Spontaneous μ-τ Reflection Symmetry Breaking in Neutrino Phenomenology
Abstract
1. Introduction
2. Spontaneous - Reflection Symmetry Breaking
2.1. The Majorana Case
2.2. The Dirac Case
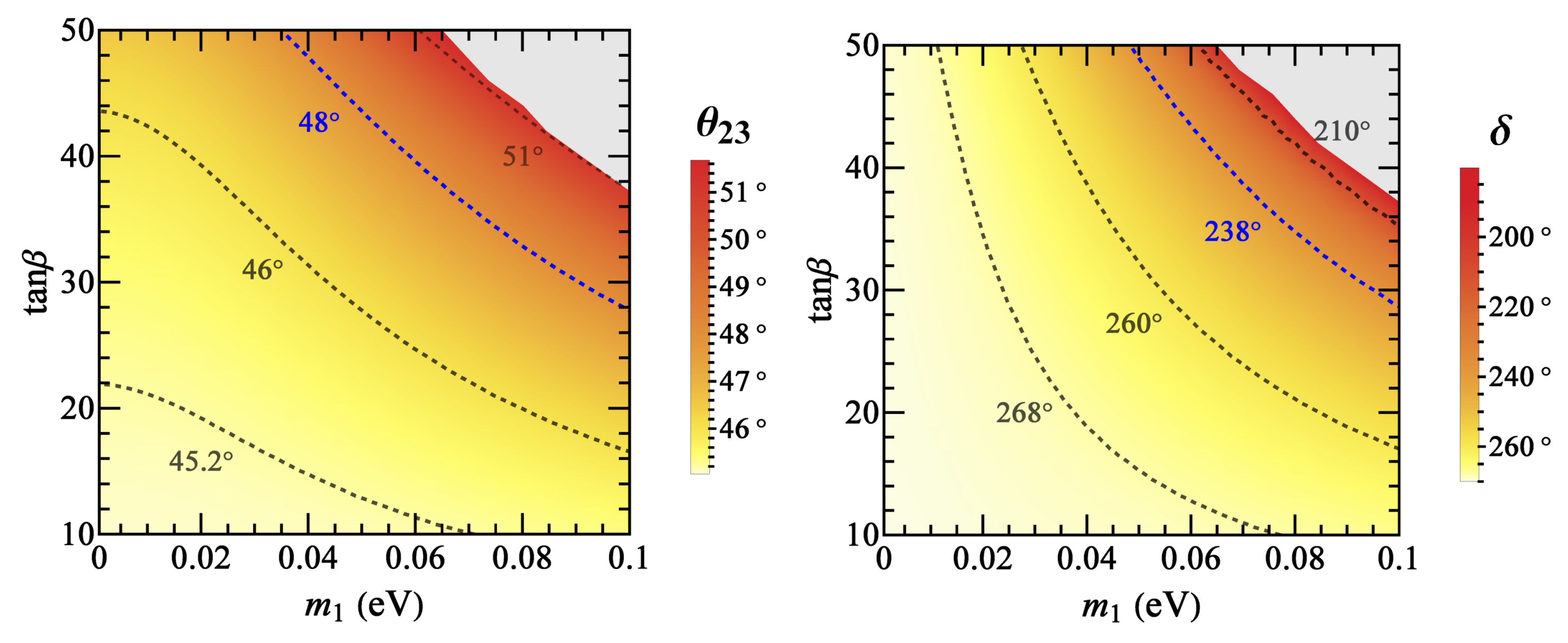
3. Numerical Exploration
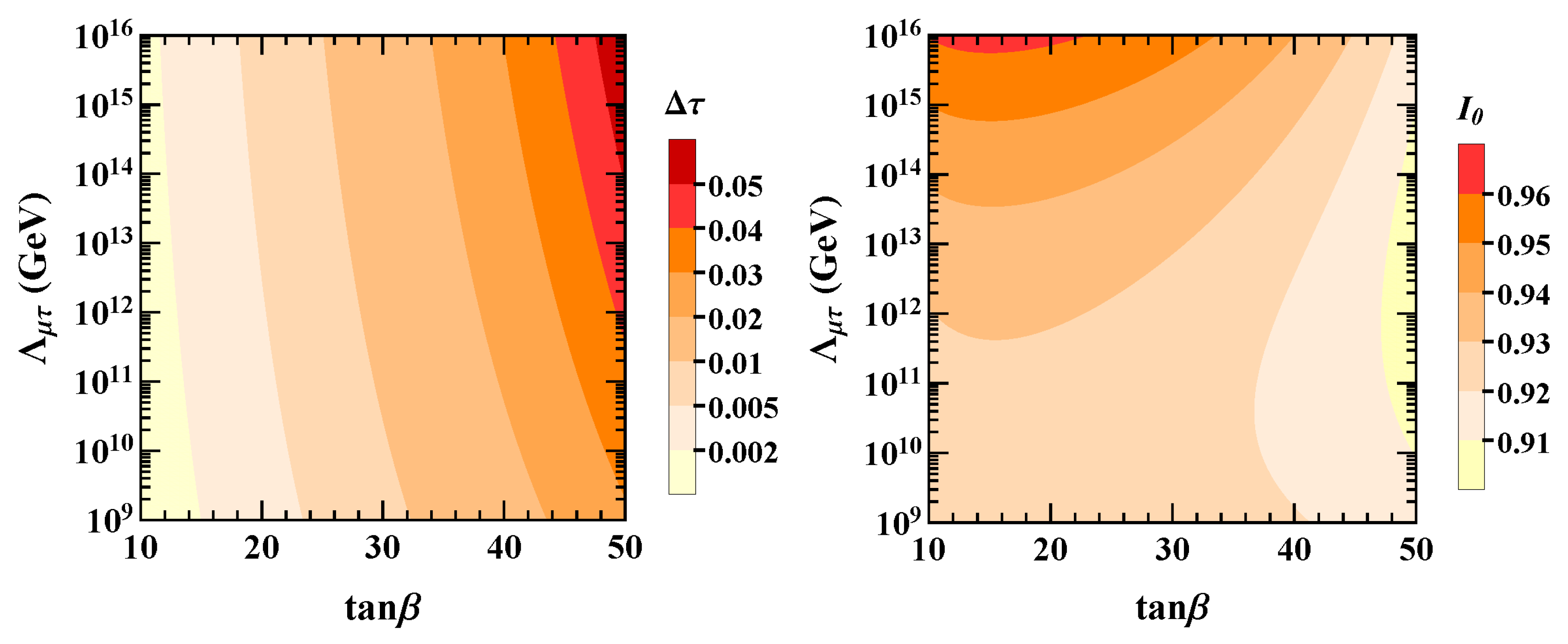
3.1. The Majorana Case
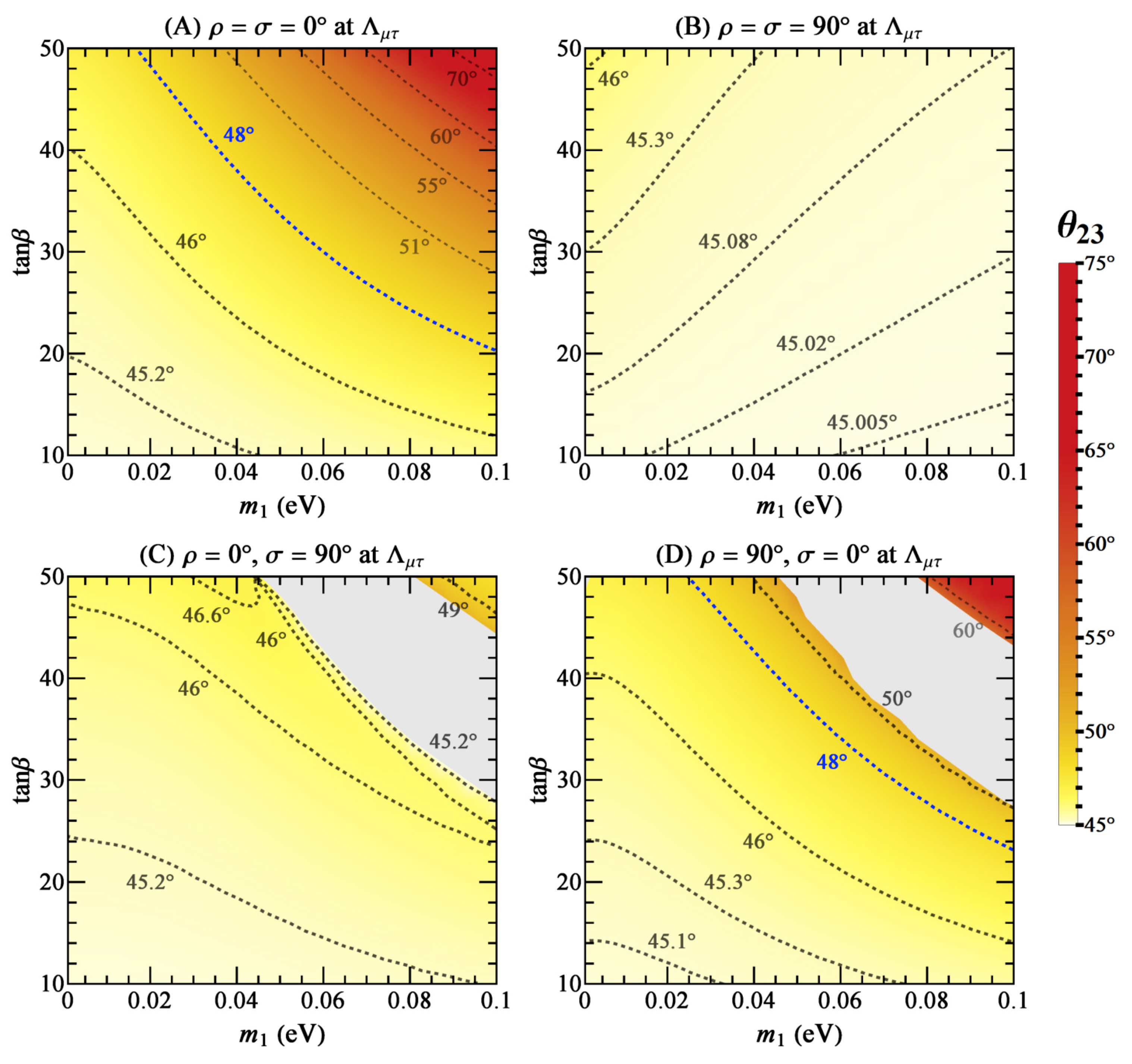
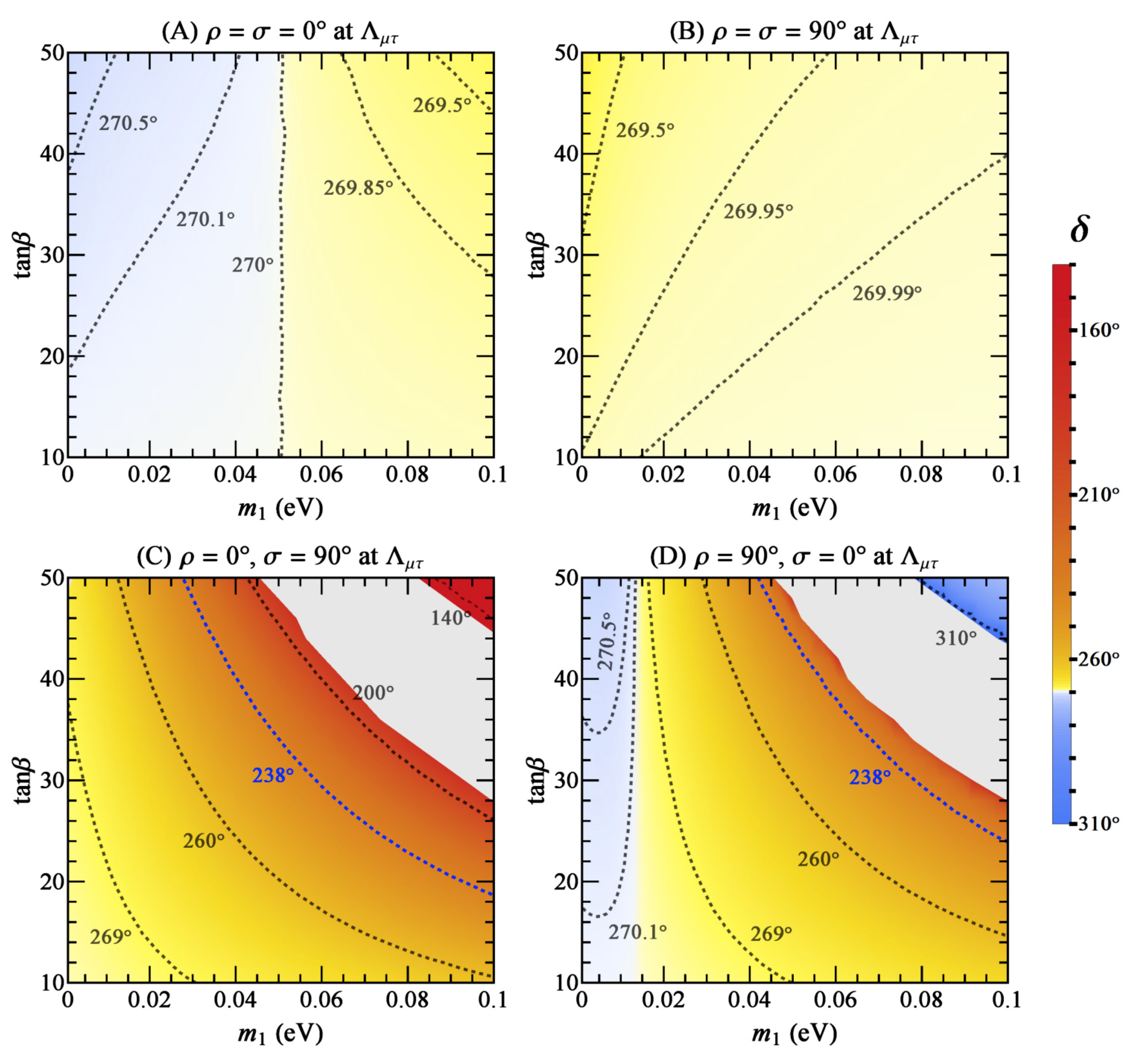
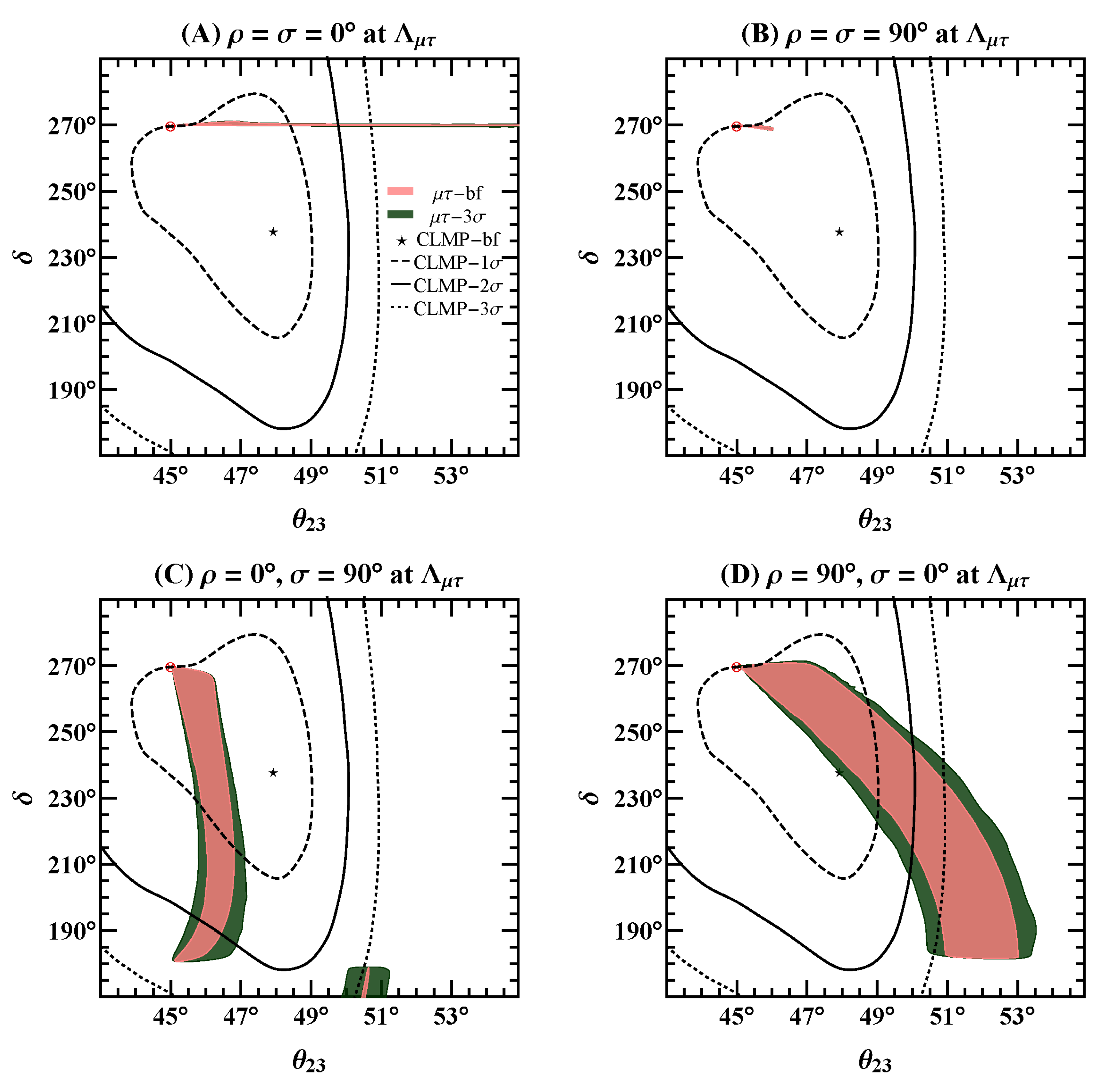
3.2. The Dirac Case
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Tanabashi, M.; Particle Data Group; et al. Review of Particle Physics. Phys. Rev. D 2018, 98, 030001. [Google Scholar]
- Maki, Z.; Nakagawa, M.; Sakata, S. Remarks on the unified model of elementary particles. Prog. Theor. Phys. 1962, 28, 870–880. [Google Scholar]
- Pontecorvo, B. Neutrino Experiments and the Problem of Conservation of Leptonic Charge. Sov. Phys. JETP 1968, 26, 984–988. [Google Scholar]
- Minkowski, P. μ → eγ at a Rate of One Out of 109 Muon Decays? Phys. Lett. B 1977, 67, 421–428. [Google Scholar] [CrossRef]
- Yanagida, T. Horizontal Symmetry And Masses Of Neutrinos. In Proceedings of the Workshop on the Unified Theories and the Baryon Number in the Universe, Tsukuba, Japan, 13–14 February 1979; Sawada, O., Sugamoto, A., Eds.; National Laboratory for High Energy Physics: Tsukuba, Japan, 1979; p. 95. [Google Scholar]
- Gell-Mann, M.; Ramond, P.; Slansky, R. Complex Spinors and Unified Theories. In Supergravity; van Nieuwenhuizen, P., Freedman, D., Eds.; North Holland Publishing Co.: Amsterdam, The Netherlands, 1979; p. 315. [Google Scholar]
- Glashow, S.L. The Future of Elementary Particle Physics. In Quarks and Leptons; Lévy, M., Basdevant, J.L., Speiser, D., Weyers, J., Gastmans, R., Jacob, M., Eds.; Springer: Boston, MA, USA, 1980; p. 687. [Google Scholar]
- Mohapatra, R.N.; Senjanovic, G. Neutrino Mass and Spontaneous Parity Violation. Phys. Rev. Lett. 1980, 44, 912. [Google Scholar]
- Fogli, G.L.; Lisi, E.; Marrone, A.; Palazzo, A.; Rotunno, A.M. Hints of θ13 > 0 from global neutrino data analysis. Phys. Rev. Lett. 2008, 101, 141801. [Google Scholar] [CrossRef] [PubMed]
- An, F.P.; Bai, J.Z.; Balantekin, A.B.; Band, H.R.; Beavis, D.; Beriguete, W.; Bishai, M.; Blyth, S.; Boddy, K.; Brown, R.L.; et al. Observation of electron-antineutrino disappearance at Daya Bay. Phys. Rev. Lett. 2012, 108, 171803. [Google Scholar] [PubMed]
- Capozzi, F.; Lisi, E.; Marrone, A.; Palazzo, A. Current unknowns in the three neutrino framework. Prog. Part. Nucl. Phys. 2018, 102, 48–72. [Google Scholar]
- Babu, K.S.; Ma, E.; Valle, J.W.F. Underlying A(4) symmetry for the neutrino mass matrix and the quark mixing matrix. Phys. Lett. B 2003, 552, 207–213. [Google Scholar] [CrossRef]
- Harrison, P.F.; Scott, W.G. μ-τ reflection symmetry in lepton mixing and neutrino oscillations. Phys. Lett. B 2002, 547, 219–228. [Google Scholar] [CrossRef]
- Xing, Z.Z.; Zhao, Z.H. A review of μ-τ flavor symmetry in neutrino physics. Rept. Prog. Phys. 2016, 79, 076201. [Google Scholar] [CrossRef] [PubMed]
- Luo, S.; Xing, Z.Z. Resolving the octant of θ23 via radiative μ-τ symmetry breaking. Phys. Rev. D 2014, 90, 073005. [Google Scholar]
- Zhou, Y.L. μ-τ reflection symmetry and radiative corrections. arXiv, 2014; arXiv:1409.8600. [Google Scholar]
- Zhao, Z.H. Breakings of the neutrino μ-τ reflection symmetry. J. High Energy Phys. 2017, 2017, 023. [Google Scholar] [CrossRef]
- Rodejohann, W.; Xu, X.J. Trimaximal μ-τ reflection symmetry. Phys. Rev. D 2017, 96, 055039. [Google Scholar]
- Liu, Z.C.; Yue, C.X.; Zhao, Z.H. Neutrino μ-τ reflection symmetry and its breaking in the minimal seesaw. J. High Energy Phys. 2017, 2017, 102. [Google Scholar]
- Xing, Z.Z.; Zhang, D.; Zhu, J.Y. The μ-τ reflection symmetry of Dirac neutrinos and its breaking effect via quantum corrections. J. High Energy Phys. 2017, 2017, 135. [Google Scholar]
- Nath, N.; Xing, Z.Z.; Zhang, J. μ-τ Reflection Symmetry Embedded in Minimal Seesaw. Eur. Phys. J. C 2018, 78, 289. [Google Scholar] [CrossRef]
- Huang, G.Y.; Xing, Z.Z.; Zhu, J.Y. Correlation of normal neutrino mass ordering with upper octant of θ23 and third quadrant of δ via spontaneous μ-τ symmetry breaking. arXiv, 2018; arXiv:1806.06640. [Google Scholar]
- Ellis, J.R.; Lola, S. Can neutrinos be degenerate in mass? Phys. Lett. B 1999, 458, 310. [Google Scholar] [CrossRef]
- Fritzsch, H.; Xing, Z.Z. Mass and flavor mixing schemes of quarks and leptons. Prog. Part. Nucl. Phys. 2000, 45, 1–81. [Google Scholar] [CrossRef]
- Feroz, F.; Hobson, M.P. Multimodal nested sampling: An efficient and robust alternative to MCMC methods for astronomical data analysis. Mon. Not. R. Astron. Soc. 2008, 384, 449–463. [Google Scholar] [CrossRef]
- Feroz, F.; Hobson, M.P.; Bridges, M. MultiNest: An efficient and robust Bayesian inference tool for cosmology and particle physics. Mon. Not. R. Astron. Soc. 2009, 398, 1601–1614. [Google Scholar] [CrossRef]
- Feroz, F.; Hobson, M.P.; Cameron, E.; Pettitt, A.N. Importance Nested Sampling and the MultiNest Algorithm. arXiv, 2013; arXiv:1306.2144. [Google Scholar]
- Xing, Z.Z.; Zhang, H.; Zhou, S. Impacts of the Higgs mass on vacuum stability, running fermion masses and two-body Higgs decays. Phys. Rev. D 2012, 86, 013013. [Google Scholar]
- Elias-Miro, J.; Espinosa, J.R.; Giudice, G.F.; Isidori, G.; Riotto, A.; Strumia, A. Higgs mass implications on the stability of the electroweak vacuum. Phys. Lett. B 2012, 709, 222–228. [Google Scholar]

© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, G.-Y.; Xing, Z.-Z.; Zhu, J.-Y. Spontaneous μ-τ Reflection Symmetry Breaking in Neutrino Phenomenology. Universe 2018, 4, 141. https://doi.org/10.3390/universe4120141
Huang G-Y, Xing Z-Z, Zhu J-Y. Spontaneous μ-τ Reflection Symmetry Breaking in Neutrino Phenomenology. Universe. 2018; 4(12):141. https://doi.org/10.3390/universe4120141
Chicago/Turabian StyleHuang, Guo-Yuan, Zhi-Zhong Xing, and Jing-Yu Zhu. 2018. "Spontaneous μ-τ Reflection Symmetry Breaking in Neutrino Phenomenology" Universe 4, no. 12: 141. https://doi.org/10.3390/universe4120141
APA StyleHuang, G.-Y., Xing, Z.-Z., & Zhu, J.-Y. (2018). Spontaneous μ-τ Reflection Symmetry Breaking in Neutrino Phenomenology. Universe, 4(12), 141. https://doi.org/10.3390/universe4120141





