Changes in Emission Properties Associated with the Emission Shifts in PSR J0344−0901
Abstract
1. Introduction
2. The Model
2.1. Detectable Variation in the Plasma Flow
2.2. Measuring the Changes
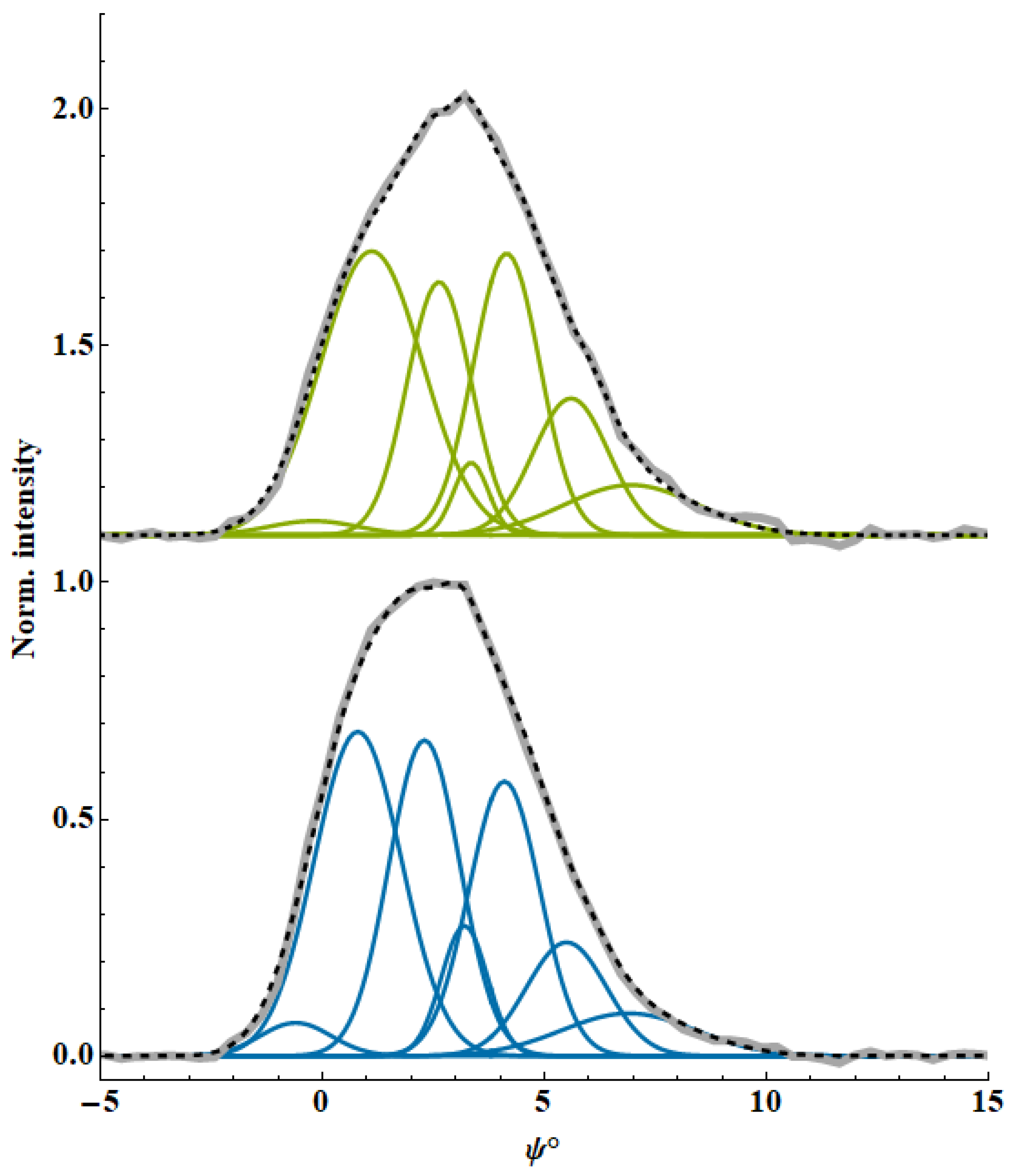
3. Gaussian Fitting and Changes in the Emission States
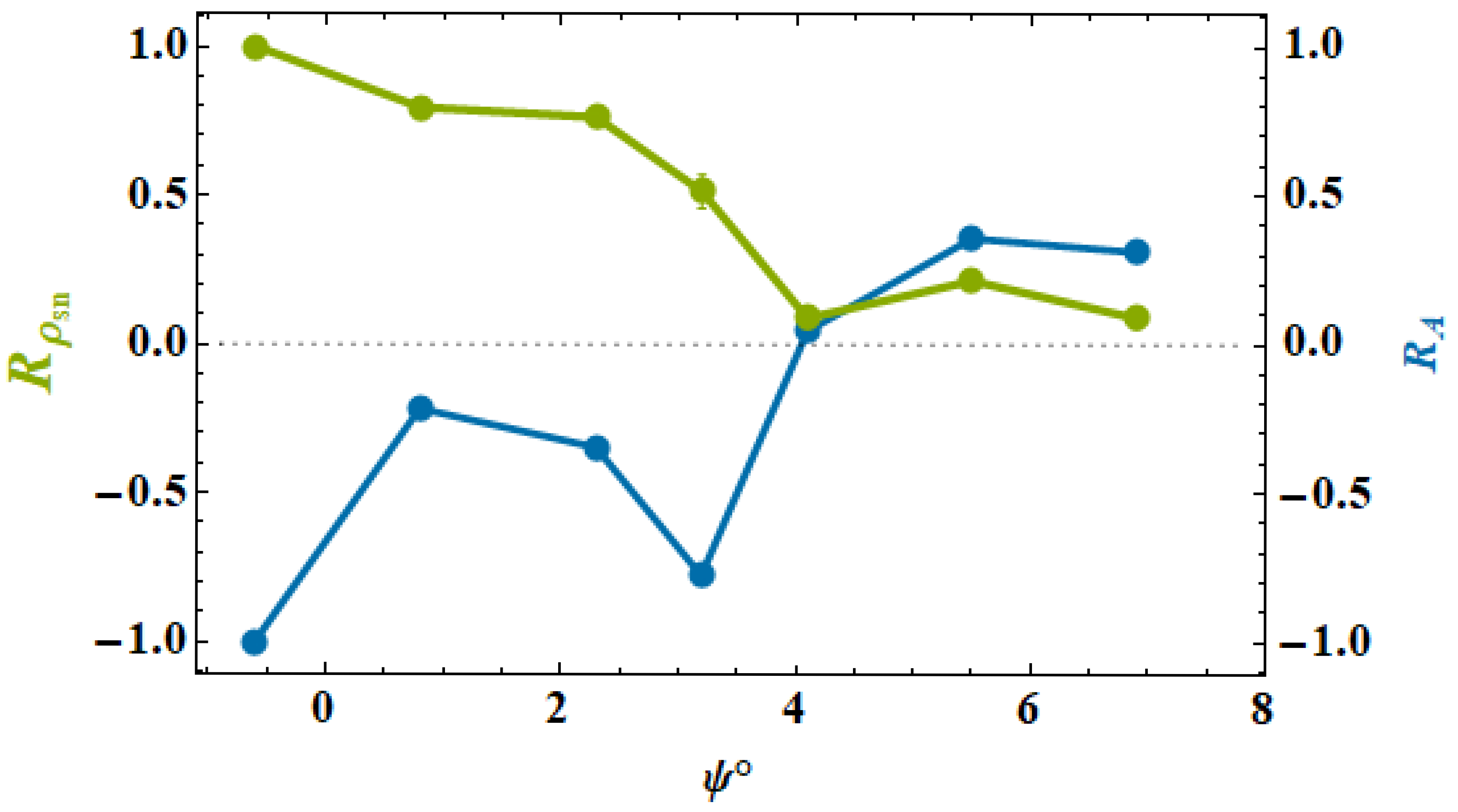
3.1. Changes of the Profile
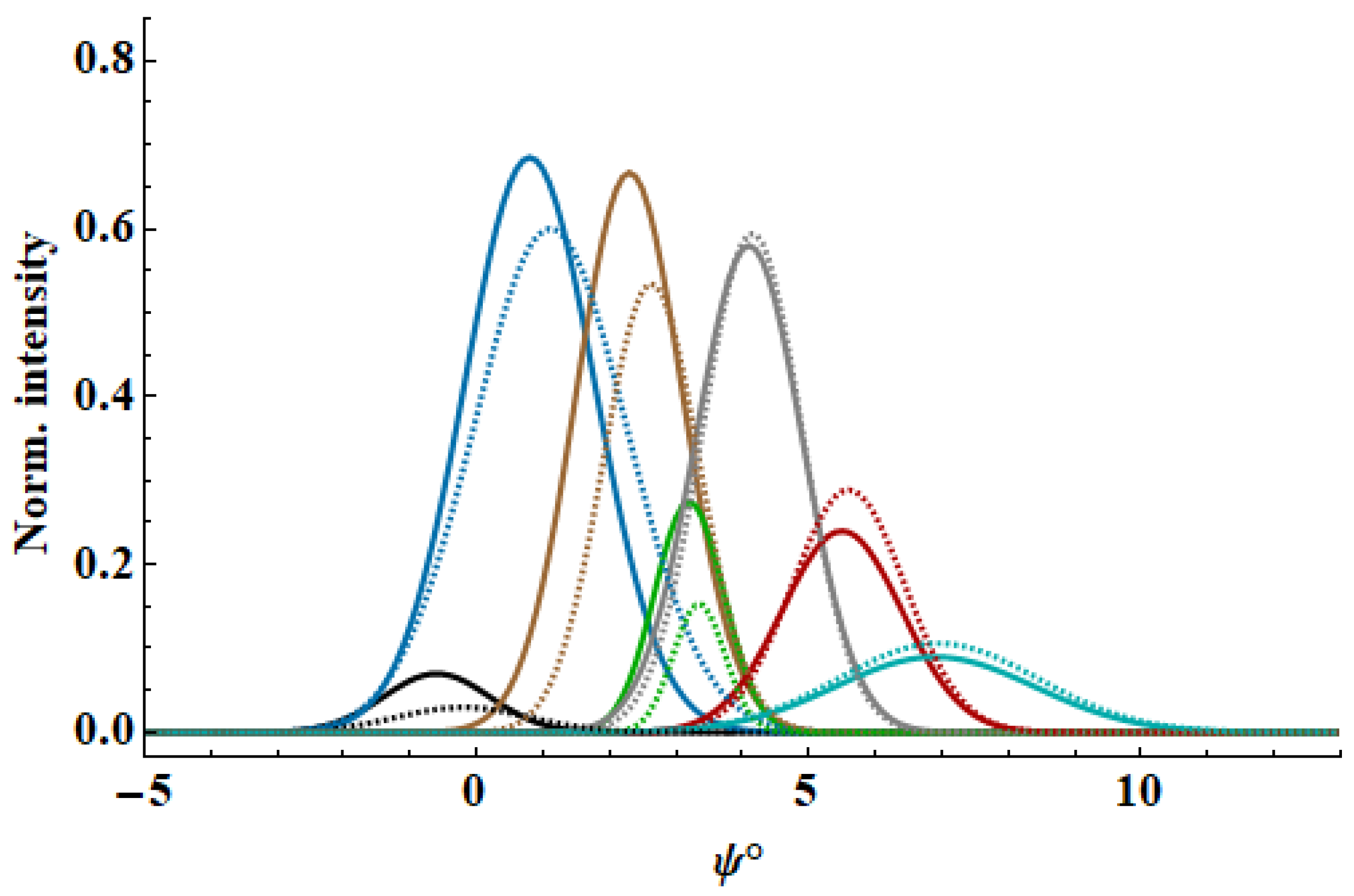
3.2. The Results
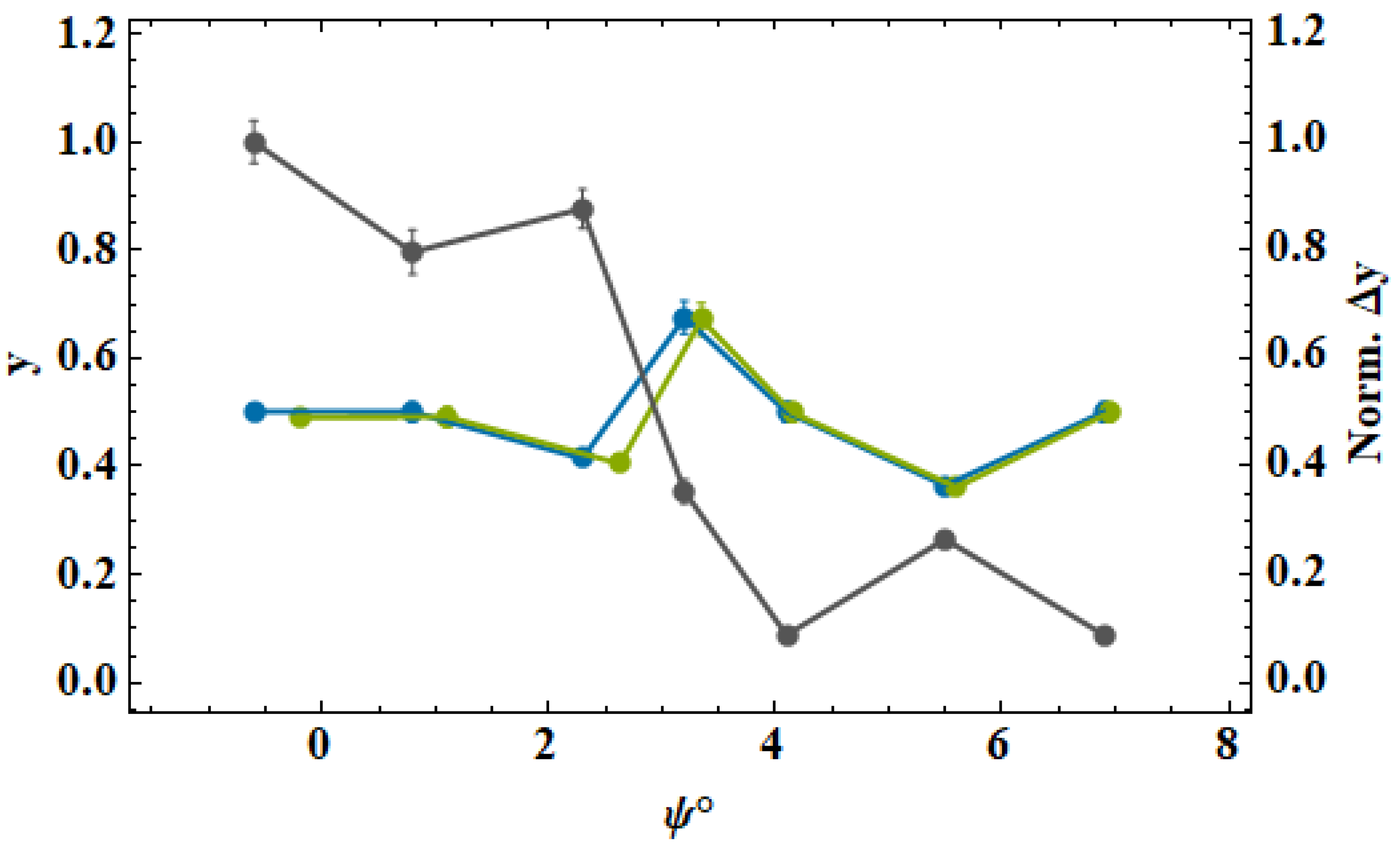
4. Properties of the Emission Shift
4.1. Irregular Subpulse Drifting
4.2. Changes in and the Emission Height
4.3. Changes in the Plasma Density and the Peak Intensity
4.4. Conditions for the Emission Shift
5. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. The Electric and Magnetic Fields
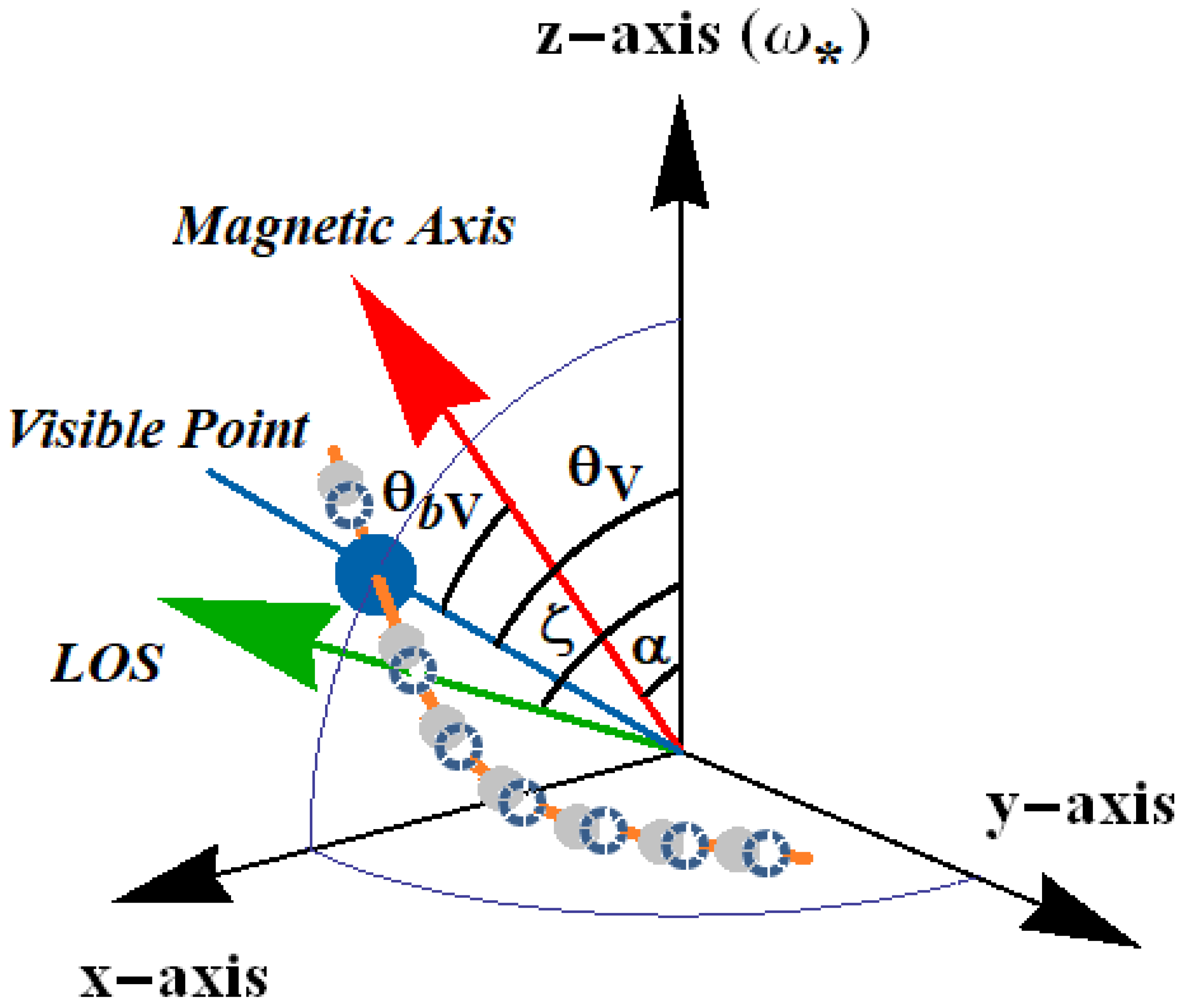
Appendix B. The Geometry for Pulsar Visibility
References
- Backer, D.C. Pulsar Nulling Phenomena. Nature 1970, 228, 42. [Google Scholar] [CrossRef] [PubMed]
- Rankin, J.M. Toward an Empirical Theory of Pulsar Emission. III. Mode Changing, Drifting Subpulses, and Pulse Nulling. Astrophys. J. 1986, 301, 901. [Google Scholar] [CrossRef]
- Wang, N.; Manchester, R.N.; Johnston, S. Pulsar nulling and mode changing. Mon. Not. R. Astron. Soc. 2007, 377, 1383. [Google Scholar] [CrossRef]
- Lyne, A.G. Mode Changing in Pulsar Radiation. Mon. Not. R. Astron. Soc. 1971, 153, 27P. [Google Scholar] [CrossRef]
- Kramer, M.; Lyne, A.G.; O’Brien, J.T.; Jordan, C.A.; Lorimer, D.R. A Periodically Active Pulsar Giving Insight into Magnetospheric Physics. Science 2006, 312, 549. [Google Scholar] [CrossRef]
- Camilo, F.; Ransom, S.M.; Chatterjee, S.; Johnston, S.; Demorest, P. PSR J1841−0500: A radio pulsar that mostly is not there. Astrophys. J. 2012, 746, 63. [Google Scholar] [CrossRef]
- Lorimer, D.R.; Lyne, A.G.; McLaughlin, M.A.; Kramer, M.; Pavlov, G.G.; Chang, C. Radio and X-ray observations of the intermittent pulsar J1832+0029. Astrophys. J. 2012, 758, 141. [Google Scholar] [CrossRef]
- Lyne, A.G.; Stappers, B.W.; Freire, P.C.C.; Hessels, J.W.T.; Kaspi, V.N.; Allen, B.; Bogdanov, S.; Brazier, A.; Camilo, F.; Cardoso, F.; et al. Two long-term intermittent pulsars discovered in the PALFA survey. Astrophys. J. 2017, 834, 72. [Google Scholar] [CrossRef]
- Singha, J.; Surnis, M.P.; Joshi, B.C.; Tarafdar, P.; Rana, P.; Susobhanan, A.; Girgaonkar, R.; Kolhe, N.; Agarwal, N.; Desai, S.; et al. Evidence for profile changes in PSR J1713+0747 using the uGMRT. Mon. Not. R. Astron. Soc. 2021, 507, L57. [Google Scholar] [CrossRef]
- Jennings, R.J.; Cordes, J.M.; Chatterjee, S.; McLaughlin, M.A.; Demorest, P.B.; Arzoumanian, Z.; Baker, P.T.; Blumer, H.; Brook, P.R.; Cohen, T.; et al. An Unusual Pulse Shape Change Event in PSR J1713+0747 Observed with the Green Bank Telescope and CHIME. Astrophys. J. 2024, 964, 179. [Google Scholar] [CrossRef]
- Rankin, J.M.; Rodriguez, C.; Wright, G.A.W. Bistable profile illumination in pulsars B0919+06 and B1859+07. Mon. Not. R. Astron. Soc. 2006, 370, 673. [Google Scholar] [CrossRef]
- Wen, Z.G.; Yan, W.M.; Yuan, J.P.; Wang, H.G.; Chen, J.L.; Mijit, M.; Yuen, R.; Wang, N.; Tu, Z.Y.; Dang, S.J. The Mode Switching in Pulsar J1326−6700. Astrophys. J. 2020, 904, 72. [Google Scholar] [CrossRef]
- Tedila, H.M.; Yuen, R.; Wang, N.; Li, D.; Wen, Z.G.; Yan, W.M.; Yuan, J.P.; Han, X.H.; Wang, P.; Zhu, W.W.; et al. Investigation of Profile Shifting and Subpulse Movement in PSR J0344−0901 with FAST. Astrophys. J. 2024, 965, 144. [Google Scholar] [CrossRef]
- Wahl, H.M.; Orfeo, D.J.; Rankin, J.M.; Weisberg, J.M. Quasi-periodicities in the anomalous emission events in pulsars B1859+07 and B0919+06. Mon. Not. R. Astron. Soc. 2016, 461, 3740. [Google Scholar] [CrossRef]
- Li, D.; Wang, P.; Qian, L.; Krco, M.; Dunning, A.; Jiang, P.; Yue, Y.L.; Jin, C.; Zhu, Y.; Pan, Z.; et al. FAST in Space: Considerations for a Multibeam, Multipurpose Survey Using China’s 500-m Aperture Spherical Radio Telescope (FAST). IEEE Microw. 2018, 19, 112. [Google Scholar] [CrossRef]
- Ruderman, M.; Sutherland, P.G. Theory of pulsars: Polar gaps, sparks, and coherent microwave radiation. Astrophys. J. 1975, 196, 51. [Google Scholar] [CrossRef]
- Deshpande, A.A.; Rankin, J.M. Pulsar Magnetospheric Emission Mapping: Images and Implications of Polar Cap Weather. Astrophys. J. 1999, 524, 1008. [Google Scholar] [CrossRef]
- Deshpande, A.A.; Rankin, J.M. The topology and polarization of sub-beams associated with the ‘drifting’ sub-pulse emission of pulsar B0943+10-I. Analysis of Arecibo 430- and 111-MHz observations. Mon. Not. R. Astron. Soc. 2001, 322, 438. [Google Scholar] [CrossRef]
- Melrose, D.B.; Yuen, R. Non-corotating models for pulsar magnetospheres. Mon. Not. R. Astron. Soc. 2014, 437, 262. [Google Scholar] [CrossRef]
- Goldreich, P.; Julian, W.H. Pulsar Electrodynamics. Astrophys. J. 1969, 157, 869. [Google Scholar] [CrossRef]
- Deutsch, A.J. The electromagnetic field of an idealized star in rigid rotation in vacuo. Ann. D’Astrophys. 1955, 18, 1. [Google Scholar]
- Johnston, S.; Weisberg, J.M. Profile morphology and polarization of young pulsars. Mon. Not. R. Astron. Soc. 2006, 368, 1856. [Google Scholar] [CrossRef]
- Cordes, J.M. Observational limits on the location of pulsar emission regions. Astrophys. J. 1978, 222, 1006. [Google Scholar] [CrossRef]
- Hibschman, J.; Arons, J. Pair Multiplicities and Pulsar Death. Astrophys. J. 2001, 554, 624. [Google Scholar] [CrossRef]
- Kijak, J.; Gil, J. Radio emission altitude in pulsars. Astron. Astrophys. 2003, 397, 969. [Google Scholar] [CrossRef]
- Arons, J. Pair creation above pulsar polar caps - Steady flow in the surface acceleration zone and polar CAP X-ray emission. Astrophys. J. 1981, 248, 1099. [Google Scholar] [CrossRef]
- Jones, P.B. Radio pulsars: A theory of subpulse formation and drift. Mon. Not. R. Astron. Soc. 1984, 209, 569. [Google Scholar] [CrossRef]
- Kazbegi, A.Z.; Machabeli, G.Z.; Melikidze, G.I. The Nature of Pulsar Subpulse Drift. Aust. J. Phys. 1991, 44, 573. [Google Scholar] [CrossRef]
- Clemens, J.C.; Rosen, R. Observations of Nonradial Pulsations in Radio Pulsars. Astrophys. J. 2004, 609, 340. [Google Scholar] [CrossRef]
- Godoberidze, G.; Machabeli, G.Z.; Melrose, D.B.; Luo, Q. On the origin of the drifting subpulses in radio pulsars. Mon. Not. R. Astron. Soc. 2005, 360, 669. [Google Scholar] [CrossRef]
- Gil, J.A.; Sendyk, M. Spark Model for Pulsar Radiation Modulation Patterns. Astrophys. J. 2000, 541, 351. [Google Scholar] [CrossRef]
- Gangadhara, R.T. Pulsar Radio Emission Altitude from Curvature Radiation. Astrophys. J. 2004, 609, 335. [Google Scholar] [CrossRef]
- Yuen, R.; Melrose, D.B. Visibility of Pulsar Emission: Motion of the Visible Point. Publ. Astron. Soc. Aust. 2014, 31, e039. [Google Scholar] [CrossRef]
- Dyks, J.; Rudak, B.; Harding, A.K. On the Methods of Determining the Radio Emission Geometry in Pulsar Magnetospheres. Astrophys. J. 2004, 607, 939. [Google Scholar] [CrossRef]
- Krishnamohan, S.; Downs, G.S. Intensity dependence of the pulse profile and polarization of the VELA pulsar. Astrophys. J. 1983, 265, 372. [Google Scholar] [CrossRef]
- Kramer, M. Geometrical analysis of average pulsar profiles using multi-component Gaussian FITS at several frequencies. II. Individual results. Astron. Astrophys. Suppl. 1994, 107, 527. [Google Scholar]
- Kramer, M.; Wielebinski, R.; Jessner, A.; Gil, J.A.; Seiradakis, J.H. Geometrical analysis of average pulsar profiles using multi-component Gaussian FITS at several frequencies. I. Method and analysis. Astron. Astrophys. Suppl. Ser. 1994, 107, 515. [Google Scholar]
- Smits, J.M.; Mitra, D.; Stappers, B.W.; Kuijpers, J.; Weltevrede, P.; Jessner, A.; Gupta, Y. The geometry of PSR B0031−07. Astron. Astrophys. 2007, 465, 575. [Google Scholar] [CrossRef]
- Wu, X.; Gao, X.; Rankin, J.M.; Xu, W.; Malofeev, V.M. Gaussian Component Decomposition and the Five-Component Profile of Pulsar 1451−68. Astron. J. 1998, 116, 1984. [Google Scholar] [CrossRef]
- Drake, F.D.; Craft, H.D. Second Periodic Pulsation in Pulsars. Nature 1968, 220, 231. [Google Scholar] [CrossRef]
- Weltevrede, P.; Edwards, R.T.; Stappers, B. The subpulse modulation properties of pulsars at 21 cm. Astron. Astrophys. 2006, 445, 243. [Google Scholar] [CrossRef]
- Weltevrede, P.; Stappers, B.W.; Edwards, R.T. The subpulse modulation properties of pulsars at 92 cm and the frequency dependence of subpulse modulation. Astron. Astrophys. 2007, 469, 607. [Google Scholar] [CrossRef]
- van Leeuwen, A.G.J.; Timokhin, A.N. On plasma rotation and drifting subpulses in pulsars: Using aligned pulsar B0826−34 as a voltmeter. Astrophys. J. 2012, 752, 155. [Google Scholar] [CrossRef]
- Sun, S.N.; Yan, W.M.; Wang, N.; Wang, H.G.; Wang, S.Q.; Dang, S.J. Polarimetric observations of PSR J0614+2229 and PSR J1938+2213 using FAST. Astrophys. J. 2022, 934, 57. [Google Scholar] [CrossRef]
- van Leeuwen, A.G.J.; Stappers, B.W.; Ramachandran, R.; Rankin, J.M. Probing drifting and nulling mechanisms through their interaction in PSR B0809+74. Astron. Astrophys. 2003, 399, 223. [Google Scholar] [CrossRef]
- Dyks, J.; Rudak, B.; Demorest, P. The nature of pulsar radio emission. Mon. Not. R. Astron. Soc. 2010, 401, 1781. [Google Scholar] [CrossRef]
- Beskin, V.; Gurevich, A.V.; Istomin, Y.N. Physics of the Pulsar Magnetosphere; CUP: New York, NY, USA, 1993. [Google Scholar] [CrossRef]
- Melrose, D.B.; Yuen, R. Obliquely rotating pulsars: Screening of the inductive electric field. Astrophys. J. 2012, 745, 169. [Google Scholar] [CrossRef]
- Manchester, R.N.; Taylor, J.H. Pulsars; W. H. Freeman: San Francisco, CA, USA, 1977; Available online: https://www.biblio.com/9780716703587 (accessed on 10 April 2025).
- Cordes, J.M. Coherent radio emission from pulsars. Space Sci. Rev. 1979, 24, 567. [Google Scholar] [CrossRef]
- Lyubarskii, Y.E. Generation of pulsar radio emission. Astron. Astrophys. 1996, 308, 809. Available online: https://adsabs.harvard.edu/full/1996A%26A...308..809L (accessed on 10 April 2025). [CrossRef]
- Sturrock, P. A Model of Pulsars. Astrophys. J. 1971, 164, 529. [Google Scholar] [CrossRef]
- Hankins, T.H.; Kern, J.S.; Weatherall, J.C.; Eilek, J.A. Nanosecond radio bursts from strong plasma turbulence in the Crab pulsar. Nature 2003, 422, 141. [Google Scholar] [CrossRef] [PubMed]
- Dyks, J.; Rudak, B.; Rankin, J.M. A model for double notches and bifurcated components in radio profiles of pulsars and magnetars. Astron. Astrophys. 2007, 465, 981. [Google Scholar] [CrossRef]
- Jessner, A.; Popov, M.V.; Kondratiev, V.I.; Kovalev, Y.Y.; Graham, D.; Zensus, A.; Soglasnov, V.A.; Bilous, A.V.; Moshkina, O.A. Giant pulses with nanosecond time resolution detected from the Crab pulsar at 8.5 and 15.1 GHz. Astron. Astrophys. 2010, 524, A60. [Google Scholar] [CrossRef]
- Wang, H.G.; Pi, F.P.; Zheng, X.P.; Deng, C.L.; Wen, S.Q.; Ye, F.; Guan, K.Y.; Liu, Y.; Xu, L.Q. A fan beam model for radio pulsars. I. observational evidence. Astrophys. J. 2014, 789, 73. [Google Scholar] [CrossRef]
- Melrose, D.B.; Yuen, R. Pulsar electrodynamics: An unsolved problem. J. Plasma Phys. 2016, 82, 635820202. [Google Scholar] [CrossRef]
- Xu, R.; Wang, W. Repeating fast radio bursts reveal the secret of pulsar magnetospheric activity. Astron. Nachr. 2023, 345, e20230153. [Google Scholar] [CrossRef]
- Maciesiak, K.; Gil, J.; Ribeiro, V.A.R.M. On the pulse-width statistics in radio pulsars – I. Importance of the interpulse emission. Mon. Not. R. Astron. Soc. 2011, 414, 1314. [Google Scholar] [CrossRef]
- Cordes, J.M.; Kramer, M.; Lazio, T.J.W.; Stappers, B.W.; Backer, D.C.; Johnston, S. Pulsars as tools for fundamental physics & astrophysics. New Astron. Rev. 2004, 48, 1413. [Google Scholar] [CrossRef]
- Timokhin, A.N. A model for nulling and mode changing in pulsars. Mon. Not. R. Astron. Soc. 2010, 408, L41. [Google Scholar] [CrossRef]
- Wright, G. Pulsar emission patterns seen as evidence for magnetospheric interactions. Mon. Not. R. Astron. Soc. 2022, 514, 4046. [Google Scholar] [CrossRef]
- Montoli, A.; Antonelli, M.; Magistrelli, F.; Pizzochero, P.M. Bayesian estimate of the superfluid moments of inertia from the 2016 glitch in the Vela pulsar. Astron. Astrophys. 2020, 642, A223. [Google Scholar] [CrossRef]
- Jiménez Cruz, N.M.; Malhotra, A.; Tasinato, G.; Zavala, I. Measuring kinematic anisotropies with pulsar timing arrays. Phys. Rev. D 2024, 110, 063526. [Google Scholar] [CrossRef]
- Hassall, T.E.; Stappers, B.W.; Hessels, J.W.T.; Kramer, M.; Alexov, A.; Anderson, K.; Coenen, T.; Karastergiou, A.; Keane, E.F.; Kondratiev, V.I.; et al. Wide-band simultaneous observations of pulsars: Disentangling dispersion measure and profile variations. Astron. Astrophys. 2012, 543, A66. [Google Scholar] [CrossRef]
- Cheng, K.S.; Ruderman, M.A.; Zhang, L. A three-dimensional outer magnetospheric gap model for gamma-ray pulsars: Geometry, pair production, emission morphologies, and phase-resolved spectra. Astrophys. J. 2000, 537, 964. [Google Scholar] [CrossRef]
- Krzeszowski, K.; Mitra, D.; Gupta, Y.; Kijak, J.; Gil, J.; Acharyya, A. On the aberration–retardation effects in pulsars. Mon. Not. R. Astron. Soc. 2009, 393, 1617. [Google Scholar] [CrossRef]
- Wang, N. Xinjiang Qitai 110 m radio telescope. Sci. Sin. Phys. Mech. Astron. 2014, 44, 783. (In Chinese) [Google Scholar] [CrossRef]
- Wang, N.; Xu, Q.; Ma, J.; Liu, Z.Y.; Liu, Q.; Zhang, H.L.; Pei, X.; Chen, M.Z.; Manchester, R.N.; Lee, K.J.; et al. The Qitai radio telescope. Sci. China Phys. Mech. Astron. 2023, 66, 289512. [Google Scholar] [CrossRef]
- Hones, E.W.J.; Bergeson, J.E. Electric field generated by a rotating magnetized sphere. J. Geophys. Res. 1965, 70, 4951. [Google Scholar] [CrossRef]
- Melrose, D.B. Rotational effects on the distribution of thermal plasma in the magnetosphere of jupiter. Planet. Space. Sci. 1967, 15, 381. [Google Scholar] [CrossRef]
- Melrose, D.B. The models for radio emission from pulsars - the outstanding issues. J. Astrophys. Astr. 1995, 16, 137. [Google Scholar] [CrossRef]
- Lyutikov, M.; Blandford, R.D.; Machabeli, G. On the nature of pulsar radio emission. Mon. Not. R. Astron. Soc. 1999, 305, 338. [Google Scholar] [CrossRef]

| Comp. | A | y | |||||
|---|---|---|---|---|---|---|---|
| Non-shifted profile | |||||||
| 1 | −0.60 | 0.070 | 0.80 | 2.64 | 0.502 ± 0.014 | 0.531 ± 0.013 | |
| 2 | 0.80 | 0.685 | 0.98 | 8.5 | 2.58 | 0.501 ± 0.018 | 0.532 ± 0.017 |
| 3 | 2.30 | 0.667 | 0.80 | 33.3 | 3.00 | 0.415 ± 0.012 | 0.612 ± 0.011 |
| 4 | 3.20 | 0.275 | 0.52 | 43.6 | 3.54 | 0.675 ± 0.030 | 0.369 ± 0.028 |
| 5 | 4.10 | 0.580 | 0.80 | 50.6 | 4.28 | 0.501 ± 0.018 | 0.532 ± 0.017 |
| 6 | 5.50 | 0.240 | 0.90 | 58.2 | 5.89 | 0.364 ± 0.012 | 0.660 ± 0.011 |
| 7 | 6.90 | 0.090 | 1.50 | 63.5 | 8.01 | 0.501 ± 0.018 | 0.533 ± 0.017 |
| Shifted profile | |||||||
| 1 | −0.20 | 0.030 | 1.00 | −3.4 | 2.57 | 0.491 ± 0.014 | 0.541 ± 0.013 |
| 2 | 1.11 | 0.600 | 1.15 | 18.3 | 2.66 | 0.492 ± 0.018 | 0.540 ± 0.017 |
| 3 | 2.63 | 0.535 | 0.72 | 36.8 | 3.13 | 0.405 ± 0.012 | 0.621 ± 0.011 |
| 4 | 3.35 | 0.153 | 0.39 | 44.5 | 3.62 | 0.671 ± 0.030 | 0.372 ± 0.028 |
| 5 | 4.15 | 0.595 | 0.75 | 50.6 | 4.28 | 0.500 ± 0.018 | 0.533 ± 0.017 |
| 6 | 5.60 | 0.289 | 0.85 | 58.7 | 6.02 | 0.361 ± 0.012 | 0.663 ± 0.011 |
| 7 | 6.95 | 0.106 | 1.50 | 63.5 | 8.01 | 0.500 ± 0.018 | 0.534 ± 0.017 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuen, R.; Tedila, H.M. Changes in Emission Properties Associated with the Emission Shifts in PSR J0344−0901. Universe 2025, 11, 165. https://doi.org/10.3390/universe11050165
Yuen R, Tedila HM. Changes in Emission Properties Associated with the Emission Shifts in PSR J0344−0901. Universe. 2025; 11(5):165. https://doi.org/10.3390/universe11050165
Chicago/Turabian StyleYuen, Rai, and Habtamu M. Tedila. 2025. "Changes in Emission Properties Associated with the Emission Shifts in PSR J0344−0901" Universe 11, no. 5: 165. https://doi.org/10.3390/universe11050165
APA StyleYuen, R., & Tedila, H. M. (2025). Changes in Emission Properties Associated with the Emission Shifts in PSR J0344−0901. Universe, 11(5), 165. https://doi.org/10.3390/universe11050165







