Quantum Loop Corrections in the Modified Gravity Model of Starobinsky Inflation with Primordial Black Hole Production
Abstract
1. Introduction
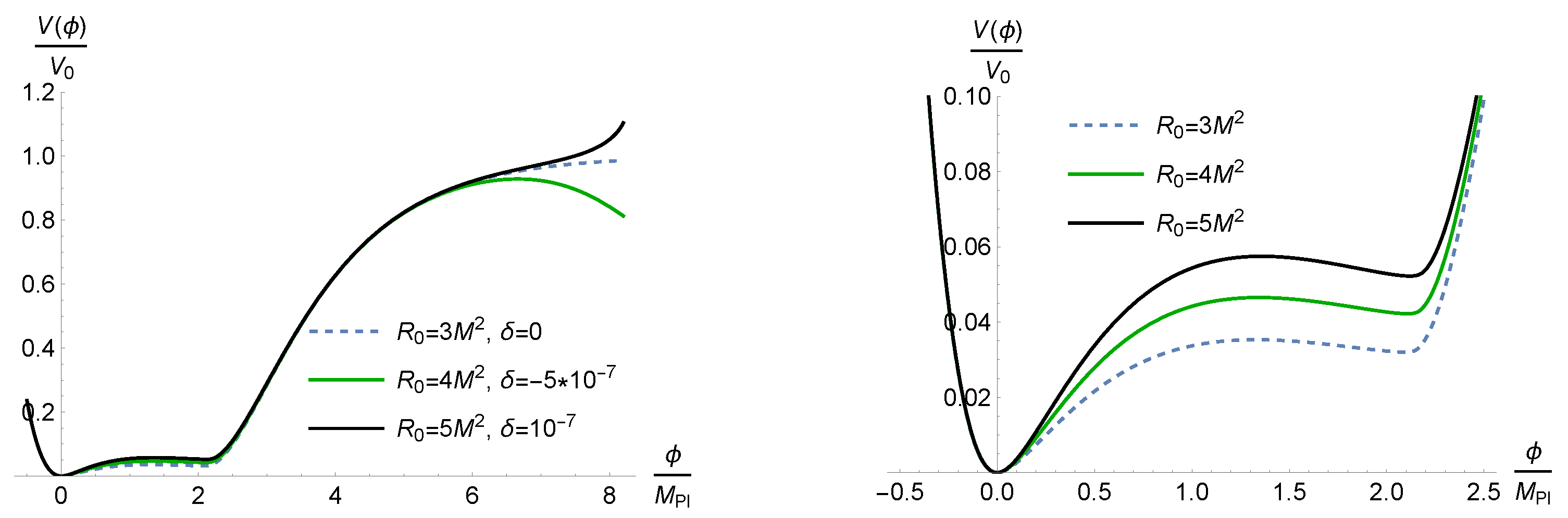
2. The Model
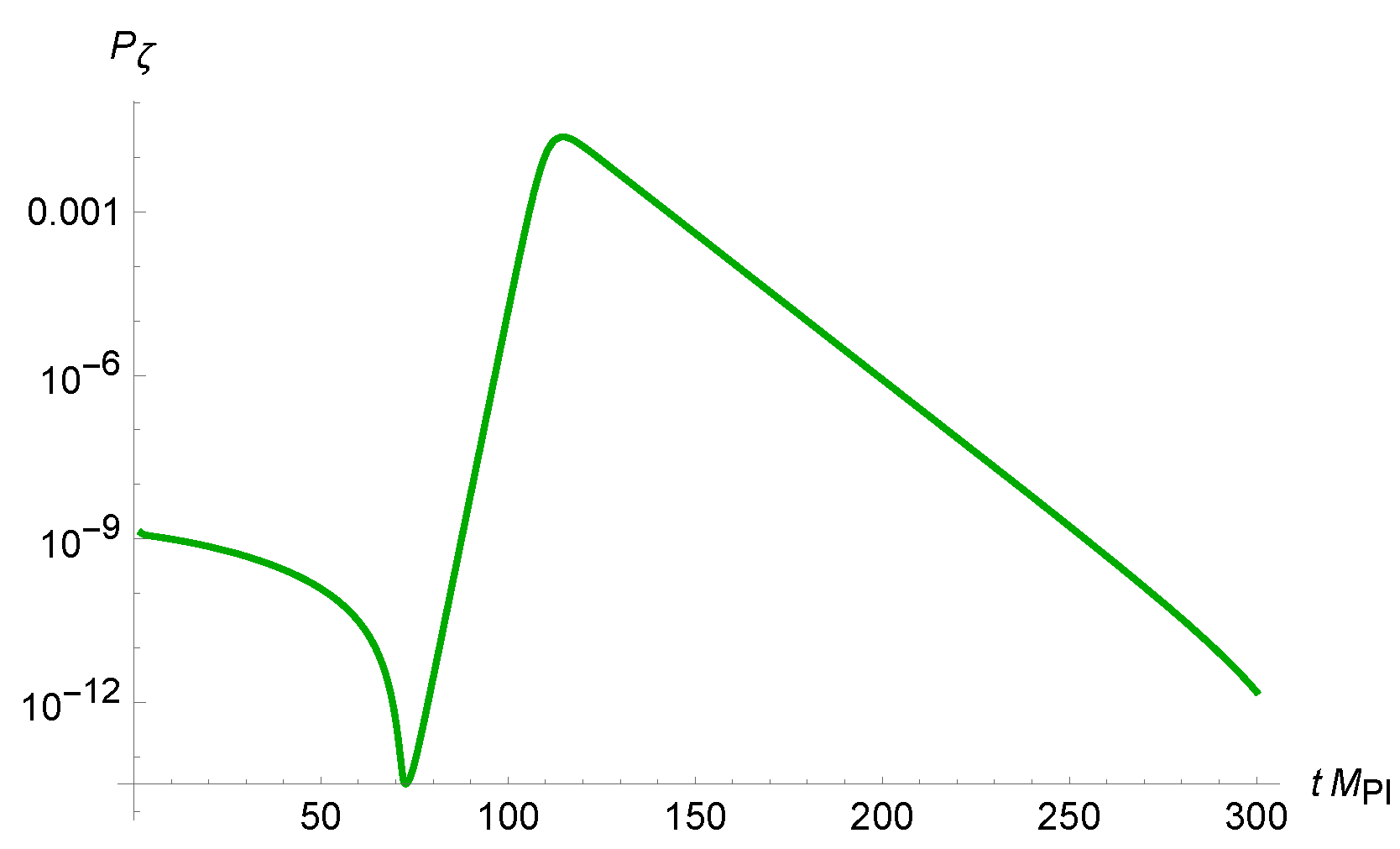
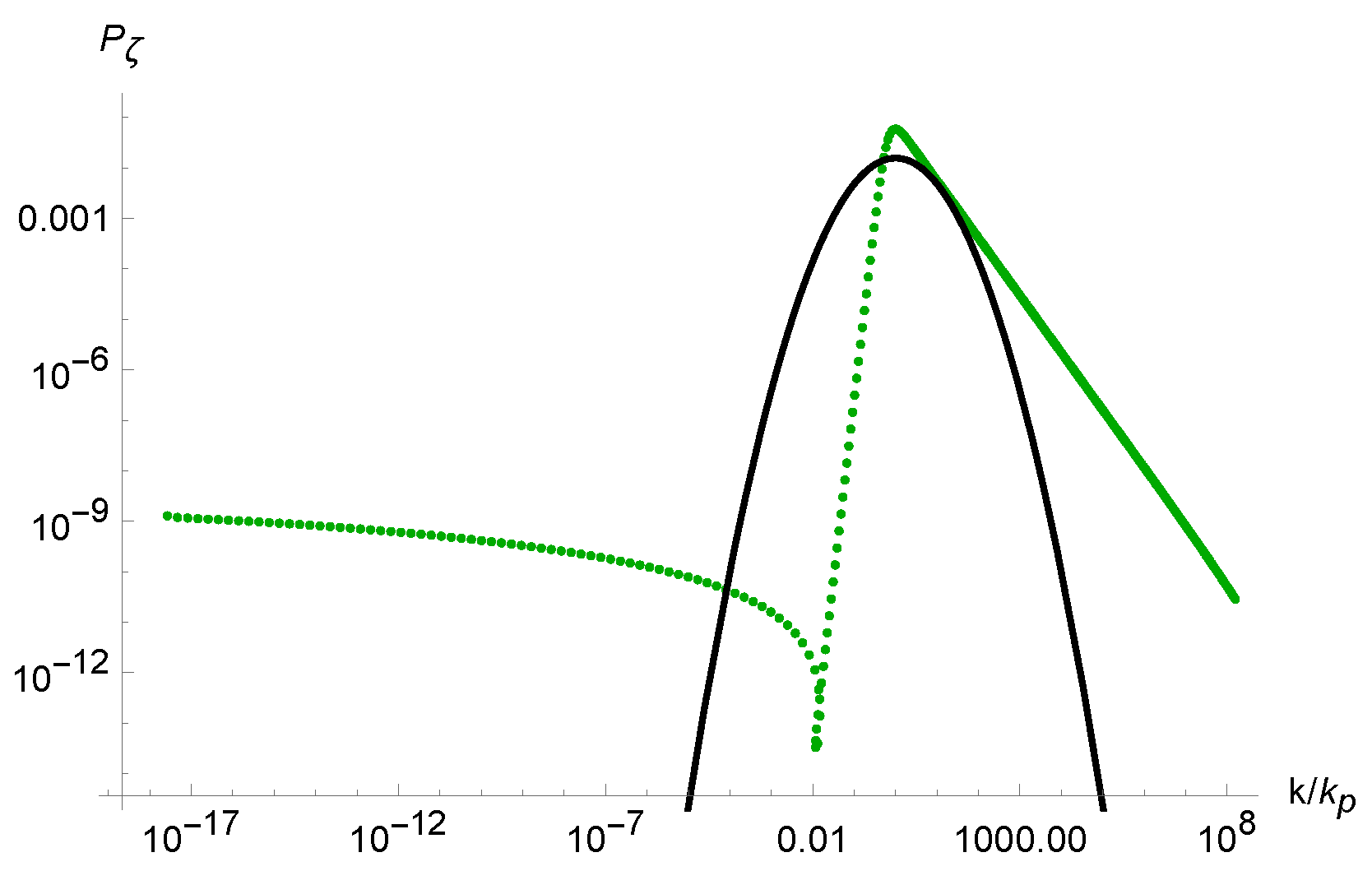
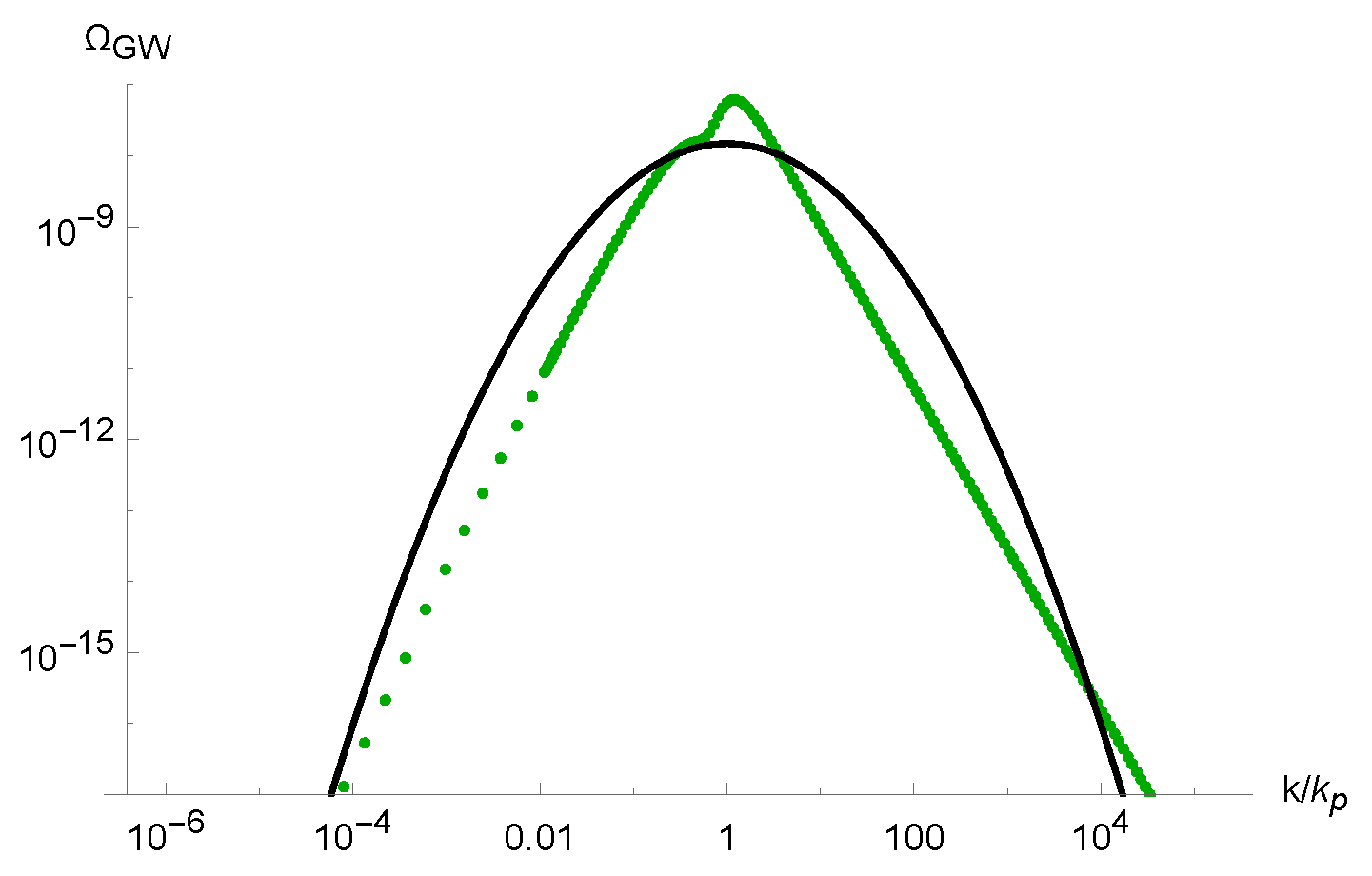
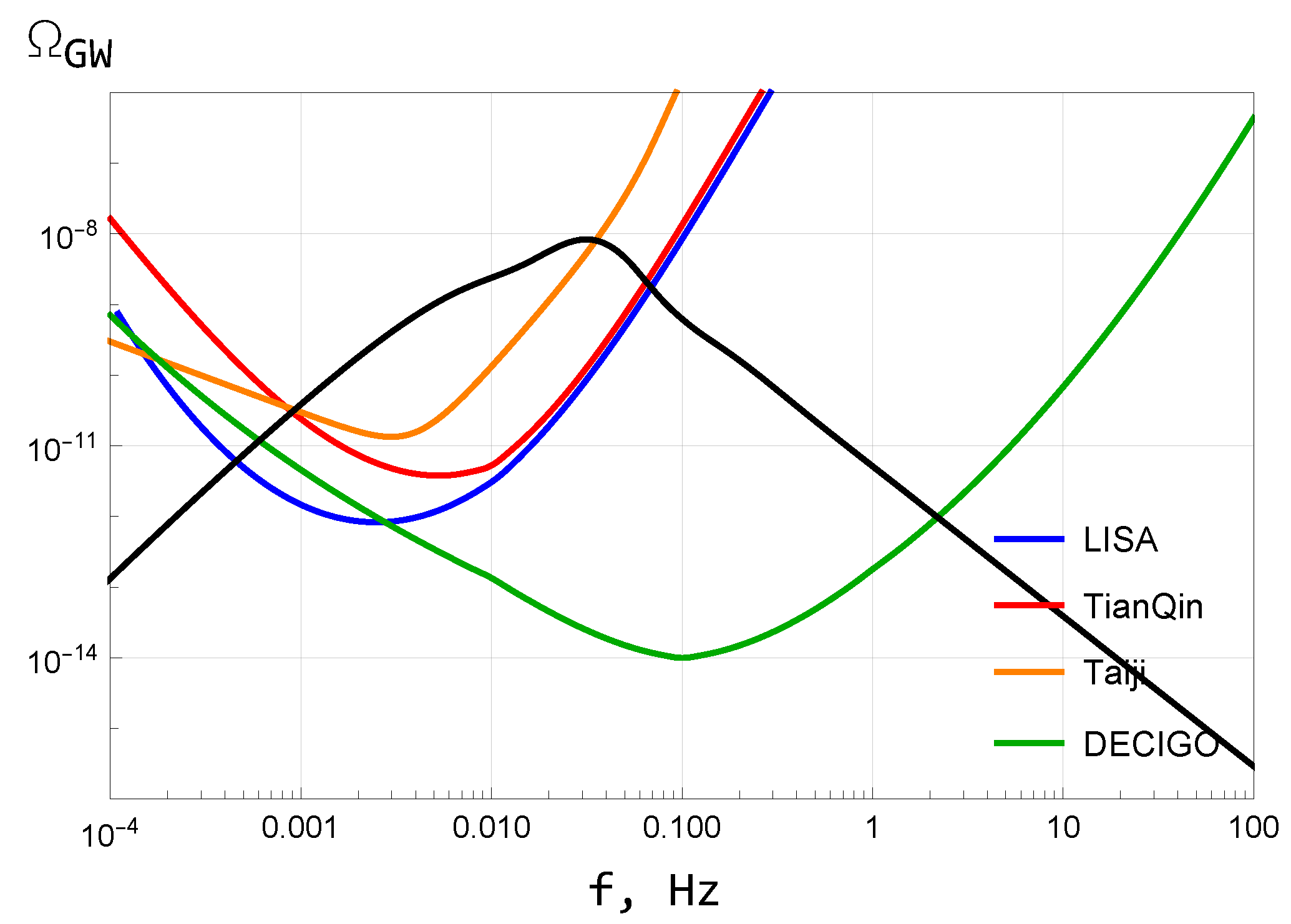
3. PBH-Induced GW and PBH-DM Density Fraction
4. Loop Corrections
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | It differs from Ref. [20] where was related to the dark energy scale. |
| 2 | See, however, Ref. [45] for a partial comparison. |
References
- Starobinsky, A.A. A new type of isotropic cosmological models without singularity. Phys. Lett. B 1980, 91, 99–102. [Google Scholar] [CrossRef]
- Ketov, S.V. On the equivalence of Starobinsky and Higgs inflationary models in gravity and supergravity. J. Phys. A 2020, 53, 084001. [Google Scholar] [CrossRef]
- Ivanov, V.R.; Ketov, S.V.; Pozdeeva, E.O.; Vernov, S.Y. Analytic extensions of Starobinsky model of inflation. J. Cosmol. Astropart. Phys. 2022, 3, 058. [Google Scholar] [CrossRef]
- Akrami, Y.; Arroja, F.; Ashdown, M.; Aumont, J.; Baccigalupi, C.; Ballardini, M.; Banday, A.J.; Barreiro, R.B.; Bartolo, N.; Basak, S.; et al. Planck 2018 results. X. Constraints on inflation. Astron. Astrophys. 2020, 641, A10. [Google Scholar] [CrossRef]
- Ade, P.A. et al. [BICEP, Keck Collaboration] Improved Constraints on Primordial Gravitational Waves using Planck, WMAP, and BICEP/Keck Observations through the 2018 Observing Season. Phys. Rev. Lett. 2021, 127, 151301. [Google Scholar] [CrossRef]
- Tristram, M.; Banday, A.J.; Górski, K.M.; Keskitalo, R.; Lawrence, C.R.; Andersen, K.J.; Barreiro, R.B.; Borrill, J.; Colombo, L.P.L.; Eriksen, H.K.; et al. Improved limits on the tensor-to-scalar ratio using BICEP and Planck data. Phys. Rev. D 2022, 105, 083524. [Google Scholar] [CrossRef]
- Frolovsky, D.; Ketov, S.V.; Saburov, S. Formation of primordial black holes after Starobinsky inflation. Mod. Phys. Lett. A 2022, 37, 2250135. [Google Scholar] [CrossRef]
- Saburov, S.; Ketov, S.V. Improved Model of Primordial Black Hole Formation after Starobinsky Inflation. Universe 2023, 9, 323. [Google Scholar] [CrossRef]
- Kamenshchik, A.Y.; Pozdeeva, E.O.; Tribolet, A.; Tronconi, A.; Venturi, G.; Vernov, S.Y. The Superpotential Method and the Amplification of Inflationary Perturbations. arXiv 2024. [Google Scholar] [CrossRef]
- Geller, S.R.; Qin, W.; McDonough, E.; Kaiser, D.I. Primordial black holes from multifield inflation with nonminimal couplings. Phys. Rev. D 2022, 106, 063535. [Google Scholar] [CrossRef]
- Cole, P.S.; Gow, A.D.; Byrnes, C.T.; Patil, S.P. Primordial black holes from single-field inflation: A fine-tuning audit. arXiv 2023. [Google Scholar] [CrossRef]
- Karam, A.; Koivunen, N.; Tomberg, E.; Vaskonen, V.; Veermäe, H. Anatomy of single-field inflationary models for primordial black holes. J. Cosmol. Astropart. Phys. 2023, 3, 013. [Google Scholar] [CrossRef]
- Kristiano, J.; Yokoyama, J. Ruling Out Primordial Black Hole Formation From Single-Field Inflation. arXiv 2024. [Google Scholar] [CrossRef]
- Choudhury, S.; Panda, S.; Sami, M. Quantum loop effects on the power spectrum and constraints on primordial black holes. J. Cosmol. Astropart. Phys. 2023, 11, 066. [Google Scholar] [CrossRef]
- Cheng, S.-L.; Lee, D.-S.; Ng, K.-W. Primordial perturbations from ultra-slow-roll single-field inflation with quantum loop effects. arXiv 2024. [Google Scholar] [CrossRef]
- Firouzjahi, H.; Riotto, A. Primordial Black Holes and Loops in Single-Field Inflation. arXiv 2023. [Google Scholar] [CrossRef]
- Davies, M.W.; Iacconi, L.; Mulryne, D.J. Numerical 1-loop correction from a potential yielding ultra-slow-roll dynamics. arXiv 2024. [Google Scholar] [CrossRef]
- Cai, Y.-F.; Chen, X.; Namjoo, M.H.; Sasaki, M.; Wang, D.-G.; Wang, Z. Revisiting non-Gaussianity from non-attractor inflation models. J. Cosmol. Astropart. Phys. 2018, 5, 12. [Google Scholar] [CrossRef]
- Abolhasani, A.A.; Firouzjahi, H.; Naruko, A.; Sasaki, M. Delta N Formalism in Cosmological Perturbation Theory; WSP: London, UK, 2019. [Google Scholar] [CrossRef]
- Appleby, S.A.; Battye, R.A.; Starobinsky, A.A. Curing singularities in cosmological evolution of F(R) gravity. J. Cosmol. Astropart. Phys. 2010, 6, 5. [Google Scholar] [CrossRef]
- Carr, B.; Kohri, K.; Sendouda, Y.; Yokoyama, J. Constraints on primordial black holes. Rept. Prog. Phys. 2021, 84, 116902. [Google Scholar] [CrossRef]
- Maeda, K.-I. Towards the Einstein-Hilbert Action via Conformal Transformation. Phys. Rev. D 2021, 39, 3159. [Google Scholar] [CrossRef]
- Frolovsky, D.; Ketov, S.V. Fitting power spectrum of scalar perturbations for primordial black hole production during inflation. Astronomy 2023, 2, 47–57. [Google Scholar] [CrossRef]
- Espinosa, J.R.; Racco, D.; Riotto, A. A Cosmological Signature of the SM Higgs Instability: Gravitational Waves. J. Cosmol. Astropart. Phys. 2018, 9, 012. [Google Scholar] [CrossRef]
- Kohri, K.; Terada, T. Semianalytic calculation of gravitational wave spectrum nonlinearly induced from primordial curvature perturbations. Phys. Rev. D 2018, 97, 123532. [Google Scholar] [CrossRef]
- Pi, S.; Sasaki, M. Gravitational Waves Induced by Scalar Perturbations with a Lognormal Peak. J. Cosmol. Astropart. Phys. 2020, 9, 037. [Google Scholar] [CrossRef]
- Domènech, G. Scalar Induced Gravitational Waves Review. Universe 2021, 7, 398. [Google Scholar] [CrossRef]
- Luca, V.D.; Franciolini, G.; Riotto, A. NANOGrav Data Hints at Primordial Black Holes as Dark Matter. Phys. Rev. Lett. 2021, 126, 041303. [Google Scholar] [CrossRef] [PubMed]
- Agazie, G. et al. [NANOGrav Collaboration] The NANOGrav 15 yr Data Set: Evidence for a Gravitational-wave Background. Astrophys. J. Lett. 2023, 951, L8. [Google Scholar] [CrossRef]
- Amaro-Seoane, P. et al. [LISA Collaboration] Laser Interferometer Space Antenna. arXiv 2017. [Google Scholar] [CrossRef]
- Smith, T.L.; Caldwell, R. LISA for Cosmologists: Calculating the Signal-to-Noise Ratio for Stochastic and Deterministic Sources. Phys. Rev. D 2019, 100, 104055. [Google Scholar] [CrossRef]
- Luo, J. et al. [TianQin Collaboration] TianQin: A space-borne gravitational wave detector. Class. Quant. Grav. 2016, 33, 035010. [Google Scholar] [CrossRef]
- Gong, X.; Lau, Y.K.; Xu, S.; Amaro-Seoane, P.; Bai, S.; Bian, X.; Cao, Z.; Chen, G.; Chen, X.; Ding, Y.; et al. Descope of the ALIA mission. J. Phys. Conf. Ser. 2015, 610, 012011. [Google Scholar] [CrossRef]
- Ruan, W.-H.; Guo, Z.-K.; Cai, R.-G.; Zhang, Y.-Z. Taiji program: Gravitational-wave sources. Int. J. Mod. Phys. A 2020, 35, 2050075. [Google Scholar] [CrossRef]
- Kudoh, H.; Taruya, A.; Hiramatsu, T.; Himemoto, Y. Detecting a gravitational-wave background with next-generation space interferometers. Phys. Rev. D 2006, 73, 064006. [Google Scholar] [CrossRef]
- Thrane, E.; Romano, J.D. Sensitivity curves for searches for gravitational-wave backgrounds. Phys. Rev. D 2013, 88, 124032. [Google Scholar] [CrossRef]
- Schmitz, K. New Sensitivity Curves for Gravitational-Wave Signals from Cosmological Phase Transitions. J. High Energy Phys. 2021, 1, 97. [Google Scholar] [CrossRef]
- Aldabergenov, Y.; Addazi, A.; Ketov, S.V. Testing Primordial Black Holes as Dark Matter in Supergravity from Gravitational Waves. Phys. Lett. B 2021, 814, 136069. [Google Scholar] [CrossRef]
- Press, W.H.; Schechter, P. Formation of galaxies and clusters of galaxies by selfsimilar gravitational condensation. Astrophys. J. 1974, 187, 425–438. [Google Scholar] [CrossRef]
- Inomata, K.; Kawasaki, M.; Mukaida, K.; Tada, Y.; Yanagida, T.T. Inflationary Primordial Black Holes as All Dark Matter. Phys. Rev. D 2017, 96, 043504. [Google Scholar] [CrossRef]
- Aldabergenov, Y.; Addazi, A.; Ketov, S.V. Primordial black holes from modified supergravity. Eur. Phys. J. C 2020, 80, 917. [Google Scholar] [CrossRef]
- Franciolini, G.; Kehagias, A.; Matarrese, S.; Riotto, A. Primordial Black Holes from Inflation and non-Gaussianity. J. Cosmol. Astropart. Phys. 2018, 3, 16. [Google Scholar] [CrossRef]
- Germani, C.; Sheth, R.K. The Statistics of Primordial Black Holes in a Radiation-Dominated Universe: Recent and New Results. Universe 2023, 9, 421. [Google Scholar] [CrossRef]
- Figueroa, D.G.; Raatikainen, S.; Rasanen, S.; Tomberg, E. Implications of stochastic effects for primordial black hole production in ultra-slow-roll inflation. J. Cosmol. Astropart. Phys. 2022, 5, 27. [Google Scholar] [CrossRef]
- Firouzjahi, H. One-loop corrections in power spectrum in single field inflation. J. Cosmol. Astropart. Phys. 2023, 10, 6. [Google Scholar] [CrossRef]
- Inomata, K. Curvature Perturbations Protected Against One Loop. arXiv 2024. [Google Scholar] [CrossRef]
- Kristiano, J.; Yokoyama, J. Generating large primordial fluctuations in single-field inflation for PBH formation. arXiv 2024. [Google Scholar] [CrossRef]
- Kristiano, J.; Yokoyama, J. Comparing sharp and smooth transitions of the second slow-roll parameter in single-field inflation. arXiv 2024. [Google Scholar] [CrossRef]
- Tada, Y.; Terada, T.; Tokuda, J. Cancellation of quantum corrections on the soft curvature perturbations. J. High Energy Phys. 2024, 1, 105. [Google Scholar] [CrossRef]
- Dvali, G.; Eisemann, L.; Michel, M.; Zell, S. Black hole metamorphosis and stabilization by memory burden. Phys. Rev. D 2020, 102, 103523. [Google Scholar] [CrossRef]
- Michel, M.; Zell, S. The Timescales of Quantum Breaking. Fortsch. Phys. 2023, 71, 2300163. [Google Scholar] [CrossRef]
- Alexandre, A.; Dvali, G.; Koutsangelas, E. New Mass Window for Primordial Black Holes as Dark Matter from Memory Burden Effect. arXiv 2024. [Google Scholar] [CrossRef]
- Thoss, V.; Burkert, A.; Kohri, K. Breakdown of Hawking Evaporation opens new Mass Window for Primordial Black Holes as Dark Matter Candidate. arXiv 2024. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saburov, S.; Ketov, S.V. Quantum Loop Corrections in the Modified Gravity Model of Starobinsky Inflation with Primordial Black Hole Production. Universe 2024, 10, 354. https://doi.org/10.3390/universe10090354
Saburov S, Ketov SV. Quantum Loop Corrections in the Modified Gravity Model of Starobinsky Inflation with Primordial Black Hole Production. Universe. 2024; 10(9):354. https://doi.org/10.3390/universe10090354
Chicago/Turabian StyleSaburov, Sultan, and Sergei V. Ketov. 2024. "Quantum Loop Corrections in the Modified Gravity Model of Starobinsky Inflation with Primordial Black Hole Production" Universe 10, no. 9: 354. https://doi.org/10.3390/universe10090354
APA StyleSaburov, S., & Ketov, S. V. (2024). Quantum Loop Corrections in the Modified Gravity Model of Starobinsky Inflation with Primordial Black Hole Production. Universe, 10(9), 354. https://doi.org/10.3390/universe10090354





