Soft X-ray Spectrum Changes over the 35-Day Cycle in Hercules X-1 Observed with AstroSat SXT
Abstract
1. Introduction
2. Data and Analysis
2.1. Lightcurve Analysis
2.2. X-ray Spectrum Analysis
3. Results
4. Discussion
4.1. Lightcurve of Her X-1
4.2. Spectrum of Her X-1
4.3. Comparison with Previous Work
5. Summary and Conclusions
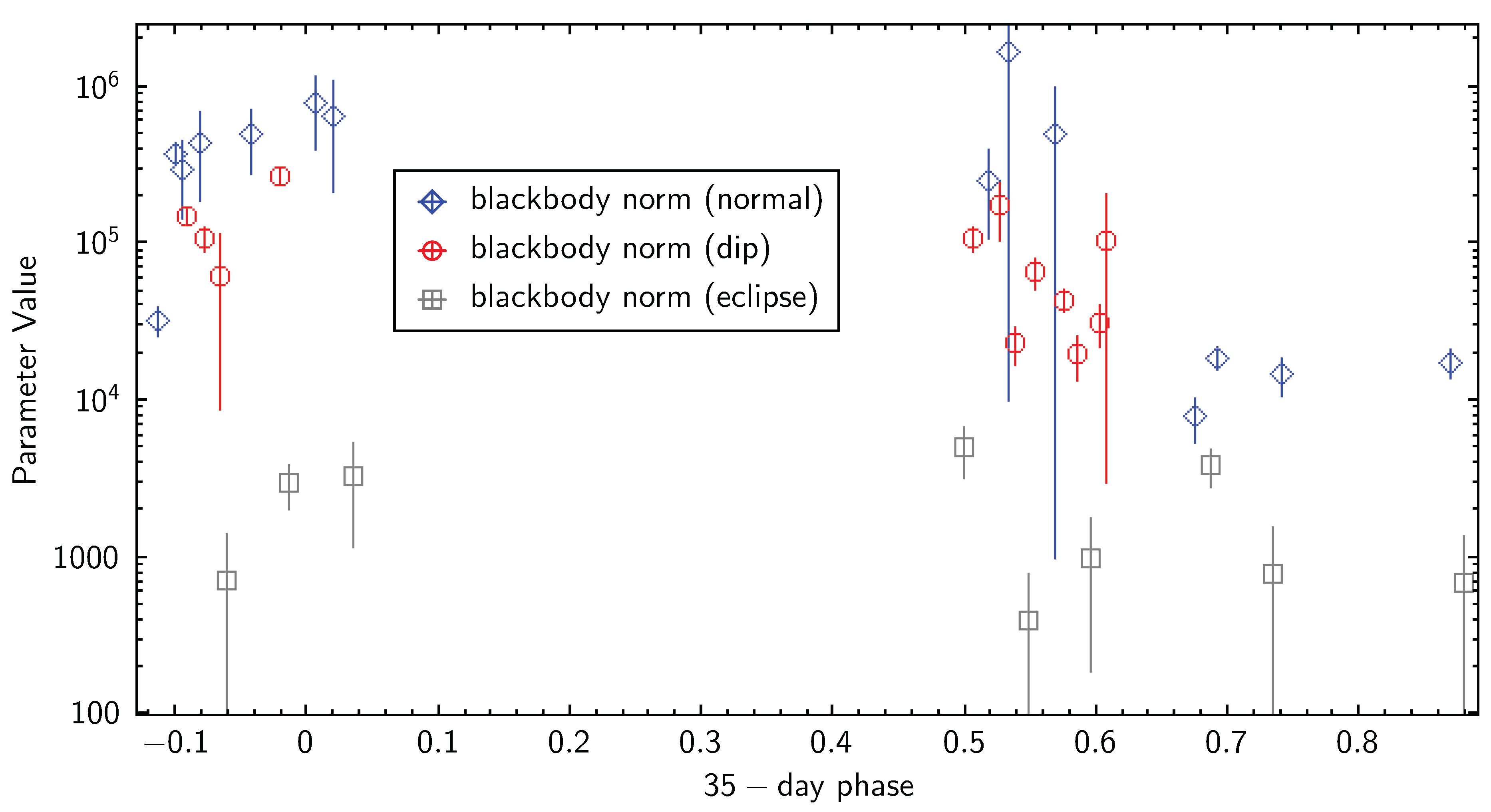
- The powerlaw normalization is nearly constant during eclipses at all 35-day phases. This means that the scattering corona in Her X-1 is not variable with 35-day phase.
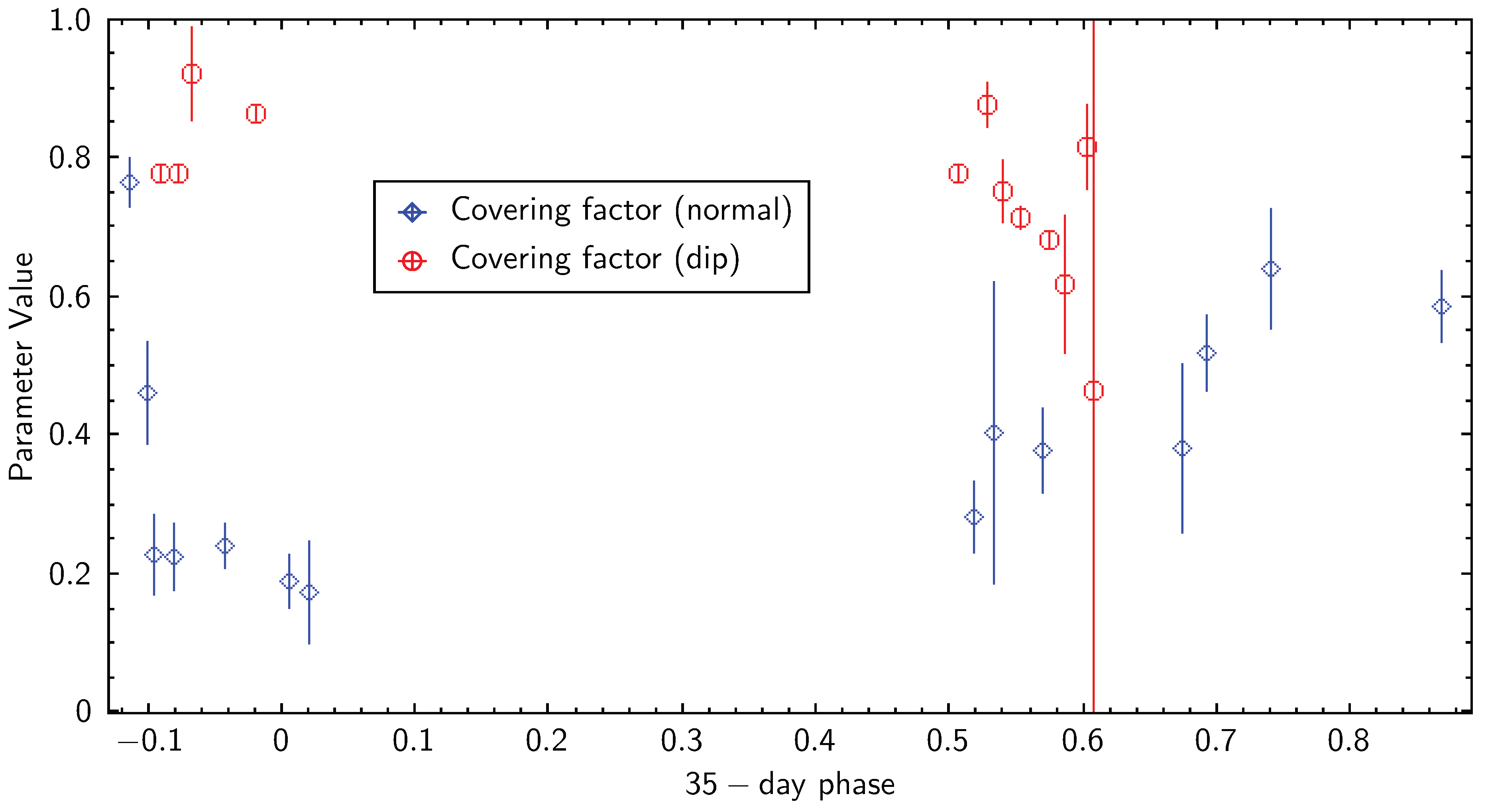
- The column density for dips and outside dips is the same. Dips have a significant increase in covering fraction compared to non-dip times.
- The blackbody component, which originates from the hot inner edge of the accretion disk, was measured as a function of 35-day phase. During dips, it is smaller by a factor of ∼2–3. During LS and during eclipses, there are only small residual components of ∼4% (for LS) and ∼1% (for eclipses), which are emissions scattered by the part of the corona that is larger than the accretion disk (for LS) or larger than the companion star (for eclipses). The blackbody has a different 35-day variability compared to the powerlaw norm, which is likely related to the extended nature of the blackbody (area ∼ km2).
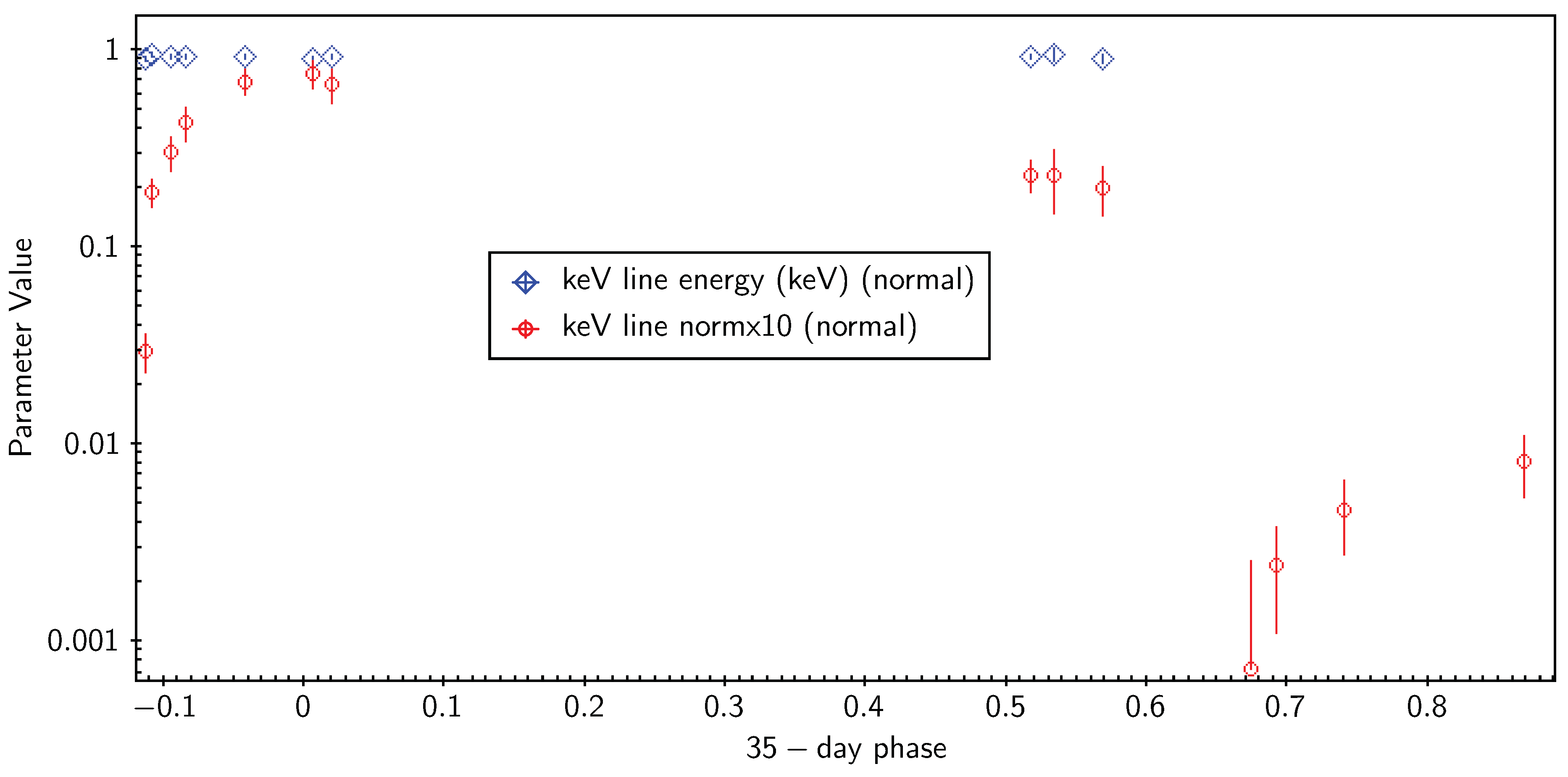
- The 1 keV line norm has similar 35-day variability to the powerlaw norm and different from that of the blackbody norm. This is consistent with the 1 keV component originating from a small region, similar to the powerlaw component. This region may be located along the magnetospheric flow of matter from the inner edge of the accretion disk onto the neutron star.
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Her X-1 | Hercules X-1 |
| SXT | Soft X-ray Telescope |
| MH | Main High |
| SH | Short High |
| LS | Low State |
| BAT | Burst Alert Telescope |
| RXTE | Rossi X-ray Timing Explorer |
| NUV | near ultraviolet |
| FUV | far ultraviolet |
| LAXPC | Large Area X-ray Proportional Counter |
| CZTI | Cadmium Zinc Telluride Imager |
| PC | Photon Counting |
| MJD | Modified Julian Date |
| MAXI | Monitor for All sky X-ray Instrument |
| PL | powerlaw model in Xspec |
| BB | bbodyrad in Xspec |
| ASM | All Sky Monitor |
| PCA | Proportional Counter Array |
| NuSTAR | Nuclear Spectroscopic Telescope Array |
References
- Scott, D.; Leahy, D.; Wilson, R. The 35 Day Evolution of the Hercules X-1 Pulse Profile: Evidence for a Resolved Inner Disk Occultation of the Neutron Star. Astrophys. J. 2000, 539, 392S. [Google Scholar] [CrossRef]
- Reynolds, A.; Quaintrell, H.; Still, M.; Roche, P.; Chakrabarty, D.; Levine, S. A new mass estimate for Hercules X-1. Mon. Not. R. Astron. Soc. 1997, 288, 43–52. [Google Scholar] [CrossRef][Green Version]
- Leahy, D.; Abdallah, M. HZ Her: Stellar Radius from X-ray Eclipse Observations, Evolutionary State, and a New Distance. Astrophys. J. 2014, 793, 79. [Google Scholar] [CrossRef][Green Version]
- Gerend, D.; Boynton, P. Optical clues to the nature of Hercules X-1/HZ Herculis. Astrophys. J. 1976, 209, 652. [Google Scholar] [CrossRef]
- Leahy, D.A.; Marshall, H. Extreme-Ultraviolet Explorer Observations of Hercules X-1 at the End of the Short High State. Astrophys. J. 1999, 521, 328. [Google Scholar] [CrossRef]
- Leahy, D.A. An accretion column model for the accreting pulsar Her X-1. Mon. Not. R. Astron. Soc. 2004, 348, 932–936. [Google Scholar] [CrossRef]
- Becker, P.; Wolff, M. Thermal and Bulk Comptonization in Accretion-powered X-ray Pulsars. Astrophys. J. 2007, 654, 435–457. [Google Scholar] [CrossRef]
- Inoue, H. X-ray emissions from magnetic polar regions of neutron stars. Publ. Astron. Soc. Jpn. 2020, 72, 12. [Google Scholar] [CrossRef]
- McCray, R.; Shull, M.; Boynton, P.; Deeter, J.; Holt, S.; White, N. Einstein Observatory pulse-phase spectroscopy of HER X-1. Astrophys. J. 1982, 262, 301. [Google Scholar] [CrossRef]
- Staubert, R.; Shakura, N.I.; Postnov, K.; Wilms, J.; Rothschild, R.E.; Coburn, W.; Rodina, L.; Klochkov, D. Discovery of a flux-related change of the cyclotron line energy in Hercules X-1. Astron. Astrophyics 2007, 465, L25–L28. [Google Scholar] [CrossRef]
- Vasco, D.; Klochkov, D.; Staubert, R. Hercules X-1: The positive cyclotron line energy/luminosity correlation. Astron. Astrophyics 2011, 532, A99. [Google Scholar] [CrossRef]
- Shakura, N.; Ketsaris, N.; Prokhorov, M.; Postnov, K. RXTE highlights of the 34.85-day cycle of HER X-1. Mon. Not. R. Astron. Soc. 1998, 300, 992–998. [Google Scholar] [CrossRef][Green Version]
- Scott, D.; Leahy, D. Rossi X-ray Timing Explorer All-Sky Monitor Observations of the 35 Day Cycle of Hercules X-1. Astrophys. J. 1999, 510, 974–985. [Google Scholar] [CrossRef]
- Leahy, D.; Wang, Y. Swift/BAT and RXTE/ASM Observations of the 35 day X-ray Cycle of Hercules X-1. Astrophys. J. 2020, 902, 146. [Google Scholar] [CrossRef]
- Leahy, D.; Wang, Y. The 35-Day Cycle of Hercules X-1 in Multiple Energy Bands from MAXI and Swift/BAT Monitoring. Universe 2021, 7, 160. [Google Scholar] [CrossRef]
- Petterson, J. Hercules X-1: A neutron star with a twisted accretion disk? Astrophys. J. 1975, 201, L61–L64. [Google Scholar] [CrossRef]
- Leahy, D. Modelling RXTE/ASM observations of the 35-d cycle in Her X-1. Mon. Not. R. Astron. Soc. 2002, 334, 847–854. [Google Scholar] [CrossRef]
- Leahy, D.; Postma, J.; Chen, Y. AstroSat UVIT Observations of Her X-1. Astrophys. J. 2020, 889, 131. [Google Scholar] [CrossRef]
- Inoue, H. Reproductions of super-orbital X-ray light-curves with the precessing accretion ring model and implications on accretion flows through accretion rings. Publ. Astron. Soc. Jpn. 2019, 71, 36. [Google Scholar] [CrossRef]
- Crosa, L.; Boynton, P. Periodic mass transfer in HER X-1/HZ Her. Astrophys. J. 1980, 235, 999–1015. [Google Scholar] [CrossRef]
- Igna, C.D.; Leahy, D.A. Light-curve dip production through accretion stream-accretion disc impact in the HZ Her/Her X-1 binary star system. Mon. Not. R. Astron. Soc. 2012, 425, 8. [Google Scholar] [CrossRef][Green Version]
- Leahy, D. Hercules X-1: Using Eclipse to Measure the X-ray Corona. Astrophys. J. 2015, 800, 32. [Google Scholar] [CrossRef]
- Singh, K.P.; Tandon, S.N.; Agrawal, P.C.; Antia, H.M.; Manchanda, R.K.; Yadav, J.S.; Seetha, S.; Ramadevi, M.C.; Rao, A.R.; Bhattacharya, D.; et al. ASTROSAT mission. SPIE Conf. Ser. 2014, 9144, 91441S. [Google Scholar]
- Singh, K.P.; Stewart, G.C.; Westergaard, N.J.; Bhattacharayya, S.; Chandra, S.; Chitnis, V.R.; Dewangan, G.C.; Kothare, A.T.; Mirza, I.M.; Mukerjee, K.; et al. Soft X-ray Focusing Telescope Aboard AstroSat: Design, Characteristics and Performance. J. Astrophys. Astron. 2017, 38, 29. [Google Scholar] [CrossRef]
- Staubert, R.; Klochkov, D.; Wilms, J. Updating the orbital ephemeris of Hercules X-1; rate of decay and eccentricity of the orbit. Astron. Astrophys. 2009, 500, 883. [Google Scholar] [CrossRef]
- Leahy, D.; Gonzalez-Enriquez, S. The RXTE/PCA 35-Day X-ray Lightcurve of Hercules X-1. Int. J. Astron. Astrophys. 2023, 13, 172–194. [Google Scholar] [CrossRef]
- Choi, C.; Nagase, F.; Makino, F.; Dotani, T.; Min, K. An X-ray Spectroscopic Study of the Pre-Eclipse Dips of Hercules X-1. Astrophys. J. 1994, 422, 799. [Google Scholar] [CrossRef]
- Leahy, D.; Yoshida, A.; Matsuoka, M. Spectral Evolution during Pre-Eclipse Dips in Hercules X-1. Astrophys. J. 1994, 434, 341. [Google Scholar] [CrossRef]
- Leahy, D.; Chen, Y. AstroSat SXT Observations of Her X-1. Astrophys. J. 2019, 871, 152. [Google Scholar] [CrossRef]
- Mihara, T.; Ohashi, T.; Makishima, K.; Nagase, F.; Kitamoto, S.; Koyama, K. X-ray Spectrum of Hercules X-1 in the Low State of the 35-Day Cycle. Publ. Astron. Soc. Jpn. 1991, 43, 501. [Google Scholar]
- Leahy, D. GINGA observations of absorption dips in Hercules X-1. Mon. Not. R. Astron. Soc. 1997, 287, 622–628. [Google Scholar] [CrossRef][Green Version]
- Kuster, M.; Wilms, J.; Staubert, R.; Heindl, W.A.; Rothschild, R.E.; Shakura, N.I.; Postnov, K.A. Probing the outer edge of an accretion disk: A Her X-1 turn-on observed with RXTE. Astron. Astrophys. 2005, 443, 753. [Google Scholar] [CrossRef]
- Brumback, M.C.; Hickox, R.C.; Fürst, F.S.; Pottschmidt, K.; Tomsick, J.A.; Wilms, J.; Staubert, R.; Vrtilek, S. A Broadband X-ray View of the Precessing Accretion Disk and Pre-eclipse Dip in the Pulsar Her X-1 with NuSTAR and XMM-Newton. Astrophys. J. 2021, 909, 186. [Google Scholar] [CrossRef]
- Fuerst, F.; Grefenstette, B.W.; Staubert, R.; Tomsick, J.A.; Bachetti, M.; Barret, D.; Bellm, E.C.; Boggs, S.E.; Chenevez, J.; Christensen, F.E.; et al. The Smooth Cyclotron Line in Her X-1 as Seen with Nuclear Spectroscopic Telescope Array. Astrophys. J. 2013, 799, 69. [Google Scholar] [CrossRef]
- Postnov, K.; Shakura, N.; Staubert, R.; Kochetkova, A.; Klochkov, D.; Wilms, J. Variable neutron star free precession in Hercules X-1 from evolution of RXTE X-ray pulse profiles with phase of the 35-d cycle. Mon. Not. R. Astron. Soc. 2013, 435, 1147–1164. [Google Scholar] [CrossRef]
- Mönkkönen, J.; Tsygankov, S.S.; Mushtukov, A.A.; Doroshenko, V.; Suleimanov, V.F.; Poutanen, J. Constraints on the magnetic field structure in accreting compact objects from aperiodic variability. Mon. Not. R. Astron. Soc. 2022, 515, 571–580. [Google Scholar] [CrossRef]
- Asami, F.; Enoto, T.; Iwakiri, W.; Yamada, S.; Tamagawa, T.; Mihara, T.; Nagase, F. Broad-band spectroscopy of Hercules X-1 with Suzaku. Publ. Astron. Soc. Jpn. 2014, 66, 44. [Google Scholar] [CrossRef]
- Fiume, D.D.; Orlandini, M.; Cusumano, G.; Del Sordo, S.; Feroci, M.; Frontera, F.; Oosterbroek, T.; Palazzi, E.; Parmar, A.N.; Santangelo, A.; et al. The broad-band (0.1–200 keV) spectrum of HER X-1 observed with BeppoSAX. Astron. Astrophys. 1998, 329, L41. [Google Scholar]
- Leahy, D.; Abdallah, M. Spectral Evolution of Hercules X-1 over Main High State from RXTE/PCA Observations. J. High Energy Phys. Gravit. Cosmol. 2022, 8, 896–918. [Google Scholar] [CrossRef]
- Abdallah, M.; Leahy, D. Spectral signature of atmospheric reflection in Hercules X-1/HZ Hercules during low and short high states. Mon. Not. R. Astron. Soc. 2015, 453, 4222–4231. [Google Scholar] [CrossRef]
- Garg, A.; Rawat, D.; Bhargava, Y.; Méndez, M.; Bhattacharyya, S. Flux-resolved Spectropolarimetric Evolution of the X-ray Pulsar Hercules X-1 Using IXPE. Astrophys. J. Lett. 2023, 948, L10. [Google Scholar] [CrossRef]
- Doroshenko, V.; Poutanen, J.; Tsygankov, S.S.; Suleimanov, V.F.; Bachetti, M.; Caiazzo, I.; Costa, E.; Di Marco, A.; Heyl, J.; La Monaca, F.; et al. Determination of X-ray pulsar geometry with IXPE polarimetry. Nat. Astron. 2022, 6, 1433–1443. [Google Scholar] [CrossRef]
- Heyl, J.; Doroshenko, V.; González-Caniulef, D.; Caiazzo, I.; Poutanen, J.; Mushtukov, A.; Tsygankov, S.S.; Kirmizibayrak, D.; Bachetti, M.; Pavlov, G.G.; et al. X-ray Polarization Reveals the Precessions of the Neutron Star in Hercules X-1. arXiv 2023, arXiv:2311.0366. [Google Scholar]
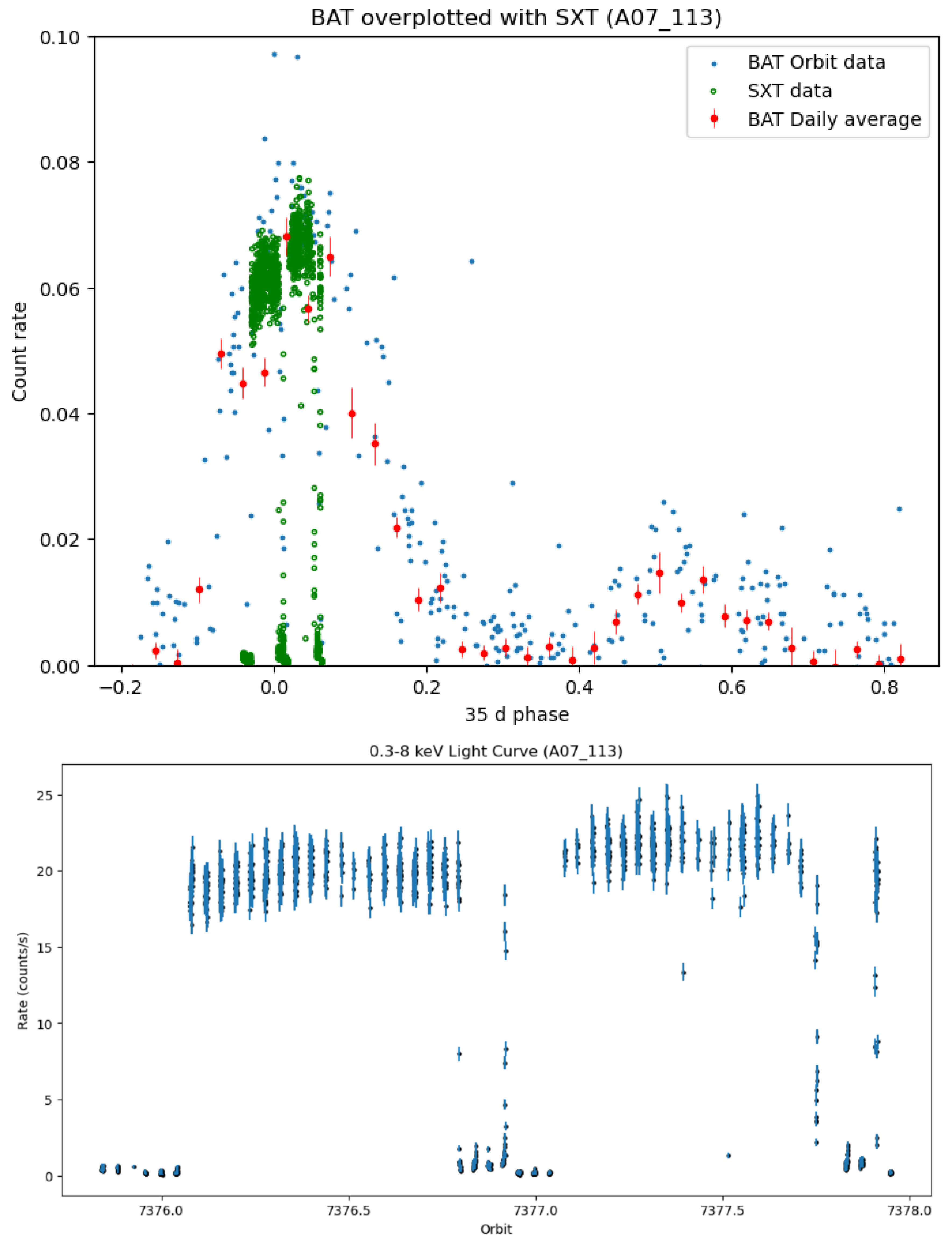
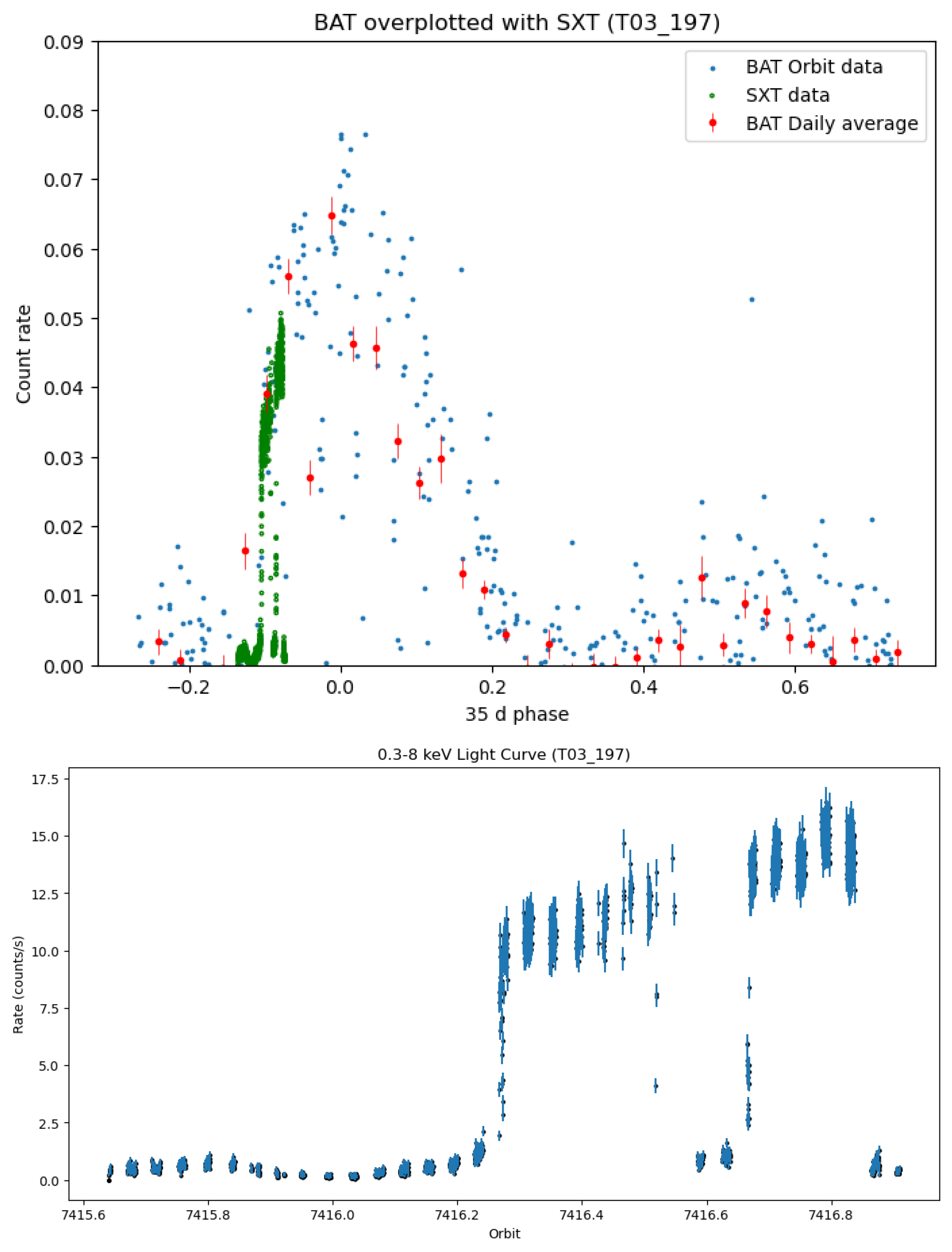
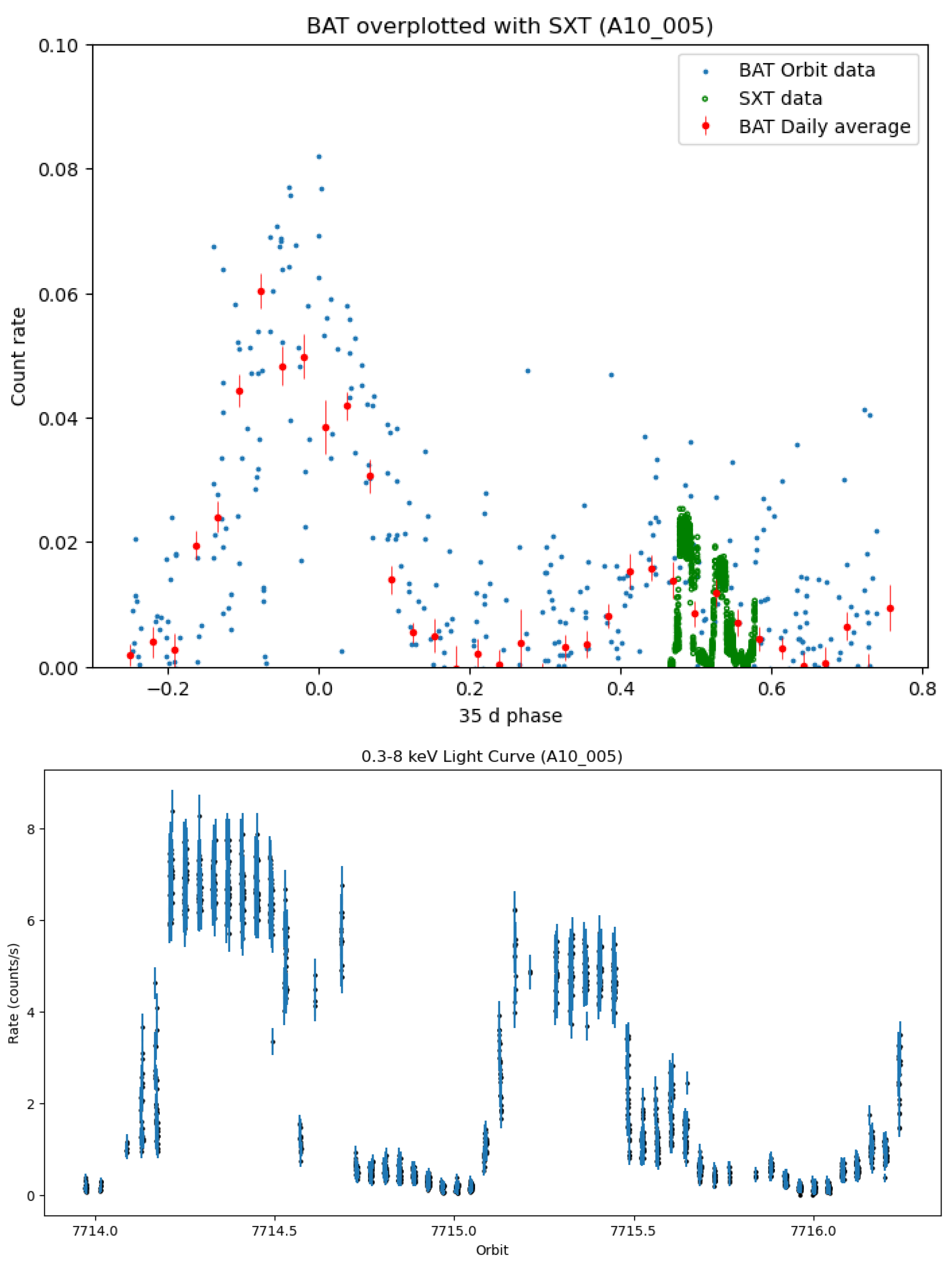
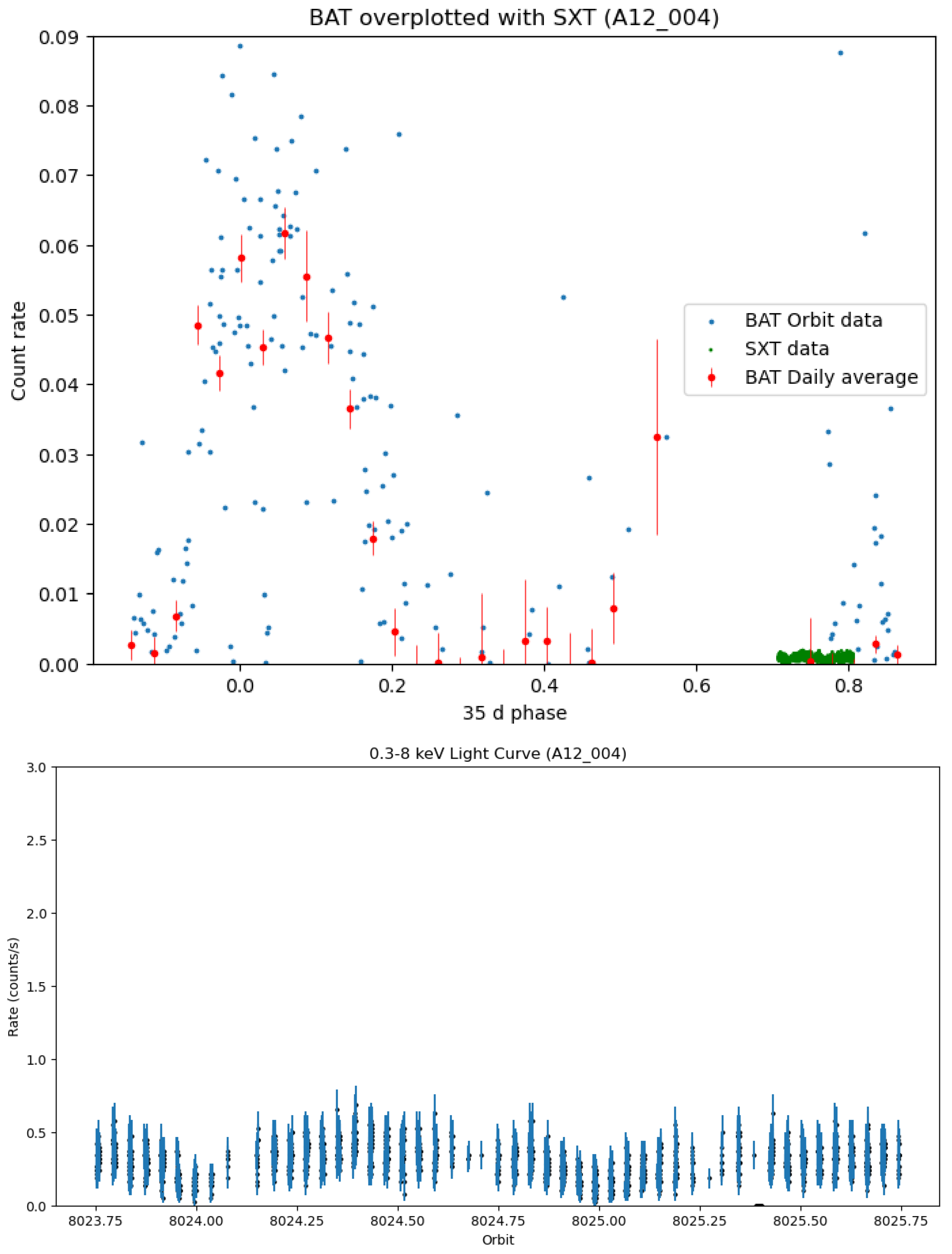




| Observation | Name | MJD Start | Duration(s) | Exposure(s) |
|---|---|---|---|---|
| A07_113: | ||||
| 5.890003284 | 1.262 | 1.524 | ||
| 5.890023116 | 1.303 | 2.912 | ||
| 5.890043410 | 1.057 | 2.123 | ||
| 5.890165747 | 1.841 | 4.964 | ||
| 5.890192234 | 1.306 | 3.259 | ||
| 5.890213748 | 4.662 | 9.541 | ||
| 5.890300361 | 1.814 | 2.513 | ||
| 5.890361353 | 8.702 | 8.702 | ||
| T03_197: | ||||
| 5.896769825 | 4.161 | 1.106 | ||
| 5.896822291 | 1.348 | 4.027 | ||
| 5.896842591 | 2.551 | 1.064 | ||
| 5.896876420 | 1.224 | 1.224 | ||
| 5.896877837 | 4.054 | 9.886 | ||
| 5.896924762 | 1.733 | 4.118 | ||
| 5.896944823 | 2.487 | 1.000 | ||
| 5.896977908 | 6.897 | 3.179 | ||
| A10_005: | ||||
| 5.947491474 | 6.597 | 1.079 | ||
| 5.947511075 | 1.304 | 2.606 | ||
| 5.947531371 | 4.810 | 1.182 | ||
| 5.947593065 | 6.443 | 6.443 | ||
| 5.947600323 | 1.110 | 7.489 | ||
| 5.947619319 | 3.056 | 7.898 | ||
| 5.947659910 | 1.302 | 3.997 | ||
| 5.947680205 | 7.177 | 2.294 | ||
| 5.947694658 | 4.144 | 6.728 | ||
| 5.947747858 | 2.470 | 6.605 | ||
| 5.947781735 | 3.634 | 5.161 | ||
| 5.947829039 | 1.300 | 3.930 | ||
| 5.947849334 | 1.884 | 5.233 | ||
| 5.947876395 | 7.774 | 7.774 | ||
| A12_004: | ||||
| 6.000159288 | 2.473 | 6.811 | ||
| 6.000193090 | 1.305 | 3.138 | ||
| 6.000214675 | 1.229 | 2.270 | ||
| 6.000362104 | 1.760 | 4.294 | ||
| 6.000382471 | 9.995 | 2.117 |
| State/Example | ( cm−2) | Covering Factor, | PL Norm (Photons/keV/cm2/s at 1 keV) | PL | 1 keV Line Norm (Photons/cm2/s) | BB Norm () b | BB kT (keV) | Fe K Line Norm (Photons/cm2/s) |
|---|---|---|---|---|---|---|---|---|
| MH | free | free | free | free | free | free | free | free |
| SH | free | free | free | free | free | free | free | omitted |
| LS | free | free | free | fixed | free | free | fixed | omitted |
| 0.95 | 0.10 | |||||||
| dip | free | free | free | fixed | free | free | fixed | omitted |
| 0.95 | 0.10 | |||||||
| eclipse | fixed | fixed | free | fixed | omitted | free | fixed | omitted |
| 13 | 0.24 | 0.95 | 0.10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Leahy , D.; Sharma , R. Soft X-ray Spectrum Changes over the 35-Day Cycle in Hercules X-1 Observed with AstroSat SXT. Universe 2024, 10, 298. https://doi.org/10.3390/universe10070298
Leahy D, Sharma R. Soft X-ray Spectrum Changes over the 35-Day Cycle in Hercules X-1 Observed with AstroSat SXT. Universe. 2024; 10(7):298. https://doi.org/10.3390/universe10070298
Chicago/Turabian StyleLeahy , Denis, and Riddhiman Sharma . 2024. "Soft X-ray Spectrum Changes over the 35-Day Cycle in Hercules X-1 Observed with AstroSat SXT" Universe 10, no. 7: 298. https://doi.org/10.3390/universe10070298
APA StyleLeahy , D., & Sharma , R. (2024). Soft X-ray Spectrum Changes over the 35-Day Cycle in Hercules X-1 Observed with AstroSat SXT. Universe, 10(7), 298. https://doi.org/10.3390/universe10070298







