Premerger Phenomena in Neutron Star Binary Coalescences
Abstract
:1. Introduction
1.1. Structure and Purpose of This Review
1.2. Remarks on Notation
2. Neutron Star Macrostructure
2.1. Equations of State
2.1.1. WFF Family
2.1.2. APR Family
2.1.3. SLy Family
2.1.4. BSk Family
2.2. Rotation and Binary Alignment
2.3. Magnetic Fields
- ★
- ★
- ★
- The morphology of pulsed emissions are highly varied, with some systems displaying long-term epochs of nulling or interpulses. Interpulse phenomenology in radio pulsars can be qualitatively explained by an oblique rotator with a multipolar magnetic field, as the emissions are then composed of multiple components [96]. The X-ray light curve from the magnetar SGR1900+14 also displays interpulse-like phenomena, which may be contributed by multiple hotspots on the surface [97]. Zhang et al. [98] suggest that starspots (i.e., localised and multipolar fields) may emerge through Hall evolution near the poles of neutron stars that are hovering around the death line, sporadically allowing the hosts to pulse and possibly explaining nulling (see also [99]).
- ★
- Multipoles are typically generated via cascade phenomena through Hall drift [99] or plastic flows [100] in neutron star crusts if the “magnetic Reynolds number” is sufficiently large ( G [83]), and likely also through ambipolar diffusion in the core [101]. For crustal fields with a high-degree of relative helicity, inverse cascades can instead operate [102], where energy is transferred from small to large scales, though this presupposes an initially multipolar field. Either way, Hall–Ohm simulation outputs are supported by observations of neutron-star cooling and (more speculatively) field decay.
- ★
- Many pulsar braking indices differ from the canonical dipole value of three, which points towards a complicated field geometry, anomalous braking torques, and/or mass-loaded winds initiating a different spin-down behaviour [103,104]. Glitch activity [105], Hall waves launched from superconducting phase transitions [106], or inclination angle evolution [107] may also notably affect this index, .
- ★
- Simulations of accretion show that even small accretion columns (or ‘magnetic mountains’) warp field lines far from the column itself [84,86,93], with field line compression within the equatorial belt persisting over long, Ohmic timescales [87]. Given that all neutron stars born from core collapse exhibit some degree of fallback accretion at birth from a temporary disc of bound material, one might expect all stars to have ‘buried’ and multipolar components [108]. Even ignoring this possibility, particle production and backflow in the magnetosphere will gradually advect field lines, instigating some (small) degree of multipolarity surviving over diffusion timescales. Such considerations were initially motivated by the observation that neutron stars in low-mass X-ray binaries (LMXBs; undergoing Roche lobe overflow) tend to possess unusually low magnetic field strengths [109].
- ★
- Cyclotron resonant scattering line energies demand that a number of accreting and isolated neutron stars possess local fields (much) stronger than those implied by global, dipole-field observations [110].
- ★
- Current bundles injected into the magnetosphere by crustal motions twist the fields there, inducing multipolarity [111]. Models of neutron star activity often invoke crustal failures as seeding events for outbursts (such as glitches or flares, e.g., [112,113,114]), and therefore, such injections may be common. In fact, crust failures are one of the mechanisms proposed for premerger precursors; see Section 6.
- ★
- Precession in magnetars, such as 4U 0142+61 [115] and SGR 1900+14 [116], are most straightforwardly explained through a (sub)crustal toroidal field of strength G: such a field introduces a prolate distortion along the magnetic axis, which then becomes misaligned with the rotation axis, causing free precession (see also [117,118]).
- ★
- ★
- Dynamo models aiming to explain the intense fields of magnetars rely on poloidal–toroidal amplification cycles. More generally, differential rotation will wind up poloidal lines. Given that some differential rotation is inescapable, as the spin-down torque applied to the surface cannot instantaneously influence the core (i.e., there is a crust–core lag; [122]), toroidal components should be generated.
- ★
3. Neutron Star Microstructure
3.1. Basic Elements of Crustal Physics
Supporting Stress
3.2. Breaking Strain
Mountains
3.3. Stratification Gradients
3.4. Superfluidity and Superconductivity
4. The Mechanics of Late Inspirals: Gravitational Waves
4.1. Tides: General Theory
4.1.1. Mathematical Description and Calculation Methods
4.1.2. Tides: Equilibrium
4.1.3. Tides: Dynamical
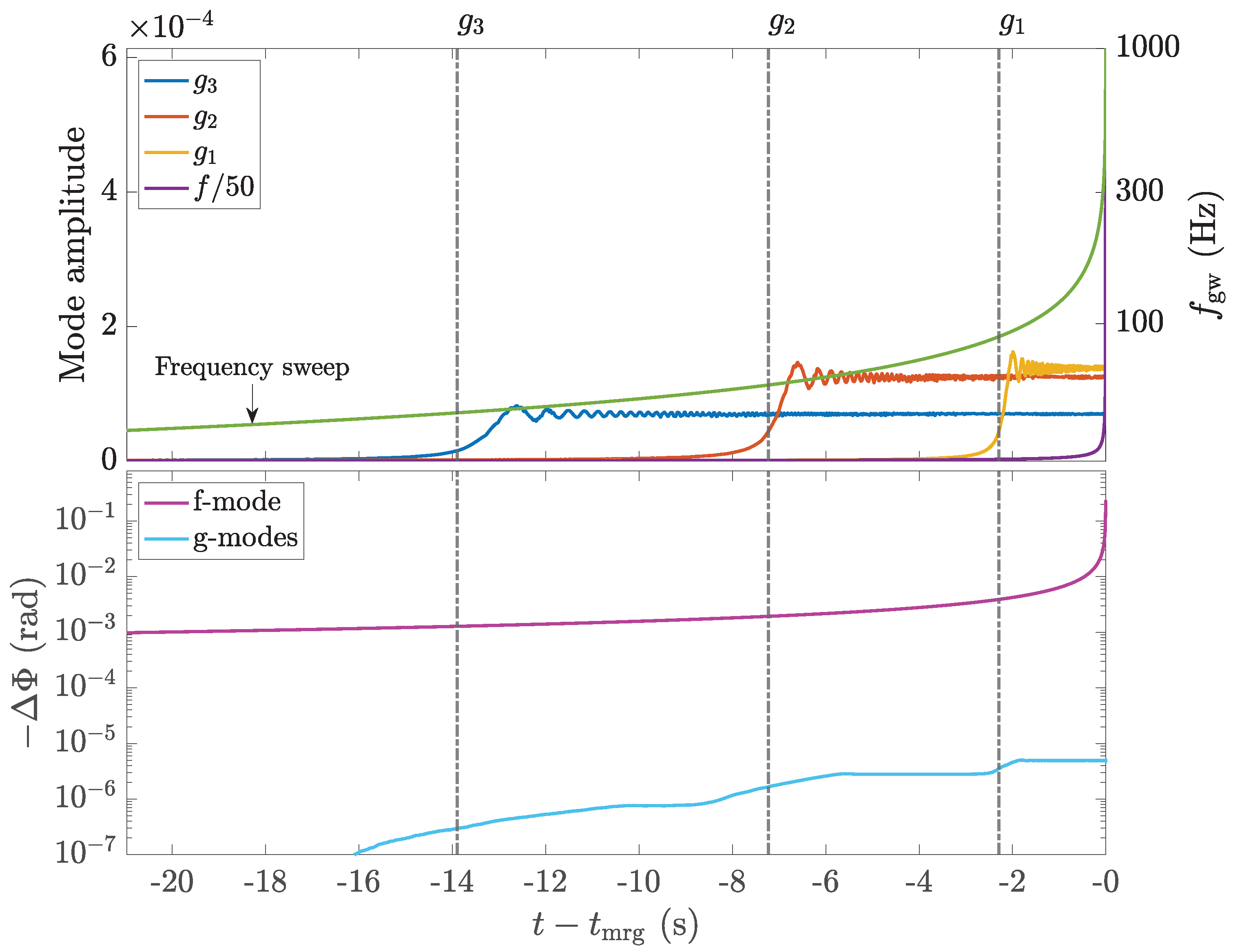
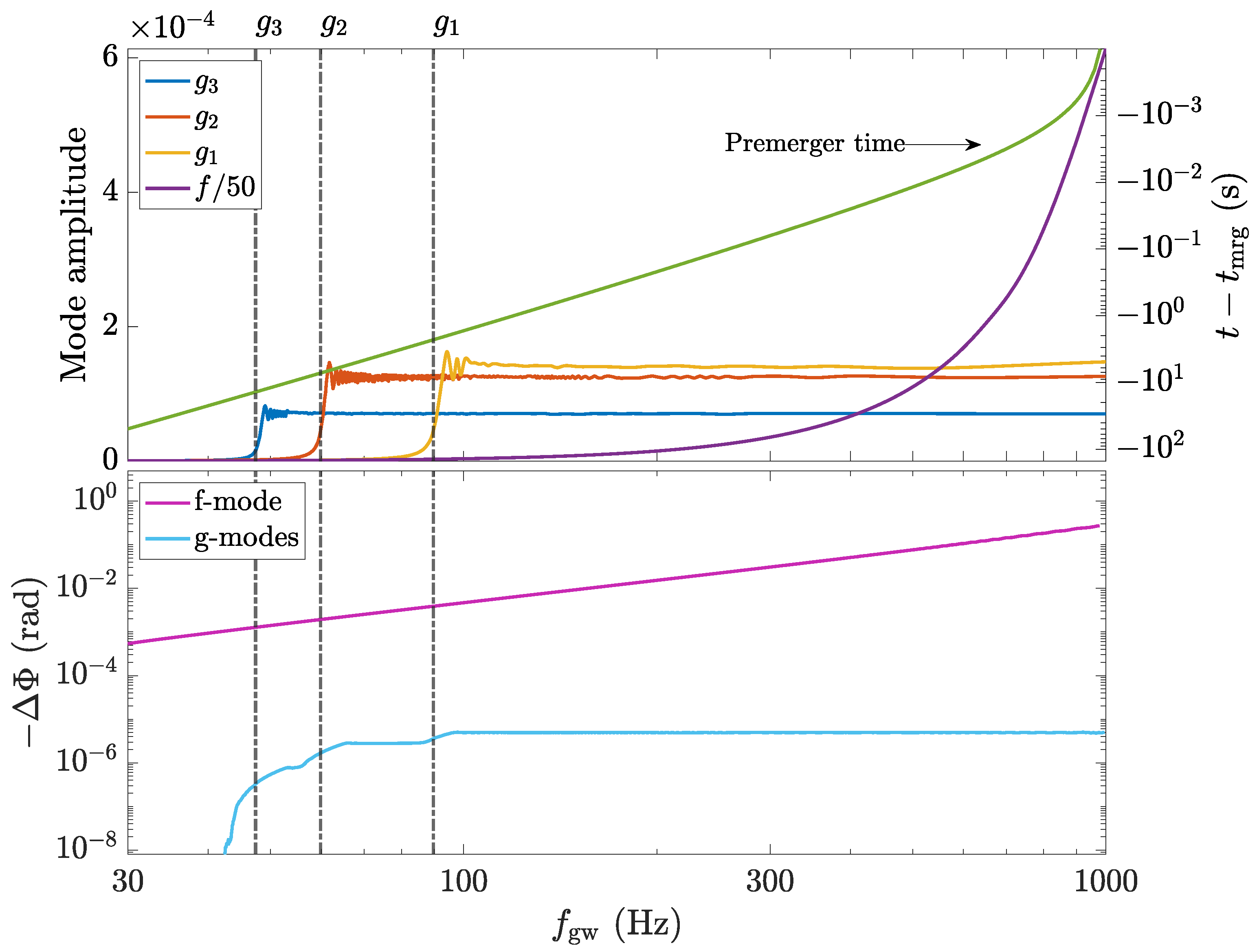
4.2. Spectral Modulations: General Considerations
4.2.1. Tidal Corrections
4.2.2. Curvature (Frame-Dragging)
4.3. Spin Effects
- (i)
- Centrifugal forces deform the star starting at order . For a uniform density object, the rotational oblateness is estimated by , where is the Keplerian break up value (e.g., [316]). In much the same way that equilibrium tides impact the evolution through a quadrupolar deformation, so too does the rotation (e.g., [317]).
- (ii)
- Modes are generally split into prograde and retrograde families through the azimuthal number m. That is to say, when , the mode frequencies and eigenfunctions depend on m, which can be either positive or negative. This splitting leads to a more complicated set of couplings and dynamical tides (as described in Section 4.1.3). Moreover, if the star is spinning sufficiently fast such that while , an allegedly retrograde mode instead appears as prograde in the inertial frame (24)—from which radiation reaching infinity is measured—and thus is subject to the Chandrasekhar–Friedman–Schutz (CFS) instability [313,314,315,318].
- (iii)
- Spin–orbit misalignment changes the Wigner coefficient and hence the tidal coupling, which couples in with point ii above.
- (iv)
Tidal Spin-up
4.4. Magnetic Effects
Internal Fields?
4.5. Thermal and Viscous Effects
Crust Melting?
4.6. Residual Eccentricity
4.7. Remarks on Merger Simulations and Future Challenges
5. Precursor Flares: Observations
5.1. Statistical Preliminaries
5.2. GRBs: Short, Long, or Ultra-Long?
Excluded Events
5.3. Rarity
5.4. Spectral Inferences
5.5. Waiting Times
5.6. Some Exceptional Precursors
5.6.1. GRB 211211A
5.6.2. GRB 180703B
5.6.3. GRB 180511437
5.6.4. GRB 191221A
5.6.5. GRB 090510
5.6.6. GRB 230307A
5.7. Connections with Post-Merger Phenomena
6. Precursor Flares: Theory
6.1. Delay Timescales: Post-Merger Jets
6.2. Magnetospheric Interaction and Unipolar Inductor
6.3. Resonant Failure: Luminosity and Timescales
6.3.1. Energetics
6.3.2. Resonance Window Duration
6.3.3. Launching Timescale
6.4. Resonant Failure: Some Important Families
6.4.1. i-Modes
6.4.2. g-Modes
6.4.3. Ocean Modes
6.4.4. f-Modes
6.4.5. r-Modes
6.5. Late-Stage Dynamos?
6.6. Post-Merger Models
7. Multimessenger Outlook
- Love numbers. The effective parameter , the quadrupolar member of which is defined by (39), is directly visible in the gravitational waveform at a leading PN order (see Table 2). Since this quantity depends on the stellar masses and integrals taken over by the internal density, it is clear that EOS information can be gleaned. This is effectively illustrated in Figure 2, showing mass–radius contours from GW170817.
- Asteroseismology. Dynamical tides also imprint themselves on the waveform. However, since these emerge at finite, non-zero frequencies, while the former appear already “at infinity”, they are generally subleading. It has been estimated that only in of neutron-star mergers will one be able to cleanly isolate the impact of dynamical tides with current detectors [292]. However, owing to the discussion provided in Section 4.1.3, there are open questions in this direction which are worth revisiting. For example, the g-modes may be comparable contributors to the dephasing if the f-mode frequency is very high (cf. Figure 9). Strong magnetic fields may also be important, either through modulating the mode frequencies directly or instigating an electromotive spin-up (Section 4.4), which continuously shifts the spectra. Out-of-equilibrium effects could also distort the spectrum in a complicated, time-dependent way [186].
- Gamma-ray precursors. Depending on the ignition mechanism, different kinds of information may be discernible. For premerger precursors, we argue the resonant failure picture can adequately explain all the observational characteristics (Section 6.3), though admittedly this is due to the huge range of QNM properties that neutron stars can exhibit. As resonances are obviously tied to the mode spectra, everything above applies here too but in the gamma-ray band. Similarly, since the activity of these modes in the crust is the relevant aspect here, microphysical inputs become critical. With the above three (Love number, dynamical tides, and precursors), one may thus learn about both macro- and microphysical elements of the stars taking part in a merger. This can be combined self-consistently with the properties of the post-merger remnant to deduce generative elements of GRBs (e.g., heavier stars will more likely promptly collapse, leading to faster jet break-out; see [377] and Table 4).
- Radio flares. Radio activity can be incited premerger from a few different channels. For instance, there may be shock-powered radio emissions through interactions taking place in the accelerating, binary wind left in the wake of the inspiral [336,432]. These are likely to be in the form of FRBs or “giant pulse” like phenomena in the ∼GHz band. Another possibility discussed by [28] is that acceleration zones may form in regions of interwoven magnetic fields, which could produce coherent, millisecond bursts in radio frequencies that are theoretically observable out to ∼Gpc distances. Such observations could reveal information about the magnetospheric plasma and radio activation mechanisms, which can be used to deduce information about the general pulsar engine and all that can be conveyed about the stellar structure (see [433]). There is also the possibility of post-merger radio activity associated with a neutron star collapse as described in Section 6.1. The Square Kilometer Array (SKA) will go a long way towards detection prospects.
- X-ray flares. We have earlier described how the emission mechanism for GRB precursors resembles that of giant flares from magnetars in terms of energy extraction and propagation. Given that X-ray activity from magnetars is commonplace (and arguably a defining feature), the same applies for premerger stars with strong fields. X-ray emission mechanisms in a premerger system are described by [434], the key ingredient being the non-linear development of magnetospheric Alfvén waves (see also [401]). Such observations may reveal crucial information about the local magnetic field strength and radiation transport physics, from which information about neutron-star evolutionary pathways can be deduced. X-ray activity may also be related to magnetic instabilities arising by late-stage dynamo activity or magnetic reconfiguration, more generally (see Section 6.5 and also [158,435] for instance). The planned ECLAIRs telescope will help enable searches for X-rays out to cosmological distances.
- Neutrinos. Neutrinos could be emitted from a premerger system through at least two distinct means. One involves tidal heating. As described in Section 4.5, rapid episodes of heating could lead to chemical imbalance and the production of neutrinos, which could be theoretically observed for a close merger. Such observations would provide important information on the microphysical heat capacity. The second mechanism is indirectly via the production of cosmic rays. As discussed by Coppin and van Eijndhoven [436], it is thought that GRBs could be sources of ultra-high-energy cosmic rays. In this case, the interaction of high-energy protons in relativistic wind wakes (as above) near the source could produce neutrinos carrying a non-negligible fraction of the proton energy. Ice-Cube has reported the detection of some cosmic neutrinos [437,438,439], while the sources are only identified in a few cases; next-generation neutrino observatories (like IceCube-Gen2; [440]) will synergise with other instruments to better constrain the sources (e.g., [441]). In addition, such observations could be used to place constraints on binary neutron star merger abundances and provide tests of the standard model [442]. For instance, neutrinos are thought to carry magnetic moments [443,444] through which they interact with the ambient magnetic field that depends on whether they are of a Dirac or Majorana nature [445]. Thus, the neutrino flux from a strongly magnetised environment, such as the engine of GRBs [446,447], may encode the neutrino’s nature (e.g., [448]). An excellent review of future neutrino observatories, and instruments capable of follow-up, are described in Tables 1 and 2 of [449].
A Brief Look at Future Possibilities
8. Conclusions
- ★
- Magnetospheric interactions will accelerate the inspiral and lead to electromotive spin-up (quantified in Section 4.4), both of which may be visible in GWs either directly (dephasing) or indirectly (shifting mode frequencies as a function of time). Flares, radio pulsations, and/or FRBs may also be triggered by reconnection; such observations can place constraints on the large-scale, far-field dipole moments of old stars.
- ★
- Strong internal fields ( G) could, in principle, reveal themselves by shifting the stellar QNM frequencies or tidal Love numbers (Section Internal Fields). This could also be used to statistically constrain the presence or absence of superconducting states, as the superconductor tension force may also shift these properties even if B itself is partially expelled.
- ★
- Near-surface fields could be constrained by bright, non-thermal precursors if the magnetic field dominates the energy transport process (e.g., the maximum luminosity is set by the near-surface field; see Section 6.3.1).
- ★
- Remnant properties may be tied to the strength of the fields of the inspiralling constituents. Although very recent, high-resolution simulations suggest the magnetisation of the remnant may be set independently by dynamo activity [381], and disk material may still be highly magnetised [373], which could prolong subsequent GRB activity by setting up magnetic barriers that sporadically halt accretion. This channel could constrain internal fields and disc dynamics more generally.
- ★
- Field longevity is a critical question in the precursor scenario. How is it possible that magnetars take place in mergers when the anticipated decay timescales are orders of magnitude lower than a typical inspiral time? If the answer is that it is impossible, we appear to be left with only two options, being either that (i) bright, non-thermal precursors must be post-merger phenomena (Section 6.6), or (ii) the field is generated by dynamo activity in the seconds leading up to coalescence (Section 6.5). Either of these would lead to constraints on jet formation and propagation together with the microphysics of the crust where the dynamo would operate. If such fields can persist into late times, this may call into question assumptions about field evolution generally (e.g., whether one can ignore meridional circulation when evolving the induction equation [333]).
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
| 1 | For an up-to-date catalogue of neutron-star mass measurements, at least where there are no substantial systematics due to mass transfer or mass loss, see https://www3.mpifr-bonn.mpg.de/staff/pfreire/NS_masses.html, accessed on 27 November 2024. There are supposedly heavier “spiders”, though these are highly dynamical and also rotating rapidly. |
| 2 | These and many other EOS can be obtained in a tabulated form at [46]: https://compose.obspm.fr/home/, accessed on 27 November 2024. |
| 3 | The BSk EOS table can be generated by the resources provided in https://www.ioffe.ru/astro/NSG/BSk/, accessed on 27 November 2024 or the CompOSE catalogue. |
| 4 | |
| 5 | This is no longer strictly true in GR (or when including complicated microphysics capable of independent, secular responses) as the metric variables, even in the Regge-Wheeler gauge for example, cannot be uniquely expressed in terms of this displacement (cf. w-modes, which exist even in the no-fluid limit where ). We remark also that the inclusion of an elastic region does not complicate the formal analysis here [225]. |
| 6 | Two ways of generalising overlaps to GR have been proposed in [224] and [228]. The orthogonality between modes and the sum rule for tidal overlaps [229] are equally respected by both definitions, while only the former predicts a vanishing g-mode overlap in the zero stratification limit (at least for a simple, constant law; see Section 3.3). This issue is related to overlap “leakage” discussed in Section 6.4.1. Throughout, we adopt the definition of [224]. |
| 7 | This raises a subtle issue: it could be that the GRB is missed (e.g., beamed away) but a precursor is launched in the direction of Earth. The precursor may therefore be mistaken for the actual GRB since such events can be spectrally similar in some instances (see also Section 5.4). |
| 8 | In GR, modes are not strictly orthogonal but the inner product between them is much smaller than the extent of this leakage; see also Note 6. |
References
- Lattimer, J.M. Neutron Stars and the Nuclear Matter Equation of State. Annu. Rev. Nucl. Part. Sci. 2021, 71, 433. [Google Scholar] [CrossRef]
- Oertel, M.; Hempel, M.; Klähn, T.; Typel, S. Equations of state for supernovae and compact stars. Rev. Mod. Phys. 2017, 89, 015007. [Google Scholar] [CrossRef]
- Özel, F.; Freire, P. Masses, Radii, and the Equation of State of Neutron Stars. Annu. Rev. Astron. Astrophys. 2016, 54, 401–440. [Google Scholar] [CrossRef]
- Shibata, M.; Taniguchi, K.; Uryū, K. Merger of binary neutron stars of unequal mass in full general relativity. Phys. Rev. D 2003, 68, 084020. [Google Scholar] [CrossRef]
- Shibata, M.; Uryū, K.ō. Simulation of merging binary neutron stars in full general relativity: Γ = 2 case. Phys. Rev. D 2000, 61, 064001. [Google Scholar] [CrossRef]
- Falcke, H.; Rezzolla, L. Fast radio bursts: The last sign of supramassive neutron stars. Astron. Astrophys. 2014, 562, A137. [Google Scholar] [CrossRef]
- Kaplan, J.D.; Ott, C.D.; O’Connor, E.P.; Kiuchi, K.; Roberts, L.; Duez, M. The Influence of Thermal Pressure on Equilibrium Models of Hypermassive Neutron Star Merger Remnants. The Astrophys. J. 2014, 790, 19. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Glampedakis, K. Magnetically supramassive neutron stars. Phys. Rev. D 2022, 105, L061302. [Google Scholar] [CrossRef]
- Levan, A.J.; Gompertz, B.P.; Salafia, O.S.; Bulla, M.; Burns, E.; Hotokezaka, K.; Izzo, L.; Lamb, G.P.; Malesani, D.B.; Oates, S.R.; et al. Heavy-element production in a compact object merger observed by JWST. Nature 2024, 626, 737. [Google Scholar] [CrossRef]
- Troja, E.; Fryer, C.L.; O’Connor, B.; Ryan, G.; Dichiara, S.; Kumar, A.; Ito, N.; Gupta, R.; Wollae-ger, R.T.; Norris, J.P.; et al. A nearby long gamma-ray burst from a merger of compact objects. Nature 2022, 612, 228–231. [Google Scholar] [CrossRef] [PubMed]
- Abbott, B.P. et al. [LIGO Scientific and Virgo Collaborations] GW170817: Observation of gravitational waves from a binary neutron star inspiral. Phys. Rev. Lett. 2017, 119, 161101. [Google Scholar] [PubMed]
- Goldstein, A.; Veres, P.; Burns, E.; Briggs, M.S.; Hamburg, R.; Kocevski, D.; Wilson-Hodge, C.A.; Preece, R.D.; Poolakkil, S.; Roberts, O.J.; et al. An ordinary short gamma-ray burst with extraordinary implications: Fermi-GBM detection of GRB 170817A. Astrophys. J. Lett. 2017, 848, L14. [Google Scholar] [CrossRef]
- Abbott, B.P. et al. [LIGO Scientific and Virgo Collaborations] Tests of general relativity with the binary black hole signals from the LIGO-Virgo catalog GWTC-1. Phys. Rev. D 2019, 100, 104036. [Google Scholar] [CrossRef]
- Chatziioannou, K. Neutron-star tidal deformability and equation-of-state constraints. Gen. Relativ. Gravit. 2000, 52, 109. [Google Scholar] [CrossRef]
- Dietrich, T.; Hinderer, T.; Samajdar, A. Interpreting binary neutron star mergers: Describing the binary neutron star dynamics, modelling gravitational waveforms, and analyzing detections. Gen. Relativ. Gravit. 2021, 53, 27. [Google Scholar] [CrossRef]
- Wang, J.; Liu, L. Electromagnetic Precursors of Short Gamma-Ray Bursts as Counterparts of Gravitational Waves. Galaxies 2021, 9, 104. [Google Scholar] [CrossRef]
- Baiotti, L.; Rezzolla, L. Binary neutron star mergers: A review of Einstein’s richest laboratory. Rep. Prog. Phys. 2017, 80, 096901. [Google Scholar] [CrossRef]
- Burns, E. Neutron star mergers and how to study them. Living Rev. Relativ. 2020, 23, 4. [Google Scholar]
- Ciolfi, R. Short gamma-ray burst central engines. Int. J. Mod. Phys. D 2018, 27, 1842004. [Google Scholar] [CrossRef]
- Faber, J.A.; Rasio, F.A. Binary neutron star mergers. Living Rev. Relativ. 2012, 15, 8. [Google Scholar] [CrossRef]
- Kiuchi, K. General relativistic magnetohydodynamics simulations for binary neutron star mergers. arXiv 2024, arXiv:2405.10081. [Google Scholar]
- Radice, D.; Perego, A.; Hotokezaka, K.; Fromm, S.A.; Bernuzzi, S.; Roberts, L.F. Binary neutron star mergers: Mass ejection, electromagnetic counterparts, and nucleosynthesis. Astrophys. J. 2018, 869, 130. [Google Scholar] [CrossRef]
- Sarin, N.; Lasky, P.D. The evolution of binary neutron star post-merger remnants: A review. Gen. Relativ. Gravit. 2021, 53, 59. [Google Scholar] [CrossRef]
- Shibata, M.; Hotokezaka, K. Merger and mass ejection of neutron star binaries. Annu. Rev. Nucl. Part. Sci. 2019, 69, 41–64. [Google Scholar] [CrossRef]
- Coppin, P.; de Vries, K.D.; van Eijndhoven, N. Identification of gamma-ray burst precursors in Fermi-GBM bursts. Phys. Rev. D 2020, 102, 103014. [Google Scholar] [CrossRef]
- Deng, H.-Y.; Peng, Z.-Y.; Chen, J.-M.; Yin, Y.; Li, T. A Study of the Spectral properties of Gamma-Ray Bursts with the Precursors and Main bursts. Astrophys. J. 2024, 970, 67. [Google Scholar] [CrossRef]
- Wang, J.-S.; Peng, Z.-K.; Zou, J.-H.; Zhang, B.-B.; Zhang, B. Stringent search for precursor emission in short grbs from fermi/gbm data and physical implications. Astrophys. J. Lett. 2020, 902, L42. [Google Scholar] [CrossRef]
- Cooper, A.J.; Gupta, O.; Wadiasingh, Z.; Wijers, R.A.M.J.; Boersma, O.M.; Andreoni, I.; Rowlinson, A.; Gourdji, K. Pulsar revival in neutron star mergers: Multimessenger prospects for the discovery of pre-merger coherent radio emission. Mon. Not. R. Astron. Soc. 2023, 519, 3923–3946. [Google Scholar] [CrossRef]
- Hansen, B.M.S.; Lyutikov, M. Radio and X-ray signatures of merging neutron stars. Mon. Not. R. Astron. Soc. 2001, 322, 695–701. [Google Scholar] [CrossRef]
- Palenzuela, C.; Lehner, L.; Ponce, M.; Liebling, S.L.; Anderson, M.; Neilsen, D.; Motl, P. Electromagnetic and gravitational outputs from binary-neutron-star coalescence. Phys. Rev. Lett. 2013, 111, 061105. [Google Scholar] [CrossRef]
- Wada, T.; Shibata, M.; Ioka, K. Analytic properties of the electromagnetic field of binary compact stars and electromagnetic precursors to gravitational waves. Prog. Theor. Exp. Phys. 2020, 2020, 103E01. [Google Scholar] [CrossRef]
- Wang, J.-S.; Peng, F.-K.; Wu, K.; Dai, Z.-G. Pre-merger electromagnetic counterparts of binary compact stars. Astrophys. J. 2018, 868, 19. [Google Scholar] [CrossRef]
- Dichiara, S.; Tsang, D.; Troja, E.; Neill, D.; Norris, J.P.; Yang, Y.H. A luminous precursor in the extremely bright GRB 230307A. Astrophys. J. Lett. 2023, 954, L29. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Kuan, H.-J.; Reboul-Salze, A.; Kokkotas, K.D. Magnetic amplification in premerger neutron stars through resonance-induced magnetorotational instabilities. Phys. Rev. D 2024, 109, 103023. [Google Scholar] [CrossRef]
- Xiao, S.; Zhang, Y.Q.; Zhu, Z.P.; Xiong, S.L.; Gao, H.; Xu, D.; Zhang, S.N.; Peng, W.X.; Li, X.B.; Zhang, P.; et al. The peculiar precursor of a gamma-ray burst from a binary merger involving a magnetar. Astrophys. J. 2024, 970, 6. [Google Scholar] [CrossRef]
- Thompson, C.; Duncan, R.C. Neutron star dynamos and the origins of pulsar magnetism. Astrophys. J. 1993, 408, 194–217. [Google Scholar] [CrossRef]
- Thompson, C.; Duncan, R.C. The soft gamma repeaters as very strongly magnetized neutron stars-I. Radiative mechanism for outbursts. Mon. Not. R. Astron. Soc. 1995, 275, 255–300. [Google Scholar] [CrossRef]
- Enoto, T.; Kisaka, S.; Shibata, S. Observational diversity of magnetized neutron stars. Rep. Prog. Phys. 2019, 82, 106901. [Google Scholar] [CrossRef]
- Olausen, S.A.; Kaspi, V.M. The McGill magnetar catalog. Astrophys. J. Suppl. Ser. 2014, 212, 6. [Google Scholar] [CrossRef]
- Hoyos, J.; Reisenegger, A.; Valdivia, J.A. Magnetic field evolution in neutron stars: One-dimensional multi-fluid model. Astron. Astrophys. 2008, 487, 789–803. [Google Scholar] [CrossRef]
- Alford, M.G.; Harris, S.P. β equilibrium in neutron-star mergers. Phys. Rev. C 2018, 98, 065806. [Google Scholar] [CrossRef]
- O’Boyle, M.F.; Markakis, C.; Stergioulas, N.; Read, J.S. Parametrized equation of state for neutron star matter with continuous sound speed. Phys. Rev. D 2020, 102, 083027. [Google Scholar] [CrossRef]
- Alford, M.; Braby, M.; Paris, M.; Reddy, S. Hybrid stars that masquerade as neutron stars. Astrophys. J. 2005, 629, 969. [Google Scholar] [CrossRef]
- Doroshenko, V.; Suleimanov, V.; Pühlhofer, G.; Santangelo, A. A strangely light neutron star within a supernova remnant. Nat. Astron. 2022, 6, 1444–1451. [Google Scholar] [CrossRef]
- Ofengeim, D.D.; Shternin, P.S.; Piran, T. A three-parameter characterization of neutron stars’ mass-radius relation and equation of state. arXiv 2024, arXiv:2404.17647. [Google Scholar] [CrossRef]
- Typel, S.; Oertel, M.; Klähn, T. CompOSE CompStar online supernova equations of state harmonising the concert of nuclear physics and astrophysics compose.obspm.fr. Phys. Part. Nucl. 2015, 46, 633–664. [Google Scholar] [CrossRef]
- Krüger, C.J.; Ho, W.C.G.; Andersson, N. Seismology of adolescent neutron stars: Accounting for thermal effects and crust elasticity. Phys. Rev. D 2015, 92, 063009. [Google Scholar] [CrossRef]
- Kuan, H.-J.; Suvorov, A.G.; Kokkotas, K.D. Measuring spin in coalescing binaries of neutron stars that show double precursors. Astron. Astrophys. 2023, 676, A59. [Google Scholar] [CrossRef]
- Kuan, H.-J.; Krüger, C.J.; Suvorov, A.G.; Kokkotas, K.D. Constraining equation-of-state groups from g-mode asteroseismology. Mon. Not. R. Astron. Soc. 2022, 513, 4045–4056. [Google Scholar] [CrossRef]
- Lattimer, J.M.; Prakash, M. Neutron star structure and the equation of state. Astrophys. J. 2001, 550, 426. [Google Scholar] [CrossRef]
- Raithel, C.A.; Most, E.R. Characterizing the Breakdown of Quasi-universality in Postmerger Gravitational Waves from Binary Neutron Star Mergers. Astrophys. J. Lett. 2022, 933, L39. [Google Scholar] [CrossRef]
- Sedrakian, A.; Li, J.J.; Weber, F. Heavy baryons in compact stars. Prog. Part. Nucl. Phys. 2023, 131, 104041. [Google Scholar] [CrossRef]
- Greif, S.K.; Hebeler, K.; Lattimer, J.M.; Pethick, C.J.; Schwenk, A. Equation of state constraints from nuclear physics, neutron star masses, and future moment of inertia measurements. Astrophys. J. 2020, 901, 155. [Google Scholar] [CrossRef]
- Wiringa, R.B.; Fiks, V.; Fabrocini, A. Equation of state for dense nucleon matter. Phys. Rev. C 1988, 38, 1010. [Google Scholar] [CrossRef] [PubMed]
- Wiringa, R.B.; Stoks, V.G.J.; Schiavilla, R. Accurate nucleon-nucleon potential with charge-independence breaking. Phys. Rev. C 1995, 51, 38. [Google Scholar] [CrossRef]
- Akmal, A.; Pandharipande, V.R.; Ravenhall, D.G. Equation of state of nucleon matter and neutron star structure. Phys. Rev. C 1998, 58, 1804. [Google Scholar] [CrossRef]
- Anzuini, F.; Melatos, A.; Dehman, C.; Viganò, D.; Pons, J.A. Fast cooling and internal heating in hyperon stars. Mon. Not. R. Astron. Soc. 2022, 509, 2609–2623. [Google Scholar]
- Schneider, A.S.; Constantinou, C.; Muccioli, B.; Prakash, M. Akmal-Pandharipande-Ravenhall equation of state for simulations of supernovae, neutron stars, and binary mergers. Phys. Rev. C 2019, 100, 025803. [Google Scholar] [CrossRef]
- Burgio, G.F.; Schulze, H.J.; Vidaña, I.; Wei, J.B. Neutron stars and the nuclear equation of state. Prog. Part. Nucl. Phys. 2021, 120, 103879. [Google Scholar] [CrossRef]
- Chabanat, E.; Bonche, P.; Haensel, P.; Meyer, J.; Schaeffer, R. A Skyrme parametrization from subnuclear to neutron star densities. Nucl. Phys. A 1997, 627, 710–746. [Google Scholar] [CrossRef]
- Chabanat, E.; Bonche, P.; Haensel, P.; Meyer, J.; Schaeffer, R. A Skyrme parametrization from subnuclear to neutron star densities Part II. Nuclei far from stabilities. Nucl. Phys. A 1998, 635, 231–256. [Google Scholar] [CrossRef]
- Douchin, F.; Haensel, P. A unified equation of state of dense matter and neutron star structure. Astron. Astrophys. 2001, 380, 151–167. [Google Scholar] [CrossRef]
- Gulminelli, F.; Raduta, A.R. Unified treatment of subsaturation stellar matter at zero and finite temperature. Phys. Rev. C 2015, 92, 055803. [Google Scholar] [CrossRef]
- Raduta, A.R.; Gulminelli, F. Nuclear statistical equilibrium equation of state for core collapse. Nucl. Phys. A 2019, 983, 252–275. [Google Scholar] [CrossRef]
- Schneider, A.S.; Roberts, L.F.; Ott, C.D. A New Open-Source Nuclear Equation of State Framework based on the Liquid-Drop Model with Skyrme Interaction. Phys. Rev. C 2017, 96, 065802. [Google Scholar] [CrossRef]
- Marino, A.; Dehman, C.; Kovlakas, K.; Rea, N.; Pons, J.A.; Viganò, D. Constraints on the dense matter equation of state from young and cold isolated neutron stars. Nat. Astron. 2024, 8, 1020–1030. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J.M. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XIII. The 2012 atomic mass evaluation and the symmetry coefficient. Phys. Rev. C 2013, 88, 024308. [Google Scholar] [CrossRef]
- Pearson, J.M.; Chamel, N.; Goriely, S.; Ducoin, C. Inner crust of neutron stars with mass-fitted Skyrme functionals. Phys. Rev. C 2012, 85, 065803. [Google Scholar] [CrossRef]
- Pearson, J.M.; Chamel, N.; Potekhin, A.Y.; Fantina, A.F.; Ducoin, C.; Dutta, A.K.; Goriely, S. Unified equations of state for cold non-accreting neutron stars with Brussels–Montreal functionals–I. Role of symmetry energy. Mon. Not. R. Astron. Soc. 2018, 481, 2994–3026. [Google Scholar] [CrossRef]
- Pearson, J.M.; Goriely, S.; Chamel, N. Properties of the outer crust of neutron stars from Hartree-Fock-Bogoliubov mass models. Phys. Rev. C 2011, 83, 065810. [Google Scholar] [CrossRef]
- Potekhin, A.Y.; Fantina, A.F.; Chamel, N.; Pearson, J.M.; Goriely, S. Analytical representations of unified equations of state for neutron-star matter. Astron. Astrophys. 2013, 560, A48. [Google Scholar] [CrossRef]
- Dobaczewski, J.; Stoitsov, M.V.; Nazarewicz, W. Skyrme-HFB deformed nuclear mass table. AIP Conf. Proc. 2004, 726, 51–56. [Google Scholar] [CrossRef]
- Fantina, A.F.; Zdunik, J.L.; Chamel, N.; Pearson, J.M.; Suleiman, L.; Goriely, S. Accreting neutron stars from the nuclear energy-density functional theory. II. Equation of state and global properties. Astron. Astrophys. 2022, 665, A74. [Google Scholar] [CrossRef]
- Stockinger, G.; Janka, H.T.; Kresse, D.; Melson, T.; Ertl, T.; Gabler, M.; Gessner, A.; Wongwathanarat, A.; Tolstov, A.; Leung, S.C.; et al. Three-dimensional models of core-collapse supernovae from low-mass progenitors with implications for Crab. Mon. Not. R. Astron. Soc. 2020, 496, 2039–2084. [Google Scholar] [CrossRef]
- Fields, C.E. The Three-dimensional Collapse of a Rapidly Rotating 16 M⊙ Star. Astrophys. J. Lett. 2022, 924, L15. [Google Scholar] [CrossRef]
- Varma, V.; Müller, B. 3D simulations of magnetoconvection in a rapidly rotating supernova progenitor. Mon. Not. R. Astron. Soc. 2023, 526, 5249–5262. [Google Scholar] [CrossRef]
- Varma, V.; Müller, B.; Schneider, F.R.N. 3D simulations of strongly magnetized non-rotating supernovae: Explosion dynamics and remnant properties. Mon. Not. R. Astron. Soc. 2023, 518, 3622–3636. [Google Scholar] [CrossRef]
- Patruno, A.; Haskell, B.; D’Angelo, C. Gravitational waves and the maximum spin frequency of neutron stars. Astrophys. J. 2012, 746, 9. [Google Scholar] [CrossRef]
- Tauris, T.M.; Kramer, M.; Freire, P.C.C.; Wex, N.; Janka, H.T.; Langer, N.; Podsiadlowski, P.; Bozzo, E.; Chaty, S.; Kruckow, M.U.; et al. Formation of double neutron star systems. Astrophys. J. 2017, 846, 170. [Google Scholar] [CrossRef]
- Andrews, J.J.; Mandel, I. Double neutron star populations and formation channels. Astrophys. J. Lett. 2019, 880, L8. [Google Scholar] [CrossRef]
- van den Heuvel, E.P.J. High-Mass X-Ray Binaries: Illuminating the Passage from Massive Binaries to Merging Compact Objects; Oskinova, L.M., Bozzo, E., Bulik, T., Gies, D.R., Eds.; Cambridge University Press: Cambridge, UK, 2019; Volume 346, pp. 1–13. [Google Scholar]
- Fonseca, E.; Stairs, I.H.; Thorsett, S.E. A comprehensive study of relativistic gravity using PSR B1534+ 12. Astrophys. J. 2014, 787, 82. [Google Scholar] [CrossRef]
- Glampedakis, K.; Suvorov, A.G. Modelling spin-up episodes in accreting millisecond X-ray pulsars. Mon. Not. R. Astron. Soc. 2021, 508, 2399–2411. [Google Scholar] [CrossRef]
- Melatos, A.; Payne, D.J.B. Gravitational radiation from an accreting millisecond pulsar with a magnetically confined mountain. Astrophys. J. 2005, 623, 1044. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Melatos, A. Testing modified gravity and no-hair relations for the Kerr-Newman metric through quasiperiodic oscillations of galactic microquasars. Phys. Rev. D 2016, 93, 024004. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Melatos, A. Relaxation by thermal conduction of a magnetically confined mountain on an accreting neutron star. Mon. Not. R. Astron. Soc. 2019, 484, 1079–1099. [Google Scholar] [CrossRef]
- Vigelius, M.; Melatos, A. Resistive relaxation of a magnetically confined mountain on an accreting neutron star. Mon. Not. R. Astron. Soc. 2009, 395, 1985–1998. [Google Scholar] [CrossRef]
- Zhu, X.; Thrane, E.; Osłowski, S.; Levin, Y.; Lasky, P.D. Inferring the population properties of binary neutron stars with gravitational-wave measurements of spin. Phys. Rev. D 2018, 98, 043002. [Google Scholar]
- Dexheimer, V.; Franzon, B.; Gomes, R.O.; Farias, R.L.S.; Avancini, S.S.; Schramm, S. What is the magnetic field distribution for the equation of state of magnetized neutron stars? Phys. Lett. B 2017, 773, 487–491. [Google Scholar] [CrossRef]
- Bilous, A.V.; Ransom, S.M.; Demorest, P. Unusually bright single pulses from the binary pulsar B1744–24A: A case of strong lensing? Astrophys. J. 2019, 877, 125. [Google Scholar] [CrossRef]
- Riley, T.E. et al. [NICER Collaboration] A NICER view of the massive pulsar PSR J0740+ 6620 informed by radio timing and XMM-Newton spectroscopy. Astrophys. J. Lett. 2021, 918, L27. [Google Scholar] [CrossRef]
- Kalapotharakos, C.; Wadiasingh, Z.; Harding, A.K.; Kazanas, D. The multipolar magnetic field of the millisecond pulsar PSR J0030+ 0451. Astrophys. J. 2021, 907, 63. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Melatos, A. Recycled pulsars with multipolar magnetospheres from accretion-induced magnetic burial. Mon. Not. R. Astron. Soc. 2020, 499, 3243–3254. [Google Scholar] [CrossRef]
- Chen, K.; Ruderman, M. Pulsar death lines and death valley. Astrophys. J. 1993, 402, 264–270. [Google Scholar] [CrossRef]
- Hibschman, J.A.; Arons, J. Pair multiplicities and pulsar death. Astrophys. J. 2001, 554, 624. [Google Scholar] [CrossRef]
- Barnard, J.J.; Arons, J. Pair production and pulsar cutoff in magnetized neutron stars with nondipolar magnetic geometry. Astrophys. J. 1982, 254, 713–734. [Google Scholar] [CrossRef]
- Beloborodov, A.M.; Thompson, C. Corona of magnetars. Astrophys. J. 2007, 657, 967. [Google Scholar] [CrossRef]
- Zhang, B.; Gil, J.; Dyks, J. On the origins of part-time radio pulsars. Mon. Not. R. Astron. Soc. 2007, 374, 1103–1107. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Mastrano, A.; Geppert, U. Gravitational radiation from neutron stars deformed by crustal Hall drift. Mon. Not. R. Astron. Soc. 2016, 459, 3407–3418. [Google Scholar] [CrossRef]
- Gourgouliatos, K.N.; De Grandis, D.; Igoshev, A. Magnetic field evolution in neutron star crusts: Beyond the hall effect. Symmetry 2022, 14, 130. [Google Scholar] [CrossRef]
- Gusakov, M.E.; Kantor, E.M.; Ofengeim, D.D. Evolution of the magnetic field in neutron stars. Phys. Rev. D 2017, 96, 103012. [Google Scholar] [CrossRef]
- Dehman, C.; Brandenburg, A. Reality of Inverse Cascading in Neutron Star Crusts. arXiv 2024, arXiv:2408.08819. [Google Scholar] [CrossRef]
- Barsukov, D.P.; Tsygan, A.I. The influence of nondipolar magnetic field and neutron star precession on braking indices of radiopulsars. Mon. Not. R. Astron. Soc. 2010, 409, 1077–1087. [Google Scholar] [CrossRef]
- Melatos, A. Bumpy spin-down of anomalous x-ray pulsars: The link with magnetars. Astrophys. J. 1999, 519, L77. [Google Scholar] [CrossRef]
- Johnston, S.; Galloway, D. Pulsar braking indices revisited. Mon. Not. R. Astron. Soc. 1999, 306, L50–L54. [Google Scholar] [CrossRef]
- Bransgrove, A.; Levin, Y.; Beloborodov, A.M. Giant Hall Waves Launched by Superconducting Phase Transition in Pulsars. arXiv 2024, arXiv:2408.10888. [Google Scholar]
- Johnston, S.; Karastergiou, A. Pulsar braking and the P–P˙ diagram. Mon. Not. R. Astron. Soc. 2017, 467, 3493–3499. [Google Scholar] [CrossRef]
- Melatos, A.; Priymak, M. Gravitational radiation from magnetically funneled supernova fallback onto a magnetar. Astrophys. J. 2014, 794, 170. [Google Scholar] [CrossRef]
- Bhattacharya, D.; van den Heuvel, E.P.J. Formation and evolution of binary and millisecond radio pulsars. Phys. Rep. 1991, 203, 1–124. [Google Scholar] [CrossRef]
- Staubert, R.; Trümper, J.; Kendziorra, E.; Klochkov, D.; Postnov, K.; Kretschmar, P.; Pottschmidt, K.; Haberl, F.; Rothschild, R.E.; Santangelo, A.; et al. Cyclotron lines in highly magnetized neutron stars. Astron. Astrophys. 2019, 622, A61. [Google Scholar] [CrossRef]
- Beloborodov, A.M. Untwisting magnetospheres of neutron stars. Astrophys. J. 2009, 703, 1044. [Google Scholar] [CrossRef]
- Baym, G.; Pines, D. Neutron starquakes and pulsar speedup. Ann. Phys. 1971, 66, 816–835. [Google Scholar] [CrossRef]
- Dall’Osso, S.; Stella, L. Millisecond Magnetars. In Millisecond Pulsars; Astrophysics and Space Science Library; Bhattacharyya, S., Papitto, A., Bhattacharya, D., Eds.; Springer: Cham, Switzerland, 2022; Volume 465, pp. 245–280. [Google Scholar]
- Suvorov, A.G.; Kokkotas, K.D. Young magnetars with fracturing crusts as fast radio burst repeaters. Mon. Not. R. Astron. Soc. 2019, 488, 5887–5897. [Google Scholar] [CrossRef]
- Makishima, K.; Enoto, T.; Hiraga, J.S.; Nakano, T.; Nakazawa, K.; Sakurai, S.; Sasano, M.; Murakami, H. Possible evidence for free precession of a strongly magnetized neutron star in the magnetar 4U 0142+ 61. Phys. Rev. Lett. 2014, 112, 171102. [Google Scholar] [CrossRef] [PubMed]
- Makishima, K.; Tamba, T.; Aizawa, Y.; Odaka, H.; Yoneda, H.; Enoto, T.; Suzuki, H. Discovery of 40.5 ks hard X-ray pulse-phase modulations from SGR 1900+ 14. Astrophys. J. 2021, 923, 63. [Google Scholar] [CrossRef]
- Mastrano, A.; Suvorov, A.G.; Melatos, A. Neutron star deformation due to poloidal–toroidal magnetic fields of arbitrary multipole order: A new analytic approach. Mon. Not. R. Astron. Soc. 2015, 447, 3475–3485. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Kokkotas, K.D. Evidence for magnetar precession in X-ray afterglows of gamma-ray bursts. Astrophys. J. Lett. 2020, 892, L34. [Google Scholar] [CrossRef]
- Akgün, T.; Reisenegger, A.; Mastrano, A.; Marchant, P. Stability of magnetic fields in non-barotropic stars: An analytic treatment. Mon. Not. R. Astron. Soc. 2013, 433, 2445–2466. [Google Scholar] [CrossRef]
- Herbrik, M.; Kokkotas, K.D. Stability analysis of magnetized neutron stars—A semi-analytic approach. Mon. Not. R. Astron. Soc. 2017, 466, 1330–1347. [Google Scholar] [CrossRef]
- Tayler, R.J. The Adiabatic Stability of Stars Containing Magnetic Fields–I: T OROIDAL F IELDS. Mon. Not. R. Astron. Soc. 1973, 161, 365–380. [Google Scholar] [CrossRef]
- Melatos, A. Fast fossil rotation of neutron star cores. Astrophys. J. 2012, 761, 32. [Google Scholar] [CrossRef]
- Dehman, C.; Viganò, D.; Pons, J.A.; Rea, N. 3D code for MAgneto-Thermal evolution in Isolated Neutron Stars, MATINS: The magnetic field formalism. Mon. Not. R. Astron. Soc. 2023, 518, 1222–1242. [Google Scholar] [CrossRef]
- Pons, J.A.; Perna, R. Magnetars versus high magnetic field pulsars: A theoretical interpretation of the apparent dichotomy. Astrophys. J. 2011, 741, 123. [Google Scholar] [CrossRef]
- Suvorov, A.G. Wave-optical effects in the microlensing of continuous gravitational waves by star clusters. Astrophys. J. 2022, 930, 13. [Google Scholar] [CrossRef]
- Braithwaite, J.; Nordlund, Å. Stable magnetic fields in stellar interiors. Astron. Astrophys. 2006, 450, 1077–1095. [Google Scholar] [CrossRef]
- Lorimer, D.R.; Kramer, M. Handbook of Pulsar Astronomy; Cambridge University Press: Cambridge, UK, 2004; Volume 4. [Google Scholar]
- Goldreich, P.; Reisenegger, A. Magnetic field decay in isolated neutron stars. Astrophys. J. 1992, 395, 250–258. [Google Scholar] [CrossRef]
- Gourgouliatos, K.N.; Lander, S.K. Axisymmetric magneto-plastic evolution of neutron-star crusts. Mon. Not. R. Astron. Soc. 2021, 506, 3578–3587. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Melatos, A. Evolutionary implications of a magnetar interpretation for GLEAM-X J162759. 5–523504.3. Mon. Not. R. Astron. Soc. 2023, 520, 1590–1600. [Google Scholar] [CrossRef]
- Aguilera, D.N.; Pons, J.A.; Miralles, J.A. The impact of magnetic field on the thermal evolution of neutron stars. Astrophys. J. 2008, 673, L167. [Google Scholar] [CrossRef]
- Gourgouliatos, K.N.; Cumming, A. Hall attractor in axially symmetric magnetic fields in neutron star crusts. Phys. Rev. Lett. 2014, 112, 171101. [Google Scholar] [CrossRef]
- Igoshev, A.P.; Popov, S.B. How to make a mature accreting magnetar. Mon. Not. R. Astron. Soc. 2018, 473, 3204–3210. [Google Scholar] [CrossRef]
- Ho, W.C.G.; Andersson, N.; Graber, V. Dynamical onset of superconductivity and retention of magnetic fields in cooling neutron stars. Phys. Rev. C 2017, 96, 065801. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Glampedakis, K. Does the Gamma-Ray Binary LS I+ 61° 303 Harbor a Magnetar? Astrophys. J. 2022, 940, 128. [Google Scholar] [CrossRef]
- Eichler, D.; Livio, M.; Piran, T.; Schramm, D.N. Nucleosynthesis, neutrino bursts and γ-rays from coalescing neutron stars. Nature 1989, 340, 126–128. [Google Scholar] [CrossRef]
- Rosswog, S.; Liebendörfer, M. High-resolution calculations of merging neutron stars—II. Neutrino emission. Mon. Not. R. Astron. Soc. 2003, 342, 673–689. [Google Scholar] [CrossRef]
- Sekiguchi, Y.; Kiuchi, K.; Kyutoku, K.; Shibata, M. Gravitational waves and neutrino emission from the merger of binary neutron stars. Phys. Rev. Lett. 2011, 107, 051102. [Google Scholar] [CrossRef] [PubMed]
- Chamel, N.; Haensel, P. Physics of neutron star crusts. Living Rev. Relativ. 2008, 11, 10. [Google Scholar] [CrossRef]
- Farouki, R.T.; Hamaguchi, S. Thermal energy of the crystalline one-component plasma from dynamical simulations. Phys. Rev. E 1993, 47, 4330. [Google Scholar] [CrossRef]
- Gittins, F.; Celora, T.; Beri, A.; Andersson, N. Modelling Neutron-Star Ocean Dynamics. Universe 2023, 9, 226. [Google Scholar] [CrossRef]
- Gudmundsson, E.H.; Pethick, C.J.; Epstein, R.I. Structure of neutron star envelopes. Astrophys. J. 1983, 272, 286–300. [Google Scholar] [CrossRef]
- Lorenz, C.P.; Ravenhall, D.G.; Pethick, C.J. Neutron star crusts. Phys. Rev. Lett. 1993, 70, 379. [Google Scholar] [CrossRef]
- Negele, J.W.; Vautherin, D. Neutron star matter at sub-nuclear densities. Nucl. Phys. A 1973, 207, 298–320. [Google Scholar] [CrossRef]
- Harutyunyan, A.; Sedrakian, A. Electrical conductivity of a warm neutron star crust in magnetic fields. Phys. Rev. C 2016, 94, 025805. [Google Scholar] [CrossRef]
- Truesdell, C.; Noll, W. ; The Non-Linear Field Theories of Mechanics [Electronic Resource]; Springer: Berlin, Heidelberg, Germany, 2004. [Google Scholar]
- Andersson, N.; Comer, G.L. Relativistic fluid dynamics: Physics for many different scales. Living Rev. Relativ. 2021, 24, 3. [Google Scholar] [CrossRef]
- Sotani, H.; Suvorov, A.G.; Kokkotas, K.D. Couplings of torsional and shear oscillations in a neutron star crust. Phys. Rev. D 2024, 110, 023035. [Google Scholar] [CrossRef]
- Pethick, C.J.; Potekhin, A.Y. Liquid crystals in the mantles of neutron stars. Phys. Lett. B 1998, 427, 7–12. [Google Scholar] [CrossRef]
- Strohmayer, T.; Ogata, S.; Iyetomi, H.; Ichimaru, S.; van Horn, H.M. The shear modulus of the neutron star crust and nonradial oscillations of neutron stars. Astrophys. J. 1991, 375, 679–686. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Hughto, J. Molecular dynamics simulation of shear moduli for Coulomb crystals. arXiv 2008, arXiv:0812.2650. [Google Scholar]
- Baiko, D.A. Shear modulus of neutron star crust. Mon. Not. R. Astron. Soc. 2011, 416, 22–31. [Google Scholar] [CrossRef]
- Beloborodov, A.M.; Levin, Y. Thermoplastic waves in magnetars. Astrophys. J. Lett. 2014, 794, L24. [Google Scholar] [CrossRef]
- Carter, B.; Quintana, H. Foundations of general relativistic high-pressure elasticity theory. Proc. R. Soc. Lond. Ser. A 1972, 331, 57–83. [Google Scholar]
- Kojima, Y. Correct criterion of crustal failure driven by intense magnetic stress in neutron stars. arXiv 2024, arXiv:2408.14100. [Google Scholar] [CrossRef]
- Kerin, A.D.; Melatos, A. Mountain formation by repeated, inhomogeneous crustal failure in a neutron star. Mon. Not. R. Astron. Soc. 2022, 514, 1628–1644. [Google Scholar] [CrossRef]
- Ruderman, M. Crust-breaking by neutron superfluids and the VELA pulsar glitches. Astrophys. J. 1976, 203, 213–222. [Google Scholar] [CrossRef]
- Suvorov, A.G. The radio shut-off, glitch, and X-ray burst in 1E 1547.0-5408 interpreted through magnetic reconfiguration. Mon. Not. R. Astron. Soc. 2023, 523, 4089–4096. [Google Scholar] [CrossRef]
- Lander, S.K.; Andersson, N.; Antonopoulou, D.; Watts, A.L. Magnetically driven crustquakes in neutron stars. Mon. Not. R. Astron. Soc. 2015, 449, 2047–2058. [Google Scholar] [CrossRef]
- Jones, P.B. Nature of fault planes in solid neutron star matter. Astrophys. J. 2003, 595, 342. [Google Scholar] [CrossRef]
- Li, X.; Levin, Y.; Beloborodov, A.M. Magnetar outbursts from avalanches of Hall waves and crustal failures. Astrophys. J. 2016, 833, 189. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Kadau, K. Breaking strain of neutron star crust and gravitational waves. Phys. Rev. Lett. 2009, 102, 191102. [Google Scholar] [CrossRef] [PubMed]
- Kozhberov, A.A.; Yakovlev, D.G. Deformed crystals and torsional oscillations of neutron star crust. Mon. Not. R. Astron. Soc. 2020, 498, 5149–5158. [Google Scholar] [CrossRef]
- Smoluchowski, R. Frequency of pulsar starquakes. Phys. Rev. Lett. 1970, 24, 923. [Google Scholar] [CrossRef]
- Kittel, C. Introduction to Solid State Physics; Wiley: New York, NY, USA, 1976. [Google Scholar]
- Ruderman, R. Neutron star crustal plate tectonics. II-Evolution of radio pulsar magnetic fields. III-Cracking, glitches, and gamma-ray bursts. Astrophys. J. 1991, 382, 576–593. [Google Scholar] [CrossRef]
- Chugunov, A.I.; Horowitz, C.J. Breaking stress of neutron star crust. Mon. Not. R. Astron. Soc. 2010, 407, L54–L58. [Google Scholar] [CrossRef]
- Hoffman, K.; Heyl, J. Mechanical properties of non-accreting neutron star crusts. Mon. Not. R. Astron. Soc. 2012, 426, 2404–2412. [Google Scholar] [CrossRef]
- Fattoyev, F.J.; Horowitz, C.J.; Lu, H. Crust breaking and the limiting rotational frequency of neutron stars. arXiv 2018, arXiv:1804.04952. [Google Scholar]
- Baiko, D.A.; Chugunov, A.I. Breaking properties of neutron star crust. Mon. Not. R. Astron. Soc. 2018, 480, 5511–5516. [Google Scholar] [CrossRef]
- Caplan, M.E.; Schneider, A.S.; Horowitz, C.J. Elasticity of nuclear pasta. Phys. Rev. Lett. 2018, 121, 132701. [Google Scholar] [CrossRef] [PubMed]
- Kozhberov, A.A. Breaking properties of multicomponent neutron star crust. Mon. Not. R. Astron. Soc. 2023, 523, 4855–4858. [Google Scholar] [CrossRef]
- Baiko, D.A. Liquid-phase epitaxy of neutron star crusts and white dwarf cores. Mon. Not. R. Astron. Soc. 2024, 528, 408–417. [Google Scholar] [CrossRef]
- Aerts, C.; Christensen-Dalsgaard, J.; Kurtz, D.W. Asteroseismology; Springer: Dordrecht, The Netherlands, 2010. [Google Scholar]
- Unno, W.; Osaki, Y.; Ando, H.; Shibahashi, H. Nonradial Oscillations of Stars; University of Tokyo Press: Tokyo, Japan, 1979. [Google Scholar]
- Andersson, N.; Pnigouras, P. Exploring the effective tidal deformability of neutron stars. Phys. Rev. D 2020, 101, 083001. [Google Scholar] [CrossRef]
- Ho, W.C.G.; Andersson, N. New dynamical tide constraints from current and future gravitational wave detections of inspiralling neutron stars. Phys. Rev. D 2023, 108, 043003. [Google Scholar] [CrossRef]
- Xu, W.; Lai, D. Resonant tidal excitation of oscillation modes in merging binary neutron stars: Inertial-gravity modes. Phys. Rev. D 2017, 96, 083005. [Google Scholar] [CrossRef]
- Haensel, P.; Potekhin, A.Y. Analytical representations of unified equations of state of neutron-star matter. Astron. Astrophys. 2004, 428, 191–197. [Google Scholar] [CrossRef]
- Hiscock, W.A.; Lindblom, L. Stability and causality in dissipative relativistic fluids. Ann. Phys. 1983, 151, 466–496. [Google Scholar] [CrossRef]
- Israel, W. Nonstationary irreversible thermodynamics: A causal relativistic theory. Ann. Phys. 1976, 100, 310–331. [Google Scholar] [CrossRef]
- Israel, W.; Stewart, J.M. Transient relativistic thermodynamics and kinetic theory. Ann. Phys. 1979, 118, 341–372. [Google Scholar] [CrossRef]
- Stewart, J.M. On transient relativistic thermodynamics and kinetic theory. Proc. R. Soc. Lond. Ser. A 1977, 357, 59–75. [Google Scholar]
- Andersson, N.; Pnigouras, P. The g-mode spectrum of reactive neutron star cores. Mon. Not. R. Astron. Soc. 2019, 489, 4043–4048. [Google Scholar] [CrossRef]
- Counsell, A.R.; Gittins, F.; Andersson, N. The impact of nuclear reactions on the neutron-star g-mode spectrum. Mon. Not. R. Astron. Soc. 2024, 531, 1721–1729. [Google Scholar] [CrossRef]
- Hammond, P.; Hawke, I.; Andersson, N. Thermal aspects of neutron star mergers. Phys. Rev. D 2021, 104, 103006. [Google Scholar] [CrossRef]
- Ripley, J.L.; K, R.A.H.; Yunes, N. Probing internal dissipative processes of neutron stars with gravitational waves during the inspiral of neutron star binaries. Phys. Rev. D 2023, 108, 103037. [Google Scholar] [CrossRef]
- Saketh, M.V.S.; Zhou, Z.; Ghosh, S.; Steinhoff, J.; Chatterjee, D. Investigating tidal heating in neutron stars via gravitational Raman scattering. arXiv 2024, arXiv:2407.08327. [Google Scholar] [CrossRef]
- Ripley, J.L.; Hegade KR, A.; Chandramouli, R.S.; Yunes, N. A constraint on the dissipative tidal deformability of neutron stars. Nat. Astron. 2024, 8, 1277–1283. [Google Scholar] [CrossRef]
- Shapiro, S.L.; Teukolsky, S.A. Black Holes, White Dwarfs and Neutron Stars: The Physics of Compact Objects; Wiley: New York, NY, USA, 1983. [Google Scholar]
- Haskell, B.; Sedrakian, A. Superfluidity and Superconductivity in Neutron Stars. In The Physics and Astrophysics of Neutron Stars; Astrophysics and Space Science Library; Rezzolla, L., Pizzochero, P., Jones, D.I., Rea, N., Vidaña, I., Eds.; Springer: Cham, Switzerland, 2018; Volume 457, p. 401. [Google Scholar]
- Migdal, A.B. Superfluidity and the moments of inertia of nuclei. Nucl. Phys. 1959, 13, 655–674. [Google Scholar] [CrossRef]
- Ginzburg, V.L.; Kirzhniz, D.A. On the superfluidity of neutron stars. Zh. Eksperim Teor. Fiz. 1964, 47, 2006. [Google Scholar]
- Drissi, M.; Rios, A. Many-body approximations to the superfluid gap and critical temperature in pure neutron matter. Eur. Phys. J. A 2022, 58, 90. [Google Scholar] [CrossRef]
- Gandolfi, S.; Palkanoglou, G.; Carlson, J.; Gezerlis, A.; Schmidt, K.E. The 1S0 pairing gap in neutron matter. Condens. Matter 2022, 7, 19. [Google Scholar] [CrossRef]
- Krotscheck, E.; Papakonstantinou, P.; Wang, J. Triplet pairing in neutron matter. Astrophys. J. 2023, 955, 76. [Google Scholar] [CrossRef]
- Andersson, N. A superfluid perspective on neutron star dynamics. Universe 2021, 7, 17. [Google Scholar] [CrossRef]
- Ye, C.S.; Fong, W.-F.; Kremer, K.; Rodriguez, C.L.; Chatterjee, S.; Fragione, G.; Rasio, F.A. On the rate of neutron star binary mergers from globular clusters. Astrophys. J. 2020, 888, L10. [Google Scholar] [CrossRef]
- Wolf, R.A. Some effects of the strong interactions on the properties of neutron-star matter. Astrophys. J. 1966, 145, 834. [Google Scholar] [CrossRef]
- Passamonti, A.; Andersson, N.; Ho, W.C.G. Buoyancy and g-modes in young superfluid neutron stars. Mon. Not. R. Astron. Soc. 2016, 455, 1489–1511. [Google Scholar] [CrossRef]
- Kantor, E.M.; Gusakov, M.E. Composition temperature-dependent g modes in superfluid neutron stars. Mon. Not. R. Astron. Soc. 2014, 442, L90–L94. [Google Scholar] [CrossRef]
- Yu, H.; Weinberg, N.N. Resonant tidal excitation of superfluid neutron stars in coalescing binaries. Mon. Not. R. Astron. Soc. 2017, 464, 2622–2637. [Google Scholar] [CrossRef]
- Rau, P.B.; Wasserman, I. Compressional modes in two-superfluid neutron stars with leptonic buoyancy. Mon. Not. R. Astron. Soc. 2018, 481, 4427–4444. [Google Scholar] [CrossRef]
- Yu, H.; Weinberg, N.N.; Arras, P.; Kwon, J.; Venumadhav, T. Beyond the linear tide: Impact of the non-linear tidal response of neutron stars on gravitational waveforms from binary inspirals. Mon. Not. R. Astron. Soc. 2023, 519, 4325–4343. [Google Scholar] [CrossRef]
- Yu, H.; Arras, P.; Weinberg, N.N. Dynamical tides during the inspiral of rapidly spinning neutron stars: Solutions beyond mode resonance. Phys. Rev. D 2024, 110, 024039. [Google Scholar] [CrossRef]
- Lander, S.K. Magnetic fields in superconducting neutron stars. Phys. Rev. Lett. 2013, 110, 071101. [Google Scholar] [CrossRef]
- Suvorov, A.G. Ultra-compact X-ray binaries as dual-line gravitational-wave sources. Mon. Not. R. Astron. Soc. 2021, 503, 5495–5503. [Google Scholar] [CrossRef]
- Glampedakis, K.; Andersson, N.; Samuelsson, L. Magnetohydrodynamics of superfluid and superconducting neutron star cores. Mon. Not. R. Astron. Soc. 2011, 410, 805–829. [Google Scholar] [CrossRef]
- Taam, R.E.; Sandquist, E.L. Common envelope evolution of massive binary stars. Annu. Rev. Astron. Astrophys. 2000, 38, 113–141. [Google Scholar] [CrossRef]
- Peters, P.C. Gravitational radiation and the motion of two point masses. Phys. Rev. 1964, 136, 1224. [Google Scholar] [CrossRef]
- Peters, P.C.; Mathews, J. Gravitational radiation from point masses in a Keplerian orbit. Phys. Rev. 1963, 131, 435. [Google Scholar] [CrossRef]
- Lai, D.; Rasio, F.A.; Shapiro, S.L. Equilibrium, stability and orbital evolution of close binary systems. Astrophys. J. 1994, 423, 344. [Google Scholar] [CrossRef]
- Lai, D.; Rasio, F.A.; Shapiro, S.L. Hydrodynamic instability and coalescence of binary neutron stars. Astrophys. J. 1994, 420, 811. [Google Scholar] [CrossRef]
- Kochanek, C.S. Coalescing binary neutron stars. Astrophys. J. 1992, 398, 234–247. [Google Scholar] [CrossRef]
- Pnigouras, P.; Gittins, F.; Nanda, A.; Andersson, N.; Jones, D.I. The dynamical tides of spinning Newtonian stars. Mon. Not. R. Astron. Soc. 2024, 527, 8409–8428. [Google Scholar] [CrossRef]
- Knee, A.M.; Romero-Shaw, I.M.; Lasky, P.D.; McIver, J.; Thrane, E. A Rosetta Stone for eccentric gravitational waveform models. Astrophys. J. 2022, 936, 172. [Google Scholar] [CrossRef]
- Pitre, T.; Poisson, E. General relativistic dynamical tides in binary inspirals without modes. Phys. Rev. D 2024, 109, 064004. [Google Scholar] [CrossRef]
- Lai, D.; Wu, Y. Resonant tidal excitations of inertial modes in coalescing neutron star binaries. Phys. Rev. D 2006, 74, 024007. [Google Scholar] [CrossRef]
- Press, W.H.; Teukolsky, S.A. On formation of close binaries by two-body tidal capture. Astrophys. J. 1977, 213, 183–192. [Google Scholar] [CrossRef]
- Reisenegger, A.; Goldreich, P. Excitation of neutron star normal modes during binary inspiral. Astrophys. J. 1994, 426, 688–691. [Google Scholar] [CrossRef]
- Zahn, J.P. Les marées dans une étoile double serrée. Ann. d’Astrophys. 1966, 29, 313. [Google Scholar]
- Zahn, J.P. Tidal friction in close binary stars. Astron. Astrophys. 1977, 57, 383–394. [Google Scholar]
- Ho, W.C.G.; Lai, D. Resonant tidal excitations of rotating neutron stars in coalescing binaries. Mon. Not. R. Astron. Soc. 1999, 308, 153–166. [Google Scholar]
- Kuan, H.-J.; Suvorov, A.G.; Kokkotas, K.D. General-relativistic treatment of tidal g-mode resonances in coalescing binaries of neutron stars–I. Theoretical framework and crust breaking. Mon. Not. R. Astron. Soc. 2021, 506, 2985–2998. [Google Scholar] [CrossRef]
- Passamonti, A.; Andersson, N.; Pnigouras, P. Dynamical tides in neutron stars: The impact of the crust. Mon. Not. R. Astron. Soc. 2021, 504, 1273–1293. [Google Scholar] [CrossRef]
- Strohmayer, T.E. Oscillations of rotating neutron stars. Astrophys. J. 1991, 372, 573–591. [Google Scholar] [CrossRef]
- Denis, J. Tidal Perturbation of the Non-Radial Oscillations of a Star. Astron. Astrophys. 1972, 20, 151. [Google Scholar]
- Miao, Z.; Zhou, E.; Li, A. Resolving phase transition properties of dense matter through tidal-excited g-mode from inspiralling neutron stars. Astrophys. J. 2024, 964, 31. [Google Scholar] [CrossRef]
- Reisenegger, A. Multipole moments of stellar oscillation modes. Astrophys. J. 1994, 432, 296. [Google Scholar] [CrossRef]
- Alexander, M.E. Tidal resonances in binary star systems. Mon. Not. R. Astron. Soc. 1987, 227, 843–861. [Google Scholar] [CrossRef]
- Alexander, M.E. Tidal resonances in binary star systems: Pt. 2. Slowly rotating stars. Mon. Not. R. Astron. Soc. 1988, 235, 1367. [Google Scholar] [CrossRef]
- Schäfer, G.; Jaranowski, P. Hamiltonian formulation of general relativity and post-Newtonian dynamics of compact binaries. Living Rev. Relativ. 2024, 27, 2. [Google Scholar] [CrossRef]
- Kokkotas, K.D.; Schafer, G. Tidal and tidal-resonant effects in coalescing binaries. Mon. Not. R. Astron. Soc. 1995, 275, 301–308. [Google Scholar] [CrossRef]
- Schäfer, G. The gravitational quadrupole radiation-reaction force and the canonical formalism of ADM. Ann. Phys. 1985, 161, 81–100. [Google Scholar] [CrossRef]
- Yagi, K.; Stein, L.C.; Yunes, N. Challenging the presence of scalar charge and dipolar radiation in binary pulsars. Phys. Rev. D 2016, 93, 024010. [Google Scholar] [CrossRef]
- Thorne, K.S. Multipole expansions of gravitational radiation. Rev. Mod. Phys. 1980, 52, 299. [Google Scholar] [CrossRef]
- Yunes, N.; Yagi, K.; Pretorius, F. Theoretical physics implications of the binary black-hole mergers GW150914 and GW151226. Phys. Rev. D 2016, 94, 084002. [Google Scholar] [CrossRef]
- Andersson, N.; Gittins, F.; Yin, S.; Panosso Macedo, R. Building post-Newtonian neutron stars. Class. Quantum Gravity 2023, 40, 025016. [Google Scholar] [CrossRef]
- Wagoner, R.V.; Malone, R.C. Post-Newtonian neutron stars. Astrophys. J. 1974, 189, L75. [Google Scholar] [CrossRef]
- Minaev, S.; Will, C.M. Compact binary systems in scalar-tensor gravity:<? format?> Equations of motion to 2.5 post-Newtonian order. Phys. Rev. D 2013, 87, 084070. [Google Scholar]
- Blanchet, L.; Damour, T.; Iyer, B.R.; Will, C.M.; Wiseman, A.G. Gravitational-radiation damping of compact binary systems to second post-Newtonian order. Phys. Rev. Lett. 1995, 74, 3515. [Google Scholar] [CrossRef]
- Ioka, K.; Taniguchi, K. Gravitational waves from inspiraling compact binaries with magnetic dipole moments. Astrophys. J. 2000, 537, 327. [Google Scholar] [CrossRef]
- Poisson, E. Gravitational waves from inspiraling compact binaries: The quadrupole-moment term. Phys. Rev. D 1998, 57, 5287. [Google Scholar] [CrossRef]
- Blanchet, L. Gravitational-wave tails of tails. Class. Quantum Gravity 1998, 15, 113. [Google Scholar] [CrossRef]
- Damour, T.; Nagar, A.; Villain, L. Measurability of the tidal polarizability of neutron stars in late-inspiral gravitational-wave signals. Phys. Rev. D 2012, 85, 123007. [Google Scholar] [CrossRef]
- Yagi, K. Multipole love relations. Phys. Rev. D 2014, 89, 043011. [Google Scholar] [CrossRef]
- Abdelsalhin, T.; Gualtieri, L.; Pani, P. Post-Newtonian spin-tidal couplings for compact binaries. Phys. Rev. D 2018, 98, 104046. [Google Scholar] [CrossRef]
- Henry, Q.; Faye, G.; Blanchet, L. Tidal effects in the gravitational-wave phase evolution of compact binary systems to next-to-next-to-leading post-Newtonian order. Phys. Rev. D 2020, 102, 044033. [Google Scholar] [CrossRef]
- Castro, G.; Gualtieri, L.; Maselli, A.; Pani, P. Impact and detectability of spin-tidal couplings in neutron star inspirals. Phys. Rev. D 2022, 106, 024011. [Google Scholar] [CrossRef]
- Suvorov, A.G. Monopolar and quadrupolar gravitational radiation from magnetically deformed neutron stars in modified gravity. Phys. Rev. D 2018, 98, 084026. [Google Scholar] [CrossRef]
- Cutler, C.; Flanagan, E.E. Gravitational waves from merging compact binaries: How accurately can one extract the binary’s parameters from the inspiral waveform? Phys. Rev. D 1994, 49, 2658. [Google Scholar] [CrossRef]
- Finn, L.S.; Chernoff, D.F. Observing binary inspiral in gravitational radiation: One interferometer. Phys. Rev. D 1993, 47, 2198. [Google Scholar] [CrossRef]
- Damour, T. Gravitational Radiation; Deruelle, N., Piran, T., Eds.; North-Holland: Amsterdam, The Netherlands, 1983; p. 58. [Google Scholar]
- Binnington, T.; Poisson, E. Relativistic theory of tidal Love numbers. Phys. Rev. D 2009, 80, 084018. [Google Scholar] [CrossRef]
- Damour, T.; Nagar, A. Relativistic tidal properties of neutron stars. Phys. Rev. D 2009, 80, 084035. [Google Scholar] [CrossRef]
- Pani, P.; Gualtieri, L.; Abdelsalhin, T.; Jiménez-Forteza, X. Magnetic tidal Love numbers clarified. Phys. Rev. D 2018, 98, 124023. [Google Scholar] [CrossRef]
- Pani, P.; Gualtieri, L.; Ferrari, V. Tidal Love numbers of a slowly spinning neutron star. Phys. Rev. D 2015, 92, 124003. [Google Scholar] [CrossRef]
- Hansen, R.O. Multipole moments of stationary space-times. J. Math. Phys. 1974, 15, 46–52. [Google Scholar] [CrossRef]
- Gürsel, Y. Multipole moments for stationary systems: The equivalence of the Geroch-Hansen formulation and the Thorne formulation. Gen. Relativ. Gravit. 1983, 15, 737–754. [Google Scholar] [CrossRef]
- Damour, T.; Soffel, M.; Xu, C. General-relativistic celestial mechanics. I. Method and definition of reference systems. Phys. Rev. D 1991, 43, 3273. [Google Scholar] [CrossRef]
- Pappas, G.; Sotiriou, T.P. Multipole moments in scalar-tensor theory of gravity. Phys. Rev. D 2015, 91, 044011. [Google Scholar] [CrossRef]
- Bernuzzi, S.; Nagar, A.; Dietrich, T.; Damour, T. Modeling the dynamics of tidally interacting binary neutron stars up to the merger. Phys. Rev. Lett. 2015, 114, 161103. [Google Scholar] [CrossRef]
- Bini, D.; Damour, T.; Faye, G. Effective action approach to higher-order relativistic tidal interactions<? format?> in binary systems and their effective one body description. Phys. Rev. D 2012, 85, 124034. [Google Scholar]
- Hinderer, T.; Taracchini, A.; Foucart, F.; Buonanno, A.; Steinhoff, J.; Duez, M.; Kidder, L.E.; Pfeiffer, H.P.; Scheel, M.A.; Szilagyi, B.; et al. Effects of neutron-star dynamic tides on gravitational waveforms within the effective-one-body approach. Phys. Rev. Lett. 2016, 116, 181101. [Google Scholar] [CrossRef]
- Baiotti, L.; Damour, T.; Giacomazzo, B.; Nagar, A.; Rezzolla, L. Analytic modeling of tidal effects in the relativistic inspiral of binary neutron stars. Phys. Rev. Lett. 2010, 105, 261101. [Google Scholar] [CrossRef]
- Bernuzzi, S.; Thierfelder, M.; Brügmann, B. Accuracy of numerical relativity waveforms from binary neutron star mergers<? format?> and their comparison with post-Newtonian waveforms. Phys. Rev. D 2012, 85, 104030. [Google Scholar]
- Damour, T.; Nagar, A. Effective one body description of tidal effects in inspiralling compact binaries. Phys. Rev. D 2010, 81, 084016. [Google Scholar] [CrossRef]
- Flanagan, É.É.; Hinderer, T. Constraining neutron-star tidal Love numbers with gravitational-wave detectors. Phys. Rev. D 2008, 77, 021502. [Google Scholar] [CrossRef]
- Hinderer, T. Tidal Love numbers of neutron stars. Astrophys. J. 2008, 677, 1216. [Google Scholar] [CrossRef]
- Hinderer, T.; Lackey, B.D.; Lang, R.N.; Read, J.S. Tidal deformability of neutron stars with realistic equations of state<? format?> and their gravitational wave signatures in binary inspiral. Phys. Rev. D 2010, 81, 123016. [Google Scholar]
- Buonanno, A.; Damour, T. Effective one-body approach to general relativistic two-body dynamics. Phys. Rev. D 1999, 59, 084006. [Google Scholar] [CrossRef]
- Buonanno, A.; Damour, T. Transition from inspiral to plunge in binary black hole coalescences. Phys. Rev. D 2000, 62, 064015. [Google Scholar] [CrossRef]
- Bini, D.; Damour, T. Gravitational self-force corrections to two-body tidal interactions and the effective one-body formalism. Phys. Rev. D 2014, 90, 124037. [Google Scholar] [CrossRef]
- Andersson, N. A gravitational-wave perspective on neutron-star seismology. Universe 2021, 7, 97. [Google Scholar] [CrossRef]
- Andersson, N.; Kokkotas, K.D. Towards gravitational wave asteroseismology. Mon. Not. R. Astron. Soc. 1998, 299, 1059–1068. [Google Scholar] [CrossRef]
- Kokkotas, K.D.; Apostolatos, T.A.; Andersson, N. The inverse problem for pulsating neutron stars: A ‘fingerprint analysis’ for the supranuclear equation of state. Mon. Not. R. Astron. Soc. 2001, 320, 307–315. [Google Scholar] [CrossRef]
- Pratten, G.; Schmidt, P.; Hinderer, T. Gravitational-wave asteroseismology with fundamental modes from compact binary inspirals. Nat. Commun. 2020, 11, 2553. [Google Scholar] [CrossRef]
- Colaiuda, A.; Kokkotas, K.D. Magnetar oscillations in the presence of a crust. Mon. Not. R. Astron. Soc. 2011, 414, 3014–3022. [Google Scholar] [CrossRef]
- Gabler, M.; Cerdá-Durán, P.; Stergioulas, N.; Font, J.A.; Müller, E. Magnetoelastic oscillations of neutron stars with dipolar magnetic fields. Mon. Not. R. Astron. Soc. 2012, 421, 2054–2078. [Google Scholar] [CrossRef]
- Gabler, M.; Cerdá-Durán, P.; Stergioulas, N.; Font, J.A.; Müller, E. Coherent magneto-elastic oscillations in superfluid magnetars. Mon. Not. R. Astron. Soc. 2016, 460, 4242–4257. [Google Scholar] [CrossRef]
- Lockitch, K.H.; Friedman, J.L. Where are the r-modes of isentropic stars? Astrophys. J. 1999, 521, 764. [Google Scholar] [CrossRef]
- Papaloizou, J.; Pringle, J.E. On the rotational modes of fully convective stars with application to close binary systems. Mon. Not. R. Astron. Soc. 1981, 195, 743–753. [Google Scholar] [CrossRef]
- Kuan, H.-J.; Kokkotas, K.D. f-mode imprints on gravitational waves from coalescing binaries involving aligned spinning neutron stars. Phys. Rev. D 2022, 106, 064052. [Google Scholar] [CrossRef]
- Kuan, H.-J.; Kokkotas, K.D. Last three seconds: Packed message delivered by tides in binary neutron star mergers. Phys. Rev. D 2023, 108, 063026. [Google Scholar] [CrossRef]
- Lai, D. Resonant oscillations and tidal heating in coalescing binary neutron stars. Mon. Not. R. Astron. Soc. 1994, 270, 611–629. [Google Scholar] [CrossRef]
- Pratten, G.; Schmidt, P.; Williams, N. Impact of dynamical tides on the reconstruction of the neutron star equation of state. Phys. Rev. Lett. 2022, 129, 081102. [Google Scholar] [CrossRef]
- Williams, N.; Pratten, G.; Schmidt, P. Prospects for distinguishing dynamical tides in inspiralling binary neutron stars with third generation gravitational-wave detectors. Phys. Rev. D 2022, 105, 123032. [Google Scholar] [CrossRef]
- Tsang, D.; Read, J.S.; Hinderer, T.; Piro, A.L.; Bondarescu, R. Resonant shattering of neutron star crusts. Phys. Rev. Lett. 2012, 108, 011102. [Google Scholar] [CrossRef]
- Sullivan, A.G.; Alves, L.M.B.; Márka, Z.; Bartos, I.; Márka, S. Gamma-ray burst precursors from tidally resonant neutron star oceans: Potential implications for GRB 211211A. Mon. Not. R. Astron. Soc. 2024, 527, 7722–7730. [Google Scholar] [CrossRef]
- Kokkotas, K.D.; Schmidt, B.G. Quasi-normal modes of stars and black holes. Living Rev. Relativ. 1999, 2, 2. [Google Scholar] [CrossRef]
- Andersson, N.; Pnigouras, P. The phenomenology of dynamical neutron star tides. Mon. Not. R. Astron. Soc. 2021, 503, 533–539. [Google Scholar] [CrossRef]
- Gamba, R.; Bernuzzi, S. Resonant tides in binary neutron star mergers: Analytical-numerical relativity study. Phys. Rev. D 2023, 107, 044014. [Google Scholar] [CrossRef]
- Ma, S.; Yu, H.; Chen, Y. Excitation of f-modes during mergers of spinning binary neutron star. Phys. Rev. D 2020, 101, 123020. [Google Scholar] [CrossRef]
- Steinhoff, J.; Hinderer, T.; Buonanno, A.; Taracchini, A. Dynamical tides in general relativity: Effective action and effective-one-body Hamiltonian. Phys. Rev. D 2016, 94, 104028. [Google Scholar] [CrossRef]
- Steinhoff, J.; Hinderer, T.; Dietrich, T.; Foucart, F. Spin effects on neutron star fundamental-mode dynamical tides: Phenomenology and comparison to numerical simulations. Phys. Rev. Res. 2021, 3, 033129. [Google Scholar] [CrossRef]
- Gittins, F.; Andersson, N. Neutron-star seismology with realistic, finite-temperature nuclear matter. arXiv 2024, arXiv:2406.05177. [Google Scholar]
- Cowling, T.G.; Newing, R.A. The Oscillations of a Rotating Star. Astrophys. J. 1949, 109, 149. [Google Scholar] [CrossRef]
- Gaertig, E.; Kokkotas, K.D. Oscillations of rapidly rotating relativistic stars. Phys. Rev. D 2008, 78, 064063. [Google Scholar] [CrossRef]
- Ledoux, P. The Nonradial Oscillations of Gaseous Stars and the Problem of Beta Canis Majoris. Astrophys. J. 1951, 114, 373. [Google Scholar] [CrossRef]
- Yoshida, S.; Rezzolla, L.; Karino, S.; Eriguchi, Y. Frequencies of f-modes in differentially rotating relativistic stars and secular stability limits. Astrophys. J. 2002, 568, L41. [Google Scholar] [CrossRef]
- Dudi, R.; Dietrich, T.; Rashti, A.; Brügmann, B.; Steinhoff, J.; Tichy, W. High-accuracy simulations of highly spinning binary neutron star systems. Phys. Rev. D 2022, 105, 064050. [Google Scholar] [CrossRef]
- Read, J.S.; Lackey, B.D.; Owen, B.J.; Friedman, J.L. Constraints on a phenomenologically parametrized neutron-star equation of state. Phys. Rev. D 2009, 79, 124032. [Google Scholar] [CrossRef]
- Akcay, S.; Bernuzzi, S.; Messina, F.; Nagar, A.; Ortiz, N.; Rettegno, P. Effective-one-body multipolar waveform for tidally interacting binary neutron stars up to merger. Phys. Rev. D 2019, 99, 044051. [Google Scholar] [CrossRef]
- Nagar, A.; Bernuzzi, S.; Del Pozzo, W.; Riemenschneider, G.; Akcay, S.; Carullo, G.; Fleig, P.; Ba-bak, S.; Tsang, K.W.; Colleoni, M.; et al. Time-domain effective-one-body gravitational waveforms for coalescing compact binaries with nonprecessing spins, tides, and self-spin effects. Phys. Rev. D 2018, 98, 104052. [Google Scholar] [CrossRef]
- Abac, A.; Dietrich, T.; Buonanno, A.; Steinhoff, J.; Ujevic, M. New and robust gravitational-waveform model for high-mass-ratio binary neutron star systems with dynamical tidal effects. Phys. Rev. D 2024, 109, 024062. [Google Scholar] [CrossRef]
- Dietrich, T.; Bernuzzi, S.; Tichy, W. Closed-form tidal approximants for binary neutron star gravitational waveforms constructed from high-resolution numerical relativity simulations. Phys. Rev. D 2017, 96, 121501. [Google Scholar] [CrossRef]
- Dietrich, T.; Samajdar, A.; Khan, S.; Johnson-McDaniel, N.K.; Dudi, R.; Tichy, W. Improving the NRTidal model for binary neutron star systems. Phys. Rev. D 2019, 100, 044003. [Google Scholar] [CrossRef]
- Dietrich, T.; Khan, S.; Dudi, R.; Kapadia, S.J.; Kumar, P.; Nagar, A.; Ohme, F.; Pannarale, F.; Sa-majdar, A.; Bernuzzi, S.; et al. Matter imprints in waveform models for neutron star binaries: Tidal and self-spin effects. Phys. Rev. D 2019, 99, 024029. [Google Scholar] [CrossRef]
- Williams, N.; Schmidt, P.; Pratten, G. Phenomenological model of gravitational self-force enhanced tides in inspiralling binary neutron stars. arXiv 2024, arXiv:2407.08538. [Google Scholar]
- Suvorov, A.G.; Kokkotas, K.D. Precursor flares of short gamma-ray bursts from crust yielding due to tidal resonances in coalescing binaries of rotating, magnetized neutron stars. Phys. Rev. D 2020, 101, 083002. [Google Scholar] [CrossRef]
- Blanchet, L. Post-Newtonian theory for gravitational waves. Living Rev. Relativ. 2024, 27, 4. [Google Scholar] [CrossRef]
- Detweiler, S. Consequence of the gravitational self-force for circular orbits of the Schwarzschild geometry. Phys. Rev. D 2008, 77, 124026. [Google Scholar] [CrossRef]
- Chandrasekhar, S. Solutions of two problems in the theory of gravitational radiation. Phys. Rev. Lett. 1970, 24, 611. [Google Scholar] [CrossRef]
- Friedman, J.L.; Schutz, B.F. Langrangian perturbation theory of nonrelativistic fluids. Astrophys. J. 1978, 221, 937–957. [Google Scholar] [CrossRef]
- Friedman, J.L.; Schutz, B.F. Secular instability of rotating Newtonian stars. Astrophys. J. 1978, 222, 281–296. [Google Scholar] [CrossRef]
- Krüger, C.J.; Kokkotas, K.D.; Manoharan, P.; Völkel, S.H. Fast rotating neutron stars: Oscillations and instabilities. Front. Astron. Space Sci. 2021, 8, 736918. [Google Scholar] [CrossRef]
- Doneva, D.D.; Gaertig, E.; Kokkotas, K.D.; Krüger, C. Gravitational wave asteroseismology of fast rotating neutron stars with realistic equations of state. Phys. Rev. D 2013, 88, 044052. [Google Scholar] [CrossRef]
- Andersson, N.; Kokkotas, K.D. The r-mode instability in rotating neutron stars. Int. J. Mod. Phys. D 2001, 10, 381–441. [Google Scholar] [CrossRef]
- Cutler, C.; Apostolatos, T.A.; Bildsten, L.; Finn, L.S.; Flanagan, E.E.; Kennefick, D.; Markovic, D.M.; Ori, A.; Poisson, E.; Sussman, G.J.; et al. The last three minutes: Issues in gravitational-wave measurements of coalescing compact binaries. Phys. Rev. Lett. 1993, 70, 2984. [Google Scholar] [CrossRef] [PubMed]
- Kidder, L.E. Coalescing binary systems of compact objects to (post) 5/2-Newtonian order. V. Spin effects. Phys. Rev. D 1995, 52, 821. [Google Scholar] [CrossRef]
- Kidder, L.E.; Will, C.M.; Wiseman, A.G. Spin effects in the inspiral of coalescing compact binaries. Phys. Rev. D 1993, 47, R4183. [Google Scholar] [CrossRef] [PubMed]
- Passamonti, A.; Haskell, B.; Andersson, N.; Jones, D.I.; Hawke, I. Oscillations of rapidly rotating stratified neutron stars. Mon. Not. R. Astron. Soc. 2009, 394, 730–741. [Google Scholar] [CrossRef]
- Bernuzzi, S.; Dietrich, T.; Tichy, W.; Brügmann, B. Mergers of binary neutron stars with realistic spin. Phys. Rev. D 2014, 89, 104021. [Google Scholar] [CrossRef]
- Lai, D. Dynamical tides in rotating binary stars. Astrophys. J. 1997, 490, 847. [Google Scholar] [CrossRef]
- Fuller, J.; Lai, D. Tidal excitations of oscillation modes in compact white dwarf binaries–I. Linear theory. Mon. Not. R. Astron. Soc. 2011, 412, 1331–1340. [Google Scholar] [CrossRef]
- Racine, É.; Phinney, E.S.; Arras, P. Non-dissipative tidal synchronization in accreting binary white dwarf systems. Mon. Not. R. Astron. Soc. 2007, 380, 381–398. [Google Scholar] [CrossRef]
- Lai, D. DC circuit powered by orbital motion: Magnetic interactions in compact object binaries and exoplanetary systems. Astrophys. J. 2012, 757, L3. [Google Scholar] [CrossRef]
- Goldreich, P.; Lynden-Bell, D. Io, a Jovian unipolar inductor. Astrophys. J. 1969, 156, 59–78. [Google Scholar] [CrossRef]
- Piro, A.L. Magnetic interactions in coalescing neutron star binaries. Astrophys. J. 2012, 755, 80. [Google Scholar] [CrossRef]
- Crinquand, B.; Cerutti, B.; Dubus, G. Kinetic modeling of the electromagnetic precursor from an axisymmetric binary pulsar coalescence. Astron. Astrophys. 2019, 622, A161. [Google Scholar] [CrossRef]
- Giacomazzo, B.; Rezzolla, L.; Baiotti, L. Can magnetic fields be detected during the inspiral of binary neutron stars? Mon. Not. R. Astron. Soc. 2009, 399, L164–L168. [Google Scholar] [CrossRef]
- Zhu, Z.; Li, A.; Rezzolla, L. Tidal deformability and gravitational-wave phase evolution of magnetized compact-star binaries. Phys. Rev. D 2020, 102, 084058. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Glampedakis, K. Magnetic equilibria of relativistic axisymmetric stars: The impact of flow constants. Phys. Rev. D 2023, 108, 084006. [Google Scholar] [CrossRef]
- Arras, P.; Weinberg, N.N. Urca reactions during neutron star inspiral. Mon. Not. R. Astron. Soc. 2019, 486, 1424–1436. [Google Scholar] [CrossRef]
- Ghosh, S.; Pradhan, B.K.; Chatterjee, D. Tidal heating as a direct probe of strangeness inside neutron stars. Phys. Rev. D 2024, 109, 103036. [Google Scholar] [CrossRef]
- Meszaros, P.; Rees, M.J. Tidal heating and mass loss in neutron star binaries-Implications for gamma-ray burst models. Astrophys. J. 1992, 397, 570–575. [Google Scholar] [CrossRef]
- Kanakis-Pegios, A.; Koliogiannis, P.S.; Moustakidis, C.C. Thermal effects on tidal deformability in the last orbits of an inspiraling binary neutron star system. Phys. Lett. B 2022, 832, 137267. [Google Scholar] [CrossRef]
- Alford, M.; Harutyunyan, A.; Sedrakian, A. Bulk viscous damping of density oscillations in neutron star mergers. Particles 2000, 3, 34. [Google Scholar] [CrossRef]
- Pan, Z.; Lyu, Z.; Bonga, B.; Ortiz, N.; Yang, H. Probing crust meltdown in inspiraling binary neutron stars. Phys. Rev. Lett. 2020, 125, 201102. [Google Scholar] [CrossRef]
- Watts, A.L. Thermonuclear burst oscillations. Annu. Rev. Astron. Astrophys. 2012, 50, 609–640. [Google Scholar] [CrossRef]
- Lindblom, L.; Owen, B.J.; Ushomirsky, G. Effect of a neutron-star crust on the r-mode instability. Phys. Rev. D 2000, 62, 084030. [Google Scholar] [CrossRef]
- Mardling, R.A. The Role of Chaos in the Circularization of Tidal Capture Binaries. I. The Chaos Boundary. Astrophys. J. 1995, 450, 722. [Google Scholar] [CrossRef]
- Vick, M.; Lai, D. Dynamical tides in highly eccentric binaries: Chaos, dissipation, and quasi-steady state. Mon. Not. R. Astron. Soc. 2018, 476, 482–495. [Google Scholar] [CrossRef]
- Chirenti, C.; Gold, R.; Miller, M.C. Gravitational waves from f-modes excited by the inspiral of highly eccentric neutron star binaries. Astrophys. J. 2017, 837, 67. [Google Scholar] [CrossRef]
- Wang, J.-S.; Lai, D. Evolution of inspiralling neutron star binaries: Effects of tidal interactions and orbital eccentricities. Phys. Rev. D 2020, 102, 083005. [Google Scholar] [CrossRef]
- Yang, H. Inspiralling eccentric binary neutron stars: Orbital motion and tidal resonance. Phys. Rev. D 2019, 100, 064023. [Google Scholar] [CrossRef]
- Vick, M.; Lai, D. Tidal effects in eccentric coalescing neutron star binaries. Phys. Rev. D 2019, 100, 063001. [Google Scholar] [CrossRef]
- Takátsy, J.; Kocsis, B.; Kovács, P. Observability of dynamical tides in merging eccentric neutron star binaries. arXiv 2024, arXiv:2407.17560. [Google Scholar] [CrossRef]
- Shibata, M. Effects of tidal resonances in coalescing compact binary systems. Prog. Theor. Phys. 1994, 91, 871–883. [Google Scholar] [CrossRef]
- Gabler, M.; Sperhake, U.; Andersson, N. Nonlinear radial oscillations of neutron stars. Phys. Rev. D 2009, 80, 064012. [Google Scholar] [CrossRef]
- Koshut, T.M.; Kouveliotou, C.; Paciesas, W.S.; van Paradijs, J.; Pendleton, G.N.; Briggs, M.S.; Fishman, G.J.; Meegan, C.A. Gamma-ray burst precursor activity as observed with BATSE. Astrophys. J. 1995, 452, 145. [Google Scholar] [CrossRef]
- Troja, E.; Rosswog, S.; Gehrels, N. Precursors of short gamma-ray bursts. Astrophys. J. 2010, 723, 1711. [Google Scholar] [CrossRef]
- Zhong, S.-Q.; Dai, Z.-G.; Cheng, J.-G.; Lan, L.; Zhang, H.-M. Precursors in short gamma-ray bursts as a possible probe of progenitors. Astrophys. J. 2019, 884, 25. [Google Scholar] [CrossRef]
- Abbott, B.P. et al. [LIGO Scientific and Virgo Collaborations] Search for gravitational waves associated with gamma-ray bursts detected by Fermi and Swift during the LIGO–Virgo run O3b. Astrophys. J. 2022, 928, 186. [Google Scholar] [CrossRef]
- Thöne, C.C.; De Ugarte Postigo, A.; Fryer, C.L.; Page, K.L.; Gorosabel, J.; Aloy, M.A.; Perley, D.A.; Kouveliotou, C.; Janka, H.T.; Mimica, P.; et al. The unusual γ-ray burst GRB 101225A from a helium star/neutron star merger at redshift 0.33. Nature 2011, 480, 72–74. [Google Scholar] [CrossRef] [PubMed]
- Li, L.-X.; Paczyński, B. Transient events from neutron star mergers. Astrophys. J. 1998, 507, L59. [Google Scholar] [CrossRef]
- Valenti, S.; Sand, D.J.; Yang, S.; Cappellaro, E.; Tartaglia, L.; Corsi, A.; Jha, S.W.; Reichart, D.E.; Haislip, J.; Kouprianov, V. The discovery of the electromagnetic counterpart of GW170817: Kilonova AT 2017gfo/DLT17ck. Astrophys. J. 2017, 848, L24. [Google Scholar] [CrossRef]
- Chase, E.A.; O’Connor, B.; Fryer, C.L.; Troja, E.; Korobkin, O.; Wollaeger, R.T.; Ristic, M.; Fontes, C.J.; Hungerford, A.L.; Herring, A.M. Kilonova detectability with wide-field instruments. Astrophys. J. 2022, 927, 163. [Google Scholar] [CrossRef]
- Yang, B.; Jin, Z.-P.; Li, X.; Covino, S.; Zheng, X.-Z.; Hotokezaka, K.; Fan, Y.-Z.; Piran, T.; Wei, D.-M. A possible macronova in the late afterglow of the long–short burst grb 060614. Nat. Commun. 2015, 6, 7323. [Google Scholar] [CrossRef]
- Deng, Q.; Zhang, Z.B.; Li, X.J.; Chang, H.Y.; Zhang, X.L.; Zhen, H.Y.; Sun, H.; Pan, Q.; Dong, X.F. Reclassifying Swift Gamma-Ray Bursts with Diverse Duration Distributions. Astrophys. J. 2022, 940, 5. [Google Scholar] [CrossRef]
- Rowlinson, A.; O’Brien, P.T.; Metzger, B.D.; Tanvir, N.R.; Levan, A.J. Signatures of magnetar central engines in short GRB light curves. Mon. Not. R. Astron. Soc. 2013, 430, 1061–1087. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Kokkotas, K.D. Precessing magnetars as central engines in short gamma-ray bursts. Mon. Not. R. Astron. Soc. 2021, 502, 2482–2494. [Google Scholar] [CrossRef]
- Minaev, P.Y.; Pozanenko, A.S. Precursors of short gamma-ray bursts in the SPI-ACS/INTEGRAL experiment. Astron. Lett. 2017, 43, 1. [Google Scholar] [CrossRef]
- Qin, S.-M.; Jiang, L.-Y.; Wang, X.-G. GRB 130310A: Very high peak energy and thermal emission. Res. Astron. Astrophys. 2021, 21, 072. [Google Scholar] [CrossRef]
- Virgili, F.J.; Zhang, B.; O’Brien, P.; Troja, E. Are all short–hard gamma-ray bursts produced from mergers of compact stellar objects? Astrophys. J. 2011, 727, 109. [Google Scholar] [CrossRef]
- Pavan, A.; Ciolfi, R.; Kalinani, J.V.; Mignone, A. Short gamma-ray burst jet propagation in binary neutron star merger environments. Mon. Not. R. Astron. Soc. 2021, 506, 3483–3498. [Google Scholar] [CrossRef]
- Tsang, D. Shattering flares during close encounters of neutron stars. Astrophys. J. 2013, 777, 103. [Google Scholar] [CrossRef]
- Meredith, T.R.L.; Wynn, G.A.; Evans, P.A. An analysis of the effect of data processing methods on magnetic propeller models in short GRBs. Mon. Not. R. Astron. Soc. 2023, 519, 418–431. [Google Scholar] [CrossRef]
- Kuan, H.-J.; Suvorov, A.G.; Kokkotas, K.D. General-relativistic treatment of tidal g-mode resonances in coalescing binaries of neutron stars–II. As triggers for precursor flares of short gamma-ray bursts. Mon. Not. R. Astron. Soc. 2021, 508, 1732–1744. [Google Scholar] [CrossRef]
- Gehrels, N. Confidence limits for small numbers of events in astrophysical data. Astrophys. J. 1986, 303, 336–346. [Google Scholar] [CrossRef]
- Rastinejad, J.C.; Gompertz, B.P.; Levan, A.J.; Fong, W.; Nicholl, M.; Lamb, G.P.; Malesani, D.B.; Nugent, A.E.; Oates, S.R.; Tanvir, N.R.; et al. A kilonova following a long-duration gamma-ray burst at 350 Mpc. Nature 2022, 612, 223–227. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Kuan, H.J.; Kokkotas, K.D. Quasi-periodic oscillations in precursor flares via seismic aftershocks from resonant shattering. Astron. Astrophys. 2022, 664, A177. [Google Scholar] [CrossRef]
- Kiuchi, K.; Kyutoku, K.; Sekiguchi, Y.; Shibata, M.; Wada, T. High resolution numerical relativity simulations for the merger of binary magnetized neutron stars. Phys. Rev. D 2014, 90, 041502. [Google Scholar] [CrossRef]
- Chirenti, C.; Dichiara, S.; Lien, A.; Miller, M.C. Evidence of a Strong 19.5 Hz Flux Oscillation in Swift BAT and Fermi GBM Gamma-Ray Data from GRB 211211A. Astrophys. J. 2024, 967, 26. [Google Scholar] [CrossRef]
- Duncan, R.C. Global seismic oscillations in soft gamma repeaters. Astrophys. J. Lett. 1998, 498, L45. [Google Scholar] [CrossRef]
- Fernández, R.; Metzger, B.D. Electromagnetic signatures of neutron star mergers in the advanced LIGO era. Annu. Rev. Nucl. Part. Sci. 2016, 66, 23–45. [Google Scholar] [CrossRef]
- Zhang, B. The delay time of gravitational wave—gamma-ray burst associations. Front. Phys. 2019, 14, 64402. [Google Scholar] [CrossRef]
- Ravi, V.; Lasky, P.D. The birth of black holes: Neutron star collapse times, gamma-ray bursts and fast radio bursts. Mon. Not. R. Astron. Soc. 2014, 441, 2433–2439. [Google Scholar] [CrossRef]
- Ciolfi, R.; Kastaun, W.; Kalinani, J.V.; Giacomazzo, B. First 100 ms of a long-lived magnetized neutron star formed in a binary neutron star merger. Phys. Rev. D 2019, 100, 023005. [Google Scholar] [CrossRef]
- Ciolfi, R. Collimated outflows from long-lived binary neutron star merger remnants. Mon. Not. R. Astron. Soc. 2020, 495, L66–L70. [Google Scholar] [CrossRef]
- Kiuchi, K.; Reboul-Salze, A.; Shibata, M.; Sekiguchi, Y. A large-scale magnetic field produced by a solar-like dynamo in binary neutron star mergers. Nat. Astron. 2024, 8, 298–307. [Google Scholar] [CrossRef]
- Paschalidis, V.; Etienne, Z.B.; Shapiro, S.L. Importance of cooling in triggering the collapse of hypermassive neutron stars. Phys. Rev. D 2012, 86, 064032. [Google Scholar] [CrossRef]
- Mösta, P.; Radice, D.; Haas, R.; Schnetter, E.; Bernuzzi, S. A magnetar engine for short GRBs and kilonovae. Astrophys. J. 2020, 901, L37. [Google Scholar] [CrossRef]
- Ruiz, M.; Shapiro, S.L.; Tsokaros, A. Jet launching from binary black hole-neutron star mergers: Dependence on black hole spin, binary mass ratio, and magnetic field orientation. Phys. Rev. D 2018, 98, 123017. [Google Scholar] [CrossRef]
- Shibata, M.; Taniguchi, K. Merger of black hole and neutron star in general relativity: Tidal disruption, torus mass, and gravitational waves. Phys. Rev. D 2008, 77, 084015. [Google Scholar] [CrossRef]
- Nampalliwar, S.; Suvorov, A.G.; Kokkotas, K.D. Testing horizon topology with electromagnetic observations. Phys. Rev. D 2020, 102, 104035. [Google Scholar] [CrossRef]
- Spivey, R.J. Quasars: A supermassive rotating toroidal black hole interpretation. Mon. Not. R. Astron. Soc. 2000, 316, 856–874. [Google Scholar] [CrossRef]
- Lu, M.-X.; Li, L.; Wang, X.-G.; Deng, C.-M.; Liang, Y.-F.; Lin, D.-B.; Liang, E.-W. Searching for Associations between Short Gamma-Ray Bursts and Fast Radio Bursts. Astrophys. J. 2024, 961, 45. [Google Scholar] [CrossRef]
- Moroianu, A.; Wen, L.; James, C.W.; Ai, S.; Kovalam, M.; Panther, F.H.; Zhang, B. An assessment of the association between a fast radio burst and binary neutron star merger. Nat. Astron. 2023, 7, 579–589. [Google Scholar] [CrossRef]
- McWilliams, S.T.; Levin, J. Electromagnetic extraction of energy from black-hole–neutron-star binaries. Astrophys. J. 2011, 742, 90. [Google Scholar] [CrossRef]
- Most, E.R.; Philippov, A.A. Electromagnetic precursors to gravitational-wave events: Numerical simulations of flaring in pre-merger binary neutron star magnetospheres. Astrophys. J. 2020, 893, L6. [Google Scholar] [CrossRef]
- Carrasco, F.; Shibata, M.; Reula, O. Magnetospheres of black hole-neutron star binaries. Phys. Rev. D 2021, 104, 063004. [Google Scholar] [CrossRef]
- Most, E.R.; Philippov, A.A. Electromagnetic precursors to black hole–neutron star gravitational wave events: Flares and reconnection-powered fast radio transients from the late inspiral. Astrophys. J. 2023, 956, L33. [Google Scholar] [CrossRef]
- Metzger, B.D.; Zivancev, C. Pair fireball precursors of neutron star mergers. Mon. Not. R. Astron. Soc. 2016, 461, 4435–4440. [Google Scholar] [CrossRef]
- Daugherty, J.K.; Harding, A.K. Electromagnetic cascades in pulsars. Astrophys. J. 1982, 252, 337–347. [Google Scholar] [CrossRef]
- Medin, Z.; Lai, D. Pair cascades in the magnetospheres of strongly magnetized neutron stars. Mon. Not. R. Astron. Soc. 2010, 406, 1379–1404. [Google Scholar] [CrossRef]
- Mignani, R.P.; Testa, V.; Caniulef, D.G.; Taverna, R.; Turolla, R.; Zane, S.; Wu, K. Evidence for vacuum birefringence from the first optical polarimetry measurement of the isolated neutron star RX J1856. 5-3754. Mon. Not. R. Astron. Soc. 2017, 465, 492–500. [Google Scholar] [CrossRef]
- Taverna, R.; Turolla, R.; Muleri, F.; Heyl, J.; Zane, S.; Baldini, L.; González-Caniulef, D.; Bachetti, M.; Rankin, J.; Caiazzo, I.; et al. Polarized x-rays from a magnetar. Science 2022, 378, 646–650. [Google Scholar] [CrossRef]
- Popov, S.B.; Taverna, R.; Turolla, R. Probing the surface magnetic field structure in RX J1856. 5-3754. Mon. Not. R. Astron. Soc. 2017, 464, 4390–4398. [Google Scholar] [CrossRef]
- Hulsman, J. POLAR-2: A large scale gamma-ray polarimeter for GRBs. In Space Telescopes and Instrumentation 2020: Ultraviolet to Gamma Ray, Society of Photo-Optical Instrumentation Engineers (SPIE) Conference Series; den Herder, J.-W.A., Nikzad, S., Nakazawa, K., Eds.; SPIE Optical Engineering Press: Bellingham, WA, USA, 2020; Volume 11444, p. 114442V. [Google Scholar]
- Most, E.R.; Kim, Y.; Chatziioannou, K.; Legred, I. Nonlinear Alfvén-wave Dynamics and Premerger Emission from Crustal Oscillations in Neutron Star Mergers. arXiv 2024, arXiv:2407.17026. [Google Scholar] [CrossRef]
- Neill, D.; Tsang, D.; van Eerten, H.; Ryan, G.; Newton, W.G. Resonant shattering flares in black hole-neutron star and binary neutron star mergers. Mon. Not. R. Astron. Soc. 2022, 514, 5385–5402. [Google Scholar] [CrossRef]
- Neill, D.; Preston, R.; Newton, W.G.; Tsang, D. Constraining the Nuclear Symmetry Energy with Multimessenger Resonant Shattering Flares. Phys. Rev. Lett. 2023, 130, 112701. [Google Scholar] [CrossRef] [PubMed]
- Reed, B.T.; Fattoyev, F.J.; Horowitz, C.J.; Piekarewicz, J. Implications of PREX-2 on the equation of state of neutron-rich matter. Phys. Rev. Lett. 2021, 126, 172503. [Google Scholar] [CrossRef] [PubMed]
- Finn, L.S. G-modes in zero-temperature neutron stars. Mon. Not. R. Astron. Soc. 1987, 227, 265–293. [Google Scholar] [CrossRef]
- McDermott, P.N. Density discontinuity g-modes. Mon. Not. R. Astron. Soc. 1990, 245, 508. [Google Scholar]
- Sotani, H.; Tominaga, K.; Maeda, K.-I. Density discontinuity of a neutron star and gravitational waves. Phys. Rev. D 2001, 65, 024010. [Google Scholar] [CrossRef]
- Dong, W.; Melatos, A. Gravitational waves from non-radial oscillations of stochastically accreting neutron stars. Mon. Not. R. Astron. Soc. 2024, 530, 2822–2839. [Google Scholar] [CrossRef]
- McDermott, P.N.; van Horn, H.M.; Hansen, C.J. Nonradial oscillations of neutron stars. Astrophys. J. 1988, 325, 725–748. [Google Scholar] [CrossRef]
- Kuan, H.-J.; Suvorov, A.G.; Doneva, D.D.; Yazadjiev, S.S. Gravitational waves from accretion-induced descalarization in massive scalar-tensor theory. Phys. Rev. Lett. 2022, 129, 121104. [Google Scholar] [CrossRef]
- McDermott, P.N.; Hansen, C.J.; van Horn, H.M.; Buland, R. The nonradial oscillation spectra of neutron stars. Astrophys. J. 1985, 297, L37–L40. [Google Scholar] [CrossRef]
- Strohmayer, T.E. Density discontinuities and the g-mode oscillation spectra of neutron stars. Astrophys. J. 1993, 417, 273. [Google Scholar] [CrossRef]
- Chambers, F.R.N.; Watts, A.L. Relativistic ocean r-modes during type-I X-ray bursts. Mon. Not. R. Astron. Soc. 2020, 491, 6032–6044. [Google Scholar] [CrossRef]
- Nättilä, J.; Cho, J.Y.K.; Skinner, J.W.; Most, E.R.; Ripperda, B. Neutron Star Atmosphere–Ocean Dynamics. Astrophys. J. 2024, 971, 37. [Google Scholar] [CrossRef]
- van Baal, B.F.A.; Chambers, F.R.N.; Watts, A.L. Waves in thin oceans on oblate neutron stars. Mon. Not. R. Astron. Soc. 2020, 496, 2098–2106. [Google Scholar] [CrossRef]
- Sullivan, A.G.; Alves, L.M.B.; Spence, G.O.; Leite, I.P.; Veske, D.; Bartos, I.; Márka, Z.; Márka, S. Multimessenger emission from tidal waves in neutron star oceans. Mon. Not. R. Astron. Soc. 2023, 520, 6173–6189. [Google Scholar] [CrossRef]
- Thorne, K.S. Tidal stabilization of rigidly rotating, fully relativistic neutron stars. Phys. Rev. D 1998, 58, 124031. [Google Scholar] [CrossRef]
- Andersson, N.; Kokkotas, K.D. Gravitational waves and pulsating stars: What can we learn from future observations? Phys. Rev. Lett. 1996, 77, 4134. [Google Scholar] [CrossRef]
- Manoharan, P.; Kokkotas, K.D. Finding universal relations using statistical data analysis. Phys. Rev. D 2024, 109, 103033. [Google Scholar] [CrossRef]
- Andersson, N. A new class of unstable modes of rotating relativistic stars. Astrophys. J. 1998, 502, 708. [Google Scholar] [CrossRef]
- Friedman, J.L.; Morsink, S.M. Axial instability of rotating relativistic stars. Astrophys. J. 1998, 502, 714. [Google Scholar] [CrossRef]
- Kokkotas, K.D.; Schwenzer, K. R-mode astronomy. Eur. Phys. J. A 2016, 52, 38. [Google Scholar] [CrossRef]
- Andersson, N.; Kokkotas, K.; Schutz, B.F. Gravitational radiation limit on the spin of young neutron stars. Astrophys. J. 1999, 510, 846. [Google Scholar] [CrossRef]
- Antonopoulou, D.; Espinoza, C.M.; Kuiper, L.; Andersson, N. Pulsar spin-down: The glitch-dominated rotation of PSR J0537-6910. Mon. Not. R. Astron. Soc. 2018, 473, 1644–1655. [Google Scholar] [CrossRef]
- Andersson, N.; Antonopoulou, D.; Espinoza, C.M.; Haskell, B.; Ho, W.C.G. The enigmatic spin evolution of PSR J05370-6910: R-modes, gravitational waves, and the case for continued timing. Astrophys. J. 2018, 864, 137. [Google Scholar] [CrossRef]
- Rezzolla, L.; Lamb, F.K.; Marković, D.; Shapiro, S.L. Properties of r modes in rotating magnetic neutron stars. II. Evolution of the r modes and stellar magnetic field. Phys. Rev. D 2001, 64, 104014. [Google Scholar] [CrossRef]
- Balbus, S.A.; Hawley, J.F. A powerful local shear instability in weakly magnetized disks. I-Linear analysis. II-Nonlinear evolution. Astrophys. J. 1991, 376, 214–233. [Google Scholar] [CrossRef]
- Duez, M.D.; Liu, Y.T.; Shapiro, S.L.; Shibata, M.; Stephens, B.C. Evolution of magnetized, differentially rotating neutron stars:<? format?> Simulations in full general relativity. Phys. Rev. D 2006, 73, 104015. [Google Scholar]
- Dehman, C.; Pons, J.A. On the Origin of Magnetar Fields: Chiral Magnetic Instability in Neutron Star Crusts. arXiv 2024, arXiv:2408.05281. [Google Scholar]
- Mészáros, P.; Rees, M.J. Steep slopes and preferred breaks in gamma-ray burst spectra: The role of photospheres and comptonization. Astrophys. J. 2000, 530, 292. [Google Scholar] [CrossRef]
- Lyutikov, M. Electrodynamics of binary neutron star mergers. Mon. Not. R. Astron. Soc. 2019, 483, 2766–2777. [Google Scholar] [CrossRef]
- Sridhar, N.; Zrake, J.; Metzger, B.D.; Sironi, L.; Giannios, D. Shock-powered radio precursors of neutron star mergers from accelerating relativistic binary winds. Mon. Not. R. Astron. Soc. 2021, 501, 3184–3202. [Google Scholar] [CrossRef]
- Beskin, V.S. Radio pulsars: Already fifty years! Phys. Uspekhi 2018, 61, 353. [Google Scholar] [CrossRef]
- Beloborodov, A.M. Emission of magnetar bursts and precursors of neutron star mergers. Astrophys. J. 2021, 921, 92. [Google Scholar] [CrossRef]
- Mastrano, A.; Suvorov, A.G.; Melatos, A. Interpreting the AXP 1E 2259+ 586 antiglitch as a change in internal magnetization. Mon. Not. R. Astron. Soc. 2015, 453, 522–530. [Google Scholar] [CrossRef]
- Coppin, P.; van Eijndhoven, N. IceCube search for high-energy neutrinos produced in the precursor stages of gamma-ray bursts. In Proceedings of the 36th International Cosmic Ray Conference (ICRC2019), Madison, WI, USA, 24 July–1 August 2019; Volume 36, p. 859. [Google Scholar]
- Aartsen, M.G. et al. [IceCube Collaboration] Evidence for high-energy extraterrestrial neutrinos at the IceCube detector. Science 2013, 342, 1242856. [Google Scholar] [PubMed]
- Aartsen, M.G. et al. [IceCube Collaboration] A combined maximum-likelihood analysis of the high-energy astrophysical neutrino flux measured with IceCube. Astrophys. J. 2015, 809, 98. [Google Scholar] [CrossRef]
- Abbasi, R. et al. [IceCube Collaboration] IceCube high-energy starting event sample: Description and flux characterization with 7.5 years of data. Phys. Rev. D 2021, 104, 022002. [Google Scholar] [CrossRef]
- Aartsen, M.G. et al. [IceCube-Gen2 Collaboration] IceCube-Gen2: The window to the extreme Universe. J. Phys. G 2021, 48, 060501. [Google Scholar] [CrossRef]
- Murase, K.; Bartos, I. High-energy multimessenger transient astrophysics. Annu. Rev. Nucl. Part. Sci. 2019, 69, 477. [Google Scholar] [CrossRef]
- Anchordoqui, L.A.; Barger, V.; Cholis, I.; Goldberg, H.; Hooper, D.; Kusenko, A.; Learned, J.G.; Marfatia, D.; Pakvasa, S.; Paul, T.C.; et al. Cosmic Neutrino Pevatrons: A Brand New Pathway to Astronomy, Astrophysics, and Particle Physics. J. High Energy Astrophys. 2014, 1, 1. [Google Scholar] [CrossRef]
- Fujikawa, K.; Shrock, R. Magnetic moment of a massive neutrino and neutrino-spin rotation. Phys. Rev. Lett. 1980, 45, 963. [Google Scholar] [CrossRef]
- Shrock, R.E. Electromagnetic properties and decays of Dirac and Majorana neutrinos in a general class of gauge theories. Nucl. Phys. B 1982, 206, 359–379. [Google Scholar] [CrossRef]
- Giunti, C.; Studenikin, A. Neutrino electromagnetic interactions: A window to new physics. Rev. Mod. Phys. 2015, 87, 531–591. [Google Scholar] [CrossRef]
- Vietri, M. On the acceleration of ultra high energy cosmic rays in gamma ray bursts. Astrophys. J. 1995, 453, 883–889. [Google Scholar] [CrossRef]
- Waxman, E. Cosmological gamma-ray bursts and the highest energy cosmic rays. Phys. Rev. Lett. 1995, 75, 386. [Google Scholar] [CrossRef] [PubMed]
- Brdar, V.; Cheng, T.; Kuan, H.-J.; Li, Y.-Y. Magnetar-powered neutrinos and magnetic moment signatures at IceCube. J. Cosmol. Astropart. Phys. 2024, 2024, 026. [Google Scholar] [CrossRef]
- Guépin, C.; Kotera, K.; Oikonomou, F. High-energy neutrino transients and the future of multi-messenger astronomy. Nat. Rev. Phys. 2022, 4, 697–712. [Google Scholar] [CrossRef]
- Branchesi, M.; Maggiore, M.; Alonso, D.; Badger, C.; Banerjee, B.; Beirnaert, F.; Belgacem, E.; Bhagwat, S.; Boileau, G.; Borhanian, S.; et al. Science with the Einstein Telescope: A comparison of different designs. J. Cosmol. Astropart. Phys. 2023, 2023, 068. [Google Scholar] [CrossRef]
- Hild, S.; Abernathy, M.; Acernese, F.; Amaro-Seoane, P.; Andersson, N.; Arun, K.; Barone, F.; Barr, B.; Barsuglia, M.; Beker, M.; et al. Sensitivity studies for third-generation gravitational wave observatories. Class. Quantum Gravity 2011, 28, 094013. [Google Scholar] [CrossRef]
- Maggiore, M.; Van Den Broeck, C.; Bartolo, N.; Belgacem, E.; Bertacca, D.; Bizouard, M.A.; Branchesi, M.; Clesse, S.; Foffa, S.; García-Bellido, J.; et al. Science case for the Einstein telescope. J. Cosmol. Astropart. Phys. 2020, 2020, 050. [Google Scholar] [CrossRef]
- Punturo, M.; Abernathy, M.; Acernese, F.; Allen, B.; Andersson, N.; Arun, K.; Barone, F.; Barr, B.; Barsuglia, M.; Beker, M.; et al. The Einstein Telescope: A third-generation gravitational wave observatory. Class. Quantum Gravity 2010, 27, 194002. [Google Scholar] [CrossRef]
- Abbott, B.P. et al. [LIGO Scientific Collaboration] Exploring the sensitivity of next generation gravitational wave detectors. Class. Quantum Gravity 2017, 34, 044001. [Google Scholar] [CrossRef]
- Evans, M.; Adhikari, R.X.; Afle, C.; Ballmer, S.W.; Biscoveanu, S.; Borhanian, S.; Brown, D.A.; Chen, Y.; Eisenstein, R.; Gruson, A.; et al. A horizon study for cosmic explorer: Science, observatories, and community. arXiv 2021, arXiv:2109.09882. [Google Scholar]
- Reitze, D.; Adhikari, R.X.; Ballmer, S.; Barish, B.; Barsotti, L.; Billingsley, G.L.; Brown, D.A.; Chen, Y.; Coyne, D.; Eisenstein, R.; et al. Cosmic explorer: The US contribution to gravitational-wave astronomy beyond LIGO. Bull. Am. Astron. Soc. 2019, 51, 035. [Google Scholar]
- Evans, M.; Corsi, A.; Afle, C.; Ananyeva, A.; Arun, K.G.; Ballmer, S.; Bandopadhyay, A.; Barsotti, L.; Baryakhtar, M.; Berger, E.; et al. Cosmic Explorer: A submission to the NSF MPSAC ngGW subcommittee. arXiv 2023, arXiv:2306.13745. [Google Scholar]
- Amaro-Seoane, P.; Andrews, J.; Arca Sedda, M.; Askar, A.; Baghi, Q.; Balasov, R.; Bartos, I.; Bavera, S.S.; Bellovary, J.; Berry, C.P.L.; et al. Astrophysics with the laser interferometer space antenna. Living Rev. Relativ. 2023, 26, 2. [Google Scholar]
- Destounis, K.; Suvorov, A.G.; Kokkotas, K.D. Testing spacetime symmetry through gravitational waves from extreme-mass-ratio inspirals. Phys. Rev. D 2020, 102, 064041. [Google Scholar] [CrossRef]
- Destounis, K.; Suvorov, A.G.; Kokkotas, K.D. Gravitational wave glitches in chaotic extreme-mass-ratio inspirals. Phys. Rev. Lett. 2021, 126, 141102. [Google Scholar] [CrossRef]
- Berti, E.; Cardoso, V.; Starinets, A.O. Quasinormal modes of black holes and black branes. Class. Quantum Gravity 2009, 26, 163001. [Google Scholar] [CrossRef]
- Konoplya, R.A.; Zhidenko, A. Quasinormal modes of black holes: From astrophysics to string theory. Rev. Mod. Phys. 2011, 83, 793–836. [Google Scholar] [CrossRef]
- Suvorov, A.G.; Völkel, S.H. Exact theory for the Rezzolla-Zhidenko metric and self-consistent calculation of quasinormal modes. Phys. Rev. D 2021, 103, 044027. [Google Scholar] [CrossRef]
- Chirenti, C.; Dichiara, S.; Lien, A.; Miller, M.C.; Preece, R. Kilohertz quasiperiodic oscillations in short gamma-ray bursts. Nature 2023, 613, 253–256. [Google Scholar] [CrossRef]
- Zink, B.; Lasky, P.D.; Kokkotas, K.D. Are gravitational waves from giant magnetar flares observable? Phys. Rev. D 2012, 85, 024030. [Google Scholar] [CrossRef]
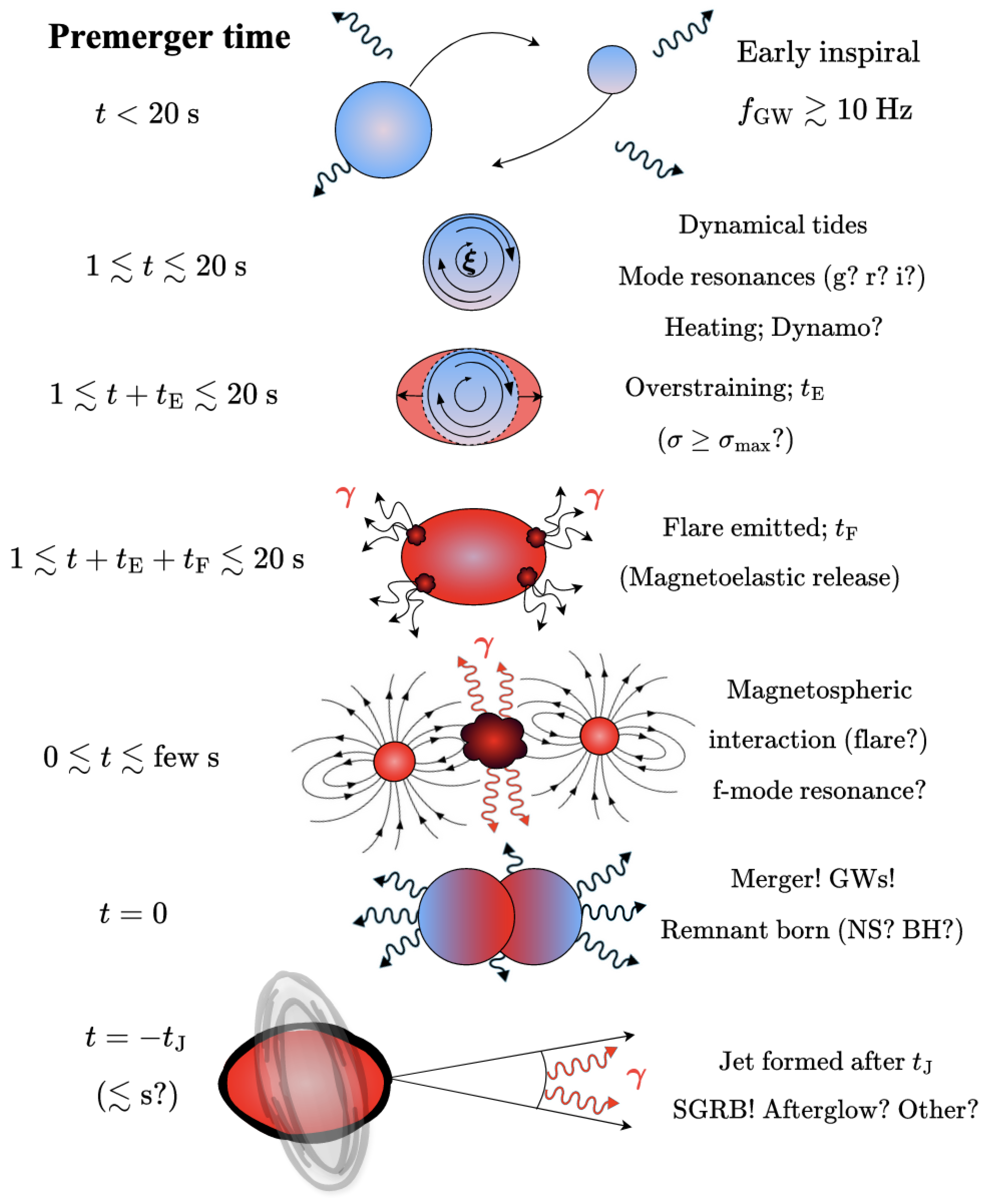


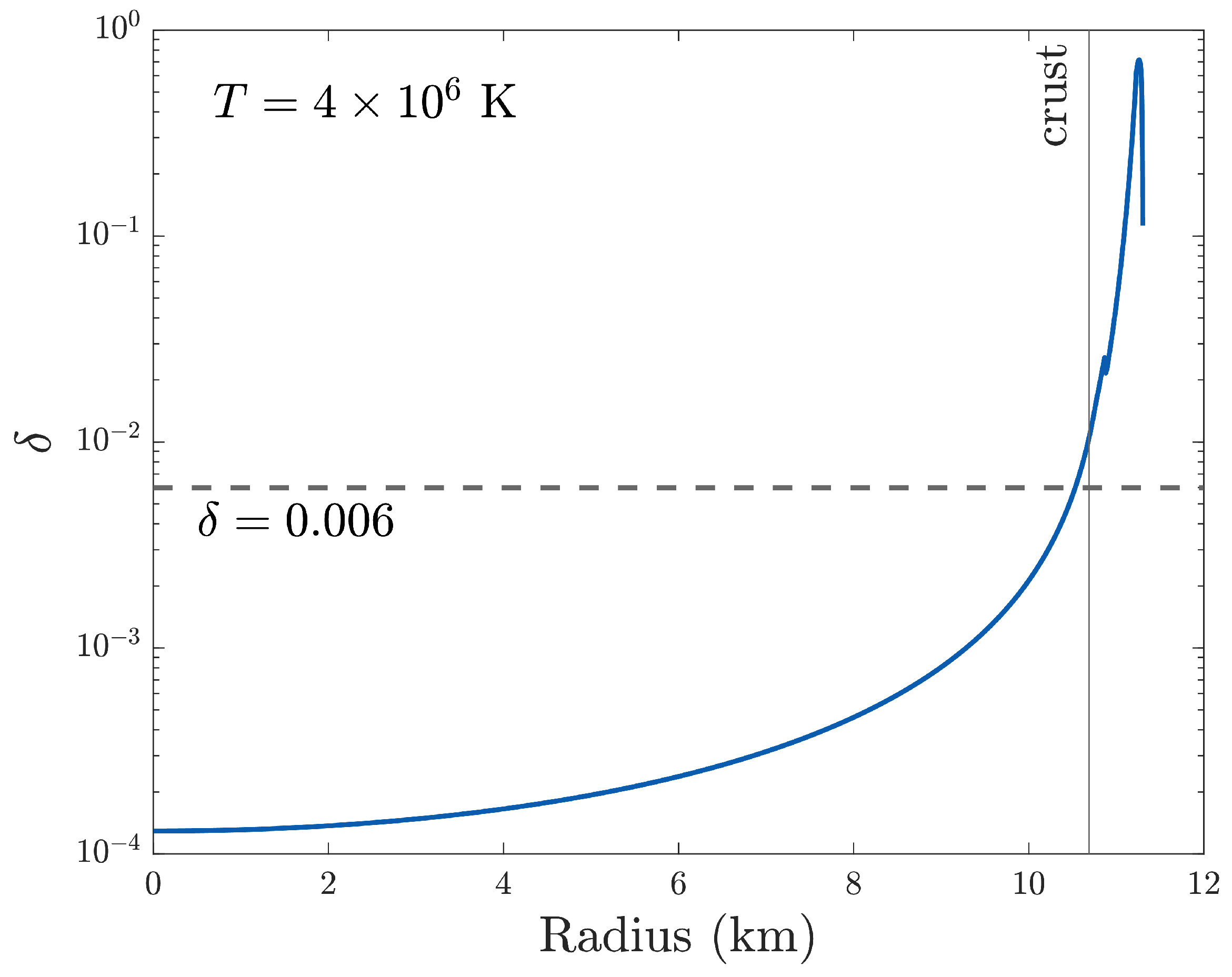
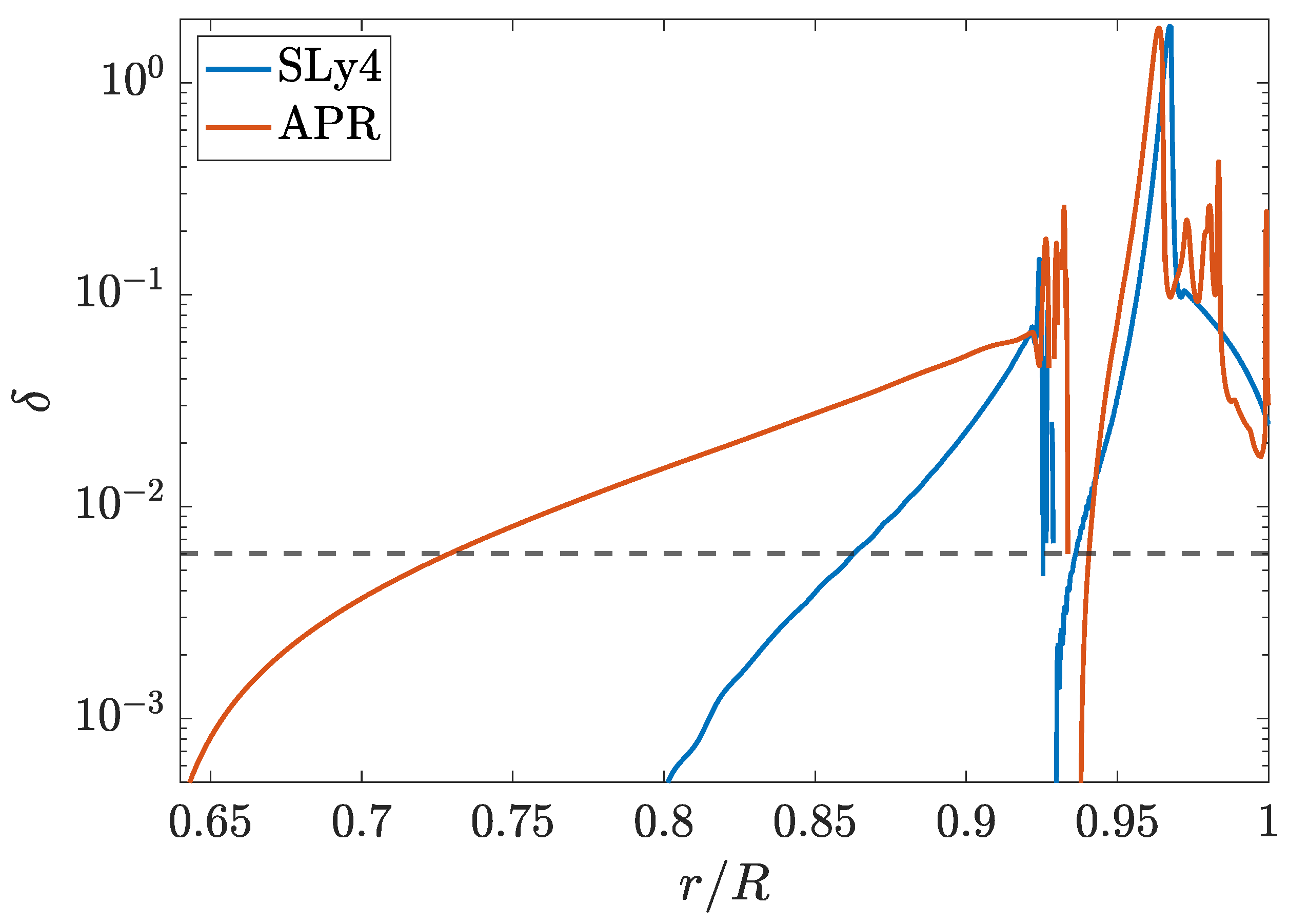

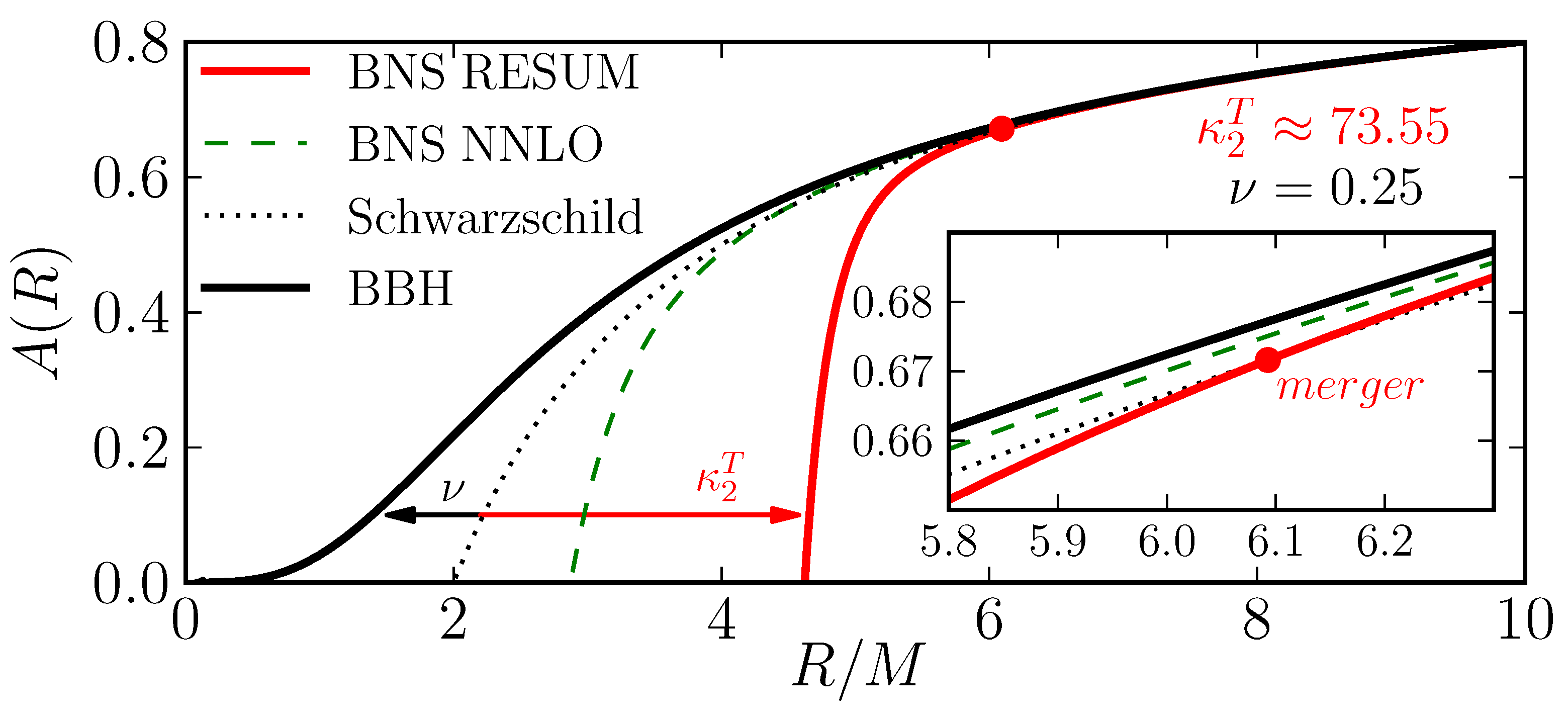
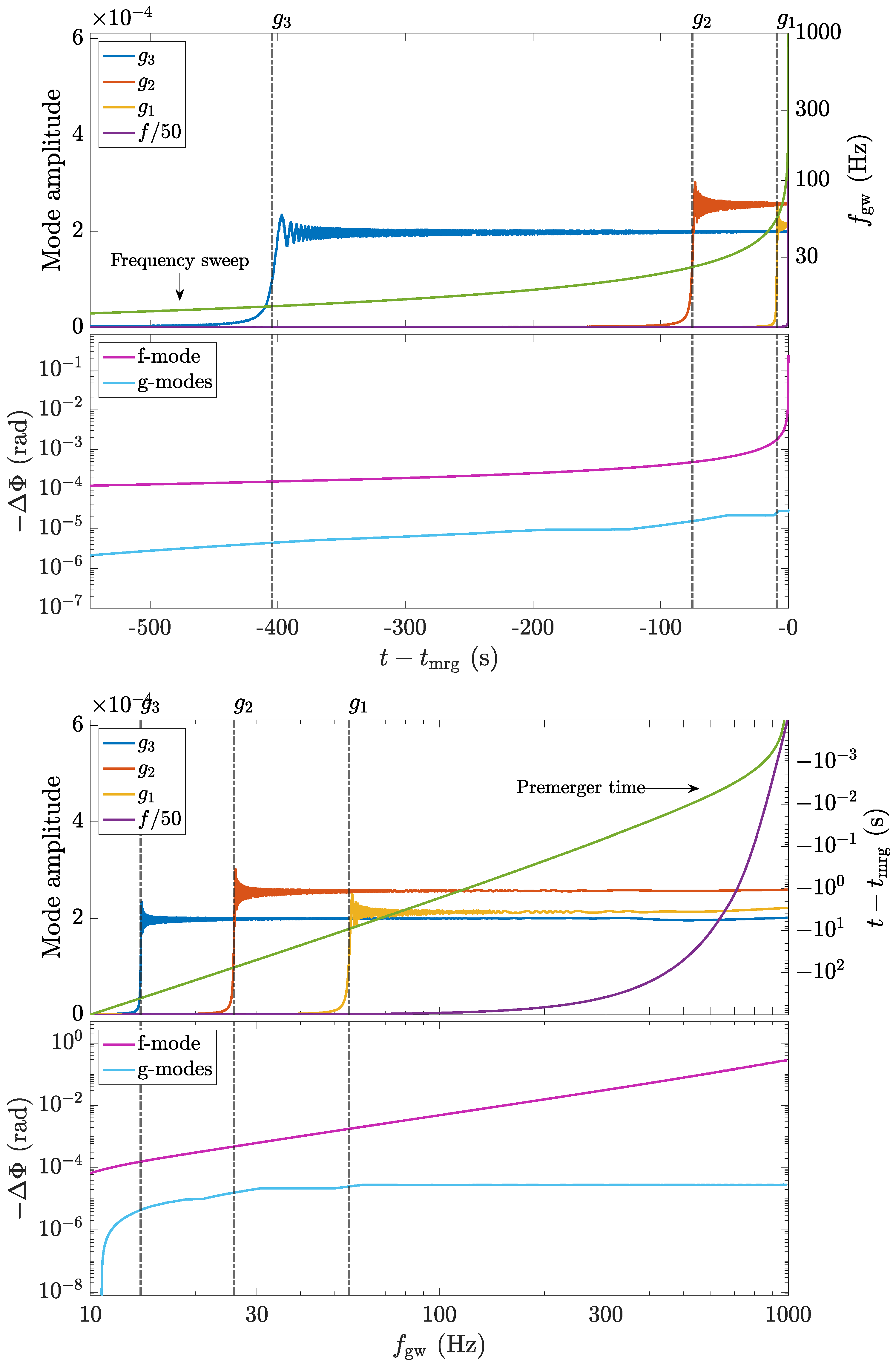


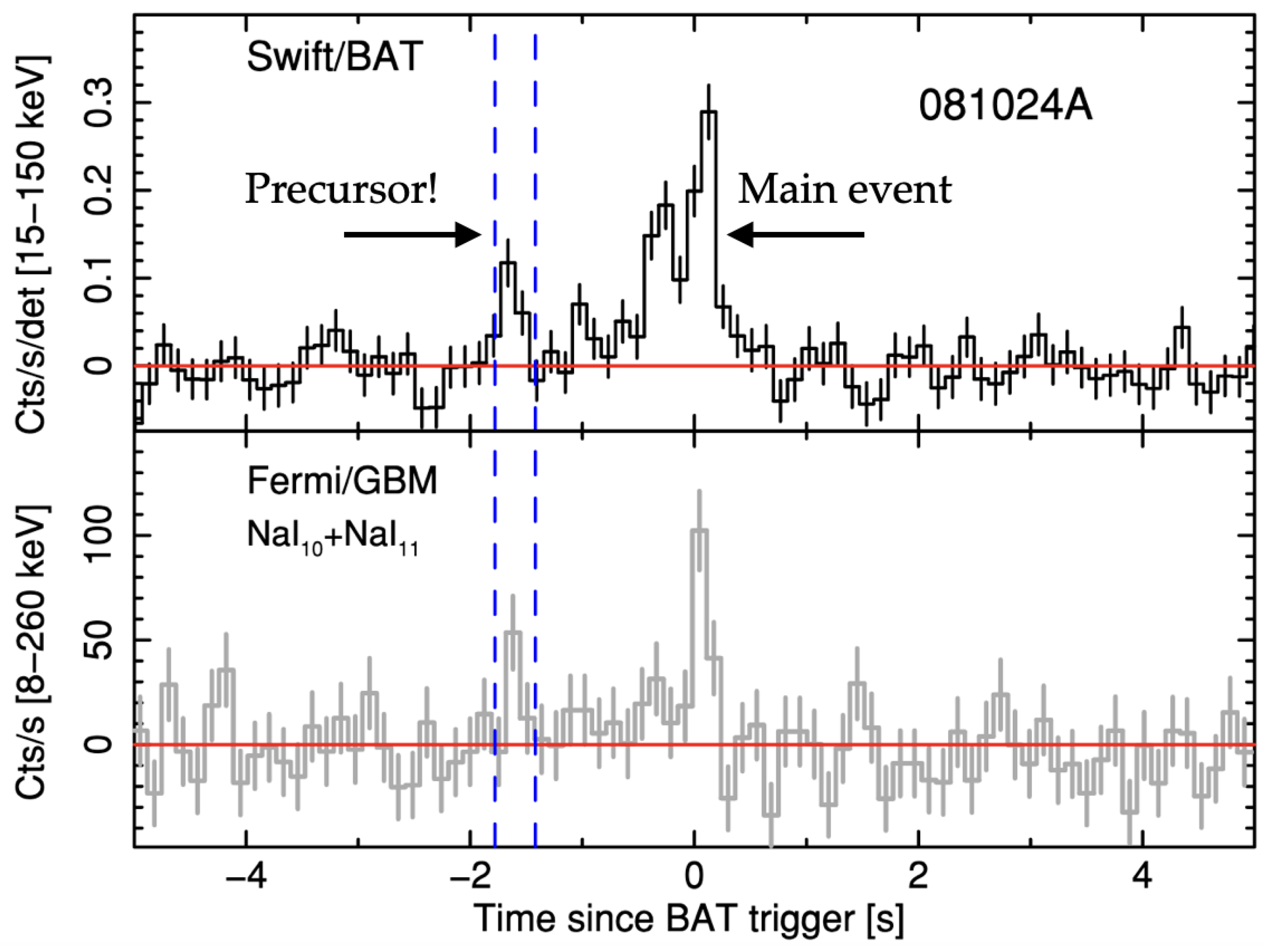
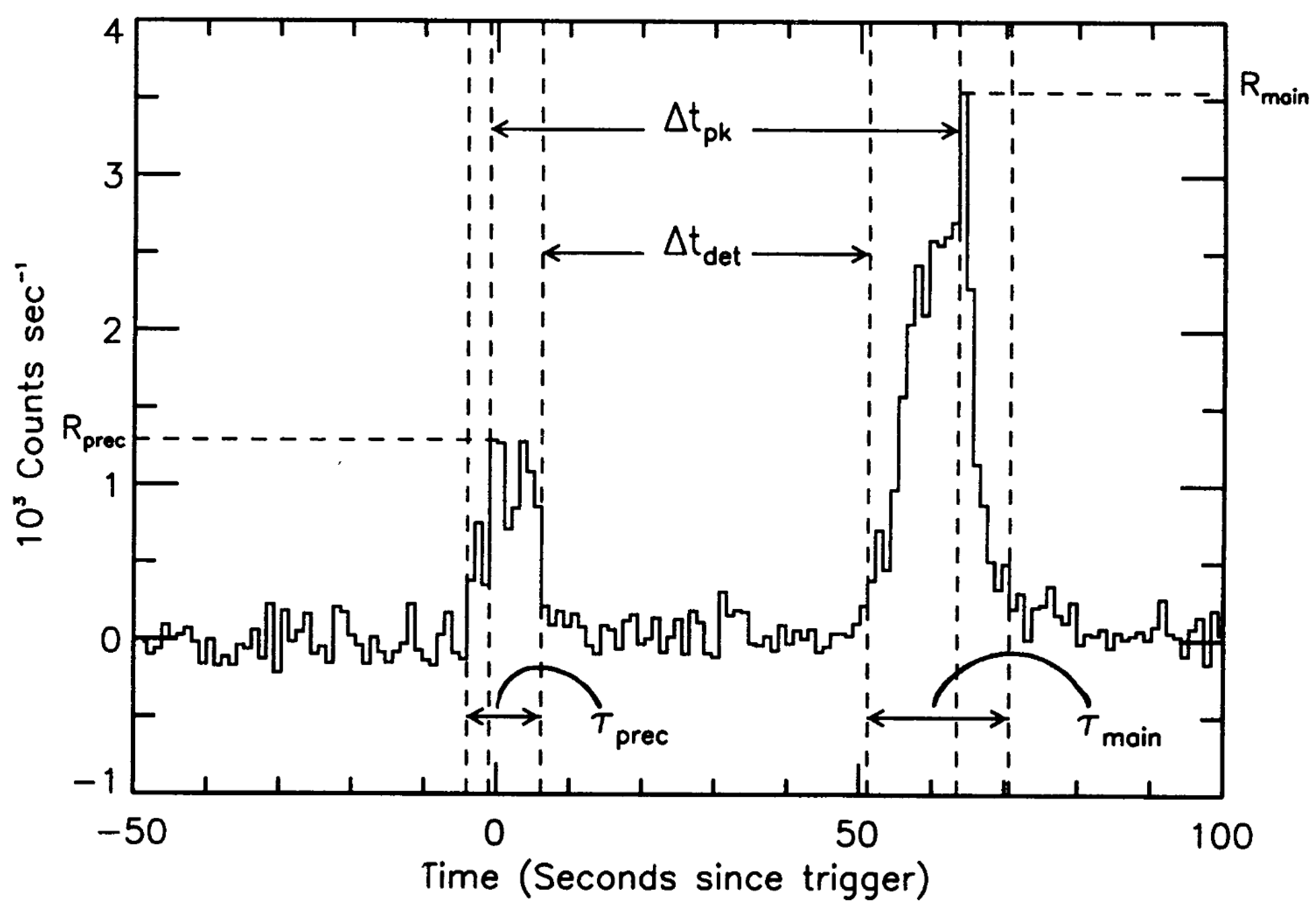
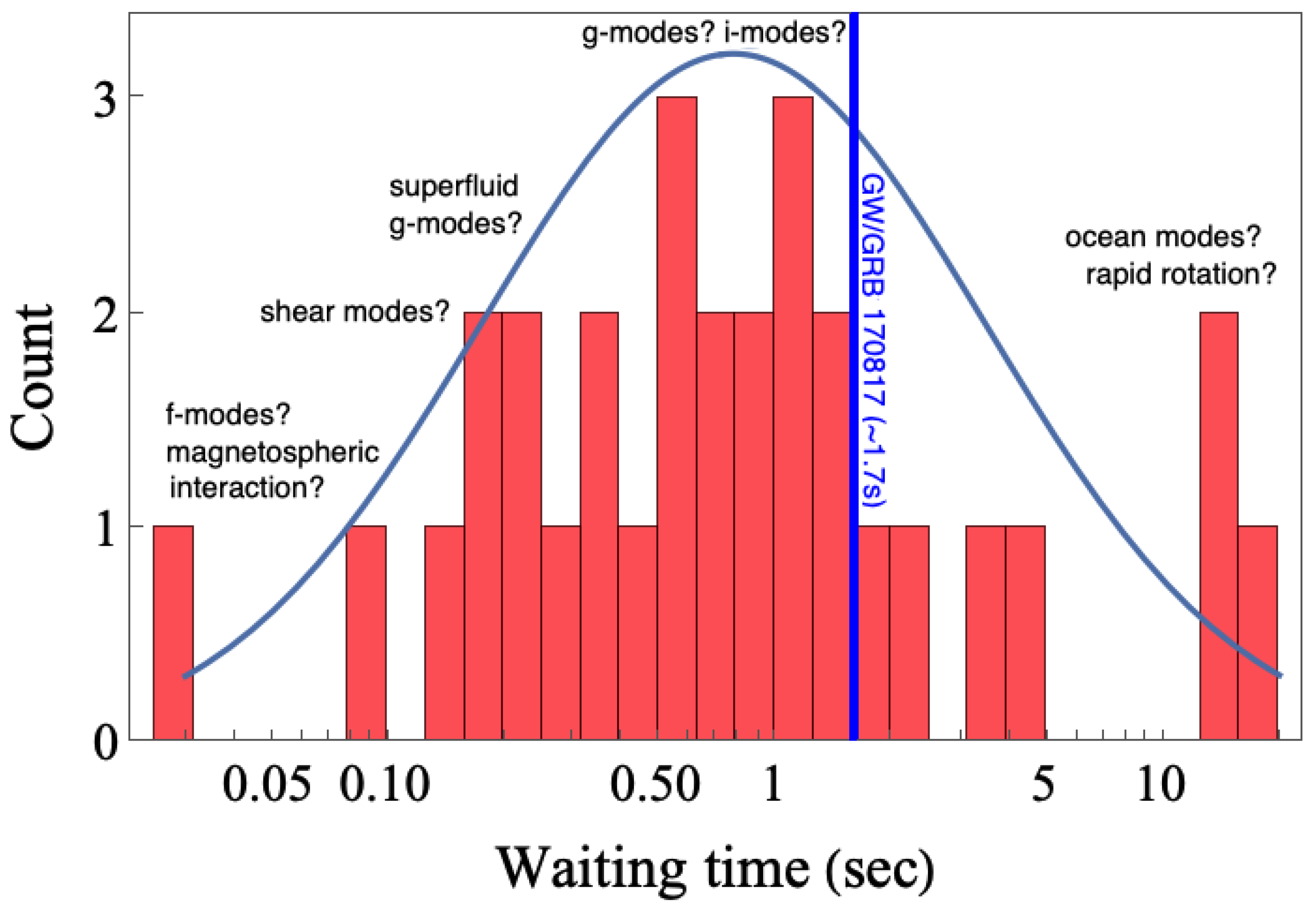
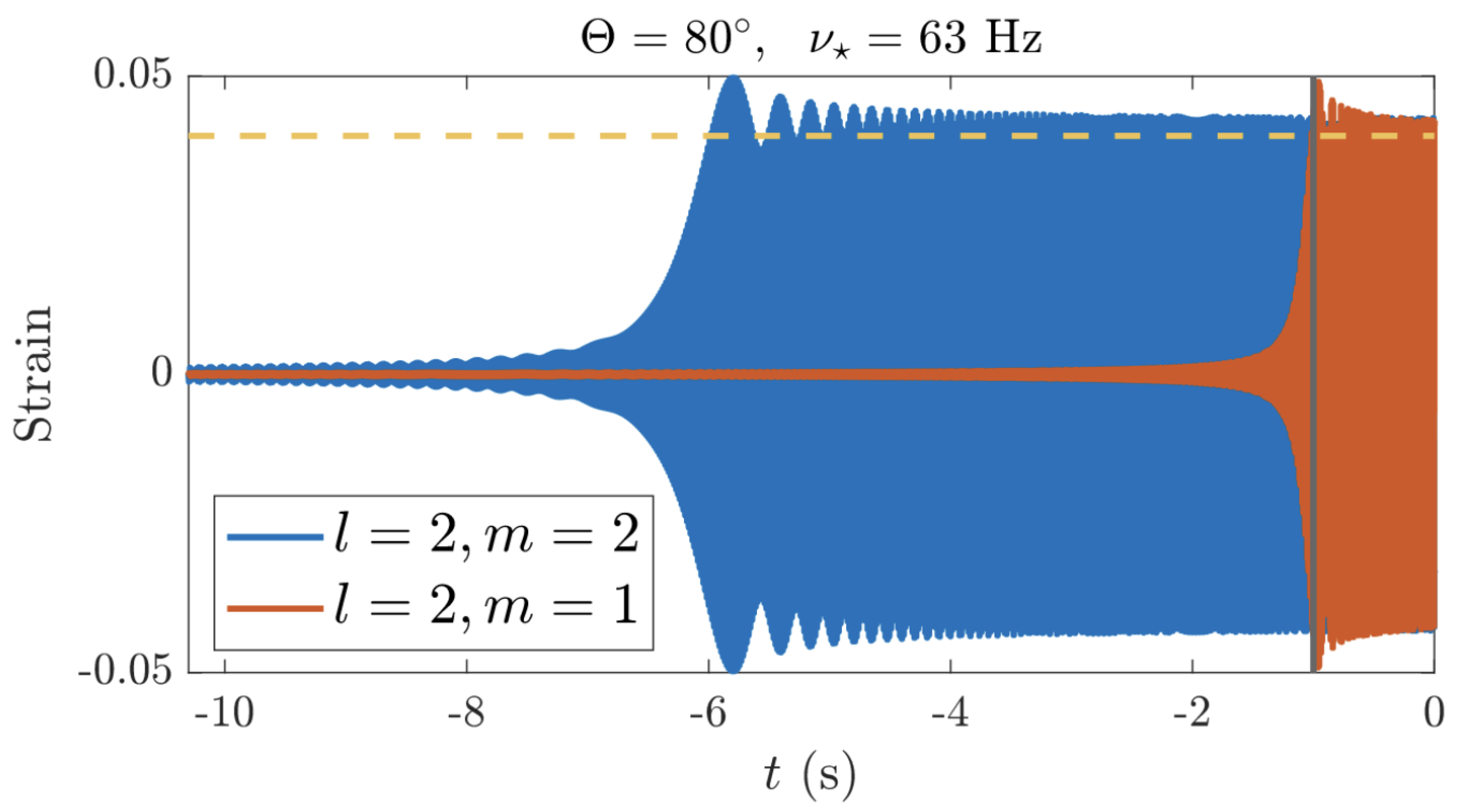
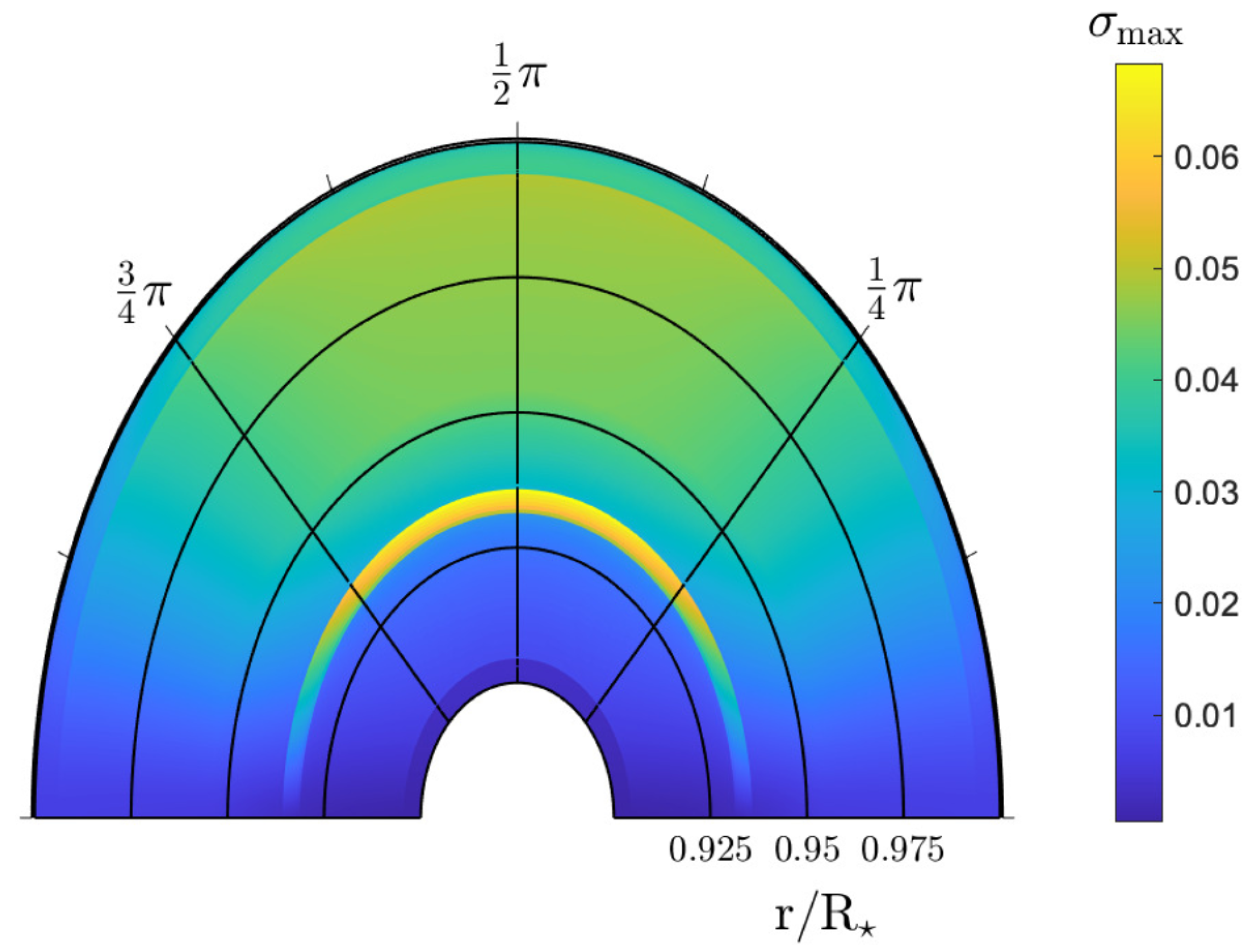

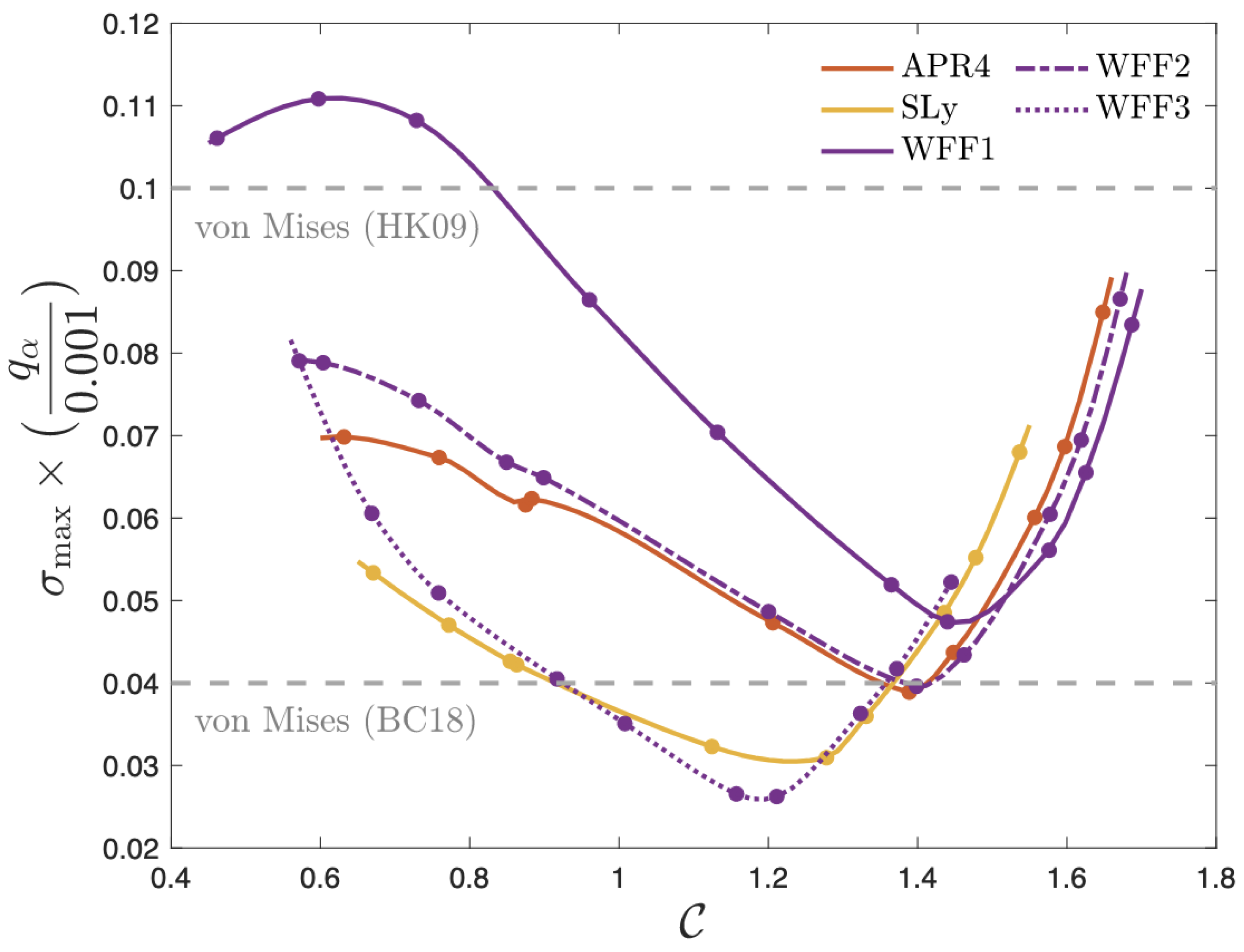

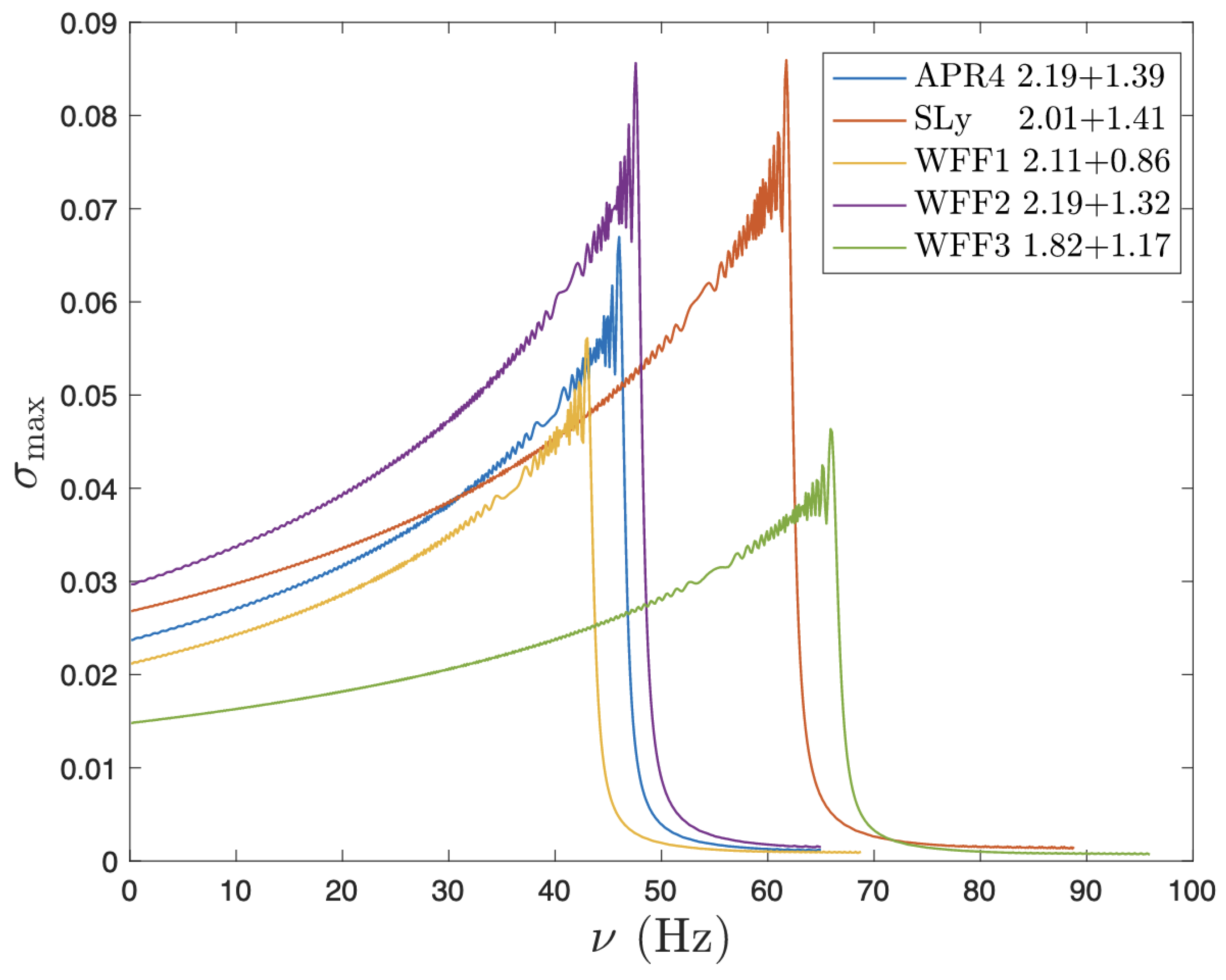

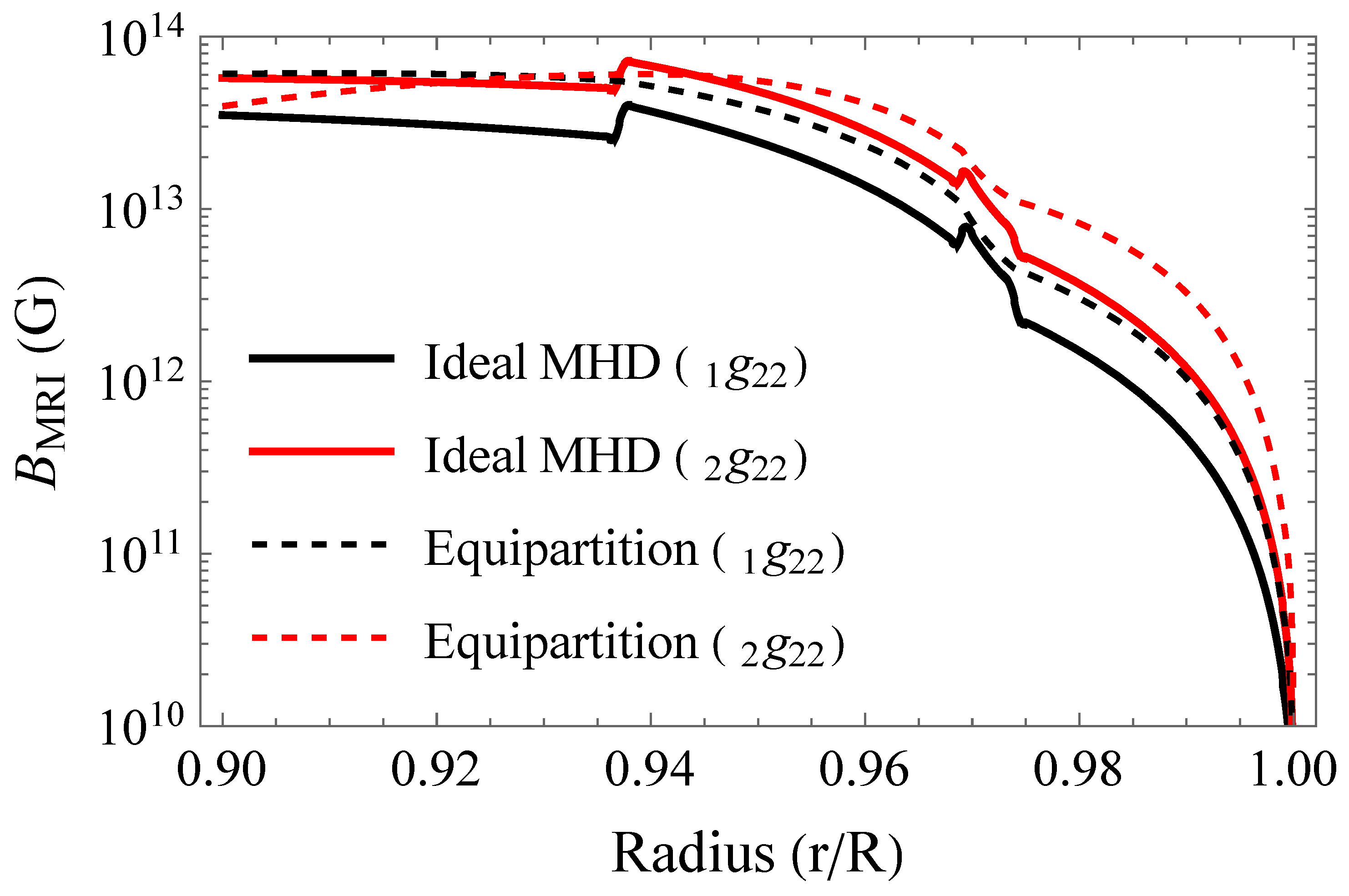
| Physical Model/Simulation Setup | (Dimensionless) | Reference(s) |
|---|---|---|
| Imperfect (alkali) metals | [164] | |
| Perfect one-component crystal | [165] | |
| Li crystals ( Mg, K) | [166] | |
| Energy and event rates of magnetar flares | [37] | |
| Perfect, defective, and poly-crystal () | [162] | |
| Perfect body-centred cubic crystal | [167] | |
| Pure and imperfect crystals (various compositions) | [168] | |
| Maximum strain set by spin limit ( kHz) | [169] | |
| Polycrystalline crust (anisotropic, variable) | () | [170] |
| Idealised nuclear pasta ( MeV) | [171] | |
| Deformed mono-crystals | [163] | |
| Multi-ion (strongly ordered) crystal ( MeV) | [172] | |
| Near-equilibrium, stretched lattice (various composition) | [173] |
| Post-Newtonian Order | Effect(s) | Reference(s) |
|---|---|---|
| 0 | Energy deposited into modes | [230,231] |
| 1 | Stellar structure; pericentre advance | [238,239] |
| 1.5 | Scalar-field contributions to dynamics (non-GR) | [235,240] |
| 1.5 | Spin–orbit coupling; tail backscatter | [241] |
| 2 | Self–spin, spin–spin, quadrupole–monopole couplings, mag. dipoles | [242,243] |
| 2.5 | Quadrupole formula for GWs | [211] |
| 3 | Gravitational tails of tails | [244] |
| 4 | Dissipative tidal number | [187,188] |
| 5 | Gravitoelectric quadrupole Love number | [245,246] |
| 6 | Gravitomagnetic quadrupole Love number | [247,248] |
| Spin-tidal coupling | [247,249] |
| Source | Precursor Duration (s) | Relative Delay (s) | GRB Duration (s) | Remarkable Features |
|---|---|---|---|---|
| 150922A | Peak flux larger than that of the main pulse | |||
| 100223110 | - | |||
| 080702A | XAM; Stable magnetar? | |||
| 160804180 | - | |||
| 170709334 | Thermal precursor and main GRB | |||
| 111117A | XAM; Stable magnetar? Debated ; | |||
| 100702A | XAM; s | |||
| 180703B | Thermal spectra; long-duration precursor | |||
| 060502B | Debated | |||
| 100827455 | Debated waiting time [353] | |||
| 230307A | s | LGRB but Kilonova? erg/s(!) | ||
| 090510 (I) | Double! XAM; ; peaks in 15–50 keV band | |||
| 081216 | Debated spectra [26] | |||
| 071112B | - | |||
| 150604434 | - | |||
| 100213A | - | |||
| 181126A | - | |||
| 081024A | Debated ; XAM; Collapse time s? | |||
| 211211A | LGRB; Kilonova; QPOs main and prec(!); XAM | |||
| 140209A | Debated ( s?); LGRB?; Strongly thermal | |||
| 101208498 | - | |||
| 141102A | Thermal spectra | |||
| 170726794 | - | |||
| 170802638 | - | |||
| 071030 | Data appear lost (see [353])? Debated | |||
| 100717 | Strongly non-thermal. Debated | |||
| 130310A | Debated spectra [364]; Magnetar flare? QPOs | |||
| 180511437 | LGRB? Debated ; Longest precursor | |||
| 090510 (II) | Double! Peaks around ∼300 keV | |||
| 191221A | - |
| System | Engine | Mechanism | (s) | (s; #) | (s) | (s) | |||
|---|---|---|---|---|---|---|---|---|---|
| (s) | (ms) | (s) | |||||||
| BH-NS | BH | accretion | ∼ 0 | ∼ 0 | ; | < few | ∼3 | ||
| BH-NS | HMNS/BH | accretion | ; (*) | ∼ 0 | ; | ≲ few (‡) | ≲1 | >5 (*) | |
| BH-NS | HMNS/BH | magnetic | ∼ 0 | (†) | <1 | ; | ≲ few (‡) | ≲1 | ∼5 |
| NS-NS | BH | accretion | ∼ 0 | ∼ 0 | ; | < few | ∼3 | ||
| NS-NS | HMNS/BH | accretion | ; (*) | ∼ 0 | ; | ≲ few | >5 (*) | ||
| NS-NS | HMNS/BH | magnetic | ∼ 0 | (†) | ; | ∼ few | > 3 | ||
| NS-NS | SMNS/NS | accretion | ∼ 0 | ; | ≲ few | ≳ 3 | |||
| NS-NS | SMNS/NS | magnetic | ∼ 0 | (†) | (**) | (**) | ∼ few | ≲1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suvorov, A.G.; Kuan, H.-J.; Kokkotas, K.D. Premerger Phenomena in Neutron Star Binary Coalescences. Universe 2024, 10, 441. https://doi.org/10.3390/universe10120441
Suvorov AG, Kuan H-J, Kokkotas KD. Premerger Phenomena in Neutron Star Binary Coalescences. Universe. 2024; 10(12):441. https://doi.org/10.3390/universe10120441
Chicago/Turabian StyleSuvorov, Arthur G., Hao-Jui Kuan, and Kostas D. Kokkotas. 2024. "Premerger Phenomena in Neutron Star Binary Coalescences" Universe 10, no. 12: 441. https://doi.org/10.3390/universe10120441
APA StyleSuvorov, A. G., Kuan, H.-J., & Kokkotas, K. D. (2024). Premerger Phenomena in Neutron Star Binary Coalescences. Universe, 10(12), 441. https://doi.org/10.3390/universe10120441






