Effect of Link Misalignment in the Optical-Internet of Underwater Things
Abstract
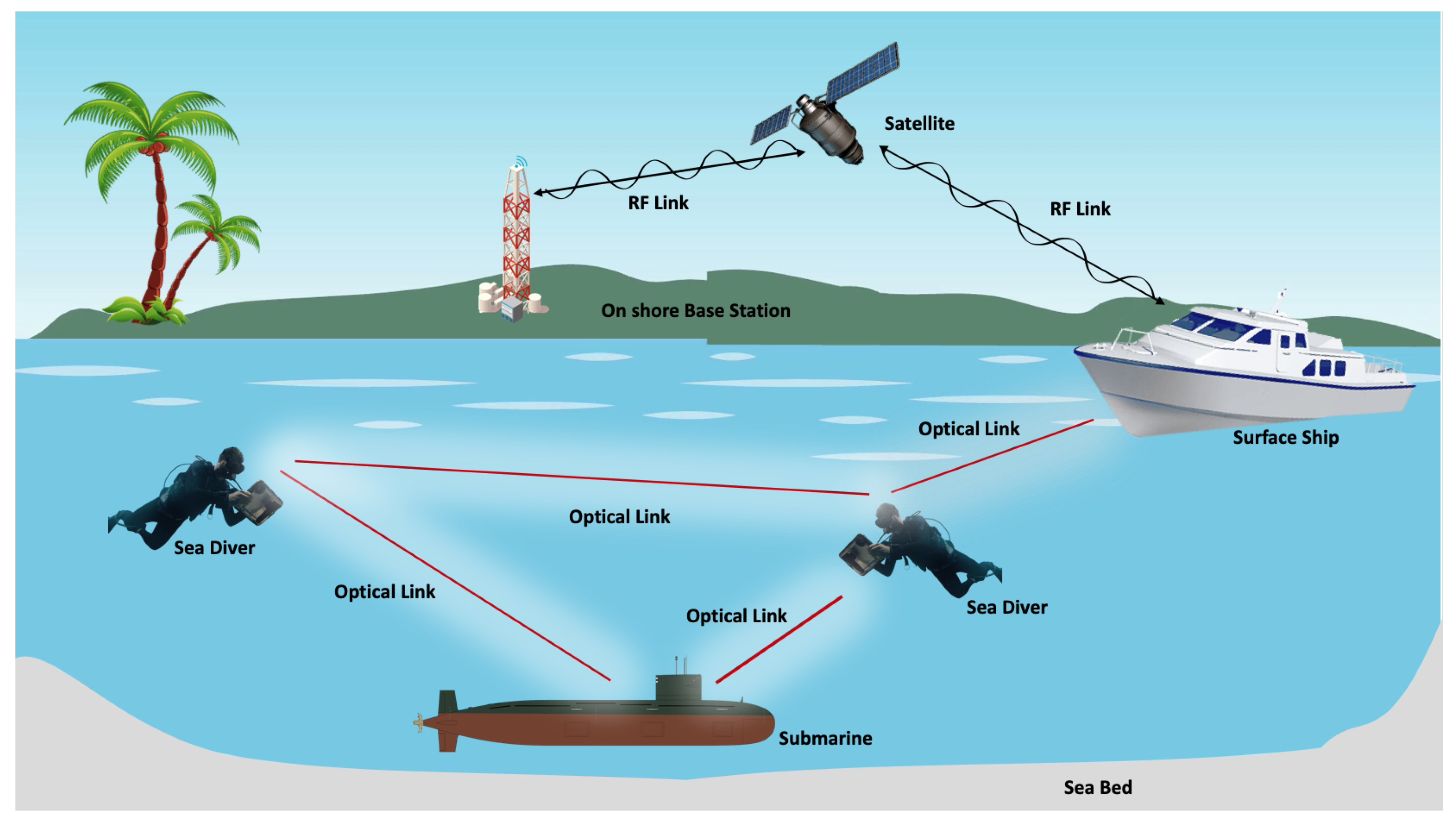
1. Introduction
- First, we investigate the misalignment between Tx and Rx for a single-input-single-output (SISO) O-IoUT system by considering various optical beams such as Plane, Gaussian, and Spherical.
- Then, we analyze the effect of divergence angle for all these beams in the presence of link misalignment.
- Furthermore, we derive the BER expression for the O-IoUT link when there is a misalignment between the Tx and the Rx.
- Lastly, the proposed approach for link misalignment in O-IoUT has been evaluated based on normalized received power, maximum acceptable literal offset (MALO), and BER performance.
2. Related Work
2.1. Literature on O-IoUT
2.2. O-IoUT: Requirements and Challenges
2.2.1. Absorption and Scattering
2.2.2. Multi-path fading and Time Jitter
2.2.3. Pointing and Link Misalignment
3. Proposed System Modeling and Effect of Misalignment
- A well-known UWOC channel model is considered for the system evaluation, i.e., the radiative transfer equation (RTE) [13]. The best consideration of RTE is given by Beer Lambert’s law, where there is only straight-line transmission of light without considering the multi-path scattering. This simplification along with radiance of light R is expressed as;in (1), the term r is known as the transmission distance, and a is the attenuation coefficient in context of absorption of light and scattering of light . Hence, their relationship is given byNote that here we consider normalized received power because the underwater optical wireless channel is highly dynamic, and instantaneous power can significantly vary over time and may leads to inappropriate estimation. Furthermore, Monte-Carlo simulation technique is utilized along with (1) for better tracing of photons.
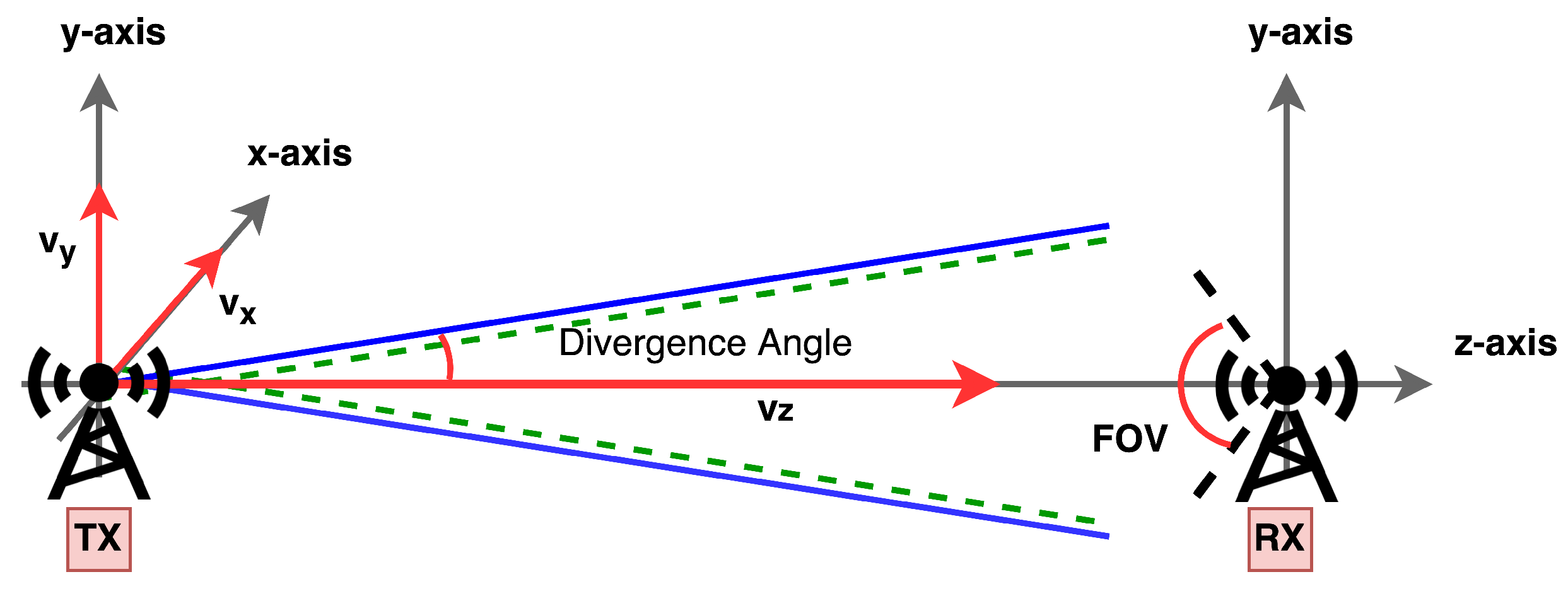
- Monte-Carlo simulations are used for the Tx modeling, where the initial position of the Tx is with moving directional cosines of the photons . At the Tx end, both polar angles and radial angles must be set precisely to obtain the initial track. The gets updated in each scattering event during the transmission phase along the path of propagation and is calculated recursively. The variations in and for both the Tx and Rx are depicted in Figure 3. It should be noted that is taken as a random variable in the range of .
- The most important part is to measure the antecedent position of each and every photon of the considered Gaussian beam [54,55]. To analyze the overall distribution of the Gaussian beam, it is of greater importance to first plot the probability density function (PDF) and cumulative distribution function (CDF). Hence, the PDF and CDF for the considered radial position are expressed aswhich on simplification results aswhere , and is known as the waist of Gaussian beam. In the above PDF, is represented aswhere the term is a random number selected uniformly from the range . Afterwards, the initial positions are expressed as . According to [56], the divergence angle of the Tx is taken at . After modeling the Tx for the given UWOC system, the initial direction cosines are represented byDuring modeling phase, the photons are considered to be randomly dispersed in a circular region with a defined smaller area. With such an assumption, the PDF for the position of is radial, and is given in [55] for each photon as,whereHere, i is the radius of the defined area. The photons’ initial position is attained by , and their direction is taken along the channel that can be expressed asIn order to appropriately model the spherical beam, it is assumed that most of the photons commenced from the original source having initial coordinates as . The photonic radiation is readily considered to propagate only along –direction, and assumed to be radiated from point isotropic source in a frontward hemisphere [54]. In the context of polar angle, the PDF and CDF are then expressed as;andwhere is a source function defined for the considered spherical beam [54]. Subsequently, at , can be expressed as .
- The photons traveling in an underwater environment usually deviate from the defined direction after striking with water particles. In the initial state, the weights of these photons are made equal to unity. These initial state weights will further be reduced by water albedo at each scattering event. The albedo of water is termed as the ratio of coefficient of scattering and coefficient of attenuation as . It basically defines the percentage drop in weights of the photons caused by the absorption occurred as a result of interaction with different particles present in water. During this interaction, the path followed by these photons can be properly traced by three random parameters such as , , and directly associated with the trajectory. Among these parameters, is the distance between two consecutive interactions and is expressed as followswhere is taken as a random variable and obtained from interval . It should be noted that the parameters and defined another scattering direction for these photons as compared to the prior direction that was defined due to the interaction by the coordinate system. However, at each scattering event, the new scattering direction is transformed into a more generalized coordinate system as depicted in Figure 3. The details for implementation of such a scenario using Monte Carlo simulations can be found in [54,56]. It is easy to estimate and for each photon at every transmission phase. This, in turn highly impact the speed of designed simulation due to the complex analytical computation of from the designated CDF. According to [57], it can be determined by a specific function known as the scattering phase function (SPF). However, according to [56], the numerical calculations take a lot of time to calculate photonic propagation as well as the realization of the channel at each phase. In contrast, to avoid the time-consuming process, can be calculated from the SPF function in a much efficient way. During the simulations stage, a variation of this technique known as Henyey Greenstein (HG) SPF [57] is used, which is expressed aswhere s represents the asymmetry of HG that solely depends upon features of the medium, and is approximately equal to the average of in all scattering directions. However, function HG can only anticipate the scattering function for angles having values . This is following the Petzold’s data given in [55], and cannot predict minute frontward scattering angles. In contrast, HG-SPF based realization can predict the polar angle in terms of random numeric value for in each scattering event [54], and is given byWhen the weights of the photons significantly drop than the defined threshold and , it follows the scattering phenomena along the channel in both clean and turbid water. The procedure of rouletting is adopted for the termination of these scattered photons along the propagation path [56].
- On the Rx side, only those photons are received which are in the limits of FOV and aperture of the Rx. Furthermore, the weights of photons at different axis, and the distance traveled are recorded for calculation of various quantities such as effect on link misalignment, MALO, and BER performance.
4. Bit Error Rate Analysis for Link Misalignment
5. Results and Discussions
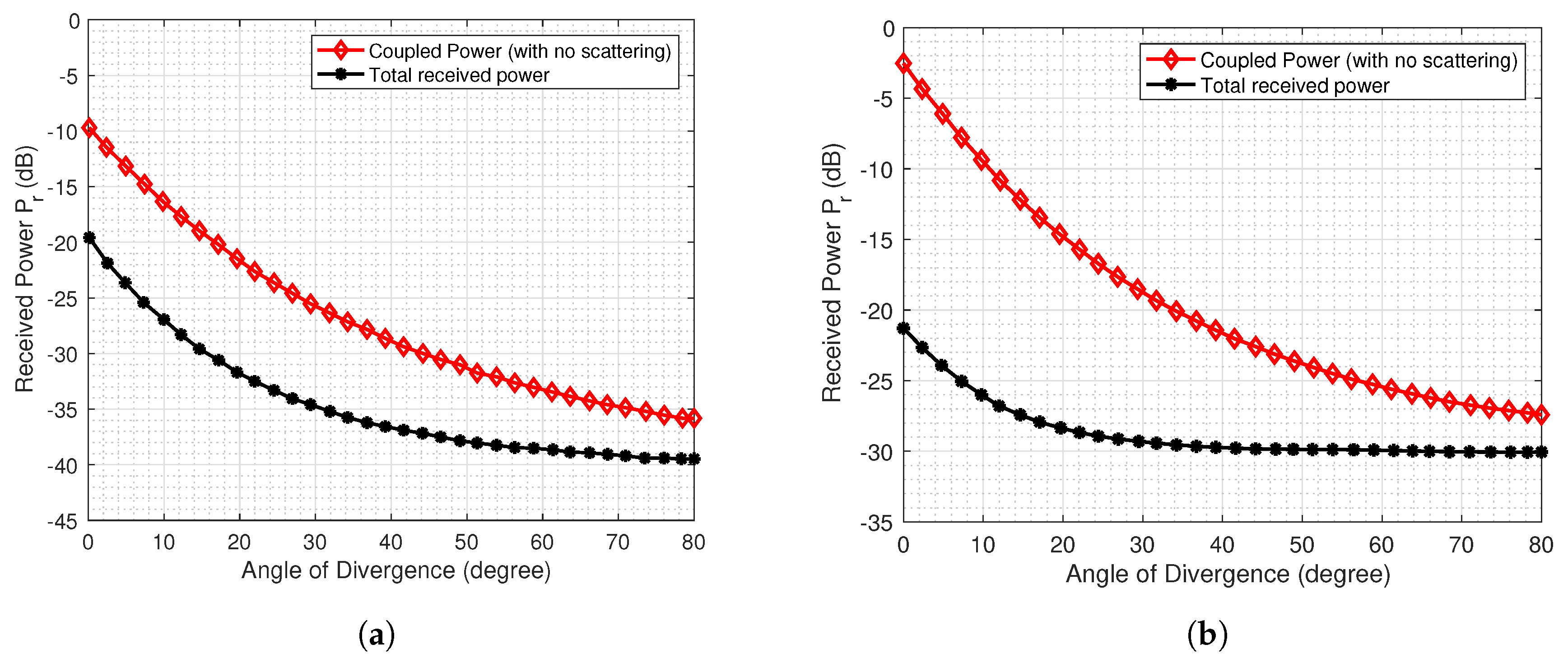
5.1. Impact on Link Misalignment
5.2. Maximum Acceptable Literal Offset (MALO) for Rx
5.3. BER Calculation
6. Conclusions and Future Work
Author Contributions
Funding
Conflicts of Interest
References
- Ali, M.; Jayakody, D.N.; Chursin, Y.; Affes, S.; Dmitry, S. Recent Advances and Future Directions on Underwater Wireless Communications. Arch. Comput. Methods Eng. 2019, 26, 1–34. [Google Scholar] [CrossRef]
- Kao, C.C.; Lin, Y.S.; Wu, G.D.; Huang, C.J. A comprehensive study on the internet of underwater things: Applications, challenges, and channel models. Sensors 2017, 17, 1477. [Google Scholar] [CrossRef] [PubMed]
- Domingo, M.C. An overview of the internet of underwater things. J. Netw. Comput. Appl. 2012, 35, 1879–1890. [Google Scholar] [CrossRef]
- Gussen, C.; Diniz, P.; Campos, M.; Martins, W.A.; Costa, F.M.; Gois, J.N. A survey of underwater wireless communication technologies. J. Commun. Inf. Syst. 2016, 31, 242–255. [Google Scholar] [CrossRef]
- Saeed, N.; Alouini, M.S.; Al-Naffouri, T.Y. Accurate 3D Localization of Selected Smart Objects in Optical Internet of Underwater Things. IEEE Internet of Things J. 2020, 7, 937–947. [Google Scholar] [CrossRef]
- Demirors, E.; Sklivanitis, G.; Melodia, T.; Batalama, S.N.; Pados, D.A. Software-defined underwater acoustic networks: Toward a high-rate real-time reconfigurable modem. IEEE Commun. Mag. 2015, 53, 64–71. [Google Scholar] [CrossRef]
- Khalil, R.A.; Saeed, N.; Jan, T.; Ashraf, M. Multiple Object Localization in Underwater Wireless Communication Systems using the Theory of Gravitation. In Proceedings of the 25th International Conference on Mechatronics and Machine Vision in Practice (M2VIP), Stuttgart, Germany, 20–22 November 2018; pp. 1–6. [Google Scholar]
- Saeed, N.; Celik, A.; Al-Naffouri, T.Y.; Alouini, M.S. Underwater optical wireless communications, networking, and localization: A survey. Ad Hoc Netw. 2019, 94, 101935. [Google Scholar] [CrossRef]
- Kaushal, H.; Kaddoum, G. Underwater optical wireless communication. IEEE Access 2016, 4, 1518–1547. [Google Scholar] [CrossRef]
- Uysal, M.; Capsoni, C.; Ghassemlooy, Z.; Boucouvalas, A.; Udvary, E. Optical Wireless Communications: An Emerging Technology; Springer: Berlin, Germany, 2016. [Google Scholar]
- Saeed, N.; Celik, A.; Al-Naffouri, T.Y.; Alouini, M.S. Energy Harvesting Hybrid Acoustic-Optical Underwater Wireless Sensor Networks Localization. Sensors 2017, 18, 51. [Google Scholar] [CrossRef]
- Zeng, Z. A Survey of Underwater Wireless Optical Communication. Ph.D. Thesis, University of British Columbia, Vancouver, BC, USA, 2015. [Google Scholar]
- Gabriel, C.; Khalighi, M.A.; Bourennane, S.; Léon, P.; Rigaud, V. Monte-Carlo-based channel characterization for underwater optical communication systems. J. Opt. Commun. Netw. 2013, 5, 1–12. [Google Scholar] [CrossRef]
- Boucouvalas, A.C.; Peppas, K.P.; Yiannopoulos, K.; Ghassemlooy, Z. Underwater optical wireless communications with optical amplification and spatial diversity. IEEE Photonics Technol. Lett. 2016, 28, 2613–2616. [Google Scholar] [CrossRef]
- Dalgleish, F.R.; Shirron, J.J.; Rashkin, D.; Giddings, T.E.; Dalgleish, A.K.V.; Cardei, I.; Ouyang, B.; Caimi, F.M.; Cardei, M. Physical layer simulator for undersea free-space laser communications. Opt. Eng. 2014, 53, 051410. [Google Scholar] [CrossRef]
- Tang, S.; Dong, Y.; Zhang, X. Impulse response modeling for underwater wireless optical communication links. IEEE Trans. Commun. 2013, 62, 226–234. [Google Scholar] [CrossRef]
- Anguita, D.; Brizzolara, D.; Parodi, G.; Hu, Q. Optical wireless underwater communication for AUV: Preliminary simulation and experimental results. In Proceedings of the OCEANS, Santander, Spain, 6–9 June 2011; pp. 1–5. [Google Scholar]
- Dong, Y.; Tang, S.; Zhang, X. Effect of random sea surface on downlink underwater wireless optical communications. IEEE Commun. Lett. 2013, 17, 2164–2167. [Google Scholar] [CrossRef]
- Dong, Y.; Zhang, H.; Zhang, X. On impulse response modeling for underwater wireless optical MIMO links. In Proceedings of the IEEE/CIC International Conference on Communications in China (ICCC), Shanghai, China, 13–15 October 2014; pp. 151–155. [Google Scholar]
- Xu, J.; Song, Y.; Yu, X.; Lin, A.; Kong, M.; Han, J.; Deng, N. Underwater wireless transmission of high-speed QAM-OFDM signals using a compact red-light laser. Opt. Express 2016, 24, 8097–8109. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Hui, L.; Dong, Y. Angle of arrival analysis for underwater wireless optical links. IEEE Commun. Lett. 2015, 19, 2162–2165. [Google Scholar] [CrossRef]
- Liou, E.C.; Kao, C.C.; Chang, C.H.; Lin, Y.S.; Huang, C.J. Internet of Underwater Things: Challenges and routing protocols. In Proceedings of the IEEE International Conference on Applied System Invention (ICASI), Chiba, Japan, 13–17 April 2018; pp. 1171–1174. [Google Scholar]
- Peppas, K.P.; Boucouvalas, A.C.; Ghassemloy, Z. Performance of underwater optical wireless communication with multi-pulse pulse-position modulation receivers and spatial diversity. IET Optoelectron. 2017, 11, 180–185. [Google Scholar] [CrossRef]
- Arnon, S. Underwater optical wireless communication network. Opt. Eng. 2010, 49, 015001. [Google Scholar] [CrossRef]
- Cai, Y.; Lin, Q. Hollow elliptical Gaussian beam and its propagation through aligned and misaligned paraxial optical systems. JOSA A 2004, 21, 1058–1065. [Google Scholar] [CrossRef]
- Borah, D.K.; Voelz, D.G. Pointing Error Effects on Free-Space Optical Communication Links in the Presence of Atmospheric Turbulence. J. Light. Technol. 2009, 27, 3965–3973. [Google Scholar] [CrossRef]
- Farid, A.A.; Hranilovic, S. Outage Capacity Optimization for Free-Space Optical Links With Pointing Errors. J. Light. Technol. 2007, 25, 1702–1710. [Google Scholar] [CrossRef]
- Sandalidis, H.G.; Tsiftsis, T.A.; Karagiannidis, G.K.; Uysal, M. BER Performance of FSO Links over Strong Atmospheric Turbulence Channels with Pointing Errors. IEEE Commun. Lett. 2008, 12, 44–46. [Google Scholar] [CrossRef]
- Jamali, M.V.; Mirani, A.; Parsay, A.; Abolhassani, B.; Nabavi, P.; Chizari, A.; Khorramshahi, P.; Abdollahramezani, S.; Salehi, J.A. Statistical Studies of Fading in Underwater Wireless Optical Channels in the Presence of Air Bubble, Temperature, and Salinity Random Variations. IEEE Trans. Commun. 2018, 66, 4706–4723. [Google Scholar] [CrossRef]
- Miramirkhani, F.; Uysal, M. Visible Light Communication Channel Modeling for Underwater Environments With Blocking and Shadowing. IEEE Access 2018, 6, 1082–1090. [Google Scholar] [CrossRef]
- Zhang, H.; Dong, Y. Link misalignment for underwater wireless optical communications. In Proceedings of the Advances in Wireless and Optical Communications (RTUWO), Riga, Latvia, 5–6 November 2015; pp. 215–218. [Google Scholar]
- Tang, S.; Dong, Y.; Zhang, X. On link misalignment for underwater wireless optical communications. IEEE Commun. Lett. 2012, 16, 1688–1690. [Google Scholar] [CrossRef]
- Liu, J.; Dong, Y.; Zhang, H. On received intensity for misaligned underwater wireless optical links. In Proceedings of the OCEANS, Shanghai, China, 10–13 April 2016; pp. 1–4. [Google Scholar]
- Zeng, Z.; Fu, S.; Zhang, H.; Dong, Y.; Cheng, J. A survey of underwater optical wireless communications. IEEE Commun. Surv. Tutorials 2016, 19, 204–238. [Google Scholar] [CrossRef]
- Smart, J.H. Underwater optical communications systems part 1: Variability of water optical parameters. In Proceedings of the IEEE Military Communications Conference (MILCOM)), Atlantic City, NJ, USA, 17–20 October 2005; pp. 1140–1146. [Google Scholar]
- Giles, J.W.; Bankman, I.N. Underwater optical communications systems. Part 2: Basic design considerations. In Proceedings of the IEEE Military Communications Conference(MILCOM), Atlantic City, NJ, USA, 17–20 October 2005; pp. 1700–1705. [Google Scholar]
- Liu, W.; Zou, D.; Wang, P.; Xu, Z.; Yang, L. Wavelength dependent channel characterization for underwater optical wireless communications. In Proceedings of the IEEE International Conference on Signal Processing, Communications and Computing (ICSPCC), Guilin, China, 5–8 August 2014; pp. 895–899. [Google Scholar]
- Farr, N.; Chave, A.; Freitag, L.; Preisig, J.; White, S.; Yoerger, D.; Titterton, P. Optical modem technology for seafloor observatories. In Proceedings of the OCEANS, Washington, DC, USA, 17–23 September 2005; pp. 928–934. [Google Scholar]
- de Hulst, V.; Christoffel, H. Multiple Light Scattering: Tables, Formulas and Applications; Academic Press: Cambridge, MA, USA, 1980. [Google Scholar]
- Li, J.; Ma, Y.; Zhou, Q.; Zhou, B.; Wang, H. Monte Carlo study on pulse response of underwater optical channel. Opt. Eng. 2012, 51, 066001. [Google Scholar] [CrossRef]
- Zhang, H.; Dong, Y.; Zhang, X. On stochastic model for underwater wireless optical links. In Proceedings of the International Conference on Communications in China (ICCC), Shanghai, China, 13–15 October 2014; pp. 156–160. [Google Scholar]
- Zhang, H.; Dong, Y. General stochastic channel model and performance evaluation for underwater wireless optical links. IEEE Trans. Wireless Commun. 2015, 15, 1162–1173. [Google Scholar] [CrossRef]
- Cox, W.; Muth, J. Simulating channel losses in an underwater optical communication system. JOSA A 2014, 31, 920–934. [Google Scholar] [CrossRef]
- Tang, S.; Zhang, X.; Dong, Y. On impulse response for underwater wireless optical links. In Proceedings of the OCEANS, Bergen, Norway, 10–14 June 2013; pp. 1–4. [Google Scholar]
- Choudhary, A.; Jagadeesh, V.; Muthuchidambaranathan, P. Pathloss analysis of NLOS underwater wireless optical communication channel. In Proceedings of the International Conference on Electronics and Communication Systems (ICECS), Coimbatore, India, 13–14 February 2014; pp. 1–4. [Google Scholar]
- Tang, S.; Dong, Y.; Zhang, X. On path loss of NLOS underwater wireless optical communication links. In Proceedings of the OCEANS, Bergen, Norway, 10–14 June 2013; pp. 1–3. [Google Scholar]
- Jamali, M.V.; Salehi, J.A. On the BER of multiple-input multiple-output underwater wireless optical communication systems. In Proceedings of the 2015 4th International Workshop on Optical Wireless Communications (IWOW), Istanbul, Turkey, 7–8 September 2015; pp. 26–30. [Google Scholar]
- Khalighi, M.A.; Uysal, M. Survey on free space optical communication: A communication theory perspective. IEEE Commun. Surv. Tutorials 2014, 16, 2231–2258. [Google Scholar] [CrossRef]
- Yi, X.; Li, Z.; Liu, Z. Underwater optical communication performance for laser beam propagation through weak oceanic turbulence. Appl. Opt. 2015, 54, 1273–1278. [Google Scholar] [CrossRef] [PubMed]
- Hanson, F.; Radic, S. High bandwidth underwater optical communication. Appl. Opt. 2008, 47, 277–283. [Google Scholar] [CrossRef] [PubMed]
- Yang, F.; Cheng, J.; Tsiftsis, T.A. Free-space optical communication with nonzero boresight pointing errors. IEEE Trans. Commun. 2014, 62, 713–725. [Google Scholar] [CrossRef]
- Johnson, L.J.; Green, R.J.; Leeson, M.S. Underwater optical wireless communications: Depth dependent variations in attenuation. Appl. Opt. 2013, 52, 7867–7873. [Google Scholar] [CrossRef]
- Cai, Y.; Zhang, L. Propagation of a hollow Gaussian beam through a paraxial misaligned optical system. Opt. Commun. 2006, 265, 607–615. [Google Scholar] [CrossRef]
- Leathers, R.A.; Downes, T.V.; Davis, C.O.; Mobley, C.D. Monte Carlo Radiative Transfer Simulations for Ocean Optics: A Practical Guide; Technical Report; Naval Research Lab Washington Dc Applied Optics Branch: Washington, DC, USA, 2004. [Google Scholar]
- Jacques, S.L. Monte Carlo modeling of light transport in tissue (steady state and time of flight). In Optical-Thermal Response of Laser-Irradiated Tissue; Springer: Berlin, Germany, 2010; pp. 109–144. [Google Scholar]
- Cox, W.C., Jr. Simulation, Modeling, and Design of Underwater Optical Communication Systems; North Carolina State University: Raleigh, NC, USA, 2012. [Google Scholar]
- Mobley, C.D. Light and Water: Radiative Transfer in Natural Waters; Academic Press: Cambridge, MA, USA, 1994. [Google Scholar]
- Hemmati, H. Deep Space Optical Communications; John Wiley & Sons: Hoboken, NJ, USA, 2006; Volume 11. [Google Scholar]
- Cochenour, B.; Mullen, L.; Muth, J. Temporal response of the underwater optical channel for high-bandwidth wireless laser communications. IEEE J. Ocean. Eng. 2013, 38, 730–742. [Google Scholar] [CrossRef]
- Wells, W.H. Theory of small angle scattering. In Optics of the Sea; NATO: Brussels, Belgium, 1973; Volume 61. [Google Scholar]




| UWOC Channel | Merits | Shortcomings | References |
|---|---|---|---|
| Beer Lambert’s law | Simple to implement | Less accurate | [34,35,36] |
| Chlorophyl Monte-Carlo | Accurate in simulation environment | Less efficient for errors | [37,38] |
| Analytical Radiative Transfer Equation (RTE) | Provide accurate results | Offer difficulty in solving | [39,40] |
| Analytical stochastic | Efficient for performance analysis | Difficult derivation with limited assumptions | [41,42] |
| Numerical RTE | Easy to program, providing accurate results | Efficiency is low in case of error | [43,44] |
| NLOS technique | NLOS technique for UWOC | Less accurate in case of surface reflection | [45,46] |
| Turbulence model | Turbulence technique for errors in UWOC | Less accurate in case of turbulence | [47,48] |
| Parameters | Value |
|---|---|
| Number of Photons | |
| s | 0.924 |
| wavelength | 520 nm |
| Tx beam width | 1 mm |
| Rx aperture diameter | 0.18 m |
| Rx NEP | |
| Rx aperture FOV | |
| Link span (clean water) | 0–25 m |
| Link span (turbid water) | 0–10 m |
| Albedo (clean water) | 0.247 |
| Albedo (turbid water) | 0.8329 |
| , , (clean water) | 0.114, 0.037, 0.151 |
| , , (turbid water) | 0.366, 1.824, 2.19 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khalil, R.A.; Babar, M.I.; Saeed, N.; Jan, T.; Cho, H.-S. Effect of Link Misalignment in the Optical-Internet of Underwater Things. Electronics 2020, 9, 646. https://doi.org/10.3390/electronics9040646
Khalil RA, Babar MI, Saeed N, Jan T, Cho H-S. Effect of Link Misalignment in the Optical-Internet of Underwater Things. Electronics. 2020; 9(4):646. https://doi.org/10.3390/electronics9040646
Chicago/Turabian StyleKhalil, Ruhul Amin, Mohammad Inayatullah Babar, Nasir Saeed, Tariqullah Jan, and Ho-Shin Cho. 2020. "Effect of Link Misalignment in the Optical-Internet of Underwater Things" Electronics 9, no. 4: 646. https://doi.org/10.3390/electronics9040646
APA StyleKhalil, R. A., Babar, M. I., Saeed, N., Jan, T., & Cho, H.-S. (2020). Effect of Link Misalignment in the Optical-Internet of Underwater Things. Electronics, 9(4), 646. https://doi.org/10.3390/electronics9040646








