Wideband Band-Pass Filter Design Using Coupled Line Cross-Shaped Resonator
Abstract
1. Introduction
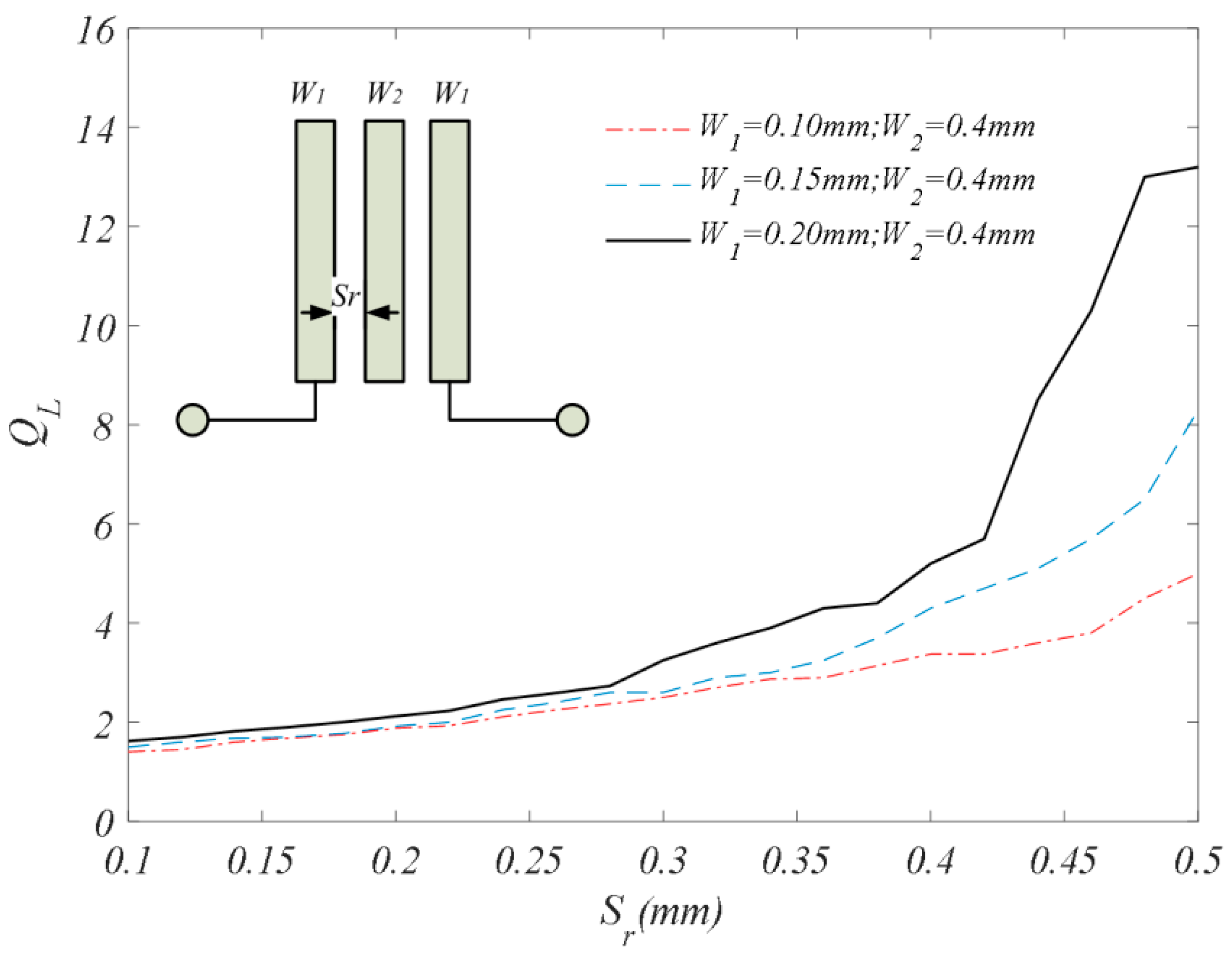
2. Filter Analysis and Design
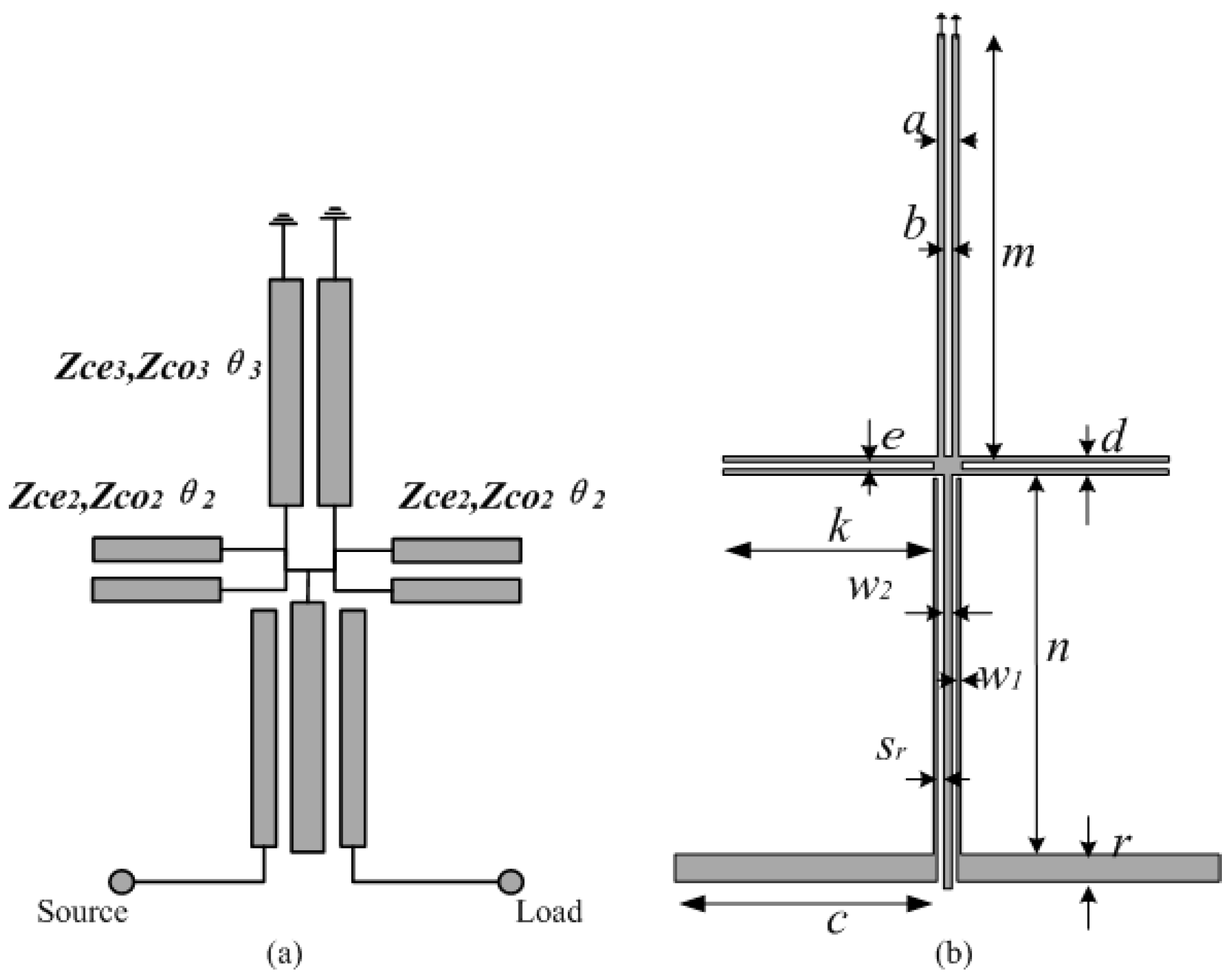
2.1. Bandpass Filter’s Equivalent Circuit Analysis
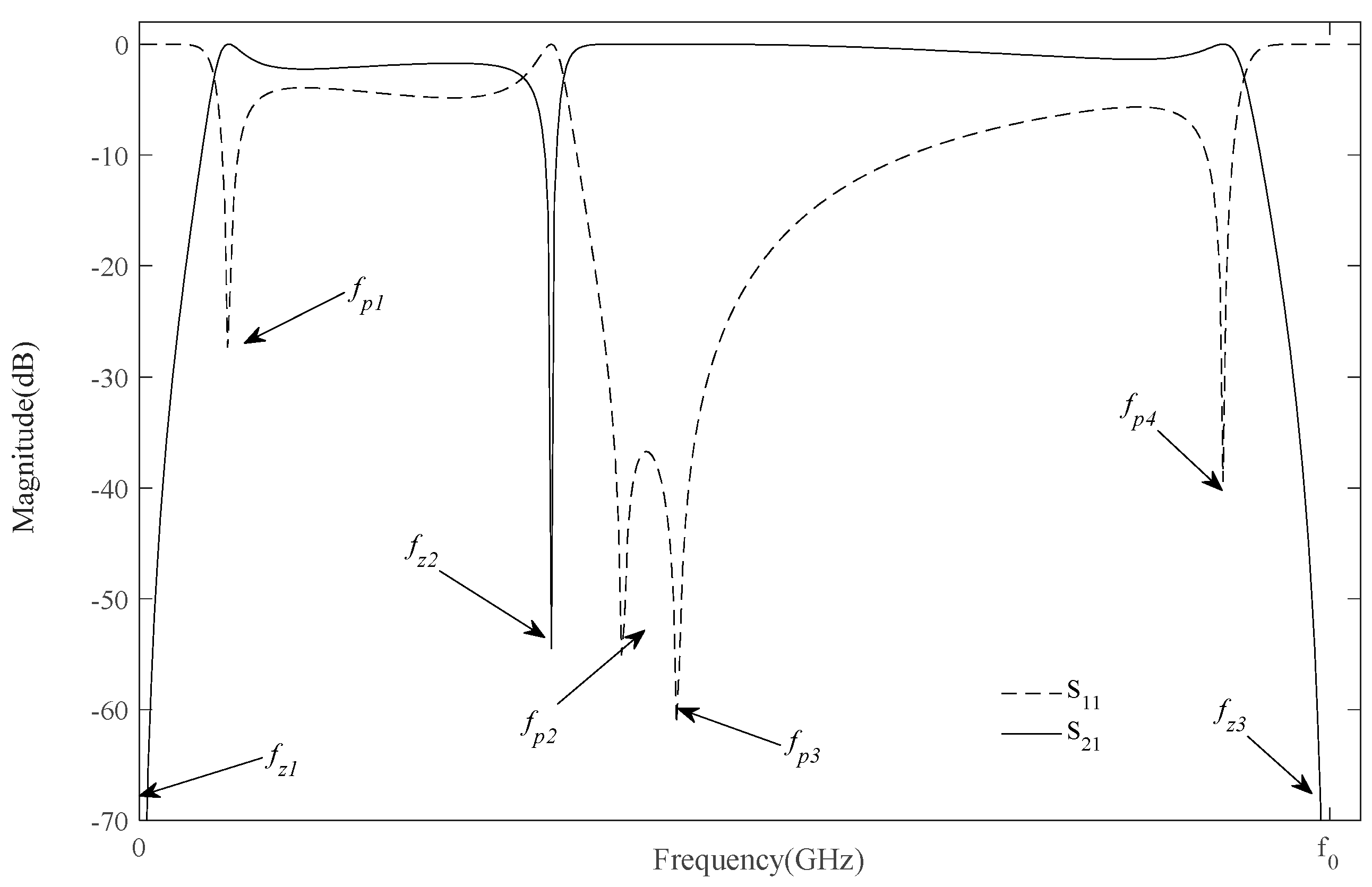
2.2. Transmission Zero Analysis
2.3. Transmission Poles Analysis
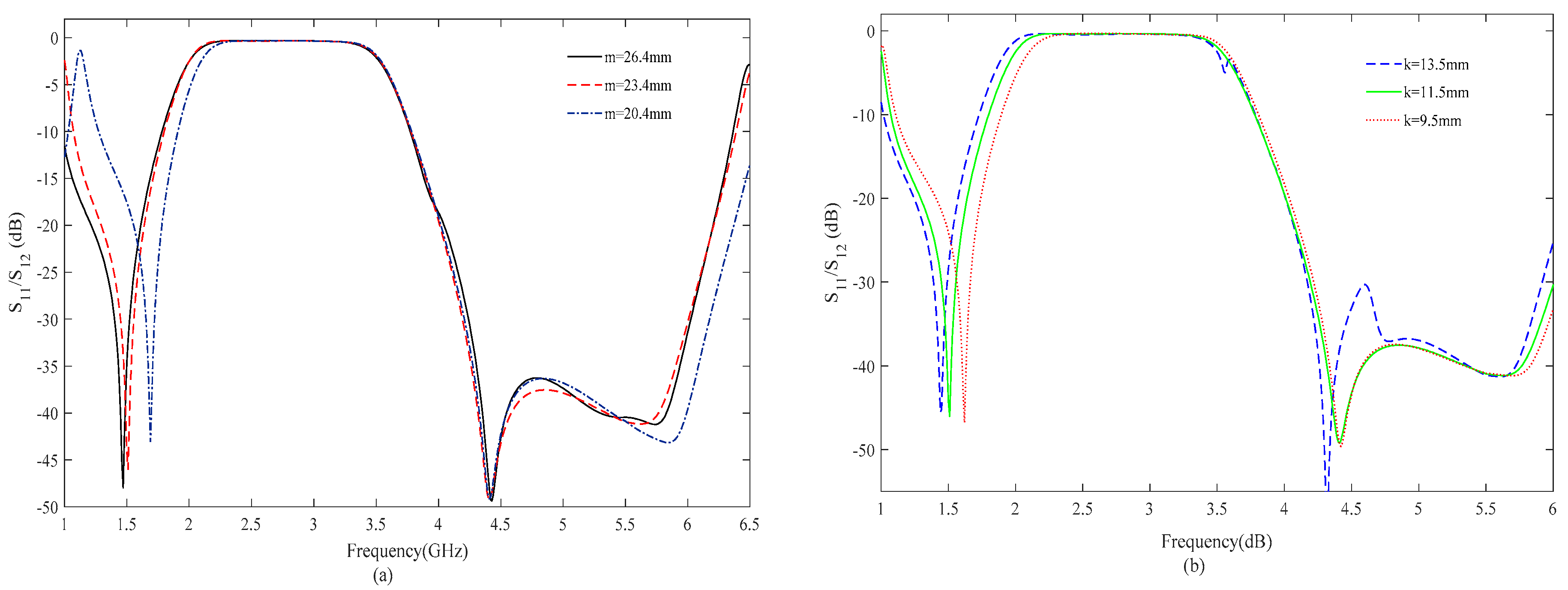
3. Filter’s Results and Discussion
3.1. Filter’s Results
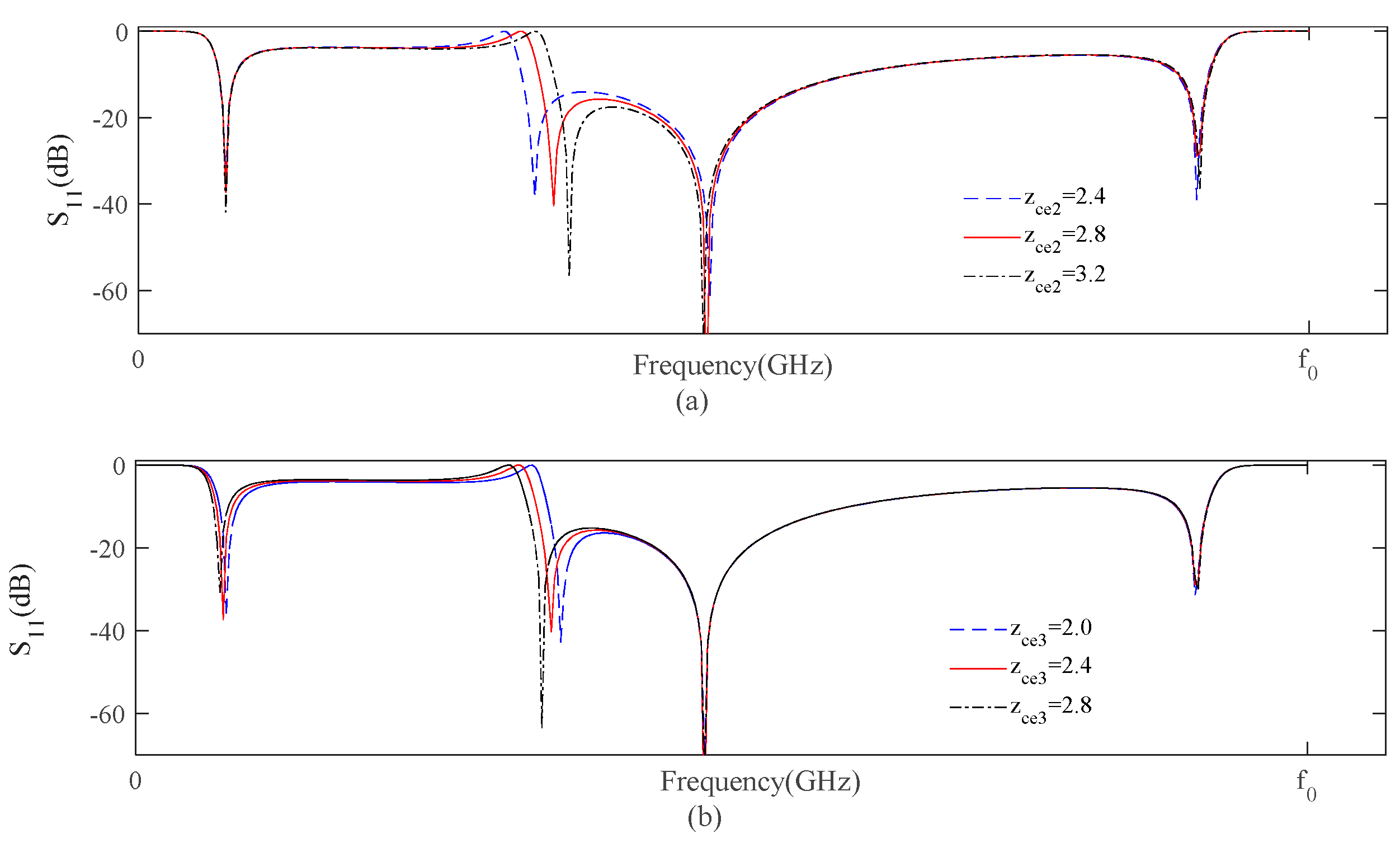
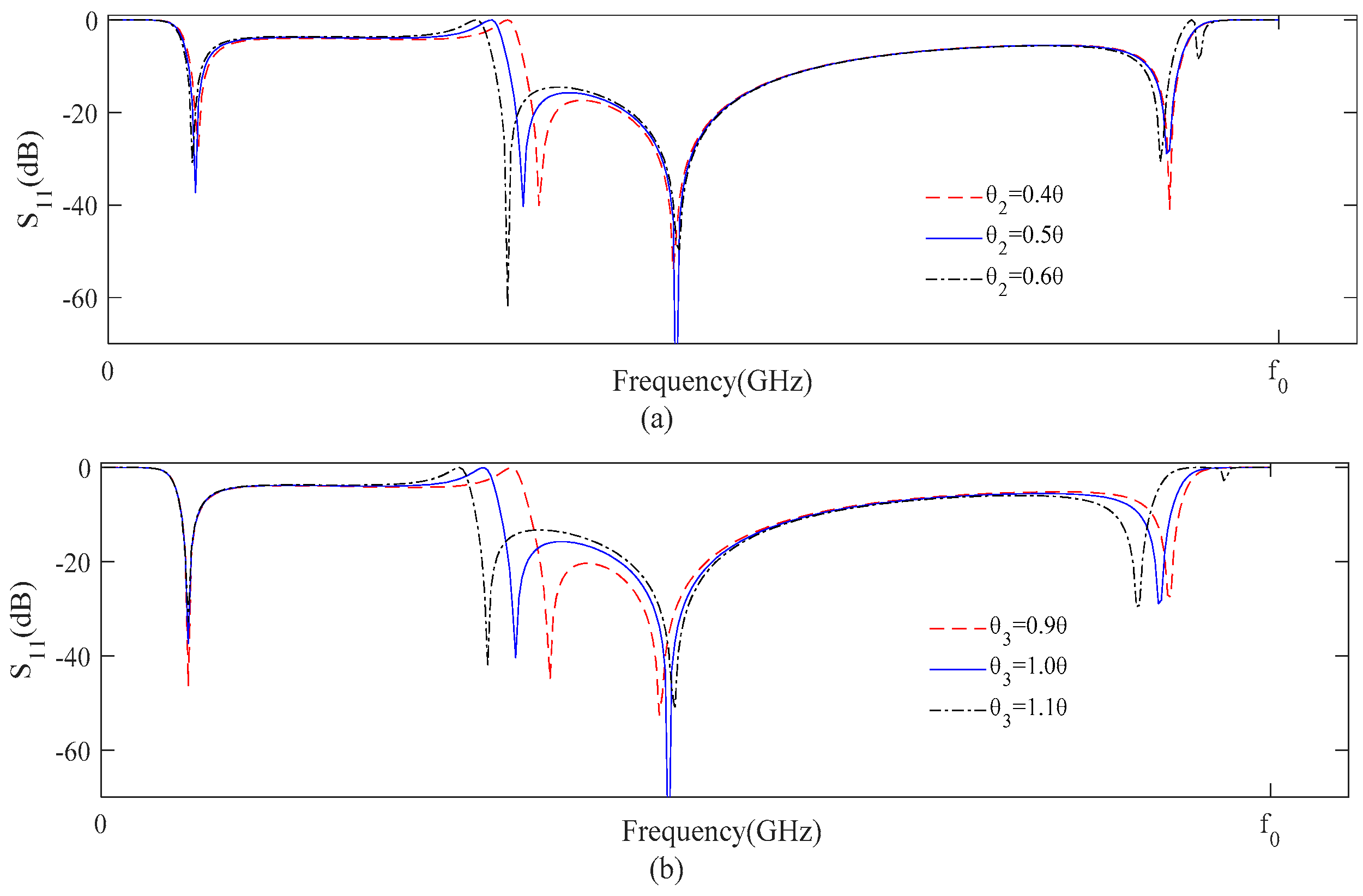
3.2. Filter Performance Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Wang, H.; Yang, G.; Kang, W.; Miao, C.; Wu, W. Application of Cross-Shaped Resonator to the Ultra Wideband Bandpass Filter Design. IEEE Microw. Wirel. Components Lett. 2011, 21, 667–669. [Google Scholar] [CrossRef]
- Da Xu, K.; Zhang, F.; Liu, Y.; Nie, W. High Selectivity Seventh-order Wideband Bandpass filter Using Coupled Lines and Open-sorted Stubs. Electron. Lett. 2018, 54, 223–225. [Google Scholar]
- Xu, K.-D.; Li, D.; Liu, Y. High-Selectivity Wideband Bandpass Filter Using Simple Coupled Lines With Multiple Transmission Poles and Zeros. IEEE Microw. Wirel. Components Lett. 2019, 29, 107–109. [Google Scholar] [CrossRef]
- Li, L.; Li, Z.-F. Side-Coupled Shorted Microstrip Line for Compact Quasi-Elliptic Wideband Bandpass Filter Design. IEEE Microw. Wirel. Components Lett. 2010, 20, 322–324. [Google Scholar] [CrossRef]
- Ahmadi, A.; Makki, S.V.; Lalbakhsh, A.; Majidifar, S. A Novel Dual-Mode Wideband Bandpass Filter. ACES J. 2014, 29, 735–742. [Google Scholar]
- Lalbakhsh, A.; Jamshidi, M.B.; Siahkamari, H.; Ghaderi, A.; Golestanifar, A.; Linhart, R.; Talla, J.; Simorangkir, R.B.; Mandal, K. A compact lowpass filter for satellite communication systems based on transfer function analysis. AEU Int. J. Electron. Commun. 2020, 124, 153318. [Google Scholar] [CrossRef]
- Zhang, W.; Feng, F.; Zhang, J.; Zhao, Z.; Ma, J.; Zhang, Q. Parallel Decomposition Approach to Wide-Range Parametric Modeling With Applications to Microwave Filters. IEEE Trans. Microw. Theory Tech. 2020, 68, 5288–5306. [Google Scholar] [CrossRef]
- Sheikhi, A.; Alipour, A.; Mir, A. Design and Fabrication of an Ultra-Wide Stopband Compact Bandpass Filter. IEEE Trans. Circuits Syst. II Express Briefs 2019, 67, 265–269. [Google Scholar] [CrossRef]
- Rao, Y.; Qian, H.J.; Yang, B.; Gomez-Garcia, R.; Luo, X. Dual-Band Bandpass Filter and Filtering Power Divider With Ultra-Wide Upper Stopband Using Hybrid Microstrip/DGS Dual-Resonance Cells. IEEE Access 2020, 8, 23624–23637. [Google Scholar] [CrossRef]
- Guo, Z.-C.; Zhu, L.; Wong, S.-W. A Quantitative Approach for Direct Synthesis of Bandpass Filters Composed of Transversal Resonators. IEEE Trans. Circuits Syst. II Express Briefs 2018, 66, 577–581. [Google Scholar] [CrossRef]
- Bi, X.-K.; Cheng, T.; Cheong, P.; Ho, S.-K.; Tam, K.-W. Design of Dual-Band Bandpass Filters with Fixed and Reconfigurable Bandwidths Based on Terminated Cross-Shaped Resonators. IEEE Trans. Circuits Syst. II: Express Briefs 2018, 66, 317–321. [Google Scholar] [CrossRef]
- Bi, X.-K.; Teng, C.; Cheong, P.; Ho, S.-K.; Tam, K.-W. Wideband bandpass filters with reconfigurable bandwidth and fixed notch bands based on terminated cross-shaped resonator. IET Microw. Antennas Propag. 2019, 13, 796–803. [Google Scholar] [CrossRef]
- Feng, W.; Che, W. Wideband balanced bandpass filter based on three-line coupled structure. Electron. Lett. 2012, 48, 1006–1008. [Google Scholar] [CrossRef]
- Lalbakhsh, A.; Alizadeh, S.M.; Ghaderi, A.; Golestanifar, A.; Mohamadzade, B.; Jamshidi, M.B.; Mandal, K.; Mohyuddin, W. A Design of a Dual-Band Bandpass Filter Based on Modal Analysis for Modern Communication Systems. Electronics 2020, 9, 1770. [Google Scholar] [CrossRef]
- Moradi, B.; Fernández-García, R.; Gil, I. Meander Microwave Bandpass filter on a Flexible Textile Substrate. Electronics 2019, 8, 11. [Google Scholar] [CrossRef]
- Xu, J.; Miao, C.; Wu, W. A compact and high isolation dual-mode dual-band bandpass filter with tunable transmission zeros. J. Electromagn. Waves Appl. 2012, 26, 2390–2397. [Google Scholar] [CrossRef]
- Wang, H.; Gao, L.M.; Tam, K.W.; Kang, W.; Wu, W. A Wideband Differential Bandpass Filter With Multiple Differential- and Common-Mode Transmission Zeros Using Cross-Shaped Resonator. IEEE Microw. Wirel. Compon. Lett. 2014, 24, 854–856. [Google Scholar] [CrossRef]





| Ref. | Center Frequency (GHz) | Lower Stopband (dB) | Upper Stopband (dB) | RL (dB) | IL (dB) | Size |
|---|---|---|---|---|---|---|
| [1] | 6.65 | <−20 | <−20 | 20 | 0.35 | 0.5 × 0.79 |
| [2] | 1.93 | <−40 | <−35 | 20 | 0.4 | 0.56 × 0.23 |
| [3] | 2.05 | <−32 | <−20 | 20 | 0.6 | 0.48 × 0.24 |
| [5] | 8.28 | <−20 | <−25 | 15 | 1.9 | 1.12 × 0.45 |
| [10] | 3 | <−10 | <−18 | 16 | 1.28 | 0.18 × 0.175 |
| [13] | 6.71 | <−30 | <−25 | 15 | 1.45 | 0.5 × 0.04 |
| [14] | 7.6 | <−20 | <−10 | 16 | 0.6 | 0.64 × 0.31 |
| This work | 2.95 | <−15 | <−35 | 20 | 0.4 | 0.12 × 0.23 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
La, D.-S.; Guan, X.; Chen, S.-M.; Li, Y.-Y.; Guo, J.-W. Wideband Band-Pass Filter Design Using Coupled Line Cross-Shaped Resonator. Electronics 2020, 9, 2173. https://doi.org/10.3390/electronics9122173
La D-S, Guan X, Chen S-M, Li Y-Y, Guo J-W. Wideband Band-Pass Filter Design Using Coupled Line Cross-Shaped Resonator. Electronics. 2020; 9(12):2173. https://doi.org/10.3390/electronics9122173
Chicago/Turabian StyleLa, Dong-Sheng, Xin Guan, Shuai-Ming Chen, Yu-Ying Li, and Jing-Wei Guo. 2020. "Wideband Band-Pass Filter Design Using Coupled Line Cross-Shaped Resonator" Electronics 9, no. 12: 2173. https://doi.org/10.3390/electronics9122173
APA StyleLa, D.-S., Guan, X., Chen, S.-M., Li, Y.-Y., & Guo, J.-W. (2020). Wideband Band-Pass Filter Design Using Coupled Line Cross-Shaped Resonator. Electronics, 9(12), 2173. https://doi.org/10.3390/electronics9122173





