High-Order Differential Feedback Control for Quadrotor UAV: Theory and Experimentation
Abstract
:1. Introduction
- (1)
- The HOD accuracy in estimating the derivatives and model function is higher than the ESO accuracy because of the higher-order filtering property and derivative form, which will be explained in the main text.
- (2)
- The ADRC uses the ESO to estimate the unknown model. The HODFC designs a control filter for estimating the unknown model. Because the system satisfies , once is estimated using the HOD, the control quantity contains the unknown model information . Because the control input is unresolved, it cannot be used, so we design a control filter to obtain to replace . Thus, the unknown model can be estimated indirectly. This method is different from the ADRC; still, it can overcome the problem of unknown function and disturbance.
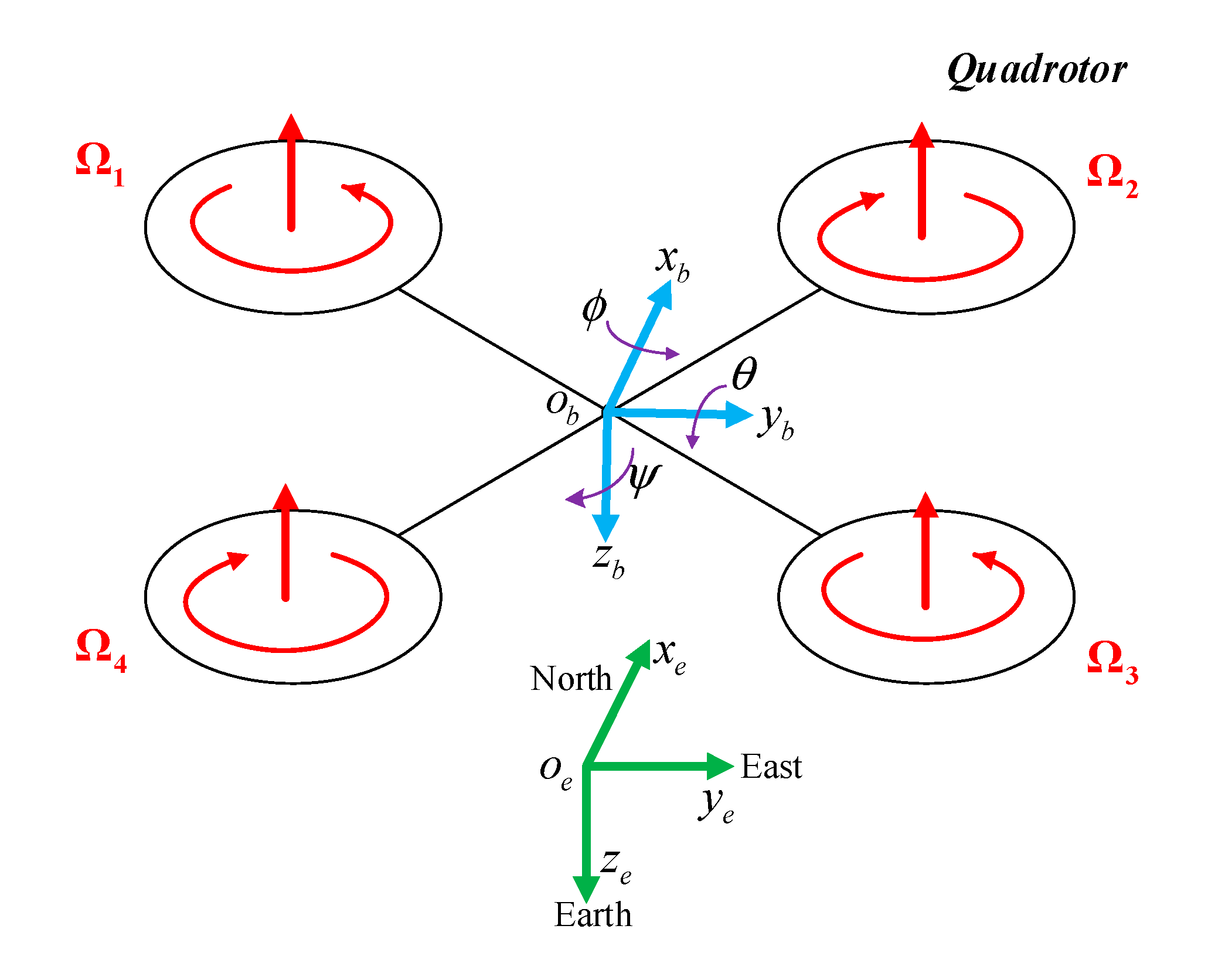
2. Quadrotor Model
3. HODFC Scheme for QUAV
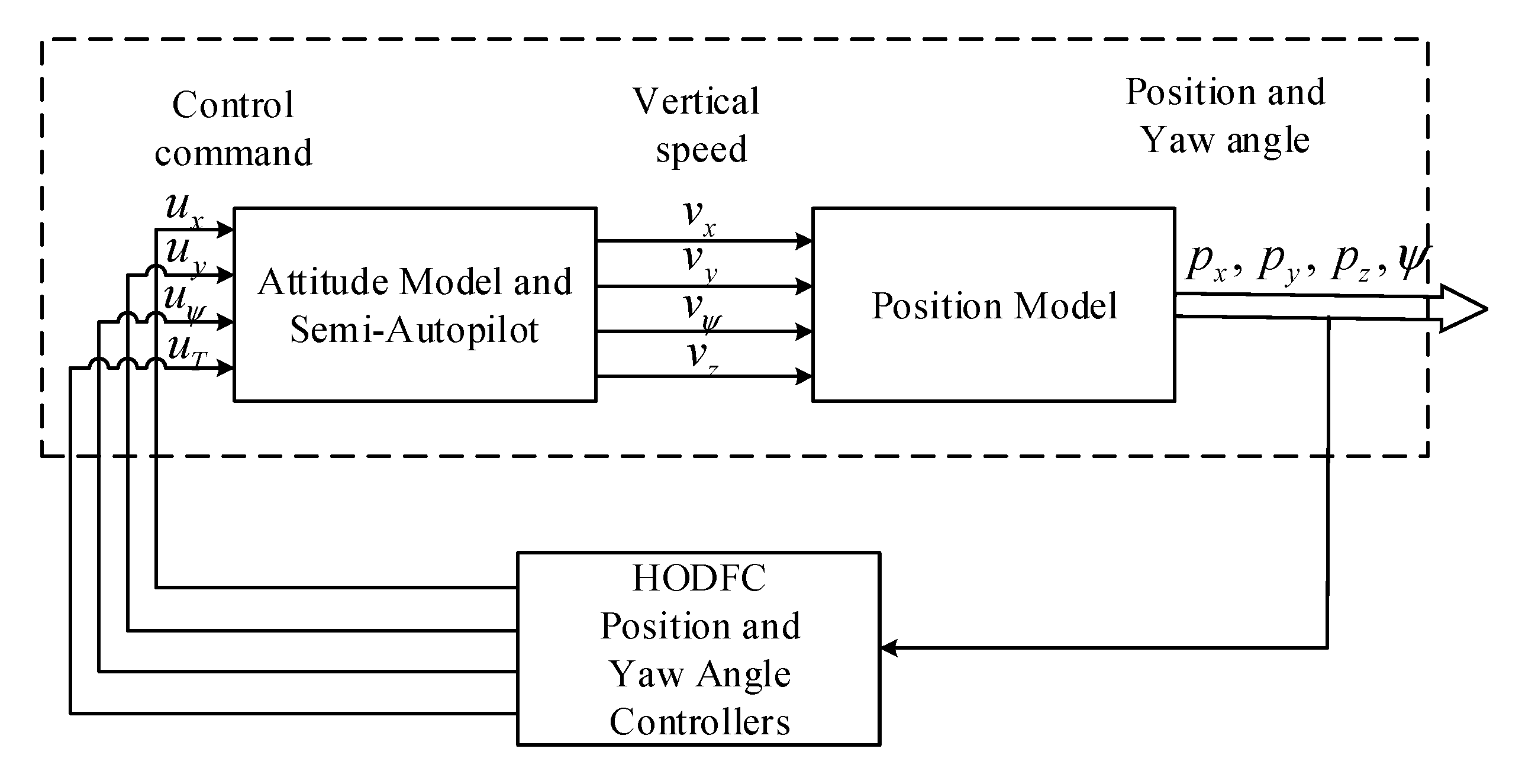
3.1. Quadrotor Control Structure with Semi-Autopilot
3.2. HOD Design
- (1)
- It has a filtering process because the number of integrators is more than the order of the extracted derivatives.
- (2)
- It has the derivative form, thereby having higher estimation accuracy.
- (3)
- It is simple, with only one parameter to adjust.
- (4)
- It is stable.
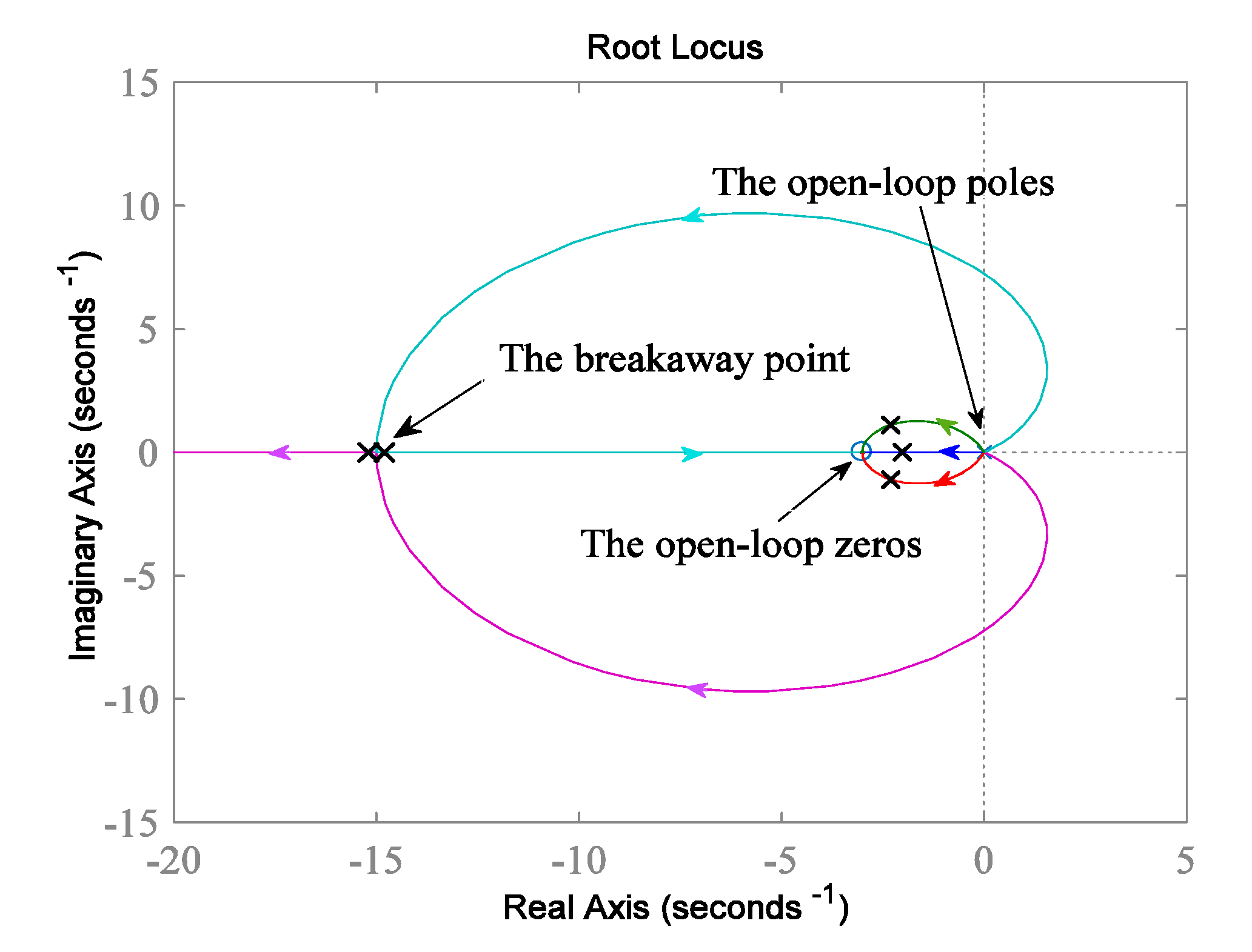
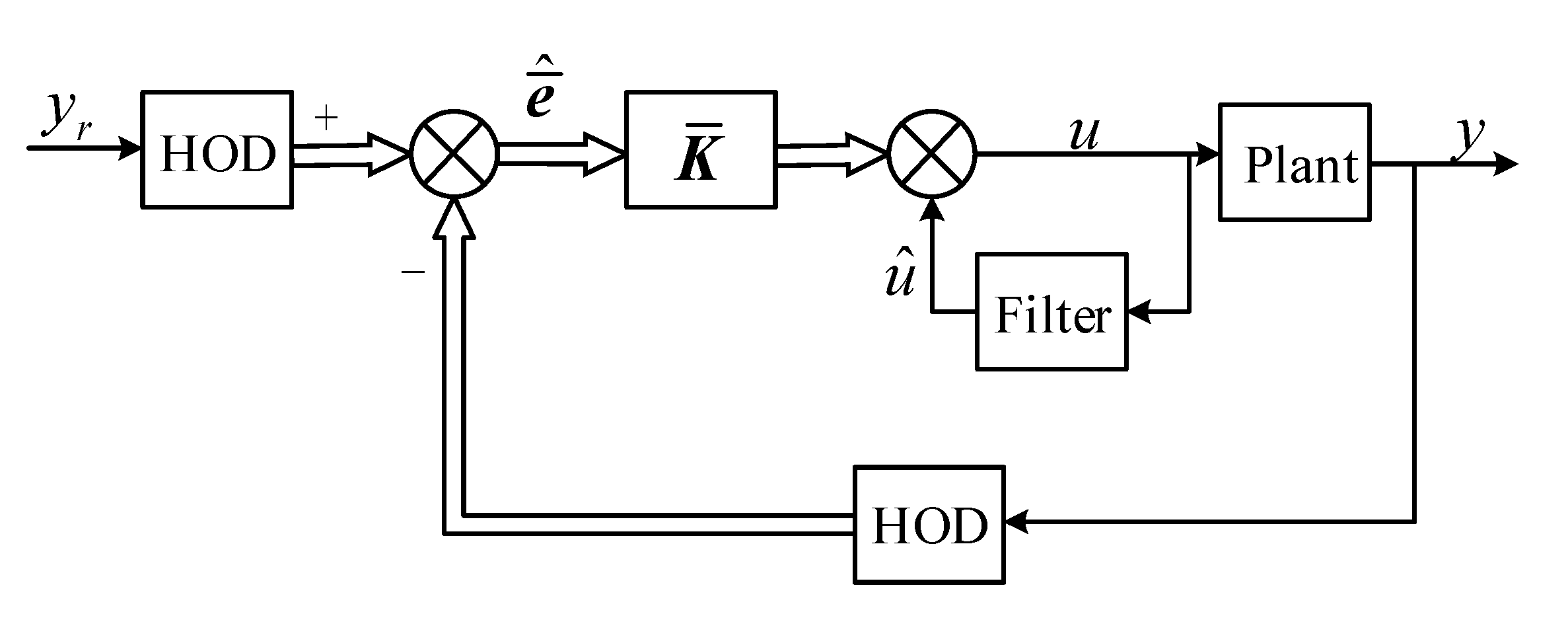
3.3. HODFC Design
- (1)
- It does not rely on the system model and disturbance.
- (2)
- It makes the closed-loop system stable and behave in the desired assigned poles.
- (3)
- The control filter is designed to compensate for the unknown nonlinear model and disturbances.
4. Experimental Results
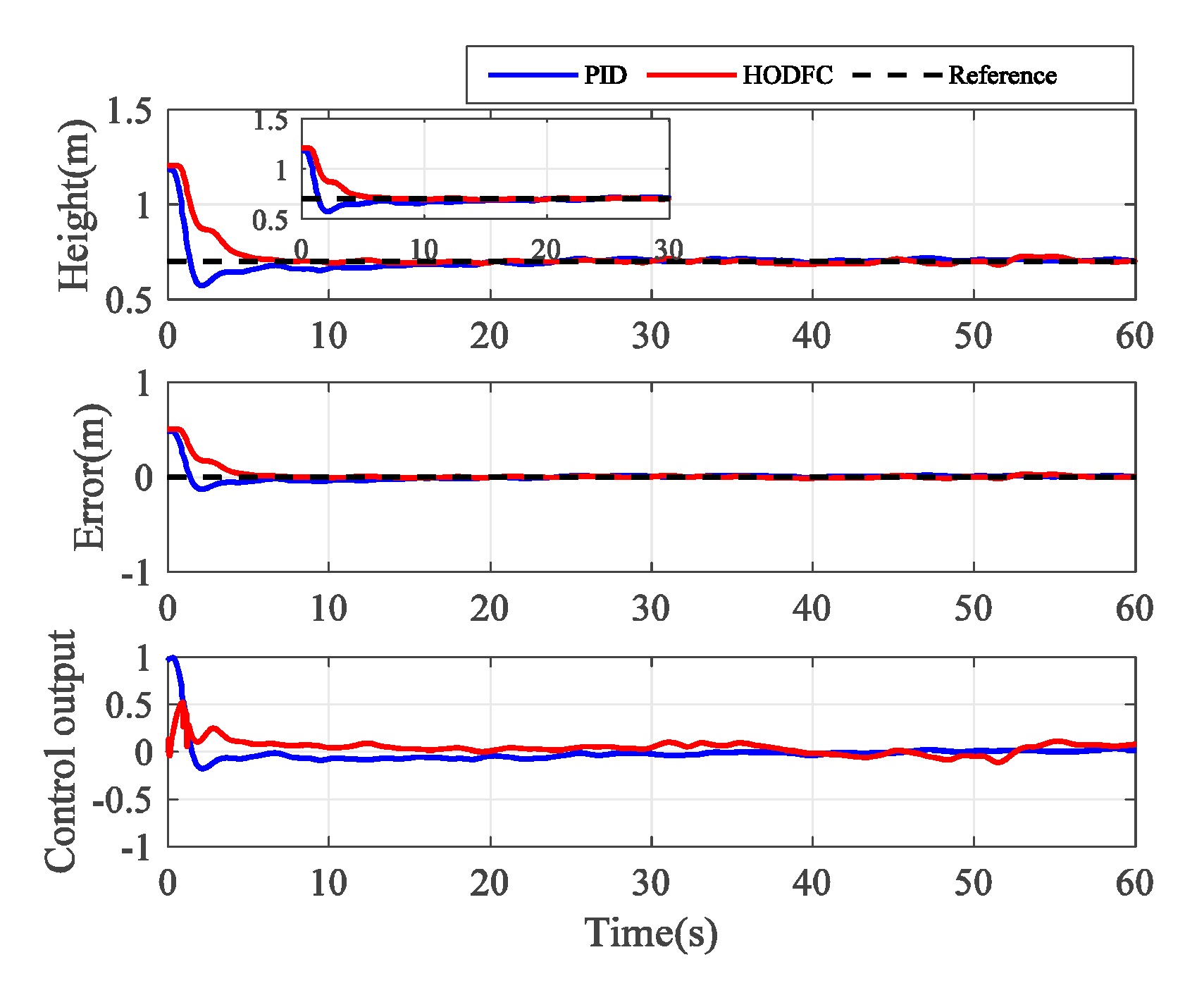
4.1. Static Trajectory Tracking
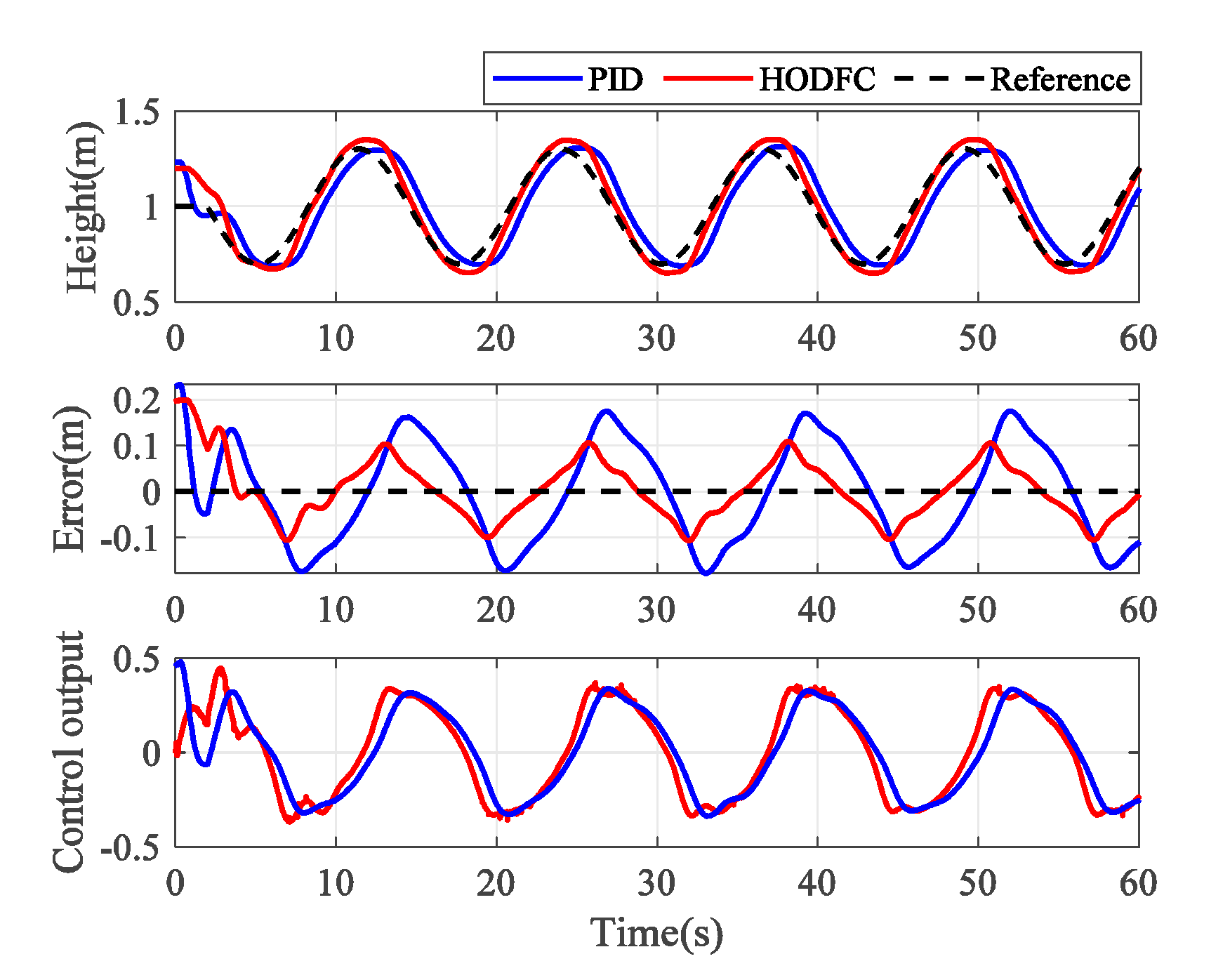
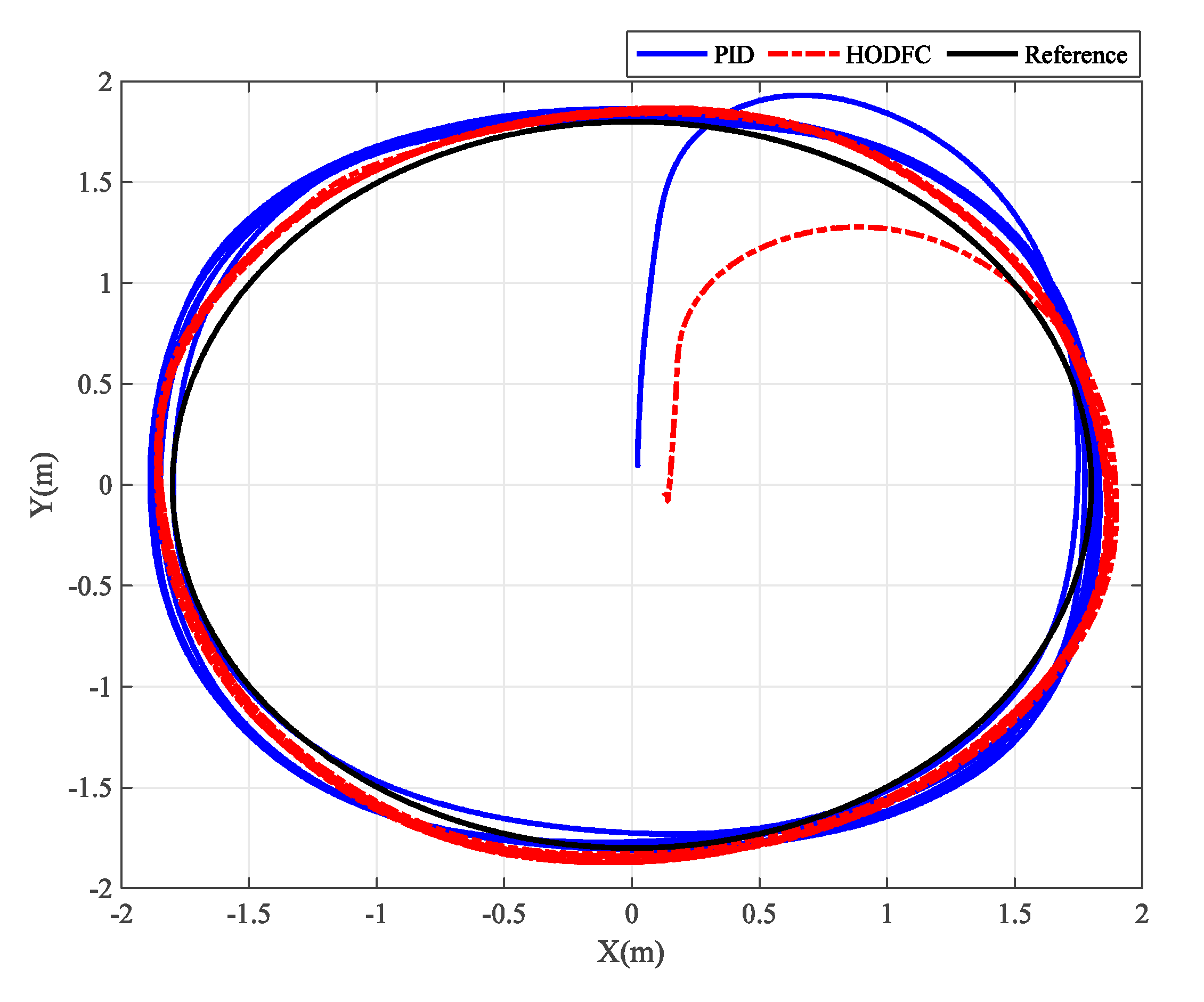
4.2. Dynamic Trajectory Tracking
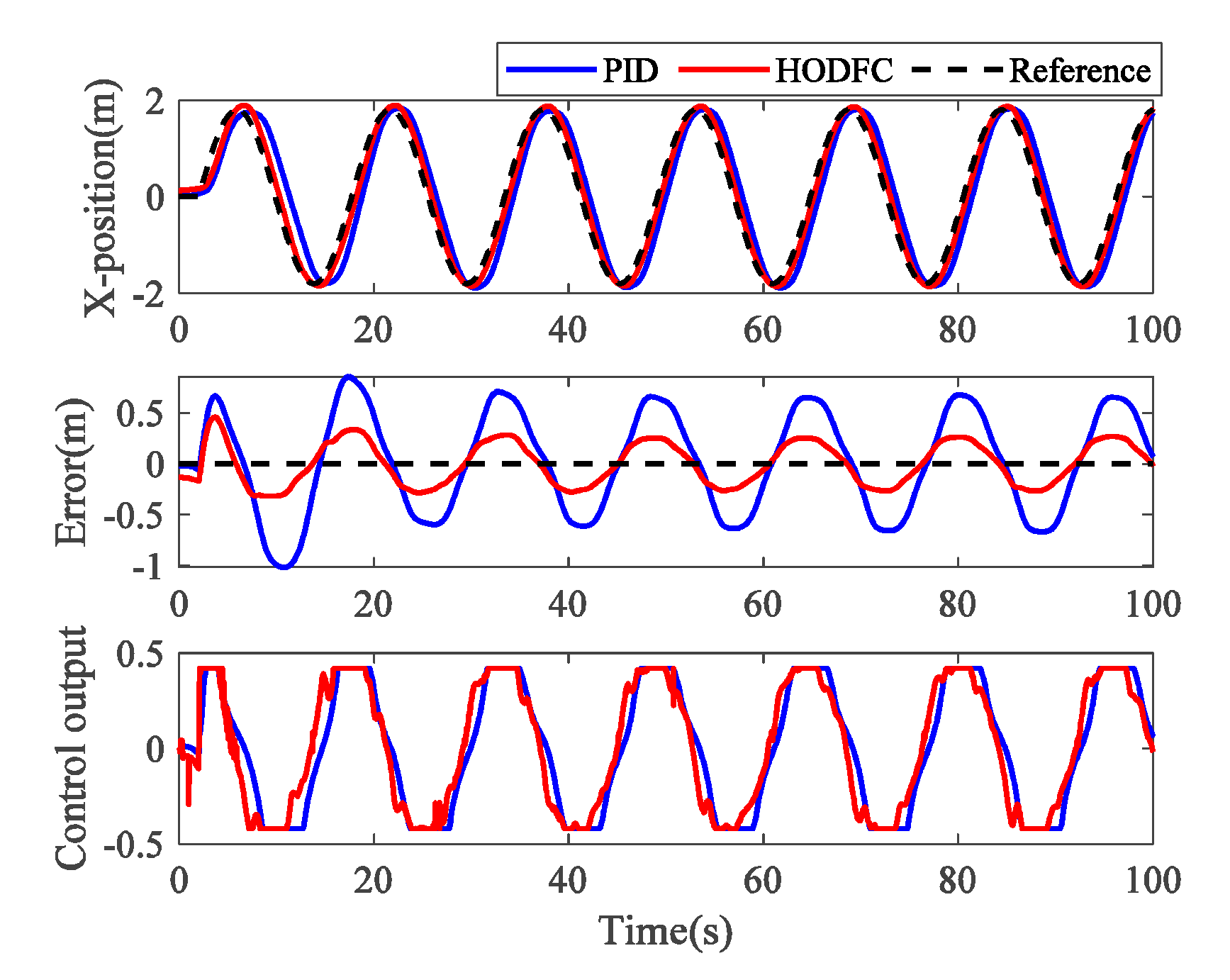
4.3. Experiments with Disturbance
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Qi, G.; Huang, D. Modeling and dynamical analysis of a small-scale unmanned helicopter. Nonlinear Dyn. 2019, 98, 2131–2145. [Google Scholar] [CrossRef]
- Hood, S.; Benson, K.; Hamod, P.; Madison, D.; O’Kane, J.M.; Rekleitis, I. Bird’s eye view cooperative exploration by UGV and UAV. In Proceedings of the International Conference on Unmanned Aircraft Systems, Miami, FL, USA, 13–16 June 2017; pp. 247–255. [Google Scholar]
- Yuan, C.; Liu, Z.; Zhang, Y. Aerial images-based forest fire detection for firefighting using optical remote sensing techniques and unmanned aerial vehicles. J. Intell. Robot. Syst. 2017, 88, 1–20. [Google Scholar] [CrossRef]
- Pierson, A.; Ataei, A.; Paschalidis, I.; Schwager, M. Cooperative multi-quadrotor pursuit of an evader in an environment with no-fly zones. In Proceedings of the IEEE International Conference on Robotics and Automation, Stockholm, Sweden, 16–21 May 2016; pp. 320–326. [Google Scholar]
- Bi, H.; Qi, G.; Hu, J. Modeling and analysis of chaos and bifurcations for the attitude system of a quadrotor unmanned aerial vehicle. Complexity 2019, 2019, 6313925-1-16. [Google Scholar] [CrossRef]
- Li, Y.; Song, S. A survey of control algorithms for quadrotor unmanned helicopter. In Proceedings of the IEEE International Conference on Advanced Computational Intelligence, Nanjing, China, 18–20 October 2012; pp. 365–369. [Google Scholar]
- Mu, B.; Zhang, K.; Shi, Y. Integral sliding mode flight controller design for a quadrotor and the application in a heterogeneous multi-agent system. IEEE Trans. Ind. Electron. 2017, 64, 9389–9398. [Google Scholar] [CrossRef]
- Tian, B.; Liu, L.; Lu, H.; Zuo, Z.; Zong, Q.; Zhang, Y. Multivariable finite time attitude control for quadrotor UAV: Theory and experimentation. IEEE Trans. Ind. Electron. 2017, 65, 2567–2577. [Google Scholar] [CrossRef]
- Liu, H.; Wang, X.; Zhong, Y. Quaternion-based robust attitude control for uncertain robotic quadrotors. IEEE Trans. Ind. Inform. 2015, 11, 406–415. [Google Scholar] [CrossRef]
- Salih, A.; Moghavvemi, M.; Mohamed, H.; Gaeid, K.S. Flight PID controller design for a UAV quadrotor. Sci. Res. Essays 2010, 5, 3660–3667. [Google Scholar]
- Vázquez, S.; Valenzuela, J. A new nonlinear PI/PID controller for quadrotor posture regulation. In Proceedings of the IEEE Electronics, Robotics and Automotive Mechanics Conference, Morelos, Mexico, 28 September–1 October 2010; pp. 642–647. [Google Scholar]
- Gao, Y.; Chen, D.; Li, R. Research on control algorithm of microquadrotor aircraft. Comput. Mod. 2011, 194, 4–7. [Google Scholar]
- Sangyam, T.; Laohapiengsak, P.; Chongcharoen, W.; Nilkhamhang, I. Path tracking of UAV using self-tuning PID controller based on fuzzy logic. In Proceedings of the Society of Instrument and Control Engineers Conference, Taipei, Taiwan, 18–21 August 2010; pp. 1265–1269. [Google Scholar]
- Efe, M. Neural network assisted computationally simple PIλDμ control of a quadrotor UAV. IEEE Trans. Ind. Inform. 2011, 7, 354–361. [Google Scholar] [CrossRef]
- Han, J. From PID to active disturbance rejection control. IEEE Trans. Ind. Electron. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Gao, Z. Scaling and bandwidth-parameterization based controller tuning. In Proceedings of the American Control Conference, Minneapolis, MN, USA, 14–16 June 2006; pp. 4989–4996. [Google Scholar]
- Nie, Z.; Liu, R. Inverted pendulum angle control design and experimental studies under complex conditions. Inf. Control 2016, 45, 506–512. [Google Scholar]
- Sun, M.; Wang, Z.; Wang, Y.; Chen, Z. On low-velocity compensation of brushless dc servo in the absence of friction model. IEEE Trans. Ind. Electron. 2013, 60, 3897–3905. [Google Scholar] [CrossRef]
- Chen, Z.; Cheng, Y.; Sun, M.; Sun, Q. Surveys on theory and engineering applications for linear active disturbance rejection control. Inf. Control 2017, 46, 257–266. [Google Scholar]
- Zhao, Z.; Guo, B. On convergence of nonlinear active disturbance rejection control for SISO nonlinear systems. J. Dyn. Control Syst. 2016, 22, 385–412. [Google Scholar] [CrossRef]
- Yang, H.; Cheng, L.; Xia, Y.; Yuan, Y. Active disturbance rejection attitude control for a dual closed-loop quadrotor under gust wind. IEEE Trans. Control Syst. Technol. 2018, 26, 1400–1405. [Google Scholar] [CrossRef]
- Li, X.; Chen, Y. Reduced-order active disturbance rejection control for quadrotor aircraft. Electron. Opt. Control 2019, 26, 43–48, 72. [Google Scholar]
- Qi, G.; Chen, Z.; Yuan, Z. Model-free control of affine chaotic systems. Phys. Lett. A 2005, 344, 189–202. [Google Scholar] [CrossRef]
- Qi, G.; Chen, Z.; Yuan, Z. Adaptive high order differential feedback control for affine nonlinear system. Chaos Solitons Fractals 2008, 37, 308–315. [Google Scholar] [CrossRef]
- Xue, W.; Xue, Y.; Qi, G.; Gao, J.; Zheng, S. Study of high order differential feedback control of DC-link voltage in active power filter. In Proceedings of the IEEE International Conference on Automation and Logistics, Jinan, China, 18–21 August 2007; pp. 1063–1066. [Google Scholar]
- Du, S.; Wyk, B.; Qi, G.; Tu, C. Chaotic system synchronization with an unknown master model using a hybrid HOD active control approach. Chaos Solitons Fractals 2009, 42, 1900–1913. [Google Scholar] [CrossRef]
- Nyabundi, S.; Qi, G.; Hamam, Y.; Munda, J. DC motor control via high order differential feedback control. In Proceedings of the AFRICON, Nairobi, Kenya, 23–25 September 2009; pp. 1–5. [Google Scholar]
- Shi, X.; Dai, Y.; Liu, Z.; Qi, G. High order differentialfeedback controller and its application in servo control system of NC machine tools. In Proceedings of the IEEE International Conference on System Science, Yichang, China, 12–14 November 2010; pp. 241–244. [Google Scholar]
- Faradja, P.; Qi, G. Robustness based comparison between a sliding mode controller and a model free controller with the approach of synchronization of nonlinear systems. In Proceedings of the International Conference on Control, Automation and Systems, Busan, Korea, 13–16 October 2015; pp. 36–40. [Google Scholar]
- Agee, J.; Bingul, Z.; Kizir, S. Higher-order differential feedback control of a flexible-joint manipulator. J. Vib. Control 2015, 21, 1976–1986. [Google Scholar] [CrossRef]
- Agee, J.; Bingul, Z.; Kizir, S. Trajectory and vibration control of a single-link flexible joint manipulator using a distributed higher order differential feedback controller. J. Dyn. Syst. Meas. Control 2017, 139, 081006. [Google Scholar] [CrossRef]
- Ayas, M.; Sahin, E.; Altas, I. High order differential feedback controller design and implementation for a stewart platform. J. Vib. Control 2019, 26, 976–988. [Google Scholar] [CrossRef]
- Ayas, M. Design of an optimized fractional high-order differential feedback controller for an AVR system. Electr. Eng. 2019, 101, 1221–1233. [Google Scholar] [CrossRef]
- Yi, K.; Gu, F.; Yang, L.; He, Y.; Han, J. Sliding model control for a quadrotor slung load system. In Proceedings of the Chinese Control Conference, Dalian, China, 26–28 July 2017; pp. 3694–3703. [Google Scholar]
- Guo, Y.; Jiang, B.; Zhang, Y. A novel robust attitude control for quadrotor aircraft subject to actuator faults and wind gusts. IEEE/CAA J. Autom. Sin. 2018, 5, 292–300. [Google Scholar] [CrossRef]
- Zhao, B.; Xian, B.; Zhang, Y.; Zhang, X. Nonlinear robust adaptive tracking control of a quadrotor UAV via immersion and invariance methodology. IEEE Trans. Ind. Electron. 2015, 62, 2891–2902. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, Z.; Zhang, X.; Sun, Q.; Sun, M. A novel control scheme for quadrotor UAV based upon active disturbance rejection control. Aerosp. Sci. Technol. 2018, 79, 601–609. [Google Scholar] [CrossRef]
- Qi, G.; Chen, Z.; Xue, W.; Yuan, Z. A new differential state estimator and controller for nonlinear stochastic system. Chin. J. Electron. 2004, 32, 693–696. [Google Scholar]










| Parameter | Yaw | Height | Position | Position |
|---|---|---|---|---|
| 5 | 7 | 5 | 5 | |
| 30 | 15 | 25 | 25 |
| Parameter | Yaw | Height | Position | Position |
|---|---|---|---|---|
| 1 | 2 | 0.8 | 0.8 | |
| 0.2 | 0.2 | 0.04 | 0.04 | |
| 0.05 | 0.01 | 0.18 | 0.18 |
| Controller | MAE | IAE | ITAE | |
|---|---|---|---|---|
| Height with fixed value | HODFC | 0.0223 | 1.3376 | 9.6850 |
| PID | 0.0238 | 1.4273 | 17.9791 | |
| Improvement Ratio | 1.6073 | 1.0671 | 1.8564 | |
| Yaw with fixed value | HODFC | 0.0052 | 0.3087 | 2.2582 |
| PID | 0.0055 | 0.3311 | 6.5964 | |
| Improvement Ratio | 1.0577 | 1.0726 | 2.9211 |
| Controller | MAE | IAE | ITAE | |
|---|---|---|---|---|
| Height with a sinusoidal function | HODFC | 0.0538 | 3.2279 | 89.2979 |
| PID | 0.0977 | 5.8636 | 179.4122 | |
| Improvement Ratio | 1.8160 | 1.8165 | 2.0091 | |
| Yaw with a sinusoidal function | HODFC | 0.0238 | 1.4290 | 37.6433 |
| PID | 0.1059 | 6.3561 | 191.7916 | |
| Improvement Ratio | 4.4496 | 4.4479 | 5.0950 | |
| Spatial plane trajectory | HODFC | 0.3243 | 32.4210 | 1.3776 × 103 |
| PID | 0.7197 | 71.9629 | 3.3282 × 103 | |
| Improvement Ratio | 2.2192 | 2.2196 | 2.4159 |
| Controller | MAE | IAE | ITAE | |
|---|---|---|---|---|
| Height with human interference | HODFC | 0.0192 | 1.1513 | 24.8912 |
| PID | 0.0311 | 1.8679 | 55.8895 | |
| Improvement Ratio | 1.6198 | 1.6224 | 2.2454 | |
| Yaw with human interference | HODFC | 0.0147 | 0.8813 | 15.2536 |
| PID | 0.0291 | 1.7456 | 51.1210 | |
| Improvement Ratio | 1.9796 | 1.9807 | 3.3514 | |
| Yaw with wind disturbance | HODFC | 0.0165 | 0.9870 | 14.6846 |
| PID | 0.0279 | 1.6721 | 49.8645 | |
| Improvement Ratio | 1.6909 | 1.6941 | 3.3957 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, G.; Ma, S.; Guo, X.; Li, X.; Guo, J. High-Order Differential Feedback Control for Quadrotor UAV: Theory and Experimentation. Electronics 2020, 9, 2001. https://doi.org/10.3390/electronics9122001
Qi G, Ma S, Guo X, Li X, Guo J. High-Order Differential Feedback Control for Quadrotor UAV: Theory and Experimentation. Electronics. 2020; 9(12):2001. https://doi.org/10.3390/electronics9122001
Chicago/Turabian StyleQi, Guoyuan, Shengli Ma, Xitong Guo, Xia Li, and Jianchuan Guo. 2020. "High-Order Differential Feedback Control for Quadrotor UAV: Theory and Experimentation" Electronics 9, no. 12: 2001. https://doi.org/10.3390/electronics9122001
APA StyleQi, G., Ma, S., Guo, X., Li, X., & Guo, J. (2020). High-Order Differential Feedback Control for Quadrotor UAV: Theory and Experimentation. Electronics, 9(12), 2001. https://doi.org/10.3390/electronics9122001




