Microwave Imaging by Means of Lebesgue-Space Inversion: An Overview
Abstract
1. Introduction
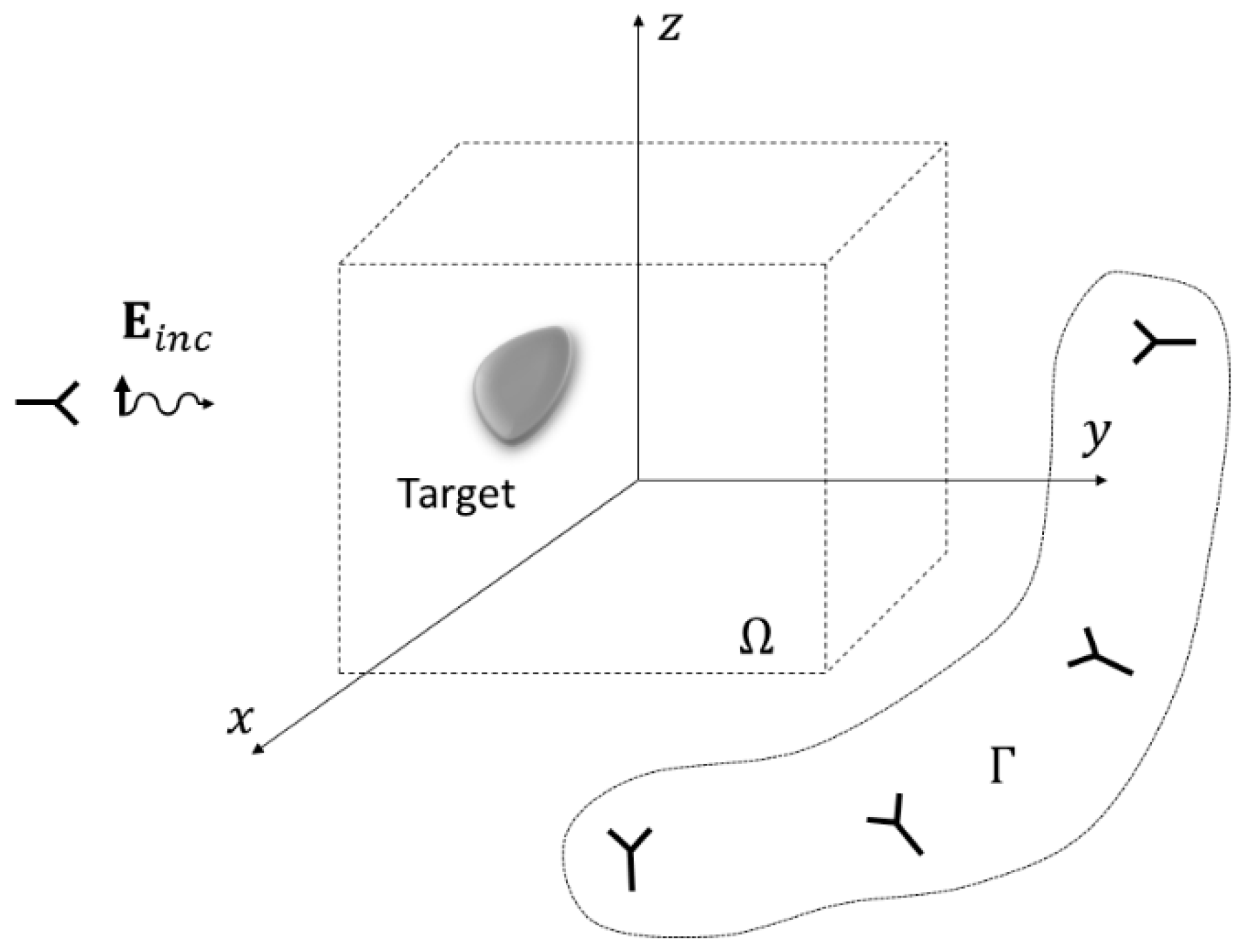
2. Overview of the Inverse-Scattering Problem Formulation
3. Newton-Type Methods in Banach Spaces
3.1. Classical Mathematical Foundations in Hilbert Spaces
| Algorithm1. Two level (outer–inner iterations) inexact Newton method for the nonlinear Equation (5) |
|
3.2. Extension to Banach Spaces
- (III)
- INNER STEP: Find a (regularized) solution of the linear Equation (11) by means of an iterative minimization, with respect to, of the-th residual. Specifically, letbe the inner initial guess. Then, for, compute:until a certain stopping rule is satisfied (e.g., a maximum number of inner iterationsis reached or the norm of the functionalfalls below a specified threshold). The obtained regularized solution of the-th linear system (11) is denoted as.
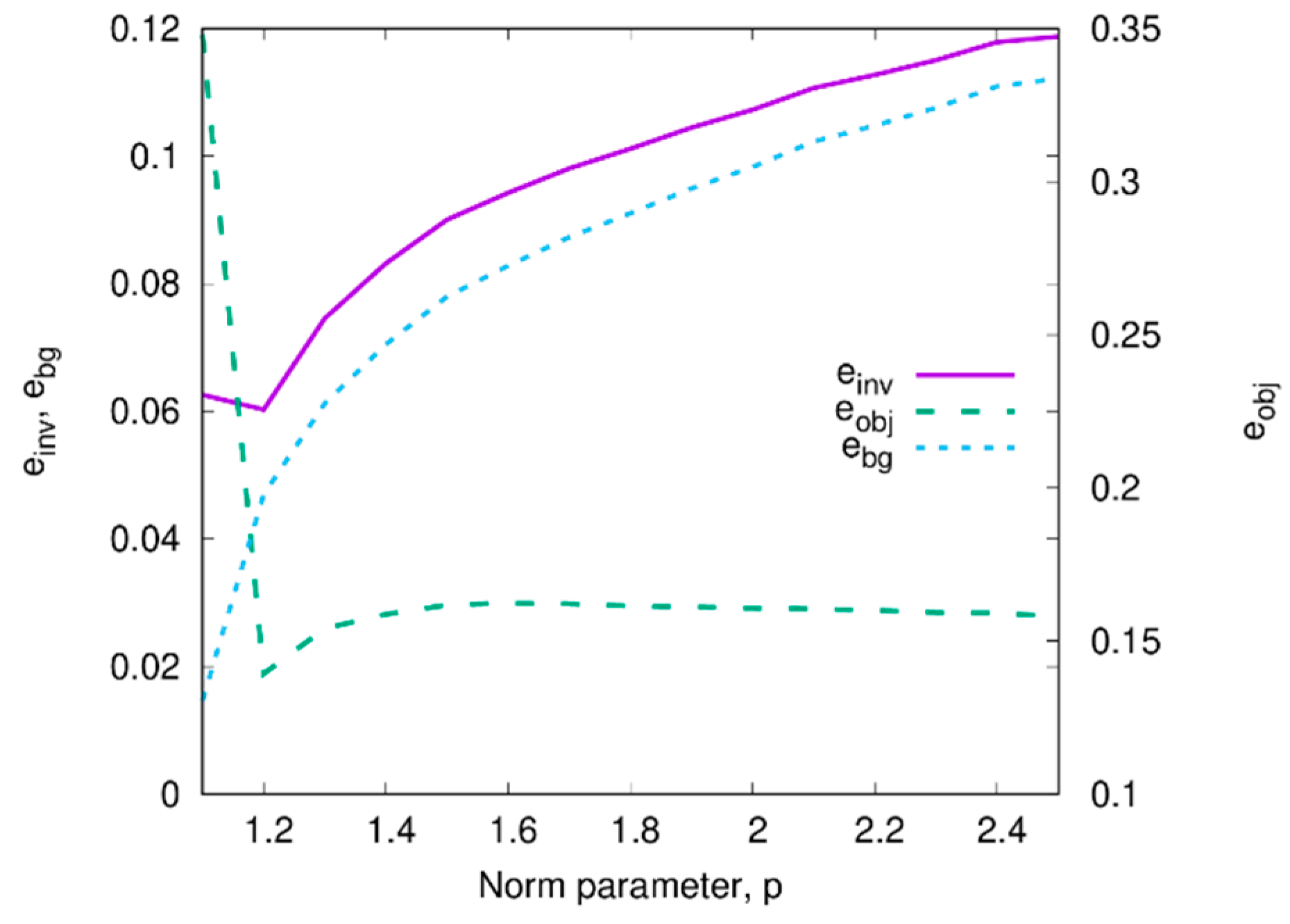
3.3. The Role of the Exponent Parameter in the Lebesgue Spaces Solution
- (i)
- since , the “cost” functional is convex, consequently no other local minima arise in the context of regularization, and differentiable;
- (ii)
- the duality maps and are always single-valued.
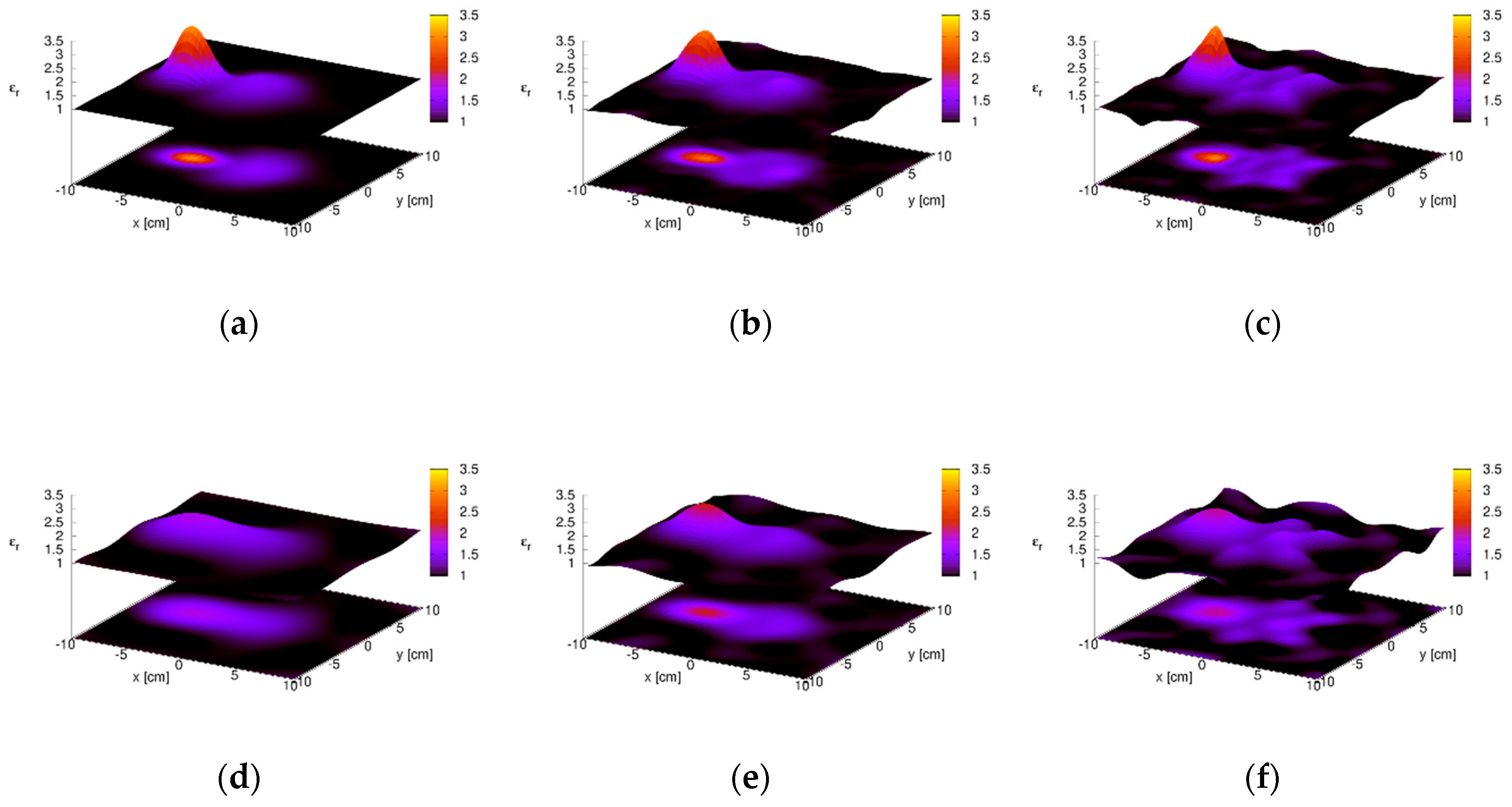
3.4. A Reconstruction Example
4. Multifrequency Lebesgue-Space Inversion
A Reconstruction Example
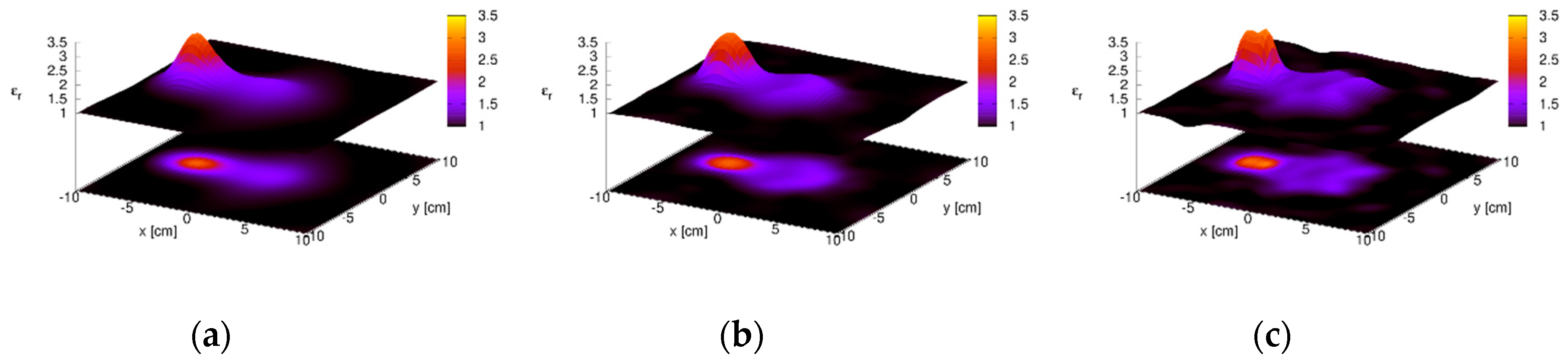
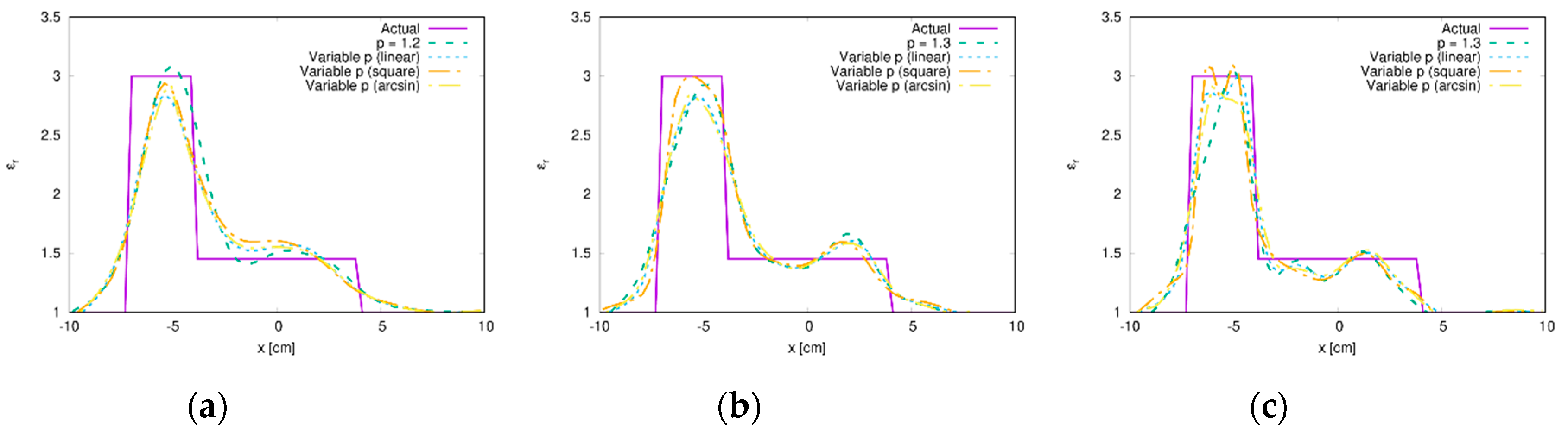
5. The Novel Variable-Exponent Approach
5.1. Strategies for Choosing the Variable Exponent Function
5.2. A Reconstruction Example
- Linear →
- Square →
- Square root →
- Arcsin →
- Sine →
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Nikolova, N.K. Introduction to Microwave Imaging; Cambridge University Press: Cambridge, UK, 2017; ISBN 978-1-316-08426-7. [Google Scholar]
- Chen, X. Computational Methods for Electromagnetic Inverse Scattering; John Wiley & Sons: South Tower, Singapore, 2018. [Google Scholar]
- Vacca, M.; Tobon, J.A.; Pulimeno, A.; Sarwar, I.; Vipiana, F.; Casu, M.R.; Solimene, R. A COTS-Based Microwave Imaging System for Breast-Cancer Detection. IEEE Trans. Biomed. Circuits Syst. 2017, 11, 804–814. [Google Scholar]
- Brancaccio, A.; Leone, G.; Solimene, R. Single-frequency subsurface remote sensing via a non-cooperative source. J. Electromagn. Waves Appl. 2016, 30, 1147–1161. [Google Scholar] [CrossRef]
- Coli, V.L.; Tournier, P.; Dolean-Maini, V.; Kanfoud, I.E.; Pichot, C.; Migliaccio, C.; Blanc-Féraud, L. Detection of Simulated Brain Strokes Using Microwave Tomography. IEEE J. Electromagn. RF Microwaves Med. Biol. 2019, 1. [Google Scholar] [CrossRef]
- Solimene, R.; Soldovieri, F.; Prisco, G.; Pierri, R. Three-Dimensional Through-Wall Imaging Under Ambiguous Wall Parameters. IEEE Trans. Geosci. Remote. Sens. 2009, 47, 1310–1317. [Google Scholar] [CrossRef]
- Pastorino, M.; Randazzo, A. Microwave Imaging Methods and Applications; Artech House: Norwood, MA, USA, 2018; ISBN 978-1-63081-348-2. [Google Scholar]
- Burfeindt, M.J.; Shea, J.D.; Van Veen, B.D.; Hagness, S.C. Beamforming-Enhanced Inverse Scattering for Microwave Breast Imaging. IEEE Trans. Antennas Propag. 2014, 62, 5126–5132. [Google Scholar] [CrossRef] [PubMed]
- Zoughi, R. Microwave Non-Destructive Testing and Evaluation; Kluwer Academic Publishers: Dordrecht, The Newtherlands, 2000; ISBN 0-412-62500-8. [Google Scholar]
- Bevacqua, M.; Crocco, L.; Di Donato, L.; Isernia, T.; Palmeri, R. Exploiting sparsity and field conditioning in subsurface microwave imaging of nonweak buried targets. Radio Sci. 2016, 51, 301–310. [Google Scholar] [CrossRef]
- Frezza, F.; Pajewski, L.; Ponti, C.; Schettini, G. Through-wall electromagnetic scattering by N conducting cylinders. J. Opt. Soc. Am. A 2013, 30, 1632–1639. [Google Scholar] [CrossRef] [PubMed]
- Palmeri, R.; Bevacqua, M.T.; Crocco, L.; Isernia, T.; Di Donato, L. Microwave Imaging via Distorted Iterated Virtual Experiments. IEEE Trans. Antennas Propag. 2017, 65, 829–838. [Google Scholar] [CrossRef]
- Solimene, R.; Leone, G. MUSIC Algorithms for Grid Diagnostics. IEEE Geosci. Remote. Sens. Lett. 2013, 10, 226–230. [Google Scholar] [CrossRef]
- Solimene, R.; Soldovieri, F.; Prisco, G. A Multiarray Tomographic Approach for Through-Wall Imaging. IEEE Trans. Geosci. Remote. Sens. 2008, 46, 1192–1199. [Google Scholar] [CrossRef]
- Zhong, Y.; Lambert, M.; Lesselier, D.; Chen, X. A new integral equation method to solve highly nonlinear inverse scattering problems. IEEE Trans. Antennas Propag. 2016, 64, 1788–1799. [Google Scholar] [CrossRef]
- Shumakov, D.S.; Nikolova, N.K. Fast Quantitative Microwave Imaging With Scattered-Power Maps. IEEE Trans. Microwave. Theory Tech. 2018, 66, 439–449. [Google Scholar] [CrossRef]
- Shah, P.; Khankhoje, U.K.; Moghaddam, M. Inverse scattering using a joint L1–L2 norm-based regularization. IEEE Trans. Antennas Propag. 2016, 64, 1373–1384. [Google Scholar] [CrossRef]
- Taskin, U.; Ozdemir, O. Sparsity Regularized Nonlinear Inversion for Microwave Imaging. IEEE Geosci. Remote. Sens. Lett. 2017, 14, 2220–2224. [Google Scholar] [CrossRef]
- Salucci, M.; Oliveri, G.; Anselmi, N.; Viani, F.; Fedeli, A.; Pastorino, M.; Randazzo, A. Three-dimensional electromagnetic imaging of dielectric targets by means of the multiscaling inexact-Newton method. J. Opt. Soc. Am. A 2017, 34, 1119–1131. [Google Scholar] [CrossRef] [PubMed]
- Azghani, M.; Marvasti, F. L2-regularized Iterative Weighted Algorithm for Inverse Scattering. IEEE Trans. Antennas Propag. 2016, 64, 2293–2300. [Google Scholar] [CrossRef]
- Rabbani, M.; Tavakoli, A.; Dehmollaian, M. A Hybrid Quantitative Method for Inverse Scattering of Multiple Dielectric Objects. IEEE Trans. Antennas Propag. 2016, 64, 977–987. [Google Scholar] [CrossRef]
- Gilmore, C.; Abubakar, A.; Hu, W.; Habashy, T.M.; van den Berg, P.M. Microwave biomedical data inversion using the finite-difference contrast source inversion method. IEEE Trans. Antennas Propag. 2009, 57, 1528–1538. [Google Scholar] [CrossRef]
- De Zaeytijd, Jü.; Franchois, A.; Eyraud, C.; Geffrin, J.-M. Full-wave three-dimensional microwave imaging with a regularized Gauss–Newton method–Theory and experiment. IEEE Trans. Antennas Propag. 2007, 55, 3279–3292. [Google Scholar] [CrossRef]
- Bisio, I.; Fedeli, A.; Lavagetto, F.; Pastorino, M.; Randazzo, A.; Sciarrone, A.; Tavanti, E. A numerical study concerning brain stroke detection by microwave imaging systems. Multimedia Tools Appl. 2018, 77, 9341–9363. [Google Scholar] [CrossRef]
- Abubakar, A.; Habashy, T.M.; Pan, G.; Li, M.-K. Application of the Multiplicative Regularized Gauss–Newton Algorithm for Three-Dimensional Microwave Imaging. IEEE Trans. Antennas Propag. 2012, 60, 2431–2441. [Google Scholar] [CrossRef]
- Pastorino, M. Stochastic Optimization Methods Applied to Microwave Imaging: A Review. IEEE Trans. Antennas Propag. 2007, 55, 538–548. [Google Scholar] [CrossRef]
- Schuster, T.; Kaltenbacher, B.; Hofmann, B.; Kazimierski, K.S. Regularization Methods in Banach Spaces; De Gruyter: Berlin, Germany, 2012; ISBN 978-3-11-025524-9. [Google Scholar]
- Schopfer, F.; Louis, A.K.; Schuster, T. Nonlinear iterative methods for linear ill-posed problems in Banach spaces. Inverse Prob. 2006, 22, 311–329. [Google Scholar] [CrossRef]
- Hein, T.; Kazimierski, K.S. Accelerated Landweber iteration in Banach spaces. Inverse Prob. 2010, 26. [Google Scholar] [CrossRef]
- Estatico, C.; Pastorino, M.; Randazzo, A. A Novel Microwave Imaging Approach Based on Regularization in Lp Banach Spaces. IEEE Trans. Antennas Propag. 2012, 60, 3373–3381. [Google Scholar] [CrossRef]
- Estatico, C.; Fedeli, A.; Pastorino, M.; Randazzo, A. A Multi-Frequency Inexact-Newton Method in Lp Banach Spaces for Buried Objects Detection. IEEE Trans. Antennas Propag. 2015, 63, 4198–4204. [Google Scholar] [CrossRef]
- Estatico, C.; Pastorino, M.; Randazzo, A.; Tavanti, E. Three-Dimensional Microwave Imaging in Lp Banach Spaces: Numerical and Experimental Results. IEEE Trans. Comput. Imaging 2018, 4, 609–623. [Google Scholar] [CrossRef]
- Estatico, C.; Fedeli, A.; Pastorino, M.; Randazzo, A. Buried object detection by means of a Lp Banach-space inversion procedure. Radio Sci. 2015, 50, 41–51. [Google Scholar] [CrossRef]
- Estatico, C.; Fedeli, A.; Pastorino, M.; Randazzo, A. Quantitative Microwave Imaging Method in Lebesgue Spaces With Nonconstant Exponents. IEEE Trans. Antennas Propag. 2018, 66, 7282–7294. [Google Scholar] [CrossRef]
- Quarteroni, A.; Sacco, R.; Saleri, F. Numerical Mathematics, 2nd ed.; Springer: Berlin, Germany, 2006; ISBN 978-3-540-34658-6. [Google Scholar]
- Boccacci, P.; Bertero, M. Introduction to Inverse Problems in Imaging; CRC Press: Boca Raton, FL, USA, 1998. [Google Scholar]
- Bozza, G.; Pastorino, M. An Inexact Newton-Based Approach to Microwave Imaging Within the Contrast Source Formulation. IEEE Trans. Antennas Propag. 2009, 57, 1122–1132. [Google Scholar] [CrossRef]
- Randazzo, A.; Oliveri, G.; Massa, A.; Pastorino, M. Electromagnetic inversion with the multiscaling inexact Newton method–experimental validation. Microwave. Opt. Technol. Lett. 2011, 53, 2834–2838. [Google Scholar] [CrossRef]
- Belkebir, K.; Saillard, M. Testing inversion algorithms against experimental data: inhomogeneous targets. Inverse Prob. 2005, 21, S1–S3. [Google Scholar] [CrossRef]
- Geffrin, J.-M.; Sabouroux, P.; Eyraud, C. Free space experimental scattering database continuation: experimental set-up and measurement precision. Inverse Probl. 2005, 21, S117–S130. [Google Scholar] [CrossRef]
- Estatico, C.; Fedeli, A.; Pastorino, M.; Randazzo, A. A Banach Space Regularization Approach for Multifrequency Microwave Imaging. Int. J. Antennas Propag. 2016, 2016, 1–8. [Google Scholar] [CrossRef]
- Diening, L.; Harjulehto, P.; Hästö, P.; Ruzicka, M. Lebesgue and Sobolev Spaces with Variable Exponents; Springe: Berlin, Germany, 2011. [Google Scholar]
- Dinca, G.; Matei, P. Geometry of Sobolev spaces with variable exponent: smoothness and uniform convexity. C. R. Math. 2009, 347, 885–889. [Google Scholar] [CrossRef]


| Frequency [GHz] | Lebesgue (Optimal Norm) | Hilbert | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| popt | einv | eobj | ebg | tm [s] | einv | eobj | ebg | tm [s] | |||
| 2 | 1.2 | 0.060 | 0.14 | 0.047 | 5 | 11.78 | 0.11 | 0.16 | 0.098 | 6 | 12.54 |
| 3 | 1.3 | 0.082 | 0.13 | 0.074 | 10 | 17.26 | 0.12 | 0.13 | 0.12 | 12 | 16.93 |
| 4 | 1.3 | 0.096 | 0.13 | 0.091 | 8 | 18.62 | 0.16 | 0.15 | 0.17 | 21 | 21.50 |
| KLW | einv | eobj | ebg |
|---|---|---|---|
| 25 | 0.13 | 0.16 | 0.13 |
| 50 | 0.14 | 0.15 | 0.14 |
| 75 | 0.14 | 0.15 | 0.14 |
| 100 | 0.14 | 0.14 | 0.14 |
| Number of Frequencies | Lebesgue (Optimal Norm) | Hilbert | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| popt | einv | eobj | ebg | tm [s] | einv | eobj | ebg | tm [s] | |||
| 2 | 1.3 | 0.057 | 0.12 | 0.047 | 4 | 117.87 | 0.083 | 0.13 | 0.076 | 13 | 133.57 |
| 3 | 1.3 | 0.049 | 0.088 | 0.043 | 6 | 205.97 | 0.066 | 0.098 | 0.061 | 16 | 221.65 |
| 4 | 1.4 | 0.045 | 0.075 | 0.040 | 10 | 313.20 | 0.059 | 0.081 | 0.055 | 21 | 330.66 |
| 5 | 1.4 | 0.041 | 0.066 | 0.036 | 19 | 454.80 | 0.052 | 0.074 | 0.048 | 24 | 451.44 |
| 6 | 1.4 | 0.039 | 0.062 | 0.036 | 17 | 611.34 | 0.049 | 0.071 | 0.045 | 27 | 592.21 |
| 7 | 1.5 | 0.038 | 0.060 | 0.034 | 19 | 772.71 | 0.046 | 0.068 | 0.042 | 28 | 744.73 |
| Map Type | 2 GHz | 3 GHz | 4 GHz | ||||||
|---|---|---|---|---|---|---|---|---|---|
| einv | eobj | ebg | einv | eobj | ebg | einv | eobj | ebg | |
| Linear | 0.076 | 0.15 | 0.064 | 0.073 | 0.12 | 0.065 | 0.085 | 0.10 | 0.082 |
| Sine | 0.076 | 0.15 | 0.064 | 0.071 | 0.12 | 0.062 | 0.11 | 0.12 | 0.10 |
| Quadratic | 0.079 | 0. 16 | 0.066 | 0.070 | 0.11 | 0.063 | 0.098 | 0.11 | 0.095 |
| Square root | 0.077 | 0.14 | 0.065 | 0.077 | 0.13 | 0.069 | 0.088 | 0.11 | 0.084 |
| Arcsin | 0.077 | 0.15 | 0.066 | 0.078 | 0.12 | 0.070 | 0.092 | 0.11 | 0.090 |
| Map Type | 2 GHz | 3 GHz | 4 GHz | |||
|---|---|---|---|---|---|---|
| tm [s] | tm [s] | tm [s] | ||||
| Linear | 11 | 12.61 | 8 | 17.41 | 6 | 19.05 |
| Sine | 9 | 12.98 | 4 | 16.19 | 8 | 21.80 |
| Quadratic | 7 | 12.74 | 7 | 16.71 | 6 | 18.98 |
| Square root | 11 | 13.06 | 8 | 17.75 | 7 | 19.83 |
| Arcsin | 10 | 12.72 | 8 | 17.59 | 7 | 19.60 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Estatico, C.; Fedeli, A.; Pastorino, M.; Randazzo, A. Microwave Imaging by Means of Lebesgue-Space Inversion: An Overview. Electronics 2019, 8, 945. https://doi.org/10.3390/electronics8090945
Estatico C, Fedeli A, Pastorino M, Randazzo A. Microwave Imaging by Means of Lebesgue-Space Inversion: An Overview. Electronics. 2019; 8(9):945. https://doi.org/10.3390/electronics8090945
Chicago/Turabian StyleEstatico, Claudio, Alessandro Fedeli, Matteo Pastorino, and Andrea Randazzo. 2019. "Microwave Imaging by Means of Lebesgue-Space Inversion: An Overview" Electronics 8, no. 9: 945. https://doi.org/10.3390/electronics8090945
APA StyleEstatico, C., Fedeli, A., Pastorino, M., & Randazzo, A. (2019). Microwave Imaging by Means of Lebesgue-Space Inversion: An Overview. Electronics, 8(9), 945. https://doi.org/10.3390/electronics8090945







