A Theoretical Study on the Operation Principle of Hybrid Solar Cells
School of Engineering and IT, Charles Darwin University, Darwin NT 0909, Australia
*
Author to whom correspondence should be addressed.
Academic Editor: Mohan Jacob
Electronics 2015, 4(2), 303-310; https://doi.org/10.3390/electronics4020303
Received: 13 February 2015 / Revised: 15 May 2015 / Accepted: 25 May 2015 / Published: 29 May 2015
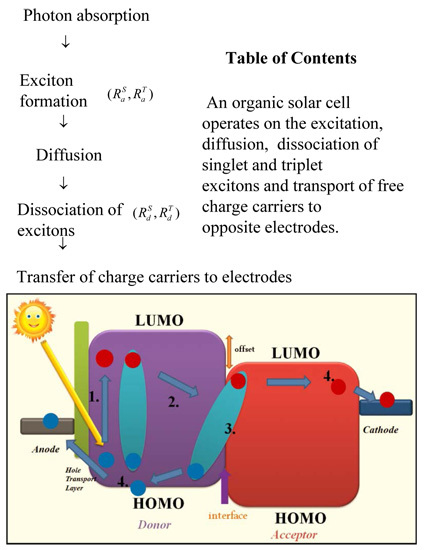
In this work, the operation mechanism of hybrid solar cells is studied explicitly. The excitation, diffusion and dissociation of singlet and triplet excitons and charge transport of free charge carriers are studied and their corresponding rates are calculated for a flexible P3HT:SiNW hybrid solar cell. The rates are found to be faster for singlet than triplet excitons. Possible loss mechanisms in hybrid solar cells have also been highlighted.
View Full-Text
Keywords:
hybrid solar cells; excitation; diffusion; dissociation
▼
Show Figures
Graphical abstract
This is an open access article distributed under the Creative Commons Attribution License which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited
MDPI and ACS Style
Narayan, M.; Singh, J. A Theoretical Study on the Operation Principle of Hybrid Solar Cells. Electronics 2015, 4, 303-310. https://doi.org/10.3390/electronics4020303
AMA Style
Narayan M, Singh J. A Theoretical Study on the Operation Principle of Hybrid Solar Cells. Electronics. 2015; 4(2):303-310. https://doi.org/10.3390/electronics4020303
Chicago/Turabian StyleNarayan, Monishka; Singh, Jai. 2015. "A Theoretical Study on the Operation Principle of Hybrid Solar Cells" Electronics 4, no. 2: 303-310. https://doi.org/10.3390/electronics4020303
Find Other Styles
Search more from Scilit
