Elimination of Static Angular Error and Stability Enhancement for Active Power-Synchronized Converter Under a Weak Grid
Abstract
1. Introduction
2. Modeling and Analysis of Angular Errors in Vector Current Control Employing Active Power Synchronization
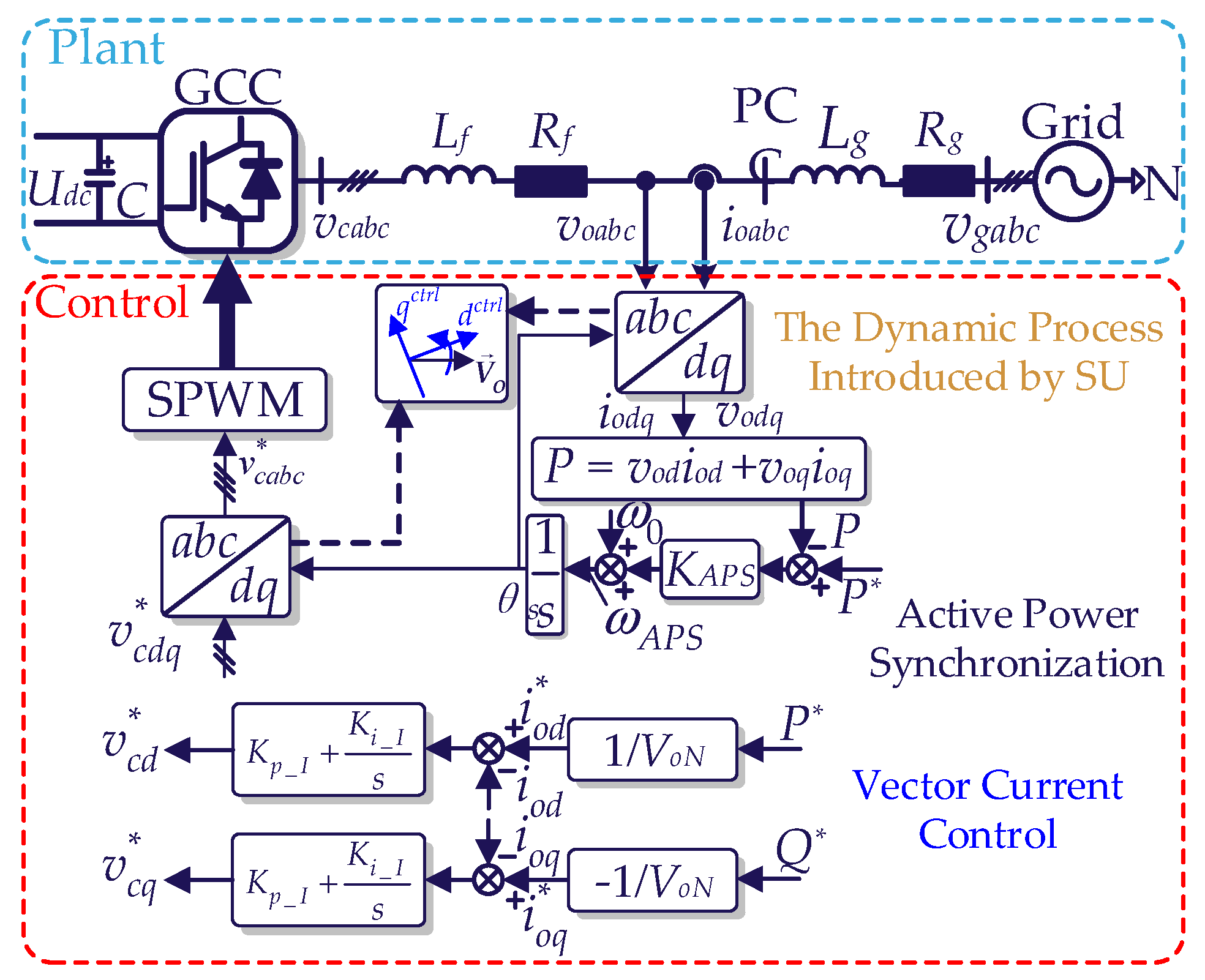
2.1. Description of the GCC System
2.2. Analysis and Calculation of Operating Points for Grid-Connected Converter Employing Phase-Locked Loop
3. Design Process of the Composite Direct Damping Controller
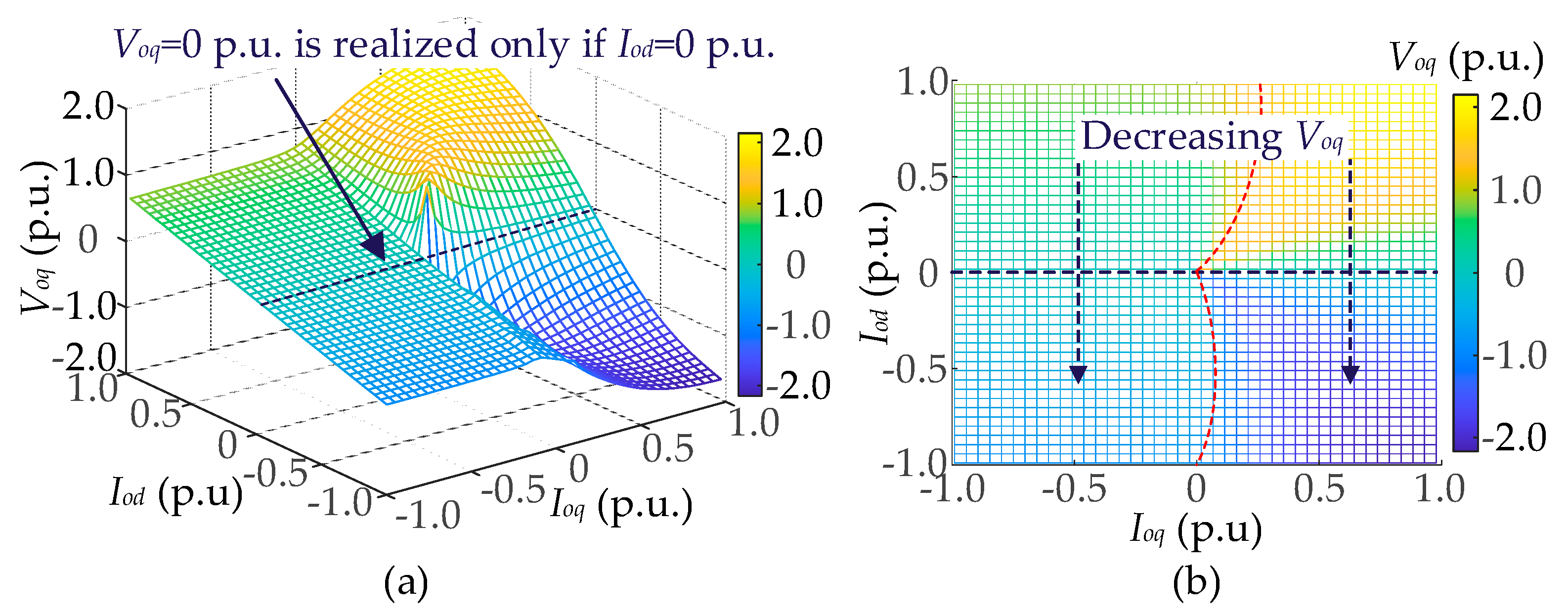
3.1. Derivation of the Closed-Loop SISO Model and Analysis of Angular Error for Grid-Connected Converter Employing Active Power Synchronization
3.2. Derivation of the Closed-Loop SISO Model and Analysis of Angular Error of Grid-Connected Converter Employing Conventional Phase-Locked Loop and Phase-Locked Loop with Second-Order Generalized Integer
3.3. Analysis of GCC Employing APS and PI-APS for Stability Risk and Static Angular Error
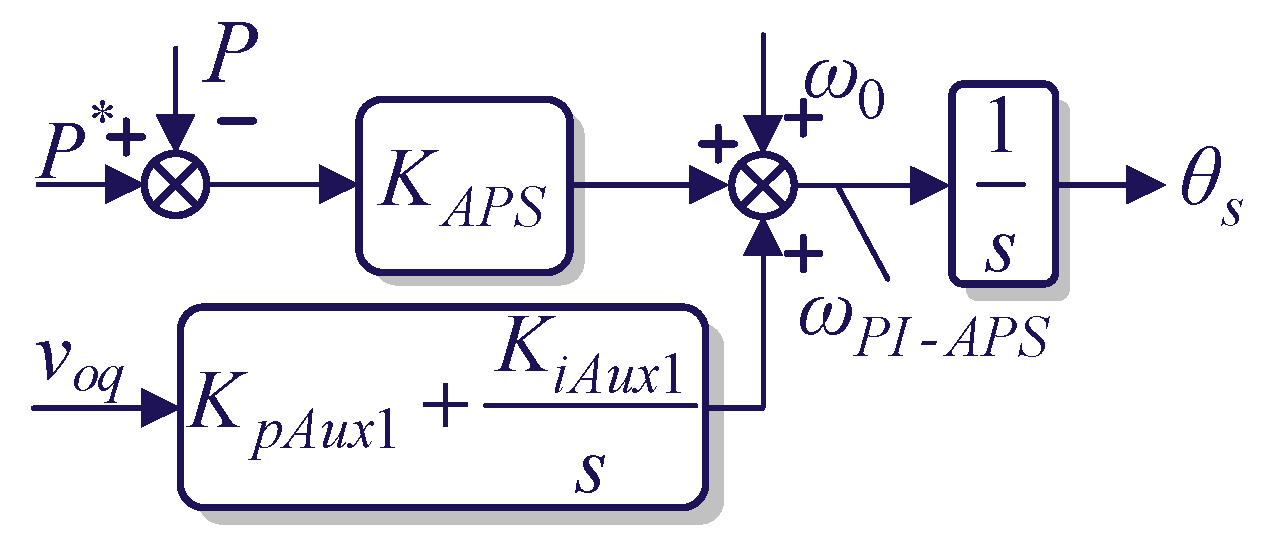
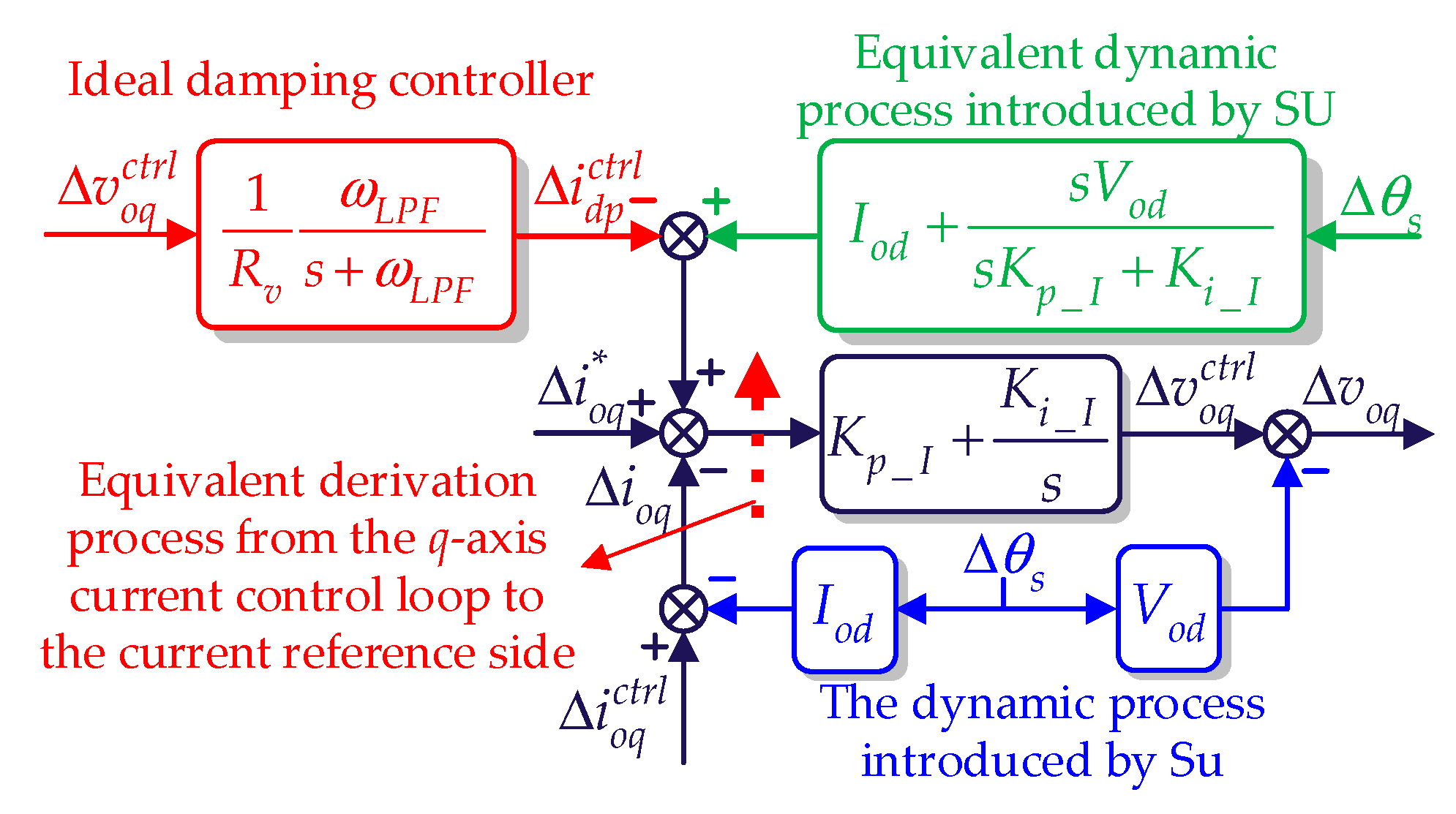
3.4. The Design Process of the Proposed Composite Direct Damping Controller
3.4.1. Mechanism Analysis and Design of the Proposed Composite Direct Damping Controller
- (1)
- The selected variables, which are adopted by the proposed damping controller, should reflect fluctuations in the output angle of the SU so that the damping effect can be guaranteed.
- (2)
- The proposed damping controller functions only in the transient state and its inclusion should not affect the steady-state current reference value.
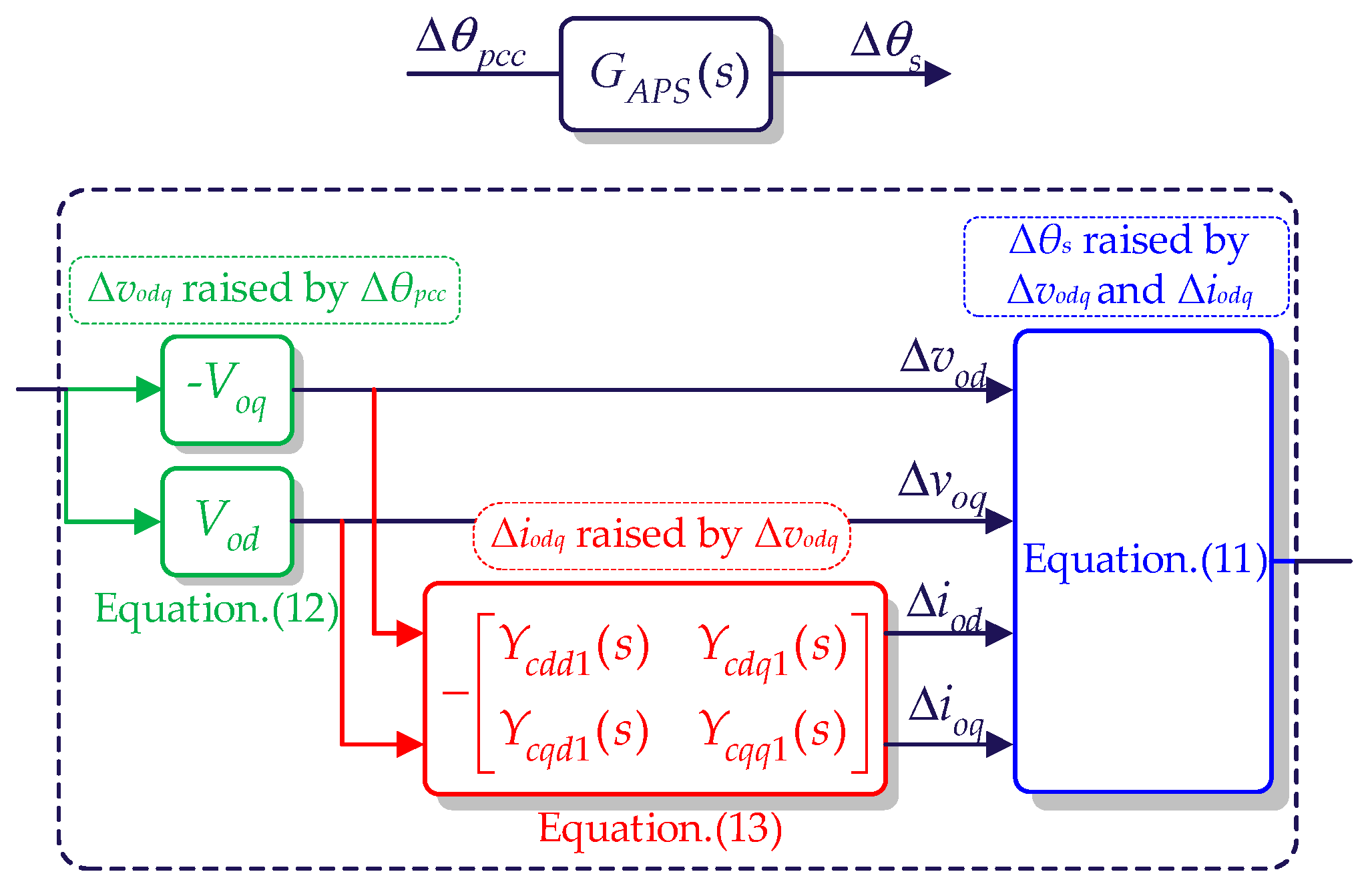
3.4.2. Impact of Angle Perturbations of PCC Voltage on the Output Angle in Active Power-Based Synchronization Method with the Direct Damping and Proportional–Integral Controller
4. Dynamic Performance Analysis and Stability Evaluation of Grid-Connected Converter Employing the Proposed Composite Direct Damping Controller
4.1. Dynamic Performance Analysis of Active Power Synchronization with the Proportional–Integral Controller and the Proposed Direct Damping Controller
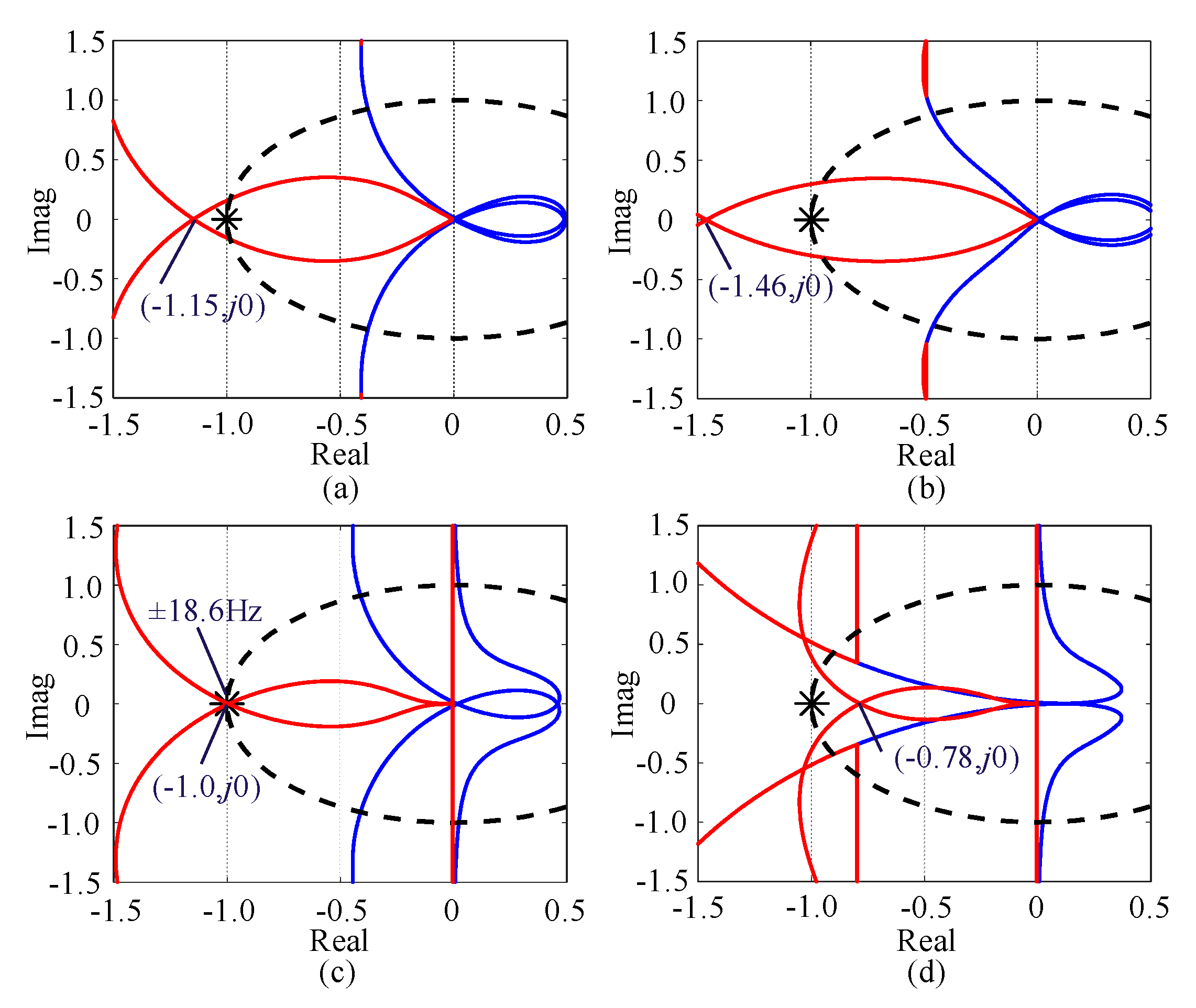
4.2. Stability Analysis Based on Generalized Nyquist Criterion
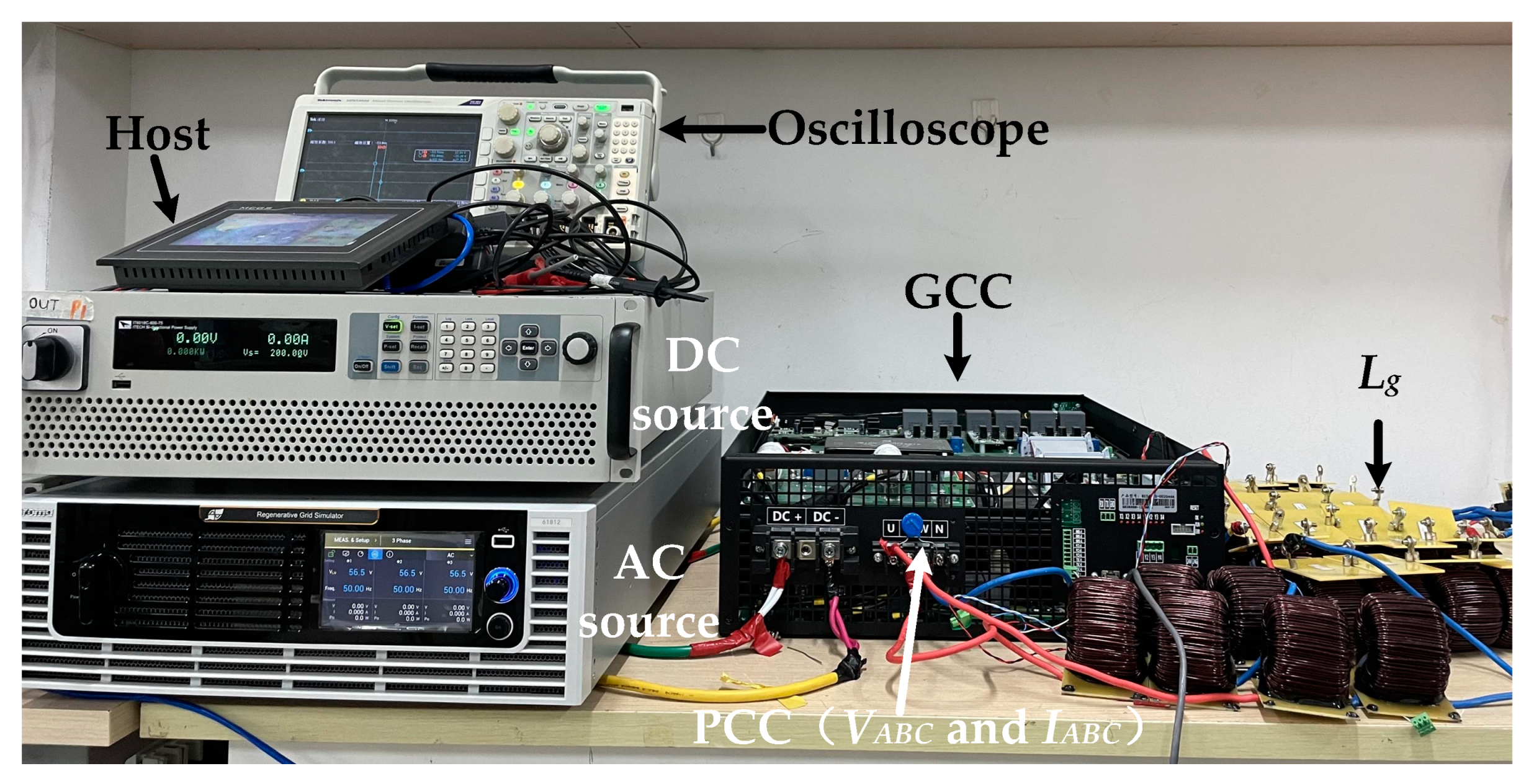
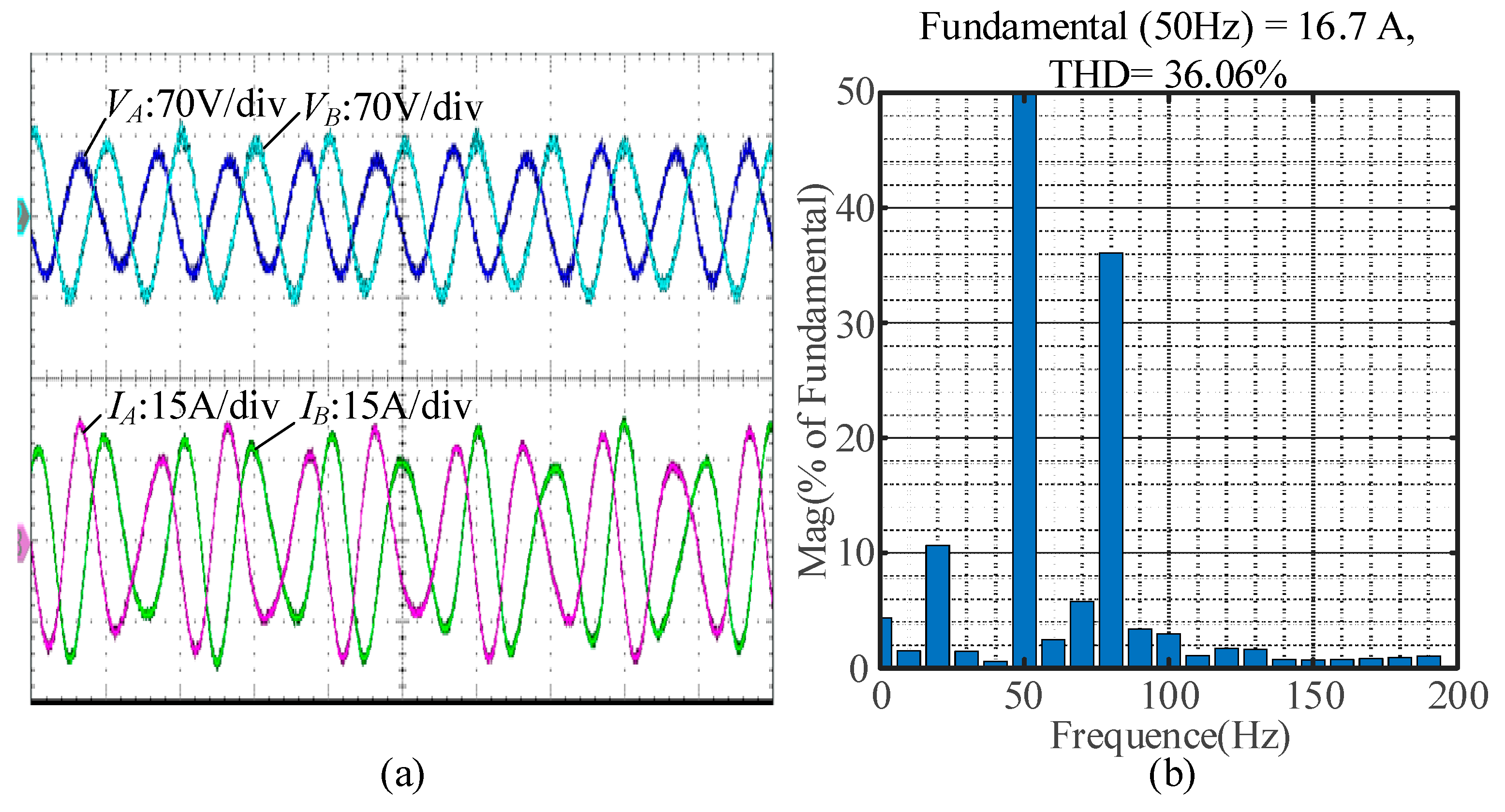
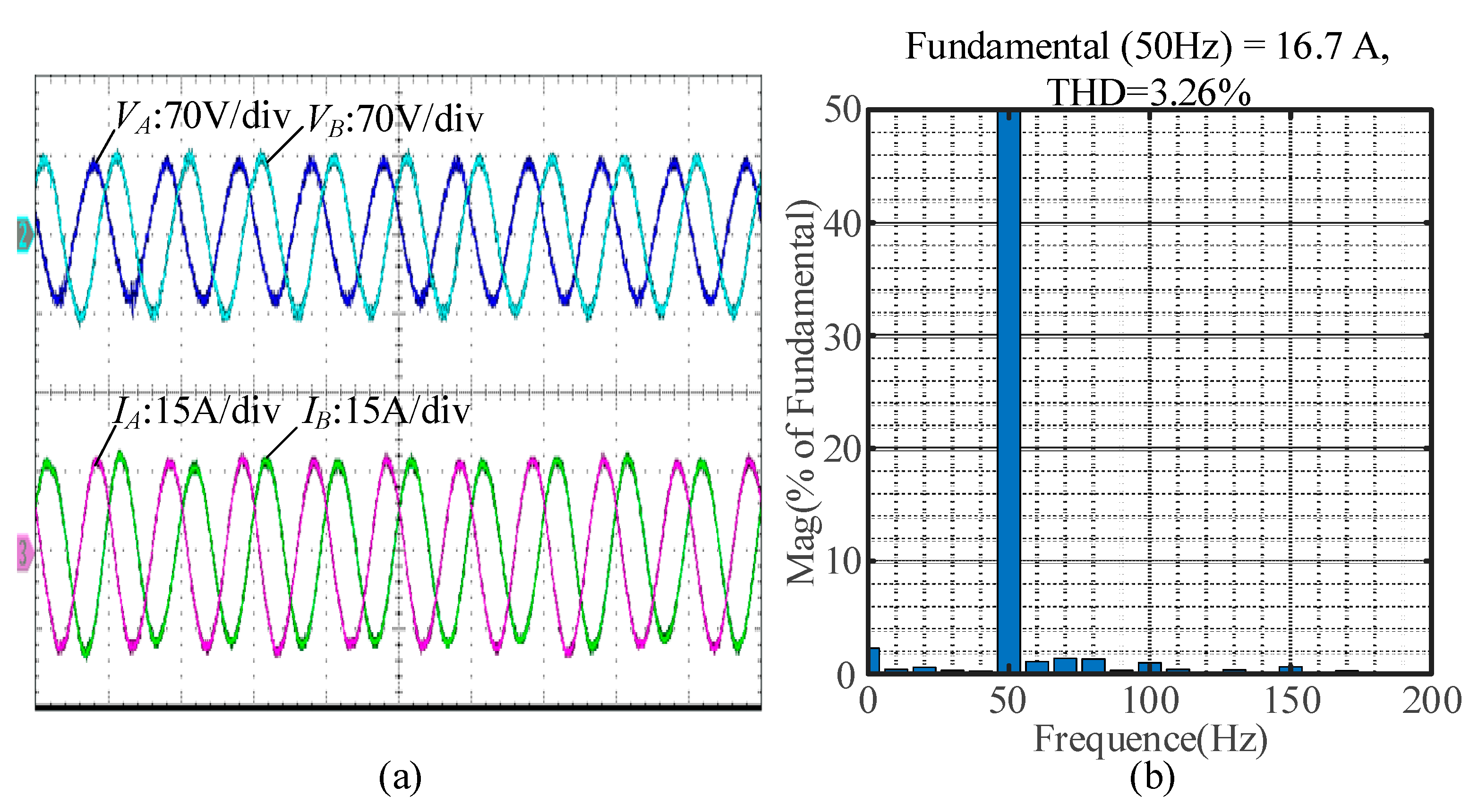
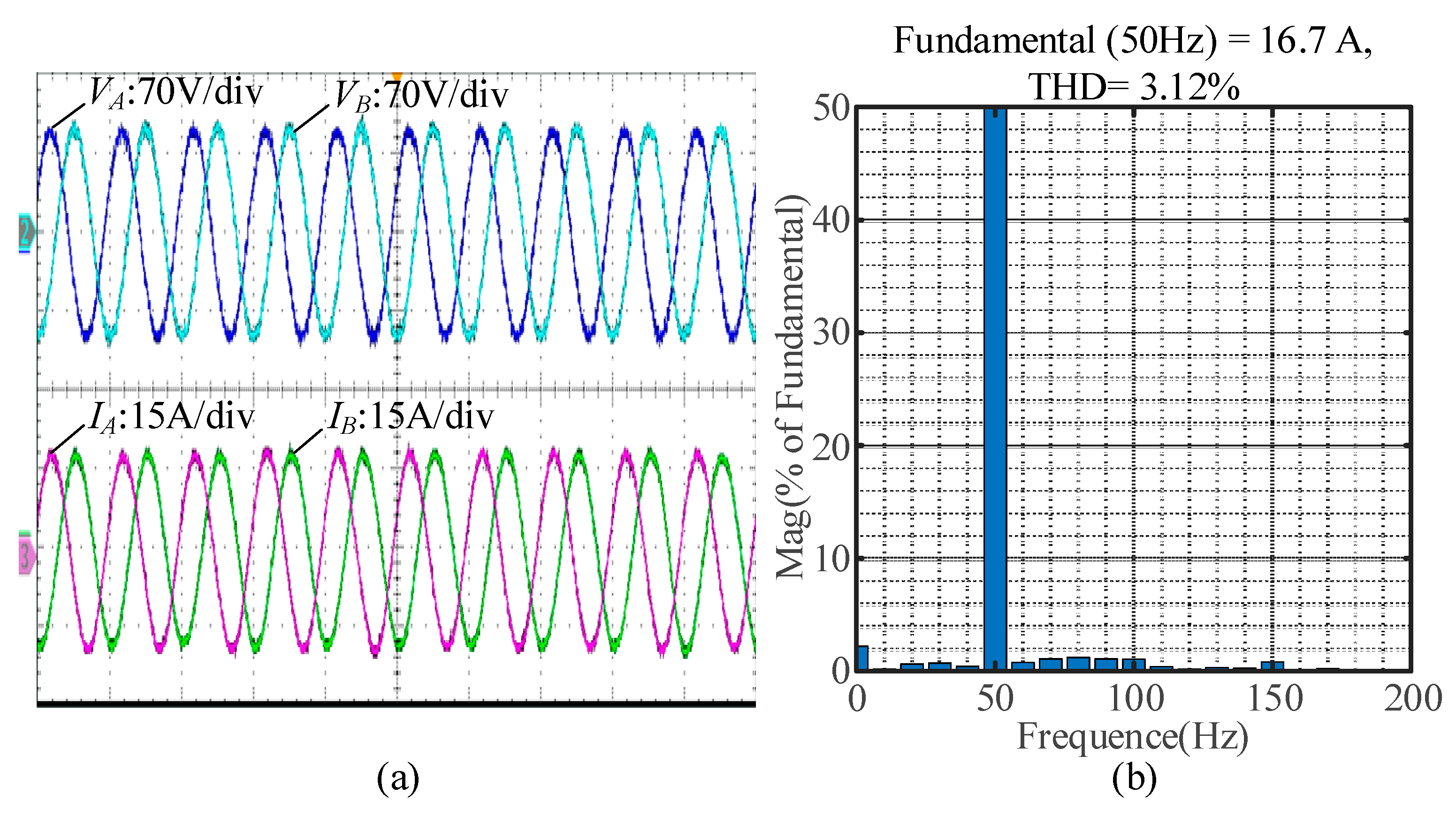
5. Experimental Verification
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lorenzen, S.L.; Nielsen, A.B.; Bede, L. Control of a grid-connected converter during weak grid conditions. In Proceedings of the 2016 IEEE 7th International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Vancouver, BC, Canada, 27–30 June 2016; pp. 1–6. [Google Scholar] [CrossRef]
- Egea-Alvarez, A.; Barker, C.; Hassan, F.; Gomis-Bellmunt, O. Capability curves of a VSC-HVDC connected to a weak AC grid considering stability and power limits. In Proceedings of the 11th IET International Conference on AC and DC Power Transmission, Birmingham, UK, 10–12 February 2015; pp. 1–5. [Google Scholar] [CrossRef]
- Aghazadeh, A.; Davari, M.; Nafisi, H.; Blaabjerg, F. Grid Integration of a Dual Two-Level Voltage-Source Inverter Considering Grid Impedance and Phase-Locked Loop. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 401–422. [Google Scholar] [CrossRef]
- Harnefors, L.; Bongiorno, M.; Lundberg, S. Input-Admittance Calculation and Shaping for Controlled Voltage-Source Converters. IEEE Trans. Ind. Electron. 2007, 54, 3323–3334. [Google Scholar] [CrossRef]
- Wen, B.; Boroyevich, D.; Burgos, R.; Mattavelli, P.; Shen, Z. Analysis of D-Q Small-Signal Impedance of Grid-Tied Inverters. IEEE Trans. Power Electron. 2016, 31, 675–687. [Google Scholar] [CrossRef]
- Cespedes, M.; Sun, J. Adaptive Control of Grid-Connected Inverters Based on Online Grid Impedance Measurements. IEEE Trans. Sustain. Energy 2014, 5, 516–523. [Google Scholar] [CrossRef]
- Midtsund, T.; Suul, J.A.; Undeland, T. Evaluation of current controller performance and stability for voltage source converters connected to a weak grid. In Proceedings of the 2nd International Symposium on Power Electronics for Distributed Generation Systems, Hefei, China, 16–18 June 2010; pp. 382–388. [Google Scholar] [CrossRef]
- Zhu, D.; Zhou, S.; Zou, X.; Kang, Y. Improved Design of PLL Controller for LCL-Type Grid-Connected Converter in Weak Grid. IEEE Trans. Power Electron. 2020, 35, 4715–4727. [Google Scholar] [CrossRef]
- Li, X.; Lin, H. A Design Method of Phase-Locked Loop for Grid-Connected Converters Considering the Influence of Current Loops in Weak Grid. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 8, 2420–2429. [Google Scholar] [CrossRef]
- Wu, X.; Li, X.; Yuan, X.; Geng, Y. Grid Harmonics Suppression Scheme for LCL-Type Grid-Connected Inverters Based on Output Admittance Revision. IEEE Trans. Sustain. Energy 2015, 6, 411–421. [Google Scholar] [CrossRef]
- Yi, H.; Wang, X.; Blaabjerg, F.; Zhuo, F. Impedance Analysis of SOGI-FLL-Based Grid Synchronization. IEEE Trans. Power Electron. 2017, 32, 7409–7413. [Google Scholar] [CrossRef]
- Zhang, Y.; Yang, X.; Zhang, Z. High-Efficiency Broadband Electroacoustic Energy Conversion Using Non-Foster-Inspired Circuit and Adaptively Switched Capacitor. IEEE Trans. Ind. Electron. 2025, 1–12. [Google Scholar] [CrossRef]
- Huang, L.; Wu, C.; Zhou, D.; Blaabjerg, F. A Double-PLLs-Based Impedance Reshaping Method for Extending Stability Range of Grid-Following Inverter Under Weak Grid. IEEE Trans. Power Electron. 2022, 37, 4091–4104. [Google Scholar] [CrossRef]
- Gui, Y.; Wang, X.; Blaabjerg, F.; Pan, D. Control of Grid-Connected Voltage-Source Converters: The Relationship Between Direct-Power Control and Vector-Current Control. IEEE Ind. Electron. Mag. 2019, 13, 31–40. [Google Scholar] [CrossRef]
- Gui, Y.; Wang, X.; Blaabjerg, F. Vector Current Control Derived from Direct Power Control for Grid-Connected Inverters. IEEE Trans. Power Electron. 2019, 34, 9224–9235. [Google Scholar] [CrossRef]
- Gui, Y.; Kim, C.; Chung, C.C.; Guerrero, J.M.; Guan, Y.; Vasquez, J.C. Improved Direct Power Control for Grid-Connected Voltage Source Converters. IEEE Trans. Ind. Electron. 2018, 65, 8041–8051. [Google Scholar] [CrossRef]
- Wu, C.; Xiong, X.; Blaabjerg, F. Impedance Analysis of Voltage Source Converter Based on Voltage Modulated Matrix. In Proceedings of the 2021 IEEE Energy Conversion Congress and Exposition (ECCE), Vancouver, BC, Canada, 10–14 October 2021; pp. 887–892. [Google Scholar] [CrossRef]
- Zhang, L.; Harnefors, L.; Nee, H. Power-Synchronization Control of Grid-Connected Voltage-Source Converters. IEEE Trans. Power Syst. 2010, 25, 809–820. [Google Scholar] [CrossRef]
- Bahrani, B. Power-Synchronized Grid-Following Inverter Without a Phase-Locked Loop. IEEE Access 2021, 9, 112163–112176. [Google Scholar] [CrossRef]
- Mansour, M.Z.; Ravanji, M.H.; Karimi, A.; Bahrani, B. Linear Parameter-Varying Control of a Power-Synchronized Grid-Following Inverter. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 2547–2558. [Google Scholar] [CrossRef]
- Gu, Y.; Green, T.C. Power System Stability with a High Penetration of Inverter-Based Resources. Proc. IEEE 2022, 111, 832–853. [Google Scholar] [CrossRef]
- Asensio, A.P.; Gómez, S.A.; Rodriguez-Amenedo, J.L.; Cardiel-Álvarez, M.Á. Reactive Power Synchronization Method for Voltage-Sourced Converters. IEEE Trans. Sustain. Energy 2019, 10, 1430–1438. [Google Scholar] [CrossRef]
- Sun, Z.; Sun, Y.; Lin, J.; Xie, S.; Han, H.; Wu, Z. Impedance Modeling and Stability Analysis of Single-Phase LCL-type Grid-Connected VSC Based on Harmonic Linearization. In Proceedings of the 2021 IEEE 12th Energy Conversion Congress & Exposition—Asia (ECCE-Asia), Singapore, 24–27 May 2021; pp. 2143–2148. [Google Scholar] [CrossRef]
- Huang, L.; Wu, C.; Zhou, D.; Blaabjerg, F. A Simplified SISO Small-Signal Model for Analyzing Instability Mechanism of Grid-Forming Inverter under Stronger Grid. In Proceedings of the 2021 IEEE 22nd Workshop on Control and Modelling of Power Electronics (COMPEL), Cartagena, Colombia, 2–5 November 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Liao, Y.; Wang, X.; Liu, F.; Xin, K.; Liu, Y. Sub-Synchronous Control Interaction in Grid-Forming VSCs with Droop Control. In Proceedings of the 2019 4th IEEE Workshop on the Electronic Grid (eGRID), Xiamen, China, 11–14 November 2019; pp. 1–6. [Google Scholar] [CrossRef]












| Name | Value | |
|---|---|---|
| Grid | Grid voltage (peak value), VgN | 80 V |
| Grid frequency, ω0 | 100π rad/s | |
| Short circuit ratio, SCR | 1.8 | |
| R/X ratio of grid impedance, Rg/Xg | 0.01 | |
| Grid impedance, Rg, Lg | 0.0267 Ω, 8.5 mH | |
| GCC | Rated power of inverter, SN | 2000 VA |
| Rated voltage (peak value), VoN | 80 V | |
| Rated current (peak value), IoN | 16.7 A | |
| DC-bus voltage, Vdc | 300 V | |
| Switching frequency, fsw = 1/Tsw | 20 kHz | |
| Filter inductor impedance, Rf, Lf, | 0.1 Ω, 1 mH | |
| The gain of active power synchronization, KAPS | 0.008 | |
| The bandwidth of the current PI controller, ωc | 2576 rad/s | |
| Parameters of the current PI controller, KpI = ωcLf, KiI = ωc2Lf/10 | 2.58, 663.6 |
| Name | Value | |
|---|---|---|
| PLL | KpPLL1 and KiPLL1 | 1.35 and 72.8 |
| SOGI-PLL | KSOGI, ωSOGI,KpPLL2 and KiPLL2 | 0.7, 314 rad/s, 2.48 and 246.5 |
| PI-APS | KAPS, KpAux1 and KiAux1 | 0.008, 1.64 and 43.09 |
| DP-APS | KAPS, Rv, ωLPF and KiAux2 | 0.008, 4.05 Ω, 314 rad/s and 43.69 |
| Name of Synchronization Unit | Phase Margin | Overshoot Percentage |
|---|---|---|
| PLL | Unstable | 30% |
| SOGI-PLL | Unstable | 27% |
| PI-APS | Critically stable | 15% |
| DP-APS | Stable | 6% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, T.; Wu, C.; Wang, Y. Elimination of Static Angular Error and Stability Enhancement for Active Power-Synchronized Converter Under a Weak Grid. Electronics 2025, 14, 1781. https://doi.org/10.3390/electronics14091781
Zhao T, Wu C, Wang Y. Elimination of Static Angular Error and Stability Enhancement for Active Power-Synchronized Converter Under a Weak Grid. Electronics. 2025; 14(9):1781. https://doi.org/10.3390/electronics14091781
Chicago/Turabian StyleZhao, Tong, Chao Wu, and Yong Wang. 2025. "Elimination of Static Angular Error and Stability Enhancement for Active Power-Synchronized Converter Under a Weak Grid" Electronics 14, no. 9: 1781. https://doi.org/10.3390/electronics14091781
APA StyleZhao, T., Wu, C., & Wang, Y. (2025). Elimination of Static Angular Error and Stability Enhancement for Active Power-Synchronized Converter Under a Weak Grid. Electronics, 14(9), 1781. https://doi.org/10.3390/electronics14091781






