An Integrated Ultra-Wideband Balun Topology and Its Application in Mixer Design †
Abstract
:1. Introduction
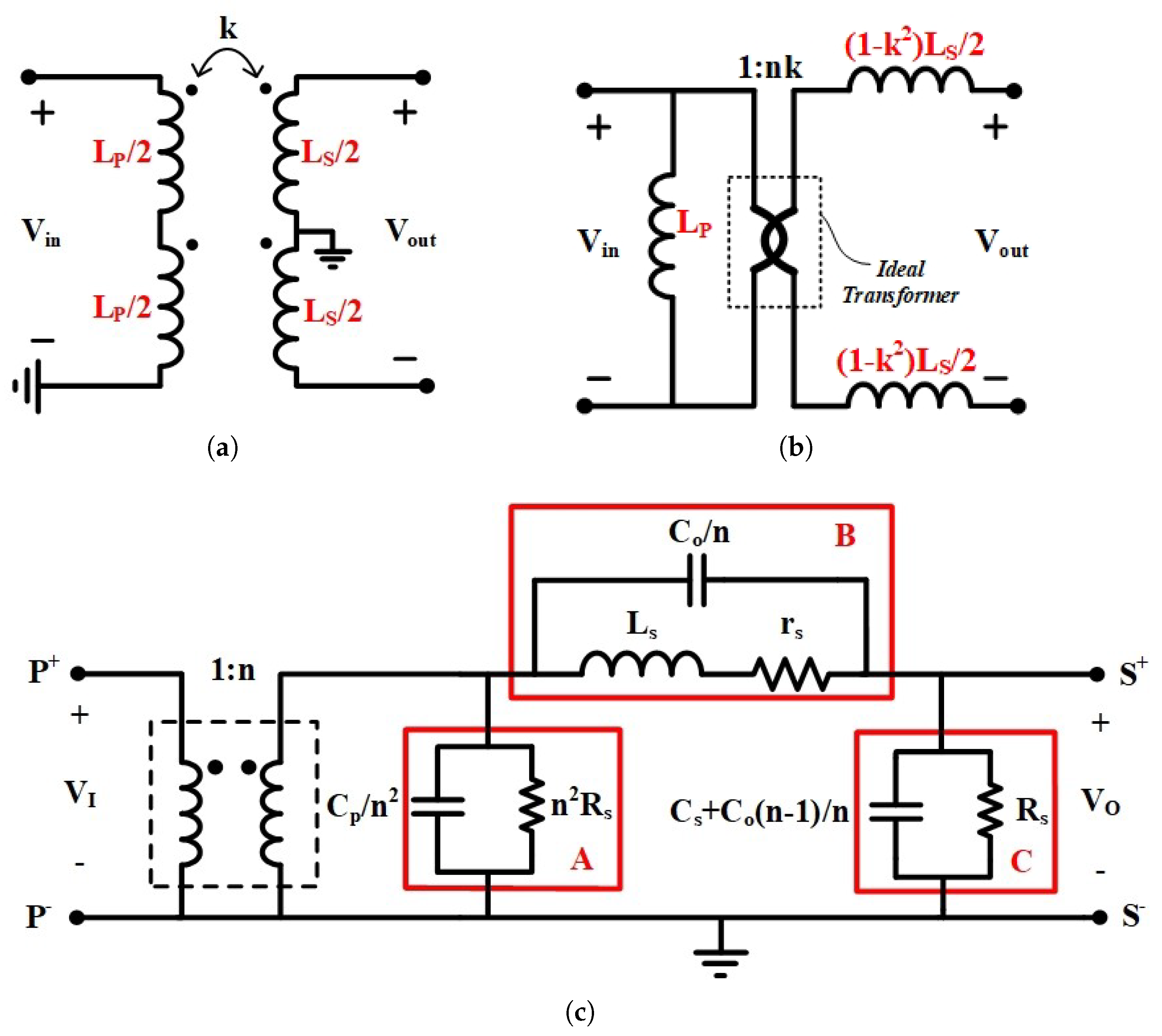
2. Theroretical Analysis
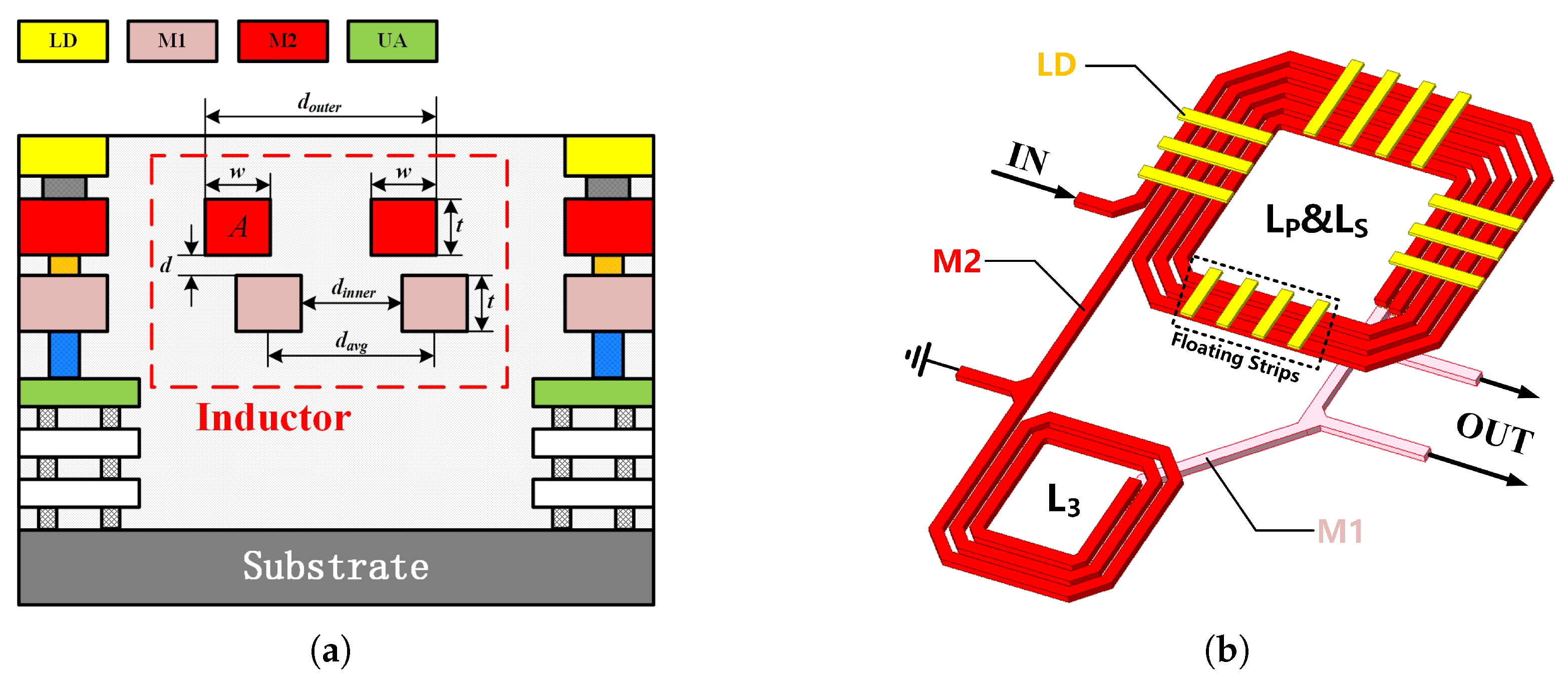
2.1. Integrated Transformer
2.2. Voltage Transformer Balun
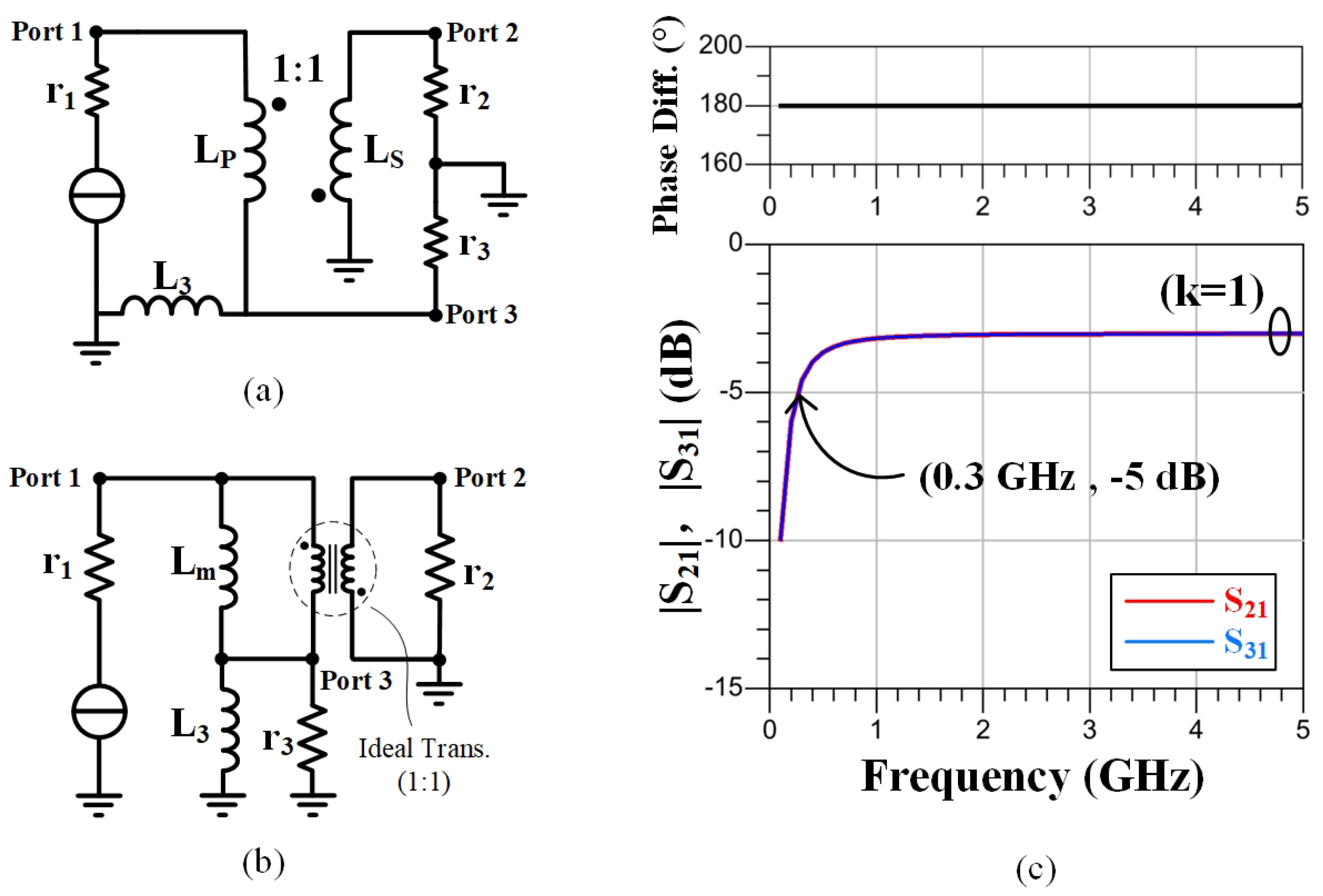
2.3. Current Transformer Balun
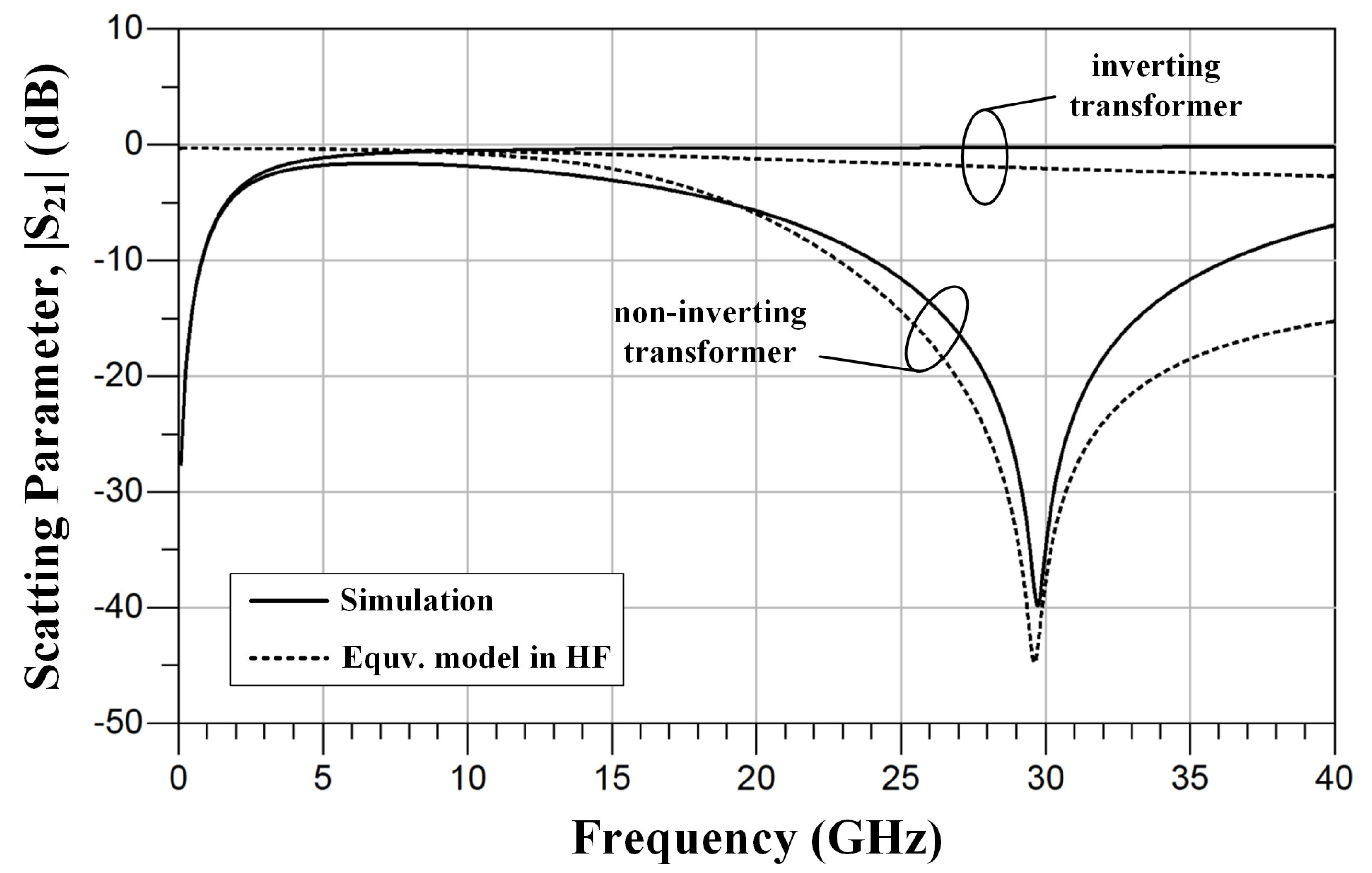
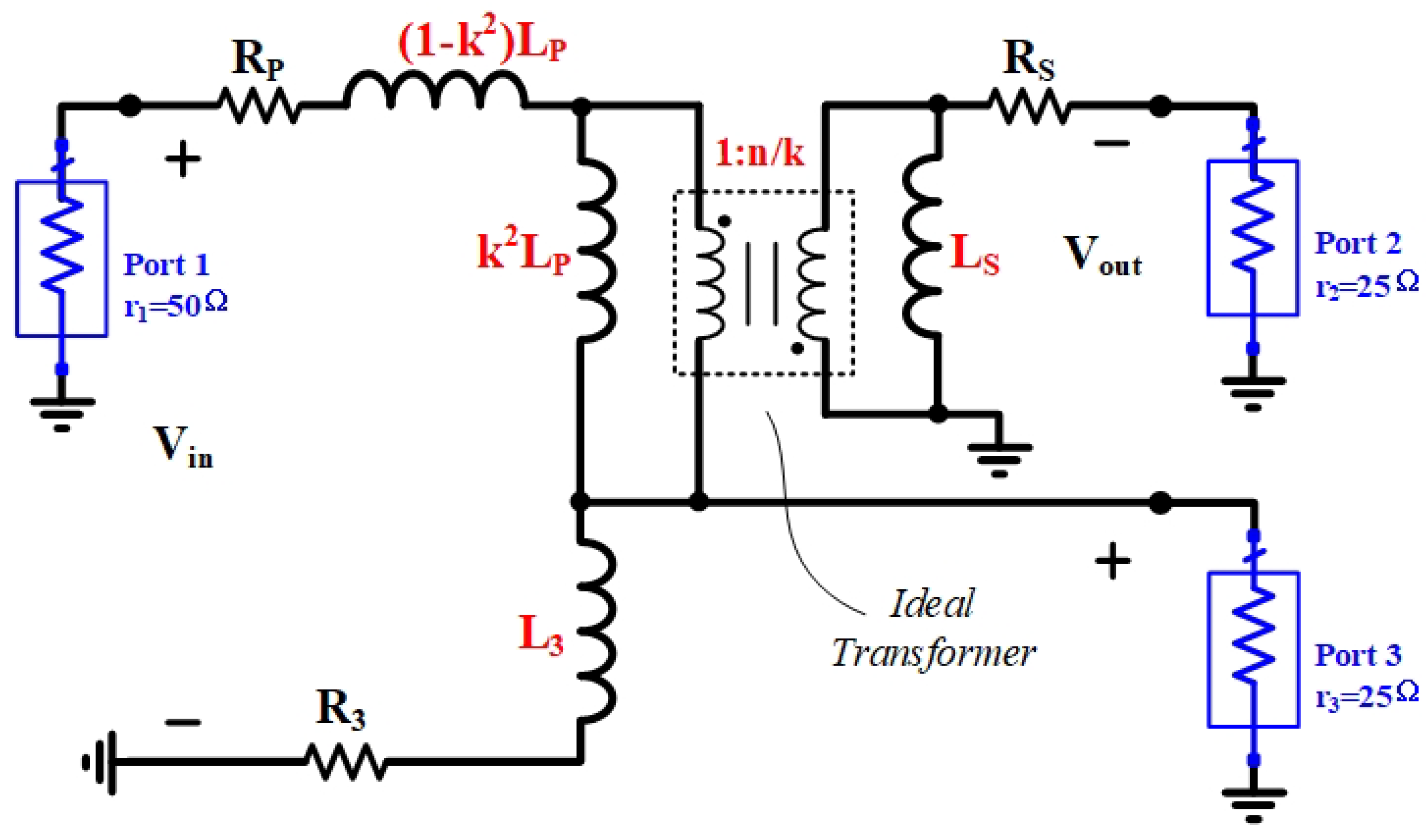
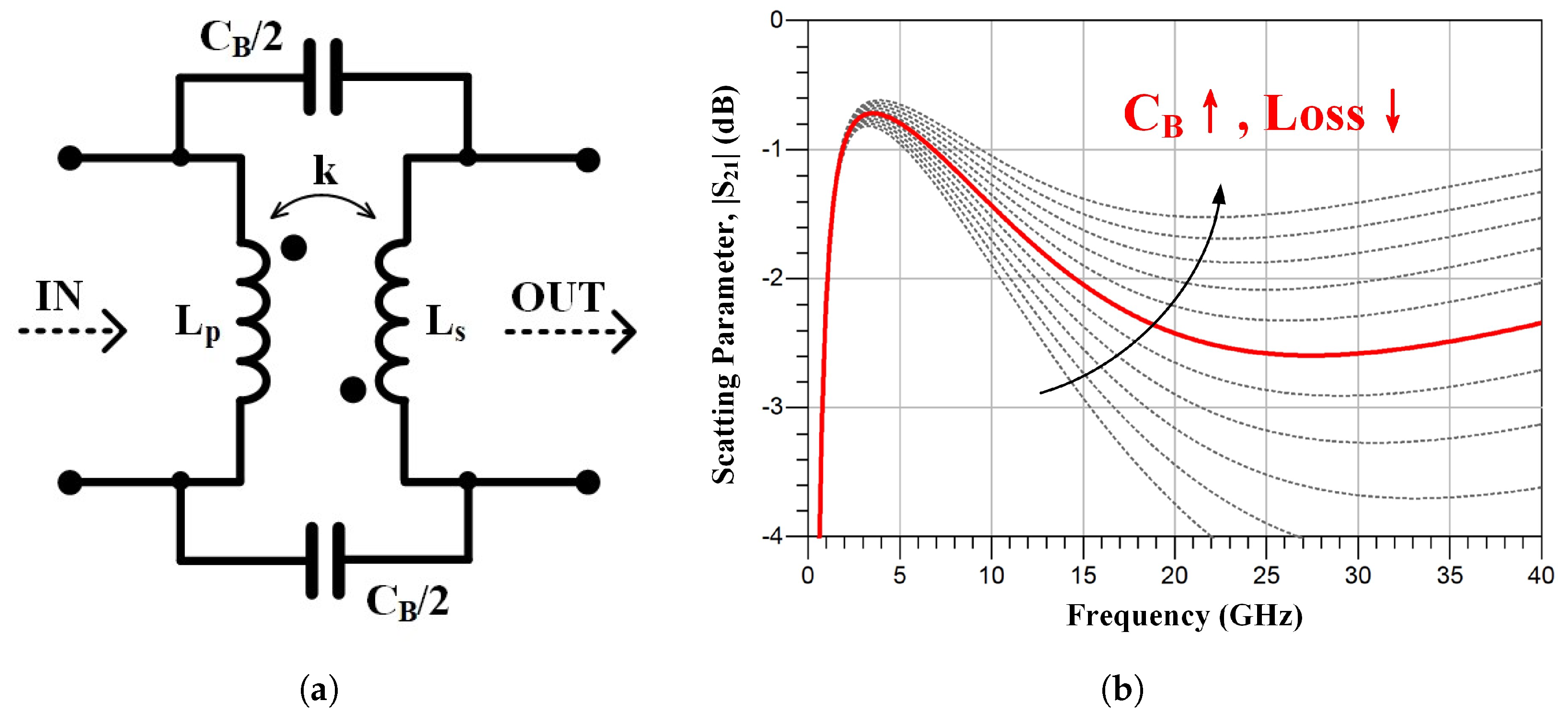
2.4. Ultra-Wideband Balun
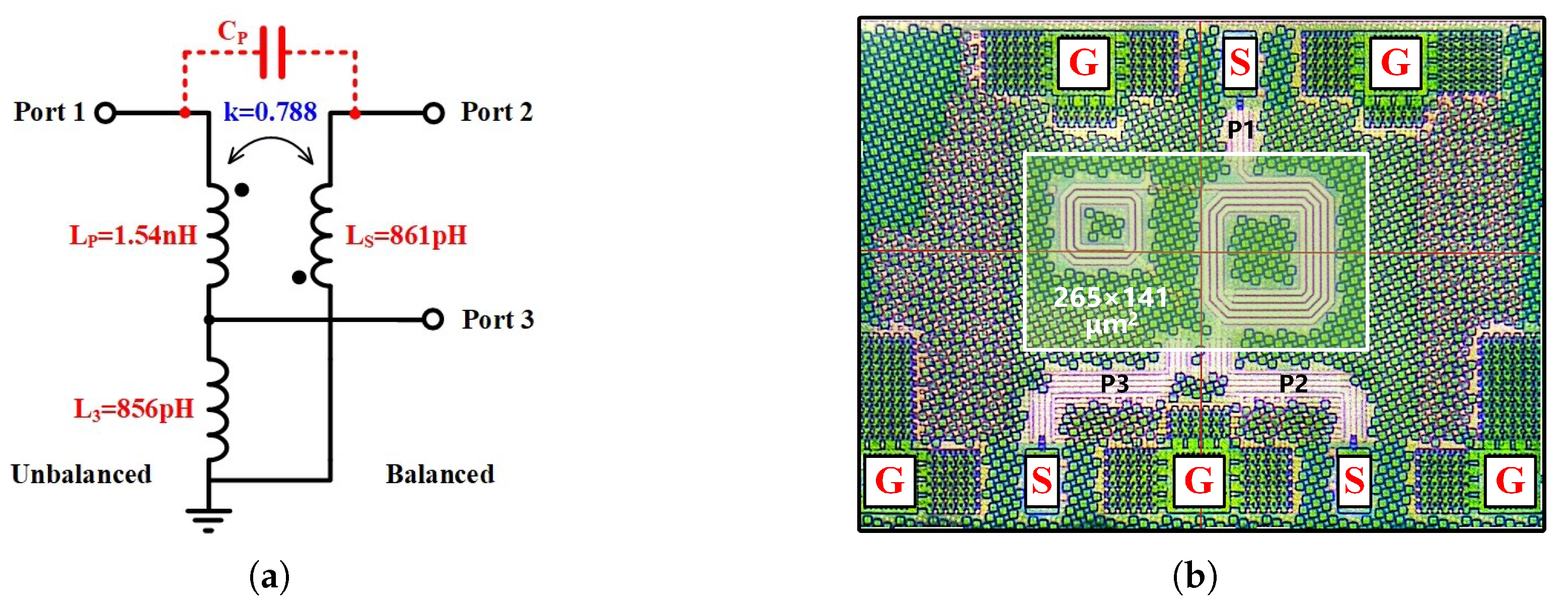
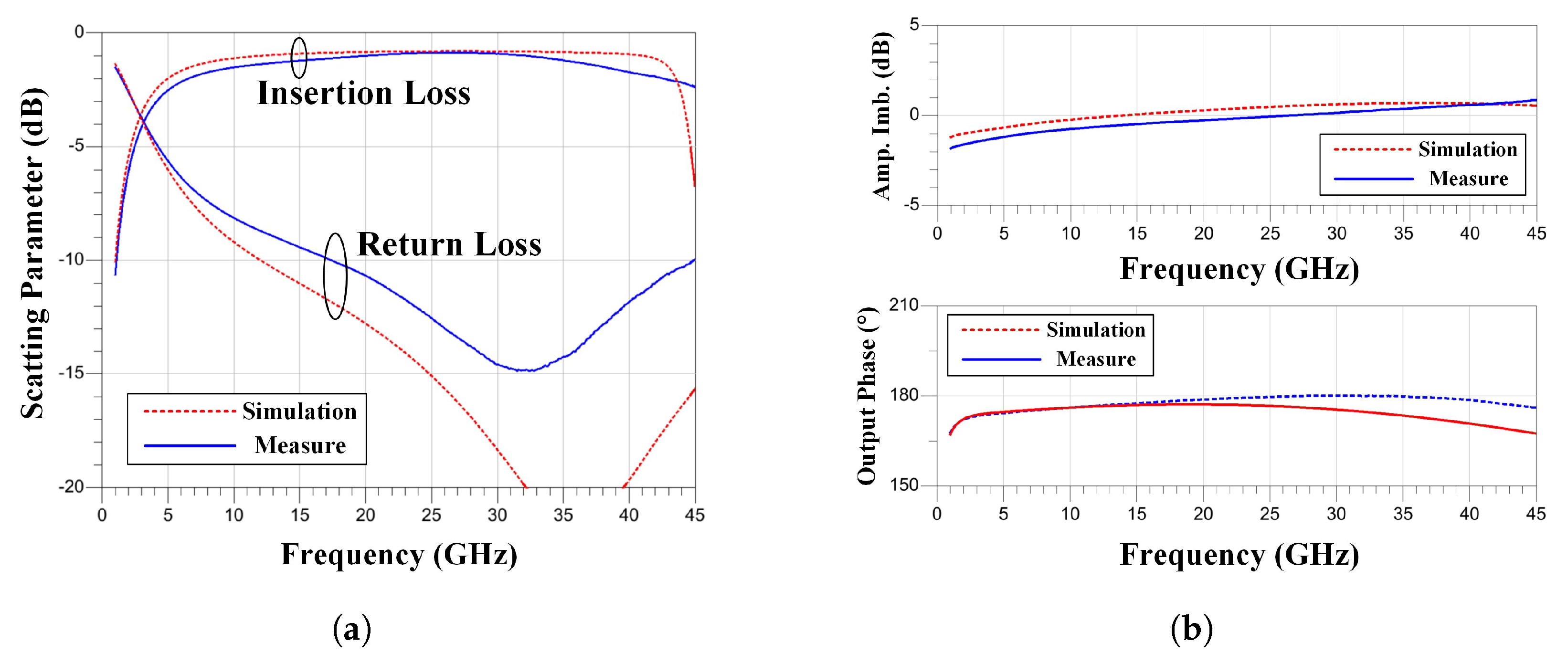
3. Balun Design, Simulation, and Measurement
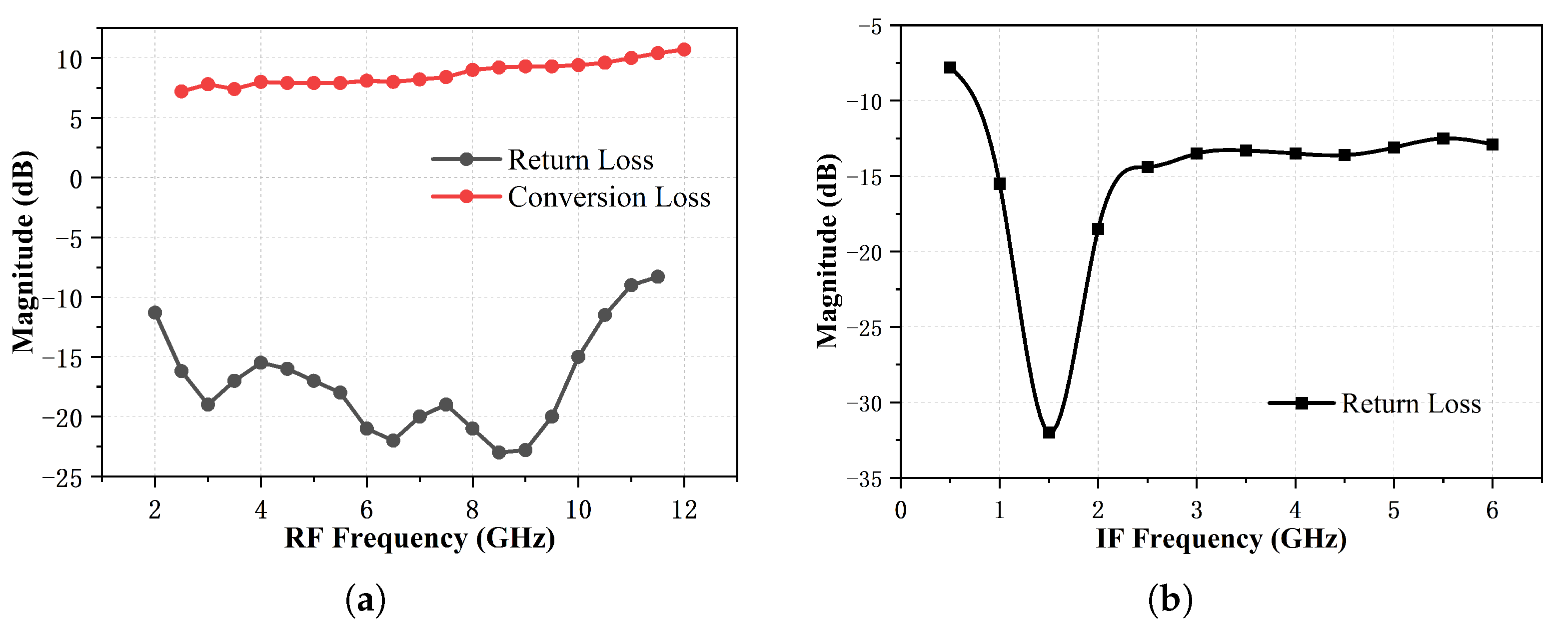
4. Mixer with an Ultra-Wideband Balun
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ma, S.; Wu, T.; Zhang, J.; Ren, J. A 5G Wireless Event-Driven Sensor Chip for Online Power-Line Disturbances Detecting Network in 0.25 μm GaAs Process. IEEE Trans. Ind. Electron. 2021, 68, 5271–5280. [Google Scholar] [CrossRef]
- Chakraborty, S.; Milner, L.E.; Zhu, X.; Hall, L.T.; Sevimli, O.; Heimlich, M.C. A K-Band Frequency Doubler with 35-dB Fundamental Rejection Based on Novel Transformer Balun in 0.13-μm SiGe Technology. IEEE Electron Device Lett. 2016, 37, 1375–1378. [Google Scholar] [CrossRef]
- Chakraborty, S.; Milner, L.E.; Zhu, X.; Sevimli, O.; Parker, A.E.; Heimlich, M.C. An Edge-Coupled Marchand Balun With Partial Ground for Excellent Balance in 0.13 μm SiGe Technology. IEEE Trans. Circuits Syst. II Express Briefs 2021, 68, 226–230. [Google Scholar] [CrossRef]
- Huang, C.H.; Chen, C.H.; Horng, T.S. Design of integrated planar Marchand balun using physical transformer model. In Proceedings of the 2009 Asia Pacific Microwave Conference, Singapore, 7–10 December 2009; pp. 1004–1007. [Google Scholar] [CrossRef]
- Tseng, S.C.; Meng, C.; Chang, C.H.; Wu, C.K.; Huang, G.W. Monolithic Broadband Gilbert Micromixer With an Integrated Marchand Balun Using Standard Silicon IC Process. IEEE Trans. Microw. Theory Tech. 2006, 54, 4362–4371. [Google Scholar] [CrossRef]
- Marchand, N. Transmission line conversion transformers. Electronics 1944, 17, 142–145. [Google Scholar]
- Deb, P.K.; Moyra, T.; Bhhattacharyya, B.K. Miniaturized and enhanced bandwidth Marchand Balun using CSRR. IET Microw. Antennas Propag. 2021, 15, 788–796. [Google Scholar] [CrossRef]
- Costantini, A.; Lawrence, B.; Mahon, S.; Harvey, J.; McCulloch, G.; Bessemoulin, A. Broadband Active and Passive Balun Circuits: Functional Blocks for Modern Millimeter-Wave Radio Architectures. In Proceedings of the 2006 European Microwave Integrated Circuits Conference, Manchester, UK, 10–13 October 2006; pp. 421–424. [Google Scholar] [CrossRef]
- Chen, T.; Chang, K.; Wang, H.; Dow, G.; Liu, L.; Bui, S.; Lin, T. Broadband monolithic passive baluns and monolithic double-balanced mixer. In Proceedings of the 1991 IEEE MTT-S International Microwave Symposium Digest, Boston, MA, USA, 10–14 July 1991; Volume 2, pp. 861–864. [Google Scholar] [CrossRef]
- Dai, Y.S.; Li, X.; Zhu, D. Ultra compact wideband Balun design and realization based on LTC. J. Microw. 2014, 30, 4. [Google Scholar]
- Wu, B.; Ji, Y.; Fang, G. An improved ultra wide-bandwidth planar Balun. J. Univ. Chin. Acad. Sci. 2010, 27, 507–511. [Google Scholar]
- Lin, Z.; Zhang, S.; Jia, D.; Liang, X.; Deng, J.; Nian, F. Design of a 35-95 GHz fundamental monolithic mixer based on a novel IF extraction structure. IEICE Electron. Express 2019, 16, 20190602. [Google Scholar] [CrossRef]
- Wang, X. Novel Broadband Balun Structures and Their Applications. Chinese Patent CN116799463A; granted, 15 September 2023. [Google Scholar]
- Zhu, X.; Xu, H.; Xia, T.; Wang, X. Invited Paper: Comparison and Analysis of on-Chip Broadband Integrated Balun. In Proceedings of the 2024 IEEE 67th International Midwest Symposium on Circuits and Systems (MWSCAS), Springfield, MA, USA, 11–14 August 2024; pp. 152–156. [Google Scholar] [CrossRef]
- Long, J. Monolithic transformers for silicon RF IC design. IEEE J. Solid-State Circuits 2000, 35, 1368–1382. [Google Scholar] [CrossRef]
- Jurgen, R.K. Ideal Transformers and Coupled Inductors. In Linear Circuits; Addison-Wesley: Reading, MA, USA, 1982; Chapter 5. [Google Scholar]
- Pozar, D.M. Microwave Engineering, 4th ed.; Original English edition; John Wiley & Sons: Hoboken, NJ, USA, 2011; p. 752. [Google Scholar]
- Que, X.; Jiang, L.; Wang, Y. A Novel Compact Uniplanar Isolation Circuit for Three-Port Baluns. In Proceedings of the 2024 IEEE/MTT-S International Microwave Symposium—IMS 2024, Washington, DC, USA, 16–21 June 2024; pp. 289–292. [Google Scholar] [CrossRef]
- Sevick, J.; W2FMI. More On The 1:1 Balun. CQ Amateur Radio 1994, 50, 26–46. [Google Scholar]
- Yu, W.; Sengal, U. Demystifying RF Transformers. Microw. J. 2020, 62, 52. [Google Scholar]
- Yang, Y.; Wu, Y.; Zhuang, Z.; Kong, M.; Wang, W.; Wang, C. An Ultraminiaturized Bandpass Filtering Marchand Balun Chip With Spiral Coupled Lines Based on GaAs Integrated Passive Device Technology. IEEE Trans. Plasma Sci. 2020, 48, 3067–3075. [Google Scholar] [CrossRef]
- Yang, G.; Wang, K.; Wang, Z. 20.8-51 GHz highly balanced CMOS Balun. Electron. Lett. 2017, 53, 1202–1203. [Google Scholar] [CrossRef]
- Chakraborty, S.; Milner, L.E.; Zhu, X.; Parker, A.; Heimlich, M. Analysis and Comparison of Marchand and Transformer Baluns Applied in GaAs. IEEE Trans. Circuits Syst. II Express Briefs 2022, 69, 4278–4282. [Google Scholar] [CrossRef]
- Chang, C.F.; Lin, Y.S. On-chip transformer-coupled balun bandpass filter for 5-GHz applications. In Proceedings of the 2015 IEEE MTT-S International Microwave Symposium, Phoenix, AZ, USA, 17–22 May 2015; pp. 1–4. [Google Scholar] [CrossRef]
- Li, Y.; Zhu, X.W.; Tian, L.; Liu, R.J. Compact 2-18 GHz on-chip Balun based on centrosymmetric spiral transformers. Electron. Lett. 2020, 56, 229–230. [Google Scholar] [CrossRef]
- Wang, X.; Beckwith, W.; Schiltz, T. Broadband Integrated RF/Microwave/Millimeter Mixer with Integrated Balun(s). U.S. Patent US9,312,815; granted, 12 April 2016. [Google Scholar]
- Analog Devices, Inc. LTC5549 Data Sheet; Analog Devices, Inc.: Wilmington, MA, USA, 2020; Available online: https://www.analog.com/media/en/technical-documentation/data-sheets/5549fa.pdf (accessed on 1 June 2024).
- Chakraborty, S.; Milner, L.E.; Parker, A.; Heimlich, M. A Folded Edge-Coupled Transformer Balun in GaAs with Excellent Balance from 9 to 20 GHz. In Proceedings of the 2018 48th European Microwave Conference (EuMC), Madrid, Spain, 23–27 September 2018; pp. 1101–1104. [Google Scholar] [CrossRef]

| Reference | Amp. Imb. [dB] | Phase Imb. [deg] | Insertion Loss [dB] | Bandwidth [GHz] | Relative Bandwidth | Area [mm2] | Technology | Topology |
|---|---|---|---|---|---|---|---|---|
| [21] | <0.35 | <5.26 | 1.5 | 2.39∼5.68 | 81.5% | 2.07 | IPD | MB |
| [22] | <0.3 | <2 | 2 | 20.8∼51 | 84.1% | 0.043 | 0.18 m CMOS | MB |
| [23] | <0.7 | <1.2 | 1.2 | 30∼65 | 73.7% | 0.12 | 0.1 m GaAs | MB |
| [24] | <0.63 | <3.7 | 2.5 | 4.6∼6.1 | 28% | 1 ** | GaAs | TB |
| [25] | <1 | <3 | 3 | 2∼18 | 160% | 0.21 | 1P2M GaAs | TB |
| [23] | <0.2 | <0.9 | 1.2 | 28.5∼46.5 | 45% | 0.06 | 0.1 m GaAs | TB |
| This Work | <1.01 | <5 | 1.2 | 4.1∼45+s | 167% | 0.037 | 45 nm RFE | / |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, X.; Xu, H.; Beckwith, B.; Wang, X. An Integrated Ultra-Wideband Balun Topology and Its Application in Mixer Design. Electronics 2025, 14, 1631. https://doi.org/10.3390/electronics14081631
Zhu X, Xu H, Beckwith B, Wang X. An Integrated Ultra-Wideband Balun Topology and Its Application in Mixer Design. Electronics. 2025; 14(8):1631. https://doi.org/10.3390/electronics14081631
Chicago/Turabian StyleZhu, Xu, Hanzhong Xu, Bill Beckwith, and Xudong Wang. 2025. "An Integrated Ultra-Wideband Balun Topology and Its Application in Mixer Design" Electronics 14, no. 8: 1631. https://doi.org/10.3390/electronics14081631
APA StyleZhu, X., Xu, H., Beckwith, B., & Wang, X. (2025). An Integrated Ultra-Wideband Balun Topology and Its Application in Mixer Design. Electronics, 14(8), 1631. https://doi.org/10.3390/electronics14081631






