The Pole-to-Ground Fault Current Calculation Method and Impact Factor Investigation for Monopole DC Grids
Abstract
1. Introduction
- (1)
- An accurate pole-to-ground fault current calculation method in multi-terminal monopole DC grids is proposed, based on a simplified RLC-equivalent model, where the accuracy and calculation efficiency can be both guaranteed.
- (2)
- Based on the proposed method, varied components and their parameters’ impact on pole-to-ground fault currents have been investigated. And the most influential components are also discovered.
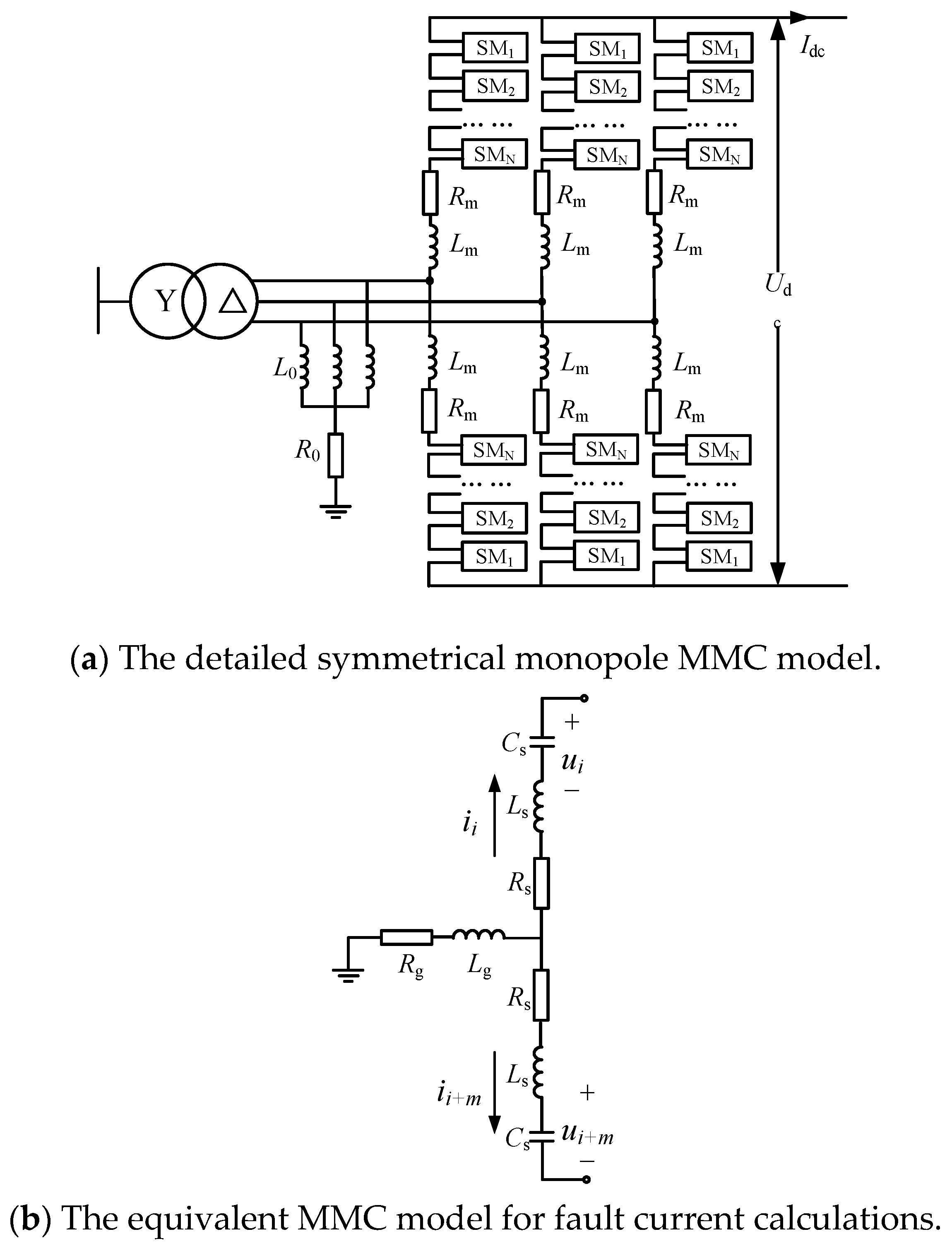
2. The Pole-to-Ground Fault Characteristic in Symmetrical Monopole DC System and Equivalent Circuit
2.1. Symmetrical Monopole DC Converter
2.2. The Equivalent Model for Symmetrical Monopole Converter
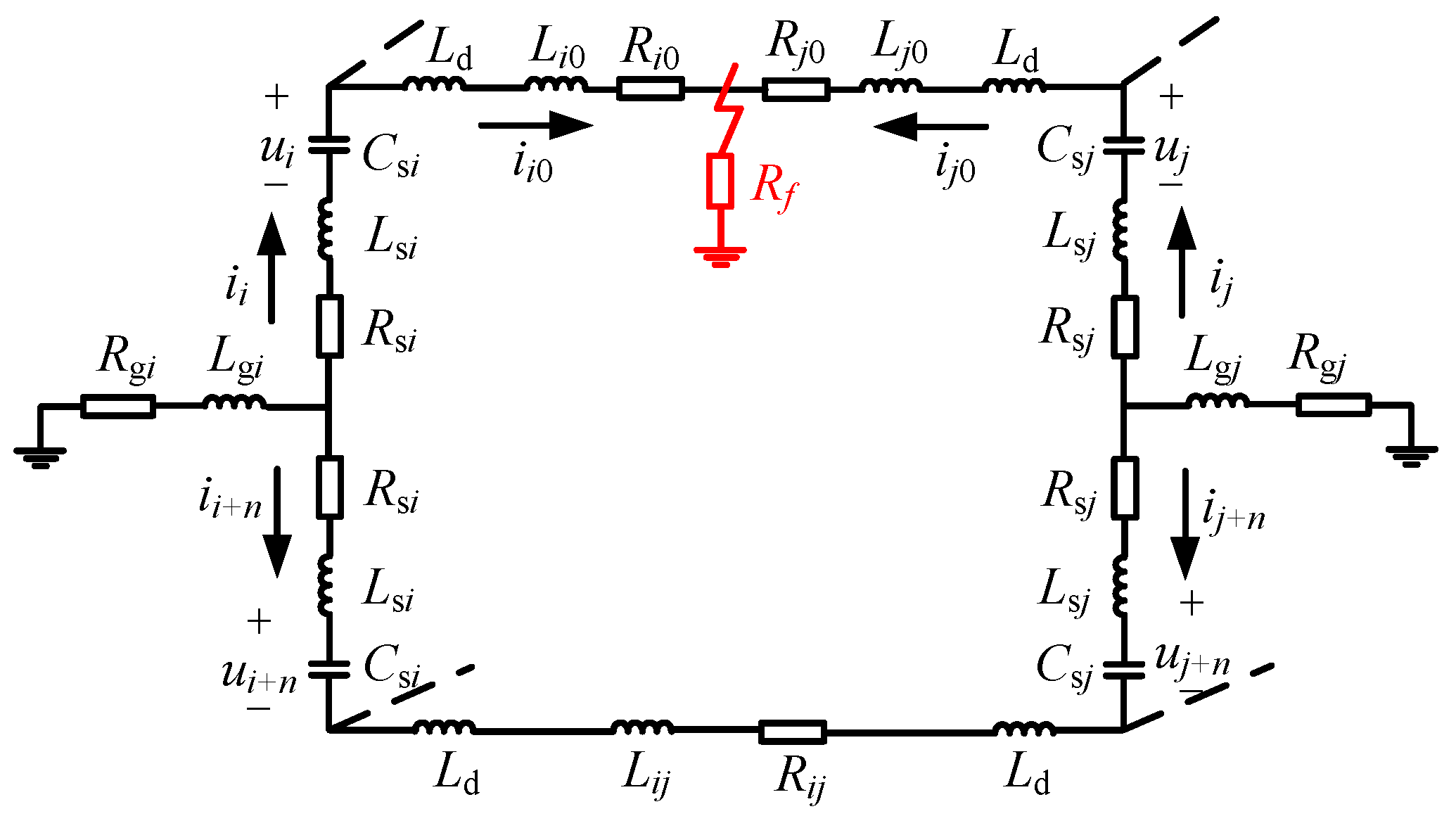
3. The Fault Current Calculation Method for Symmetrical Monopole DC Grids
3.1. The Calculation Model Based on the Equivalent Model
3.2. Mathematical Equations Before Fault
3.3. The Revised Mathematical Equations After Fault
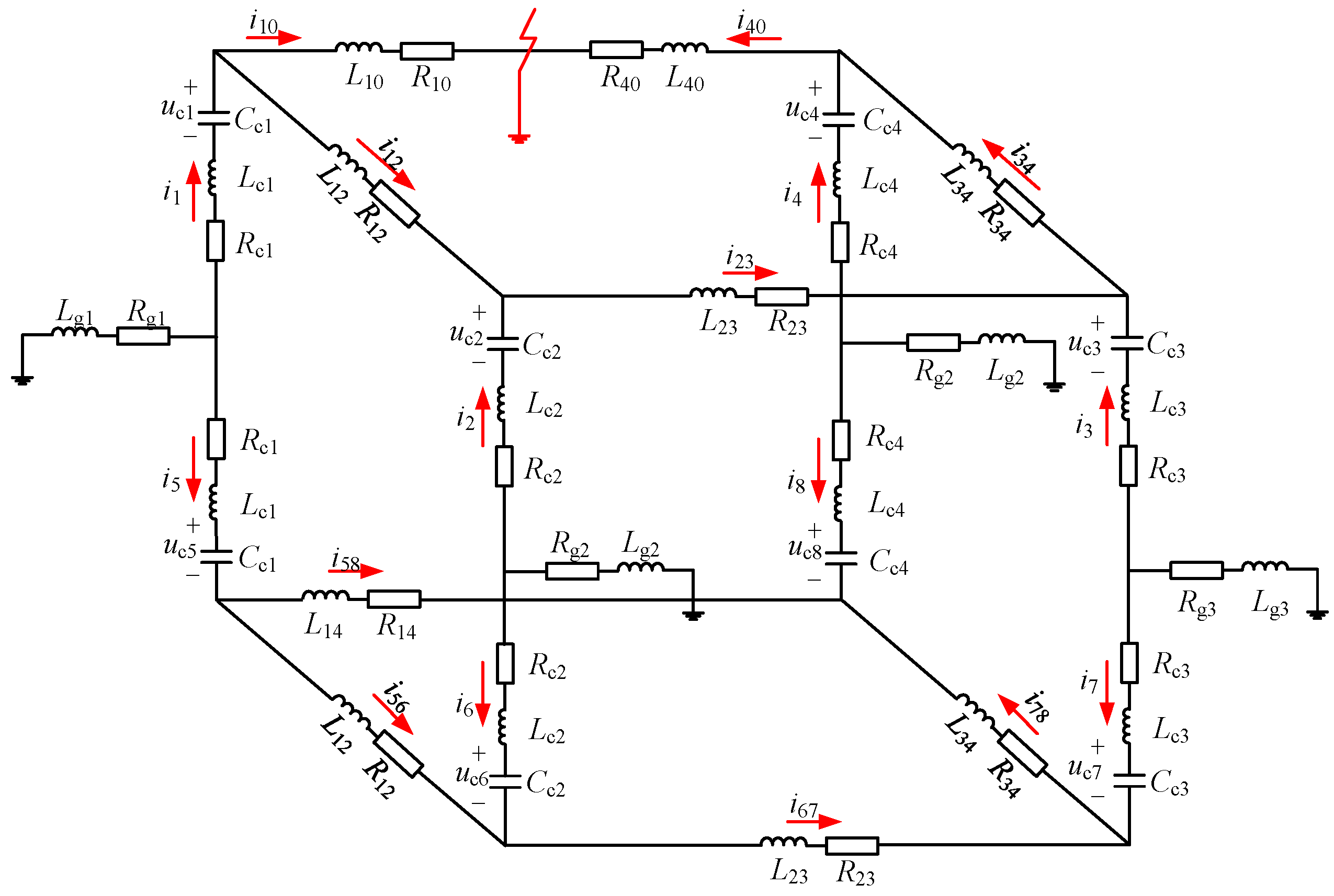
4. Verification of Pole-to-Ground Fault Current Calculation in Monopole DC Grid
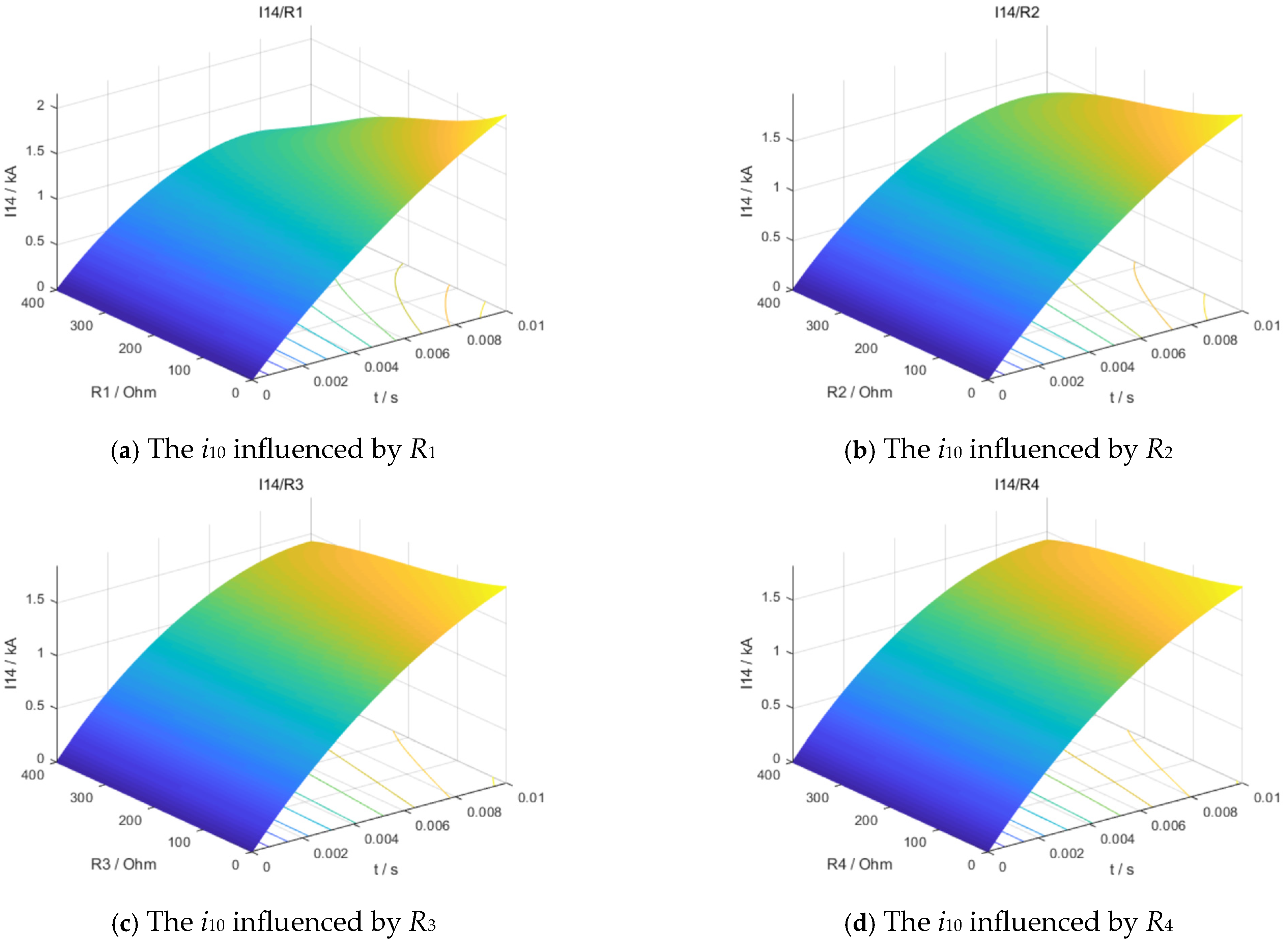
5. Pole-to-Ground Fault Current Impact Factors Investigation
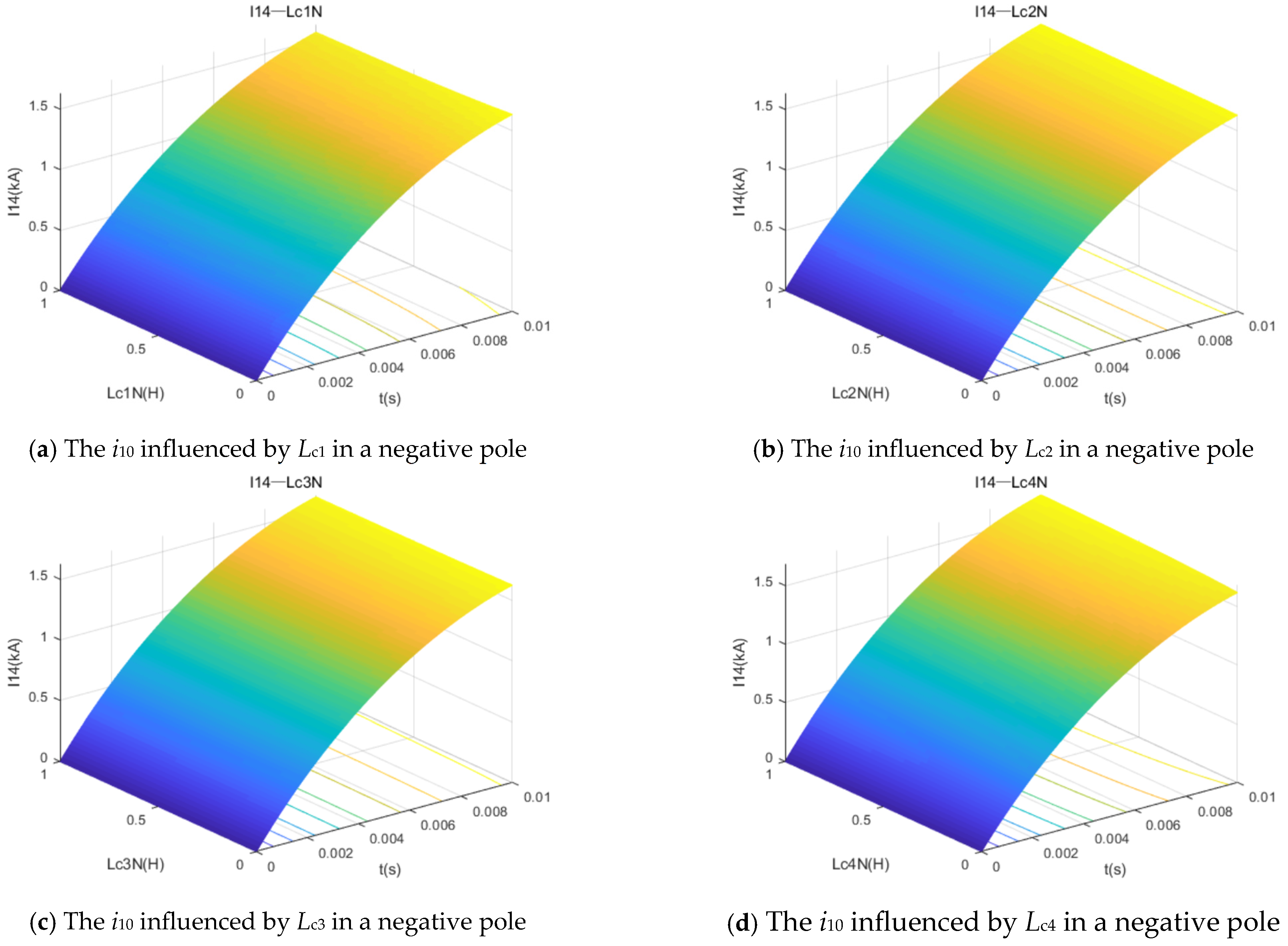
5.1. The DC Line Parameters’ Impact Analysis
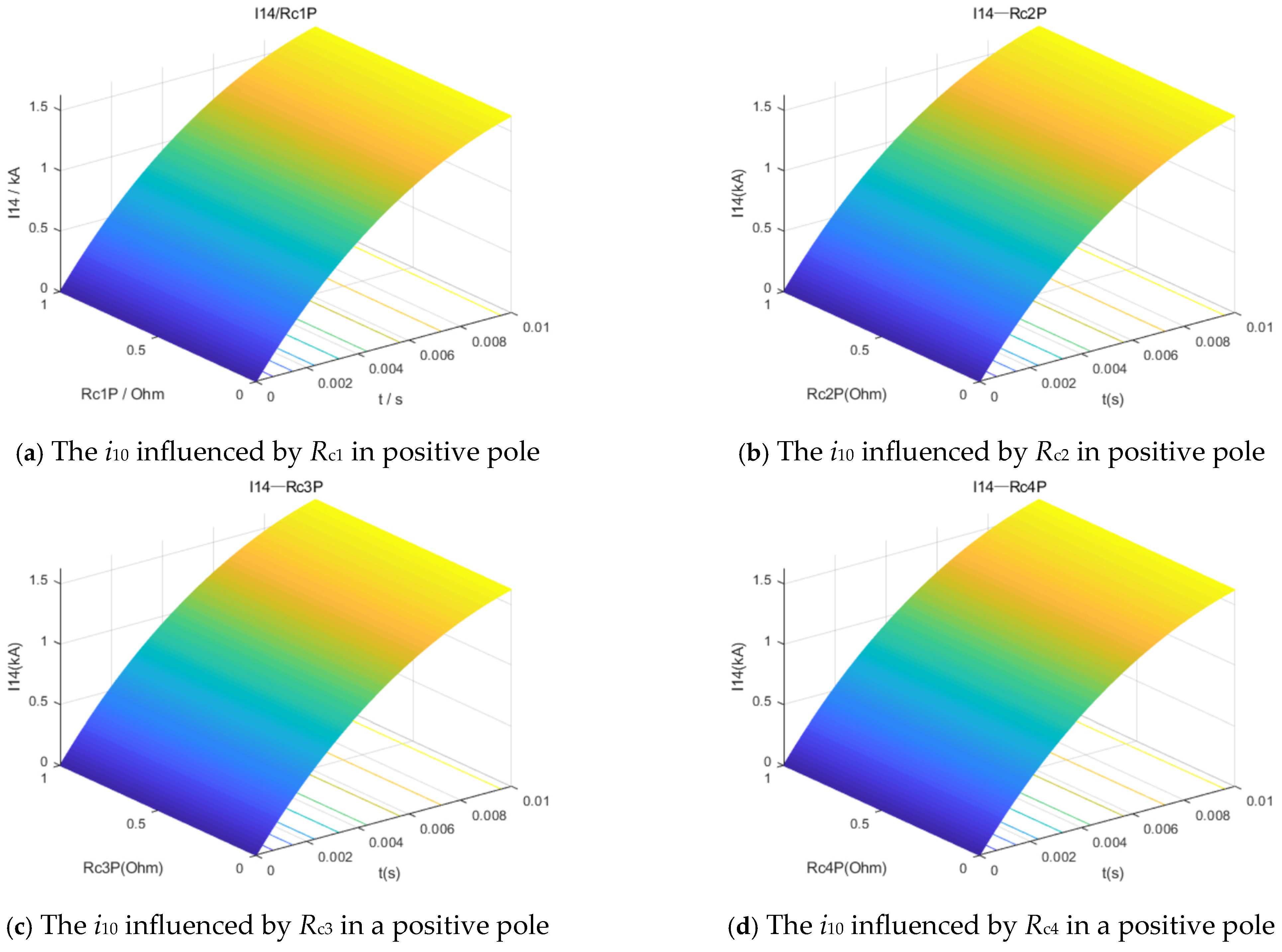
5.2. The Grounding Electrode Parameters’ Impact Analysis
5.3. The Converter Parameters’ Impact Analysis
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhou, H.; Li, B.; Jiang, Q.; Liu, T.; Zhang, Y.; Yin, Y. The Extreme Temperature Weather Impact Mechanism Analysis of MMC-HVDC’s Harmonic Impedance and Its Dynamic Stability. Energies 2024, 17, 6044. [Google Scholar] [CrossRef]
- Jiang, Q.; Zeng, X.; Li, B.; Wang, S.; Liu, T.; Chen, Z.; Wang, T.; Zhang, M. Time-sharing frequency coordinated control strategy for PMSG-based wind turbine. IEEE J. Emerg. Sel. Top. Circuits Syst. 2022, 12, 268–278. [Google Scholar] [CrossRef]
- Verma, N.; Kumar, N.; Gupta, S.; Malik, H.; Márquez, F.P.G. Review of sub-synchronous interaction in wind integrated power systems: Classification, challenges, and mitigation techniques. Prot. Control. Mod. Power Syst. 2023, 8, 17. [Google Scholar] [CrossRef]
- Wang, P.; Jiang, Q.; Li, B.; Liu, T.; Li, X.; Chen, G.; Zeng, X.; Blaabjerg, F. Ultra-low frequency oscillation analysis considering thermal-hydro power proportion. Int. J. Electr. Power Energy Syst. 2023, 148, 108919. [Google Scholar] [CrossRef]
- Li, Q.; Li, B.; Jiang, Q.; Liu, T.; Yue, Y.; Zhang, Y. A Novel Location Method for Interline Power Flow Controllers Based on Entropy Theory. Prot. Control. Mod. Power Syst. 2024, 9, 70–81. [Google Scholar] [CrossRef]
- Roy, T.K.; Ghosh, S.K.; Saha, S. Robust Backstepping Global Integral Terminal Sliding Mode Controller to Enhance Dynamic Stability of Hybrid AC/DC Microgrids. Prot. Control. Mod. Power Syst. 2023, 8, 8. [Google Scholar] [CrossRef]
- Kok, C.L.; Tang, H.; Teo, T.H.; Koh, Y.Y. A DC-DC Converter with Switched-Capacitor Delay Deadtime Controller and Enhanced Unbalanced-Input Pair Zero-Current Detector to Boost Power Efficiency. Electronics 2024, 13, 1237. [Google Scholar] [CrossRef]
- Yu, J.; Li, Y.; Wan, J.; Zhou, F.; Zhang, M.; Cao, L. Tri-State Modulation with Operating Losses Minimization for a Soft-Switching Bidirectional DC-DC Converter. Prot. Control. Mod. Power Syst. 2024, 9, 58–70. [Google Scholar] [CrossRef]
- Jiang, Q.; Tao, Y.; Li, B.; Liu, T.; Chen, Z.; Blaabjerg, F.; Wang, P. Joint Limiting Control Strategy Based on Virtual Impedance Shaping for Suppressing DC Fault Current and Arm Current in MMC-HVDC Systems. J. Mod. Power Syst. Clean Energy 2023, 11, 2003–2014. [Google Scholar] [CrossRef]
- Pang, H.; Wei, X. Research on key technology and equipment for Zhangbei 500kV DC grid. In Proceedings of the 2018 International Power Electronics Conference (IPEC-Niigata 2018-ECCE Asia), Niigata, Japan, 20–24 May 2018; IEEE: Piscataway, NJ, USA, 2018. [Google Scholar]
- Zhang, Y.; Zhang, W.; Peng, Q.; Li, B.; Tao, Y.; Zhang, M.; Liu, T.; Blaabjerg, F. Impact of grid topology on pole-to-ground fault current in bipolar DC grids: Mechanism and evaluation. J. Mod. Power Syst. Clean Energy 2022, 11, 434–445. [Google Scholar] [CrossRef]
- Han, X.; Jiang, Q.; Liu, T.; Li, B.; Ding, L.; Chen, G. Research on ultra-low frequency oscillation caused by hydro power in hydro-dominant power system. In Proceedings of the 2018 International Conference on Power System Technology (POWERCON), Guangzhou, China, 6–9 November 2018; IEEE: Piscataway, NJ, USA, 2018; pp. 1909–1914. [Google Scholar]
- Tao, Y.; Li, B.; Liu, T.; Jiang, Q.; Blaabjerg, F. Practical Fault Current Level Evaluation and Limiting Method of Bipolar HVdc Grid Based on Topology Optimization. IEEE Syst. J. 2021, 16, 4466–4476. [Google Scholar] [CrossRef]
- Zhang, L.; Zou, Y.; Yu, J.; Qin, J.; Vittal, V.; Karady, G.G.; Shi, D.; Wang, Z. Modeling, control, and protection of modular multilevel converter-based multi-terminal HVDC systems: A review. CSEE J. Power Energy Syst. 2017, 3, 340–352. [Google Scholar] [CrossRef]
- Hou, J.; Song, G.; Fan, Y. Fault identification scheme for protection and adaptive reclosing in a hybrid multi-terminal HVDC system. Prot. Control. Mod. Power Syst. 2023, 8, 23. [Google Scholar] [CrossRef]
- Li, C.; Zhao, C.; Xu, J.; Ji, Y.; Zhang, F.; An, T. A Pole-to-Pole Short-Circuit Fault Current Calculation Method for DC Grids. IEEE Trans. Power Syst. 2017, 32, 4943–4953. [Google Scholar] [CrossRef]
- Liu, Y.; Huang, M.; Zha, X.; Iu, H.H. Short-Circuit Current Estimation of Modular Multilevel Converter Using Discrete-Time Modeling. IEEE Trans. Power Electron. 2019, 34, 40–45. [Google Scholar] [CrossRef]
- Adam, G.P.; Abdelsalam, I.; Fletcher, J.E.; Burt, G.M.; Holliday, D.; Finney, S.J. New Efficient Submodule for a Modular Multilevel Converter in Multiterminal HVDC Networks. IEEE Trans. Power Electron. 2017, 32, 4258–4278. [Google Scholar] [CrossRef]
- Etxegarai, A.; Larruskain, D.M.; Iturregi, A.; Saldaña, G.; Apiñaniz, S. Performance of a superconducting breaker for the protection of HVDC grids. IET Gener. Transm. Distrib. 2020, 14, 997–1004. [Google Scholar] [CrossRef]
- Yao, L.Z.; Yao, Z.Q.; Lin, Z. Research on DC Fault Characteristics of Modular Multilevel Converter-Based High-Voltage DC Transformer. Power Syst. Technol. 2016, 40, 1051–1058. [Google Scholar]
- Wang, S.S.; Zhou, X.Y.; Tang, G.F.; He, Z.Y.; Teng, L.T.; Bao, H.L. Analysis of Submodule Overcurrent in Modular Multilevel Converter HVDC During DC Pole-to-Pole Short Circuit. Proc. CSEE (Chin. J. Electr. Eng.) 2011, 31, 7. [Google Scholar]
- Wang, W.R.; He, Z.Y.; Li, G.Q.; Xin, Y.C.; Gu, G.H. Recursive Calculation Method for DC Fault Current in MMC-HVDC Considering AC Influence. Proc. CSEE (Chin. J. Electr. Eng.) 2019, 39, 313–320. [Google Scholar]
- Yang, H.; Dong, Y.; Li, W.; He, X. Average-value model of modular multilevel converters considering capacitor voltage ripple. IEEE Trans. Power Deliv. 2016, 32, 723–732. [Google Scholar] [CrossRef]






| iip/i(i+m)(p+m) | ipi/i(p+m)(i+m) | ipj/i(p+m)(j+m) | ijp/i(j+m)(p+m) | ipq/i(p+m)(q+m) | iqp/i(q+m)(p+m) |
|---|---|---|---|---|---|
| Ri | −Ri | Rj | −Rj | 0 | 0 |
| iip | ipi | ipj | ijp | ipq | iqp |
|---|---|---|---|---|---|
| Rci | −Rci | Rcj | −Rcj | 0 | 0 |
| i(i+m)(p+m) | i(p+m)(i+m) | i(p+m)(j+m) | i(j+m)(p+m) | i(p+m)(q+m) | i(q+m)(p+m) |
|---|---|---|---|---|---|
| Rci | −Rci | Rcj | −Rcj | 0 | 0 |
| Converter No. | Converter Control Modes | Converter Parameters | |||
|---|---|---|---|---|---|
| L0 (mH) | R0 (Ω) | C0 (μF) | NSM | ||
| 1 | P = 750 MW Q = 0 Mvar | 100 | 0.2722 | 7500 | 200 |
| 2 | P = 1500 MW Q = 0 Mvar | 50 | 0.1816 | 15,000 | 200 |
| 3 | P = −1500 MW Q = 0 Mvar | 50 | 0.1816 | 15,000 | 200 |
| 4 | Udc = 500 kV | 100 | 1.089 | 7500 | 200 |
| Q = 0 Mvar | |||||
| Line No. | Length (km) | Resistance (Ω/km) | Inductance (mH/km) | Smooth Conductor Inductance (mH) |
|---|---|---|---|---|
| L12 | 100 | 0.0198 | 0.82 | 150 |
| L14 | 300 | 0.0198 | 0.82 | 150 |
| L23 | 400 | 0.0198 | 0.82 | 150 |
| L34 | 200 | 0.0198 | 0.82 | 150 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, L.; Yi, W.; Deng, P.; Ma, S.; Kuang, D.; Cai, H. The Pole-to-Ground Fault Current Calculation Method and Impact Factor Investigation for Monopole DC Grids. Electronics 2025, 14, 1067. https://doi.org/10.3390/electronics14061067
Chen L, Yi W, Deng P, Ma S, Kuang D, Cai H. The Pole-to-Ground Fault Current Calculation Method and Impact Factor Investigation for Monopole DC Grids. Electronics. 2025; 14(6):1067. https://doi.org/10.3390/electronics14061067
Chicago/Turabian StyleChen, Liang, Wei Yi, Pan Deng, Shen Ma, Da Kuang, and Hongyu Cai. 2025. "The Pole-to-Ground Fault Current Calculation Method and Impact Factor Investigation for Monopole DC Grids" Electronics 14, no. 6: 1067. https://doi.org/10.3390/electronics14061067
APA StyleChen, L., Yi, W., Deng, P., Ma, S., Kuang, D., & Cai, H. (2025). The Pole-to-Ground Fault Current Calculation Method and Impact Factor Investigation for Monopole DC Grids. Electronics, 14(6), 1067. https://doi.org/10.3390/electronics14061067





