Highly Robust Active Damping Approach for Grid-Connected Current Feedback Using Phase-Lead Compensation

Abstract

The grid-current-feedback active damping (GCFAD) strategy has been widely utilized in LCL-type cascaded H-bridge static var generator (SVG) systems. Although digital control in GCFAD enhances sampling accuracy, it introduces delays in the subsequent calculation and execution processes, thereby reducing the effective damping region and weakening the robustness of the LCL-type cascaded H-bridge SVG system under a broad domain of grid impedance changes. This paper expands the effective damping area from (0, f_R) (f_R ∈ (f_s/6, f_s/3)) to (0, 0.45f_s) by introducing a phase-lead compensator into the active damping feedback loop to reduce the delay. This reduces the negative effect of the digital control on the system’s effective damping region and significantly enhances its robustness under an extensive domain of grid impedance variations. The improved GCFAD is employed to establish the system’s discrete domain model and the controller parameters are adjusted in conjunction with the mentioned model. Simulation outcomes indicate the efficiency of the presented approach.

1. Introduction

The integration of nonlinear loads has exacerbated issues such as low power factor, harmonic pollution, and three-phase imbalances in the power grid, leading to a significant decline in power quality. The LCL-type cascaded H-bridge static var generator (SVG), a novel compensation device, is widely used in power systems for reactive power compensation, owing to its superior dynamic adjustment and harmonic suppression capabilities [1]. However, connecting the cascaded H-bridge SVG to the grid may induce high-frequency resonance due to its interaction with the LCL filter. Additionally, large variations in grid impedance can shift resonance frequencies, impairing system stability and distorting the output current [2,3,4]. In weak grid environments, such as those with high-penetration renewable energy or long-distance transmission systems characterized by low short-circuit ratios (SCR) or high impedance ratios (ZR), this resonance issue is particularly severe and poses a potential threat to the safe operation of power systems.

Traditional methods used to address the resonant problem of LCL filters involve passive and active damping. Passive damping [5,6,7,8], which suppresses resonance by introducing actual resistance, shows significant results, but increases power consumption and hardware costs. Active damping [9,10,11,12,13,14,15], especially the grid-current-feedback active damping (GCFAD) approach based on virtual resistance, has gradually become mainstream owing to its flexibility and lack of extra power consumption [16,17,18,19,20]. However, control delay narrows the effective damping region, leading to poor performance of the system in complex grid environments, where it struggles to cope with grid impedance variations. Studies on active damping strategies [9,10,11,12,13,14,15] indicate that the virtual resistance method simulates the damping effect of actual resistance by feeding back the appropriate state variables. While its flexibility enhances system stability, control delay remains an issue in digital systems. Control delay shrinks the effective damping region [18], making the system prone to instability under significant variations in the grid impedance.

Various studies have attempted to improve the GCFAD strategy to address the issue of control delay. A study [21] analyzed the impact of delay on virtual impedance and proposed an active damping critical value model, revealing the intrinsic relationship between control delay and system stability. However, it did not fully bring up the effect of grid impedance variations on the system’s resonant frequency, restricting its applicability when grid impedance undergoes significant variations. Some methods, like real-time sampling and multi-sampling techniques, have been proposed to reduce control delay by directly modifying the sampling method [22,23,24]. However, delays cannot be entirely eliminated due to computational speed limitations, and there is a risk of introducing aliasing and switching noise, which can affect system stability. Other studies [19,20] introduced a delay compensation loop within the active damping loop to extend the effective damping region, thus improving system stability. However, existing compensators have limited capacity, and the effective damping region extension is restricted. Besides, the system lacks robustness and adaptability, particularly in weak grid environments. Overall, since existing research has not effectively expanded the damping region of the system, stable operation cannot be ensured under significant variations in grid impedance. Further optimization is required to improve system robustness and adaptability.

To address the above problems, the current study presents a highly robust GCFAD approach using phase-lead compensation. Section 2 configures and models a typical cascaded H-bridge SVG system containing LCL filtering links and presents the system control structure using the conventional GCFAD strategy. Section 3 analyzes the adverse effect of digital control delay on the conventional GCFAD. Section 4 introduces a phase-lead compensator, whose optimal coefficient is derived, which is chosen as 0.95. The compensated GCFAD parameters are then redesigned and analyzed to guarantee the system’s stability. The compensator significantly expands the GCFAD system’s effective damping area from (0, f_R) (f_R ∈ (f_s/6, f_s/3)) to (0, 0.45f_s), thereby improving the system’s stability and robustness under a broad domain of grid impedance changes. Section 5 provides the simulation and comparative analysis outcomes to evaluate the capability of the improved robust GCFAD strategy to effectively suppress resonance and enhance the quality of grid-connected (GC) current.

2. Modeling of the LCL-Type Cascaded H-Bridge SVG System

2.1. Main Circuit Topology

Figure 1 presents the structure diagram of the cascaded H-bridge SVG system. The main circuit adopts a star structure, where H-bridge modules are cascaded to form three phases linked to the grid via an LCL-type filter. In Figure 1, u_pcck(k = a, b, c) describes the grid connection point voltage; u_sk(k = a, b, c) describes the grid voltage; L_gk(k = a, b, c) describes the grid inductance; the SVG side inductance L₁, the filter capacitor C, and the grid-side inductance L₂ form the LCL-type filter; i_1k(k = a, b, c) and i_2k(k = a, b, c) describe the currents of L₁ and L₂, respectively; u_ck(k = a, b, c) describes the filter capacitor voltage; u_dc represents the equivalent single H-bridge DC voltage of the three-phase DC side; C_dc represents the equivalent capacitance.

To more efficiently analyze the digital control delay, the following assumptions are made for system simplification. All power switches are assumed to be ideal switches, ignoring the effects of switching dead time. The inductors and capacitors in the filter are considered ideal components, excluding parasitic resistance. The grid voltage is assumed to be a perfectly symmetrical three-phase ideal sinusoidal wave.

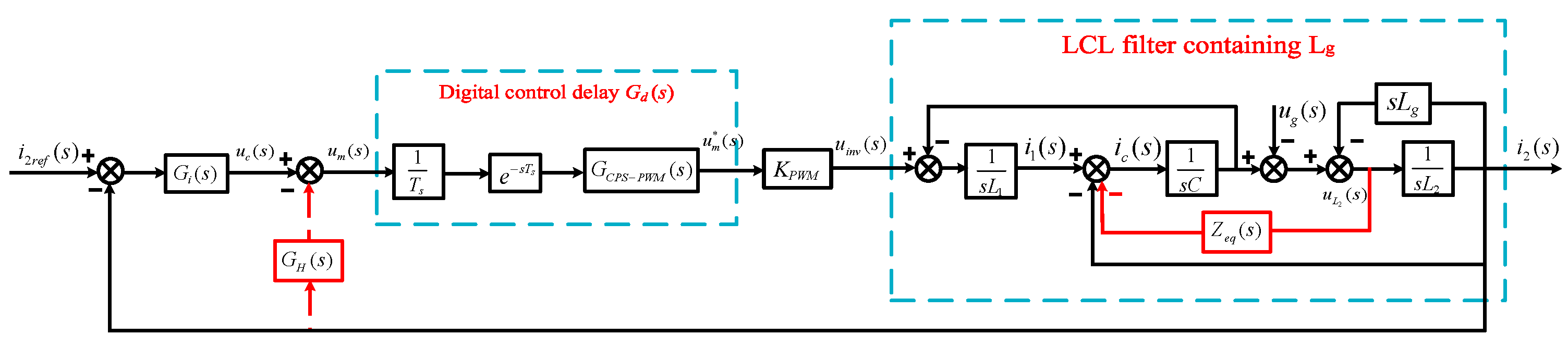
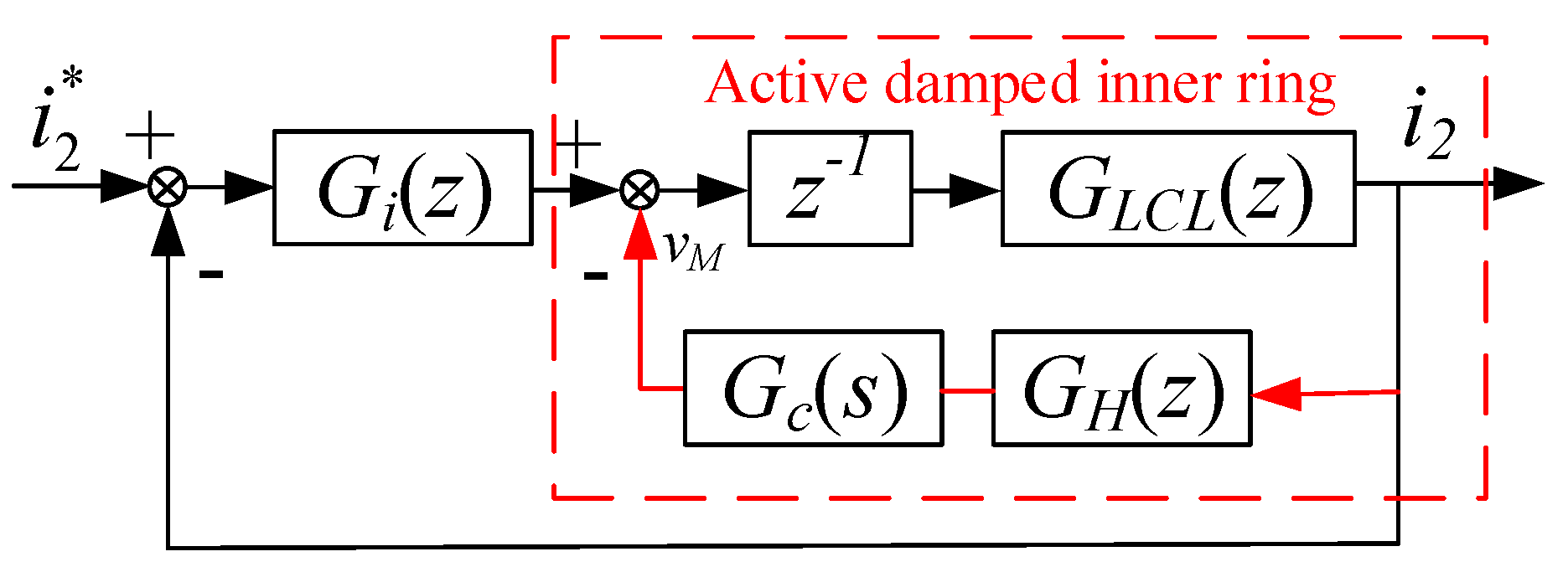
2.2. System Control Framework

The control framework of the LCL-type cascaded H-bridge SVG is typically divided into an inner current loop and an outer voltage loop. The current study mainly focuses on the inner current loop while neglecting the impact of the outer voltage one. A pulse width modulation (PWM) CPS-PWM is employed in a two-phase stationary coordinate system, with a sampling rate f_s equal to the switching rate f_sw. The current loop employs a proportional–integral (PI) controller. Figure 2 describes the traditional GCFAD system’s equivalent control block diagram in the continuous domain.

In Figure 2, G_i(s) is the current controller; K_PWM indicates the H-bridge SVG’s equivalent gain; G_d(s) describes the digital control system’s equivalent delay element; and G_H(s) is the high-pass filter (HPF). The current controller G_i(s) is a PI regulator whose transfer function (TF) is given by:

$$G_i(s)=K_p+{\displaystyle \frac{K_i}{s}}$$

(1)

K_p represents the proportional coefficient; K_i represents the resonance coefficient.

G_d(s) denotes the system control delay formed by the sampling process, computational delay, and zero-order hold, described as follows:

$$G_d(s)={\displaystyle \frac{1}{T_s}}{e}^{-s{T}_s}\cdot T_s{e}^{-0.5s{T}_s}=e^{-1.5s{T}_s}$$

(2)

The active damping function G_H(s) comprises a first-order HPF and a transfer function. Its expression is:

$$G_H(\mathrm{s})=-{\displaystyle \frac{s{K}_H}{s+{\omega }_d}}$$

(3)

where K_H describes the damping coefficient and ω_d indicates the cutoff angular frequency.

From Figure 2, the LCL filter’s equivalent TF from u_inv(s) to i₂(s), denoted by G_LCL(s), can be expressed as:

$$G_{LCL}(s)={\displaystyle \frac{i_2(s)}{u_{inv}(s)}}={\displaystyle \frac{1}{s^3{L}_1(L_2+L_g)C+s(L_1+L_2+L_g)}}={\displaystyle \frac{1}{s{L}_1(L_2+L_g)C}}{\displaystyle \frac{1}{s^2+{\omega }_r^2}}$$

(4)

where ω_r indicates the LCL filter’s resonant angular frequency, calculated as follows:

$${\omega }_r=\sqrt{{\displaystyle \frac{L_1+L_2+L_g}{C{L}_1(L_2+L_g)}}}$$

(5)

3. Impact of Digital Control Delay on Traditional GCFAD

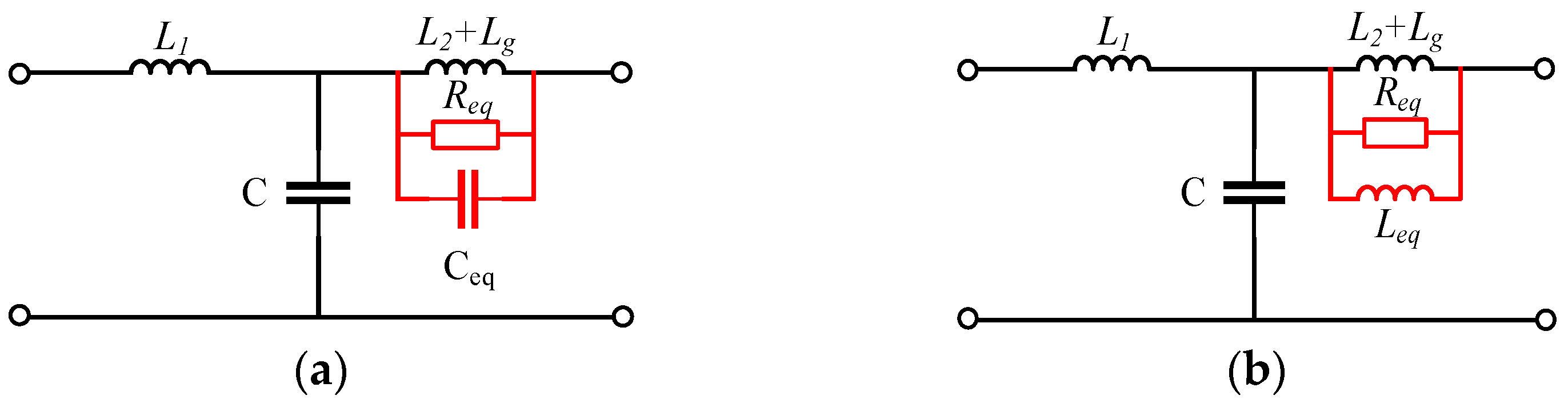
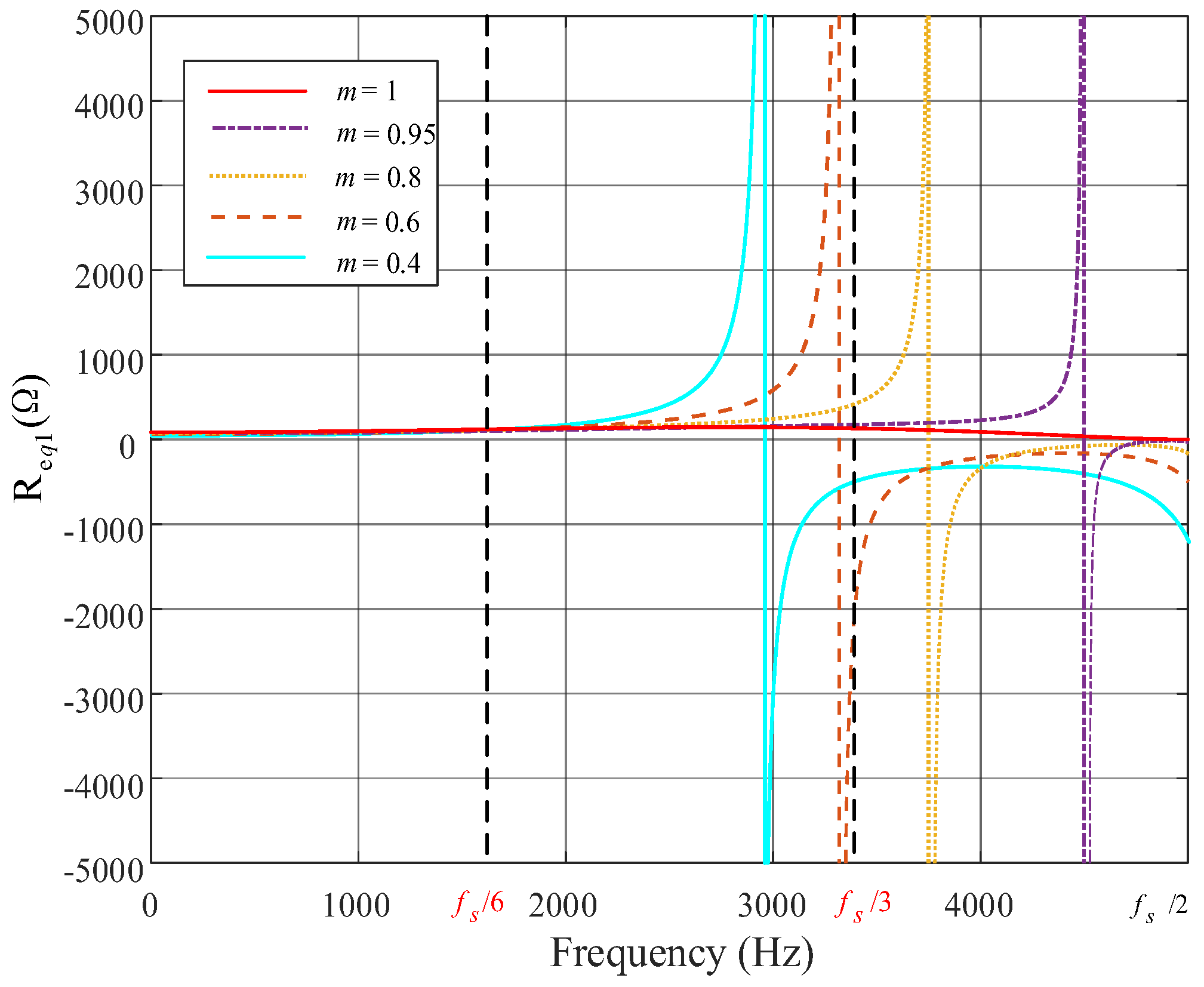
3.1. Virtual Impedance Analysis Under Digital Control

Figure 2 can be transformed accordingly to obtain the equivalent virtual impedance of the GCFAD strategy and analyze its physical essence. Specifically, the starting point of the active damping function G_H(s) is adjusted from the GC current i₂(s) to the grid-side inductive voltage u_L2(s), and its endpoint is changed to the output terminal of the cascaded H-bridge SVG-side inductive current i₁(s). This forms the equivalent control block diagram presented in Figure 3, reflecting the active damping strategy’s physical nature. This strategy essentially connects a virtual impedance Z_eq(s) in parallel across the two terminals of the grid-side inductance L₂, which can be expressed as:

$$Z_{eq}(s)={\displaystyle \frac{-s(s+{\omega }_d)L_1(L_2+L_g)e^{1.5s{T}_S}}{K_{PWM}{K}_H}}$$

(6)

When G_d(s) = 1, the virtual impedance Z_eq(s) is unaffected by digital control delays. In this case, the grid-current-feedback active damping strategy is equivalent to parallel connecting a virtual impedance, which remains positive in the frequency range (0, f_s/2), across both ends of L₂. This configuration helps dampen the resonance peaks across all resonant frequency ranges of the LCL filter. However, digital control delays narrow the effective damping range of the GCFAD method, limiting it to (0, f_R), where f_R ∈ (f_s/6, f_s/3).

After introducing virtual impedance, the resonant characteristics of the LCL filter will be improved. In the positive resistance region, Z_eq(s) can effectively dampen the system’s resonance peaks, reducing their amplitude and, thus, suppressing system instability.

By inserting s = jω into (6), the frequency-domain representation of Z_eq is obtained as:

$$Z_{eq}(j\omega )={\displaystyle \frac{\omega L_1(L_2+L_g)(\omega -j{\omega }_d)}{K_{PWM}{K}_H}}[\cos (1.5\omega T_s)+j\sin (1.5\omega T_s)]$$

(7)

Equation (7) describes a parallel combination of a resistance R_eq(ω) and a reactance X_eq(ω), expressed as follows:

$$Z_{\mathrm{eq}}(j\omega )=R_{\mathrm{eq}}(\omega )//X_{\mathrm{eq}}(\omega )$$

(8)

R_eq(ω) and X_eq(ω) are calculated as:

$$\left\{\begin{array}{l}{R}_{\mathrm{eq}}(\omega )={\displaystyle \frac{\omega L_1(L_2+L_g)({\omega }^2+{\omega }_d^2)}{K_{PWM}{K}_{H}M(\omega )}}\\ X_{\mathrm{eq}}(\omega )={\displaystyle \frac{\omega L_1(L_2+L_g)({\omega }^2+{\omega }_d^2)}{K_{PWM}{K}_{H}N(\omega )}}\end{array}\right.$$

(9)

where

$$\left\{\begin{array}{l}M(\omega )=\omega \cos (1.5\omega T_s)+{\omega }_d\sin (1.5\omega T_s)\\ N(\omega )=\omega \sin (1.5\omega T_s)-{\omega }_d\cos (1.5\omega T_s)\end{array}\right.$$

(10)

The frequency features of R_eq and X_eq can be obtained from Equation (9). When R_eq exhibits positive resistance features, it effectively suppresses the LCL filter’s resonance, as presented in Figure 4. R_eq exhibits positive resistance behavior within the frequency range (0, f_R), which corresponds to the system’s valid damping range, and negative resistance behavior within (f_R, f_s/2), where f_R ∈ (f_s/6, f_s/3). In the most severe case, the frequency boundary f_R of the positive and negative resistances of R_eq is f_s/6. When the system’s resonant frequency f_r is greater than f_s/6, i.e., f_r > f_R, the negative resistance characteristic of R_eq will degrade the system’s robustness to grid impedance, particularly when f_r = f_R, where the system cannot maintain stability.

From the analysis above, it can be concluded that using the GCFAD method not only virtualizes resistance, but also introduces an equivalent inductance or capacitance, and the equivalent reactance X_eq (either capacitive or inductive) causes a shift in f_r. Figure 5 presents the equivalent virtual impedance circuit.

From Figure 5, the resonant frequency of the system’s loop gain is obtained as:

$$f_r^{\prime }=\left\{\begin{array}{l}{\displaystyle \frac{1}{2\pi }}\sqrt{{\displaystyle \frac{L_1+L_2+L_g}{(C+C_{eq})L_1(L_2+L_g)}}},X_{eq}=C_{eq}\\ {\displaystyle \frac{1}{2\pi }}\sqrt{{\displaystyle \frac{L_1+L_2+L_g}{C{L}_1(L_2+L_g)}}+{\displaystyle \frac{1}{C{L}_{eq}}}},X_{eq}=L_{eq}\end{array}\right.$$

(11)

3.2. Stability Verification of the Traditional GCFAD System

From Figure 3, the transfer function of the loop gain of the digital-controlled LCL-type GC cascaded H-bridge SVG, denoted by T_D(s), can be expressed as:

$$T_D(s)={\displaystyle \frac{1}{s^3{L}_1{L}_{2}C}}\cdot {\displaystyle \frac{G_i(s)K_{PWM}{e}^{-1.5s{T}_s}}{s^2+{\omega }_r^2+\frac{s}{C{Z}_{eq}(s)}}}$$

(12)

As shown in (12), the numerator of T_D(s) includes the control delay term e^−1.5sTs, which causes phase lag and limits the control loop’s bandwidth; the equivalent impedance Z_eq of the actively damped loop in the denominator restricts the control loop’s bandwidth, which influences the system’s dynamic efficiency.

Figure 6 shows the loop gain’s Bode diagram under various grid impedances for the digital-controlled cascaded H-bridge SVG based on Equation (12) and the system parameters in

Table 1. The GC system parameters.

Parameter	Symbol	Value
Grid voltage (RMS)	ug	10,000 V
Fundamental frequency	f0	50 Hz
Rated capacity of the device	S	±10 Mvar
Submodule switching frequency	fSM	1000 Hz
Number of submodules	N	10
DC input voltage	Udc	1500 V
DC side capacitance	Cdc	3000 μF
Filter capacitance	C	3.86 μF
SVG-side inductance	L1	3.5 mH
Grid-side inductance	L2	1.5 mH
Equivalent switching frequency	fsw	10 kHz
Sampling rate	fs	10 kHz
Proportional gain	Kp	0.316
Integral gain	Ki	19.85

RMS: root mean square, f_sw = N × f_SM.

.

As illustrated in Figure 6, the wide range of grid impedance L_g changes causes the resonant peak frequency to cross the positive and negative dividing frequency f_R of the virtual resistor R_eq. Combined with Figure 4, it is known that, when f_r > f_R, the virtual resistor Req exhibits a negative resistance characteristic. In this case, the active damping controller amplifies the resonant frequency signal instead of suppressing it, ultimately leading to system instability. Furthermore, Equations (5) and (11) show that the loop gain’s resonant frequency f_r’ is greater than f_r, indicating that R_eq < 0 at f_r’. For the traditional GCFAD method, the phase frequency response of the open-loop TF T_D(s) never crosses −180°. In addition, T_D(s) has poles in the right half-plane (RHP) when f_r’ > f_R. Therefore, from the Nyquist stability criterion, the system is unable to operate stably when f_r’ > f_R.

To improve the system’s stability and robustness, the delay should be reduced to increase the system’s boundary frequency, ensuring f_R > f_r’. This will avoid negative resistance in the frequency range lower than f_r’. If f_R = f_s/2, R_eq has a positive resistance within the Nyquist frequency, significantly improving system performance.

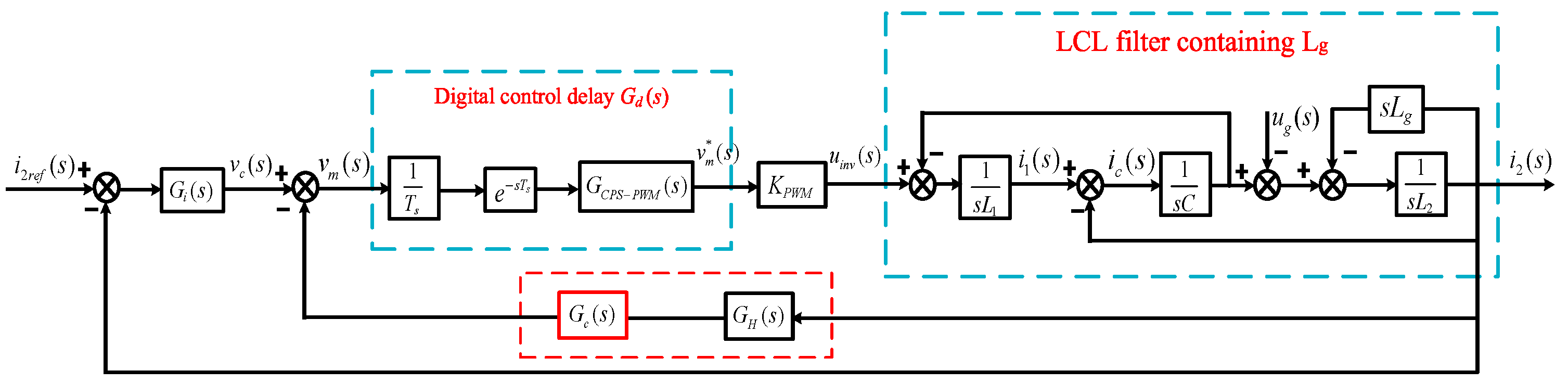
4. Highly Robust GCFAD Strategy Based on Phase-Lead Compensator

The analysis in Section 3 indicates that the valid damping zone of the traditional GCFAD system is confined to the range (0, f_R), where f_R lies within the range of (f_s/6, f_s/3). Since the grid impedance variations can cause shifts in the system’s resonant frequency, the narrow valid damping zone may lead to the virtual resistance falling into the negative resistance region. Therefore, the traditional damping approach should be refined to enlarge the efficient damping area and improve the system’s robustness. The current work introduces a phase-lead compensator in the damping loop to counteract the negative effects of digital delay on the effective damping region, as presented in Figure 7.

The TF of the delay compensation link, denoted by G_c(s), is represented as:

$$G_c(s)={({\displaystyle \frac{1+m}{1+m{e}^{-s{T}_s}}})}^2$$

(13)

where 0 ≤ m ≤ 1 and where m represents the degree of compensation. When m = 0, no compensation is applied, and, when m = 1, full compensation is applied. Within the Nyquist frequency range (0, f_s/2), replacing $e^{s{T}_s}$ with z in (13) gives:

$$G_c(z)={({\displaystyle \frac{1+m}{1+m{z}^{-1}}})}^2$$

(14)

According to Equation (14), the delay compensator’s frequency response characteristics can be changed by adjusting the value of m, as depicted in Figure 8.

As presented in Figure 8, the phase provided by the compensator increases as the value of m increases. When m = 1, the phase at the Nyquist frequency is 180°, while the corresponding excessive amplitude gain amplifies high-frequency noise, resulting in system instability. Therefore, a trade-off must be made between the phase and the amplitude of the compensation link to attain a satisfactory compromise between phase compensation while considering the stability of the system, and m ∈ (0.8, 1) was selected after the analysis.

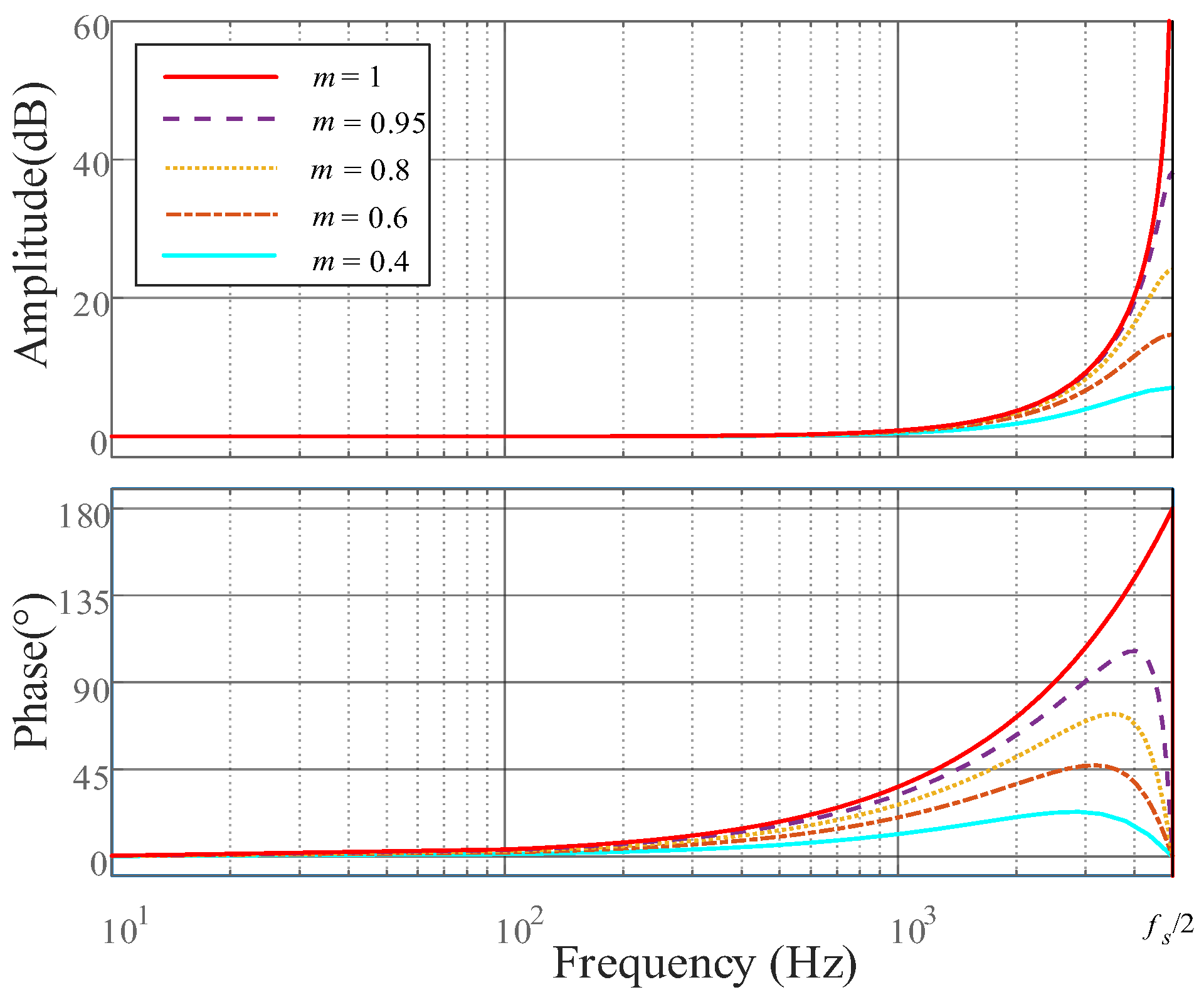
4.1. Effective Damping Zone Analysis

After adding the phase-lead compensation link, the virtual equivalent impedance Z_eq1 connected in parallel at both ends of L₂ can be expressed as:

$$Z_{eq1}(s)={\displaystyle \frac{-s(s+{\omega }_d)L_1(L_2+L_g)e^{1.5s{T}_S}{(1+m{e}^{-s{T}_s})}^2}{K_{PWM}{K}_H{(1+m)}^2}}$$

(15)

Substituting s = jω into (15) gives the frequency-domain representation for Z_eq1 as:

$$Z_{eq1}(j\omega )={\displaystyle \frac{L_1(L_2+L_g)}{K_{PWM}{K}_H{(1+m)}^2}}(M_1+j{N}_1)$$

(16)

$$\left\{\begin{array}{l}{M}_1={\omega }^2[\cos 3\pi f{T}_s+(m^2+2m)\cos \pi f{T}_s]+\omega {\omega }_d[\sin 3\pi f{T}_s+(2m-m^2)\sin \pi f{T}_s]\\ N_1={\omega }^2[\sin 3\pi f{T}_s+(2m-m^2)\sin \pi f{T}_s]-\omega {\omega }_d[\cos 3\pi f{T}_s+(m^2+2m)\cos \pi f{T}_s]\end{array}\right.$$

(17)

This can be equivalently represented by a resistance R_eq1(ω) in parallel with a reactance X_eq1(ω):

$$Z_{\mathrm{eq}1}(j\omega )=R_{\mathrm{eq}1}(\omega )//X_{\mathrm{eq}1}(\omega )$$

(18)

where the frequency-domain representations of R_eq1(ω) and X_eq1(ω) are:

$$\left\{\begin{array}{l}{R}_{\mathrm{eq}1}(\omega )={\displaystyle \frac{L_1(L_2+L_g)}{K_{PWM}{K}_H{(1+m)}^2}}{\displaystyle \frac{M_1^2+N_1^2}{M_1}}\\ X_{\mathrm{eq}1}(\omega )={\displaystyle \frac{L_1(L_2+L_g)}{K_{PWM}{K}_H{(1+m)}^2}}{\displaystyle \frac{M_1^2+N_1^2}{N_1}}\end{array}\right.$$

(19)

Combining Equations (17) and (19), the frequency characteristics of R_eq1 and X_eq1 for different values of m are illustrated in Figure 9.

As presented in Figure 9, the positive and negative dividing frequencies of the equivalent damping resistance R_eq1 are improved after introducing the delay compensation link, and the system’s effective damping area is effectively enlarged with the increase of m. To determine the optimal value of the compensation coefficient m, the closed-loop poles (CLPs) are obtained by changing the compensation coefficient m using the parameters given in Table 1, as presented in Figure 10.

It can be observed that, as m increases, the CLPs progressively shift outside the unit circle. To ensure the system stability when the system’s actual resonant frequency traverses the dividing frequency under a weak grid, the maximum value of m was chosen as 0.95 to attain a wide positive damping range (0, 0.45f_s), and the system’s effective damping region covered almost all possible resonant frequencies. Therefore, extending the effective damping region by phase-lead compensation significantly improves the system’s stability, especially under complex grid situations, improving the system’s adaptability and robustness.

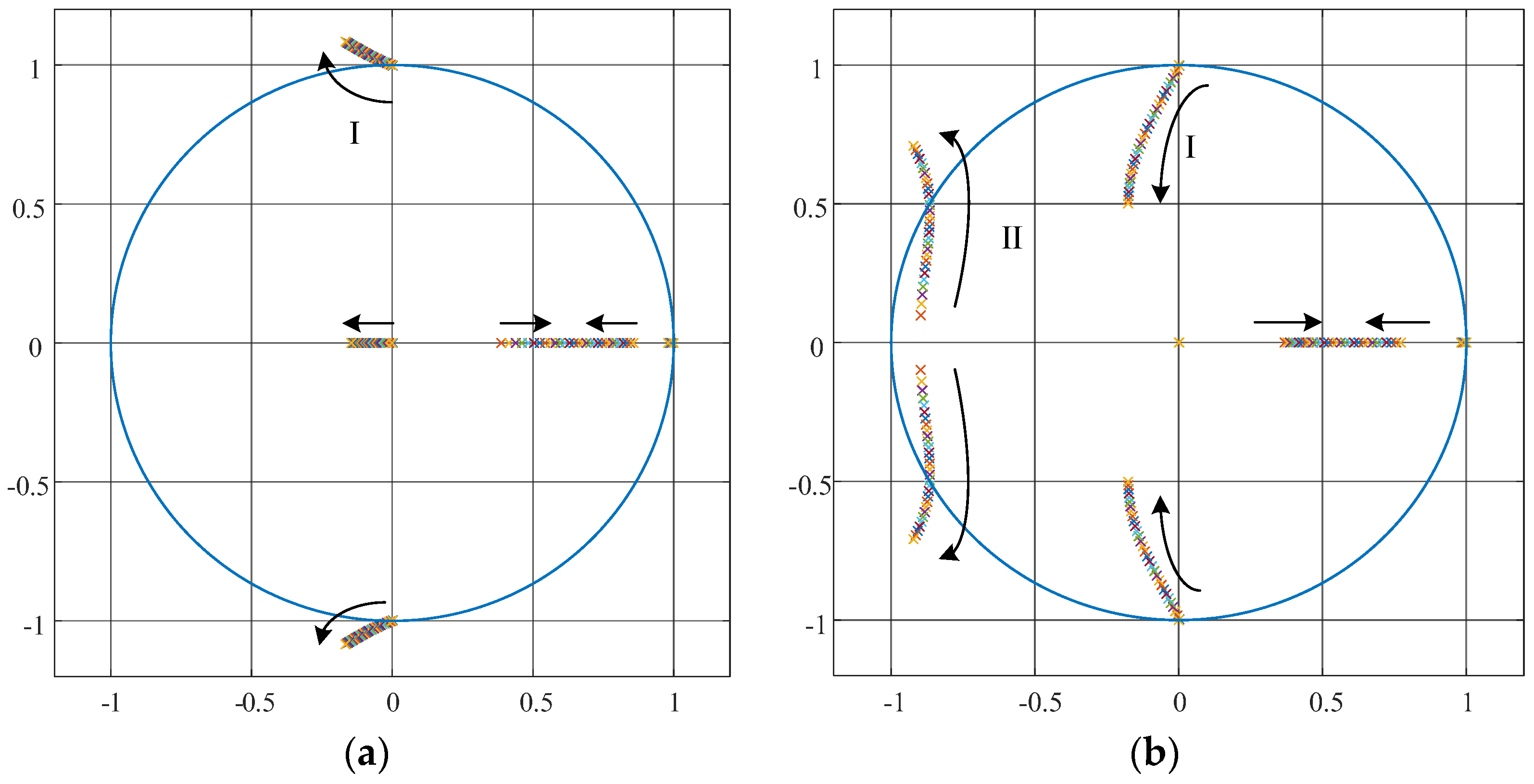
4.2. Design and Stability Analysis of GC Current Feedback Coefficient K_H

The magnitude of the GC current feedback coefficient affects the number of RHP poles and the magnitude margin of the system’s loop gain. The pole distribution of the loop gain of the LCL-type cascaded H-bridge SVG system should be analyzed after the compensation. Due to the existence of nonlinear links in the open-loop gain derived from the s-domain control block diagram, the continuous links in the control system shown in Figure 2 should be discretized for ease of analysis, forming the system control’s z-domain block diagram presented in Figure 11.

Where G_LCL(z) is the z-domain transfer function discretized by the series connection of the zero-order keeper link and the main circuit of the cascaded H-bridge SVG, which is expressed as:

$$G_{LCL}(z)={\displaystyle \frac{K_{PWM}}{{\omega }_r(L_1+L_2+L_g)}}[{\displaystyle \frac{{\omega }_r{T}_s}{z-1}}-{\displaystyle \frac{(z-1)\sin ({\omega }_r{T}_s)}{z^2-2z\cos ({\omega }_r{T}_s)+1}}]$$

(20)

G_H(z) indicates the discrete TF of the active damped feedback function G_H(s) discretized using the bilinear method, which can solve the frequency-domain aliasing distortion problem caused by time-domain sampling, and its expression is:

$$G_H(z)=Z{[{\displaystyle \frac{-s{K}_H}{s+{\omega }_d}}]}_{s={\displaystyle \frac{2}{T_s}}{\displaystyle \frac{z-1}{z+1}}}={\displaystyle \frac{-2K_H(z-1)}{(2+{\omega }_d{T}_s)z+({\omega }_d{T}_s-2)}}$$

(21)

For the high-frequency case, the G_PI(s) is equivalent to a K_p link. The z-domain open-loop TF, using the improved GCFAD system, can be attained.

$$T_D(z)={\displaystyle \frac{\begin{array}{l}\left\{K_{p}A\right\{{\omega }_r{T}_s[z^2-2z\cos ({\omega }_r{T}_s)+1]\\ -{(z-1)}^2\sin ({\omega }_r{T}_s)\}\cdot [(2+{\omega }_d{T}_s)z+({\omega }_d{T}_s-2)]\cdot {(z+m)}^2\}\end{array}}{\begin{array}{l}\{(z-1)\cdot \{z[z^2-2z\cos ({\omega }_r{T}_s)+1]\cdot [(2+{\omega }_d{T}_s)z+({\omega }_d{T}_s-2)]\cdot {(z+m)}^2\\ -2A{K}_H{(1+m)}^2\cdot \{{\omega }_r{T}_s[z^2-2z\cos ({\omega }_r{T}_s)+1]-{(z-1)}^2\sin ({\omega }_r{T}_s)\}\left\}\right\}\end{array}}}$$

(22)

where

$$A={\displaystyle \frac{K_{PWM}}{{\omega }_{\mathrm{r}}(L_1+L_2+L_{\mathrm{g}})}}$$

(23)

After selecting the compensation coefficient to be m = 0.95, the system’s closed-loop polar plot for various values of the grid-connected current feedback coefficient K_H (K_H ∈ (0, 1)) was plotted, as presented in Figure 12:

Figure 12a illustrates the pole distribution of the closed-loop TF of the system prior to time-delay compensation. As depicted in Figure 12a, In region Ⅰ, the CLPs moves outside the unit circle as the value of K_H increases. In contrast, for small values of K_H, the pole, although still within the unit circle, is positioned near its boundary, resulting in a limited stability margin and suboptimal damping performance for the uncompensated system.

Figure 12b illustrates the CLP distribution of the compensated system. In region Ⅰ, for the same value of K_H, the CLPs of the compensated system are positioned closer to the inner unit circle, resulting in an improved stability margin. This demonstrates that the presented delay compensation approach efficiently enhances the stability of the GC current under weak grid conditions and strengthens the system’s robustness. In region Ⅱ, as K_H increases, the CLPs of the system move away from the circle. According to automatic control theory, poles closer to the origin provide better damping performance. To address the impact of system parameter changes on pole position, a comprehensive analysis was conducted to determine an optimal K_H value. In this study, K_H = 0.5 was chosen to fulfill the system’s stability requirements.

Since the cutoff frequency ω_d is positively correlated with K_H, it is necessary to obtain the range of ω_d constraints that provides an open-loop gain with no RHP poles. Based on the selected values of m and K_H, the closed-loop system’s polar plot for different values of ω_d (ω_d ∈ (0, ω_r)) is shown in Figure 13.

As described in Figure 13, with the gradual growth of ω_d, the CLPs in the I-area will gradually approach the unit circle, ω_d = 0.6. A ω_r ≈ 9425 was taken to locate the poles of the open-loop gain in the left half-plane (LHP) while obtaining a satisfactory stability amplitude margin.

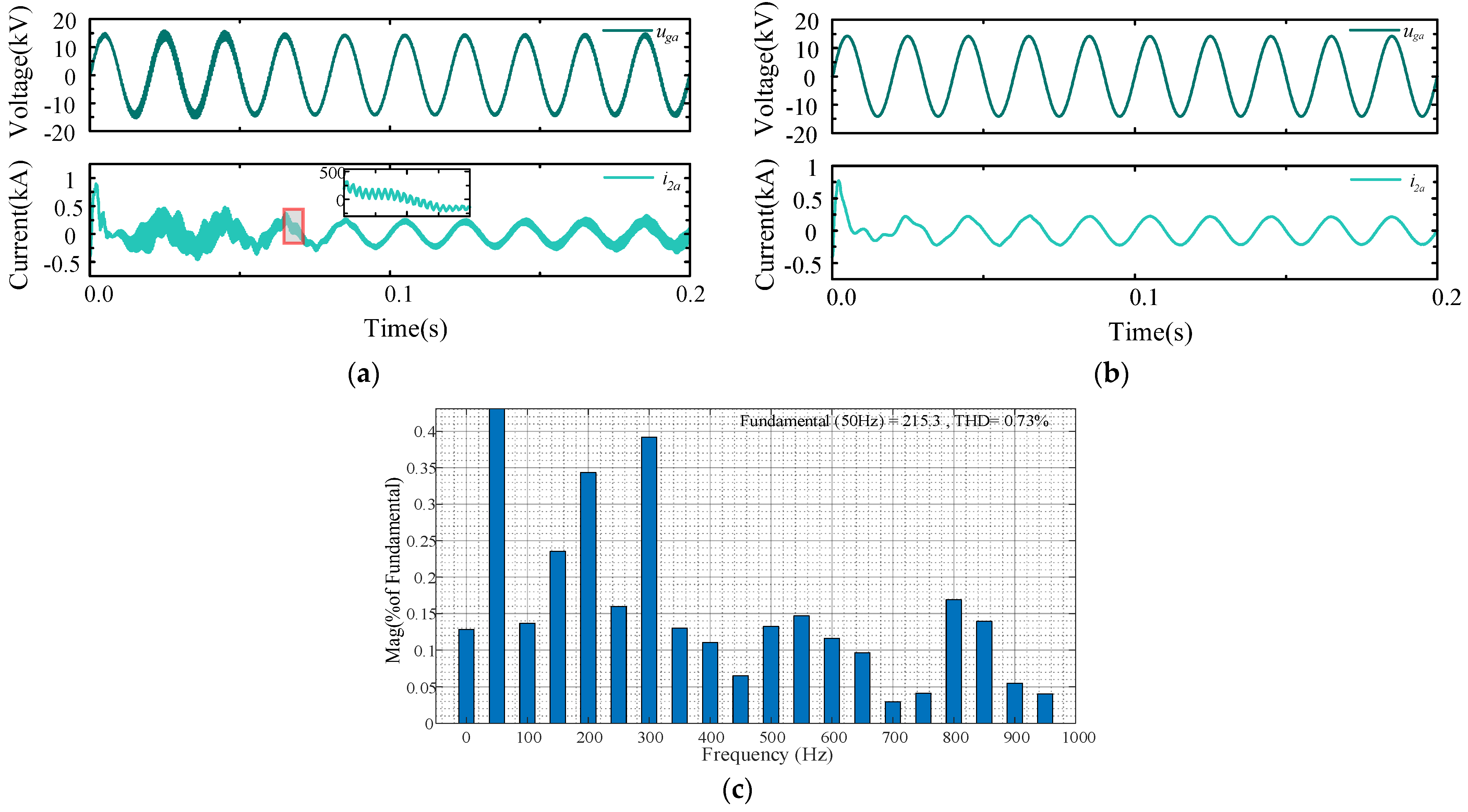
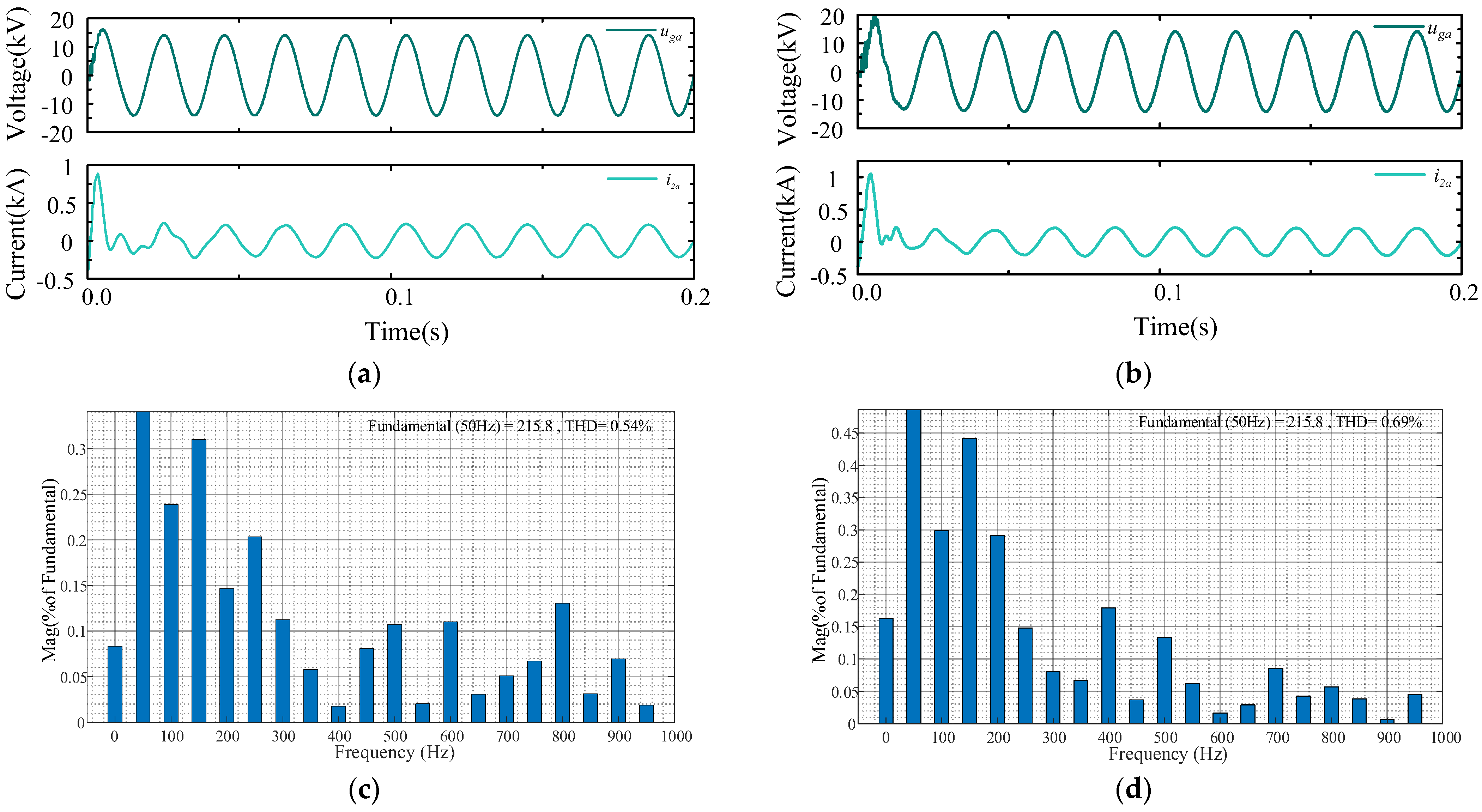
5. Simulation and Verification

To assess the accuracy and feasibility of the presented approach, a highly robust GCFAD system simulation model was developed by MATLAB R2022b/Simulink. Table 1 presents the system’s main circuit parameters.

Figure 14 presents the A-phase grid-connected voltage and grid-connected current of the GCFAD system before and after compensation for L_g = 0 and f_r = 2.49 kHz. As shown in Figure 14a, the resonance occurred with the conventional GCFAD system, causing a risk of instability. Figure 14b presents the system’s A-phase GC voltage and current using highly robust GCFAD. A comparison with Figure 14a shows that the compensated system can effectively suppress system resonance at the resonant frequency. As presented in Figure 14c, the THD of the system with highly robust GCFAD was only 0.77%, satisfying the grid-connection requirements.

Figure 15 describes the system’s simulated waveforms after the compensation for various values of the grid impedance. Combined with the case of Figure 14b, the system was kept stable when the grid impedance changed within 0–10.62 mH, demonstrating that the proposed highly robust GCFAD strategy can provide better stability and robustness under a broad domain of grid impedance changes.

Figure 16 illustrates the dynamic waveform of the load surge current for different values of the grid impedance. At t = 0.5 s, the load changed from 4 Mvar inductive load to 4 Mvar capacitive load.

As presented in Figure 16, the grid-connected current was rapidly regulated and provided stable operation after two IF cycles after a sudden load change. This delivered an effective damping effect while also reflecting the system’s rapid dynamic response.

The above simulation results indicate that the proposed highly robust GCFAD strategy can provide a satisfactory damping effect and a wide damping region, thereby improving the system’s adaptability to the weak grid. This demonstrates the effectiveness of the highly robust GCFAD method in suppressing harmonics and improving system stability and power quality.

6. Conclusions

This paper investigates the stability and harmonic suppression of the GCFAD control system to solve the insufficient robustness and poor harmonic suppression of conventional control strategies under complex grid conditions. The current study shows that the traditional GCFAD control strategy has limited harmonic suppression capability and poor system stability and robustness under complex grid conditions due to the delay effect. For this reason, the current study introduces a highly robust GCFAD control approach using phase overrun compensation. Simulations verify the effectiveness of this method under different operating conditions. The subsequent conclusions can be obtained from the simulation outcomes:

(1)

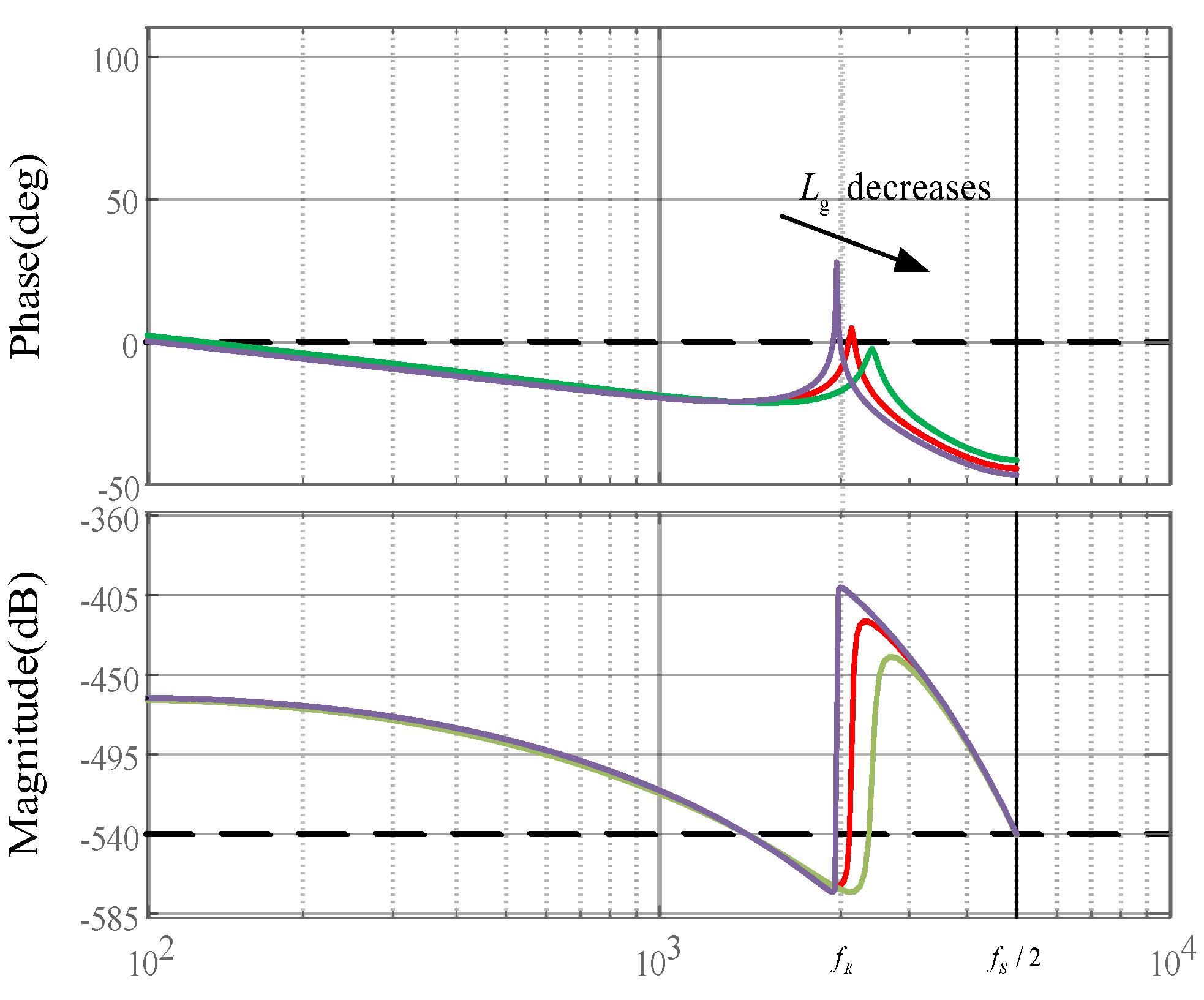
Affected by the digital control delay, the efficient damping area using the uncompensated GCFAD system is only (0, f_R) (f_R ∈ (f_s/6, f_s/3)). In a weak grid environment, the narrower the effective damping range, the poorer the system robustness.

(2)

To reduce the adverse effect of the digital control delay, the current work introduces the phase overrun compensation to counteract the control delay. As a result, the system’s efficient damping area is expanded from (0, f_R)(f_R ∈ (f_s/6, f_s/3)) to (0, 0.45f_s), and the wider effective damping range is more conducive to the LCL filter’s parameter design and the response to the grid impedance variations, thereby improving the system’s stability and robustness.

(3)

The stability of the highly robust GCFAD system is rigorously verified, and the optimal active damped feedback link parameters are obtained by combining the polar plots of the closed-loop system. The simulation outcomes confirm the efficiency of the presented approach.