Research Status and Latest Progress of Magnetic Field Modulation Motors
Abstract
1. Introduction
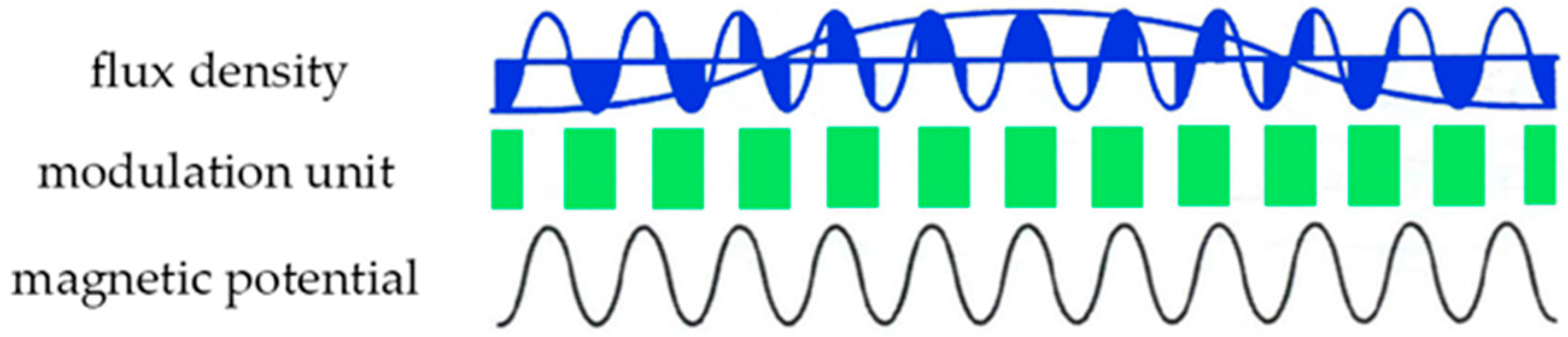
2. Basic Principles of Magnetic Field Modulation
3. Research on Motor Topology
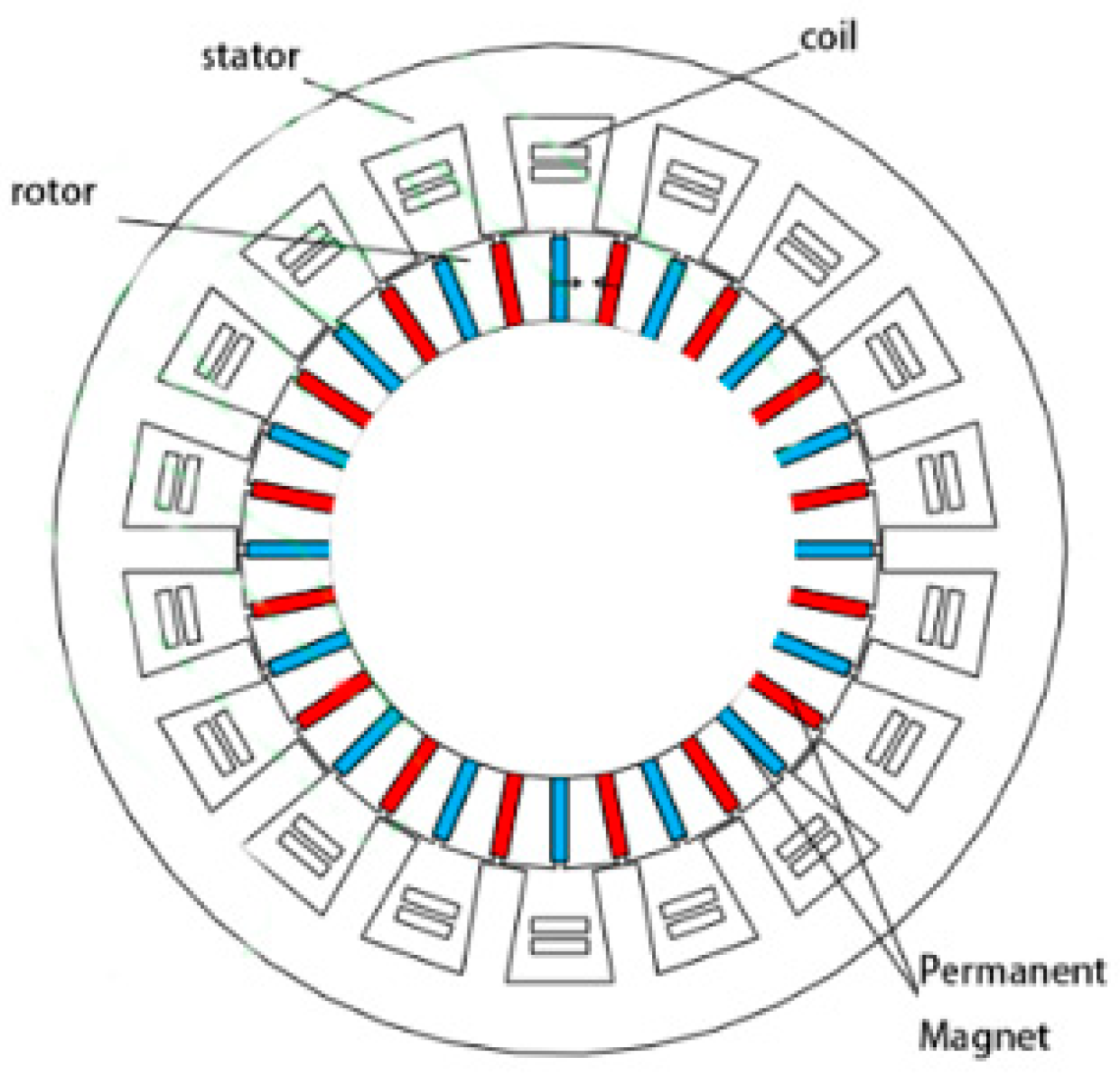
3.1. Rotor Permanent Magnet Type (Permanent Magnet Vernier Motor)
3.2. Stator Permanent Magnet Type
3.2.1. Switched Flux Linkage Permanent Magnet Motor
3.2.2. Flux Reversal Permanent Machine
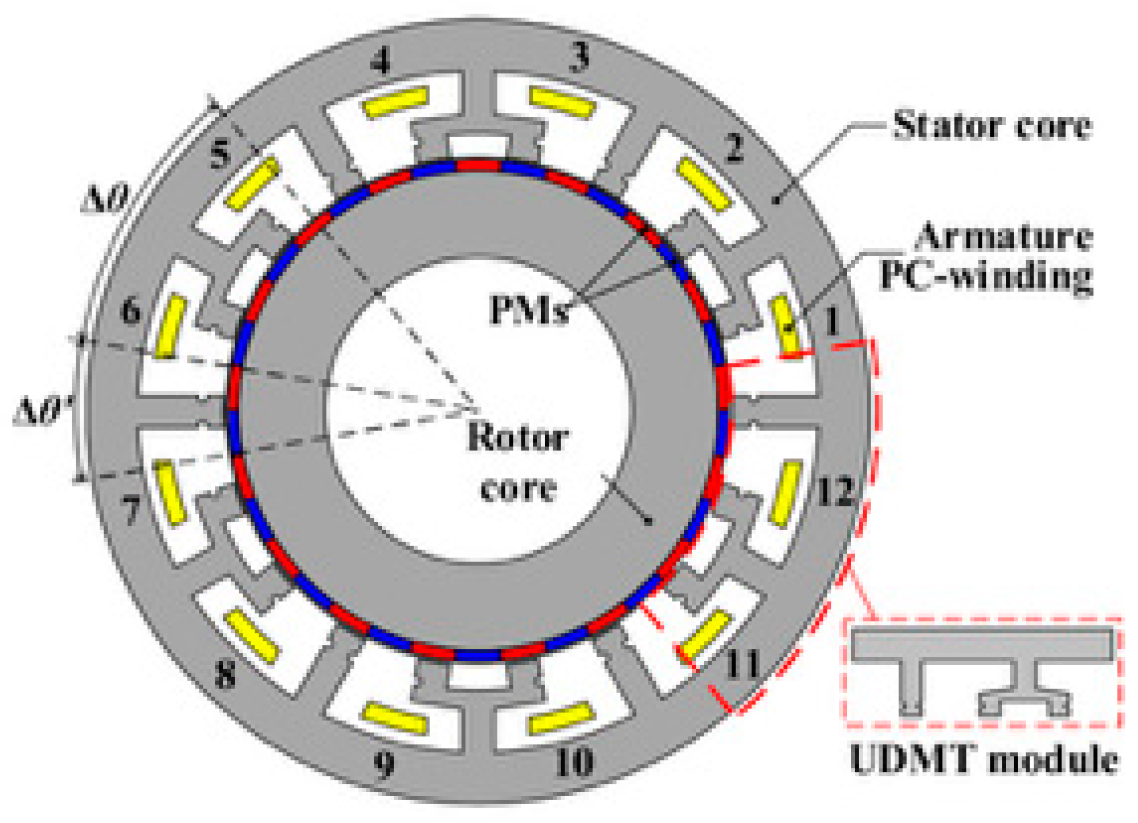
3.3. Double Permanent Magnet Type
4. Motor Characteristics Study
4.1. Torque Capacity
4.1.1. Torque Density Improvement
Harmonic Optimization
Control Strategies
4.1.2. Torque Ripple Reduction
Harmonic Optimization
Control Strategies
4.2. Power Factor
4.2.1. Air Gap Magnetic Flux
4.2.2. Winding Inductance
4.3. MFMM Characteristic Analytical Method
5. Development Trend and Application Prospect of MFMM
- (1)
- Excessive torque ripple. MFMMs have a stronger ability to utilize field harmonics than ordinary motors, but we should not ignore the fact that the motor is completed on the basis of increasing all sub-harmonics. How to improve the effective working sub-harmonics and reduce non-working sub-harmonics will always be the focus of research on MFMMs. In terms of motor structure, in the future, the sinusoidal nature of the air gap magnetic field can be optimized, and harmonic torque can be reduced by improving the magnetic barrier structure of the rotor/stator (such as layered magnetic barriers, asymmetric magnetic pole design) or adopting a multipole logarithmic combination. Alternatively, a hybrid excitation structure can be used to dynamically adjust the magnetic field strength to suppress torque pulsation. Advanced motor control strategies can also be adopted, such as the harmonic current injection method and intelligent algorithms, that apply deep reinforcement learning to optimize the current waveform and switching frequency in real-time and adaptively suppress pulsation.
- (2)
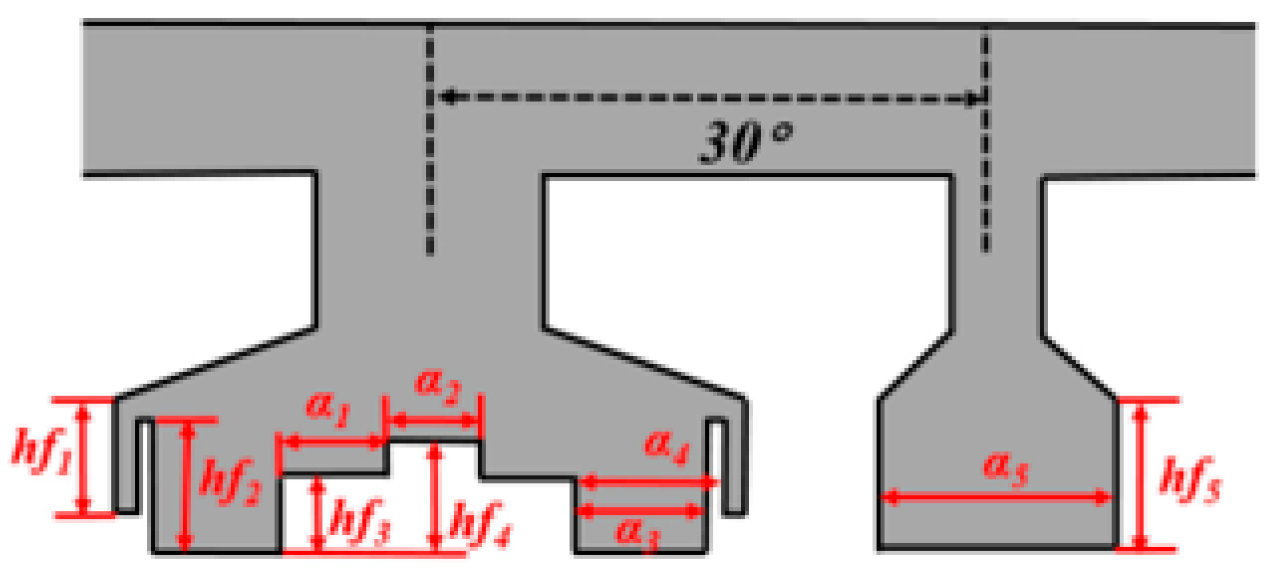
- Complicated motor structure. The introduction of the “field modulation” principle has already increased the complexity of the MFMM structure compared with conventional machines. In recent years, MFMM structural models of more and more complex structures have been proposed in order to improve the motor performance. Although these motors have excellent performance, their structure is extremely complex, and their processing and maintenance are extremely difficult, which is not conducive to large-scale popularization and application. In the future, modular design and symmetry optimization may be adopted to solve motor design problems. For example, using standardized functional modules (such as interchangeable magnetic pole units and integrated windings) can reduce the assembly complexity while maintaining modulation performance. the magnetic field modulation structure can be simplified through symmetrical magnetic circuits or quasi uniform air gap design while meeting the torque requirements. In the field of motor manufacturing, 3D printing can be used to achieve complex topologies that are difficult to process with traditional techniques, while reducing the number of parts.
- (3)
- The PF is generally low, particularly in high pole ratio designs. Although the torque output is large, most of the energy is consumed by reactive power, limiting its application in the high-precision field. In the future, dynamic adjustable electromagnetic modulation units, such as auxiliary excitation windings, can be introduced to optimize the air gap magnetic field distribution in real-time and reduce reactive current demand. Alternatively, a bidirectional magnetic field modulation structure of the rotor/stator can be used to offset the inductive reactive power component through phase compensation. At the same time, advanced intelligent control strategies can be adopted to detect the harmonic reactive power in real-time, inject high-frequency currents of specific phases (similar to active filtering), and offset the reactive power components of low-order harmonics. In terms of material innovation, gradient magnetic materials and programmable magnetic materials can be used to achieve a natural sinusoidal magnetic field and reduce the reactive magnetization current.
- (4)
- Large loss. Limited by material performance, hysteresis and eddy current loss are difficult to break through, resulting in low energy efficiency and high operation costs, which limits its application in the high-precision and high-efficiency fields. Therefore, reducing loss and improving efficiency has become a key issue in the field of MFMMs. Whether this problem can be solved will directly affect the development prospect and market potential of MFMMs. In the future, various composite materials, such as nanocrystalline soft magnetic alloys and amorphous alloy graphene composite magnetic cores, can be utilized to achieve the self-suppression of eddy current losses and block eddy current paths under high-frequency magnetic fields. In terms of winding technology, superconducting materials can be used in key areas to achieve zero resistance electrification.
- (5)
- Fault diagnosis faces new challenges. The unique magnetic field modulation mechanism of MFMMs has resulted in significant differences in its fault characteristics compared with traditional motors, mainly manifested in three aspects. Firstly, the failure of the modulation unit (such as modulation pole deformation or demagnetization) will generate special harmonic characteristics, requiring the development of dedicated modulation harmonic analysis algorithms. Secondly, the coupling effect of multiple physical fields makes it difficult for single sensor data to accurately reflect the nature of the fault. Therefore, multisource information fusion technology is needed such as combining high frequency current signals, vibration spectra, and infrared thermography for comprehensive diagnosis. Thirdly, the dynamic modulation process leads to time varying fault characteristics, and traditional threshold detection methods are prone to misjudgment. The latest solution includes building a deep learning based digital twin model to achieve early fault warning by comparing the difference in magnetic field distribution between the measured data and health benchmarks, developing adaptive resonance demodulation technology to effectively extract the characteristic frequencies of mechanical damage in modulation units; and applying transfer learning algorithms to solve the problem of fault feature drift under different operating conditions. These innovative methods provide new ideas for the intelligent operation and maintenance of MFMMs.
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, R.; Wang, B.; Cai, H.; Cheng, M.; Hua, W. Research on the Magnetic Field Modulation Effect of PMVM from the Perspective of Armature Magnetic Field. IEEE Trans. Ind. Electron. 2024, 72, 3392–3402. [Google Scholar] [CrossRef]
- Qu, R.; Li, D.; Zhao, Y. The Origin, Development and Challenge of Flux Modulation Machine. Proc. CSEE 2024, 44, 7361–7381. [Google Scholar]
- Wang, R.; Wang, B.; Tian, D.; Cai, H.; Cheng, M.; Hua, W. Slot Pole Combination Analysis of FSCW-PMVM on Magnetic Field Modulation Performance. IEEE Trans. Transp. Electrif. 2024, 11, 5665–5675. [Google Scholar] [CrossRef]
- Hsieh, M.F.; Chang, C.Y.; Huynh, T.A.; Dorrell, D.J. Design and Analysis of Consequent-pole Permanent Magnet Vernier Motor with Cancellation of Even-Order Harmonics. IEEE Trans. Magn. 2023, 59, 8204406. [Google Scholar] [CrossRef]
- Tlali, P.M.; Wang, R.J.; Gerber, S.; Botha, C.D.; Kamper, J.M. Design and performance comparison of vernier and conventional PM synchronous wind generators. IEEE Trans. Ind. Appl. 2020, 56, 2570–2579. [Google Scholar] [CrossRef]
- Lin, H.; Zhang, Y.; Yang, H.; Fang, S.; Huang, Y. Overview and Recent Developments of Permanent Magnet Vernier Machines. Proc. CSEE 2016, 36, 5021–5034+5127. [Google Scholar]
- Wu, F.; MEl-Refaie, A. Permanent magnet vernier machine: A review. IET Electr. Power Appl. 2019, 13, 127–137. [Google Scholar] [CrossRef]
- Hua, W.; Zhu, X. Overview of Flux-reversal Permanent Magnet Machine and Its Key Technologies. Proc. CSEE 2020, 40, 2657–2670. [Google Scholar]
- Chu, Y.; Liu, Z.; Zhao, W.; Liu, G. Overview of Iron Loss Calculation Methods for Flux Modulation Electric Machines. Proc. CSEE 2024, 1–14. [Google Scholar] [CrossRef]
- Li, X.; Cheng, M.; Zou, G.; Li, S. Principle and Analysis of a New Flux-Concentrating Field-Modulated Permanent-Magnet Wind Power Generator. Trans. China Electrotech. Soc. 2014, 29, 1–9. [Google Scholar]
- Liu, W.; Wang, J.; Lipo, T.A. A consequent pole single rotor single stator vernier design to effectively improve torque density of an industrial PM drive. IEEE Trans. Ind. Electron. 2023, 70, 255–264. [Google Scholar] [CrossRef]
- Wei, F.R.; Zhu, Z.Q.; Qu, H. Analysis of novel dual-PM Vernier machines. In Proceedings of the 2021 Sixteenth International Conference on Ecological Vehicles and Renewable Energies (EVER), Monte-Carlo, Monaco, 5–7 May 2021; pp. 1–8. [Google Scholar]
- Ghods, M.; Faiz, J.; Gorginpour, H.; Bazrafshan, M.A.; Nøland, J.K. Equivalent magnetic network modeling of variable-reluctance fractional-slot v-shaped vernier permanent magnet machine based on numerical conformal mapping. IEEE Trans. Transp. Electrif. 2023, 9, 3880–3893. [Google Scholar] [CrossRef]
- Xu, G.; Chen, J.; Chen, Q. A Novel Inverted T-type Consequent High Torque Density Permanent Magnet Vernier Motor. Proc. CSEE 2024, 1–8. Available online: https://link.cnki.net/urlid/10.1289.TM.20240425.1058.002 (accessed on 30 March 2025).
- Takahashi, H.; Shimomura, S. Study of Magnetic Circuit to Estimate Air Gap Magnetic Flux Density and Output Torque in Spoke Type Permanent Magnet Vernier Machine. In Proceedings of the 2020 23rd International Conference on Electrical Machines and Systems (ICEMS), Hamamatsu, Japan, 24–27 November 2020; pp. 894–897. [Google Scholar]
- Chen, Q.; Fan, Y.; Lei, Y.; Wang, X. Multi-objective optimization design of unequal halbach array permanent magnet vernier motor based on optimization algorithm. IEEE Trans. Ind. Appl. 2022, 58, 6014–6023. [Google Scholar] [CrossRef]
- Ardestani, M.; Arish, N.; Yaghobi, H. A new HTS dual stator linear permanent magnet Vernier machine with Halbach array for wave energy conversion. Phys. C Supercond. Appl. 2020, 569, 1353593. [Google Scholar] [CrossRef]
- Wang, X.; Jiang, S.; Qu, C. Structural optimization design of Halbach array permanent magnet cursor motor. Proc. CSEE 2023, 27, 140–147. [Google Scholar]
- Du, K.; Xu, L.; Zhao, W.; Liu, G. Analysis and design of a fault-tolerant permanent magnet vernier machine with improved power factor. IEEE Trans. Ind. Electron. 2022, 69, 4353–4363. [Google Scholar] [CrossRef]
- Fang, L.; Li, D.; Ren, X.; Qu, R. A novel permanent magnet vernier machine with coding-shaped tooth. IEEE Trans. Ind. Electron. 2021, 69, 6058–6068. [Google Scholar] [CrossRef]
- Du, Y.; He, Z.; Zhu, X.; Xiao, F.; Zhang, C.; Xu, L.; Zuo, Y.; Quan, L. A novel pole-changing permanent magnet vernier motor. IEEE Trans. Ind. Electron. 2022, 70, 6110–6120. [Google Scholar] [CrossRef]
- Yang, F.; Xie, Y.; Cai, W.; Wang, L.; Ye, B.; He, S.; Shao, J. The Magnetic Field Analytical Calculation of Permanent Magnet Vernier Synchronous Machine With Saddle-Shaped Permanent Magnets. IEEE Trans. Magn. 2023, 59, 8100213. [Google Scholar] [CrossRef]
- He, Z.; Xiao, F.; Du, Y.; Zhu, X. Comparison of Permanent Magnet Vernier Motors with Evenly and Unevenly Distributed Modulation Teeth. IEEE Trans. Magn. 2023, 59, 8203005. [Google Scholar] [CrossRef]
- Xie, Y.; Zhang, Y.; Yang, F.; Wang, Z.; Qi, G. Design and analysis of novel main and secondary tooth permanent magnet vernier motor with hourglass-shaped magnetic insulation. Electr. Mach. Control 2024, 28, 115–123+134. [Google Scholar]
- Liang, Z.; Qu, R.; Li, D.; Ren, X.; Gao, Y. Analysis of a Consequent-Pole Spoke-Array Vernier Permanent Magnet Machine. Trans. China Electrotech. Soc. 2020, 35, 3173–3181. [Google Scholar]
- Zhang, Y. Research on Spoke-Type Vernier Permanent Magnet Direct-Drive Wind Turbine Generator. Ph.D. Thesis, Huazhong University of Science and Technology, Wuhan, China, 2022. [Google Scholar]
- Siddiqi, M.R.; Yazdan, T.; Im, J.H.; Humza, M.; Hur, A.J. Experimental verification of dual airgap PMVM having yokeless rotor for extended speed application. IEEE Access 2022, 10, 133915–133925. [Google Scholar] [CrossRef]
- Kesgin, M.G.; Han, P.; Taran, N.; Ionel, D.M. Optimal study of a high specific torque vernier-type axial-flux PM machine with two different stators and a single winding. In Proceedings of the 2020 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 11–15 October 2020; pp. 4064–4067. [Google Scholar]
- Yang, F. Research on Novel Built-In Saddle-Shaped Permanent Magnet Vernier Machine. Ph.D. Thesis, Harbin University of Science and Technology, Harbin, China, 2024. [Google Scholar]
- Song, Z.; Liu, C.; Chai, F.; Zhao, H. Modular design of an efficient permanent magnet Vernier machine. IEEE Trans. Magn. 2020, 56, 1–6. [Google Scholar] [CrossRef]
- Du, K.; Zhao, W.; Xu, L.; Ji, J. Design of a new fault-tolerant linear permanent-magnet vernier machine. IEEE J. Emerg. Sel. Top. Ind. Electron. 2020, 1, 172–181. [Google Scholar] [CrossRef]
- Xie, K.; Li, D.; Qu, R.; Ren, X.; Shah, M.R.; Pan, Y. A new perspective on the PM vernier machine mechanism. IEEE Trans. Ind. Appl. 2019, 55, 1420–1429. [Google Scholar] [CrossRef]
- Zhou, Y.; Gao, Y.; Qu, R.; Cheng, Y.; Shi, C. A novel dual-stator HTS linear vernier generator for direct drive marine wave energy conversion. IEEE Trans. Appl. Supercond. 2019, 29, 5201806. [Google Scholar] [CrossRef]
- Arish, N. Electromagnetic performance analysis of linear vernier machine with PM and HTS-Bulk. Phys. C Supercond. Appl. 2020, 579, 1353751. [Google Scholar] [CrossRef]
- Shi, Y.; Ching, T.W.; Zhong, J.; Jian, L. A dual-stator HTS modular linear vernier motor for long stroke applications. IEEE Trans. Appl. Supercond. 2022, 32, 5201608. [Google Scholar] [CrossRef]
- Jing, L.; Kui, Z.; Yang, K.; Min, Z.; Su, Z.; Wang, Y. Genetic-Algorithm-Based Optimization of Dual Stator PM Vernier Machine with Spoke and HTS Bulks. J. Electr. Eng. Technol. 2023, 18, 3743–3750. [Google Scholar] [CrossRef]
- Wu, L.; Zhu, J.; Fang, Y. A novel doubly-fed flux-switching permanent magnet machine with armature windings wound on both stator poles and rotor teeth. IEEE Trans. Ind. Electron. 2020, 67, 10223–10232. [Google Scholar] [CrossRef]
- Cao, J.; Guo, X.; Fu, W.; Wang, R.; Liu, Y.; Lin, L. A method to improve torque density in a flux-switching permanent magnet machine. Energies 2020, 13, 5308. [Google Scholar] [CrossRef]
- Chen, Y.; Liang, J. Torque Ripples Reduction for Flux Switching Permanent Magnet Motor. Micromotors 2021, 54, 18–22. [Google Scholar]
- Wang, P.; Hua, W.; Hu, M.; Zhang, Z.; Wang, Y.; Cheng, M. Design and Performance Analysis of Flux-switching Permanent Magnet Machine with Cos-chamfering Rotor. Proc. CSEE 2022, 42, 8372–8382. [Google Scholar]
- Chen, Y.; Zhuang, J.; Ding, Y.; Li, X. Optimal design and performance analysis of double stator multi-excitation flux-switching machine. IEEE Trans. Appl. Supercond. 2019, 29, 0601205. [Google Scholar] [CrossRef]
- Shu, Q.; Zhou, L.; Cao, R. Design and analysis of a Tubular Flux-Switching Permanent Magnet Linear Motor for aviation actuator. In Proceedings of the 2022 IEEE 5th International Electrical and Energy Conference (CIEEC), Nanjing, China, 27–29 May 2022; pp. 4232–4237. [Google Scholar]
- Fard, J.R.; Ardebili, M. Design and control of a novel yokeless axial flux-switching permanent-magnet motor. IEEE Trans. Energy Convers. 2019, 34, 631–642. [Google Scholar] [CrossRef]
- Wang, Y. Research on Optimal Design of High Thrust Density Electrically-Excited Flux-Switching Linear Machines. Master’s Thesis, Zhejiang University of Science and Technology, Hangzhou, China, 2023. [Google Scholar]
- Zhu, X.; Fan, D.; Mo, L.; Chen, Y.; Quan, L. Multi-objective optimization design of a double-rotor flux-switching permanent magnet machine considering multimode operation. IEEE Trans. Ind. Electron. 2019, 66, 641–653. [Google Scholar] [CrossRef]
- Hussain, S.; Khan, F.; Ullah, W.; Ullah, B.; Khan, A.B. Development of a low-cost modular structure fault tolerant field excited flux switching linear machine for urban rail transit. IEEE Access 2021, 9, 165854–165864. [Google Scholar] [CrossRef]
- Hussain, S.; Khan, F.; Ullah, W.; Ullah, B.; Khan, B.; Qasim, M. Design and Analysis of E-Core Modular and Complementary Fault Tolerant Field Excited Flux Switching Linear Machines. In Proceedings of the 2021 International Conference on Computing, Electronic and Electrical Engineering (ICE Cube), Quetta, Pakistan, 26–27 October 2021; pp. 1–6. [Google Scholar]
- Ullah, N.; Basit, A.; Khan, F.; Shah, Y.A.; Khan, A.; Waheed, O.; Usman, A.A. Design and optimization of complementary field excited linear flux switching machine with unequal primary tooth width and segmented secondary. IEEE Access 2019, 7, 106359–106371. [Google Scholar] [CrossRef]
- Omar, M.F.; Sulaiman, E.; Ahmad, M.Z.; Othman, S.; Soomro, H.; Jusoh, L. Comparison Between Segmental and Salient Rotors of Single-phase Field Excitation Flux Switching Motor with Non-Overlap Windings for Lightweight Applications. In Proceedings of the 2019 IEEE Student Conference on Research and Development (SCORED), Bandar Seri Iskandar, Malaysia, 15–17 October 2019; pp. 27–32. [Google Scholar]
- Cao, R.; Su, E. New double-sided wound field flux-switching linear motor with non-overlapping winding. In Proceedings of the 2019 IEEE International Electric Machines & Drives Conference (IEMDC), San Diego, CA, USA, 12–15 May 2019; pp. 1746–1751. [Google Scholar]
- Jiang, W.; Huang, W.; Lin, X.; Zhao, Y.; Wu, X.; Zhao, Y.; Dong, D.; Jiang, X. A novel stator wound field flux switching machine with the combination of overlapping armature winding and asymmetric stator poles. IEEE Trans. Ind. Electron. 2021, 69, 2737–2748. [Google Scholar] [CrossRef]
- Khan, B.; Khan, F.; Ahmad, N.; Faraz, G.; Ahmad, R.; Naveed, K. Preliminary study of a new octane modular stator field excited flux switching motor for high speed applications. In Proceedings of the 2019 2nd International Conference on Computing, Mathematics and Engineering Technologies (ICOMET), Sukkur, Pakistan, 30–31 January 2019; pp. 1–5. [Google Scholar]
- Ma, W.; Wang, X.; Wang, Y.; Li, X. A HTS-excitation modular flux-switching linear machine with static seals. IEEE Access 2019, 7, 32009–32018. [Google Scholar] [CrossRef]
- Yao, X. Research on Control System of Primary Staggered Double-sided Linear Flux switching Permanent Magnet Motor. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2022. [Google Scholar]
- Zhao, M.; Wei, Y.; Yang, H.; Xu, M.; Han, F.; Deng, G.; Hou, D.; Zhang, P. Development and analysis of novel flux-switching transverse-flux permanent magnet linear machine. IEEE Trans. Ind. Electron. 2019, 66, 4923–4933. [Google Scholar] [CrossRef]
- Li, H.; Zhu, Z.Q. Influence of adjacent teeth magnet polarities on the performance of flux reversal permanent magnet machine. IEEE Trans. Ind. Appl. 2019, 55, 354–365. [Google Scholar] [CrossRef]
- Du, D.; Zhao, J. Low Harmonics Design and Performance Analysis of Flux Reverse Permanent Magnet Motor. Micromotor 2021, 49, 1–7+16. [Google Scholar]
- Dmitrievskii, V.; Prakht, V.; Kazakbaev, V. Novel rotor design for high-speed flux reversal motor. Energy Rep. 2020, 6, 1544–1549. [Google Scholar] [CrossRef]
- Nie, Y. Research on a New Type of Magnetic Flux Reverse Transverse Magnetic Field Permanent Magnet Linear Motor. Master’s Thesis, Nanjing University of Information Science and Technology, Nanjing, China, 2024. [Google Scholar]
- Liu, X. Design of Dual Stator Flux Reverse Transverse Flux Permanent Magnet Linear Motor. Master’s Thesis, Nanjing University of Information Science and Technology, Nanjing, China, 2024. [Google Scholar]
- Liu, Y. Design and Analysis of Flux Reverse Permanent Magnet Galvanometer Motor. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2023. [Google Scholar]
- Boroujeni, S.T.; Emami, S.P.; Jalali, P. Analytical modeling of eccentric PM-inset machines with a slotless armature. IEEE Trans. Energy Convers. 2019, 34, 1466–1474. [Google Scholar] [CrossRef]
- Pan, Z.; Fang, S.; Lin, H.; Yang, H.; Xue, S. A new double-sided flux reversal arc permanent magnet machine with enhanced torque density capability. IEEE Trans. Magn. 2019, 55, 8102506. [Google Scholar] [CrossRef]
- Shen, Y.; Zhu, S.; He, Y.; Li, Z.; Zhao, W.; Lu, Q.; Lee, C.H.T. Investigation and Comparative Study of Double-Sided Yokeless Asymmetric Flux Reversal Permanent Magnet Linear Machine. IEEE Trans. Ind. Electron. 2024, 72, 756–767. [Google Scholar] [CrossRef]
- Ryu, J.; Hahn, I. Axially Stacked Multiphase Flux Reversal Machine. In Proceedings of the IECON 2019–45th Annual Conference of the IEEE Industrial Electronics Society, Lisbon, Portugal, 14–17 October 2019; Volume 1, pp. 928–933. [Google Scholar]
- Liu, C.C.; Wang, D.Y.; Wang, S.P.; Wang, Y.H. A novel flux reversal claw pole machine with soft magnetic composite cores. IEEE Trans. Appl. Supercond. 2020, 30, 5202905. [Google Scholar] [CrossRef]
- Yang, K.; Zhao, F.; Yu, J.; Wang, Y. A Novel Partitioned-Stator Flux Reversal Machine With Two Sets of Permanent Magnet. IEEE Trans. Magn. 2023, 59, 8102506. [Google Scholar] [CrossRef]
- Sarshar, M.R.; Kondelaji, M.A.J.; Mirsalim, M. Analysis of a Synthesized Slot Permanent Magnet Flux Reversal Motor. In Proceedings of the 2023 3rd International Conference on Electrical Machines and Drives (ICEMD), Tehran, Iran, 20–21 December 2023; pp. 1–4. [Google Scholar]
- Yang, T.; Chau, K.T.; Ching, T.W.; Xue, Z.; Zhao, H. Design and Analysis of Double-Rotor Flux-Reversal PM Magnetic Differential Motor With Suppressed Rotor Coupling. IEEE Trans. Transp. Electrif. 2023, 10, 5506–5519. [Google Scholar] [CrossRef]
- Saeidabadi, S.; Parsa, L.; Corzine, K.; Kovacs, C.; Haugan, T.J. A Partially Superconducting Flux Reversal Machine with High Power Density. In Proceedings of the 2023 IEEE International Electric Machines & Drives Conference (IEMDC), San Francisco, CA, USA, 15–18 May 2023; pp. 1–6. [Google Scholar]
- Yu, Y.; Nakamura, K. Design and Analysis of High Performance Flux Reversal Motor with Cross-Pole Shape Stator. In Proceedings of the 2024 International Conference on Electrical Machines (ICEM), Torino, Italy, 1–4 September 2024; pp. 1–6. [Google Scholar]
- Zhang, B.; Huang, R.; Dong, Z.; Liu, Y.; Liu, C. Design of a Novel Six-Phase Outer Rotor Transverse Flux Reversal PM Motor for Distributed Aviation Propulsion System. In Proceedings of the 2023 26th International Conference on Electrical Machines and Systems (ICEMS), Zhuhai, China, 5–8 November 2023; pp. 2342–2346. [Google Scholar]
- Chen, C.; Qu, R.; Bianchi, N. Comparative Analysis of Novel Asymmetric Consequent-Pole and Conventional Flux Reversal Permanent Magnet Machines. IEEE Trans. Energy Convers. 2023, 38, 2560–2570. [Google Scholar] [CrossRef]
- Shen, Y.; Lu, Q.; Shi, T.; Xia, C. Analysis and evaluation of hybrid-excited doubly salient permanent magnet linear machine with DC-biased armature current. IEEE Trans. Ind. Appl. 2021, 57, 3666–3677. [Google Scholar] [CrossRef]
- Jiang, T.; Zhao, W.; Xu, L. Analysis of split-tooth stator PM vernier machines with zero-sequence current excitation. IEEE Trans. Ind. Electron. 2023, 70, 1229–1238. [Google Scholar] [CrossRef]
- Lin, Q.; Niu, S.; Cai, F.; Fu, W.; Shang, L. Design and optimization of a novel dual-PM machine for electric vehicle applications. IEEE Trans. Veh. Technol. 2020, 69, 14391–14400. [Google Scholar] [CrossRef]
- Li, Y.; Yang, H.; Lin, H.; Liu, W.; Zhao, X. Torque generation mechanism and performance evaluation of a dual-sided pm machine with stator U-shaped magnets. IEEE Trans. Ind. Appl. 2021, 58, 250–260. [Google Scholar] [CrossRef]
- Huang, H.; Li, D.; Qu, R.; Gao, X.; Han, B. Design and analysis of T-shaped consequent pole dual PM Vernier machines with differential magnetic network method. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 4546–4555. [Google Scholar] [CrossRef]
- Huang, H.; Li, D.; Ren, X.; Qu, R. Analysis and reduction methods of cogging torque in dual pm vernier machines with unevenly distributed split teeth. IEEE Trans. Ind. Appl. 2022, 58, 4637–4647. [Google Scholar] [CrossRef]
- Gao, Y.; Doppelbauer, M.; Qu, R.; Li, D.; Ding, H. Synthesis of a flux modulation machine with permanent magnets on both stator and rotor. IEEE Trans. Ind. Appl. 2021, 57, 294–305. [Google Scholar] [CrossRef]
- Yang, K.; Zhao, F.; Wang, Y. Analysis of double-layer permanent-magnet flux reversal machines with different permanent magnet arrangements in stator. IEEE Trans. Magn. 2021, 57, 8100805. [Google Scholar] [CrossRef]
- Jia, S.; Yan, K.; Liang, D.; Qu, R.; Liu, J.; He, J. A novel DC-biased current dual PM Vernier machine. IEEE Trans. Ind. Appl. 2021, 57, 4595–4605. [Google Scholar] [CrossRef]
- Yang, X.; Kou, B.; Luo, J.; Zhang, H. Electromagnetic design of a dual-consequent-pole transverse flux motor. IEEE Trans. Energy Convers. 2020, 35, 1547–1558. [Google Scholar] [CrossRef]
- Luo, J.; Kou, B.; Yang, X.; Zhang, H.; Zhang, L. Development, design, and analysis of a dual-consequent-pole transverse flux linear machine for direct-drive applications. IEEE Trans. Ind. Electron. 2020, 68, 6097–6108. [Google Scholar] [CrossRef]
- Meng, Y. Research on Dual-Stator Flux Modulated Machine with Multiple-Permanent Magnet Excitation. Ph.D Thesis, Southeast University, Nanjing, China, 2022. [Google Scholar]
- Wang, Y.; Xu, W.; Zhang, X.; Ma, W. Harmonic analysis of air gap magnetic field in flux-modulation double-stator electrical-excitation synchronous machine. IEEE Trans. Ind. Electron. 2020, 67, 5302–5312. [Google Scholar] [CrossRef]
- Li, Y.; Ding, S.; Hang, J.; Li, W. A New Perspective on High-Torque-Density Mechanism of Permanent Magnet Vernier Machines. IEEE Trans. Energy Convers. 2024, 39, 2337–2348. [Google Scholar] [CrossRef]
- Xie, S.; Zuo, Y.; Chen, H.; Cao, L.; Yuan, X.; Han, B.S.; Hoang, C.C.; Lee, C.H.T. Investigation on stator shifting technique for permanent magnet vernier machines with two-slot pitch winding. IEEE Trans. Ind. Electron. 2023, 70, 6585–6596. [Google Scholar] [CrossRef]
- Pu, W. Harmonic Enhancement Design and Optimization of Flux-Modulated Permanent Magnet In-Wheel Motors. Ph.D Thesis, Jiangsu University, Zhenjiang, China, 2023. [Google Scholar]
- Pu, W.; Quan, L.; Xiang, Z.; Zhu, X.; Xue, G. Multi-harmonic-layered design and optimization of a flux-concentrated PM vernier motor considering harmonic sensitivity. IEEE Trans. Transp. Electrif. 2024, 10, 648–659. [Google Scholar] [CrossRef]
- Ling, Z.; Gu, Y.; Zhao, W.; Ji, J.; Zeng, Y. Topology Evolution and Torque Improvement Mechanism of Magnetic Field Modulated Machine Based on Energy Analysis Method. Proc. CSEE 2024, 45, 3236–3247. [Google Scholar]
- Xiang, Z.; Zhou, Y.; Zhu, X.; Ju, H.; Quan, L. Research on Torque Performance Improvement of Stator Permanent Magnet Field Modulation Motor Based on Magnetic Field Compensation. Proc. CSEE 2024, 44, 9062–9076. [Google Scholar]
- Fan, D.; Quan, L.; Zhu, X.; Xiang, Z. Design and optimization of double-stator vernier permanent magnet motor with improved torque characteristics based on flux modulation theory. IEEE Trans. Magn. 2022, 58, 8202707. [Google Scholar] [CrossRef]
- Shi, L.; Yan, B. Electromagnetic characteristics analysis of electric excitation segmental rotor flux-switching generator. Electr. Mach. Control 2021, 25, 110–118. [Google Scholar]
- Liu, W.; Yang, H.; Lin, H.; Qin, L. Hybrid analytical modeling of air-gap magnetic field in asymmetric-stator-pole flux reversal permanent magnet machine considering slotting effect. IEEE Trans. Ind. Electron. 2022, 69, 1739–1749. [Google Scholar] [CrossRef]
- Khazaee, A.; Zarchi, H.A.; Markadeh, G.R.A. Real-time maximum torque per ampere control of brushless DC motor drive with minimum torque ripple. IEEE Trans. Power Electron. 2020, 35, 1194–1199. [Google Scholar] [CrossRef]
- Mosaddegh Hesar, H.; Abootorabi Zarchi, H.; Ayaz Khoshhava, M. Online maximum torque per ampere control for induction motor drives considering iron loss using input–output feedback linearisation. IET Electr. Power Appl. 2019, 13, 2113–2120. [Google Scholar] [CrossRef]
- Hesar, H.M.; Zarchi, H.A.; Markadeh, G.A.; Khazaee, A. Maximum Torque Per Total Ampere Strategy for Vector-Controlled Brushless Doubly Fed Induction Machine Drive Taking Iron Loss Into Account. IEEE Trans. Power Electron. 2022, 37, 13598–13605. [Google Scholar] [CrossRef]
- Hesar, H.M.; Zarchi, H.A.; Markadeh, G.A. Online MTPTA and MTPIA control of brushless doubly fed induction motor drives. IEEE Trans. Power Electron. 2021, 36, 691–701. [Google Scholar] [CrossRef]
- Chen, X.; Hua, H.; Xu, S. Torque Improvement by Injecting Third Harmonics in Flux Reversal Permanent Magnet Machines. In Proceedings of the 2023 IEEE 6th Student Conference on Electric Machines and Systems (SCEMS), Huzhou, China, 7–9 December 2023; pp. 1–6. [Google Scholar]
- Amin, F.; Sulaiman, E.B.; Jenal, M.; Soomro, H.A.; Soomro, I.A. A New Field Oriented Control Strategy for Single Phase FEFSM. In Proceedings of the 2019 IEEE International Conference on Automatic Control and Intelligent Systems (I2CACIS), Selangor, Malaysia, 29 June 2019; pp. 268–272. [Google Scholar]
- Xiang, Z.; Lu, Z.; Zhu, X.; Jiang, M.; Fan, D.; Quan, L. Research on magnetic coupling characteristic of a double rotor flux-switching PM machine from the perspective of air-gap harmonic groups. IEEE Trans. Ind. Electron. 2022, 69, 12551–12563. [Google Scholar] [CrossRef]
- Xiang, Z.; Zhou, Y.; Zhu, X.; Quan, L.; Fan, D.; Liu, Q. Research on characteristic airgap harmonics of a double rotor flux-modulated PM motor based on harmonic dimensionality reduction. IEEE Trans. Transp. Electrif. 2024, 10, 5750–5761. [Google Scholar] [CrossRef]
- Yan, D.; Chen, W.; Wang, Z.; Wang, H.; Shi, T.; Xia, C. The Torque Ripple Reduction in PMAREL Machine Using Time-Space Harmonics Analysis of Air-Gap Flux Density. IEEE Trans. Ind. Electron. 2022, 69, 2390–2401. [Google Scholar] [CrossRef]
- Diao, K.; Sun, X.; Lei, G.; Bramerdorfer, G.; Guo, Y.; Zhu, J. System-level robust design optimization of a switched reluctance motor drive system considering multiple driving cycles. IEEE Trans. Energy Convers. 2021, 36, 348–357. [Google Scholar] [CrossRef]
- Liu, Z.; Huang, X.; Liu, B.; Geng, W.; Chen, G.; Zhang, J.; Chen, Q. A Modified Deadbeat Predictive Flux Control for Reducing Torque Ripple at Low Modulation Ratio. In Proceedings of the 2023 IEEE Vehicle Power and Propulsion Conference (VPPC), Milan, Italy, 24–27 October 2023; pp. 1–6. [Google Scholar]
- Yang, Q.; Guo, S. Multi-objective Orthogonal Particle Swarm Optimization of Permanent Magnet Vernier Motor. Mech. Sci. Technol. Aerosp. Eng. 2023, 42, 1996–2002. [Google Scholar]
- Zhao, Y.; Li, D.; Ren, X.; Zou, T.; Qu, R. Design trade-off between torque density and power factor in surface-mounted PM vernier machines through closed-form per-unit equations. IEEE Trans. Ind. Appl. 2023, 59, 3265–3277. [Google Scholar] [CrossRef]
- Zhu, Z.Q.; Liu, Y. Analysis of air-gap field modulation and magnetic gearing effect in fractional-slot concentrated-winding permanent-magnet synchronous machines. IEEE Trans. Ind. Electron. 2018, 65, 3688–3698. [Google Scholar] [CrossRef]
- Gorginpour, H. Dual-stator consequent-pole Vernier PM motor with improved power factor. IET Electr. Power Appl. 2019, 13, 652–661. [Google Scholar] [CrossRef]
- Xu, L.; Wu, W.; Zhao, W. Airgap magnetic field harmonic synergetic optimization approach for power factor improvement of PM vernier machines. IEEE Trans. Ind. Electron. 2022, 69, 12281–12291. [Google Scholar] [CrossRef]
- Wu, D.; Xiang, Z.; Zhu, X.; Quan, L.; Jiang, M.; Liu, Y. Optimization design of power factor for an in-wheel vernier PM machine from the perspective of air-gap harmonic modulation. IEEE Trans. Ind. Electron. 2021, 68, 9265–9276. [Google Scholar] [CrossRef]
- Wu, D.; Xiang, Z.; Quan, L.; Zhu, X.; Jiang, M.; Zhu, T. Research on power factor characteristic for a flux-modulated permanent magnet motor from perspective of magnetic source topologies effect. IEEE Trans. Appl. Supercond. 2021, 31, 5206206. [Google Scholar] [CrossRef]
- Bai, J.; Liu, J.; Liu, G.; Liu, Y.; Zheng, P. Investigation of the power factor of magnetic-field modulated brushless double-rotor machine. IEEE Trans. Power Electron. 2021, 36, 423–432. [Google Scholar] [CrossRef]
- Zhu, J.; Zuo, Y.; Chen, H.; Chen, J.; Lee, C.H.T. Deep-investigated analytical modeling of a surface permanent magnet vernier motor. IEEE Trans. Ind. Electron. 2022, 69, 12336–12347. [Google Scholar] [CrossRef]
- YShi, T.W. Ching. Power factor analysis of dual-stator permanent magnet vernier motor with consideration on turn-number assignment of inner and outer stator windings. IEEE Trans. Magn. 2021, 57, 8200405. [Google Scholar]
- Wang, T.; Zhu, X.; Xiang, Z.; Yi, F.; Jiang, M. Improvement of Power Factor and Torque of a PM Vernier Motor from Perspective of Armature and PM Field Harmonics. IEEE Trans. Transp. Electrif. 2023, 10, 6064–6075. [Google Scholar] [CrossRef]
- Yu, Y.; Chai, F.; Pei, Y.; Doppelbauer, M.; Lee, C.H.T. Investigation of Power Factor Characteristic in Permanent Magnet Motors for in-Wheel Direct Drive Application. IEEE Trans. Energy Convers. 2024, 39, 1699–1710. [Google Scholar] [CrossRef]
- Zhou, J.; Fan, D. Production Mechanism of Power Factor of V-Type Vernier Permanent Magnet Machine and Improvement Method. Trans. China Electrotech. Soc. 2023, 38, 3789–3799. [Google Scholar]
- Lu, K.; Li, X.; Yan, X.; Jiang, M.; Zhu, X. Harmonic Analysis and Electromagnetic Torque Calculation of the Flux-concentrated Filed-modulated Permanent Magnet Machine. Micromotors 2023, 56, 24–28. [Google Scholar]
- Ji, J.; Pan, X.; Zhao, W.; Geng, T. Operation Principle Analysis of Air Gap Magnetic Field Modulation in Five Phase Fault Tolerant Flux Switching Permanent Magnet Motor. Proc. CSEE 2017, 37, 6227–6236. [Google Scholar]
- Zhao, Y. Low pole-pair ratio integration design of permanent magnet vernier machine with improved power factor. IEEE Trans. Ind. Electron. 2023, 71, 2820–2830. [Google Scholar] [CrossRef]
- Fang, L.; Li, D.; Qu, R. Torque improvement of vernier permanent magnet machine with larger rotor pole pairs than stator teeth number. IEEE Trans. Ind. Electron. 2023, 70, 12648–12659. [Google Scholar] [CrossRef]
- Pu, W.; Xiang, Z.; Zhu, X.; Quan, L.; Fan, D. Research on enhanced harmonic effect of a dual-PM-excited flux-modulated motor. IEEE Trans. Appl. Supercond. 2021, 31, 5203906. [Google Scholar] [CrossRef]
- Shi, Y.; Jian, L.; Ching, T. Quantitative identification of airgap flux density harmonics contributing to back EMF in dual-permanent-magnet-excited machine. IEEE Trans. Magn. 2021, 58, 8100705. [Google Scholar] [CrossRef]





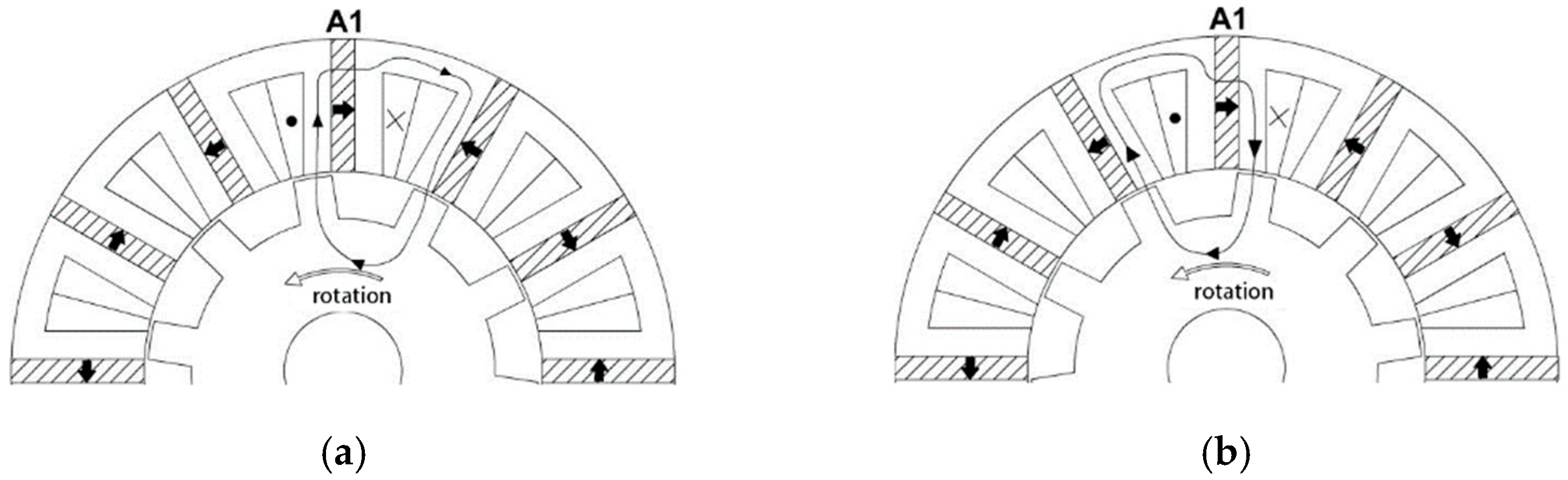

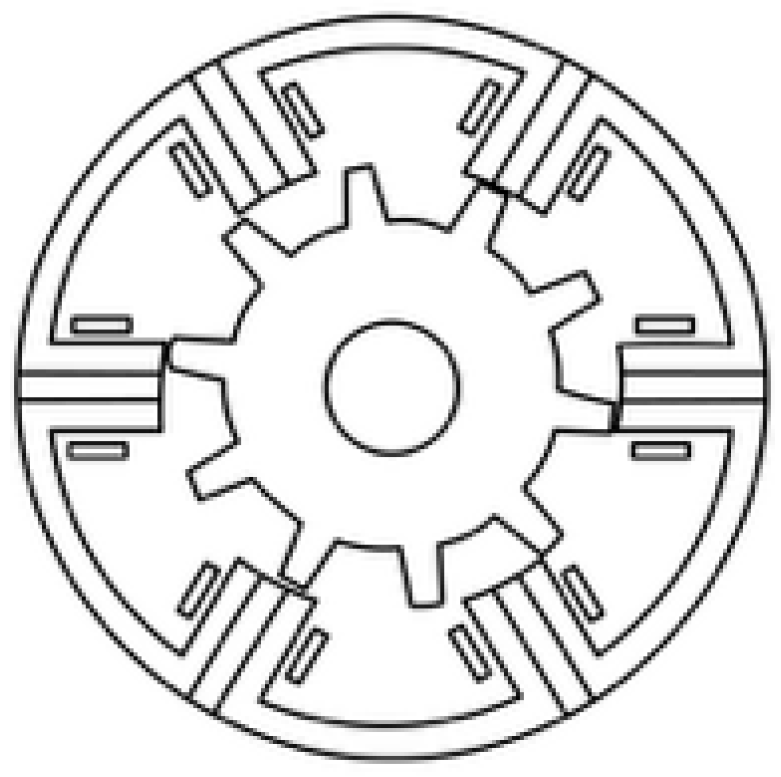
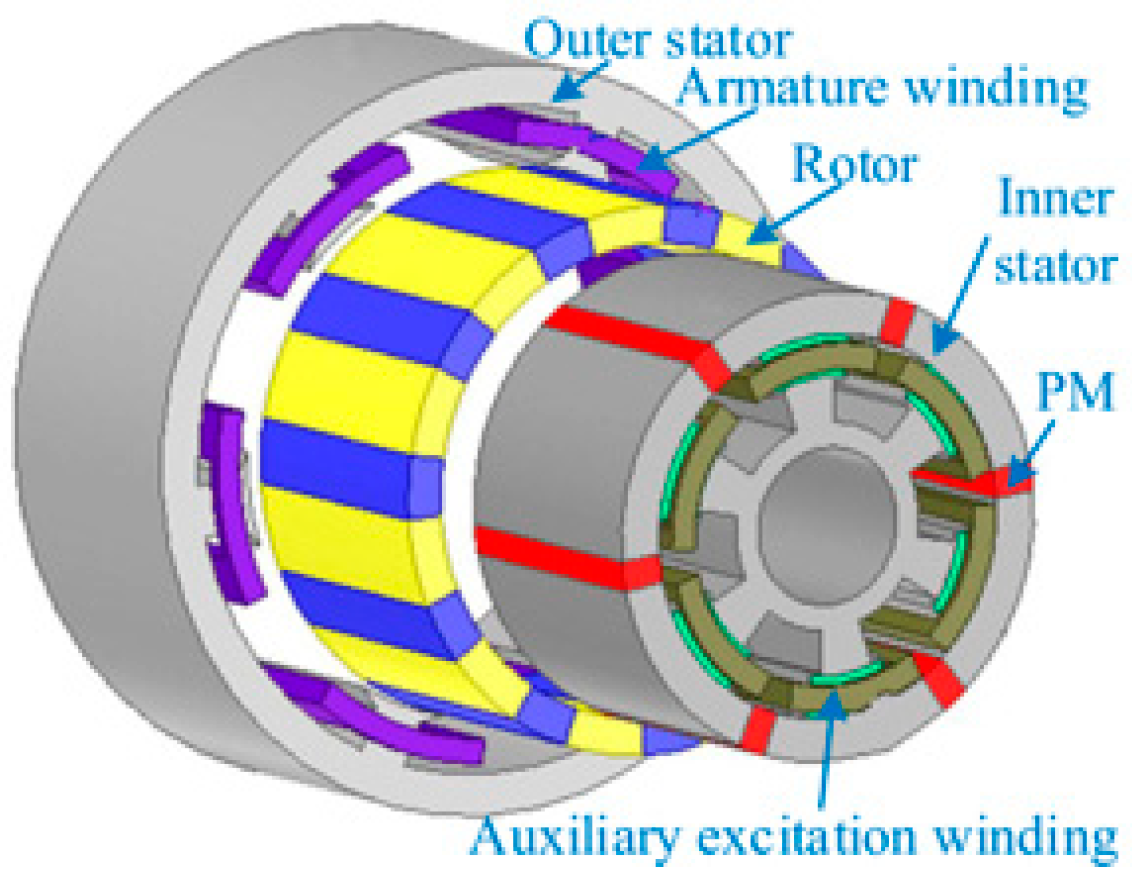
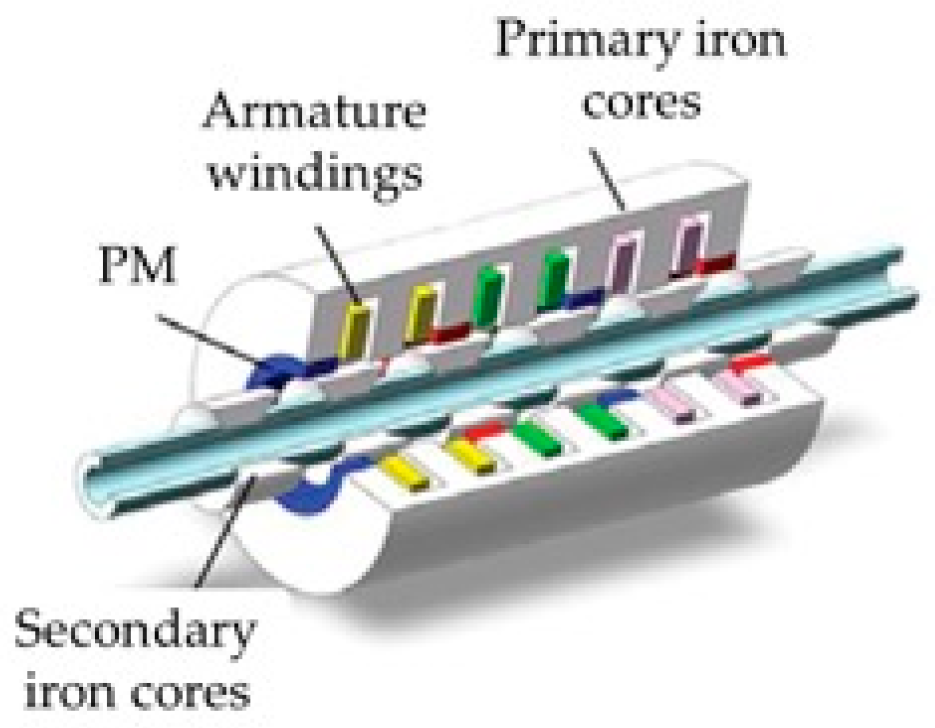
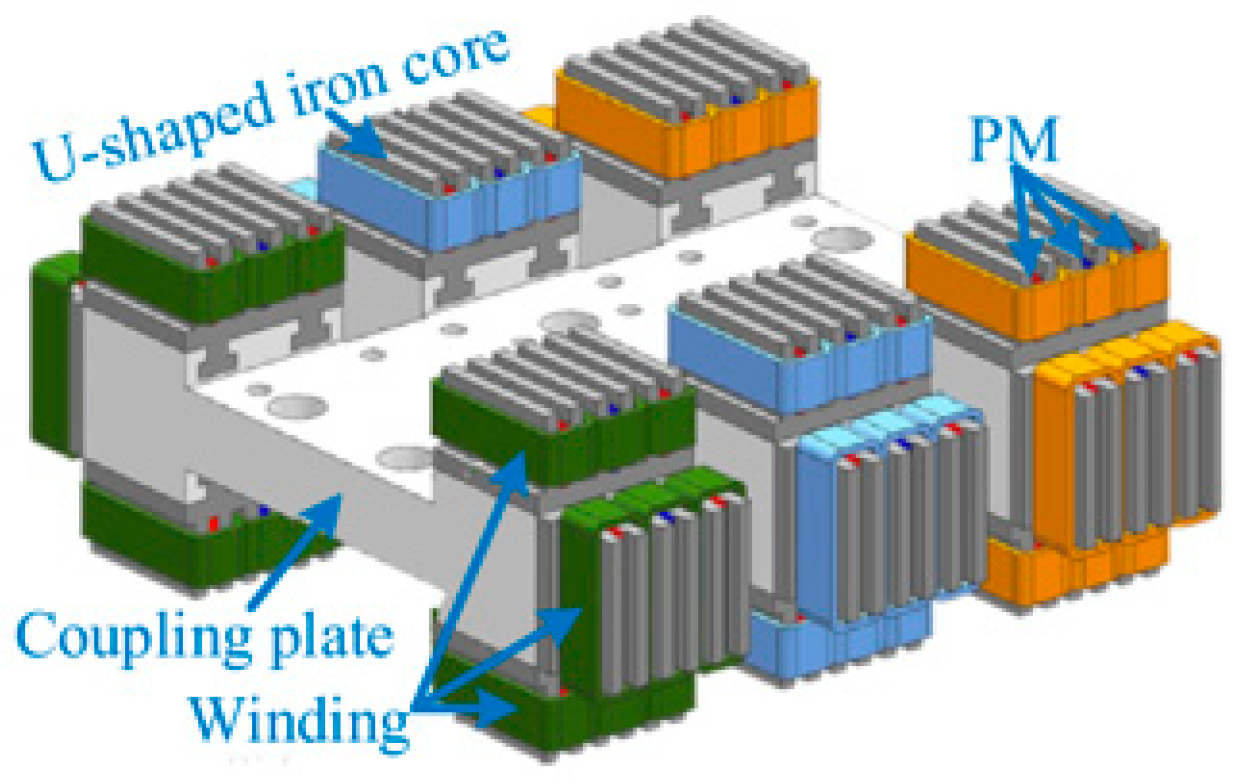
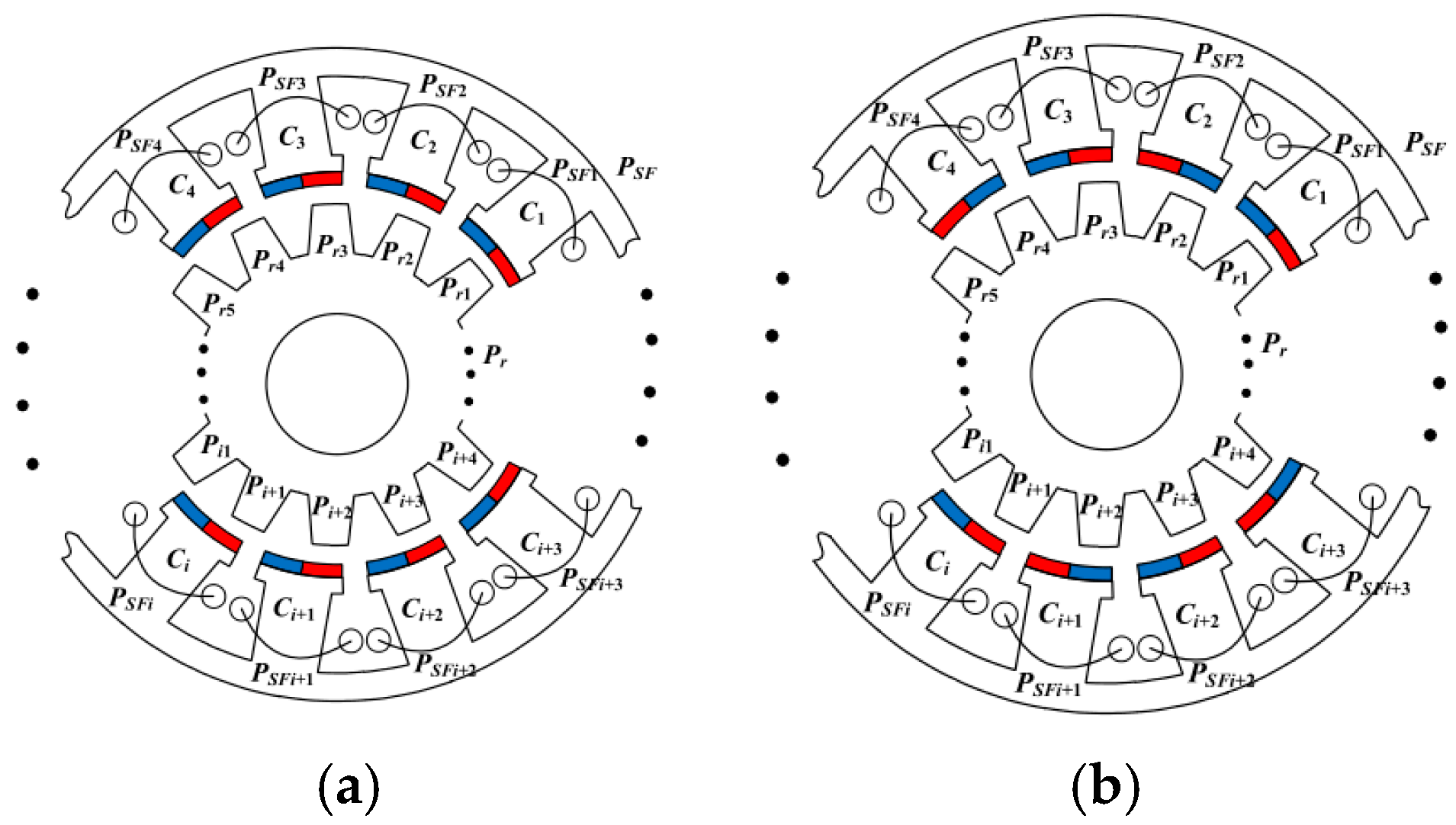
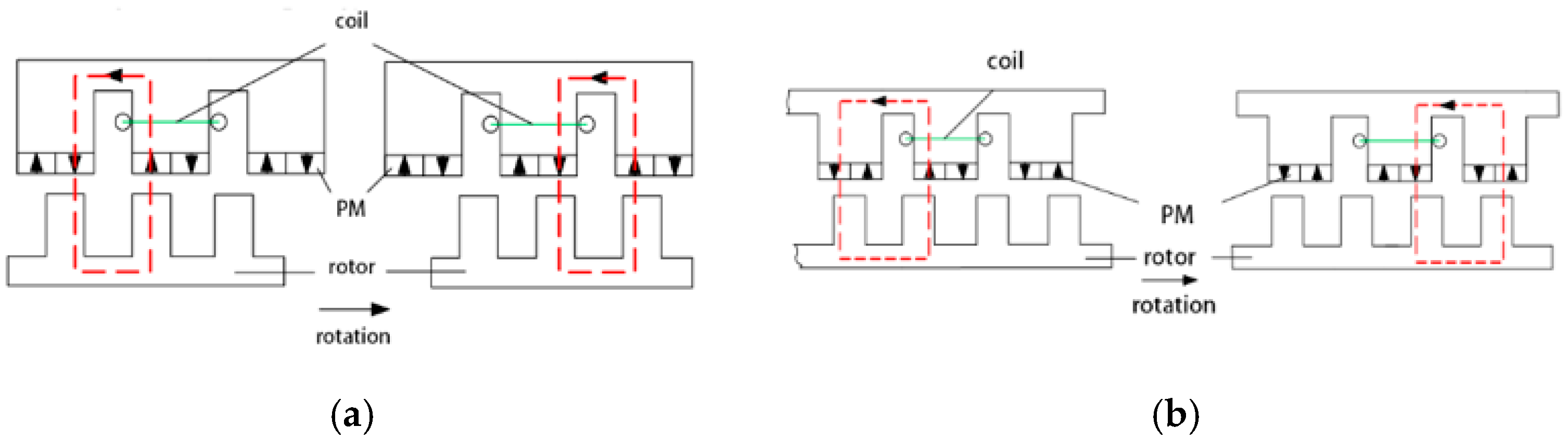

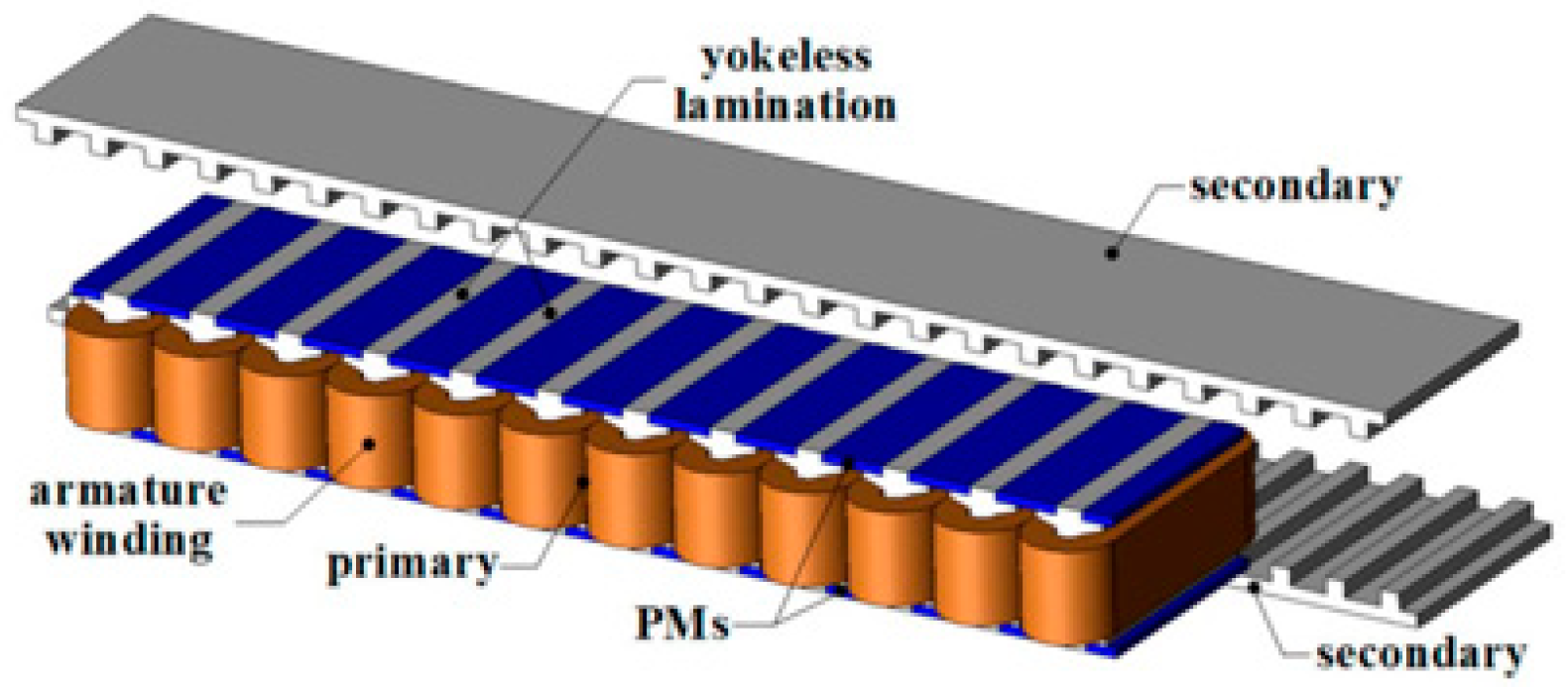

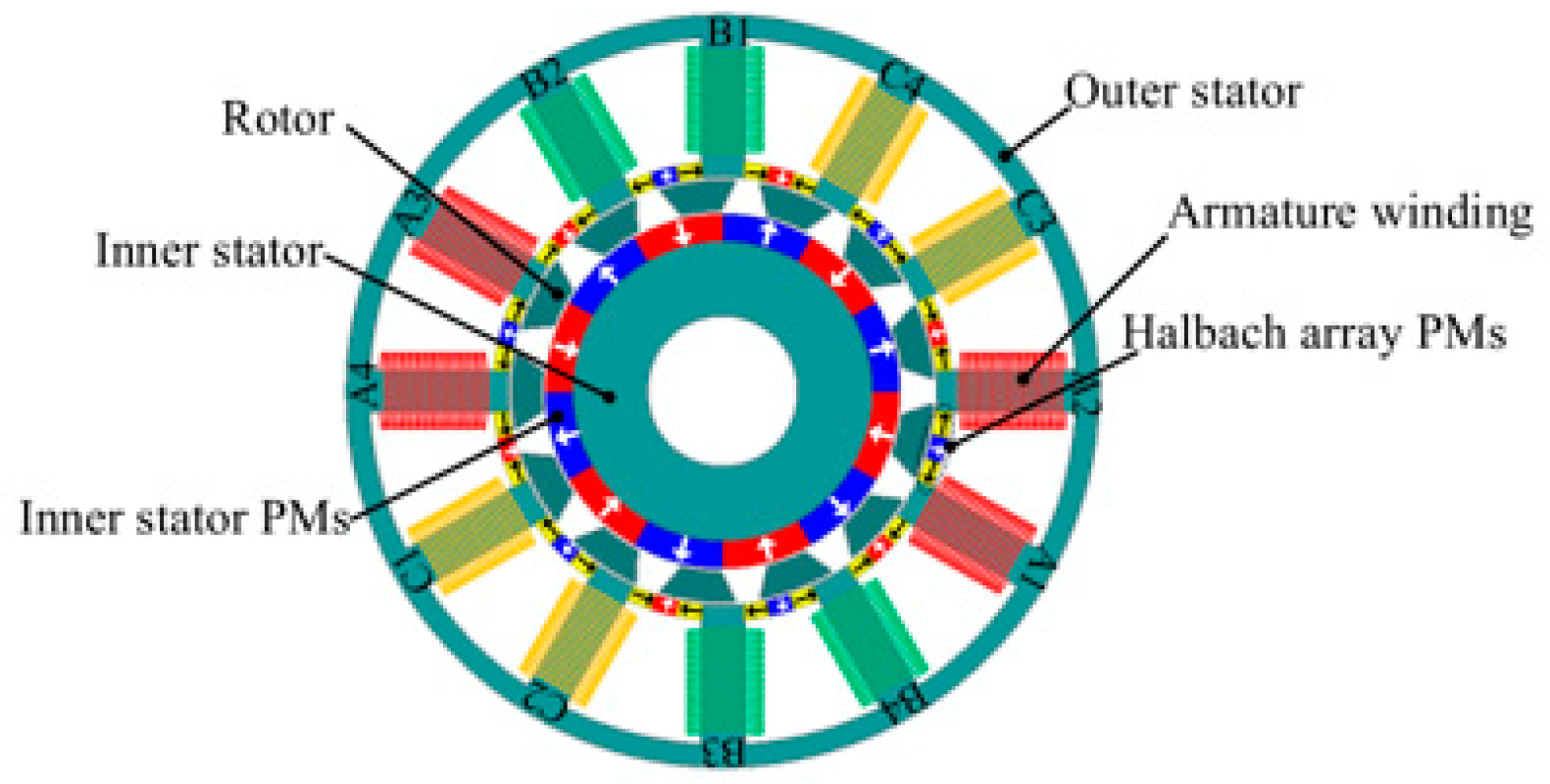
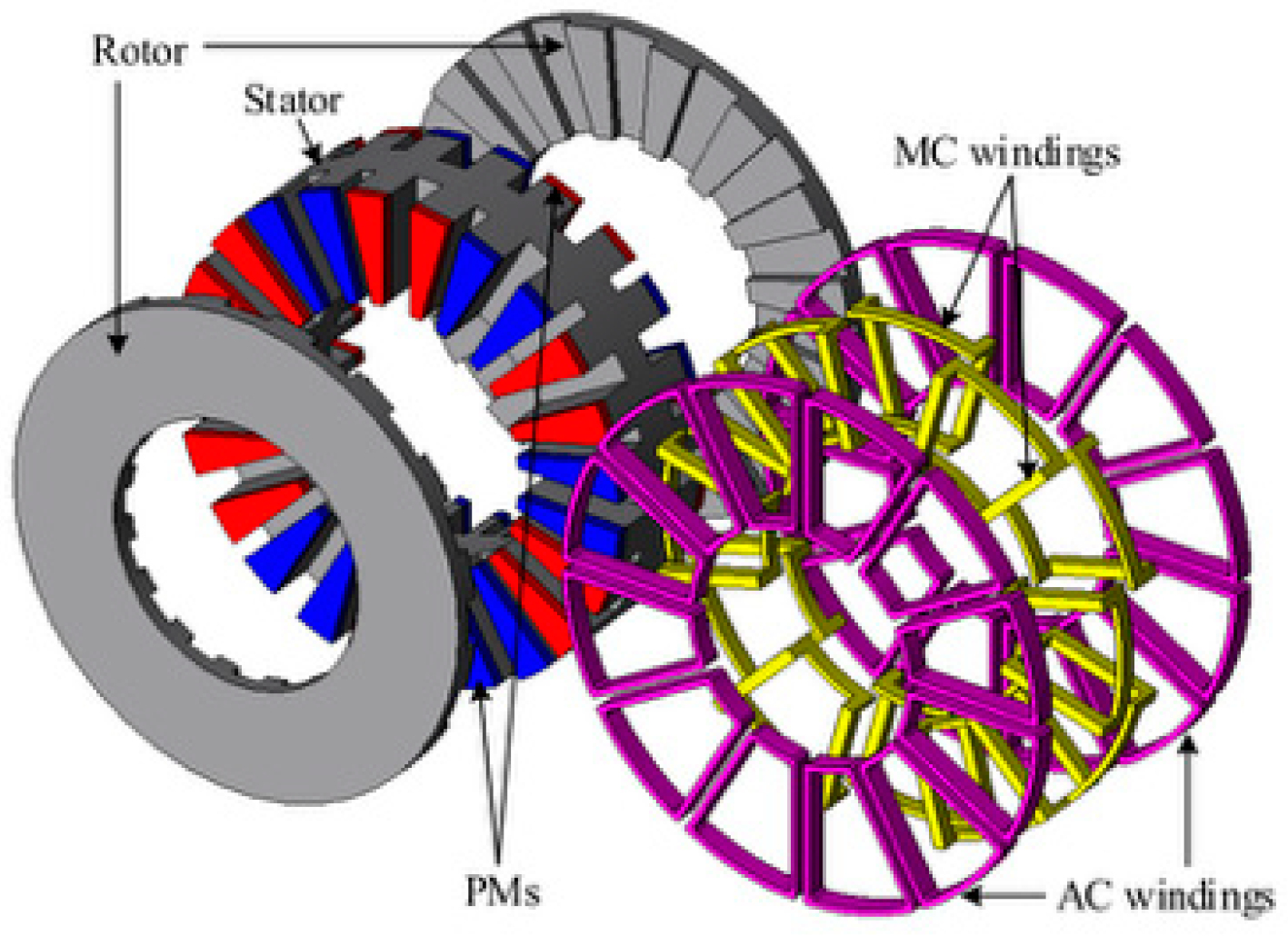

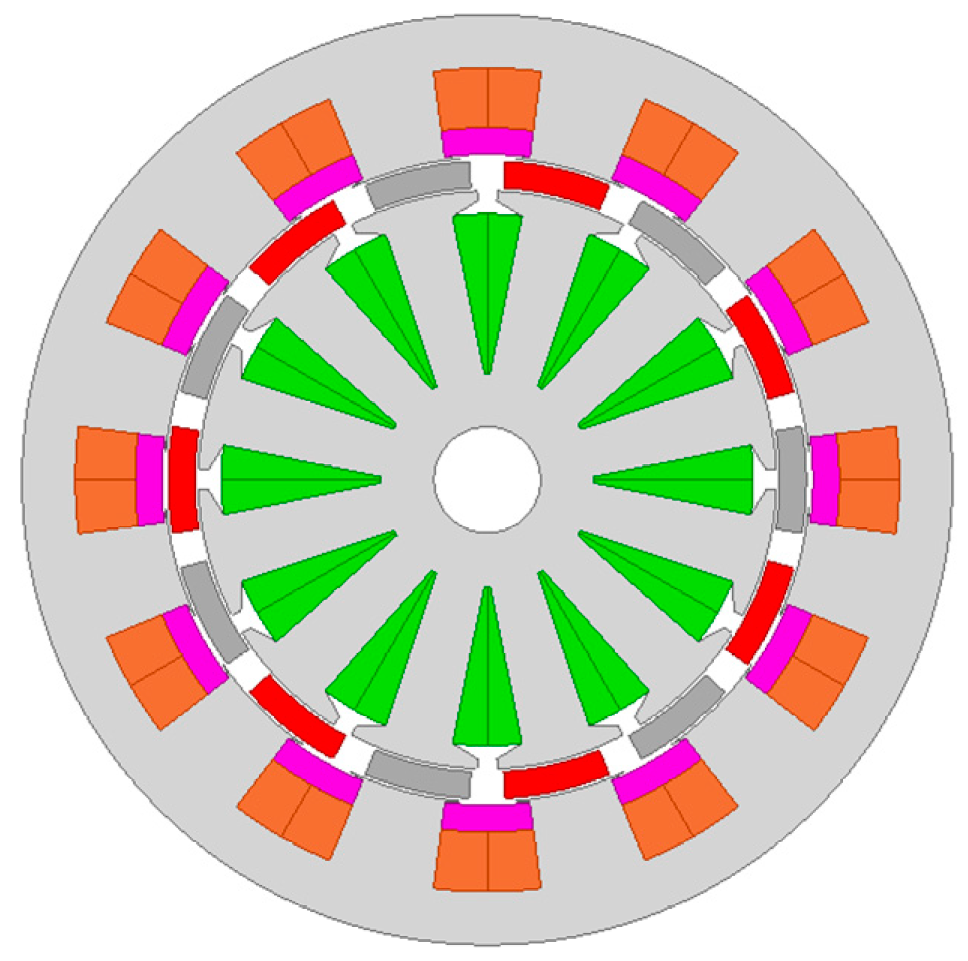
| References | Torque | Torque Ripple | Power Factor | Efficiency | |
|---|---|---|---|---|---|
| [10] | 36% | \ | \ | 30.8% | |
| [14] | 13% | −31.5% | \ | −2% | |
| [15] | 27.9% | −7% | \ | \ | |
| [18] | 40.8~69.01% | −11.74% | \ | \ | |
| [19] | 37% | \ | 0.21 | \ | |
| [20] | 30% | −62% | \ | \ | |
| [21] | High speed | −47.5% | −6.9% | \ | \ |
| Low speed | 43.3% | −4% | \ | \ | |
| [22,23] | 35.4% | −0.1% | \ | \ | |
| [24] | 7% | −5% | \ | \ | |
| [26] | 83% | \ | \ | \ | |
| [28] | 37.5% | \ | \ | −0.9% | |
| [29] | 3.2% | −0.6% | \ | \ | |
| [31] | 6.7% | −4.7% | \ | \ | |
| [32,33] | 36% | \ | 0.38–0.58 | \ | |
| Application Scenarios/Technical Requirements | References | Advantages | Application Area |
|---|---|---|---|
| Magnetic field modulation and magnetic circuit optimization | [14,15,22,23,24] | Increase torque density, reduce magnetic reluctance and leakage | Industrial high torque equipment, wind power generation |
| High torque density design | [18,25,26,29] | Increased torque density and reduced vibration, suitable for MW level loads | Electric vehicles, large generator sets |
| Reliability and fault-tolerant design | [19,31] | Improved power factor, enhanced fault tolerance, and reduced thrust fluctuations | Industrial servo, rail transit drive |
| Compact and axial integration | [28] | Shortened axial length and increased torque density | Ship propulsion and aviation actuation systems |
| Exploring cutting-edge technologies | [21,33] | Expansion of speed range ≥ 1 times, significant increase in superconducting torque | Wide speed domain drive and superconducting motor pre research |
| Reference | Torque | Torque Ripple | Power Factor | Efficiency |
|---|---|---|---|---|
| [39] | −4.2% | −20% | \ | \ |
| [40] | −3.6% | −91.4% | \ | \ |
| [41] | 28.2% | −71.8% | \ | 2.8% |
| [42] | 21% | −7.2% | \ | \ |
| [43] | 18.7% | −92.1% | \ | 1.23% |
| [49] | 210% | \ | \ | −12.8% |
| [50] | 40% | 5.71% | −0.01 | 2.39% |
| [51] | 25.7% | −21.27% | \ | \ |
| [52] | 80% | −38.7% | \ | \ |
| [53] | 7.5% | −76% | \ | \ |
| [55] | 140% | −24% | \ | \ |
| Application Scenarios/Technical Requirements | References | Advantages | Application Area |
|---|---|---|---|
| Torque pulsation suppression and structural optimization | [39,40,42,43] | Reduced torque ripple, improved efficiency, and reduced cogging torque | High precision servo system, linear drive |
| High torque density and excitation enhancement | [41,44,49,51,52] | Torque density improvement, heat dissipation optimization, magnetic saturation suppression | Industrial heavy-duty equipment, rail transit |
| High temperature superconductivity and new excitation technology | [53] | Low torque ripple, strong fault tolerance, and cost reduction | Superconducting motor, high reliability drive |
| Control strategy and improvement of operational accuracy | [54,55] | Improved position/velocity estimation accuracy, homogenized thrust, and enhanced phase independence | Precision positioning system, CNC machine tool |
| References | Torque | Torque Ripple | Power Factor | Efficiency |
|---|---|---|---|---|
| [57] | 0.6% | 21.9% | 0.17 | −23.5% |
| [58] | −20% | −27% | \ | 2.7% |
| [59,60] | 40.6% | −50% | \ | \ |
| [61] | 28.4% | −29.56% | \ | 2.8% |
| [63] | 0.3% | −5.9% | \ | \ |
| [64] | 59% | −2.5% | 0.14 | \ |
| [66] | 0 | −7.1% | 0.1 | 0 |
| [67] | 257% | \ | \ | \ |
| [68] | 48.7% | \ | \ | \ |
| [69] | 2.8% | \ | \ | \ |
| [70] | 100% | \ | \ | 1.17% |
| [71,72] | 16% | \ | \ | 12% |
| [73] | 55.7% | 40% | \ | 16.4% |
| [74,75] | 69.4% | \ | −0.7 | 12.3% |
| Application Scenarios/Technical Requirements | References | Advantages | Application Area |
|---|---|---|---|
| Torque pulsation suppression and harmonic optimization | [57,58,65,73] | Reduced torque ripple and increased back electromotive force | High precision servo system, CNC machine tool |
| High torque density and structural innovation | [59,60,63,66,68,69] | Torque density increase, thrust density increase, dynamic response optimization | Industrial heavy-duty equipment, magnetic differential system |
| Long distance and high thrust applications | [64,70] | Enhanced thrust density, suitable for long travel, and improved high-temperature resistance performance | Rail transit, long-distance transportation system |
| Efficiency improvement and loss reduction | [61,71,72] | PM eddy current loss reduction, efficiency improvement | Energy saving motor, high-frequency drive system |
| Hybrid excitation and advanced control | [67,74,75] | Improved flexibility of magnetic flux regulation and reduced risk of stator saturation | Hybrid drive, intelligent demagnetization system |
| References | Torque | Torque Ripple | Power Factor | Efficiency |
|---|---|---|---|---|
| [77] | 40.4% | \ | \ | 2.04% |
| [78] | 12% | \ | \ | \ |
| [79] | −7% | −97% | \ | \ |
| [80,81] | 80% | 0.09 | 0.12 | 0.03% |
| [82] | 10.6%% | \ | \ | 0.2% |
| [83,84] | −7.2% | −2.45% | 0.07 | \ |
| [85] | 168%% | −0.87% | 0.03 | 3.05% |
| Application Scenarios/Technical Requirements | References | Advantages | Application Area |
|---|---|---|---|
| High torque density and magnetic field modulation optimization | [77,78,80,81,85] | Increased torque density, improved power factor, and enhanced bidirectional magnetic field modulation effect | Electric vehicle drive, industrial heavy-duty equipment |
| Low cogging torque and vibration suppression | [79] | Reduced cogging torque and improved operational stability | Precision servo system, medical equipment |
| Hybrid excitation and flexible adjustment of magnetic flux | [82,85] | Expanded magnetic flux regulation range, combining high torque density and dynamic magnetization capability | Hybrid power system, intelligent demagnetization drive |
| Lateral magnetic flux and special topology applications | [83,84] | Rotating motor torque increases, linear motor thrust density increases, suitable for compact space layout | Aerospace actuators, linear propulsion systems |
| References | Torque Density |
|---|---|
| [88] | 6.6% |
| [90] | 13.2% |
| [91] | 39% |
| [92] | 34% |
| [93] | 11.5% |
| [94] | 14.9% |
| [95] | 3.8% |
| [96] | 33% |
| [98,99] | 13.02% |
| [100] | 7.2% |
| [101] | 15% |
| Application Scenarios/Technical Requirements | References | Advantages | Application Area |
|---|---|---|---|
| Harmonic optimization and magnetic field design | [86,87,88,89,90,91,92] | Optimization of harmonic utilization efficiency and enhancement of back electromotive force | High torque industrial equipment, wind turbines |
| Intelligent control and algorithm optimization | [93,94,95,98,99,100,101] | Improved computational accuracy and accelerated dynamic response speed | Electric vehicle drive, precision servo system |
| High frequency performance and electromagnetic compatibility | [100,101] | Reduced electromagnetic interference and controllable high-frequency losses | High frequency power supply system, aerospace equipment |
| Torque density and efficiency improvement | [96,97,98,99] | Improved efficiency and reduced iron loss | Industrial energy-saving motors, hybrid power systems |
| Reference | Torque Density |
|---|---|
| [102] | −57% |
| [103] | −4.88% |
| [104] | −59.94% |
| [105] | −60% |
| [106] | −39.3% |
| [107] | −8.23% |
| Application Scenarios/Technical Requirements | References | Advantages | Application Area |
|---|---|---|---|
| Harmonic mechanism analysis and optimization | [102,103,104] | Quantify harmonic contributions and establish a ‘harmonic—torque’ model | Electric vehicle drive, industrial robots |
| Intelligent optimization and control strategy | [105,106,107] | Improved low-speed torque accuracy and shortened comprehensive performance optimization cycle | New generation motor prototype design, military special motor |
| Reference | Power Factor |
|---|---|
| [111] | 0.16 |
| [112] | 0.14 |
| [113] | 0.03 |
| [19] | 0.21 |
| [114] | 0.2 |
| [115] | 0.23 |
| [116] | 0.06 |
| [117] | 0.07 |
| [119] | 0.13 |
| Application Scenarios/Technical Requirements | References | Advantages | Application Area |
|---|---|---|---|
| Optimization of air gap magnetic flux harmonics | [19,111,112,113] | Topology optimization of magnetic sources and analysis of magnetic field coupling | Industrial variable frequency drive, new energy generation system |
| Magnetic leakage suppression and precise modeling | [114,115] | Slot leakage sensing control, conformal mapping modeling | High precision servo motors, aerospace actuation systems |
| Collaborative design of winding inductance | [116,117,118,119] | Harmonic inductance relationship modeling, layered optimization | Electric vehicle drive, military special motor |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, B.; Qiao, M.; Xia, Y. Research Status and Latest Progress of Magnetic Field Modulation Motors. Electronics 2025, 14, 1927. https://doi.org/10.3390/electronics14101927
Wu B, Qiao M, Xia Y. Research Status and Latest Progress of Magnetic Field Modulation Motors. Electronics. 2025; 14(10):1927. https://doi.org/10.3390/electronics14101927
Chicago/Turabian StyleWu, Bo, Mingzhong Qiao, and Yihui Xia. 2025. "Research Status and Latest Progress of Magnetic Field Modulation Motors" Electronics 14, no. 10: 1927. https://doi.org/10.3390/electronics14101927
APA StyleWu, B., Qiao, M., & Xia, Y. (2025). Research Status and Latest Progress of Magnetic Field Modulation Motors. Electronics, 14(10), 1927. https://doi.org/10.3390/electronics14101927







