The Condition Number Perspective in Modeling and Designing an Electronic IDBIC Converter
Abstract
1. Introduction
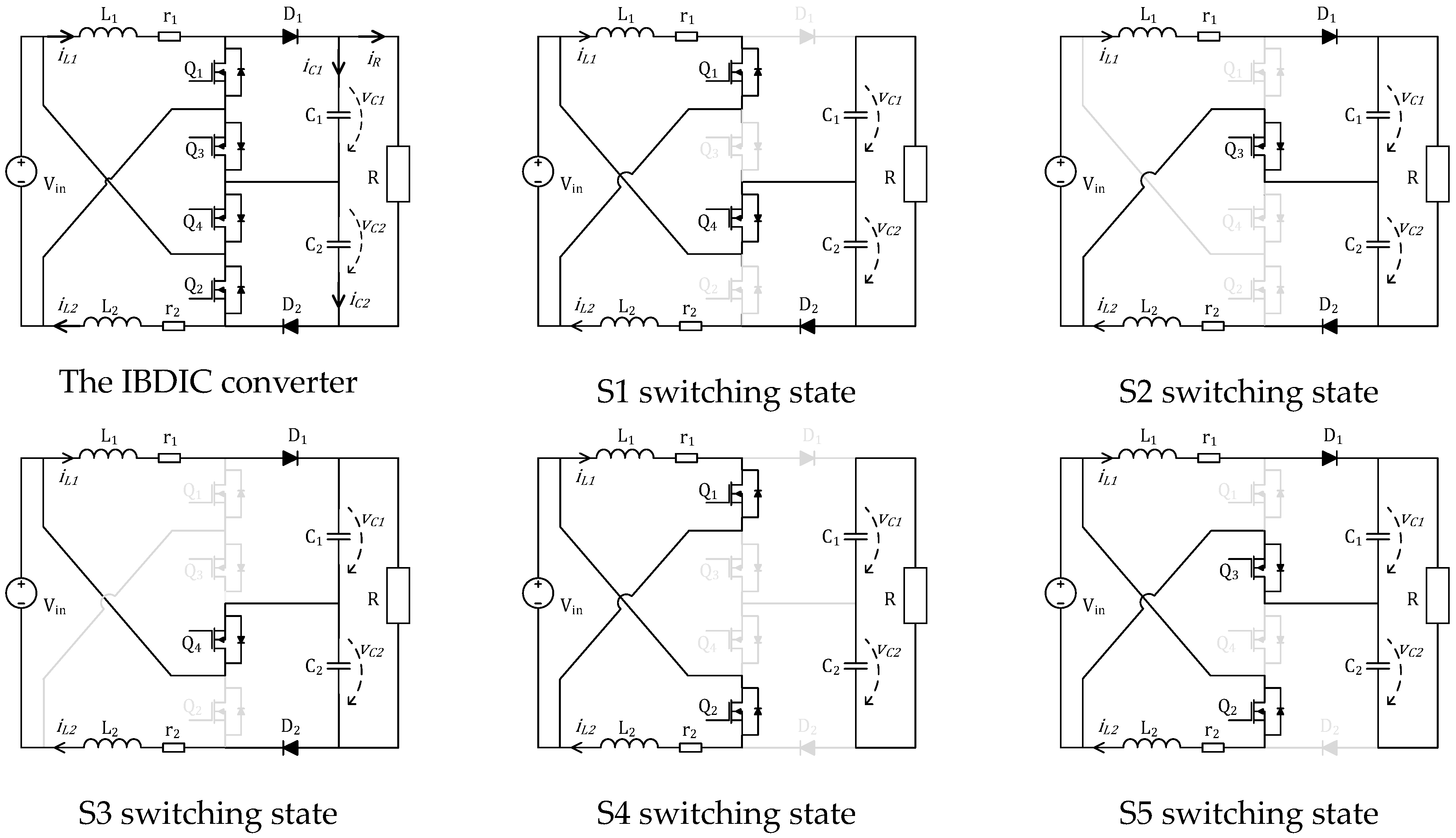
2. Averaged Switching Model of the Converter
2.1. General Representation of the Switching States in CCM
2.2. State-Space Average at CCM Operation and a Duty Cycle below 50%
2.3. State-Space Average at CCM Operation and a Duty Cycle over 50%
3. The Condition Number Background
3.1. The Norm of a Matrix
3.2. The Condition Number of a Matrix
4. The Converter’s Condition Number
4.1. Converter with a Duty Cycle below 50%
- All values of the circuit’s components are positive;
- Considering the differences between the values of the same type of component are very small, it can be approximated that , and ;
- Operating at a duty cycle smaller than 50% results in .
- To establish the maximum between and , both terms are multiplied by , which results in
- Knowing that, in practice [13], , the approximation is made. The relation through which the maximum is defined results in
- In relation (18), we can observe that the norm of the system matrix, hence the condition number, depends on the ratio between the capacitor and inductor values with respect to the duty cycle. By taking into consideration the left-hand/right-hand side positioning of the terms in relation (18) and the order of the terms in relation (15), the norm of is obtained as follows:
- In an equivalent manner, the maximum between and is determined. After obtaining a common denominator, the numerators of and are observed for establishing , as shown in relation (20):
- Considering that a small value of is desired, the approximation will be made. As such, in a practical application [13], we consider that and result in . After some operations, the relation from which the maximum value is derived stands as
- In this situation, the norm of the inverse matrix is also defined by the capacitor and inductor values ratio and the duty cycle. Considering the positioning of the maximum in relation (21) and the terms in relation (16), the norm of results in
4.2. Converter with a Duty Cycle above 50%
- The values of the ratios and are positive
- The values of and are defined as and ;
- has a positive value, less or equal to 1;
- has a value less than or equal to 1, and it can be a negative value;
- can be a negative value, in which case it cannot be a valid solution for the norm;
- By analyzing the possible norm values, the condition number of the matrix can be determined as follows:
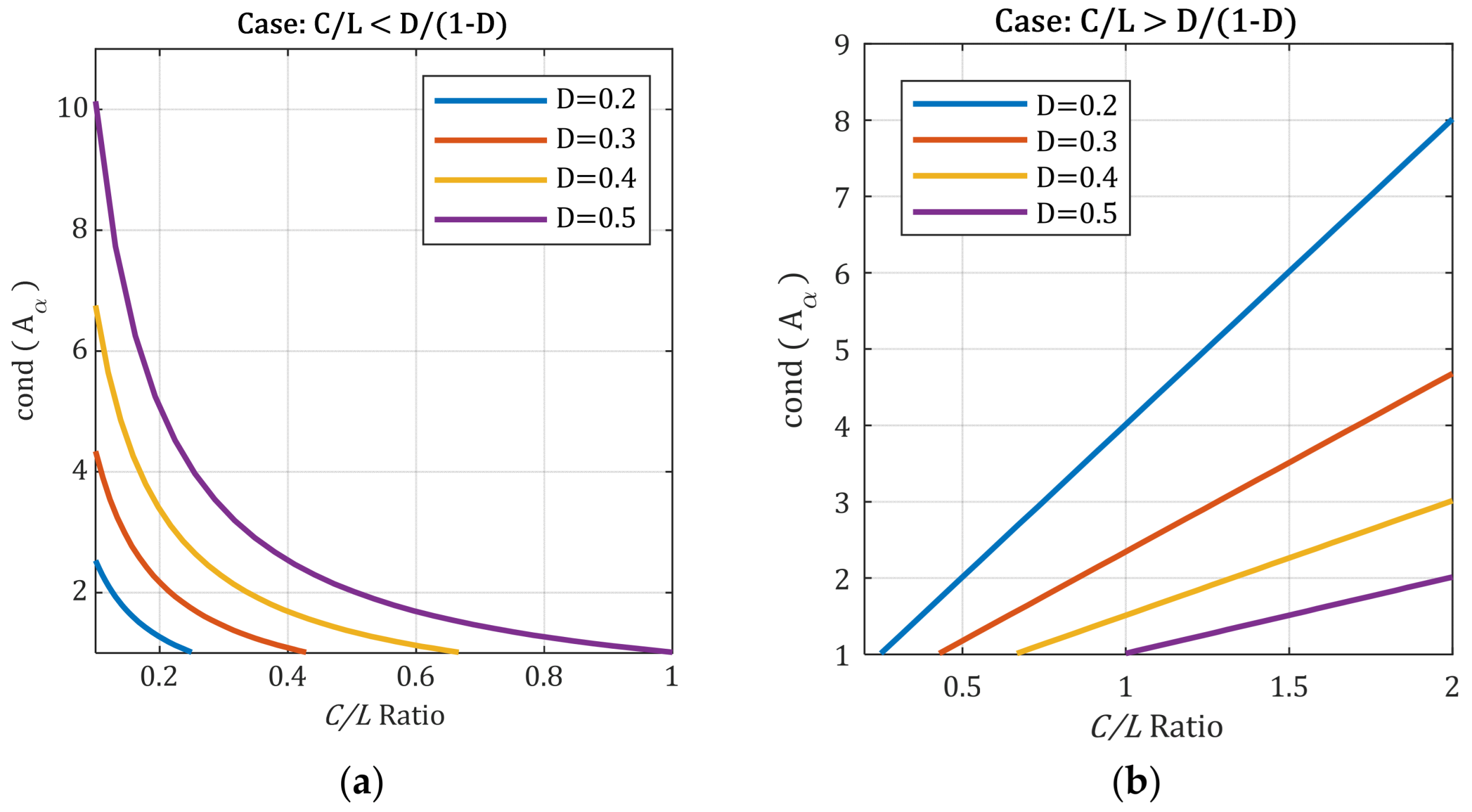
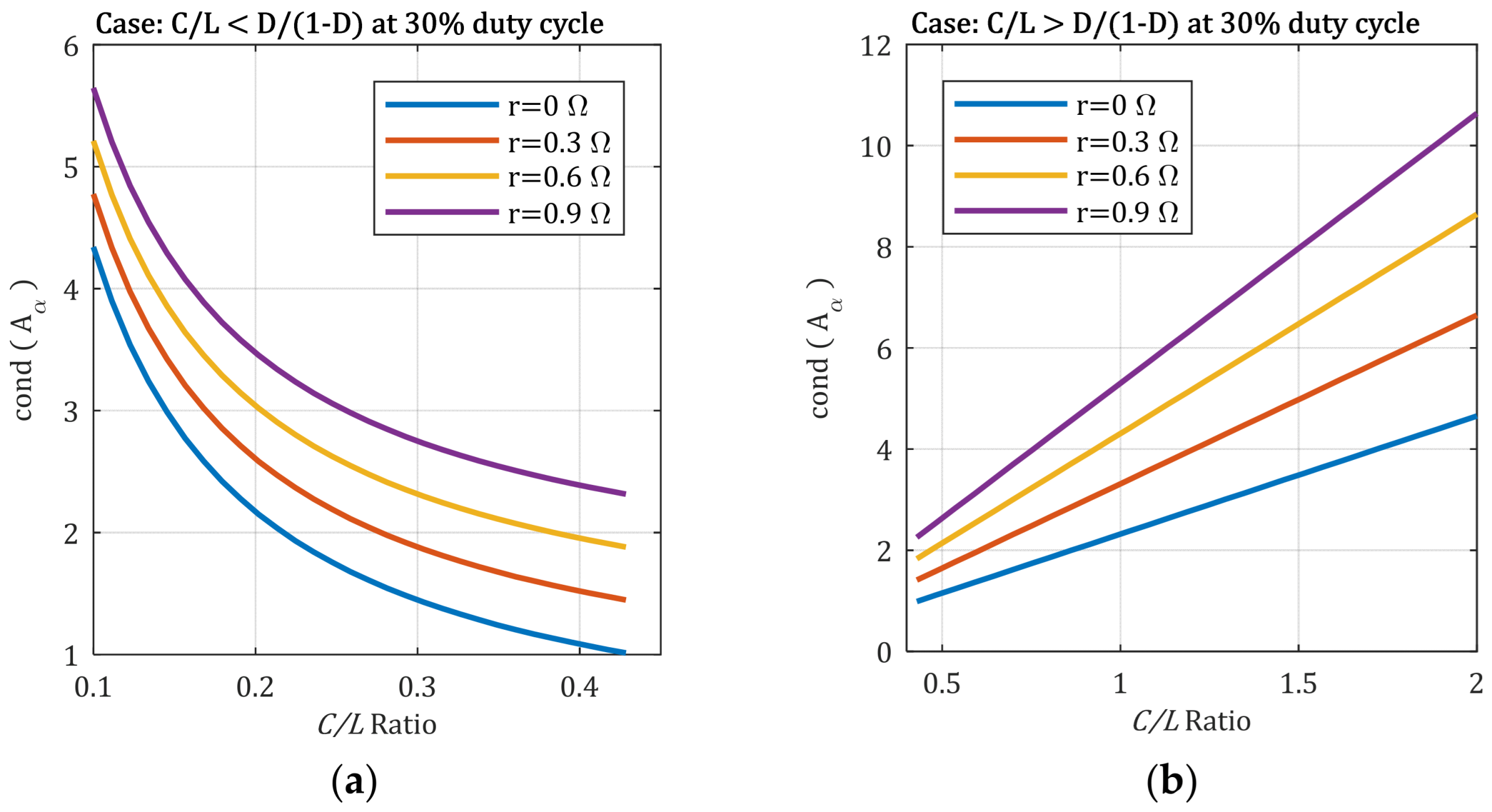
5. The Behavior of the Condition Number
5.1. Converter Operating below a 50% Duty Cycle
5.2. Converter Operating above a 50% Duty Cycle
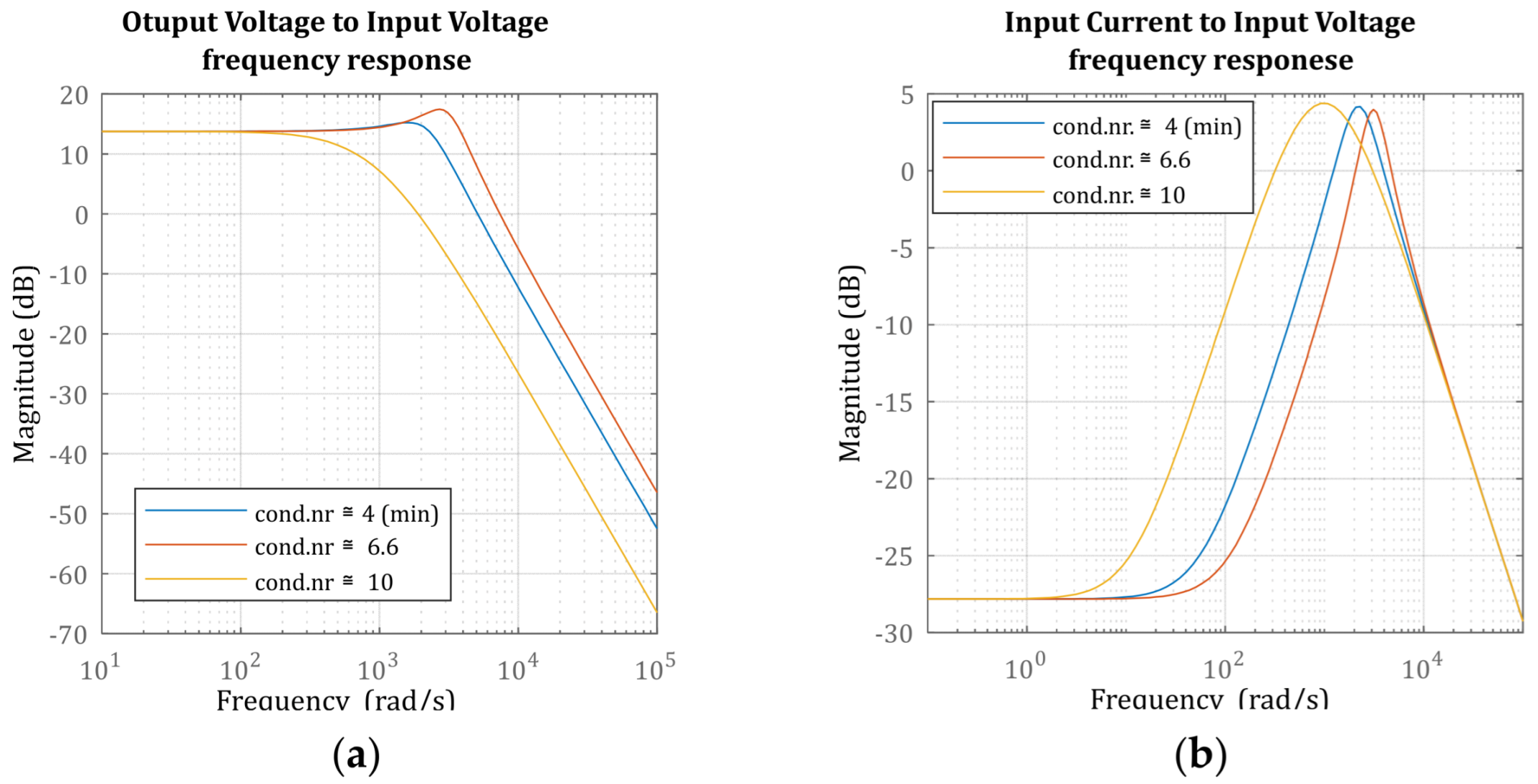
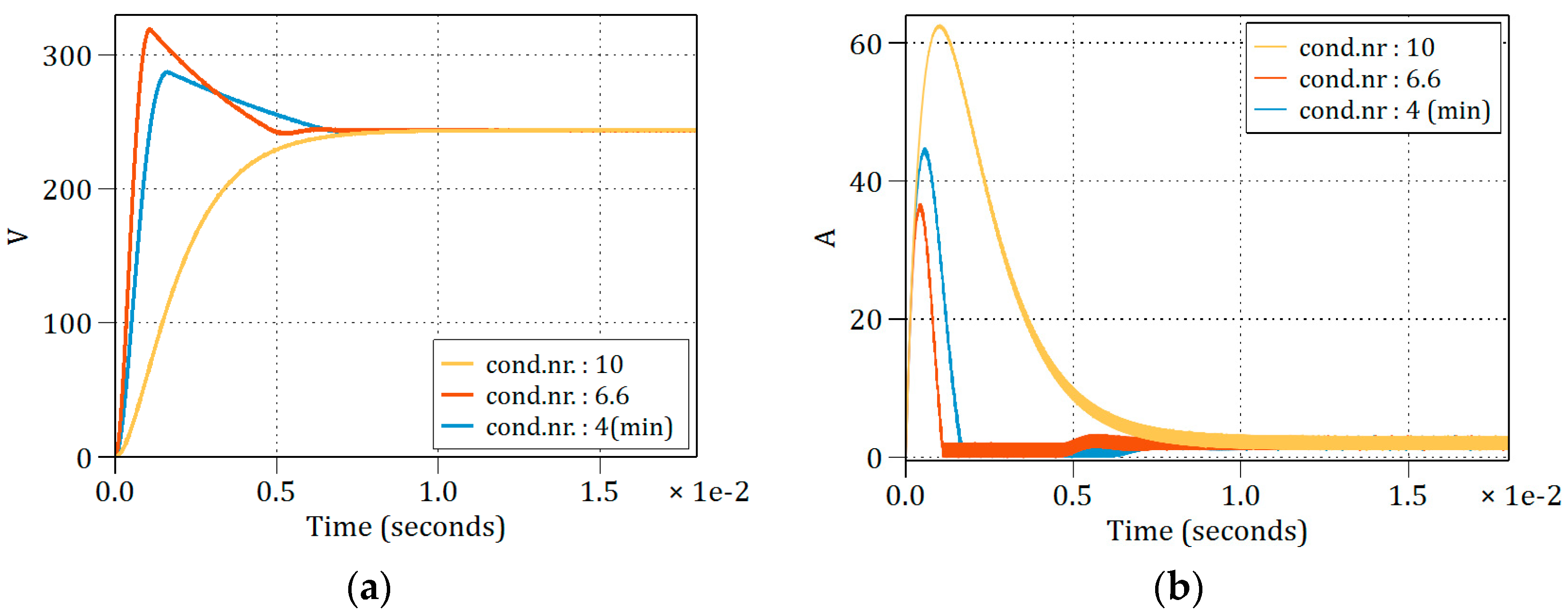
6. The Condition Number and the Converter’s Performance
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yan, D.; Yang, C.; Hang, L.; He, Y.; Luo, P.; Shen, L.; Zeng, P. Review of General Modeling Approaches of Power Converters. Chin. J. Electr. Eng. 2021, 7, 27–36. [Google Scholar] [CrossRef]
- Yue, X.; Wang, X.; Blaabjerg, F. Review of Small-Signal Modeling Methods Including Frequency-Coupling Dynamics of Power Converters. IEEE Trans. Power Electron. 2019, 34, 3313–3328. [Google Scholar] [CrossRef]
- Burdio, J.M.; Martinez, A.; Garcia, J.R. Derivation of Some Classical Modeling Methods for Power Electronic Converters from a Unified Model. In Proceedings of the PESC Record—27th Annual IEEE Power Electronics Specialists Conference, Baveno, Italy, 23–27 June 1996; IEEE: New York, NY, USA; Volume 2, pp. 1382–1387. [Google Scholar]
- Caliskan, V.A.; Verghese, O.C.; Stankovic, A.M. Multifrequency Averaging of DC/DC Converters. IEEE Trans. Power Electron. 1999, 14, 124–133. [Google Scholar] [CrossRef]
- Kwon, J.B.; Wang, X.; Bak, C.L.; Blaabjerg, F. Modeling and Simulation of DC Power Electronics Systems Using Harmonic State Space (HSS) Method. In Proceedings of the 2015 IEEE 16th Workshop on Control and Modeling for Power Electronics (COMPEL), Vancouver, BC, Canada, 12–15 July 2015; IEEE: New York, NY, USA; pp. 1–8. [Google Scholar]
- Jaen, C.; Pindado, R.; Pou, J.; Sala, V. Adaptive Model Applied to PWM DC-DC Converters Using Averaging Techniques. In Proceedings of the 2006 IEEE International Symposium on Industrial Electronics, Montreal, QC, Canada, 9–13 July 2006; IEEE: New York, NY, USA; pp. 1347–1352. [Google Scholar]
- Meher, T.; Majhi, S.; Ramana, K.V. Hammerstein Modeling of Buck Converter Using Relay Feedback. In Proceedings of the TENCON 2019—2019 IEEE Region 10 Conference (TENCON), Kochi, India, 17–20 October 2019; IEEE: New York, NY, USA; pp. 637–642. [Google Scholar]
- Shah, C.; Vasquez-Plaza, J.D.; Campo-Ossa, D.D.; Patarroyo-Montenegro, J.F.; Guruwacharya, N.; Bhujel, N.; Trevizan, R.D.; Rengifo, F.A.; Shirazi, M.; Tonkoski, R.; et al. Review of Dynamic and Transient Modeling of Power Electronic Converters for Converter Dominated Power Systems. IEEE Access 2021, 9, 82094–82117. [Google Scholar] [CrossRef]
- Alcaide, A.M.; Buticchi, G.; Chub, A.; Dalessandro, L. Design and Control for High-Reliability Power Electronics: State-of-the-Art and Future Trends. IEEE J. Emerg. Sel. Top. Ind. Electron. 2024, 5, 50–61. [Google Scholar] [CrossRef]
- Kim, M.; Yun, H.-J. A Basic Design Tool for Grid-Connected AC–DC Converters Using Silcon Carbide MOSFETs. Electronics 2023, 12, 4828. [Google Scholar] [CrossRef]
- Hinov, N.; Gocheva, P.; Gochev, V. Index Matrix-Based Modeling and Simulation of Buck Converter. Mathematics 2023, 11, 4756. [Google Scholar] [CrossRef]
- Ebrahimian, R.; Baldick, R. State Estimator Condition Number Analysis. IEEE Trans. Power Syst. 2001, 16, 273–279. [Google Scholar] [CrossRef]
- Suciu, V.M.; Salcu, S.I.; Pacuraru, A.M.; Pintilie, L.N.; Szekely, N.C.; Teodosescu, P.D. Independent Double-Boost Interleaved Converter with Three-Level Output. Appl. Sci. 2021, 11, 5993. [Google Scholar] [CrossRef]
- Szekely, N.C.; Salcu, S.I.; Suciu, V.M.; Pintilie, L.N.; Fasola, G.I.; Teodosescu, P.D. Power Factor Correction Application Based on Independent Double-Boost Interleaved Converter (IDBIC). Appl. Sci. 2022, 12, 7209. [Google Scholar] [CrossRef]
- Ascher, U.M.; Greif, C. A First Course in Numerical Methods; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 2011; ISBN 978-0-89871-997-0. [Google Scholar]
- Trefethen, L.N.; Bau, D. III. Numerical Linear Algebra; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 1997; ISBN 0-89871-361-7. [Google Scholar]
- Sauer, T. (Ed.) Numerical Analysis, 2nd ed.; Pearson: Boston, MA, USA, 2012; ISBN 978-0-321-78367-7. [Google Scholar]
- Burden, A.M.; Faires, J.D.; Burden, R.L. Numerical Analysis, 10th ed.; Cengage Learning: Boston, MA, USA, 2016; ISBN 978-1-305-25366-7. [Google Scholar]

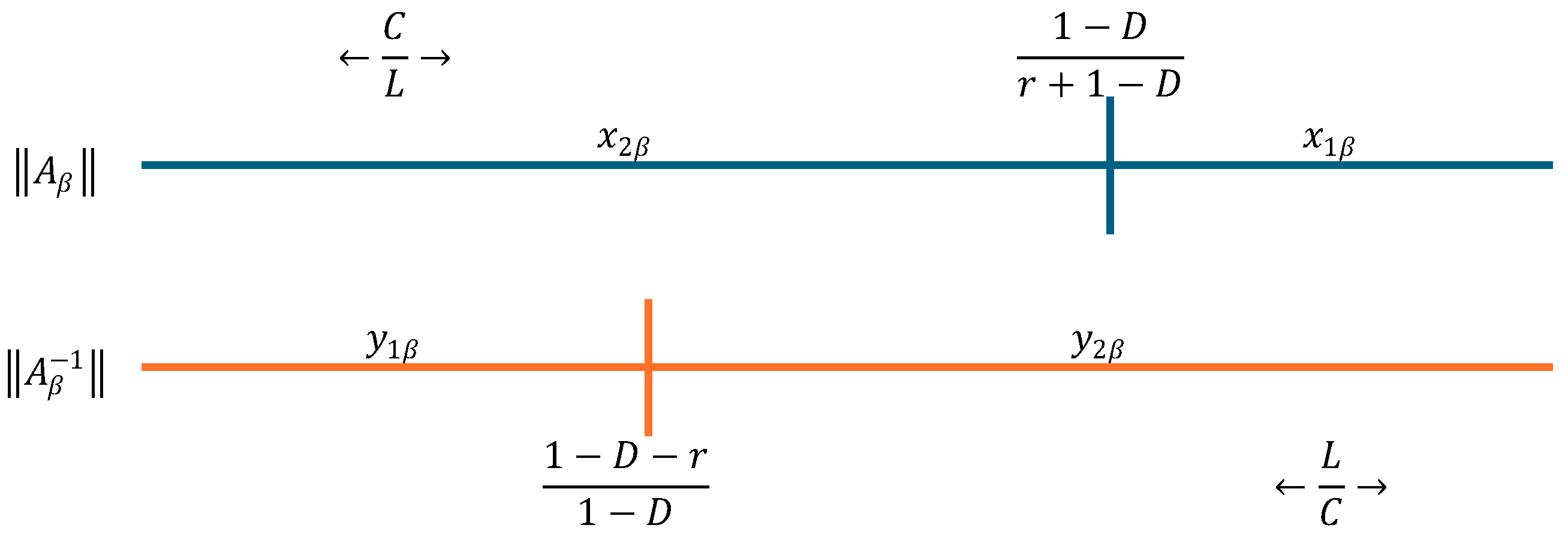





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salcu, S.I.; Suciu, V.M.; Teodosescu, P.D.; Mathe, Z. The Condition Number Perspective in Modeling and Designing an Electronic IDBIC Converter. Electronics 2024, 13, 1302. https://doi.org/10.3390/electronics13071302
Salcu SI, Suciu VM, Teodosescu PD, Mathe Z. The Condition Number Perspective in Modeling and Designing an Electronic IDBIC Converter. Electronics. 2024; 13(7):1302. https://doi.org/10.3390/electronics13071302
Chicago/Turabian StyleSalcu, Sorin Ionut, Vasile Mihai Suciu, Petre Dorel Teodosescu, and Zsolt Mathe. 2024. "The Condition Number Perspective in Modeling and Designing an Electronic IDBIC Converter" Electronics 13, no. 7: 1302. https://doi.org/10.3390/electronics13071302
APA StyleSalcu, S. I., Suciu, V. M., Teodosescu, P. D., & Mathe, Z. (2024). The Condition Number Perspective in Modeling and Designing an Electronic IDBIC Converter. Electronics, 13(7), 1302. https://doi.org/10.3390/electronics13071302






