Susceptibility to Low-Frequency Breakdown in Full-Wave Models of Liquid Crystal-Coaxially-Filled Noise-Shielded Analog Phase Shifters
Abstract
1. Introduction
2. Materials and Methods
- The size must ensure pure TEM mode operation at all frequency points, without exciting any higher-order modes. The highest frequency under consideration (60 GHz in this case) is most susceptible to higher-order mode excitation. Therefore, the central pin diameter (Dcore) is chosen as 0.23 mm, the industry-standard for 60 GHz millimeter-wave operation, to guarantee single-mode behavior.
- TLC and Dcore are calculated using the characteristic impedance () formula derived from TEM mode analysis [47]. With one parameter fixed, the other is determined according to Equation (2):
- TLC must also comply with liquid crystal operational constraints, specifically within the classically defined rubbing interaction range (i.e., it should not be too thick). Using the 60 GHz case as a baseline is more appropriate than using lower frequencies, as a larger Dcore leads to a thicker TLC under the impedance-matching condition.
- Finally, designing the geometry based on the highest frequency point (60 GHz) amplifies the LFB vulnerability. For long-wavelength applications (e.g., 1 Hz, 1 kHz, and 1 MHz as listed in Table 1), the TLC (0.34876 mm) and Dcore (0.23 mm) dimensions, optimized for the 60 GHz standard, offer a highly area-efficient solution to the 50 Ω impedance-matching equation. However, LFB may still occur in full-wave computational simulations, as the wave-like behavior becomes infinitesimally small relative to the tiny structure. The multi-scale evolution of the LFB susceptibility is intuitively schematized in Figure 2. The multiple frequencies presented in this study correspond to the transmitted signal (or low-frequency biasing signal) undergoing phase shifting and subsequent analysis through simulations, where the quasi-static or low-frequency bias cannot be simulated independently (separately). It is crucial to carefully assess the potential computational impact (e.g., LFB errors) of the liquid crystal (LC) material’s low-frequency bias on the simulation of the transmitted signal across various frequencies, in addition to the practical coupling effects (crosstalk) between the mixed signals and the influence of noise from instrumentation.
3. Results
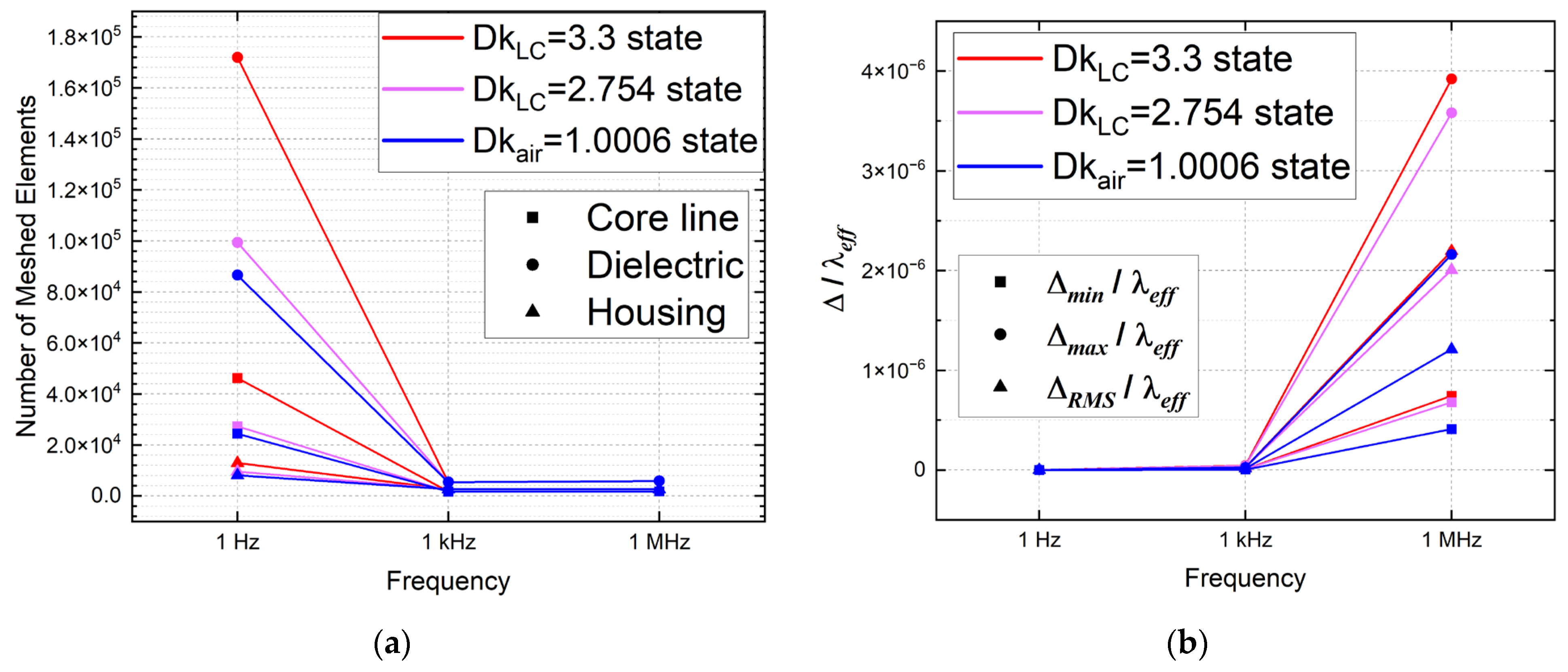
3.1. Comparison of Solution Statistics
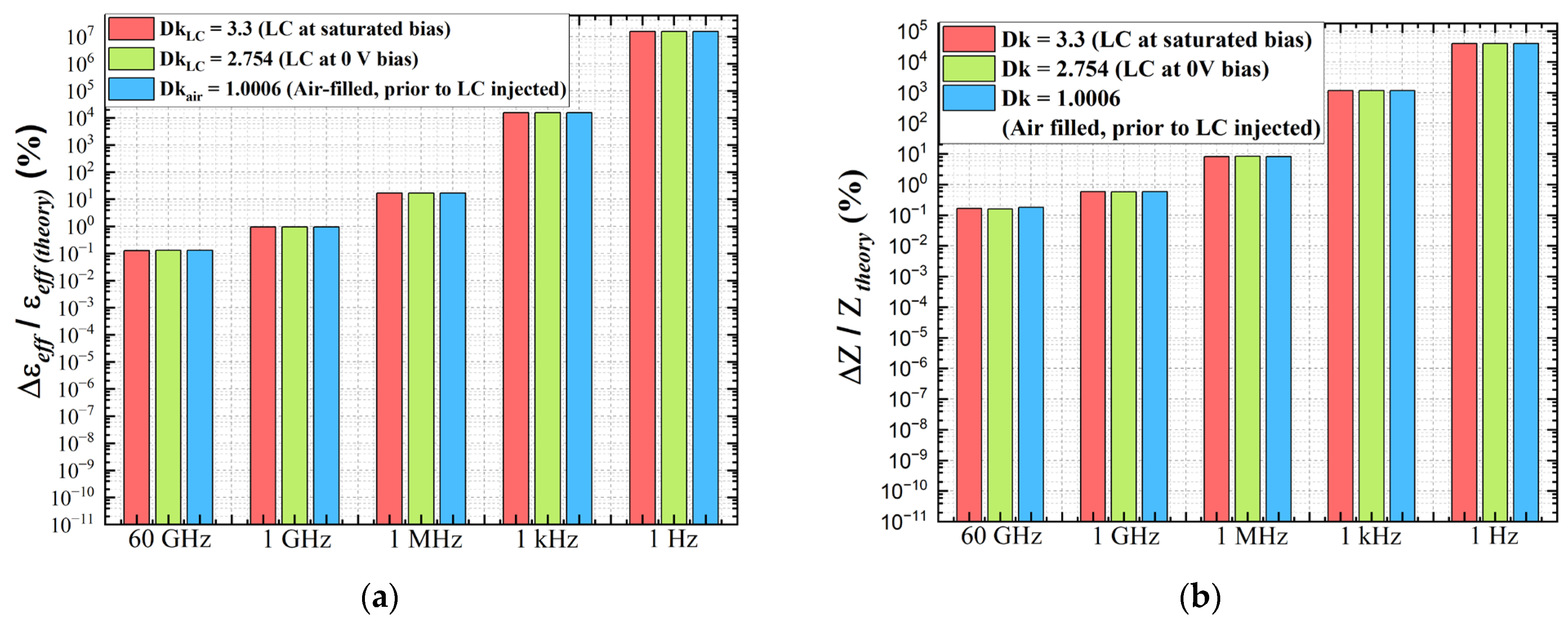
3.2. Errors in Cross-Spectrum Performance Prediction of Cross-Sections
3.3. Mesh Illustrations
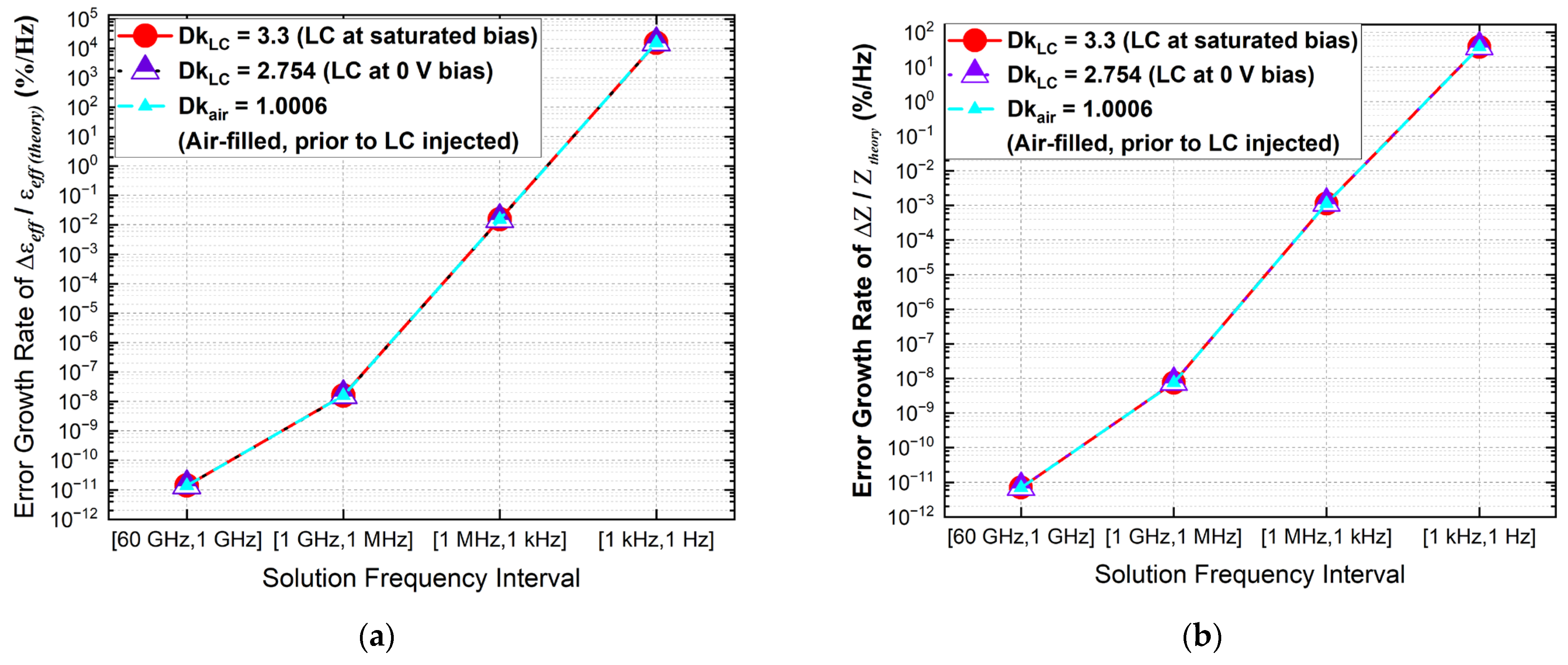
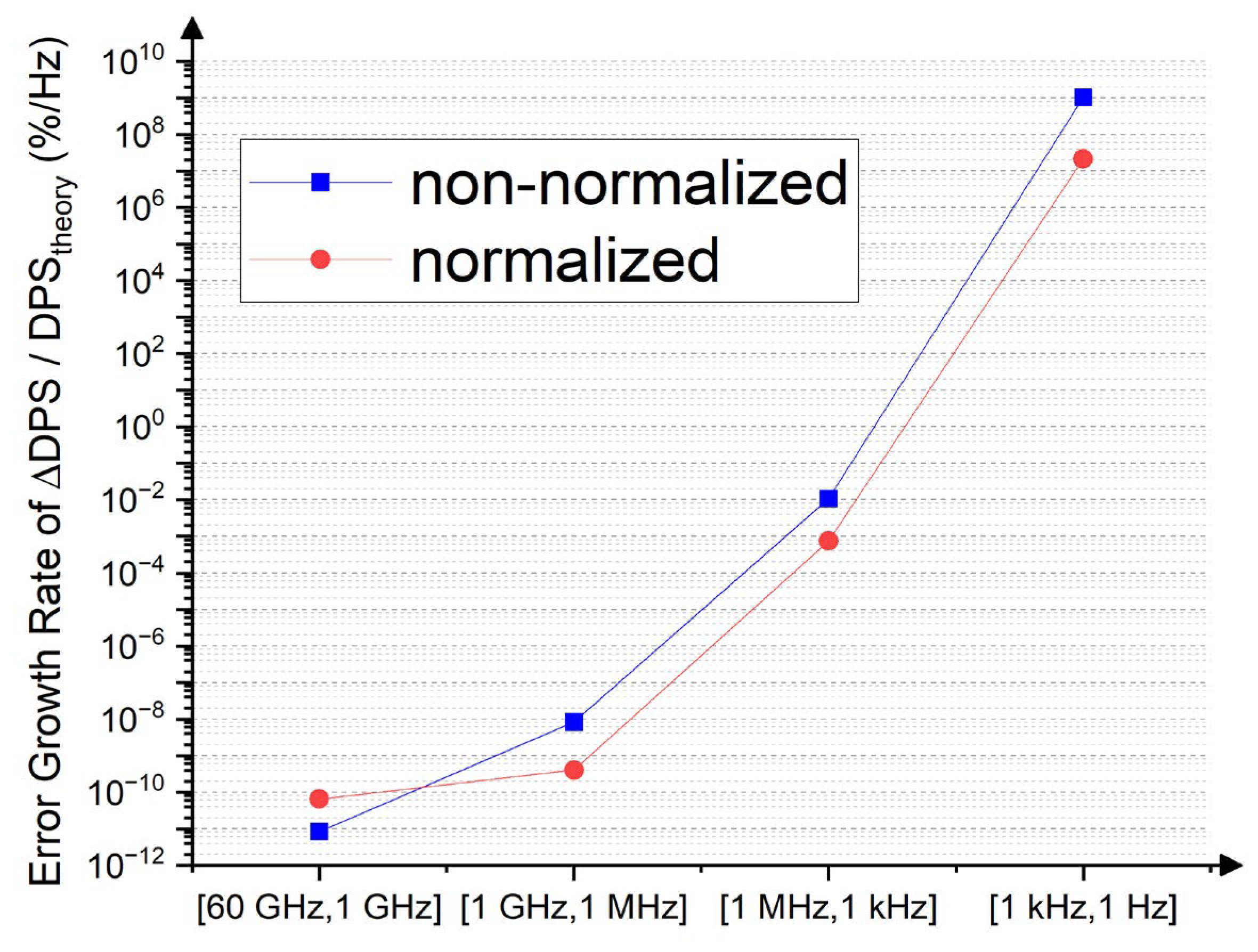
3.4. Errors in Differential Phase Shift Behavior
4. Discussion and Generalizations
4.1. Analysis of Root Causes and Associated Complexities
4.2. Implications for Next-Generation B5G/6G Communication-Sensing Integrated Platforms
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| Nomenclatures | Abbreviations |
| Low-frequency breakdown | LFB |
| Cross-section | XS |
| Liquid crystal | LC |
| Liquid crystal thickness | TLC |
| Core line diameter | Dcore |
| Length | L |
| Polyimide | PI |
| Direct current | DC |
| Alternating current | AC |
| Extremely low frequency | ELF |
| Super low frequency | SLF |
| Ultra-low frequency | ULF |
| Microwave-wave | MW |
| Millimeter-wave | mmW |
| Electromagnetic interference | EMI |
| Kilometer | km |
| Megameter | Mm |
| Gigahertz | GHz |
| Terahertz | THz |
| Root mean square | RMS |
| Scattering parameters | S parameters |
| Forward transmission coefficient | S21 |
| Forward reflection coefficient | S11 |
| Characteristic impedance | ZC |
| Differential phase shift | DPS |
| Dielectric constant | Dk |
| Dissipation factor | DF |
| Effective permittivity | |
| Effective wavelength | |
| Two dimensional | 2D |
| Three dimensional | 3D |
| Fifth-generation wireless | 5G |
| Sixth-generation wireless | 6G |
| Wireless Gigabit | WiGig |
| Advanced driver assistance systems | ADAS |
| Light detection and ranging | LiDAR |
| Internet of Things | IoT |
| Internet of Nano-Things | IoNT |
| Finite-element method | FEM |
| High-frequency structure simulator | HFSS |
| High-performance computing | HPC |
| Partial-differential equation | PDE |
| Integral equation | IE |
| Artificial intelligence | AI |
Appendix A
References
- Boyarsky, M.; Sleasman, T.; Imani, M.F.; Gollub, J.N.; Smith, D.R. Electronically steered metasurface antenna. Sci. Rep. 2021, 11, 4693. [Google Scholar] [CrossRef] [PubMed]
- Mumcu, G.; Kacar, M.; Mendoza, J. Mm-Wave Beam Steering Antenna with Reduced Hardware Complexity Using Lens Antenna Subarrays. IEEE Antennas Wirel. Propag. Lett. 2018, 17, 1603–1607. [Google Scholar] [CrossRef]
- Nikfalazar, M.; Sazegar, M.; Mehmood, A.; Wiens, A.; Friederich, A.; Maune, H. Two-Dimensional Beam-Steering Phased-Array Antenna With Compact Tunable Phase Shifter Based on BST Thick Films. IEEE Antennas Wirel. Propag. Lett. 2017, 16, 585–588. [Google Scholar] [CrossRef]
- Ali, M.; Guzmán, R.C.; Muñoz, L.E.G.; Dijk, F.V.; Carpintero, G. Photonics-Enabled Millimetre-Wave Phased-Array Antenna with True Time Delay Beam-Steering. In Proceedings of the 2020 50th European Microwave Conference (EuMC), Utrecht, The Netherlands, 12–14 January 2021; pp. 316–319. [Google Scholar]
- Yang, D.H.; Lin, W.P. Phased-array beam steering using optical true time delay technique. Opt. Commun. 2015, 350, 90–96. [Google Scholar] [CrossRef]
- Wymeersch, H.; Saleh, S.; Nimr, A. Joint Communication and Sensing for 6G—A Cross-Layer Perspective. In Proceedings of the 2024 IEEE 4th International Symposium on Joint Communications & Sensing (JC&S), Leuven, Belgium, 19–21 March 2024; pp. 1–6. [Google Scholar]
- Abdulkarim, Y.I.; Alkurt, F.Ö.; Bakır, M.; Awl, H.N.; Muhammadsharif, F.F.; Karaaslan, M.; Appasani, B.; Badri, K.S.L.A.; Zhu, Y.Y.; Dong, J. A polarization-insensitive triple-band perfect metamaterial absorber incorporating ZnSe for terahertz sensing. J. Opt. 2022, 24, 105102. [Google Scholar] [CrossRef]
- Zhang, J.A.; Rahman, M.L.; Wu, K.; Huang, X.J.; Guo, Y.J.; Chen, S.Z. Enabling Joint Communication and Radar Sensing in Mobile Networks—A Survey. IEEE Commun. Surv. Tutor. 2022, 24, 306–345. [Google Scholar] [CrossRef]
- Abdalmalak, K.A.; Botello, G.S.; Suresh, M.I.; Falcón-Gómez, E.; Lavado, A.R.; García-Muñoz, L.E. An Integrated Millimeter-Wave Satellite Radiometer Working at Room-Temperature with High Photon Conversion Efficiency. Sensors 2022, 22, 2400. [Google Scholar] [CrossRef]
- Delamotte, T.; Schraml, M.G.; Schwarz, R.T.; Storek, K.U.; Knopp, A. Multi-Antenna-Enabled 6G Satellite Systems: Roadmap, Challenges and Opportunities. In Proceedings of the 25th International ITG Workshop on Smart Antennas, French Riviera, France, 10–12 November 2021; pp. 1–6. [Google Scholar]
- Maduranga, M.W.P.; Tilwari, V.; Rathnayake, R.M.M.R.; Sandamini, C. AI-Enabled 6G Internet of Things: Opportunities, Key Technologies, Challenges, and Future Directions. Telecom 2024, 5, 804–822. [Google Scholar] [CrossRef]
- Gimenez, S.; Roger, S.; Baracca, P.; Martín-Sacristán, D.; Monserrat, J.F.; Braun, V.; Halbauer, H. Performance Evaluation of Analog Beamforming with Hardware Impairments for mmW Massive MIMO Communication in an Urban Scenario. Sensors 2016, 16, 1555. [Google Scholar] [CrossRef]
- Cahoon, N.; Srinivasan, P.; Guarin, F. 6G Roadmap for Semiconductor Technologies: Challenges and Advances. In Proceedings of the 2022 IEEE International Reliability Physics Symposium (IRPS), Dallas, TX, USA, 27–31 March 2022; pp. 11B.1-1–11B.1-9. [Google Scholar]
- Ragonese, E. Design Techniques for Low-Voltage RF/mm-Wave Circuits in Nanometer CMOS Technologies. Appl. Sci. 2022, 12, 2103. [Google Scholar] [CrossRef]
- Lavado, A.R.; Muñoz, L.E.G.; Lioubtchenko, D.; Preu, S.; Abdalmalak, K.A.; Botello, G.S.; Vargas, D.S.; Räisänen, A.V. Planar Lens–Based Ultra-Wideband Dielectric Rod Waveguide Antenna for Tunable THz and Sub-THz Photomixer Sources. J. Infrared Milli. Terahz. Waves 2019, 40, 838–855. [Google Scholar] [CrossRef]
- Li, J.; Li, H. Modeling 0.3 THz Coaxial Single-Mode Phase Shifter Designs in Liquid Crystals with Constitutive Loss Quantifications. Crystals 2024, 14, 364. [Google Scholar] [CrossRef]
- Jakoby, R.; Gaebler, A.; Weickhmann, C. Microwave liquid crystal enabling technology for electronically steerable antennas in SATCOM and 5G millimeter-wave systems. Crystals 2020, 10, 514. [Google Scholar] [CrossRef]
- Garbovskiy, Y.; Zagorodnii, V.; Krivosik, P.; Lovejoy, J.; Camley, R.E.; Celinski, Z.; Glushchenko, A.; Dziaduszek, J.; Dąbrowski, R. Liquid crystal phase shifters at millimetre wave frequencies. J. Appl. Phys. 2012, 111, 054504. [Google Scholar] [CrossRef]
- Li, J.; Chu, D. Liquid Crystal-Based Enclosed Coplanar Waveguide Phase Shifter for 54–66 GHz Applications. Crystals 2019, 9, 650. [Google Scholar] [CrossRef]
- Kim, D.; Kim, K.; Saeed, M.H.; Choi, S.; Na, J.H. Fast Reconfigurable Phase Shifter Based on a Chiral Liquid Crystal Configuration. IEEE Access 2023, 11, 60817–60826. [Google Scholar] [CrossRef]
- White, J.F. Diode Phase Shifters for Array Antennas. IEEE Trans. Microw. Theory Tech. 1974, 22, 658–674. [Google Scholar] [CrossRef]
- Massoni, E.; Desmarres, D.; Ezzeddineet, H.; Sittler, F.; Thomas, S.C.; Benoit-Gonin, J.; Tosetti, E.; Moscatelli, A.; Di Paola, N.; Lemarchand, O. An Integrated Passive Device RF Front-End for Narrow-Band Internet-of-Things Modules. In Proceedings of the 2021 IEEE MTT-S International Microwave Filter Workshop (IMFW), Perugia, Italy, 17–19 November 2021; pp. 221–223. [Google Scholar]
- Ballo, A.; Grasso, A.D.; Palumbo, G. Very-Low-Voltage Charge Pump Topologies for IoT Applications. IEEE Trans. Circuits Syst. I Regul. Pap. 2023, 70, 2283–2292. [Google Scholar] [CrossRef]
- Bozzi, M.; Moscato, S.; Silvestri, L.; Massoni, E.; Delmonte, N.; Rocco, G.M. Novel materials and fabrication technologies for SIW components for the Internet of Things. In Proceedings of the 2016 IEEE International Workshop on Electromagnetics: Applications and Student Innovation Competition (iWEM), Nanjing, China, 16–18 May 2016; pp. 1–3. [Google Scholar]
- Sala, R.D.; Centurelli, F.; Scotti, G.; Palumbo, G. Rail to Rail ICMR and High Performance ULV Standard-Cell-Based Comparator for Biomedical and IoT Applications. IEEE Access 2024, 12, 4642–4659. [Google Scholar] [CrossRef]
- Alabdulatif, A.; Thilakarathne, N.N.; Lawal, Z.K.; Fahim, K.E.; Zakari, R.Y. Internet of Nano-Things (IoNT): A Comprehensive Review from Architecture to Security and Privacy Challenges. Sensors 2023, 23, 2807. [Google Scholar] [CrossRef]
- Liakos, K.G.; Georgakilas, G.K.; Plessas, F.C.; Kitsos, P. GAINESIS: Generative Artificial Intelligence NEtlists SynthesIS. Electronics 2022, 11, 245. [Google Scholar] [CrossRef]
- Pantoli, L.; Leoni, A.; Marinković, Z. Calibration Strategy for Tunable Devices Based on Artificial Neural Network Modelling. In Proceedings of the 2020 5th International Conference on Smart and Sustainable Technologies (SpliTech), Split, Croatia, 23–26 September 2020; pp. 1–3. [Google Scholar]
- Kalapothas, S.; Flamis, G.; Kitsos, P. Efficient Edge-AI Application Deployment for FPGAs. Information 2022, 13, 279. [Google Scholar] [CrossRef]
- Li, J. Machine Learning and Digital Twinning Enabled Liquid Crystals mm-Wave Reconfigurable Devices Design and Systems Operation. In Proceedings of the 2022 IEEE MTT-S International Microwave Workshop Series on Advanced Materials and Processes for RF and THz Applications (IMWS-AMP), Guangzhou, China, 27–29 November 2022; pp. 1–3. [Google Scholar]
- Weil, C.; Luessem, G.; Jakoby, R. Tunable inverted-microstrip phase shifter device using nematic liquid crystals. In Proceedings of the 2002 IEEE MTT-S International Microwave Symposium Digest, Seattle, WA, USA, 2–7 June 2002; pp. 367–371. [Google Scholar]
- Li, J. Rethinking Liquid Crystal Tunable Phase Shifter Design with Inverted Microstrip Lines at 1–67 GHz by Dissipative Loss Analysis. Electronics 2023, 12, 421. [Google Scholar] [CrossRef]
- Althuwayb, A.A. The Dilemma of Resolving the Low-Frequency Breakdown Problem in Microwave Components via Traditional and Improved Finite-Element Time-Domain Techniques. IEEE Access 2022, 10, 42171–42180. [Google Scholar] [CrossRef]
- Zhu, J.; Jiao, D. A Theoretically Rigorous Full-Wave Finite-Element-Based Solution of Maxwell’s Equations From dc to High Frequencies. IEEE Trans. Adv. Packag. 2010, 33, 1043–1050. [Google Scholar] [CrossRef]
- Moore, R.K. Radio communication in the sea. IEEE Spectr. 1967, 4, 42–51. [Google Scholar] [CrossRef]
- Turner, R.W. Submarine Communication Antenna Systems. Proc. Ire 2007, 47, 735–739. [Google Scholar] [CrossRef]
- Zhu, J.; Jiao, D. A Rigorous Solution to the Low-Frequency Breakdown in Full-Wave Finite-Element-Based Analysis of General Problems Involving Inhomogeneous Lossless/Lossy Dielectrics and Nonideal Conductors. IEEE Trans. Microw. Theory Tech. 2011, 59, 3294–3306. [Google Scholar] [CrossRef]
- Zhu, J.; Jiao, D. Fast Full-Wave Solution That Eliminates the Low-Frequency Breakdown Problem in a Reduced System of Order One. IEEE Trans. Compon. Packag. Manuf. Technol. 2012, 2, 1871–1881. [Google Scholar]
- Zhu, J.; Omar, S.; Jiao, D. Solution of the Electric Field Integral Equation When It Breaks Down. IEEE Trans. Antennas Propag. 2014, 62, 4122–4134. [Google Scholar] [CrossRef]
- Qian, Z.G.; Chew, W.C. An augmented electric field integral equation for high-speed interconnect analysis. Microw. Opt. Technol. Lett. 2008, 50, 2658–2662. [Google Scholar] [CrossRef]
- Qian, Z.G.; Chew, W.C. A quantitative study on the low frequency breakdown of EFIE. Microw. Opt. Technol. Lett. 2008, 50, 1159–1162. [Google Scholar] [CrossRef]
- Zhu, J.; Jiao, D. Solution to the Low-Frequency Breakdown Problem in Computational Electromagnetics. In Computational Electromagnetics, 1st ed.; Mittra, R., Ed.; Springer: New York, NY, USA, 2014; pp. 259–316. [Google Scholar]
- Chew, W.C.; Tong, M.S.; Hu, B. Low-Frequency Problems in Integral Equations. In Integral Equation Methods for Electromagnetic and Elastic Waves, 1st ed.; Springer: Cham, Switzerland, 2009; pp. 107–134. [Google Scholar]
- Chen, X.; Zhang, M.; Fan, Z.; Ding, D. Investigation on Low-frequency Breakdown of Electric Field Integral Equation Using 16-byte Floating-point Number. In Proceedings of the 2024 Photonics & Electromagnetics Research Symposium (PIERS), Chengdu, China, 21–25 April 2024; pp. 1–4. [Google Scholar]
- Li, J.; Li, H. Symmetry Implications of a 60 GHz Inverted Microstrip Line Phase Shifter with Nematic Liquid Crystals in Diverse Packaging Boundary Conditions. Symmetry 2024, 16, 798. [Google Scholar] [CrossRef]
- Li, J.; Li, H. Assessing Vulnerabilities in Line Length Parameterization and the Per-Unit-Length Paradigm for Phase Modulation and Figure-of-Merit Evaluation in 60 GHz Liquid Crystal Phase Shifters. Symmetry 2024, 16, 1261. [Google Scholar] [CrossRef]
- Li, J.; Li, H. Liquid Crystal-Filled 60 GHz Coaxially Structured Phase Shifter Design and Simulation with Enhanced Figure of Merit by Novel Permittivity-Dependent Impedance Matching. Electronics 2024, 13, 626. [Google Scholar] [CrossRef]
- Ertman, S.; Orzechowski, K.; Rutkowska, K.; Kołodyńska, O.; Różycka, J.; Ignaciuk, A.; Wasilewska, N.; Osuch, T.; Woliński, T.R. Periodic liquid crystalline waveguiding microstructures. Sci. Rep. 2023, 13, 13896. [Google Scholar] [CrossRef] [PubMed]
- Siarkowska, A.; Chychłowski, M.; Budaszewski, D.; Jankiewicz, B.; Bartosewicz, B.; Woliński, T.R. Thermo- and electro-optical properties of photonic liquid crystal fibers doped with gold nanoparticles. Beilstein J. Nanotechnol. 2017, 8, 2790–2801. [Google Scholar] [CrossRef]
- Yeong, D.J.; Velasco-Hernandez, G.; Barry, J.; Walsh, J. Sensor and Sensor Fusion Technology in Autonomous Vehicles: A Review. Sensors 2021, 21, 2140. [Google Scholar] [CrossRef]
- Panicker, A.; Kshirsagar, U.; Pareek, B. Advanced driver assistance system (ADAS) based on sensor fusion. In Proceedings of the 7th IET Smart Cities Symposium (SCS 2023), Hybrid Conference, Bahrain, 3–5 December 2023; pp. 406–410. [Google Scholar]
- Abdulkarim, Y.I.; Bakır, M.; Yaşar, İ.; Ulutaş, H.; Karaaslan, M.; Alkurt, F.Ö.; Sabah, C.; Dong, J. Highly sensitive metamaterial-based microwave sensor for the application of milk and dairy products. Appl. Opt. 2022, 61, 1972–1981. [Google Scholar] [CrossRef]
- Ragonese, E.; Italia, A.; Palmisano, G. A 5-GHz highly integrated receiver front-end. Analog. Integr. Circ. Sig. Process 2007, 53, 3–7. [Google Scholar] [CrossRef]
- Papadopoulos, M.; Lampropoulos, K.; Kitsos, P. FPGA-Based Cloud Security Solutions for 5G Networks. In Proceedings of the 2024 IEEE International Conference on Cyber Security and Resilience (CSR), London, UK, 2–4 September 2024; pp. 913–918. [Google Scholar]
- Psychalinos, C.; Minaei, S.; Safari, L. Ultra low-power electronically tunable current-mode instrumentation amplifier for biomedical applications. AEU Int. J. Electron. Commun. 2020, 117, 153120. [Google Scholar] [CrossRef]
- Ali, M.Z.; Abohmra, A.; Usman, M.; Zahid, A.; Heidari, H.; Imran, M.A.; Abbasi, Q.H. Quantum for 6G communication: A perspective. IET Quant. Comm. 2023, 4, 112–124. [Google Scholar] [CrossRef]
- Akbar, M.A.; Khan, A.A.; Hyrynsalmi, S. Role of quantum computing in shaping the future of 6 G technology. Inf. Softw. Technol. 2024, 170, 107454. [Google Scholar] [CrossRef]
- Pantoli, L.; Stornelli, V.; Leuzzi, G. High dynamic range, low power, tunable, active filter for RF and microwave wireless applications. IET Microw. Antennas Propag. 2018, 12, 595–601. [Google Scholar] [CrossRef]
- Elwakil, A.S.; Maundy, B.J.; Psychalinos, C. A family of resonance-based tunable frequency oscillators and application to chaos generation. Int. J. Electron. Lett. 2024, 12, 495–505. [Google Scholar] [CrossRef]
- Nako, J.; Kaloudi, E.; Tsirimokou, G.; Psychalinos, C. An Electronically Tunable Universal Non-Integer-Order Filter Structure. In Proceedings of the 2024 Panhellenic Conference on Electronics & Telecommunications (PACET), Thessaloniki, Greece, 28–29 March 2024; pp. 1–4. [Google Scholar]
- Pantoli, L.; Stornelli, V.; Leuzzi, G. Low-noise tunable filter design by means of active components. Electron. Lett. 2016, 52, 86–88. [Google Scholar] [CrossRef]
- Maundy, B.J.; Elwakil, A.S.; Psychalinos, C. A novel family of tunable-frequency oscillators. AEU Int. J. Electron. Commun. 2024, 177, 155219. [Google Scholar] [CrossRef]
- Li, J. Bias Tees Integrated Liquid Crystals Inverted Microstrip Phase Shifter for Phased Array Feeds. In Proceedings of the IEEE EPS 21st International Conference on Electronic Packaging Technology (ICEPT), Guangzhou, China, 12–15 August 2020; pp. 1–5. [Google Scholar]
- Angiulli, G.; Carlo, D.D.; Amendola, G.; Arnieri, E.; Costanzo, S. Support Vector Regression Machines to Evaluate Resonant Frequency of Elliptic Substrate Integrate Waveguide Resonators. Prog. Electromagn. Res. 2008, 83, 107–118. [Google Scholar] [CrossRef]
- You, Y.H.; Kou, X.Y.; Tan, S.T. Adaptive meshing for finite element analysis of heterogeneous materials. Comput. Aided Des. 2015, 62, 176–189. [Google Scholar] [CrossRef]
- Noguchi, S.; Naoe, T.; Igarashi, H.; Matsutomo, S.; Cingoski, V.; Ahagon, A.; Kameari, A. A New Adaptive Mesh Refinement Method in FEA Based on Magnetic Field Conservation at Elements Interfaces and Non-Conforming Mesh Refinement Technique. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Arya, S.; Tiwari, G.K. Characterizing Radio Frequency Transmission and Attenuation in Underwater Wireless Communication. In Proceedings of the 2023 3rd International Conference on Smart Generation Computing, Communication and Networking (SMART GENCON), Bangalore, India, 29–31 December 2023; pp. 1–5. [Google Scholar]
- Hu, S.; Xie, H.; Li, Z. Evaluation of Electromagnetic Fields of Extremely Low-Frequency Horizontal Electric Dipoles at Sea–Air Boundaries. Electronics 2023, 12, 4165. [Google Scholar] [CrossRef]
- Che, X.; Wells, I.; Dickers, G.; Kear, P.; Gong, X. Re-evaluation of RF electromagnetic communication in underwater sensor networks. IEEE Commun. Mag. 2010, 48, 143–151. [Google Scholar] [CrossRef]
- Tritakis, V.; Mlynarczyk, J.; Contopoulos, I.; Kubisz, J.; Christofilakis, V.; Tatsis, G.; Chronopoulos, S.K.; Repapis, C. Extremely Low Frequency (ELF) Electromagnetic Signals as a Possible Precursory Warning of Incoming Seismic Activity. Atmosphere 2024, 15, 457. [Google Scholar] [CrossRef]
- Mlynarczyk, J.; Kulak, A.; Salvador, J. The Accuracy of Radio Direction Finding in the Extremely Low Frequency Range. Radio Sci. 2017, 52, 1245–1252. [Google Scholar] [CrossRef]

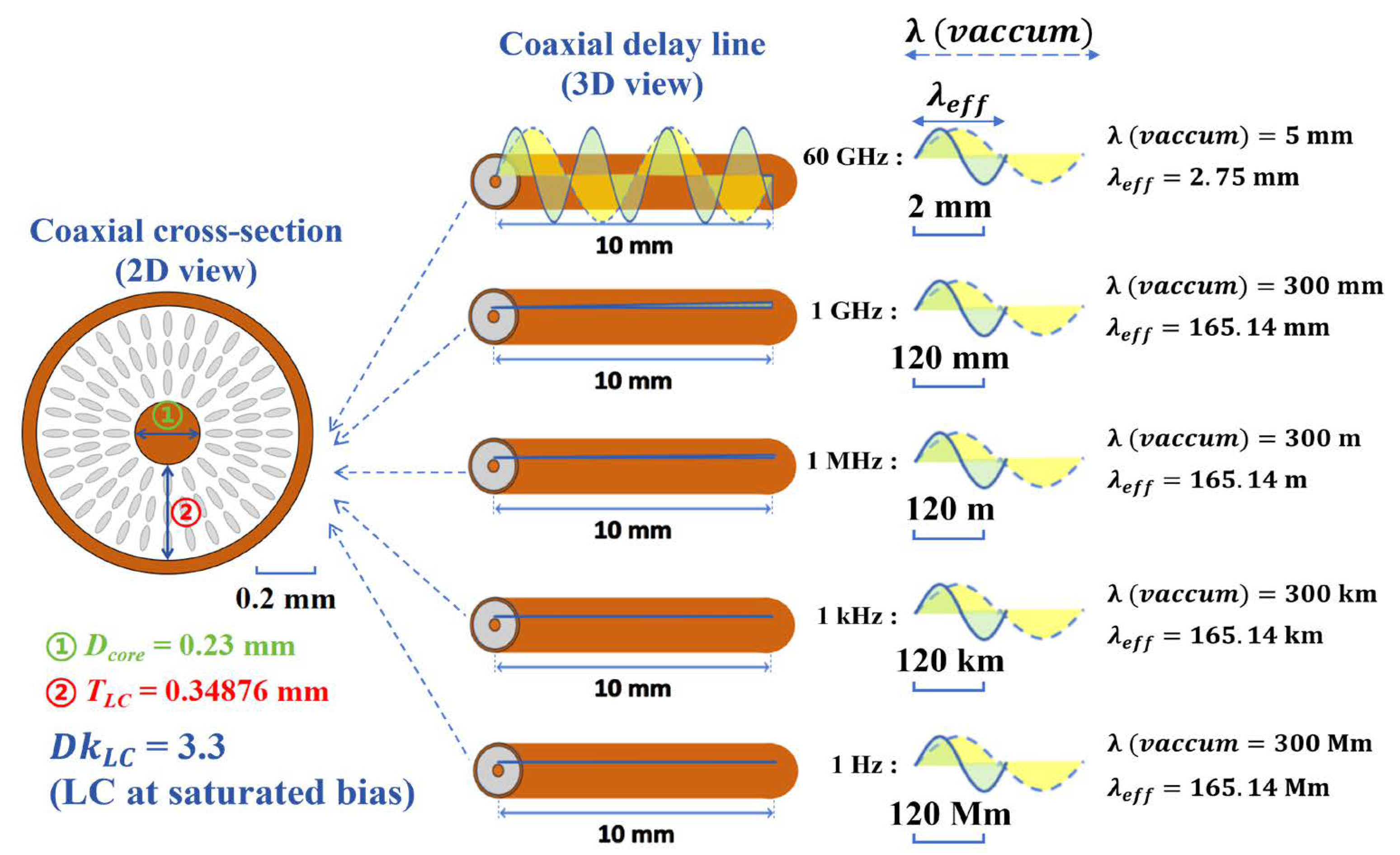


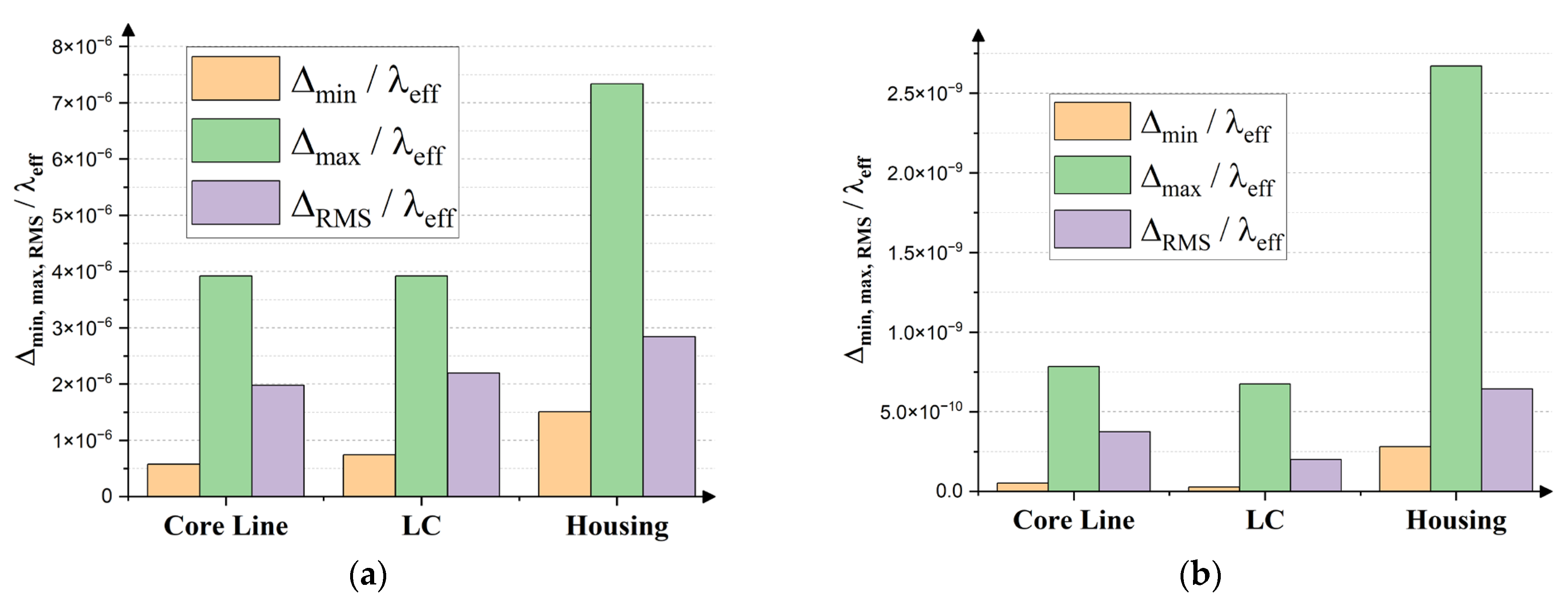


| Solution | Dcore | TLC | L | vacuum | * |
|---|---|---|---|---|---|
| 60 GHz | 0.23 mm | 0.34876 mm | 10 mm | 5 mm | 2.75 mm |
| 1 GHz | 0.23 mm | 0.34876 mm | 10 mm | 30 cm | 16.51 cm |
| 1 MHz | 0.23 mm | 0.34876 mm | 10 mm | 300 m | 165.14 m |
| 1 kHz | 0.23 mm | 0.34876 mm | 10 mm | 300 km | 165.14 km |
| 1 Hz | 0.23 mm | 0.34876 mm | 10 mm | 300,000 km | 165,144.56 km |
| Solution Frequency | Tuning States | Average Memory/Process | Elapsed Time |
|---|---|---|---|
| 60 GHz | Dk = 3.3 (LC saturated bias) | 369 MB | 00:00:14 |
| Dk = 2.754 (LC 0 V bias) | 361 MB | 00:00:14 | |
| Dk = 1.0006 (air-filled) | 292 MB | 00:00:11 | |
| 1 GHz | Dk = 3.3 (LC saturated bias) | 231 MB | 00:00:08 |
| Dk = 2.754 (LC 0 V bias) | 231 MB | 00:00:08 | |
| Dk = 1.0006 (air-filled) | 236 MB | 00:00:08 | |
| 1 MHz | Dk = 3.3 (LC saturated bias) | 251 MB | 00:00:14 |
| Dk = 2.754 (LC 0 V bias) | 253 MB | 00:00:14 | |
| Dk = 1.0006 (air-filled) | 252 MB | 00:00:14 | |
| 1 kHz | Dk = 3.3 (LC saturated bias) | 238 MB | 00:00:14 |
| Dk = 2.754 (LC 0 V bias) | 236 MB | 00:00:14 | |
| Dk = 1.0006 (air-filled) | 235 MB | 00:00:14 | |
| 1 Hz | Dk = 3.3 (LC saturated bias) | 7.24 GB | 00:11:29 |
| Dk = 2.754 (LC 0 V bias) | 4.33 GB | 00:08:27 | |
| Dk = 1.0006 (air-filled) | 3.68 GB | 00:06:19 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Li, H. Susceptibility to Low-Frequency Breakdown in Full-Wave Models of Liquid Crystal-Coaxially-Filled Noise-Shielded Analog Phase Shifters. Electronics 2024, 13, 4792. https://doi.org/10.3390/electronics13234792
Li J, Li H. Susceptibility to Low-Frequency Breakdown in Full-Wave Models of Liquid Crystal-Coaxially-Filled Noise-Shielded Analog Phase Shifters. Electronics. 2024; 13(23):4792. https://doi.org/10.3390/electronics13234792
Chicago/Turabian StyleLi, Jinfeng, and Haorong Li. 2024. "Susceptibility to Low-Frequency Breakdown in Full-Wave Models of Liquid Crystal-Coaxially-Filled Noise-Shielded Analog Phase Shifters" Electronics 13, no. 23: 4792. https://doi.org/10.3390/electronics13234792
APA StyleLi, J., & Li, H. (2024). Susceptibility to Low-Frequency Breakdown in Full-Wave Models of Liquid Crystal-Coaxially-Filled Noise-Shielded Analog Phase Shifters. Electronics, 13(23), 4792. https://doi.org/10.3390/electronics13234792










