Extended-Distance Capacitive Wireless Power Transfer System Based on Generalized Parity–Time Symmetry
Abstract
:1. Introduction
2. System Structure and Modeling
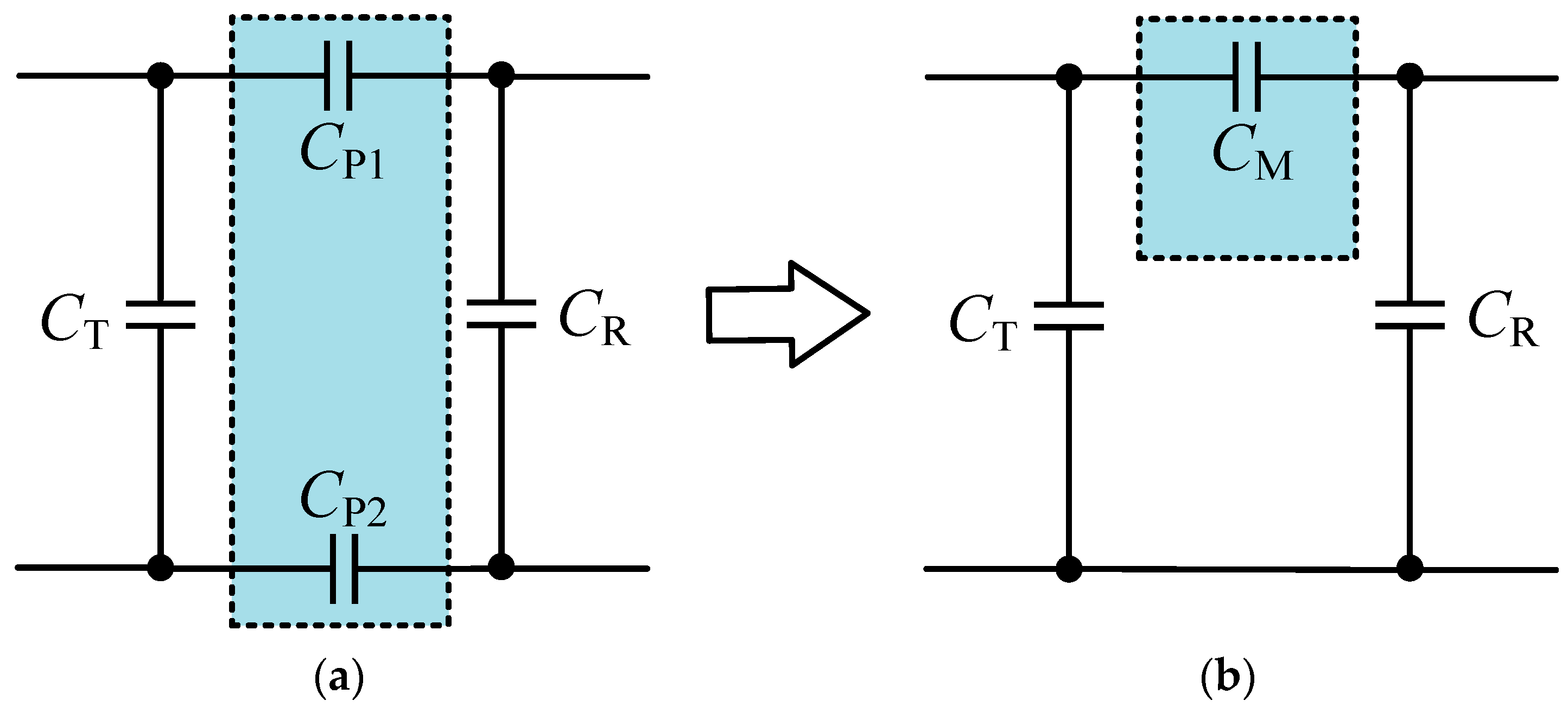
2.1. Capacitive Coupler
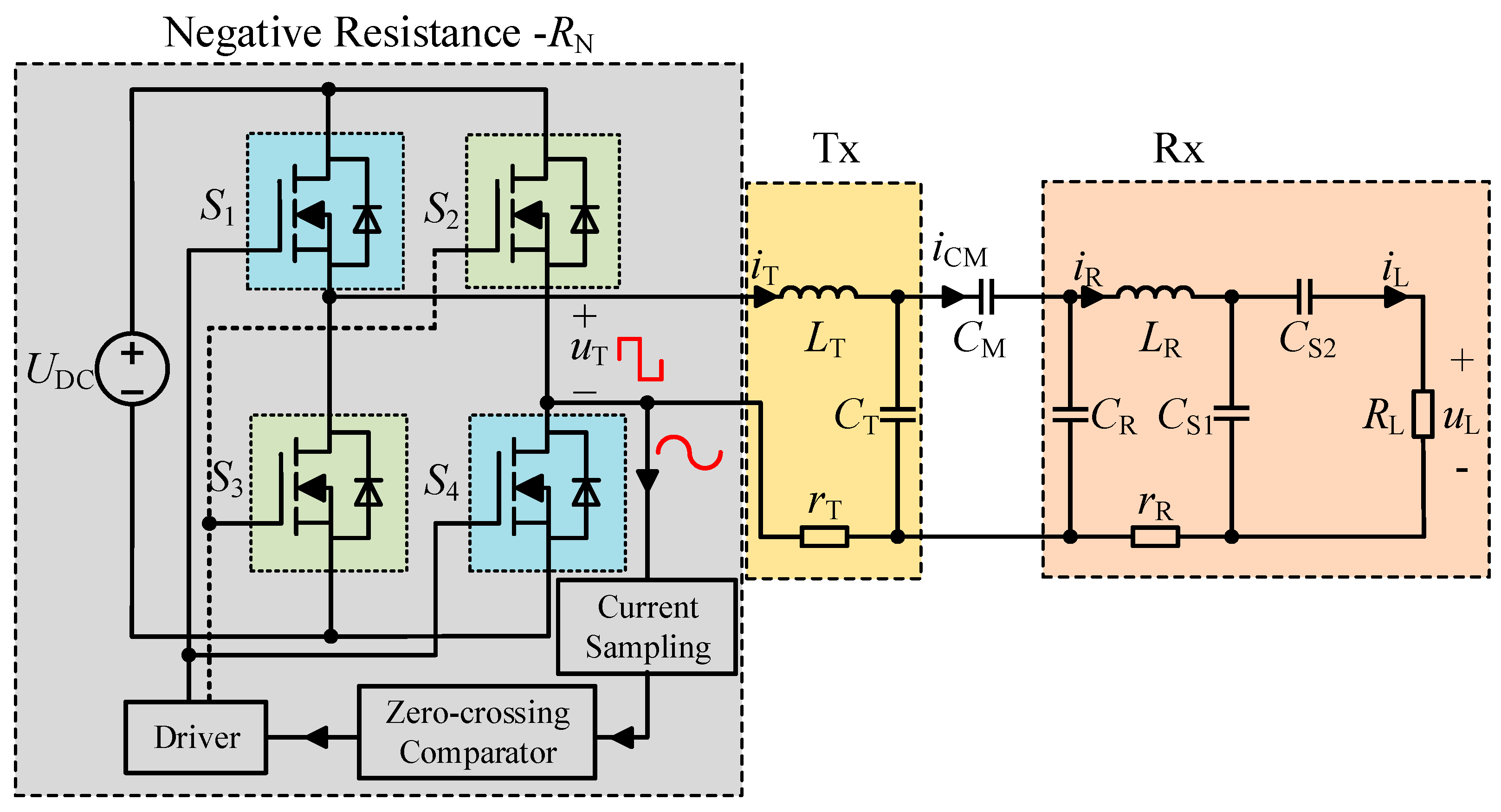
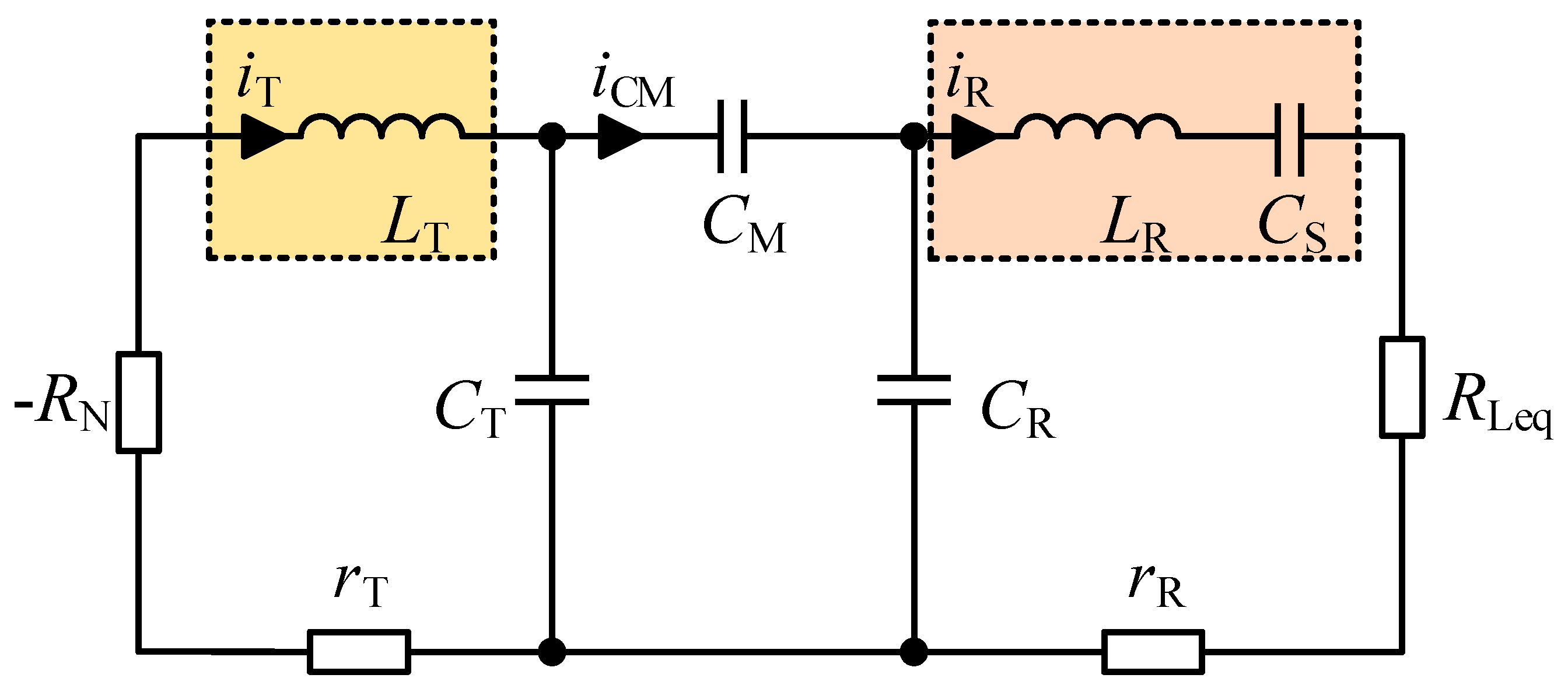
2.2. GPT-Based CPT System with S-PS Structure
2.2.1. In the Exact PT-Symmetric Region
2.2.2. In the Broken PT-Symmetric Region
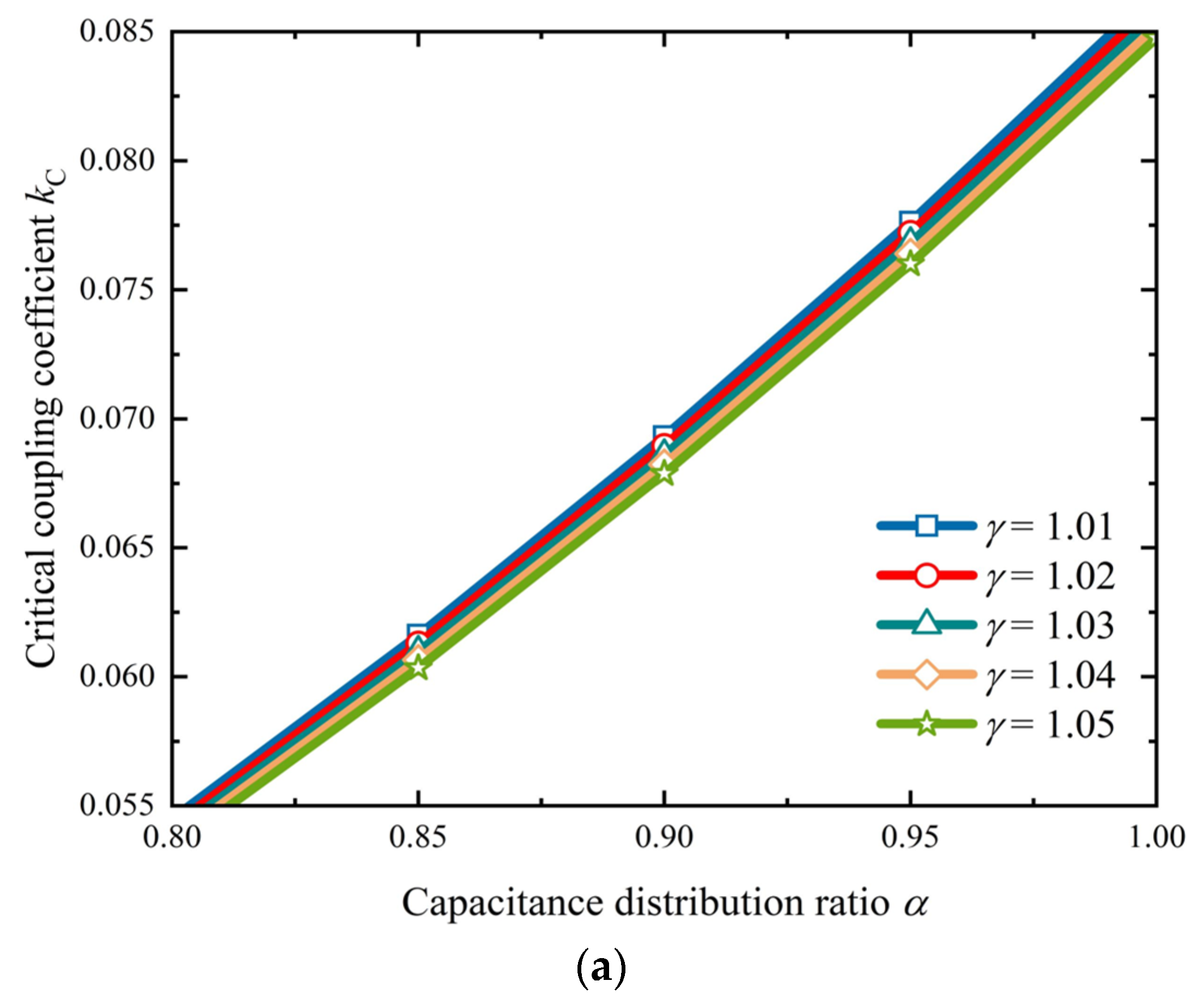
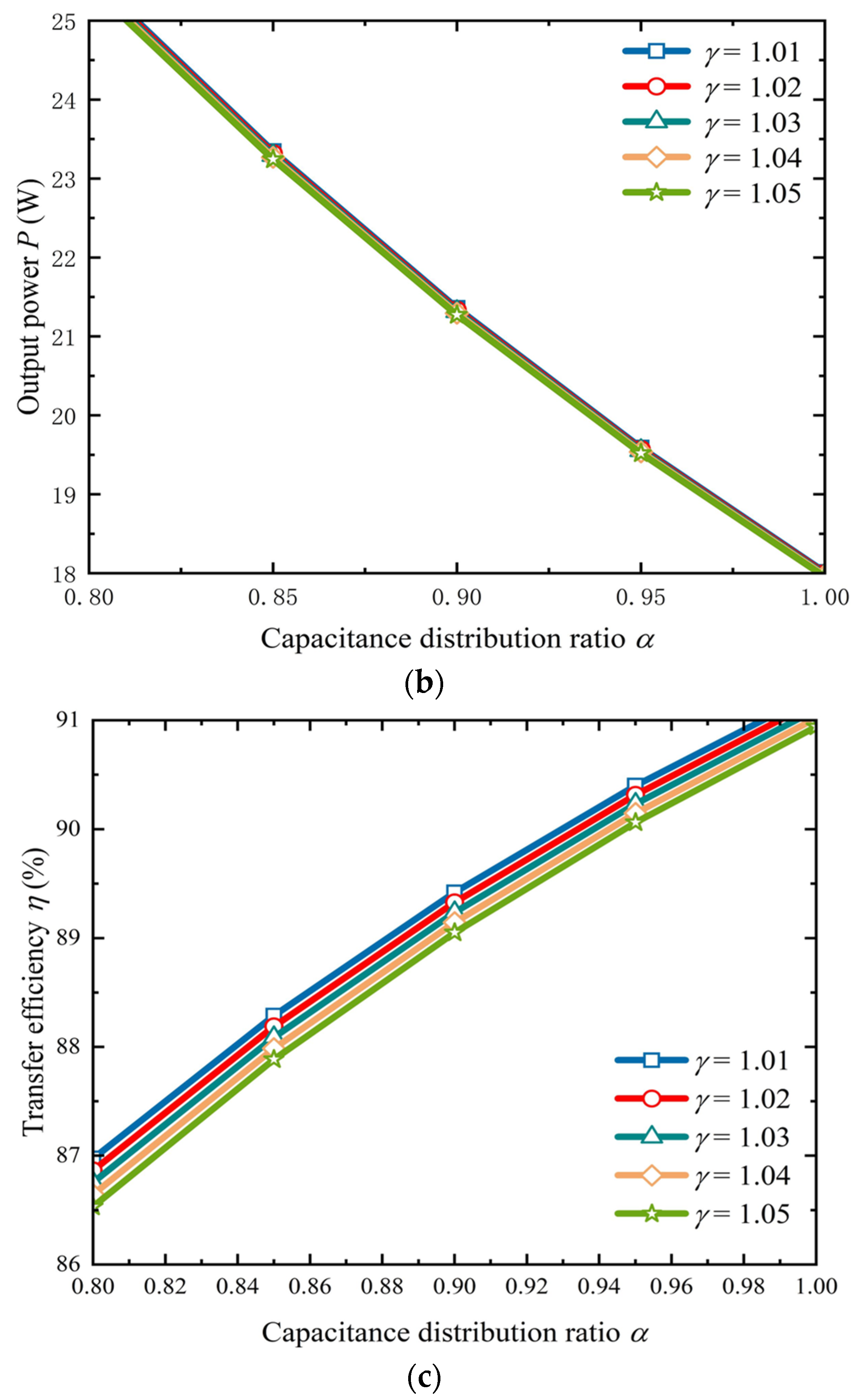
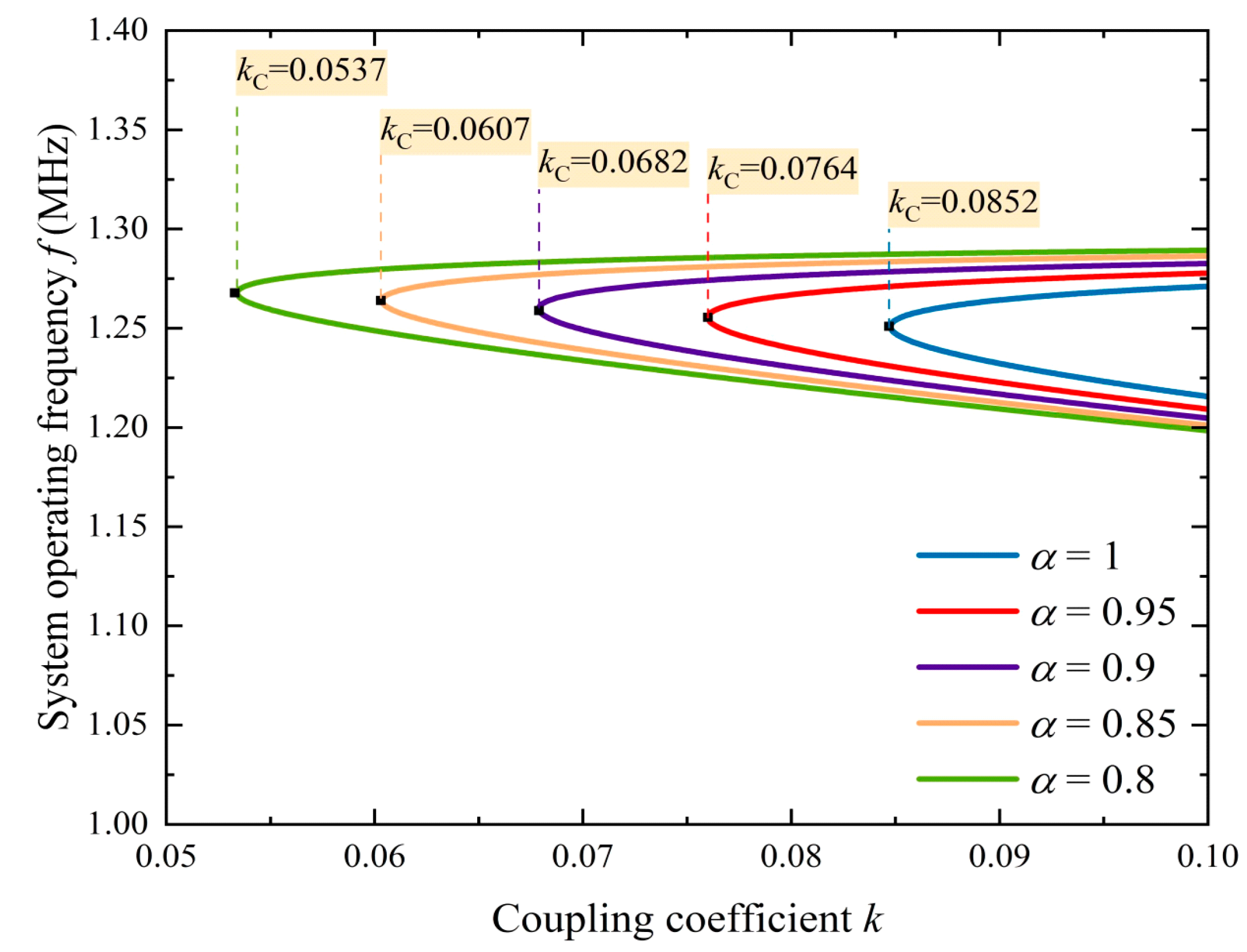
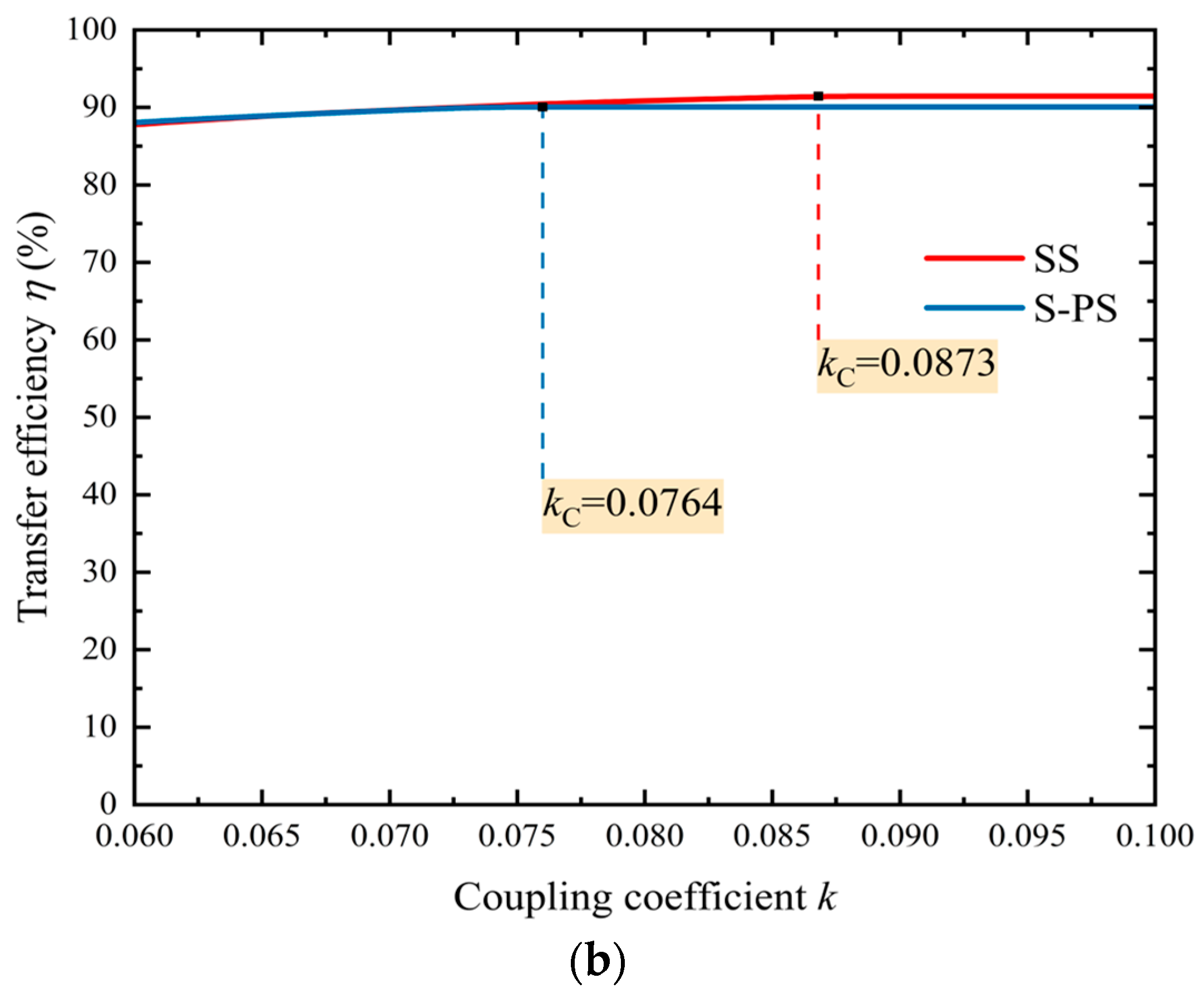
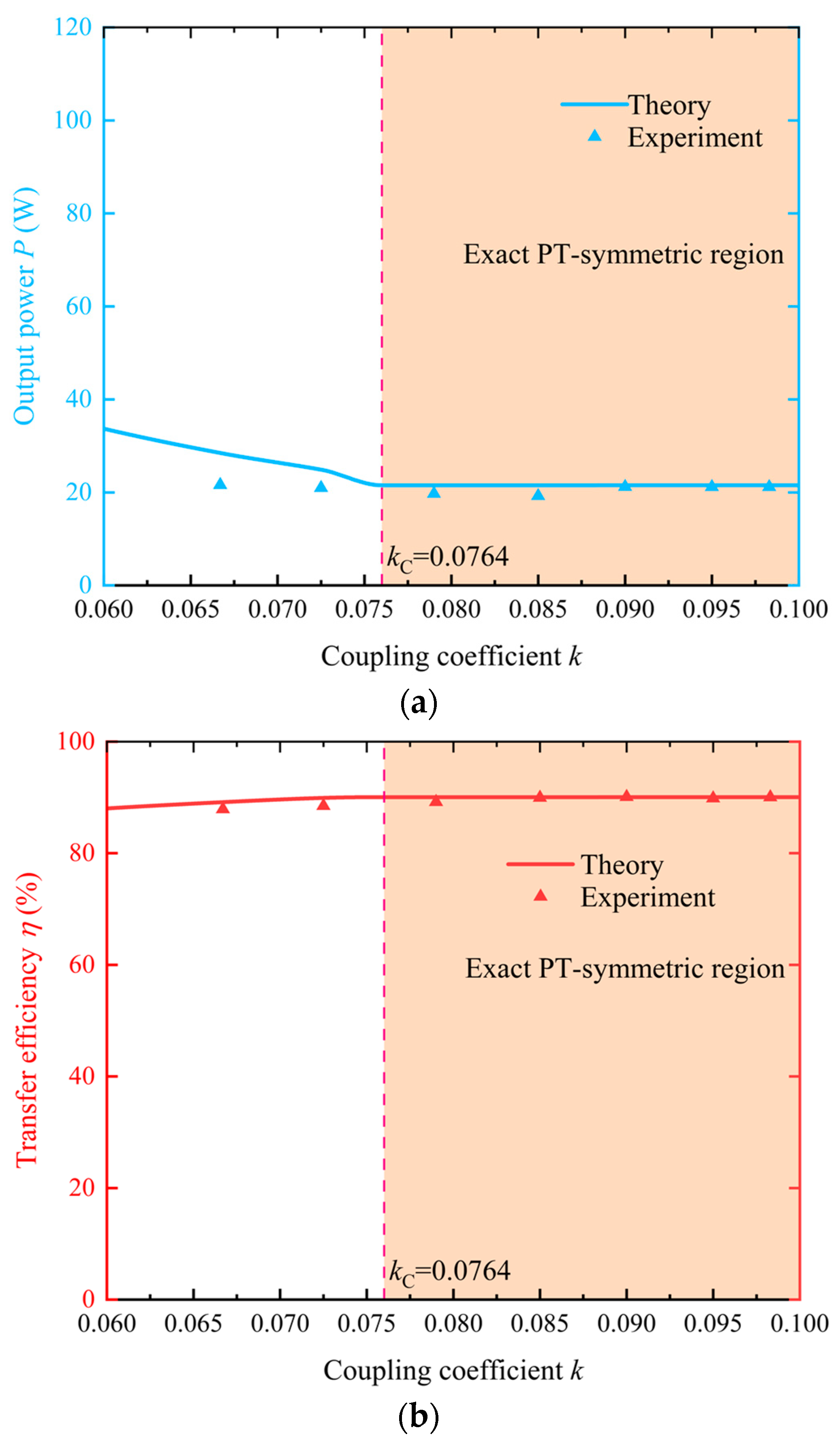
2.3. Parameter Selection and Comparison
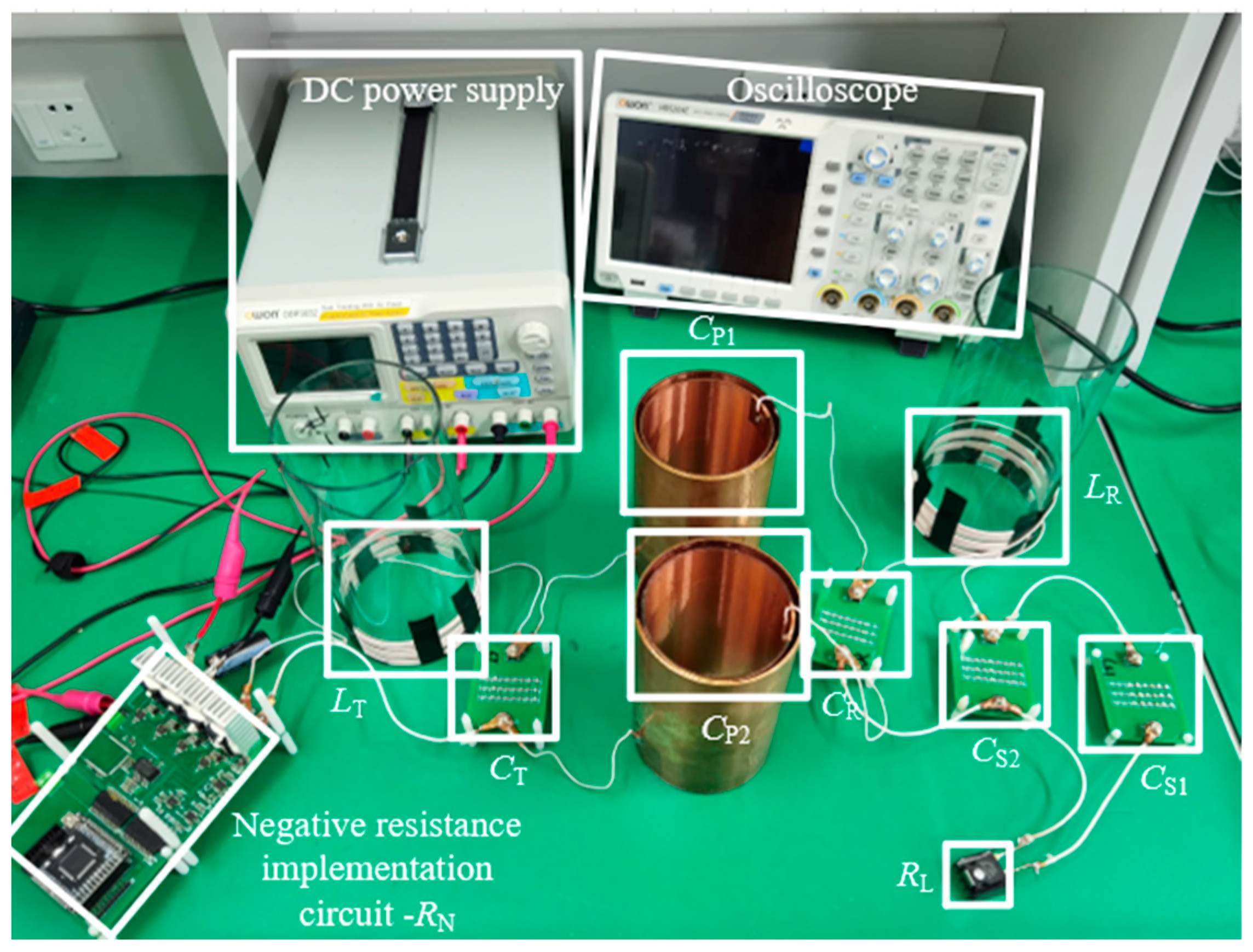
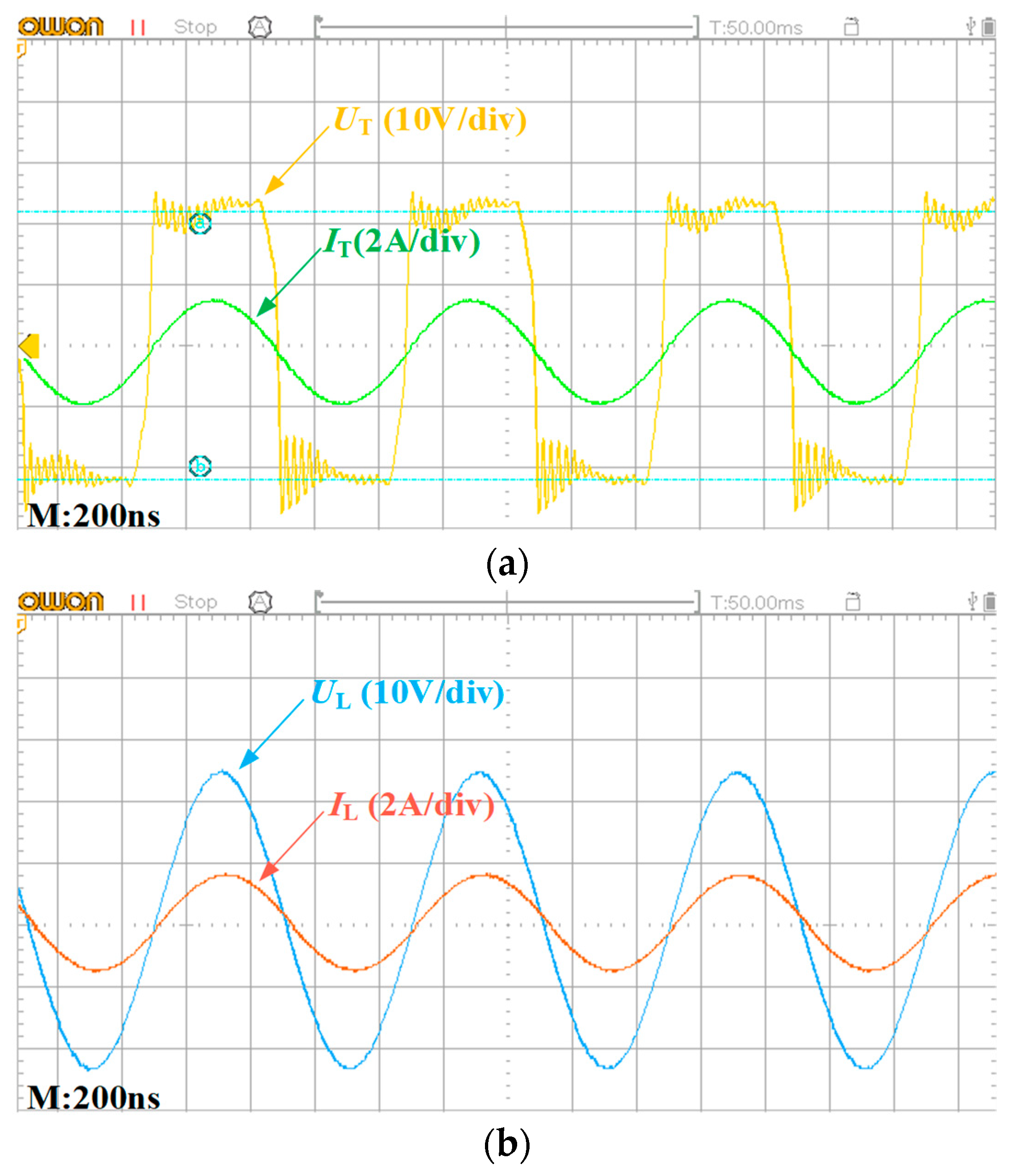
3. Experimental Verification
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Zhang, Z.; Pang, H.; Georgiadis, A.; Cecati, C. Wireless Power Transfer-An Overview. IEEE Trans. Ind. Electron. 2019, 66, 1044–1058. [Google Scholar] [CrossRef]
- Kracek, J.; Svanda, M. Analysis of Capacitive Wireless Power Transfer. IEEE Access 2019, 7, 26678–26683. [Google Scholar] [CrossRef]
- Sagar, A.; Kashyap, A.; Nasab, M.A.; Padmanaban, S.; Bertoluzzo, M.; Kumar, A.; Blaabjerg, F. A Comprehensive Review of the Recent Development of Wireless Power Transfer Technologies for Electric Vehicle Charging Systems. IEEE Access 2023, 11, 83703–83751. [Google Scholar] [CrossRef]
- Lu, F.; Zhang, H.; Mi, C. A Two-Plate Capacitive Wireless Power Transfer System for Electric Vehicle Charging Applications. IEEE Trans. Power Electron. 2018, 33, 964–969. [Google Scholar] [CrossRef]
- Yang, L.; Zhang, Y.; Li, X.; Feng, B.; Chen, X.; Huang, J.; Yang, T.; Zhu, D.; Zhang, A.; Tong, X. Comparison Survey of Effects of Hull on AUVs for Underwater Capacitive Wireless Power Transfer System and Underwater Inductive Wireless Power Transfer System. IEEE Access 2022, 10, 125401–125410. [Google Scholar] [CrossRef]
- Hossain, A.N.M.S.; Mohseni, P.; Lavasani, H.M. Design and Optimization of Capacitive Links for Wireless Power Transfer to Biomedical Implants. IEEE Trans. Biomed. Circuits Syst. 2022, 16, 1299–1312. [Google Scholar] [CrossRef] [PubMed]
- Yang, L.; Ju, M.; Zhang, B. Bidirectional Undersea Capacitive Wireless Power Transfer System. IEEE Access 2019, 7, 121046–121054. [Google Scholar] [CrossRef]
- Lu, F.; Zhang, H.; Hofmann, H.; Mi, C. A Double-Sided LCLC-Compensated Capacitive Power Transfer System for Electric Vehicle Charging. IEEE Trans. Power Electron. 2015, 30, 6011–6014. [Google Scholar] [CrossRef]
- Tamura, M.; Naka, Y.; Murai, K.; Nakata, T. Design of a Capacitive Wireless Power Transfer System for Operation in Fresh Water. IEEE Trans. Microw. Theory 2018, 66, 5873–5884. [Google Scholar] [CrossRef]
- Qing, X.; Su, Y.; Hu, A.P.; Dai, X.; Liu, Z. Dual-Loop Control Method for CPT System Under Coupling Misalignments and Load Variations. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 10, 4902–4912. [Google Scholar] [CrossRef]
- Cai, C.; Liu, X.; Wu, S.; Chen, X.; Chai, W.; Yang, S. A Misalignment Tolerance and Lightweight Wireless Charging System via Reconfigurable Capacitive Coupling for Unmanned Aerial Vehicle Applications. IEEE Trans. Power Electron. 2022, 38, 22–26. [Google Scholar] [CrossRef]
- Jiang, C.; Wei, B.; Xu, C.; Wu, X.; He, H. Efficiency Improvement Under Coupler Misalignment for Dual-Transmitter and Single-Receiver Capacitive Power Transfer System. IEEE Trans. Power Electron. 2023, 38, 14872–14883. [Google Scholar] [CrossRef]
- Jiang, Y.; Zhou, Y.; Zhang, B.; Chen, W.; Chen, Q.; Shu, X. A Robust Capacitive Power Transfer System via Fractional-Order Autonomous Circuit. IEEE J. Emerg. Sel. Top. Power Electron. 2024, 12, 3258–3267. [Google Scholar] [CrossRef]
- Liu, Z.; Hu, H.; Su, Y.G.; Sun, Y.; Chen, F.; Deng, P. A Double-Receiver Compact SCC-WPT System With CV/CC Output for Mobile Devices Charging/Supply. IEEE Trans. Power Electron. 2023, 38, 9230–9245. [Google Scholar] [CrossRef]
- Wei, X.; Shen, X.; Jiang, J.; Luo, S.; Zhu, Y.; Ma, H. An Improved Design Method Based on a Multiplate Coupler Structure for Capacitive Power Transfer With Wide Ranges of Misalignment and Loads. IEEE J. Emerg. Sel. Top. Power Electron. 2024, 12, 5311–5322. [Google Scholar] [CrossRef]
- van Ieperen, A.; Derammelaere, S.; Minnaert, B. Coupling-Independent Capacitive Wireless Power Transfer With One Transmitter and Multiple Receivers Using Frequency Bifurcation. IEEE Open J. Power Electron. 2024, 5, 891–901. [Google Scholar] [CrossRef]
- Lian, J.; Qu, X. An LCLC-LC-Compensated Capacitive Power Transferred Battery Charger With Near-Unity Power Factor and Configurable Charging Profile. IEEE Trans. Ind. Appl. 2021, 58, 1053–1060. [Google Scholar] [CrossRef]
- Shinde, K.; Koizumi, H. Capacitive Power Transfer System Using a Load-Independent Class E Zero Voltage Switching Parallel Resonant Inverter and a Class D Voltage-Driven Rectifier. IEEE Trans. Circuits Syst. II Express Briefs 2024, 71, 4366–4370. [Google Scholar] [CrossRef]
- Zhou, W.; Chen, Z.; Zhang, Q.; Li, Z.; Huang, L.; Mai, R.; He, Z. Design and Analysis of CPT System With Wide-Range ZVS and Constant Current Charging Operation Using 6.78 MHz Class-E Power Amplifier. IEEE J. Emerg. Sel. Top. Power Electron. 2024, 12, 3211–3225. [Google Scholar] [CrossRef]
- Assawaworrarit, S.; Yu, X.; Fan, S. Robust wireless power transfer using a nonlinear parity–time-symmetric circuit. Nature 2017, 546, 387–390. [Google Scholar] [CrossRef]
- Gu, W.; Qiu, D.; Shu, X.; Zhang, B.; Xiao, W.; Chen, Y. A Constant Output Capacitive Wireless Power Transfer System Based on Parity-Time Symmetric. IEEE Trans. Circuits Syst. II Express Briefs 2023, 70, 2585–2589. [Google Scholar] [CrossRef]
- Assawaworrarit, S.; Fan, S. Robust and efficient wireless power transfer using a switch-mode implementation of a nonlinear parity time symmetric circuit. Nature Electron. 2020, 3, 273–279. [Google Scholar] [CrossRef]
- Chen, P.Y.; Sakhdari, M.; Hajizadegan, M.; Cui, Q.; Cheng, M.M.C.; El-Ganainy, R.; Alù, A. Generalized parity-time symmetry condition for enhanced sensor telemetry. Nature Electron. 2018, 1, 297–304. [Google Scholar] [CrossRef]
- Zhang, H.; Lu, F.; Hofmann, H.; Liu, W.; Mi, C.C. A Four-Plate Compact Capacitive Coupler Design and LCL-Compensated Topology for Capacitive Power Transfer in Electric Vehicle Charging Application. IEEE Trans. Power Electron. 2016, 31, 8541–8551. [Google Scholar]
- Wu, X.; Su, Y.; Hu, A.P.; Qing, X.; Hou, X. Multiobjective Parameter Optimization of a Four-Plate Capacitive Power Transfer System. IEEE J. Emerg. Sel. Top. Power Electron. 2020, 9, 2328–2342. [Google Scholar] [CrossRef]




| Symbols | Values | Symbols | Values |
|---|---|---|---|
| CT | 606.641 pF | RL | 15 Ω |
| CR | 606.035 pF | rT | 682.137 mΩ |
| CS1 | 11.518 nF | rR | 714.613 mΩ |
| CS2 | 606.406 pF | fT | 1.292 MHz |
| LT | 25.0061 μH | fR | 1.293 MHz |
| LR | 26.2493 μH | UDC | 21 V |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shu, X.; Ou, R.; Wu, G.; Yang, J.; Jiang, Y. Extended-Distance Capacitive Wireless Power Transfer System Based on Generalized Parity–Time Symmetry. Electronics 2024, 13, 4731. https://doi.org/10.3390/electronics13234731
Shu X, Ou R, Wu G, Yang J, Jiang Y. Extended-Distance Capacitive Wireless Power Transfer System Based on Generalized Parity–Time Symmetry. Electronics. 2024; 13(23):4731. https://doi.org/10.3390/electronics13234731
Chicago/Turabian StyleShu, Xujian, Riming Ou, Guoxin Wu, Jingjing Yang, and Yanwei Jiang. 2024. "Extended-Distance Capacitive Wireless Power Transfer System Based on Generalized Parity–Time Symmetry" Electronics 13, no. 23: 4731. https://doi.org/10.3390/electronics13234731
APA StyleShu, X., Ou, R., Wu, G., Yang, J., & Jiang, Y. (2024). Extended-Distance Capacitive Wireless Power Transfer System Based on Generalized Parity–Time Symmetry. Electronics, 13(23), 4731. https://doi.org/10.3390/electronics13234731






