Design and Modeling of Coreless Magnetoelectric Transducers for Snake-like Wave Energy Converters
Abstract
1. Introduction
2. Design of the Snake-Like WEC
3. Design and Optimization of the Coreless Magnetoelectric Transducer
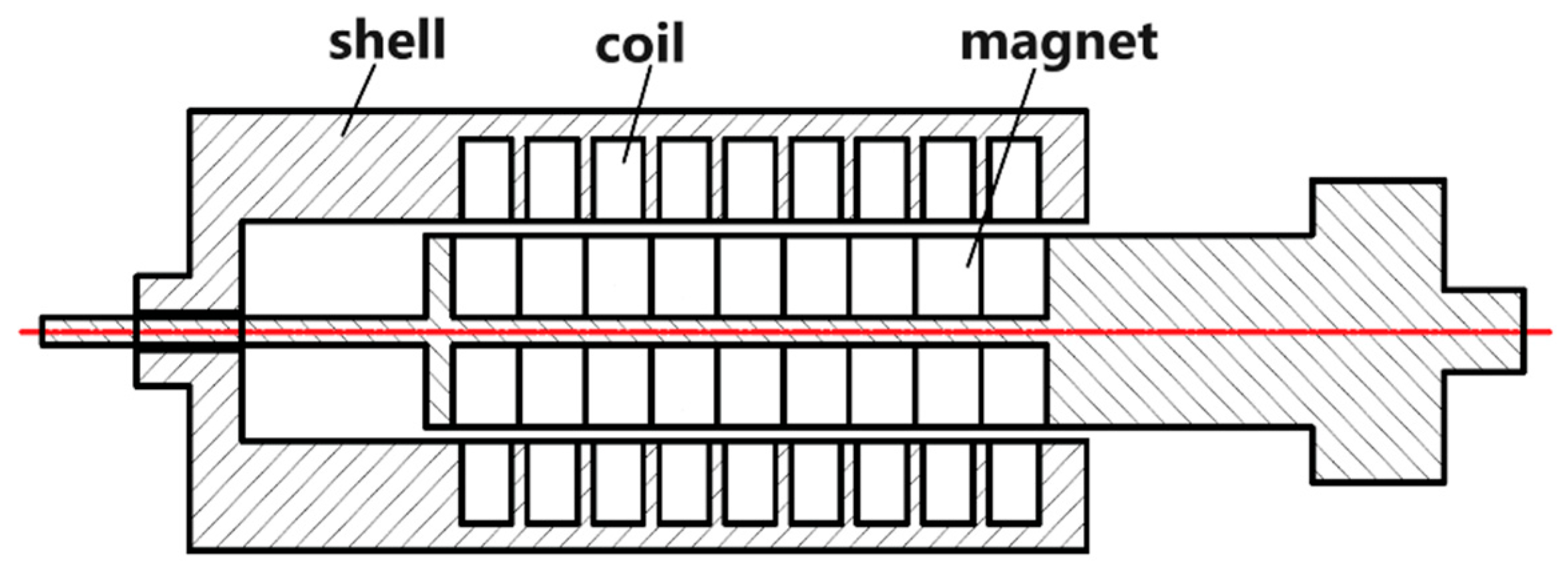
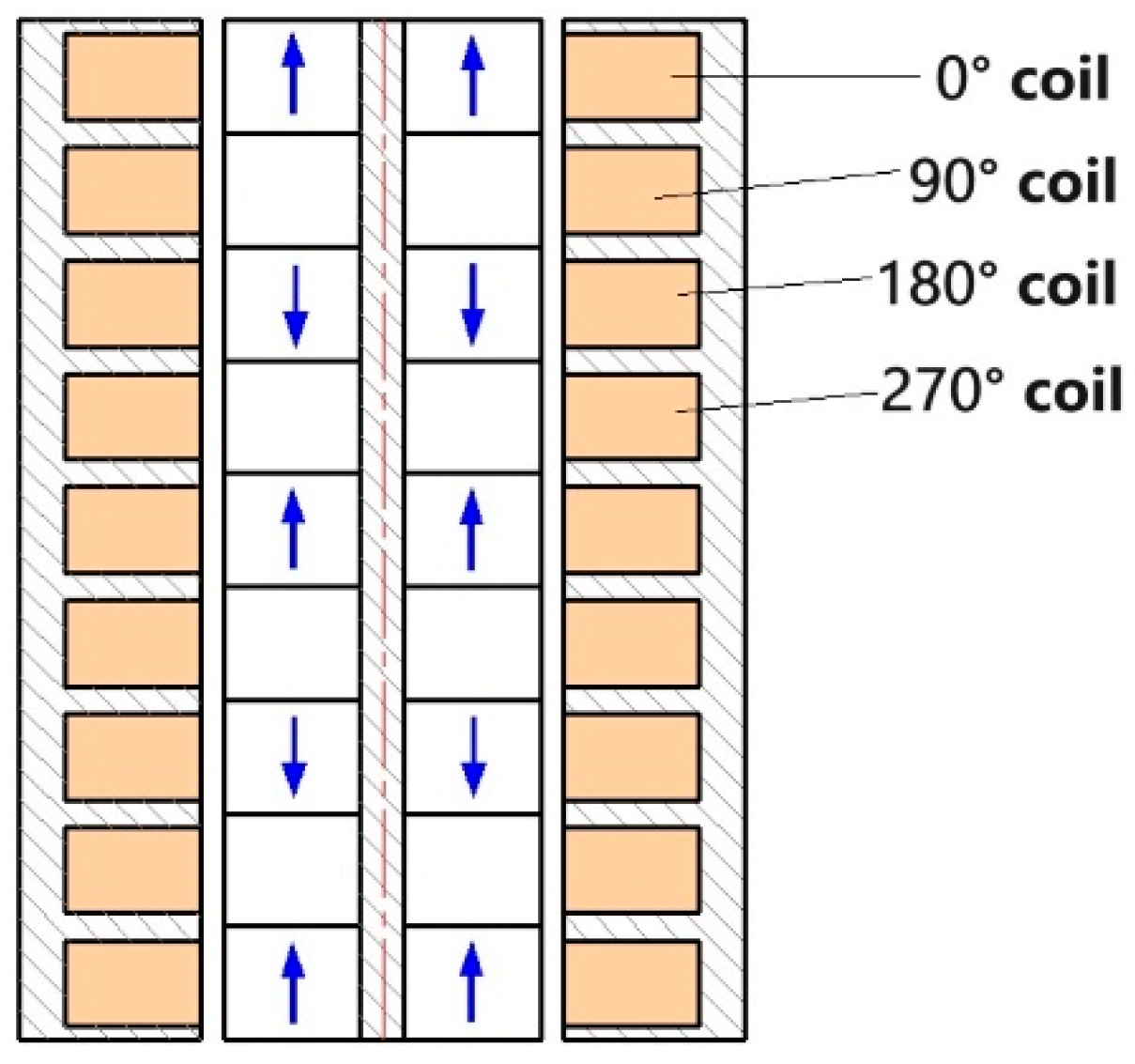
3.1. Design of the Coreless Magnetoelectric Transducer
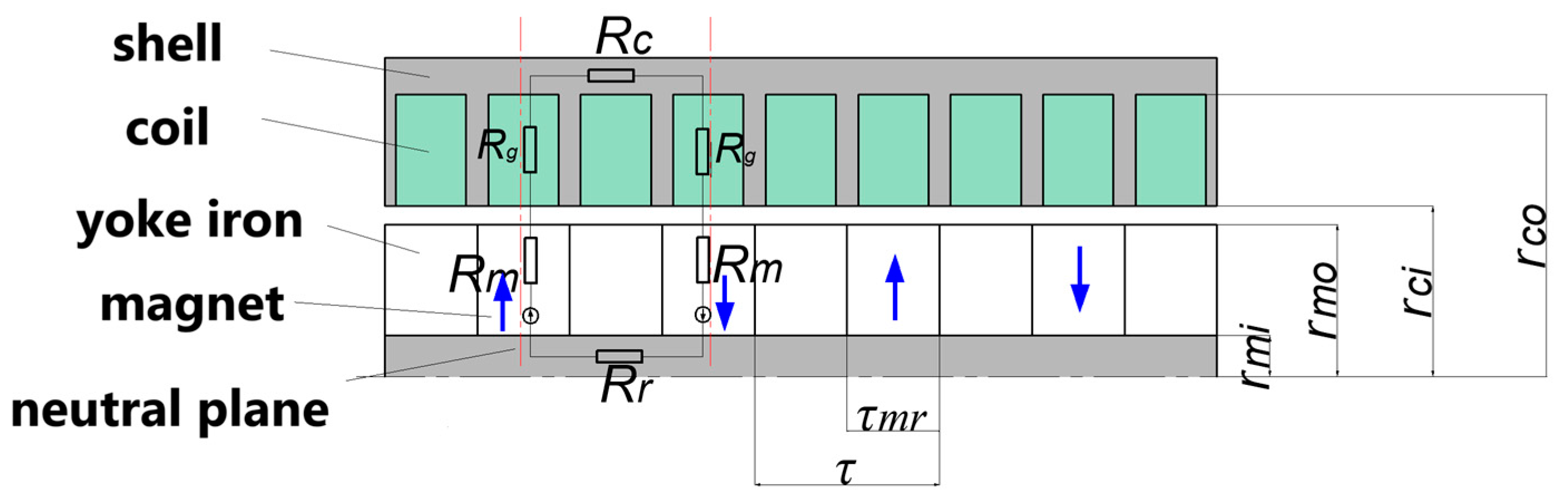
3.2. Equivalent Magnetic Circuit Model of the Coreless Magnetoelectric Transducer
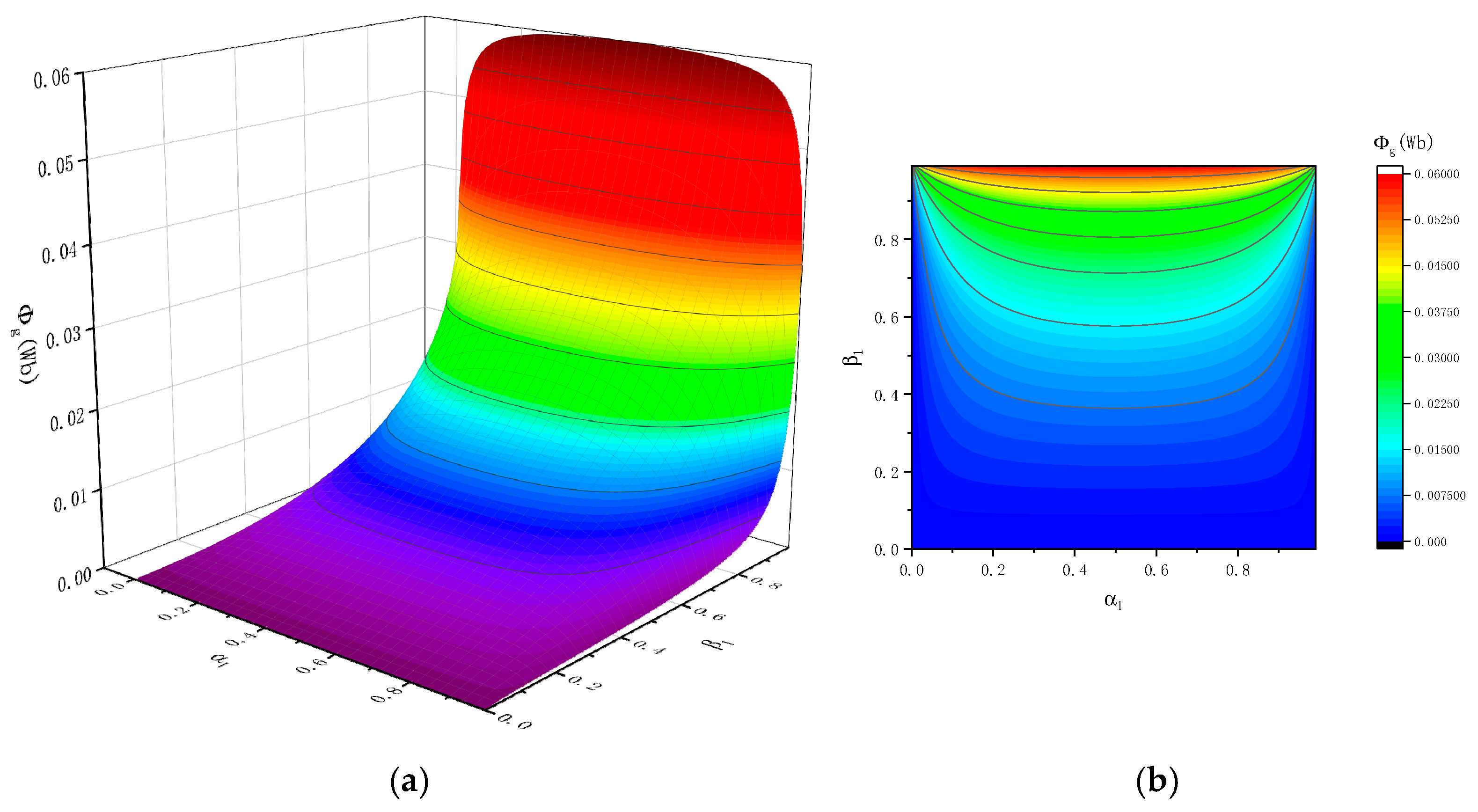
3.2.1. Equivalent Magnetic Circuit Model of the Coreless Magnetoelectric Transducer with Axial Permanent Magnets
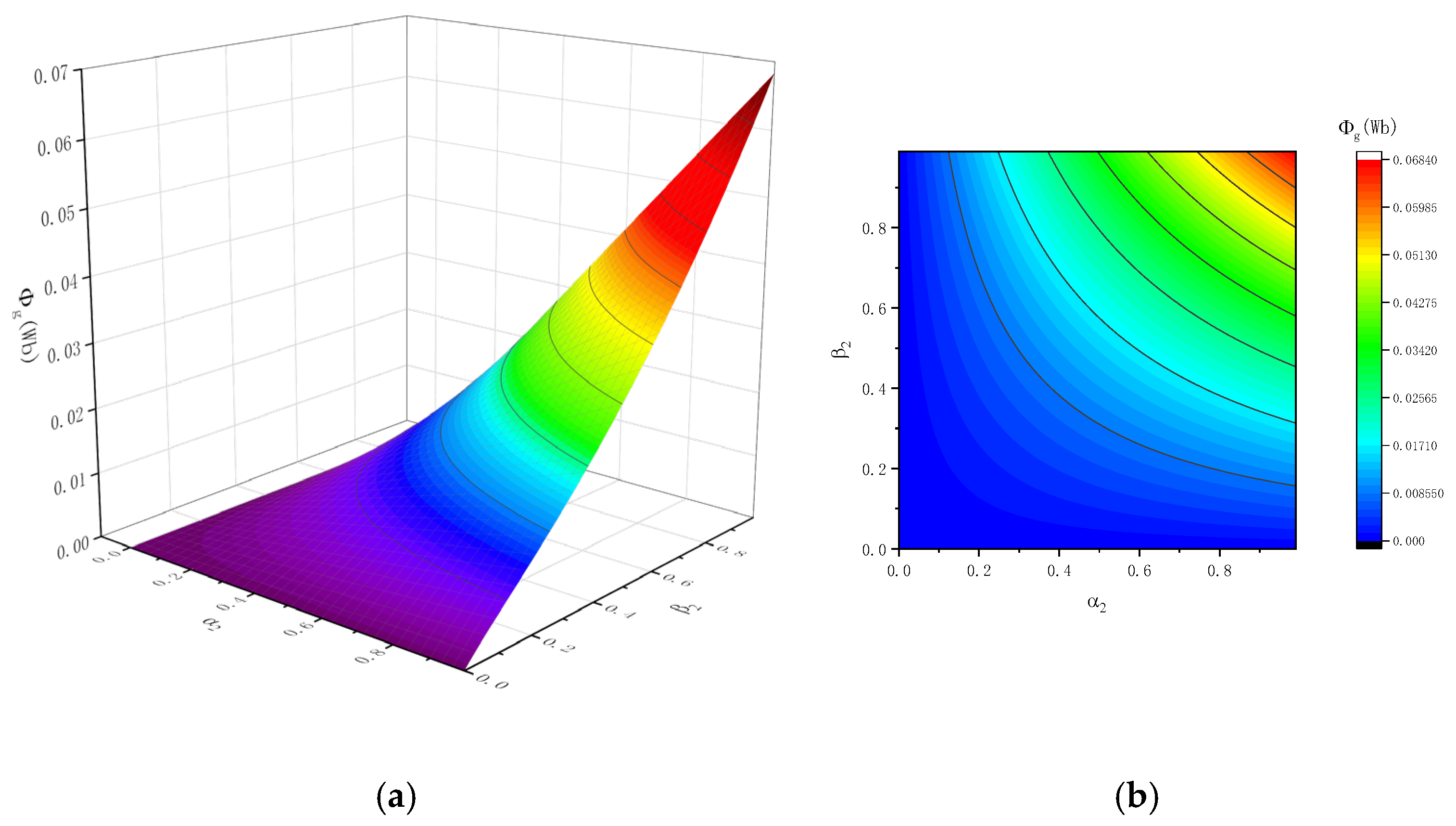
3.2.2. Equivalent Magnetic Circuit Model of the Coreless Magnetoelectric Transducer with Radial Permanent Magnets
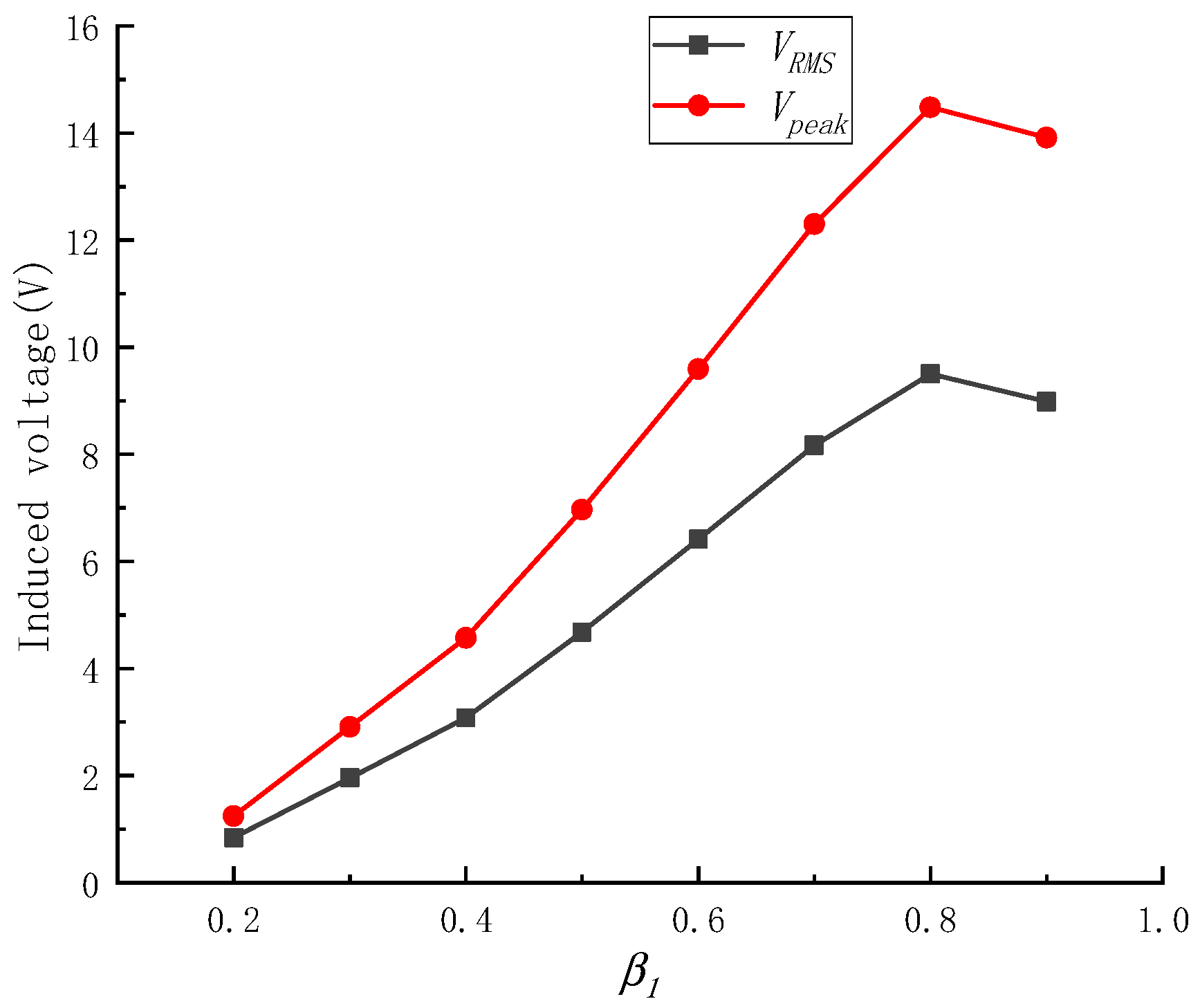
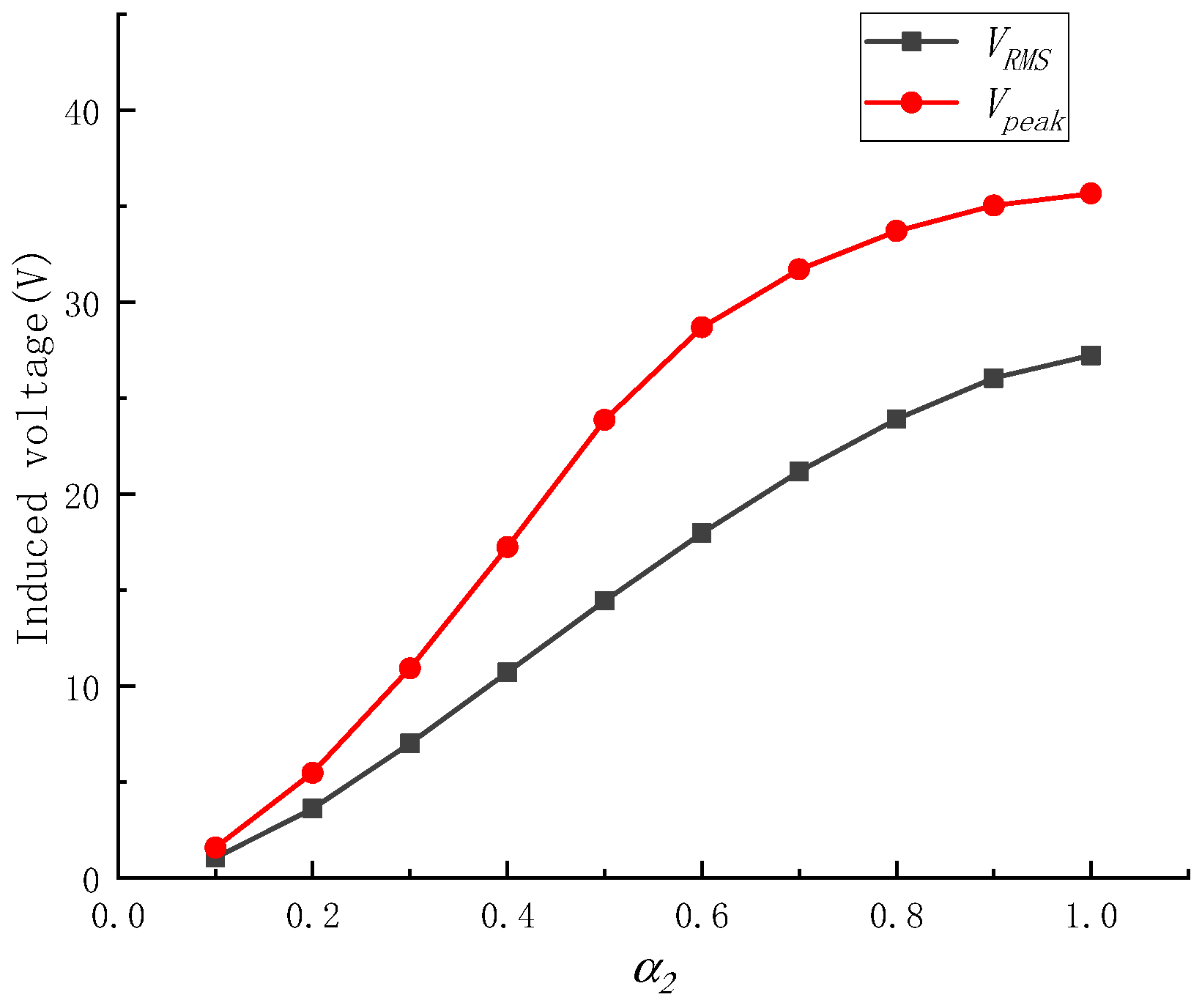
4. Results and Discussions
4.1. Simulation of the Coreless Magnetoelectric Transducer
4.1.1. Simulation of the Coreless Magnetoelectric Transducer with Axial Permanent Magnets
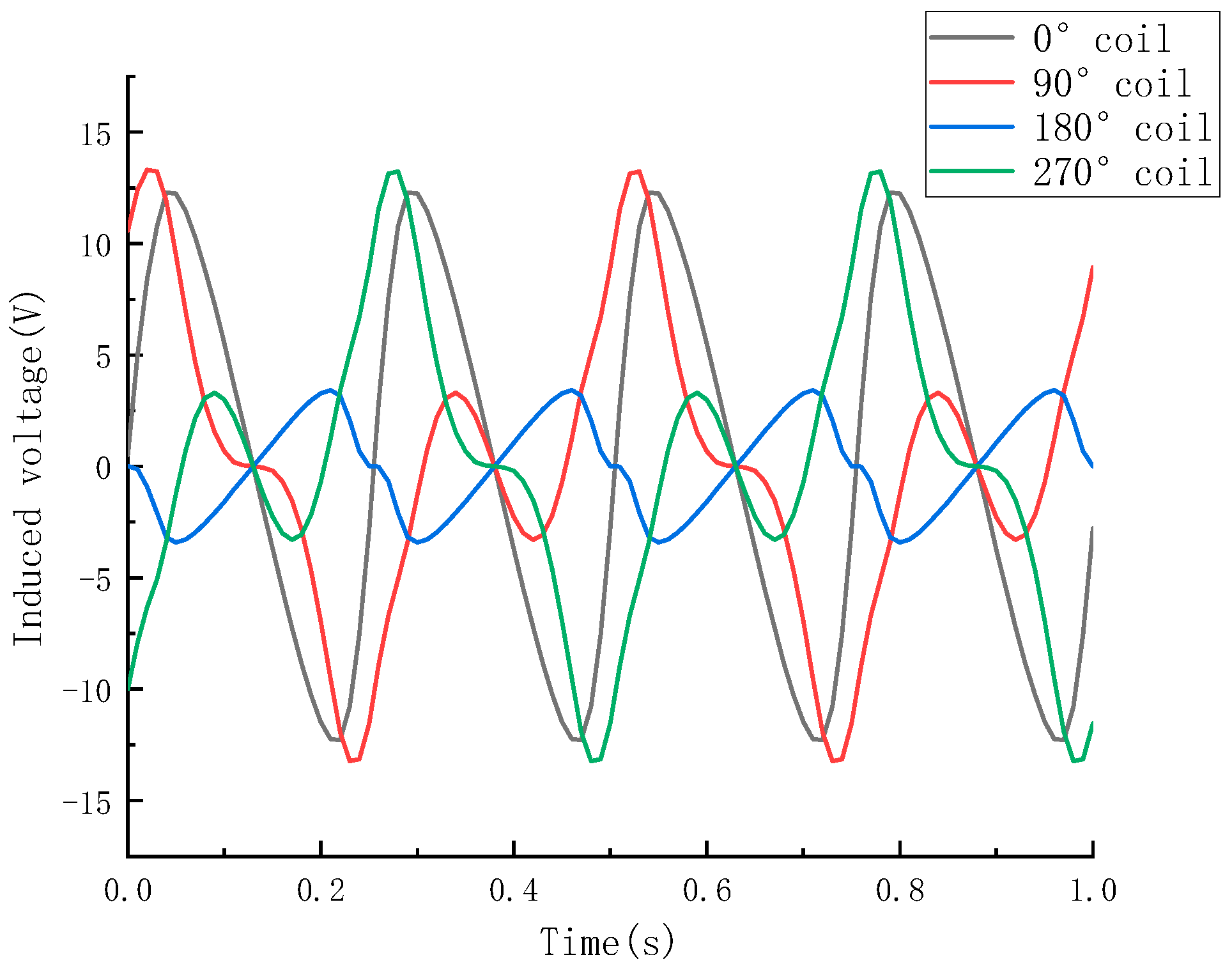
4.1.2. Simulation of the Coreless Magnetoelectric Transducer with Radial Permanent Magnets
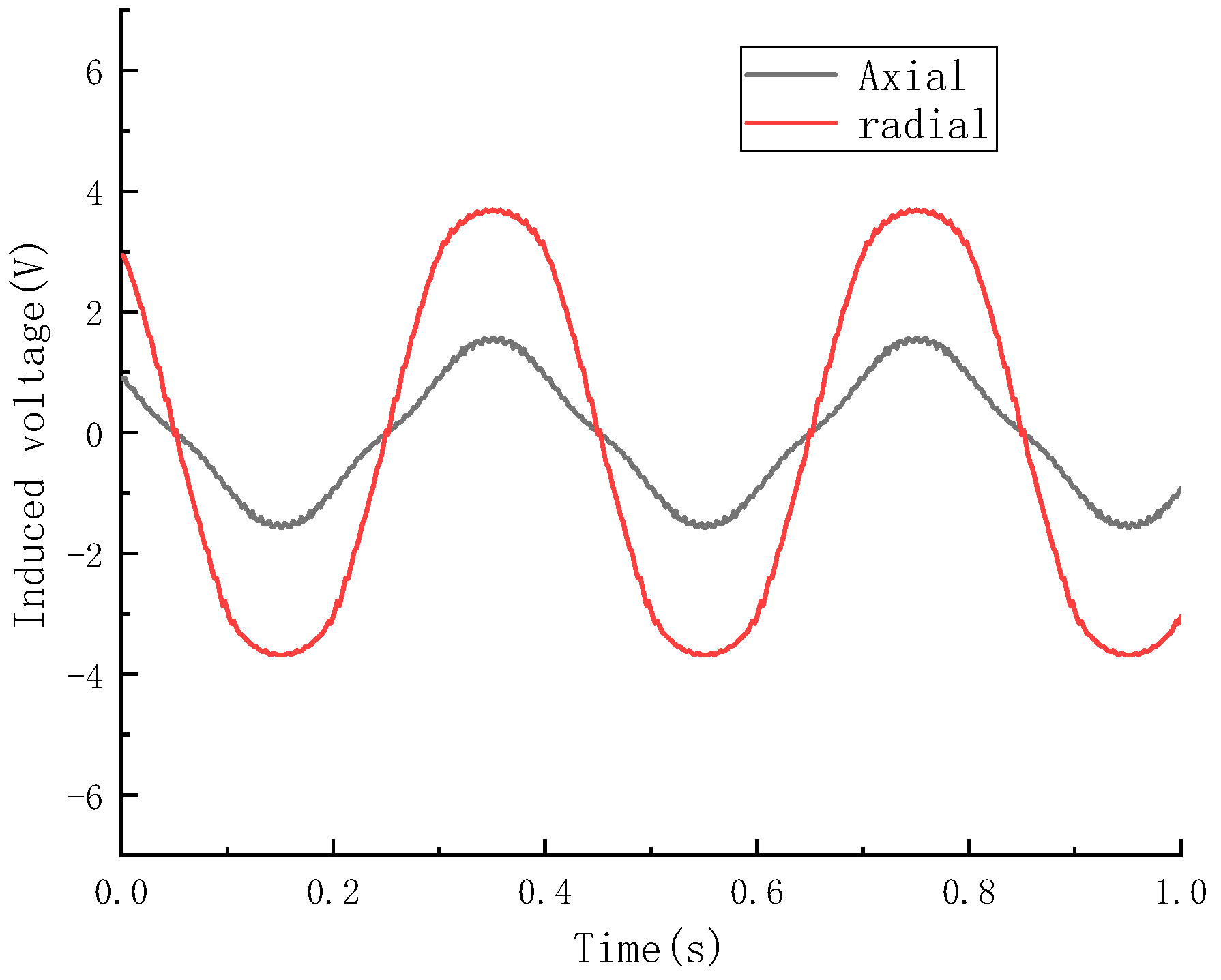
4.1.3. Comparison
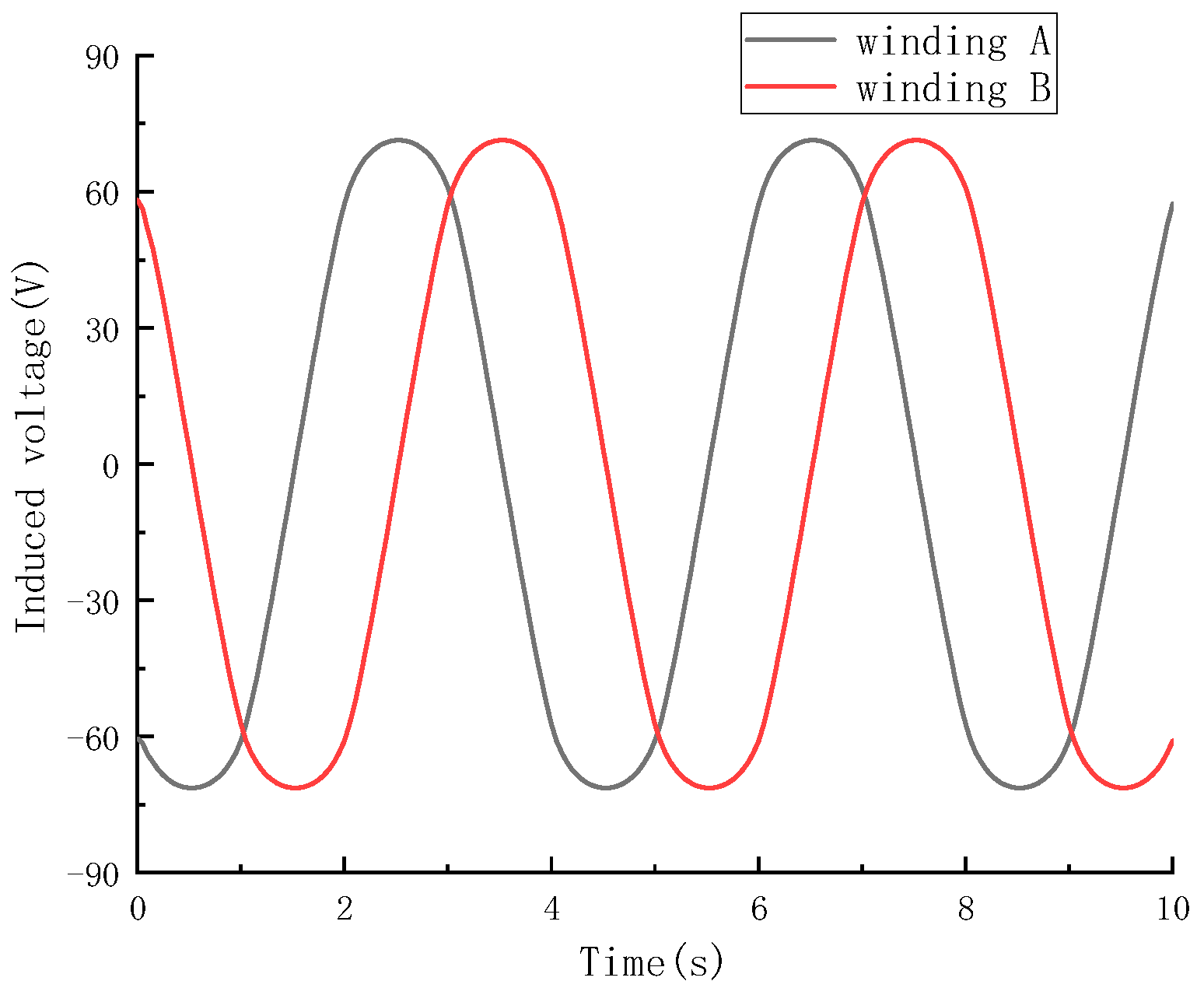
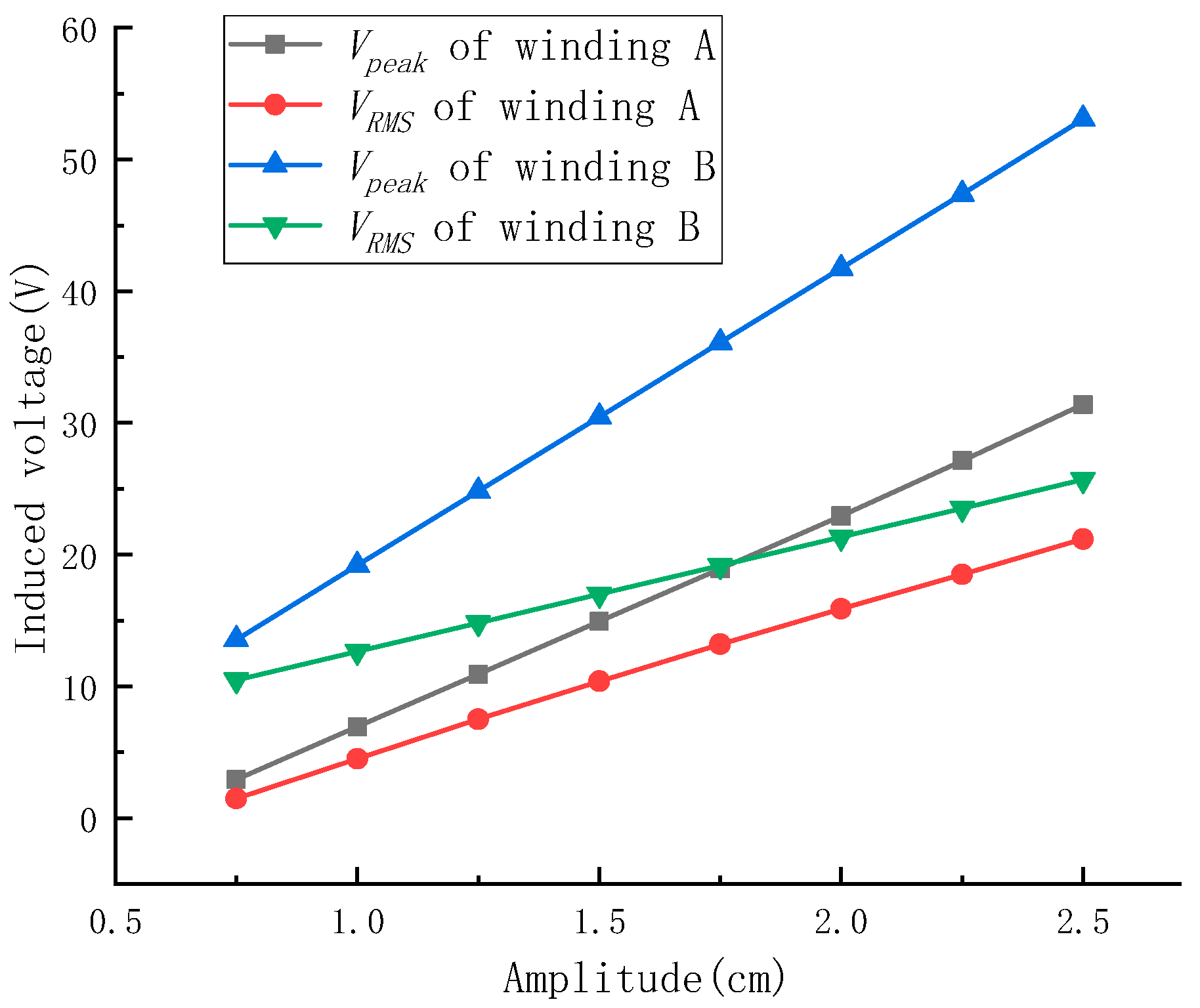
4.2. Experimental Study
4.2.1. Prototype of the Coreless Magnetoelectric Transducer with Axial Permanent Magnets
4.2.2. Prototype of the Coreless Magnetoelectric Transducer with Radial Permanent Magnets
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yu, M.; Cao, F.; Wei, Z.; Han, M.; Shi, H.; Chen, P.; Tian, H. Hydrodynamic performance and energy redistribution characteristics of wind–wave hybrid system based on different WEC microarrays. Ocean Eng. 2024, 306, 118090. [Google Scholar] [CrossRef]
- Zhao, L.; Zou, H.; Xie, X.; Guo, D.H.; Gao, Q.H.; Wu, Z.Y.; Yan, G.; Wei, K.X.; Zhang, W.M. Mechanical intelligent wave energy harvesting and self-powered marine environment monitoring. Nano Energy 2023, 108, 108222. [Google Scholar] [CrossRef]
- Cheng, Y.; Song, F.; Fu, L.; Dai, S.; Yuan, Z.; Incecik, A. Experimental investigation of a dual-pontoon WEC-type breakwater with a hydraulic-pneumatic complementary power take-off system. Energy 2024, 286, 129427. [Google Scholar] [CrossRef]
- Everett, J.; Sorokin, V.; Whittaker, C.; Aw, K. Numerical and experimental analysis of the power output performance of a point absorber WEC for nearshore wave conditions. Ocean Eng. 2024, 309, 118381. [Google Scholar] [CrossRef]
- Azam, A.; Ahmed, A.; Yi, M.; Zhang, Z.; Tan, X.; Ali, A.; Li, N. A self-stabilizing point absorber wave energy converter with a top-shaped buoy and non-linear power take-off for oceanographic applications. Ocean Eng. 2023, 288, 116018. [Google Scholar] [CrossRef]
- He, G.; Luan, Z.; Zhang, W.; He, R.; Liu, C.; Yang, K.; Yang, C.; Jing, P.; Zhang, Z. Review on research approaches for multi-point absorber wave energy converters. Renew. Energy 2023, 218, 119237. [Google Scholar] [CrossRef]
- Han, M.; Cao, F.; Shi, H.; Kou, H.; Gong, H.; Wang, C. Parametrical study on an array of point absorber wave energy converters. Ocean Eng. 2023, 272, 113857. [Google Scholar] [CrossRef]
- Ni, W.; Zhang, X.; Zhang, W.; Liang, S. Numerical investigation of adaptive damping control for raft-type wave energy converters. Renew. Energy 2021, 175, 520–531. [Google Scholar] [CrossRef]
- Liu, C.; Hu, M.; Zhao, Z.; Zeng, Y.; Gao, W.; Chen, J.; Yan, H.; Zhang, J.; Yang, Q.; Bao, G.; et al. Latching control of a raft-type wave energy converter with a hydraulic power take-off system. Ocean Eng. 2021, 236, 109512. [Google Scholar] [CrossRef]
- Opoku, F.; Uddin, M.; Atkinson, M. A review of computational methods for studying oscillating water columns–the Navier-Stokes based equation approach. Renew. Sustain. Energy Rev. 2023, 174, 113124. [Google Scholar] [CrossRef]
- Trivedi, K.; Santanu, K. Performance of a hybrid wave energy converter device consisting of a piezoelectric plate and oscillating water column device placed over an undulated seabed. Appl. Energy 2023, 333, 120627. [Google Scholar] [CrossRef]
- Xu, C.; Zuo, J.; He, Y.; Li, J. Characteristics of wave loading and viscous energy loss of a bottom-sitting U-OWC wave energy device: A validated numerical perspective. Energy 2024, 308, 132898. [Google Scholar] [CrossRef]
- He, F.; Jiang, H.; Lin, Y.; Pan, J.; Zhang, Y.; Huang, C. Multi-phase SPH-FDM and experimental investigations on the hydrodynamics of an oscillating water column wave energy device. Coast. Eng. 2024, 192, 104569. [Google Scholar] [CrossRef]
- Yazdi, H.; Ghafari, H.; Ghassemi, H.; He, G.; Karimirad, M. Wave power extraction by Multi-Salter’s duck WECs arrayed on the floating offshore wind turbine platform. Energy 2023, 278, 127930. [Google Scholar] [CrossRef]
- Su, H.; Qin, H.; Wen, Z.; Liang, H.; Jiang, H.; Mu, L. Optimization of latching control for duck wave energy converter based on deep reinforcement learning. Ocean. Eng. 2024, 309, 118531. [Google Scholar] [CrossRef]
- Jin, S.; Wang, D.; Hann, M.; Collins, K.; Conley, D.; Greaves, D. A designed two-body hinged raft wave energy converter: From experimental study to annual power prediction for the EMEC site using WEC-Sim. Ocean Eng. 2023, 267, 113286. [Google Scholar] [CrossRef]
- Abbasi, A.; Ghassemi, H. Numerical results of the dynamic response and capture factor of the two-raft-type WEC. Energy Convers. Manag. 2024, 303, 118176. [Google Scholar] [CrossRef]
- Zhao, C.; Han, M.; Johanning, L.; Shi, H. Multi-freedom effects on a raft-type wave energy convertor- hydrodynamic response and energy absorption. Ocean Eng. 2024, 305, 117964. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, H.; Zhou, X.; Guo, B.; Xu, D. Hybrid control strategy for efficiency enhancement of a raft-type wave energy converter. Energy 2024, 300, 131519. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, J.; Zhou, X.; Shi, Q.; Xu, D.; Sun, Z.; Lu, Y.; Wu, B. Robust performance improvement of a raft-type wave energy converter using a nonlinear stiffness mechanism. Int. J. Mech. Sci. 2021, 211, 106776. [Google Scholar] [CrossRef]
- Liu, C.; Hu, M.; Gao, W.; Chen, J.; Zeng, Y.; Wei, D.; Yang, Q.; Bao, G. A high-precise model for the hydraulic power take-off of a raft-type wave energy converter. Energy 2021, 215, 119107. [Google Scholar] [CrossRef]
- Molla, S.; Farrok, O.; Islam, M.; Xu, W. A systematic approach for designing a highly efficient linear electrical generator for harvesting oceanic wave energy. Renew. Energy 2023, 204, 152–165. [Google Scholar] [CrossRef]
- Curto, D.; Franzitta, V.; Guercio, A.; Miceli, R.; Nevoloso, C.; Raimondi, F.M.; Trapanese, M. An Experimental Comparison between an Ironless and a Traditional Permanent Magnet Linear Generator for Wave Energy Conversion. Energies 2022, 15, 2387. [Google Scholar] [CrossRef]
- Qiu, S.; Zhao, W.; Zhang, C.; Shek, J.K.; Wang, H. A Novel Structure of Tubular Staggered Transverse-Flux Permanent-Magnet Linear Generator for Wave Energy Conversion. IEEE Trans. Energy Convers. 2022, 37, 24–35. [Google Scholar] [CrossRef]
- Liu, C.; Chen, Y.; Dong, R.; Ye, B.-L. Optimization design of Tubular Permanent Magnet Linear Generator based on entropy model for wave energy conversion. Renew. Energy 2023, 216, 119087. [Google Scholar] [CrossRef]
- Zhang, J.; Yu, H.; Shi, Z. Analysis of a PM Linear Generator with Double Translators for Complementary Energy Generation Platform. Energies 2019, 12, 4606. [Google Scholar] [CrossRef]
- Zhang, J.; Yu, H.; Shi, Z. Design and Experiment Analysis of a Direct-Drive Wave Energy Converter with a Linear Generator. Energies 2018, 11, 735. [Google Scholar] [CrossRef]













| β1 | 0.1 | 0.2 | 0.3 | 0.4 | 0.5 | 0.6 | 0.7 | 0.8 | 0.9 |
|---|---|---|---|---|---|---|---|---|---|
| rmo (m) | 0.033 | 0.046 | 0.059 | 0.072 | 0.085 | 0.098 | 0.111 | 0.124 | 0.137 |
| N | 2900 | 2575 | 2250 | 1925 | 1600 | 1275 | 950 | 625 | 300 |
| Modeling Method | Axial | Radial | |
|---|---|---|---|
| Equivalent magnetic circuit model | α | 0.9 | 1 |
| β | 0.8 | 0.65 | |
| Simulation | α | 0.8 | 1 |
| β | 0.8 | 0.6 | |
| Vpeak | 9.59 | 35.66 | |
| VRMS | 6.42 | 27.23 | |
| Parameter | Axial | Radial |
|---|---|---|
| rmi (cm) | 5 | 5 |
| rmo (cm) | 33 | 26 |
| rci (cm) | 34 | 27 |
| rco (cm) | 40 | 40 |
| τ (cm) | 40 | 40 |
| τmr (cm) | 40 | |
| τma (cm) | 36 | |
| N | 120 | 260 |
| Number of coils | 8 | 8 |
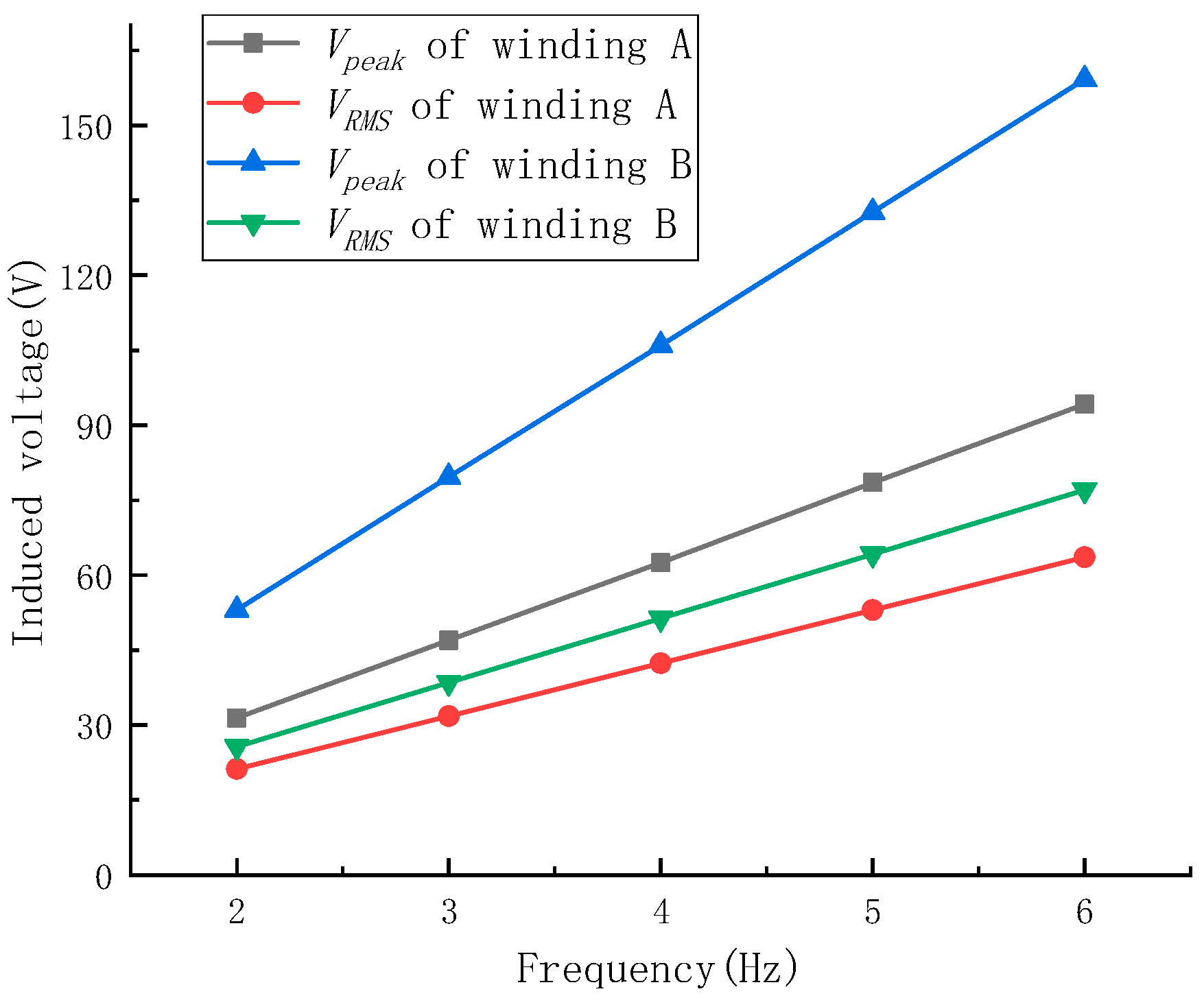
| Frequency | Axial | Radial | ||
|---|---|---|---|---|
| VRMS of Winding A | VRMS of Winding B | VRMS of Winding A | VRMS of Winding B | |
| 2 | 4.76 | 4.65 | 21.14 | 25.69 |
| 3 | 7.15 | 6.95 | 31.80 | 38.52 |
| 4 | 9.56 | 9.26 | 42.32 | 51.38 |
| 5 | 11.99 | 11.58 | 52.89 | 64.21 |
| 6 | 14.38 | 13.85 | 63.53 | 77.03 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Duan, W.; Zhang, Y.; Liu, S.; Shen, Q.; Hou, Z.; Chen, R. Design and Modeling of Coreless Magnetoelectric Transducers for Snake-like Wave Energy Converters. Electronics 2024, 13, 4092. https://doi.org/10.3390/electronics13204092
Duan W, Zhang Y, Liu S, Shen Q, Hou Z, Chen R. Design and Modeling of Coreless Magnetoelectric Transducers for Snake-like Wave Energy Converters. Electronics. 2024; 13(20):4092. https://doi.org/10.3390/electronics13204092
Chicago/Turabian StyleDuan, Weiping, Yuxiang Zhang, Shihao Liu, Qian Shen, Zhiwei Hou, and Renwen Chen. 2024. "Design and Modeling of Coreless Magnetoelectric Transducers for Snake-like Wave Energy Converters" Electronics 13, no. 20: 4092. https://doi.org/10.3390/electronics13204092
APA StyleDuan, W., Zhang, Y., Liu, S., Shen, Q., Hou, Z., & Chen, R. (2024). Design and Modeling of Coreless Magnetoelectric Transducers for Snake-like Wave Energy Converters. Electronics, 13(20), 4092. https://doi.org/10.3390/electronics13204092






