An Oscillation Stability Analysis of a Direct-Driven Wind Power Grid-Connected System Considering Low Voltage Ride though from an Energy Perspective
Abstract
1. Introduction
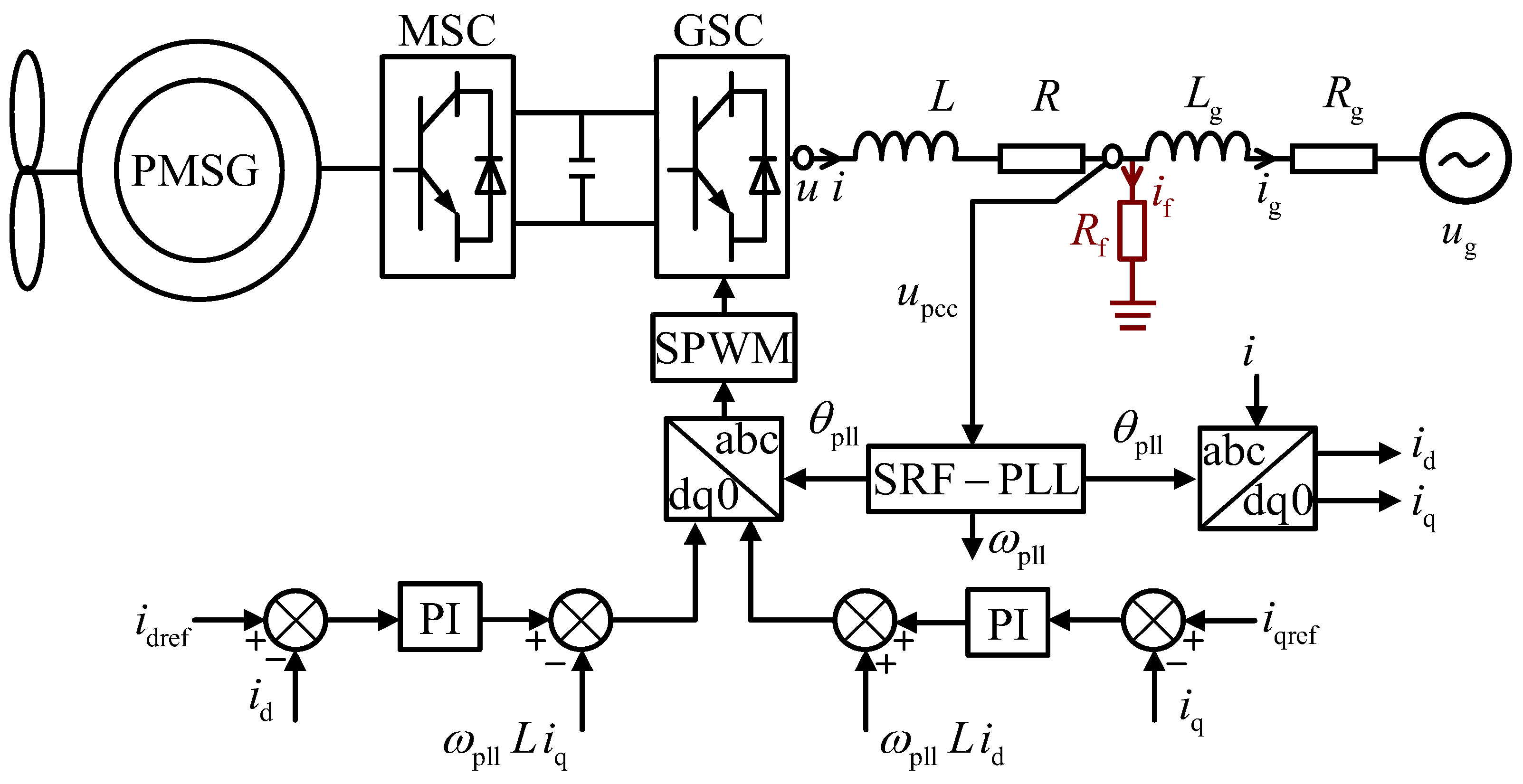
2. Dynamic Energy Model of Direct-Driven Wind-Powered Grid-Connected System during Low-Voltage Crossing
2.1. Mathematical Model of Direct-Drive Wind-Powered Grid-Connected System during Low-Voltage Crossing
2.2. Dynamic Energy Model of Direct-Drive Fan during Low-Voltage Crossing
3. Analysis of System Stability after Low-Voltage Traverse Control Switching
3.1. Dynamic Energy Stability Analysis Criterion
3.2. Influence of Energy Interaction Path on the Stability of Direct-Driven Wind Power Grid-Connected System
3.2.1. The Influence of Current Inner Loop Energy on the Oscillation Stability of the System
3.2.2. The Influence of PLL Energy on the Oscillation Stability of the System
3.2.3. The Influence of the Current Inner Loop and Phase-Locked Loop Interaction Energy on the Oscillation Stability of the System
4. Simulation Analysis
4.1. Test System
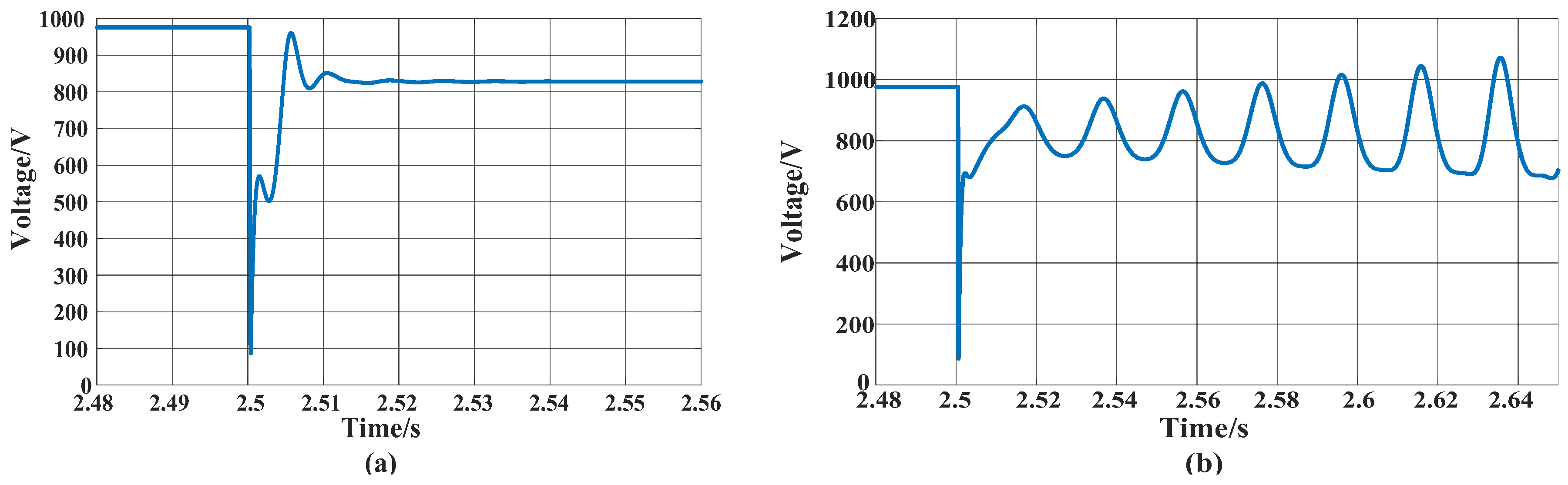
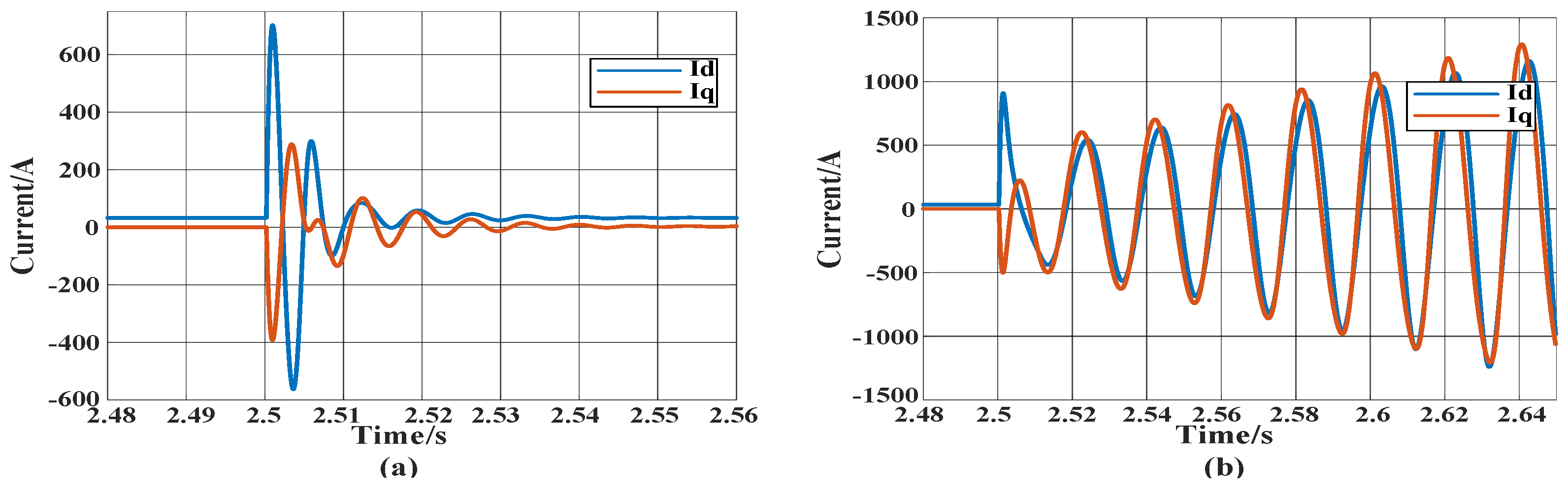
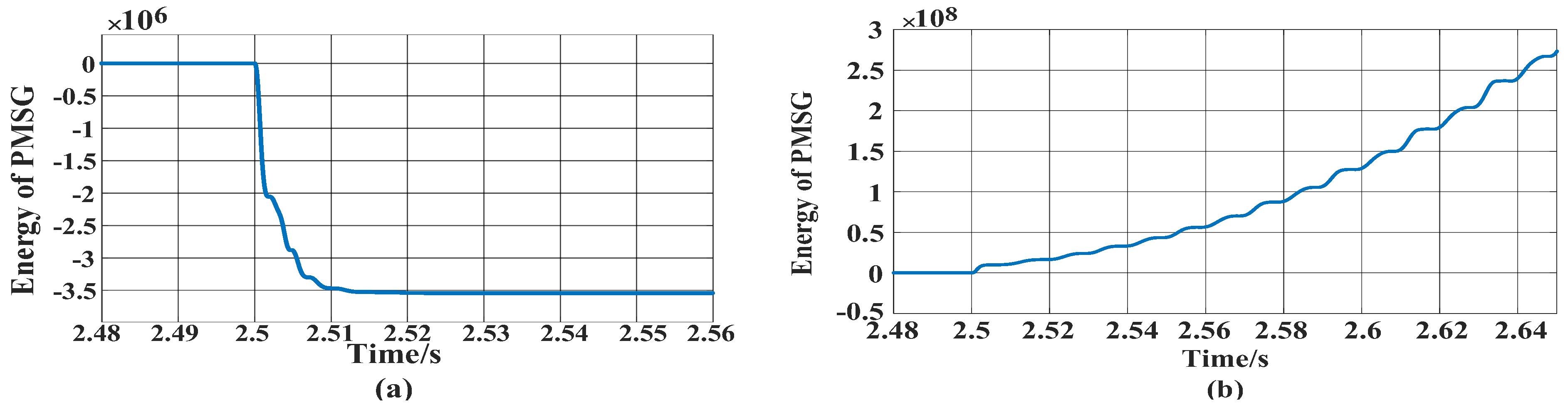
4.2. Dynamic Energy Model Verification of Direct-Driven Wind-Powered Grid-Connected System
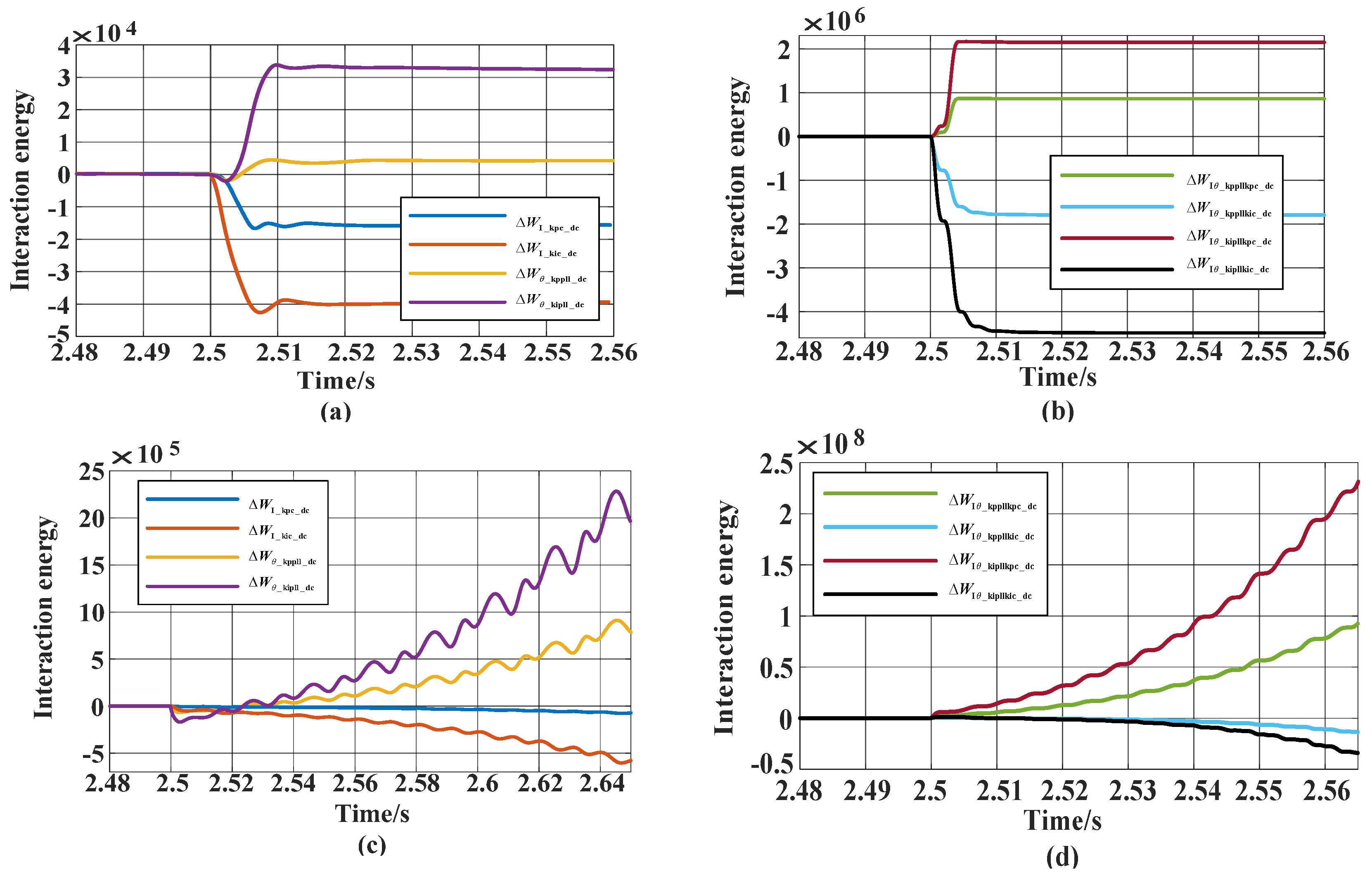
4.3. Energy Path Verification of Direct-Driven Wind-Powered Grid-Connected System
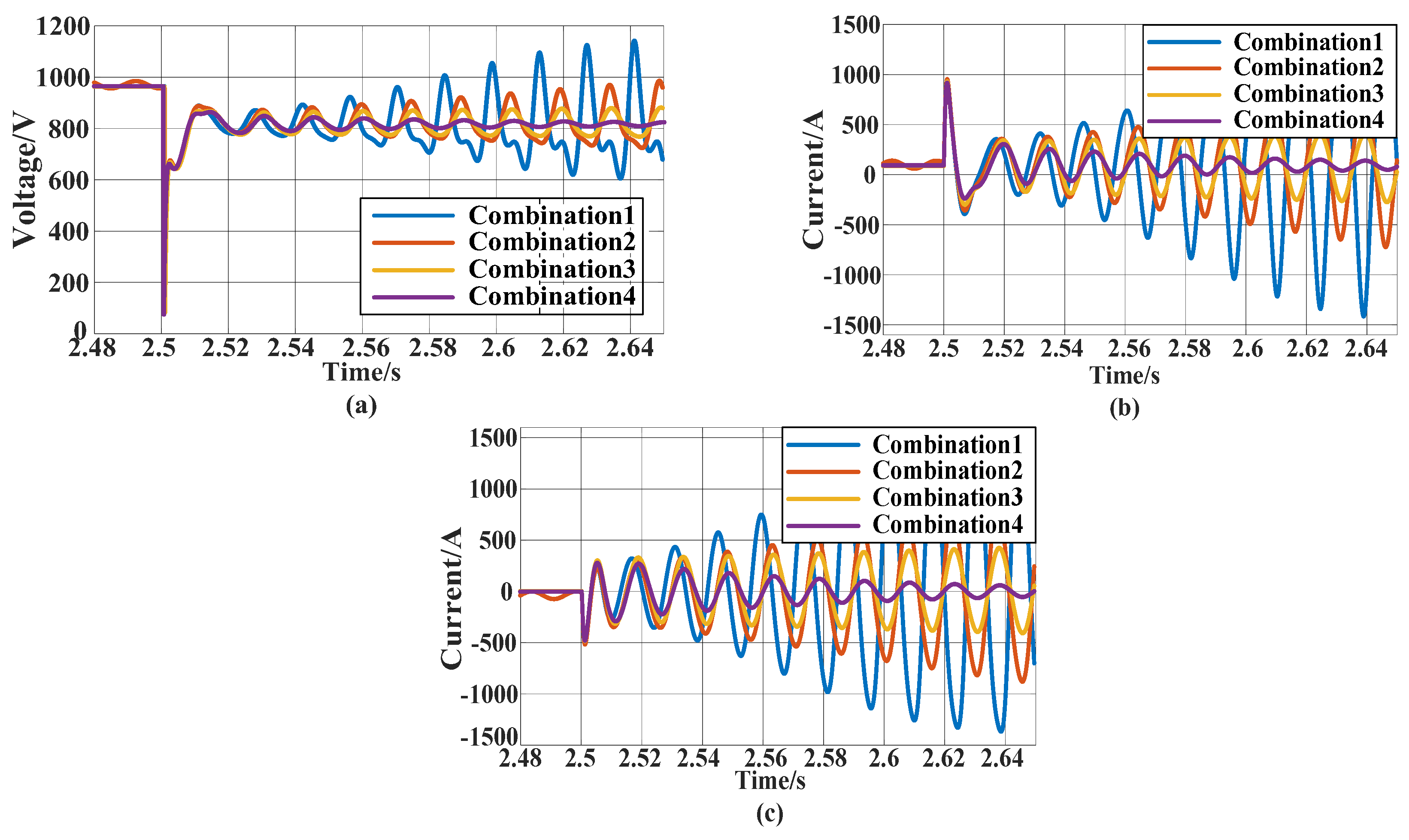
4.4. Control Parameter Influence Analysis Validation
5. Conclusions
- The dynamic energy model of a direct-driven wind-powered grid-connected system with low-voltage crossing control is derived. The energy includes line parameter energy, current inner loop energy, phase-locked loop energy, and current inner loop–phase-locked loop interaction energy.
- The primary element influencing a direct-driven wind-powered grid-connected system’s stability level is the current inner loop–PLL interaction. The direct-driven wind-powered grid-connected systems are more stable when there is a current inner loop integration–PLL interaction and a current inner loop integration–PLL proportional interaction. The grid-connected systems’ stability is not supported by the existing inner loop proportional–PLL integral interaction or inner loop proportional–PLL proportional interaction.
- The simulation test parameters of the control group were adjusted as follows: the current inner loop proportion parameter increased from 1.32 to 5.28, the current inner loop integral parameter increased from 4.48 to 6.42, the PLL proportion parameter decreased from 9.45 to 6.3, and the PLL integral parameter decreased from 50.25 to 40.2. Both theoretical and experimental results show that increasing the current inner loop integral and proportion parameters can improve the stability level of the direct-driven wind-powered grid-connected system, and reducing the phase-locked loop proportion and integral parameters can also improve the stability level of the grid-connected system.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| PMSG | permanent-magnet synchronous generator |
| SPWM | sine pulse width modulation |
| SRF-PLL | synchronous reference frame phase-locked loop |
| the output current of the converter | |
| the port voltage of the converter | |
| grid connection voltage of PMSG | |
| grid connection current of PMSG | |
| fault current at the junction point | |
| voltage at the junction point | |
| line resistance on converter side | |
| line inductance on converter side | |
| line resistance on the power grid side | |
| line inductance on the grid side | |
| transition resistance | |
| phase angle of the PLL output | |
| the angular frequency of the PLL output | |
| synchronous angular frequency | |
| proportional parameters of the inner loop of the current | |
| integral parameters of the inner loop of the current | |
| proportional parameters of PLL | |
| integral parameters of PLL | |
| Subscripts | |
| perturbation of a variable | |
| synchronously rotate the d,q axis | |
| 0 | initial value |
| ref | reference value |
Appendix A
Appendix B
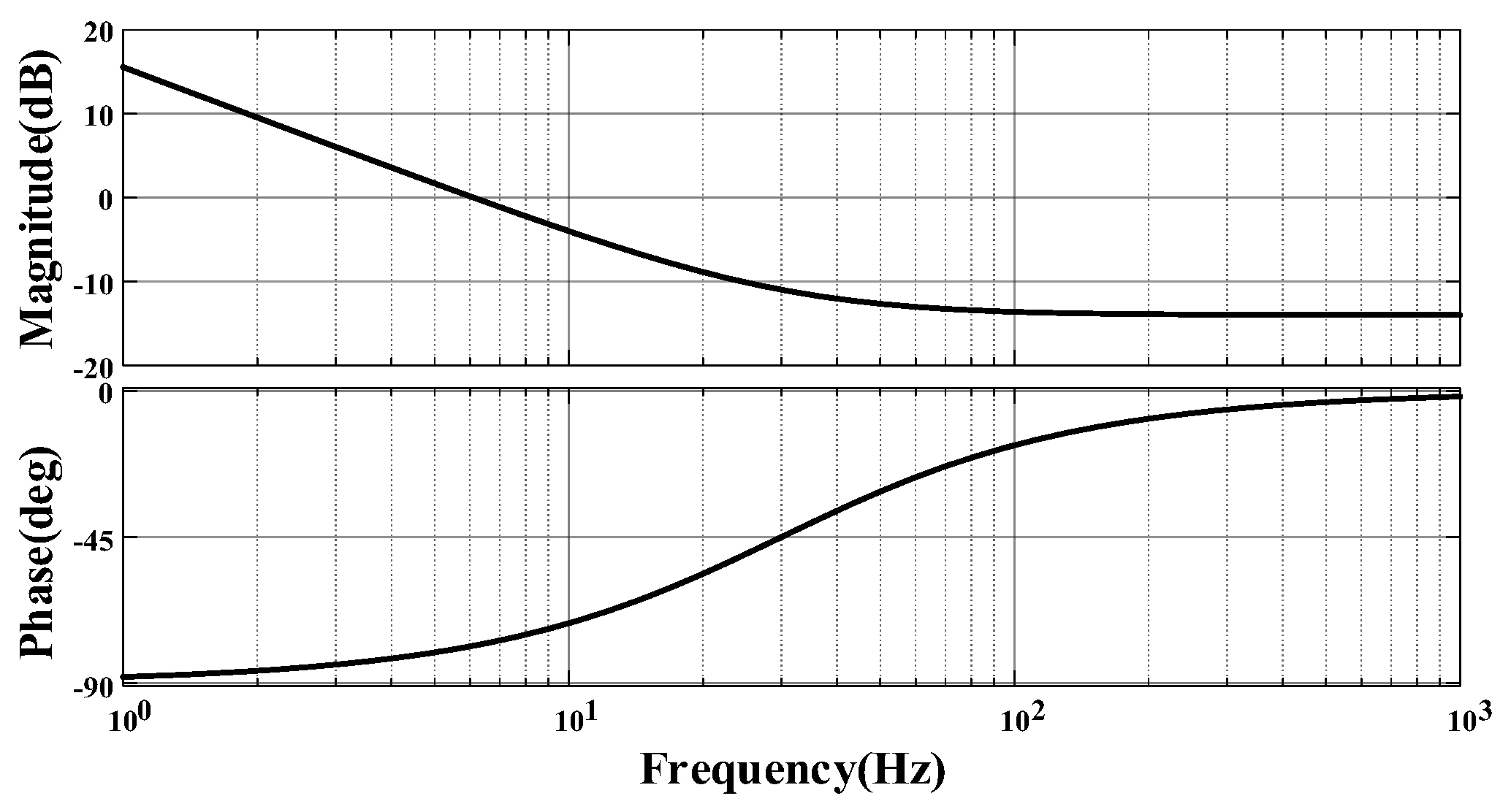

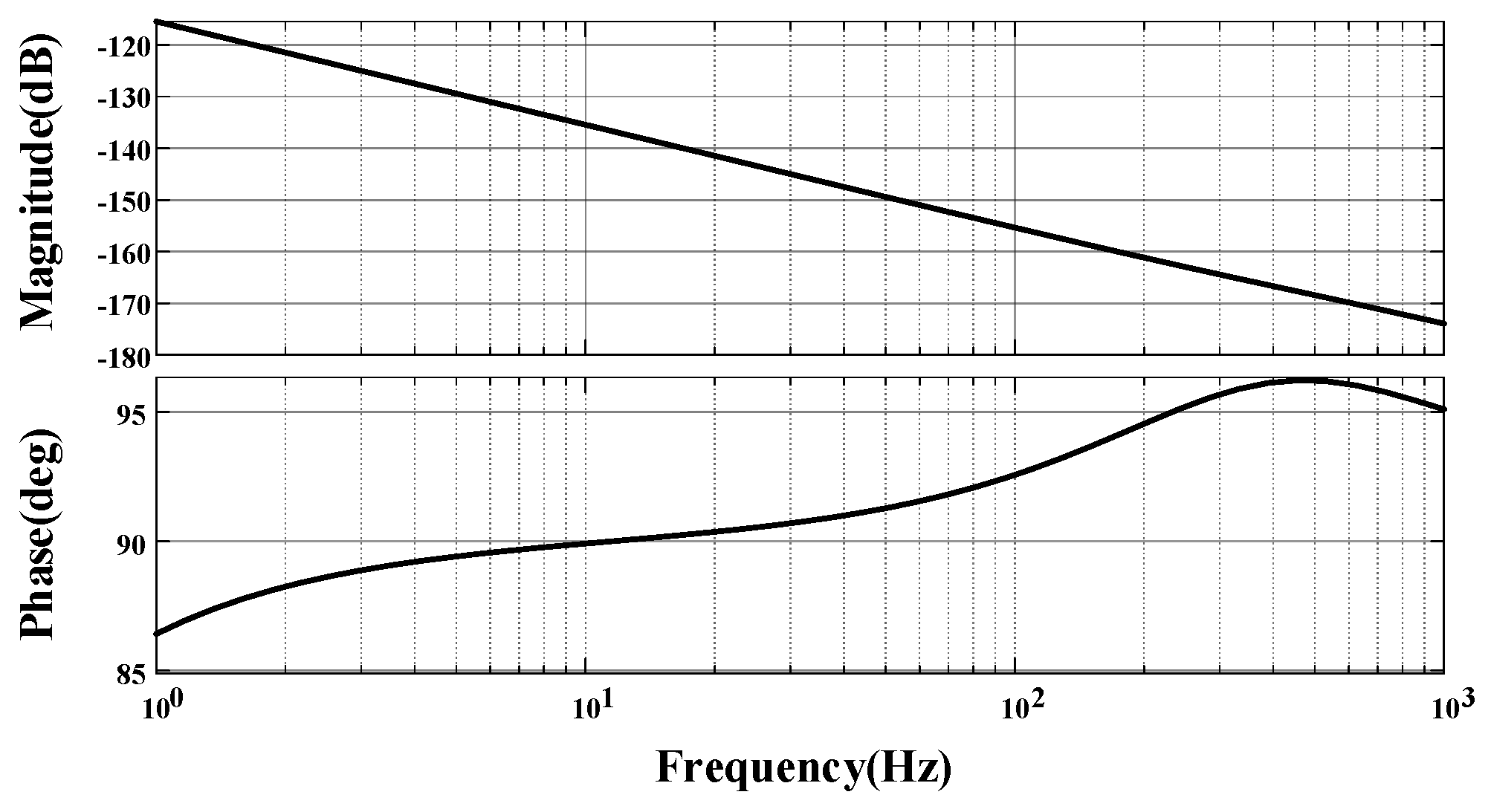
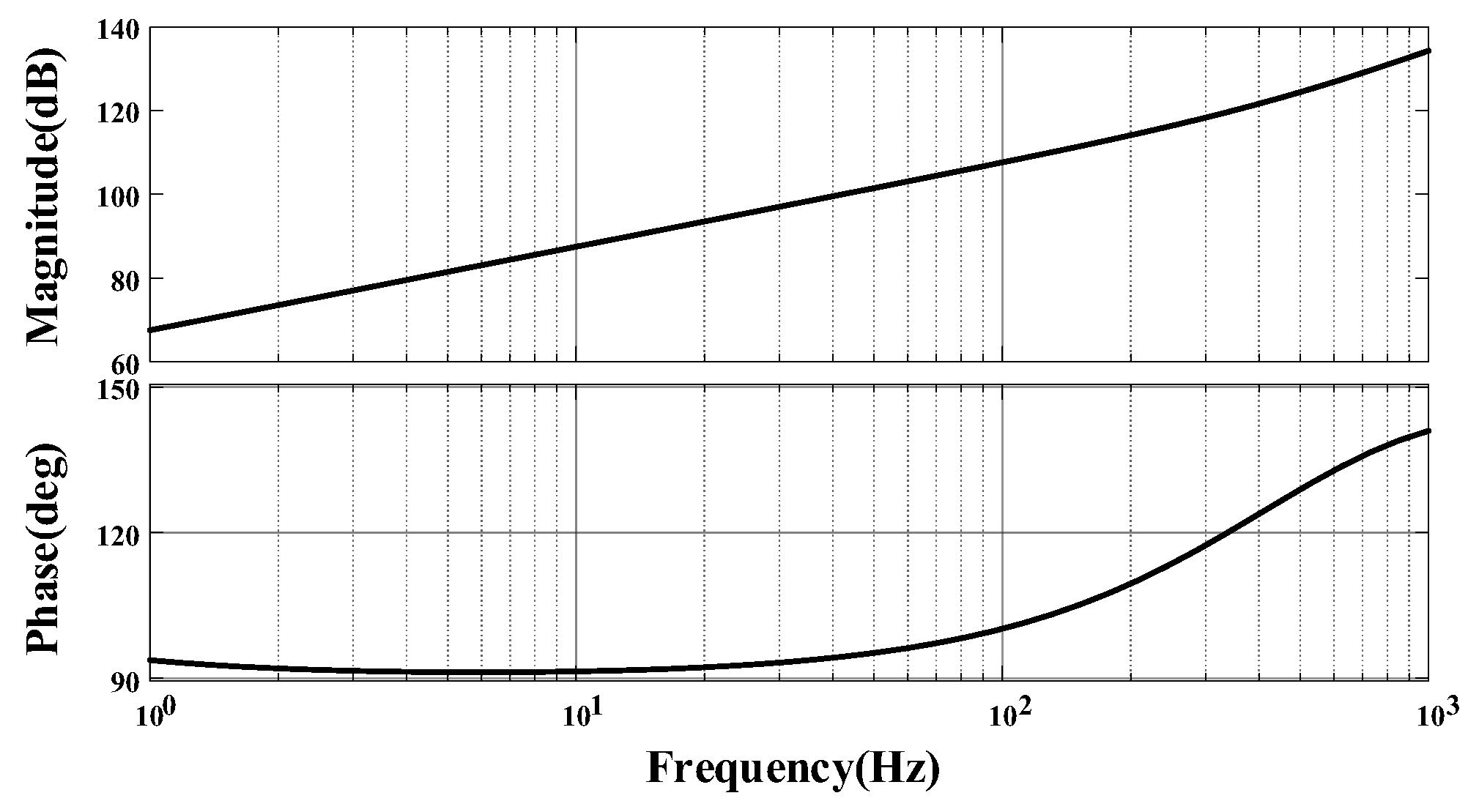
References
- Zou, X.; Du, X.; Tai, H.-M. Two-Variable Admittance Model for D-PMSG-Based Wind Turbine and Stability Criterion Based on Magnitude and Phase Contour Plot. IEEE Trans. Power Electron. 2020, 35, 1484–1498. [Google Scholar] [CrossRef]
- Zhan, Y.; Chen, W.; Xie, X. Frequency domain mode analysis of sub-synchronous oscillation in grid-connected wind power systems. Autom. Electr. Power Syst. 2020, 44, 90–97. [Google Scholar]
- Liu, B.; Li, Z.; Dong, X.; Samson, S.Y.; Chen, X.; Oo, A.M.; Lian, X.; Shan, Z.; Liu, X. Impedance Modeling and Controllers Shaping Effect Analysis of PMSG Wind Turbines. IEEE J. Emerg. Sel. Top. Power Electron. 2021, 9, 1465–1478. [Google Scholar] [CrossRef]
- Liu, B.; Li, Z.; Zhang, X.; Dong, X.; Liu, X. Impedance-Based Analysis of Control Interactions in Weak-Grid-Tied PMSG Wind Turbines. IEEE J. Emerg. Sel. Top. Circuits Syst. 2021, 11, 90–98. [Google Scholar] [CrossRef]
- Du, W.; Dong, W.; Wang, H. Small-Signal Stability Limit of a Grid-Connected PMSG Wind Farm Dominated by the Dynamics of PLLs. IEEE Trans. Power Syst. 2020, 35, 2093–2107. [Google Scholar] [CrossRef]
- Du, W.; Wang, Y.; Wang, Y.; Wang, H.F.; Xiao, X. Analytical Examination of Oscillatory Stability of a Grid-Connected PMSG Wind Farm Based on the Block Diagram Model. IEEE Trans. Power Syst. 2021, 36, 5670–5683. [Google Scholar] [CrossRef]
- Liu, F.; Li, Y.; He, G.; Li, G.; Liu, S.; Small, W.L. Signal Modeling and Stable Operation Strategy Analysis of direct-drive wind power grid-connected Converter in Extremely Weak Current Network. Electr. Power Autom. Equip. 2022, 42, 167–173. [Google Scholar]
- Wang, D.; Sun, H.; Huang, B.; Han, Y.; Mao, Y.; Zhu, T. Stability Analysis of Voltage Source Direct Drive Wind Turbine Connected to the Grid Based on Virtual Synchronous Control. High Volt. Technol. 2022, 48, 3282–3294. [Google Scholar]
- Shen, Y.; Ma, J.; Li, P.; Huo, Q.; Sun, S.; Huang, Y.; Zhao, S. Subsynchronous Oscillation suppression Strategy of Virtual synchronous doubly-fed Fan with Additional energy branch. Autom. Electr. Power Syst. 2022, 46, 83–93. [Google Scholar]
- Liu, H.; Zhang, X.; Li, Y.; Fu, Y.; Qin, L. Virtual Axis Control and Transient Energy Transfer for Double-fed Wind Turbine. In Proceedings of the CSEE, Sanya, China, 25–27 February 2022; Volume 42, pp. 7007–7019. [Google Scholar]
- Zhang, X.; Huang, Y.; Fu, Y. Analysis of transient Energy Transfer and Oscillation Characteristics of doubly-fed wind Turbine under Nonlinear Elastic Coupling. Trans. China Electrotech. Soc. 2024, 39, 1–19. [Google Scholar]
- Liu, C.; Han, J.; Shang, L.; Dong, X.; Xie, X. Modeling and Quantitative Analysis of wide-frequency oscillation mechanism of new energy Transmission System from the perspective of energy. Power Grid Technol. 2023, 47, 3980–3993. [Google Scholar]
- Ma, J.; Xu, H.; Li, X. Transient Stability Mechanism of direct-drive grid-connected Wind Power System under Strong Interaction. In Proceedings of the CSEE, Shanghai, China, 27–29 February 2024; pp. 1–13. [Google Scholar]
- Jia, K.; Liu, Q.; Yang, B.; Hou, L.; Fang, Y.; Bi, T. Fault Transient Current Analysis of Inverter with PLL Dynamic Characteristics. Power Grid Technol. 2021, 45, 4242–4251. [Google Scholar]
- Ma, J.; Gu, Y.; Shen, Y.; Zhou, Y.; Cheng, P. Stability Analysis of Power Grid Connected with Direct-Drive Wind Farm Containing Virtual Inertia Based on Integrated Dissipation Energy Model. IEEE Trans. Sustain. Energy 2021, 12, 2378–2392. [Google Scholar] [CrossRef]


| Working Condition | ||||
|---|---|---|---|---|
| Oscillation convergence | 6.2 | 12.1 | 6.3 | 40.2 |
| Oscillation divergence | 1.32 | 4.84 | 8.82 | 48.24 |
| Combination | ||||
|---|---|---|---|---|
| 1 | 1.32 | 4.84 | 9.45 | 50.25 |
| 2 | 1.32 | 6.42 | 9.45 | 50.25 |
| 3 | 1.32 | 6.42 | 6.3 | 40.2 |
| 4 | 5.28 | 6.42 | 6.3 | 40.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, B.; Wang, C.; Li, Q.; Zou, X.; Wang, D.; Hu, Y. An Oscillation Stability Analysis of a Direct-Driven Wind Power Grid-Connected System Considering Low Voltage Ride though from an Energy Perspective. Electronics 2024, 13, 3489. https://doi.org/10.3390/electronics13173489
Ren B, Wang C, Li Q, Zou X, Wang D, Hu Y. An Oscillation Stability Analysis of a Direct-Driven Wind Power Grid-Connected System Considering Low Voltage Ride though from an Energy Perspective. Electronics. 2024; 13(17):3489. https://doi.org/10.3390/electronics13173489
Chicago/Turabian StyleRen, Bixing, Chenggen Wang, Qiang Li, Xiaoming Zou, Dajiang Wang, and Yingjie Hu. 2024. "An Oscillation Stability Analysis of a Direct-Driven Wind Power Grid-Connected System Considering Low Voltage Ride though from an Energy Perspective" Electronics 13, no. 17: 3489. https://doi.org/10.3390/electronics13173489
APA StyleRen, B., Wang, C., Li, Q., Zou, X., Wang, D., & Hu, Y. (2024). An Oscillation Stability Analysis of a Direct-Driven Wind Power Grid-Connected System Considering Low Voltage Ride though from an Energy Perspective. Electronics, 13(17), 3489. https://doi.org/10.3390/electronics13173489





