A Novel DOA Estimation Algorithm Based on Robust Mixed Fractional Lower-Order Correntropy in Impulsive Noise
Abstract
1. Introduction
- Development of MFLOCR: This paper proposes a new correntropy called MFLOCR, which extends the concept of correntropy using different kernel lengths to jointly regulate the kernel function. The proposed method is based on the theory of FLOS and uses mixed correntropy with an exponential kernel instead of the traditional covariance matrix, which provides greater robustness against impulsive noise and enhances the effectiveness of the algorithm in environments with Alpha-stable distributed impulsive noise.
- MFLOCR-MUSIC algorithm: An improved DOA estimation algorithm, MFLOCR-MUSIC, was developed by combining the MFLOCR operator with the traditional MUSIC algorithm. This combination is specifically tailored for improved performance in impulsive noise environments.
- Proof of Boundedness: The proof of boundedness serves to verify the efficiency and stability of the proposed MFLOCR-MUSIC algorithm under impulsive noise and ensures its reliability in real applications.
- Superior Performance in Simulations: Simulation experiments showed that the proposed MFLOCR-MUSIC algorithm outperforms existing DOA estimation methods in various characteristic indices. It was proven to be highly accurate and robust.
2. Problem Formulation
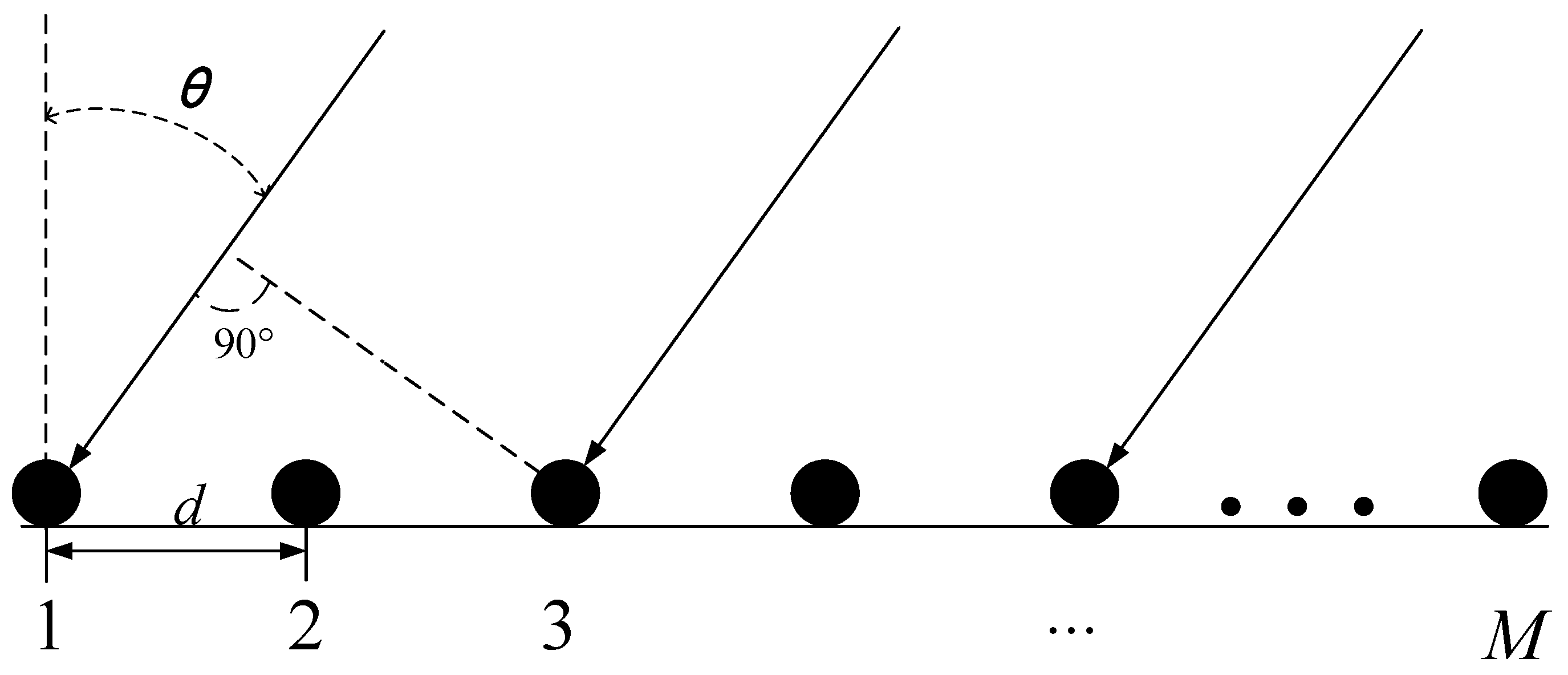
2.1. Signal Model of DOA Estimation
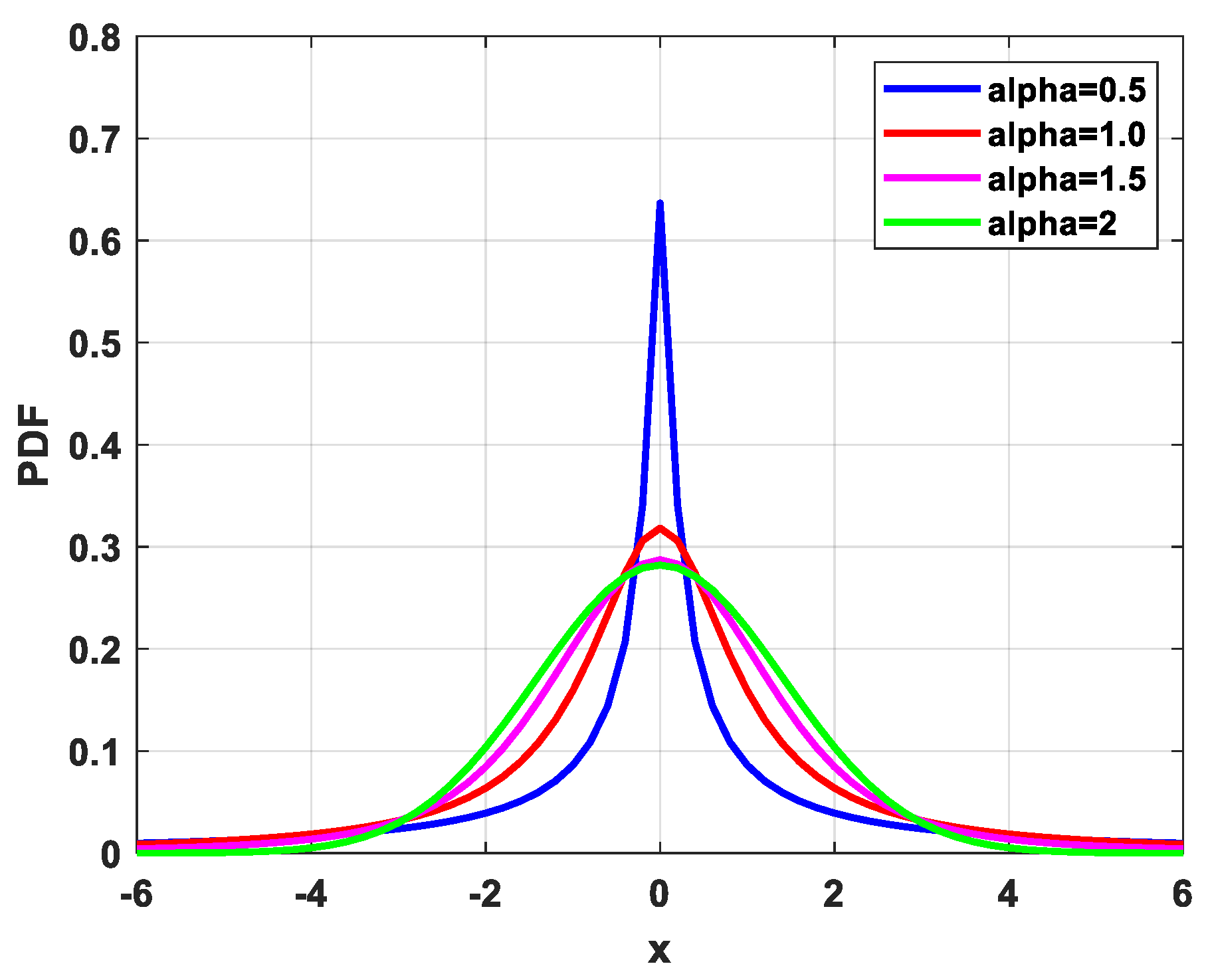
2.2. Impulsive Noise Model
2.3. Correntropy
3. The Proposed MFLOCR-MUSIC Algorithm
3.1. FLOM-MUSIC Algorithm
3.2. MFLOCR-MUSIC Algorithm
3.3. The Implementation of MFLOCR–MUSIC
3.4. The Boundedness of MFLOCR
4. Simulation
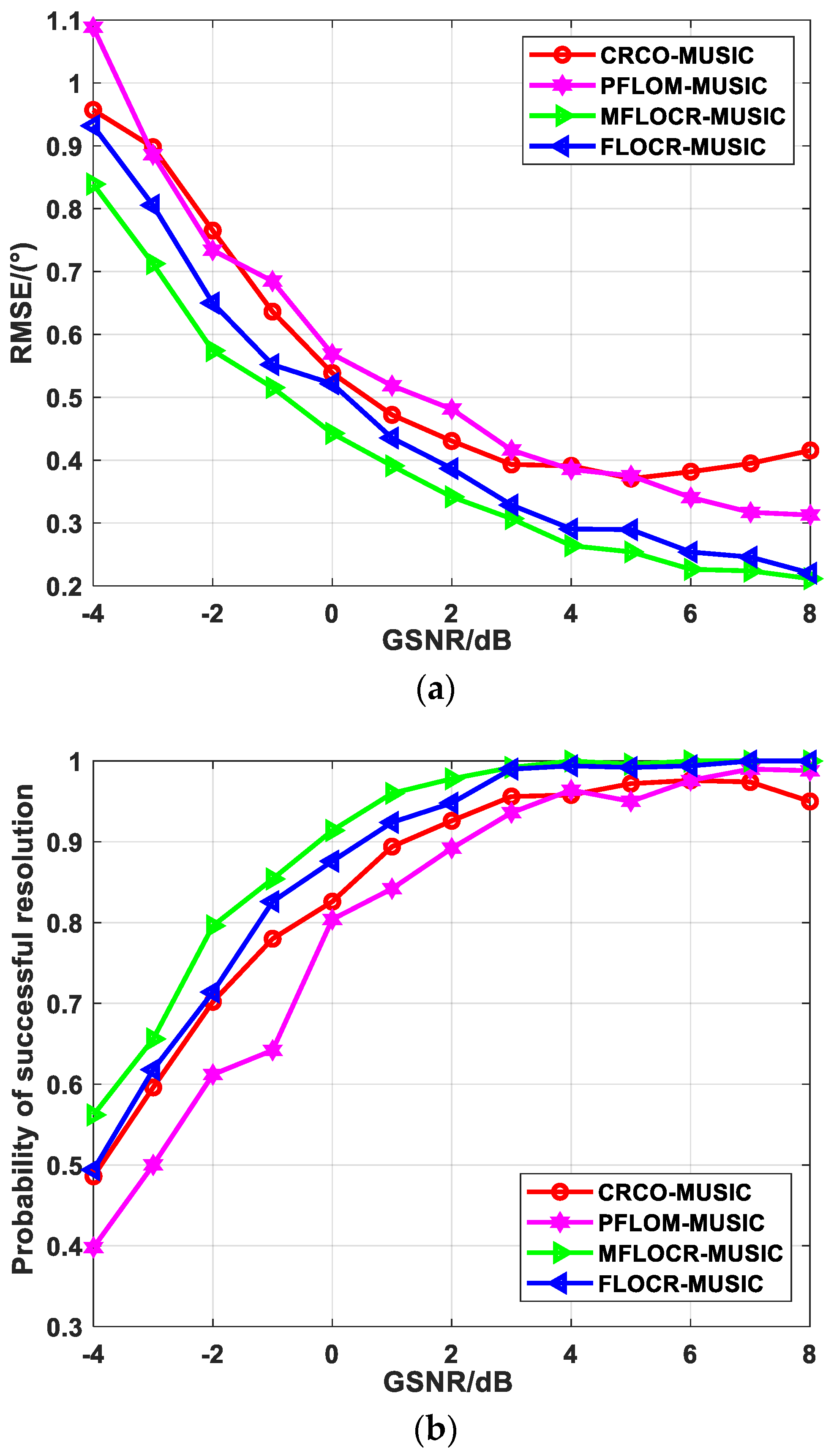
4.1. Effect of GSNR
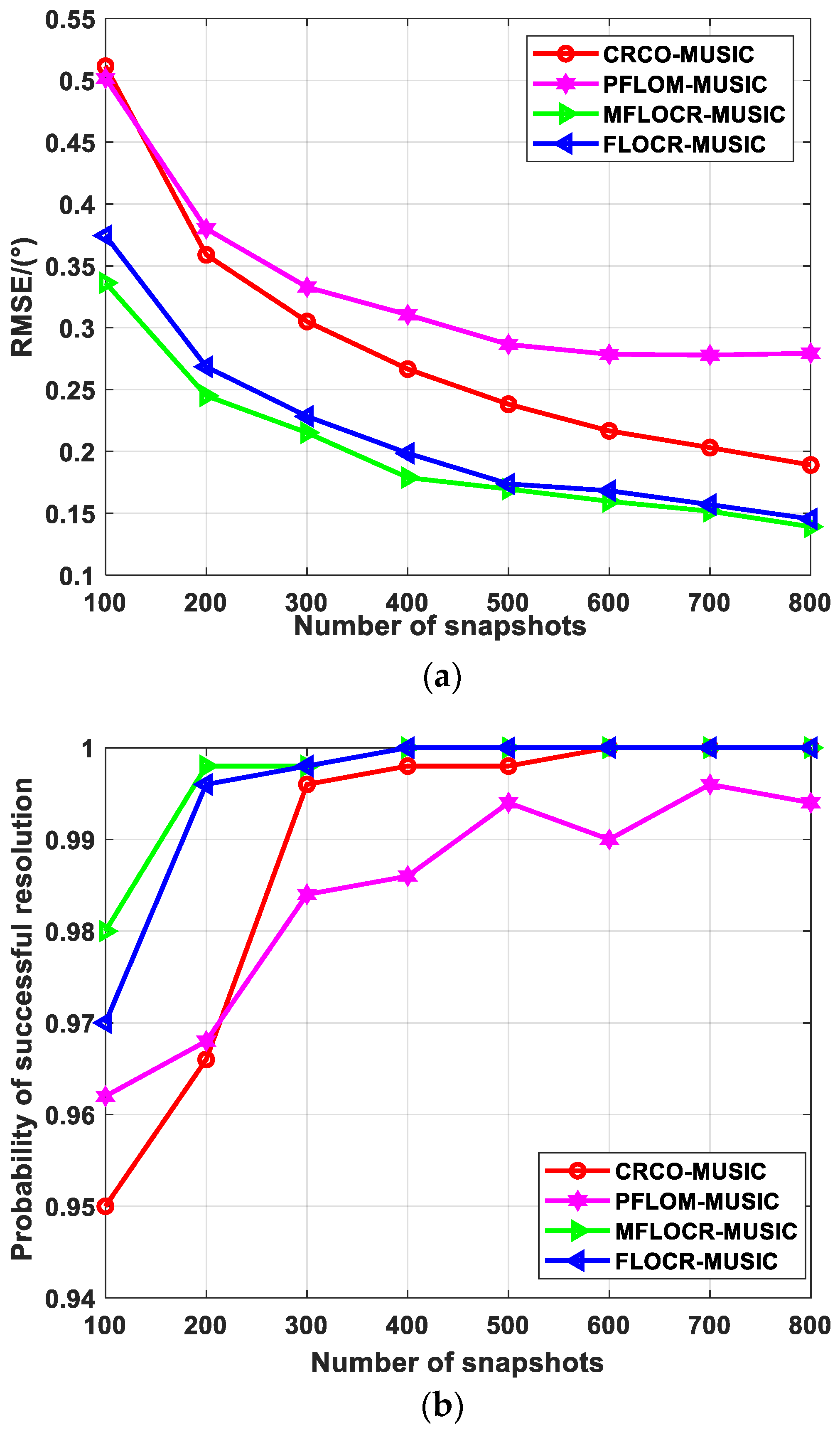
4.2. Effect of the Number of Snapshots
4.3. Characteristic Exponent
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Tang, W.; Jiang, H.; Zhang, Q. Coarray interpolation for joint DOD and DOA estimation in bistatic coprime MIMO radar via decoupled atomic norm minimization. Multidimens. Syst. Signal Process. 2022, 33, 1237–1256. [Google Scholar] [CrossRef]
- Ganguly, S.; Ghosh, J.; Kumar, P.K.; Mukhopadhyay, M. An Efficient DOA Estimation and Jammer Mitigation Method by Means of a Single Snapshot Compressive Sensing Based Sparse Coprime Array. Wirel. Pers. Commun. 2022, 123, 2737–2757. [Google Scholar] [CrossRef]
- Cui, J.; Pan, W.; Wang, H. Direction of Arrival Estimation Method Based on Eigenvalues and Eigenvectors for Coherent Signals in Impulsive Noise. Mathematics 2024, 12, 832. [Google Scholar] [CrossRef]
- Asghari, M.; Zareinejad, M.; Rezaei, S.M.; Amindavar, H. DOA Estimation of Noncircular Signals Under Impulsive Noise Using a Novel Empirical Characteristic Function-Based MUSIC. Circuits Syst. Signal Process. 2023, 42, 3706–3743. [Google Scholar] [CrossRef]
- Todros, K.; Hero, A.O. Robust Multiple Signal Classification via Probability Measure Transformation. IEEE Trans. Signal Process. 2015, 63, 1156–1170. [Google Scholar] [CrossRef]
- Hacioglu; Harmanci; Anarim; Delic. Time-of-Arrival Estimation with FLOM-MUSIC Under Impulsive Noise. In Proceedings of the 2006 IEEE 14th Signal Processing and Communications Applications, Antalya, Turkey, 17–19 April 2006; pp. 1–4. [Google Scholar]
- Qiu, S.; Ma, X.; Zhang, R.; Han, Y.; Sheng, W. A dual-resolution unitary ESPRIT method for DOA estimation based on sparse co-prime MIMO radar. Signal Process. 2023, 202, 108753. [Google Scholar] [CrossRef]
- Yuvaraj, R.; Aravind Kumar, Y.V. Performance Analysis of Optimal Analog Beamforming with Modified Esprit Doa Estimation Algorithm for Mimo Noma System Over Rayleigh Fading Channel. ECS Trans. 2022, 107, 11361. [Google Scholar] [CrossRef]
- Xiao, M.; Duan, Z.; Yang, Z. A Weighted Forward-Backward Spatial Smoothing DOA Estimation Algorithm Based on TLS-ESPRIT. IEICE Trans. Inf. Syst. 2021, 104, 881–884. [Google Scholar] [CrossRef]
- Tsihrintzis, G.A.; Nikias, C.L. Performance of optimum and suboptimum receivers in the presence of impulsive noise modeled as an alpha-stable process. IEEE Trans. Commun 1995, 43, 904–914. [Google Scholar] [CrossRef]
- Huang, F.; Zhang, Y.; Guo, Q.; Tao, J.; Zakharov, Y.; Wang, B. A robust iterative receiver for single carrier underwater acoustic communications under impulsive noise. Appl. Acoust. 2023, 210, 109438. [Google Scholar] [CrossRef]
- Zhang, X.; Ying, W.; Yang, P.; Sun, M. Parameter estimation of underwater impulsive noise with the Class B model. IET Radar Sonar Navig. 2020, 14, 1055–1060. [Google Scholar] [CrossRef]
- Nikias, C.L.; Shao, M. Signal Processing with Alpha-Stable Distributions and Applications; Wiley-Interscience: Hoboken, NJ, USA, 1995. [Google Scholar]
- Lu, L.; Zhao, H.; Champagne, B. Distributed Nonlinear System Identification in alpha-stable Noise. IEEE Signal Process. Lett. 2018, 25, 979–983. [Google Scholar] [CrossRef]
- Rožić, N.; Banelli, P.; Begušić, D.; Radić, J. Multiple-Threshold Estimators for Impulsive Noise Suppression in Multicarrier Communications. IEEE Trans. Signal Process. 2018, 66, 1619–1633. [Google Scholar] [CrossRef]
- Rabie, K.M.; Adebisi, B.; Tonello, A.M.; Nauryzbayev, G. For more energy-efficient dual-hop DF relaying power-line communication systems. IEEE Syst. J. 2017, 12, 2005–2016. [Google Scholar] [CrossRef]
- Saaifan, K.A.; Henkel, W. accepted for publication 1 decision boundary evaluation of optimum and suboptimum detectors in class-a interference. IEEE Trans. Commun. 2017, 61, 197–205. [Google Scholar] [CrossRef]
- Middleton, D. Non-Gaussian noise models in signal processing for telecommunications: New methods an results for class A and class B noise models. IEEE Trans. Inf. Theory 1999, 45, 1129–1149. [Google Scholar] [CrossRef]
- Liu, T.H.; Mendel, J.M. A subspace-based direction finding algorithm using fractional lower order statistics. IEEE Trans. Signal Process 2001, 49, 1605–1613. [Google Scholar]
- Zhang, J.; Qiu, T. The fractional lower order moments based ESPRIT algorithm for noncircular signals in impulsive noise environments. Wirel. Pers. Commun. 2017, 96, 1673–1690. [Google Scholar] [CrossRef]
- Tsakalides, P.; Nikias, C.L. The robust covariation-based MUSIC (ROC-MUSIC) algorithm for bearing estimation in impulsive noise environments. IEEE Trans. Signal Process. 1996, 44, 1623–1633. [Google Scholar] [CrossRef]
- Belkacemi, H.; Marcos, S. Robust subspace-based algorithms for joint angle/Doppler estimation in non-Gaussian clutter. Signal Process. 2007, 87, 1547–1558. [Google Scholar] [CrossRef]
- Shao, M.; Nikias, C.L. Signal processing with fractional lower order moments: Stable processes and their applications. Proc. IEEE 1993, 81, 986–1010. [Google Scholar] [CrossRef]
- Li, H.; Zhang, Y.; Dai, K. Robust Regression Based on Correntropy Induced Metric. In Proceedings of the International Conference on Wireless Communications, Networking and Applications, Wuhan, China, 16–18 December 2022; Springer: Singapore, 2022; pp. 595–603. [Google Scholar]
- Yang, J.; Cao, J.; Xue, A. Robust maximum mixture correntropy criterion-based semi-supervised ELM with variable center. IEEE Trans. Circuits Syst. II Express Briefs 2020, 67, 3572–3576. [Google Scholar] [CrossRef]
- Chen, B.; Xing, L.; Zhao, H.; Du, S.; Principe, J.C. Effects of outliers on the maximum correntropy estimation: A robustness analysis. IEEE Trans. Syst. Man Cybern. Syst. 2019, 51, 4007–4012. [Google Scholar] [CrossRef]
- Ma, W.; Qu, H.; Gui, G.; Xu, L.; Zhao, J.; Chen, B. Maximum correntropy criterion based sparse adaptive filtering algorithms for robust channel estimation under non-Gaussian environments. J. Frankl. Inst. 2015, 352, 2708–2727. [Google Scholar] [CrossRef]
- Wang, B.; Gao, S.; Ge, H.; Wang, W. A Variable Step Size for Maximum Correntropy Criterion Algorithm with Improved Variable Kernel Width. IEEE Trans. Electr. Electron. Eng. 2020, 15, 1465–1474. [Google Scholar] [CrossRef]
- Zhang, J.; Qiu, T.; Song, A.; Tang, H. A novel correntropy based DOA estimation algorithm in impulsive noise environments. Signal Process. 2014, 104, 346–357. [Google Scholar] [CrossRef]
- Luan, S.; Qiu, T.; Zhu, Y.; Yu, L. Cyclic correntropy and its spectrum in frequency estimation in the presence of impulsive noise. Signal Process. 2016, 120, 503–508. [Google Scholar] [CrossRef]
- Chen, J.; Guan, S. Correntropy-based DOA estimation algorithm under impulsive noise environments. EURASIP J. Wirel. Commun. Netw. 2020, 2020, 154. [Google Scholar] [CrossRef]
- Dai, J.; Qiu, T.; Luan, S.; Tian, Q.; Zhang, J. An Improved Toeplitz Approximation Method for Coherent DOA Estimation in Impulsive Noise Environments. Entropy 2023, 25, 960. [Google Scholar] [CrossRef]
- Wang, M.; Feng, D.Z.; Chen, M.H.; Su, T.T.; Zhang, X.J. A robust direction-of-arrival estimation method for impulsive noise environments. Signal Process. 2023, 212, 109175. [Google Scholar] [CrossRef]
- Tian, Q.; Qiu, T.; Ma, J.; Li, J.; Li, R. Robust fractional lower order correntropy algorithm for DOA estimation in impulsive noise environments. IEICE Trans. Commun. 2021, 104, 35–48. [Google Scholar] [CrossRef]
- Chen, B.; Wang, X.; Lu, N.; Wang, S.; Cao, J.; Qin, J. Mixture correntropy for robust learning. Pattern Recognit. 2018, 79, 318–327. [Google Scholar] [CrossRef]
- Cai, R.; Tian, Q.; Luo, Y. DOA estimation based on a deep neural network under impulsive noise. Signal Image Video Process. 2024, 18, 785–792. [Google Scholar] [CrossRef]
- Wang, W.; Zhao, H.; Dogancay, K.; Yu, Y.; Lu, L.; Zheng, Z. Robust Adaptive Filtering Algorithm Based on Maximum Correntropy Criteria for Censored Regression. Signal Process. 2019, 160, 88–98. [Google Scholar] [CrossRef]
- Chen, B.; Principe, J.C. Maximum Correntropy Estimation Is a Smoothed MAP Estimation. IEEE Signal Process. Lett. 2012, 19, 491–494. [Google Scholar] [CrossRef]
- Zeng, W.J.; So, H.C.; Huang, L. p-MUSIC: Robust directionof-arrival estimator for impulsive noise environments. IEEE Trans. Signal Process 2013, 61, 4296–4308. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lan, X.; Hu, J.; Zhang, Y.; Ma, S.; Tian, Y. A Novel DOA Estimation Algorithm Based on Robust Mixed Fractional Lower-Order Correntropy in Impulsive Noise. Electronics 2024, 13, 2386. https://doi.org/10.3390/electronics13122386
Lan X, Hu J, Zhang Y, Ma S, Tian Y. A Novel DOA Estimation Algorithm Based on Robust Mixed Fractional Lower-Order Correntropy in Impulsive Noise. Electronics. 2024; 13(12):2386. https://doi.org/10.3390/electronics13122386
Chicago/Turabian StyleLan, Xiaoyu, Jingyi Hu, Yudi Zhang, Shuang Ma, and Ye Tian. 2024. "A Novel DOA Estimation Algorithm Based on Robust Mixed Fractional Lower-Order Correntropy in Impulsive Noise" Electronics 13, no. 12: 2386. https://doi.org/10.3390/electronics13122386
APA StyleLan, X., Hu, J., Zhang, Y., Ma, S., & Tian, Y. (2024). A Novel DOA Estimation Algorithm Based on Robust Mixed Fractional Lower-Order Correntropy in Impulsive Noise. Electronics, 13(12), 2386. https://doi.org/10.3390/electronics13122386






