A Physical-Layer Watermarking Scheme Based on 5G NR
Abstract
1. Introduction
- (1)
- Channel coding is introduced into the scheme of watermark generation, which can reduce the bit error when the watermark is transmitted in the channel and improve the success rate of authentication. The receiver can use channel decoding to realize error detection and correction of the watermark signal so that even if a few watermark symbols are transmitted incorrectly, identity authentication can still be corrected.
- (2)
- The pseudo-user-bit is proposed and used as the parameter for watermark generation. To avoid a third party other than the legitimate communication parties stealing watermark information, it is necessary to ensure that different watermark signals are embedded for different data frames. The usual method is to hash the user bits contained in the data frame with the key information shared between the legitimate communication parties. The problem is that when the receiver makes an error in demodulating the user’s signal, the watermark signal generated by the receiver must be inconsistent with the watermark signal generated by the sender. To reduce the influence of user’s signal demodulation error on watermark authentication, the pseudo-user-bit is proposed, and it is used as the parameter of hash encryption instead of the user bit.
- (3)
- The watermark signal is embedded into the phase of DMRS, which can avoid the interference of the watermark signal in the demodulation of the user’s signal so that the embedding of the watermark does not affect the SNR of the user’s signal. Note that DMRS will be used to estimate the channel conditions, so the watermark should not affect the channel estimation. In this paper, we call this important condition the symmetry of the watermark and improve the channel coding algorithm to achieve the symmetry of the watermark.
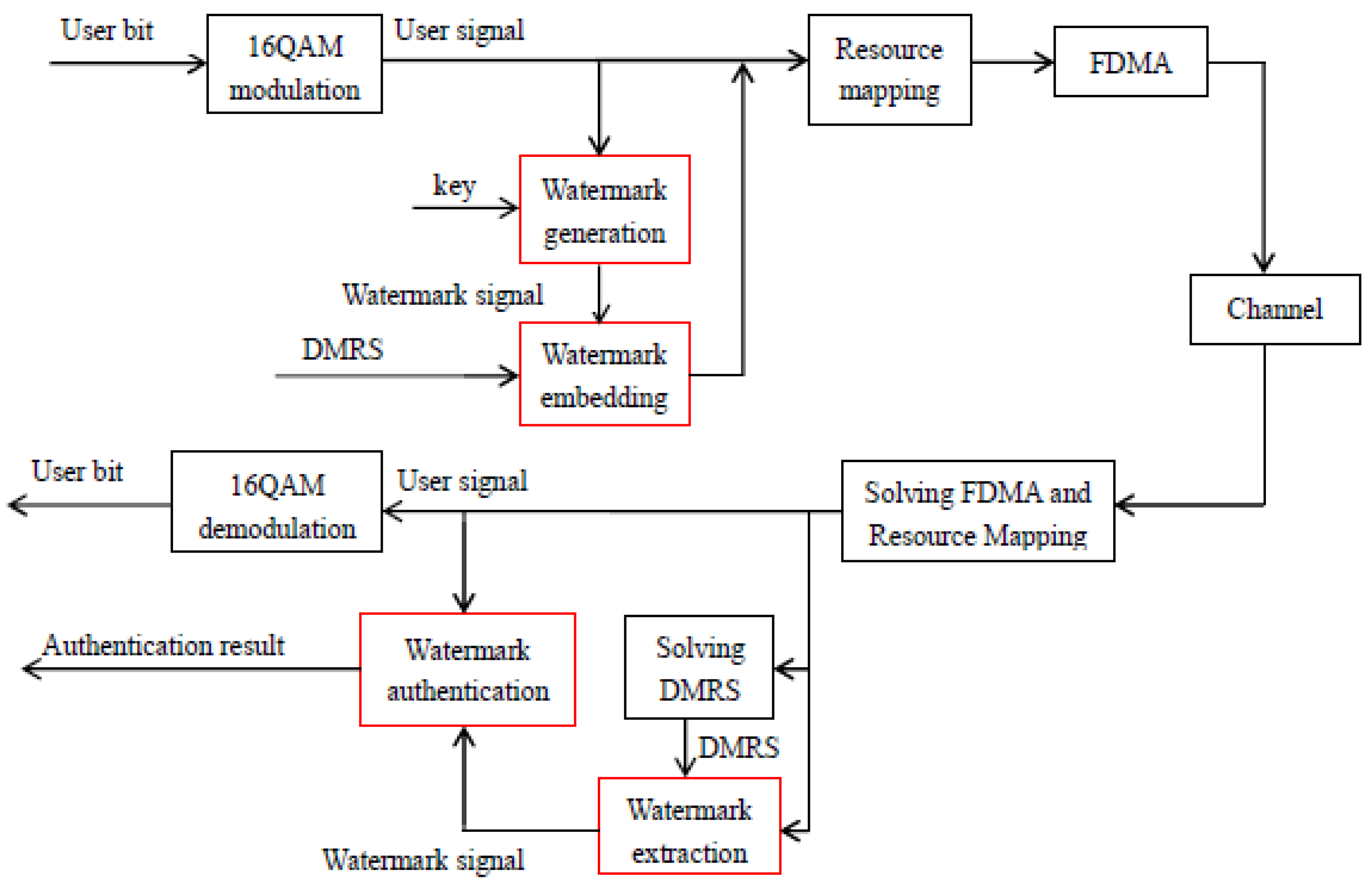
2. Watermark System Model on 5G NR
2.1. Basic Watermarking Scheme
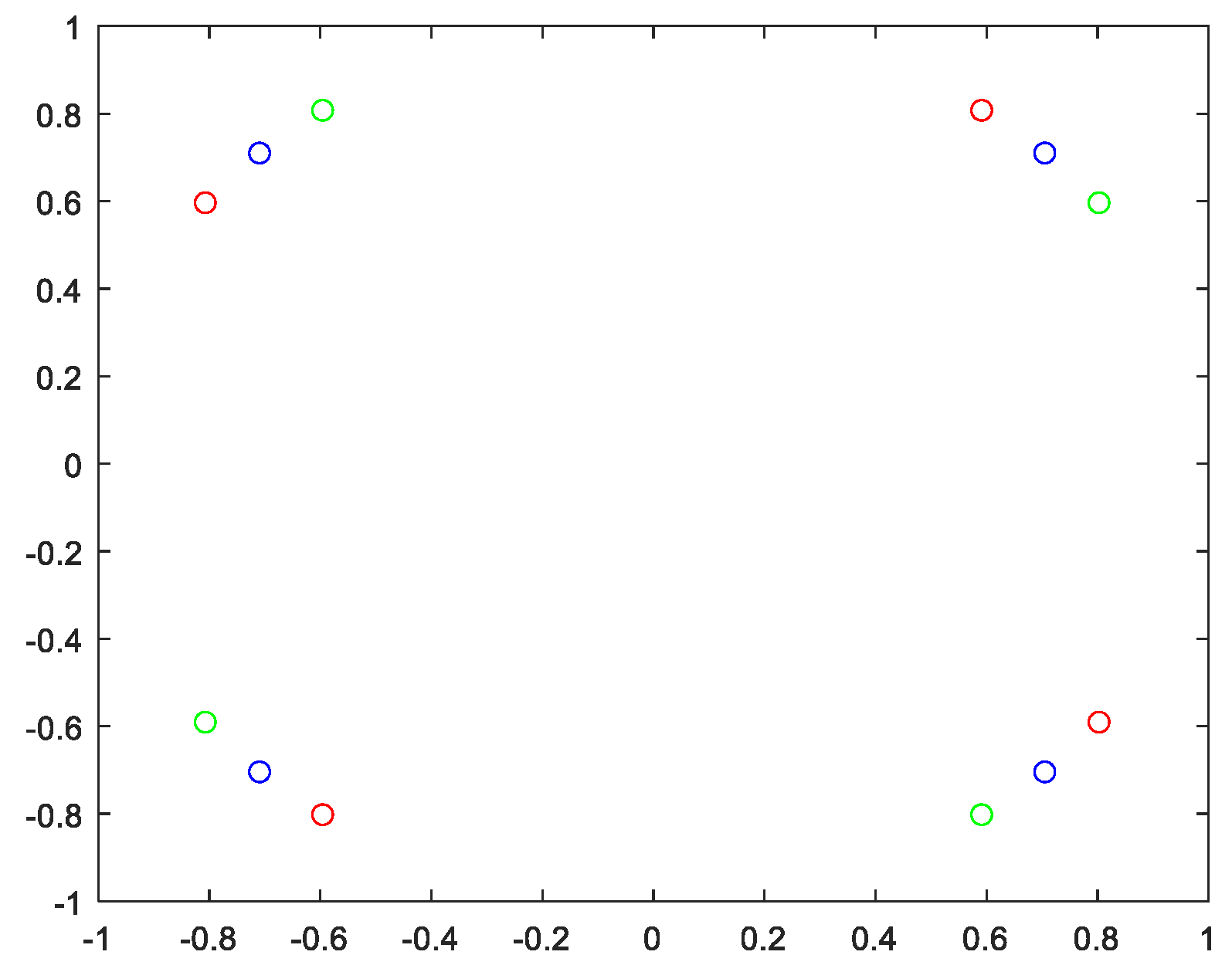
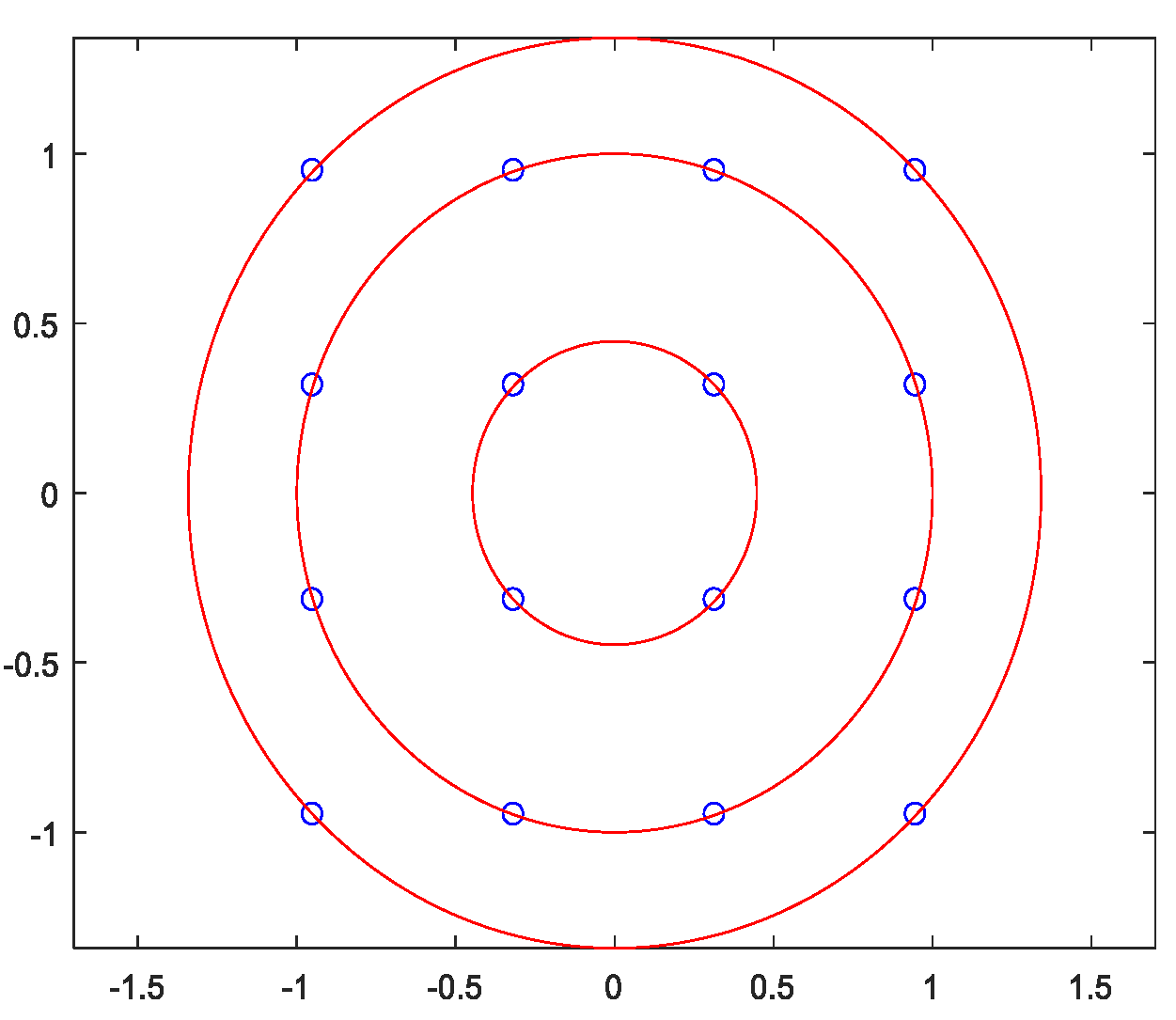
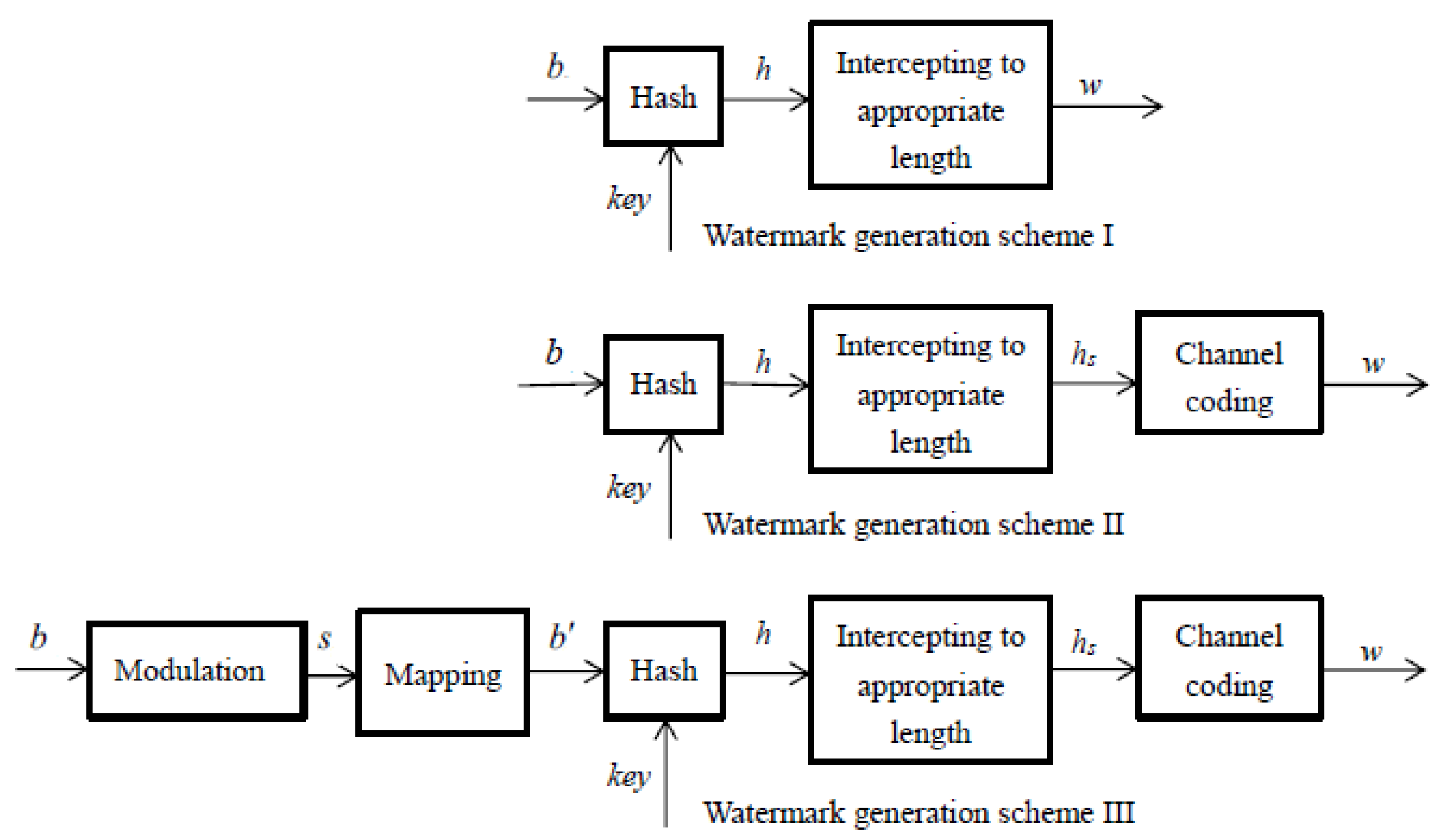
2.1.1. Watermark Generation Scheme
2.1.2. Watermark Embedding Scheme
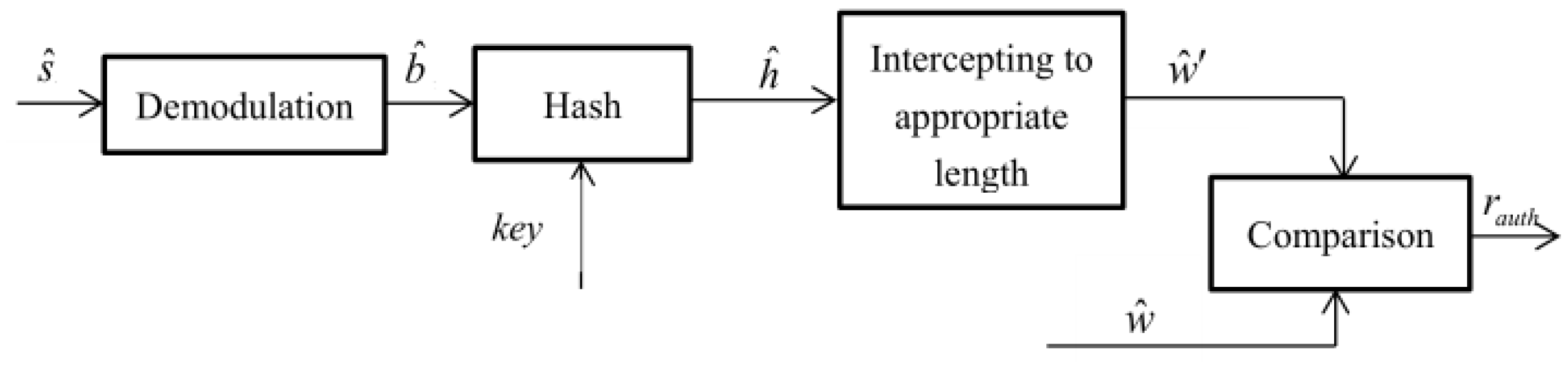
2.1.3. Watermark Extraction and Authentication Scheme
2.2. Improved Watermarking Scheme
2.2.1. Improved Watermark Generation Scheme
2.2.2. Improved Watermark Embedding Scheme
2.2.3. Improved Watermark Embedding Scheme
2.3. Performance Analysis for Bit Error Rate
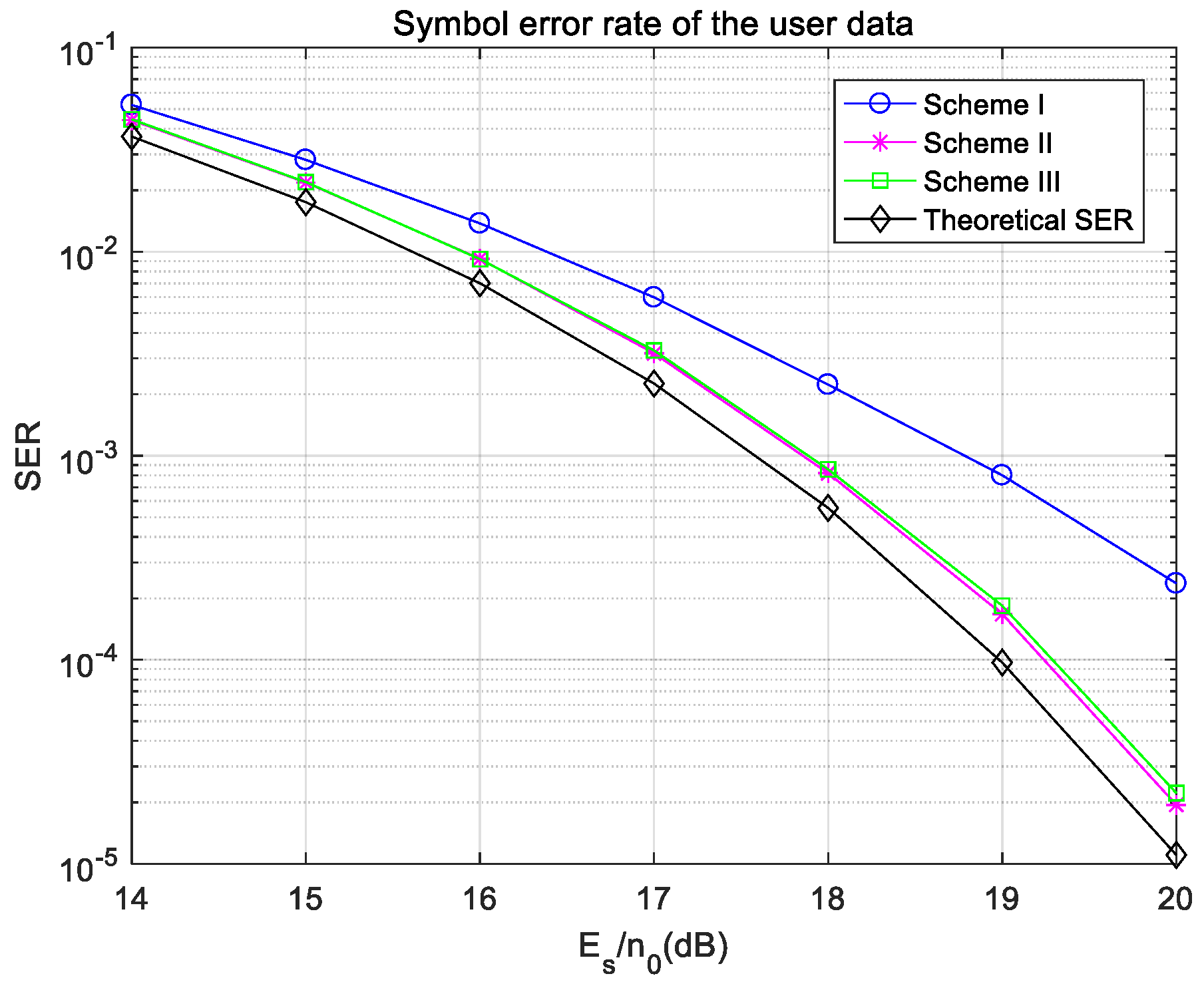
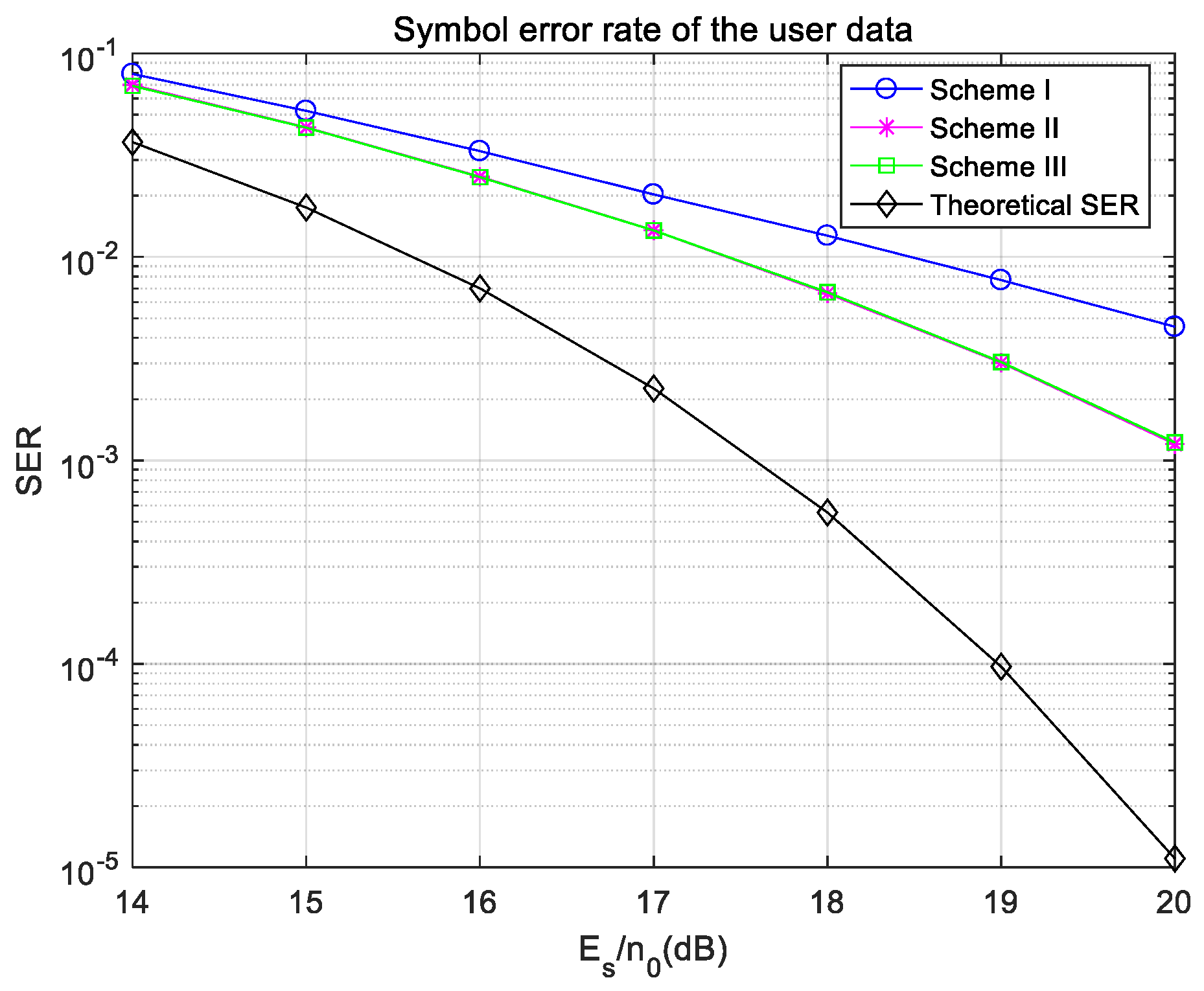
2.3.1. Symbol Error Rate of the User’s Symbol
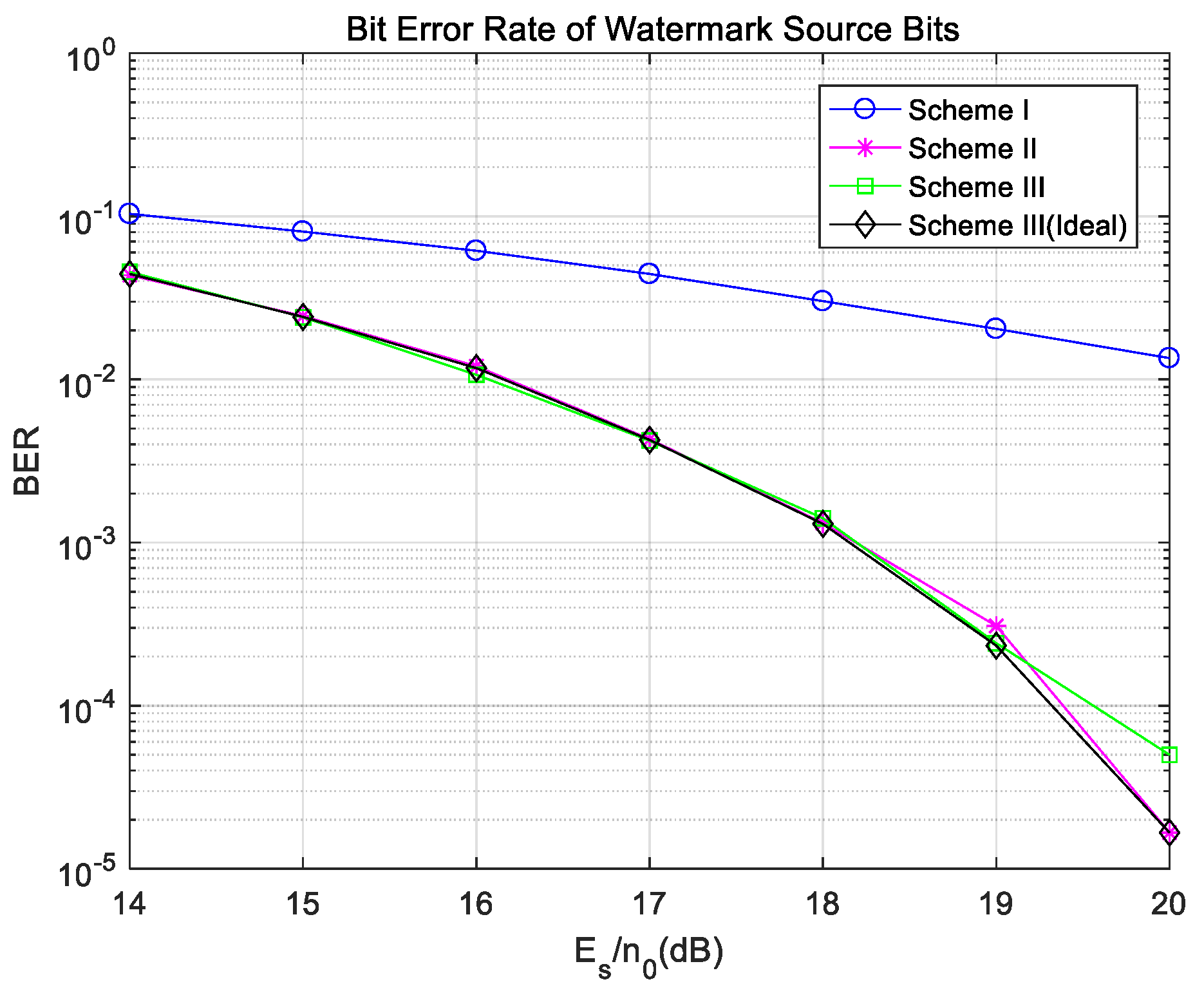
2.3.2. Bit Error Rate of the Watermark Bit
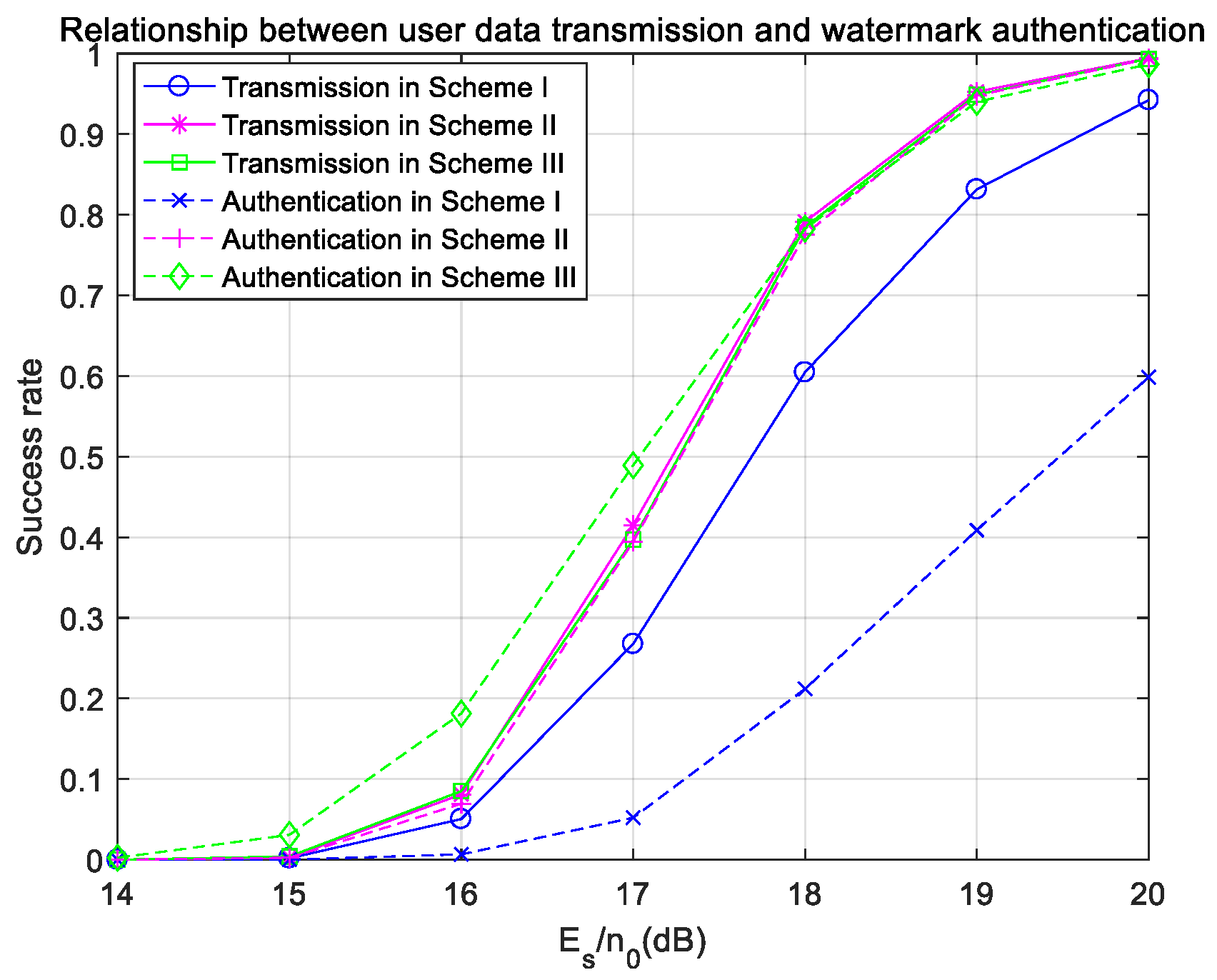
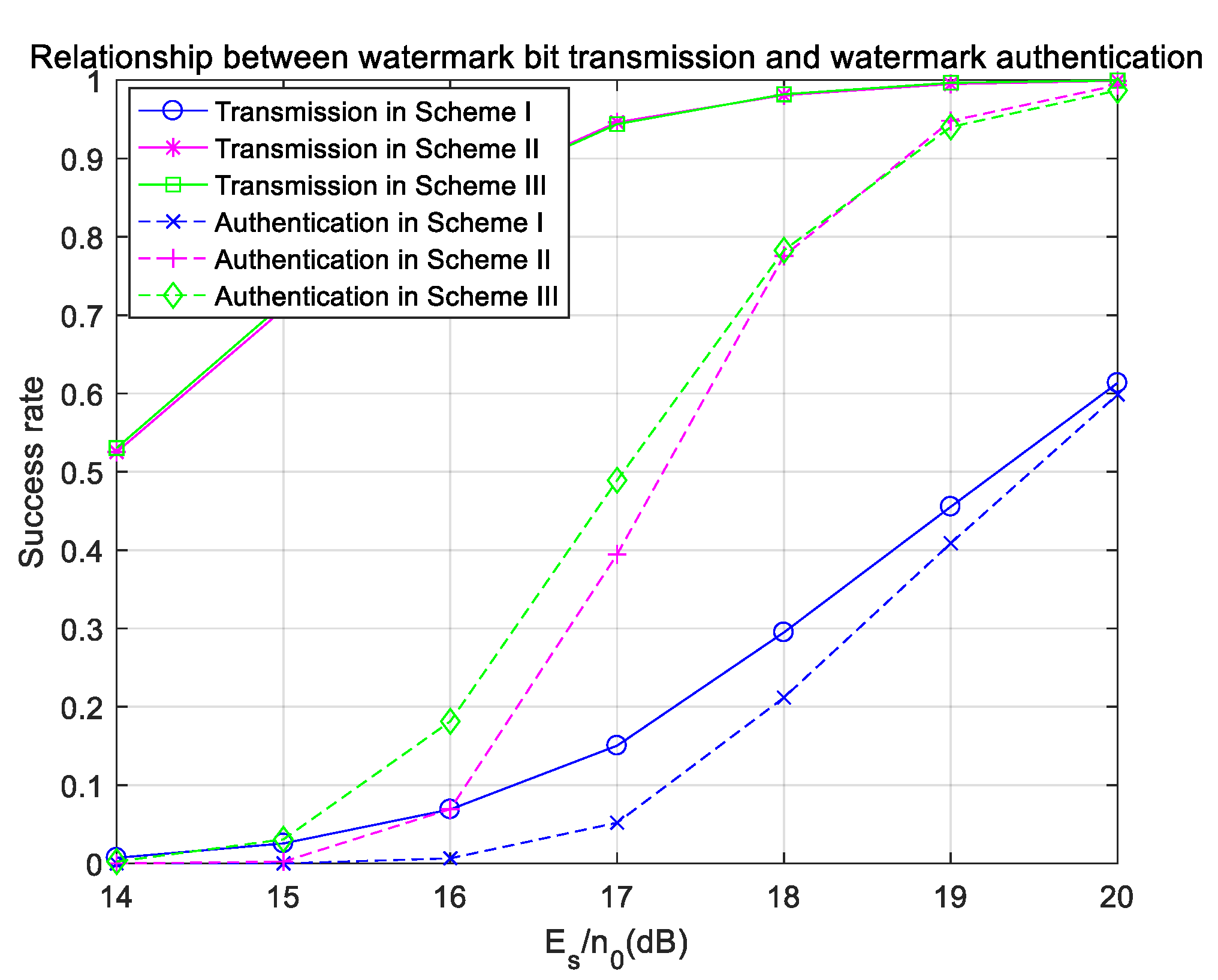
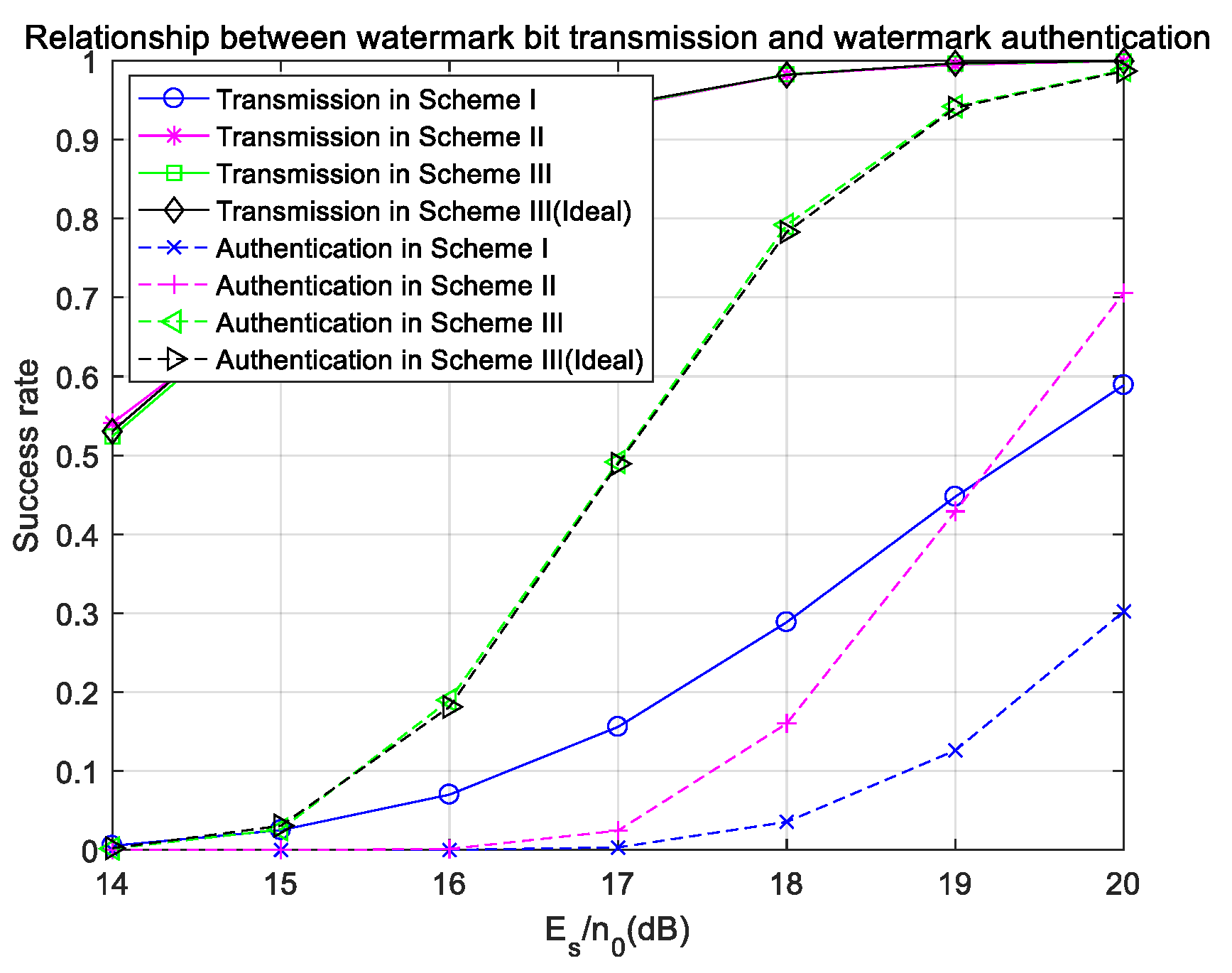
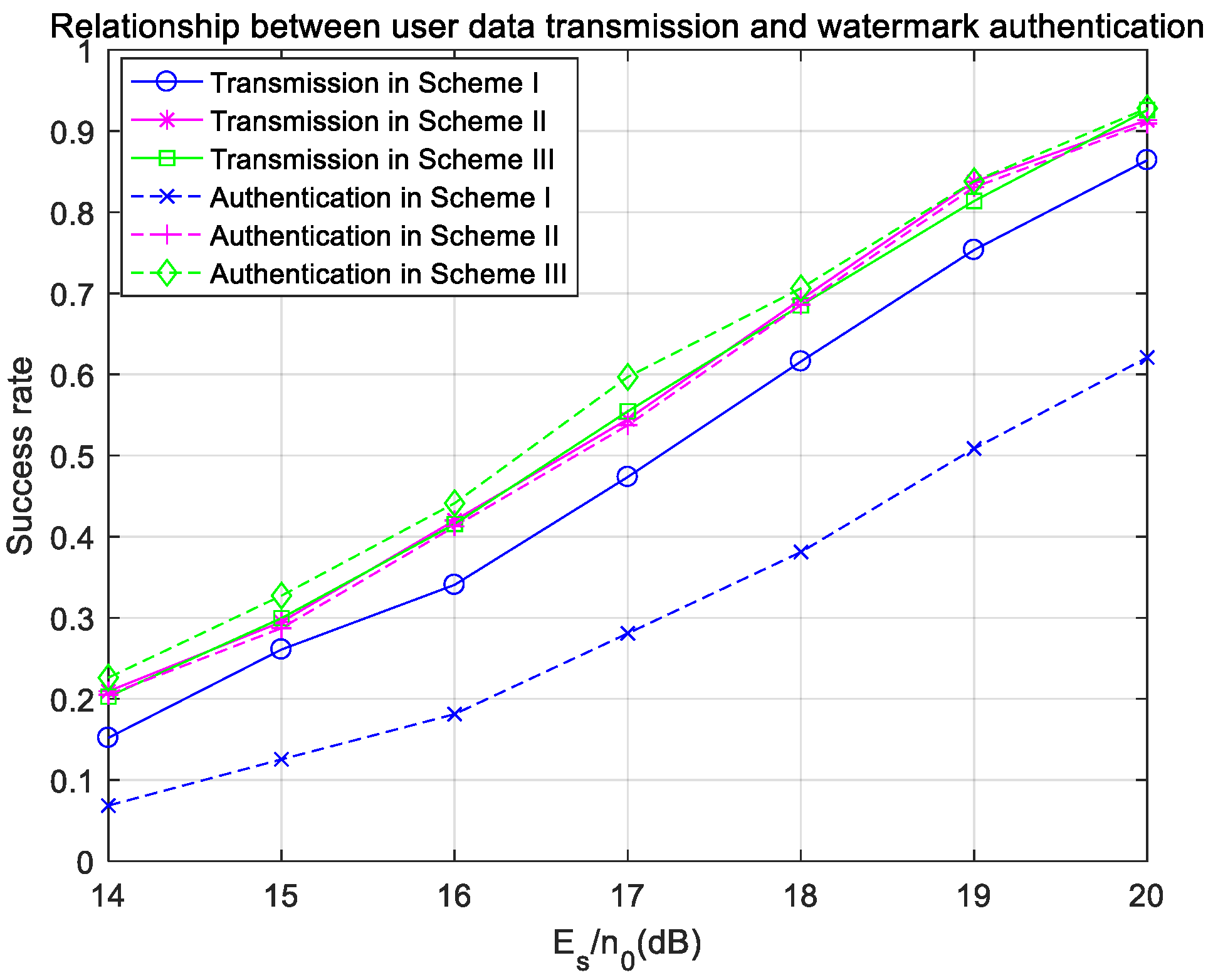
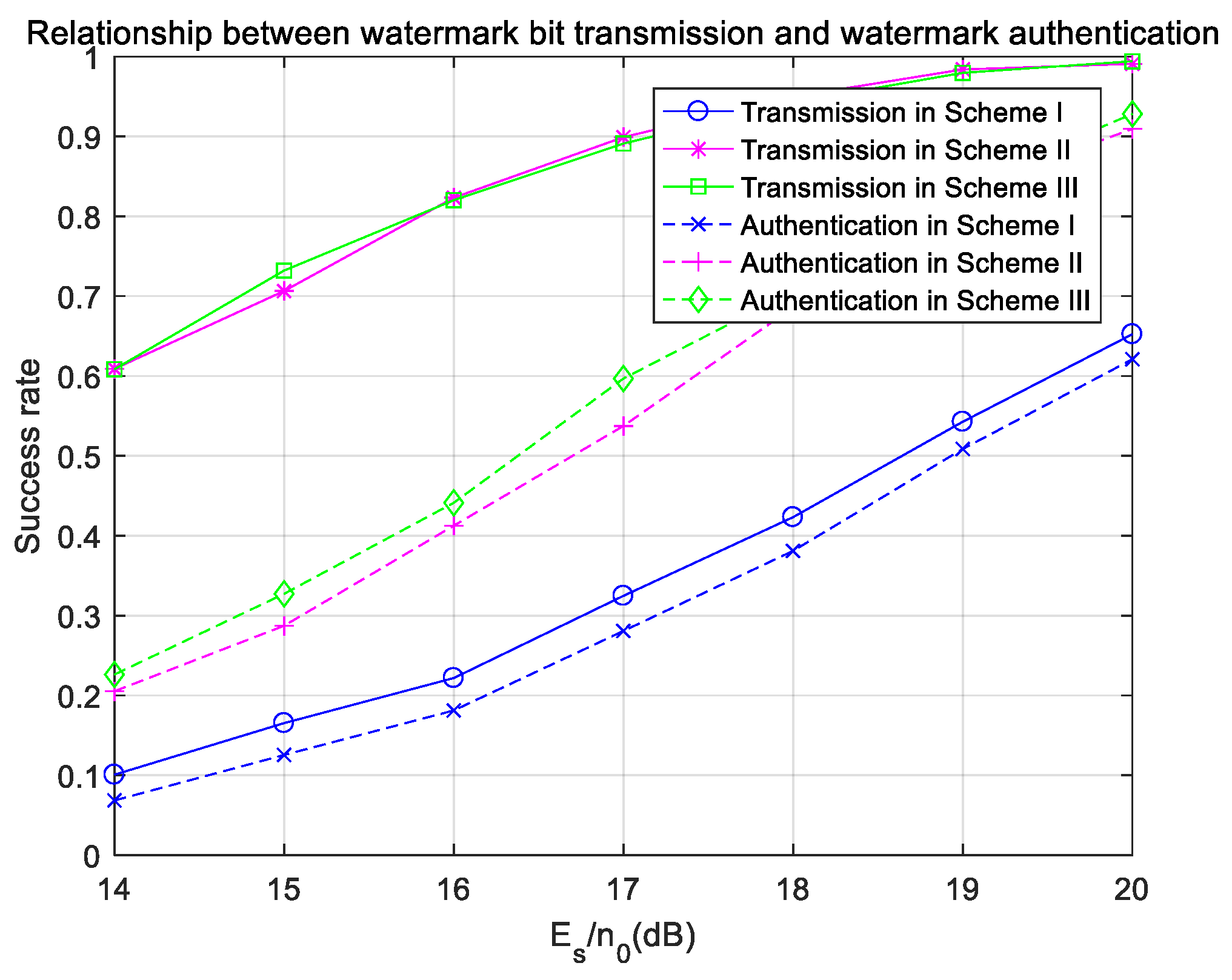
3. System Performance Simulation
3.1. System Performance in the Gaussian Channel
3.1.1. System Performance under Ideal Carrier Synchronization
3.1.2. System Performance with Carrier Frequency Offset
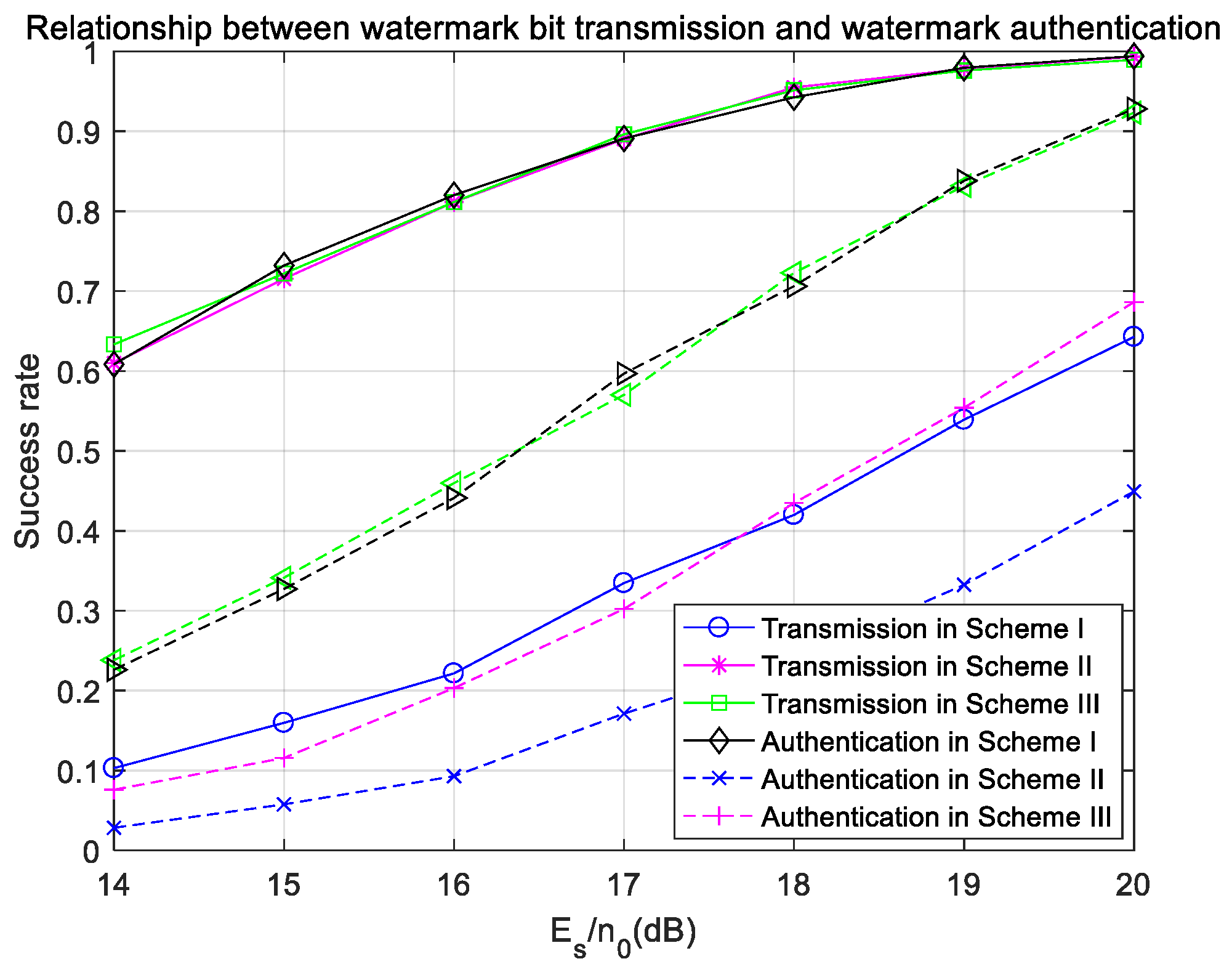
3.2. System Performance in the Fading Channel
3.2.1. System Performance under Ideal Carrier Synchronization
3.2.2. System Performance in the Presence of Carrier Frequency Offset
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Mathur, S.; Reznik, A.; Ye, C.; Mukherjee, R.; Rahman, A.; Shah, Y.; Trappe, W.; Mandayam, N. Exploiting the physical layer for enhanced security. IEEE Wirel. Commun. 2010, 17, 63–70. [Google Scholar] [CrossRef]
- Shin, M.; Ma, J.; Mishra, A.; Arbaugh, W.A. Wireless network security and interworking. Proc. IEEE 2006, 94, 455–466. [Google Scholar] [CrossRef]
- Potlapally, N.R.; Ravi, S.; Raghunathan, A.; Jha, N.K. A study of the energy consumption characteristics of cryptographic algorithms and security protocols. IEEE Trans. Mob. Comput. 2006, 5, 128–143. [Google Scholar] [CrossRef]
- Brik, V.; Banerjee, S.; Gruteser, M.; Oh, S. Wireless device identification with radiometric signatures. In Proceedings of the 14th ACM International Conference on Mobile Computing and Networking, Francisco, CA, USA, 14–19 September 2008; pp. 116–127. [Google Scholar]
- Wu, X.; Yang, Z. Physical-Layer Authentication for Multi-Carrier Transmission. IEEE Commun. Lett. 2015, 19, 74–77. [Google Scholar] [CrossRef]
- Yu, P.L.; Verma, G.; Sadler, B.M. Wireless physical layer authentication via fingerprint embedding. IEEE Commun. Mag. 2015, 53, 48–53. [Google Scholar] [CrossRef]
- Yu, P.L.; Baras, J.S.; Sadler, B.M. Physical-Layer Authentication. IEEE Trans. Inf. Forensics Secur. 2008, 3, 38–51. [Google Scholar] [CrossRef]
- Yu, P.L.; Baras, J.S.; Sadler, B.M. Multicarrier authentication at the physical layer. In Proceedings of the International Symposium on a World of Wireless, Mobile and Multimedia Networks, Newport Beach, CA, USA, 23–26 June 2008; pp. 1–6. [Google Scholar]
- Yu, P.L.; Sadler, B.M. MIMO Authentication via Deliberate Fingerprinting at the Physical Layer. IEEE Trans. Inf. Forensics Secur. 2011, 6, 606–615. [Google Scholar] [CrossRef]
- Tan, X.; Borle, K.; Du, W.; Chen, B. Cryptographic link signatures for spectrum usage authentication in cognitive radio. In Proceedings of the ACM Conference on Wireless Network Security, Hamburg, Germany, 14–17 June 2011; pp. 79–90. [Google Scholar]
- Jiang, T.; Zeng, H.; Yan, Q.; Lou, W.; Hou, Y.T. On the limitation of embedding cryptographic signature for primary transmitter authentication. IEEE Wirel. Commun. Lett. 2012, 1, 324–327. [Google Scholar] [CrossRef]
- Verma, G.; Yu, P.; Sadler, B.M. Physical layer authentication via fingerprint embedding using software-defined radios. IEEE Access 2015, 3, 81–88. [Google Scholar] [CrossRef]
- Zhang, P.; Liu, J.; Shen, Y.; Li, H.; Jiang, X. Lightweight tag-based PHY-layer authentication for IoT devices in smart cities. IEEE Internet Things J. 2020, 7, 3977–3990. [Google Scholar] [CrossRef]
- Ran, Y.; Al-Shwaily, H.; Tang, C.; Tian, G.Y.; Johnston, M. Physical layer authentication scheme with channel based tag padding sequence. IET Commun. 2019, 13, 1776–1780. [Google Scholar] [CrossRef]
- Xie, N.; Chen, Y. Pilot-Based Physical-Layer Authentication with High Covertness. IEEE Wirel. Commun. 2020, 28, 97–103. [Google Scholar] [CrossRef]
- Xie, N.; Li, Z.; Tan, H. A Survey of Physical-Layer Authentication in Wireless Communications. IEEE Commun. Surv. Tutor. 2021, 23, 282–310. [Google Scholar] [CrossRef]
- 3GPP TS 36.211 v11.0.0; LTE Physical Channels and Modulation. European Telecommunications Standards Institute: Sophia Antipolis, France, 2012.
- 3GPP TS 38.211 v16.3.0; NR Physical Channels and Modulation. European Telecommunications Standards Institute: Sophia Antipolis, France, 2020.
- Meneze, A.J.; Oorschot, P.C.; Vanstone, S.A. Handbook of Applied Cryptography; CRC Press: Boca Raton, FL, USA, 2001. [Google Scholar]
- Dang, Q.H. The Keyed-Hash Message Authentication Code (HMAC): FIPS Pub 1981; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2008. [Google Scholar]
- Hou, X.; Zhang, Z.; Kayama, H. DMRS Design and Channel Estimation for LTE-Advanced MIMO Uplink. In Proceedings of the 70th IEEE Vehicular Technology Conference Fall, Anchorage, AK, USA, 20–23 September 2009; pp. 20–23. [Google Scholar]
- Prange, E. The use of information sets in decoding cyclic codes. IRE Trans. Inf. Theory 1962, 8, 5–9. [Google Scholar] [CrossRef]
- Nylund, H.W. Characteristics of small-area signal fading on mobile circuits in the 150 MHz band. IEEE Trans. Veh. Technol. 1968, 17, 24–30. [Google Scholar] [CrossRef]
- Xu, Z.; Yuan, W. Watermark BER and Channel Capacity Analysis for QPSK-Based RF Watermarking by Constellation Dithering in AWGN Channel. IEEE Signal Process. Lett. 2017, 24, 1068–1072. [Google Scholar] [CrossRef]







| Information Code | Channel Code | Information Code | Channel Code |
|---|---|---|---|
| 0000 | 00010111 | 1000 | 10001011 |
| 0001 | 00010111 | 1001 | 10011100 |
| 0010 | 00101101 | 1010 | 10100110 |
| 0011 | 00111010 | 1011 | 10110001 |
| 0100 | 01001110 | 1100 | 11000101 |
| 0101 | 01011001 | 1101 | 11010010 |
| 0110 | 01100011 | 1110 | 11101000 |
| 0111 | 01110100 | 1111 | 11101000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, X.; Chen, W.; Xu, Z. A Physical-Layer Watermarking Scheme Based on 5G NR. Electronics 2022, 11, 3184. https://doi.org/10.3390/electronics11193184
Xie X, Chen W, Xu Z. A Physical-Layer Watermarking Scheme Based on 5G NR. Electronics. 2022; 11(19):3184. https://doi.org/10.3390/electronics11193184
Chicago/Turabian StyleXie, Xu, Wan Chen, and Zhengguang Xu. 2022. "A Physical-Layer Watermarking Scheme Based on 5G NR" Electronics 11, no. 19: 3184. https://doi.org/10.3390/electronics11193184
APA StyleXie, X., Chen, W., & Xu, Z. (2022). A Physical-Layer Watermarking Scheme Based on 5G NR. Electronics, 11(19), 3184. https://doi.org/10.3390/electronics11193184






