A New Approach to Compensator Design Based on Multi-Loop Technique and Scalable Forward Model Complexity
Abstract
:1. Introduction
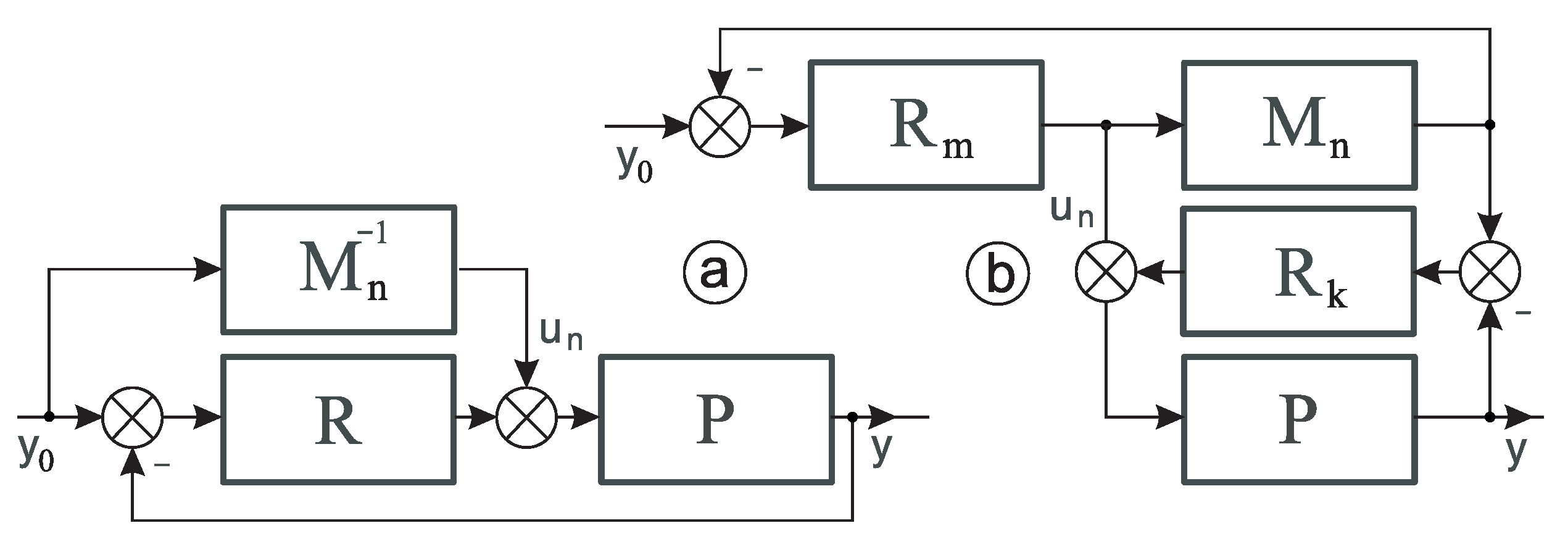
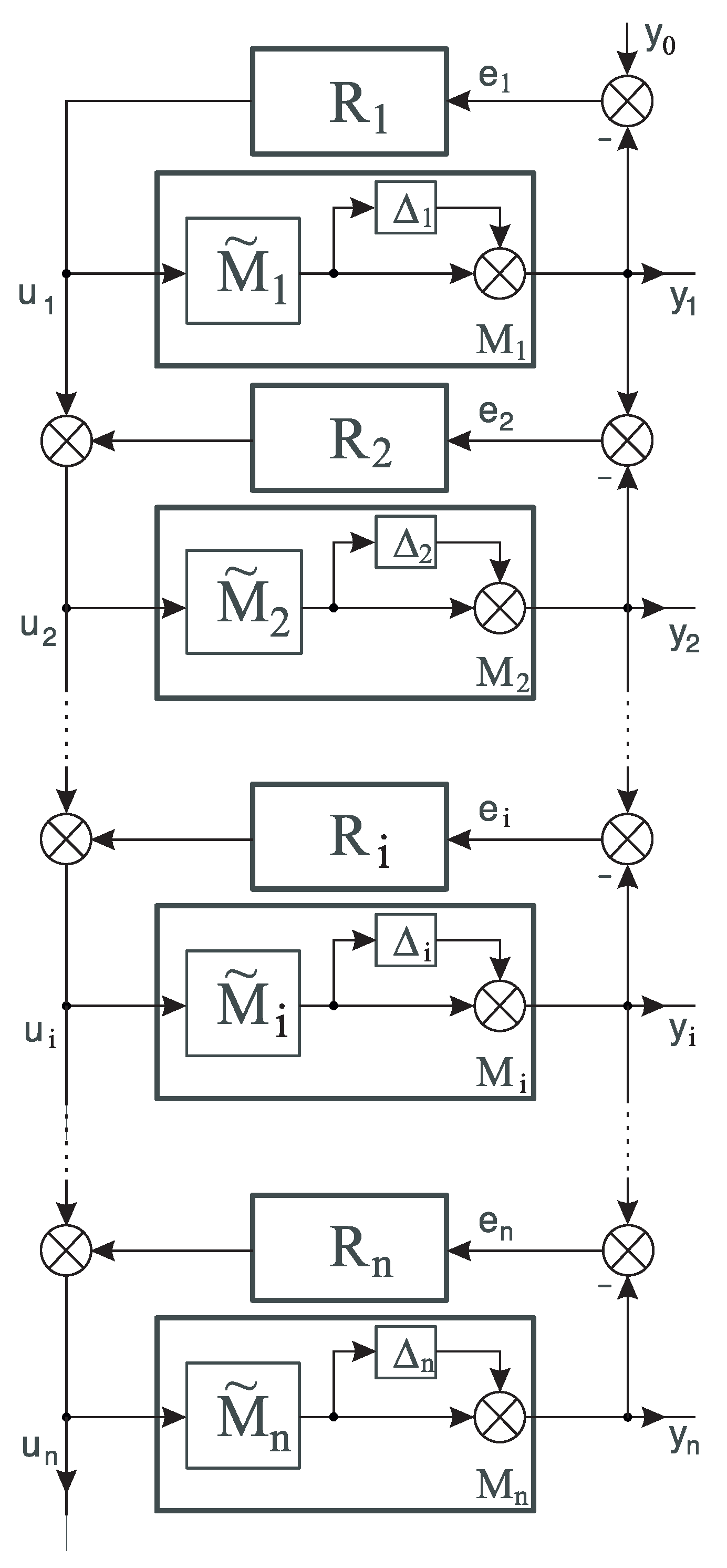
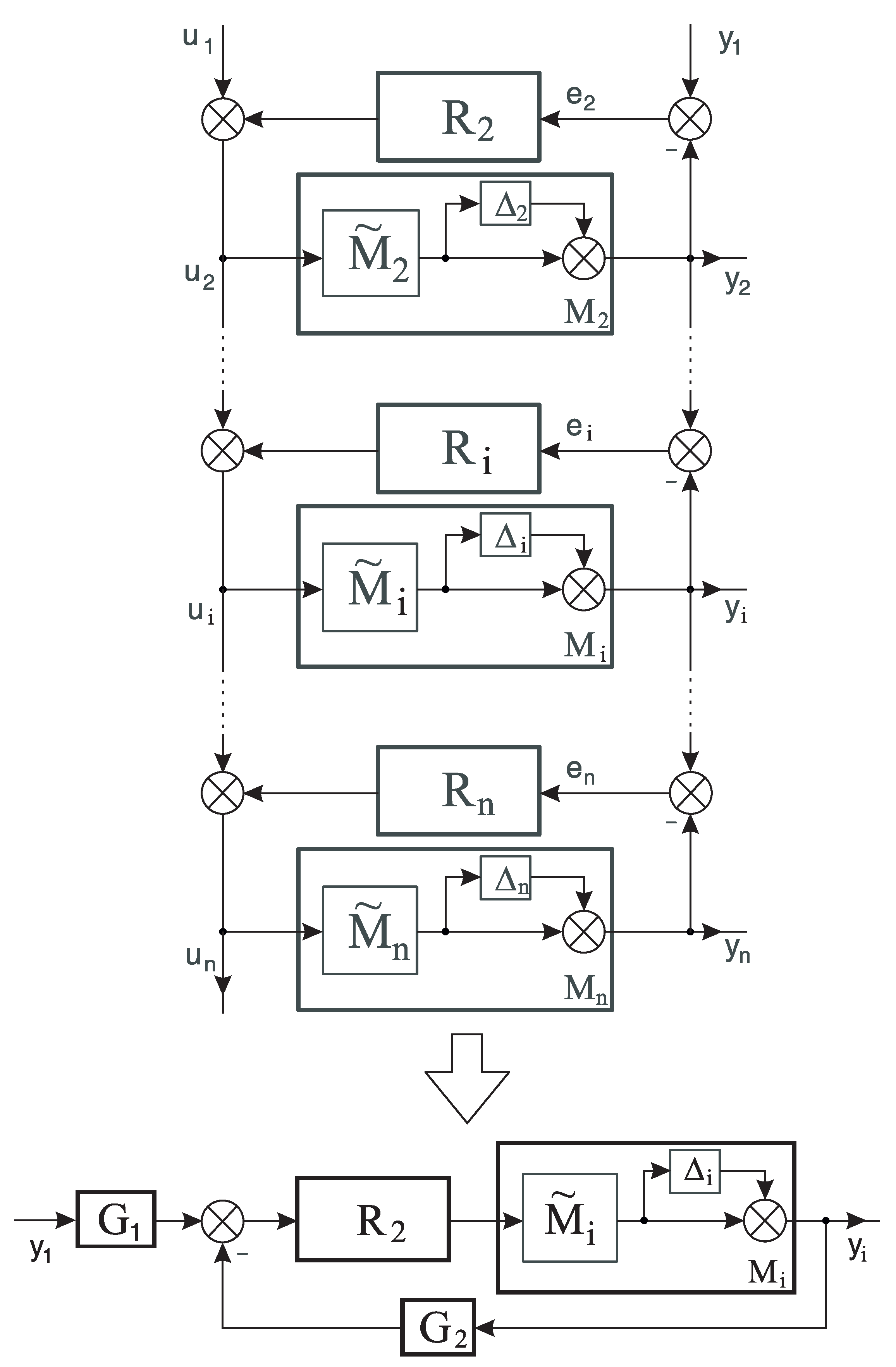
2. Multi-Loop Approach
2.1. Stability
2.2. Robustness Analysis
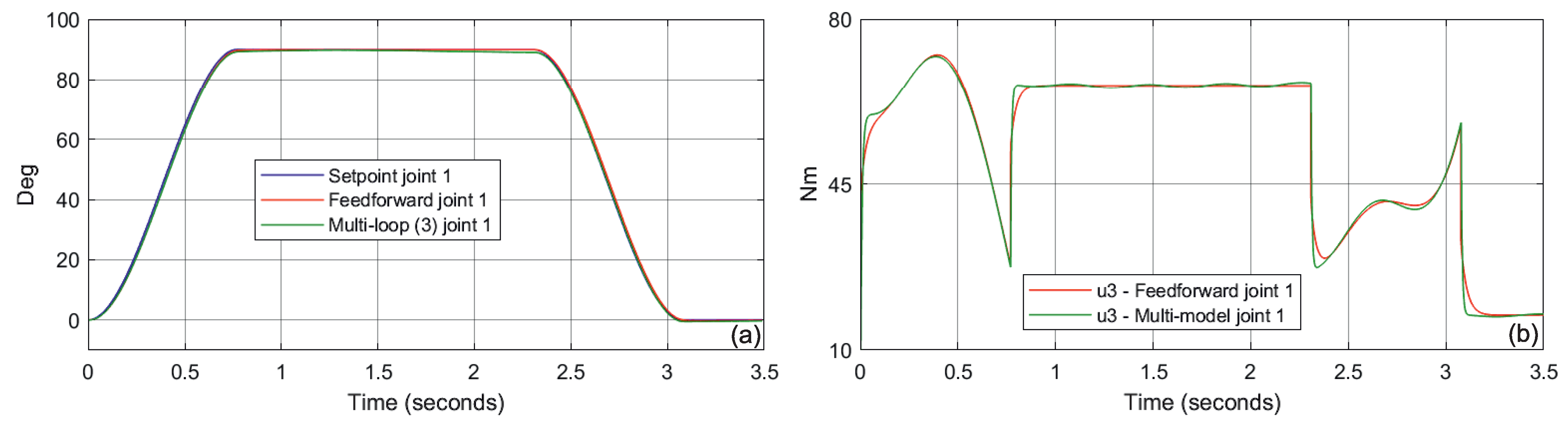
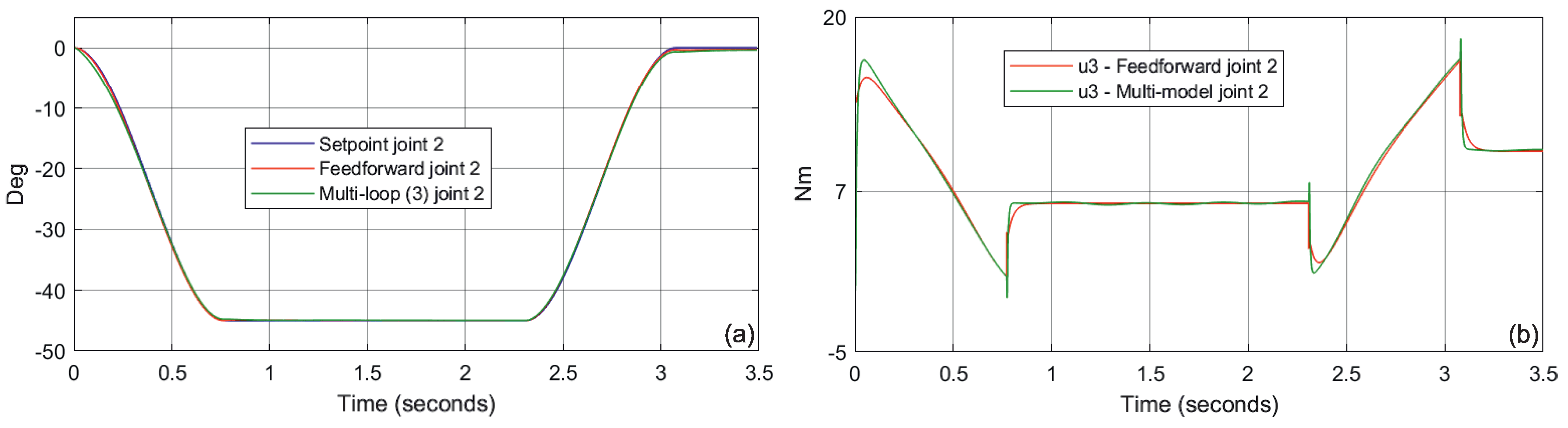
3. Results
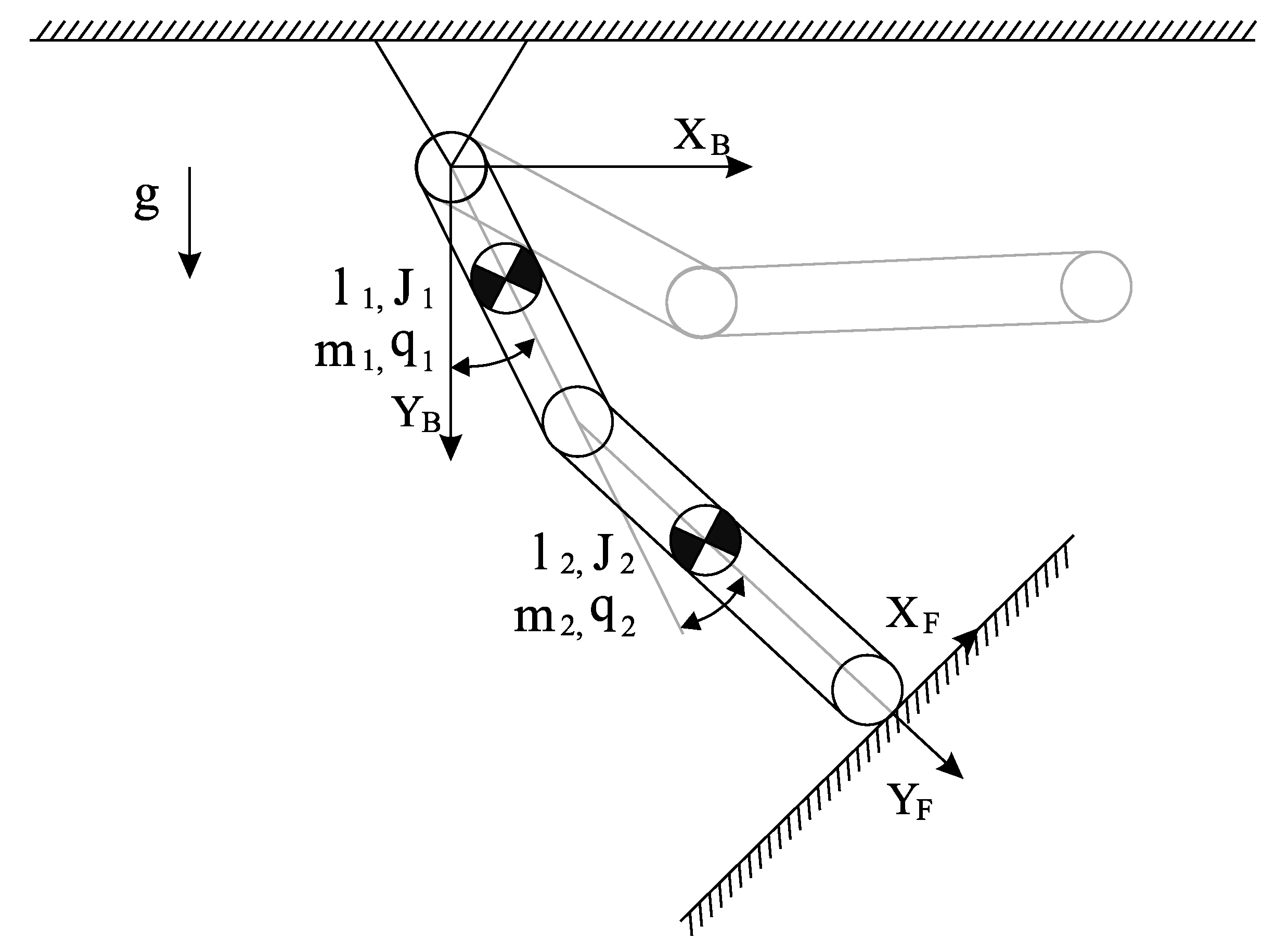
3.1. Preparation of Non-Linear Process Models
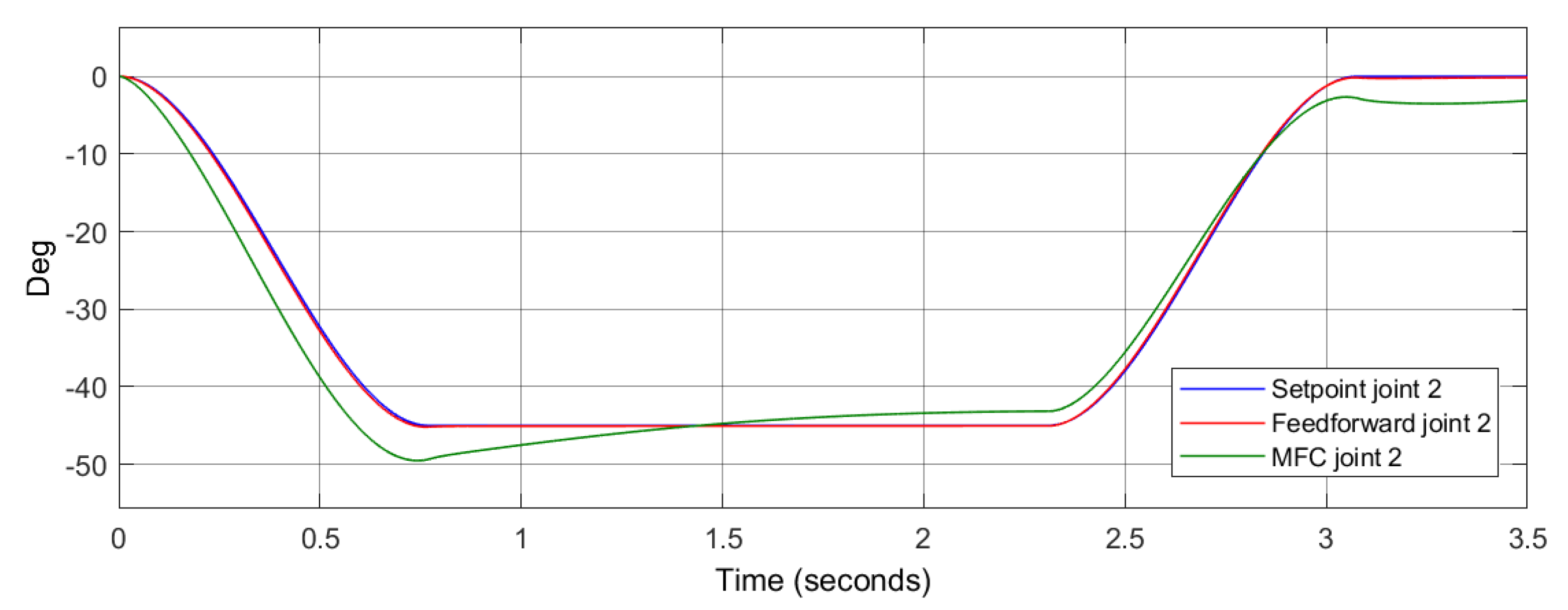
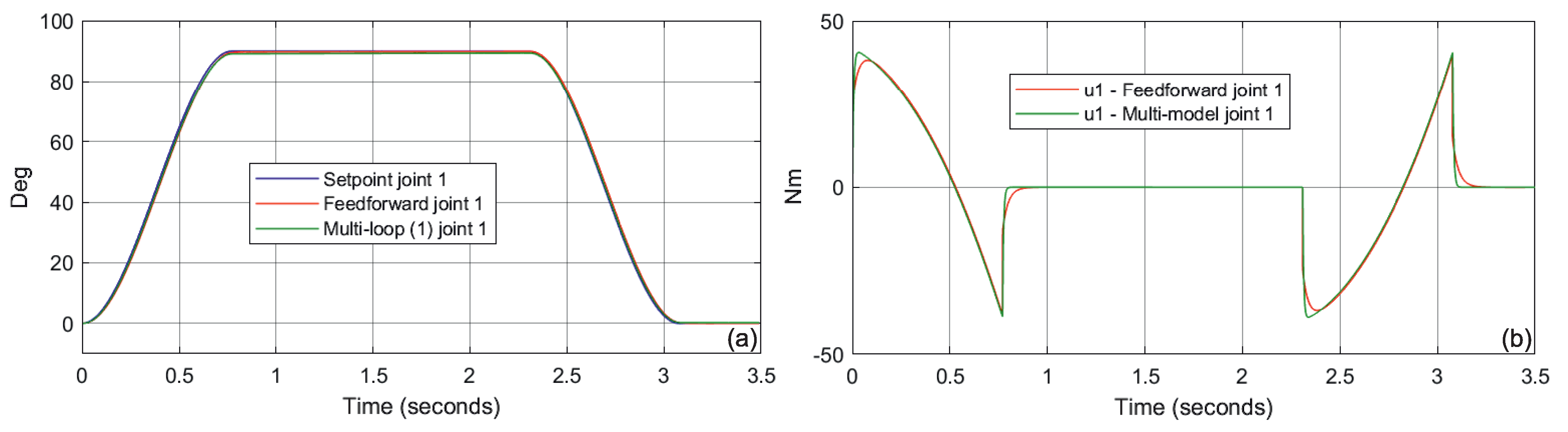
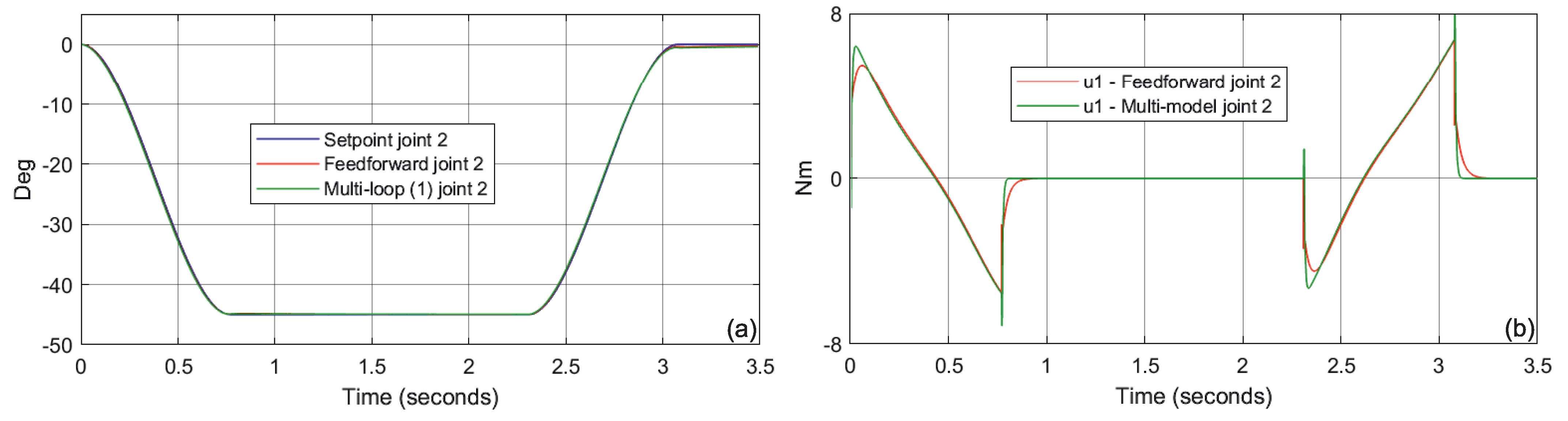
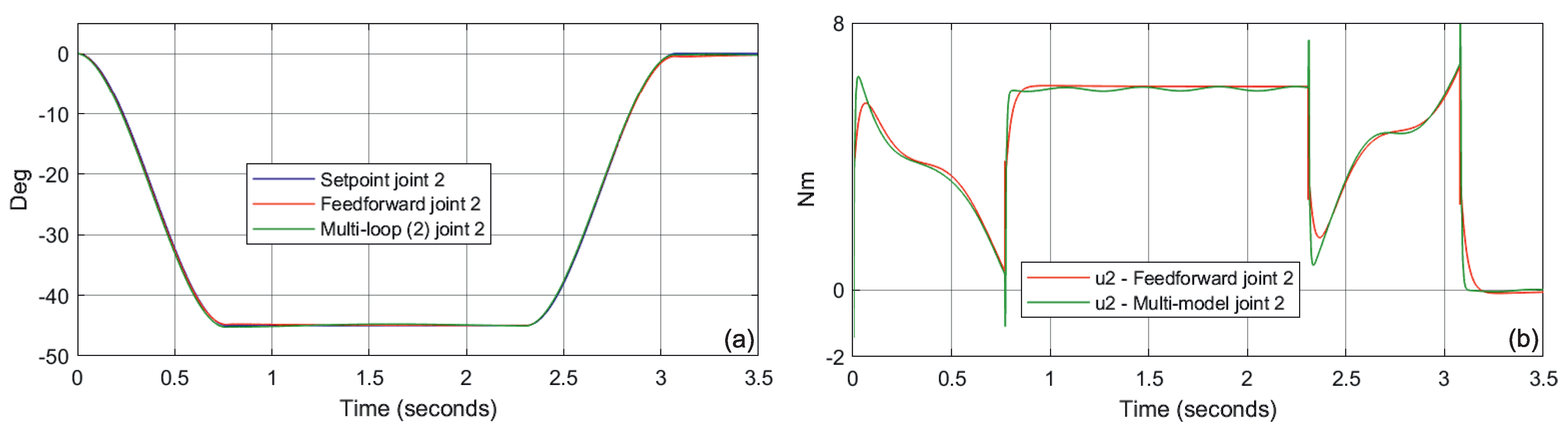
3.2. Simulation Study
4. Conclusions
Funding
Conflicts of Interest
Abbreviations
| DOF | Degree of Freedom |
| EDDA | Experimental Direct Drive Arm |
| EL | Euler–Lagrange Systems |
| IMC | Internal Model Control |
| MBC | Model-Based Control |
| MFC | Model-Following Control |
| PID | Proportional Integral Derivative |
References
- Agachi, P.S.; Nagy, Z.K.; Cristea, M.V.; Imre-Lucaci, Á. Model Based Control: Case Studies in Process Engineering; Wiley-VCH Verlag GmbH & Co. KGaA: Hoboken, NJ, USA, 2006. [Google Scholar]
- Francis, B.A.; Wonham, W.M. The internal model principle of control theory. Automatica 1976, 10, 457–465. [Google Scholar] [CrossRef]
- Shao, L.; Liu, C.; Wang, Z.; Wang, J.; Yang, X. The Temperature Control of Blackbody Radiation Source Based on IMC-PID. In Proceedings of the Conference on Mechatronics and Automation, Tianjin, China, 4–7 August 2019; pp. 1698–1702. [Google Scholar]
- Arya, P.P.; Chakrabarty, S. Robust internal model controller with increased closed-loop bandwidth for process control systems. IET Control Theory Appl. 2020, 14, 2134–2146. [Google Scholar] [CrossRef]
- Li, P.; Zhu, G.; Zhang, M. Linear Active Disturbance Rejection Control for Servo Motor Systems With Input Delay via Internal Model Control Rules. IEEE Trans. Ind. Electron. 2021, 68, 1077–1086. [Google Scholar] [CrossRef]
- Alfaro, V.M.; Vilanova, R.; Arrieta, O. Analytical robust tuning of PI controllers for first-order-plus-dead-time processes. In Proceedings of the Conference on Emerging Technologies & Factory Automation, Hamburg, Germany, 15–18 September 2008; pp. 273–280. [Google Scholar]
- Åström, K.J.; Hang, C.C.; Lim, B.C. A new Smith predictor for controlling a process with an integrator and long dead-time. IEEE Trans. Autom. Control. 1994, 39, 343–345. [Google Scholar] [CrossRef] [Green Version]
- Zhu, Q.; Yin, Z.; Zhang, Y.; Niu, J.; Li, Y.; Zhong, Y. Research on Two-Degree-of-Freedom Internal Model Control Strategy for Induction Motor Based on Immune Algorithm. IEEE Trans. Ind. Electron. 2016, 63, 1981–1992. [Google Scholar] [CrossRef]
- Cui, J.; Wang, Z.; Chen, Y.; Liu, T. Indirect iterative learning control design based on 2DOF IMC for batch processes with input delay. In Proceedings of the Conferen Chinese Control Conference, Dalian, China, 26–28 July 2017; pp. 3587–3592. [Google Scholar]
- Mikulas, H.; Bistak, B.; Vrancic, D. 2DOF IMC and Smith-Predictor-Based Control for Stabilised Unstable First Order Time Delayed Plants. Mathematics 2021, 9, 1064. [Google Scholar]
- Siciliano, B.; Khatib, O. Springer Handbook of Robotics; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Mahmoud, M.S.; Xia, Y. Applied Control Systems Design; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Roffel, B.; Betlem, B.H. Advanced Practical Process Control; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Commercial Modelica Simulation Environments. Available online: https://www.modelica.org/tools (accessed on 9 September 2021).
- Looye, G.; Thuemmel, M.; Kurze, M.; Otter, M.; Bals, J. Nonlinear Inverse Models for Control. In Proceedings of the Conference International Modelica Conference, Hamburg, Germany, 7–8 March 2005; pp. 267–279. [Google Scholar]
- Li, G.; Tsang, K.M.; Ho, S.L. A novel model following scheme with simple structure for electrical position servo systems. Int. J. Syst. Sci. 1998, 29, 959–969. [Google Scholar] [CrossRef]
- Osypiuk, R. Simple robust control structures based on the model-following concept—A theoretical analysis. Int. J. Robust Nonlinear Control 2010, 20, 1920–1929. [Google Scholar] [CrossRef]
- Shibasaki, H.; Yusof, R.; Ishida, Y. A Design Method of a Model-following Control System. Int. J. Control Autom. Syst. 2015, 13, 843–852. [Google Scholar] [CrossRef]
- Skoczowski, S. The Robust Control System With Use of Nominal Model of Controlled Plant. IFAC Proc. Vol. 2000, 33, 911–916. [Google Scholar] [CrossRef]
- Li, G.; Tsang, K.M.; Ho, S.L. Robust Fine Tuning of Optimal PID Controller With Guaranteed Robustness. IEEE Trans. Ind. Electron. 2019, 67, 4911–4920. [Google Scholar]
- Ortega, R.; Loría, A.; Nicklasson, P.J.; Sira-Ramírez, H. Passivity-Based Control of Euler-Lagrange Systems; Mechanical, Electrical and Electromechanical Applications; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Shao, K.; Tang, R.; Xu, F.; Wang, X.; Zheng, J. Adaptive sliding mode control for uncertain Euler—Lagrange systems with input saturation. J. Frankl. Inst. 2021, 358, 8356–8376. [Google Scholar] [CrossRef]
- Aranovskiy, S.; Ryadchikov, I.; Nikulchev, E.; Wang, J.; Sokolov, D. Experimental Comparison of Velocity Observers: A Scissored Pair Control Moment Gyroscope Case Study. IEEE Access 2020, 8, 21694–21702. [Google Scholar] [CrossRef]
- Aranovskiy, S.; Ryadchikov, I.; Nikulchev, E.; Wang, J.; Sokolov, D. Bias Propagation and Estimation in Homogeneous Differentiators for a Class of Mechanical Systems. IEEE Access 2020, 8, 19450–19459. [Google Scholar] [CrossRef]
- Siciliano, B.; Villani, L. Robot Force Control; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Lynch, K.M.; Park, F.C. Modern Robotics: Mechanics, Planning, and Control; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Ye, H. Stabilization of Uncertain Feedforward Nonlinear Systems With Application to Underactuated Systems. IEEE Trans. Autom. Control 2019, 64, 3484–3491. [Google Scholar] [CrossRef]
- Liu, J.; He, J.; Ho-Ching, I.H. Realization of Low-Voltage and High-Current Rectifier Module Control System Based on Nonlinear Feed-Forward PID Control. Electronics 2021, 10, 2138. [Google Scholar] [CrossRef]
- Roffel, B.; Betlem, B. Process Dynamics and Control: Modeling for Control and Prediction; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Zhao, C.; Guo, L. Control of Nonlinear Uncertain Systems by Extended PID. IEEE Trans. Autom. Control 2021, 66, 3840–3847. [Google Scholar] [CrossRef]
- Silva, G.J.; Datta, A.; Bhattacharyya, S.P. On the stability and controller robustness of some popular PID tuning rules. IEEE Trans. Autom. Control 2003, 48, 1638–1641. [Google Scholar] [CrossRef]
- Mercader, P.; Åström, K.J.; Baños, A.; Hägglund, T. Robust PID Design Based on QFT and Convex—Concave Optimization. IEEE Trans. Control. Syst. Technol. 2017, 25, 441–452. [Google Scholar] [CrossRef]
- Hagiwara, T.; Araki, M. Robust stability of sampled-data systems under possibly unstable additive/multiplicative perturbations. IEEE Trans. Autom. Control 1998, 43, 1340–1346. [Google Scholar] [CrossRef]
- Skoczowski, S.; Domek, S.; Pietrusewicz, K.; Broel-Plater, B. A method for improving the robustness of PID control. IEEE Trans. Ind. Electron. 2005, 52, 1669–1676. [Google Scholar] [CrossRef]
- Miller, R.K. Industrial Robot Handbook; Springer: New York, NY, USA, 2014. [Google Scholar]
- Gonzalez, J.J.; Widmann, G.R. Investigation of nonlinearities in the force control of real robots. IEEE Trans. Syst. Man. Cybern. 1992, 22, 1183–1193. [Google Scholar] [CrossRef]
- Spong, M. Robot Modeling and Control; John Wiley and Sons Ltd.: Hoboken, NJ, USA, 2020. [Google Scholar]
- Osypiuk, R.; Finkemeyer, B.; Skoczowski, S. Simple two degree of freedom structures and their properties. Robotica 2006, 24, 365–372. [Google Scholar] [CrossRef]
- Kozłowski, K. Modelling and Identification in Robotics; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Kvrgic, V.; Vidakovic, J. Efficient method for robot forward dynamics computation. Mech. Mach. Theor. 2020, 145, 103680. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Osypiuk, R. A New Approach to Compensator Design Based on Multi-Loop Technique and Scalable Forward Model Complexity. Electronics 2021, 10, 3049. https://doi.org/10.3390/electronics10243049
Osypiuk R. A New Approach to Compensator Design Based on Multi-Loop Technique and Scalable Forward Model Complexity. Electronics. 2021; 10(24):3049. https://doi.org/10.3390/electronics10243049
Chicago/Turabian StyleOsypiuk, Rafał. 2021. "A New Approach to Compensator Design Based on Multi-Loop Technique and Scalable Forward Model Complexity" Electronics 10, no. 24: 3049. https://doi.org/10.3390/electronics10243049
APA StyleOsypiuk, R. (2021). A New Approach to Compensator Design Based on Multi-Loop Technique and Scalable Forward Model Complexity. Electronics, 10(24), 3049. https://doi.org/10.3390/electronics10243049





