The Energy-Water Nexus: An Analysis and Comparison of Various Configurations Integrating Desalination with Renewable Power
Abstract
:1. Introduction
2. Background
2.1. Reverse Osmosis Desalination of Brackish Groundwater
2.2. Wind-Powered Desalination
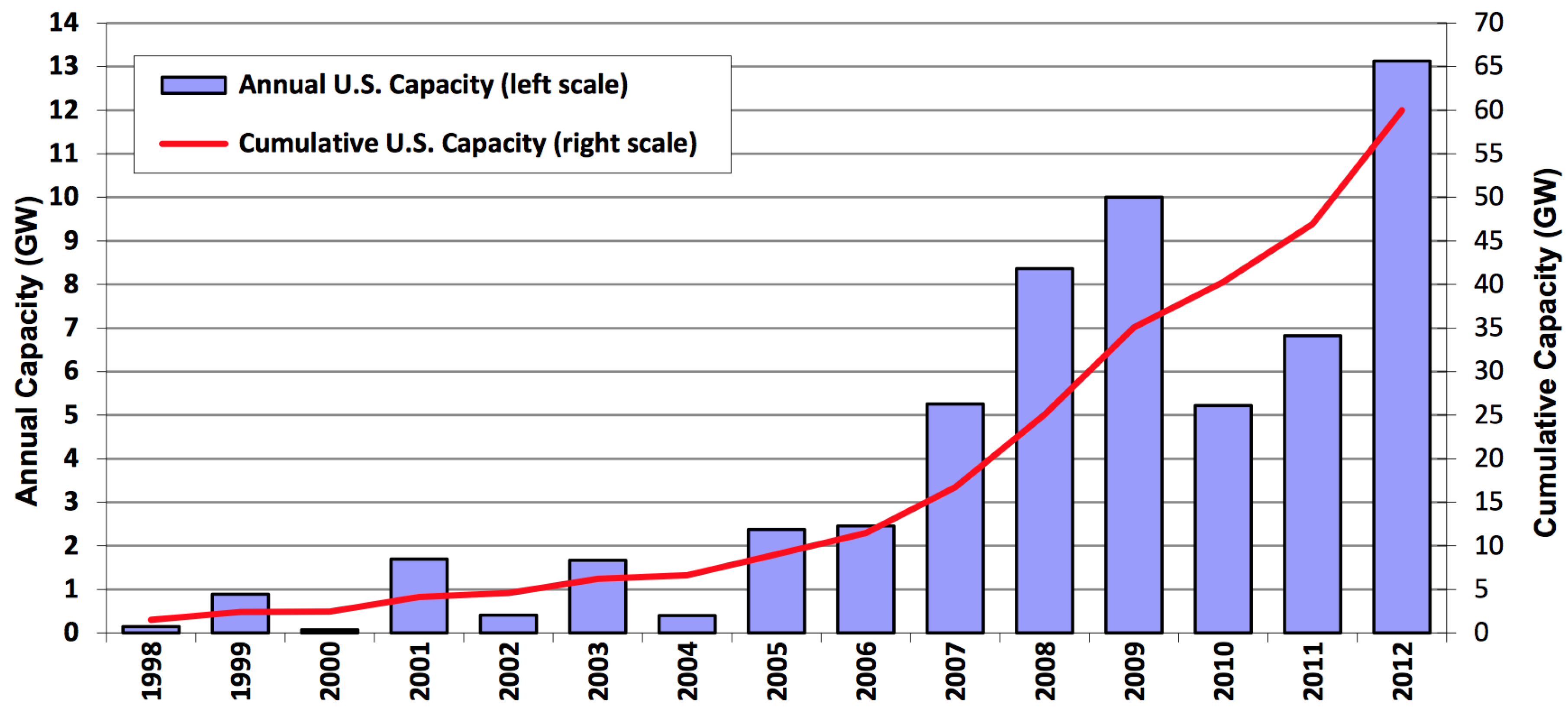
2.3. Solar-Powered Desalination
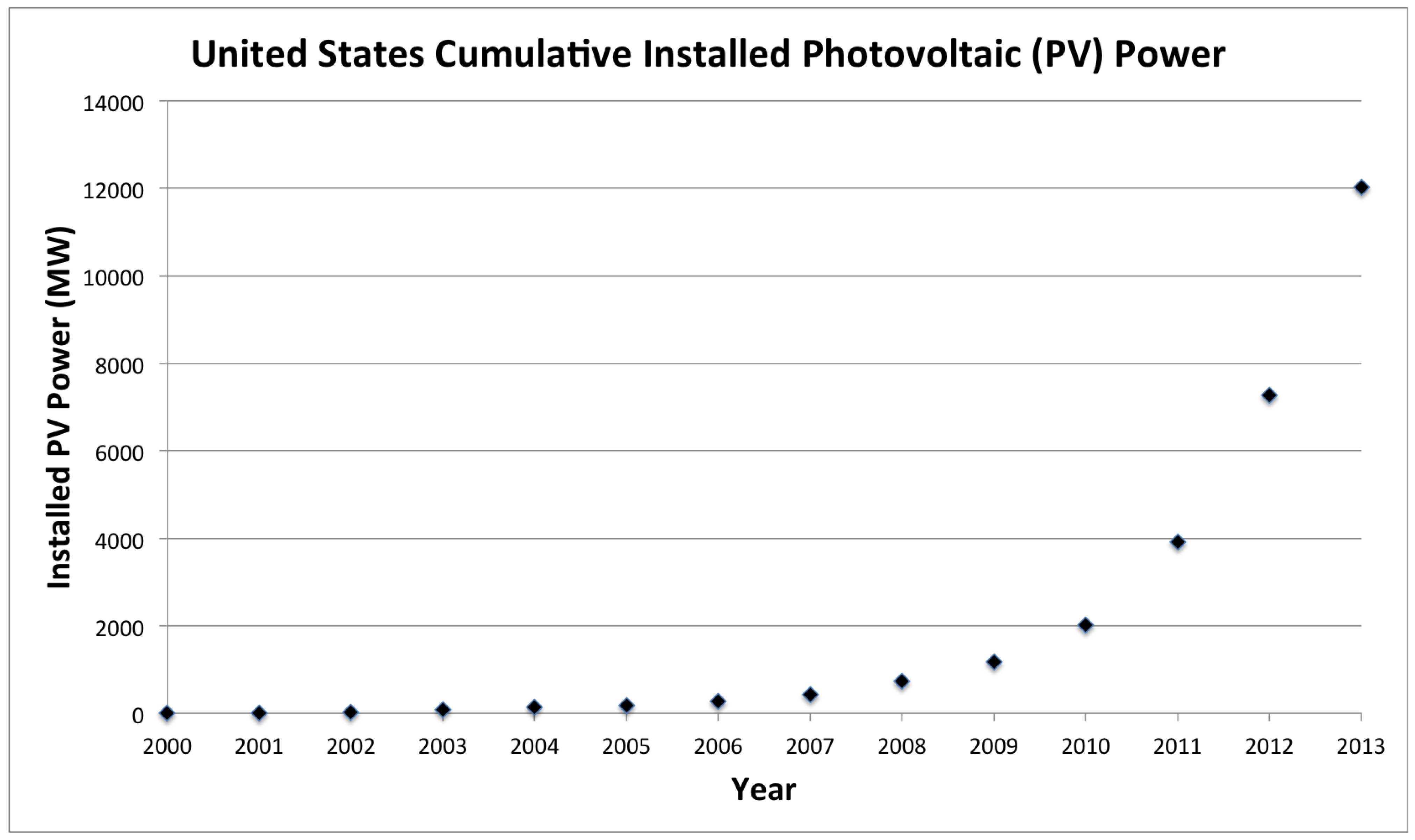
2.4. Wind/Solar-Powered RO Desalination
2.5. Photovoltaic Thermal System Used for Reverse Osmosis Desalination
2.5.1. Photovoltaic Thermal Solar Technology
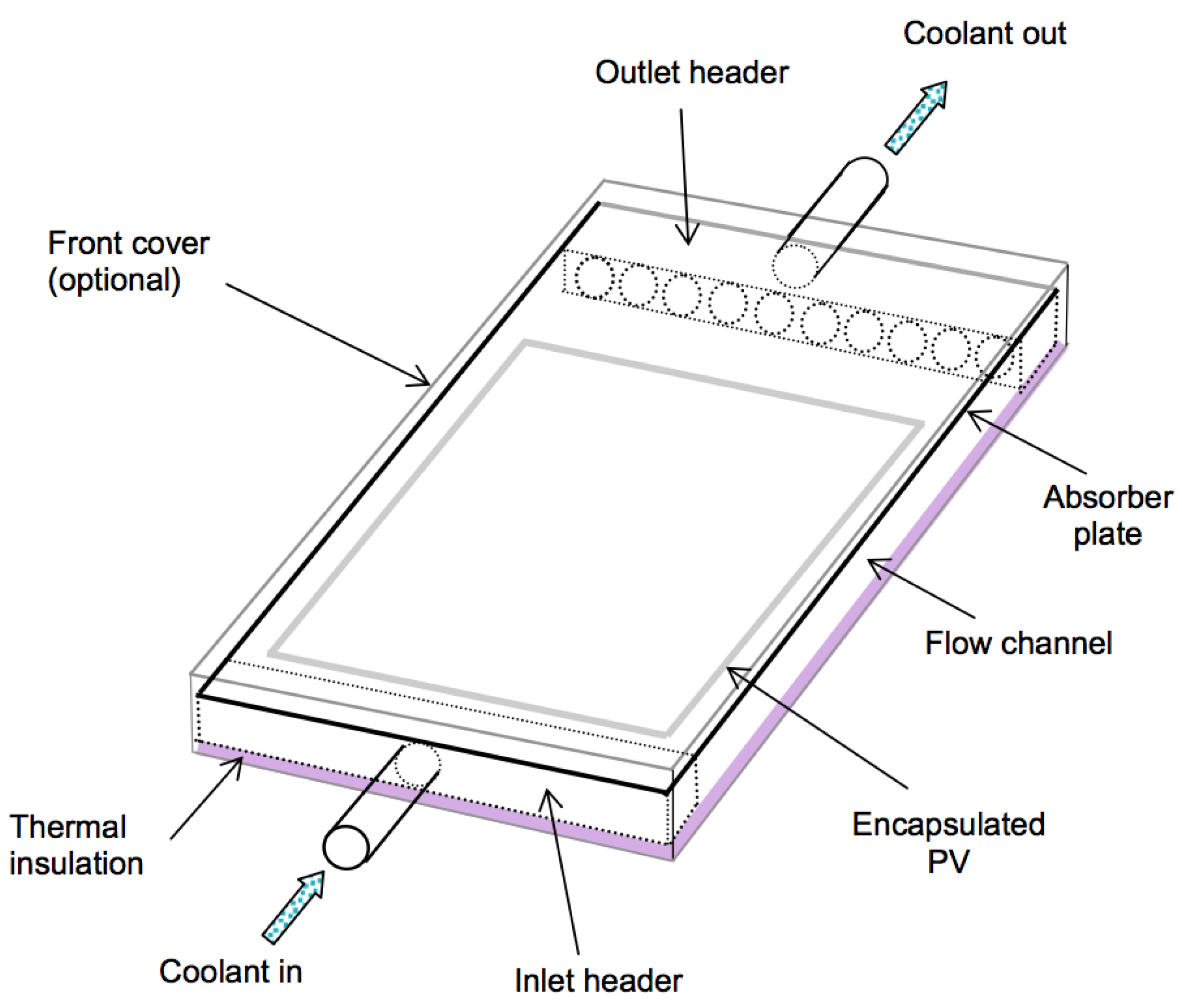
2.5.2. Reverse Osmosis Feed Water Temperature
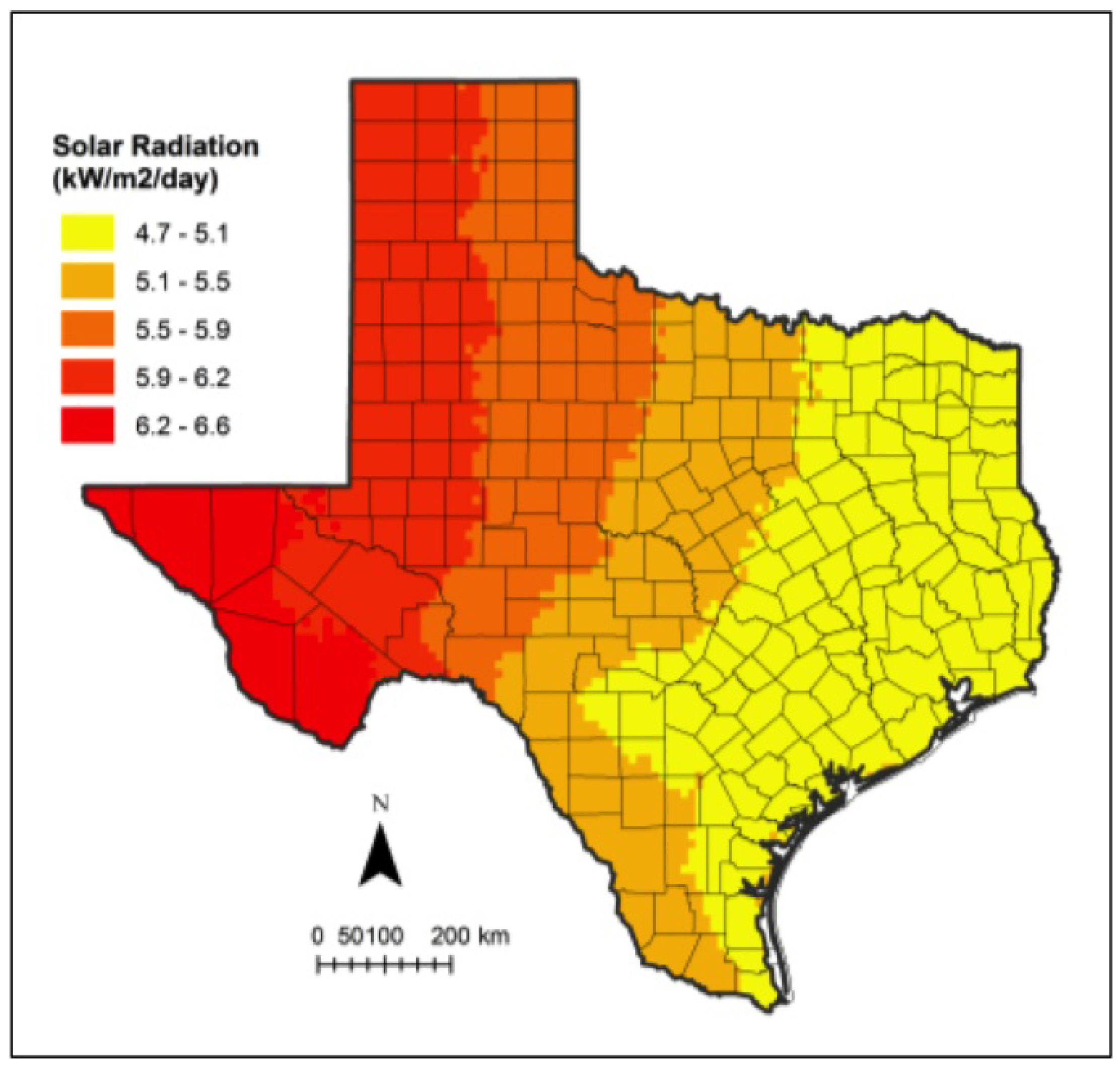
2.5.3. Texas as a Testbed
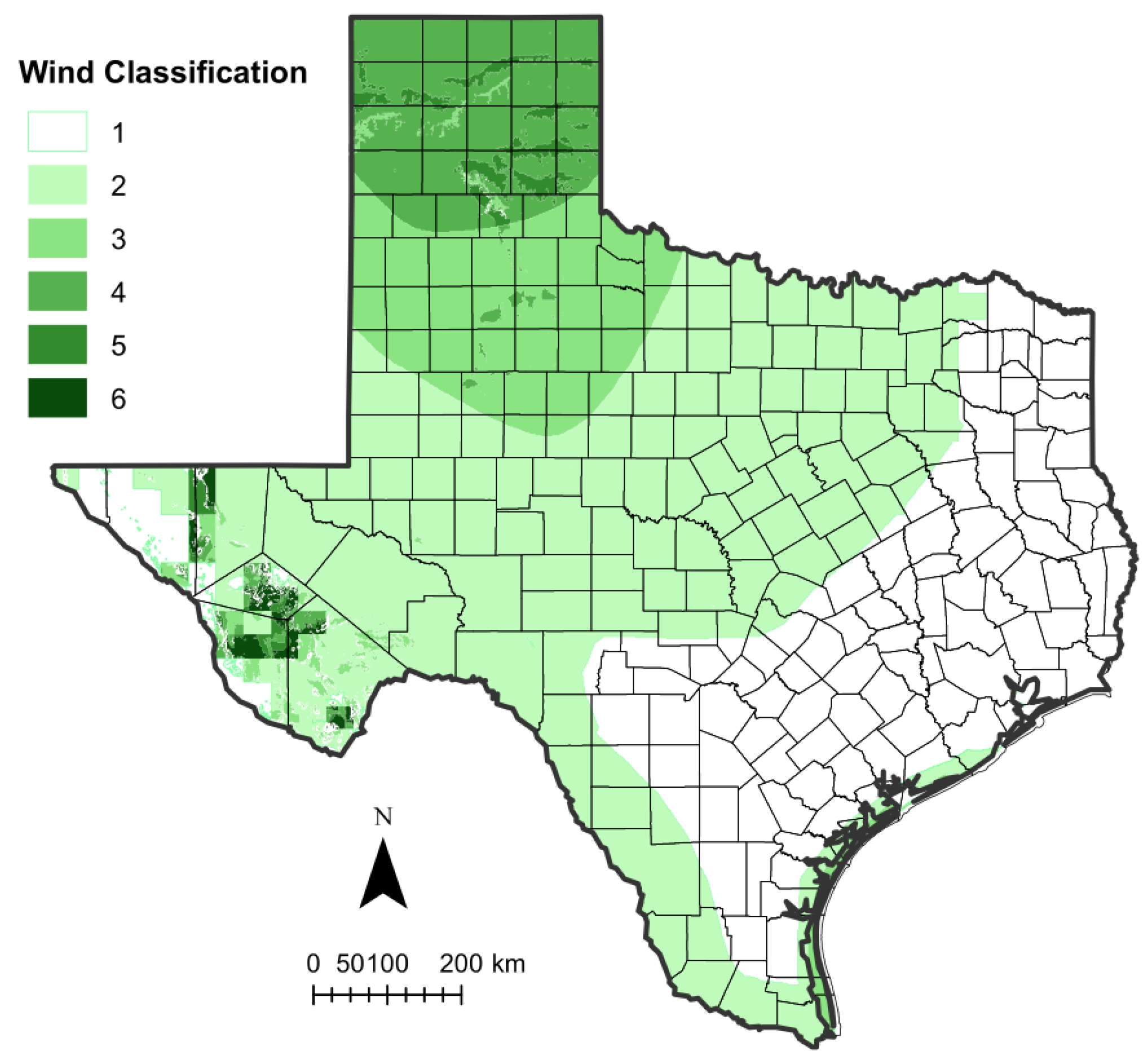
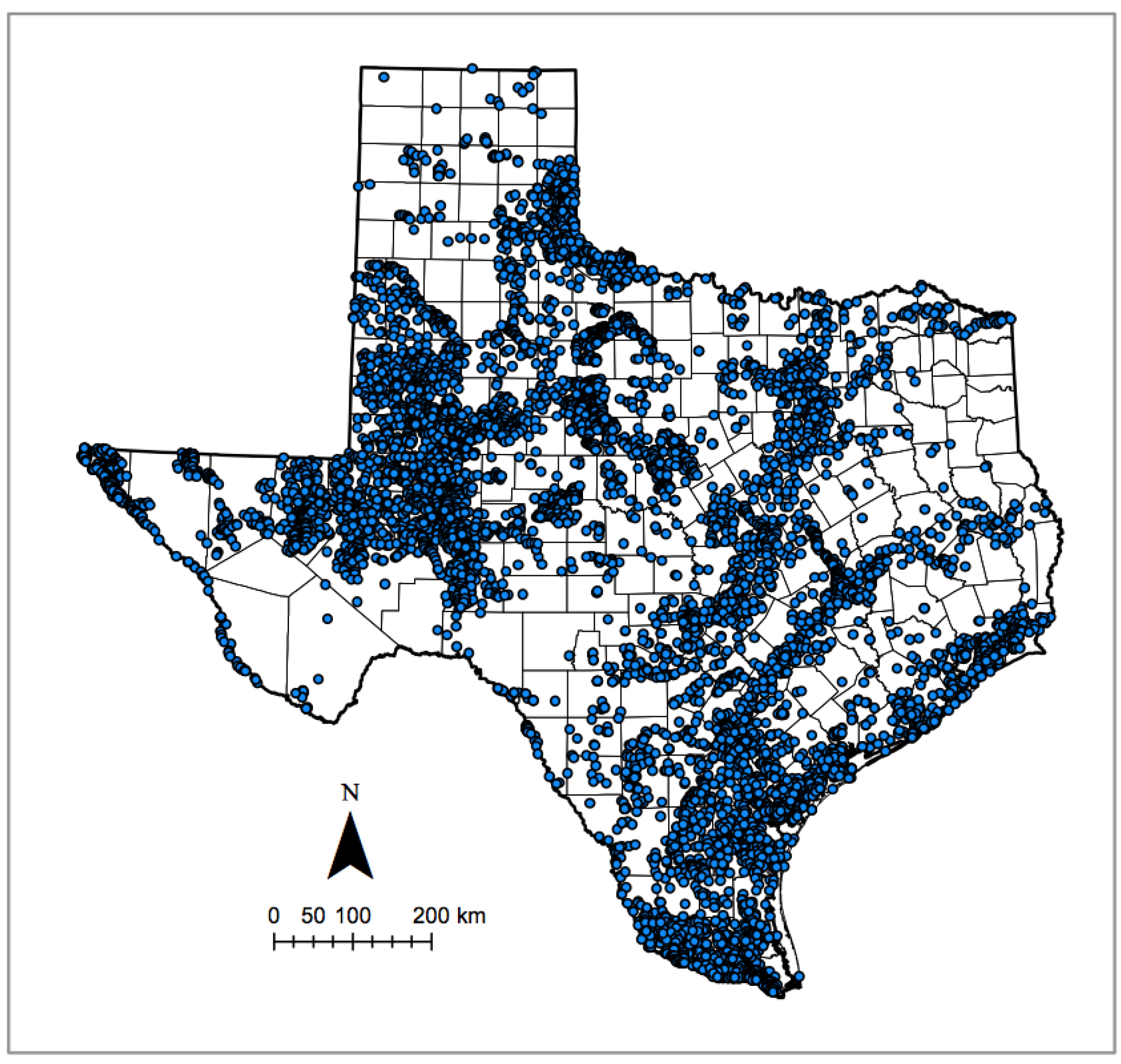
3. Modeling Methods
3.1. Overview

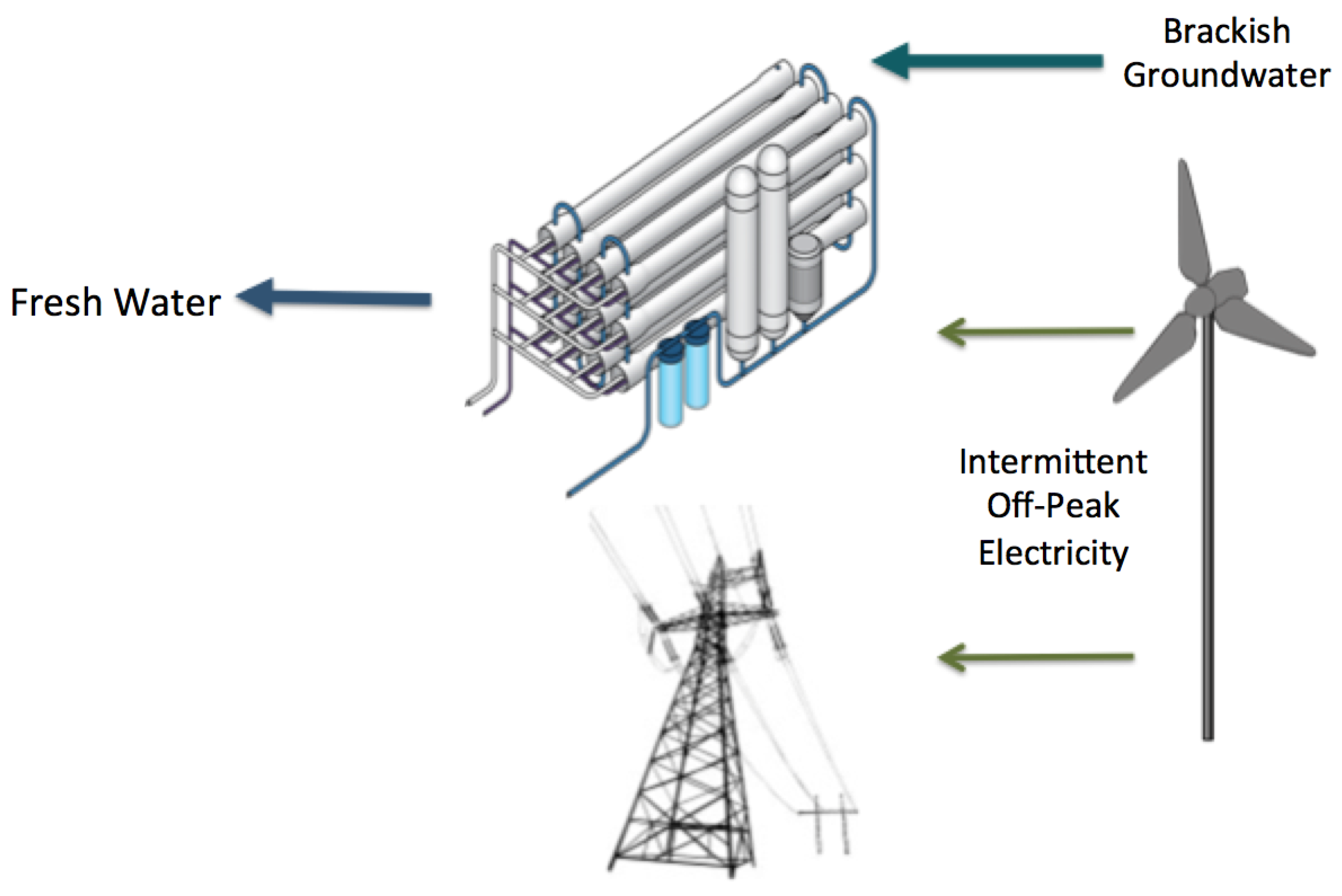

3.2. Water Treatment Model
| Parameter | Symbol | Value |
|---|---|---|
| Depth to aquifer | z | 275 m |
| Pipe length | l | 5250 m |
| Density of water | ρ | 1000 kg/m |
| Pump efficiency | 0.65 | |
| Acceleration due to gravity | g | 9.81 m/s |
| Pipe diameter | D | 0.3 m |
| Friction factor | f | 0.0162 |
| Reverse osmosis recovery factor | R | 0.8 |
| Energy intensity of reverse osmosis for Scenarios B and D | E | 1.5 kWh/m |
| Energy intensity of reverse osmosis for Scenarios A and C | E | 1.4 kWh/m |
| Desalination capacity factor | CF | 0.95 |
| Desired daily product water | G | 3000 m/day |
3.3. Energy Model
3.3.1. Solar Farm Sized for Preheating Water in Scenario C
| Parameter | Symbol | Value |
|---|---|---|
| Inverse of density of water | 0.001003 | |
| Specific enthalpy of water entering PVT panel | h | 104.89 |
| Specific enthalpy of water exiting PVT panel | h | 125.79 |
3.3.2. Solar Farm Sized for BWRO Desalination in Scenario A
3.3.3. Wind Farm Sizing for Scenarios B and C
3.4. Integrated Model
3.4.1. Water Production Revenue and Cost for Scenarios A, B, C and D
3.4.2. Integrated GAMS Model for Scenario A
3.4.3. Integrated GAMS Model for Scenario B
3.4.4. Integrated GAMS Model for Scenario C
3.4.5. Integrated GAMS Model for Scenario D
4. Results and Discussion
4.1. Overview
4.2. Water Treatment Model Results
| Scenarios A and C | Scenarios B and D | |
|---|---|---|
| Total power requirement by desalination plant | 432 kW | 440 kW |
| Power required for pumping | 194 kW | 194 kW |
| Power required for RO treatment | 238 kW | 246 kW |
4.3. Energy Model Results
| Solar Farm | Wind Farm | |
|---|---|---|
| Scenario A | 2057 kW | N/A |
| Scenario B | N/A | 1257 kW |
| Scenario C | 1644 kW | 1233 kW |
4.4. Operational Profiles from the Integrated Model
4.4.1. Operational Profiles for Scenario A
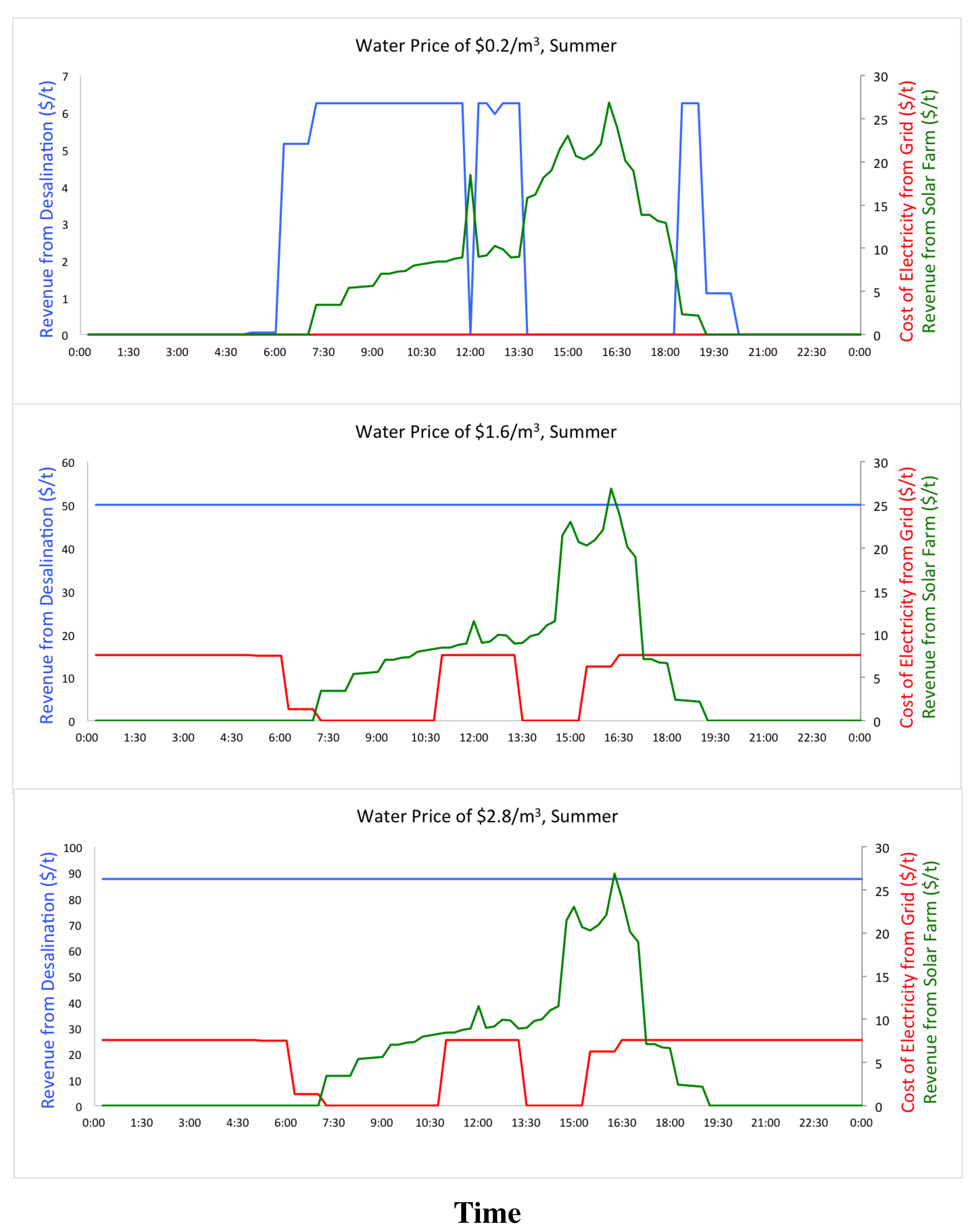
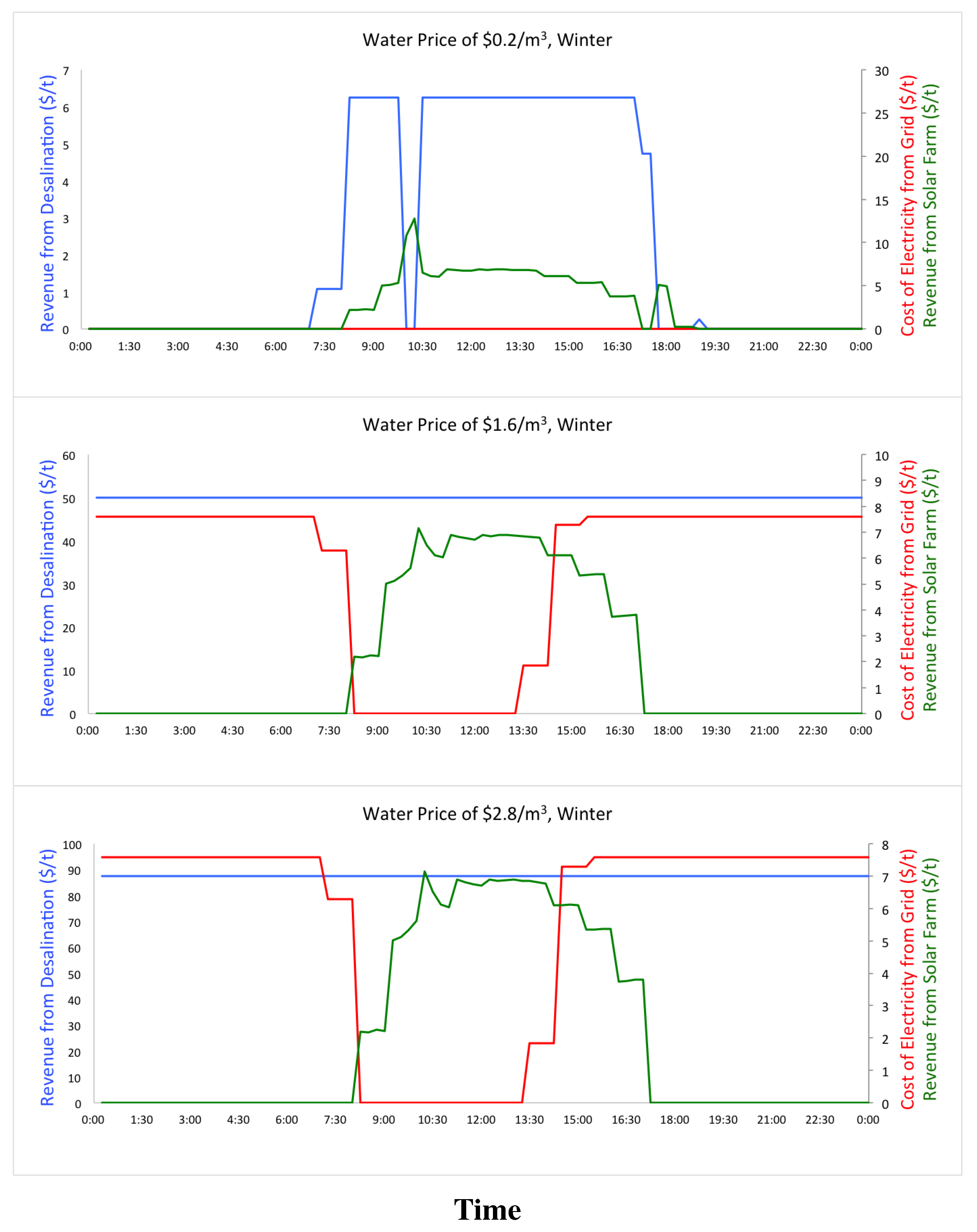
4.4.2. Operational Profiles for Scenario B
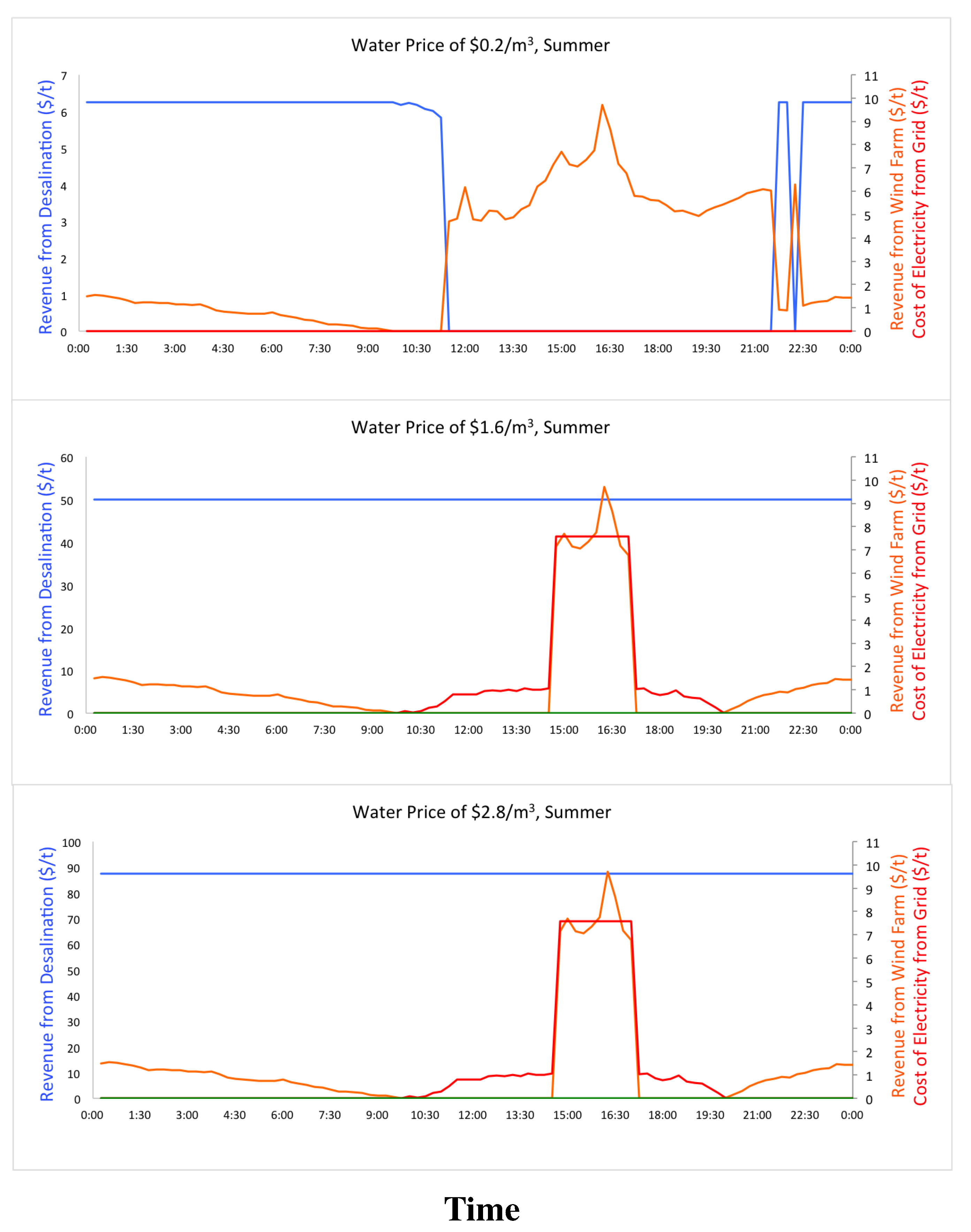
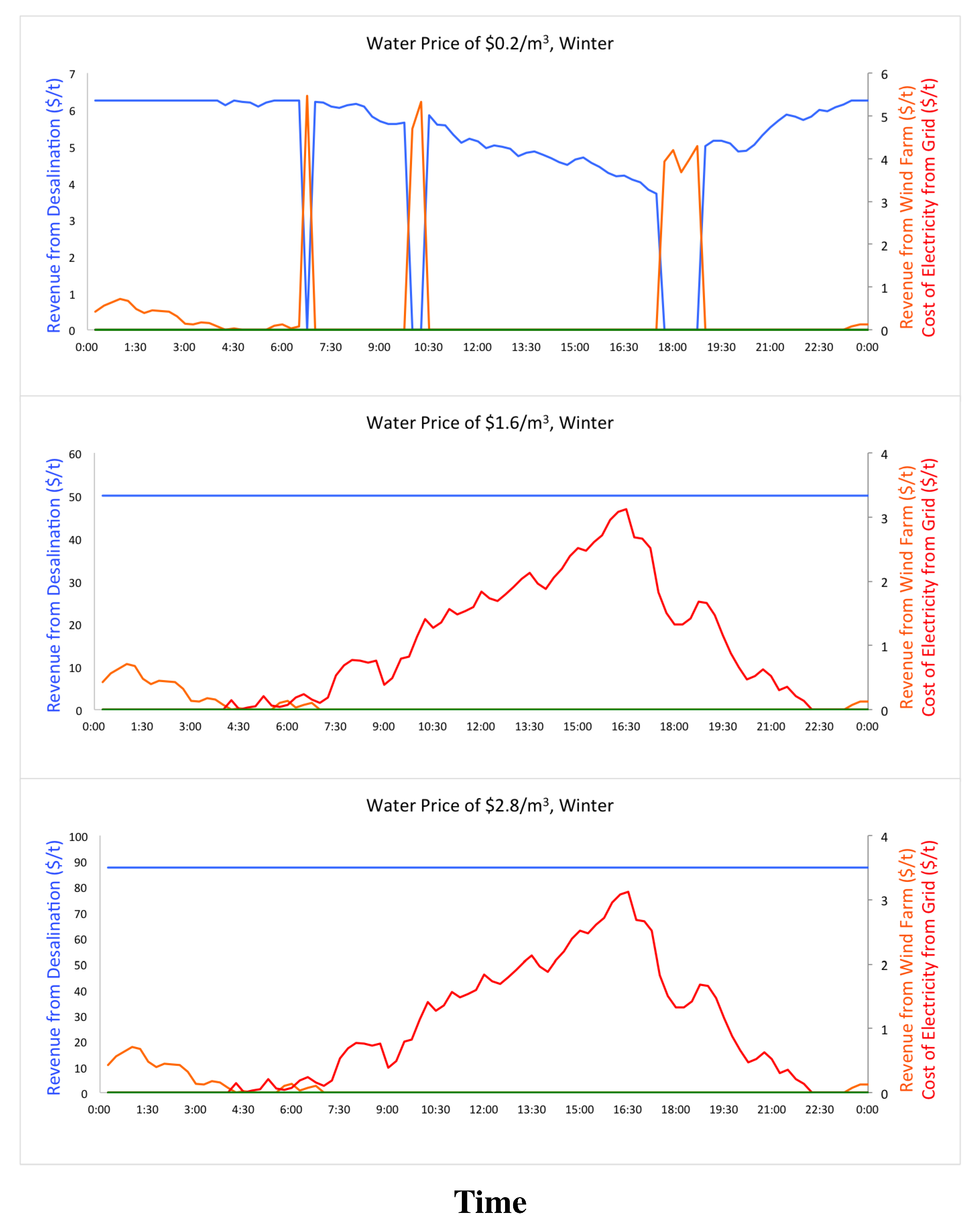
4.4.3. Operational Profiles for Scenario C
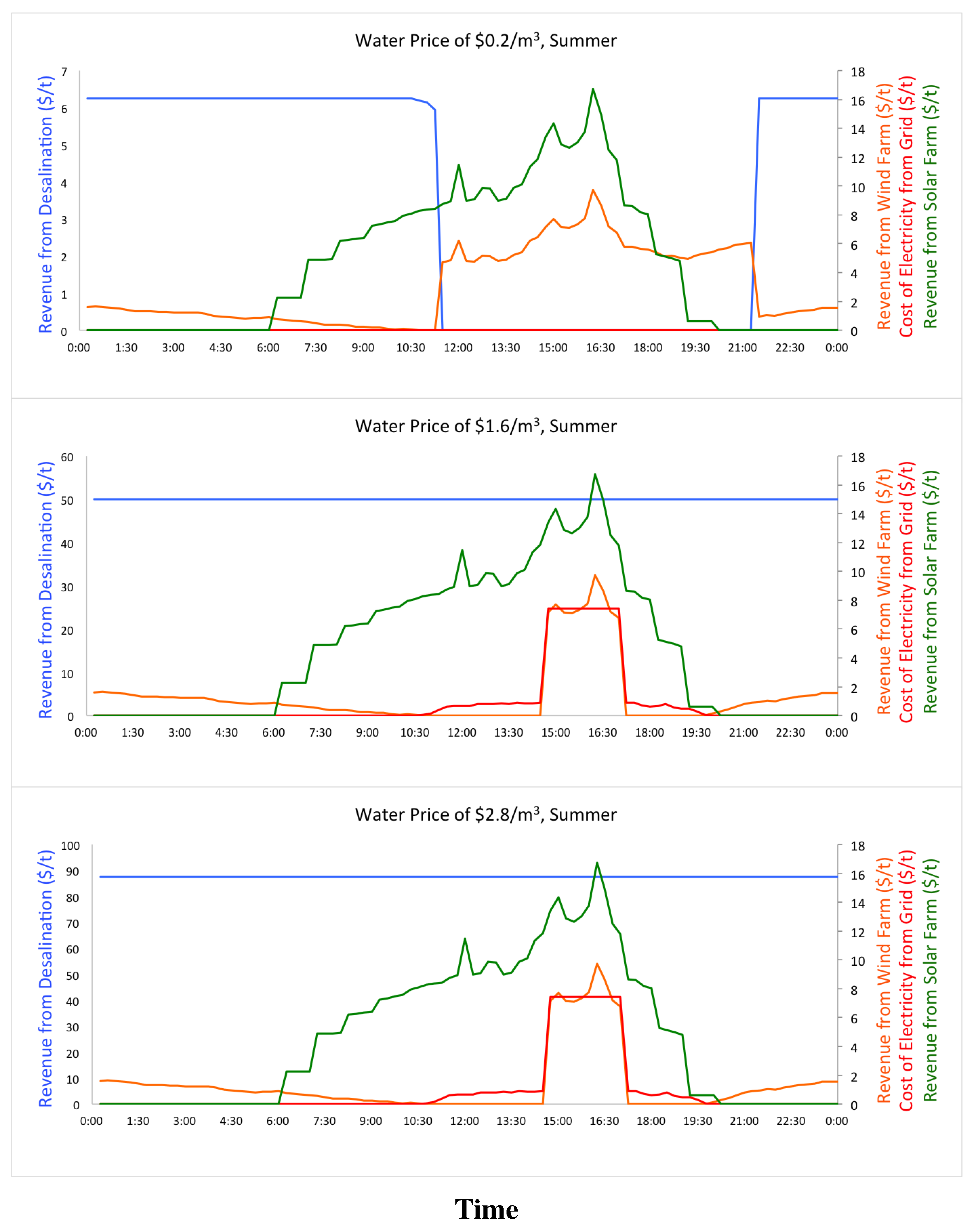
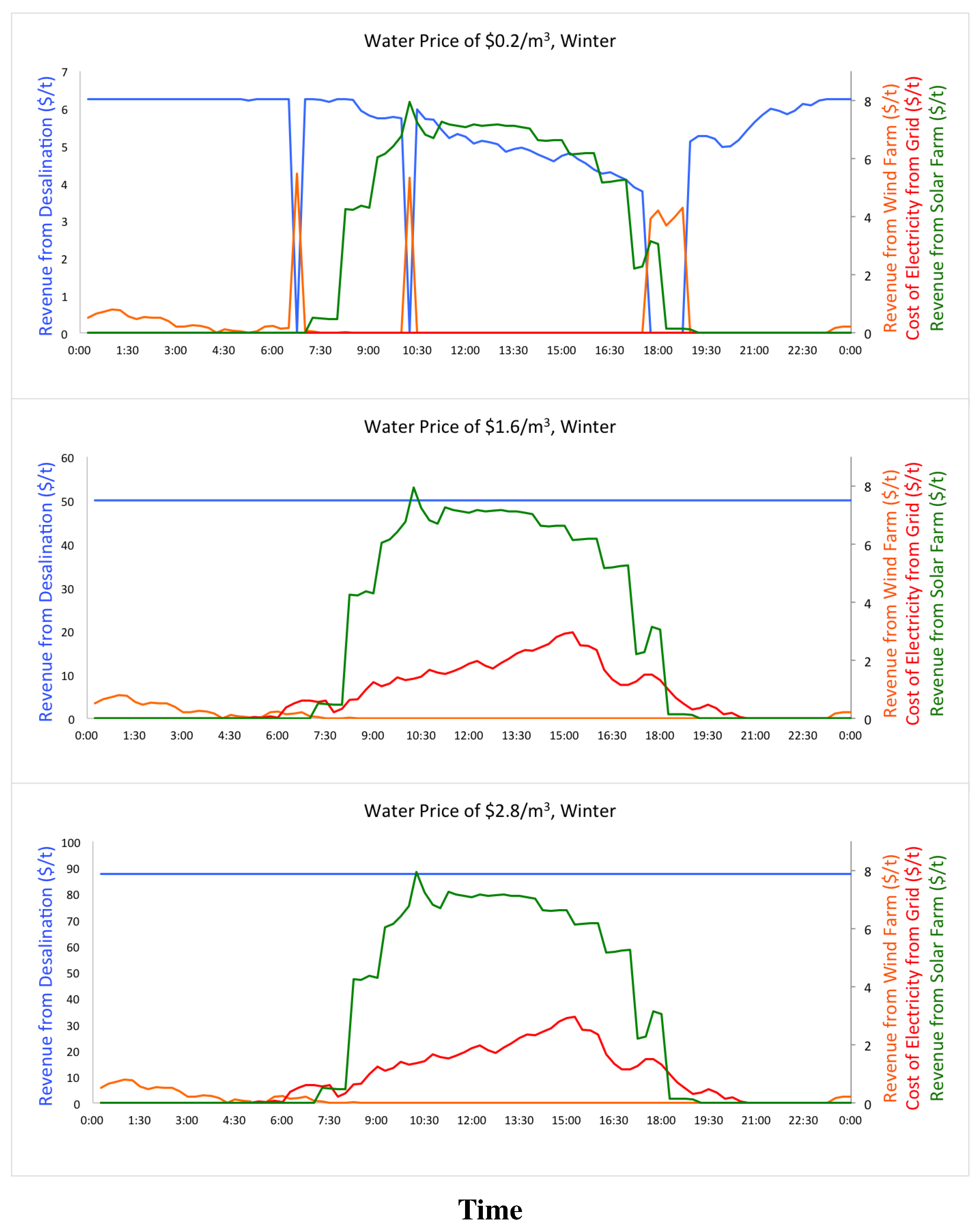
4.4.4. Operational Profiles for Scenario D
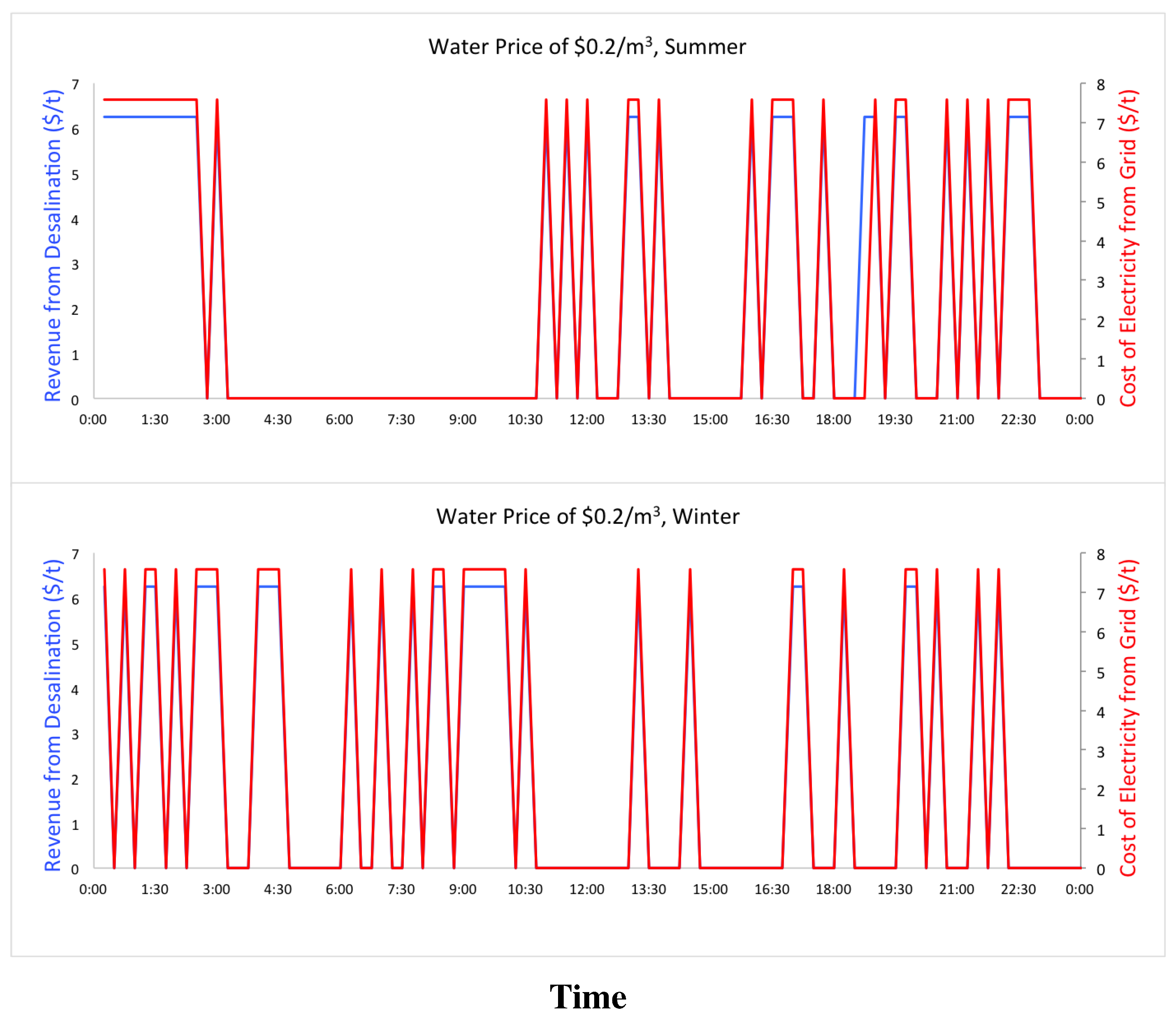
4.5. Comparison of Electricity Costs
| Water Price | $0.20 per m | $1.60 per m | $2.80 per m |
|---|---|---|---|
| Daily cost of electricity for Scenario A | $0 | $409 | $409 |
| Daily cost of electricity for Scenario B | $0 | $97 | $97 |
| Daily cost of electricity for Scenario C | $0 | $91 | $91 |
| Daily cost of electricity for Scenario D | $243 | $729 | $729 |
| Water Price | $0.20 per m | $1.60 per m | $2.80 per m |
|---|---|---|---|
| Daily cost of electricity for Scenario A | $0 | $426 | $426 |
| Daily cost of electricity for Scenario B | $0 | $89 | $89 |
| Daily cost of electricity for Scenario C | $0 | $78 | $78 |
| Daily cost of electricity for Scenario D | $243 | $729 | $729 |
4.6. Comparison of Revenues from Water and Electricity in Scenario C
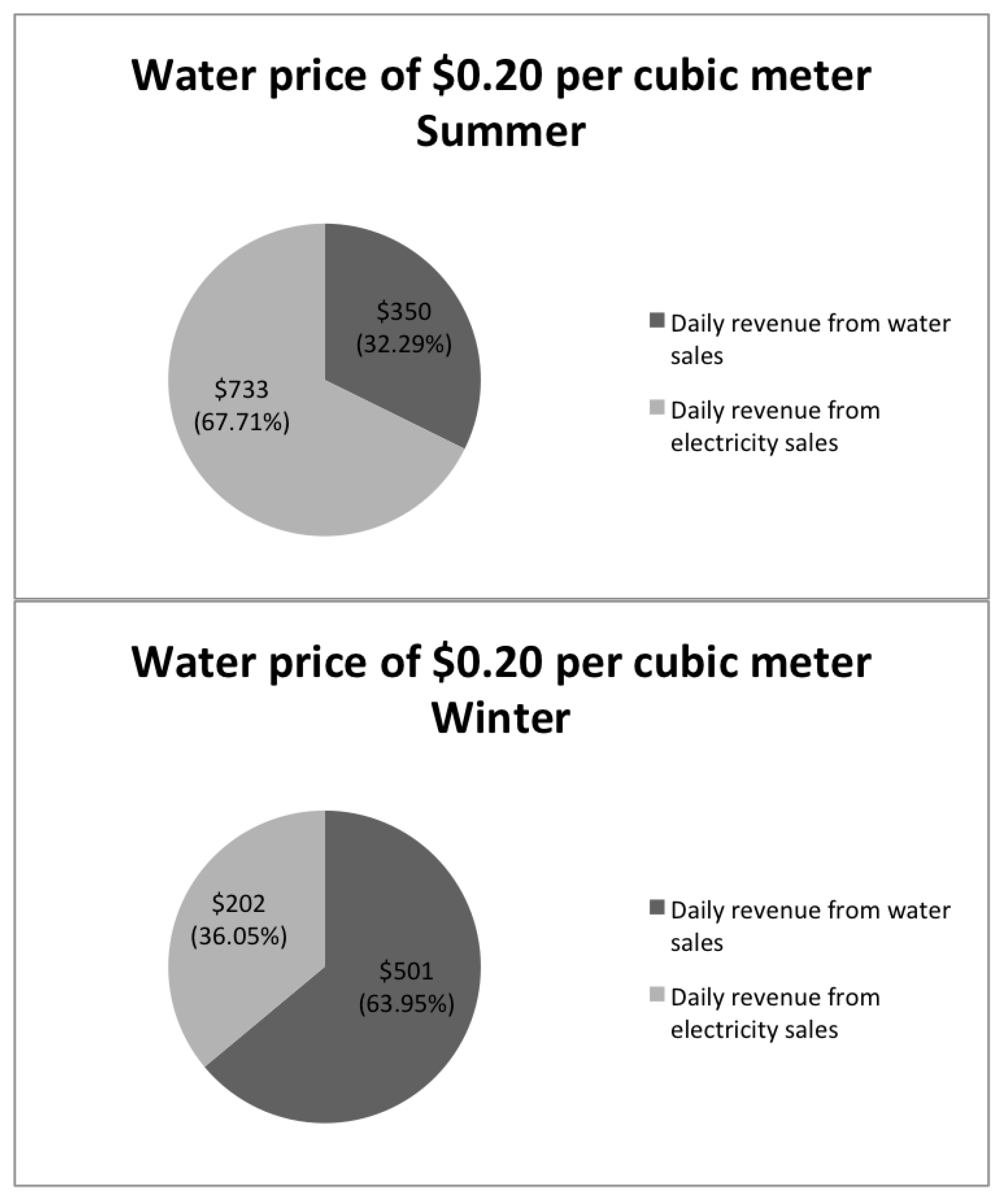


5. Conclusions
5.1. Future Work
5.2. Recommendations
Acknowledgments
Author Contributions
Conflicts of Interest
References
- United State Census Bureau. State and County Quick Facts; United State Census Bureau: Washington, DC, USA, 2010.
- United States Global Change Research Program, National Climate Assessment. Climate Change Impacts in the United States; United States Global Change Research Program: Washington, DC, USA, 2014.
- Clayton, M.; Stillwell, A.; Webber, M. Implementation of Brackish Groundwater Desalination Using Wind-Generated Electricity: A Case Study of the Energy-Water Nexus in Texas. Sustainability 2014, 6, 758–778. [Google Scholar] [CrossRef]
- Desalination : Brackish Groundwater; Texas Water Development Board: Austin, TX, USA, 2013.
- Miller, J.E. Review of Water Resources and Desalination Technologies; Sandia National Laboratories: Albuquerque, NM; Livermore, CA, USA, 2003.
- Committee on Advancing Desalination Technology. Desalination: A national perspective; The National Academies Press: Washington, DC, USA, 2008. [Google Scholar]
- Greenlee, L.F.; Lawler, D.F.; Freeman, B.D.; Marrot, B.; Moulin, P. Reverse osmosis desalination: Water sources, technology, and today’s challenges. Water Res. 2009, 43, 2317–2348. [Google Scholar] [CrossRef]
- Desalination Plant Database; Texas Water Development Board: Austin, TX, USA, 2014.
- 2012 State Water Plan; Texas Water Development Board: Austin, TX, USA, 2012.
- Mickley and Associates. Membrane Concentrate Disposal : Practices and Regulation; U.S. Department of the Interior: Denver, CO, USA, 2006.
- Rainwater, K.; Nash, P.; Song, L.; Schroeder, J. The Seminole Project: Renewable Energy for Municipal Water Desalination. J. Contemp. Water Res. Educ. 2013, 151, 50–60. [Google Scholar] [CrossRef]
- United States Department of Energy. 2012 Wind Technologies Market Report; United States Department of Energy: Washington, DC, USA, 2012.
- United States Department of Energy. 2013 Wind Technologies Market Report; United States Department of Energy: Washington, DC, USA, 2013.
- Electric Reliability Council of Texas (ERCOT). Entity-Specific Resource Output; ERCOT: Austin, TX, USA, 2012. [Google Scholar]
- Sandia National Laboratories. Electric Power Industry Need for Grid-Scale Storage Applications; Sandia National Laboratories: Albuquerque, NM, USA, 2010.
- Ma, Q.; Lu, H. Wind energy technologies integrated with desalination systems: Review and state-of-the-art. Desalination 2011, 277, 274–280. [Google Scholar] [CrossRef]
- Forstmeier, M.; Mannerheim, F.; D’Amato, F.; Shah, M.; Liu, Y.; Baldea, M.; Stella, A. Feasibility study on wind-powered desalination. Desalination 2007, 203, 463–470. [Google Scholar] [CrossRef]
- Zejli, D.; Benchrifa, R.; Bennouna, A.; Zazi, K. Economic analysis of wind-powered desalination in the south of Morocco. Desalination 2004, 165, 219–230. [Google Scholar] [CrossRef]
- Kershrnana, S.A.; Rheinl, J.; Gablerb, H. Seawater reverse osmosis powered Tom renewable energy sources— Hybrid wind/photovoltaic/grid power supply for small-scale desalination in Libya. Desalination 2002, 153, 17–23. [Google Scholar]
- United States Department of Energy. 2010 Solar Technologies Market Report; United States Department of Energy: Washington, DC, USA, 2011.
- BP Statistical Review of World Energy 2014. Available online: http://www.bp.com/content/dam/bp/pdf/Energy-economics/statistical-review-2014/BP-statistical-review-of-worldenergy-2014-full-report.pdf (accessed on 20 April 2015).
- Peterson, G.; Fries, S.; Mohn, J.; Muller, A. Wind and solar powered reverse osmosis desalination units: Description of two demonstation projects. Desalination 1979, 31, 501–509. [Google Scholar] [CrossRef]
- Headley, O. Renewable energy technologies in the Caribbean. Sol. Energy 1997, 59, 1–9. [Google Scholar] [CrossRef]
- Ghermandi, A.; Messalem, R. Solar-driven desalination with reverse osmosis: The state of the art. Desalin. Water Treat. 2009, 7, 285–296. [Google Scholar] [CrossRef]
- Weiner, D.; Fisher, D.; Moses, E.J.; Katz, B.; Meron, G. Operation experience of a solar- and wind-powered desalination demonstration plant. Desalination 2001, 137, 7–13. [Google Scholar] [CrossRef]
- Zhu, A.; A. Rahardianto, P.D.C.; Cohen, Y. Reverse Osmosis Desalination with High Permeability Membranes—Cost Optimization and Research Needs. Desalin. Water Treat. 2010, 15, 256–266. [Google Scholar]
- Gude, V.G. Energy storage for desalination processes powered by renewable energy and waste heat sources. Appl. Energy 2015, 137, 877–898. [Google Scholar] [CrossRef]
- Charcosset, C. Combining membrane processes with renewable energy technologies: Perspectives on membrane desalination, biofuels and biogas production, and microbial fuel cells. Membr. Clean Renew. Power Appl. 2014, 1, 44–62. [Google Scholar]
- Chow, T. A review on photovoltaic/thermal hybrid solar technology. Appl. Energy 2010, 87, 365–379. [Google Scholar] [CrossRef]
- Skoplaki, E.; Palyvos, J. On the temperature dependence of photovoltaic module electrical performance: A review of efficiency/power correlations. Sol. Energy 2009, 83, 614–624. [Google Scholar] [CrossRef]
- Skoplaki, E.; Palyvos, J. Operating temperature of photovoltaic modules: A survey of pertinent correlations. Renew. Energy 2009, 34, 23–29. [Google Scholar] [CrossRef]
- Solimpeks Academy. Volther Hybrid PV-T Panels; Solimpeks Academy: Karatay, Turkey, 2010. [Google Scholar]
- Mittelman, G.; Kribus, A.; Mouchtar, O.; Dayan, A. Water desalination with concentrating photovoltaic/thermal (CPVT) systems. Sol. Energy 2009, 83, 1322–1334. [Google Scholar] [CrossRef]
- El-dessouky, B.H.; Alatiqi, I.; Bingulac, S.; Ettouney, H. Steady-State Analysis of the Multiple Effect Evaporation Desalination Process. Chem. Eng. Technol. 1998, 21, 437–451. [Google Scholar] [CrossRef]
- Mittelman, G.; Kribus, A.; Dayan, A. Solar cooling with concentrating photovoltaic/thermal (CPVT) systems. Energy Convers. Manag. 2007, 48, 2481–2490. [Google Scholar] [CrossRef]
- Van Helden, W.G.J.; van Zolingen, R.J.C.; Zondag, H.a. PV thermal systems: PV panels supplying renewable electricity and heat. Progr. Photovolt.: Res. Appl. 2004, 12, 415–426. [Google Scholar] [CrossRef]
- Davis, T.; Cappelle, M. Hybrid Photovoltaic/Thermal (PV-T) Systems for Water Desalination; UTEP Center for Inland Desalination: Austin, TX, USA, 2013. [Google Scholar]
- United States Department of Energy, National Renewable Energy Labotatory. stalled Wind Capacity; United States Department of Energy: Washington, DC, USA, 2014.
- United States Department of Energy: National Renewable Energy Laboratory. Dynamic Maps, GIS Data, and Analysis Tool: U.S. 50m Wind Resource Map; United States Department of Energy: Washington, DC, USA, 2009.
- Lopez, A.; Roberts, B.; Heimiller, D.; Blair, N.; Porro, G. US Renewable Energy Technical Potentials : A GIS-Based Analysis. Contract 2012, 303, 275–3000. [Google Scholar]
- United States Department of Energy, National Renewable Energy Laboratory. Dynamic Maps, GIS Data, and Analysis Tools: Solar Maps; United States Department of Energy: Washington, DC, USA, 2014.
- United States Department of Energy, National Renewable Energy Labratory. The Open PV Project; United States Department of Energy: Washington, DC, USA, 2013.
- United States Department of Energy, National Renewable Energy Laboratory. Dynamic Maps, GIS Data, and Analysis Tools: 10-Kilometer Solar Data; United States Department of Energy: Washington, DC, USA, 2014.
- Wogan, D.M.; Webber, M.E.; da Silva, A. A Framework and Methodology for Reporting Geographically and Temporally Resolved Solar Data: A Case Study of Texas. J. Renew. Sustain. Energy 2010, 2, 053107. [Google Scholar] [CrossRef]
- Texas Water Development Board. Groundwater Database; Texas Water Development Board: Austin, TX, USA, 2009.
- Al-Karaghouli, A.; Kazmerski, L.L. Energy consumption and water production cost of conventional and renewable-energy-powered desalination processes. Renew. Sustain. Energy Rev. 2013, 24, 343–356. [Google Scholar] [CrossRef]
- Klein, G.; Krebs, M. California’s Water—Energy Relationship Final Staff Report 2005. Available online: http://www.energy.ca.gov/2005publications/CEC-700-2005-011/CEC-700-2005-011-SF.PDF (accessed on 20 April 2015).
- Semiat, R. Critical Review Energy Issues in Desalination Processes. Environ. Sci. Technol. 2008, 42, 8193–8201. [Google Scholar] [CrossRef] [PubMed]
- Texas Comptroller of Public Accounts. Liquid Assest: The State of Texas’ Water Resources. 2009. Available online: http://www.window.state.tx.us/specialrpt/water/2009/PDF/96-1360-LiquidAssets.pdf (accessed on 20 April 2015).
- Stillwell, A.S. The Energy-Water Nexus in Texas. Ph.D. Thesis, The University of Texas at Austin, Austin, TX, USA, 2010. [Google Scholar]
- Moran, M.J.; Shapiro, H.N.; Boettner, D.D.; Bailey, M.B. Fundamentals of Engineering Thermodynamics, 7 ed.; Wiley: Hoboken, NJ, USA, 2010. [Google Scholar]
- Environmental Proctection Agency. Average Temperature of Shallow Groundwater; Environmental Protection Agency: Washington, DC, USA, 2013.
- Fudholi, A.; Sopian, K.; Yazdi, M.H.; Ruslan, M.H.; Ibrahim, A.; Kazem, H.A. Performance analysis of photovoltaic thermal (PVT) water collectors. Energy Convers. Manag. 2014, 78, 641–651. [Google Scholar] [CrossRef]
- Electric Reliability Coucil of Texas. Abilene Solar Farm Entity-Specific Resource Output; Electric Reliability Council of Texas: Austin, TX, USA, 2002. [Google Scholar]
- Electric Reliability Council of Texas. Sweetwater 1 Wind Farm Entity-Specific Resource Output; Electric Reliability Council of Texas: Austin, TX, USA, 2010. [Google Scholar]
- Kendrick, D.A.; Mercado, P.R.; Amman, H.M. Computational Economics; Princeton University Press: Princeton, NJ, USA, 2006. [Google Scholar]
- Hardberger, A.; Kelly, M. From Policy to Reality; Environmental Defense Fund: NY, USA, 2008. [Google Scholar]
- Mickley, M.C. Membrace Concentrate Disposal: Practices and Regulation, Reclamation: Managing Water in the West; Mickley and Associates: Boulder, CO, USA, 2006. [Google Scholar]
- Foldager, R.A. Economics of Desalination Concentrate Disposal Methods in Inland Regions: Deep-Well Injection, Evaporative Ponds, and Salinity Gradient Solar Ponds. Ph.D. Thesis, New Mexico State University, Las Cruces, NM, USA, 2003. [Google Scholar]
- United States Department of Energy, Energy Information Administration. Annual Energy Review 2011; United States Department of Energy: Washington, DC, USA, 2012.
- Electric Reliability Council of Texas (ERCOT). Historical RTM Load Zone and Hub Prices; ERCOT: Austin, TX, USA, 2013.
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gold, G.M.; Webber, M.E. The Energy-Water Nexus: An Analysis and Comparison of Various Configurations Integrating Desalination with Renewable Power. Resources 2015, 4, 227-276. https://doi.org/10.3390/resources4020227
Gold GM, Webber ME. The Energy-Water Nexus: An Analysis and Comparison of Various Configurations Integrating Desalination with Renewable Power. Resources. 2015; 4(2):227-276. https://doi.org/10.3390/resources4020227
Chicago/Turabian StyleGold, Gary M., and Michael E. Webber. 2015. "The Energy-Water Nexus: An Analysis and Comparison of Various Configurations Integrating Desalination with Renewable Power" Resources 4, no. 2: 227-276. https://doi.org/10.3390/resources4020227
APA StyleGold, G. M., & Webber, M. E. (2015). The Energy-Water Nexus: An Analysis and Comparison of Various Configurations Integrating Desalination with Renewable Power. Resources, 4(2), 227-276. https://doi.org/10.3390/resources4020227