An Evolutionary Game Analysis of Heterogeneous Local Government Land Supply Behavior and Industrial Transfer Competition

Abstract

The implicit assumption of homogeneity in traditional economics fails to adequately explain the complex and contradictory behavior exhibited by different local governments in China during the land supply process. The issue of land supply is characterized by practical chaos and has fallen into a theoretical research dilemma. Starting from two dimensions of heterogeneity—preferences and income—this study establishes an evolutionary game model of the limited rationality of local governments. It explores the behavioral evolution process of local governments in the context of industrial competition for land supply and its convergence trends. The research findings indicate that the land supply behavior of local governments is primarily determined by the degree of heterogeneity in preferences and income, as well as the level of compensation for losses to cooperative partners and the severity of penalties for non-cooperative behavior. If local governments’ preferences converge towards a certain threshold or if their income continually increases, they are more inclined towards cooperative behavior; otherwise, they tend to act non-cooperatively. If local governments’ revenues are heavily reliant on industrial transfers, they are more likely to engage in non-cooperative land supply behavior. This research offers theoretical insights and policy recommendations to enhance the optimal allocation of land resources in China.

1. Introduction

According to data from the Chinese Ministry of Finance, in 2021, China’s revenue from the transfer of state-owned land use rights reached a new high of CNY 8.7 trillion, a more than tenfold increase compared to CNY 0.8 trillion in 2007 [1]. As a fundamental carrier for economic activities and industrial layout and a crucial tool for industrial policies [2,3,4,5], land plays a pivotal role in optimizing spatial allocation to facilitate the rational flow of resources and construct a high-quality development layout for the national territory. The fiscal decentralization reform in 1994 strengthened the central government’s control over national fiscal management and granted local governments the authority to develop and gain revenue from land transfers. Under the current land system in China, local governments have absolute control over the primary land supply market [6]. This intertwining of land allocation and local government behavior is driven by the pursuit of financial resources through market-driven land supply, maximizing land transfer revenue to bolster local finances [7,8,9] and promoting industrial competition through preferential supply of industrial land for increased investment and local economic growth [10,11]. This dual land supply strategy, undertaken by local governments in the context of fiscal constraints and the promotion tournament mechanism, is considered a significant factor contributing to China’s economic miracle over the past three decades [12,13].

How does the development competition centered around land management unfold for Chinese local governments? Oman (2000) pointed out that, under the constraints imposed by the central government in China, local governments have limited space to compete for investments through fiscal means [14]. In the absence of financial support, the current land system provides a way for local governments to break free from existing constraints. On one hand, in the situation of scarce domestic capital, local governments can attract external industries with a tendency to relocate by offering industrial land at low prices, thereby increasing local investment. On the other hand, through the bidding and auction market mechanism, local governments can compensate for the loss incurred by low-priced industrial land transfers with high-priced transfers of residential and commercial land. This supports all aspects of local finances, enabling urban expansion and beautification [15,16]. A comprehensive examination of theory and reality suggests that the development competition with land management at its core for Chinese local governments is highly complex. While the pursuit of fiscal revenue constitutes a significant driving force for local government land supply behavior, for local officials within the internal labor market, engaging in industrial competition through the leasing rights granted by the current land system for construction land may be more crucial in promoting local economic growth and increasing the probability of political promotion.

The existing literature generally agrees that land, as the most valuable resource controlled by local governments, has become the primary means for them to acquire off-budget fiscal revenue and engage in industrial competition. Therefore, land supply strategies involve high-priced land transfers using market mechanisms to fill local finances and low-priced land transfers for industrial competition [7,11]. This consensus provides the basic research entry points for this article. However, there are two main shortcomings in existing research. First, most of the existing literature is based on the strict assumption that local governments are homogeneous competitors. Yet, China, as a vast developing country, has economically developed regions on the eastern coast and economically underdeveloped regions in the central and western areas. This reality, where competitors are not entirely homogeneous, prompts a clearer perspective on the land supply behavior among heterogeneous local governments than what the existing literature anticipates. Second, existing research primarily adopts a single perspective of fiscal or growth incentives and uses econometric methods to empirically analyze local government land transfer behavior, with insufficient emphasis on the strategic interactions among local governments. Even though a few studies theoretically consider strategic interactions in local government land supply, they do not specifically address them in empirical models. In light of these gaps, this article fully considers the heterogeneity of Chinese local governments and the existence of strategic interactions in land supply. It incorporates fiscal incentives and growth incentives into the same framework and applies evolutionary game theory and methods to focus on the analysis of local government land supply behavior, aiming to demonstrate a comprehensive form of local government competition in industrial land acquisition. Additionally, it is worth mentioning that the purpose of adopting evolutionary game theory and methods is to move beyond limiting the study to finding strategies against another type of local government, but rather to analyze the regularities of mutual constraints and interactions among heterogeneous local governments in the process of land supply behavior selection.

In conclusion, under the dual influence of fiscal revenue and economic growth incentives, Chinese local governments are no longer just providers of local public services; they are now the leaders and direct beneficiaries of local economic development [17,18,19,20]. Due to the strategy choice of local governments to provide industrial land at low prices for industrial takeover, under the effects of demonstration and incentive effects, this land supply strategy will inevitably affect the land supply behavior choices of neighboring local governments, turning land supply behavior into a game process among local governments. Simultaneously, due to widespread differences among Chinese local governments in economic development foundations, government behavior preferences, and other aspects, land supply behavior of local governments with different characteristics will inevitably differentiate during the interactive game process, presenting a realistic pattern of multiple game equilibria coexisting. Based on this, this article views the land supply behavior among Chinese local governments as a strategic interaction process. Starting from two heterogeneous dimensions—income and preferences—it establishes an evolutionary game model of local government limited rationality and explores the evolutionary process and convergence trend of local government land supply behavior in the new wave of domestic industrial transfer. This paper theoretically reveals the internal logic behind the differing land supply behaviors of Chinese local governments and offers theoretical support and policy recommendations for optimizing the allocation of land resources in China.

The remainder of this paper is structured into four parts. First, it establishes a framework for analyzing the land supply behavior of heterogeneous local governments. Second, it builds an evolutionary game model of this behavior and conducts a thorough analysis of the model’s evolutionary equilibria. Third, it performs a numerical simulation analysis of these equilibria, the heterogeneity of local governments, and other important parameters. The final part presents the conclusions and policy recommendations.

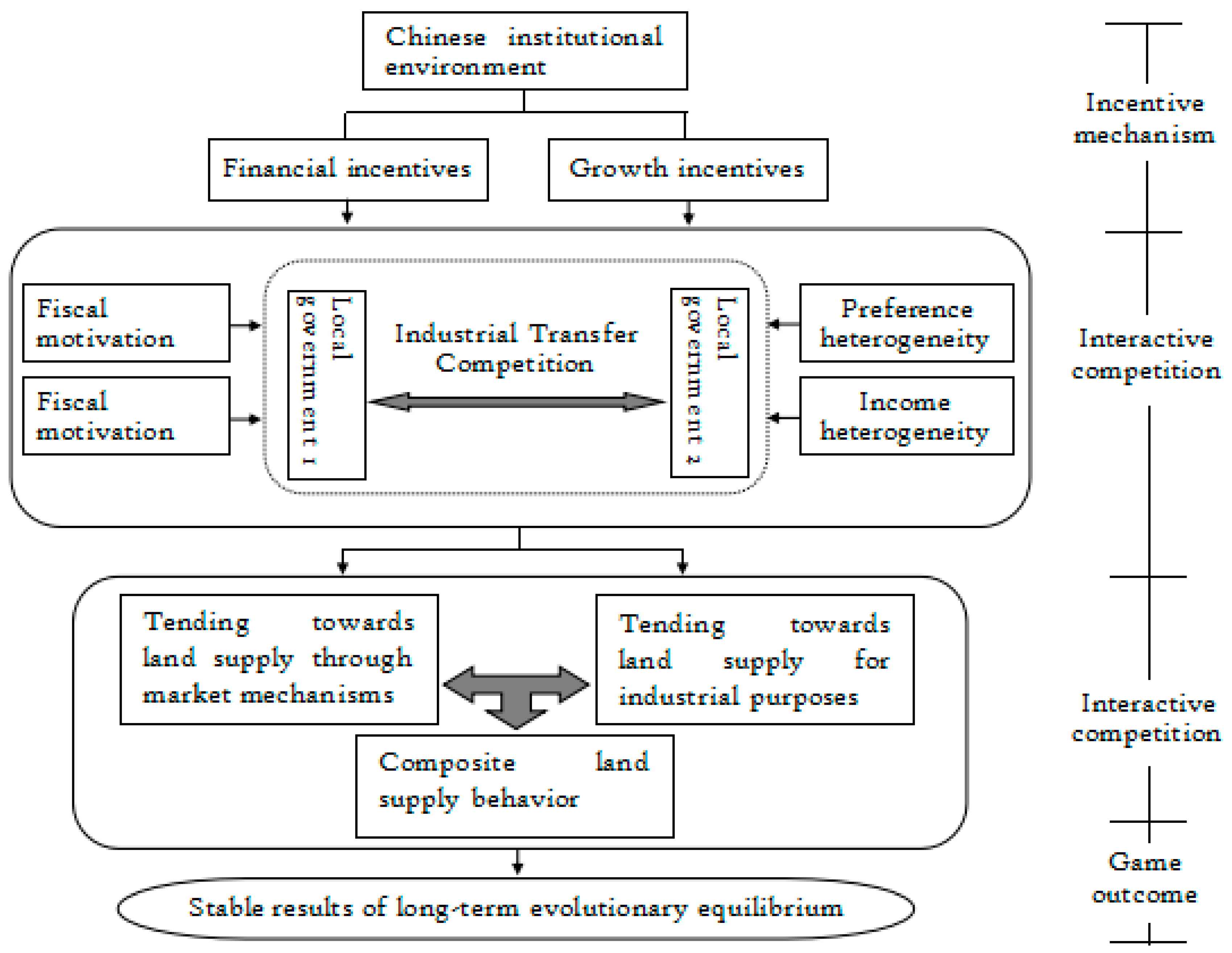
2. Analysis Framework

Heterogeneity is the opposite of homogeneity. In fact, homogeneous rational economic agents lack explanatory power for real public choice and social cooperation issues [21,22,23]. The tournament competition under central political centralization makes local governments highly attentive to the behavior of competitors, and many actions are strategic, complex, and variable [24,25,26,27,28]. Since Olson (1973) pioneeringly pointed out the importance of differences among members in influencing collective action, heterogeneity has been introduced into numerous research fields [29]. This includes the positive and negative effects of organizational member heterogeneity on group performance [30], the impact of member heterogeneity on individual choices of roles such as leaders, followers, and free-riders in organizations [31], and the effects of trader heterogeneity on financial asset prices and complex market behavior [32]. These studies have expanded on homogeneity assumptions, making their conclusions more practically meaningful. Heterogeneous local governments, in contrast to the homogenization assumption in traditional economics when studying government behavior, derive mainly from individual preferences of local governments and the economic base conditions of their regions, such as resource endowments, geographical location, and existing economic levels. It is an initial difference that non-competitive ability or effort can influence [33,34,35]. The existence of heterogeneity may allow local governments with economic advantages to achieve higher economic output performance and higher rankings without making high efforts, leading to victory in competition. This is certainly not what the central government and the public would like to see. Unlike the Western bottom-up competitive mechanism, China’s local government tournament competition is based on the evaluation mechanism of the higher-level government top-down. This institutional system fundamentally serves as a guidepost guiding the choices of local government behavior [36,37,38].

On one hand, under the fiscal system of shared taxes, the serious imbalance of fiscal revenue and expenditure in some local governments in China forces them to seek additional financial sources like land to fill the fiscal gap. This leads to local governments playing the role of economic participants in fiscal and tax matters. Meanwhile, the promotion system based on GDP growth as an assessment criterion encourages local governments to engage in fierce competition around industrial development. At this point, local governments also play the role of political participants in the government arena [39,40,41,42]. Therefore, in the current institutional environment of fiscal and growth incentives in China, as monopolists in the urban land supply market, local governments have the freedom to use land as a tool for industrial competition by adjusting the mechanism of land supply, either according to market mechanisms or by favoring industrial land supply, to meet their fiscal and promotion motives [43,44,45]. In other words, facing a new round of opportunities for domestic industrial transfer, Chinese local governments have the discretion to choose land as a tool for industrial competition, deciding whether to allocate land according to market mechanisms for short-term fiscal income or to undertake industrial transfer to promote long-term local economic growth.

On the other hand, in the socio-economic complex system, there is a widespread binary opposition between developed and underdeveloped regions [46], as well as between dominant and vulnerable groups [47]. Due to historical reasons, the location advantages of developed regions, such as those resulting from reform and opening up, as well as inherent advantages result in higher overall economic development levels for local governments in developed regions, and consequently higher government revenue levels. This gives them greater policy choice space when formulating land supply strategies. In contrast, local governments in underdeveloped regions often face lower overall economic development levels, and their government revenue levels are often stretched. They have to rely more on grabbing measures to promote regional economic growth, making intervention in the land market an important aspect of their grabbing strategies [48]. This gap between local governments is not only a difference in government income but also implies a process of interest bargaining among different groups of local governments regarding land supply. According to the theory of spatial spillover effects, under the influence of information spillover and regional competition [49,50], the land supply behavior of local governments with different income levels will make corresponding strategic adjustments based on changes in land supply behavior at the same level, especially in adjacent regions.

The aforementioned government preference heterogeneity and government revenue heterogeneity constitute important factors that influence the voluntary land supply outcomes of Chinese local governments. This naturally forms a logical framework for explaining local government land supply behavior from the perspectives of incentive mechanisms and government heterogeneity (see Figure 1). Unlike the snapshot-like descriptions given to individuals and their groups in heterogeneity research, heterogeneous local governments, although composed of individual members, have certain financial and administrative powers. They are more influenced by incentive mechanisms of the central government, and their land supply behavior is mainly determined by changing government preferences and varying degrees of government revenue gaps in strategic interactions. From the perspective of preferences, in the competition for industrial transfer, local governments favoring market mechanisms for land supply are more focused on utilizing land factors to increase fiscal revenue, thus are more willing to adopt cooperative strategies in the game. On the other hand, with local governments favoring industrial land supply, although cooperation is beneficial for improving their local fiscal revenue levels, due to greater pressure on local economic growth, they are more likely to focus on the significant role of land factors in undertaking industrial transfers, reducing their willingness to cooperate. From the perspective of income, Olson’s (2010) research indicates that the existence of income differences will reduce the level of collective effort among cooperating members [51]. Combining this with the actual situation in China, cooperative behavior in the land supply process will fully play the role of market mechanisms in land transactions, promoting current land prices, which have been suppressed for a long time, to return to normal levels and generating cooperative benefits. For local governments with higher income levels, due to their relatively large economic scale, higher urbanization levels, and larger populations, they are more likely to share more cooperative benefits. If the income gap between local governments is too large, lower-income local governments will act like the small pig in the game, always choosing a delayed strategy.

In summary, under the current institutional environment in China, heterogeneous local governments in the economic–social system mutually influence and co-evolve, forming a complex and dynamic evolving system. Previous studies on the long-term evolution game between local governments have rarely focused on the important roles played by local government preferences, income, and other factors in the game process. This paper, by introducing factors of heterogeneity, aligns the game process among local governments more closely with the actual conditions in China.

3. Methods

3.1. Basic Model Assumptions

Combining the previous analysis, to simplify the game model maximally for ease of study, the following assumptions are proposed:

(1)

Participating Entities: The participating entities in the game are Local Government 1 and Local Government 2. Both parties are groups of bounded rationality during the game, implying that it is challenging to confirm whether their behavioral choices directly lead to maximized effects. Instead, they follow a dynamic logic of “exploration, learning, adaptation, and growth” in their respective decision-making processes.

(2)

Game Strategies: Heterogeneous local governments adopt two types of behavioral strategies in the game competition for industrial undertakings based on land supply—cooperation and non-cooperation. Non-cooperation is reflected in the tendency of local governments to prioritize industrial land for the sake of regional economic growth when choosing land supply strategies. Cooperation, on the other hand, means that local governments supply land based on market mechanisms, not influencing enterprise relocation decisions based on land supply.

(3)

Cost–Benefit Parameters: When both parties adopt a cooperation strategy, the total revenue consists of land transfer fees, ancillary tax revenue from land transfer, and direct and indirect effects of industrial undertaking, denoted as $\mathrm{R}$. Assuming the allocation coefficients for Local Government 1 and Local Government 2 are $\mathsf{\alpha }$ and $1-\mathsf{\alpha }$, respectively, their revenues are $\mathsf{\alpha }\mathrm{R}$ and ($1-\mathsf{\alpha })\mathrm{R}$. If either local government adopts a non-cooperation strategy, it leads to a certain loss, $\mathrm{V}$, in total revenue. This loss is directly proportional to the preference for industrial land undertaking, denoted as ${\mathsf{\beta }}_{\mathrm{i}}\mathrm{V}$($\mathrm{i}=1,2$). In the context of the accelerated industrial transfer within China, assume the total growth effect brought by industrial transfer to the recipient local government is $\mathrm{A}$. Assuming that Local Government 1 and Local Government 2 have preference factors for competing in industrial takeover, denoted as ${\mathsf{\beta }}_1$ and ${\mathsf{\beta }}_2$, their growth effects generated through industrial takeover are ${\mathsf{\beta }}_1\mathrm{A}$ and ${\mathsf{\beta }}_2\mathrm{A}$, respectively. The stronger the preference of a local government for industrial land, the higher the growth effect it obtains. Let ${\mathrm{W}}_{\mathrm{i}}$($\mathrm{i}=1,2$) represent the financial status of a local government. The party not participating in cooperation compensates the cooperating party for the losses incurred due to its opportunistic behavior. The compensation amount is denoted as ${\mathrm{k}\mathrm{W}}_{\mathrm{i}}$, where $\mathrm{k}$ serves as both the compensation ratio and the intensity of the penalty, akin to a punishment for non-cooperative behavior.

The assumptions and their definitions for the relevant parameters are presented in

Table 1. Relevant parameters and definitions.

Parameter Assumption	Definition
$R$	Total cooperative benefits resulting from mutual cooperation.
$\alpha$	Proportion of total cooperative benefits shared by Local Government 1.
$V$	Loss incurred by local government when not cooperating.
$A$	Overall growth effect brought to the recipient local government by industrial transfer.
${\beta }_1$	Preference factor for non-cooperation of Local Government 1.
${\beta }_2$	Preference factor for non-cooperation of Local Government 2.
$W_1$	Financial status of Local Government 1.
$W_2$	Financial status of Local Government 2.
$k$	Compensation factor given by the non-cooperating party to the cooperating party.

.

Building upon these assumptions, let us further assume that $\mathrm{x}$ and $1-\mathrm{x}$ represent the probabilities of Local Government 1 choosing cooperation and non-cooperation strategies, respectively. Similarly, $\mathrm{y}$ and $1-\mathrm{y}$ represent the probabilities of Local Government 2 choosing cooperation and non-cooperation strategies, respectively. It is noteworthy that, as participants form a collective concept, the probability of individual participants choosing a specific strategy in this paper can also be considered as the proportion of participants within the group opting for that strategy. Based on these fundamental assumptions, the payoff matrix for the evolutionary game between Local Government 1 and Local Government 2 is illustrated in

Table 2. Evolutionary game payoff matrix for land supply strategies between local governments.

Strategic Choices		Local Government 2 Cooperation y	Local Government 2 Non-Cooperation 1 − y
Local Government 1	Cooperation $x$	$\alpha R$	${\alpha (R-\beta }_{2}V)+k{W}_2$
		$(1-\alpha )R$	${(1-\alpha )(R-\beta }_{2}V)-k{W}_2+{\beta }_{2}A$
	Non-cooperation $1-x$	${\alpha (R-\beta }_{1}V)-k{W}_1+{\alpha }_{1}A$	${\alpha (R-\beta }_{1}V-{\beta }_{2}V)+{\beta }_{1}A$
		${(1-\alpha )(R-\beta }_{1}V)+k{W}_1$	${(1-\alpha )(R-\beta }_{1}V-{\beta }_{2}V)+{\beta }_{2}A$

.

3.2. Copying Dynamic Equations

Based on the payoff matrix in Table 2 for the evolutionary game between Local Government 1 and Local Government 2, we can derive the following:

When Local Government 1 chooses the cooperation strategy, the expected payoff is denoted as ${\mathrm{E}}_1^{\mathrm{a}}$, expressed as:

$$E_1^a=\mathrm{y}\mathsf{\alpha }\mathrm{R}+(1-\mathrm{y})\left[{\mathsf{\alpha }(\mathrm{R}-\mathsf{\beta }}_2\mathrm{V}\right)+\mathrm{k}{\mathrm{W}}_2]$$

(1)

When Local Government 1 chooses the non-cooperation strategy, the expected payoff is denoted as ${\mathrm{E}}_1^{\mathrm{b}}$, expressed as:

$$E_1^b=\mathrm{y}\left[{\mathsf{\alpha }(\mathrm{R}-\mathsf{\beta }}_1\mathrm{V}\right)-\mathrm{k}{\mathrm{W}}_1+{\mathsf{\alpha }}_1\mathrm{A}]+(1-\mathrm{y}\left)\right[{\mathsf{\alpha }(\mathrm{R}-\mathsf{\beta }}_1\mathrm{V}-{\mathsf{\beta }}_2\mathrm{V})+{\mathsf{\beta }}_1\mathrm{A}]$$

(2)

Based on the above two equations, the expression for the average expected payoff $\overline{{\mathrm{E}}_1}$ for Local Government 1 is as follows:

$$\overline{E_1}=x{E}_1^a+(1-x)E_1^b$$

(3)

Therefore, the copying dynamic equation for the probability of Local Government 1 choosing the cooperation strategy is as follows:

$$F\left(x\right)=dx/dt=x(E_1^a-\overline{E_1})=x(1-x)(k{W}_2-{\beta }_{1}A+\alpha {\beta }_{1}V+k{W}_{1}y-k{W}_{2}y)$$

(4)

Similarly, the copying dynamic equation for the probability of Local Government 2 choosing the cooperation strategy is as follows:

$$F\left(y\right)=dy/dt=y(E_2^a-\overline{E_2})=y(y-1)({\beta }_{2}A-{\beta }_{2}V-k{W}_1+\alpha {\beta }_{2}V+k{W}_{1}x-k{W}_{2}x)$$

(5)

Here, ${\mathrm{E}}_2^{\mathrm{a}}$ and $\overline{{\mathrm{E}}_2}$ represent the expected payoff and average expected payoff, respectively, when Local Government 2 chooses the cooperation strategy.

3.3. Stability Analysis of the Model

Based on the previous analysis, the equilibrium solutions of the evolutionary game model between Local Government 1 and Local Government 2 can be analyzed through a two-dimensional dynamic system formed by their copying dynamic equations [52]. Let $\mathrm{F}\left(\mathrm{x}\right)=\mathrm{F}\left(\mathrm{y}\right)=0$, solving this system of equations leads to four pure-strategy equilibrium solutions, ${\mathrm{E}}_1\left(\mathrm{0,0}\right)$, ${\mathrm{E}}_2\left(\mathrm{1,0}\right)$, ${\mathrm{E}}_3\left(\mathrm{0,1}\right)$, and ${\mathrm{E}}_4\left(\mathrm{1,1}\right)$, and one mixed strategy solution, ${\mathrm{E}}_5({\mathrm{x}}^{\mathrm{*}},{\mathrm{y}}^{\mathrm{*}})$, where ${\mathrm{x}}^{\mathrm{*}}=\frac{{\mathsf{\beta }}_2\mathrm{A}-{\mathsf{\beta }}_2\mathrm{V}-\mathrm{k}{\mathrm{W}}_1+\mathsf{\alpha }{\mathsf{\beta }}_2\mathrm{V}}{\mathrm{k}{(\mathrm{W}}_2-{\mathrm{W}}_1)} \mathrm{a}\mathrm{n}\mathrm{d} {\mathrm{y}}^{\mathrm{*}}=\frac{\mathrm{k}{\mathrm{W}}_2-{\mathsf{\beta }}_1\mathrm{A}+\mathsf{\alpha }{\mathsf{\beta }}_1\mathrm{V}}{\mathrm{k}{(\mathrm{W}}_2-{\mathrm{W}}_1)}$.

However, these five equilibrium points are not necessarily evolutionarily stable strategies for the system. According to the method proposed by Friedman (1991), the evolutionary stability of a two-dimensional dynamic system can be deduced through a local stability analysis of the system’s Jacobian matrix [53]. To achieve this, partial derivatives of $\mathrm{F}\left(\mathrm{x}\right)$ and $\mathrm{F}\left(\mathrm{y}\right)$ are taken to obtain the Jacobian matrix $\mathrm{J}$:

$$J=\left[\begin{array}{cc}\frac{\partial F\left(x\right)}{\partial x}& \frac{\partial F\left(x\right)}{\partial y}\\ \frac{\partial F\left(y\right)}{\partial x}& \frac{\partial F\left(y\right)}{\partial y}\end{array}\right]=\left[\begin{array}{cc}{F}_{11}& F_{12}\\ F_{21}& F_{22}\end{array}\right]$$

(6)

where

$$F_{11}=(1-2x)(k{W}_2-{\beta }_{1}A+\alpha {\beta }_{1}V+k{W}_{1}y-k{W}_{2}y)$$

(7)

$$F_{12}=x(1-x)(k{W}_1-k{W}_2)$$

(8)

$$F_{21}=y(y-1)(k{W}_1-k{W}_2)$$

(9)

$$F_{22}=(2y-1)({\beta }_{2}A-{\beta }_{2}V-k{W}_1+\alpha {\beta }_{2}V+k{W}_{1}x-k{W}_{2}x)$$

(10)

Simultaneously, the trace $\mathrm{t}\mathrm{r}\mathrm{J}$ and determinant $\mathrm{d}\mathrm{e}\mathrm{t}\mathrm{J}$ of the Jacobian matrix are given by the following:

$$\begin{array}{cc}trJ=F_{11}+F_{22}& =(1-2x)(k{W}_2-{\beta }_{1}A+\alpha {\beta }_{1}V+k{W}_{1}y-k{W}_{2}y)+(2y-1)({\beta }_{2}A-{\beta }_{2}V-k{W}_1+\alpha {\beta }_{2}V+k{W}_{1}x\hfill \\ & -k{W}_{2}x)\hfill \end{array}$$

(11)

$$\begin{array}{cc}detJ=F_{11}\ast F_{22}& -F_{12}\ast F_{21}\hfill \\ & =(1-2x)(k{W}_2-{\beta }_{1}A+\alpha {\beta }_{1}V+k{W}_{1}y-k{W}_{2}y)(2y-1)({\beta }_{2}A-{\beta }_{2}V-k{W}_1+\alpha {\beta }_{2}V+k{W}_{1}x\hfill \\ & -k{W}_{2}x)-x(1-x\left)\right(k{W}_1-k{W}_2\left)y\right(y-1\left)\right(k{W}_1-k{W}_2)\hfill \end{array}$$

(12)

When the trace condition $\mathrm{t}\mathrm{r}\mathrm{J}<0$ and the determinant condition $\mathrm{d}\mathrm{e}\mathrm{t}\mathrm{J}>0$ are satisfied, it can be determined that the equilibrium solutions of the two-dimensional dynamic system correspond to evolutionarily stable strategies. Further inference from the trace and determinant conditions indicates that the equilibrium solutions for evolutionarily stable strategies must satisfy ${\mathrm{F}}_{11}<0$ and ${\mathrm{F}}_{22}<0$. By further solving the Jacobian matrix $\mathrm{J}$, the values of the five local equilibrium solutions at ${\mathrm{F}}_{11}$, ${\mathrm{F}}_{12}$, ${\mathrm{F}}_{21}$, and ${\mathrm{F}}_{22}$ are obtained, as shown in

Table 3. Specific values of local equilibrium solutions at ${\mathrm{F}}_{11}$, ${\mathrm{F}}_{12}$, ${\mathrm{F}}_{21}$, and ${\mathrm{F}}_{22}$.

Equilibrium Solution	${\mathbf{F}}_{11}$	${\mathbf{F}}_{12}$	${\mathbf{F}}_{21}$	${\mathbf{F}}_{22}$
$E_1\left(\mathrm{0,0}\right)$	$\alpha {\beta }_{1}V+k{W}_2-{\beta }_{1}A$	0	0	${\beta }_{2}V+k{W}_1-{\beta }_{2}A-\alpha {\beta }_{2}V$
$E_2\left(\mathrm{1,0}\right)$	${\beta }_{1}A-k{W}_2-\alpha {\beta }_{1}V$	0	0	${\beta }_{2}V+k{W}_2-{\beta }_{2}A-\alpha {\beta }_{2}V$
$E_3\left(\mathrm{0,1}\right)$	$\alpha {\beta }_{1}V+k{W}_1-{\beta }_{1}A$	0	0	${{\beta }_{2}A+\alpha {\beta }_{2}V-\beta }_{2}V-k{W}_1$
$E_4\left(\mathrm{1,1}\right)$	${\beta }_{1}A-k{W}_1-\alpha {\beta }_{1}V$	0	0	${{\beta }_{2}A+\alpha {\beta }_{2}V-\beta }_{2}V-k{W}_2$
$E_5(x^{*},y^{*})$	0	$M$	$N$	0

.

From Table 3, it can be observed that ${\mathrm{E}}_5({\mathrm{x}}^{\mathrm{*}},{\mathrm{y}}^{\mathrm{*}})$ has values of 0 at both ${\mathrm{F}}_{11}$ and ${\mathrm{F}}_{22}$, which clearly does not satisfy the conditions $\mathrm{t}\mathrm{r}\mathrm{J}<0$ and $\mathrm{d}\mathrm{e}\mathrm{t}\mathrm{J}>0$. Therefore, this point is not a stable point for the evolution of the system’s strategy. The stability of the other four pure-strategy equilibrium solutions can be discussed in the following four specific cases.

(1)

Scenario One: When the conditions ${\mathsf{\beta }}_1\mathrm{A}-\mathsf{\alpha }{\mathsf{\beta }}_1\mathrm{V}>\mathrm{k}{\mathrm{W}}_2$ and ${\mathsf{\beta }}_2\mathrm{A}-(1-\mathsf{\alpha }){\mathsf{\beta }}_2\mathrm{V}>\mathrm{k}{\mathrm{W}}_1$ are satisfied, the stability of the four pure-strategy equilibrium solutions is as shown in

Table 4. Analysis of evolutionarily stable strategy equilibrium points.

Equilibrium Solution	${\mathit{F}}_{11}$	${\mathit{F}}_{22}$	$\mathit{t}\mathit{r}\mathit{J}$	$\mathit{d}\mathit{e}\mathit{t}\mathit{J}$	Stability
$E_1\left(\mathrm{0,0}\right)$	$-$	$-$	$-$	$+$	$ESS$ equilibrium point
$E_2\left(\mathrm{1,0}\right)$	$+$	Uncertain	Uncertain	Uncertain	Unstable point
$E_3\left(\mathrm{0,1}\right)$	Uncertain	$+$	Uncertain	Uncertain	Unstable point
$E_4\left(\mathrm{1,1}\right)$	Uncertain	Uncertain	Uncertain	Uncertain	Saddle point

Note: Unstable point indicates a point that cannot evolve into an equilibrium point under any circumstances. Saddle point indicates a point that can evolve into an equilibrium point under certain specific conditions, likewise for the following.

.

From Table 4, it can be observed that the evolutionarily stable strategy point for the game between the two local governments is ${\mathrm{E}}_1\left(\mathrm{0,0}\right)$. This signifies a non-cooperation strategy where both local governments choose to favor industrial land supply for industrial takeover competition. For any local government, if its growth preference is strong, it will not adhere to the market mechanism of land supply and demand but will opt for the non-cooperation strategy, favoring industrial land supply. Even for a local government with a relatively weak growth preference, if the benefits from industrial takeover competition outweigh the losses it incurs and are greater than the compensation for the losses of the other party, it will still choose the non-cooperation strategy favoring industrial land supply. In this scenario, both players in the game have heterogeneous preferences satisfying ${\mathsf{\beta }}_1>\frac{{\mathrm{k}\mathrm{W}}_2}{\mathrm{A}-\mathsf{\alpha }\mathrm{V}}$ and ${\mathsf{\beta }}_2>\frac{{\mathrm{k}\mathrm{W}}_1}{\mathrm{A}-(1-\mathsf{\alpha })\mathrm{V}}$, and income heterogeneity satisfies ${\mathrm{W}}_1<\frac{{\mathsf{\beta }}_2[\mathrm{A}-(1-\mathsf{\alpha }\left)\mathrm{V}\right]}{\mathrm{k}}$ and ${\mathrm{W}}_2<\frac{{\mathsf{\beta }}_1(\mathrm{A}-\mathsf{\alpha }\mathrm{V})}{\mathrm{k}}$. This indicates that both players in the game have growth preferences stronger than a certain threshold, while income heterogeneity is strictly confined within a specific threshold by the industrial takeover. Therefore, once the growth preferences of both sides exceed a certain threshold, both will choose a land supply policy favoring industrial land for industrial takeover competition. An example of this situation is the industrial takeover competition among provinces in the central region of China.

According to Table 4, the evolutionarily stable strategy points in the game between the two local governments are when both choose the non-cooperation strategy favoring industrial land supply for industrial takeover competition. For either local government, if its preference for growth is strong, it will not adhere to the market mechanism of land supply and demand but will choose the non-cooperation strategy favoring industrial land supply. Even for a local government with a relatively weak preference for growth, if the benefits from industrial takeover competition outweigh the losses after offsetting its own losses, it will still choose the non-cooperation strategy favoring industrial land supply. In this case, both sides of the game have heterogeneous preference satisfaction in terms of growth and income, indicating that both sides have a growth preference stronger than a certain threshold, while the heterogeneity in income is strictly limited within a certain threshold by industrial takeover.

Therefore, when the growth preference of both sides surpasses a certain threshold and the heterogeneity in government income is strictly limited within a certain threshold by industrial takeover, both sides will choose the non-cooperation strategy favoring industrial land supply for industrial takeover competition. An example of this situation is the industrial takeover competition among provinces in the central region of China.

Based on this, Proposition 1 can be formulated as follows:

Proposition 1. 

When the growth preference of both sides in the game is greater than a certain threshold, and the heterogeneity in government income is strictly limited within a certain threshold by industrial takeover, both sides will choose the non-cooperation strategy.

(2)

Scenario Two: The stability of the four pure-strategy equilibrium solutions is shown in

Table 5. Evolutionarily stable strategy equilibrium point analysis.

Equilibrium Solution	${\mathit{F}}_{11}$	${\mathit{F}}_{22}$	$\mathit{t}\mathit{r}\mathit{J}$	$\mathit{d}\mathit{e}\mathit{t}\mathit{J}$	Stability
$E_1\left(\mathrm{0,0}\right)$	$+$	Uncertain	Uncertain	Uncertain	Unstable point
$E_2\left(\mathrm{1,0}\right)$	$-$	$-$	$-$	$+$	$ESS$ equilibrium point
$E_3\left(\mathrm{0,1}\right)$	Uncertain	Uncertain	Uncertain	Uncertain	Unstable point
$E_4\left(\mathrm{1,1}\right)$	Uncertain	$+$	Uncertain	Uncertain	Unstable point

when ${\mathsf{\beta }}_1\mathrm{A}-\mathsf{\alpha }{\mathsf{\beta }}_1\mathrm{V}<\mathrm{k}{\mathrm{W}}_2$ and ${\mathsf{\beta }}_2\mathrm{A}-(1-\mathsf{\alpha }){\mathsf{\beta }}_2\mathrm{V}-\mathrm{k}{\mathrm{W}}_2>0$.

From Table 5, it can be observed that the evolutionarily stable strategy point in the game between the two local governments is ${\mathrm{E}}_2\left(\mathrm{1,0}\right)$. In this scenario, Local Government 1 chooses the cooperation strategy of freely supplying residential and industrial land according to market mechanisms, while Local Government 2 chooses the non-cooperation strategy, leaning towards industrial land. For Local Government 1, the compensation provided by the other party outweighs the benefits of choosing to compete for industrial land, so it will choose the cooperative strategy of supplying land according to market mechanisms. On the other hand, for Local Government 2, the benefits of adopting a strategy favoring industrial land competition, after subtracting the losses generated by this strategy and the compensation given to the other party, still result in a positive income. Therefore, it will choose the non-cooperation strategy.

In this situation, both parties in the game prefer heterogeneity, satisfying ${\mathsf{\beta }}_1<\frac{{\mathrm{k}\mathrm{W}}_2}{\mathrm{A}-\mathsf{\alpha }\mathrm{V}}$ and ${\mathsf{\beta }}_2>\frac{{\mathrm{k}\mathrm{W}}_2}{\mathrm{A}-(1-\mathsf{\alpha })\mathrm{V}}$. The income of Local Government 2 satisfies ${\frac{{\mathsf{\beta }}_1(\mathrm{A}-\mathsf{\alpha }\mathrm{V})}{\mathrm{k}}<\mathrm{W}}_2<\frac{{\mathsf{\beta }}_2[\mathrm{A}-(1-\mathsf{\alpha }\left)\mathrm{V}\right]}{\mathrm{k}}$, indicating that the growth preferences of both parties are constrained by two threshold values in opposite directions. The party constrained by the positive threshold will have its income strictly limited within a certain range by industrial takeover. Therefore, regardless of the strategy chosen by the other party, the non-cooperation strategy of favoring industrial land supply for industrial takeover competition will be the dominant strategy. Through long-term game evolution, the other party will effectively identify this dominant strategy, and considering maximizing its own interests, it will eventually abandon the non-cooperation strategy of competition and choose the cooperation strategy of freely supplying residential and industrial land according to market mechanisms. An example of this situation is the industrial takeover competition between developed central regions and underdeveloped peripheral areas in some provinces in central and western China.

Thus, Proposition 2 can be formulated as follows:

Proposition 2. 

When the growth preferences of both parties in the game are constrained by two threshold values in opposite directions, and the government income of the party constrained by the positive threshold is strictly limited within a certain range by industrial takeover, the party with growth preferences constrained by the positive threshold will choose the non-cooperation strategy, while the party with growth preferences constrained by the negative threshold will choose the cooperation strategy.

(3)

Scenario Three: When ${\mathsf{\beta }}_1\mathrm{A}-\mathsf{\alpha }{\mathsf{\beta }}_1\mathrm{V}-\mathrm{k}{\mathrm{W}}_1>0$ and $\mathrm{k}{\mathrm{W}}_1>{\mathsf{\beta }}_2\mathrm{A}-(1-\mathsf{\alpha }){\mathsf{\beta }}_2\mathrm{V}$, the stability of the four pure-strategy equilibrium solutions is as shown in

Table 6. Evolutionarily stable strategy equilibrium point analysis.

Equilibrium Solution	${\mathit{F}}_{11}$	${\mathit{F}}_{22}$	$\mathit{t}\mathit{r}\mathit{J}$	$\mathit{d}\mathit{e}\mathit{t}\mathit{J}$	Stability
$E_1\left(\mathrm{0,0}\right)$	Uncertain	$+$	Uncertain	Uncertain	Unstable point
$E_2\left(\mathrm{1,0}\right)$	Uncertain	Uncertain	Uncertain	Uncertain	Saddle point
$E_3\left(\mathrm{0,1}\right)$	$-$	$-$	$-$	$+$	$ESS$ equilibrium point
$E_4\left(\mathrm{1,1}\right)$	$+$	Uncertain	Uncertain	Uncertain	Unstable point

.

From Table 6, it can be observed that the evolutionarily stable strategy point in the game between the two local governments is ${\mathrm{E}}_3\left(\mathrm{0,1}\right)$. In other words, Local Government 1 chooses the non-cooperation strategy, leaning towards industrial land supply, while Local Government 2 opts for the cooperation strategy of supplying land according to market mechanisms. For Local Government 1, the benefits from adopting a strategy favoring industrial land for industrial development, after deducting the losses incurred by this strategy and compensating the other party, still result in positive net gains. Therefore, it chooses the non-cooperation strategy. On the other hand, for Local Government 2, the rational choice is to adopt the cooperation strategy of supplying land according to market mechanisms because the loss compensation offered by the other party exceeds the benefits of choosing to compete for industrial land development. In this scenario, both players in the game exhibit heterogeneous preferences, satisfying ${\mathsf{\beta }}_1>\frac{{\mathrm{k}\mathrm{W}}_1}{\mathrm{A}-\mathsf{\alpha }\mathrm{V}}$ and ${\mathsf{\beta }}_2<\frac{{\mathrm{k}\mathrm{W}}_1}{\mathrm{A}-(1-\mathsf{\alpha })\mathrm{V}}$. The income of Local Government 1 meets the condition ${\frac{{\mathsf{\beta }}_2[\mathrm{A}-(1-\mathsf{\alpha }\left)\mathrm{V}\right]}{\mathrm{k}}<\mathrm{W}}_1<\frac{{\mathsf{\beta }}_1(\mathrm{A}-\mathsf{\alpha }\mathrm{V})}{\mathrm{k}}$. This indicates that the growth preferences of both players in the game are constrained by two threshold values in opposite directions. Moreover, the income of the player constrained in the positive direction is strictly limited within a certain range by the industrial development activities. This symmetry contrasts with the situation described in the second scenario.

(4)

Scenario Four: When ${\mathsf{\beta }}_1\mathrm{A}-\mathrm{k}{\mathrm{W}}_1-\mathsf{\alpha }{\mathsf{\beta }}_1\mathrm{V}<0$ and ${\mathsf{\beta }}_2\mathrm{A}-(1-\mathsf{\alpha }){\mathsf{\beta }}_2\mathrm{V}-\mathrm{k}{\mathrm{W}}_2<0$, the stability of the four pure-strategy equilibrium solutions is presented in

Table 7. Evolutionarily stable strategy equilibrium point analysis.

Equilibrium Solution	${\mathbf{F}}_{11}$	${\mathbf{F}}_{22}$	$\mathbf{t}\mathbf{r}\mathbf{J}$	$\mathbf{d}\mathbf{e}\mathbf{t}\mathbf{J}$	Stability
${\mathrm{E}}_1\left(\mathrm{0,0}\right)$	Uncertain	Uncertain	Uncertain	Uncertain	Saddle point
${\mathrm{E}}_2\left(\mathrm{1,0}\right)$	Uncertain	$+$	Uncertain	Uncertain	Unstable point
${\mathrm{E}}_3\left(\mathrm{0,1}\right)$	$+$	Uncertain	Uncertain	Uncertain	Unstable point
${\mathrm{E}}_4\left(\mathrm{1,1}\right)$	$-$	$-$	$-$	$+$	$ESS$ equilibrium point

.

From Table 7, it can be observed that the evolutionarily stable strategy point in the game between the two local governments is ${\mathrm{E}}_4\left(\mathrm{1,1}\right)$, meaning both local governments choose the cooperation strategy of supplying land according to market mechanisms. For either player in the game, adopting the non-cooperation strategy favoring industrial land for industrial development results in a growth effect that, after offsetting losses and compensating the other party, is less than zero. Therefore, the rational choice for both players is to adopt the cooperation strategy of supplying land according to market mechanisms. In this scenario, both players in the game exhibit heterogeneous preferences, satisfying ${\mathsf{\beta }}_1<\frac{{\mathrm{k}\mathrm{W}}_1}{\mathrm{A}-\mathsf{\alpha }\mathrm{V}}$ and ${\mathsf{\beta }}_2<\frac{{\mathrm{k}\mathrm{W}}_2}{\mathrm{A}-(1-\mathsf{\alpha })\mathrm{V}}$. The income heterogeneity is characterized by ${\mathrm{W}}_1>\frac{{\mathsf{\beta }}_1(\mathrm{A}-\mathsf{\alpha }\mathrm{V})}{\mathrm{k}}$ and ${\mathrm{W}}_2>\frac{{\mathsf{\beta }}_2[\mathrm{A}-(1-\mathsf{\alpha }\left)\mathrm{V}\right]}{\mathrm{k}}$. This indicates that both players in the game have government incomes exceeding a certain threshold, and the gap in heterogeneous preferences is strictly confined within a specific threshold. Consequently, a compensation system linked to government income will eliminate the intrinsic motivation for both sides to engage in industrial land competition. The cooperative relationship between the two sides is thus very stable. For example, industrial land competition among some developed cities in the eastern coastal areas of China is relatively uncommon. Therefore, Proposition 3 can be stated as follows:

Proposition 3. 

When the government income of both players in the game exceeds a certain threshold, and the growth preference is strictly confined within a specific threshold, both sides will choose the cooperation strategy.

4. Simulation Analysis

To provide a more concrete illustration of the land supply behavior of heterogeneous local governments in the competition for industrial transfer, this study utilizes Matlab 2018a software to conduct simulation analysis on the evolutionary game model.

4.1. Simulation Analysis of System Evolutionary Equilibrium

The relevant parameters are assigned values satisfying the conditions of the equilibrium points, as specified in

Table 8. Evolutionarily stable strategy equilibrium point analysis.

Equilibrium Solution	$\mathsf{\alpha }$	$\mathbf{V}$	$\mathbf{A}$	${\mathsf{\beta }}_1$	${\mathsf{\beta }}_2$	${\mathbf{W}}_1$	${\mathbf{W}}_2$	$\mathbf{k}$
${\mathrm{E}}_1\left(\mathrm{0,0}\right)$	0.5	20	60	0.3	0.4	70	50	0.2
${\mathrm{E}}_2\left(\mathrm{1,0}\right)$	0.5	20	60	0.1	0.6	70	50	0.2
${\mathrm{E}}_3\left(\mathrm{0,1}\right)$	0.5	20	60	0.6	0.1	70	50	0.2
${\mathrm{E}}_4\left(\mathrm{1,1}\right)$	0.5	20	60	0.05	0.15	$70$	$50$	$0.2$

.

Based on the assigned values in Table 8, the equilibrium points ${\mathrm{E}}_1\left(\mathrm{0,0}\right)$, ${\mathrm{E}}_2\left(\mathrm{1,0}\right)$, ${\mathrm{E}}_3\left(\mathrm{0,1}\right)$, and ${\mathrm{E}}_4\left(\mathrm{1,1}\right)$ correspond to Figure 2a, Figure 2b, Figure 2c, and Figure 2d, respectively. As observed from the figure, as long as the parameter values satisfy the conditions of the equilibrium points, regardless of the initial strategy choices, the final strategy choices of both players in the game will converge towards the equilibrium points.

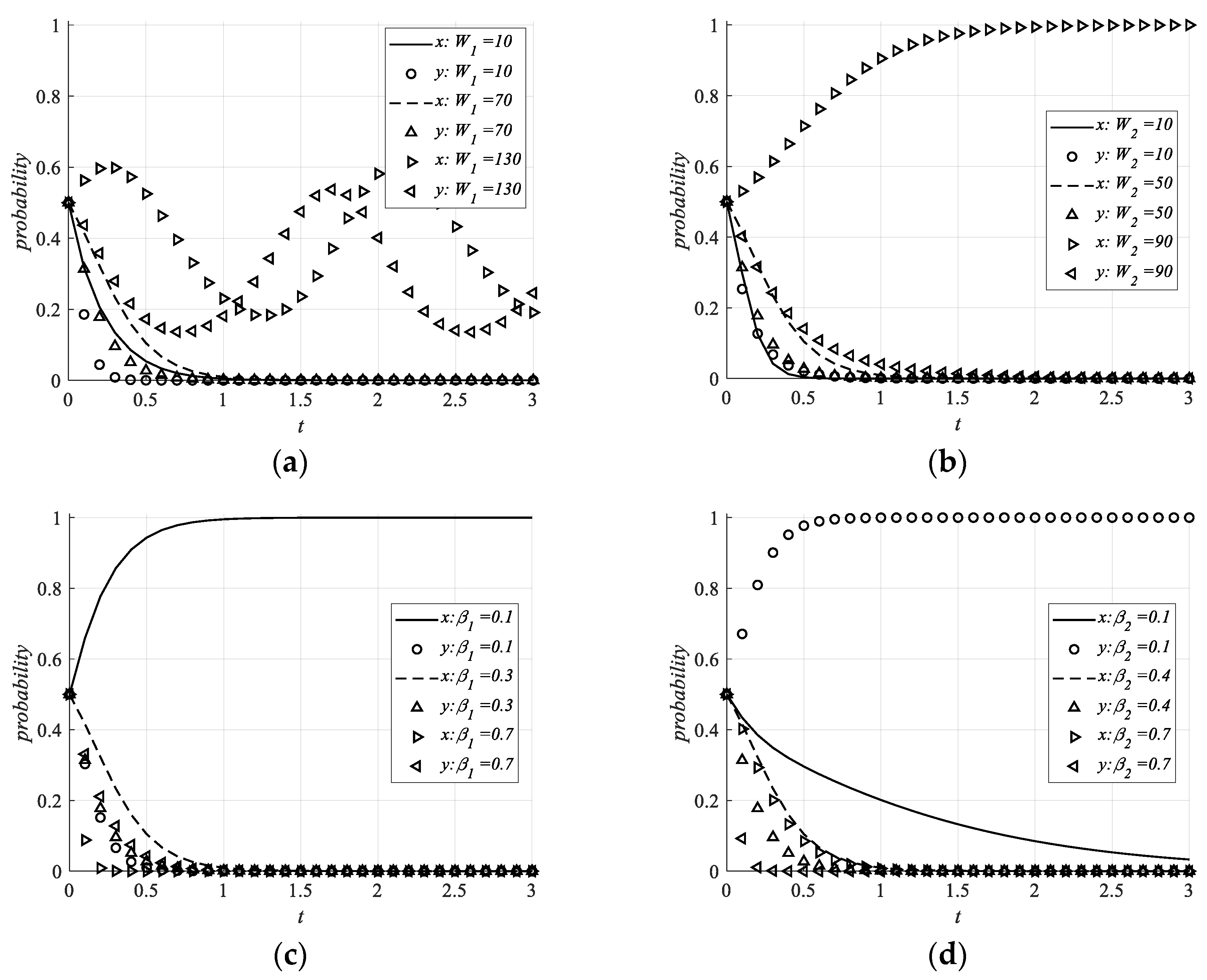
4.2. Simulation of the Impact of Government Heterogeneity on Industrial Transfer Competition Behavior

Figure 2a illustrates the scenario where governments engage in industrial transfer competition. In order to explore the evolutionary behavior of heterogeneous local governments in industrial transfer competition, this study takes $\mathsf{\alpha }=0.5$, $\mathrm{V}=20$, $\mathrm{A}=60$, ${\mathsf{\beta }}_1=0.3$, ${\mathsf{\beta }}_2=0.4$, ${\mathrm{W}}_1=70$, ${\mathrm{W}}_2=50$, and $\mathrm{k}=0.2$ as the base set of parameter values. The impact of income heterogeneity and preference heterogeneity on the behavior of local governments in industrial transfer competition is then investigated. When other parameters remain constant, ${\mathrm{W}}_1$ is assigned values of 10, 70, and 130 to obtain Figure 3a, and ${\mathrm{W}}_2$ is assigned values of 10, 50, and 90 to obtain Figure 3b. Similarly, ${\mathsf{\beta }}_1$ is assigned values of 0.1, 0.3, and 0.7 to obtain Figure 3c, and ${\mathsf{\beta }}_2$ is assigned values of 0.1, 0.3, and 0.7 to obtain Figure 3d.

From Figure 3a,b, it can be observed that in the presence of preference heterogeneity, as the income of the government with lower preference increases, the probability of choosing the land supply behavior strategy for both sides will regularly fluctuate within the (0, 1) interval. However, as the income of the government with higher preference increases, the government with lower preference will evolve from non-cooperative behavior to cooperative behavior, while the government with higher preference continues its original non-cooperative behavior. From Figure 3c,d, it can be seen that a decrease in the preference of either party in the game will lead to the evolution of its own land supply behavior from non-cooperation to cooperation, while the behavior of the other party remains unaffected.

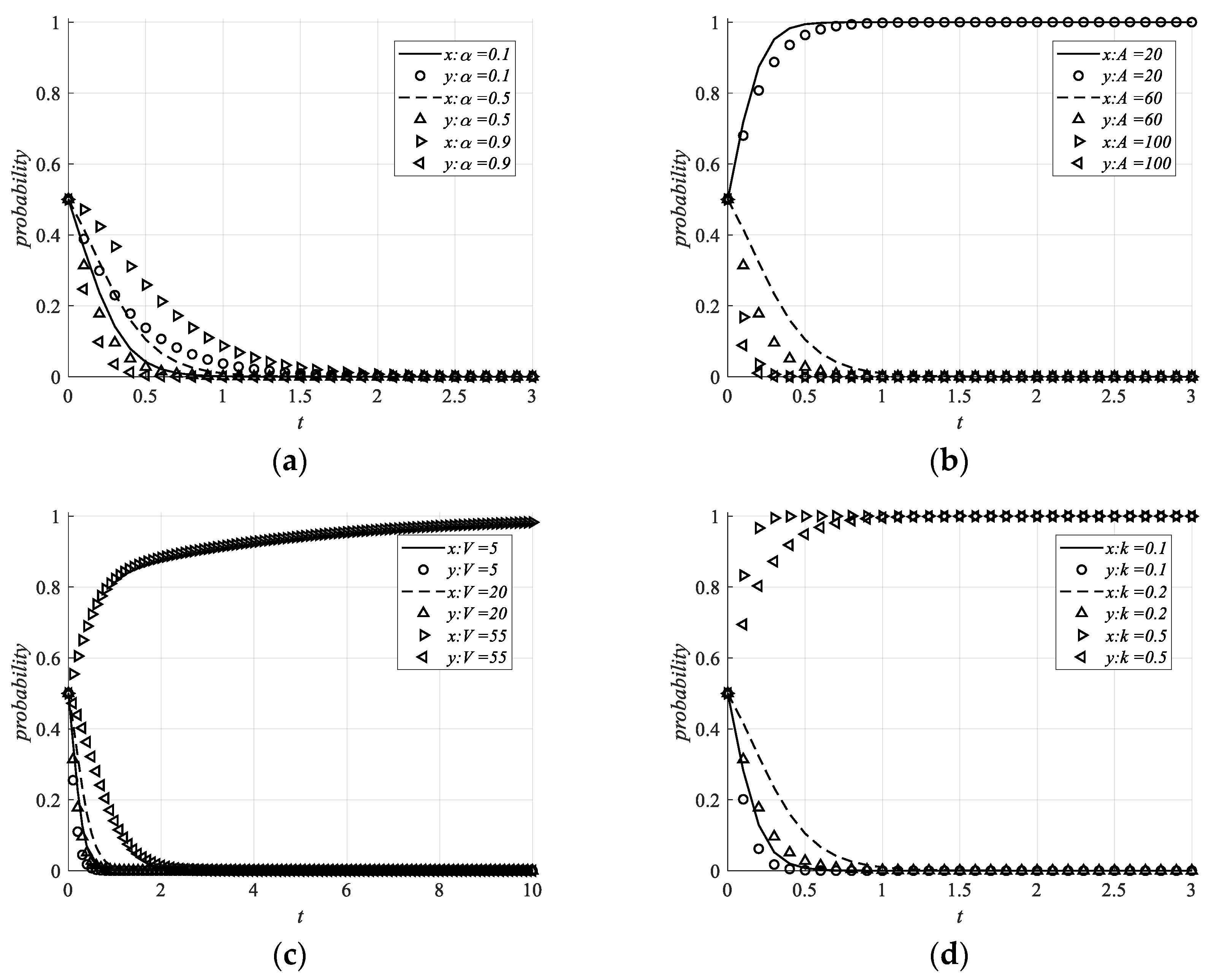
4.3. Simulation of the Impact of Other Parameters on Local Government’s Industrial Competition Behavior for Industry Absorption

Building upon the parameter settings in Figure 4a, we analyze the influence of other key variables in the model on local government’s competitive behavior in industry absorption through a bias towards industrial land use. With the other parameters held constant, we vary $\mathsf{\alpha }$ to values of 0.1, 0.5, and 0.9, resulting in Figure 4a. Similarly, assigning values of 20, 60, and 100 to $\mathrm{A}$ produces Figure 4b, values of 5, 20, and 55 for $\mathrm{V}$ generate Figure 4c, and values of 0.1, 0.2, and 0.5 for $\mathrm{k}$ lead to Figure 4d.

From Figure 4a, it is evident that the proportion of total benefits created by cooperative behavior between local governments has no significant impact on their land supply behavior. Figure 4b reveals that as the overall growth effects diminish for the local government receiving the industry transfer, both parties shift from competitive behavior towards cooperative behavior through a bias towards industrial land use. Figure 4c indicates that when the losses from non-cooperation by the local government increase, the side with relatively smaller growth preference or higher government income evolves from non-cooperative to cooperative behavior, adopting market mechanisms for land supply. Conversely, the side with larger growth preference or lower government income remains unchanged in its land supply behavior. Figure 4d illustrates that when one party chooses non-cooperation and the compensatory factor to the cooperating party steadily increases, both parties undergo a significant transformation from non-cooperative to cooperative behavior in the context of industrial competition for land absorption.

5. Conclusions and Policy Recommendations

Under the dual incentive mechanism of finance and revenue, local governments in China generally possess a certain degree of discretionary power over land use within their jurisdiction. In order to gain more fiscal revenue or attract more industrial transfer, the land supply behavior of local governments, which exhibit preferences for heterogeneity and income heterogeneity, inevitably varies. In this context, this paper constructs an evolutionary game model to systematically deduce the land supply behavior choices of local governments in the context of industrial transfer competition, with a particular focus on analyzing the impact of the heterogeneity of local government characteristics. The following three main research conclusions are drawn: (1) The land supply behavior of local governments mainly depends on the degree of preference and income heterogeneity, as well as the compensation level for losses to cooperative partners and the punishment intensity for non-cooperative behavior. (2) Convergence in preferences or divergence in income favor cooperative behavior and market-based land supply, while divergence in preferences or convergence in income lead to non-cooperative behavior, fostering industrial transfer competition through preferential supply of industrial land. (3) The greater the dependence of local governments’ income on industrial transfer, the more likely they are to adopt non-cooperative land supply behavior.

Based on the research conclusions, promoting cooperation in land supply behavior among Chinese local governments and avoiding excessive industrial transfer competition hinges on fully considering the heterogeneity of local governments and exploring effective paths to meet the current needs of regional economic coordination. The specific policy recommendations are as follows:

Firstly, the transfer payment system should be improved to narrow the income gap between local governments. If the income gap between local governments is too large, lower-income local governments may adopt a dominant strategy of competing in industrial land supply. Since income disparities between local governments cannot be leveled in the short term due to differences in geographical conditions, resource endowments, or economic development levels, it is crucial to improve the intergovernmental transfer payment system to regulate excessive income disparities. On one hand, the central government should establish a systematic, standardized, and directional vertical fiscal transfer payment system to provide necessary financial support for the development of underdeveloped regions. On the other hand, it should explore horizontal fiscal transfer payment systems between local governments, such as ecological compensation transfer payment systems, paired assistance transfer payment systems, and paired support transfer payment systems, to complement vertical transfer payments and enhance cooperation between local governments.

Secondly, the new development concept should be implemented, and the reform of existing incentive mechanisms should be deepened. With the initiation of the new journey to comprehensively build a socialist modernized society, the incentive mechanisms that have long promoted competition among local governments must undergo reform. Regarding land supply behavior, embedding the new development concept becomes the logical starting point for inspiring cooperative behavior among local governments. Specifically, the central government should integrate the five development concepts into the assessment system for local governments, enhancing incentive policies for local governments from both fiscal and growth perspectives to further stimulate cooperative land supply behavior. In terms of fiscal incentives, reforming the central–local fiscal relationship can guide local governments to adjust land transfer strategies. The central government should emphasize coordination and the sharing of ideas, concentrate the use of financial resources, balance the expenditure of funds required for coordination, implement the “housing is for living, not for speculation” policy, and break the real estate dependence syndrome of some local governments. Regarding growth incentives, reforming the assessment system for local governments can reduce GDP competition between them. The central government should focus on innovation, green development, and openness; assess local governments and their key officials based on performance; fundamentally change the preference for performance-oriented industries in land resource allocation; and reverse the competitive behavior of local governments in using low-priced industrial land for industrial transfer due to growth competition.

Thirdly, the market-oriented reform of land should be deepened, restraining the land supply behavior of local governments. In the current land market in China, land resources are not entirely allocated according to market mechanisms. As land suppliers, local governments, under the influence of fiscal and growth incentives, often engage in significant resource misallocation and negative externalities in their land supply behavior. Following the requirements of the “Opinions on Constructing a More Perfect Market-based Allocation System for Factors” issued in 2020, it is essential to deepen the market-oriented allocation reform of land, improve the efficiency of land factor allocation, and stimulate the vitality of the land market. Specifically, local governments should, on the basis of fully respecting market economic laws, self-restrain their monopoly behavior as primary suppliers and primary allocators in the land market. They should allocate land resources based on the real needs of local residential and industrial land, bringing land prices back to rational levels and guiding land resources to flow freely to the commercial and industrial sectors based on their real value. On the other hand, regarding the reform of market-oriented land, the central government should vigorously cooperate by further optimizing the fiscal system reform to promote the matching of financial rights and responsibilities of local governments. This fundamentally restrains the tendency of local governments to short debt and long investment in land finance, thereby reducing the dependence of local governments on land finance.

Fourthly, there should be a focus on internal source dynamics, and the external dependence of local governments should be weakened. When the income of local governments primarily depends on external industrial transfer, local governments will inevitably seek to increase local government revenue through industrial transfer competition. Under the condition of limited industrial transfer scale, local governments adopting a strategy of supplying industrial land is inevitable. Therefore, in the current context of increasing resource and environmental constraints, local governments should not rely mainly on external industrial transfer to achieve regional development. Instead, they should choose a path based on internal source dynamics grounded in regional endowment foundations. Specifically, it is necessary to fully explore and activate existing tangible factors such as land, labor, capital, and resources, as well as intangible factors such as institutional and cultural environments, relying on these internal factors to achieve optimal allocation through the market, fully exploiting the productive efficiency of factors, and achieving intrinsic development. Moreover, relying on local industries, it is essential to maximize the extension of the value chain within the region, seize the opportunity of constructing a unified national market, actively integrate into the national dual circulation development strategy, and use industrial linkage effects to extend service chains and supply chains nationally and globally, ultimately forming a regional industrial chain system led by the local region. Furthermore, governments should move beyond the traditional static capability perspective centered on land, position industrial transfer acceptance within the broader context of regional economic coordinated development, and strengthen the building of dynamic capabilities such as configuration, learning, and openness.

Despite this paper’s in-depth analysis of the land supply behavior of Chinese local governments, there are two notable shortcomings: first, the simplification of the model through the aggregation of some parameters has reduced the model’s explanatory and predictive capabilities; second, the paper focuses solely on the heterogeneity of local government land supply behavior. It is undeniable that many other factors influence this behavior, and the non-comprehensive exploration might negatively affect the generalizability of the research conclusions. From the internal logic of academic research in this field, these limitations highlight a direction worth further exploration: comprehensively considering the factors influencing local government land supply behavior and thus constructing a more generalized evolutionary game model to systematically analyze and reveal the evolutionary laws of local government land supply behavior.