The Decision of Production Systems with Quality-Contingent Demand and Condition-Based Maintenance
Abstract
:1. Introduction
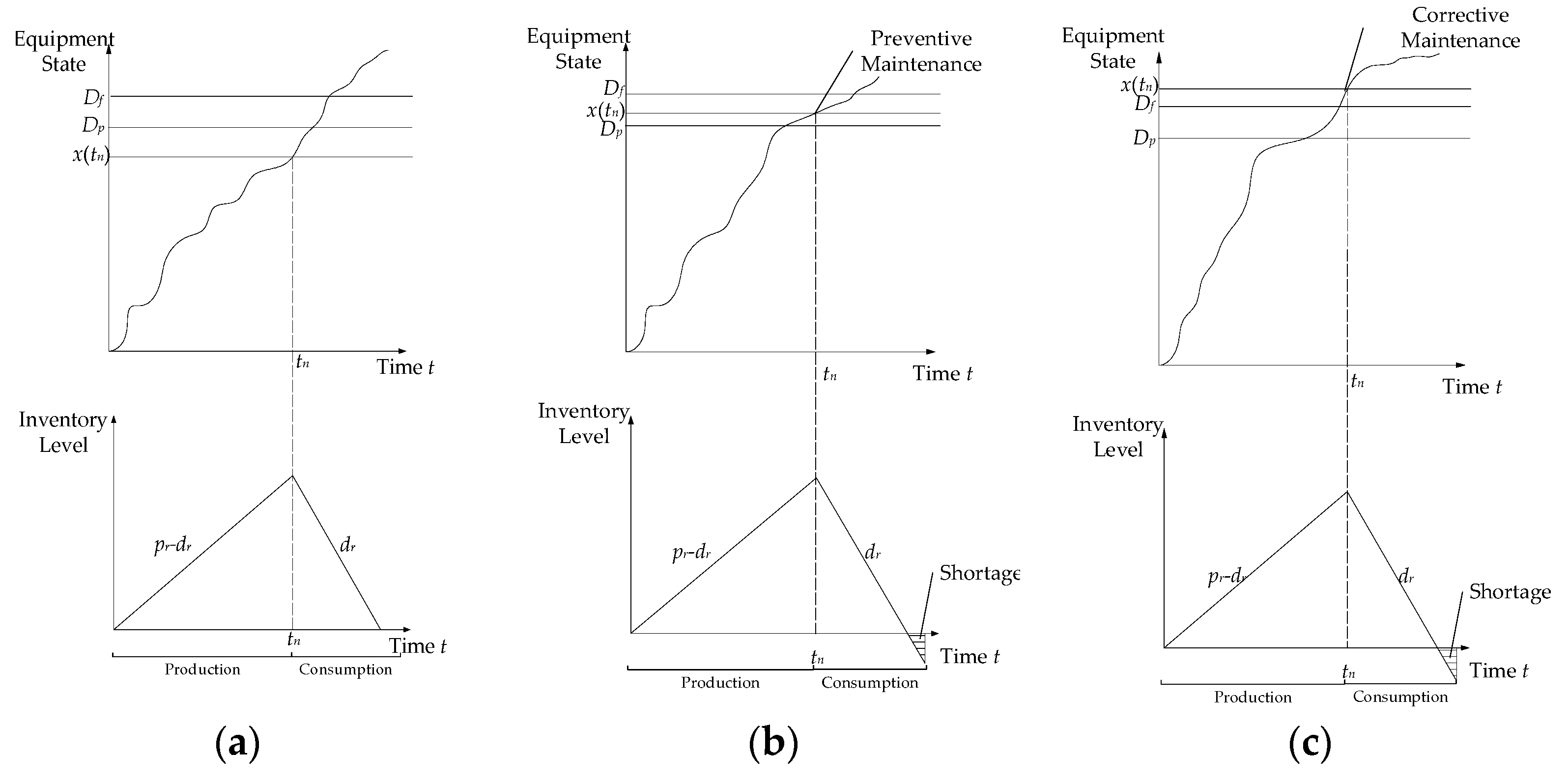
2. System Description and Assumptions
2.1. System Description
2.2. Assumptions
- The production rate is constant; the demand rate is only related to product quality and remains unchanged in a production cycle.
- The unqualified products only include defective products, and they can be repaired and completed instantly. The repaired products are all low-quality qualified products.
- Production is intermittent and demand is continuous.
- The equipment only deteriorates without sudden failure, and the equipment is restored to a new state after maintenance.
3. Model Formulation
3.1. Steady-State Probability Density of Equipment State
3.2. Defective Rate and Demand Rate
- The number of low-quality qualified products produced in the production process is:
- The number of low-quality qualified products repaired is:
3.3. The Integrated Model
- Situation 1
- Situation 2
- Situation 3
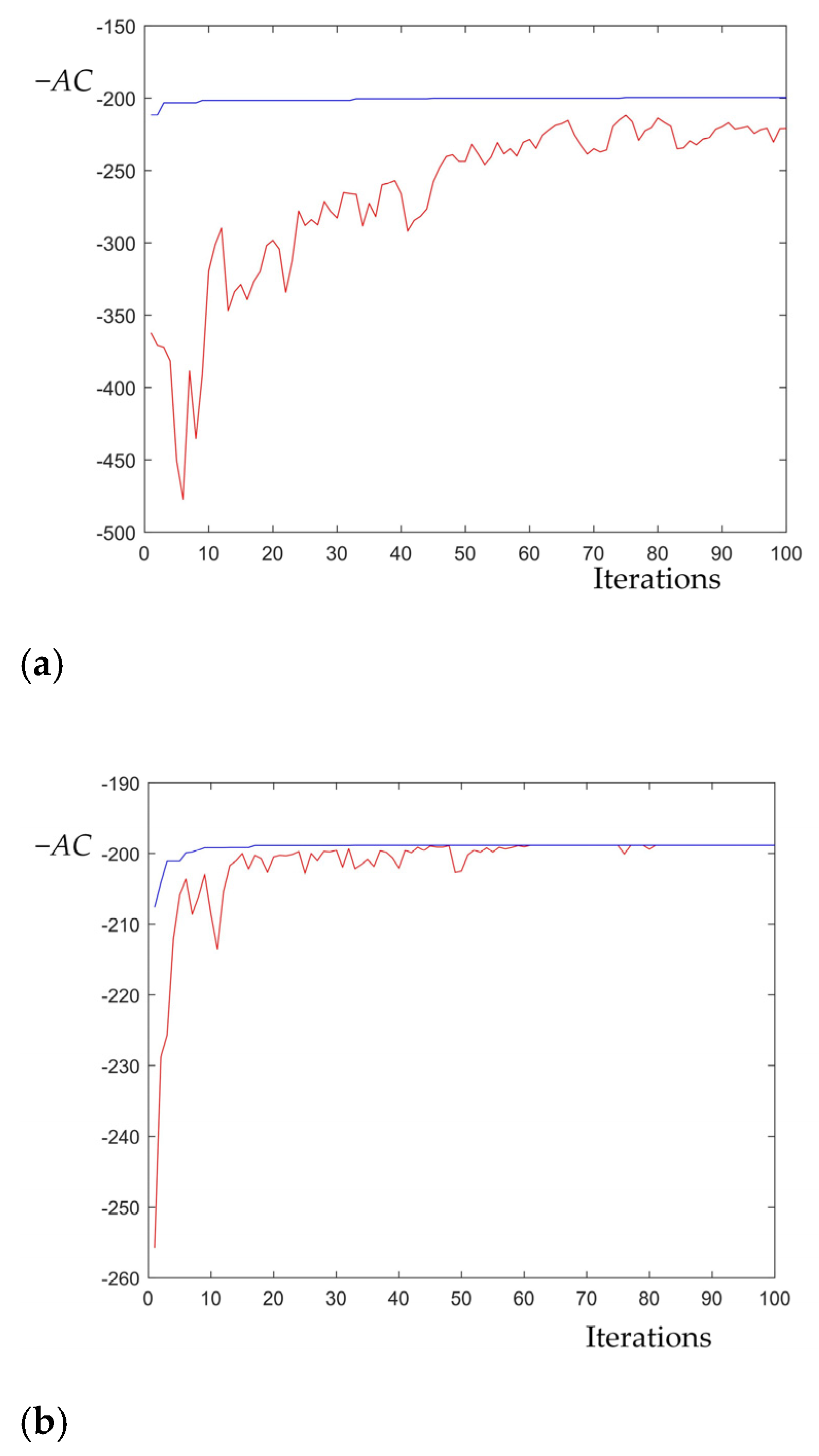
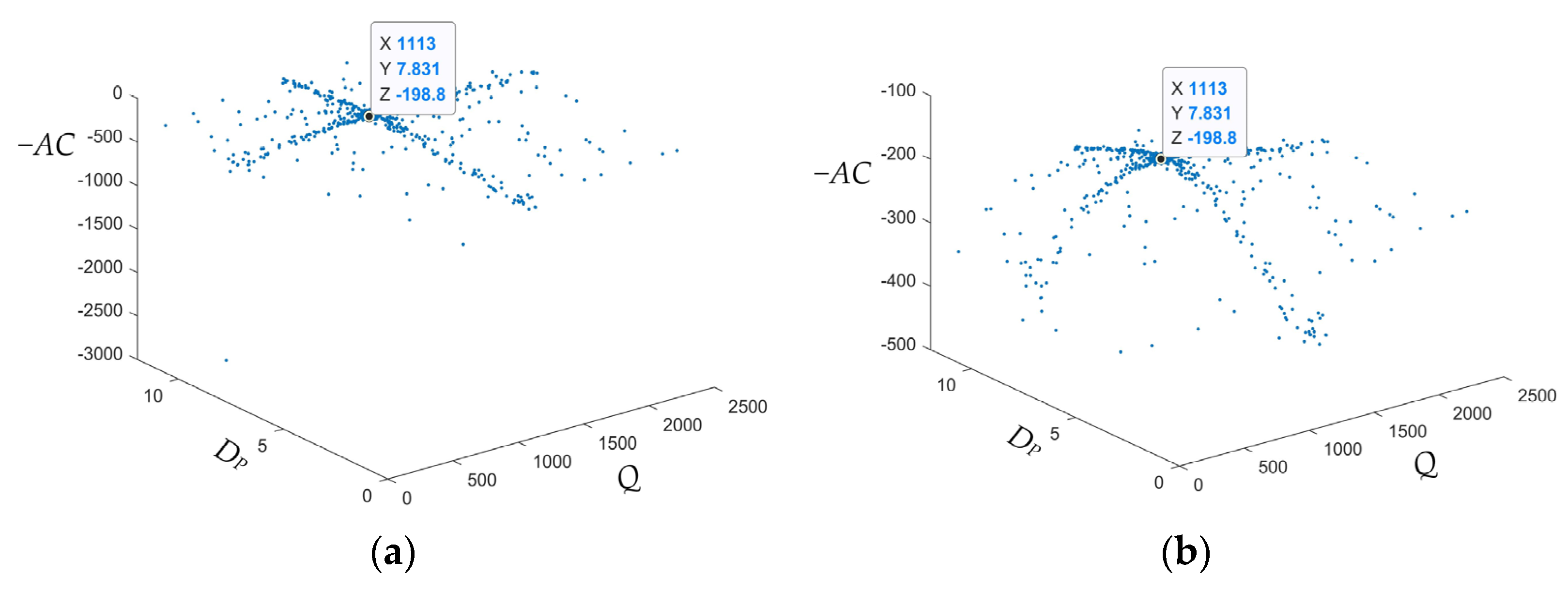
4. Numerical Analysis
4.1. Case Study
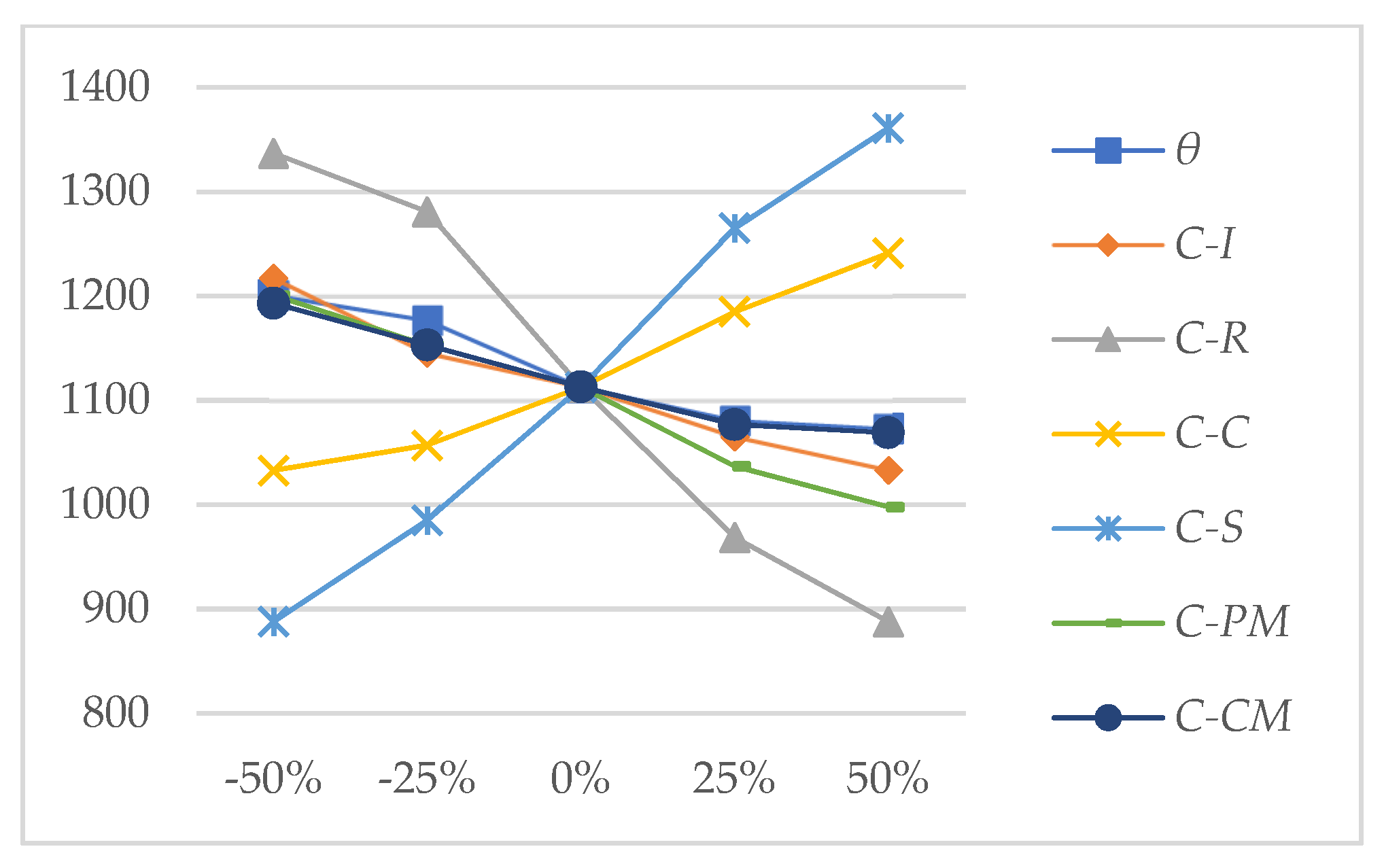
4.2. Sensitivity Analysis
- The optimal economic quantity Q* increases significantly with the increase in unit shortage cost, and decreases significantly with the rise of the unit repair cost.
- The optimal preventive maintenance threshold Dp* increases significantly with the increase in single preventive maintenance cost, and decreases significantly with the rise of single corrective maintenance cost.
- The average cost rate AC* increases significantly with the increase in single preventive maintenance cost, and decreases with the rise of fixed defective rate in production.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Schreiber, M.; Vernickel, K.; Richter, C.; Reinhart, G. Integrated production and maintenance planning in cyber-physical production systems. Procedia CIRP 2019, 79, 534–539. [Google Scholar] [CrossRef]
- Zheng, F.; He, J.; Liu, M. An exact Epsilon-constraint algorithm for the bi-objective optimization problem of scheduling staple fiber production. Oper. Res. Manag. Sci. 2018, 27, 1–8. [Google Scholar]
- Zhang, X.; Zeng, J. Deterioration state space partitioning method for opportunistic maintenance modelling of identical multi-unit systems. Int. J. Prod. Res. 2015, 140, 176–190. [Google Scholar] [CrossRef]
- Liu, X.; Feng, Z. Joint optimization of condition-based maintenance and EPQ based on the random sufficient growth mode. Syst. Eng.-Theory Pract. 2019, 39, 251–258. [Google Scholar]
- Liao, G. Production and maintenance policies for an EPQ model with perfect repair, rework, free-repair warranty, and preventive maintenance. IEEE Trans. Syst. Man Cybern. Syst. 2016, 46, 1129–1139. [Google Scholar] [CrossRef]
- Peng, H.; van Houtum, G.J. Joint optimization of condition-based maintenance and production lot-sizing. Eur. J. Oper. Res. 2016, 253, 94–107. [Google Scholar] [CrossRef]
- Jafari, L.; Makis, V. Joint optimal lot sizing and preventive maintenance policy for a production facility subject to condition monitoring. Int. J. Prod. Econ. 2015, 169, 156–168. [Google Scholar] [CrossRef]
- Zhang, X.; Xia, T.; Pan, E.; Li, Y. Integrated optimization on production scheduling and imperfect preventive maintenance considering multi-degradation and learning-forgetting effects. Flex. Serv. Manuf. J. 2021, 1–32. [Google Scholar] [CrossRef]
- Lu, S.; Pei, J.; Liu, X.; Pardalos, P.M. A hybrid DBH-VNS for high-end equipment production scheduling with machine failures and preventive maintenance activities. J. Comput. Appl. Math. 2021, 384, 113195. [Google Scholar] [CrossRef]
- Cadi, A.A.E.; Gharbi, A.; Dhouib, K.; Artiba, A. Joint production and preventive maintenance controls for unreliable and imperfect manufacturing systems. J. Manuf. Syst. 2021, 58, 263–279. [Google Scholar] [CrossRef]
- Liao, W.; Chen, M.; Yang, X. Joint optimization of preventive maintenance and production scheduling for parallel machines system. J. Intell. Fuzzy Syst. 2017, 32, 913–923. [Google Scholar] [CrossRef]
- Marquez, A.C.; Yin, X.; Liu, X. The Maintenance Management Framework: Models and Methods for Complex Systems Maintenance; National Defence Industry Press: Beijing, China, 2013; pp. 49–50. [Google Scholar]
- Cassady, C.R.; Kutanoglu, E. Integrating preventive maintenance planning and production scheduling for a single machine. IEEE Trans. Reliab. 2005, 54, 304–3097. [Google Scholar] [CrossRef]
- Khatab, A.; Diallo, C.; Aghezzaf, E.H.; Venkatadri, U. Integrated production quality and condition-based maintenance optimisation for a stochastically deteriorating manufacturing system. Int. J. Prod. Res. 2019, 57, 2480–2497. [Google Scholar] [CrossRef]
- Fitouhi, M.C.; Nourelfath, M. Integrating noncyclical preventive maintenance scheduling and production planning for a single machine. Int. J. Prod. Econ. 2012, 136, 344–351. [Google Scholar] [CrossRef]
- Lu, Z.; Zhang, Y.; Han, X. Integrating run-based preventive maintenance into the capacitated lot sizing problem with reliability constraint. Int. J. Prod. Res. 2013, 51, 1379–1391. [Google Scholar] [CrossRef]
- Lu, Z.; Xue, J.; Yang, Y. The decision of economic production quantity with quality-contingent demand and perfect preventative maintenance. Chin. J. Manag. Sci. 2020, 28, 71–78. [Google Scholar]
- Liu, X.; Wang, W.; Peng, R. An integrated production, inventory and preventive maintenance model for a multi-product production system. Reliab. Eng. Syst. Saf. 2015, 137, 76–86. [Google Scholar] [CrossRef]
- Lin, W.; Lu, Z.; Han, X. Joint optimisation of production, maintenance and quality for batch production system subject to varying operational conditions. Int. J. Prod. Res. 2019, 57, 7552–7566. [Google Scholar]
- Bouslah, B.; Gharbi, A.; Pellerin, R. Integrated production, sampling quality control and maintenance of deteriorating production systems with AOQL constraint. Omega 2016, 61, 110–126. [Google Scholar] [CrossRef]
- Sun, H.; Xia, X.; Liu, B. Inventory lot sizing policies for a closed-loop hybrid system over a finite product life cycle. Comput. Ind. Eng. 2020, 142, 106340. [Google Scholar] [CrossRef]
- Priyamvada, R.; Khanna, A.; Jaggi, C.K. An inventory model under price and stock dependent demand for controllable deterioration rate with shortages and preservation technology investment: Revisited. Opaearch 2021, 58, 181–202. [Google Scholar] [CrossRef]
- Choodowicz, E.; Orowski, P. Development of new hybrid discrete-time perishable inventory model based on Weibull distribution with time-varying demand using system dynamics approach. Comput. Ind. Eng. 2021, 154, 107151. [Google Scholar] [CrossRef]
- Seifbarghy, M.; Nouhi, K.; Mahmoud, A. Contract design in a supply chain considering price and quality dependent demand with customer segmentation. Int. J. Prod. Econ. 2015, 167, 108–118. [Google Scholar] [CrossRef]
- Wangn, S.; Liu, M. A branch and bound algorithm for single-machine production scheduling integrated with preventive maintenance planning. Int. J. Prod. Res. 2013, 51, 847–868. [Google Scholar] [CrossRef]
- Liu, Q.; Dong, M.; Chen, F.F.; Liu, W.; Ye, C. Multi-objective imperfect maintenance optimization for production system with an intermediate buffer. J. Manuf. Syst. 2020, 56, 452–462. [Google Scholar] [CrossRef]
- Pasandideh, S.; Niaki, S. A genetic algorithm approach to optimize a multi-products EPQ model with discrete delivery orders and constrained space. Appl. Math. Comput. 2008, 195, 506–514. [Google Scholar] [CrossRef]
- Rezaei, J.; Davoodi, M. Multi-objective models for lot-sizing with supplier selection. Int. J. Prod. Econ. 2011, 130, 77–86. [Google Scholar] [CrossRef]
- Van Noortwijk, J.M. A survey of the application of gamma processes in maintenance. Reliab. Eng. Syst. Saf. 2009, 94, 2–21. [Google Scholar] [CrossRef]
- Grall, A.; Dieulle, L.; Berenguer, C.; Roussignol, M. Continuous-time predictive-maintenance scheduling for a deteriorating system. IEEE Trans. Reliab. 2002, 51, 141–150. [Google Scholar] [CrossRef] [Green Version]
- Yang, X.; Guan, K. Joint Optimization Strategy of Production Planning and Condition-Based Maintenance Considering Limitation of Defective Rate. Ind. Eng. Manag. 2021, 1–17. Available online: http://kns.cnki.net/kcms/detail/31.1738.T.20210621.1829.004.html (accessed on 13 February 2022).



| Parameters | Description |
|---|---|
| Q | economic production quantity, decision variable |
| Dp | preventive maintenance threshold, decision variable |
| Df | equipment failure threshold |
| pr | the production rate of the system |
| dr | the demand rate of the system |
| θ | fixed low-quality product ratio in qualified products |
| CI | unit product inventory cost |
| CR | unit unqualified product repair cost |
| CS | unit product shortage cost |
| CC | detection cost each time |
| CPM | preventive maintenance cost each time |
| CCM | corrective maintenance cost each time |
| x(t) | state of the equipment at time t |
| s(x) | the steady-state probability density function of equipment state x |
| g1(tpm) | probability density function of preventive maintenance time tpm |
| g2(tcm) | probability density function of corrective maintenance time tcm |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| pr (unit/day) | 200 | Df | 12 |
| dmax (unit/day) | 160 | CI (Yuan/unit/day) | 0.5 |
| θ | 0.1 | CR (Yuan/unit) | 10 |
| p0 | 0.004 | CS (Yuan/unit) | 20 |
| η | 0.071 | CC (Yuan/Each time) | 150 |
| α | 0.0046 | CPM (Yuan/Each time) | 1800 |
| β | 1.26 | CCM (Yuan/Each time) | 4500 |
| μ | 0.1 |
| Parameter | Variation | The Optimal Quantity Q* | The Optimal PM Threshold Dp* | The Average Cost Rate AC* |
|---|---|---|---|---|
| - | Basic case | 1113 | 7.831 | 198.7637 |
| θ | −50% | 1201 | 7.6735 | 207.8333 |
| −25% | 1177 | 7.7005 | 203.2433 | |
| 25% | 1081 | 7.9021 | 194.477 | |
| 50% | 1073 | 7.9547 | 190.3974 | |
| CI | −50% | 1217 | 7.5325 | 191.6533 |
| −25% | 1145 | 7.7108 | 195.3027 | |
| 25% | 1065 | 7.9359 | 202.1052 | |
| 50% | 1033 | 8.048 | 205.3371 | |
| CR | −50% | 1337 | 7.215 | 181.8428 |
| −25% | 1281 | 7.5831 | 191.0104 | |
| 25% | 969 | 8.1578 | 206.1737 | |
| 50% | 888 | 8.6392 | 212.4117 | |
| CC | −50% | 1033 | 8.0755 | 188.186 |
| −25% | 1057 | 7.9303 | 193.5904 | |
| 25% | 1185 | 7.6226 | 203.6904 | |
| 50% | 1241 | 7.4475 | 208.3536 | |
| CS | −50% | 888 | 8.2554 | 183.1767 |
| −25% | 985 | 8.0255 | 192.0107 | |
| 25% | 1265 | 7.5141 | 204.1839 | |
| 50% | 1361 | 7.316 | 208.5963 | |
| CPM | −50% | 1201 | 6.367 | 153.026 |
| −25% | 1153 | 7.2189 | 176.8459 | |
| 25% | 1037 | 8.365 | 220.6385 | |
| 50% | 998 | 9.2227 | 235.0724 | |
| CCM | −50% | 1193 | 9.9544 | 167.593 |
| −25% | 1153 | 8.5109 | 189.6088 | |
| 25% | 1077 | 7.2975 | 206.448 | |
| 50% | 1069 | 6.9545 | 210.8357 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, Z.; Wang, H.; Zhang, H. The Decision of Production Systems with Quality-Contingent Demand and Condition-Based Maintenance. Systems 2022, 10, 20. https://doi.org/10.3390/systems10010020
Gao Z, Wang H, Zhang H. The Decision of Production Systems with Quality-Contingent Demand and Condition-Based Maintenance. Systems. 2022; 10(1):20. https://doi.org/10.3390/systems10010020
Chicago/Turabian StyleGao, Zhenhua, Hongjun Wang, and Hongliang Zhang. 2022. "The Decision of Production Systems with Quality-Contingent Demand and Condition-Based Maintenance" Systems 10, no. 1: 20. https://doi.org/10.3390/systems10010020
APA StyleGao, Z., Wang, H., & Zhang, H. (2022). The Decision of Production Systems with Quality-Contingent Demand and Condition-Based Maintenance. Systems, 10(1), 20. https://doi.org/10.3390/systems10010020






