Aberrant Water Structure Dynamics in B16 Melanoma-Bearing Mice by Time Domain Refractometry Analysis
Abstract
Simple Summary
Abstract
1. Introduction
2. Materials and Methods
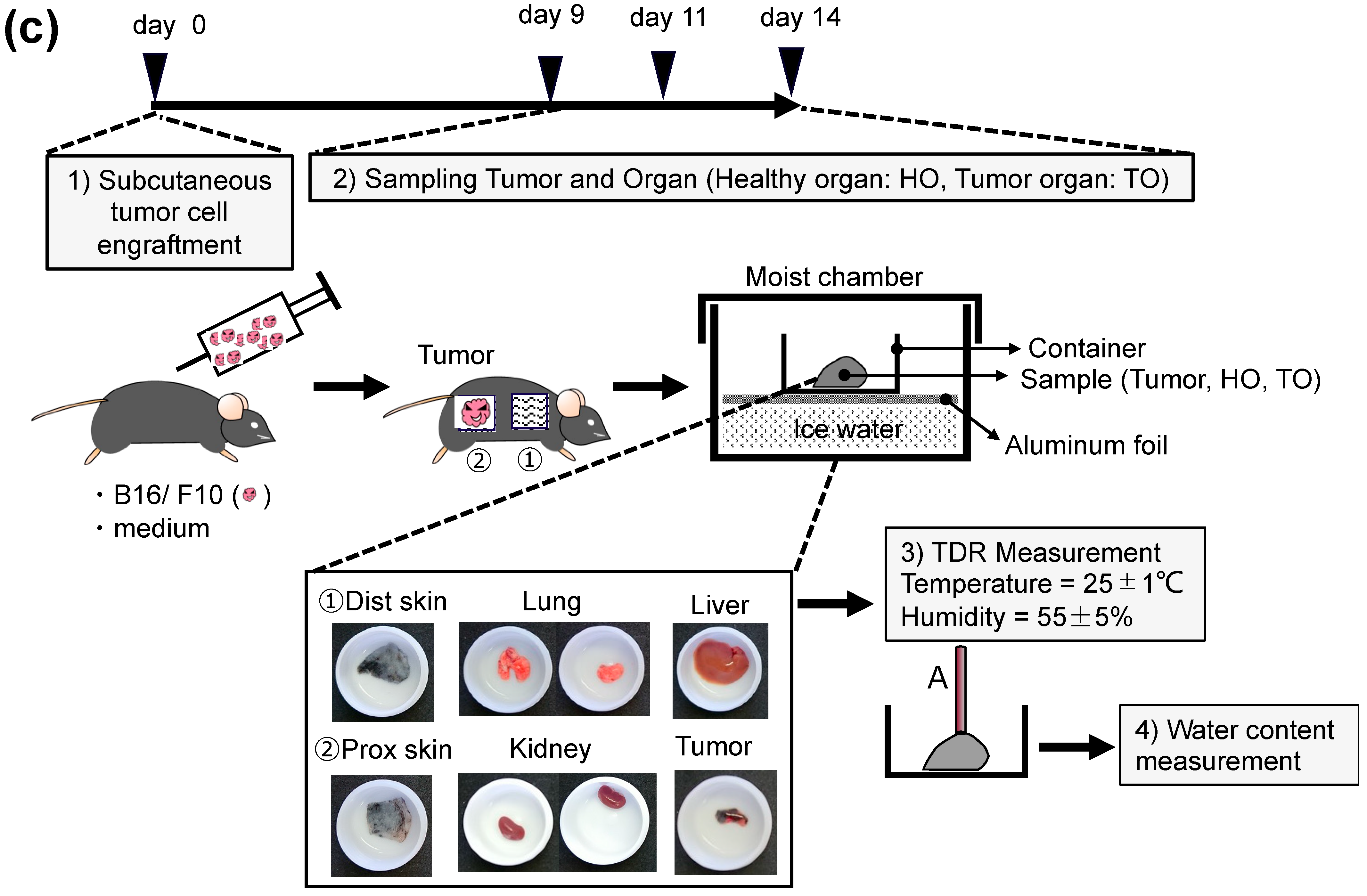
2.1. Construction of B16 Tumor-Bearing Mice
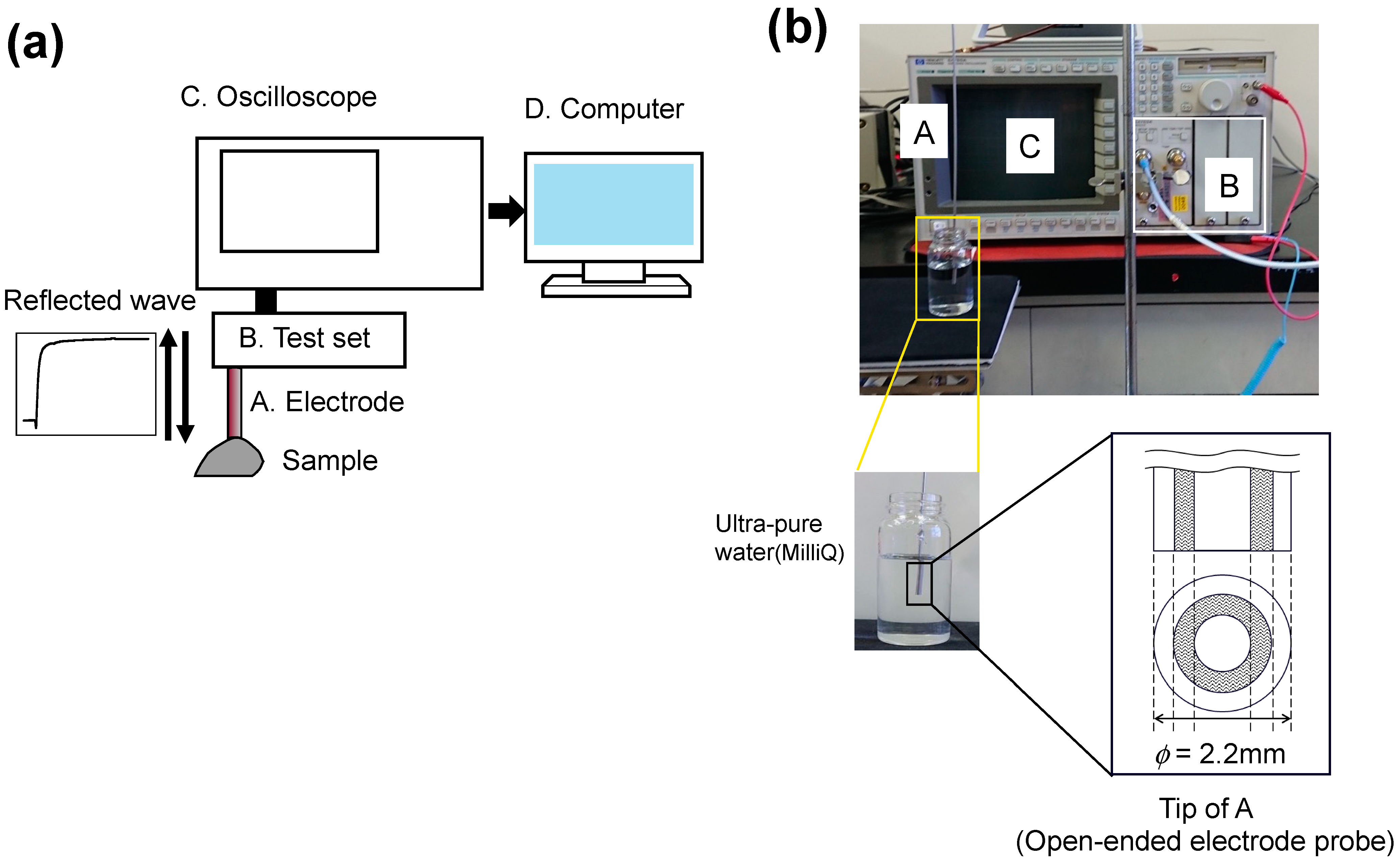
2.2. Dielectric Relaxation Measurement
2.3. Sampling of the Organ and Tumor Tissues for TDR
2.4. Analytical Method of the Dielectric Relaxation Result of a Measurement
2.5. Measurement of the Water Content
2.6. Statistical Analysis
3. Results
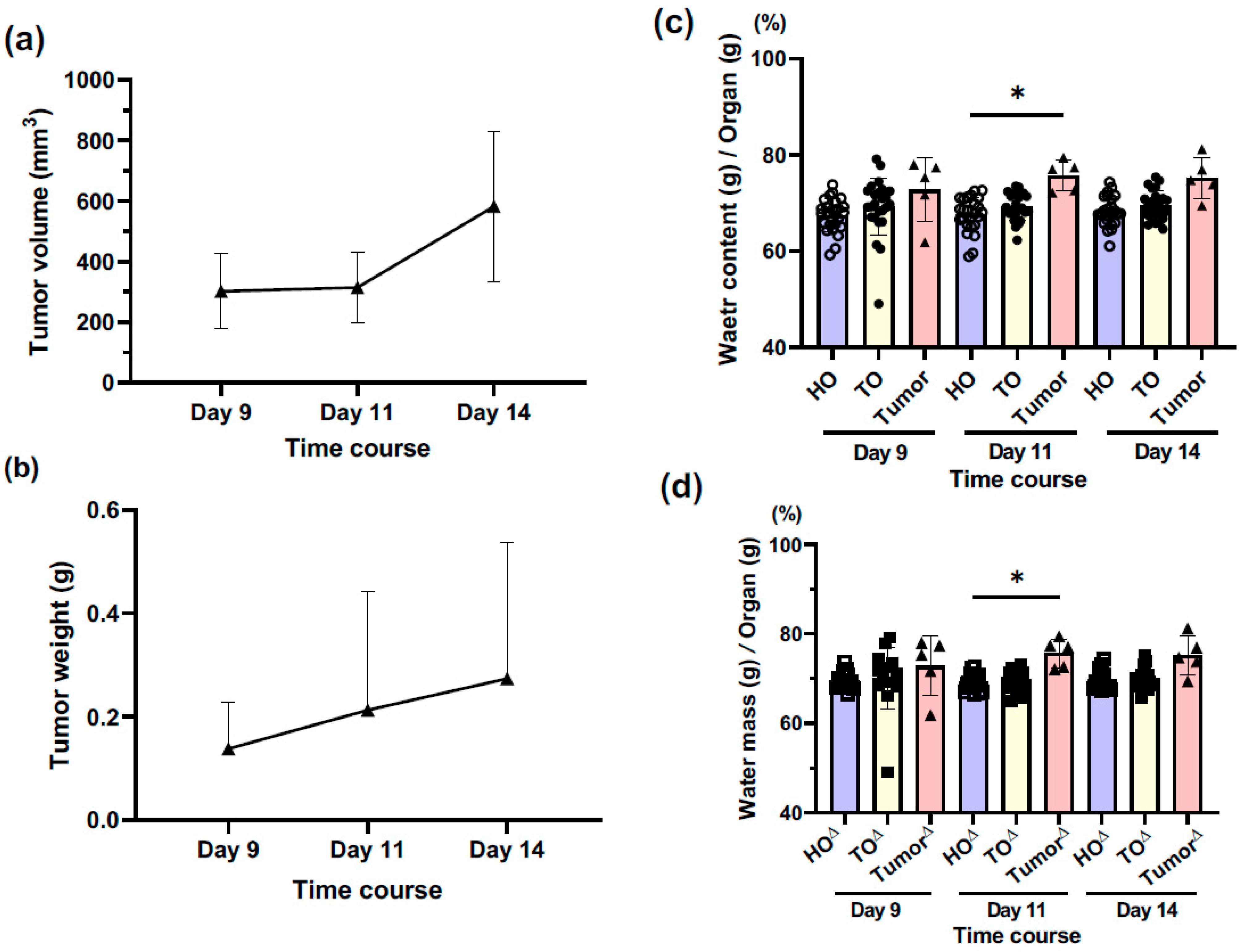
3.1. How do Tumor Volume and Mass Vary during the Observation Period?
3.2. How Does the Water Content of Tumor Tissues Change over Time?
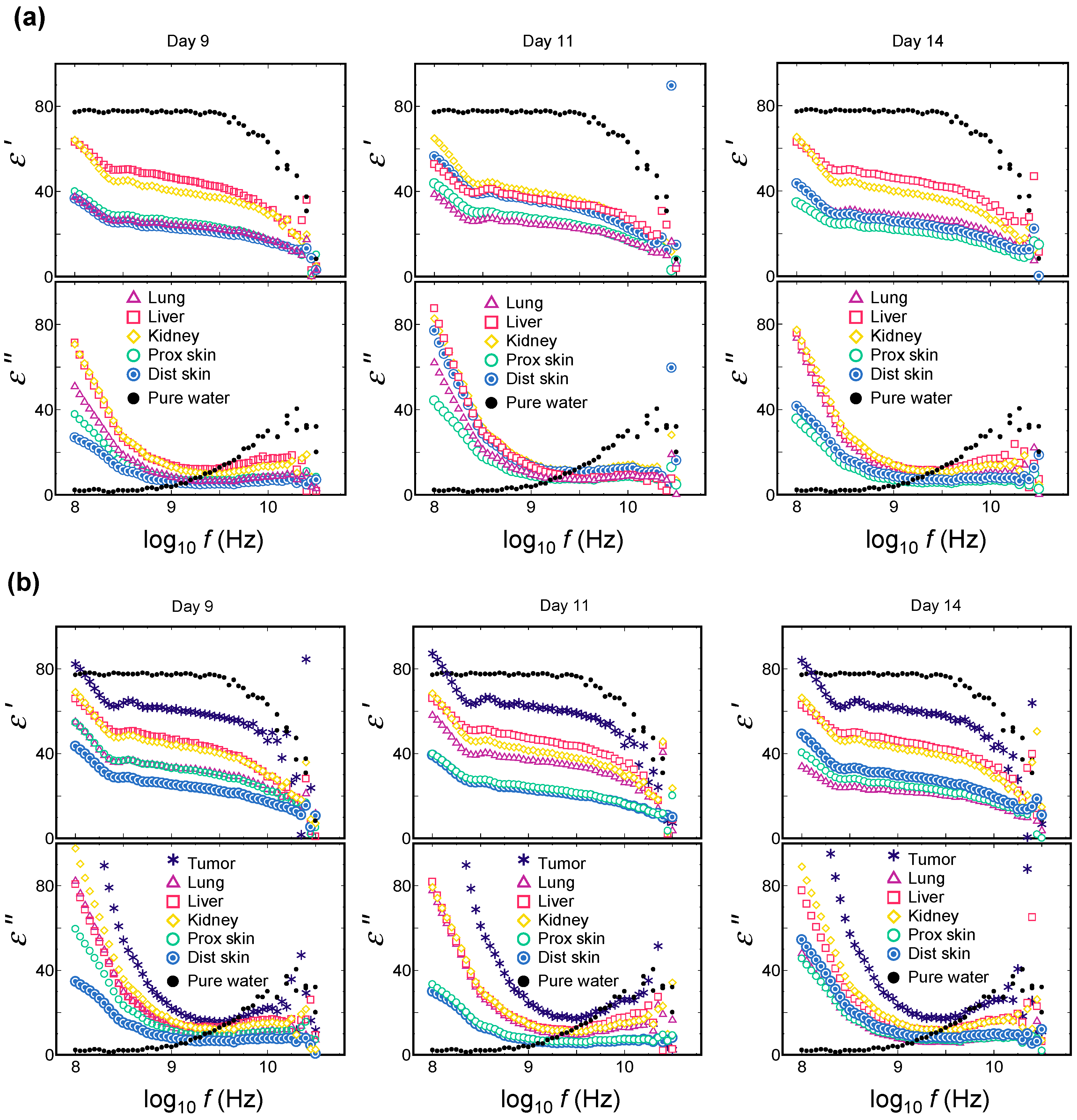
3.3. How Is It Consistent with Existing Measurements of Dielectric Constant and Conductivity?
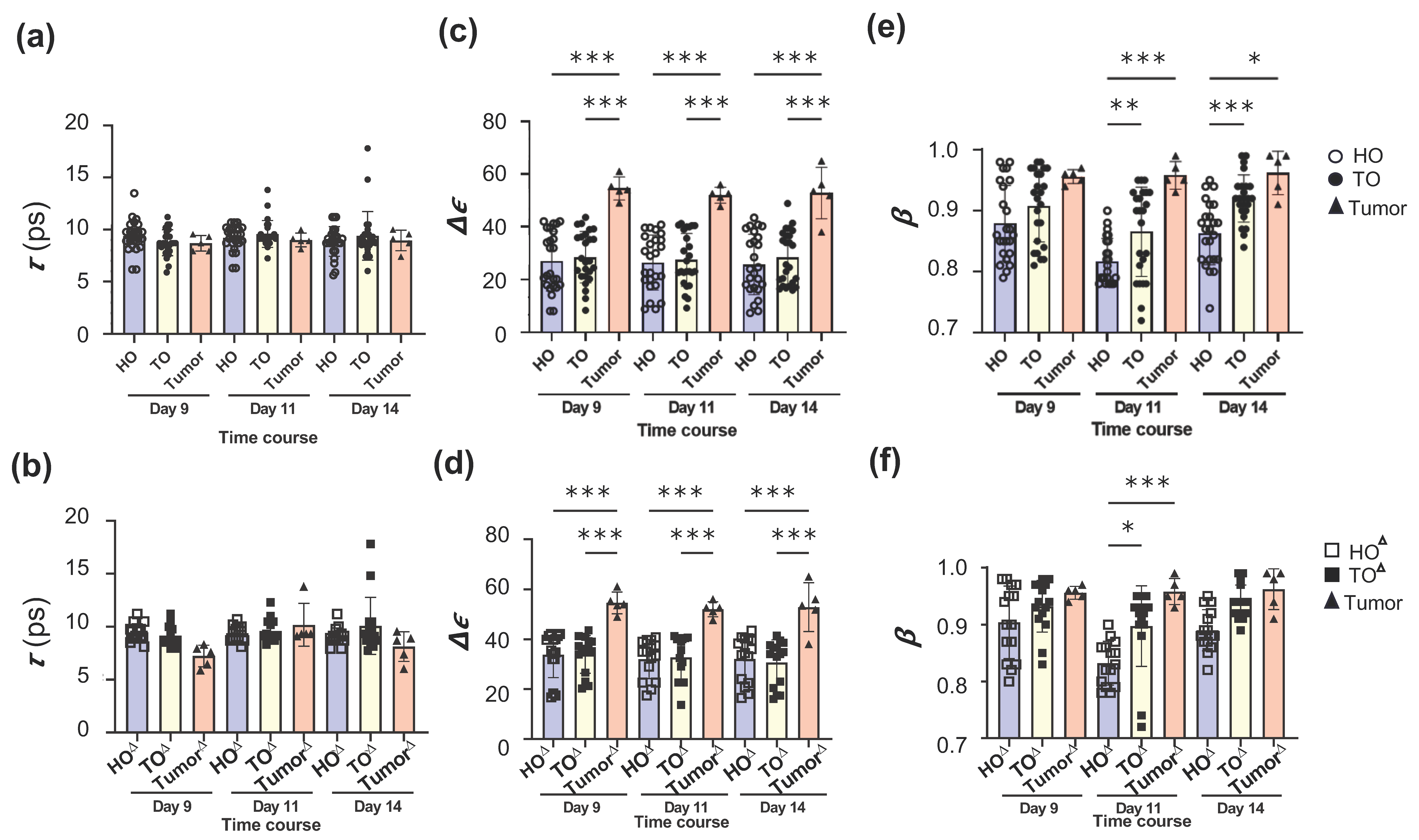
3.4. Does Tumor Tissue Exhibit a Different Dielectric Relaxation Curve Than Other Organs?
3.5. Where on the τ-β Diagram Is the Tumor Tissue Distributed?
3.6. Does Tumor Tissue Show Different Characteristics for Δε and β Than HO and TO?
4. Discussion
4.1. Tumor Growth and Water Content in Tumor-Bearing and Healthy Mice
4.2. Aberrant Dielectric Ex Vivo WSD of Tumor-Bearing Mice Versus Healthy Mice
4.3. The Pathophysiological Mechanism of Aberrant Dielectric Ex Vivo WSD in Tumor-Bearing Mice
4.4. Different Dielectric Ex Vivo WSD of Tumor-Bearing and Septic Mice
4.5. Study Limitations and Future Experiments
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Serra-Prat, M.; Lorenzo, I.; Palomera, E.; Yebenes, J.C.; Campins, L.; Cabre, M. Intracellular Water Content in Lean Mass is Associated with Muscle Strength, Functional Capacity, and Frailty in Community-Dwelling Elderly Individuals. A Cross-Sectional Study. Nutrients 2019, 11, 661. [Google Scholar] [CrossRef]
- Penet, M.F.; Kakkad, S.; Wildes, F.; Bhujwalla, Z.M. Water and Collagen Content Are High in Pancreatic Cancer: Implications for Quantitative Metabolic Imaging. Front. Oncol. 2020, 10, 599204. [Google Scholar] [CrossRef]
- Hayashi, Y.; Miura, N.; Shinyashiki, N.; Yagihara, S. Free water content and monitoring of healing processes of skin burns studied by microwave dielectric spectroscopy in vivo. Phys. Med. Biol. 2005, 50, 599–612. [Google Scholar] [CrossRef]
- Heys, K.R.; Friedrich, M.G.; Truscott, R.J. Free and bound water in normal and cataractous human lenses. Investig. Ophthalmol. Vis. Sci. 2008, 49, 1991–1997. [Google Scholar] [CrossRef]
- Carneiro, I.; Carvalho, S.; Henrique, R.; Oliveira, L.; Tuchin, V.V. Simple multimodal optical technique for evaluation of free/bound water and dispersion of human liver tissue. J. Biomed. Opt. 2017, 22, 125002. [Google Scholar] [CrossRef] [PubMed]
- Yamada, S.; Tsuboi, Y.; Yokoyama, D.; Kikuchi, J. Polymer composition optimization approach based on feature extraction of bound and free water using time-domain nuclear magnetic resonance. J. Magn. Reson. 2023, 351, 107438. [Google Scholar] [CrossRef] [PubMed]
- Tielrooij, K.J.; Paparo, D.; Piatkowski, L.; Bakker, H.J.; Bonn, M. Dielectric relaxation dynamics of water in model membranes probed by terahertz spectroscopy. Biophys. J. 2009, 97, 2484–2492. [Google Scholar] [CrossRef] [PubMed]
- Zarzycki, P.; Gilbert, B. Temperature-dependence of the dielectric relaxation of water using non-polarizable water models. Phys. Chem. Chem. Phys. 2020, 22, 1011–1018. [Google Scholar] [CrossRef]
- Martins, M.L.; Bordallo, H.N.; Arrese-Igor, S.; Alegria, A.; Colmenero de Leon, J. Effect of Paclitaxel in the Water Dynamics of MCF-7 Breast Cancer Cells Revealed by Dielectric Spectroscopy. ACS Omega 2020, 5, 18602–18607. [Google Scholar] [CrossRef]
- Asami, K.; Takahashi, K.; Shirahige, K. Progression of cell cycle monitored by dielectric spectroscopy and flow-cytometric analysis of DNA content. Yeast 2000, 16, 1359–1363. [Google Scholar] [CrossRef]
- Jansson, H.; Bergman, R.; Swenson, J. Relation between solvent and protein dynamics as studied by dielectric spectroscopy. J. Phys. Chem. B 2005, 109, 24134–24141. [Google Scholar] [CrossRef] [PubMed]
- Yagihara, S.; Kita, R.; Shinyashiki, N.; Saito, H.; Maruyama, Y.; Kawaguchi, T.; Shoji, K.; Saito, T.; Aoyama, T.; Shimazaki, K.; et al. Physical Meanings of Fractal Behaviors of Water in Aqueous and Biological Systems with Open-Ended Coaxial Electrodes. Sensors 2019, 19, 2606. [Google Scholar] [CrossRef] [PubMed]
- Yagihara, S.; Watanabe, S.; Abe, Y.; Asano, M.; Shimizu, K.; Saito, H.; Maruyama, Y.; Kita, R.; Shinyashiki, N.; Kundu, S.K. Universal Behavior of Fractal Water Structures Observed in Various Gelation Mechanisms of Polymer Gels, Supramolecular Gels, and Cement Gels. Gels 2023, 9, 506. [Google Scholar] [CrossRef] [PubMed]
- Fornes-Leal, A.; Garcia-Pardo, C.; Frasson, M.; Pons Beltran, V.; Cardona, N. Dielectric characterization of healthy and malignant colon tissues in the 0.5–18 GHz frequency band. Phys. Med. Biol. 2016, 61, 7334–7346. [Google Scholar] [CrossRef] [PubMed]
- Marta Guardiola, S.B.; Fernández-Esparrach, G.; O’Callaghan, J.M.; Romeu, J.; Cuatrecasas, M.; Córdova, H.; González Ballester, M.A.; Camara, O. Dielectric properties of colon polyps, cancer, and normal mucosa: Ex vivo measurements from 0.5 to 20 GHz. Med. Phys. 2018, 45, 3768–3782. [Google Scholar] [CrossRef] [PubMed]
- Hessinger Née Reimann, C.; Bazrafshan, B.; Hübner, F.; Schmidt, S.; Schüler, M.; Panahi, B.; Kaltenbach, B.; Polkowski, C.; Vogl, T.J.; Jakoby, R. Dielectric Contrast Between Normal and Tumor Ex-Vivo Human Liver Tissue. IEEE Access 2019, 7, 164113–164119. [Google Scholar] [CrossRef]
- Gavdush, A.A.; Chernomyrdin, N.V.; Komandin, G.A.; Dolganova, I.N.; Nikitin, P.V.; Musina, G.R.; Katyba, G.M.; Kucheryavenko, A.S.; Reshetov, I.V.; Potapov, A.A.; et al. Terahertz dielectric spectroscopy of human brain gliomas and intact tissues ex vivo: Double-Debye and double-overdamped-oscillator models of dielectric response. Biomed. Opt. Express 2021, 12, 69–83. [Google Scholar] [CrossRef] [PubMed]
- Otagiri, R.; Kawai, H.; Takatsuka, M.; Shinyashiki, N.; Ito, A.; Ikeguchi, R.; Aoyama, T. Interfacial polarization of in vivo rat sciatic nerve with crush injury studied via broadband dielectric spectroscopy. PLoS ONE 2021, 16, e0252589. [Google Scholar] [CrossRef]
- Szerement, J.; Saito, H.; Furuhata, K.; Yagihara, S.; Szyplowska, A.; Lewandowski, A.; Kafarski, M.; Wilczek, A.; Majcher, J.; Woszczyk, A.; et al. Dielectric Properties of Glass Beads with Talc as a Reference Material for Calibration and Verification of Dielectric Methods and Devices for Measuring Soil Moisture. Materials 2020, 13, 1968. [Google Scholar] [CrossRef]
- Cole, R.H. Evaluation of dielectric behavior by time domain spectroscopy. I. Dielectric response by real time analysis. J. Phys. Chem. 1975, 79, 1459–1469. [Google Scholar] [CrossRef]
- Cole, R.H. Evaluation of dielectric behavior by time domain spectroscopy. II. Complex permittivity. J. Phys. Chem. 1975, 79, 1469–1474. [Google Scholar] [CrossRef]
- Cole, R.H.; Mashimo, S.; Winsor IV, P. Evaluation of dielectric behavior by time domain spectroscopy. 3. Precision difference methods. J. Phys. Chem. 1980, 84, 786–793. [Google Scholar] [CrossRef]
- Restuccia, L.; Kluitenberg, G. On generalizations of the Debye equation for dielectric relaxation. Phys. A 1988, 154, 157–182. [Google Scholar] [CrossRef]
- Gabriel, S.; Lau, R.W.; Gabriel, C. The dielectric properties of biological tissues: III. Parametric models for the dielectric spectrum of tissues. Phys. Med. Biol. 1996, 41, 2271–2293. [Google Scholar] [CrossRef]
- Etoz, S.; Brace, C.L. Development of Water Content Dependent Tissue Dielectric Property Models. IEEE J. Electromagn. RF Microw. Med. Biol. 2019, 3, 105–110. [Google Scholar] [CrossRef]
- Hussein, M.; Awwad, F.; Jithin, D.; El Hasasna, H.; Athamneh, K.; Iratni, R. Breast cancer cells exhibits specific dielectric signature in vitro using the open-ended coaxial probe technique from 200 MHz to 13.6 GHz. Sci. Rep. 2019, 9, 4681. [Google Scholar] [CrossRef] [PubMed]
- Heileman, K.L.; Tabrizian, M. Dielectric spectroscopy platform to measure MCF10A epithelial cell aggregation as a model for spheroidal cell cluster analysis. Analyst 2017, 142, 1601–1607. [Google Scholar] [CrossRef] [PubMed]
- Hutchenreuther, J.; Vincent, K.; Norley, C.; Racanelli, M.; Gruber, S.B.; Johnson, T.M.; Fullen, D.R.; Raskin, L.; Perbal, B.; Holdsworth, D.W.; et al. Activation of cancer-associated fibroblasts is required for tumor neovascularization in a murine model of melanoma. Matrix Biol. 2018, 74, 52–61. [Google Scholar] [CrossRef]
- Ziyad, S.; Iruela-Arispe, M.L. Molecular mechanisms of tumor angiogenesis. Genes Cancer 2011, 2, 1085–1096. [Google Scholar] [CrossRef]
- Pishko, G.L.; Muldoon, L.L.; Pagel, M.A.; Schwartz, D.L.; Neuwelt, E.A. Vascular endothelial growth. factor blockade alters magnetic resonance imaging biomarkers of vascular function and decreases barrier permeability in a rat model of lung cancer brain metastasis. Fluids Barriers CNS 2015, 12, 5. [Google Scholar] [CrossRef]
- Novak, E.M.; Metzger, M.; Chammas, R.; da Costa, M.; Dantas, K.; Manabe, C.; Pires, J.; de Oliveira, A.C.; Bydlowski, S.P. Downregulation of TNF-alpha and VEGF expression by Sp1 decoy oligodeoxynucleotides in mouse melanoma tumor. Gene Ther. 2003, 10, 1992–1997. [Google Scholar] [CrossRef] [PubMed][Green Version]
- El-Masry, T.; Al-Shaalan, N.; Tousson, E.; Buabeid, M.; Al-Ghadeer, A. Potential therapy of vitamin B17 against Ehrlich solid tumor induced changes in Interferon gamma, Nuclear factor kappa B, DNA fragmentation, p53, Bcl2, survivin, VEGF and TNF-alpha Expressions in mice. Pak. J. Pharm. Sci. 2020, 33, 393–401. [Google Scholar] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Furuhata, K.; Masuda, H.; Sato, A.; Miyata, K.; Shinyashiki, N.; Kita, R.; Imagawa, K.; Akamatsu, T.; Yagihara, S. Aberrant Water Structure Dynamics in B16 Melanoma-Bearing Mice by Time Domain Refractometry Analysis. Biology 2023, 12, 1250. https://doi.org/10.3390/biology12091250
Furuhata K, Masuda H, Sato A, Miyata K, Shinyashiki N, Kita R, Imagawa K, Akamatsu T, Yagihara S. Aberrant Water Structure Dynamics in B16 Melanoma-Bearing Mice by Time Domain Refractometry Analysis. Biology. 2023; 12(9):1250. https://doi.org/10.3390/biology12091250
Chicago/Turabian StyleFuruhata, Kahori, Haruchika Masuda, Atsuko Sato, Kumiko Miyata, Naoki Shinyashiki, Rio Kita, Kotaro Imagawa, Tadashi Akamatsu, and Shin Yagihara. 2023. "Aberrant Water Structure Dynamics in B16 Melanoma-Bearing Mice by Time Domain Refractometry Analysis" Biology 12, no. 9: 1250. https://doi.org/10.3390/biology12091250
APA StyleFuruhata, K., Masuda, H., Sato, A., Miyata, K., Shinyashiki, N., Kita, R., Imagawa, K., Akamatsu, T., & Yagihara, S. (2023). Aberrant Water Structure Dynamics in B16 Melanoma-Bearing Mice by Time Domain Refractometry Analysis. Biology, 12(9), 1250. https://doi.org/10.3390/biology12091250





