Neural Field Continuum Limits and the Structure–Function Partitioning of Cognitive–Emotional Brain Networks
Abstract
Simple Summary
Abstract
1. Introduction
2. What Is the “Standard Network View” of Brain Structure and Function?
3. Scalability of Local Bidirectional Metaplasticity in Neural Fields
4. Local Parameters force Continuum Limits on the Partitioning and Embedding of Cognitive-Emotional Neural Fields
5. Continuum Limits on a Hybrid Classical-Quantum Model of Neural Fields
5.1. Local Control Parameters limit Structure and Function of Hybrid Classical-Quantum Neural Fields
5.2. State Transitions and Modularity of Hybrid Classical-Quantum Neural Fields
6. Summary
- Neuromanifolds with metaplastic control variables simulate scalable network behavior.
- Simulations identify structure-function limits unresolved by graph analysis of imaged brains.
- Embedded brain networks optimally evolve between exotic computational phases.
- Exotic phases yield degenerate structure-function homogeneities unrealistic of healthy brains.
- Some network partitioning, not unconstrained embeddedness, maximizes brain functionality.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Glossary
- Classical and Quantum Computational Phases are computational states of complex systems, such as natural or technological neural networks, which obey respective classical or quantum statistical mechanics.
- Classical Networks are complex biological and technological networks whose connectivity tends to follow Maxwell-Boltzmann classical statistics rather than Bose-Einstein or Fermi-Dirac quantum statistics.
- Combinatorial and Computational Complexity are classes of complexity defined or measured by a system’s structural (e.g., degree of embeddedness or partitioning) and processing features (e.g., resource allocation and capacity, processing speed, etc.).
- Degenerate Homogeneity is a limiting condition of network structure-function which converges onto a single state, value, or distribution.
- Deterministic Singularity is the operational and/or organizational convergence of a complex biological or technological classical network onto one indistinguishable macroscopic state. The connectivity of these classical networks obeys Maxwell-Boltzmann, Shannon-Einstein, and other classical statistics when nodal strength is described as separate fitness or energy levels and nodal links take on the identity of particle states functioning under associative-like preferential attachment rules. In such cases, a thermodynamic control parameter dictates system behavior and is often represented with a computational annealing parameter, such as space or time. Singularity occurs in the thermodynamic limit.
- Effectively Connected Network is a brain network showing correlated activity supported by known anatomical connectivity.
- Eigenfunctions are a type of eigenvector with nonzero functions acted on by linear functions (e.g., matric operators) in a defined function space which render scalar eigenvalues designating amplitudes for eigenfunctions.
- Equipotentiality is neurophysiological and behavioral phenomena, often attributed to Karl Spencer Lashley, which enable intact cortical brain areas to perform functions, such as memory, lost by other cortical brain areas due to physical damage. The concept of equipotentiality supports and is consistent with overlapping or embedded distributed parallel networks capable of plastic reorganization and functional redundancy. Originally, equipotentiality and the Law of Mass Action were presented as counterarguments to observed structure-function relationships strictly localized to discrete brain areas, such as speech production and comprehension associated with brain language centers.
- Euler Characteristic or Number, named after Leonard Euler, is an algebraic topological measure of spatially invariant structures with a various properties, including, but not limited to, properties for summation, products, fibration, and covering.
- Fitness Level is the nodal weighting parameter for networks governed by associative-like preferential attachment rules.
- Geodesics are paths in Riemannian space that connote spatiotemporal changes in field properties, such as energy or entropy levels which shape field contours and other topological features.
- Graph Theory is the mathematical application of graphs and their properties to describe the behavior and organization of computational objects, such as natural and artificial neural networks. These objects comprise a collection of nodes or vertices connected by directed or undirected edges.
- Hebbian Learning Rules, named after Donald Hebb, are iterative adaptive control mechanisms utilizing activity-dependent bidirectional or dual-processes associative learning rules to either strengthen or weaken nodal connections of an associative (biological or technological) network. The set of learning rules (e.g., cooperativity, coactivity or associativity, synaptic or nodal efficacy/weight, etc.) governing this kind of feedback/feedforward regulation, whether at neuronal synaptic junctions or other nodal forms of computational circuitry, may fall under different subclassifications, such as nonlinear and nonstochastic Hebbian learning, dynamic stochastic synaptic plasticity, spike timing-dependent plasticity, and quantum bidirectional plasticity. When natural or simulated synaptic plasticity occurs that violates Hebbian rules, it is said to be antiHebbian in nature.
- Metaplasticity is the modulation of synaptic plasticity caused by neuromodulatory chemicals and cellular processes.
- Model-Free Functional Network is a biological or technological network organized by activity correlations without presumptive physical connectivity.
- Multistability refers to the activity of a dynamic system with topological minima and maxima representative of different, adaptive system combinatorial and computational states. Systems that are multistable maintain a capacity to organize and operate in states of differential fitness to try to maximize system outcomes under varying contexts.
- Neural Field Theory represents a broad class of mathematical or computational models of brain network organization and operation. This class of models, including mean field theory, relativistic Shannon-Einstein theory, quantum field theory, and other models, employs physiologically relevant control variables, such as synaptic plasticity rules, to approximate the full dynamic range of neural structure-function evolution across different spatiotemporal (neuromanifold) scales. Besides successfully modeling biologically observed local and global brain behavior via relativistic and quantum physics, field theories remain unmatched in their capacity to predict the outcomes of control variable perturbation on brain state.
- Neuroeconomic Principles of Cognitive Computation enlist cellular energetics and organismal bioenergetics concepts and metrics to determine energy or entropy savings and consumption associated with the respective thermodynamic or informational work of scalable cell-based cognitive processes.
- Pleuripotency, sometimes reported as being first described by Rafael Lorente de Nó, is neurophysiological phenomena similar to equipotentiality that endow brain cells, particularly neurons, and circuits, such as cortical columns, with the ability to perform in highly plastic multifunctional capacities which assume traits found for various other cell types and different local brain circuitry. Pleuripotency depends on the nature of structure-function connectivity and may adapt to both weakly and strongly correlated neuronal activity.
- Poincaré or Fundamental Groups, named after Henri Poincaré, are topologically algebriac classes of homotopic space, such as Minkowski spacetime isometries, that define, for example, simply connected (or continuous path-connected), nonsimply connected, and disconnected Riemannian space through invariant or equivalent loop structures.
- Probabilistic Condensation is the operational and/or organizational convergence of a complex biological or technological quantum network onto one indistinguishable macroscopic state. The connectivity of these quantum networks obeys Bose-Einstein statistics when nodal strength is described as separate fitness or energy levels and nodal links take on the identity of particle states functioning under associative-like preferential attachment rules. In such cases, a thermodynamic control parameter dictates system behavior and is often represented with a computational annealing parameter, such as space, time, or the “critical tunneling field strength”. Condensation occurs in the thermodynamic limit.
- Quantum Networks are complex biological and technological networks whose connectivity tends to follow either Bose-Einstein or Fermi-Dirac quantum statistics rather than Maxwell-Boltzmann classical statistics.
- Quantum or Bloch Spheres, named after Felix Bloch, are quantum-mechanical geometric visualizations mapped onto a unitary 2-sphere Riemannian space with mutual orthogonal basis state vectors serving as antipodes (or poles). Spheres correspond to 2D Hilbert spaces, with surface points being pure quantum states and interior points being mixed ones.
- Resting State or Intrinsically Connected Neural Activity is correlated spontaneous wakeful brain activity.
- Riemannian Neuromanifold, named after Bernhard Riemann, is a neural field or matrix that conforms to Riemannian geometry of curved space, such as positive and negative curvature, not explained by flat 3D Euclidean geometry. The structure-function relationships of such manifolds are simplified and more fully captured by expanding the Pythagorean theorem to arbitrary dimensions with a metric tensor that describes relative local and global properties of a field.
- Small-World and Scale-Free Networks are classes of complex biological and technological networks with unique structure-function properties, such as the distribution, number, and local size of nodal neighborhoods, the length of connecting paths, and network growth rates. The global behavior and organization of a complex network tends to evolve according to a logarithmic law of connectivity for small-world objects and to a power law of connectivity for scale-free objects.
- Structure–Function Embedding and Partitioning is the respective encapsulation, comparmentalization, discetization, or parcellation of a biological or technological network into structural and functional units.
- Thermodynamic Bound is the structure-function limit forced by a control parameter on a biological or technological system, such as natural and artificial neural networks. Control parameters may be actual absolute ambient temperature or computational annealing parameters, such as space, time, or the “critical tunneling field strength”.
- Topographic Network Theory is the physical or logical description of network structure and function using various mathematical models, including, among other frameworks, mean-field, Shannon-Einstein, and quantum theories.
- Wolfram Complexity Classes, named after Stephen Wolfram, are a set of four computational complexity classes numbered in ascending order of complexity. Class I complexity displays simple behavior, with nearly all initial conditions driving the system toward the same uniform final state. Class II complexity returns many different final states, with each containing a certain set of simple structures that either always remain the same or cycle through structural iterations after repeated computational steps. Class III complexity shows more complicated behavior, appearing almost random with some simple small-scale structural elements always present. Class IV complexity presents a mixture of ordered and random behavior, with local simple structures which interact in a highly complex manner.
References
- Pessoa, L. The Cognitive-Emotional Brain: From Interactions to Integration; The MIT Press: Cambridge, MA, USA, 2013. [Google Scholar]
- Dewsbury, D.A. Monkey Farm: A History of the Yerkes Laboratories of Primate Biology, Orange Park, Florida, 1930; Associated University Presses: Cranbury, NJ, USA, 2006. [Google Scholar]
- Hearst, E. The First Century of Experimental Psychology; Lawrence Erlbaum Associates, Inc.: Hillsdale, NJ, USA, 1965. [Google Scholar]
- Larriva-Sahd, J.A. Some predictions of Rafael Lorente de Nó 80 years later. Front. Neuroanat. 2014, 8, 147. [Google Scholar] [CrossRef] [PubMed]
- Bullmore, E.; Sporns, O. Complex brain networks: Graph theoretical analysis of structural and functional systems. Nat. Rev. Neurosci. 2009, 10, 186–198. [Google Scholar] [CrossRef] [PubMed]
- Damoiseaux, J.S.; Greicius, M.D. Greater than the sum of its parts: A review of studies combining structural connectivity and resting-state functional connectivity. Brain Struct. Funct. 2009, 213, 525–533. [Google Scholar] [CrossRef] [PubMed]
- Kelso, J.A.S. Dynamic Patterns: The Self-Organization of Brain and Behavior; The MIT Press: Cambridge, MA, USA, 1995. [Google Scholar]
- Clark, K.B. A statistical mechanics definition of insight. In Computational Intelligence; Floares, A., Ed.; Nova Science Publishers, Inc.: Hauppauge, NY, USA, 2012; pp. 139–162. [Google Scholar]
- Clark, K.B. Evolution of affective and linguistic disambiguation under social eavesdropping pressures. Behav. Brain Sci. 2014, 37, 551–552. [Google Scholar] [CrossRef]
- Clark, K.B. Cognitive completeness of quantum teleportation and superdense coding in neuronal response regulation and plasticity. Proc. R. Soc. B Biol. Sci. 2014. Available online: https://royalsocietypublishing.org/action/downloadSupplement?doi=10.1098%2Frspb.2013.3056&file=royprsb_el.pdf (accessed on 2 November 2022).
- Clark, K.B.; Hassert, D.L. Undecidability and opacity of metacognition in animals and humans. Front. Psychol. 2013, 4, 171. [Google Scholar] [CrossRef]
- Krahl, S.; Clark, K. Vagus nerve stimulation for epilepsy: A review of central mechanisms. Surg. Neurol. Int. 2012, 3, S255–S259. [Google Scholar] [CrossRef] [PubMed]
- Clark, K.B. Basis for a neuronal version of Grover’s quantum algorithm. Front. Mol. Neurosci. 2014, 7, 29. [Google Scholar] [CrossRef]
- Malenka, R.C.; Bear, M.F. LTP and LTD: An embarrassment of riches. Neuron 2004, 55, 5–21. [Google Scholar] [CrossRef]
- Landman, K.A.; Fernando, A.E.; Zhang, D.; Newgreen, D.F. Building stable chains with motile agents: Insights into the morphology of enteric neural crest cell migration. J. Theor. Biol. 2011, 276, 250–268. [Google Scholar] [CrossRef]
- Xu, J.X.; Deng, X. Biological modeling of complex chemotaxis behavior for C. elegans under speed regulation—A dynamic neural network approach. J. Comput. Neurosci. 2013, 35, 19–37. [Google Scholar] [CrossRef]
- Cuntz, H.; Forstner, F.; Borst, A.; Häusser, M. One Rule to Grow Them All: A General Theory of Neuronal Branching and Its Practical Application. PLoS Comput. Biol. 2010, 6, e1000877. [Google Scholar] [CrossRef] [PubMed]
- Duckro, D.E.; Quinn, D.W.; Gardner, S.J. Neural Network Pruning with Tukey-Kramer Multiple Comparison Procedure. Neural Comput. 2002, 14, 1149–1168. [Google Scholar] [CrossRef] [PubMed]
- Miyoshi, S.; Okada, M. Storage Capacity Diverges with Synaptic Efficiency in an Associative Memory Model with Synaptic Delay and Pruning. IEEE Trans. Neural Netw. 2004, 15, 1215–1227. [Google Scholar] [CrossRef] [PubMed]
- Toussaint, U.V.; Gori, S.; Dose, V. Invariance priors for Bayesian feed-forward neural networks. Neural Netw. 2006, 19, 1550–1557. [Google Scholar] [CrossRef]
- Aizenman, C.D.; Pratt, K.G. There’s more than one way to scale a synapse. Neuron 2008, 58, 651–652. [Google Scholar] [CrossRef] [PubMed]
- Godfrey, K.B.; Eglen, S.J.; Swindale, N.V. A multicomponent model of the developing retinocollicular pathway incorpprating axonal and synaptic growth. PLoS Comput. Biol. 2009, 5, e1000600. [Google Scholar] [CrossRef] [PubMed]
- Needleman, L.A.; McAllister, A.K. Seeing the Light: Insulin Receptors and the CNS. Neuron 2008, 58, 653–655. [Google Scholar] [CrossRef]
- Swindale, N.V. The development of topography in the visual cortex: A review of models. Network 1996, 7, 161–247. [Google Scholar] [CrossRef]
- Robinson, P.A. Physical brain connectomics. Phys. Rev. E 2019, 99, 012421. [Google Scholar] [CrossRef]
- Buzsáki, G. Rhythms of the Brain; Oxford University Press: Oxford, UK, 2006. [Google Scholar]
- Friston, K. Beyond phrenology: What can neuroimaging tell us about distributed circuitry? Annu. Rev. Neurosci. 2002, 25, 221–250. [Google Scholar] [CrossRef]
- Hutchison, R.M.; Womelsdorf, T.; Allen, E.A.; Bandettini, P.A.; Calhoun, V.D.; Corbetta, M.; Della Penna, S.; Duyn, J.H.; Glover, G.H.; Gonzalez-Castillo, J.; et al. Dynamic function connectivity: Promise, issues, and interpretations. NeuroImage 2013, 80, 360–378. [Google Scholar] [CrossRef] [PubMed]
- Pessoa, L. Précise of the cognitive-emotional brain. Behav. Brain Sci. 2014, 38, e71. [Google Scholar] [CrossRef] [PubMed]
- Glaser, E.M.; Ruchkin, D.S. Principles of Neurobiological Signal Analysis; Academic Press: New York, NY, USA, 1976. [Google Scholar]
- Meunier, D.; Achard, S.; Morcom, A.; Bullmore, E. Age-related changes in modular organization of human brain functional networks. Neuroimage 2009, 44, 715–723. [Google Scholar] [CrossRef]
- Schneidman, E.; Berry, M.J.; 2nd Segev, R.; Bialek, W. Weak pairwise correlations imply strongly correlated network states in a neural population. Nature 2006, 440, 1007–1012. [Google Scholar] [CrossRef]
- Mantini, D.; Gerits, A.; Nelissen, K.; Durand, J.-B.; Joly, O.; Simone, L.; Sawamura, H.; Wardak, C.; Orban, G.; Buckner, R.L.; et al. Default Mode of Brain Function in Monkeys. J. Neurosci. 2011, 31, 12954–12962. [Google Scholar] [CrossRef] [PubMed]
- Tyszka, J.M.; Kennedy, D.P.; Adolphs, R.; Paul, L. Intact Bilateral Resting-State Networks in the Absence of the Corpus Callosum. J. Neurosci. 2011, 31, 15154–15162. [Google Scholar] [CrossRef]
- Allport, D.A. Distributed memory, modular systems and dysphasia. In Current Perspectives in Dysphasia; Newman, S., Epstein, R., Eds.; Churchill Livingstone: Edinburgh, UK, 1985; pp. 32–60. [Google Scholar]
- Arieli, A.; Sterkin, A.; Grinvald, A.; Aertsen, A. Dynamics of ongoing activity: Explanation of the large variability in evoked cortical responses. Science 1996, 273, 1868–1871. [Google Scholar] [CrossRef]
- Bi, G.-Q.; Poo, M.-M. Synaptic modification by correlated activity: Hebb’s postulate revisited. Annu. Rev. Neurosci. 2001, 24, 139–166. [Google Scholar] [CrossRef]
- Hebb, D.O. The Organization of Behavior: A Neuropsychological Theory; Wiley: New York, NY, USA, 1949. [Google Scholar]
- Roberts, P.D.; Lean, T.K. Anti-Hebbian spike-timing-dependent plasticity and adaptive sensory processing. Front. Comput. Neurosci. 2010, 4, 156. [Google Scholar] [CrossRef]
- Salinas, E.; Sejnowski, T.J. Correlated neuronal activity and the flow of neural information. Nat. Rev. Neurosci. 2001, 2, 539–550. [Google Scholar] [CrossRef]
- Sejnowski, T.J. The book of Hebb. Neuron 1999, 24, 773–776. [Google Scholar] [CrossRef] [PubMed]
- Cooper, S.; Donald, O. Hebb’s synapse and learning rule: A history and commentary. Neurosci. Biobehav. Rev. 2008, 28, 851–874. [Google Scholar] [CrossRef] [PubMed]
- Feldman, D.E. Synaptic mechanisms for plasticity in neocortex. Annu. Rev. Neurosci. 2009, 32, 33–55. [Google Scholar] [CrossRef] [PubMed]
- Paulsen, O.; Sejnowski, T. Natural patterns of activity and long-term synaptic plasticity. Curr. Opin. Neurobiol. 2000, 10, 172–179. [Google Scholar] [CrossRef]
- Damaraju, E.; Huang, Y.M.; Barrett, L.F.; Pessoa, L. Affective learning enhances activity and functional connectivity in early visual cortex. Neuropsychologia 2009, 47, 2480–2487. [Google Scholar] [CrossRef]
- Huber, K.M.; Sawtell, N.B.; Bear, M.F. Brain-derived neurotrophic factor alters the synaptic modification threshold in visual cortex. Neuropharmacology 1998, 37, 571–579. [Google Scholar] [CrossRef]
- Meliza, C.D.; Dan, Y. Receptive-field modification in rat visual cortex induced by paired visual stimulation and single-cell spiking. Neuron 2006, 49, 183–189. [Google Scholar] [CrossRef]
- Salgado, H.; Köhr, G.; Treviño, M. Noradrenergic ‘tone’ determines dichotomous control of cortical spike-timing-dependent plasticity. Sci. Rep. 2012, 2, 417. [Google Scholar] [CrossRef]
- Song, S.; Abbott, L. Cortical development and remapping through spike timing-dependent plasticity. Neuron 2001, 32, 339–350. [Google Scholar] [CrossRef]
- Bear, M.F. Bidirectional synaptic plasticity: From theory to reality. Philos. Trans. R. Soc. B Biol. Sci. 2003, 358, 649–655. [Google Scholar] [CrossRef]
- Bienenstock, E.L.; Cooper, L.; Munro, L. Theory for the development of neuron selectivity: Orientation specificity and binocular interaction in visual cortex. J. Neurosci. 1982, 21, 32–48. [Google Scholar] [CrossRef] [PubMed]
- Oja, E. A simplified neuron model as a principal component analyzer. J. Math. Biol. 1982, 15, 267–273. [Google Scholar] [CrossRef] [PubMed]
- Oja, E.; Karhunen, J. On stochastic approximation of the eigenvectors and eigenvalues of the expectation of a random matrix. J. Math. Anal. Appl. 1985, 106, 69–84. [Google Scholar] [CrossRef]
- Maass, W.; Zador, A.M. Dynamic stochastic synapses as computational units. Neural Comput. 1999, 11, 903–917. [Google Scholar] [CrossRef]
- Caporale, N.; Dan, Y. Spike timing-dependent plasticity: A Hebbian learning rule. Annu. Rev. Neurosci. 2008, 31, 25–46. [Google Scholar] [CrossRef]
- Frégnac, V.; Pananceau, M.; René, A.; Huguet, N.; Marre, O.; Levy, M.; Shulz, D.E. A re-examinatin of Hebbian-covariance rules and spike-timing-dependent plasticity in cat visual cortex in vivo. Front. Synaptic Neurosci. 2010, 2, 147. [Google Scholar] [CrossRef]
- Pawlak, V.; Greenberg, D.S.; Sprekeler, H.; Gerstner, W.; Kerr, J.N.D. Changing the response of cortical neurons from subthreshold to suprathreshold using single spikes in vivo. eLIFE 2013, 2, e00012. [Google Scholar] [CrossRef]
- Schmidt, S.L.; Chew, E.Y.; Bennett, D.V.; Hammad, M.A.; Fröhlich, F. Differential effects of cholinergic and noradrenergic neuromodulation on spontaneous cortical network dynamics. Neuropharmacology 2013, 72, 259–273. [Google Scholar] [CrossRef]
- Young, J.M.; Waleszczyk, W.J.; Wang, C.; Calford, M.B.; Dreher, B.; Obermayer, K. Cortical reorganization consistent with spike timing-but not correlation-dependent plasticity. Nat. Neurosci. 2007, 10, 887–895. [Google Scholar] [CrossRef]
- Marder, E.; Goaillard, J.M. Variability, compensation and homeostasis in neuron and network function. Nat. Rev. Neurosci. 2006, 7, 563–574. [Google Scholar] [CrossRef]
- Turrigiano, G.G. The self-tuning neuron: Synaptic scaling of excitatory synapses. Cell 2008, 135, 422–435. [Google Scholar] [CrossRef] [PubMed]
- Amari, S.; Park, H.; Ozeki, T. Singularities affect dynamics of learning in neuromanifolds. Neural Comput. 2006, 18, 1007–1065. [Google Scholar] [CrossRef] [PubMed]
- Clark, K.B. The humanness of artificial nonnormative personalities. Behav. Brain Sci. 2017, 40, e259. [Google Scholar] [CrossRef] [PubMed]
- Goldberg, J.; Holthoff, K.; Yuste, R. A problem with Hebb and local spikes. Trends Neurosci. 2002, 25, 433–435. [Google Scholar] [CrossRef]
- Stein, L.; Belluzzi, J.D. Cellular investigations of behavioral reinforcement. Neurosci. Biobehav. Rev. 1989, 13, 69–80. [Google Scholar] [CrossRef]
- Stein, L.; Xue, B.G.; Belluzzi, J.D. In Vitro reinforcement of hippocampal bursting: A search for Skinner’s atoms of behavior. J. Exp. Anal. Behav. 1994, 61, 155–168. [Google Scholar] [CrossRef]
- Kirkwood, A.; Rozas, C.; Kirkwood, J.; Perez, F.; Bear, M.F. Modulation of long-term synaptic depression in visual cortex by acetylcholine and norepinephrine. J. Neurosci. 1999, 19, 1599–1609. [Google Scholar] [CrossRef]
- Neuman, R.S.; Harley, C.W. Long-lasting potentiation of the dentate gyrus population spike by norepinephrine. Brain Res. 1983, 273, 162–165. [Google Scholar] [CrossRef]
- Bialas, A.R.; Stevens, B. TGF-β signaling regulates neuronal C1q expression and developmental synaptic refinement. Nat. Neurosci. 2013, 16, 1773–1782. [Google Scholar] [CrossRef]
- Battaglia, D.; Witt, A.; Wolf, F.; Geisel, T. Dynamic effective connectivity of inter-areal brain circuits. PLoS Comput. Biol. 2012, 8, e1002438. [Google Scholar] [CrossRef]
- Bressloff, P.C.; Webber, M.A. Neural field model of binocular rivalry waves. J. Comput. Neurosci. 2012, 32, 233–252. [Google Scholar] [CrossRef] [PubMed]
- Friston, K.J.; Kahan, J.; Razi, A.; Stephan, K.E.; Sporns, O. On nodes and modes in resting state fMRI. NeuroImage 2014, 99, 533–547. [Google Scholar] [CrossRef] [PubMed]
- Jirsa, V.K. Connectivity and dynamics of neural information processing. Neuroinformatics 2004, 2, 183–204. [Google Scholar] [CrossRef] [PubMed]
- Vuksanović, V.; Hövel, P. Functional connectivity of distant cortical regions: Role of remote synchronization and symmetry in interactions. NeuroImage 2014, 97, 1–8. [Google Scholar] [CrossRef]
- Capolupo, A.; Freeman, W.J.; Vitello, G. Dissipation of ‘dark energy’ by cortex in knowledge retrieval. Phys. Life Rev. 2013, 10, 85–94. [Google Scholar] [CrossRef]
- Chauvet, G.A. An n-level field theory of biological neural networks. J. Math. Biol. 1993, 31, 771–795. [Google Scholar] [CrossRef]
- Haeusler, S.; Schuch, K.; Maass, W. Motif distribution, dynamical properties, and computational performance of two data-based cortical microcircuit templates. J. Physiol. 2009, 103, 73–87. [Google Scholar] [CrossRef]
- Lerchner, A.; Sterner, G.; Hertz, J.; Ahmadi, M. Mean field theory for a balanced hypercolumn model of orientation selectivity in primary visual cortex. Network 2006, 17, 131–150. [Google Scholar] [CrossRef]
- Perlovsky, L.I. Neural Networks and Intellect: Using Model-Based Concepts; Oxford University Press: New York, NY, USA, 2001. [Google Scholar]
- Romani, S.; Amit, D.J.; Mongillo, G. Mean-field analysis of selective persistent activity in presence of short-term synaptic depression. J. Comput. Neurosci. 2006, 20, 201–217. [Google Scholar] [CrossRef]
- Clark, K.B. Bose-Einstein condensates form in heuristics learned by ciliates deciding to signal ‘social’ commitments. BioSystems 2010, 99, 167–178. [Google Scholar] [CrossRef]
- Clark, K.B. Arrhenius-kinetics evidence for quantum tunneling in microbial “social” decision rates. Commun. Integr. Biol. 2010, 3, 540–544. [Google Scholar] [CrossRef] [PubMed]
- Clark, K.B. Social biases determine spatiotemporal sparseness of ciliate mating heuristics. Commun. Integr. Biol. 2012, 5, 3–11. [Google Scholar] [CrossRef] [PubMed]
- Clark, K.B. Insight and analysis problem solving in microbes to machines. Prog. Biophys. Mol. Biol. 2015, 119, 183–193. [Google Scholar] [CrossRef] [PubMed]
- Clark, K.B. Classical and quantum cell-cell signaling by microbial life on Earth and possible other livable worlds. Bull. Am. Astron. Soc. 2021, 53, 32. [Google Scholar]
- Clark, K.B. Classical and quantum information processing in aneural to neural cellular decision making on Earth and perhaps beyond. Bull. Am. Astron. Soc. 2021, 53, 33. [Google Scholar]
- Bianconi, G.; Barabási, A.-L. Bose-Einstein condensation in complex networks. Phys. Rev. Lett. 2001, 86, 5632–5635. [Google Scholar] [CrossRef]
- Bianconi, G. Growing Cayley trees described by a Fermi distribution. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2002, 66, 036116. [Google Scholar] [CrossRef]
- Bianconi, G. Quantum statistics in complex networks. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2002, 66, 056123. [Google Scholar] [CrossRef]
- Bianconi, G. Size of quantum networks. Phys. Rev. E Stat. Nonlinear Soft Matter Phys. 2003, 67, 056119. [Google Scholar] [CrossRef]
- Clark, K.B. Quantum decision corrections for the neuroeconomics of irrational movement control and goal attainment. Behav. Brain Sci. 2021, 44, e127. [Google Scholar] [CrossRef]
- Killoran, N. Continuous-variable quantum neural networks. Phys. Rev. Res. 2019, 1, 033063. [Google Scholar] [CrossRef]
- Maass, W. Networks of spiking neurons: The third generation of neural network models. Neural Netw. 1997, 10, 1659–1671. [Google Scholar] [CrossRef]
- Buesing, L.; Bill, J.; Nessler, B.; Maass, W. Neural dynamics as sampling: A model for stochastic computation in recurrent networks of spiking neurons. PLoS Comput. Biol. 2011, 7, e1002211. [Google Scholar] [CrossRef] [PubMed]
- Buonomano, D.V.; Maass, W. State-dependent computations: Spatiotemporal processing in cortical networks. Nature Reviews Neurosci. 2009, 10, 113–125. [Google Scholar] [CrossRef] [PubMed]
- Steimer, A.; Maass, W.; Douglas, R. Belief propagation in networks of spiking neurons. Neural Comput. 2009, 21, 2502–2523. [Google Scholar] [CrossRef] [PubMed]
- Hoerzer, G.M.; Legenstein, R.; Maass, W. Emergence of complex computational structures from chaotic neural networks through reward-modulated Hebbian learning. Cereb. Cortex 2014, 24, 677–690. [Google Scholar] [CrossRef]
- Clark, K.B. Origins of learned reciprocity in solitary ciliates searching grouped ‘courting’ assurances at quantum efficiencies. BioSystems 2010, 99, 27–41. [Google Scholar] [CrossRef]
- Clark, K.B. On classical and quantum error-correction in ciliate mate selection. Commun. Integr. Biol. 2010, 3, 374–378. [Google Scholar] [CrossRef]
- da Silva, A.J.; Ludermir, T.B.; de Oliveira, W.R. Quantum perceptron over a field and neural network architecture selection in a quantum computer. Neural Netw. 2016, 76, 55–64. [Google Scholar] [CrossRef]
- Fagerholm, E.D.; Foulkes, W.M.C.; Friston, K.J.; Moran, R.J.; Leech, R. Rendering neuronal state equations compatible with the principle of stationary action. J. Math. Neurosci. 2021, 11, 10. [Google Scholar] [CrossRef]
- Hu, W. Towards a real quantum neuron. Nat. Sci. 2018, 10, 99–109. [Google Scholar] [CrossRef]
- Kapoor, A.; Wiebe, N.; Svore, K. Quantum perceptron models. Adv. Neural Inf. Process. Syst. 2016, 29, 3999–4007. [Google Scholar]
- Kristensen, L.B.; Degroote, M.; Wittek, P.; Aspuru-Guzik, A.; Zinner, N.T. An artificial spiking quantum neuron. NPJ Quantum Inf. 2021, 7, 59. [Google Scholar] [CrossRef]
- Monteiro, C.A.; Filho, G.I.S.; Costa, M.H.J.; de Paula Neto, F.M.; de Oliveira, W.R. Quantum neuron with real weights. Neural Netw. 2021, 143, 698–708. [Google Scholar] [CrossRef]
- Shang, F.H. Quantum-inspired neural network with quantum weights and real weights. Open J. Appl. Sci. 2015, 5, 609–617. [Google Scholar] [CrossRef]
- Tacchino, F.; Macchiavello, C.; Gerace, D.; Bajoni, D. An artificial neuron implemented on an actual quantum processor. Npj Quantum Inf. 2019, 5, 26. [Google Scholar] [CrossRef]
- Yan, S.; Qi, H.; Cui, W. Nonlinear quantum neuron: A fundamental building block for quantum neural networks. Phys. Rev. A 2020, 102, 052421. [Google Scholar] [CrossRef]
- Bialas, P.; Spiechowicz, J.; Luczka, J. Quantum analogue of energy equipartition theorem. arXiv 2018, arXiv:1805.04012. [Google Scholar] [CrossRef]
- Maronese, M.; Destri, C.; Prati, E. Quantum activation functions for quantum neural networks. Quantum Inf. Process. 2022, 21, 128. [Google Scholar] [CrossRef]
- Nielsen, M.A.; Chuang, L.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Carter, A.H. Classical and Statistical Thermodynamics; Prentice-Hall, Inc.: New Jersey, NJ, USA, 2001. [Google Scholar]
- Griffiths, D.J. Introduction to Quantum Mechanics; Addison-Wesley: Toronto, ON, Canada, 2005. [Google Scholar]
- Gottwald, S.; Braun, D.A. Two kinds of free energy and the Bayesian revolution. PLOS Comput. Biol. 2020, 16, e1008420. [Google Scholar] [CrossRef]
- Gunji, Y.-P.; Shinohara, S.; Basios, V. Connecting the free energy principle with quantum cognition. Front. Neurorobotics 2022, 16, 910161. [Google Scholar] [CrossRef] [PubMed]
- Bennett, C.H. Notes on Landauer’s principle, reversible computation, and Maxwell’s demon. Stud. Hist. Philos. Mod. Phys. 2003, 340, 501–510. [Google Scholar] [CrossRef]
- Ladyman, J.; Presnell, S.; Short, A.J.; Groisman, B. The connection between logical and thermodynamic irreversibility. Stud. Hist. Philos. Mod. Phys. 2007, 38, 58–79. [Google Scholar] [CrossRef]
- Bekenstein, J.D. Black holes and information theory. Contemp. Phys. 2004, 45, 31–43. [Google Scholar] [CrossRef]
- McMahon, R.J. Chemical reactions involving quantum tunneling. Science 2003, 299, 833–834. [Google Scholar] [CrossRef]
- Hawkes, R.B.; Holberton, D.V. Myonemal contraction of Spirostomum. III The thermal dependence of contraction, relaxation and excitation-contraction coupling. J. Cell Physiol. 1975, 87, 253–264. [Google Scholar] [CrossRef]
- Holwill, M.E.J.; Silvester, N.R. The thermal dependence of flagellar activity in Strigomonus oncopelti. J. Exp. Biol. 1965, 42, 537–544. [Google Scholar] [CrossRef]
- Holwill, M.E.J.; Silvester, N.R. Thermodynamic aspects of flagellar activity. J. Exp. Biol. 1967, 47, 249–265. [Google Scholar] [CrossRef]
- Sleigh, M.A. Metachronism and frequency of beat in the peristomial cilia of Stentor. J. Exp. Biol. 1956, 33, 15–28. [Google Scholar] [CrossRef]
- De Neys, W. Advancing theorizing about slow-and-fast thinking. Behav. Brain Sci. 2022. [Google Scholar] [CrossRef]
- Tipler, P.A. Physics for Scientists and Engineers; Worth Publishers: New York, NY, USA, 1991. [Google Scholar]
- Clark, K.B. Ciliates learn to diagnose and correct classical error syndromes in mating strategies. Front. Microbiol. 2013, 4, 229. [Google Scholar] [CrossRef] [PubMed]
- Jarzynski, C. Nonequilibrium equality for free energy differences. Phys. Rev. Lett. 1997, 78, 2690. [Google Scholar] [CrossRef]
- Prentis, J.J.; Fedak, W.A. Energy conservation in quantum mechanics. Am. J. Phys. 2002, 72, 580. [Google Scholar] [CrossRef]
- Langton, C.G. Computation at the edge of chaos: Phase transitions and emergent computation. Phys. D 1990, 42, 12–37. [Google Scholar] [CrossRef]
- Wolfram, S. Universality and complexity in cellular automata. Phys. D 1984, 10, 1–35. [Google Scholar] [CrossRef]
- Gekle, S.; Main, J.; Bartsch, T.; Uzer, T. Extracting multidimensional phase space topology from periodic orbits. Phys. Rev. Lett. 2007, 97, 104101. [Google Scholar] [CrossRef]
- Takens, F. Detecting strange attractors in turbulence. In Dynamical Systems and Turbulence; Rand, D., Yong, L., Eds.; Springer: Berlin, Germany, 1981; pp. 366–381. [Google Scholar]
- Mukta, K.N.; Gao, K.; Robinson, P.A. Neural field theory of evoked response potentials in a spherical brain geometry. Phys. Rev. E 2019, 99, 062304. [Google Scholar] [CrossRef]
- Jumarie, G. Relative Information: Theories and Applications; Springer Series in Synergetics; Springer: Berlin/Heidelberg, Germany, 1990. [Google Scholar]
- Ezaki, T.; Watanabe, T.; Ohzeki, M.; Masuda, N. Energy landscape analysis of neuroimaging data. Philosophical Transactions of the Royal Society A 2017, 375, 20160287. [Google Scholar] [CrossRef]
- Mukta, K.N.; Robinson, P.A.; Pagès, J.C.; Gabay, N.C.; Gao, X. Evoked response activity eigenmode analysis in a convoluted cortex via neural field theory. Phys. Rev. E 2020, 102, 062303. [Google Scholar] [CrossRef]
- Nunez, P.L. Generation of human EEG by a combination of long and short range neocortical interactions. Brain Topogr. 1989, 1, 199–215. [Google Scholar] [CrossRef]
- Buice, M.A.; Chow, C.C. Beyond mean field theory: Statisitcal field theory for neural networks. J. Stat. Mech. 2013, 2013, P03003. [Google Scholar] [CrossRef]
- Deco, G.; Jirsa, V.K.; Robinson, P.A.; Breakspear, M.; Friston, K. The dynamic brain: From spiking neurons to neural masses and cortical fields. PLoS Comput. Biol. 2008, 4, e1000092. [Google Scholar] [CrossRef] [PubMed]
- Fung, P.K.; Robinson, P.A. Neural field theory of synaptic metaplasticity with applications to theta burst simulation. J. Theor. Biol. 2014, 340, 164–178. [Google Scholar] [CrossRef] [PubMed]
- Pinotsis, D.A.; Leite, M.; Friston, K.J. On conductance-based neural field models. Front. Comput. Neurosci. 2013, 7, 158. [Google Scholar] [CrossRef]
- Robinson, P.A. Neural field theory of synaptic plasticity. J. Theor. Biol. 2011, 285, 156–163. [Google Scholar] [CrossRef]
- Sanz-Leon, P.; Robinson, P.A.; Knock, S.A.; Drysdale, P.M.; Abeysuriya, R.G.; Fung, F.K.; Rennie, C.J.; Zhao, X. NFTsim: Theory and simulation of multiscale neural field dynamics. PLoS Comput. Biol. 2018, 14, e1006387. [Google Scholar] [CrossRef]
- Bowers, J.S.; Malhotra, G.; Dujmović, M.; Montero, M.L.; Tsvetkov, C.; Biscione, V.; Puebla, G.; Adolfi, F.; Hummel, J.E.; Heaton, R.F.; et al. Deep problems with neural network models of human vision. Behav. Brain Sci. 2022, 1–74. [Google Scholar] [CrossRef] [PubMed]
- Gleichgerrcht, E.; Kocher, M.; Bonilha, L. Connectomics and graph theory analyses: Novel insights into network abnormalities in epilepsy. PLoS Comput. Biol. 2015, 7, 158. [Google Scholar] [CrossRef]
- Szalkai, B.; Varga, B.; Grolmusz, V. The graph of our mind. Brain Sci. 2021, 11, 342. [Google Scholar] [CrossRef]
- Thibat, F. New ways of understanding brain microcircuitry. Dialogues Clin. Neurosci. 2018, 20, 83–84. [Google Scholar] [CrossRef]
- Zhang, Y.; Jia, S.; Yu, Z.; Huang, T.; Liu, J.K.; Tian, Y. Probabilistic inference of binary Markov random fields in spiking neural networks through mean-field approximation. Neural Netw. 2020, 126, 42–51. [Google Scholar] [CrossRef] [PubMed]
- Calimera, A.; Macii, E.; Poncino, M. The human brain project and neuromorphic computing. Funct. Neurol. 2013, 28, 191–196. [Google Scholar] [PubMed]
- McDonough, I.M.; Nashiro, K. Network complexity as a measure of information processing across resting-state networks: Evidence from the Human Connectome Project. Front. Hum. Neurosci. 2014, 8, 409. [Google Scholar] [CrossRef] [PubMed]
- Sporns, O.; Tononi, G.; Kötter, R. The human connectome: A structural description of the human brain. PLoS Comput. Biol. 2005, 1, e42. [Google Scholar] [CrossRef]
- Gkigkitzis, I.; Haranas, I.; Kotsireas, I. Biological relevance of network architecture. Adv. Exp. Med. Biol. 2017, 988, 1–29. [Google Scholar]
- Subramanian, I.; Verma, S.; Kumar, S.; Jere, A.; Anamika, K. Multi-omics data integration, interpretation, and Its application. Bioinform. Biol. Insights 2020, 14, 1177932219899051. [Google Scholar] [CrossRef]
- Yan, J.; Risacher, S.L.; Shen, L.; Saykin, A.J. Network approaches to systems biology analysis of complex disease: Integrative methods for multi-omics data. Brief. Bioinf. 2018, 19, 1370–1381. [Google Scholar] [CrossRef]
- Gao, X.; Robinson, P.A. Importance of self-connections for brain connectivity and spectral connectomics. Biol. Cybern. 2020, 114, 643–651. [Google Scholar] [CrossRef]
- Müller, E.J.; Munn, B.R.; Aquino, K.M.; Shine, J.M.; Robinson, P.A. The music of the hemispheres: Cortical eigenmodes as a physical basis for large-scale brain activity and connectivity patterns. Front. Hum. Neurosci. 2022, 16, 1062487. [Google Scholar] [CrossRef]
- Petkoski, S.; Jirsa, V.K. Normalizing the brain connectome for communication through synchronization. Netw. Neurosci. 2022, 6, 722–744. [Google Scholar] [CrossRef]
- Pang, J.C.; Aquino, K.M.; Oldehinkel, M.; Robinson, P.A.; Fulcher, B.D.; Breakspear, M.; Fornito, A. Geometric constraints on human brain function. BioRxiv 2022. [Google Scholar] [CrossRef]
- Robinson, P.A.; Henderson, J.A.; Gabay, N.C.; Aquino, K.M.; Babaie-Janvier, T.; Gao, X. Determination of dynamic brain connectivity via spectral analysis. Front. Hum. Neurosci. 2021, 15, 655576. [Google Scholar] [CrossRef] [PubMed]
- del Zangari Balzo, G. Statistical field theory of the transmission of nerve impulses. Theor. Biol. Med. Model. 2021, 18, 1. [Google Scholar] [CrossRef] [PubMed]
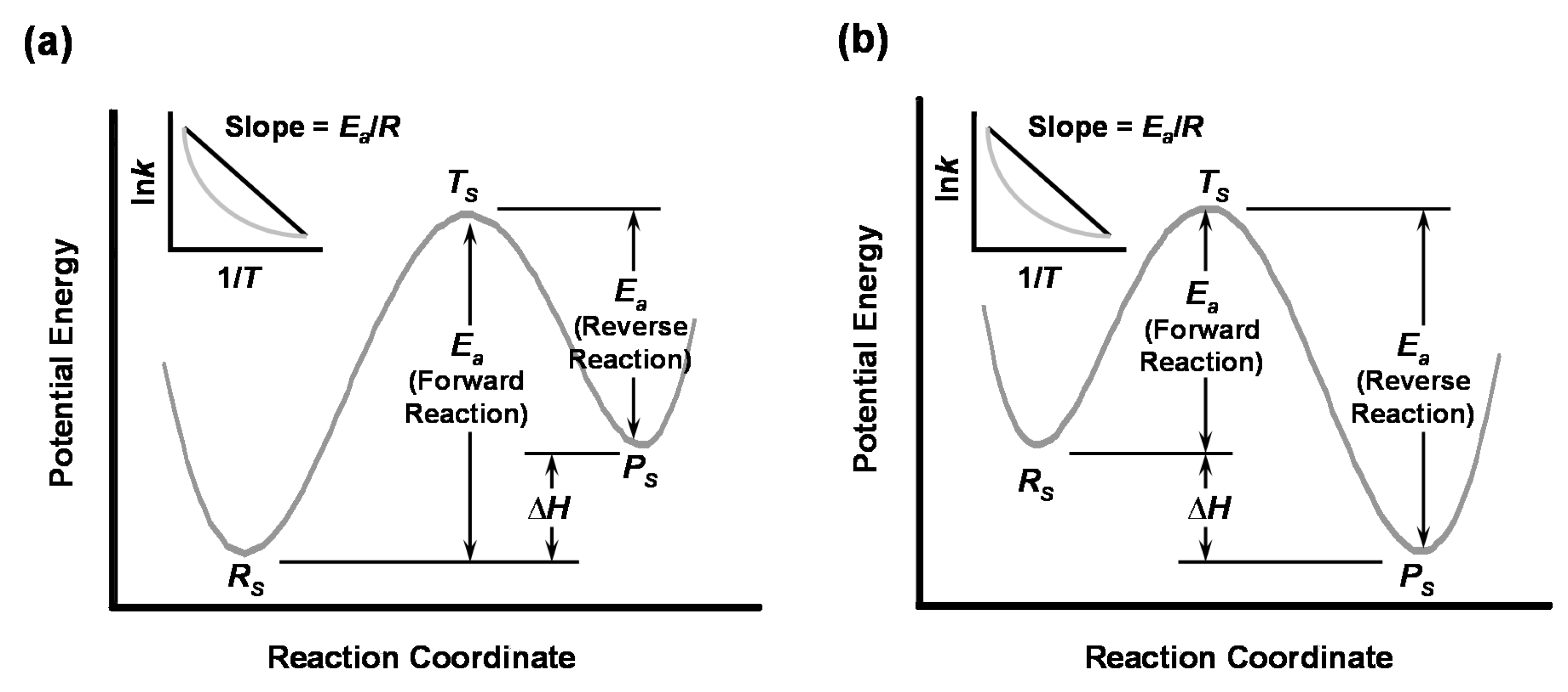
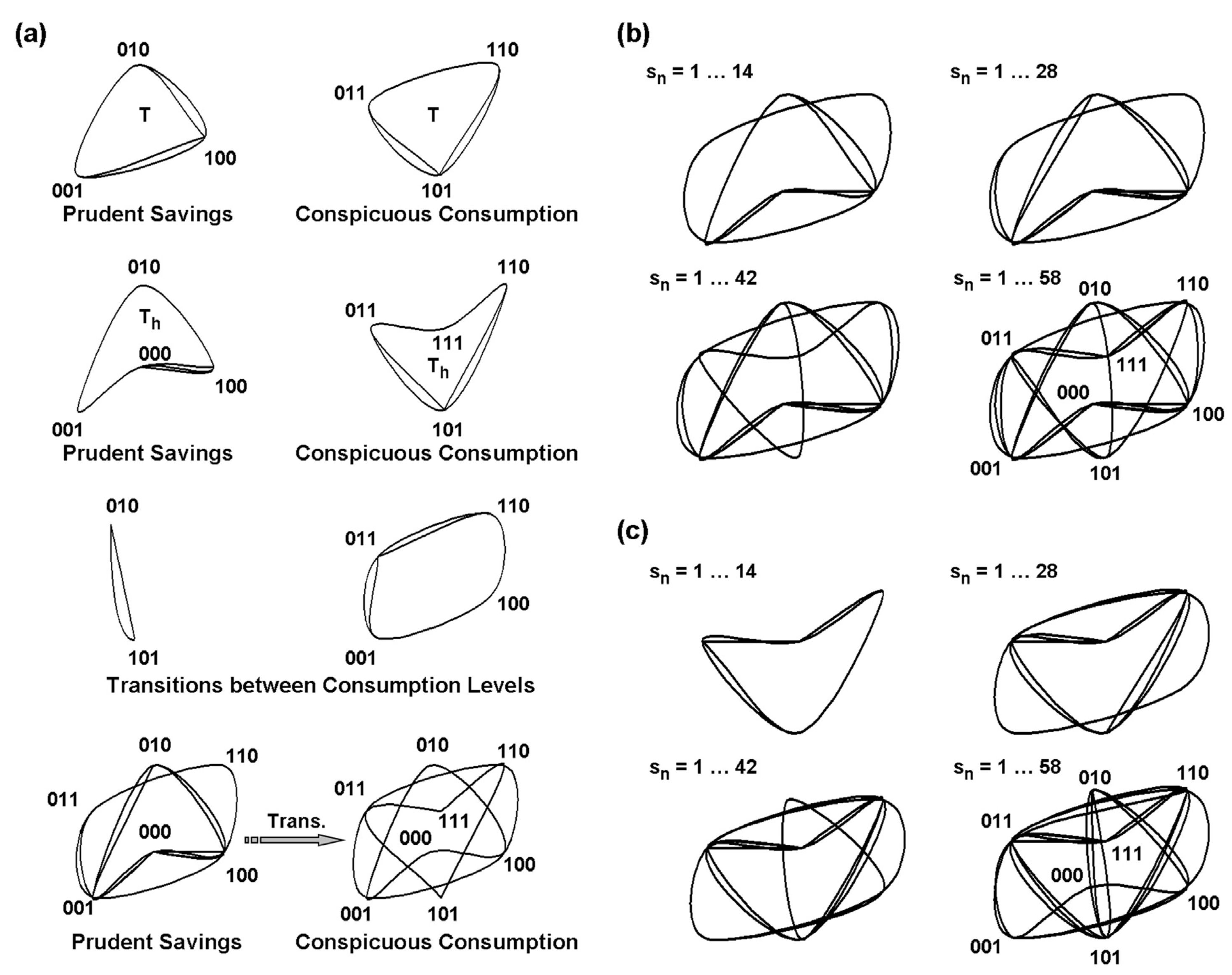

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Clark, K.B. Neural Field Continuum Limits and the Structure–Function Partitioning of Cognitive–Emotional Brain Networks. Biology 2023, 12, 352. https://doi.org/10.3390/biology12030352
Clark KB. Neural Field Continuum Limits and the Structure–Function Partitioning of Cognitive–Emotional Brain Networks. Biology. 2023; 12(3):352. https://doi.org/10.3390/biology12030352
Chicago/Turabian StyleClark, Kevin B. 2023. "Neural Field Continuum Limits and the Structure–Function Partitioning of Cognitive–Emotional Brain Networks" Biology 12, no. 3: 352. https://doi.org/10.3390/biology12030352
APA StyleClark, K. B. (2023). Neural Field Continuum Limits and the Structure–Function Partitioning of Cognitive–Emotional Brain Networks. Biology, 12(3), 352. https://doi.org/10.3390/biology12030352




