Relationship between Performance and Inter-Limb Asymmetries Using Flywheel Resistance Device in Elite Youth Female Basketball Players
Abstract
:Simple Summary
Abstract
1. Introduction
2. Materials and Methods
2.1. Participants
2.2. Design and Procedures
2.2.1. Linear Sprint
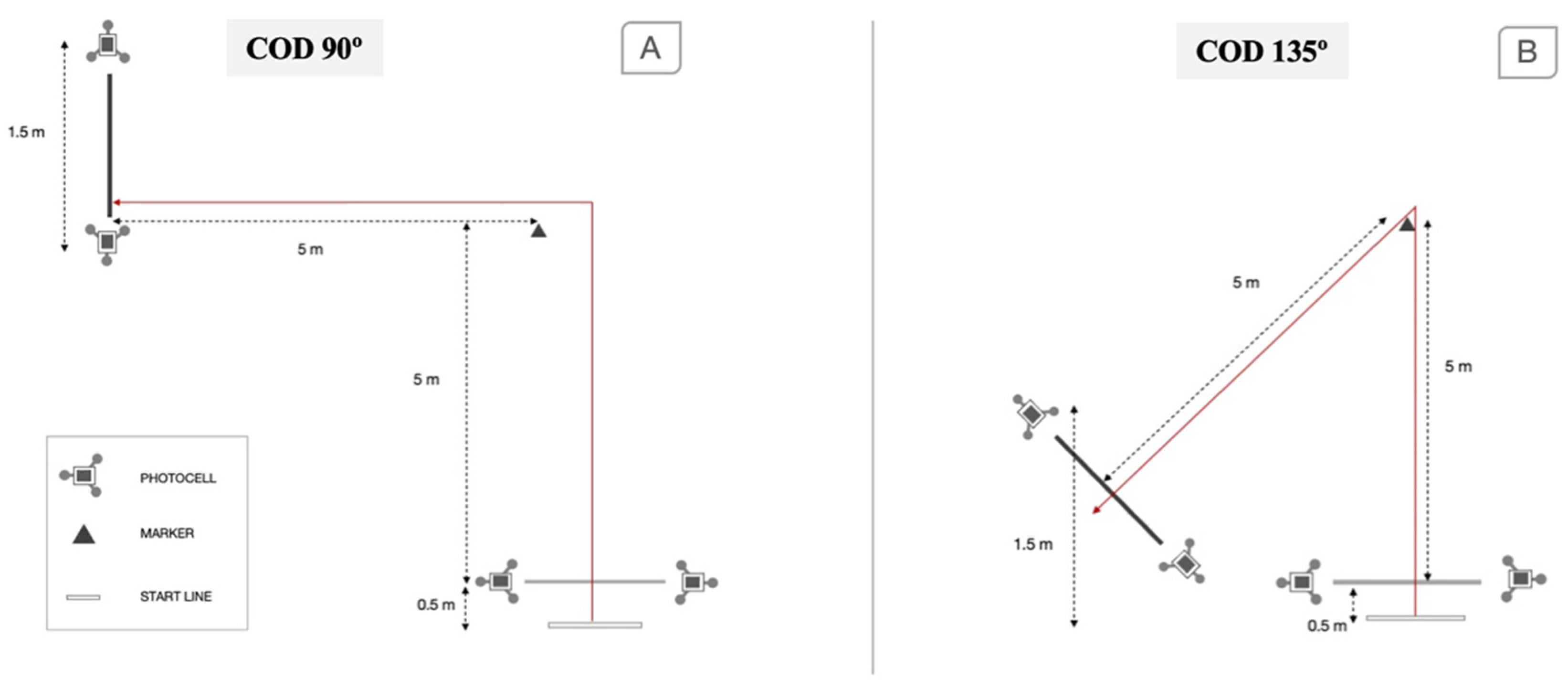
2.2.2. Change in Direction Speed Tests
2.2.3. Single Leg Countermovement Jump Tests
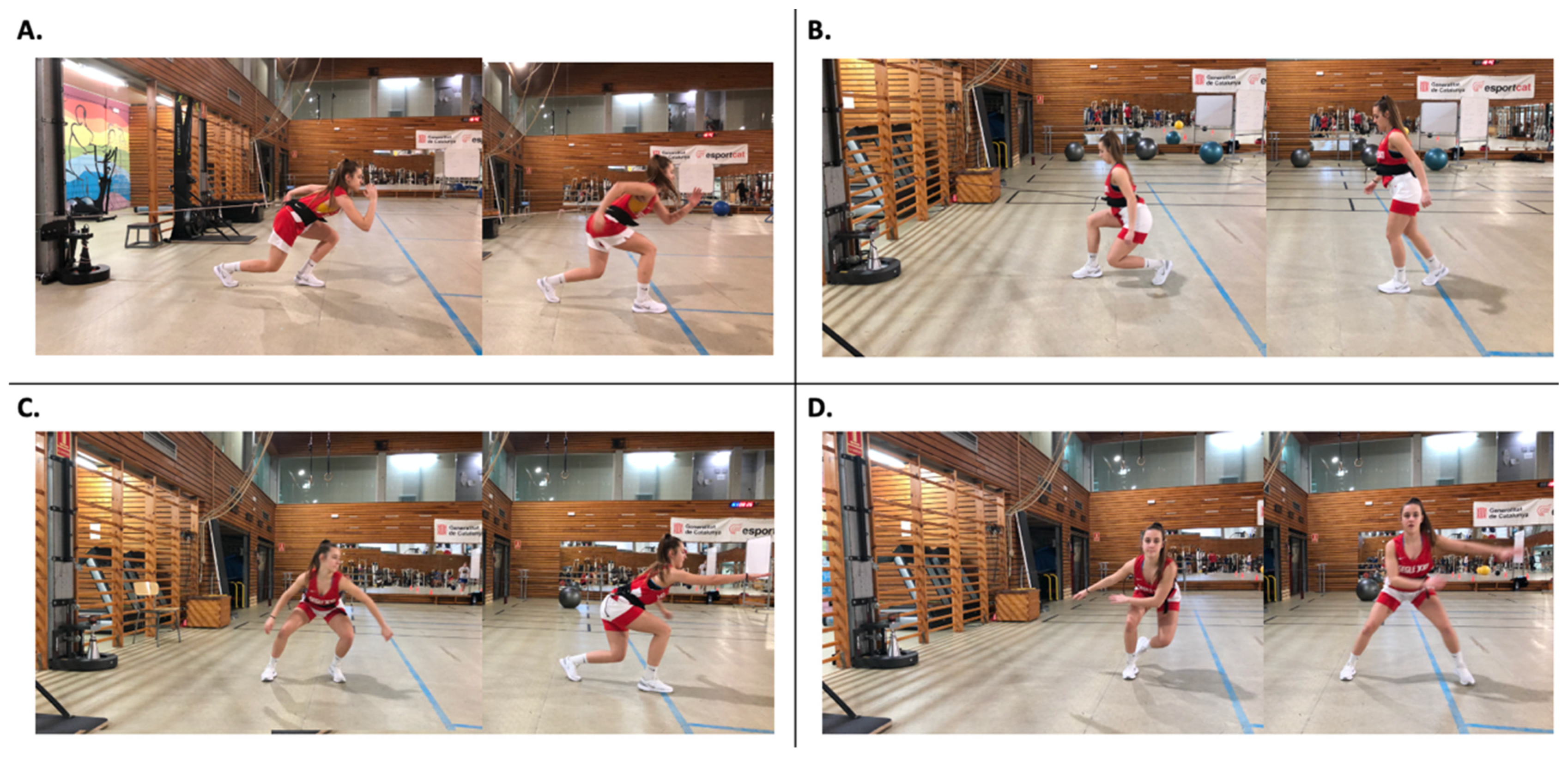
2.2.4. Flywheel Resistance Skills
2.3. Statistical Analysis
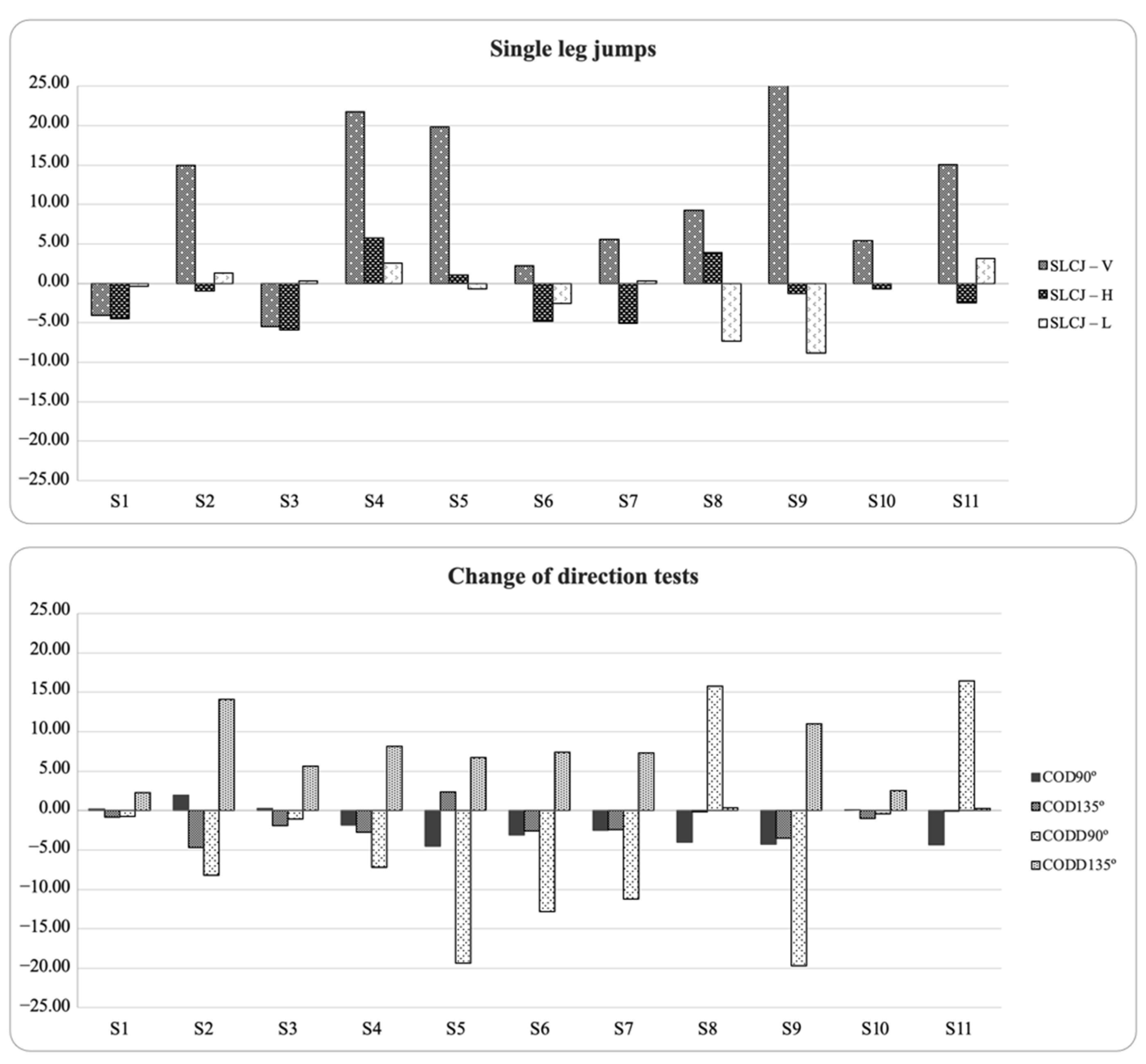
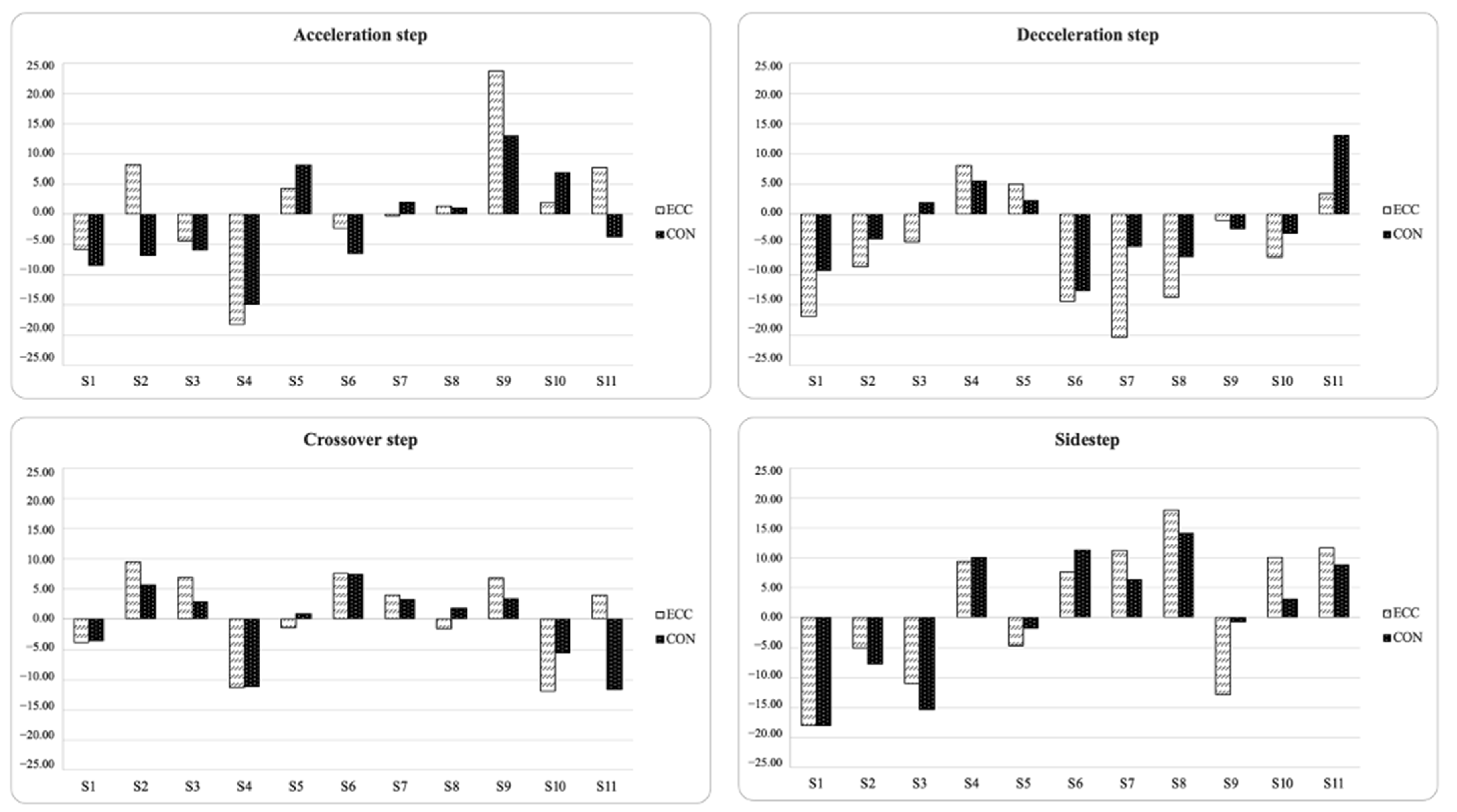
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Castagna, C.; Manzi, V.; D’Ottavio, S.; Annino, G.; Padua, E.; Bishop, D. Relation between Maximal Aerobic Power and the Ability to Repeat Sprints in Young Basketball Players. J. Strength Cond. Res. 2007, 21, 1172. [Google Scholar] [CrossRef] [PubMed]
- Banda, D.S.; Beitzel, M.M.; Kammerer, J.D.; Salazar, I.; Lockie, R.G. Lower-body power relationships to linear speed, change-of-direction speed, and high-intensity running performance in di collegiate women’s basketball players. J. Hum. Kinet. 2019, 68, 223–232. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Loturco, I.; Pereira, L.A.; Freitas, T.T.; Alcaraz, P.E.; Zanetti, V.; Bishop, C.; Jeffreys, I. Maximum acceleration performance of professional soccer players in linear sprints: Is there a direct connection with change-of-direction ability? PLoS ONE 2019, 14, e0216806. [Google Scholar] [CrossRef] [PubMed]
- Bahr, R.; Krosshaug, T. Understanding injury mechanisms: A key component of preventing injuries in sport. Br. J. Sports Med. 2005, 39, 324–329. [Google Scholar] [CrossRef]
- Fort-Vanmeerhaeghe, A.; Mila-Villarroel, R.; Pujol, M.; Arboix-Alió, J.; Bishop, C. Higher vertical jumping asymmetries and lower physical performance are indicators of increased injury incidence in youth team-sport athletes. J. Strength Cond. Res. 2020. ahead of Print. [Google Scholar] [CrossRef]
- Bishop, C.; Turner, A.; Read, P. Effects of inter-limb asymmetries on physical and sports performance: A systematic review. J. Sports Sci. 2018, 36, 1135–1144. [Google Scholar] [CrossRef]
- Bishop, C.; Lake, J.; Loturco, I.; Papadopoulos, K.; Turner, A.; Read, P. Interlimb asymmetries: The need for an individual approach to data analysis. J. Strength Cond. Res. 2021, 35, 695–701. [Google Scholar] [CrossRef]
- Fort-Vanmeerhaeghe, A.; Bishop, C.; Buscà, B.; Vicens-Bordas, J.; Arboix-Alió, J. Seasonal variation of inter-limb jumping asymmetries in youth team-sport athletes. J. Sports Sci. 2021, 39, 2850–2858. [Google Scholar] [CrossRef]
- Hewit, J.; Cronin, J.; Hume, P. Asymmetry in multi-directional jumping tasks. Phys. Ther. Sport 2012, 13, 238–242. [Google Scholar] [CrossRef]
- Fort-Vanmeerhaeghe, A.; Gual, G.; Romero-Rodriguez, D.; Unnitha, V. Lower limb neuromuscular asymmetry in volleyball and basketball players. J. Hum. Kinet. 2016, 50, 135–143. [Google Scholar] [CrossRef]
- Fort-Vanmeerhaeghe, A.; Montalvo, A.M.; Sitjà-Rabert, M.; Kiefer, A.W.; Myer, G.D. Neuromuscular asymmetries in the lower limbs of elite female youth basketball players and the application of the skillful limb model of comparison. Phys. Ther. Sport 2015, 16, 317–323. [Google Scholar] [CrossRef]
- Bishop, C.; Pereira, L.A.; Reis, V.P.; Read, P.; Turner, A.N.; Loturco, I. Comparing the magnitude and direction of asymmetry during the squat, countermovement and drop jump tests in elite youth female soccer players. J. Sports Sci. 2019, 38, 1296–1303. [Google Scholar] [CrossRef]
- Bishop, C.; Read, P.; Chavda, S.; Jarvis, P.; Brazier, J.; Bromley, T.; Turner, A. Magnitude or direction? Seasonal variation of interlimb asymmetry in elite academy soccer players. J. Strength Cond. Res. 2020, 36, 1031–1037. [Google Scholar] [CrossRef]
- Bell, D.R.; Sanfilippo, J.L.; Binkley, N.; Heiderscheit, B.C. Lean mass asymmetry influences force and power asymmetry during jumping in collegiate athletes. J. Strength Cond. Res. 2014, 28, 884–891. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bishop, C.; Read, P.; McCubbine, J.; Turner, A. Vertical and horizontal asymmetries are related to slower sprinting and jump performance in elite youth female soccer players. J. Strength Cond. Res. 2018, 35, 56–63. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Fort-Vanmeerhaeghe, A.; Bishop, C.; Buscà, B.; Aguilera-Castells, J.; Vicens-Bordas, J.; Gonzalo-Skok, O. Inter-limb asymmetries are associated with decrements in physical performance in youth elite team sports athletes. PLoS ONE 2020, 15, e0229440. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Arboix-Alió, J.; Buscà, B.; Busquets, A.; Aguilera-Castells, J.; de Pablo, B.; Montalvo, A.M.; Fort-Vanmeerhaeghe, A. Relationship between Inter-Limb asymmetries and physical performance in rink hockey players. Symmetry 2020, 12, 2035. [Google Scholar] [CrossRef]
- Hoffman, J.R.; Ratamess, N.A.; Klatt, M.; Faigenbaum, A.D.; Kang, J. Do bilateral power deficits influence direction-specific movement patterns? Res. Sports Med. 2007, 15, 125–132. [Google Scholar] [CrossRef]
- Madruga-Parera, M.; Bishop, C.; Fort-Vanmeerhaeghe, A.; Beltran-Valls, M.; Skok, O.; Romero-Rodríguez, D. Interlimb asymmetries in youth tennis players: Relationships with performance. J. Strength Cond. Res. 2019, 34, 2815–2823. [Google Scholar] [CrossRef]
- Madruga-Parera, M.; Bishop, C.; Beato, M.; Fort-Vanmeerhaeghe, A.; Gonzalo-Skok, O.; Romero-Rodríguez, D. Relationship between interlimb asymmetries and speed and change of direction speed in youth handball players. J. Strength Cond. Res. 2019, 35, 3482–3490. [Google Scholar] [CrossRef]
- Raya-González, J.; Bishop, C.; Gómez-Piqueras, P.; Veiga, S.; Viejo-Romero, D.; Navandar, A. Strength, jumping, and change of direction speed asymmetries are not associated with athletic performance in elite academy soccer players. Front. Psychol. 2020, 11, 175. [Google Scholar] [CrossRef] [Green Version]
- Nuñez Sanchez, F.J.; Sáez de Villarreal, E. Does flywheel paradigm training improve muscle volume and force? A meta-analysis. J. Strength Cond. Res. 2017, 31, 3177–3186. [Google Scholar] [CrossRef] [PubMed]
- Raya-González, J.; de Keijzer, K.L.; Bishop, C.; Beato, M. Effects of flywheel training on strength-related variables in female populations. A systematic review. Res. Sports Med. 2021, 11, 1–18. [Google Scholar] [CrossRef] [PubMed]
- Vicens-Bordas, J.; Esteve, E.; Fort-Vanmeerhaeghe, A.; Bandholm, T.; Thorborg, K. Is inertial flywheel resistance training superior to gravity-dependent resistance training in improving muscle strength? A systematic review with meta-analyses. J. Sci. Med. Sport 2018, 21, 75–83. [Google Scholar] [CrossRef]
- Mirwald, R.L.; Baxter-Jones, A.D.G.; Bailey, D.A.; Beunen, G.P. An assessment of maturity from anthropometric measurements. Med. Sci. Sports Exerc. 2002, 34, 689–694. [Google Scholar] [CrossRef] [PubMed]
- Yeado, M.; Kato, T.; Kerwin, D. Measuring running speed using photocells. J. Sports Sci. 1999, 17, 249–257. [Google Scholar] [CrossRef] [PubMed]
- Nimphius, S.; Callaghan, S.J.; Spiteri, T.; Lockie, R.G. Change of Direction Deficit: A more isolated measure of change of direction performance than total 505 time. J. Strength Cond. Res. 2016, 30, 3024–3032. [Google Scholar] [CrossRef]
- Bosco, C.; Luhtanen, P.; Komi, P.V. A simple method for measurement of mechanical power in jumping. Eur. J. Appl. Physiol. Occup. Physiol. 1983, 50, 273–282. [Google Scholar] [CrossRef]
- Koo, T.K.; Li, M.Y. A Guideline of Selecting and Reporting Intraclass Correlation Coefficients for Reliability Research. J. Chiropr. Med. 2016, 15, 155–163. [Google Scholar] [CrossRef] [Green Version]
- Cormack, S.J.; Newton, R.U.; McGuigan, M.R.; Doyle, T.L.A. Reliability of measures obtained during single and repeated countermovement jumps. Int. J. Sports Physiol. Perform. 2008, 3, 131–144. [Google Scholar] [CrossRef] [Green Version]
- Viera, A.; Garrett, J. Understanding interobserver agreement: The kappa statistic. Fam. Med. 2005, 37, 360–363. [Google Scholar]
- Cohen, J. A coefficient of agreement for nominal scales. Educ. Psychol. Meas. 1960, 20, 37–46. [Google Scholar] [CrossRef]
- Impellizzeri, F.M.; Rampinini, E.; Maffiuletti, N.; Marcora, S.M. A vertical jump force test for assessing bilateral strength asymmetry in athletes. Med. Sci. Sports Exerc. 2007, 39, 2044–2050. [Google Scholar] [CrossRef] [Green Version]
- Bishop, C.; Read, P.; Chavda, S.; Turner, A. Asymmetries of the lower limb: The calculation conundrum in strength training and conditioning. Strength Cond. J. 2016, 38, 27–32. [Google Scholar] [CrossRef] [Green Version]
- Cohen, J. Statistical Power Analysis for Behavioural Science; Lawrence Erlbaum: Hillsdale, NJ, USA, 1988. [Google Scholar]
- Hopkins, W.G.; Marshall, S.W.; Batterham, A.M.; Hanin, J. Progressive statistics for studies in sports medicine and exercise science. Med. Sci. Sports Exerc. 2009, 41, 3–13. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Stephens, T.M.; Lawson, B.R.; DeVoe, D.E.; Reiser, R.F. Gender and bilateral differences in single-leg countermovement jump performance with comparison to a double-leg jump. J. Appl. Biomech. 2007, 23, 190–202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vázquez-Guerrero, J.; Suarez-Arrones, L.; Casamichana, D.; Rodas, G. Comparing external total load, acceleration and deceleration outputs in elite basketball players across positions during match play. Kinesiology 2018, 50, 228–234. [Google Scholar] [CrossRef] [Green Version]
- Tous-Fajardo, J.; Maldonado, R.A.; Quintana, J.M.; Pozzo, M.; Tesch, P.A. The flywheel leg-curl machine: Offering eccentric overload for hamstring development. Int. J. Sports Physiol. Perform. 2006, 1, 293–298. [Google Scholar] [CrossRef] [Green Version]
- Bishop, C.; Berney, J.; Lake, J.; Loturco, I.; Blagrove, R.; Turner, A.; Read, P. Bilateral deficit during jumping tasks: Relationship with speed and change of direction speed performance. J. Strength Cond. Res. 2019, 35, 1833–1840. [Google Scholar] [CrossRef]
- Madruga-Parera, M.; Bishop, C.; Read, P.; Lake, J.; Brazier, J.; Romero-Rodriguez, D. Jumping-based asymmetries are negatively associated with jump, change of direction, and repeated sprint performance, but not linear speed, in adolescent handball athletes. J. Hum. Kinet. 2020, 71, 47–58. [Google Scholar] [CrossRef] [Green Version]
- Arboix-Alió, J.; Bishop, C.; Benet, A.; Buscà, B.; Aguilera-Castells, J.; Fort-Vanmeerhaeghe, A. Assessing the magnitude and direction of asymmetry in unilateral jump and change of direction speed tasks in youth female team-sport athletes. J. Hum. Kinet. 2021, 79, 15–27. [Google Scholar] [CrossRef]
- Sayers, M.G.L. Influence of test distance on change of direction speed test results. J. Strength Cond. Res. 2015, 29, 2412–2416. [Google Scholar] [CrossRef] [PubMed]
- Spiteri, T.; Cochrane, J.L.; Hart, N.H.; Haff, G.G.; Nimphius, S. Effect of strength on plant foot kinetics and kinematics during a change of direction task. Eur. J. Sport Sci. 2013, 13, 646–652. [Google Scholar] [CrossRef] [PubMed]
| Test | Mean ± SD | p | ES | Asymmetry (%) | ICC (95% CI) | CV (%) | |
|---|---|---|---|---|---|---|---|
| Acceleration step-CON (w) | HPL | 615.35 ± 138.19 | 0.008 | 0.31 | 6.36 ± 5.27 | 0.98 (0.95–0.99) | 22.46 |
| LPL | 574.85 ± 126.13 | 0.98 (0.96–0.99) | 21.94 | ||||
| Acceleration step-ECC (w) | HPL | 639.07 ± 135.30 | 0.005 | 0.34 | 6.24 ± 5.33 | 0.97 (0.93–0.99) | 21.17 |
| LPL | 596.97 ± 119.75 | 0.98 (0.95–0.99) | 20.06 | ||||
| Crossover step-CON (w) | HPL | 558.60 ± 87.23 | 0.001 | 0.34 | 4.79 ± 2.92 | 0.97 (0.93–0.99) | 15.62 |
| LPL | 530.87 ± 75.96 | 0.98 (0.95–0.99) | 14.31 | ||||
| Crossover step-ECC (w) | HPL | 601.76 ± 80.27 | 0.001 | 0.52 | 6.6 ± 4.00 | 0.96 (0.90–0.99) | 13.34 |
| LPL | 561.58 ± 74.06 | 0.99 (0.97–0.99) | 13.19 | ||||
| Deceleration step-CON (w) | HPL | 462.28 ± 52.30 | 0.002 | 0.46 | 5.95 ± 4.17 | 0.97 (0.92–0.99) | 11.31 |
| LPL | 435.77 ± 60.56 | 0.98 (0.94–0.99) | 13.90 | ||||
| Deceleration step-ECC (w) | HPL | 522.15 ± 61.48 | 0.001 | 0.67 | 8.81 ± 6.61 | 0.94 (0.84–0.98) | 11.77 |
| LPL | 476.99 ± 72.09 | 0.96 (0.89–0.99) | 15.11 | ||||
| Sidestep-CON (w) | HPL | 467.01 ± 79.55 | 0.000 | 0.47 | 8.84 ± 5.63 | 0.98 (0.95–0.99) | 17.03 |
| LPL | 427.42 ± 88.43 | 0.98 (0.95–0.99) | 20.69 | ||||
| Sidestep-ECC (w) | HPL | 508.06 ± 100.57 | 0.000 | 0.59 | 10.86 ± 4.37 | 0.98 (0.95–0.99) | 19.79 |
| LPL | 452.28 ± 87.72 | 0.98 (0.95–0.99) | 19.40 | ||||
| SLCJ-V (m) | HPL | 0.16 ± 0.03 | 0.002 | 0.67 | 11.75 ± 7.79 | 0.97 (0.91–0.99) | 8.37 |
| LPL | 0.14 ± 0.03 | 0.97 (0.90–0.99) | 9.68 | ||||
| SLCJ-H (m) | HPL | 1.58 ± 0.11 | 0.017 | 0.42 | 2.45 ± 2.75 | 0.81 (0.29–0.95) | 6.96 |
| LPL | 1.54 ± 0.08 | 0.86 (0.35–0.96) | 5.19 | ||||
| SLCJ-L (m) | HPL | 1.53 ± 0.09 | 0.011 | 0.44 | 2.61 ± 2.80 | 0.80 (0.31–0.95) | 5.88 |
| LPL | 1.49 ± 0.09 | 0.80 (0.05–0.93) | 6.04 | ||||
| COD 135° (s) | HPL | 3.02 ± 0.15 | 0.001 | 0.27 | 1.26 ± 0.94 | 0.93 (0.87–0.97) | 4.97 |
| LPL | 3.06 ± 0.15 | 0.96 (0.85–0.99) | 4.90 | ||||
| COD 90° (s) | HPL | 2.60 ± 0.11 | 0.006 | 0.40 | 1.60 ± 1.50 | 0.92 (0.72–0.98) | 4.23 |
| LPL | 2.65 ± 0.14 | 0.95 (0.89–0.99) | 5.28 | ||||
| COD deficit 135° (s) | HPL | 1.06 ± 0.10 | 0.001 | 0.40 | 6.53 ± 6.01 | 0.97 (0.95–0.98) | 9.43 |
| LPL | 1.10 ± 0.10 | 0.97 (0.94–0.99) | 9.09 | ||||
| COD deficit 90° (s) | HPL | 0.64 ± 0.06 | 0.006 | 0.71 | 3.62 ± 2.74 | 0.95 (0.91–0.97) | 9.38 |
| LPL | 0.69 ± 0.08 | 0.96 (0.92–0.98) | 11.59 | ||||
| 10 m sprint (s) | 1.96 ± 0.08 | 4.08 | |||||
| % Asymmetry | SLCJ-V | SLCJ-H | SLCJ-L | COD 135 | COD 90 | COD Deficit 135 | COD Deficit 90 | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | |
| Acceleration step-CON | 0.12 | −0.33 | −0.41 | −0.37 | −0.09 | −0.17 | 0.46 | 0.47 | 0.52 | 0.38 | −0.06 | −0.03 | −0.17 | 0.00 |
| Acceleration step-ECC | −0.36 | −0.43 | −0.17 | −0.16 | 0.20 | 0.00 | 0.30 | 0.30 | 0.48 | 0.26 | 0.15 | 0.04 | 0.32 | 0.24 |
| Crossover step-CON | 0.11 | 0.07 | 0.20 | 0.48 | 0.11 | 0.38 | −0.12 | −0.13 | 0.01 | −0.04 | 0.17 | 0.19 | 0.36 | 0.26 |
| Crossover step-ECC | 0.22 | 0.17 | 0.04 | 0.06 | −0.13 | 0.02 | −0.44 | −0.49 | −0.42 | −0.53 | −0.44 | −0.58 | −0.45 | −0.36 |
| Decceleration step-CON | 0.06 | −0.07 | 0.20 | −0.23 | 0.42 | 0.27 | 0.29 | 0.36 | 0.29 | 0.32 | 0.25 | 0.27 | 0.14 | 0.29 |
| Decceleration step-ECC | −0.33 | −0.25 | 0.09 | −0.73 | 0.30 | 0.04 | −0.12 | −0.12 | −0.27 | −0.10 | −0.07 | −0.12 | −0.24 | −0.24 |
| Sidestep-CON | −0.34 | −0.12 | −0.52 | −0.32 | −0.61 * | −0.60 | 0.45 | 0.43 | 0.45 | 0.45 | 0.78 ** | 0.72 * | 0.81 ** | 0.74 ** |
| Sidestep-ECC | −0.37 | −0.44 | −0.10 | 0.14 | 0.11 | −0.26 | 0.11 | 0.15 | 0.06 | 0.26 | 0.17 | 0.23 | 0.16 | 0.29 |
| % Asymmetry | Acceleration Step-CON | Acceleration Step-ECC | Crossover Step-CON | Crossover Step-ECC | Decceleration Step-CON | Decceleration Step-ECC | Sidestep-CON | Sidestep-ECC | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | HPL | LPL | |
| SLCJ-V | 0.22 | 0.16 | 0.22 | 0.18 | 0.49 | 0.33 | 0.28 | 0.26 | 0.35 | 0.33 | 0.20 | 0.38 | 0.50 | 0.56 | 0.44 | 0.55 |
| SLCJ-H | 0.19 | 0.16 | 0.15 | 0.16 | 0.04 | 0.26 | 0.02 | 0.00 | 0.26 | 0.18 | 0.22 | 0.17 | −0.25 | −0.26 | −0.11 | −0.16 |
| SLCJ-L | −0.26 | −0.15 | −0.29 | −0.47 | −0.18 | −0.18 | −0.19 | −0.07 | −0.40 | −0.52 | 0.51 | −0.62 | 0.15 | 0.07 | 0.28 | 0.14 |
| COD 135° | −0.36 | −0.34 | −0.29 | −0.02 | −0.23 | −0.30 | −0.36 | −0.11 | −0.30 | −0.18 | 0.24 | 0.07 | −0.02 | 0.00 | −0.02 | 0.09 |
| COD 90° | 0.02 | 0.10 | −0.02 | −0.16 | 0.19 | 0.03 | 0.06 | 0.19 | −0.17 | −0.26 | −0.37 | −0.37 | 0.29 | 0.24 | 0.25 | 0.19 |
| COD deficit 135° | −0.31 | −0.30 | −0.23 | 0.06 | −0.19 | −0.26 | −0.31 | −0.06 | −0.26 | −0.11 | 0.31 | 0.16 | 0.01 | 0.28 | −0.01 | 0.13 |
| COD deficit 90° | −0.02 | 0.05 | −0.06 | −0.17 | 0.18 | 0.01 | 0.05 | 0.16 | −0.18 | −0.26 | −0.36 | −0.35 | 0.26 | 0.22 | 0.19 | 0.17 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fort-Vanmeerhaeghe, A.; Benet-Vigo, A.; Montalvo, A.; Arboix, A.; Buscà, B.; Arboix-Alió, J. Relationship between Performance and Inter-Limb Asymmetries Using Flywheel Resistance Device in Elite Youth Female Basketball Players. Biology 2022, 11, 812. https://doi.org/10.3390/biology11060812
Fort-Vanmeerhaeghe A, Benet-Vigo A, Montalvo A, Arboix A, Buscà B, Arboix-Alió J. Relationship between Performance and Inter-Limb Asymmetries Using Flywheel Resistance Device in Elite Youth Female Basketball Players. Biology. 2022; 11(6):812. https://doi.org/10.3390/biology11060812
Chicago/Turabian StyleFort-Vanmeerhaeghe, Azahara, Ariadna Benet-Vigo, Alicia Montalvo, Adrià Arboix, Bernat Buscà, and Jordi Arboix-Alió. 2022. "Relationship between Performance and Inter-Limb Asymmetries Using Flywheel Resistance Device in Elite Youth Female Basketball Players" Biology 11, no. 6: 812. https://doi.org/10.3390/biology11060812
APA StyleFort-Vanmeerhaeghe, A., Benet-Vigo, A., Montalvo, A., Arboix, A., Buscà, B., & Arboix-Alió, J. (2022). Relationship between Performance and Inter-Limb Asymmetries Using Flywheel Resistance Device in Elite Youth Female Basketball Players. Biology, 11(6), 812. https://doi.org/10.3390/biology11060812









