Investigation of Flow Behavior and Porous Medium Resistance Coefficients for Metallic-Cloth Fibers
Abstract
1. Introduction
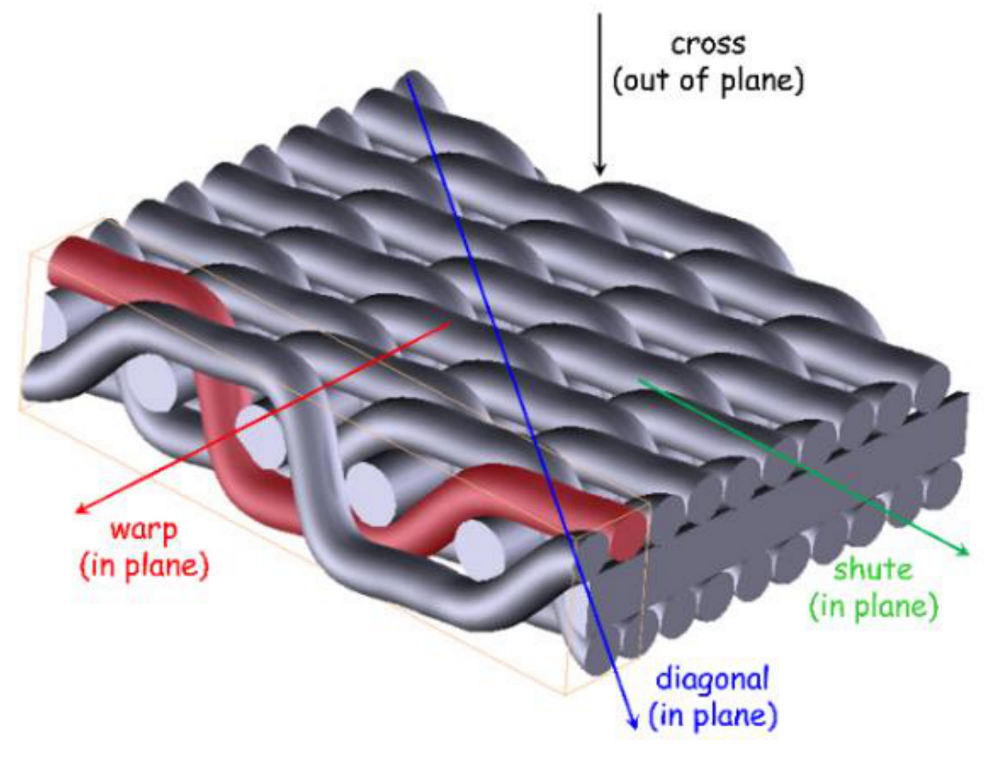
- Warp (in-plane direction);
- Shute (in-plane direction);
- Diagonal (in-plane direction);
- Cross (out-of-plane direction).
2. Materials and Methods
2.1. Materials
2.2. Methods
2.2.1. Cloth Weave Geometry and Issues
- Plain weave;
- Twill weave;
- Dutch weave;
- Plain Dutch weave;
- Reverse Dutch weave;
- Dutch twill weave;
- Dutch twill double weave;
- Stranded weave.
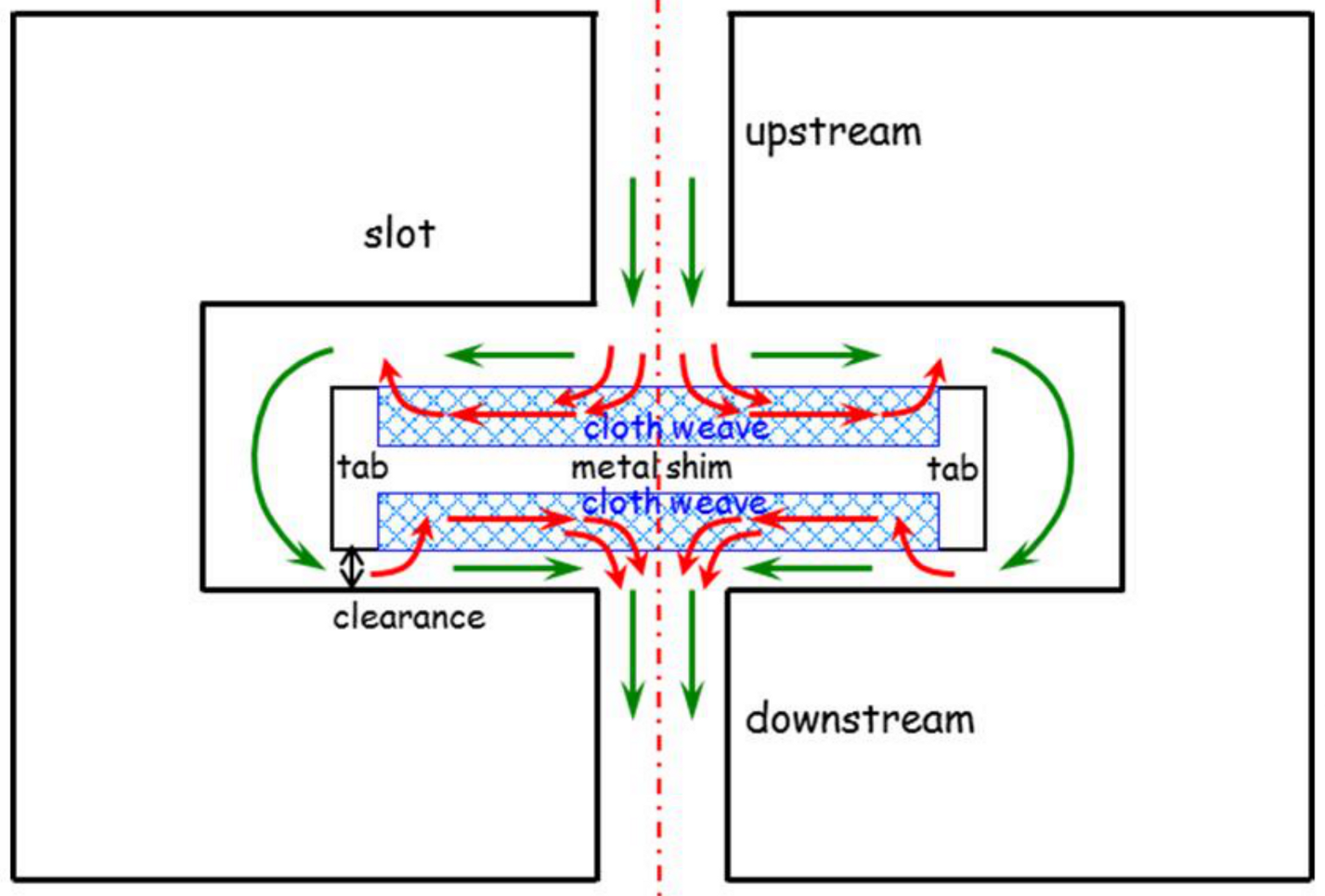
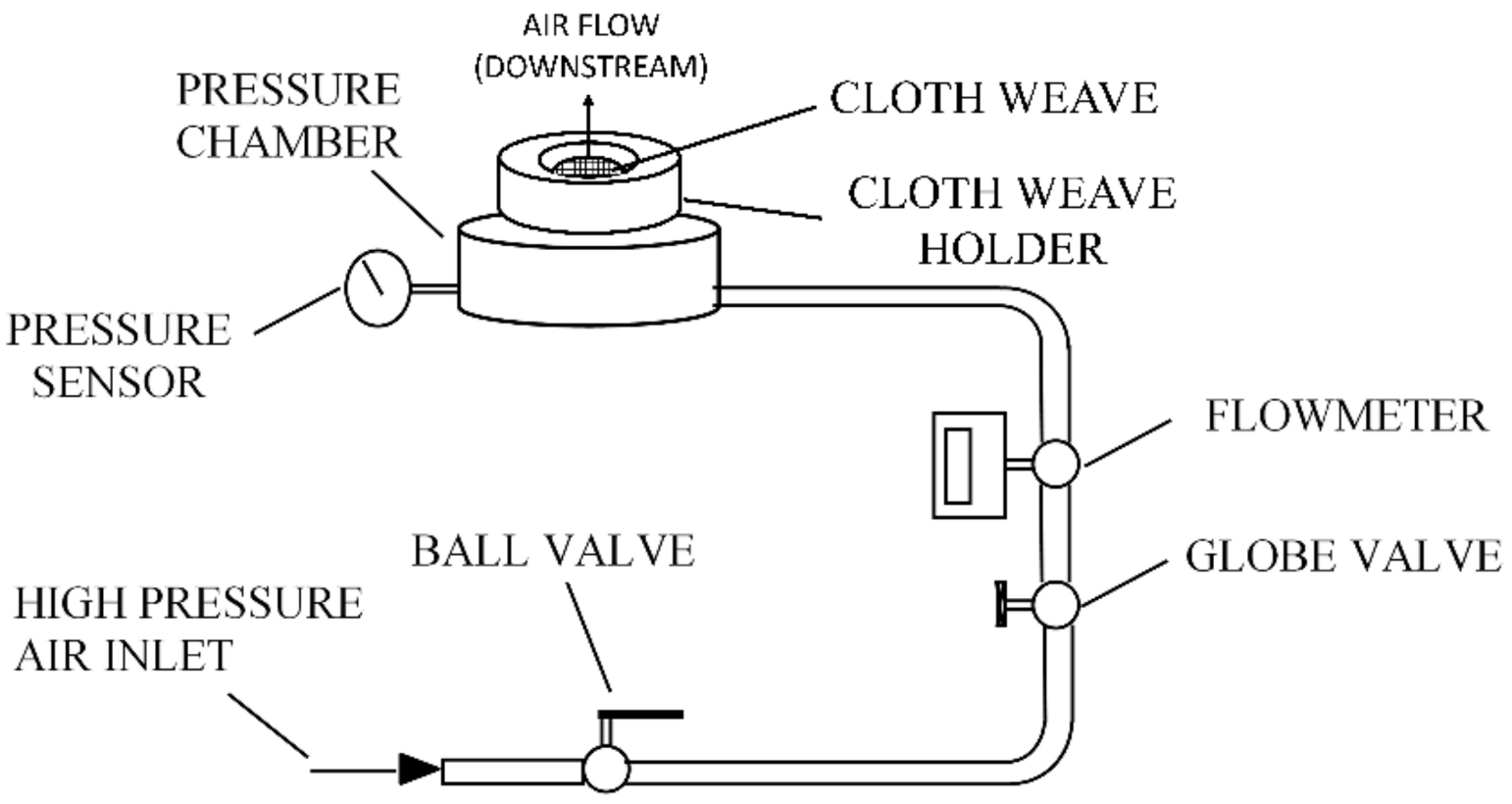
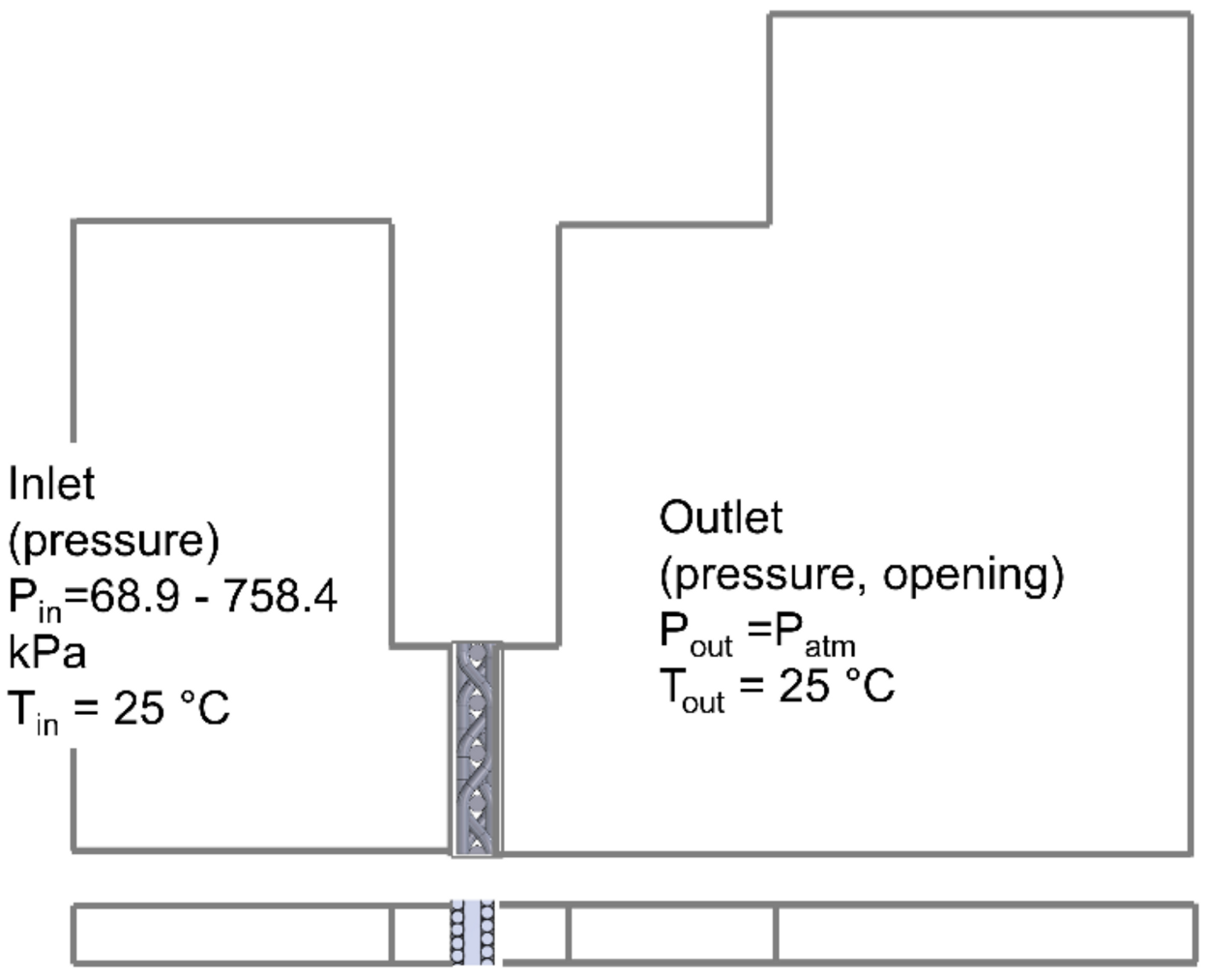
2.2.2. Experimental Setup
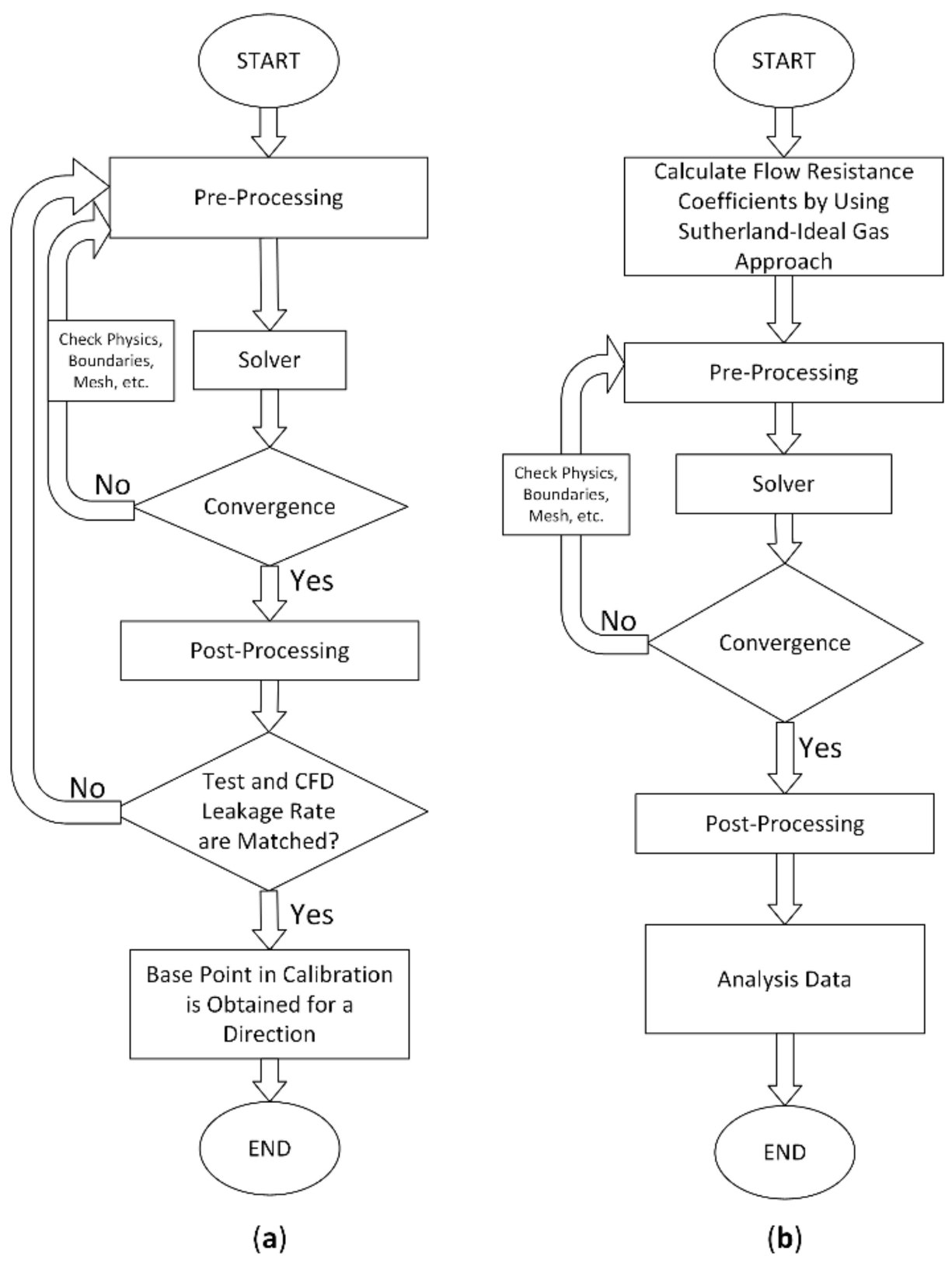
2.2.3. The Sutherland-Ideal Gas Approach for Porous Metallic-Cloth Fibers
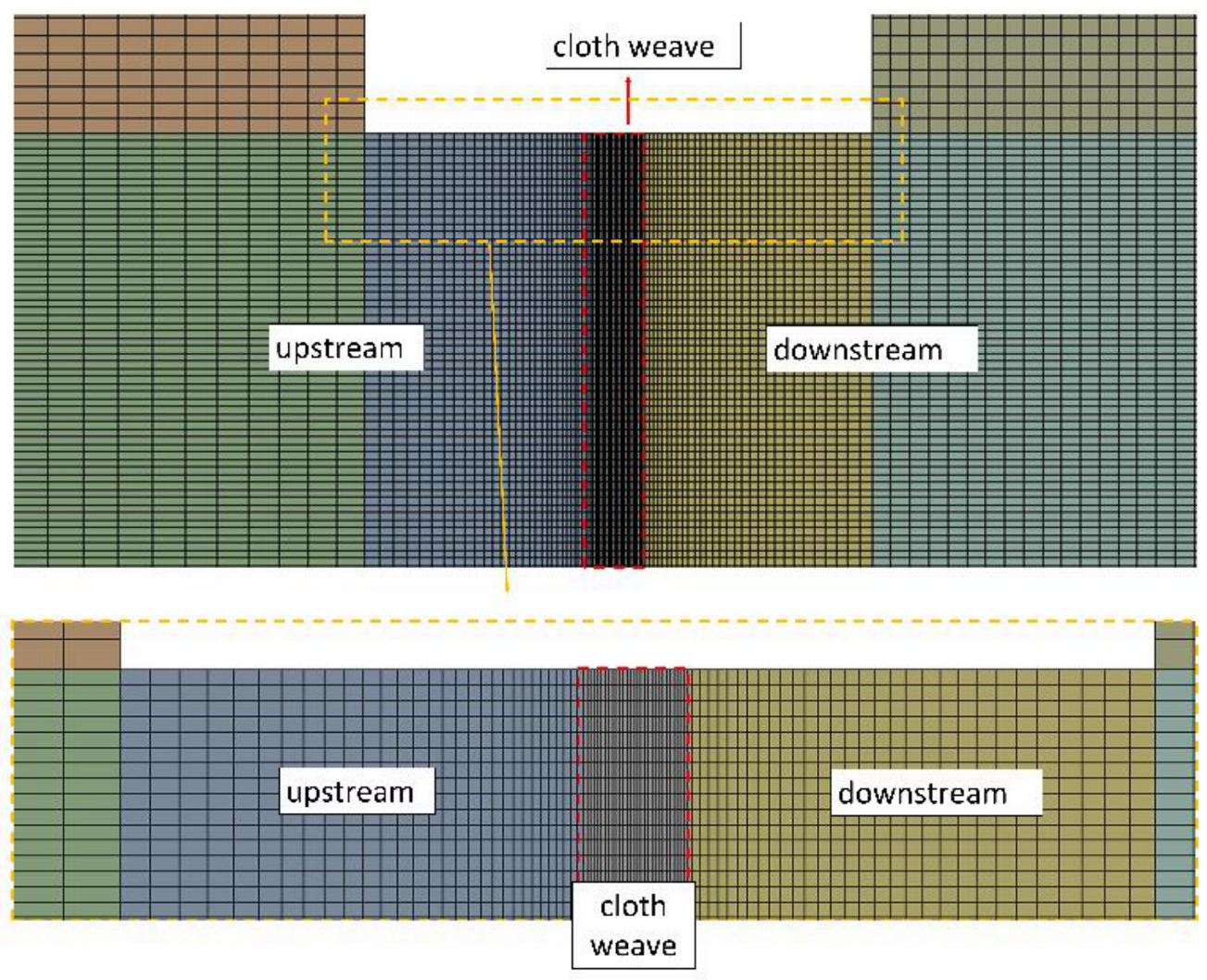
2.2.4. CFD Model
- For in-plane CFD analysis (warp, shute, and diagonal), the CFD model was built in a 3-D cartesian coordinate system (Figure 7). Three cell thicknesses were considered in the third direction. The symmetry boundary conditions were applied on two surfaces to reduce the computational domain and time.
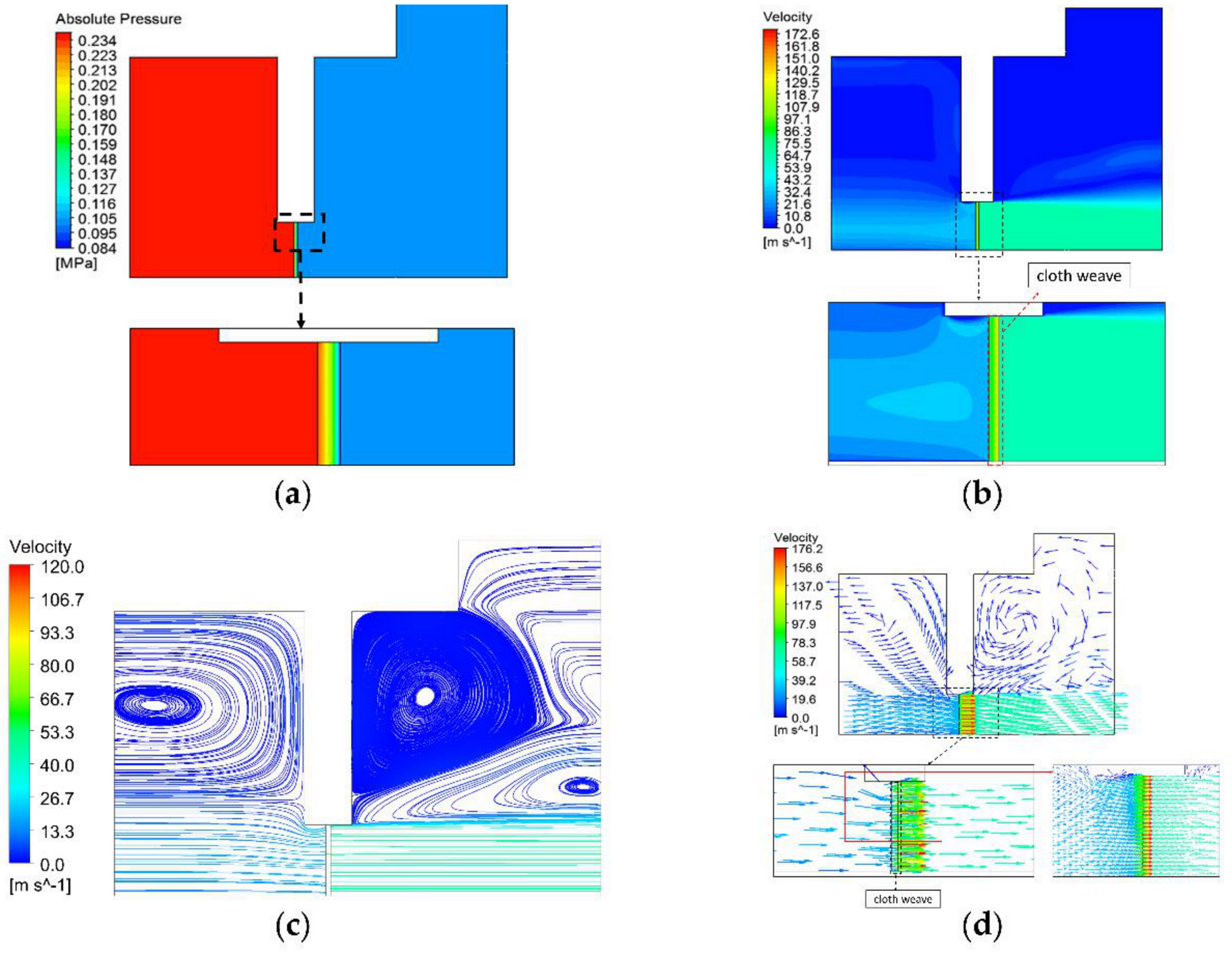
- For the out-of-plane direction of cross flow, a section model with 1° in the 3-D cylindrical coordinate system was used since a circular piece of metallic-cloth fiber was tested in the sample holders (Figure 8).
- The working fluid was air.
- The metallic-cloth fibers were modeled as a porous medium, for which the flow resistance coefficients were prescribed by using the Sutherland-ideal gas approach. The weight and volume of the experimental sample were measured. Then, the porosity was calculated as 0.364 by using the weight, density, and volume of the experimental sample. It was defined to the metallic-cloth fiber domain in CFD analyses.
- Steady-state CFD analyses were performed.
- The flow was compressible and turbulent.
- Static pressure and static temperature values were defined on the inlet boundary. The opening pressure and temperature boundary conditions were utilized for the outlet boundary.
- The smooth wall roughness was defined for static walls. For the smooth wall roughness, the dimensionless sand-grain roughness was between 0 and 5. Adiabatic and no-slip wall conditions were used for the walls. The maximum y+ value reached 5.
- In the porous weave domain, the pressure variation in transverse directions was zero. Therefore, the resistance coefficients were assumed to be isotropic in a CFD analysis for each direction of warp, shute, and diagonal.
- The momentum, mass, and turbulence kinetic energy residuals were set to 10−5 for convergence. Most of the converged CFD cases provided residuals less than 10−8. In some high pressure-ratio CFD cases (Pd/Pu < 0.1), the residuals were between 10−5 and 10−6. For all the CFD cases, several convergence criteria for the velocity, temperature, pressure, turbulence quantities, and inlet and outlet mass flow rates were met.
2.2.5. Equivalent Gap Calculations
3. Results and Discussion
3.1. Leakage Tests
3.2. CFD Analyses and Calibration of Metallic-Cloth Fiber Flow Resistance Coefficients
| • Warp | 0.9; |
| • Shute | 0.9; |
| • Diagonal | 0.7; |
| • Cross | 0.0. |
4. Conclusions
- Leakage Tests
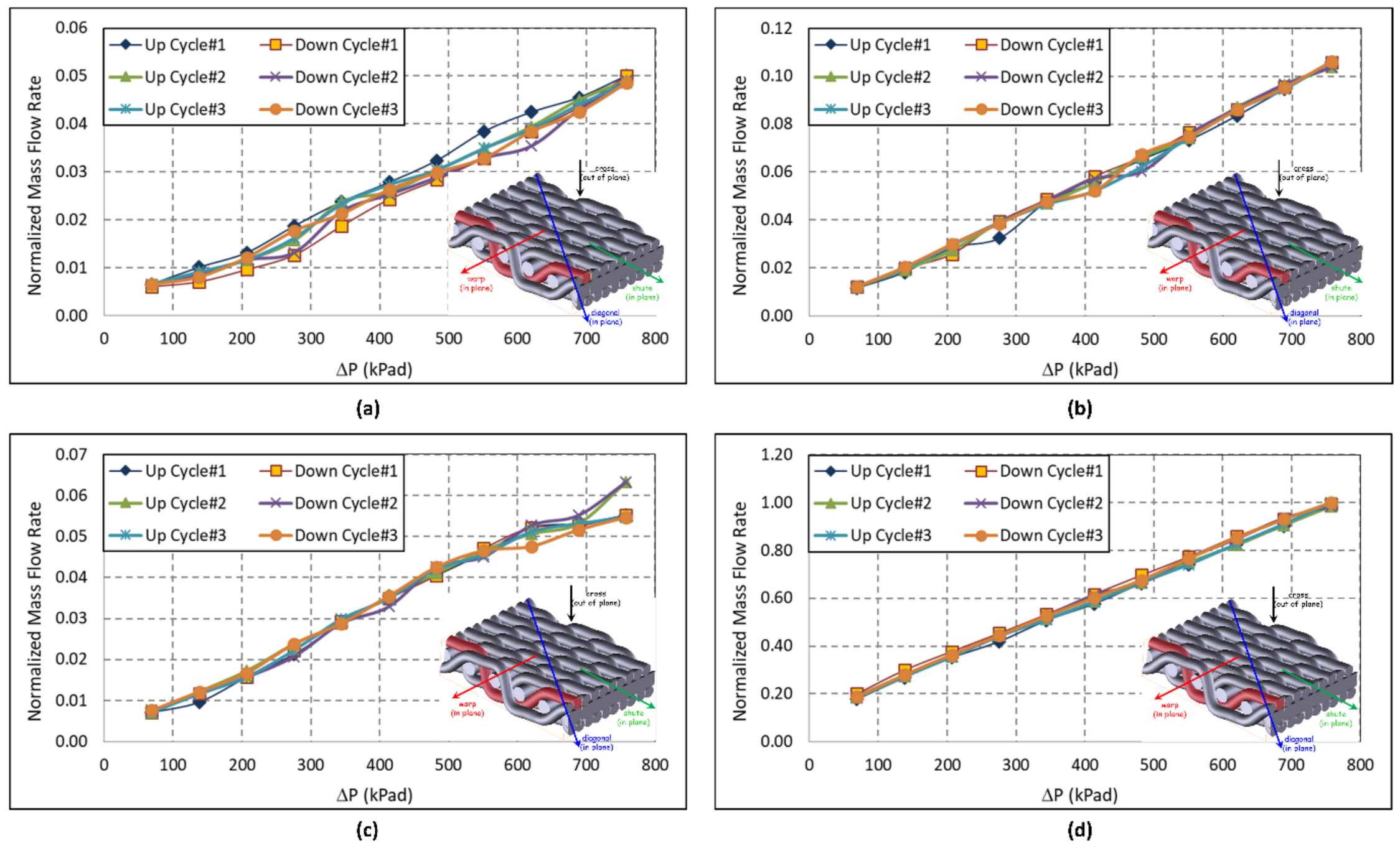
- The leakage tests show that the metallic-cloth fiber leakage is a linear function of pressure load for all the directions.
- The best–worst order for leakage performance in terms of the normalized equivalent gap was the warp, diagonal, shute, and cross directions.
- The cross direction had the highest normalized equivalent gap; therefore, it was the worst direction in terms of leakage performance. The normalized equivalent gap for the cross direction was 2–4 times higher than that for the in-plane directions (warp, shute, and diagonal).
- The leakage rate was at a minimum if the flow was in the warp direction.
- Metallic-Cloth Fiber Flow Resistance Coefficients
- For the warp and shute directions, the best match between the test and CFD leakages was obtained for Cdown = 0.9, at which the leakage difference between the test and CFD was less than 10% for all pressure loads of ΔP = 137.9–689.5 kPad (20–100 psid).
- For the diagonal direction, Cdown = 0.7 provided the minimum leakage difference of 10%.
- For the cross direction, Cdown = 0.0 provided the best leakage agreement, with the tests yielding a 0.03% difference.
- Flow Behavior
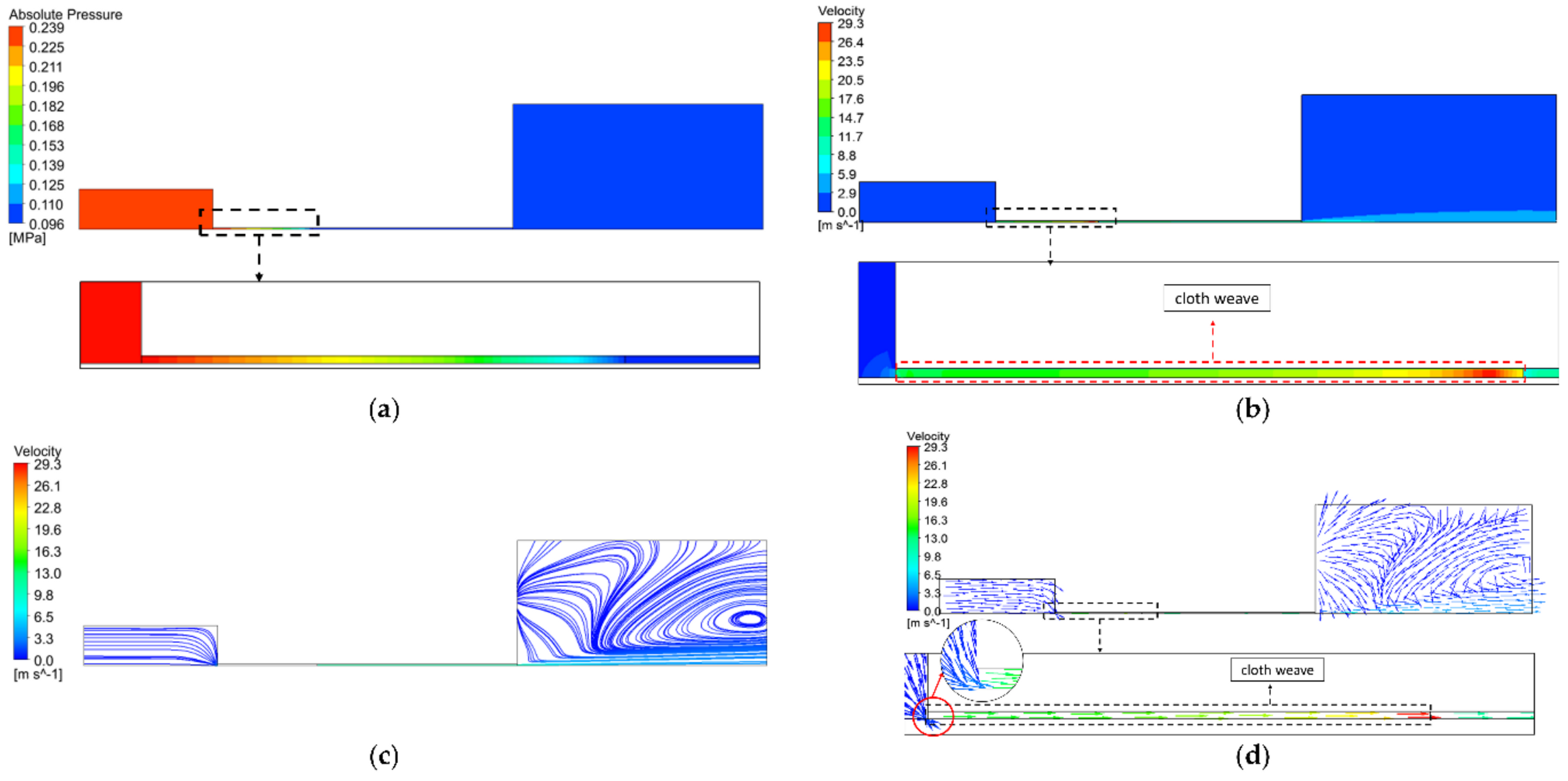
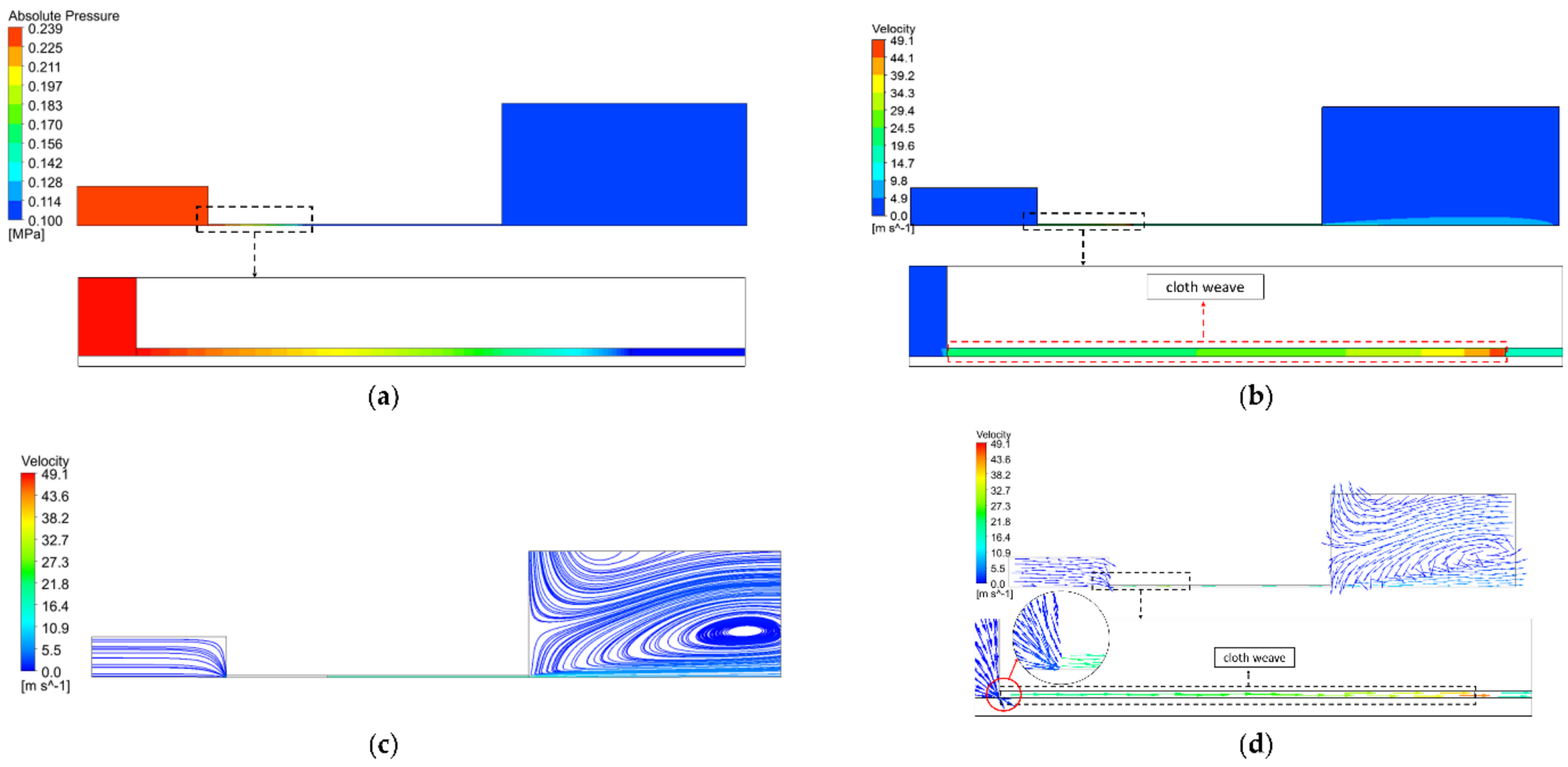
- Most of the pressure drop occurred at the downstream side.
- The flow property plots show that the flow was smoothly directed through the region of metallic-cloth fibers from the upstream side for the in-plane directions.
- The flow velocity reached its maximum value at the downstream side due to expansion.
- Future Work
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
- Uncertainty Analysis
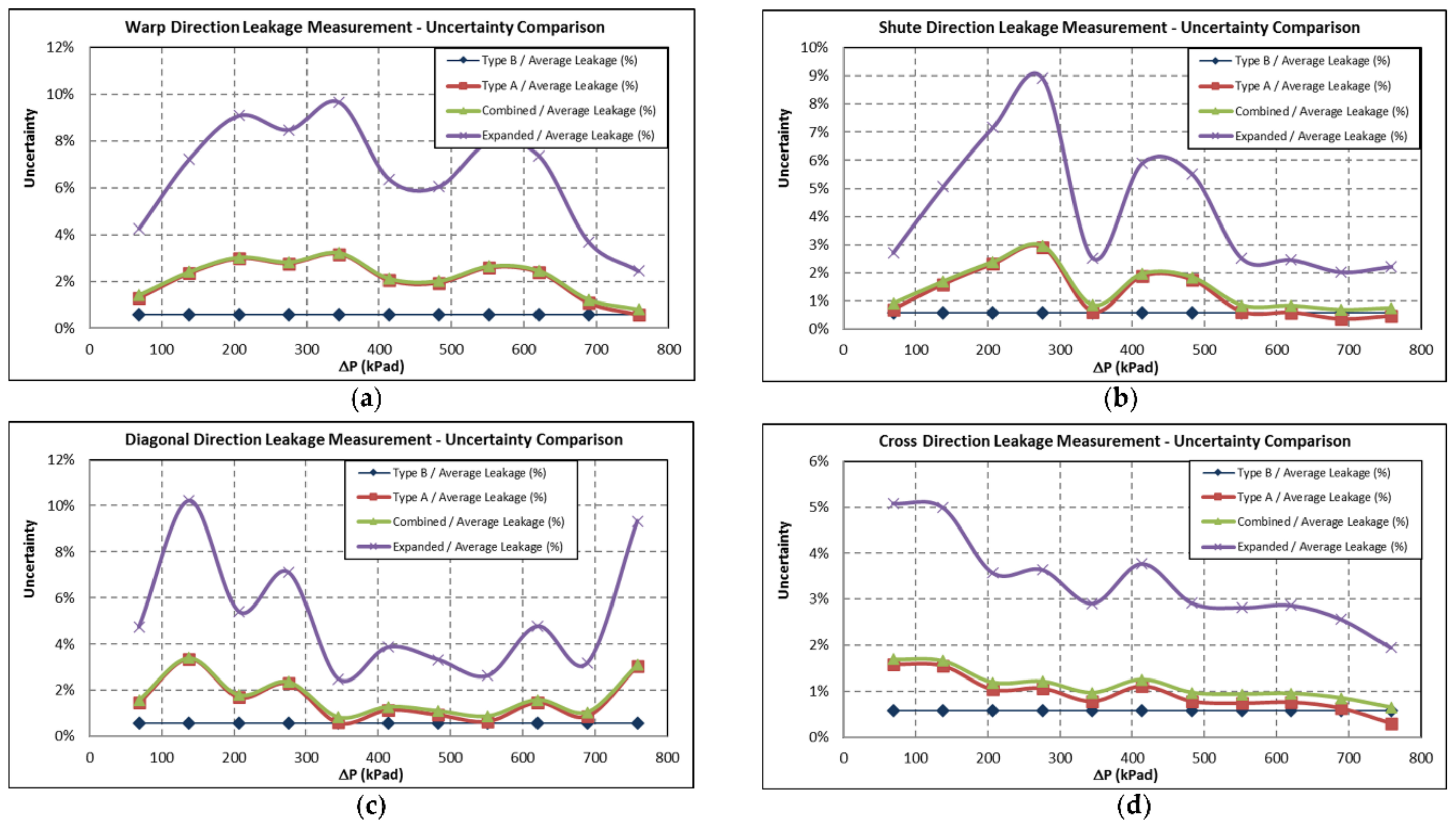
References
- Dinc, O.S.; Bagepalli, B.S.; Wolfe, C.; Aksit, M.F.; Calabrese, S. A new metal cloth stationary seal for gas turbine applications. In Proceedings of the 33rd AIAA/ASME/SAE/ASEE Joint Propulsion Conference, Seattle, WA, USA, 6–9 July 1997. AIAA Paper 97-2732. [Google Scholar] [CrossRef]
- Aksit, M.F.; Bagepalli, B.S.; Demiroglu, M.; Dinc, O.S.; Kellock, I.; Farell, T. Advanced flexible seals for gas turbine shroud applications. In Proceedings of the 35th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Los Angeles, CA, USA, 20–24 June1999. [Google Scholar] [CrossRef]
- Aksit, M.F.; Bagepalli, B.S.; Burns, J.; Stevens, P.; Vehr, J. Parasitic Corner Leakage Reduction in Gas Turbine Nozzle-Shroud Inter-Segment Locations. In Proceedings of the 37th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Salt Lake City, UT, USA, 8–11 July 2001. [Google Scholar] [CrossRef]
- Ongun, R.; Aksit, M.F.; Goktug, G. A simple model for wear of metal cloth seals. In Proceedings of the 40th AIAA/SAE/ASME/ASEE Joint Propulsion Conference & Exhibit, AIAA-2004-3892, Fort Lauderdale, FL, USA, 11–14 July 2004. [Google Scholar] [CrossRef]
- Dogu, Y. Investigation of Brush Seal Flow Characteristics Using Bulk Porous Medium Approach. J. Eng. Gas. Turbines Power 2005, 127, 136–144. [Google Scholar] [CrossRef]
- Amaki, K.; Hasegawa, T.; Narumi, T. Drag Reduction in the Flow of Aqueous Solutions of Detergent Through Mesh Screens. Nihon Reoroji Gakkaishi 2008, 36, 125–131. [Google Scholar] [CrossRef]
- Kolodziej, A.; Lojewska, J. Experimental and Modelling Study on Flow Resistance of Wire Gauzes. Chem. Eng. Process 2009, 48, 816–822. [Google Scholar] [CrossRef]
- Yoshida, Y.; Inoue, Y.; Shimosaka, A.; Shirakawa, Y.; Hidaka, J. Effect of Aperture Structure of Dutch Weave Mesh on Flow Resistivity. J. Chem. Eng. Jpn. 2015, 48, 730–741. [Google Scholar] [CrossRef]
- Chupp, R.E.; Hendricks, R.C.; Lattime, S.B.; Steinetz, B.M.; Aksit, M.F. Turbomachinery Clearance Control. Turbine Aerodynamics, Heat Transfer, Materials, and Mechanics; AIAA: Reston, VA, USA, 2007; pp. 61–188. [Google Scholar] [CrossRef]
- Bagepalli, B.S.; Aksit, M.F.; Farell, T.R. Gas-path Leakage Seal for a Turbine. US Patent No. US5934687, 10 August 1999. [Google Scholar]
- Paprotna, H.E.; Morrision, M.J. Biased Wear Resistant Turbine Seal Assembly. US Patent No. US6733234B2, 11 May 2004. [Google Scholar]
- McMahan, K.W.; Demiroglu, M.; Repikov, T.R. Spring Loaded Seal Assembly for Turbines. US Patent No. US8398090B2, 19 March 2013. [Google Scholar]
- Samudrala, O.; Sarawate, N.N. Cloth Seal for Turbo-Machinery. US Patent No. US8613451B2, 24 December 2013. [Google Scholar]
- Riggi, V.T.; Monshower, B.; Hyslop, J.D. Brazed Turbine Seal. US Patent No. US8696309B2, 15 April 2014. [Google Scholar]
- Sarawate, N.N.; Morgan, V.J.; Weber, D.W. Layered Seal for Turbomachinery. US Patent No. US9188228B2, 17 November 2015. [Google Scholar]
- Chupp, R.E.; Hendricks, R.C.; Lattime, S.B.; Steinetz, B.M. Sealing in Turbomachinery. In Proceedings of the NASA/TM-2006-214341; NASA: Cleveland, OH, USA, 2006. [Google Scholar] [CrossRef]
- Aksit, M.F.; Bagepalli, B.S.; Aslam, S. High performance combustor cloth seals. In Proceedings of the 36th AIAA/ASME/SAE/ASEE Joint Cleveland, Ohio Propulsion Conference & Exhibit, Huntsville, AL, USA, 16–19 July 2000. [Google Scholar]
- Gorgun, E.; Aksit, M.F.; Dogu, Y. A Study of Cloth Seal Leakage Performance Based on Geometry and Pressure Load. Energies 2020, 13, 5884. [Google Scholar] [CrossRef]
- Archard, J.F. Contact and rubbing of flat surfaces. J. Appl. Phys. 1953, 24, 981. [Google Scholar] [CrossRef]
- Archard, J.F.; Hirst, W. The wear of metals under unlubricated conditions. Proc. R. Soc. 1956, 236, 397–410. [Google Scholar]
- May, D.; Aktas, A.; Advani, S.G.; Berg, D.C.; Endruweit, A.; Fauster, E.; Lomov, S.V.; Long, A.; Mitschang, P.; Abaimov, S.; et al. In-Plane Permeability Characterization of Engineering Textiles Based on Radial Flow Experiments: A Benchmark Exercise. Compos. Part A Appl. Sci. Manuf. 2019, 121, 100–114. [Google Scholar] [CrossRef]
- Fauster, E.; Berg, D.C.; May, D.; Blobl, Y.; Schledjewski, R. Robust evaluation of flow front data for in-plane permeability characterization by radial flow experiments. Adv. Manuf. Polym. Compos. Sci. 2018, 4, 24–40. [Google Scholar] [CrossRef]
- Liu, X.; Ding, X.; Chen, C.; An, R.; Guo, W.; Zhang, W.; Nan, H.; Yi Wang, Y. Investigating the filtration behavior of metal fiber felt using CFD-DEM simulation. Eng. Appl. Comput. Fluid Mech. 2019, 13, 426–437. [Google Scholar] [CrossRef]
- Yeo, A.P.S.; Law, A.W.K.; Fane, A.G. Factors affecting the performance of a submerged hollow fiber bundle. J. Membr. Sci. 2006, 280, 969–982. [Google Scholar] [CrossRef]
- Ma, C.; Liu, Y.; Li, F.; Shen, C.; Huang, M.; Wang, Z.; Cao, C.; Zhou, Q.; Sheng, Y.; Sand, W. CFD simulations of fiber-fiber interaction in a hollow fiber membrane bundle: Fiber distance and position matters. Sep. Purif. Technol. 2019, 209, 707–713. [Google Scholar] [CrossRef]
- Dogu, Y.; Bahar, A.S.; Sertcakan, M.C.; Piskin, A.; Arican, E.; Kocagul, M. Computational fluid dynamics investigation of brush seal leakage performance depending on geometric dimensions and operating conditions. J. Eng. Gas. Turbines Power 2016, 138, 032506. [Google Scholar] [CrossRef]
- Li, J.; Qiu, B.; Feng, Z. Experimental and numerical investigations on the leakage flow characteristics of the labyrinth brush seal. J. Eng. Gas Turbines Power 2012, 134, 102509. [Google Scholar] [CrossRef]
- Li, J.; Obi, S.; Feng, Z. The effects of clearance sizes on labyrinth brush seal leakage performance using a Reynolds-averaged Navier-Stokes solver and non-Darcian porous medium model. Proc. Inst. Mech. Eng. Part A J. Power Energy 2009, 223, 953–964. [Google Scholar] [CrossRef]
- Ward, H.A.; Adam, I.G.; Salem, A.B.; Gamaleldin, M.W. Integral pumping rings for dual mechanical seals: Hydraulic performance evaluation using numerical simulations. Eng. Appl. Comput. Fluid Mech. 2020, 14, 923–938. [Google Scholar] [CrossRef]
- Hur, M.S.; Lee, S.I.; Moon, S.W.; Kim, T.S.; Kwak, J.S.; Kim, D.H.; Jung, I.Y. Effect of Clearance and Cavity Geometries on Leakage Performance of a Stepped Labyrinth Seal. Processes 2020, 8, 1496. [Google Scholar] [CrossRef]
- Dogu, Y.; Aksit, M.F.; Bagepalli, B.; Burns, J.; Sexton, B.; Kellock, I. Thermal and flow analysis of cloth-seal in slot for gas turbine shroud applications. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 13–15 July 1998. [Google Scholar] [CrossRef]
- Sutherland, W. The viscosity of gases and molecular force. Philos. Mag. 1893, 36, 507–531. [Google Scholar] [CrossRef]
- ANSYS. ANSYS CFX-Solver Theory Guide; Version 14; ANSYS: Canonsburg, PA, USA, 2011. [Google Scholar]
- Crudgington, P.F. Brush Seal Performance Evaluation. In Proceedings of the 34th AIAA/ASME/SAE/ASEE Joint Propulsion Conference and Exhibit, Cleveland, OH, USA, 13–15 July 1998. [Google Scholar]
- Chen, L.H.; Wood, P.E.; Jones, T.V.; Chew, J.W. An Iterative CFD and Mechanical Brush Seal Model and Comparison with Experimental Results. J. Eng. Gas. Turbines Power 1999, 121, 656–662. [Google Scholar] [CrossRef]
- Ergun, S. Fluid flow through packed columns. Chem. Eng. Prog. 1952, 48, 89–94. [Google Scholar]








| Material Property | Value |
|---|---|
| Nominal composition (weight percentage) | Cobalt (51%), nickel (10%), iron (3% max.), chromium (20%), molybdenum (1% max.), tungsten (15%), manganese (1.5%), silicon (0.4% max), carbon (0.1%) |
| Density | 9.07 g/cm3 |
| Melting range | 1330–1410 °C |
| Thermal conductivity | 10.5 W/m- °C |
| Specific heat | 403 J/kg- °C |
| Dynamic modulus of elasticity | 225 GPa |
| Ultimate tensile strength | 1015 MPa |
| Mesh Details | M1 | M2 | M3 | M4 | M5 |
|---|---|---|---|---|---|
| Number of elements | 40,000 | 50,000 | 60,000 | 80,000 | 100,000 |
| Number of nodes in height direction (metallic-cloth fiber region) | 15 | 17 | 20 | 24 | 30 |
| Number of nodes in length direction (metallic-cloth fiber region) | 120 | 140 | 160 | 180 | 200 |
| Property | Present Study | Dinc et al. Study [1] |
|---|---|---|
| Temperature | Room temperature | Room temperature |
| Pressure ratio | 0.12–0.42 | 0.07–0.25 |
| Fluid | Air | Air |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gorgun, E.; Dogu, Y.; Aksit, M.F. Investigation of Flow Behavior and Porous Medium Resistance Coefficients for Metallic-Cloth Fibers. Fibers 2020, 8, 75. https://doi.org/10.3390/fib8120075
Gorgun E, Dogu Y, Aksit MF. Investigation of Flow Behavior and Porous Medium Resistance Coefficients for Metallic-Cloth Fibers. Fibers. 2020; 8(12):75. https://doi.org/10.3390/fib8120075
Chicago/Turabian StyleGorgun, Erdem, Yahya Dogu, and Mahmut Faruk Aksit. 2020. "Investigation of Flow Behavior and Porous Medium Resistance Coefficients for Metallic-Cloth Fibers" Fibers 8, no. 12: 75. https://doi.org/10.3390/fib8120075
APA StyleGorgun, E., Dogu, Y., & Aksit, M. F. (2020). Investigation of Flow Behavior and Porous Medium Resistance Coefficients for Metallic-Cloth Fibers. Fibers, 8(12), 75. https://doi.org/10.3390/fib8120075





