Manufacturing and Spectral Features of Different Types of Long Period Fiber Gratings: Phase-Shifted, Turn-Around Point, Internally Tilted, and Pseudo-Random
Abstract
1. Introduction
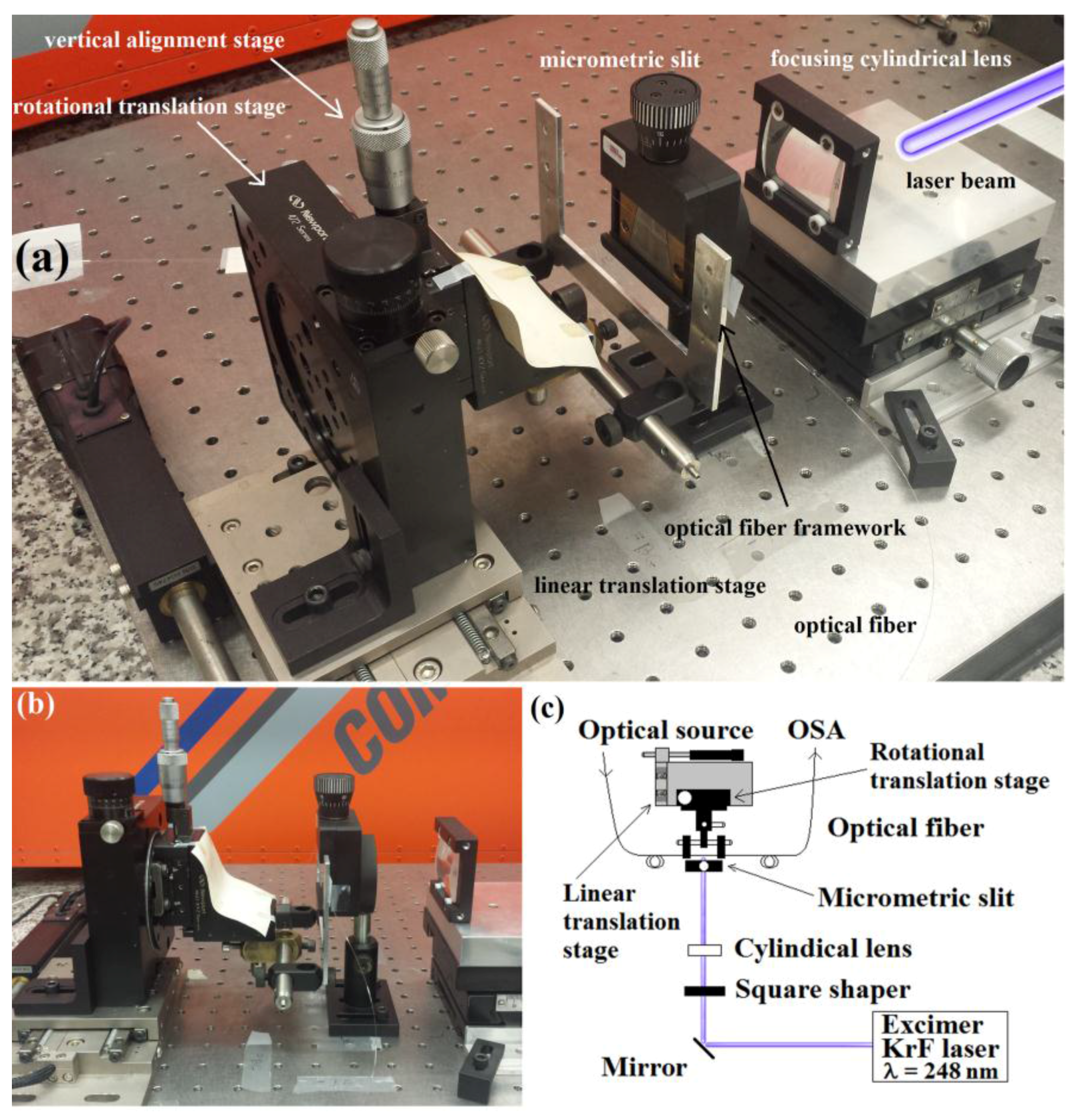
2. Materials and Methods
- a focusing cylindrical lens (focal length 10 cm) placed at a horizontal distance of 85 mm with respect to the fiber, which allows the best focusing of the laser beam on the fiber;
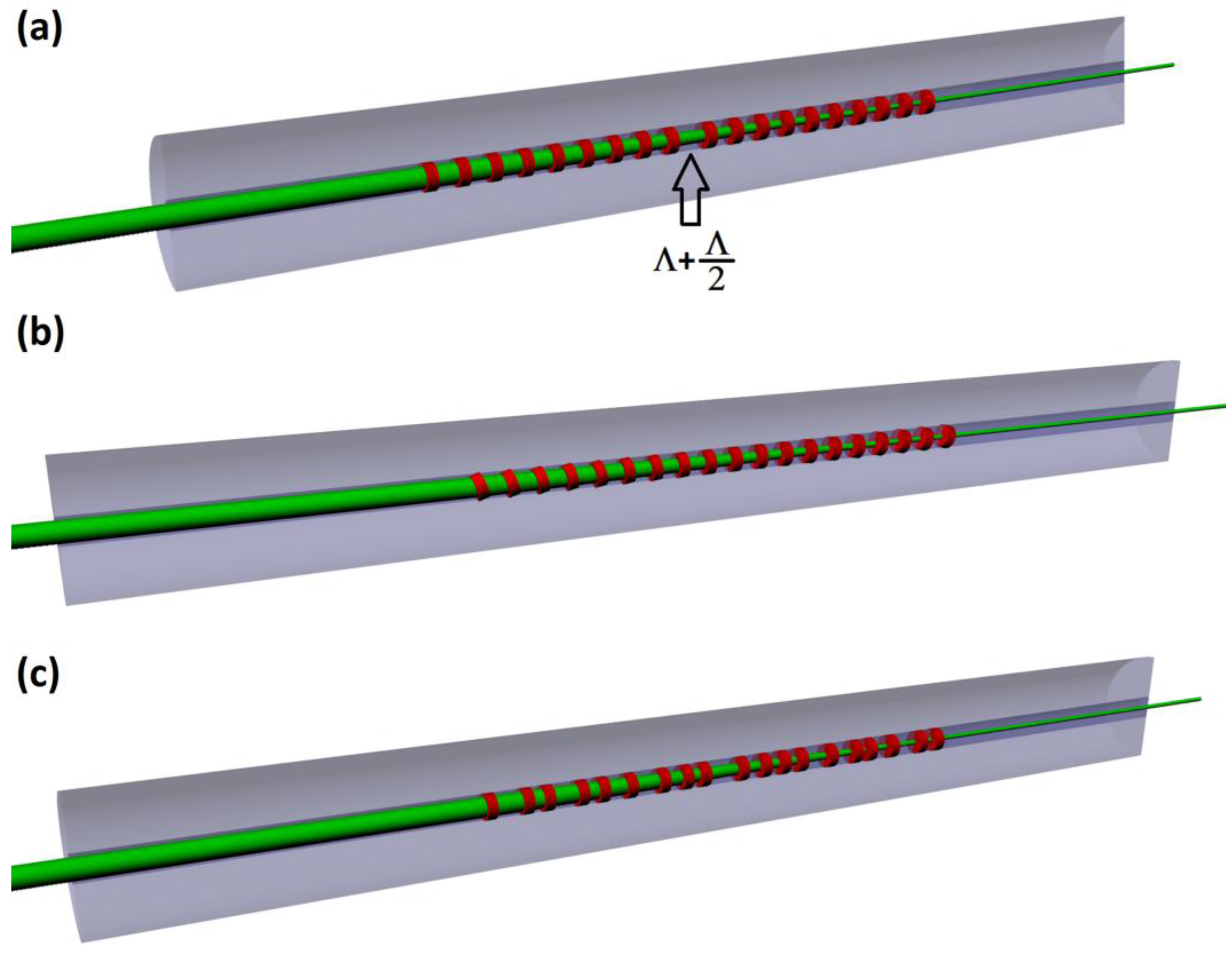
- a manual-adjusting micrometric slit that is set to half of the chosen grating period, Λ/2 (1 μm resolution; Micro-Controle, France);
- a homemade framework that allows placing the optical fiber into U-grooves and keeping it straight;
- a vertical (manual) translation stage that allows positioning the optical fiber at the same height of the laser beam (0.5 μm resolution; differential micrometer DM-13 mounted on a Newport 460A-XYZ series translation stage) (Newport Corporation, Irvine, CA 92606, USA);
- a rotational (manual) translation stage that allows the manufacturing of tilted grating planes as in Figure 2a (0.01° resolution; precision rotation stage, Newport 472 series); and
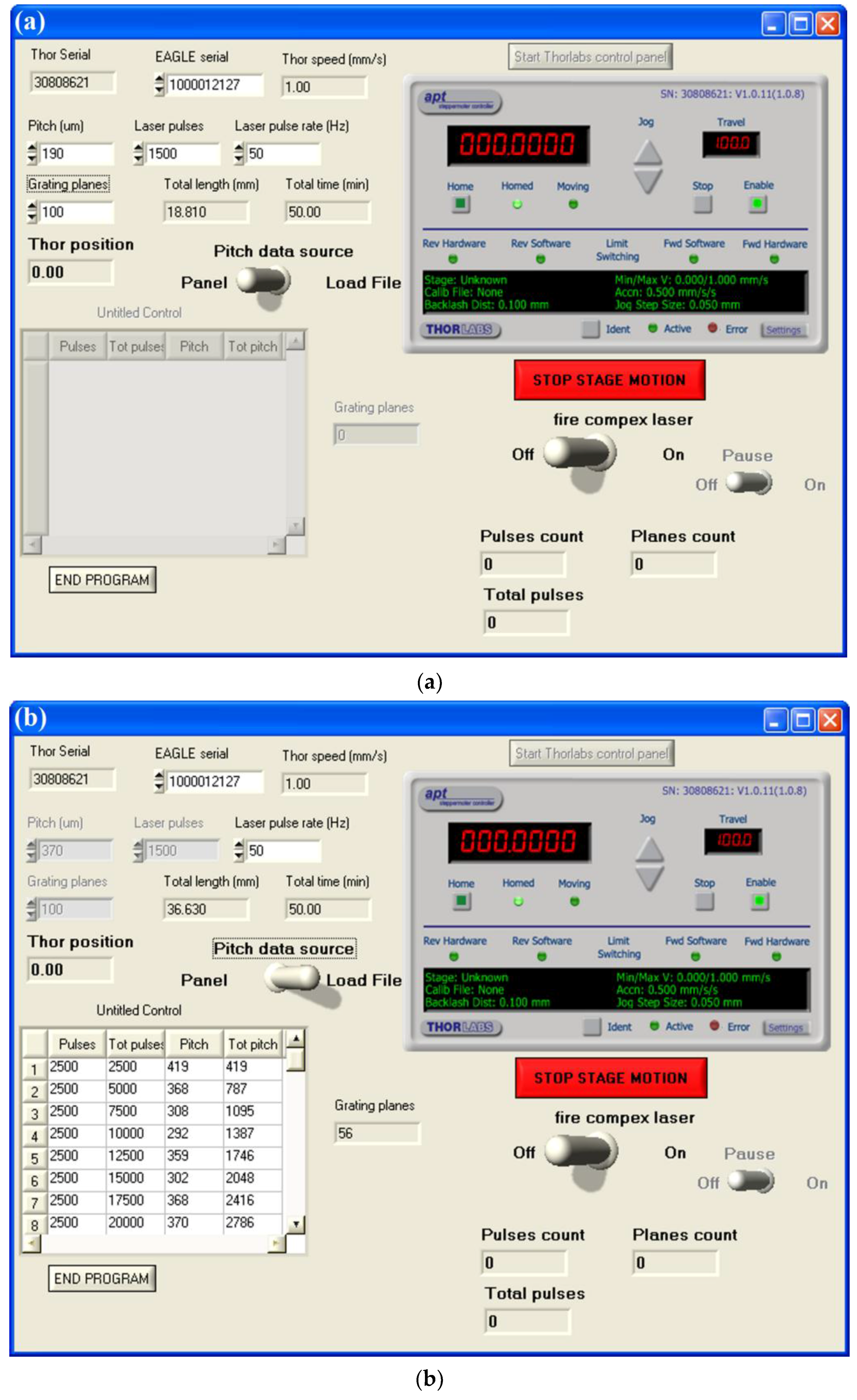
- a linear PC-controlled translation stage that allows the horizontal movement of the optical fiber according to the chosen grating period (0.1 μm resolution and 0.3 μm bidirectional repeatability; an LNR 50 series stepper motor driver controlled by an APT stepper motor controller, Thorlabs BSC001, Thorlabs Inc., Newton, NJ, USA).
3. Results and Discussion
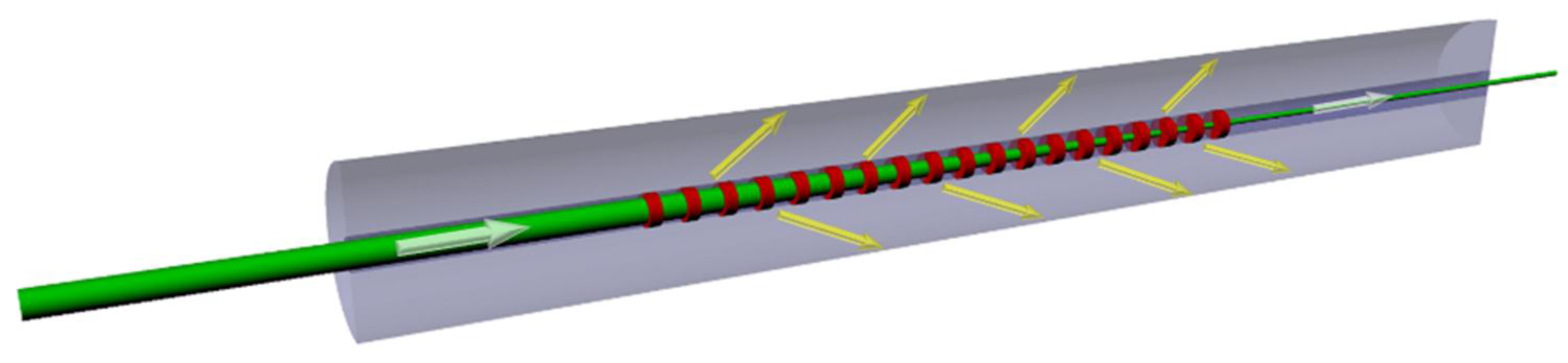
3.1. Phase-Shifted LPFGs
3.2. Turn-Around Point LPFGs
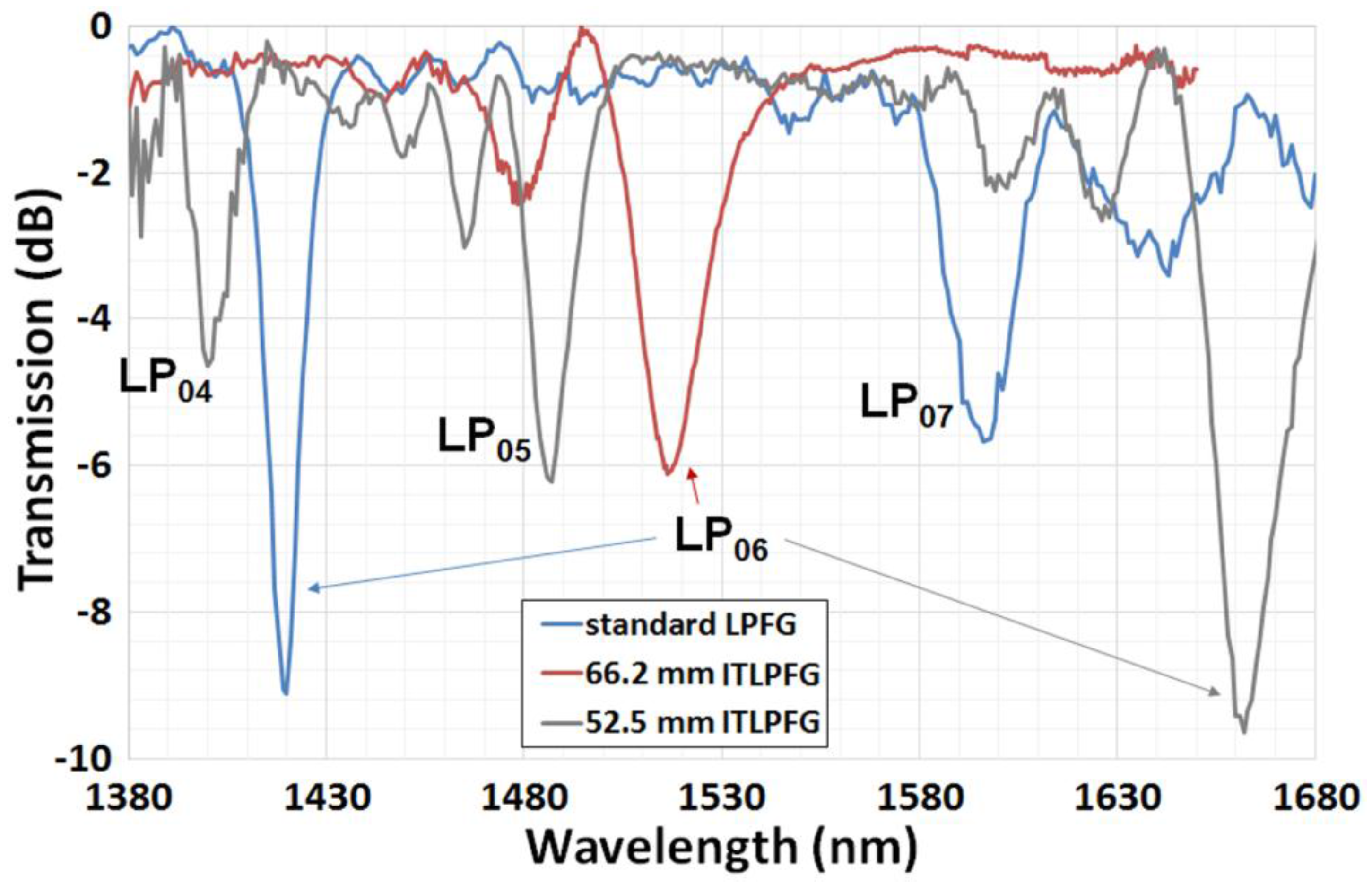
3.3. Internally-Tilted LPFGs
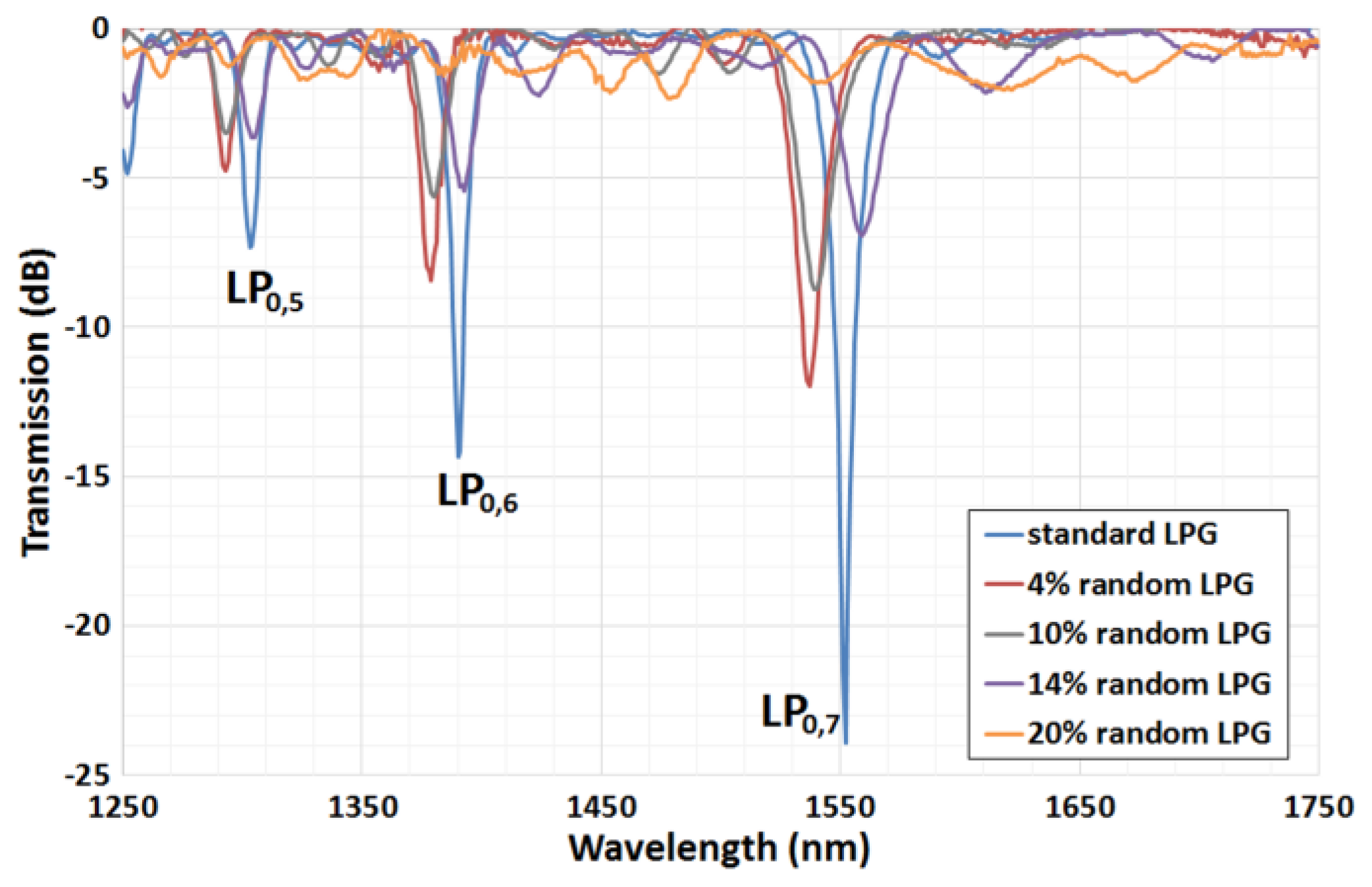
3.4. Pseudo-Random LPFGs
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Grattan, K.T.V.; Sun, T. Fiber optic sensor technology: An overview. Sens. Actuators A 2000, 82, 40–61. [Google Scholar] [CrossRef]
- Baldini, F.; Brenci, M.; Chiavaioli, F.; Giannetti, A.; Trono, C. Optical fibre gratings as tools for chemical and biochemical sensing. Anal. Bioanal. Chem. 2012, 402, 109–116. [Google Scholar] [CrossRef] [PubMed]
- Chiavaioli, F.; Baldini, F.; Tombelli, S.; Trono, C.; Giannetti, A. Biosensing with optical fibre gratings. Nanophotonics 2017, 6, 663–679. [Google Scholar] [CrossRef]
- Vaiano, P.; Carotenuto, B.; Pisco, M.; Ricciardi, A.; Quero, Q.; Consales, M.; Crescitelli, A.; Esposito, E.; Cusano, A. Lab on Fiber Technology for biological sensing applications. Laser Photonics Rev. 2016, 10, 922–961. [Google Scholar] [CrossRef]
- Vengsarkar, A.M.; Lemaire, P.J.; Judkins, J.B.; Bhatia, V.; Erdogan, T.; Sipe, J.E. Long-period fiber gratings as band-rejection filters. J. Lightwave Technol. 1996, 14, 58–65. [Google Scholar] [CrossRef]
- Wang, Z.; Ramachandran, S. Ultrasensitive long-period fiber gratings for broadband modulators and sensors. Opt. Lett. 2003, 28, 2458–2460. [Google Scholar] [CrossRef] [PubMed]
- Ashrafi, R.; Li, M.; Azaña, J. Coupling-strength-independent long-period grating designs for THz-bandwidth optical differentiators. IEEE Photonics J. 2013, 5, 7100311. [Google Scholar] [CrossRef]
- Farnesi, D.; Chiavaioli, F.; Righini, G.C.; Soria, S.; Trono, C.; Jorge, P.; Nunzi Conti, G. Long period grating-based fiber coupler to whispering gallery mode resonators. Opt. Lett. 2014, 39, 6525–6528. [Google Scholar] [CrossRef] [PubMed]
- Farnesi, D.; Chiavaioli, F.; Baldini, F.; Righini, G.C.; Soria, S.; Trono, C.; Nunzi Conti, G. Quasi-distributed and wavelength selective addressing of optical micro-resonators based on long period fiber gratings. Opt. Express 2015, 23, 21175–21180. [Google Scholar] [CrossRef] [PubMed]
- Gagné, M.; Kashyap, R. Random fiber Bragg grating Raman fiber laser. Opt. Lett. 2014, 39, 2755–2758. [Google Scholar] [CrossRef] [PubMed]
- Yin, H.; Gbadebo, A.; Turitsyna, E.G. Top-hat random fiber Bragg grating. Opt. Lett. 2015, 40, 3592–3594. [Google Scholar] [CrossRef] [PubMed]
- Kersey, A.D.; Davis, M.A.; Patrick, H.J.; LeBlanc, M.; Koo, K.; Askins, C.; Putnam, M.; Friebele, E.J. Fiber grating sensors. J. Lightwave Technol. 1997, 15, 1442–1463. [Google Scholar] [CrossRef]
- Bhatia, V.; Vengsarkar, A.M. Optical fiber long-period grating sensors. Opt. Lett. 1996, 21, 692–694. [Google Scholar] [CrossRef] [PubMed]
- Huang, Q.; Chen, H. Multi-parameter optochemical sensing based on coated cascaded long-period fiber gratings and frequency division multiplexing. Optik 2017, 132, 348–355. [Google Scholar] [CrossRef]
- Chiavaioli, F.; Biswas, P.; Trono, C.; Jana, S.; Bandyopadhyay, S.; Basumallick, N.; Giannetti, A.; Tombelli, S.; Bera, S.; Mallick, A.; et al. Sol-gel-based titania-silica thin film overlay for long period fiber grating-based biosensors. Anal. Chem. 2015, 87, 12024–12031. [Google Scholar] [CrossRef] [PubMed]
- Chiavaioli, F.; Trono, C.; Giannetti, A.; Brenci, M.; Baldini, F. Characterisation of a label-free biosensor based on long period grating. J. Biophotonics 2014, 7, 312–322. [Google Scholar] [CrossRef] [PubMed]
- Shu, X.; Zhang, L.; Bennion, I. Sensitivity characteristics of long-period fiber gratings. J. Lightwave Technol. 2002, 20, 255–266. [Google Scholar]
- Erdogan, T. Fiber grating spectra. J. Lightwave Technol. 1997, 15, 1277–1294. [Google Scholar] [CrossRef]
- Dong, X.; Zhang, H.; Liu, B.; Miao, Y. Tilted fiber Bragg gratings: Principle and sensing applications. Photonics Sens. 2011, 1, 6–30. [Google Scholar] [CrossRef]
- Guo, T.; Liu, F.; Guan, B.-O.; Albert, J. Tilted fiber grating mechanical and biochemical sensors. Opt. Laser Technol. 2016, 78, 19–33. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, L.; Bennion, I. Fabricating fibre edge filters with arbitrary spectral response based on tilted chirped grating structures. Meas. Sci. Technol. 1999, 10, L1–L3. [Google Scholar] [CrossRef]
- Chen, X.; Zhou, K.; Zhang, L.; Bennion, I. In-Fiber twist sensor based on a Fiber Bragg Grating with 81° tilted structure. IEEE Photonics Technol. Lett. 2006, 18, 2596–2598. [Google Scholar] [CrossRef]
- Agrawal, G.P.; Radic, S. Phase-shifted fiber Bragg gratings and their application for wavelength demultiplexing. IEEE Photonics Technol. Lett. 1994, 6, 995–997. [Google Scholar] [CrossRef]
- Liu, T.; Han, M. Analysis of π-Phase-Shifted Fiber Bragg Gratings for ultrasonic detection. IEEE Sens. J. 2012, 12, 2368–2373. [Google Scholar] [CrossRef]
- Chiavaioli, F.; Trono, C.; Baldini, F. Specially designed long period grating with internal geometric bending for enhanced refractive index sensitivity. Appl. Phys. Lett. 2013, 102, 231109. [Google Scholar] [CrossRef]
- Arjmand, M.; Chiavaioli, F.; Berneschi, S.; Baldini, F.; Soltanolkotabi, M.; Trono, C. Effect of induced inner curvature on refractive index sensitivity in internally tilted long-period gratings. Opt. Lett. 2016, 41, 1443–1446. [Google Scholar] [CrossRef] [PubMed]
- He, T.; Rishoj, L.; Demas, J.; Ramachandran, S. Dispersion compensation using chirped long period gratings. In Proceedings of the Conference on Lasers and Electro-Optics, San Jose, CA, USA, 5–10 June 2016. [Google Scholar]
- Verma, D.S.; Tripathi, S.M. Simultaneous measurement of temperature and strain using chirped long-period gratings. In Proceedings of the 13th International Conference on Fiber Optics and Photonics, Kanpur, India, 4–8 December 2016. [Google Scholar]
- Wong, R.Y.N.; Chehura, E.; James, S.W.; Tatam, R.P. A chirped long period grating sensor for monitoring flow direction and cure of a resin. In Proceedings of the Smart Sensor Phenomena, Technology, Networks, and Systems Integration 2013, San Diego, CA, USA, 10 March 2013. [Google Scholar]
- James, S.W.; Topliss, S.M.; Tatam, R.P. Properties of length-apodized phase-shifted LPGs operating at the phase matching turning point. J. Lightwave Technol. 2012, 30, 2203–2209. [Google Scholar] [CrossRef]
- Wong, R.Y.N.; Chehura, E.; Staines, S.E.; James, S.W.; Tatam, R.P. Fabrication of fiber optic long period gratings operating at the phase matching turning point using an ultraviolet laser. Appl. Opt. 2014, 53, 4669–4674. [Google Scholar] [CrossRef] [PubMed]
- Chiavaioli, F.; Biswas, P.; Trono, C.; Bandyopadhyay, S.; Giannetti, A.; Tombelli, S.; Basumallick, N.; Dasgupta, K.; Baldini, F. Towards sensitive label-free immunosensing by means of turn-around point long period fiber gratings. Biosens. Bioelectron. 2014, 60, 305–310. [Google Scholar] [CrossRef] [PubMed]
- Colaço, C.; Caldas, P.; Del Villar, I.; Chibante, R.; Rego, G. Arc-induced long-period fiber gratings in the dispersion turning points. IEEE J. Lightwwave Technol. 2016, 34, 4584–4590. [Google Scholar] [CrossRef]
- Szymańska, M.; Krogulski, K.; Mikulic, P.; Bock, W.J.; Śmietana, M. Sensitivity of long-period gratings modified by their bending. Proc. Eng. 2014, 87, 1180–1183. [Google Scholar] [CrossRef]
- Trono, C.; Baldini, F.; Brenci, M.; Chiavaioli, F.; Mugnaini, M. Flow cell for strain- and temperature-compensated refractive index measurements by means of cascaded optical fibre long period and Bragg gratings. Meas. Sci. Technol. 2011, 22, 075204. [Google Scholar] [CrossRef]
- Liu, Y.; Williams, J.A.R.; Zhang, L.; Bennion, I. Phase shifted and cascaded long-period fiber gratings. Opt. Commun. 1999, 164, 27–31. [Google Scholar] [CrossRef]
- Hishiki, K.; Li, H. Phase-shift formed in a long period fiber grating and its application to the measurements of temperature and refractive index. Opt. Express 2013, 21, 11901–11912. [Google Scholar] [CrossRef] [PubMed]
- Tripathi, S.M.; Bock, W.J.; Mikulic, P.; Chinnappan, R.; Ng, A.; Tolba, M.; Zourob, M. Long period grating based biosensor for the detection of Escherichia coli bacteria. Biosens. Bioelectron. 2012, 35, 308–312. [Google Scholar] [CrossRef] [PubMed]
- Biswas, P.; Basumallick, N.; Bandyopadhyay, S.; Dasgupta, K.; Ghosh, A.; Bandyopadhyay, S. Sensitivity enhancement of turn-around-point long period gratings by tuning initial coupling condition. IEEE Sens. J. 2015, 15, 1240–1245. [Google Scholar] [CrossRef]
- Chiavaioli, F.; Gouveia, C.A.J.; Jorge, P.A.S.; Baldini, F. Towards uniform metrological assessment of grating-based optical fiber sensors: From refractometers to biosensors. Biosensors 2017, 7, 32. [Google Scholar] [CrossRef] [PubMed]
- White, I.M.; Fan, X. On the performance quantification of resonant refractive index sensors. Opt. Express 2008, 16, 1020–1028. [Google Scholar] [CrossRef] [PubMed]
- Tsuda, H.; Urabe, K. Characterization of long-period grating refractive index sensors and their applications. Sensors 2009, 9, 4559–7145. [Google Scholar] [CrossRef] [PubMed]
- Lepri, S.; Trono, C.; Giacomelli, G. Complex active optical networks as a new laser concept. Phys. Rev. Lett. 2017, 118, 123901. [Google Scholar] [CrossRef] [PubMed]
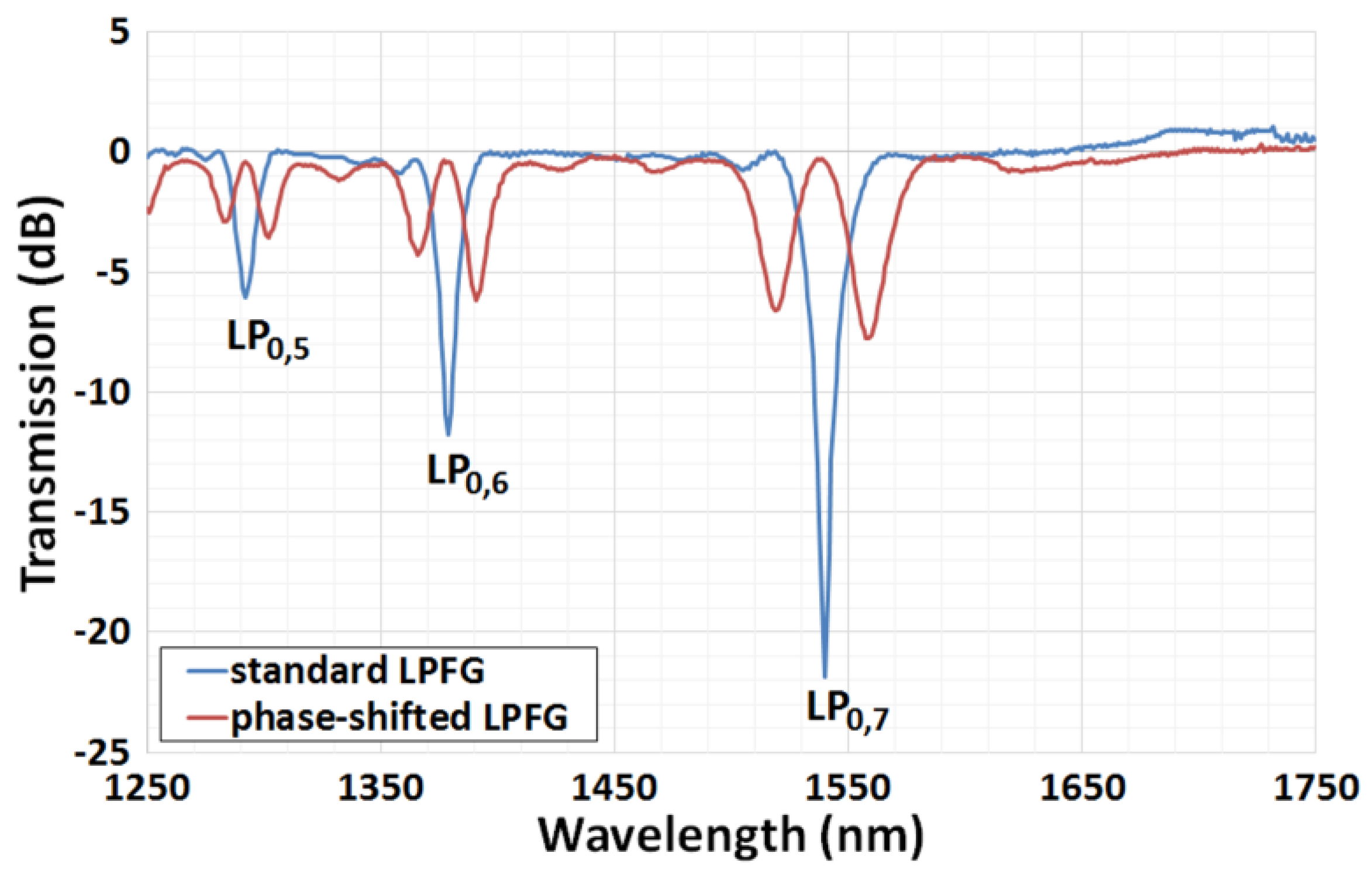
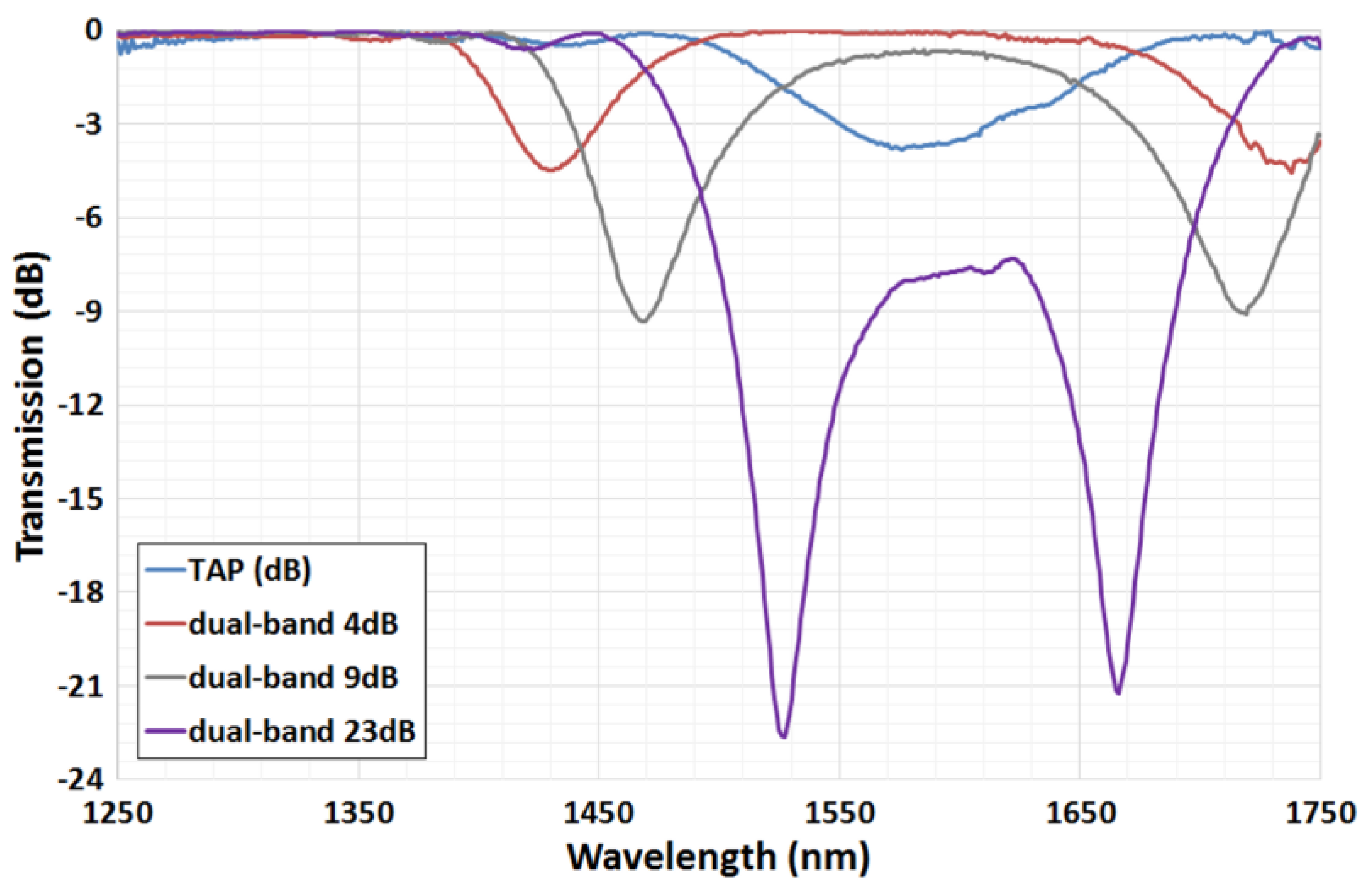
| Main Characteristics | Λ (μm) | N | Nlas | λres (nm), LP0,11 | Tmax (dB) | ∆λ (nm) | FWHM (nm) |
|---|---|---|---|---|---|---|---|
| TAP | 192 | 100 | 1000 | 1580 | 3.5 | - | 125 |
| Dual-band | 191 | 100 | 400 | 1430 (left), 1740 (right) | 4 | 310 | 55 |
| Dual-band | 191 | 100 | 1000 | 1468 (left), 1718 (right) | 9 | 250 | 45 |
| Dual-band | 191 | 112 | 1500 | 1525 (left), 1665 (right) | 23 | 140 | 21 |
| Main Characteristics | Λ (μm) | N | Nlas | R (mm) | θ | λres (nm), LP0,6 | Tmax (dB) | FWHM (nm) |
|---|---|---|---|---|---|---|---|---|
| Standard | 370 | 55 | 700 | - | - | 1419 | 9.1 | 11 |
| Internally tilted | 370 | 55 | 700 | 66.2 | 17.3° | 1516 | 6.2 | 20 |
| Internally tilted | 330 | 61 | 900 | 52.5 | 21.6° | 1662 | 9.6 | 25 |
| Main Characteristics | δ (μm) | δ (%) | λres (nm), LP0,7 | Tmax (dB) | FWHM (nm) |
|---|---|---|---|---|---|
| Standard | - | - | 1552 | 24 | 8 |
| Pseudo-random | 15 | 4.2 | 1537 | 12 | 14 |
| Pseudo-random | 35 | 9.8 | 1540 | 8.8 | 16 |
| Pseudo-random | 50 | 14.1 | 1559 | 7 | 18 |
| Pseudo-random | 70 | 19.7 | 1542 | 2 | 25 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chiavaioli, F.; Baldini, F.; Trono, C. Manufacturing and Spectral Features of Different Types of Long Period Fiber Gratings: Phase-Shifted, Turn-Around Point, Internally Tilted, and Pseudo-Random. Fibers 2017, 5, 29. https://doi.org/10.3390/fib5030029
Chiavaioli F, Baldini F, Trono C. Manufacturing and Spectral Features of Different Types of Long Period Fiber Gratings: Phase-Shifted, Turn-Around Point, Internally Tilted, and Pseudo-Random. Fibers. 2017; 5(3):29. https://doi.org/10.3390/fib5030029
Chicago/Turabian StyleChiavaioli, Francesco, Francesco Baldini, and Cosimo Trono. 2017. "Manufacturing and Spectral Features of Different Types of Long Period Fiber Gratings: Phase-Shifted, Turn-Around Point, Internally Tilted, and Pseudo-Random" Fibers 5, no. 3: 29. https://doi.org/10.3390/fib5030029
APA StyleChiavaioli, F., Baldini, F., & Trono, C. (2017). Manufacturing and Spectral Features of Different Types of Long Period Fiber Gratings: Phase-Shifted, Turn-Around Point, Internally Tilted, and Pseudo-Random. Fibers, 5(3), 29. https://doi.org/10.3390/fib5030029








