Observation of Visible Upconversion Luminescence of Soft Glass Multimode Fibers
Abstract
1. Introduction
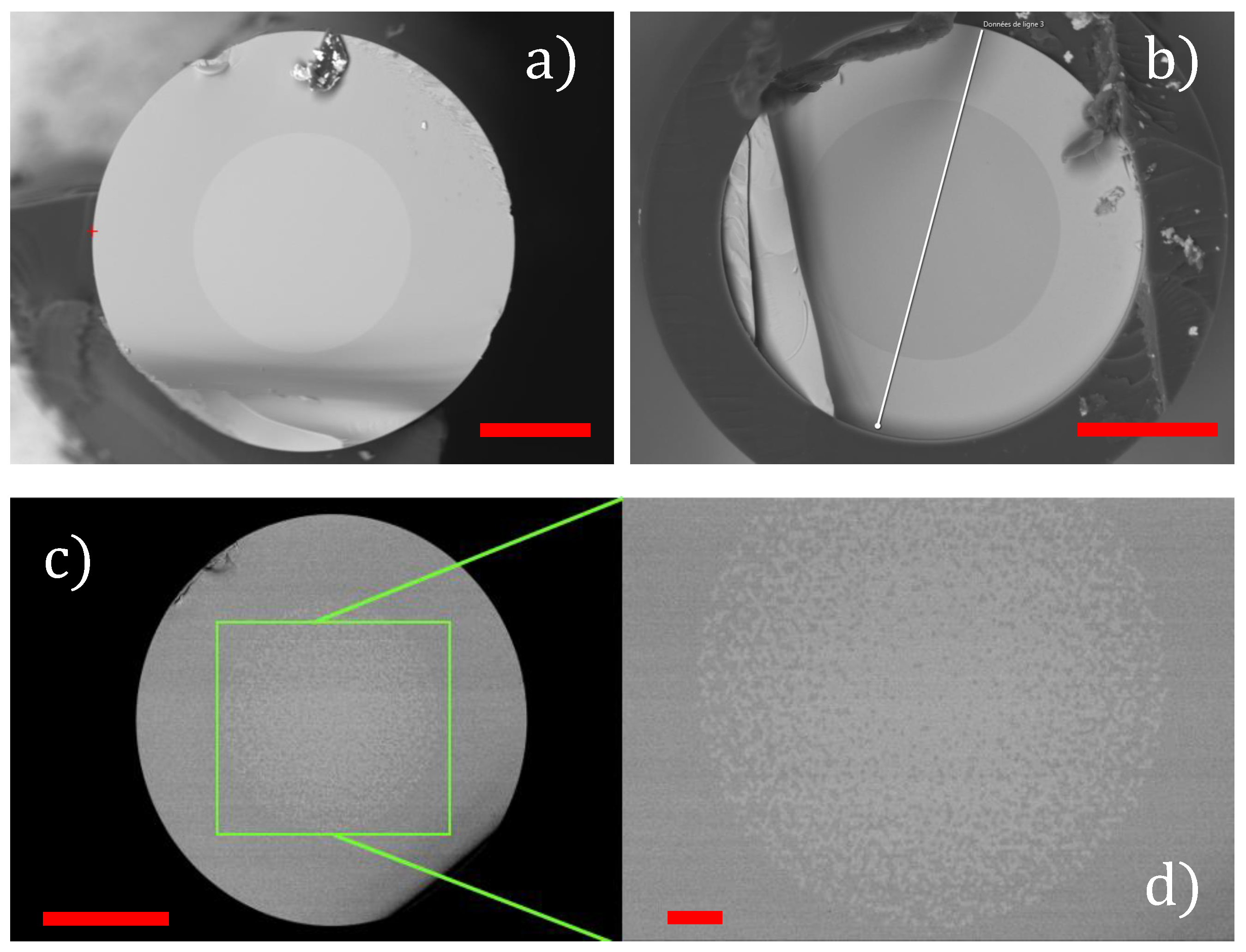
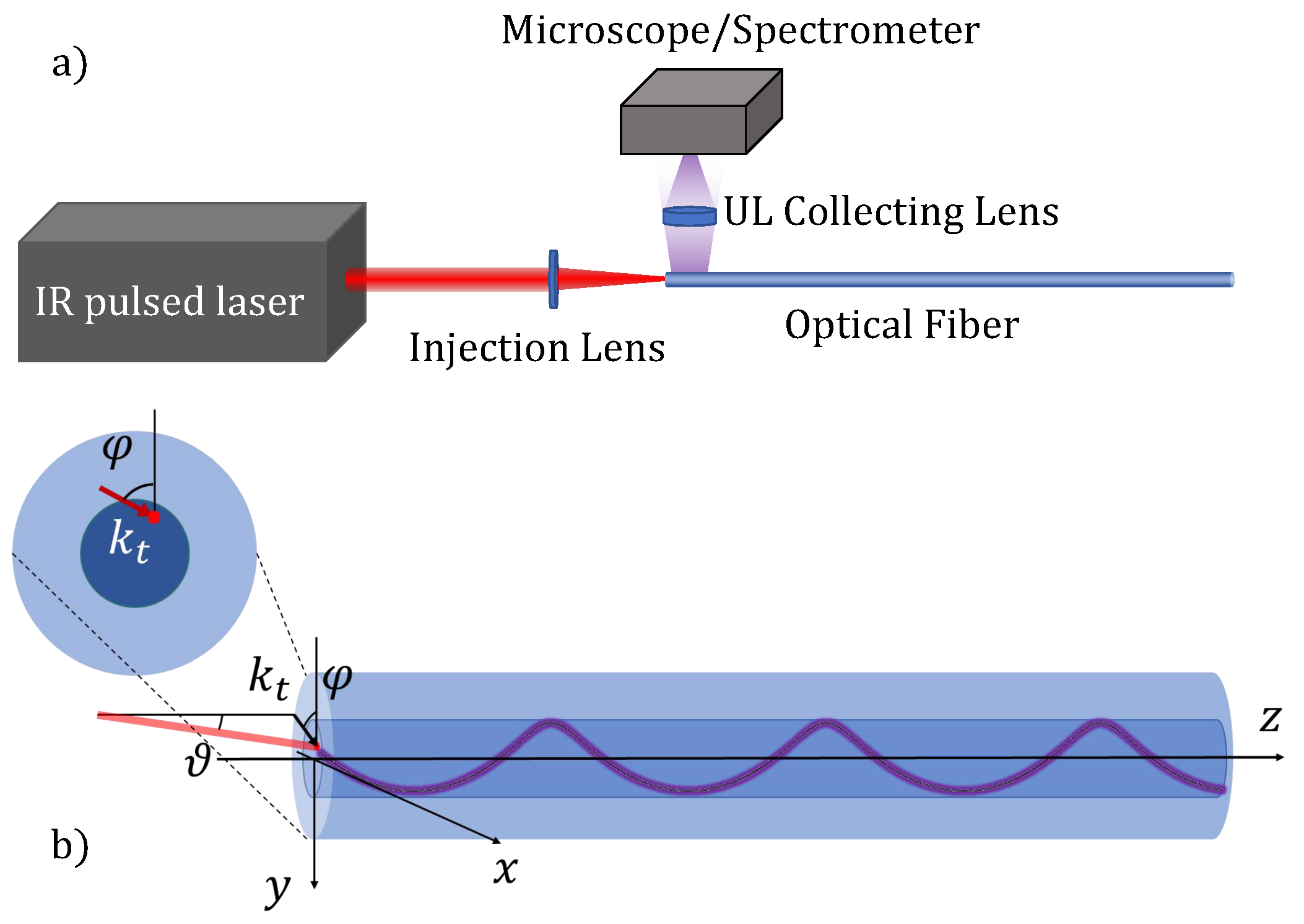
2. Materials and Methods
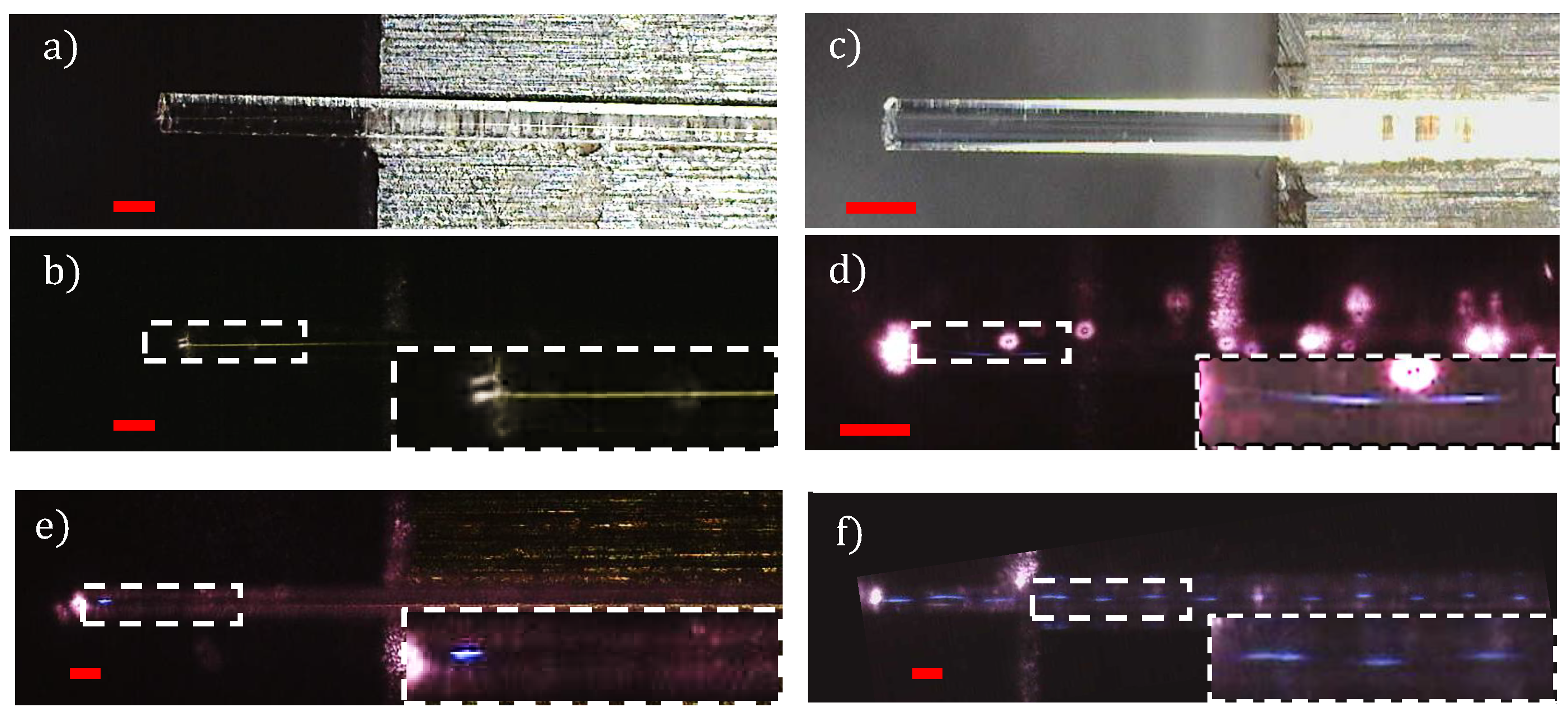
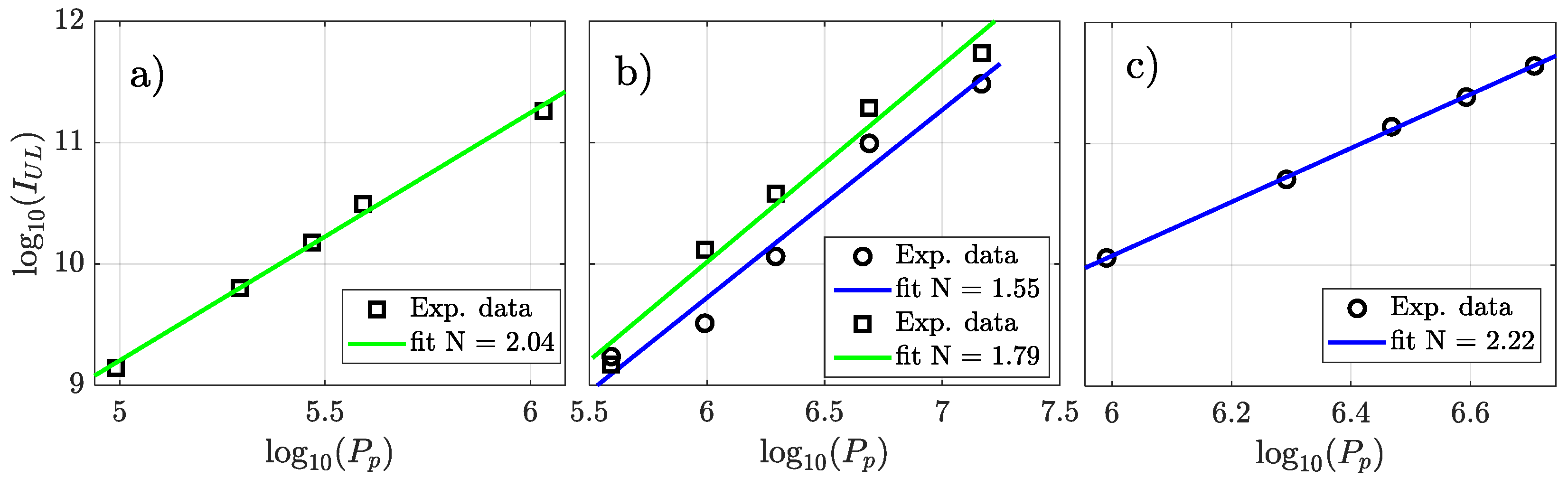
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Miller, S. Optical Fiber Telecommunications; Elsevier: Amsterdam, The Netherlands, 2012. [Google Scholar]
- Agrawal, G.P. Nonlinear fiber optics. In Nonlinear Science at the Dawn of the 21st Century; Springer: Berlin/Heidelberg, Germany, 2000; pp. 195–211. [Google Scholar]
- Petersen, C.R.; Møller, U.; Kubat, I.; Zhou, B.; Dupont, S.; Ramsay, J.; Benson, T.; Sujecki, S.; Abdel-Moneim, N.; Tang, Z.; et al. Mid-infrared supercontinuum covering the 1.4–13.3 μm molecular fingerprint region using ultra-high NA chalcogenide step-index fibre. Nat. Photonics 2014, 8, 830–834. [Google Scholar] [CrossRef]
- Saini, T.S.; Sinha, R.K. Mid-infrared supercontinuum generation in soft-glass specialty optical fibers: A review. Prog. Quantum Electron. 2021, 78, 100342. [Google Scholar] [CrossRef]
- Werle, P.; Slemr, F.; Maurer, K.; Kormann, R.; Mücke, R.; Jänker, B. Near-and mid-infrared laser-optical sensors for gas analysis. Opt. Lasers Eng. 2002, 37, 101–114. [Google Scholar] [CrossRef]
- Gao, W.; Li, X.; Wang, P.; Chen, L.; Ni, C.; Chen, L.; Chen, X.; Zhou, Y.; Zhang, W.; Hu, J.; et al. Investigation on sensing characteristics of fiber Bragg gratings based on soft glass fibers. Optik 2018, 156, 13–21. [Google Scholar] [CrossRef]
- Shi, L.; Alfano, R.R. Deep Imaging in Tissue and Biomedical Materials: Using Linear and Nonlinear Optical Methods; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Barik, A.K.; Lukose, J.; Upadhya, R.; Pai, M.V.; Kartha, V.; Chidangil, S. In vivo spectroscopy: Optical fiber probes for clinical applications. Expert Rev. Med. Devices 2022, 19, 657–675. [Google Scholar] [CrossRef]
- Wang, W.; Zhou, B.; Xu, S.; Yang, Z.; Zhang, Q. Recent advances in soft optical glass fiber and fiber lasers. Prog. Mater. Sci. 2019, 101, 90–171. [Google Scholar] [CrossRef]
- Xia, C.; Kumar, M.; Kulkarni, O.P.; Islam, M.N.; Terry Jr, F.L.; Freeman, M.J.; Poulain, M.; Mazé, G. Mid-infrared supercontinuum generation to 4.5 μm in ZBLAN fluoride fibers by nanosecond diode pumping. Opt. Lett. 2006, 31, 2553–2555. [Google Scholar] [CrossRef]
- Désévédavy, F.; Strutynski, C.; Lemière, A.; Mathey, P.; Gadret, G.; Jules, J.C.; Kibler, B.; Smektala, F. Review of tellurite glasses purification issues for mid-IR optical fiber applications. J. Am. Ceram. Soc. 2020, 103, 4017–4034. [Google Scholar] [CrossRef]
- Chen, C.; Jaluria, Y. Effects of doping on the optical fiber drawing process. Int. J. Heat Mass Transf. 2009, 52, 4812–4822. [Google Scholar] [CrossRef]
- Fokine, M. Thermal stability of chemical composition gratings in fluorine–germanium-doped silica fibers. Opt. Lett. 2002, 27, 1016–1018. [Google Scholar] [CrossRef] [PubMed]
- Nagel, S.R.; MacChesney, J.B.; Walker, K.L. An overview of the modified chemical vapor deposition (MCVD) process and performance. IEEE Trans. Microw. Theory Tech. 1982, 30, 305–322. [Google Scholar] [CrossRef]
- Méndez, A.; Morse, T.F. Specialty Optical Fibers Handbook; Elsevier: Amsterdam, The Netherlands, 2011. [Google Scholar]
- Agrawal, G.P. Invite paper: Self-imaging in multimode graded-index fibers and its impact on the nonlinear phenomena. Opt. Fiber Technol. 2019, 50, 309–316. [Google Scholar] [CrossRef]
- Poletti, F.; Horak, P. Description of ultrashort pulse propagation in multimode optical fibers. JOSA B 2008, 25, 1645–1654. [Google Scholar] [CrossRef]
- Couny, F.; Benabid, F.; Light, P. Large-pitch kagome-structured hollow-core photonic crystal fiber. Opt. Lett. 2006, 31, 3574–3576. [Google Scholar] [CrossRef]
- Strutynski, C.; Meza, R.A.; Teulé-Gay, L.; El-Dib, G.; Poulon-Quintin, A.; Salvetat, J.p.; Vellutini, L.; Dussauze, M.; Cardinal, T.; Danto, S. Stack-and-Draw Applied to the Engineering of Multi-Material Fibers with Non-Cylindrical Profiles. Adv. Funct. Mater. 2021, 31, 2011063. [Google Scholar] [CrossRef]
- Buczyński, R.; Klimczak, M.; Stefaniuk, T.; Kasztelanic, R.; Siwicki, B.; Stępniewski, G.; Cimek, J.; Pysz, D.; Stępień, R. Optical fibers with gradient index nanostructured core. Opt. Express 2015, 23, 25588–25596. [Google Scholar] [CrossRef]
- Crocco, M.C.; Mangini, F.; Filosa, R.; Solano, A.; Agostino, R.G.; Barberi, R.C.; Couderc, V.; Klimczak, M.; Filipkowski, A.; Buczynski, R.; et al. Soft glass optical fiber characterization with X-ray computed microtomography. Opt. Mater. Express 2024, 14, 70–81. [Google Scholar] [CrossRef]
- Karpate, T.; Stępniewski, G.; Kardaś, T.; Pysz, D.; Kasztalanic, R.; Stepanenko, Y.; Buczyński, R.; Krupa, K.; Klimczak, M. Quasi-periodic spectro-temporal pulse breathing in a femtosecond-pumped tellurite graded-index multimode fiber. Opt. Express 2023, 31, 13269–13278. [Google Scholar] [CrossRef]
- Eslami, Z.; Salmela, L.; Filipkowski, A.; Pysz, D.; Klimczak, M.; Buczynski, R.; Dudley, J.M.; Genty, G. Two octave supercontinuum generation in a non-silica graded-index multimode fiber. Nat. Commun. 2022, 13, 1–10. [Google Scholar]
- Mangini, F.; Ferraro, M.; Zitelli, M.; Niang, A.; Tonello, A.; Couderc, V.; Wabnitz, S. Multiphoton-absorption-excited up-conversion luminescence in optical fibers. Phys. Rev. Appl. 2020, 14, 054063. [Google Scholar] [CrossRef]
- Cho, S.H.; Kumagai, H.; Yokota, I.; Midorikawa, K.; Obara, M. Observation of self-channeled plasma formation and bulk modification in optical fibers using high-intensity femtosecond laser. Jpn. J. Appl. Phys. 1998, 37, L737. [Google Scholar] [CrossRef]
- Cho, S.H.; Kumagai, H.; Midorikawa, K.; Obara, M. Fabrication of double cladding structure in optical multimode fibers using plasma channeling excited by a high-intensity femtosecond laser. Opt. Commun. 1999, 168, 287–295. [Google Scholar] [CrossRef]
- Mangini, F.; Ferraro, M.; Zitelli, M.; Niang, A.; Mansuryan, T.; Tonello, A.; Couderc, V.; De Luca, A.; Babin, S.; Frezza, F.; et al. Helical plasma filaments from the self-channeling of intense femtosecond laser pulses in optical fibers. Opt. Lett. 2022, 47, 1–4. [Google Scholar] [CrossRef]
- Mangini, F.; Ferraro, M.; Zitelli, M.; Niang, A.; Tonello, A.; Couderc, V.; Sidelnikov, O.; Frezza, F.; Wabnitz, S. Experimental observation of self-imaging in SMF-28 optical fibers. Opt. Express 2021, 29, 12625–12633. [Google Scholar] [CrossRef]
- Hansson, T.; Tonello, A.; Mansuryan, T.; Mangini, F.; Zitelli, M.; Ferraro, M.; Niang, A.; Crescenzi, R.; Wabnitz, S.; Couderc, V. Nonlinear beam self-imaging and self-focusing dynamics in a GRIN multimode optical fiber: Theory and experiments. Opt. Express 2020, 28, 24005–24021. [Google Scholar] [CrossRef]
- Kumar, V.R.K.; George, A.; Reeves, W.; Knight, J.; Russell, P.S.J.; Omenetto, F.; Taylor, A. Extruded soft glass photonic crystal fiber for ultrabroad supercontinuum generation. Opt. Express 2002, 10, 1520–1525. [Google Scholar] [CrossRef]
- Cho, S.H.; Kumagai, H.; Midorikawa, K. Dynamics of permanent structural transformations in ZBLAN induced by self-channeled plasma filament. Opt. Mater. 2004, 26, 57–63. [Google Scholar] [CrossRef]
- Bernier, M.; Faucher, D.; Vallée, R.; Saliminia, A.; Androz, G.; Sheng, Y.; Chin, S. Bragg gratings photoinduced in ZBLAN fibers by femtosecond pulses at 800 nm. Opt. Lett. 2007, 32, 454–456. [Google Scholar] [CrossRef]
- Courrol, L.C.; Messaddeq, Y.; Messaddeq, S.H.; Ribeiro, S.J.; Samad, R.E.; de Freitas, A.Z.; Vieira Jr, N.D. Production of defects in ZBLAN, ZBLAN: Tm3+ and ZBLAN: Cr3+ glasses by ultra-short pulses laser interaction. J. Phys. Chem. Solids 2008, 69, 55–59. [Google Scholar] [CrossRef]
- Available online: https://www.thorlabs.com/newgrouppage9.cfm?objectgroup_id=15877 (accessed on 1 February 2024).
- Available online: https://leverrefluore.com/scientific-world/fluoride-fibers/general-properties/ (accessed on 1 February 2024).
- Von der Linde, D.; Schüler, H. Breakdown threshold and plasma formation in femtosecond laser–solid interaction. JOSA B 1996, 13, 216–222. [Google Scholar] [CrossRef]
- Zhihua, H.; Jianjun, W.; Honghuan, L.; Dangpeng, X.; Rui, Z.; Mingzhong, L.; Xiaofeng, W. Self-focusing length in highly multimode ultra-large-mode-area fibers. Opt. Express 2012, 20, 14604–14613. [Google Scholar] [CrossRef]
- Karlsson, M.; Anderson, D.; Desaix, M. Dynamics of self-focusing and self-phase modulation in a parabolic index optical fiber. Opt. Lett. 1992, 17, 22–24. [Google Scholar] [CrossRef]
- He, Z.; Li, W.; Yu, A.; Wu, Y.; Cai, Z. Efficient UV-visible emission enabled by 532 nm CW excitation in an Ho 3+-doped ZBLAN fiber. Opt. Express 2022, 30, 10414–10427. [Google Scholar] [CrossRef]
- Ma, J.; Sun, Y.; Yu, F.; Xue, T.; Hu, L. Boosting visible luminescence of Tb3+-activated ZBLAN fluoride glasses by Dy3+ co-doping. J. Lumin. 2021, 238, 118247. [Google Scholar] [CrossRef]
- Remillieux, A.; Jacquier, B. IR-to-visible up-conversion mechanisms in Pr3+-doped ZBLAN fluoride glasses and fibers. J. Lumin. 1996, 68, 279–289. [Google Scholar] [CrossRef]
- Peysokhan, M.; Mobini, E.; Allahverdi, A.; Abaie, B.; Mafi, A. Characterization of Yb-doped ZBLAN fiber as a platform for radiation-balanced lasers. Photonics Res. 2020, 8, 202–210. [Google Scholar] [CrossRef]
- Girard, S.; Alessi, A.; Richard, N.; Martin-Samos, L.; De Michele, V.; Giacomazzi, L.; Agnello, S.; Di Francesca, D.; Morana, A.; Winkler, B.; et al. Overview of radiation induced point defects in silica-based optical fibers. Rev. Phys. 2019, 4, 100032. [Google Scholar] [CrossRef]
- Pietros, A.R.; Rebeszko, K.; Rosenbaum, J.R.; Stone, M.P.; Brasovs, A.; Kornev, K.G.; Hawkins, T.; Cavillon, M.; Ballato, J.; Dragic, P.D. Investigation of intense visible defect luminescence from visible and infrared pumped barium fluorosilicate glass-core fiber. Opt. Mater. X 2023, 19, 100231. [Google Scholar] [CrossRef]
- Pietros, A.R.; Rebeszko, K.; Rosenbaum, J.R.; Stone, M.P.; Hawkins, T.; Cavillon, M.; Ballato, J.; Dragic, P.D. Luminescence Thermometry via Intense Green Defect Emission from an Infrared-Pumped Fluorosilicate Optical Fiber. In Proceedings of the Optical Fiber Sensors, Alexandria, VA, USA, 29 August–2 September 2022; pp. W4–W49. [Google Scholar]
- Underwood, C.C.; McMillen, C.D.; Chen, H.; Anker, J.N.; Kolis, J.W. Hydrothermal chemistry, structures, and luminescence studies of alkali hafnium fluorides. Inorg. Chem. 2013, 52, 237–244. [Google Scholar] [CrossRef]
- Wang, S.; Kershaw, S.V.; Li, G.; Leung, M.K. The self-assembly synthesis of tungsten oxide quantum dots with enhanced optical properties. J. Mater. Chem. C 2015, 3, 3280–3285. [Google Scholar] [CrossRef]
- Feng, M.; Pan, A.; Zhang, H.; Li, Z.; Liu, F.; Liu, H.; Shi, D.; Zou, B.; Gao, H. Strong photoluminescence of nanostructured crystalline tungsten oxide thin films. Appl. Phys. Lett. 2005, 86. [Google Scholar] [CrossRef]

| Sample Label | Type | Preform Method | Manufacturer | Material | Core/Cladding Size (m) | Reference |
|---|---|---|---|---|---|---|
| A | SI | CVD | Thorlabs | ZBLAN | 100/190 | [34] |
| B | SI | CVD | Verre Fluoré | ZBLAN | 90/150 | [35] |
| C | GI | Stack and draw | University of Warsaw | Tellurite | 78/137 | [22] |
| Z | Element | Sample A | Sample B | Sample C |
|---|---|---|---|---|
| 6 | C | 16.1 | 24.7 | 12.3 |
| 8 | O | - | - | 45.6 |
| 9 | F | 61.8 | 57.2 | - |
| 11 | Na | 4.1 | 2.2 | 3.8 |
| 13 | Al | 0.8 | 0.6 | 17.7 |
| 30 | Zn | - | - | 0.4 |
| 39 | Y | - | 0.5 | - |
| 40 | Zr | 11.3 | 5.9 | - |
| 41 | Nb | - | - | 0.9 |
| 52 | Te | - | - | 13.8 |
| 56 | Ba | 4.8 | 4.1 | - |
| 57 | La | 1.0 | 0.8 | - |
| 72 | Hf | 0.1 | 3.9 | - |
| 74 | W | - | - | 5.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ferraro, M.; Mangini, F.; Filosa, R.; Couderc, V.; Sun, Y.; Parra-Rivas, P.; Gemechu, W.A.; Stepniewski, G.; Filipkowski, A.; Buczynski, R.; et al. Observation of Visible Upconversion Luminescence of Soft Glass Multimode Fibers. Fibers 2024, 12, 15. https://doi.org/10.3390/fib12020015
Ferraro M, Mangini F, Filosa R, Couderc V, Sun Y, Parra-Rivas P, Gemechu WA, Stepniewski G, Filipkowski A, Buczynski R, et al. Observation of Visible Upconversion Luminescence of Soft Glass Multimode Fibers. Fibers. 2024; 12(2):15. https://doi.org/10.3390/fib12020015
Chicago/Turabian StyleFerraro, Mario, Fabio Mangini, Raffaele Filosa, Vincent Couderc, Yifan Sun, Pedro Parra-Rivas, Wasyhun A. Gemechu, Grzegorz Stepniewski, Adam Filipkowski, Ryszard Buczynski, and et al. 2024. "Observation of Visible Upconversion Luminescence of Soft Glass Multimode Fibers" Fibers 12, no. 2: 15. https://doi.org/10.3390/fib12020015
APA StyleFerraro, M., Mangini, F., Filosa, R., Couderc, V., Sun, Y., Parra-Rivas, P., Gemechu, W. A., Stepniewski, G., Filipkowski, A., Buczynski, R., & Wabnitz, S. (2024). Observation of Visible Upconversion Luminescence of Soft Glass Multimode Fibers. Fibers, 12(2), 15. https://doi.org/10.3390/fib12020015











