Development of Knitted Compression Covers for Amputated Limbs
Abstract
1. Introduction
2. Materials and Methods
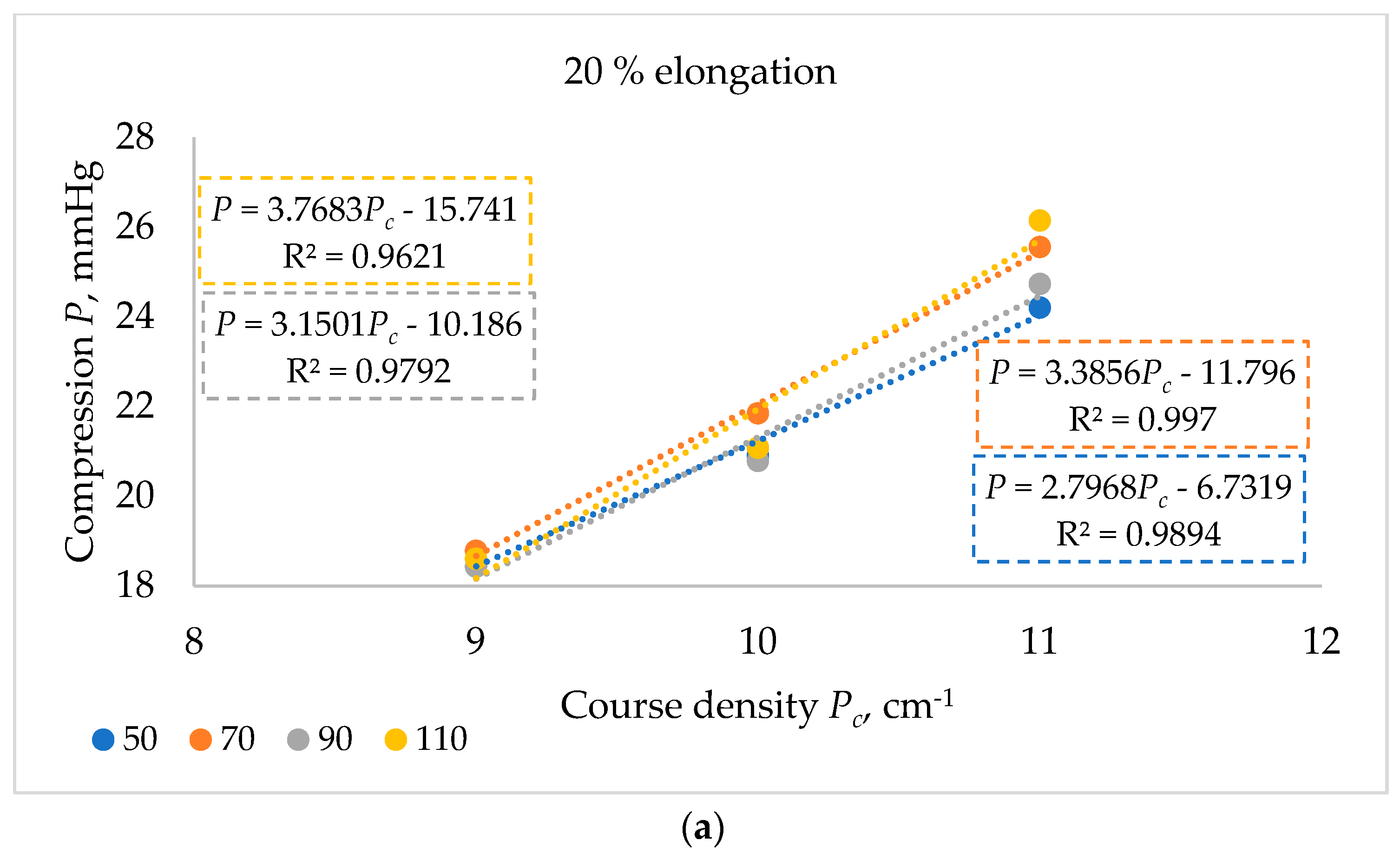
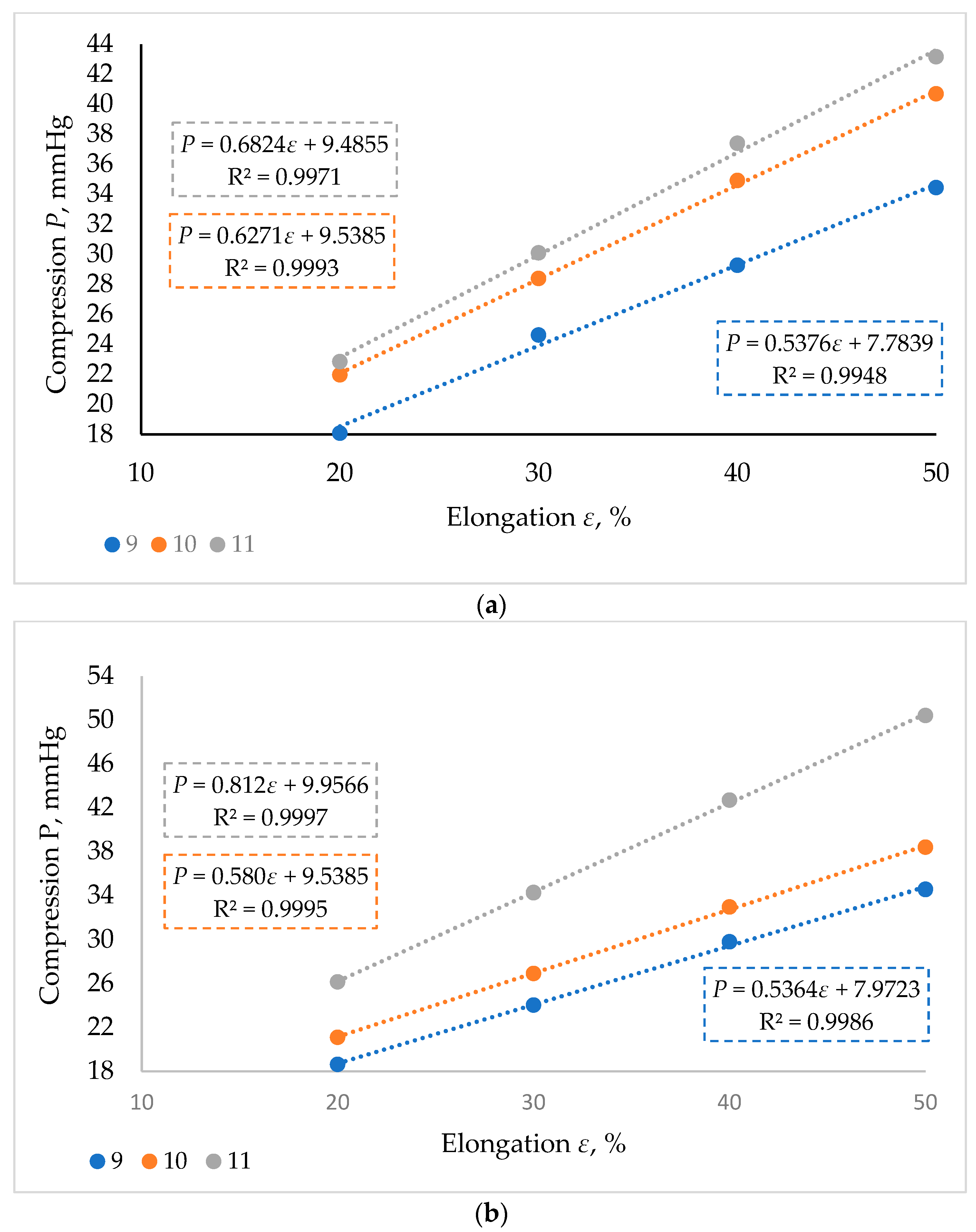
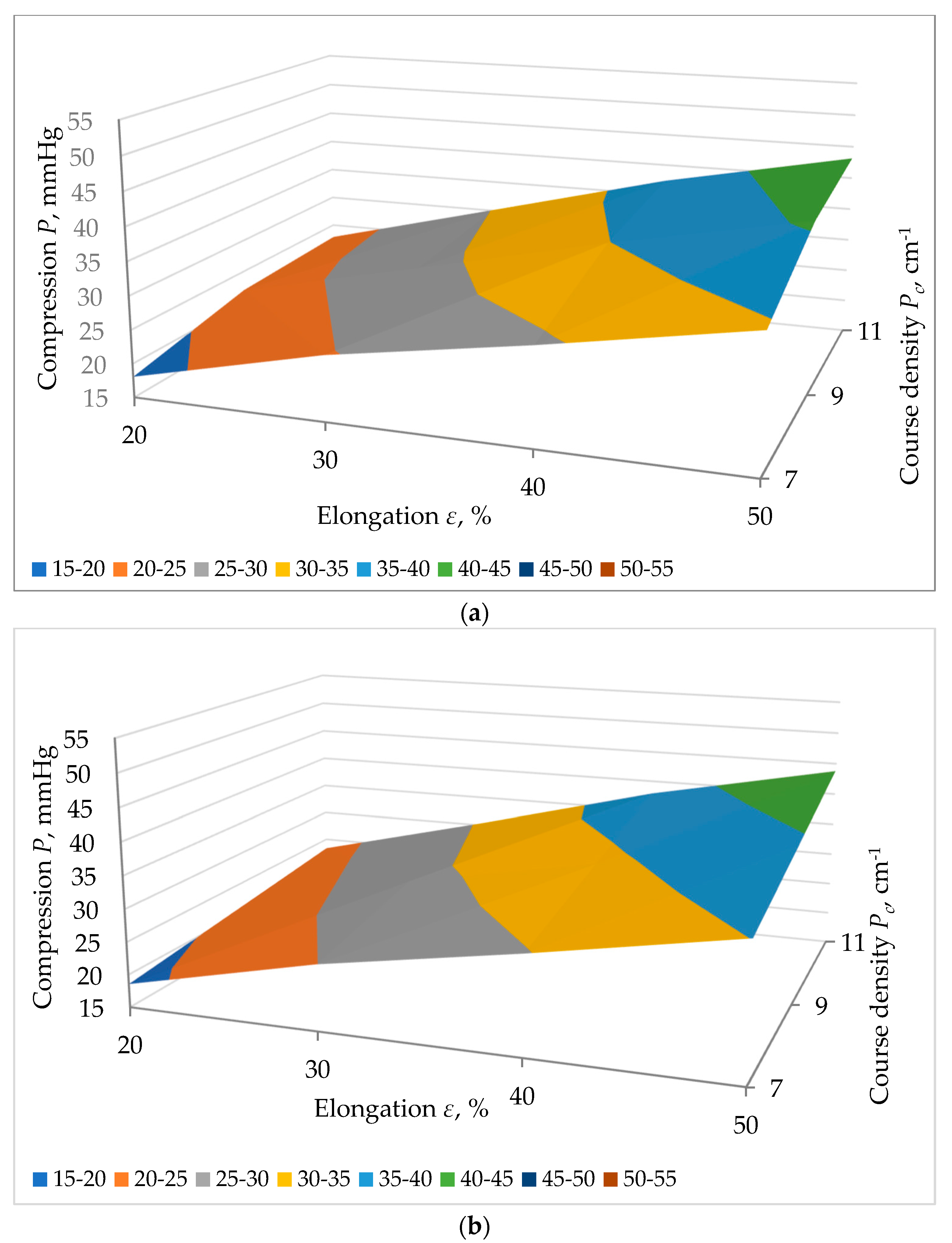
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mikucioniene, D.; Halavska, L.; Melnyk, L.; Milašius, R.; Laureckiene, G.; Arabuli, S. Classification, Structure and Construction of Functional Orthopaedic Compression Knits for Medical Application: A Review. Appl. Sci. 2024, 14, 4486. [Google Scholar] [CrossRef]
- Struszczyk, M.H.; Olejnik, M. Assessment of Prospect of Textile Technologies for Medical Devices. Fibres Text. East. Eur. 2012, 20, 28–36. [Google Scholar]
- Tekin, S.; Kanat, S. The Effects of Sensory Marketing on Clothing-Buying Behavior. Autex Res. J. 2023, 23, 315–322. [Google Scholar] [CrossRef]
- Latanska, I.; Kowalski, K.; Klonowska, M.; Jaworska, A.; Dos, J.; Karbowski, K.; Sujka, W. Biological Properties of Knitted Fabrics Used in Post-Burn Scar Rehabilitation. Autex Res. J. 2022, 22, 343–349. [Google Scholar] [CrossRef]
- Shi, Y.; Liu, R.; Lv, J.; Ye, C. Biomedical therapeutic compression textiles: Physical-mechanical property analysis to precise pressure management. J. Mech. Behav. Biomed. Mater. 2024, 151, 106392. [Google Scholar] [CrossRef] [PubMed]
- Kankariya, N. Material, structure, and design of textile-based compression devices for managing chronic edema. J. Ind. Text. 2022, 52. [Google Scholar] [CrossRef]
- Liu, R.; Guo, X.; Lao, T.T.; Little, T. A critical review on compression textiles for compression therapy: Textile-based compression interventions for chronic venous insufficiency. Text. Res. J. 2017, 87, 1121–1141. [Google Scholar] [CrossRef]
- Alisauskiene, D.; Mikucioniene, D. Influence of the Rigid Element Area on the Compression Properties of Knitted Orthopaedic Supports. Fibres Text. East. Eur. 2012, 20, 103–107. [Google Scholar]
- Tsema, E.V.; Khomenko, I.P.; Bespalenko, A.A.; Buryanov, O.A.; Mishalov, V.G.; Kikh, A.Y. Clinico-Statistical Investigation of the Extremity Amputation Level in Wounded Persons. Klin. Khirurhiia 2017, 10, 324–331. [Google Scholar]
- Melnyk, L.; Halavska, L.; Mikucioniene, D.; Dudnyk, I.; Milasius, R. Assortment and Manufacturing Methods of Stump Socks. In Proceedings of the 11th International Young Researchers Conference Industrial Engineering 2024—From Zero to Hero, Kaunas, Lithuania, 9 May 2024; pp. 129–131. [Google Scholar]
- Murray, J.C. Keloids and hypertrophic scars. Clin. Dermatol. 1994, 12, 27–37. [Google Scholar] [CrossRef] [PubMed]
- Aboalasaad, A.R.; Sirkova, B.K.; Mansoor, T.; Skenderi, Z.; Khalil, A.S. Theoretical and Experimental Evaluation of Thermal Resistance for Compression Bandages. Autex Res. J. 2022, 22, 18–25. [Google Scholar] [CrossRef]
- Zhang, L.; Sun, G.; Li, J.; Chen, Y.; Chen, X.; Gao, W.; Hu, W. The structure and pressure characteristics of graduated compression stockings: Experimental and numerical study. Text. Res. J. 2019, 89, 5218–5225. [Google Scholar] [CrossRef]
- Alisauskiene, D.; Mikucioniene, D. Prediction of Compression of Knitted Orthopaedic Supports by Inlay-Yarn Properties. Mater. Sci. Medzg. 2014, 20, 311–314. [Google Scholar] [CrossRef][Green Version]
- Yu, A.; Sukigara, S.; Takeuchi, S. Effect of inlaid elastic yarns and inlay pattern on physical properties and compression behaviour of weft-knitted spacer fabric. J. Ind. Text. 2022, 51, 2688S–2708S. [Google Scholar] [CrossRef]
- Muraliene, L.; Mikucioniene, D.; Laureckiene, G.; Brazaitis, M. New approach to evaluation of orthopaedic supports compression properties. J. Ind. Text. 2019, 49, 352–364. [Google Scholar] [CrossRef]
- LST EN ISO 13934-1:2013; Textiles—Tensile Properties of Fabrics—Part 1: Determination of Maximum Force and Elongation at Maximum Force Using the Strip Method. ISO: Geneva, Switzerland, 2000.
- LST EN ISO 139:2005; Textiles—Standard Atmospheres for Conditioning and Testing. ISO: Geneva, Switzerland, 2005.
- LST EN ISO 14971:2006; Textiles—Knitted Fabrics—Determination of Number of Stitches per Unit Length and Unit Area. iTeh, Inc.: San Francisco, CA, USA, 2006.
- RAL-GZ 387/1:2008; Medical Compression Hosiery, Quality Assurance. RAL Deutsches Institut für Gütesicherung and Kennzeichnung e.V.: Bonn, Germany, 2008.
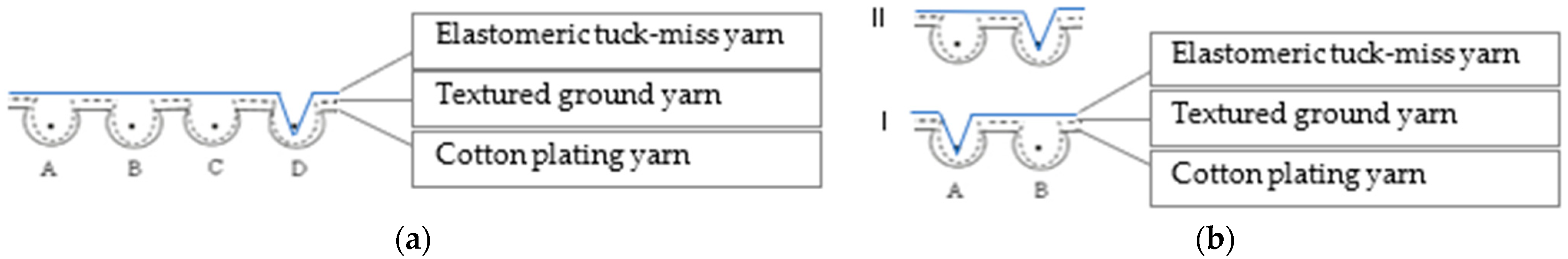

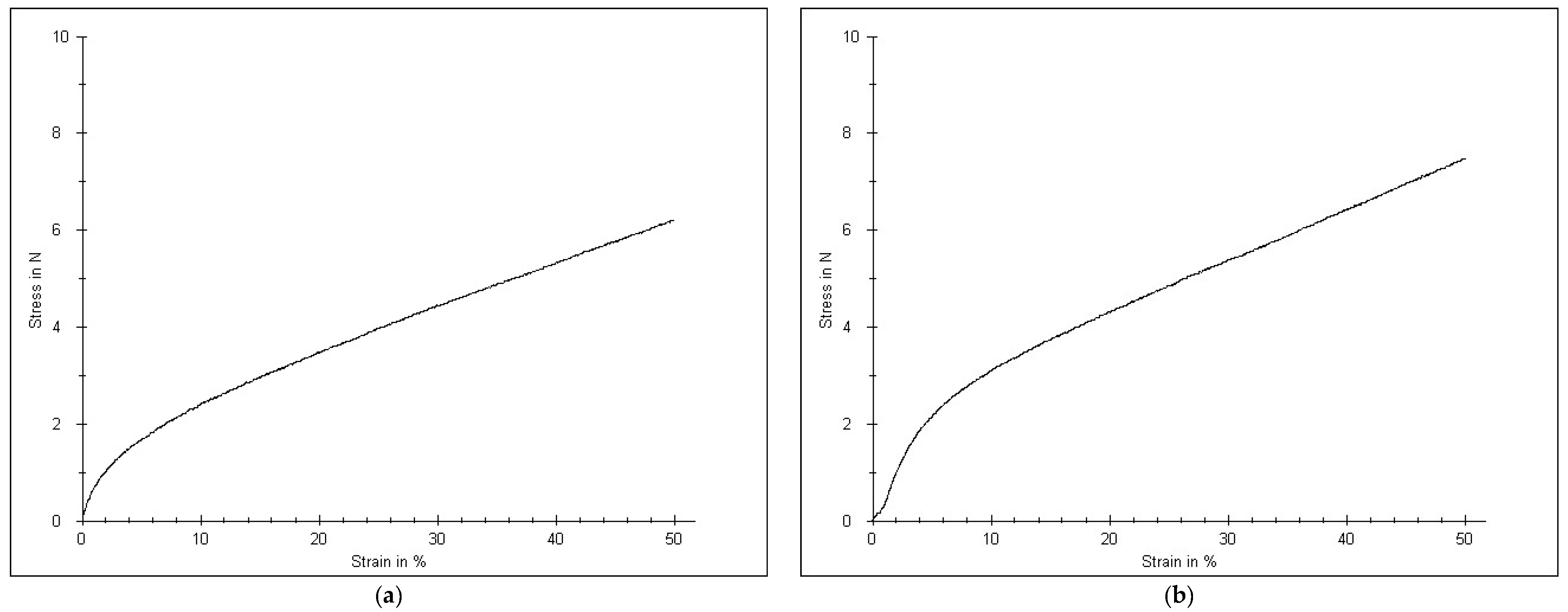
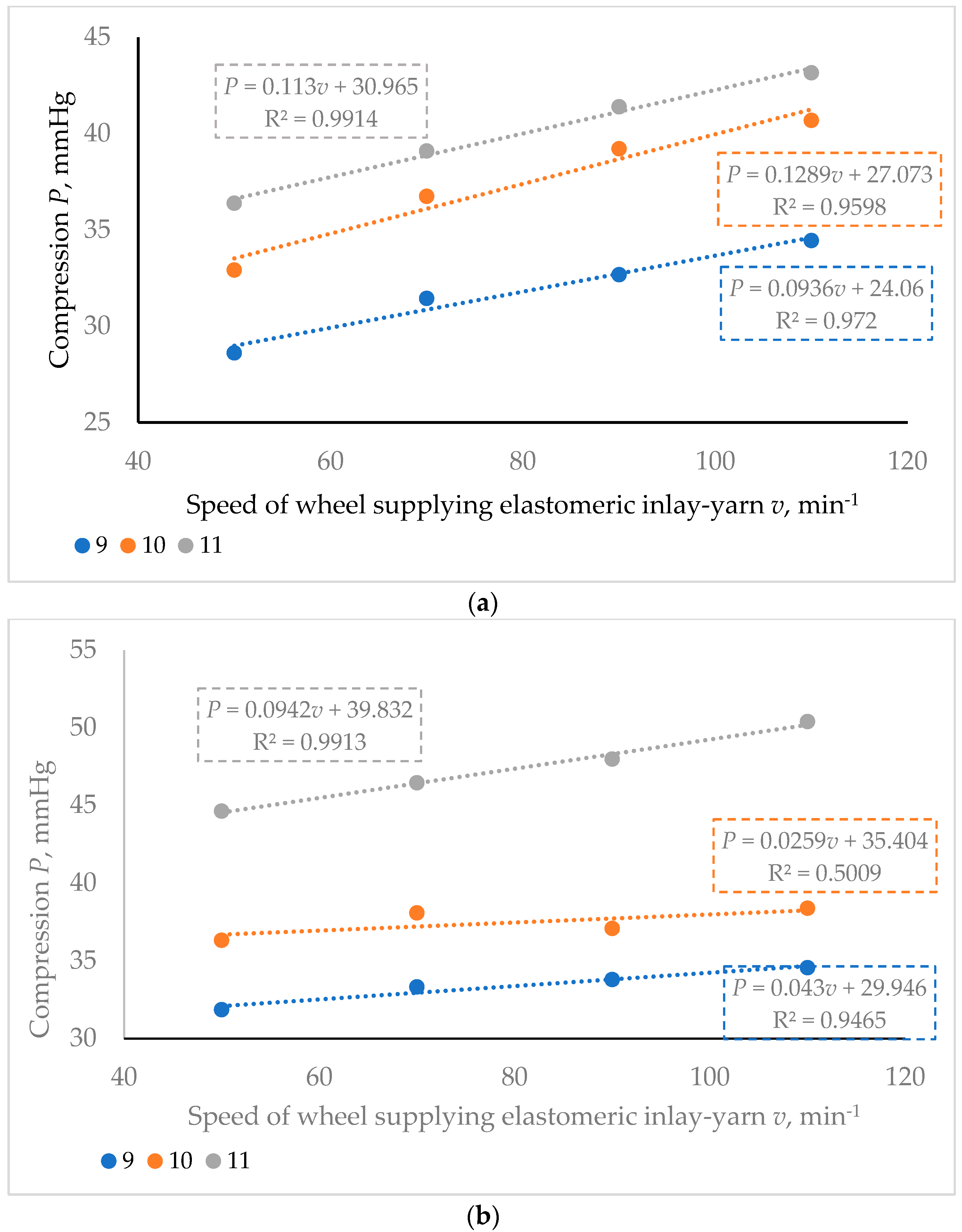

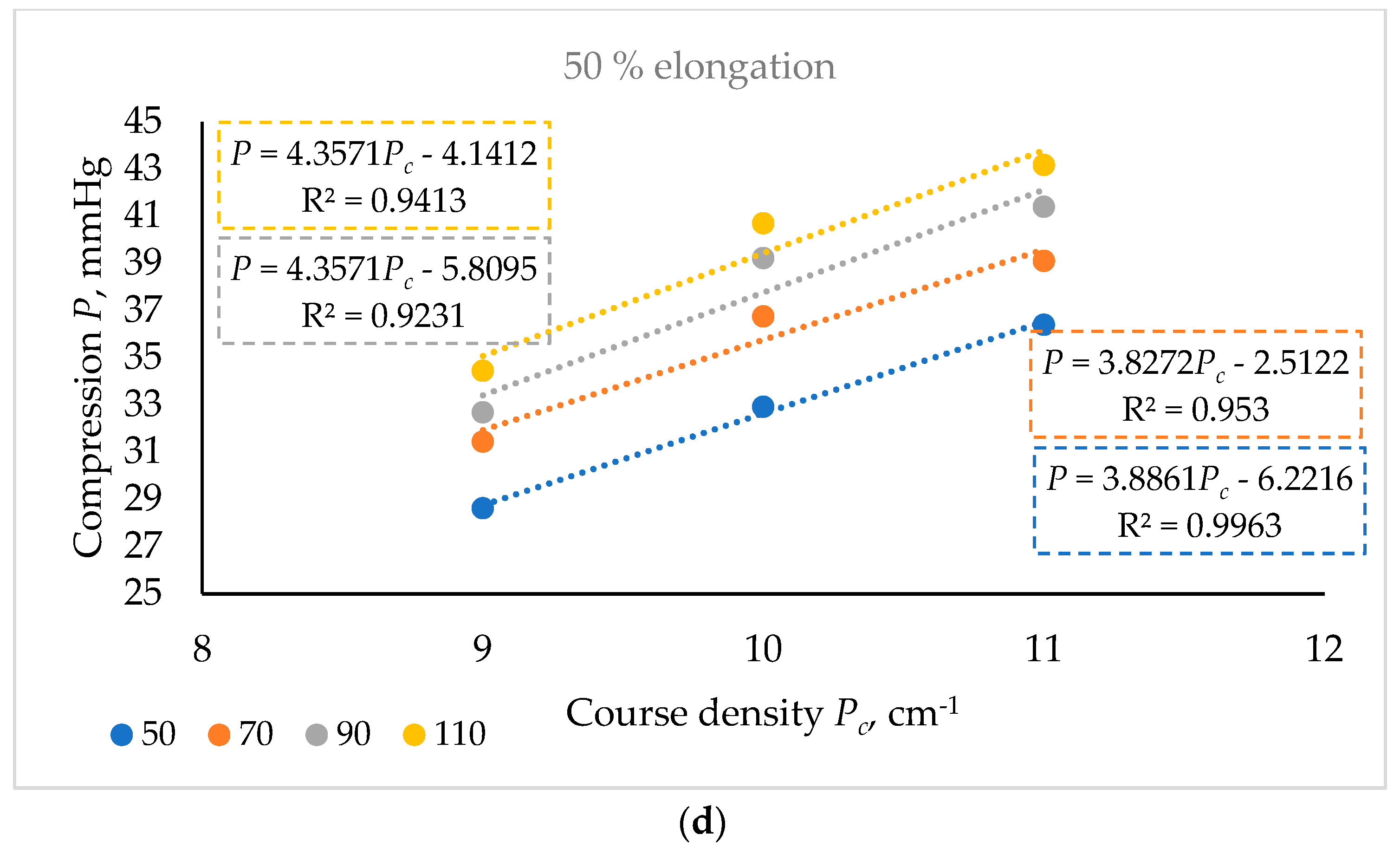


| Sample Code | Speed of Wheel Supplying Elastomeric Inlay-Yarn v, min−1 | Wale Density Pw, cm−1 | Course Density Pc, cm−1 | Area Density, M, g/m² | Loop Length of Plating Cotton Yarn, mm | Loop Length of Textured Elastomeric PA-EL Ground Yarn, mm | Average Length of Textured Elastomeric PA-EL Inlay-Yarn per One Wale, mm |
|---|---|---|---|---|---|---|---|
| 1st group of specimens with the pattern repeat 3 × 1 | |||||||
| 3 × 1/11/50 | 50 | 11 | 11 | 481.2 | 6.1 | 5.5 | 0.9 |
| 3 × 1/11/70 | 70 | 10 | 461.6 | 1.0 | |||
| 3 × 1/11/90 | 90 | 9.5 | 450.4 | 1.1 | |||
| 3 × 1/11/110 | 110 | 9 | 450.0 | 1.2 | |||
| 3 × 1/10/50 | 50 | 11 | 10 | 483.6 | 6.7 | 6.1 | 1.0 |
| 3 × 1/10/70 | 70 | 10 | 480.8 | 1.1 | |||
| 3 × 1/10/90 | 90 | 9.5 | 462.0 | 1.2 | |||
| 3 × 1/10/110 | 110 | 9 | 456.8 | 1.3 | |||
| 3 × 1/9/50 | 50 | 11 | 9 | 482.4 | 7.3 | 6.7 | 0.9 |
| 3 × 1/9/70 | 70 | 10 | 443.6 | 1.0 | |||
| 3 × 1/9/90 | 90 | 9.5 | 428.4 | 1.1 | |||
| 3 × 1/9/110 | 110 | 9 | 417.6 | 1.2 | |||
| 2nd group of specimens with the pattern repeat 1 × 1 | |||||||
| 1 × 1/11/50 | 50 | 11 | 11 | 410.8 | 5.6 | 5.2 | 0.9 |
| 1 × 1/11/70 | 70 | 10 | 410.2 | 1.0 | |||
| 1 × 1/11/90 | 90 | 9 | 409.2 | 1.0 | |||
| 1 × 1/11/110 | 110 | 8.5 | 402.4 | 1.1 | |||
| 1 × 1/10/50 | 50 | 11 | 10 | 414.4 | 6.2 | 5.8 | 0.9 |
| 1 × 1/10/70 | 70 | 10 | 404.0 | 1.0 | |||
| 1 × 1/10/90 | 90 | 9 | 390.8 | 1.1 | |||
| 1 × 1/10/110 | 110 | 8.5 | 397.6 | 1.2 | |||
| 1 × 1/9/50 | 50 | 11 | 9 | 423.2 | 6.8 | 6.4 | 0.8 |
| 1 × 1/9/70 | 70 | 10 | 416.0 | 0.9 | |||
| 1 × 1/9/90 | 90 | 9 | 403.2 | 1.0 | |||
| 1 × 1/9/110 | 110 | 8.5 | 400.8 | 1.1 | |||
| Sample Code | Tensile Force F, N | Compression P, Pa | ||||||
|---|---|---|---|---|---|---|---|---|
| 20% | 30% | 40% | 50% | 20% | 30% | 40% | 50% | |
| 3 × 1/9/50 | 2.79 | 3.60 | 4.40 | 4.86 | 2190.15 | 2826.00 | 3454.00 | 3815.10 |
| 3 × 1/10/50 | 3.05 | 4.00 | 4.65 | 5.59 | 2394.25 | 3140.00 | 3650.25 | 4388.15 |
| 3 × 1/11/50 | 3.36 | 4.30 | 5.20 | 6.18 | 2637.60 | 3375.50 | 4082.00 | 4851.30 |
| 3 × 1/9/70 | 3.03 | 3.84 | 4.58 | 5.34 | 2378.55 | 3014.40 | 3595.30 | 4191.90 |
| 3 × 1/10/70 | 3.38 | 4.30 | 5.29 | 6.24 | 2653.30 | 3375.50 | 4152.65 | 4898.40 |
| 3 × 1/11/70 | 3.59 | 4.63 | 5.72 | 6.64 | 2818.15 | 3634.55 | 4490.20 | 5212.40 |
| 3 × 1/9/90 | 2.93 | 3.85 | 4.76 | 5.55 | 2300.05 | 3022.25 | 3736.60 | 4356.75 |
| 3 × 1/10/90 | 3.48 | 4.73 | 5.69 | 6.66 | 2731.80 | 3713.05 | 4466.65 | 5228.10 |
| 3 × 1/11/90 | 3.73 | 5.00 | 6.04 | 7.03 | 2928.05 | 3925.00 | 4741.40 | 5518.55 |
| 3 × 1/9/110 | 3.07 | 4.18 | 4.97 | 5.85 | 2409.95 | 3281.30 | 3901.45 | 4592.25 |
| 3 × 1/10/110 | 3.73 | 4.82 | 5.93 | 6.91 | 2928.05 | 3783.70 | 4655.05 | 5424.35 |
| 3 × 1/11/110 | 3.88 | 5.11 | 6.35 | 7.33 | 3045.80 | 4011.35 | 4984.75 | 5754.05 |
| 1 × 1/9/50 | 3.16 | 4.03 | 4.66 | 5.41 | 2480.60 | 3163.55 | 3658.10 | 4246.85 |
| 1 × 1/10/50 | 3.55 | 4.52 | 5.50 | 6.17 | 2786.75 | 3548.20 | 4317.50 | 4843.45 |
| 1 × 1/11/50 | 4.11 | 5.58 | 6.56 | 7.58 | 3226.35 | 4380.30 | 5149.60 | 5950.30 |
| 1 × 1/9/70 | 3.19 | 4.09 | 4.89 | 5.66 | 2504.15 | 3210.65 | 3838.65 | 4443.10 |
| 1 × 1/10/70 | 3.71 | 4.66 | 5.68 | 6.47 | 2912.35 | 3658.10 | 4458.80 | 5078.95 |
| 1 × 1/11/70 | 4.34 | 5.53 | 6.82 | 7.89 | 3406.90 | 4341.05 | 5353.70 | 6193.65 |
| 1 × 1/9/90 | 3.13 | 4.00 | 4.96 | 5.74 | 2457.05 | 3140.00 | 3893.60 | 4505.90 |
| 1 × 1/10/90 | 3.53 | 4.50 | 5.38 | 6.30 | 2771.05 | 3532.50 | 4223.30 | 4945.50 |
| 1 × 1/11/90 | 4.20 | 5.55 | 6.78 | 8.15 | 3297.00 | 4356.75 | 5322.30 | 6397.75 |
| 1 × 1/9/110 | 3.16 | 4.08 | 5.06 | 5.87 | 2480.60 | 3202.80 | 3972.10 | 4607.95 |
| 1 × 1/10/110 | 3.58 | 4.57 | 5.60 | 6.52 | 2810.30 | 3587.45 | 4396.00 | 5118.20 |
| 1 × 1/11/110 | 4.44 | 5.82 | 7.25 | 8.56 | 3485.40 | 4568.70 | 5691.25 | 6719.60 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mikucioniene, D.; Halavska, L.; Laureckiene, G.; Melnyk, L.; Arabuli, S.; Milašius, R. Development of Knitted Compression Covers for Amputated Limbs. Fibers 2024, 12, 80. https://doi.org/10.3390/fib12100080
Mikucioniene D, Halavska L, Laureckiene G, Melnyk L, Arabuli S, Milašius R. Development of Knitted Compression Covers for Amputated Limbs. Fibers. 2024; 12(10):80. https://doi.org/10.3390/fib12100080
Chicago/Turabian StyleMikucioniene, Daiva, Liudmyla Halavska, Ginta Laureckiene, Liudmyla Melnyk, Svitlana Arabuli, and Rimvydas Milašius. 2024. "Development of Knitted Compression Covers for Amputated Limbs" Fibers 12, no. 10: 80. https://doi.org/10.3390/fib12100080
APA StyleMikucioniene, D., Halavska, L., Laureckiene, G., Melnyk, L., Arabuli, S., & Milašius, R. (2024). Development of Knitted Compression Covers for Amputated Limbs. Fibers, 12(10), 80. https://doi.org/10.3390/fib12100080










