Evaluation of the Maximum Strain for Different Steel-FRCM Systems in RC Beams Strengthened in Flexure
Abstract
:1. Introduction
2. Material Properties: Steel Fibres, Concrete, and Cementitious Matrix
2.1. Steel Fibres
2.2. Cementitious Matrix
2.3. Concrete
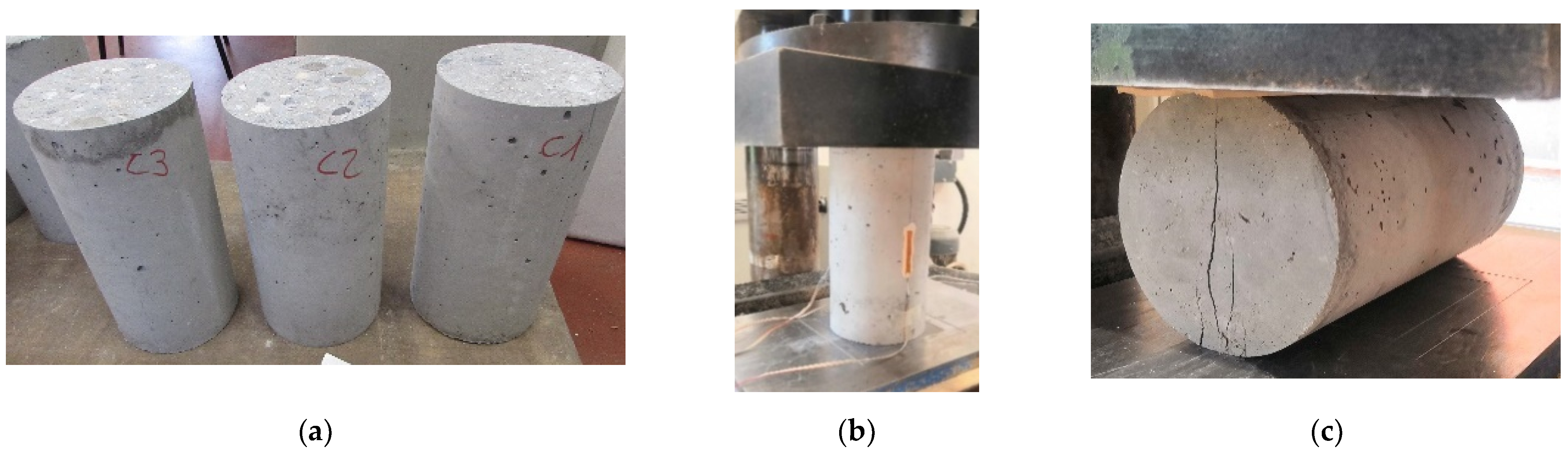
2.4. Direct Tensile Test: Test Set-Up and Results
3. Bond Behaviour
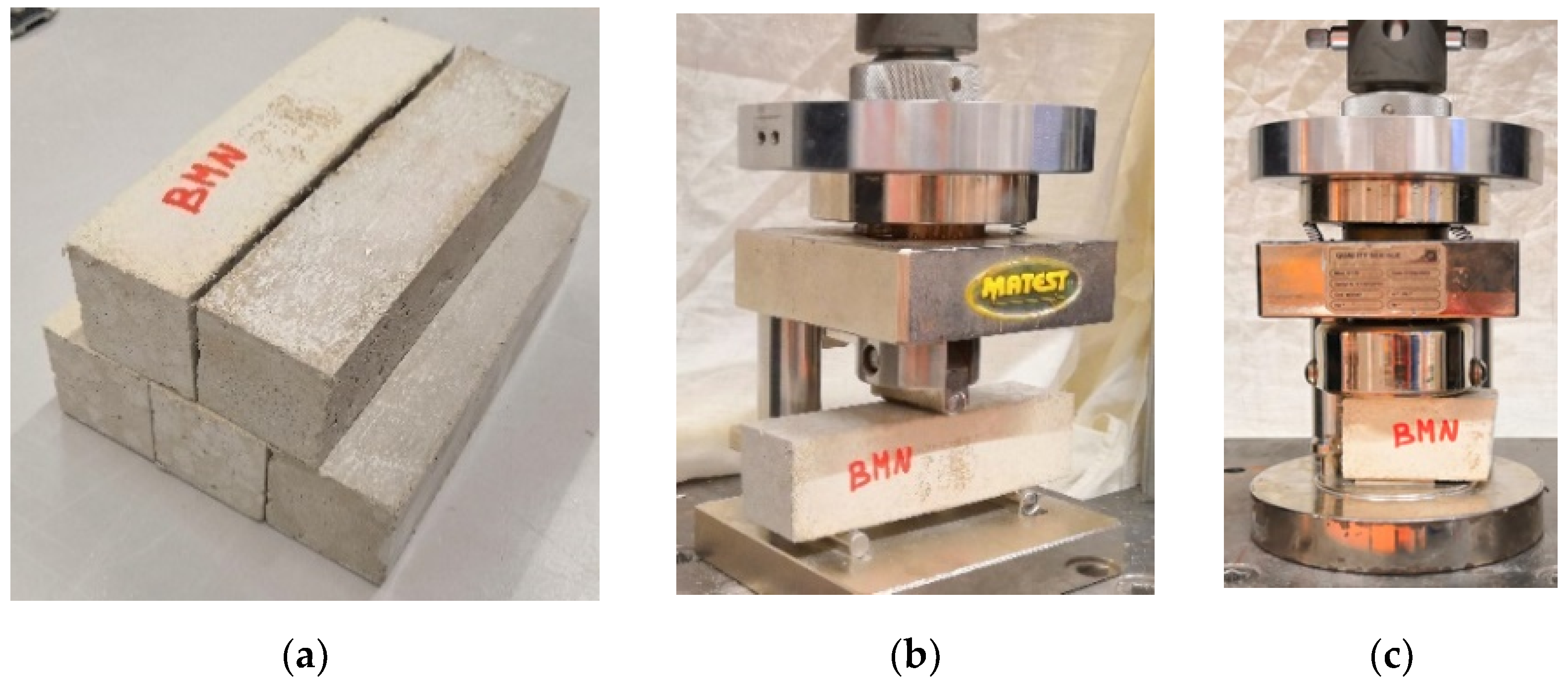
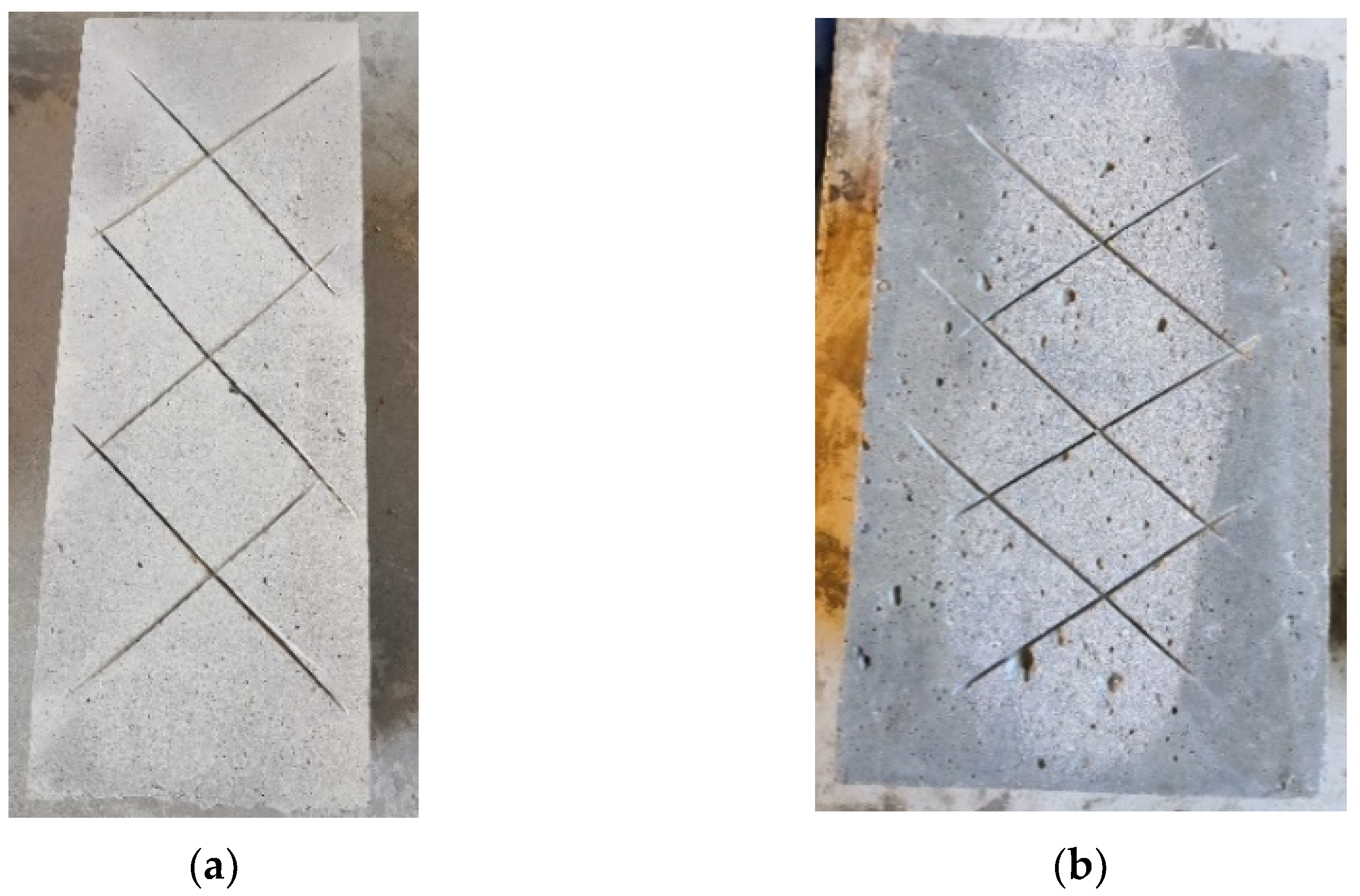
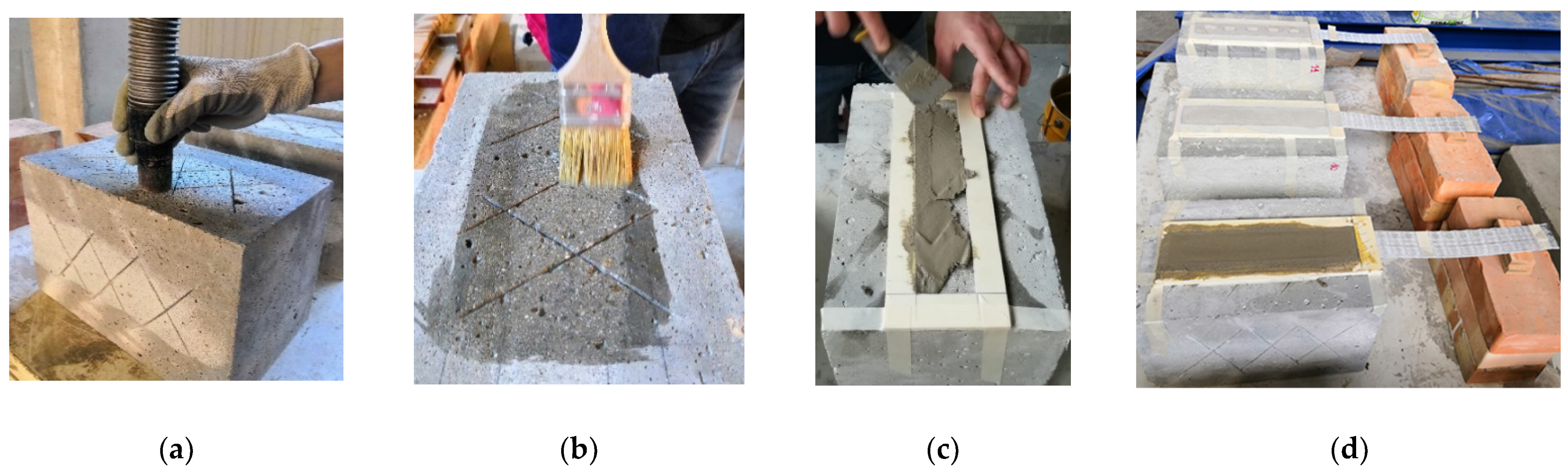
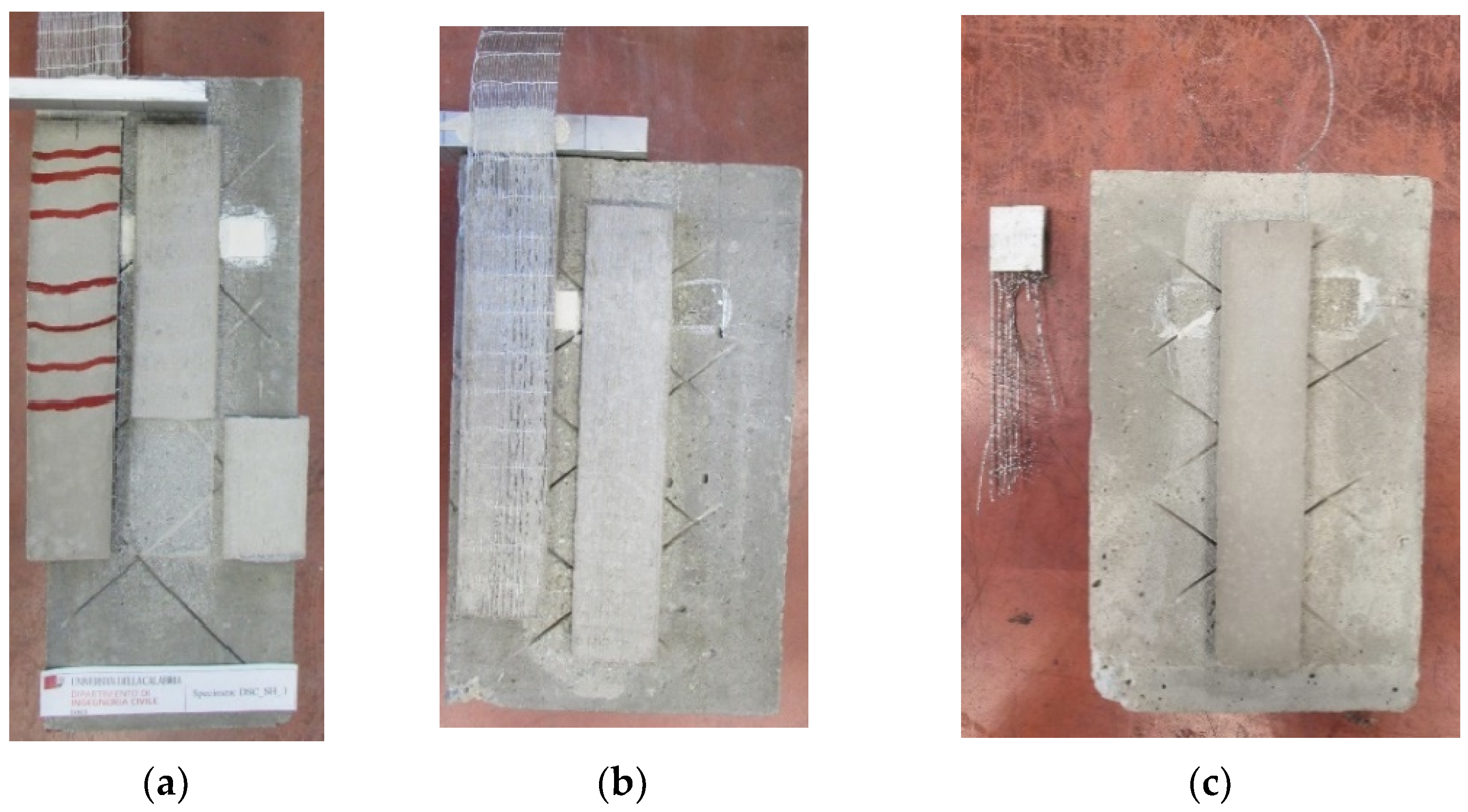
3.1. Direct Single-Lap Shear Test: Preparation and Test Set-Up
3.2. Direct Single-Lap Shear Tests: Results
4. Theoretical Calculations and Predictive Formula for Flexural Design
4.1. End Debonding Strain Value
4.2. Predictive Formula for Intermediate Debonding Strain
5. Theoretical–Experimental Comparison and Analysis
6. Conclusions
- The bond properties of Steel-FRCM composites are strongly affected by the tensile strength and mass per unit area of the steel strips. The broad variability of the components (type of steel and matrices) produces different levels of performance. In particular, with reference to the efficiency factor σmax/ff, the best bond performance is obtained using low-density steel fibre (GLV). The efficiency factors are 34.18% and 93.73% for SS and GLV, respectively.
- Different failure modes were observed from the experimental results. The debonding mainly occurs at the matrix–fibre interface (mode C) and between surface and Steel-FRCM composite (mode B), confirming the non-involvement of the concrete substrates. The failure mode of the galvanized fibre (GLV) is particularly positive, with the failure of the steel fibre outside the composite strip (without sliding).
- The procedure reported in the CNR document provides information that may be calibrated in relation to the several materials available for the strengthening of structural elements. It seems reductive to indicate a single coefficient for all the types of FRCM reinforcement.
- Specifically, high-density steel fibre reinforcement requires a transition coefficient around 2. The coefficient of 1.5 gives satisfactory results for low-density fibre. When the failure occurs due to the rupture of the reinforcement, as in the case of the GLV steel strip, the debonding phenomenon does not occur and the transition coefficient is equal to 1.
- The predictive formulas provide an accurate prediction of the strain corresponding to debonding also through the calibration of a suitable safety coefficient. They can be transferred to engineers for design practice applications, owing to their simplicity.
- The theoretical calculations were based on a database of experimental results collected from the scientific literature in order to investigate the intermediate debonding strain in the flexural strengthening applications. Based on the results, it is apparent that both procedures can be successfully used in the flexural strengthening design process, but specific suggestion should be investigated. High-mass density fibres show higher error ratios in predicting intermediate strains.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Papanicolaou, C.G.; Triantafillou, T.C.; Karlos, K.; Papathanasiou, M. Textile reinforced mortar (TRM) versus FRP as strengthening material of URM walls: In plane cyclic loading. Mater. Struct. 2007, 40, 1081–1097. [Google Scholar] [CrossRef]
- Fuseini, M.; Yousry Zaghloul, M.M.; Elkady, M.F.; El-Shazly, A.H. Evaluation of synthesized polyaniline nanofibres as corrosion protection film coating on copper substrate by electrophoretic deposition. J. Mater. Sci. 2022, 57, 6085–6101. [Google Scholar] [CrossRef]
- Yousry Zaghloul, M.M.; Yousry Zaghloul, M.M.; Yousry Zaghloul, M.M. Developments in polyester composite materials—An in-depth review on natural fibres and nano fillers. Compos. Struct. 2021, 278, 114698. [Google Scholar] [CrossRef]
- Yousry Zaghloul, M.M.; Yousry Zaghloul, M.M. Influence of flame retardant magnesium hydroxide on the mechanical properties of high density polyethylene composites. J. Reinf. Plast. Compos. 2017, 36, 1802–1816. [Google Scholar] [CrossRef]
- Ascione, G.L.; de Felice, S.; De Santis, A. qualification method for externally bonded Fibre Reinforced Cementitious Matrix (FRCM) strengthening systems. Compos. B Eng. 2015, 78, 497–506. [Google Scholar] [CrossRef]
- AC434; Proposed Acceptance Criteria for Masonry and Concrete Strengthening Using Fiber-Reinforced Cementitious Matrix (FRCM) Composite Systems. ICC-Evaluation Service: Whittier, CA, USA, 2013.
- De Santis, S.; de Felice, G. Tensile behaviour of mortar-based composites for externally bonded reinforcement systems. Compos. B Eng. 2015, 68, 401–413. [Google Scholar] [CrossRef]
- De Santis, S.; de Felice, G. Tensile behaviour and durability of mortar-based strengthening systems with glass-aramid textile. J. Key Eng. Mater. 2015, 624, 346–353. [Google Scholar] [CrossRef]
- Ombres, L.; Verre, S. Experimental and Numerical Investigation on the Steel Reinforced Grout (SRG) Composite-to-Concrete Bond. J. Compos. Sci. 2020, 4, 182. [Google Scholar] [CrossRef]
- Bencardino, F.; Nisticò, M.; Verre, S. Fibers, Experimental Investigation and Numerical Analysis of Bond Behavior in SRG-Strengthened Masonry Prisms Using UHTSS and Stainless-Steel. Fibers 2020, 8, 8. [Google Scholar] [CrossRef] [Green Version]
- Younis, A.; Ebead, U. Bond characteristics of different FRCM systems. Constr. Build. Mater. 2018, 175, 610–620. [Google Scholar] [CrossRef]
- Hadad, H.A.; Erickson, B.; Nanni, A. Flexural analysis and design of FRCM-strengthened RC beams. Constr. Build. Mater. 2020, 244, 118371. [Google Scholar] [CrossRef]
- Marcinczak, D.; Trapko, T.; Musiał, M. Shear strengthening of reinforced concrete beams with PBO-FRCM composites with anchorage. Compos. B Eng. 2019, 158, 149–161. [Google Scholar] [CrossRef]
- Feng, R.; Liu, Y.; Zhu, J.-H.; Xing, F. Flexural behaviour of C-FRCM strengthened corroded RC continuous beams. Compos. Struct. 2020, 245, 112200. [Google Scholar] [CrossRef]
- Loreto, G.; Babaeidarabad, S.; Leardini, L.; Nanni, A. RC beams shear-strengthened with fabric-reinforced-cementitious-matrix (FRCM) composite. Inter. J. Adv. Struct. Eng. (IJASE) 2015, 7, 341–352. [Google Scholar] [CrossRef] [Green Version]
- Salsavilca, J.; Yacila, J.; Tarque, N.; Camata, G. Experimental and analytical bond behaviour of masonry strengthened with steel reinforced grout (SRG). Constr. Build. Mater. 2020, 238, 117635. [Google Scholar] [CrossRef]
- Larrinaga, P.; Garmendia, L.; Piñero, I.; San-José, J.T. Flexural strengthening of low-grade reinforced concrete beams with compatible composite material: Steel Reinforced Grout (SRG). Constr. Build. Mater. 2020, 235, 117790. [Google Scholar] [CrossRef]
- Yacila, J.; Salsavilca, J.; Tarque, N.; Camata, G. Experimental assessment of confined masonry walls retrofitted with SRG under lateral cyclic loads. Eng. Struct. 2019, 199, 109555. [Google Scholar] [CrossRef]
- Wakjira, T.G.; Ebead, U. Experimental and analytical study on strengthening of reinforced concrete T-beams in shear using steel reinforced grout (SRG). Compos. B Eng. 2019, 177, 107368. [Google Scholar] [CrossRef]
- Papanikolaou, G.E.T.V.K.; Lioupis, C.; Hajirasouliha, I. Steel-Reinforced Grout (SRG) strengthening of shear-critical RC beams. Constr. Build. Mater. 2019, 216, 68–83. [Google Scholar]
- Napoli, A.; Realfonzo, R. Reinforced concrete beams strengthened with SRP/SRG systems: Experimental investigation. Constr. Build. Mater. 2015, 93, 654–677. [Google Scholar] [CrossRef]
- Aiello, M.A.; Bencardino, F.; Cascardi, A.; Realfonzo, R.; Verre, S. Masonry columns confined with fabric reinforced cementitious matrix (FRCM) systems: A round robin test. Constr. Build. Mater. 2021, 298, 123816. [Google Scholar] [CrossRef]
- ACI 549.4R-20; Guide to Design and Construction of Externally Bonded Fabric-Reinforced and Steel-Reinforced Grout Systems for Repair and Strengthening of Concrete Structures. American Concrete Institute: Farmington Hills, MI, USA, 2013.
- National Research Council (CNR). Guide for the Design and Construction of Externally Bonded Fibre Reinforced Inorganic Matrix Systems for Strengthening Existing Structures; CNR-DT 215/2018 (updated version 30 June 2020); CNR: Rome, Italy, 2020; Available online: https://www.cnr.it/en/node/12827 (accessed on 17 June 2022).
- Bencardino, F.; Carloni, C.; Condello, A.; Focacci, F.; Napoli, A.; Realfonzo, R. Flexural behaviour of RC members strengthened with FRCM: State-of-the-art and predictive formulas. Compos. B Eng. 2018, 148, 132–148. [Google Scholar] [CrossRef]
- ST3-0221. Kimia S.p.A. Available online: www.kimia.it (accessed on 10 January 2020).
- ST7-0221. Kimia S.p.A. Available online: www.kimia.it (accessed on 10 January 2020).
- ST6-0319. Kimia S.p.A. Available online: www.kimia.it (accessed on 10 January 2020).
- EN 1504-3:2005; Products and Systems for the Protection and Repair of Concrete Structures—Definitions, Requirements, Quality Control and Evaluation of Conformity—Part 3: Structural and non-Structural Repair. 2005. Available online: https://standards.iteh.ai/catalog/standards/cen/901c7ca2-4f42-4478-a8e1-7e56ee6a8475/pren-1504-3-rev (accessed on 17 June 2022).
- EN 1504-4:2004; Products and Systems for the Protection and Repair of Concrete Structures—Definitions, Requirements, Quality Control and Evaluation of Conformity—Part 4: Structural Bonding. Available online: https://standards.iteh.ai/catalog/standards/cen/ab0b5079-46c0-49ed-80ee-48254718963f/en-1504-4-2004 (accessed on 17 June 2022).
- EN 12190:1998; Product and System for the Protection and Repair Concrete Structures—Test Methods—Determination of Compressive Strength of Repair Mortar. Available online: https://standards.iteh.ai/catalog/standards/cen/4191a705-4001-4412-ae57-f21e7994bb79/en-12190-1998 (accessed on 17 June 2022).
- EN 1015-11:2019; Methods of Test for Masonry Units—Part 11: Of flexural and Compressive Strength of Hardened Mortar. Available online: https://standards.iteh.ai/catalog/standards/cen/14596d4c-119b-4a78-94e1-3fe481a29bde/en-1015-11-2019 (accessed on 17 June 2022).
- D’Antino, T.; Colombi, P.; Carloni, C.; Sneed, L.H. Estimation of a matrix-fiber interface cohesive material law in FRCM-concrete joints. Compos. Struct. 2018, 193, 103–112. [Google Scholar] [CrossRef]
- Razavizadeh, A.; Ghiassi, B.; Oliveira, D.V. Bond behavior of SRG-strengthened masonry units: Testing and numerical modeling. Constr. Build. Mater. 2014, 64, 387–397. [Google Scholar] [CrossRef]
- Bellini, A.; Aiello, M.A.; Bencardino, F.; Bello, C.B.D.C.; Castori, G.; Cecchi, A.; Ceroni, F.; Corradi, M.; D’Antino, T.; De Santis, S.; et al. Influence of different set-up parameters on the bond behavior of FRCM composites. Constr. Build. Mater. 2021, 308, 124964. [Google Scholar] [CrossRef]
- Cheng, J.F.; Teng, J.G. Anchorage strength models for FRP and steel plates bonded to concrete. J. Struct. Eng. ASCE 2001, 127, 784–791. [Google Scholar] [CrossRef]
- Mazzotti, C.; Savoia, M.; Ferracuti, B. A new single-shear set-up for stable debonding of FRP-concrete joints. Constr. Build. Mater. 2009, 23, 1529–1537. [Google Scholar] [CrossRef]
- De Felice, G.; Aiello, M.A.; Caggegi, C.; Ceroni, F.; de Santis, S.; Garbin, E.; Gattesco, N.; Hojdys, Ł.; Krajewski, P.; Kwiecien, A.; et al. Recommendation of RILEM TC 250-CSM: Test method for Textile Reinforced Mortar to substrate bond characterization. Mater. Struct. 2018, 51, 95. [Google Scholar] [CrossRef]
- EN 1990:2002; Eurocode 0: Basis of Structural Design. CEN—European Committee for Standardization: Brussels, Belgium, 2002. Available online: https://standards.iteh.ai/catalog/standards/cen/be4b05f7-4c56-4e2e-a88d-56fce873fcbc/en-1990-20022002 (accessed on 17 June 2022).
- Bencardino, F.; Nisticò, M. Predictive Strain Debonding in RC Beams Externally Strengthened with S-FRCM. In Durability and Sustainability of Concrete Structures (DSCS-2018). In Proceedings of the 2nd International Workshop, Moscow, Russia, 6–7 June 2018. [Google Scholar]
- Bencardino, F.; Condello, A. Innovative solution to retrofit RC members: Inhibiting-Repairing-Strengthening (IRS). Constr. Build. Mater. 2016, 117, 171–181. [Google Scholar] [CrossRef]
- Barton, B.; Wobbe, E.; Dharani, L.R.; Silva, P.; Birman, V.; Nanni, A.; Alkhrdaji, T.; Thomas, J.; Tunis, G. Characterization of reinforced concrete beams strengthened by steel reinforced polymer and grout (SRP and SRG) composites. Mater. Sci. Eng. A 2005, 412, 129–136. [Google Scholar] [CrossRef]
- Ombres, L.; Verre, S. Flexural Strengthening of RC Beams with Steel-Reinforced Grout: Experimental and Numerical Investigation. J. Compos. Constr. ASCE 2019, 23, 04019035. [Google Scholar] [CrossRef]
- Bencardino, F.; Condello, A.; Ashour, A.F. Single-lap shear bond tests on Steel Reinforced Geopolymeric Matrix-concrete joints. Compos. B Eng. 2017, 110, 62–71. [Google Scholar] [CrossRef] [Green Version]
- Matana, M.; Nanni, A.; Dharani, L.; Silva, P.; Tunis, G. Bond performance of steel reinforced polymer and steel reinforced grout. In Proceedings of the International Symposium on Bond Behaviour of FRP in Structures (BBFS 2005), Hong Kong, China, 7–9 December 2005. [Google Scholar]
- Realfonzo, R.; Napoli, A.; Ascione, F.; Lamberti, M. Bond behavior of SRP/SRG systems on concrete substrate: Experimental investigations. In Proceedings of the 4th Workshop on the New Boundaries of Structural Concrete, Anacapri, Italy, 29 September–1 October 2016. [Google Scholar]
- Ascione, F.; Lamberti, M.; Napoli, A.; Realfonzo, R. Experimental bond behavior of Steel Reinforced Grout systems for strengthening concrete elements. Constr. Build. Mater. 2020, 232, 117105. [Google Scholar] [CrossRef]






| Description | Label | Af,str (mm2) | nstr/50 mm | Af (mm2) | tf (mm) | ρ (g/m2) |
|---|---|---|---|---|---|---|
| Stainless Steel | SS | 0.470 | 25 | 11.75 | 0.235 | 2200 |
| Galvanized High Strength Steel | GLV | 0.519 | 8 | 4.15 | 0.083 | 650 |
| Property | Mean Value (N/mm2) | |
|---|---|---|
| Cementitious Matrix | Concrete | |
| Compressive Strength fm, fcm | 43.67 | 33.89 |
| Tensile Strength ft, fctm | 5.77 | 2.87 |
| Specimen | Fmax (N) | ff (N/mm2) |
|---|---|---|
| DT_SS_1 | 16,618.75 | 1414.36 |
| DT_SS_2 | 15,664.90 | 1333.18 |
| DT_SS_3 | 18,142.54 | 1544.05 |
| Average (C.o.V.) | 16,808.73 (0.074) | 1430.53 (0.074) |
| DT_GLV_1 | 8507.78 | 2050.07 |
| DT_GLV_2 | 8318.67 | 2004.50 |
| DT_GLV_3 | 7924.88 | 1909.61 |
| Average (C.o.V.) | 8250.44 (0.036) | 1988.06 (0.036) |
| Specimen ID | bc (mm) | pc (mm) | lc (mm) | bf (mm) |
|---|---|---|---|---|
| 1 to 4 | 150 | 150 | 400 | 50 |
| 5 to 9 | 200 | 200 | 320 |
| Specimen | Pmax (kN) | gmax (mm) | gu (mm) | σmax (MPa) | σmax/ff (%) | Failure Mode |
|---|---|---|---|---|---|---|
| DSC_SS_1 | 5.36 | 0.34 | 0.57 | 456.17 | 31.89 | B-C |
| DSC_SS_2 | 5.71 | 0.59 | 0.65 | 485.96 | 33.97 | B |
| DSC_SS_3 | 5.24 | 0.57 | 0.65 | 445.96 | 31.17 | B-C |
| DSC_SS_4 | 5.98 | 0.79 | 0.81 | 508.94 | 35.58 | B-C |
| DSC_SS_5 | 6.70 | 0.66 | 0.85 | 570.21 | 39.86 | B-C |
| DSC_SS_6 | 5.16 | 0.61 | 0.64 | 439.15 | 30.70 | B-C |
| DSC_SS_7 | 5.93 | 0.54 | 0.77 | 504.68 | 35.28 | B-C |
| DSC_SS_8 | 5.65 | 0.65 | 0.77 | 480.85 | 33.61 | B |
| DSC_SS_9 | 5.97 | 0.68 | 0.68 | 508.09 | 35.52 | B |
| Average (C.o.V.) | 5.74 (0.083) | 0.60 (0.204) | 0.71 (0.131) | 488.89 (0.083) | 34.18 (0.083) | |
| DSC_GLV_1 | 6.99 | 0.94 | 0.94 | 1684.34 | 85.34 | E |
| DSC_GLV_2 | 7.67 | 1.06 | 1.06 | 1848.19 | 93.65 | E |
| DSC_GLV_3 | 7.44 | 1.63 | 1.63 | 1792.77 | 90.84 | E |
| DSC_GLV_4 | 7.62 | 0.80 | 0.80 | 1836.08 | 93.03 | E |
| DSC_GLV_5 | 8.19 | 1.05 | 1.05 | 1973.53 | 100.00 | E |
| DSC_GLV_6 | 8.58 | 0.88 | 0.88 | 2067.82 | 104.77 | E |
| DSC_GLV_7 | 7.02 | 0.89 | 0.89 | 1691.74 | 85.72 | E |
| DSC_GLV_8 | 7.80 | 1.02 | 1.02 | 1880.57 | 95.29 | E |
| DSC_GLV_9 | 7.78 | 1.12 | 1.12 | 1874.37 | 94.97 | E |
| Average (C.o.V.) | 7.47 (0.068) | 0.93 (0.260) | 0.93 (0.260) | 1849.93 (0.066) | 93.73 (0.066) |
| System | N° of Specimens | bc (mm) | σlim,conv,m (MPa) | εlim,conv,m | sx | C.o.V. (%) | kn | εlim,conv,k | ε(α)lim,conv,k | αreal |
|---|---|---|---|---|---|---|---|---|---|---|
| SS | 4 | 150 | 474.26 | 0.00274 | 0.00019 | 7.02 | 2.63 | 0.00223 | 0.00335 | 2.16 |
| 5 | 200 | 500.60 | 0.00292 | 0.00032 | 11.03 | 2.33 | 0.00217 | 0.00325 | 2.03 | |
| 9 | 150/200 | 488.89 | 0.00284 | 0.00027 | 9.60 | 1.96 | 0.00230 | 0.00346 | 2.09 | |
| GLV | 3 | 150 | 1775.10 | 0.0117 | 0.00129 | 10.98 | 3.37 | 0.00738 | 0.0111 | 1.31 |
| 6 | 200 | 1887.35 | 0.0135 | 0.00183 | 13.50 | 2.18 | 0.00954 | 0.0143 | 1.13 | |
| 9 | 150/200 | 1849.93 | 0.0129 | 0.00182 | 14.08 | 1.96 | 0.00936 | 0.0140 | 1.18 |
| Strengthening System | εf,deb |
|---|---|
| SS | 0.00821 |
| GLV | 0.0141 |
| Experimental Work | ID | bc (mm) H (mm) h (mm) | l (mm) a (mm) bf (mm) | fcm (N/mm2) fym (N/mm2) ρf | As (mm2) A’s (mm2) | Reinforcement | εf,max |
|---|---|---|---|---|---|---|---|
| Barton et al. [42] | SRG-2 | 203 305 2438 | 2134 711.50 152 | 36.50 436.00 | 396.90 141.70 | Hardwire 3SX | 0.0065 |
| Napoli and Realfonzo [21] | SRG-1LD | 400 200 167 | 3400 1220 200 | 15.14 460.00 0.000515/0.00152 | 392.50 157.0 | Geosteel G600 | 0.0149 |
| SRG-2LD | Geosteel G600 | 0.0107 | |||||
| SRG-1MD | Geosteel G2000 | 0.0080 | |||||
| SRG-1MD-A | Geosteel G2000 | 0.0085 | |||||
| SRG-1MD-B | Geosteel G2000 | 0.0070 | |||||
| SRG-2MD | Geosteel G2000 | 0.0075 | |||||
| Bencardino and Condello [41] | A-EB | 150 250/400 365 | 3000/4500 900/1500 100/150 | 16.70 367.1 0.000657 | 401.92 100.50 | Kimisteel INOX | 0.00587 |
| B-EB | 0.00599 | ||||||
| Ombres and Verre [43] | B-1L | 140 300 269 | 4500 1600 70 | 19.4 474 0.00028 | 113.04 28.26 | Geosteel G1200 | 0.0074 |
| Experimental Work | Specimen | Fmax (kN) | σlim,conv (MPa) | εlim,conv | ε(α)lim,conv | Reinforcement Fibre |
|---|---|---|---|---|---|---|
| Matana et al. [45] | G-12-1 | 28.48 | 727.62 | 0.00364 | 0.00546 | Hardwire 3SX |
| G-12-2 | 25.37 | 648.04 | 0.00324 | 0.00486 | ||
| G-12-3 | 21.81 | 557.09 | 0.00279 | 0.00418 | ||
| Realfonzo et al. [46] | 30 LD-2 | 14.30 | 1692.98 | 0.00870 | 0.01304 | Geosteel G600 |
| 30 LD-3 | 15.73 | 1858.74 | 0.00955 | 0.01432 | ||
| 30 MD-1 | 16.64 | 655.28 | 0.00337 | 0.00505 | Geosteel G2000 | |
| 30 MD-2 | 16.01 | 630.47 | 0.00324 | 0.00486 | ||
| 30 MD-3 | 17.66 | 695.45 | 0.00357 | 0.00536 | ||
| Bencardino and Condello [44] | 200 S | 7.24 | 603.33 | 0.00319 | 0.00479 | Kimisteel INOX |
| 250 S | 7.20 | 600.00 | 0.00317 | 0.00476 | ||
| 300 | 6.17 | 514.17 | 0.00272 | 0.00408 | ||
| 400 S | 7.90 | 658.33 | 0.00348 | 0.00522 | ||
| Ascione et al. [47] | 30LM-10-1-1 | 16.43 | 972.19 | 0.00499 | 0.00749 | Geosteel G1200 |
| 30LM-10-1-2 | 17.80 | 1053.25 | 0.00541 | 0.00811 | ||
| 30LM-10-1-3 | 18.25 | 1079.88 | 0.00555 | 0.00832 |
| Steel Textile | Ef1 (MPa) | Ef2 (MPa) | Efm (MPa) | tf (mm) | ffu (MPa) | εfu | Cord Area (mm2) | Spacing (Cord/cm) | Density |
|---|---|---|---|---|---|---|---|---|---|
| Hardwire 3SX | 200,000 | 200,000 | 200,000 | 0.382 | 1701.83 | 0.0168 | 0.813 | 4.70 | M |
| Geosteel G600 | 206,000 | 183,400 | 194,700 | 0.086 | 3191.00 | 0.0219 | 0.538 | 1.57 | L |
| Geosteel G1200 | 206,000 | 183,400 | 194,700 | 0.169 | 3138.30 | 0.0214 | 0.538 | 3.14 | L-M |
| Geosteel G2000 | 206,000 | 183,400 | 194,700 | 0.254 | 3085.70 | 0.0209 | 0.538 | 4.72 | H |
| Kimisteel INOX | 189,000 | 189,000 | 189,000 | 0.240 | 1400.73 | 0.0148 | 0.470 | 5.20 | H |
| Ref. | ID | nf | εf,debAV |
|---|---|---|---|
| Barton et al. [42] | SRG-2 | 1 | 0.0077 |
| Napoli and Realfonzo [21] | SRG-1LD | 1 | 0.0145 |
| SRG-2LD | 2 | 0.0101 | |
| SRG-1MD | 1 | 0.0082 | |
| SRG-1MD-A | 1 | 0.0082 | |
| SRG-1MD-B | 1 | 0.0082 | |
| SRG-2MD | 2 | 0.0057 | |
| Bencardino and Condello [41] | B-EB | 1 | 0.0086 |
| Ombres and Verre [43] | B-1L | 1 | 0.0115 |
| B-1L-1A | 1 | 0.0115 | |
| B-1L-2A | 1 | 0.0115 |
| Rif./Strengthening System | εf,maxAV | εlim,convAV | ε(α)lim,convAV | εf,debAV | Diff1 (%) | Diff2 (%) | Density | αreal |
|---|---|---|---|---|---|---|---|---|
| Barton et al./Hardwire 3SX | 0.00650 | 0.00322 | 0.00483 | 0.00655 | −25.66 | 0.83 | M | 1.49 |
| Napoli and Realfonzo/Geosteel G600 | 0.0128 | 0.00912 | 0.0137 | 0.0123 | 6.89 | −3.72 | L | 1.40 |
| Napoli and Realfonzo/Geosteel G2000 | 0.00780 | 0.00339 | 0.00509 | 0.00761 | −34.35 | −1.84 | H | 2.28 |
| Bencardino and Condello/Kimisteel INOX | 0.00599 | 0.00314 | 0.00471 | 0.00862 | −21.30 | 43.87 | H | 1.91 |
| Ombres and Verre/Geosteel G1200 | 0.00740 | 0.00532 | 0.00797 | 0.0102 | 7.77 | 37.83 | L-M | 1.39 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bencardino, F.; Nisticò, M. Evaluation of the Maximum Strain for Different Steel-FRCM Systems in RC Beams Strengthened in Flexure. Fibers 2022, 10, 67. https://doi.org/10.3390/fib10080067
Bencardino F, Nisticò M. Evaluation of the Maximum Strain for Different Steel-FRCM Systems in RC Beams Strengthened in Flexure. Fibers. 2022; 10(8):67. https://doi.org/10.3390/fib10080067
Chicago/Turabian StyleBencardino, Francesco, and Mattia Nisticò. 2022. "Evaluation of the Maximum Strain for Different Steel-FRCM Systems in RC Beams Strengthened in Flexure" Fibers 10, no. 8: 67. https://doi.org/10.3390/fib10080067
APA StyleBencardino, F., & Nisticò, M. (2022). Evaluation of the Maximum Strain for Different Steel-FRCM Systems in RC Beams Strengthened in Flexure. Fibers, 10(8), 67. https://doi.org/10.3390/fib10080067







