Effect of PTFE Particle Size on Superhydrophobic Coating for Supercooled Water Prevention
Abstract
1. Introduction
2. Methods and Experiment
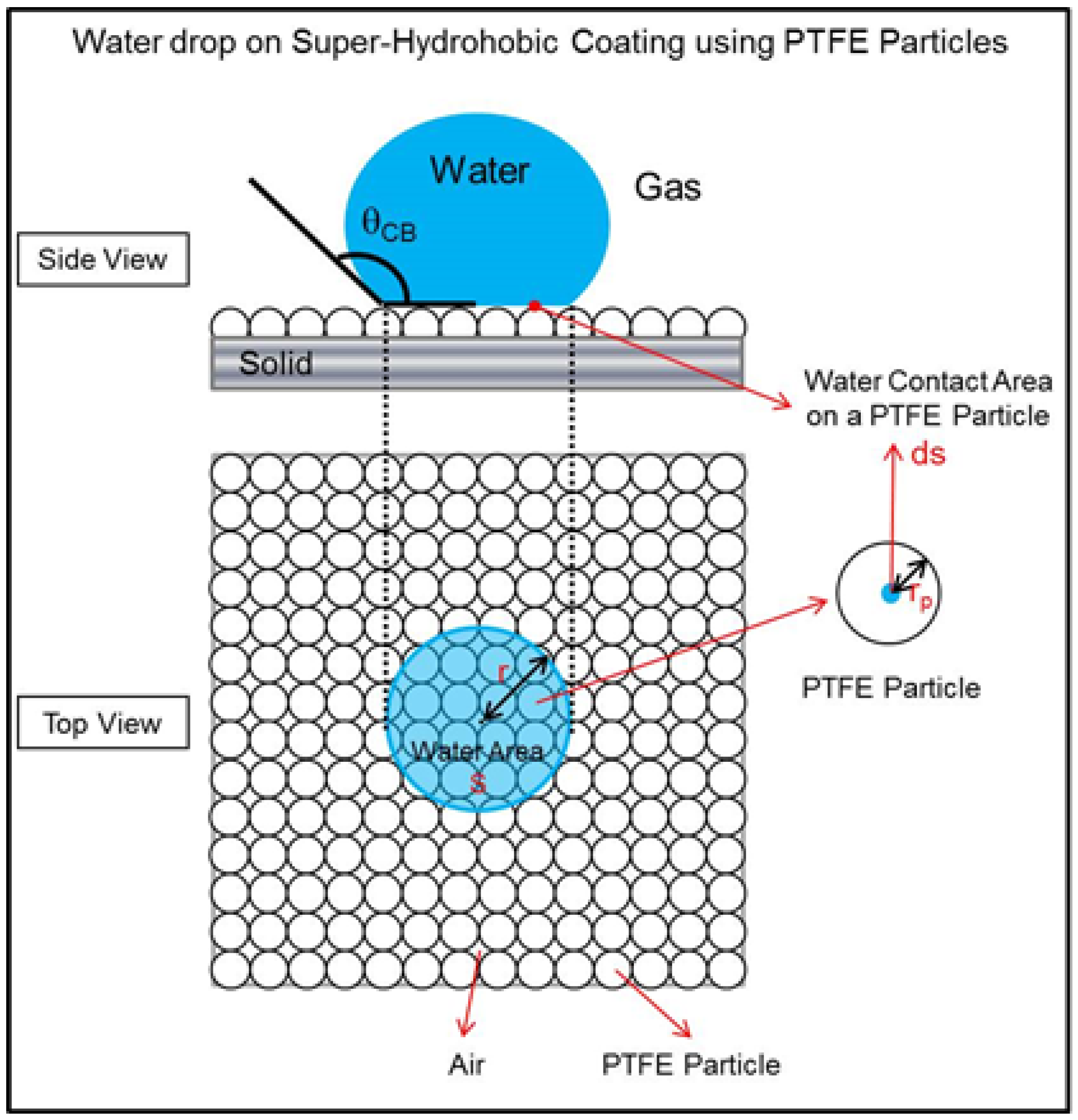
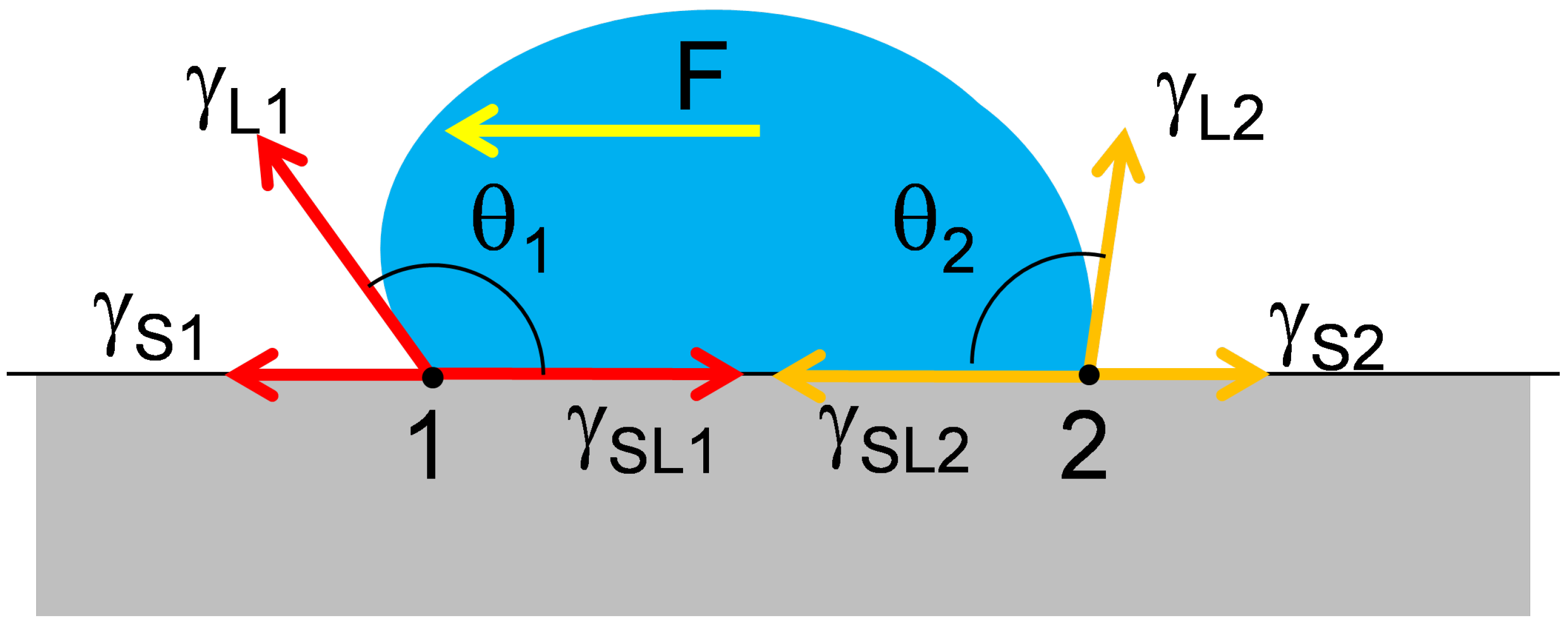
2.1. Static-Evaluation Method
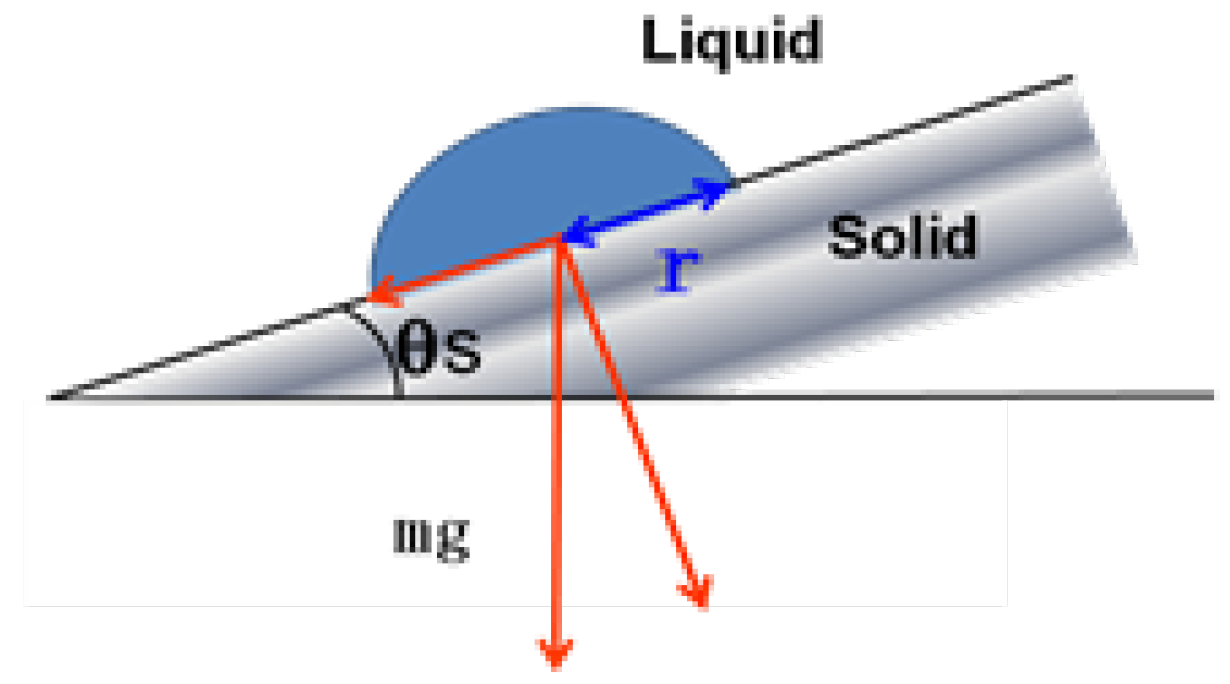
2.2. Dynamic-Evaluation Method
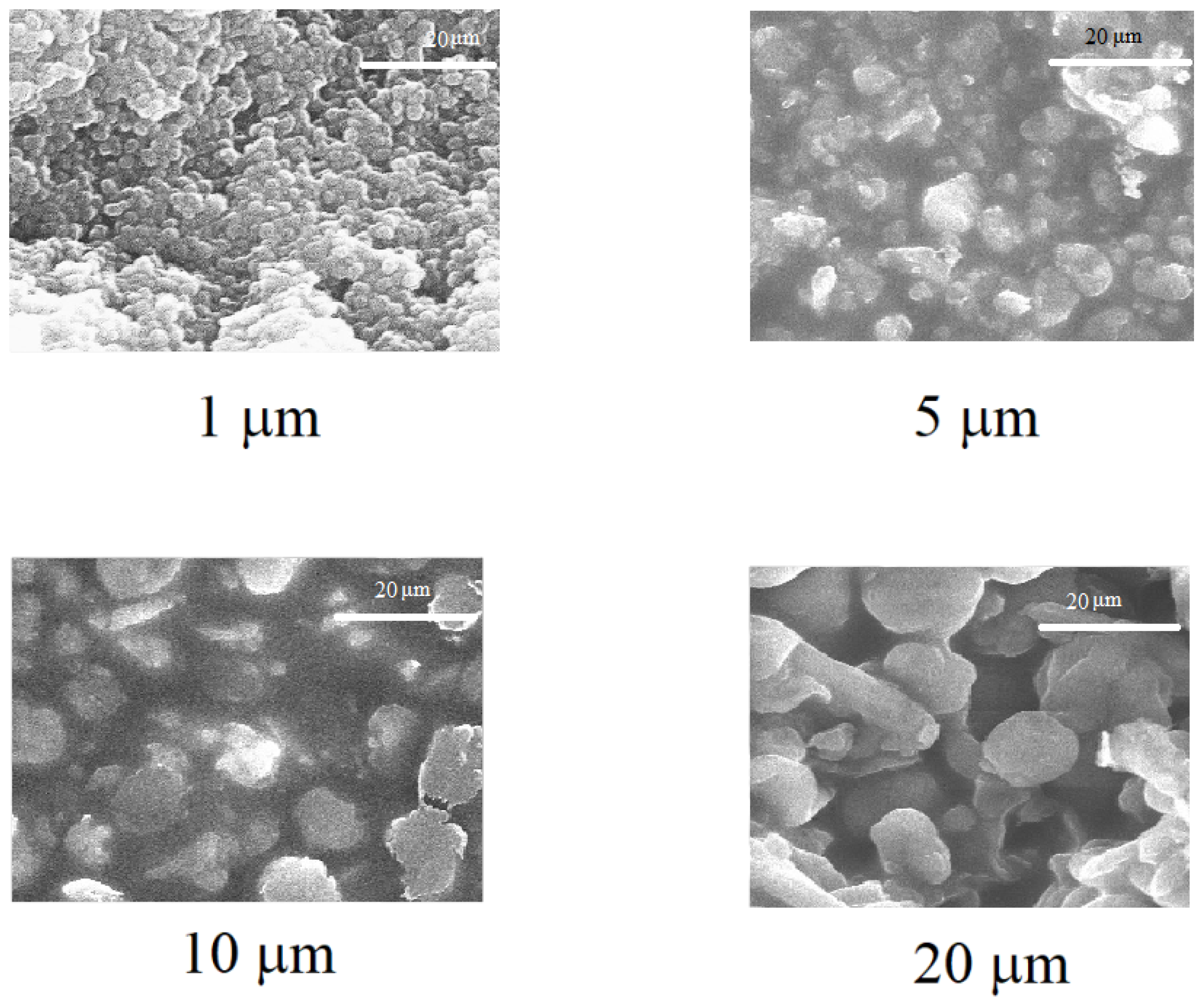
2.3. Coating Surface and Experimental Setup
3. Results and Discussion
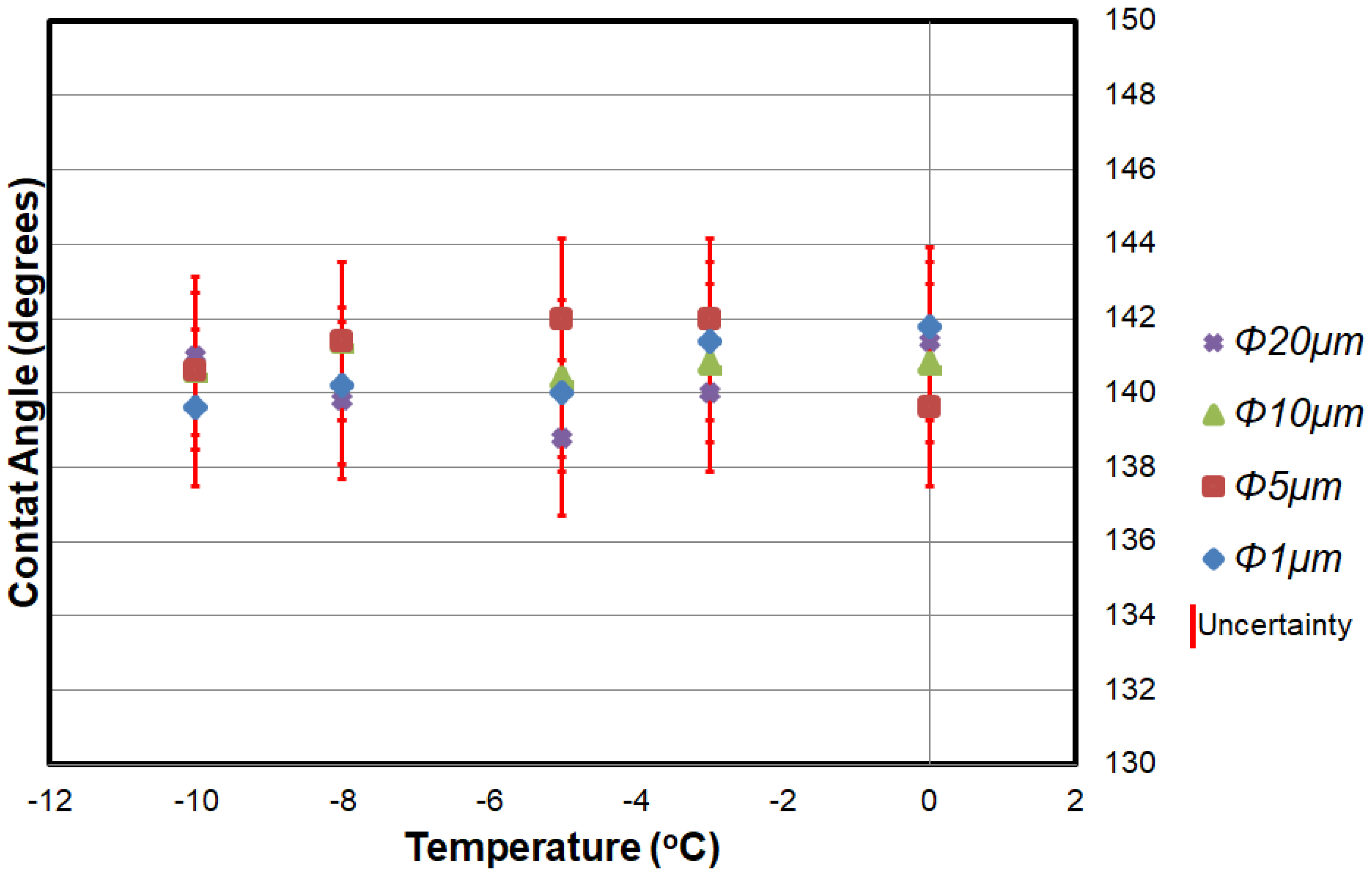
3.1. Static-Evaluation Results: Contact Angle under Supercooled Conditions
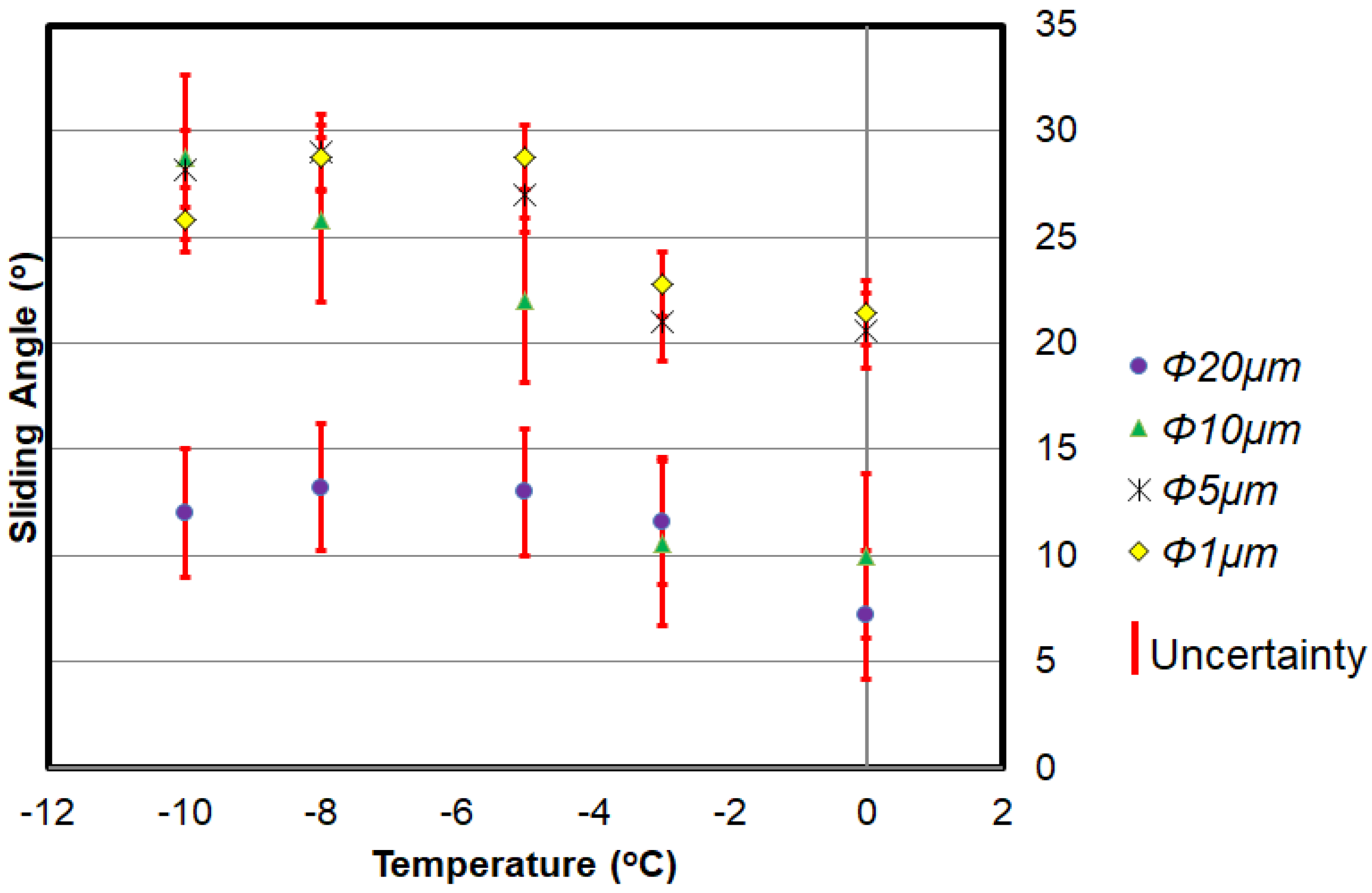
3.2. Dynamic-Evaluation Results: Sliding Angle under Supercooled Conditions
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Song, D.; Song, B.; Hu, H.; Du, X.; Du, P.; Choi, C.-H.; Rothstein, J.P. Effect of a surface tension gradient on the slip flow along a superhydrophobic air-water interface. Phys. Rev. Fluids 2018, 3, 033303. [Google Scholar] [CrossRef]
- Quere, D. Wetting and roughness. Ann. Rev. Mater. Res. 2008, 38, 71–99. [Google Scholar] [CrossRef]
- Rothstein, J. Slip on superhydrophobic surfaces. Ann. Rev. Fluid Mech. 2010, 42, 89–109. [Google Scholar] [CrossRef]
- Zhang, B.; Chen, X.; Dobnikar, J.; Wang, Z.; Zhang, X. Spontaneous Wenzel to Cassie cewetting transition on structured surfaces. Phys. Rev. Fluids 2016, 1, 073904. [Google Scholar] [CrossRef]
- Amabili, M.; Giacomello, A.; Meloni, S.; Casciola, C.M. Collapse of superhydrophobicity on nanopillared surfaces. Phys. Rev. Fluids 2017, 2, 034202. [Google Scholar] [CrossRef]
- Johnson, R.E.; Detter, R.H. Contact Angle Hysteresis. In Contact Angle, Wettability, and Adhesion; American Chemical Society: Washington, DC, USA, 1964; pp. 112–135. [Google Scholar]
- Diaz, M.E.; Savage, M.D.; Cerro, R.L. Prediction of static contact angles on the basis of molecular forces and adsorption data. Phys. Rev. E 2016, 94, 022801. [Google Scholar] [CrossRef] [PubMed]
- Varchanis, S.; Dimakopoulos, Y.; Tsamopoulos, J. Steady film flow over a substrate with rectangular trenches forming air inclusions. Phys. Rev. Fluids 2017, 2, 124001. [Google Scholar] [CrossRef]
- De Coninck, J.; Dunlop, F.; Huillet, T. Contact angles of a drop pinned on an incline. Phys. Rev. E 2017, 95, 052805. [Google Scholar] [CrossRef] [PubMed]
- Onda, T.; Shibuichi, S.; Satoh, N.; Tsujii, K. Super-water-repellent fractal surfaces. ACS J. Surf. Colloids 1996, 12, 2125–2127. [Google Scholar] [CrossRef]
- Lafuma, A.; Quere, D. Superhydrophobic states. Nat. Mater. 2003, 2, 457–460. [Google Scholar] [CrossRef] [PubMed]
- Menini, R.; Farzaneh, M. Elaboration of Al2O3/PTFE icephobic coatings for protecting aluminum surfaces. Surf. Coat. Technol. 2009, 203, 1941–1946. [Google Scholar] [CrossRef]
- Veeramasuneni, S.; Drelich, J.; Miller, J.; Yamauchi, G. Hydrophobicity of ion-plated PTFE coatings. Prog. Org. Coat. 1997, 31, 265–270. [Google Scholar] [CrossRef]
- Morita, K.; Sakaue, H. Characterization method of hydrophobic anti-icing coatings. Rev. Sci. Instrum. 2015, 86, 115108. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Q.; Liu, Y.; Wang, C. Development and evaluation of electroless Ag-PTFE composite coatings with anti-microbial and anti-corrosion properties. Appl. Surf. Sci. 2005, 252, 1620–1627. [Google Scholar] [CrossRef]
- Ling, H.; Katz, J.; Fu, M.; Hultmark, M. Effect of reynolds number and saturation level on gas diffusion in and out of a superhydrophobic surface. Phys. Rev. Fluids 2017, 2, 124005. [Google Scholar] [CrossRef]
- Hernandez-Perez, R.; García-Cordero, J.L.; Escobar, J.V. Simple scaling laws for the evaporation of droplets pinned on pillars: Transfer-rate- and diffusion-limited regimes. Phys. Rev. Fluids 2017, 96, 062803. [Google Scholar] [CrossRef] [PubMed]
- Weisend, N.A. Design of an advanced pneumatic deicer for the composite rotor blade. J. Aircr. 1989, 26, 947–950. [Google Scholar] [CrossRef]
- Zwolinski, B.J.; Eicher, L.D. High-precision viscosity of supercooled water and analysis of the extended range temperature coefficient. J. Phys. Chem. 1971, 75, 2016–2024. [Google Scholar] [CrossRef]
- Hallett, J. The temperature dependence of the viscosity of supercooled water. Proc. Phys. Soc. 1963, 82, 1046. [Google Scholar] [CrossRef]
- De Ruijter, M.; Kölsch, P.; Voué, M.; Coninck, J.D.; Rabe, J. Effect of temperature on the dynamic contact angle. Colloids Surf. A Physicochem. Eng. Asp. 1998, 144, 235–243. [Google Scholar] [CrossRef]
- Chiu, C.C.; Moore, P.B.; Shinoda, W.; Nielsen, S.O. Size-dependent hydrophobic to hydrophilic transition for nanoparticles: A molecular dynamics study. J. Chem. Phys. 2009, 131, 244706. [Google Scholar] [CrossRef] [PubMed]
- Caban-Nevarez, R.; Perales Perez, O.J. Size-dependence hydrophobicity in nanocrystalline talc produced by high-intensity planetary ball milling. MRS Proc. 2015, 1805. [Google Scholar] [CrossRef]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morita, K.; Gonzales, J.; Sakaue, H. Effect of PTFE Particle Size on Superhydrophobic Coating for Supercooled Water Prevention. Coatings 2018, 8, 426. https://doi.org/10.3390/coatings8120426
Morita K, Gonzales J, Sakaue H. Effect of PTFE Particle Size on Superhydrophobic Coating for Supercooled Water Prevention. Coatings. 2018; 8(12):426. https://doi.org/10.3390/coatings8120426
Chicago/Turabian StyleMorita, Katsuaki, Joseph Gonzales, and Hirotaka Sakaue. 2018. "Effect of PTFE Particle Size on Superhydrophobic Coating for Supercooled Water Prevention" Coatings 8, no. 12: 426. https://doi.org/10.3390/coatings8120426
APA StyleMorita, K., Gonzales, J., & Sakaue, H. (2018). Effect of PTFE Particle Size on Superhydrophobic Coating for Supercooled Water Prevention. Coatings, 8(12), 426. https://doi.org/10.3390/coatings8120426





