Interfacial Mechanics Analysis of a Brittle Coating–Ductile Substrate System Involved in Thermoelastic Contact
Abstract
:1. Introduction
2. Theory
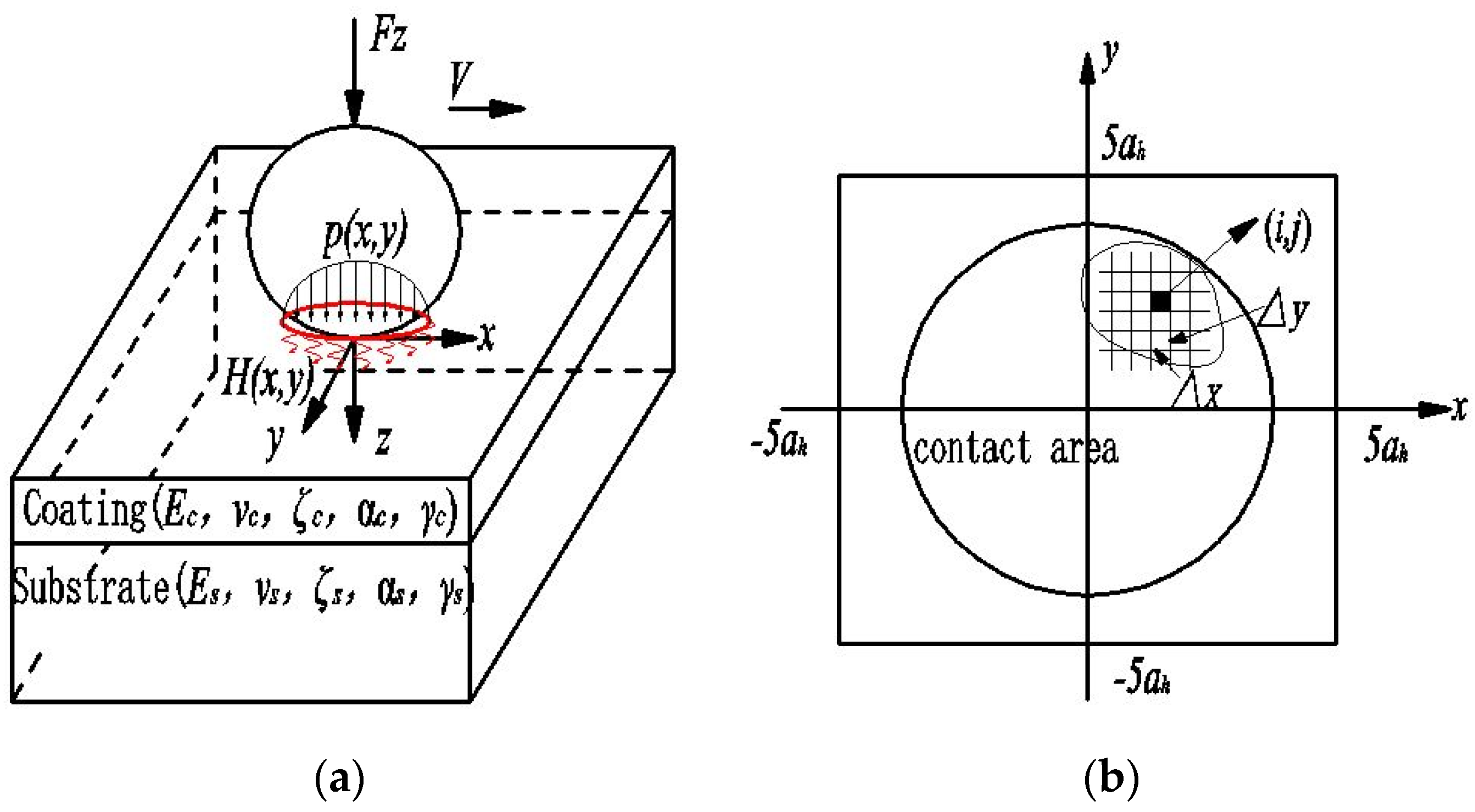
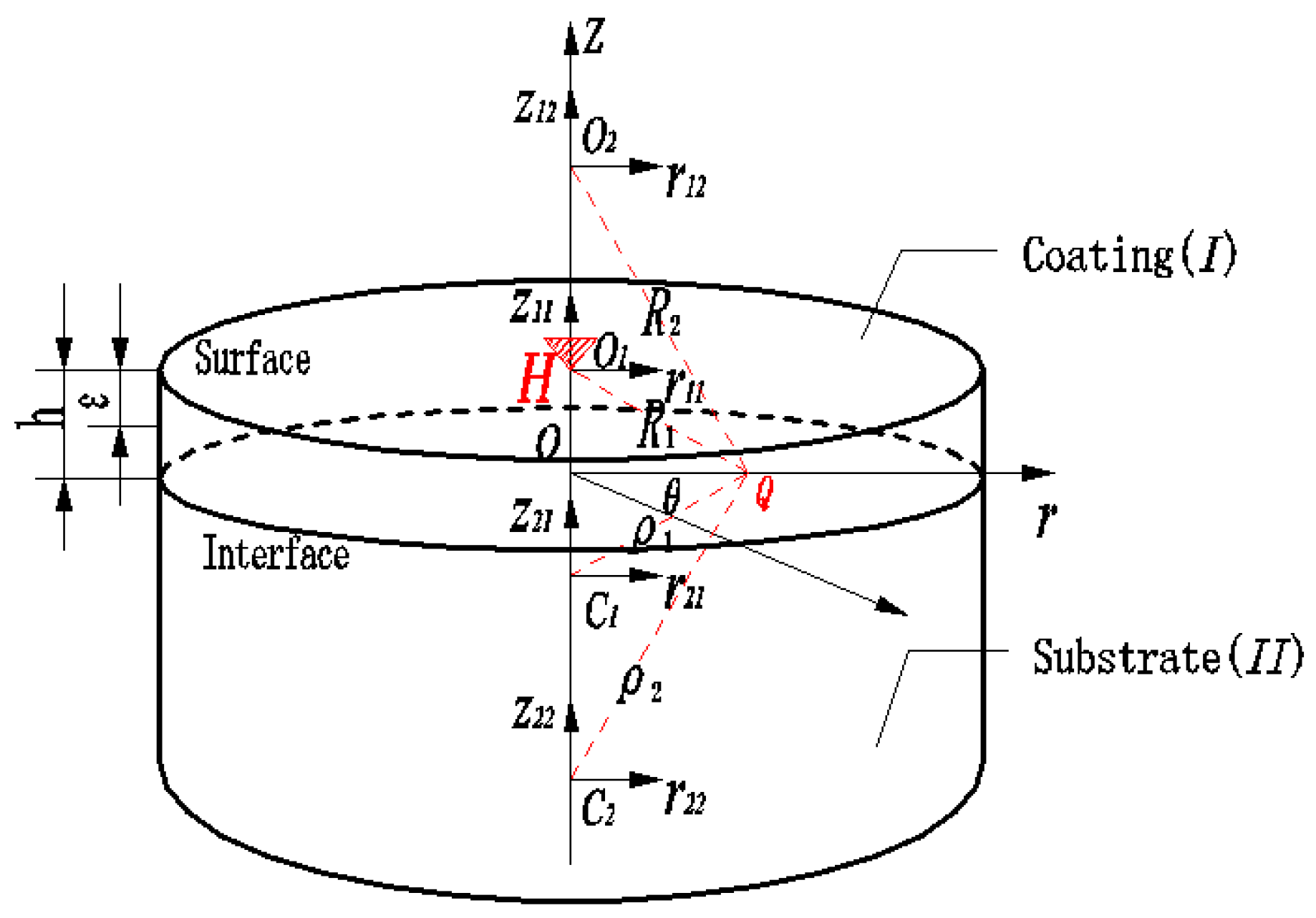
2.1. Description of the Thermoelastic Contact Model
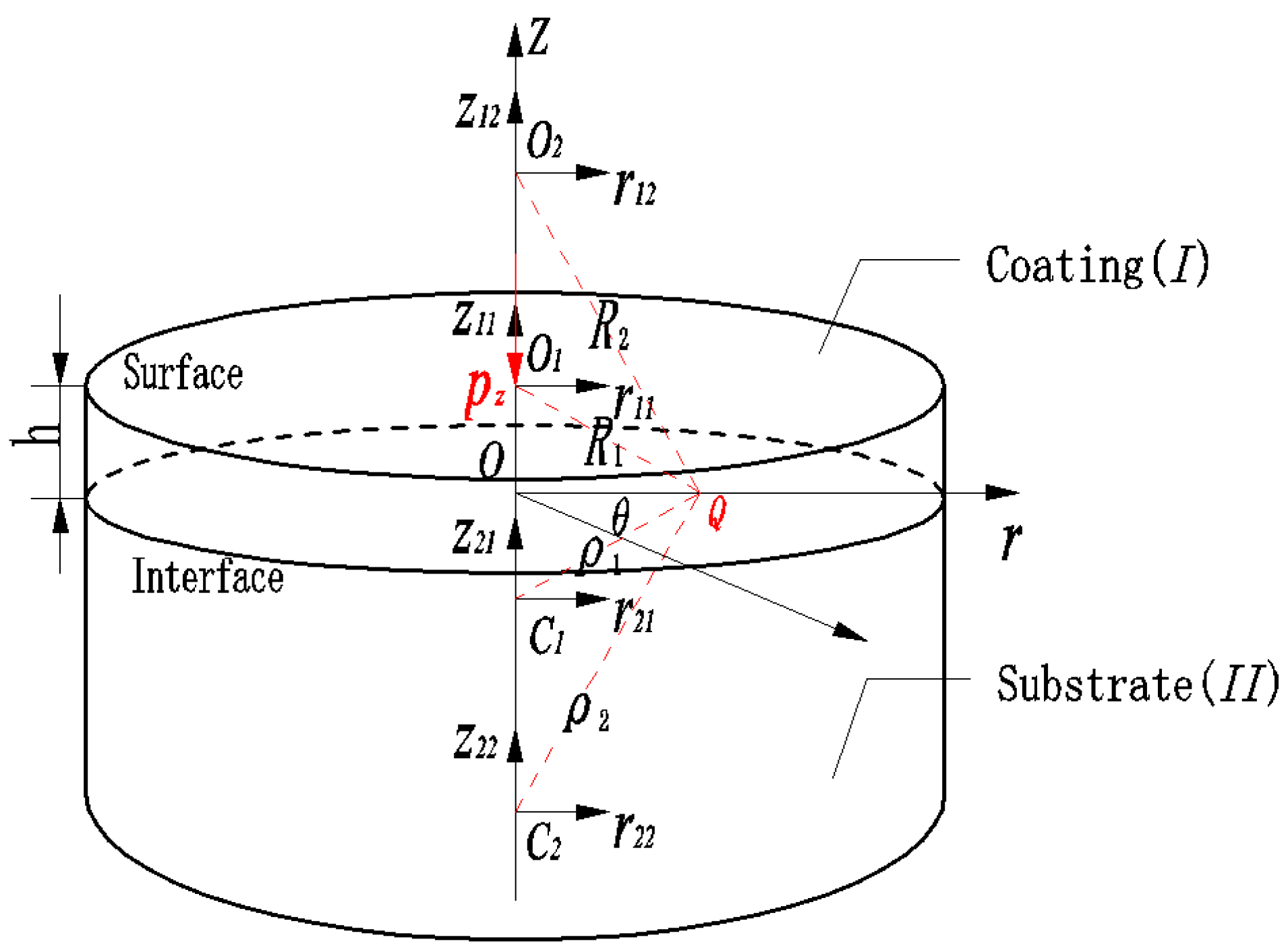
2.2. Green’s Function for a Point Normal Load Acting on the Surface of a Coated Isotropic Thermoelastic Material
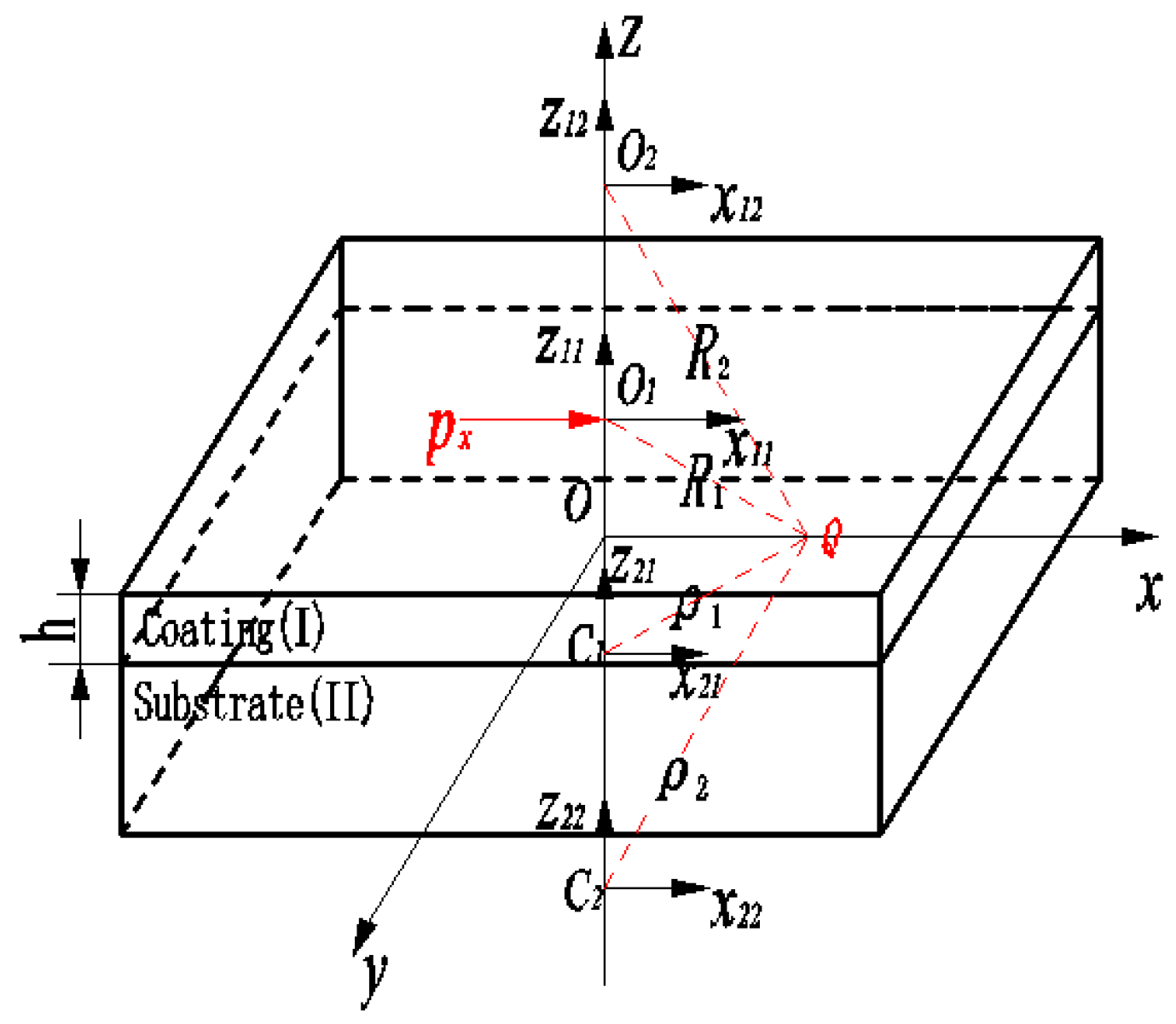
2.3. Green’s Function for a Point Tangential Load Acting on the Surface of a Coated Isotropic Thermoelastic Material
2.4. Green’s Function for a Moving Point Heat Resource Acting on the Surface of a Coated Isotropic Thermoelastic Material
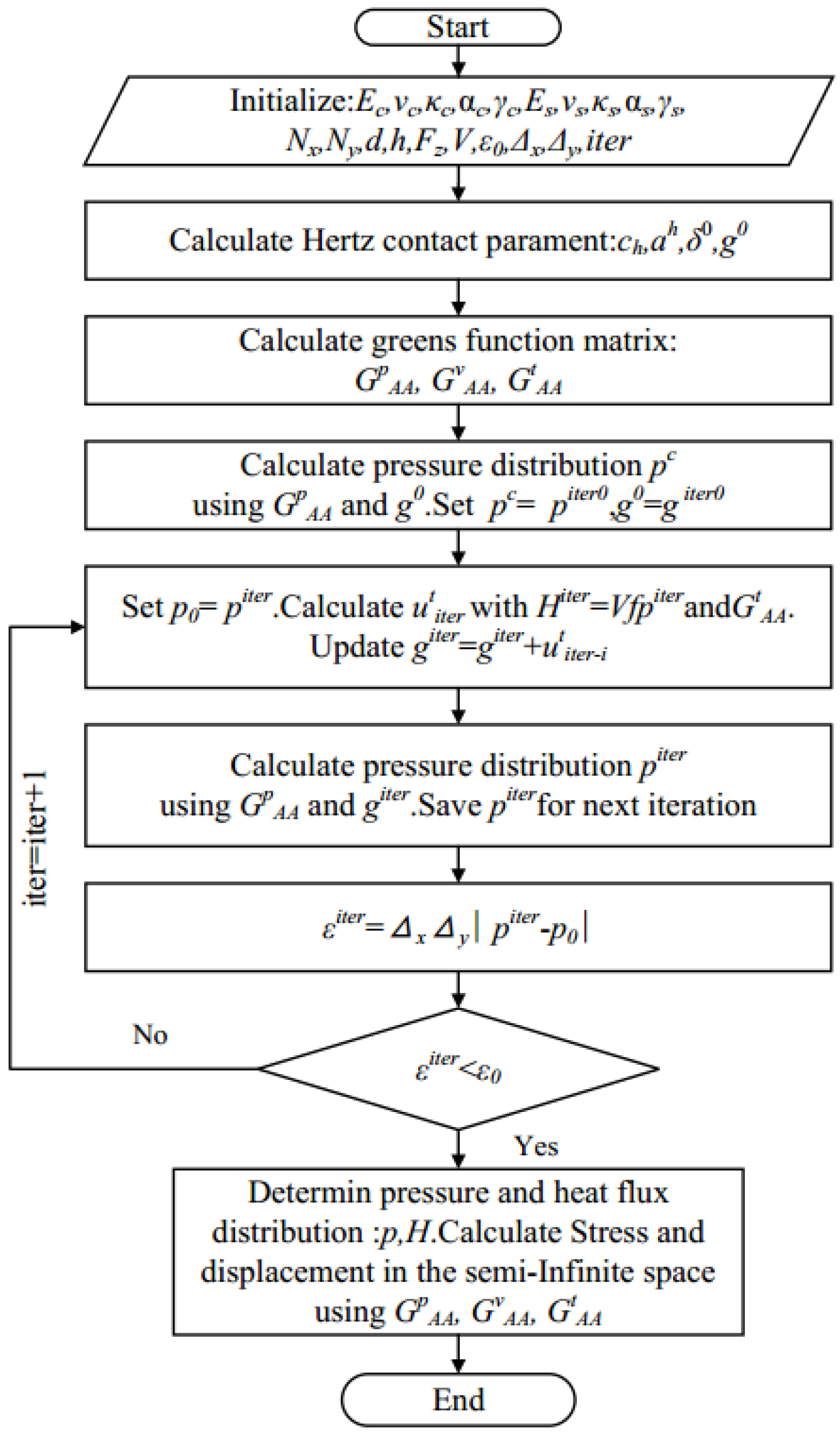
2.5. Solution Procedure
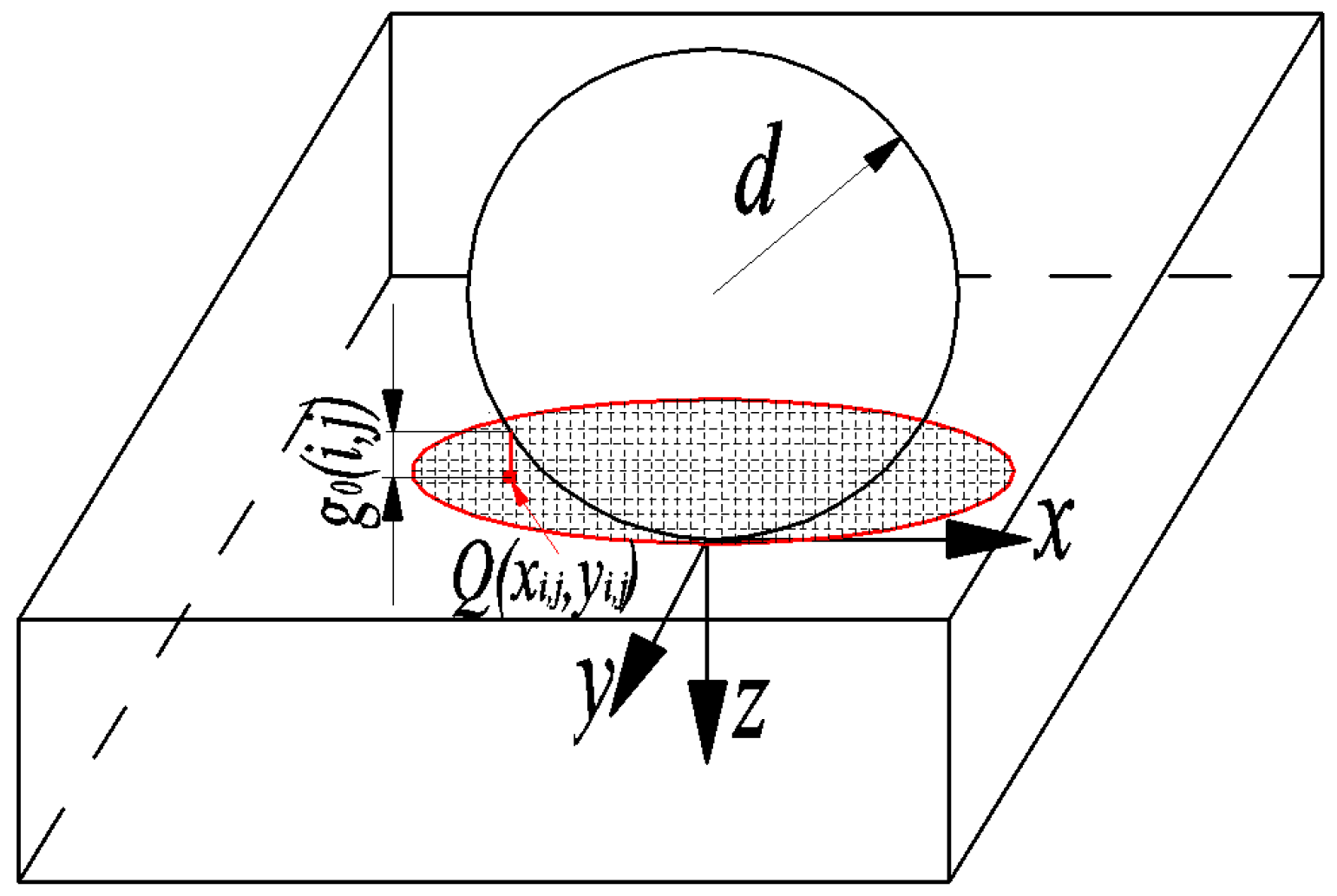
- Initialize material parameters and structural parameters. Calculate Hertz contact radius ah, Hertz contact pressure ch, Hertz contact approach δ0, and initial gap g0.
- According to the material parameters and the coating thickness, construct a matrix of Green’s functions for normal load GpAA using Equation (9) and Equations (13)–(15); construct matrix of Green’s function for thermal load GtAA using Equations (20)–(27); construct matrix of Green’s function for tangential load GvAA using Equations (16)–(19).
- Employ GpAA and the quadratic programming method, setting giter0 = g0, calculate Hertzian contact pressure distribution pc using Equations (2)–(6). Set piter0 = pc.
- Obtain surface heat flux Hiter, according to Equation (1) and piter. Employing GtAA and Hiter, calculate thermally induced surface displacement utiter. Update gap giter = giter + utiter.
- Save piter as p0, then update surface contact pressure piter by employing giter, GpAA, and the quadratic programming method.
- Calculate εiter = ΔxΔy| piter − p0| to judge convergence. If εiter < ε0, go to step 7, otherwise go to step 4 for the next iteration.
- Obtain contact pressure p and heat flux H. Calculate σAA using Equation (7) by employing GAAp, GAAv, and GAAt.
3. Results and Discussion
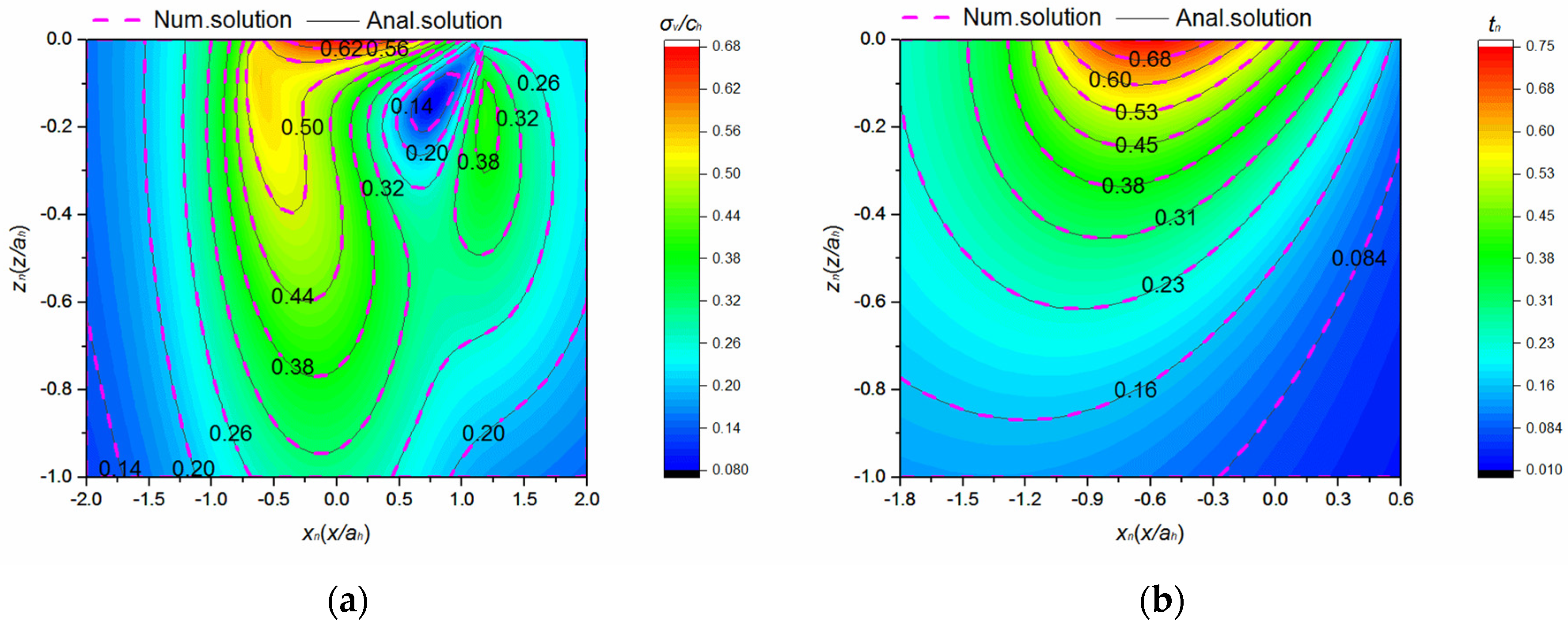
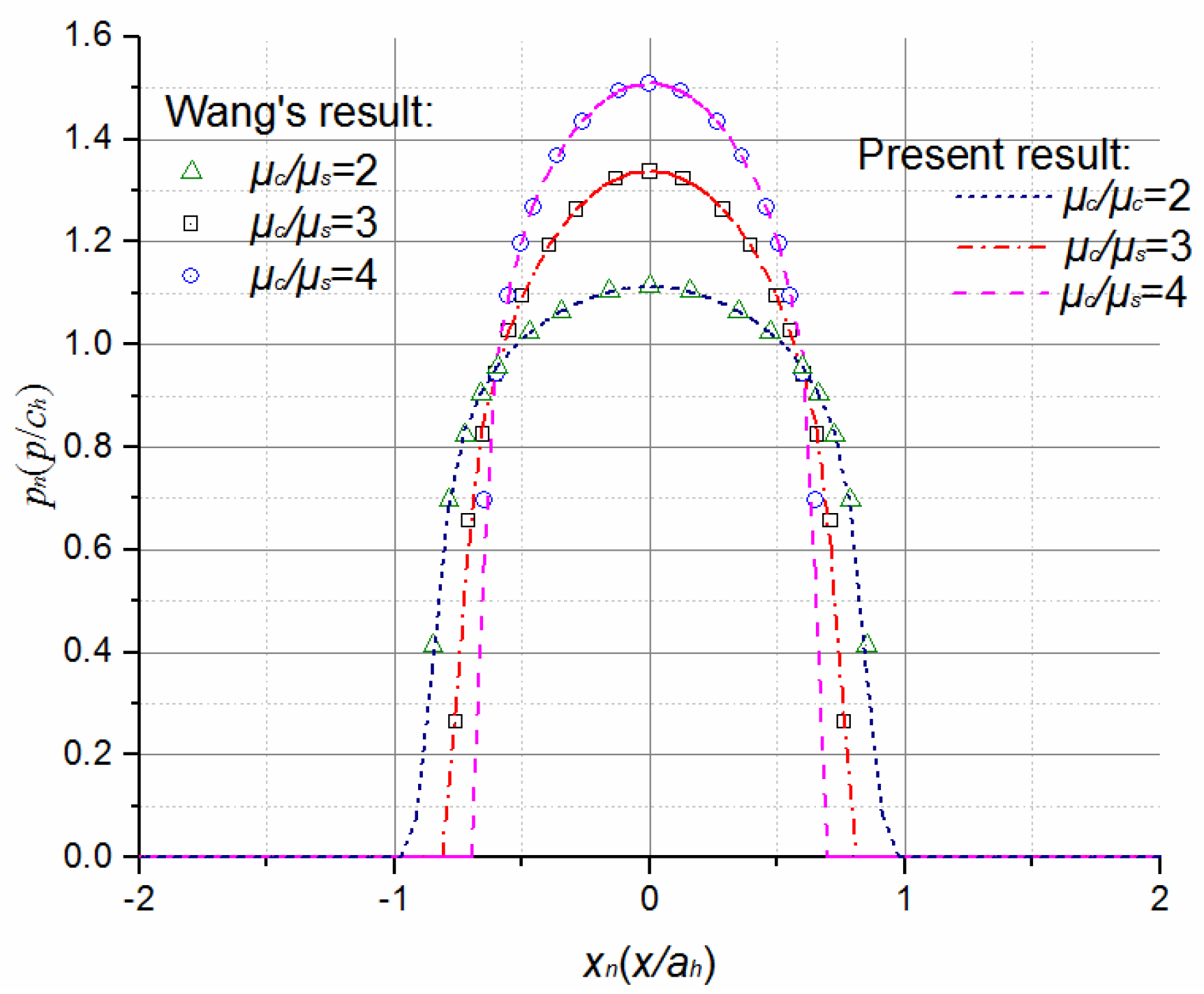
3.1. Model Verification
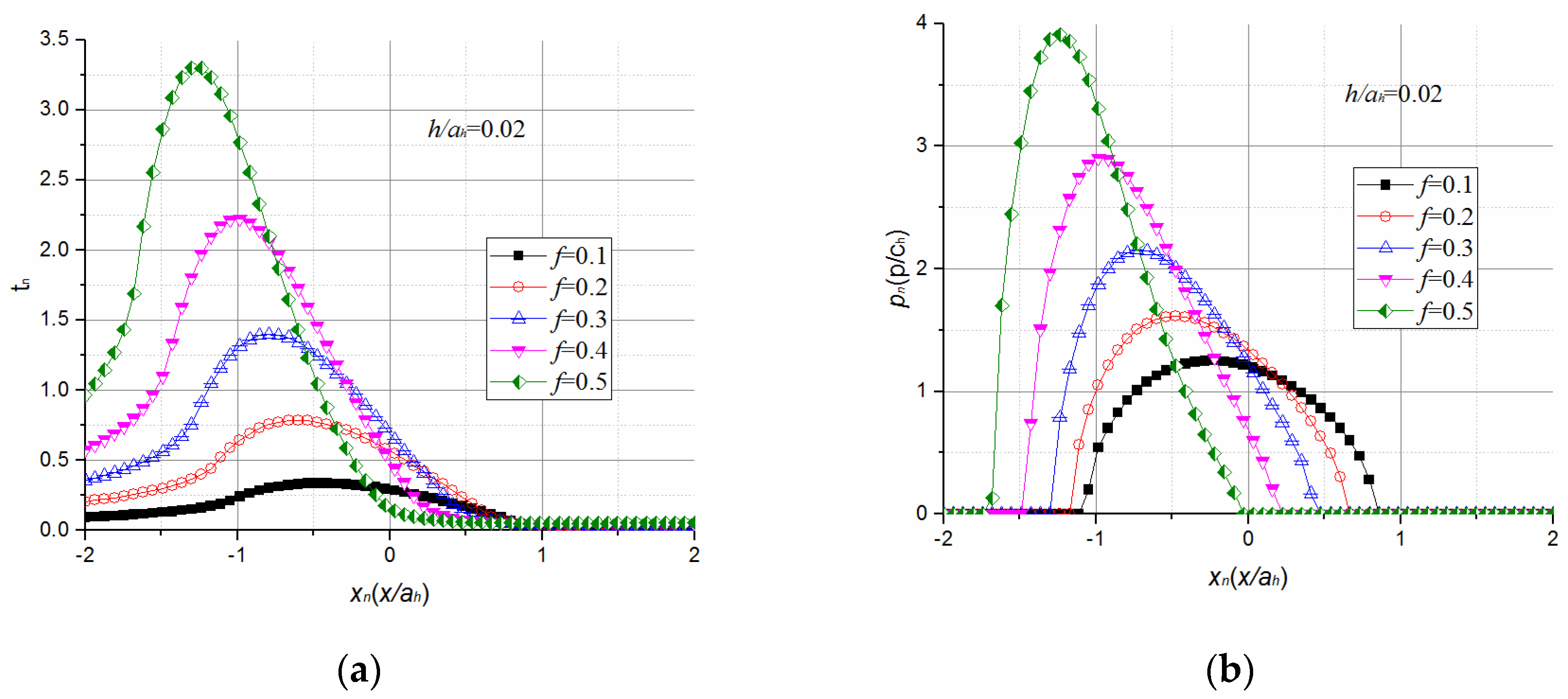
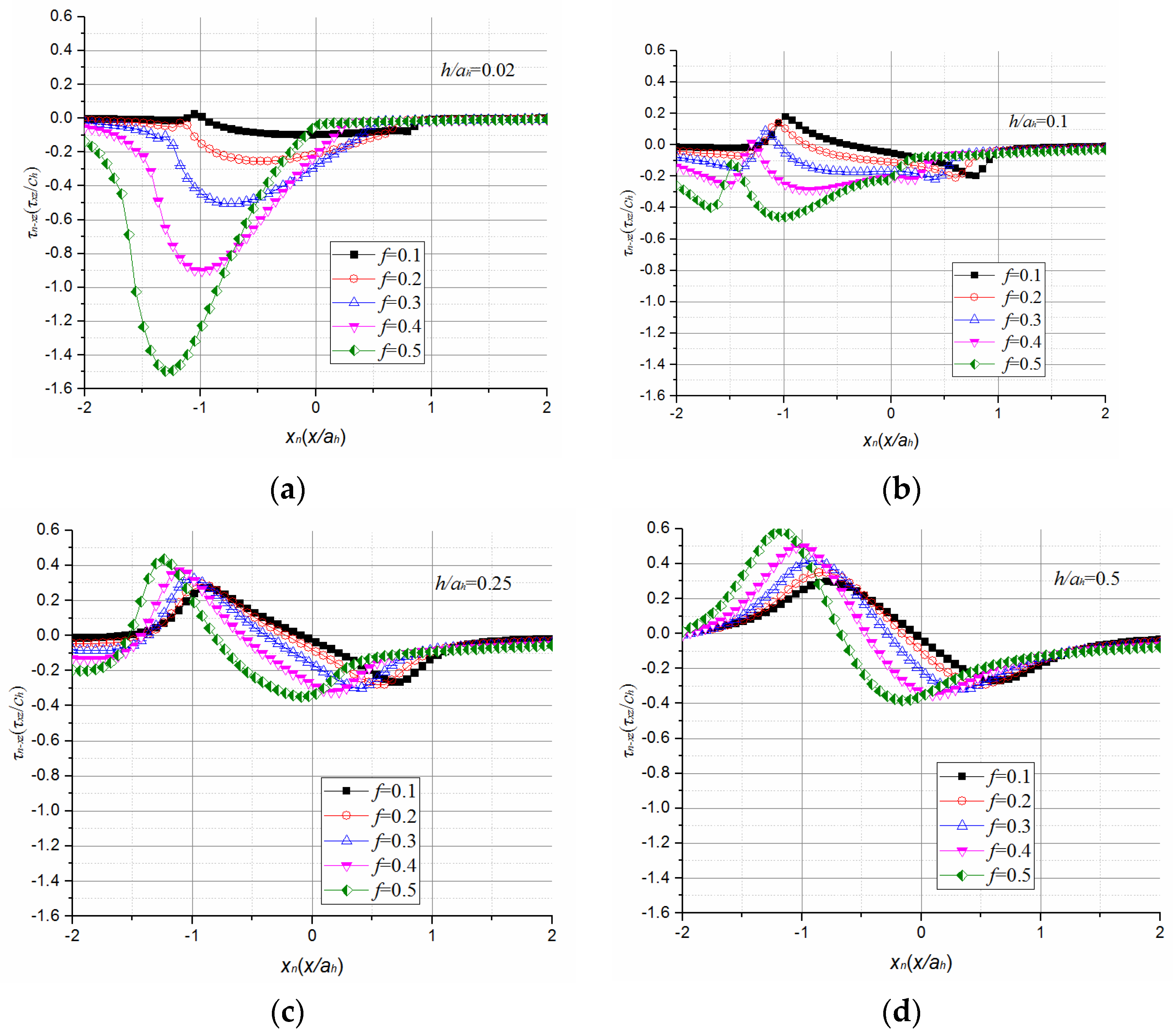
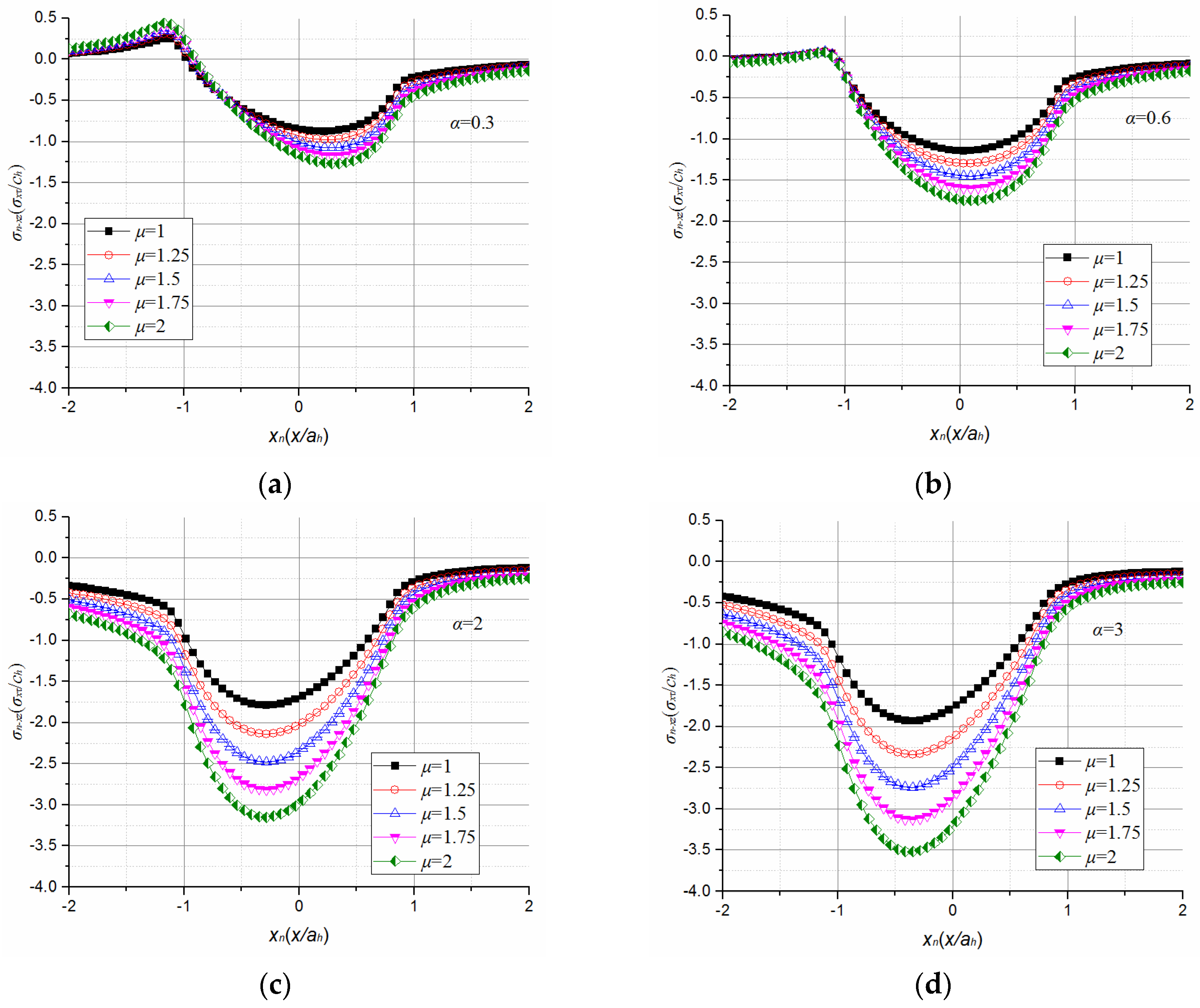
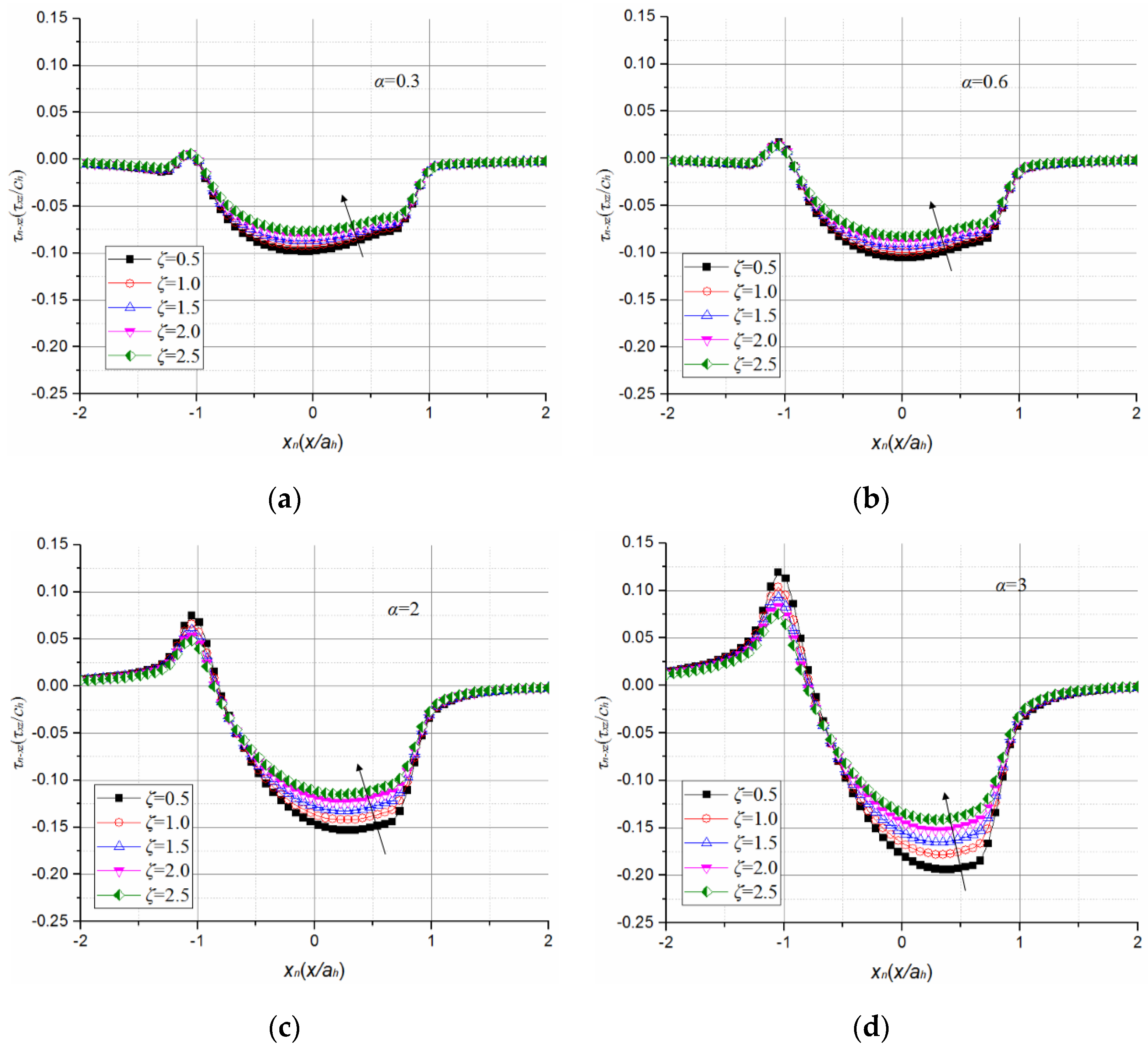
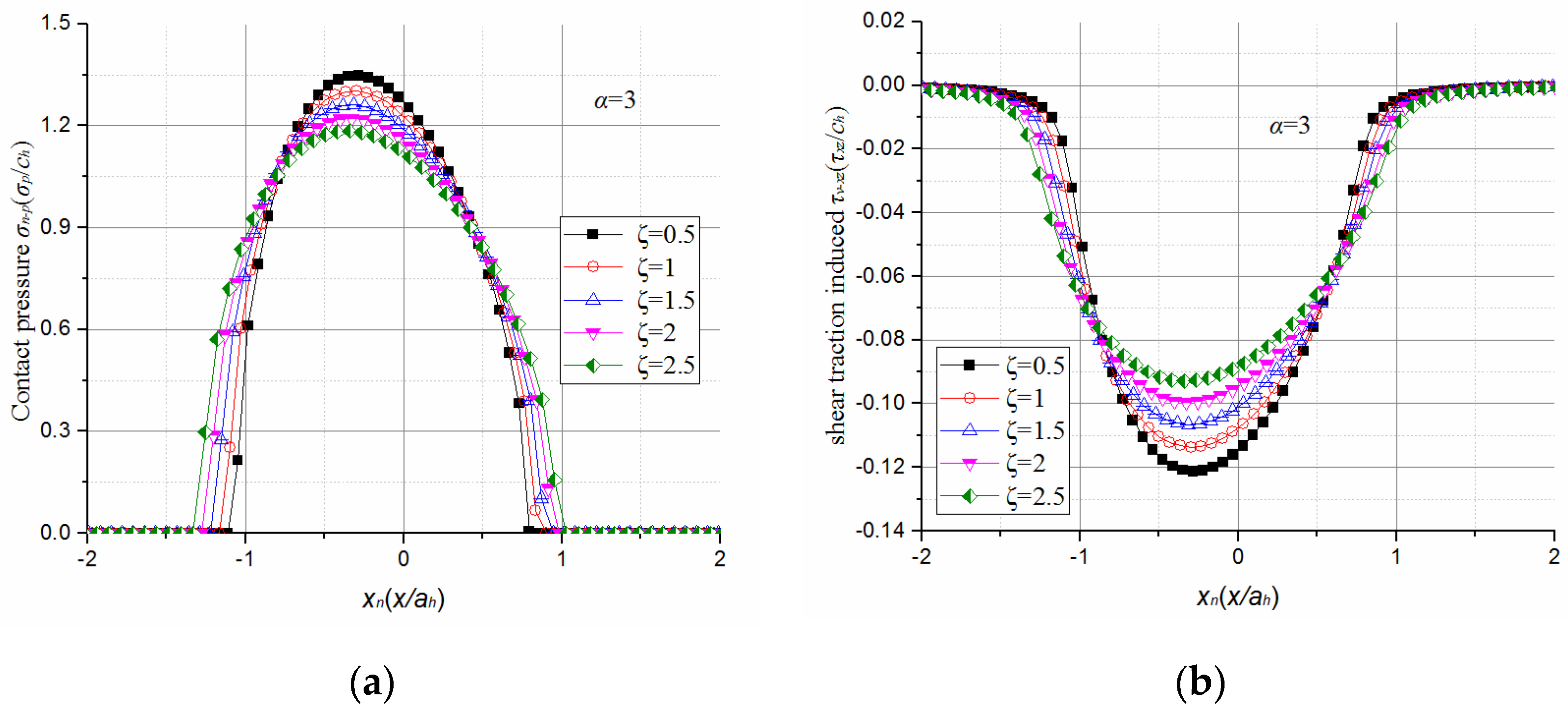
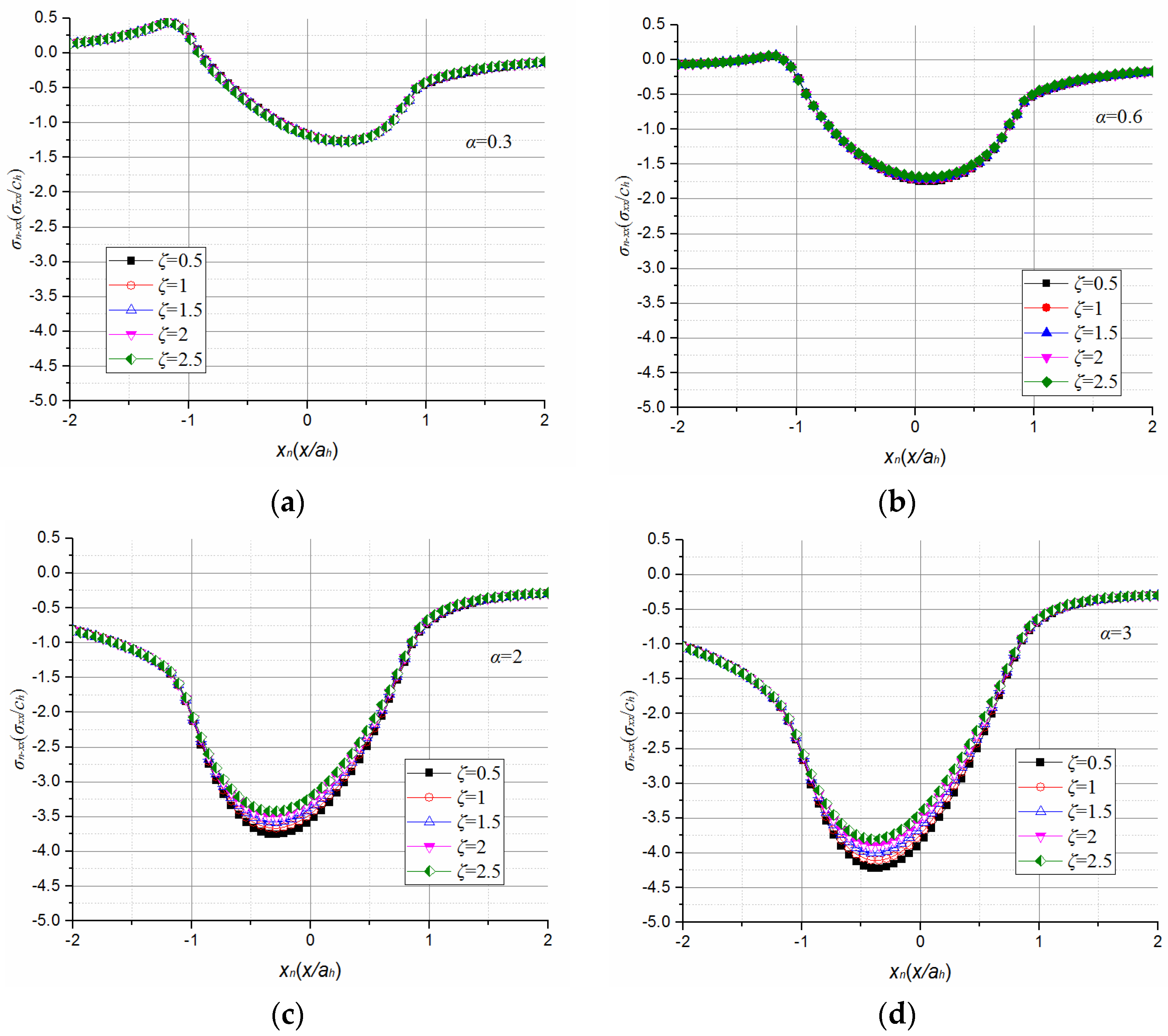
3.2. Effects of Friction Coefficient and Coating Thickness on Contact Pressure and Interface Stress Distribution
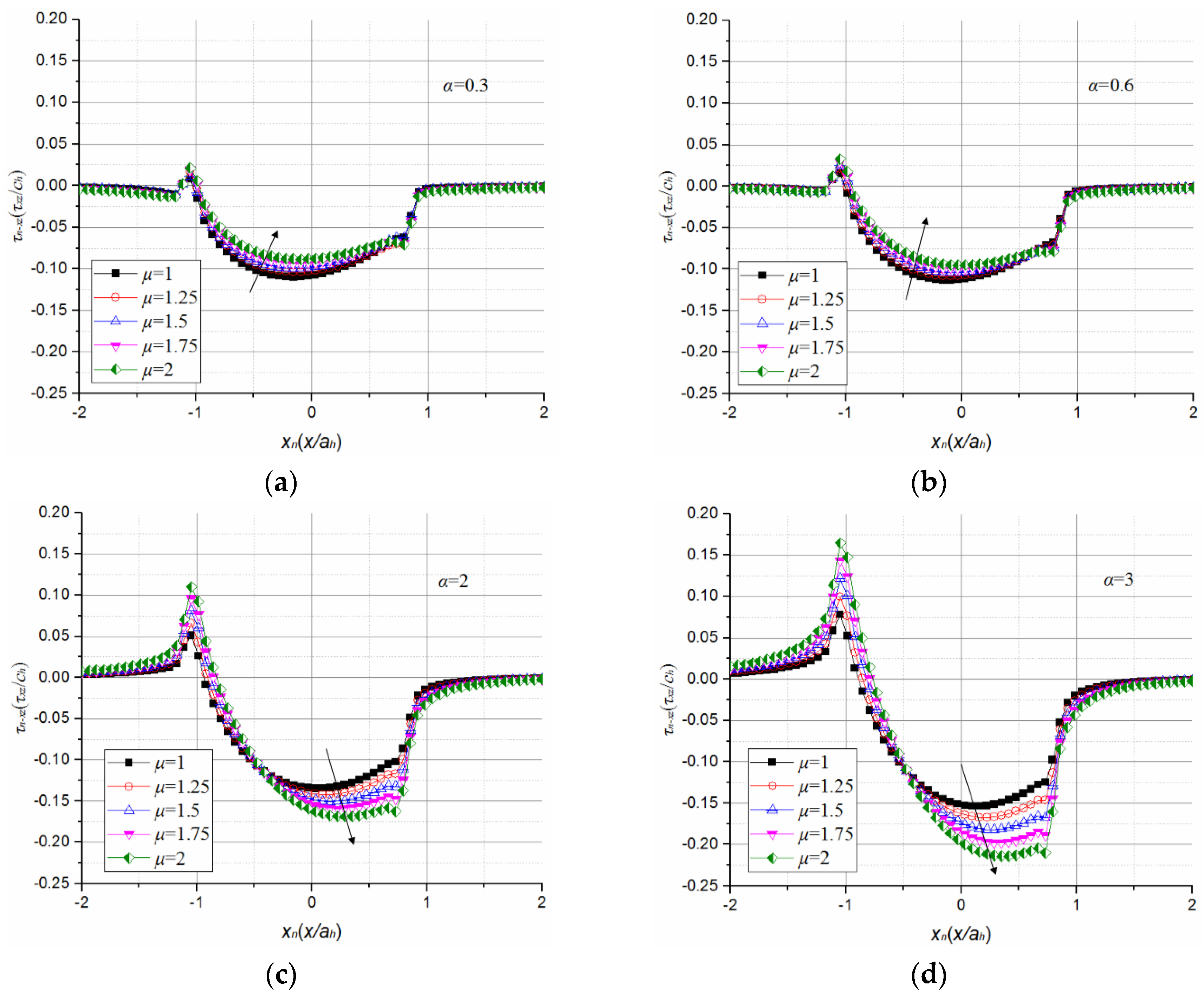
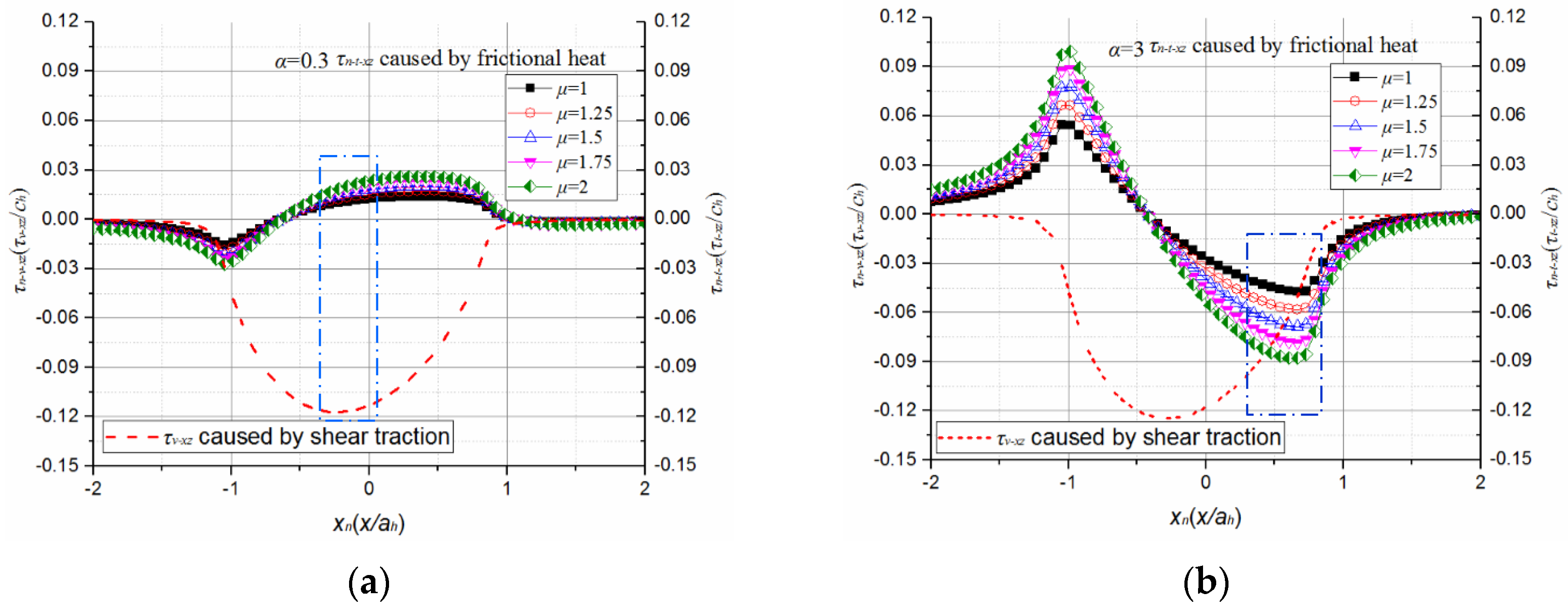
3.3. Effect of a Coating’s Elastic Modulus and Thermal Expansion Coefficient on Interface Stress Distribution
3.4. Effect of a Coating’s Thermal Conductiveness and Thermal Expansion Properties on Interface Stress Distribution
4. Conclusions
- Adequate design of coating thickness for different friction coefficients can be help in obtaining relatively low interfacial shear stress and tensile transverse stress at the same time. For brittle coatings (Ec > Es), in the case where the friction coefficient is less than 0.1, a smaller coating thickness, with h/ah < 0.02, is recommended. With a friction coefficient between 0.1 and 0.3, a coating with a thickness h/ah of about 0.1 is proposed. When the friction coefficient is greater than 0.3, coating with a thickness h/ah above 0.25 is preferable.
- Interfacial shear stress tends to be relieved by frictional heating in the case of αc/αs < 1 for brittle coatings. Meanwhile, tensile transverse stress on the interface tends to be eliminated in the case of αc/αs > 0.6. A thermal expansion coefficient ratio of 0.6 < αc/αs < 1 can be recommended as a goal for the optimization of thin hard coatings-substrate systems.
- Coating materials with enhanced thermal conductivity are shown to lower surface contact pressure concentration, and, thus, decrease interfacial shear stress. Although the interfacial tensile transverse stress is affected, to a negligible extent, by the variations of the thermal conductive coefficient of coating materials, it is predictable that the increased thermal conductivity of a coating could improve the resistance to interfacial delamination of the coating material.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Ebert, F.J. Fundamentals of design and technology of rolling element bearings. Chin. J. Aeronaut. 2010, 23, 123–136. [Google Scholar] [CrossRef]
- Tong, Y.X.; Wang, L.Q.; Gu, L. Friction and wear behaviors of Si3N4 sliding against M50 bearing steel in vacuum. Adv. Mater. Res. 2010, 97, 1681–1684. [Google Scholar] [CrossRef]
- Wang, L.Q.; Li, C.; Zheng, D.Z.; Gu, L. Nonlinear Dynamics Behaviors of a Rotor Roller Bearing System with Radial Clearances and Waviness Considered. Chin. J. Aeronaut. 2008, 21, 86–96. [Google Scholar]
- Daisuke, Y.; Richard, J.C.; Peter, A.D. Wear mechanisms of steel roller bearings protected by thin, hard and low friction coatings. Wear 2005, 259, 779–788. [Google Scholar]
- Bobzin, K.; Lugscheider, E.; Maes, M.; Gold, P.W.; Loos, J.; Kuhn, M. High-performance chromium aluminium nitride PVD coatings on roller bearings. Surf. Coat. Technol. 2004, 188, 649–654. [Google Scholar] [CrossRef]
- Nie, C.Y.; Zheng, D.Z.; Gu, L.; Zhao, X.L.; Wang, L.Q. Comparison of interface mechanics characteristics of DLC coating deposited on bearing steel and ceramics. Appl. Surf. Sci. 2014, 317, 188–197. [Google Scholar] [CrossRef]
- O’Sullivan, T.C.; King, R.B. Sliding contact stress-field due to a spherical indenter on a layered elastic half-space. ASME. J. Tribol. 1988, 110, 235–240. [Google Scholar] [CrossRef]
- Wang, Z.J.; Wang, W.Z.; Wang, H.; Dong, Z.; Hu, Y.Z. Partial slip contact analysis on three-dimensional elastic layered half Space. ASME. J. Tribol. 2010, 132, 280–290. [Google Scholar] [CrossRef]
- Liu, S.B.; Wang, Q.; Liu, G. A versatile method of discrete convolution and FFT (DC-FFT) for contact analyses. Wear 2000, 243, 101–111. [Google Scholar] [CrossRef]
- Cai, S.; Bhushan, B. A numerical three-dimensional contact model for rough, multilayered elastic/plastic solid surfaces. Wear 2005, 259, 1408–1423. [Google Scholar] [CrossRef]
- Kot, M. Contact mechanics of coating–substrate systems: Monolayer and multilayer coatings. Arch. Civ. Mech. Eng. 2012, 12, 464–470. [Google Scholar] [CrossRef]
- Komvopoulos, K. Finite element analysis of a layered elastic solid in normal contact with a rigid surface. ASME. J. Tribol. 1988, 110, 477–485. [Google Scholar] [CrossRef]
- Kral, E.R.; Komvopoulos, K.; Bogy, D.B. Finite Element Analysis of Repeated Indentation of an Elastic-Plastic Layered Medium by a Rigid Sphere, Part I: Surface Results. ASME. J. Appl. Mech. 1995, 62, 20–28. [Google Scholar] [CrossRef]
- Ye, N.; Komvopoulos, K. Three-dimensional finite element analysis of elastic–plastic layered media under thermomechanical surface loading. ASME. J. Tribol. 2003, 125, 52–59. [Google Scholar] [CrossRef]
- Yang, J.; Komvopoulos, K. Dynamic indentation of an elastic–plastic multilayered medium by a rigid cylinder. ASME. J. Tribol. 2004, 126, 18–27. [Google Scholar] [CrossRef]
- Liu, J.; Ke, L.L.; Wang, Y.S. Two-dimensional thermoelastic contact problem of functionally graded materials involving frictional heating. Int. J. Solids Struct. 2011, 48, 2536–2548. [Google Scholar] [CrossRef]
- Choi, H.J.; Paulino, G.H. Thermoelastic contact mechanics for a flat punch sliding over a graded coating/substrate system with frictional heat generation. J. Mech. Phys. 2008, 56, 1673–1692. [Google Scholar] [CrossRef]
- Neha, S.; Kumar, N.; Dash, S.; Das, C.R.; Subba, R.R.V.; Tyagi, A.K. Scratch resistance and tribological properties of DLC coatings under dry and lubrication conditions. Tribol. Int. 2012, 56, 129–140. [Google Scholar]
- Singh, R.K.; Tilbrook, M.T.; Xie, Z.H. Contact damage evolution in diamond-like carbon coatings on ductile substrates. J. Mater. Res. 2008, 23, 27–36. [Google Scholar] [CrossRef]
- Moorthy, V.; Shaw, B.A. An observation on the initiation of micro-pitting damage in as-ground and coated gears during contact fatigue. Wear 2013, 297, 878–884. [Google Scholar] [CrossRef]
- Shahsavari, S.; Desouza, A.; Bahrami, M.; Erik, K.E. Thermal analysis of air-cooled PEM fuel cells. Int. J. Hydrogen Energy 2012, 37, 18261–18271. [Google Scholar] [CrossRef]
- Johnson, K.L. Contact Mechanics; Cambridge University Press: Cambridge, UK, 1985; pp. 171–193. [Google Scholar]
- Wang, T.J.; Wang, L.Q.; Gu, L.; Zheng, D.Z. Stress analysis of elastic coated solids in point contact. Tribol. Int. 2015, 86, 52–61. [Google Scholar] [CrossRef]
- Xu, J.Q.; Mutoh, Y. Analytical solution for interface stresses due to concentrated surface force. Int. J. Mech. Sci. 2003, 45, 1877–1892. [Google Scholar] [CrossRef]
- Hou, P.F.; Jiang, H.Y.; Tong, J.; Xiong, S.M. Study on the coated isotropic thermoelastic material based on the three-dimensional Green’s function for a point heat source. Int. J. Mech. Sci. 2014, 83, 155–162. [Google Scholar] [CrossRef]
- Xu, J.Q. Interface Mechanics; Science Press: Beijing, China, 2006; pp. 70–80. [Google Scholar]
- Grylitsky, D.V.; Pauk, V.J. Some quasistationary contact problem for half-space involving heat generation and radiation. Int. J. Eng. Sci. 1995, 33, 1773–1781. [Google Scholar] [CrossRef]

© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, C.; Gu, L.; Nie, C.; Zhang, C.; Wang, L. Interfacial Mechanics Analysis of a Brittle Coating–Ductile Substrate System Involved in Thermoelastic Contact. Coatings 2017, 7, 21. https://doi.org/10.3390/coatings7020021
Zhang C, Gu L, Nie C, Zhang C, Wang L. Interfacial Mechanics Analysis of a Brittle Coating–Ductile Substrate System Involved in Thermoelastic Contact. Coatings. 2017; 7(2):21. https://doi.org/10.3390/coatings7020021
Chicago/Turabian StyleZhang, Chi, Le Gu, Chongyang Nie, Chuanwei Zhang, and Liqin Wang. 2017. "Interfacial Mechanics Analysis of a Brittle Coating–Ductile Substrate System Involved in Thermoelastic Contact" Coatings 7, no. 2: 21. https://doi.org/10.3390/coatings7020021
APA StyleZhang, C., Gu, L., Nie, C., Zhang, C., & Wang, L. (2017). Interfacial Mechanics Analysis of a Brittle Coating–Ductile Substrate System Involved in Thermoelastic Contact. Coatings, 7(2), 21. https://doi.org/10.3390/coatings7020021





