Next Article in Journal
Icephobic Properties of Superhydrophobic Coatings Developed for Aeronautical Applications
Previous Article in Journal
A Method for Preparing Electrically Conductive SiC Ceramics Through Hot-Press Sintering Followed by Nitrogen Pressure Heat Treatment
Previous Article in Special Issue
Enhanced Fracture Toughness of WC-CoCr Thermally Sprayed Coatings by the Addition of NiCrFeSiBC and Mo and Its Influence on Sliding Wear Behavior
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Coatings
Volume 15
Issue 6
10.3390/coatings15060619
coatings-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Article

Investigation of Steel Chimney Retrofitted with Nanocoating Under Earthquake Excitation Using FEM

by
Sertaç Tuhta
1 and
Furkan Günday
2,*
1
Department of Civil Engineering, Faculty of Engineering, Ondokuz Mayis University, 55139 Samsun, Turkey
2
Department of Civil Engineering, Faculty of Engineering, Giresun University, 28200 Giresun, Turkey
*
Author to whom correspondence should be addressed.
Coatings 2025, 15(6), 619; https://doi.org/10.3390/coatings15060619
Submission received: 30 April 2025 / Revised: 19 May 2025 / Accepted: 20 May 2025 / Published: 22 May 2025
(This article belongs to the Special Issue Characterization and Manufacturing of Nano-Composites and Nano-Composite Coatings)
Browse Figures
Versions Notes

Abstract

:
The aim of this research is to determine the effect of nanocoating retrofitting on the dynamic responses of industrial steel chimneys. A 20 m high industrial steel chimney was selected for nanocoating retrofitting. First, a three-dimensional finite element model of the chimney was created, and modal analysis was performed in SAP2000 software, and frequency values and mode shapes were obtained. Later, linear analyses of the chimney were made using the 1995 Kobe earthquake ground motion recording. Then, the outer surface of the chimney was retrofitted with a 1 mm MgO nanocoating, and dynamic analyses were made. Finally, the chimney’s dynamic responses before and after the MgO retrofit, such as minimum–maximum von Mises stresses and displacements, were compared. At the end of the study, it was observed that the displacements along the chimney height, especially maximum displacements, decreased from 150 mm to 140 mm, and the minimum von Mises stress at the bottom of the chimney decreased from 37.20 kPa to 30.19 kPa, and the maximum von Mises stress from 1519.27 kPa to 1332.33 kPa stresses in the analyses results. The decrease in frequency values revealed that MgO nanocoating had a positive effect on the stiffness of the chimney. It can be understood from this study that nanocoatings will be very useful in retrofitting industrial steel chimneys and have an effect on dynamic parameters.

1. Introduction

Industrial steel chimneys are tall and slender structures that need repair and strengthening in their service lives. Today, nanocoatings are becoming a popular material used for the retrofitting of steel structures. Steel chimneys are industrial structures that remove waste gases from factories. Their heights, geometries and dimensions vary according to the needs of the facilities. Industrial chimneys are made of masonry, steel, reinforced concrete and composite materials [1,2,3]. As the need for high chimneys increased, those produced from steel and its alloys came to the fore. With developing technology, very important progress has been made in the field of corrosion. The fact that they are more resistant to external forces such as earthquakes and wind also causes them to be frequently preferred [4]. As with other materials, steel chimneys need repair or strengthening throughout their service life to maintain adequate ductility over time [5]. Quite different methods are used for the repair and strengthening of structures of this type. Conventionally, one of the most frequently used methods are operations that address the entire chimney, as well as intervening in parts that require repair. The most used of these processes involves as intervening in the parts requiring repair by using FRP-based materials and if there is no structural obstacle, supporting the section with rings made of steel or composite materials [6,7,8,9]. In study [10], samples representing RC chimneys were produced and tested under increasing horizontal monotonic loads and evaluated in terms of carrying capacity, crack formation, collapse mechanism, curvature ductility, moment capacity and energy absorption capacity. The test results showed that there was an average increase of 11.5% in the samples without FRP and 27.6% in the samples with FRP wrapping compared to the theoretical moment capacity. However, sudden collapse occurred in the samples wrapped with FRP, which revealed the need for additional design and application developments in such applications. In reference [11], the Chi-Chi earthquake in Taiwan in 1999 caused serious structural damage to a 250 m high chimney located in the central part of the country. In this study, experimental and numerical analysis methods were used together to evaluate the dynamic behavior, collapse mechanism and seismic performance of the chimney after repair. The dynamic properties of the chimney were determined by ambient vibration tests and system identification methods; the instrumental variable subspace approach was applied in this process. In addition, the three-dimensional dynamic behavior of the chimney was modeled with the finite element method using SAP2000 V26 software. The buckling capacity of the structure was evaluated according to the ASCE 1975 standard [12], and the calculated stress values were found to be consistent with the damage observed in the steel flues. The obtained findings demonstrate the reliability of the modeling and provide important information about the seismic performance of the chimney. On the other hand, it is seen that the use of nanomaterials, which are the result of working with developing technology, gives very effective results. Similarly, the use of FRP-based materials is highly preferred for retrofitting [13,14,15,16,17]. According to the seismic performance level required in the chimney, increasing the cross-section, supporting it with ring-like elements from the inside or outside, adding dampers, using FRP and using nanomaterials are among the methods that give effective results in retrofitting [18,19,20,21,22,23]. Today, in addition to conventional applications, various new methods have emerged with the widespread use of nanotechnological materials in the field of civil engineering. While these methods are based on the direct use of nanomaterials, it has also become possible to apply structural elements produced from these materials. It is difficult to make a broad definition, as nanotechnology, the foundations of which were laid approximately 50 years ago, is developing very rapidly. However, according to the definition agreed upon in every branch of science, nanotechnology is the manufacture of materials and products by controlling matter with its most fundamental particle (nanocoatings, nanoparticles, etc.). At the atomic, molecular or nanoscale levels, it can be expressed as the use of very small particles of material to create new large-scale materials. Nanotechnology is defined as the study and application of structures ranging in size from 1 nanometer (nm) to 100 nanometers. During nano-level processing, various problems arise such as gravity becoming negligible, electrostatic forces and quantum effects. Another point to be noted is that the use of nano-scale particles causes the material properties to change as a result of the increase in the ratio of atoms on the surface to those inside. Nanotechnology can produce materials with many unique properties that can improve existing building materials, such as lighter and stronger structural composites, low-maintenance building elements, fabrication in complex geometric shapes, and products with better thermal insulation properties. The incorporation of nanomaterials into the composition of some materials such as concrete, steel and composites will benefit air conditioning and energy efficiency. In addition, nanomaterials applied to the surfaces of building structural elements contribute to the elimination of structural deficiencies. Thanks to nanotechnology, concrete with a high strength, steel that can be made more durable and easier to assemble and wooden elements with a long durability can be produced. Due to the new potential applications of nanometer-scale particles, nanomaterials and nanotechnologies have become topics of great scientific interest. The construction sector lags behind other major industrial sectors in terms of the potential to benefit from nanotechnology. In addition to the use of nanotechnology in construction materials, today’s popular topics regarding the potential uses of nanotechnology in the construction and development of construction materials are adding nanoparticles to increase the strength and durability of cement composites, using carbon nanotubes and nanofibers, producing low-cost and corrosion-resistant steel and the like. The use of nanotechnological materials in buildings not only prolongs their service life but also provides extra protection against various factors such as energy saving, fire resistance and corrosion. There are studies showing that the performance of building elements produced using materials such as cement-based composites, concrete, mortar, etc., is increased in terms of strength with the addition of nanomaterials [24,25,26,27,28]. In recent years, developments in structural engineering have made it possible to build bold structures with record spans and heights. However, significant challenges are encountered in the construction of such structures, such as the limitations of traditional materials and the need for continuous monitoring under complex dynamic loads. Considering the responsibilities for human life and the budget of the structures, continuous monitoring is inevitable to ensure the safe and efficient operation of structures. In this context, in the field of structural engineering, smart structures and materials with self-monitoring properties stand out among the most promising solution proposals. Although it is an area that has not yet been fully explored, current research reveals that composites reinforced with carbon-based nanomaterials have great potential as structural materials with a self-sensing capacity. Nanomaterials significantly improve the microstructure, mechanical properties and load-related properties of structural materials, thus increasing the durability and performance of structures. Therefore, the use of nanomaterials in structural engineering has become an important research topic in areas such as mechanical and electrical properties and durability [29]. Current nanoscience and nanotechnology researchers are examining these new features in depth. Because macro properties can change at the nanoscale, a significant number of new materials and processes can be created. Discussions regarding the application of nanotechnology in civil engineering, especially in construction, are critical. However, many advances in nanotechnology have the potential to be applied in the field of civil engineering. By using nanotechnological materials, various negative effects (via self-healing and waterproof concrete, self-cleaning plaster, etc.) that occur over time on building elements can be prevented. According to the studies reviewed, carbon nanotubes, one of the most widely used nanomaterials in civil engineering, are cylindrical carbon tubes that take their name from their nanometer diameter. They can be several millimeters long and have one “layer” or wall (single-walled nanotubes) or multiple walls (multi-walled nanotubes). Nanotubes have exceptional strength, unique electrical properties and efficient thermal properties. They are extremely popular because of their properties. Magnesium oxide nanoparticles, another nanomaterial especially for heat resistance, are also used to improve the properties of building elements. Most of the literature studies are on the reinforcement of reinforced concrete bridges, water tanks and chimneys with FRP-based composites. Studies on steel structures have generally focused on silos and water tanks. It is known that there are a limited number of studies on wood and other material types. It has been observed that studies in the field of civil engineering have emerged in the last ten years on nanotechnology. The majority of these studies are on the incorporation of nanoparticles into material structures and its consequences. Studies directly on the use of nanocoatings in civil engineering structures are limited [30]. It has been determined that there are reasons, such as the development of technology and the lack of application possibilities, for the small number of studies. In article [31], the dynamic behavior of glass fiber-reinforced epoxy nanocomposites was investigated. In the study, the effects of nanoclay and nanosilica additives on the mechanical properties and natural frequencies of epoxy composites were analyzed. It was observed that nanosilica and nanoclay additives added at different rates improved the dynamic properties of the composites and increased the mechanical performance of the material. In particular, it was found that 1% nanosilica additive increased the natural frequencies, but 3% nanoclay additive did not provide a significant improvement. These findings show that glass fiber-reinforced epoxy nanocomposites can be used as an effective reinforcement material to become more durable under dynamic loads and vibrations. The study aims to increase the potential of using such materials in future engineering applications by determining the effect of nanomaterials on epoxy-based composites [31]. In study [32], it was investigated how the 3D nanofiber network structure formed using bacterial cellulose and protonated chitosan improved the mechanical and thermal properties of natural fiber nanocomposites. The results showed that this structure increased the durability and flexibility of the composites [32].
This study provides an economic evaluation of the use of nanomaterials in concrete structures in the construction industry. The effective material properties of nanocomposite concrete were determined using the Halpin–Tsai micromechanical theory, and the effects of nanoparticles on mechanical properties such as elastic modulus, compressive strength and reinforcement percentage were investigated. The role of nanoparticles in the overall cost structure of concrete was analyzed using Euler theory and Hamilton’s principle. The results showed that increasing the volume percentage of nanoparticles up to 1% increased the elastic modulus by 78%, while reducing the reinforcement ratio by 142%. In economic terms, it was found that the increase in the cost of concrete by adding nanoparticles was approximately USD 1 per ton, but this cost increase was quite low compared to the mechanical improvements provided [33]. MgO coating can be applied by different methods. These methods are generally thermal spray (plasma or flame spray) application, slurry spray application, and Application as a Refractory Coating (similar to Shotcrete). The thermal spray method is applied by melting or semi-melting MgO powder at high temperatures and spraying it onto the surface. The thermal spray method has the advantages of creating a protective layer resistant to high temperatures, being chemically inert, providing resistance to corrosion and being able to obtain a solid coating similar to ceramics. However, it has the disadvantages of the high melting point of MgO and therefore requiring advanced techniques such as plasma spraying and requiring controlled cooling to prevent the sprayed layer from cracking. Spray application in slurry form consists of mixing MgO powder with water and binders and applying this suspension with a spray gun. The slurry spray method has advantages such as low equipment costs and being used for refractory surface repair or internal coating. However, it has disadvantages such as requiring drying and subsequent sintering (high-temperature cooking) and affecting the quality of the application in terms of the binder selection and particle size. In the application method of Application as a Refractory Coating, MgO can be sprayed in a similar way to refractory concrete. It is especially preferred in industrial chimneys requiring a high temperature and chemical resistance (e.g., cement, metallurgy). In addition, in MgO coating applications, special attention should be paid to cleaning and roughening the surface before spraying, selecting the appropriate binder (e.g., silicates, alumina additives) for the good adhesion of MgO to the surface, ensuring slow cooling against the risk of cracking after the application, and preventing MgO from reacting with moisture and turning into Mg(OH)2, which causes volumetric expansion and cracking [34,35,36]. Study [37] investigates the gas–liquid reaction of a Mg(OH)2 suspension with SO2. The effects of different operating parameters are investigated and a mass transfer model is developed. Th experimental results show that gas–film diffusion is the controlling mechanism of the desulfurization process. The article [38] focuses on the microstructure and biodegradable corrosion properties of Mg/MgO composite coatings by high-velocity suspension flame spraying (HVSFS). It is especially important for biomedical and environmentally friendly engineering applications.
The aim of this study is to fill a gap in the literature on the retrofitting of industrial steel chimneys with nanocoatings (MgO), which has been identified in the reviewed studies, and to compare the dynamic responses of such structures before and after reinforcement with nanocoatings. For this purpose, an industrial steel chimney with a height of 20 m, a diameter of 1 m and a thickness of 0.01 m was chosen for the reinforcement application. First, a three-dimensional (3D) finite element model (FEM) of the chimney was developed in SAP2000 software [39] using existing solid elements [40,41,42]. The 3D finite element model of the selected chimney has been validated using studies in the literature. Then, the FEM model of the steel chimney was analyzed using the ground motion of the 1995 Kobe earthquake, in which a large number of industrial facilities were severely damaged. Afterwards, the chimney body was reinforced with 0.001 m thick MgO to cover the entire outer body from bottom to top, and dynamic analyses were performed with the same ground motion mentioned above. Finally, chimney dynamic responses before and after MgO retrofitting, such as displacement, von Mises stress values, frequency and mode shapes, were compared.

2. Description of the Steel Chimney

An industrial steel chimney with a total height of 20 m was selected for the dynamic analysis before and after MgO retrofitting. The outer diameter of the chimney is 1.00 m. The wall thickness of the chimney is 0.01 m. The general structural properties of the chimney are shown in Figure 1.

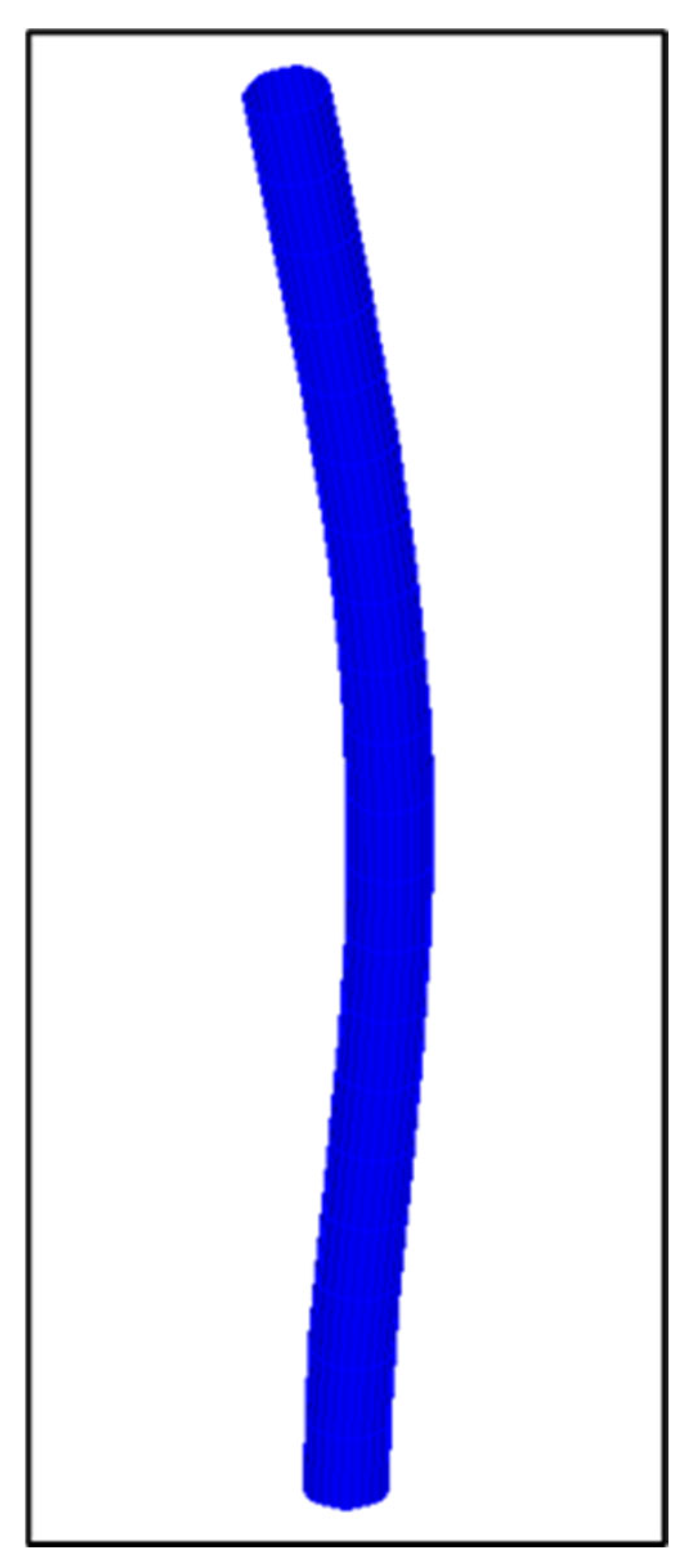
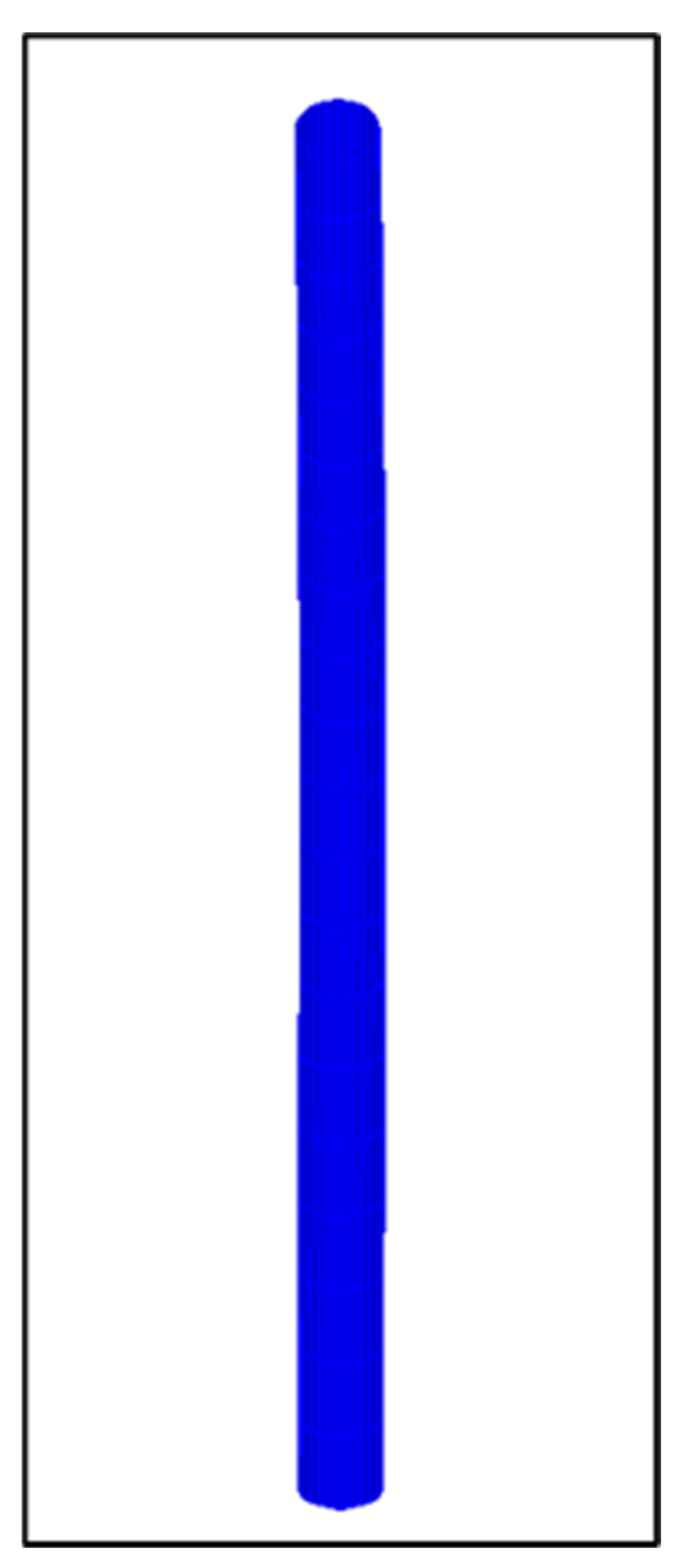
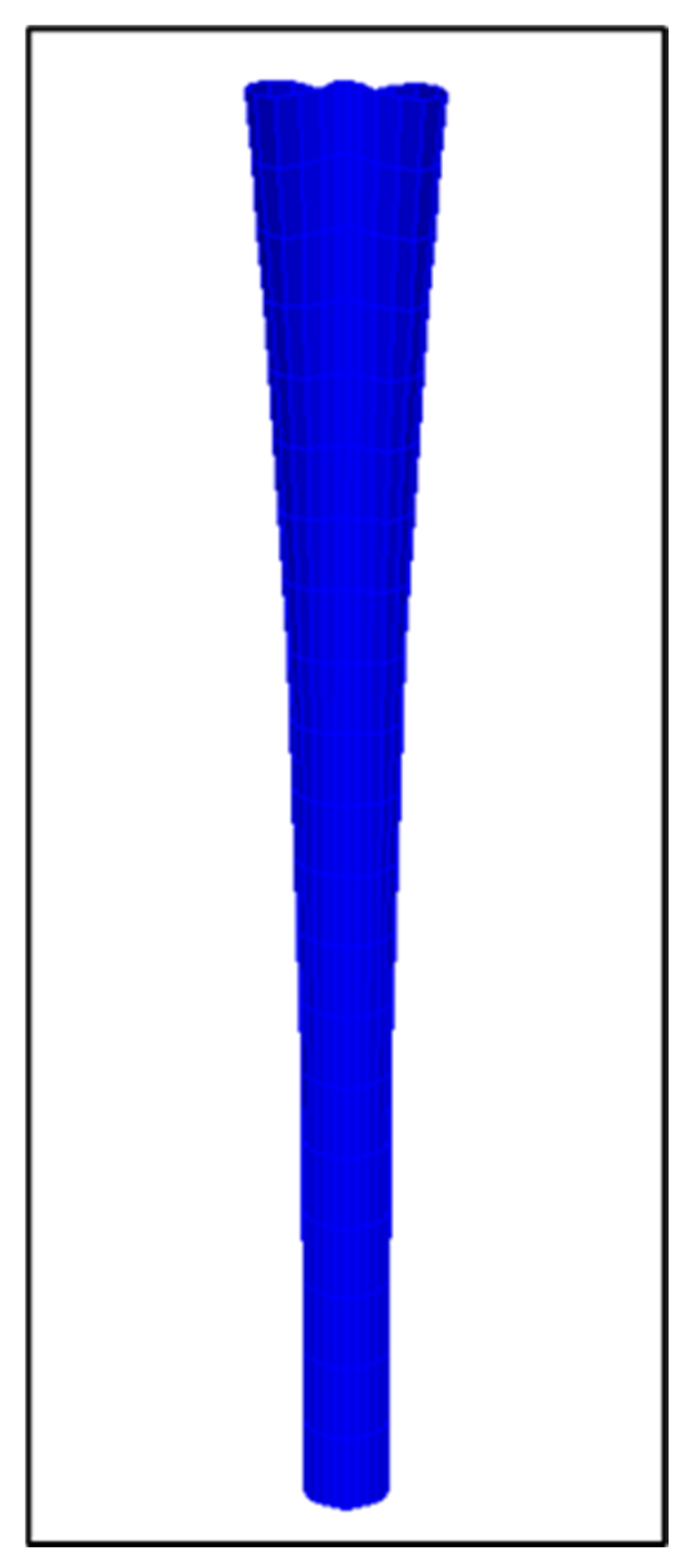
3. Finite Element Modeling of the Chimney

SAP2000 software [29] was used to create three-dimensional finite element models of the chimney before and after retrofitting with MgO. This software is used for linear, non-linear, static and dynamic analysis of 3D structures [43,44,45,46,47]. In modeling, analytical solutions yield accurate results as long as the mesh size is properly specified. Convergence studies were carried out to determine the best mesh size in steel chimney models. The 3D FEM of the selected chimney was verified using the studies in the literature. In the finite element modeling of the chimney model, a four-node shell element is used. Each shell element has nine nodes, and each node has six degrees of freedom, namely translations in the x, y and z axes and rotations about the same axes in Figure 2.
Figure 2 depicts the shape and node positions for this element type. A layered shell element was used to model being coated with MgO. This element allows numerous different material layers, with different orientations and orthotropic material properties in each layer. This element can be used to model layered thick shells or solids. A schematic view of the shell element is shown in Figure 2. For thin and tall structures like chimneys, the characterization of material properties and boundary conditions, which must be taken into account in the analytical study, is critical. In the analytical model, the linear elastic material properties of steel and MgO were assumed. The chimney was built out of steel. For steel, SAP2000 software requires input data for materials such as modulus of elasticity, Poisson’s ratio and unit weight. In Table 1, the elasticity modulus, Poisson’s ratio and mass per unit volume of non-alloy structural steel are given [48,49].
Nanomaterials can be used to strengthen reinforced concrete, masonry structures, steel and wood in the event of flexural and shear stress due to increased loading capacity, changes in building utilization, defect correction, seismic protection and compliance with revised standards. For the retrofitting process of the chosen chimney, MgO nanocoating with the material quality shown in Table 2 was employed [50,51,52,53].
After applying the ideal meshing size to the chimney, it was discovered that the total length of linear transient analyses and total volume were extremely high, making visualization of any output impossible. As a result, the meshing size was increased to a manageable level in order to simplify the analysis and output process. A total of 1920 shell elements and 1968 nodes were utilized in the analysis at the conclusion of these studies.
When compared to the thickness of the chimney wall (0.01 m), the thickness of the MgO retrofitting (0.001 m) was thin. In the retrofitting of the model, the outer surface of the chimney was covered with a single layer of MgO with a thickness of 1 mm. The MgO layer covered the entire chimney body from bottom to top. In the literature review, it was determined that a 1 mm thick MgO layer was sufficient for reinforcement. Tensile and compressive stiffness behaviors were considered for the elements. Also, the one-way fiber direction (zero degree) was considered in this study. All degrees of freedom at the base of the chimney are assumed to be connected to the ground, and it is assumed that the chimney is fixed to the ground. In other words, in both finite element models, the boundary conditions are assumed to be fixed at the base. The adjacent nodes between the MgO layered shell elements and the steel elements are interconnected to provide the assumption of perfect bonding. In this way, it is ensured that the shell elements (steel and MgO) share the same nodal points. In addition, the MgO layered shell elements and the steel shell elements are split in the same mesh size to achieve the perfect interaction described above. In this study, modal and seismic (time-history analysis) were the used load cases for the dynamic analysis. The finite element model of the chimney and the meshing before and after MgO reinforcement are shown in Figure 3 and Figure 4.
In essence, industrial chimneys are thin structures with a very homogeneous mass distribution. Thus, the higher mode effects have a significant impact on these towers’ dynamic behavior. In the time-history studies, all modes having a total modal mass participation of at least 90% were taken into account. Modal analyses were carried out to determine the frequencies and mode shapes of the chimney. The modal analysis was performed with dynamic response theories. The first five frequencies of the chimney before MgO retrofitting were obtained in the range of 2.50–7.14 Hz. In Figure 5, Figure 6, Figure 7, Figure 8 and Figure 9, the first five mode shapes and frequencies of the chimney obtained from the modal analyses are illustrated.
The first five frequencies of the chimney after MgO retrofitting were obtained in the range of 2.63–11.11 Hz. In Figure 10, Figure 11, Figure 12, Figure 13 and Figure 14, the first five mode shapes and frequencies of the chimney obtained from modal analyses are illustrated.
From Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12, Figure 13 and Figure 14, it can be seen that the same mode shapes were obtained in the modal analyses before and after MgO retrofitting.
A comparison of frequency differences in the first five modes before and after MgO retrofitting is given in Table 3.
When the changes in natural frequencies in the first five modes were compared before and after retrofitting with MgO, it was observed that the natural frequency increased by 0.13 Hz after retrofitting in the 1st Mode. This increase corresponds to approximately 5.2% compared to the pre-retrofitting situation. When the changes in the 1st Mode shapes were examined, no change was observed in the directions of the mode shapes. In both cases, the mode shape is the translational mode. According to these findings, it is understood that there is an increase in stiffness after retrofitting.
It was observed that the natural frequency increased by 0.18 Hz after retrofitting in the 2nd Mode. This increase corresponds to approximately 6.32% compared to the pre-retrofitting situation. When the changes in the 2nd Mode shapes were examined, no change was observed in the directions of the mode shapes. In both cases, the mode shape is the translational mode. According to these findings, it is understood that there is an increase in stiffness after retrofitting.
It was observed that the natural frequency increased by 0.40 Hz after retrofitting in the 3rd Mode. This increase corresponds to approximately 11.63% compared to the pre-retrofitting situation. When the changes in the 3rd Mode shapes were examined, no change was observed in the directions of the mode shapes. According to these findings, it is understood that there is an increase in stiffness after retrofitting.
It was observed that the natural frequency increased by 1.01 Hz after retrofitting in the 4th Mode. This increase corresponds to approximately 22.25% compared to the pre-retrofitting situation. When the changes in the 4th Mode shapes were examined, no change was observed in the directions of the mode shapes. In both cases, the mode shape is the torsional mode. According to these findings, it is understood that there is a significant increase in stiffness after retrofitting.
It was observed that the natural frequency increased by 3.97 Hz after retrofitting in the 5th Mode. This increase corresponds to approximately 55.60% compared to the pre-retrofitting situation. When the changes in the 5th Mode shapes were examined, no change was observed in the directions of the mode shapes. In both cases, the mode shape is the torsional mode. According to these findings, it is understood that there is a serious increase in stiffness after retrofitting.

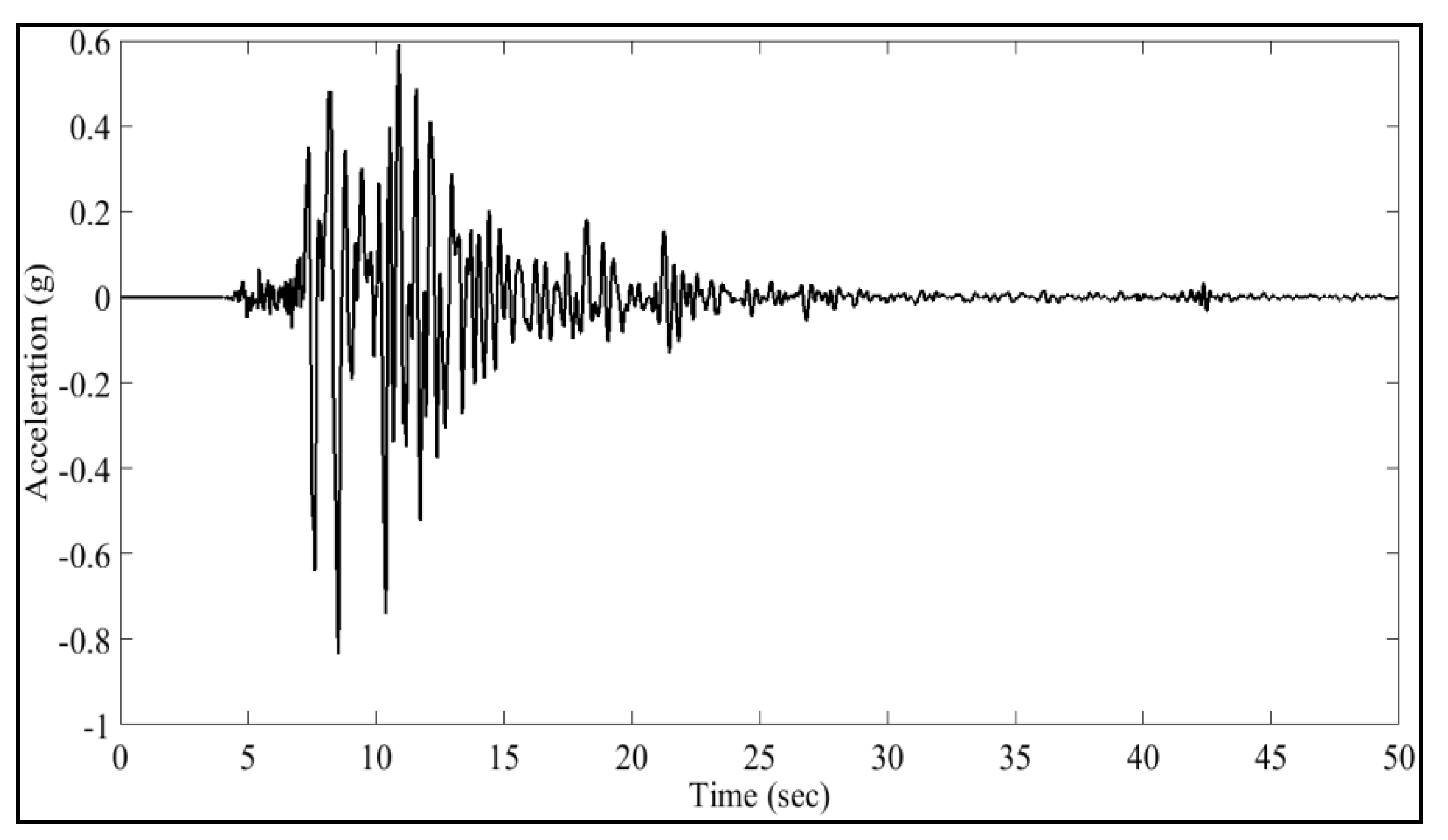
4. Dynamic Analyses of the Chimney

Linear transient analyses of chimney before and after MgO retrofitting were carried out using the ground acceleration values of the north–south component of the Kobe earthquake that occurred on 17 January 1995 (a total length of 50 s with equal intervals of 0.02 s). The time history of ground motion acceleration of the 1995 Kobe earthquake is given in Figure 15.
Rayleigh damping constants are calculated assuming a 2% damping ratio between the 1st Mode and the 5th Mode of the chimney [41,54,55,56]. Maximum horizontal displacement values under the effect of the Kobe earthquake are shown in Figure 16 and Figure 17.
It is clearly seen in the figure that the application of MgO to the chimney reduces the maximum displacement values along the height. The maximum upper displacement value, which was 150 mm before the application of MgO to the chimney, decreased to 140 mm after the application of MgO. The reduction in maximum top displacements was about 6.66% without much additional mass to the structure. As can be seen from the contour diagrams of the maximum horizontal displacement, the maximum displacements occurred at the top of the chimney.
The maximum and minimum von Mises stress contours of the chimney exposed to the Kobe earthquake are presented in Figure 18 and Figure 19.
As shown in Figure 18 and Figure 19, the maximum and minimum von Mises stresses obtained before MgO retrofitting are higher than those obtained after MgO reinforcement. In other words, it is seen from Figure 18 and Figure 19 that there is a decrease in the minimum and maximum principal stresses after MgO reinforcement. Also, there is a reduction in von Mises stresses occurring at the base of the chimney after MgO retrofitting. It can be said that MgO retrofitting is effective in reducing the minimum and maximum von Mises stresses occurring on the thin wall of the chimney.

5. Conclusions

In this study, the aim was to investigate the dynamic behavior of industrial steel chimneys before and after MgO retrofitting. A 3D finite element model of an industrial steel chimney was created in SAP2000 software and analyzed using the north–south acceleration component of the 1995 Kobe earthquake. Some of the results obtained from this study can be summarized as follows:
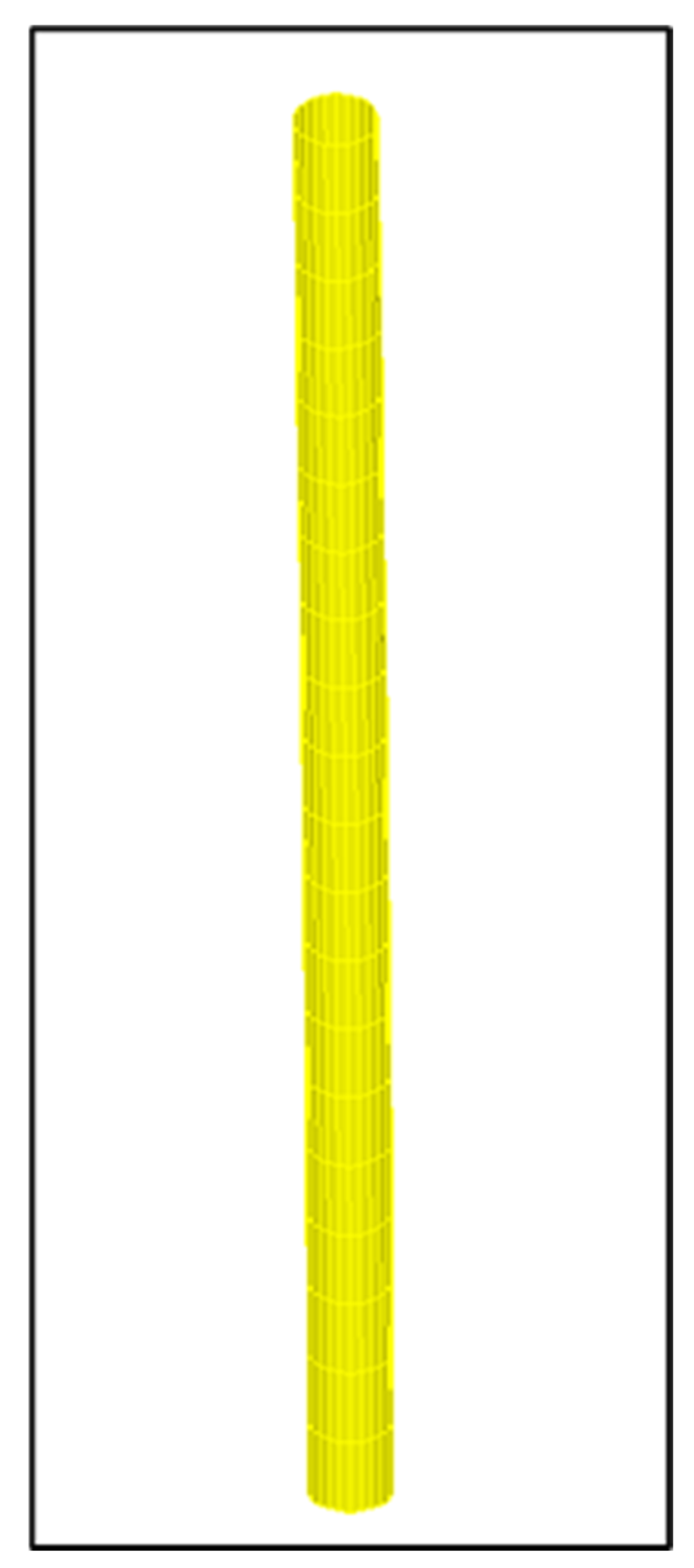
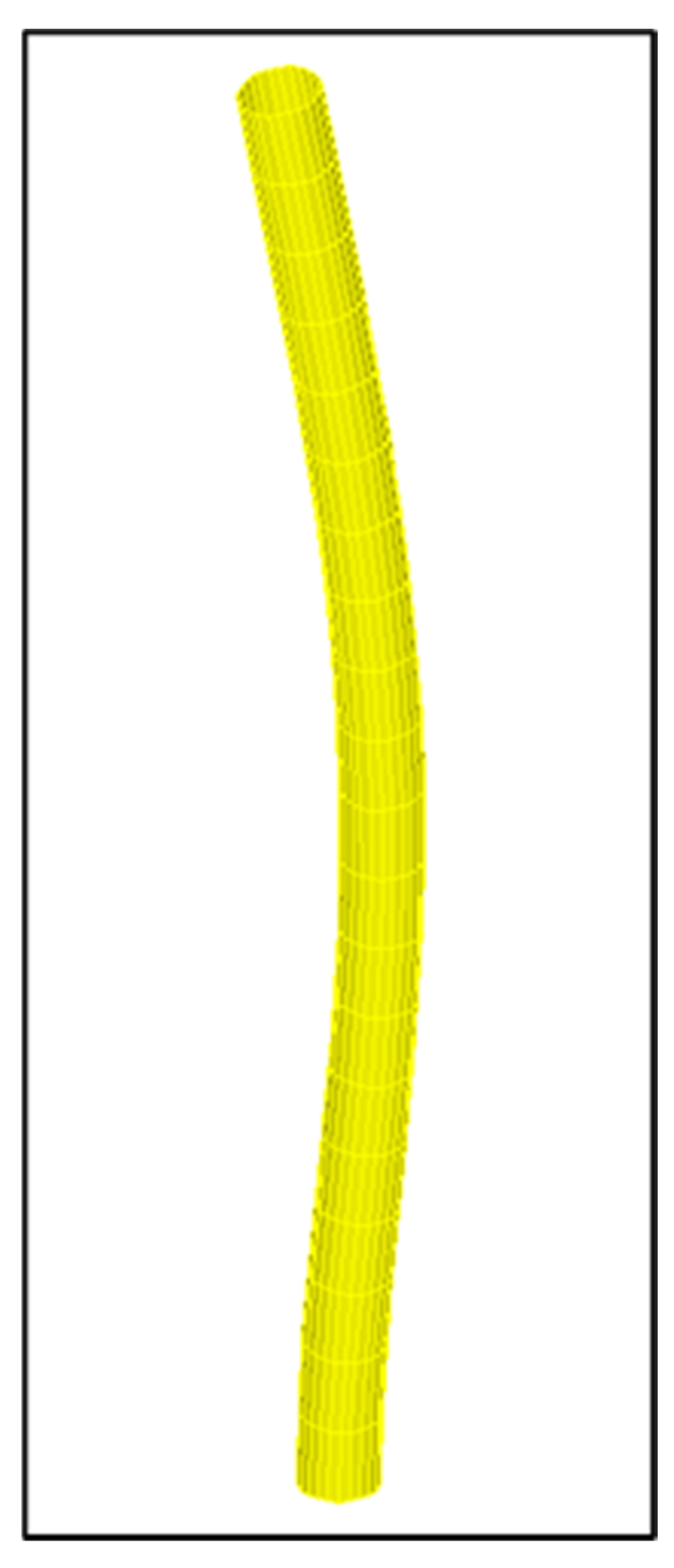
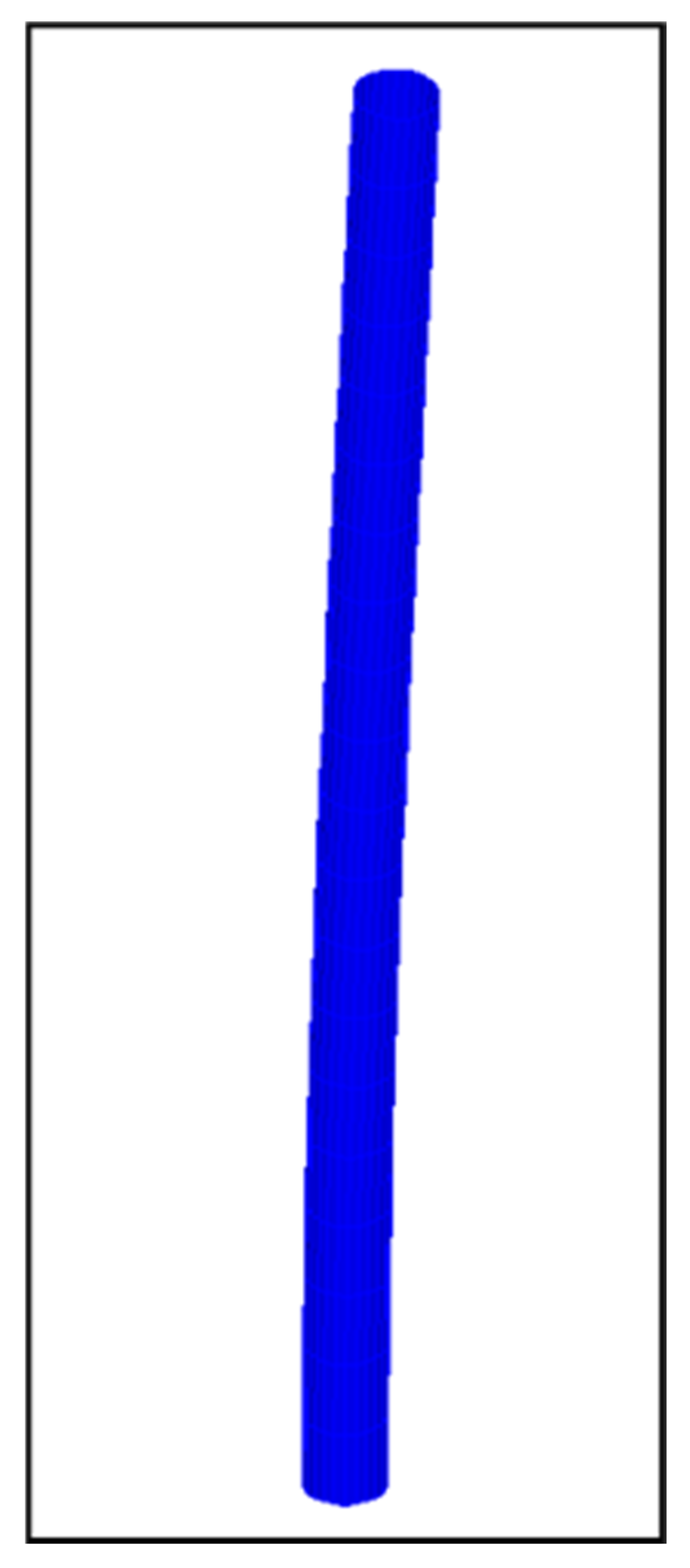
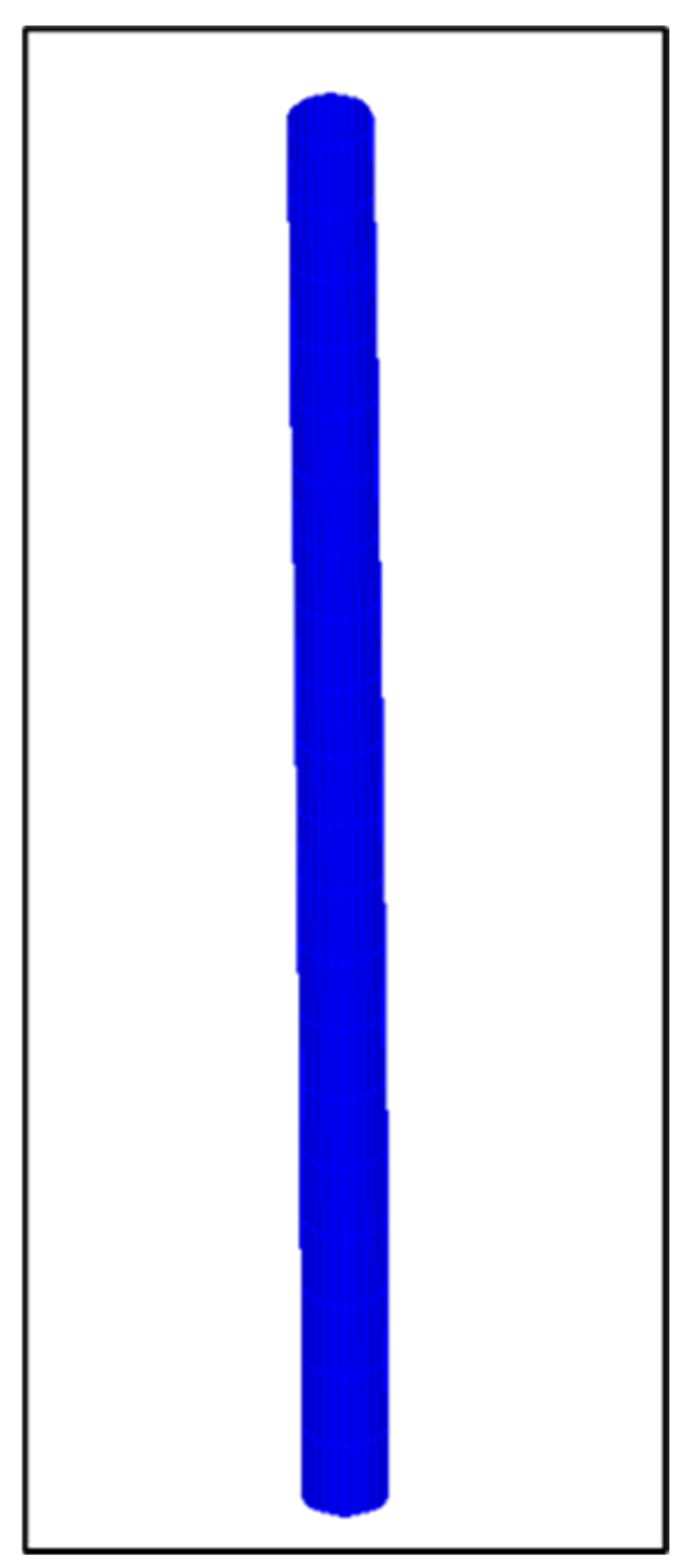
  • From the chimney modal analysis, no changes were observed in the modal shapes before and after retrofitting with MgO. A total of five frequencies were obtained in the range of 2.50–7.14 Hz and 2.63–11.11 Hz, respectively, before and after retrofitting with MgO. It has been observed that MgO increases the rigidity of the structural system;
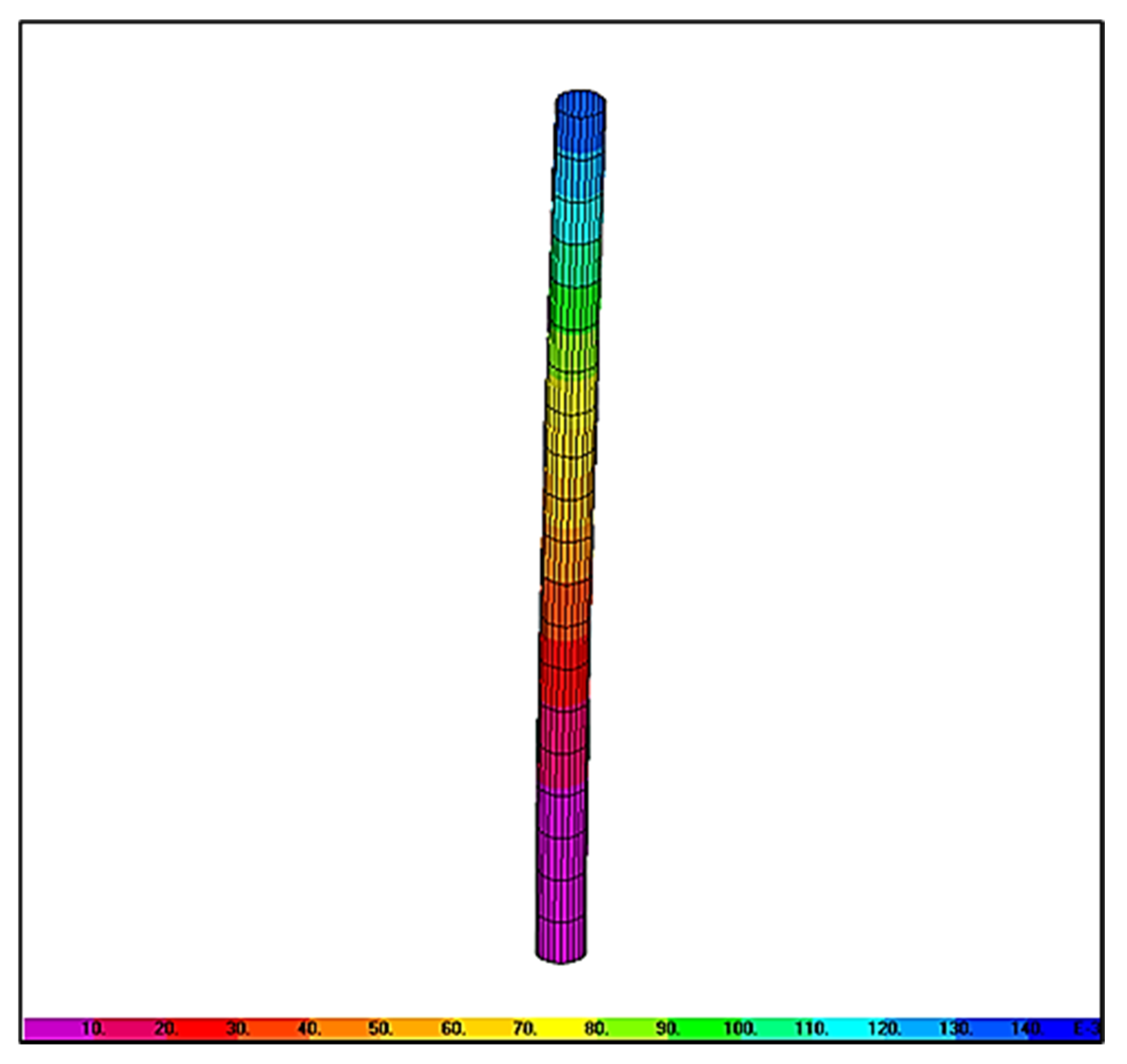
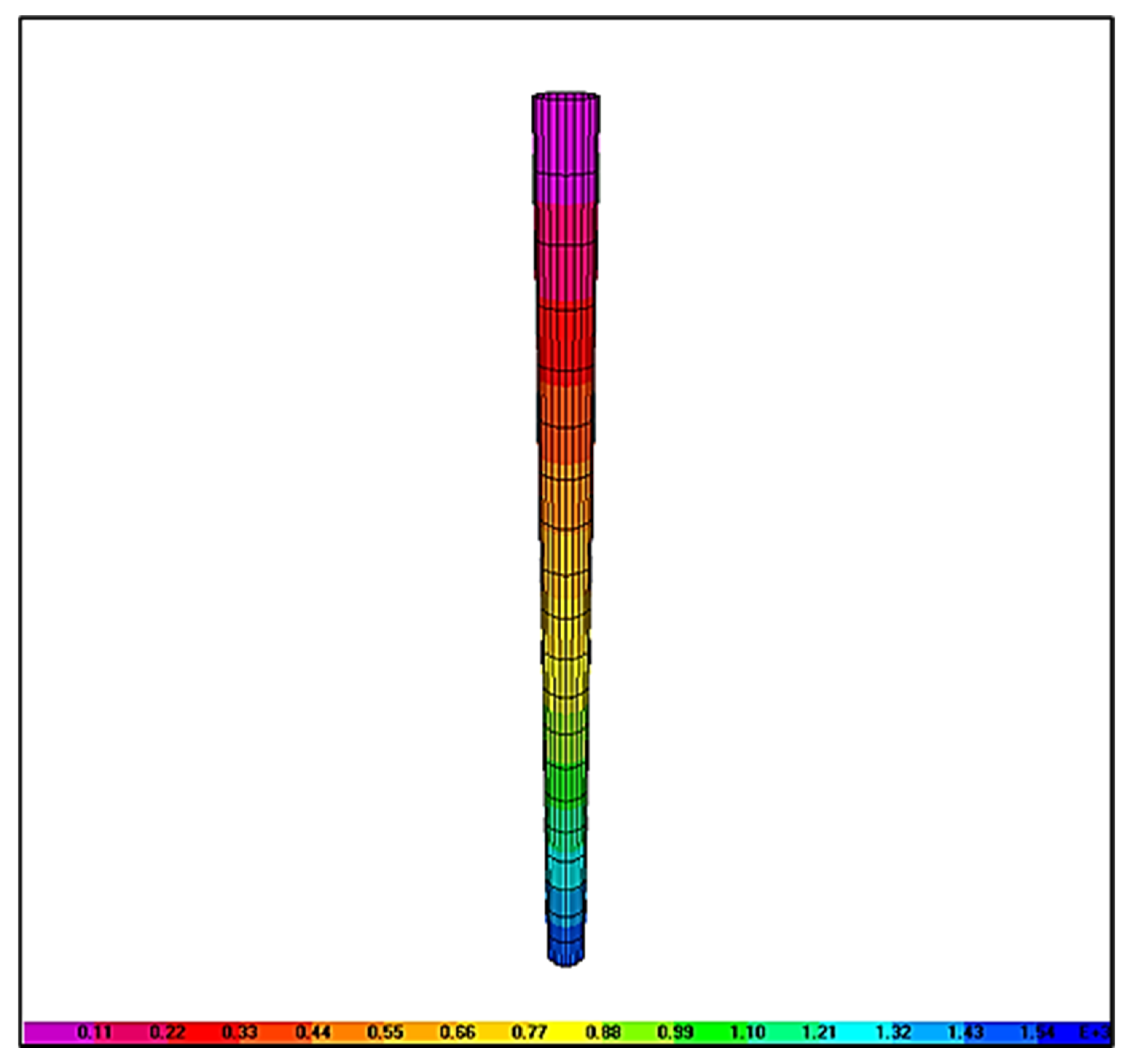
  • For both analyses, horizontal displacement values increased from bottom to top along the height of the chimney. Maximum displacements (top) were obtained before and after MgO reinforcement at 150 and 140 mm, respectively. There was an approximate 6.66% reduction in horizontal displacement without adding too much mass to the structural system, which is a superior advantage over traditional reinforcement techniques for industrial steel chimneys;
  • For both analyses, the maximum and minimum von Mises stresses formed at the bottom of the chimney. After MgO retrofitting, the minimum von Mises stress at the bottom of the chimney decreased from 37.20 kPa to 30.19 kPa, and the maximum von Mises stress from 1519.27 kPa to 1332.33 kPa. From these stress observations, it can be concluded that MgO retrofitting has an effect on stress values. Particular attention should be paid to the points where the chimneys meet the ground. With the application of MgO, the stress distribution on the chimney surface can be reduced to tolerable values without adding much additional mass to the system. This gives it a significant advantage over other traditional retrofitting techniques;
  • From the displacement and von Mises stress results obtained, it can be said that MgO increases the stiffness of the structural system (increasing frequency values). More rigidity means fewer cracks; fewer cracks lead to less corrosion and stress concentrations, resulting in less maintenance and repair costs to the user;
  • It can be seen from the study that nanocoatings are effective for the dynamic response of industrial steel chimneys and should be preferred as a new and good technique in the maintenance, repair and retrofitting of industrial steel chimneys. Considering the advantages of nanocoating such as a high strength/weight ratio, high corrosion resistance, easy application to all surfaces, long life and high fire resistance, it can be said that such materials will replace traditional retrofitting techniques in the near future;
  • Nanocomposites are slowly becoming a popular retrofitting technique among other traditional techniques. In this respect, a large number of studies should be carried out to expand the literature on similar materials;
  • In this study, a review of retrofitting a steel chimney with MgO is presented. Although the results and findings of the study belong to a specific example of steel chimney retrofitting, the methods used in this study can be applied to many steel chimneys in daily life. This study is a pioneer in demonstrating the effect of nanocoating reinforcement on the dynamic response of steel industrial chimneys;
  • In future studies, experimental studies are recommended to verify the effectiveness of MgO nanocoating in field applications and to investigate long-term durability issues such as adhesion loss, environmental effects and coating degradation that may occur over time.

Author Contributions

Conceptualization, S.T.; Methodology, S.T.; Software, S.T.; Validation, F.G.; Formal analysis, F.G.; Investigation, S.T.; Resources, S.T.; Data curation, F.G.; Writing—review and editing, F.G. All authors have read and agreed to the published version of the manuscript.

Funding

This research received no external funding.

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

Data are contained within the article; further inquiries can be directed to the authors.

Conflicts of Interest

The authors declare no conflicts of interest.

References

  1. Karaca, Z.; Türkeli, E.; Günaydın, M.; Adanur, S. Dynamic Responses of Industrial Reinforced Concrete Chimneys Strengthened with Fiber-Reinforced Polymers. Struct. Des. Tall Spec. Build. 2015, 24, 228–241. [Google Scholar] [CrossRef]
  2. Tuhta, S. OMA of Model Chimney Using Bench-Scale Earthquake Simulator. Earthq. Struct. 2019, 16, 321–327. [Google Scholar] [CrossRef]
  3. Tuhta, S.; Günday, F.; Aydın, H. Subspace Identification Using N4SID Methods Applied to Model Concrete Chimney. JournalNX 2020, 6, 415–423. [Google Scholar]
  4. Karaca, Z.; Türkeli, E. Determination and Comparison of Wind Loads for Industrial Reinforced Concrete Chimneys. Struct. Des. Tall Spec. Build. 2012, 21, 133–154. [Google Scholar] [CrossRef]
  5. López-Patiño, G.; Adam, J.M.; Gimeno, P.V.; Milani, G. Causes of Damage to Industrial Brick Masonry Chimneys. Eng. Fail. Anal. 2017, 74, 188–201. [Google Scholar] [CrossRef]
  6. Meier, U. Strengthening of Structures Using Carbon Fibre/Epoxy Composites. Constr. Build. Mater. 1995, 9, 341–351. [Google Scholar] [CrossRef]
  7. Kobatake, Y. A Seismic Retrofitting Method for Existing Reinforced Concrete Structures Using CFRP. Adv. Compos. Mater. 1998, 7, 1–22. [Google Scholar] [CrossRef]
  8. Zhang, S.; Ye, L.; Mai, Y.W. A Study on Polymer Composite Strengthening Systems for Concrete Columns. Appl. Compos. Mater. 2000, 7, 125–138. [Google Scholar] [CrossRef]
  9. Mirmiran, A.; Zagers, K.; Yuan, W. Nonlinear Finite Element Modeling of Concrete Confined by Fiber Composites. Finite Elem. Anal. Des. 2000, 35, 79–96. [Google Scholar] [CrossRef]
  10. Van Den Einde, L.; Zhao, L.; Seible, F. Use of FRP Composites in Civil Structural Applications. Constr. Build. Mater. 2003, 17, 389–403. [Google Scholar] [CrossRef]
  11. Chen, C.H. Seismic Assessment of a Retrofitted Chimney by FEM Analysis and Field Testing. Int. J. Struct. Stab. Dyn. 2004, 4, 337–359. [Google Scholar] [CrossRef]
  12. ASCE/SEI 7-75; Minimum Design Loads for Buildings and Other Structures. American Society of Civil Engineers: Reston, VA, USA, 1975.
  13. Matthys, S.; Toutanji, H.; Taerwe, L. Stress–Strain Behavior of Large-Scale Circular Columns Confined with FRP Composites. J. Struct. Eng. 2006, 132, 123–133. [Google Scholar] [CrossRef]
  14. Fawzia, S.; Al-Mahaidi, R.; Zhao, X.L.; Rizkalla, S. Strengthening of Circular Hollow Steel Tubular Sections Using High Modulus CFRP Sheets. Constr. Build. Mater. 2007, 21, 839–845. [Google Scholar] [CrossRef]
  15. Rougier, V.C.; Luccioni, B.M. Numerical Assessment of FRP Retrofitting Systems for Reinforced Concrete Elements. Eng. Struct. 2007, 29, 1664–1675. [Google Scholar] [CrossRef]
  16. Zhao, X.L.; Zhang, L. State-of-the-Art Review on FRP Strengthened Steel Structures. Eng. Struct. 2007, 29, 1808–1823. [Google Scholar] [CrossRef]
  17. Pallarés, F.J.; Ivorra, S.; Pallarés, L.; Adam, J.M. Seismic Assessment of a CFRP-Strengthened Masonry Chimney. Proc. Inst. Civ. Eng. Struct. Build. 2009, 162, 291–299. [Google Scholar] [CrossRef]
  18. Uomoto, T.; Mutsuyoshi, H.; Katsuki, F.; Misra, S. Use of Fiber Reinforced Polymer Composites as Reinforcing Material for Concrete. J. Mater. Civ. Eng. 2002, 14, 191–209. [Google Scholar] [CrossRef]
  19. Wu, G.; Wang, H.T.; Wu, Z.S.; Liu, H.Y.; Ren, Y. Experimental Study on the Fatigue Behavior of Steel Beams Strengthened with Different Fiber-Reinforced Composite Plates. J. Compos. Constr. 2012, 16, 127–137. [Google Scholar] [CrossRef]
  20. Yang, J.Q.; Smith, S.T.; Feng, P. Effect of FRP-to-Steel Bonded Joint Configuration on Interfacial Stresses: Finite Element Investigation. Thin-Walled Struct. 2013, 62, 215–228. [Google Scholar] [CrossRef]
  21. Hu, L.; Feng, P.; Zhao, X.L. Fatigue Design of CFRP Strengthened Steel Members. Thin-Walled Struct. 2017, 119, 482–498. [Google Scholar] [CrossRef]
  22. Kasimzade, A.A.; Tuhta, S. OMA of Model Steel Structure Retrofitted with CFRP Using Earthquake Simulator. Earthq. Struct. 2017, 12, 689–697. [Google Scholar] [CrossRef]
  23. Zhou, Y.; Fan, L.; Xing, F.; Lin, W.; Hu, R. Effect of nano-SiO2 modification on the seismic performance of recycled aggregate concrete shear walls. Eng. Struct. 2024, 307, 117945. [Google Scholar] [CrossRef]
  24. Timesli, A. A Cylindrical Shell Model for Nonlocal Buckling Behavior of CNTs Embedded in an Elastic Foundation Under the Simultaneous Effects of Magnetic Field, Temperature Change, and Number of Walls. Adv. Nano Res. 2021, 11, 581–593. [Google Scholar] [CrossRef]
  25. Tuhta, S. The Determination of Effect of TiO2 on Dynamic Behavior of Scaled Concrete Structure by OMA. Adv. Nano Res. 2021, 11, 641–648. [Google Scholar] [CrossRef]
  26. Tuhta, S.; Günday, F. Determination of the Effect of TiO2 on the Dynamic Behavior of Scaled Concrete Chimney by OMA. Mater. Technol. 2021, 55, 459–466. [Google Scholar] [CrossRef]
  27. Tuhta, S. The Determination of Effect of TiO2 on Dynamic Behavior of Scaled WPC Warehouse by OMA. Adv. Nano Res. 2022, 12, 65–72. [Google Scholar] [CrossRef]
  28. Günday, F. Investigation of the Effect of Coating Light Steel Container Houses with Nano-TiO2 on Dynamic Parameters Using OMA. Buildings 2025, 15, 909. [Google Scholar] [CrossRef]
  29. Krystek, M.; Górski, M. Nanomaterials in Structural Engineering. In New Uses of Micro and Nanomaterials; InTechOpen: London, UK, 2018. [Google Scholar] [CrossRef]
  30. Satankar, R.K.; Sharma, N.; Ramteke, P.M.; Panda, S.K.; Mahapatra, S.S. Acoustic Responses of Natural Fibre Reinforced Nanocomposite Structure Using Multiphysics Approach and Experimental Validation. Adv. Nano Res. 2020, 9, 263–276. [Google Scholar] [CrossRef]
  31. Erdoğan, İ.; Kısa, M.; Özen, M.; Demircan, G.; Kaya, A.İ. Cam Elyaf Takviyeli Epoksi Nanokompozitlerin Dinamik Davranışlarının İncelenmesi. Harran Üniversitesi Mühendislik Dergisi. 2023, 78, 78–90. [Google Scholar] [CrossRef]
  32. Yang, H.-B.; Zhao, X.; Wang, Q.; Ruan, Y.-H.; Liu, Z.-X.; Yue, X.; Zhu, Y.B.; Wu, H.A.; Guan, Q.-F.; Yu, S.-H. Simultaneously Strengthening and Toughening All-Natural Structural Materials via 3D Nanofiber Network Interfacial Design. Angew. Chem. Int. Ed. 2024, 63, e202408458. [Google Scholar] [CrossRef]
  33. Xie, T.; Han, W.; Chang, H.; Motaghedfer, M.R. Dynamic response of nanoparticle-containing concrete shells under moving loads. Adv. Nano Res. 2024, 16, 303–311. [Google Scholar] [CrossRef]
  34. Ctibor, P.; Nevrlá, B.; Neufuss, K.; Petrášek, J.; Sedláček, J. Plasma Spray Coatings of Natural Ores from Structural, Mechanical, Thermal, and Dielectric Viewpoints. Coatings 2020, 10, 3. [Google Scholar] [CrossRef]
  35. Shao, L.; Yu, W.; Du, X.; Shen, A.; Li, Y.; Ding, H.; Tao, S.; Wu, F. Investigating Lightweight Carbonation Curing of Waste Slurry Using Activated Magnesium Oxide: Performance Insights. Materials 2025, 18, 2084. [Google Scholar] [CrossRef]
  36. Haiden, L.; Feuchter, M.; Brunner, A.J.; Barbezat, M.; Pansare, A.; Ravindran, B.; Terziyska, V.; Pinter, G. Shining a Light on Carbon-Reinforced Polymers: Mg/MgO and TiO2 Nanomodifications for Enhanced Optical Performance. J. Compos. Sci. 2025, 9, 187. [Google Scholar] [CrossRef]
  37. Shen, Z.; Ni, M.; Guo, S.; Chen, X.; Tong, M.; Lu, J. Studies on Magnesium-Based Wet Flue Gas Desulphurization Process with a Spray Scrubber. Asian J. Chem. 2013, 25, 6727–6732. [Google Scholar] [CrossRef]
  38. Yao, H.-L.; Xia, J.; Yi, D.-L.; Liu, H.-Y.; Liu, W.-L.; Liu, J.-S.; Wang, F. Microstructure and Corrosion Properties of Biodegradable Mg/MgO Composite Coating on Mg Alloy Prepared by High Velocity Suspension Flame Spraying. J. Therm. Spray Technol. 2021, 30, 1544–1556. [Google Scholar] [CrossRef]
  39. Computers and Structures, Inc. SAP2000: Integrated Structural Analysis and Design Software, Version 26.0; Computers and Structures, Inc.: Berkeley, CA, USA, 2024.
  40. Aoki, T.; Sabia, D.; Rivella, D. Influence of Experimental Data and FE Model on Updating Results of a Brick Chimney. Adv. Eng. Softw. 2008, 39, 327–335. [Google Scholar] [CrossRef]
  41. Kasimzade, A.A. Finite Element Method: Foundation and Applications to Structural Analysis; Nobel Publication: Ankara, Turkey, 2018. [Google Scholar]
  42. Kasimzade, A.A. Structural Dynamics: Theory and Applications to Earthquake Engineering; Nobel Publication: Ankara, Turkey, 2018. [Google Scholar]
  43. Sancibrian, R.; Lombillo, I.; Sarabia, E.G.; Boffill, Y.; Wong, H.; Villegas, L. Dynamic Identification and Condition Assessment of an Old Masonry Chimney by Using Modal Testing. Procedia Eng. 2017, 199, 3410–3415. [Google Scholar] [CrossRef]
  44. Ke, L.; Li, C.; He, J.; Lu, Y.; Jiao, Y.; Liu, Y. Fatigue Evaluation and CFRP Strengthening of Diaphragm Cutouts in Orthotropic Steel Decks. Steel Compos. Struct. 2021, 39, 453–469. [Google Scholar] [CrossRef]
  45. Kumar, Y.; Gupta, A.; Tounsi, A. Size-Dependent Vibration Response of Porous Graded Nanostructure with FEM and Nonlocal Continuum Model. Adv. Nano Res. 2021, 11, 1–17. [Google Scholar] [CrossRef]
  46. Rahman, S.; Jain, A.K.; Bharti, S.D.; Datta, T.K. A Comparative Study of the Models to Predict Aeroelastic Vibrations of Circular Cylinder and Chimneys. Wind Struct. 2022, 35, 35–54. [Google Scholar] [CrossRef]
  47. Günday, F. Structural Health Monitoring of Steel Garage Model with Stochastic Subspace Identification–Covariance Variance Analysis. Buildings 2025, 15, 944. [Google Scholar] [CrossRef]
  48. EN 1993-1-1; Eurocode 3: Design of Steel Structures—Part 1-1: General Rules and Rules for Buildings. CEN: Brussels, Belgium, 2005.
  49. EN 10025-2; Hot Rolled Products of Structural Steels—Part 2: Technical Delivery Conditions for Non-Alloy Structural Steels. CEN: Brussels, Belgium, 2019.
  50. CRC. Handbook of Chemistry and Physics, 97th ed.; Haynes, W.M., Ed.; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
  51. Nair, A.K.; Gautam, A.; Buehler, M.J. Nano-mechanical behavior of MgO nanostructures. J. Appl. Phys. 2010, 107, 093510. [Google Scholar] [CrossRef]
  52. Walker, J.S.; Mould, S.T. Poisson’s ratio of magnesium oxide: Experimental and theoretical approaches. Phys. Rev. B 1991, 44, 1434. [Google Scholar] [CrossRef]
  53. Chen, Y.; Feng, Z.; Zhang, W. Effect of MgO Content on Mechanical Properties of Directionally Solidified Pure Magnesium. Mater. Res. 2021, 24, 2. [Google Scholar] [CrossRef]
  54. Chopra, A.K. Dynamics of Structures: Theory and Applications to Earthquake Engineering, 5th ed.; Pearson: Boston, MA, USA, 2017. [Google Scholar]
  55. EN 1998-1:2004; Eurocode 8: Design of Structures for Earthquake Resistance—Part 1: General Rules, Seismic Actions and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2004.
  56. ASCE/SEI 7-16; Minimum Design Loads and Associated Criteria for Buildings and Other Structures. American Society of Civil Engineers: Reston, VA, USA, 2016.
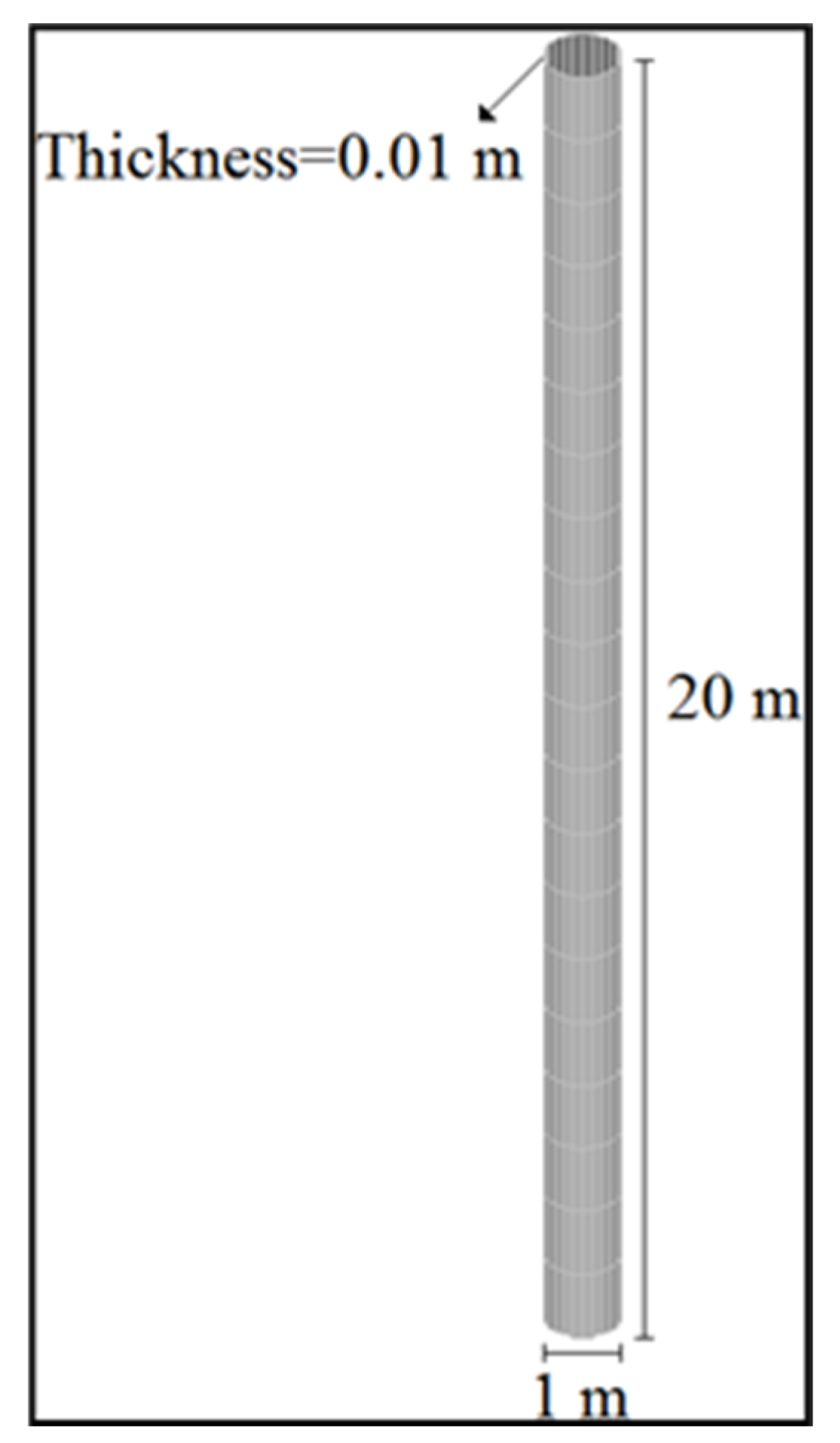
Coatings 15 00619 g001
Figure 1. Dimensions of steel chimney.
Figure 1. Dimensions of steel chimney.
Coatings 15 00619 g001
Coatings 15 00619 g002
Figure 2. A schematic view and properties of the shell element [38].
Figure 2. A schematic view and properties of the shell element [38].
Coatings 15 00619 g002
Coatings 15 00619 g003
Figure 3. Finite element model of steel chimney before MgO retrofitting.
Figure 3. Finite element model of steel chimney before MgO retrofitting.
Coatings 15 00619 g003
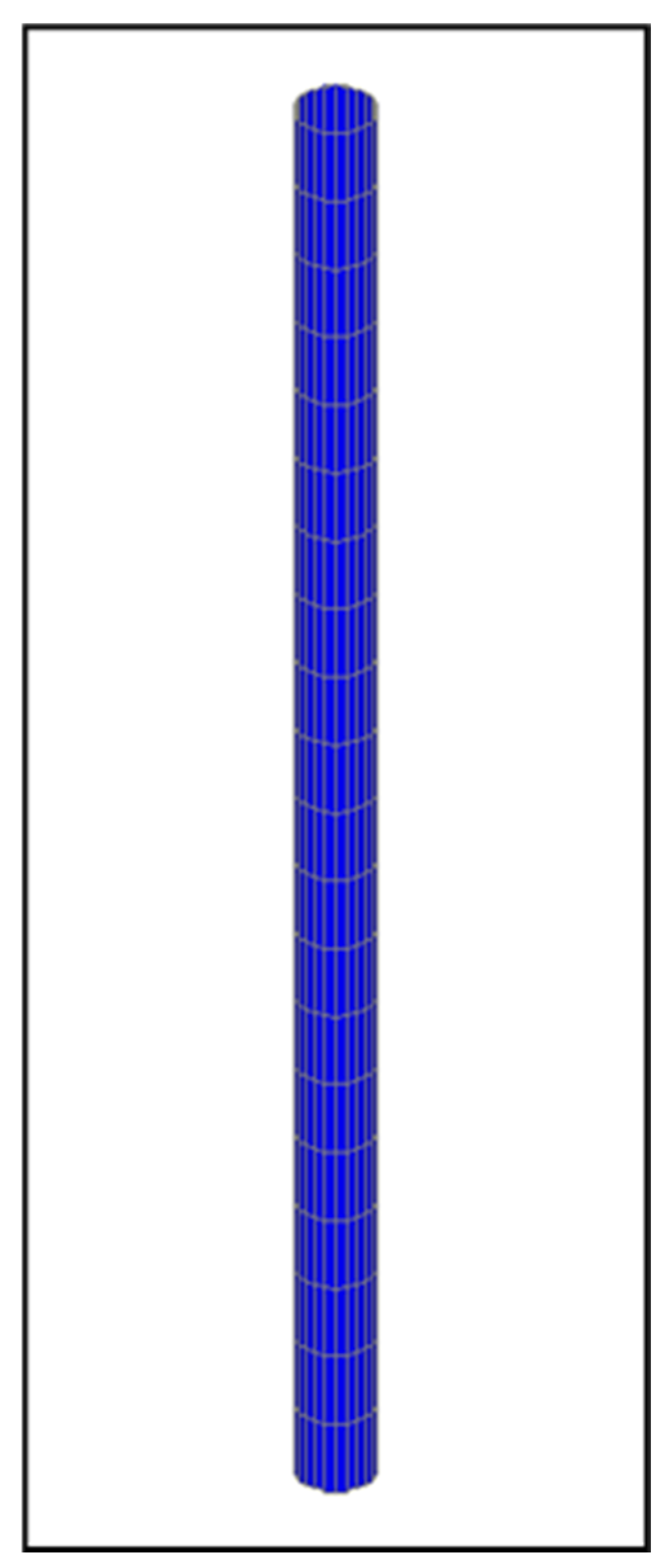
Coatings 15 00619 g004
Figure 4. Finite element model of steel chimney after MgO retrofitting.
Figure 4. Finite element model of steel chimney after MgO retrofitting.
Coatings 15 00619 g004
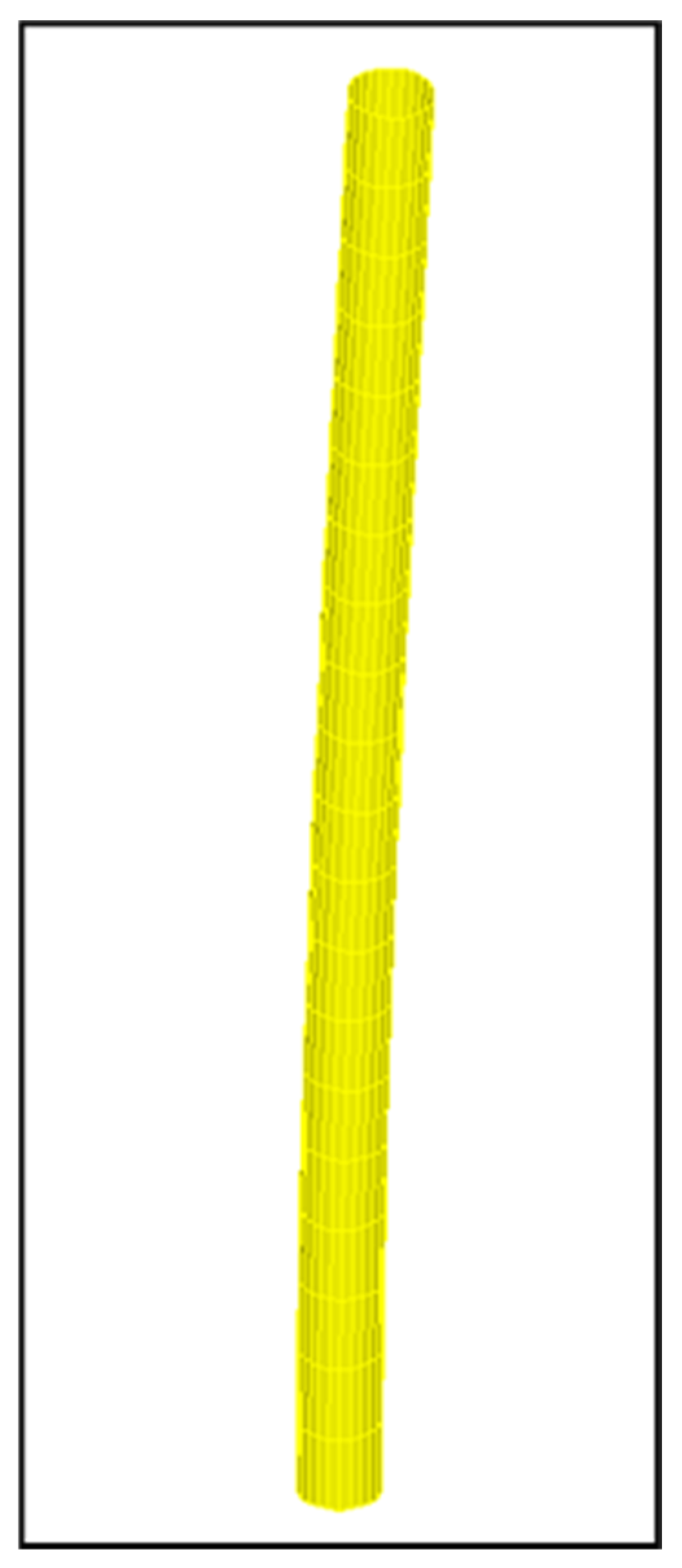
Coatings 15 00619 g005
Figure 5. The 1st Mode (f = 2.50 Hz).
Figure 5. The 1st Mode (f = 2.50 Hz).
Coatings 15 00619 g005
Coatings 15 00619 g006
Figure 6. The 2nd Mode (f = 2.85 Hz).
Figure 6. The 2nd Mode (f = 2.85 Hz).
Coatings 15 00619 g006
Coatings 15 00619 g007
Figure 7. The 3rd Mode (f = 3.44 Hz).
Figure 7. The 3rd Mode (f = 3.44 Hz).
Coatings 15 00619 g007
Coatings 15 00619 g008
Figure 8. The 4th Mode (f = 4.54 Hz).
Figure 8. The 4th Mode (f = 4.54 Hz).
Coatings 15 00619 g008
Coatings 15 00619 g009
Figure 9. The 5th Mode (f = 7.14 Hz).
Figure 9. The 5th Mode (f = 7.14 Hz).
Coatings 15 00619 g009
Coatings 15 00619 g010
Figure 10. The 1st Mode (f = 2.63 Hz).
Figure 10. The 1st Mode (f = 2.63 Hz).
Coatings 15 00619 g010
Coatings 15 00619 g011
Figure 11. The 2nd Mode (f = 3.03 Hz).
Figure 11. The 2nd Mode (f = 3.03 Hz).
Coatings 15 00619 g011
Coatings 15 00619 g012
Figure 12. The 3rd Mode (f = 3.84 Hz).
Figure 12. The 3rd Mode (f = 3.84 Hz).
Coatings 15 00619 g012
Coatings 15 00619 g013
Figure 13. The 4th Mode (f = 5.55 Hz).
Figure 13. The 4th Mode (f = 5.55 Hz).
Coatings 15 00619 g013
Coatings 15 00619 g014
Figure 14. The 5th Mode (f = 11.11 Hz).
Figure 14. The 5th Mode (f = 11.11 Hz).
Coatings 15 00619 g014
Coatings 15 00619 g015
Figure 15. The time history of ground motion acceleration of 1995 Kobe earthquake (PGA = 0.833 g).
Figure 15. The time history of ground motion acceleration of 1995 Kobe earthquake (PGA = 0.833 g).
Coatings 15 00619 g015
Coatings 15 00619 g016
Figure 16. Maximum displacement contours of chimney before MgO retrofitting.
Figure 16. Maximum displacement contours of chimney before MgO retrofitting.
Coatings 15 00619 g016
Coatings 15 00619 g017
Figure 17. Maximum displacement contours of chimney after MgO retrofitting.
Figure 17. Maximum displacement contours of chimney after MgO retrofitting.
Coatings 15 00619 g017
Coatings 15 00619 g018
Figure 18. Minimum–maximum von Mises stress contours of chimney before MgO retrofitting.
Figure 18. Minimum–maximum von Mises stress contours of chimney before MgO retrofitting.
Coatings 15 00619 g018
Coatings 15 00619 g019
Figure 19. Minimum–maximum von Mises stress contours of chimney after MgO retrofitting.
Figure 19. Minimum–maximum von Mises stress contours of chimney after MgO retrofitting.
Coatings 15 00619 g019
Table 1. Material properties considered in analysis of steel chimney.
Table 1. Material properties considered in analysis of steel chimney.
MaterialModulus of Elasticity
(kN/m2)		Poisson’s RatioMass per Unit Volume
(kN/m3)
Steel2 × 1080.307.849
Table 2. Typical properties of MgO nanocoating.
Table 2. Typical properties of MgO nanocoating.
MaterialModulus of Elasticity
(kN/m2)		Poisson’s RatioMass per Unit Volume
(kN/m3)
MgO3.3 × 1080.363.560
Table 3. The comparison of frequency for before and after MgO retrofitting.
Table 3. The comparison of frequency for before and after MgO retrofitting.
Mode12345
Before MgO retrofitting (Hz)2.502.853.444.547.14
After MgO retrofitting (Hz)2.633.033.845.5511.11
Difference (Hz)0.130.180.401.013.97
Difference (%)5.26.3211.6322.2555.60
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content.

Share and Cite

MDPI and ACS Style

Tuhta, S.; Günday, F. Investigation of Steel Chimney Retrofitted with Nanocoating Under Earthquake Excitation Using FEM. Coatings 2025, 15, 619. https://doi.org/10.3390/coatings15060619

AMA Style

Tuhta S, Günday F. Investigation of Steel Chimney Retrofitted with Nanocoating Under Earthquake Excitation Using FEM. Coatings. 2025; 15(6):619. https://doi.org/10.3390/coatings15060619

Chicago/Turabian Style

Tuhta, Sertaç, and Furkan Günday. 2025. "Investigation of Steel Chimney Retrofitted with Nanocoating Under Earthquake Excitation Using FEM" Coatings 15, no. 6: 619. https://doi.org/10.3390/coatings15060619

APA Style

Tuhta, S., & Günday, F. (2025). Investigation of Steel Chimney Retrofitted with Nanocoating Under Earthquake Excitation Using FEM. Coatings, 15(6), 619. https://doi.org/10.3390/coatings15060619

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Article metric data becomes available approximately 24 hours after publication online.
Back to TopTop