Using Preexisting Surface Cracks to Prevent Thermal Fatigue Failure and Crack Delamination in FGM Thermal Barrier Coatings
Abstract
1. Introduction
2. Description of the Model
3. Coating Architecture
4. The Construction of Pulsed Laser Boundary and the Air-Cooling Conditions
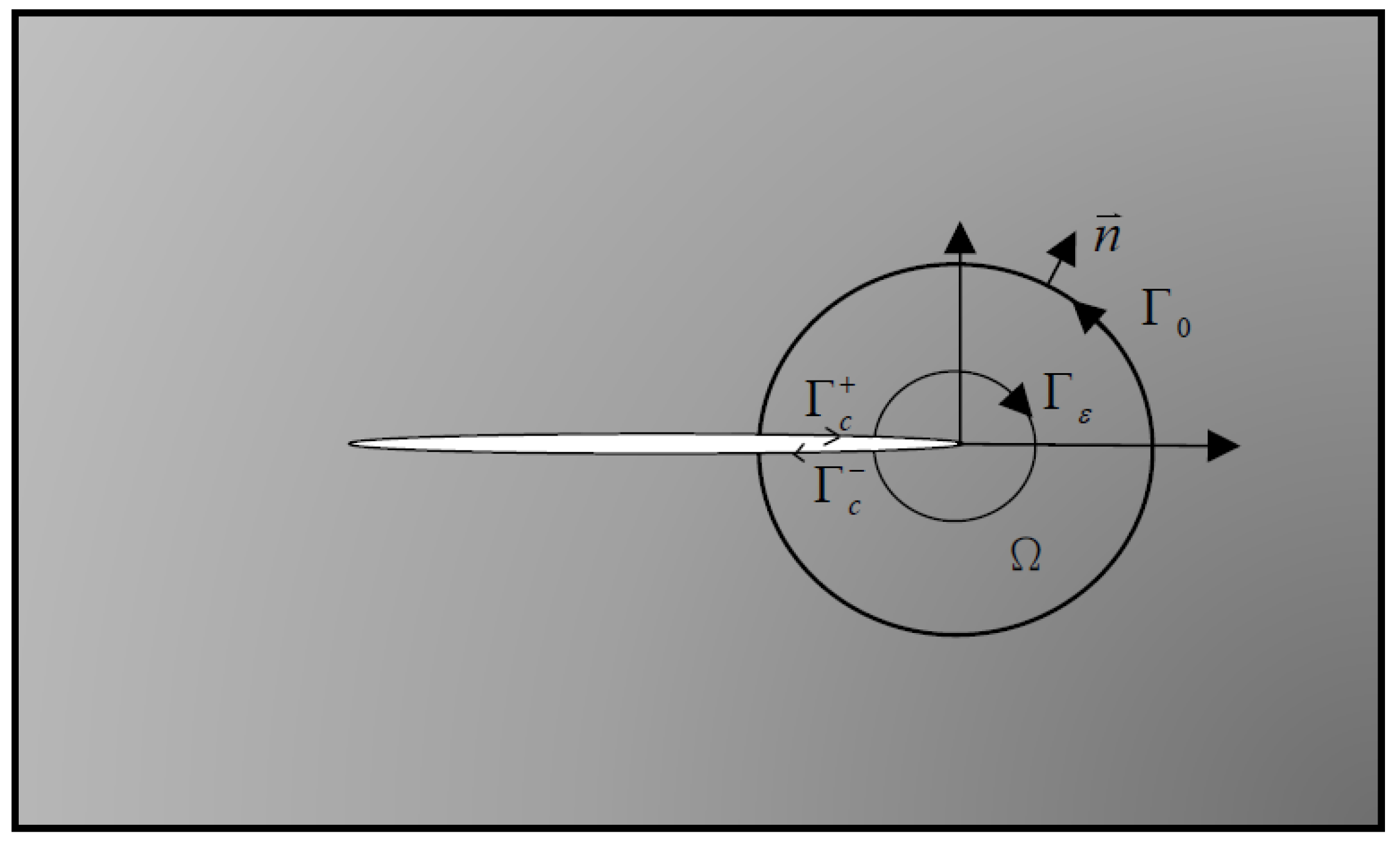
5. J-Integral for Thermally Loaded Isotropic TBC Coating
6. Displacement Correlation Technique (DCT)
7. Simulation of Thermal Shock Testing
7.1. The Evaluation of Stress Intensity Factors
7.2. Stress Analysis on the Interface and Surface
8. Conclusions
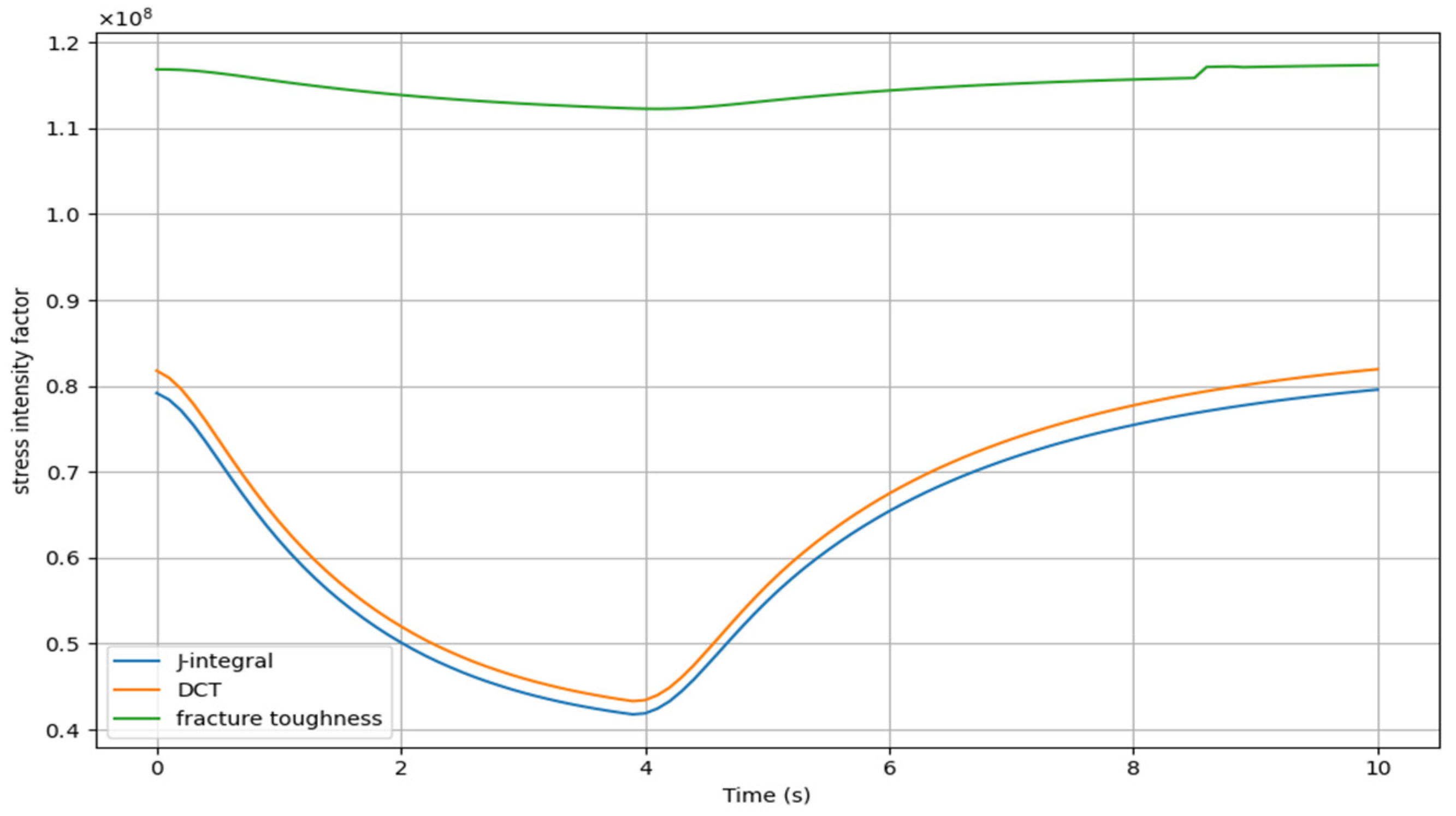
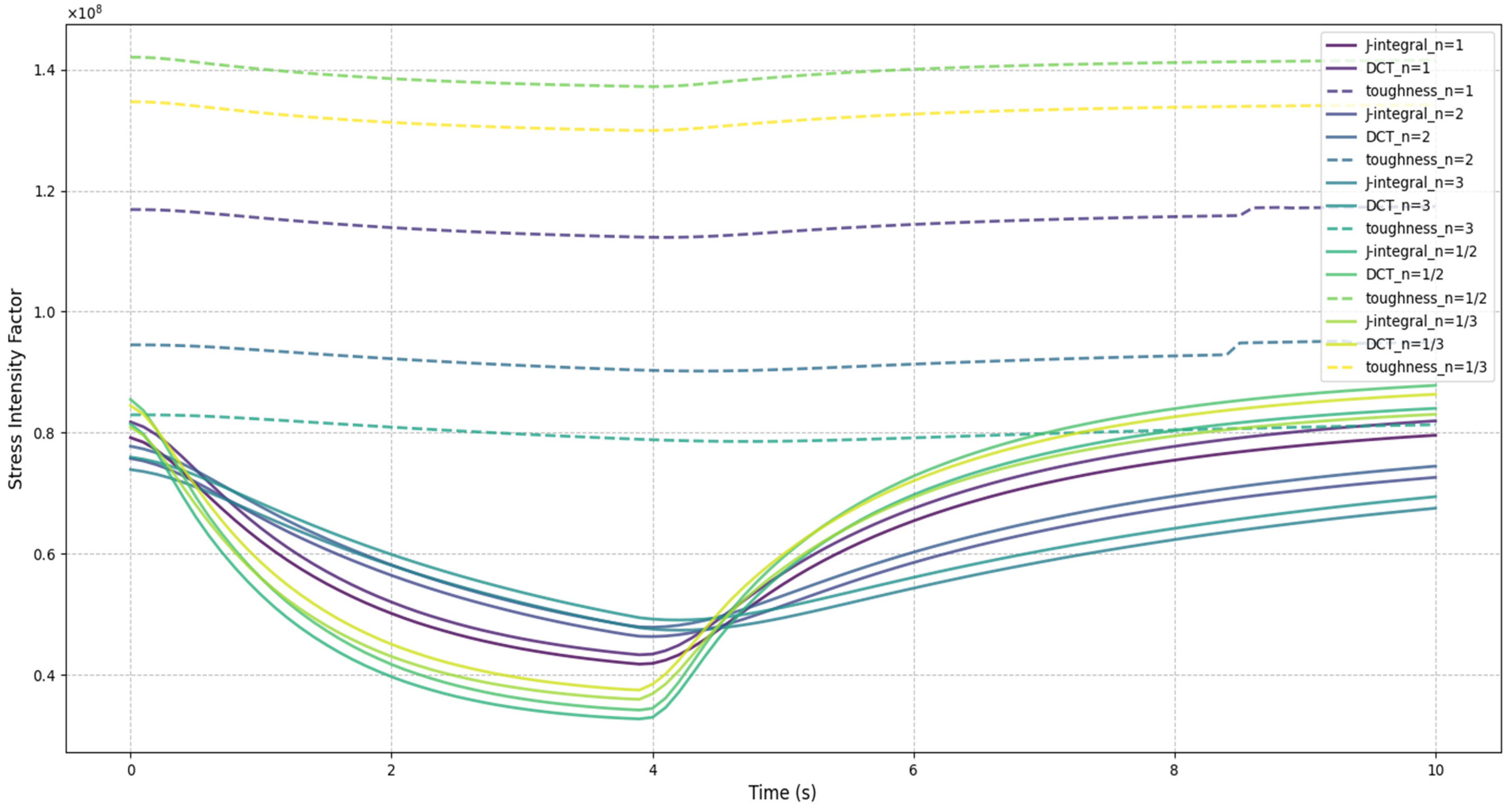
- Our analysis, based on LEFM theory and evaluated using the J-integral and DCT methods, shows a high agreement in SIF values from Figure 11, Figure 12, Figure 13 and Figure 14, with low error estimates. The presence of high-intensity preexisting cracks, as in the CPI 10 design, effectively reduces SIF values relative to fracture toughness, except when a single crack exhibits higher SIF than the fracture toughness. Additionally, given that elastoplastic or viscoplastic behaviors may occur at the crack tip under thermoelastic conditions, future work should consider methods such as the strain energy release rate and XFEM for a more thorough analysis of crack propagation.
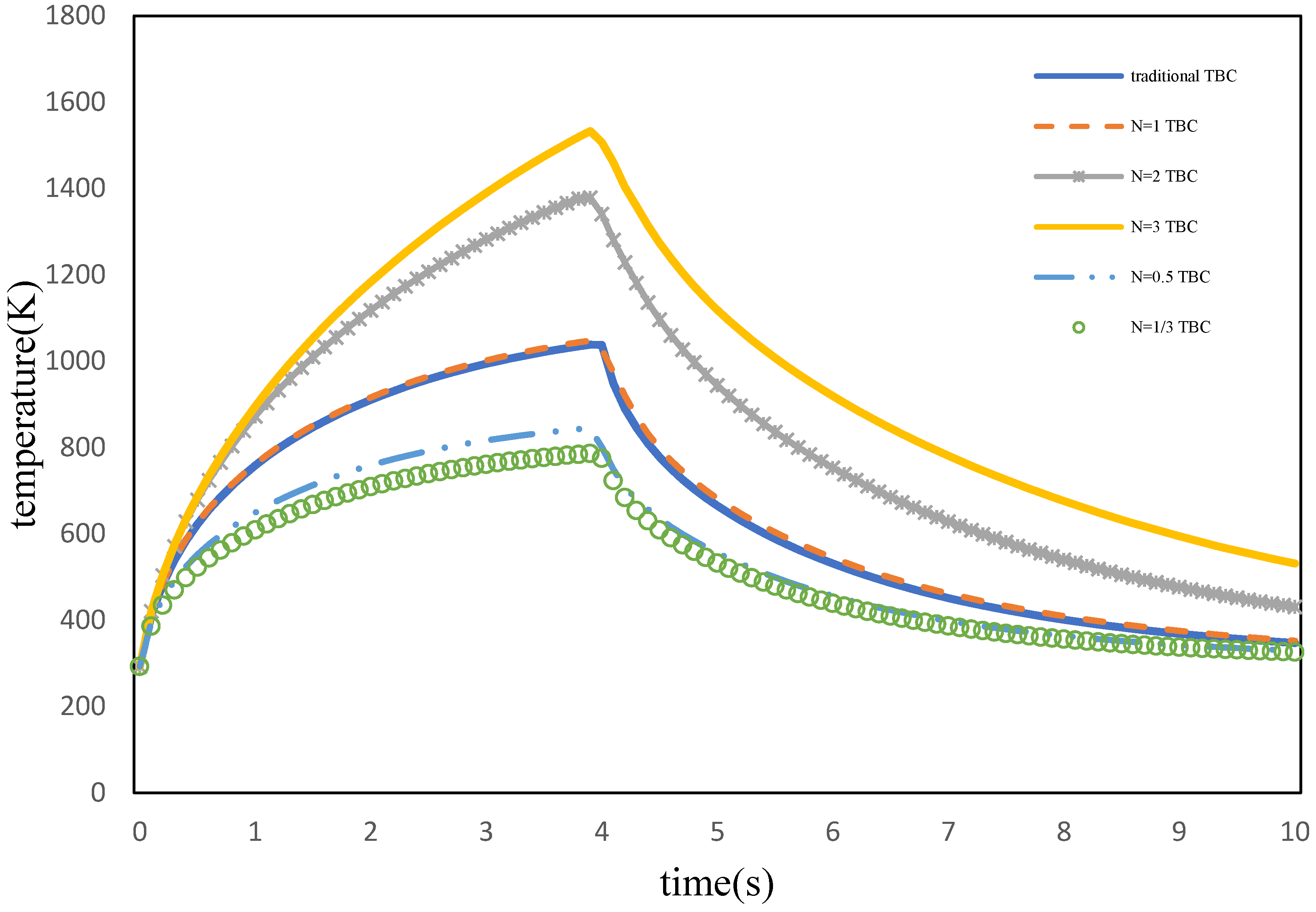
- Thermal shock resistance is a critical parameter for TBC coatings. Figure 15 demonstratehaus that traditional TBC coatings operate within a temperature range of 293.15 K to 1027 K, while an increased volume fraction mixture in the functionally graded material coating can elevate the maximum temperature to 1533 K. This finding underscores the significant impact of the volume fraction mixture on enhancing the thermal shock resistance of the coating.
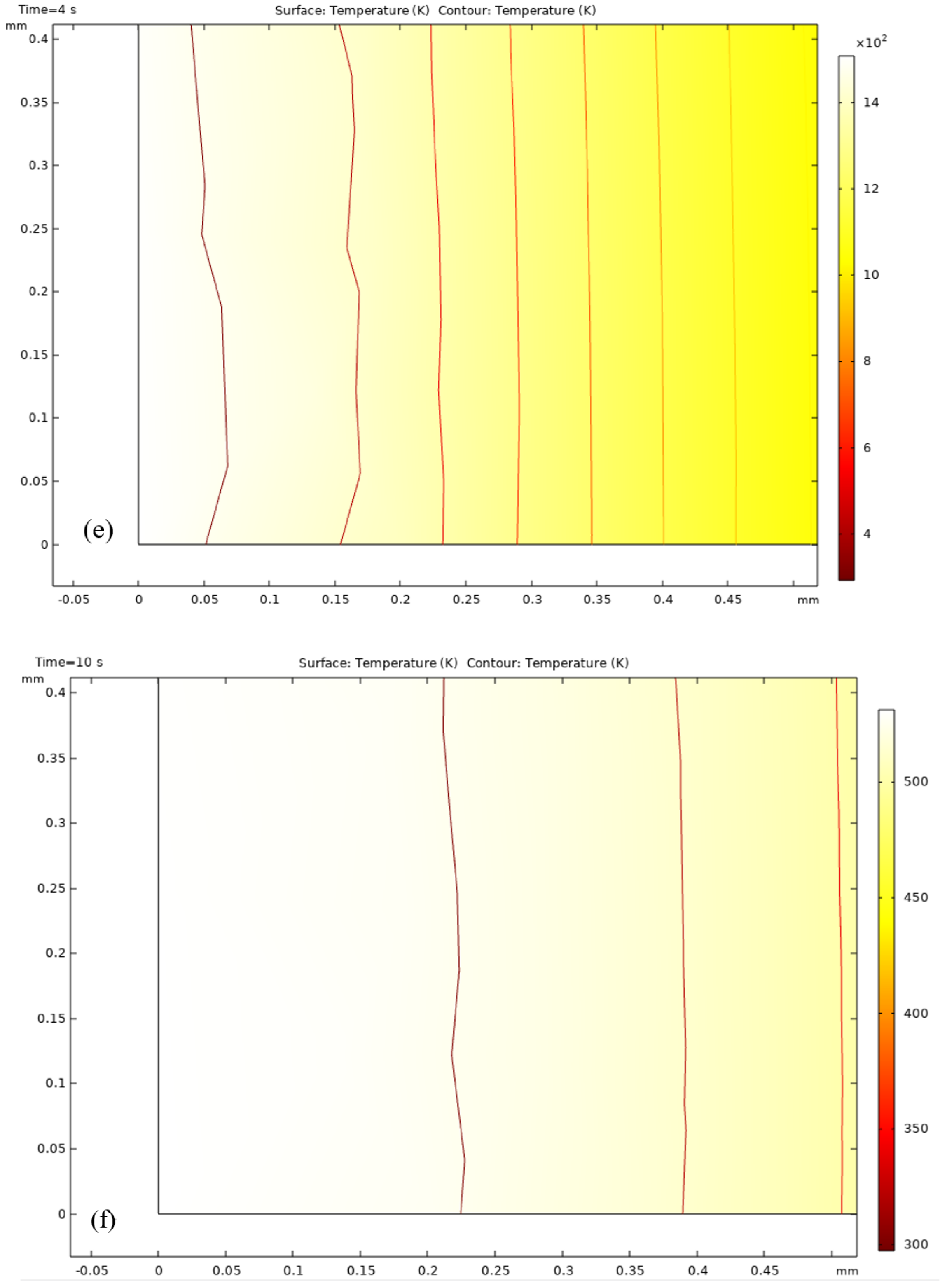
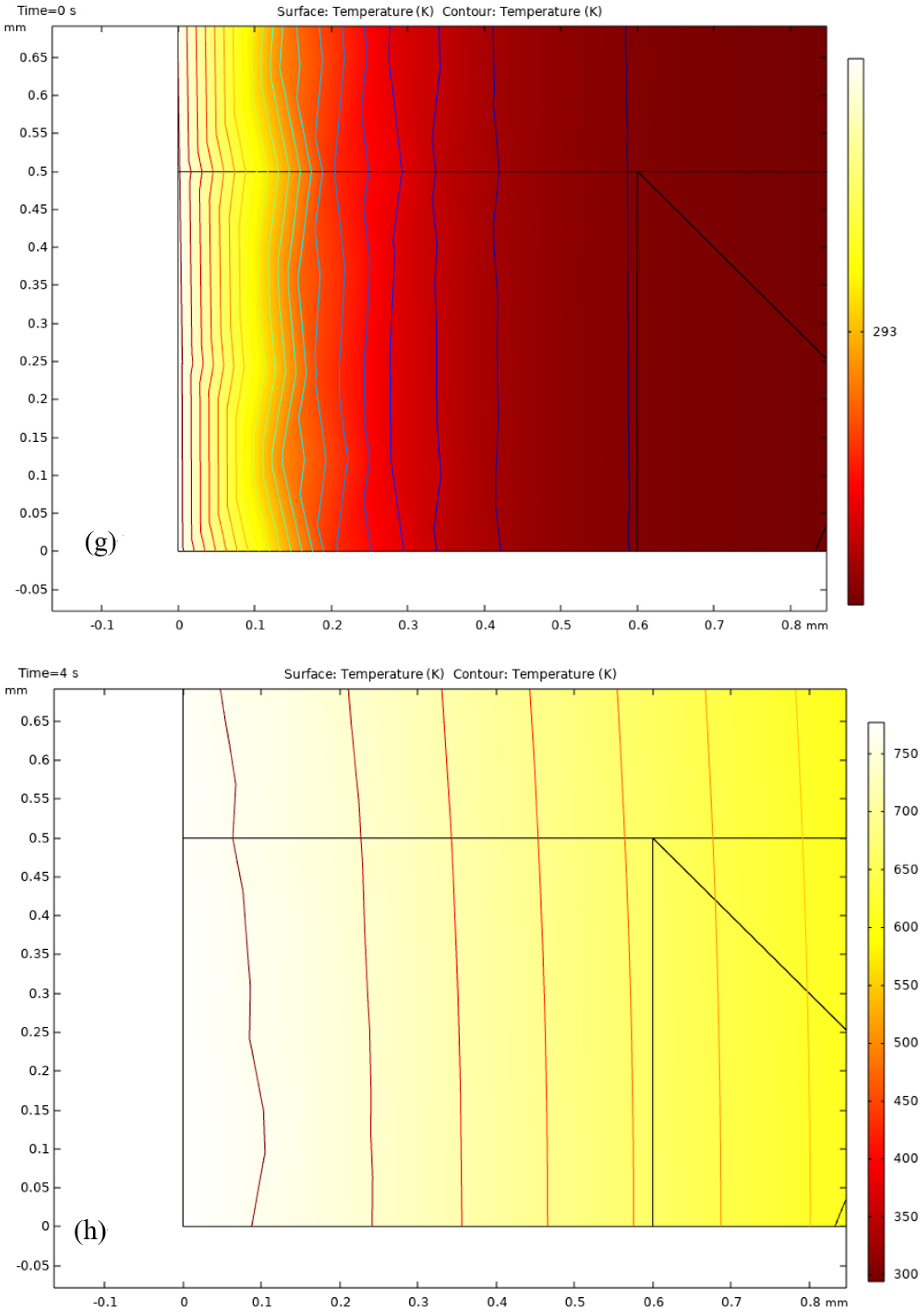
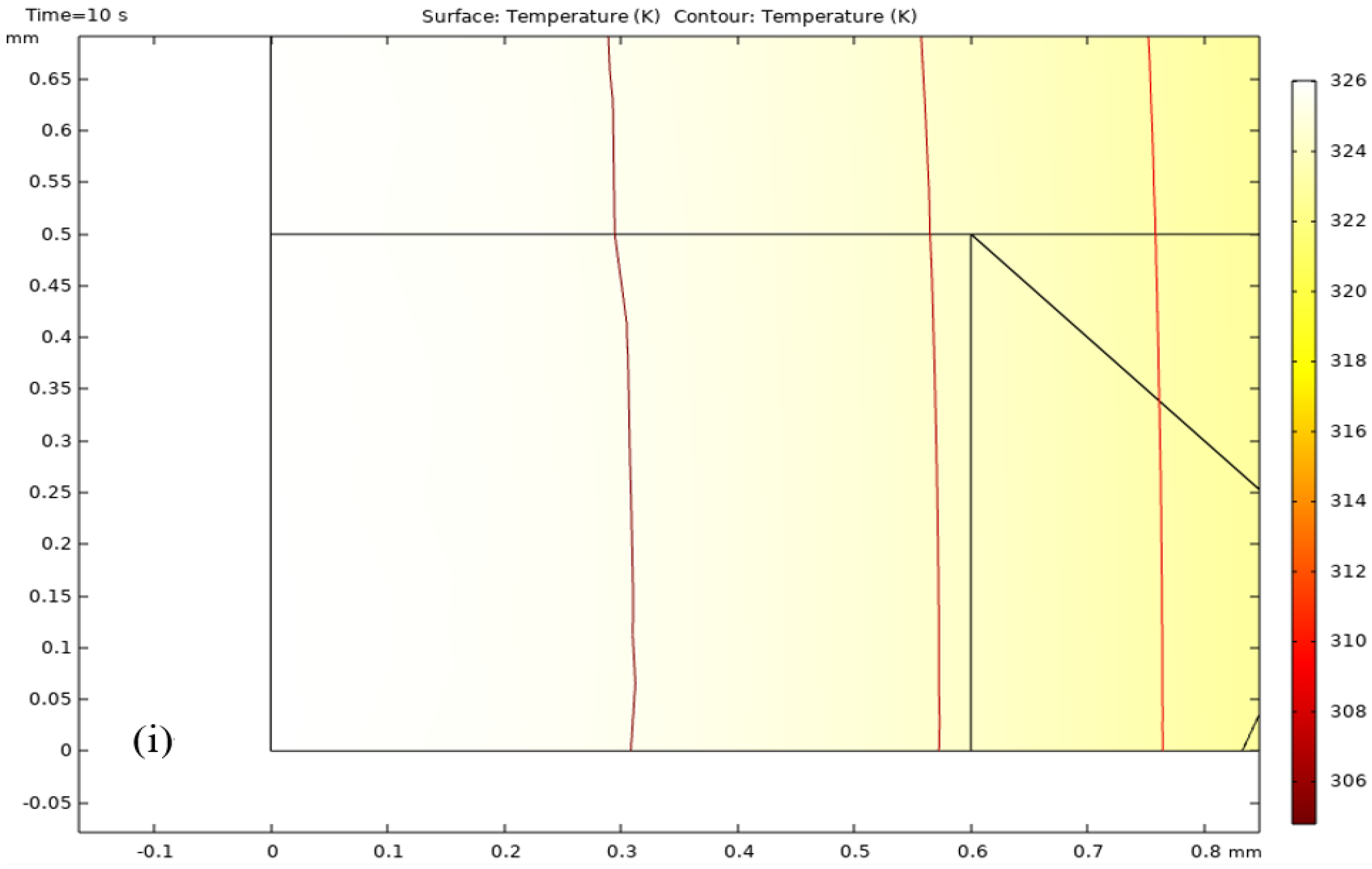
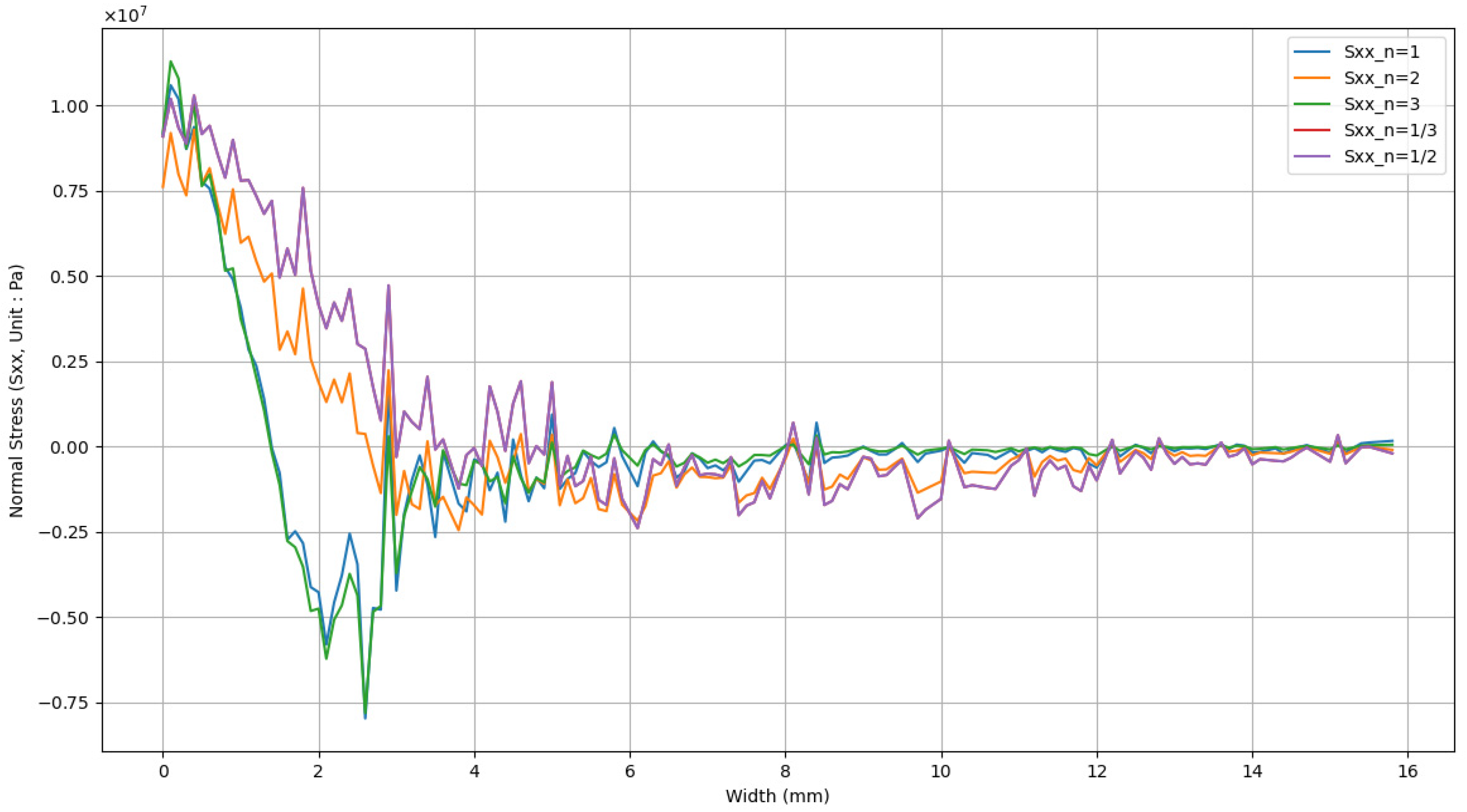
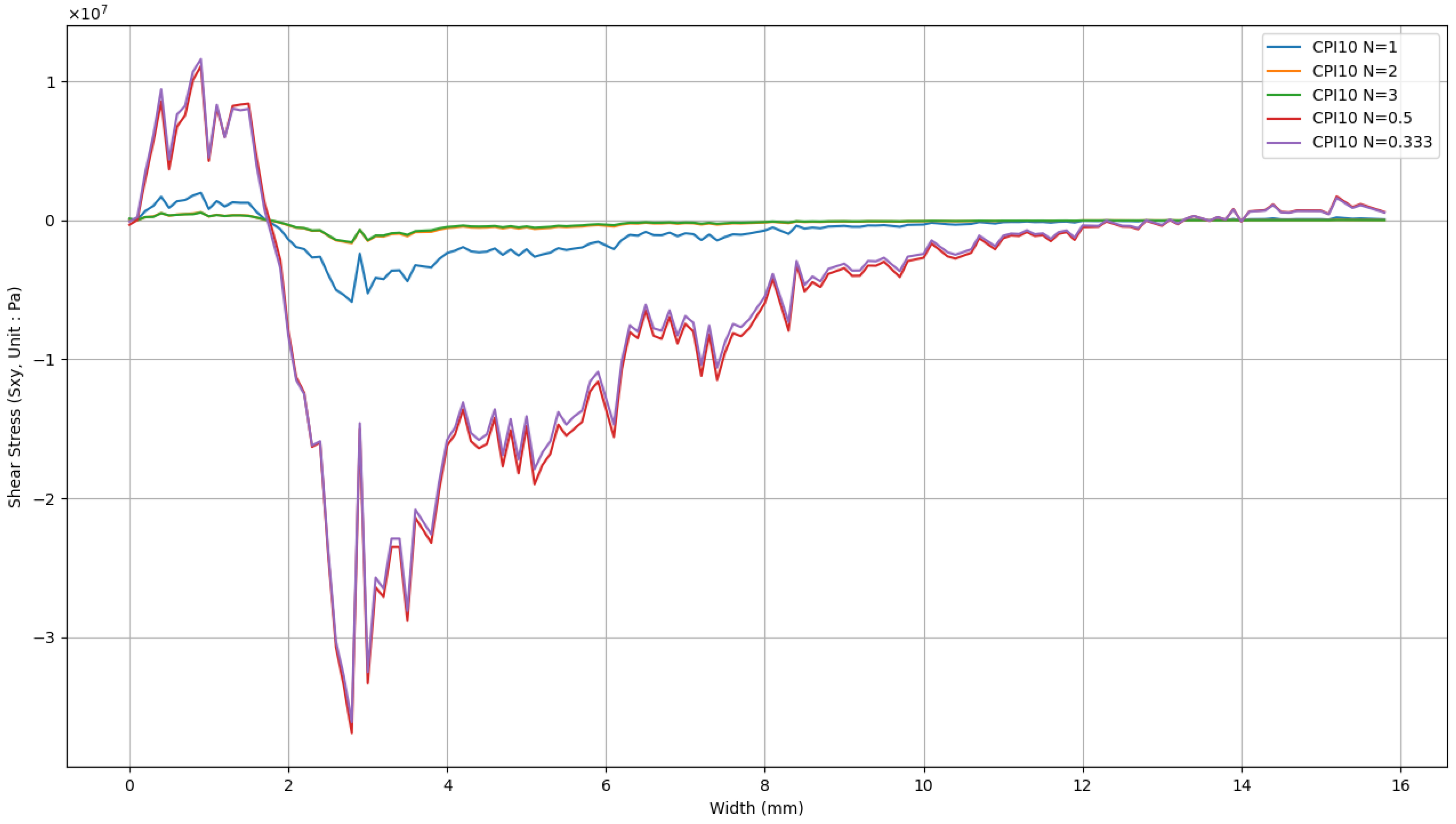
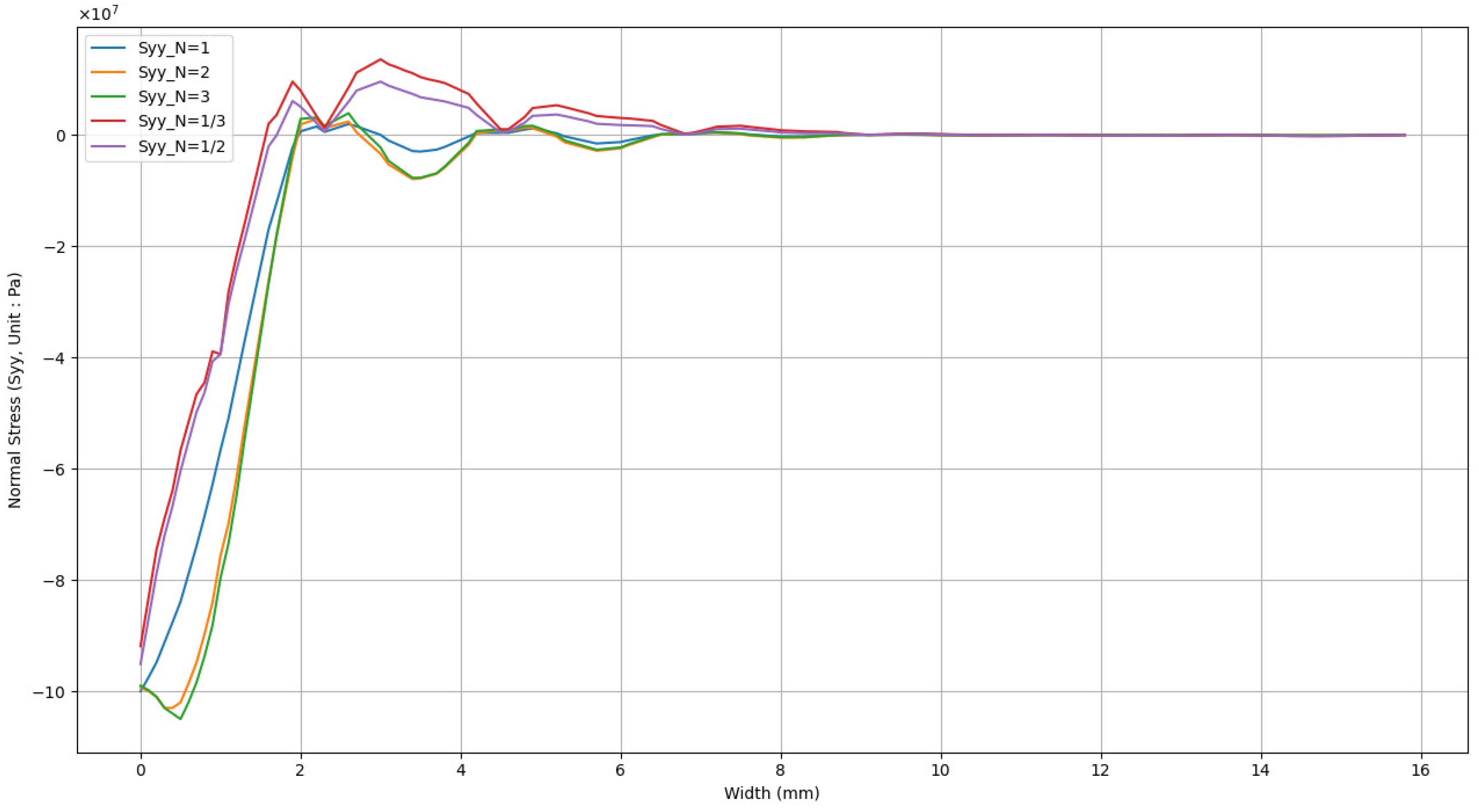
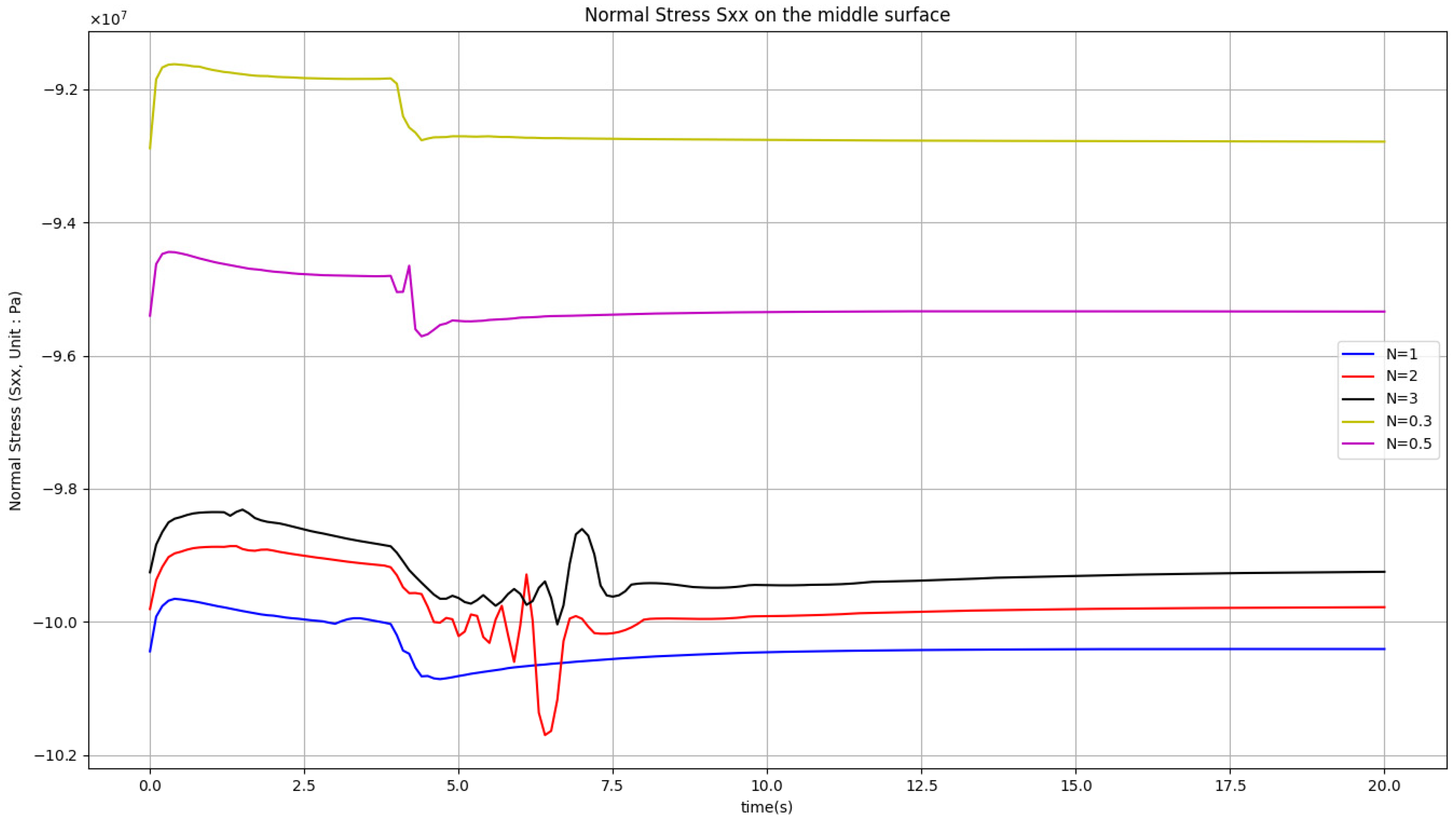
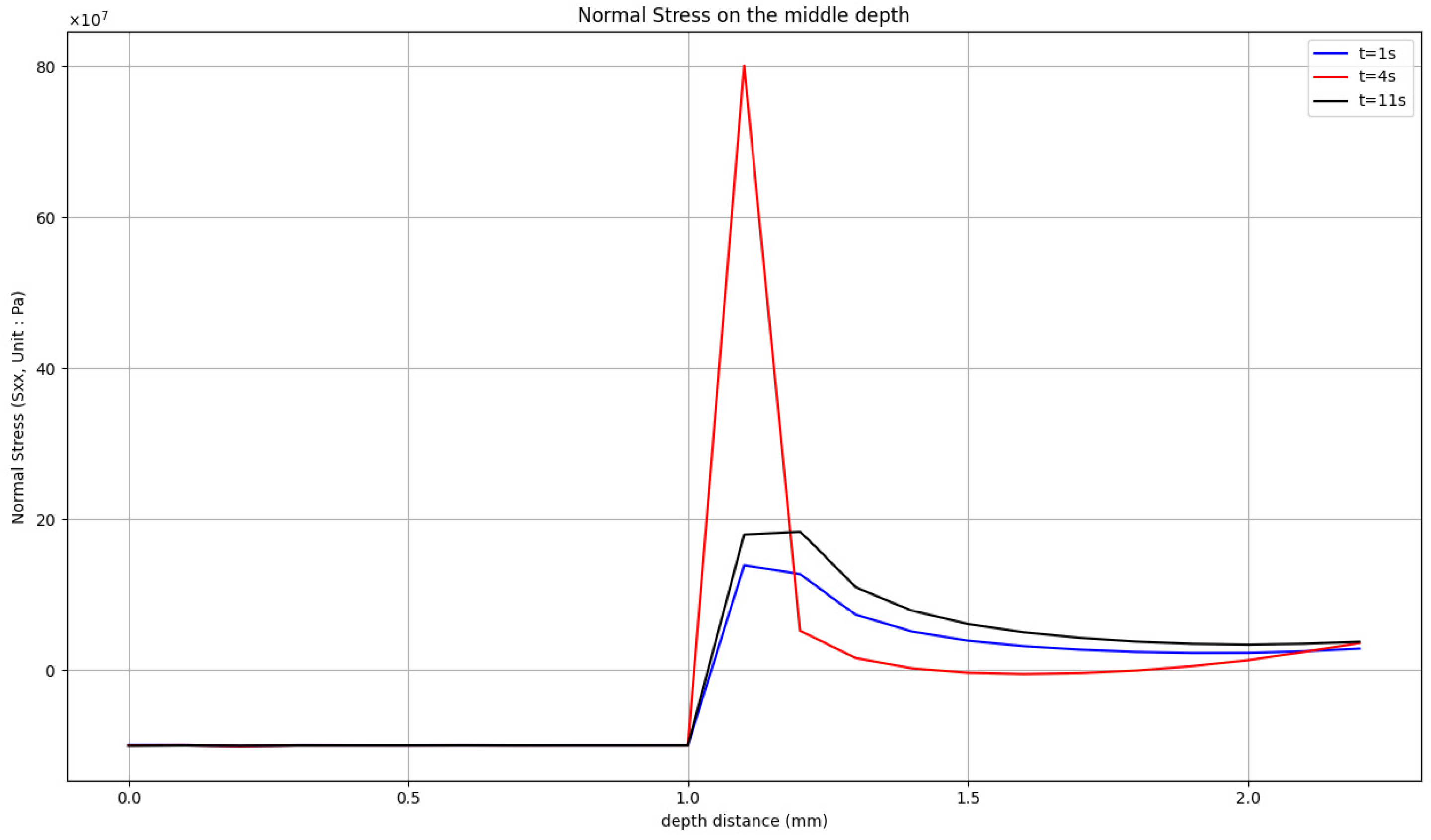
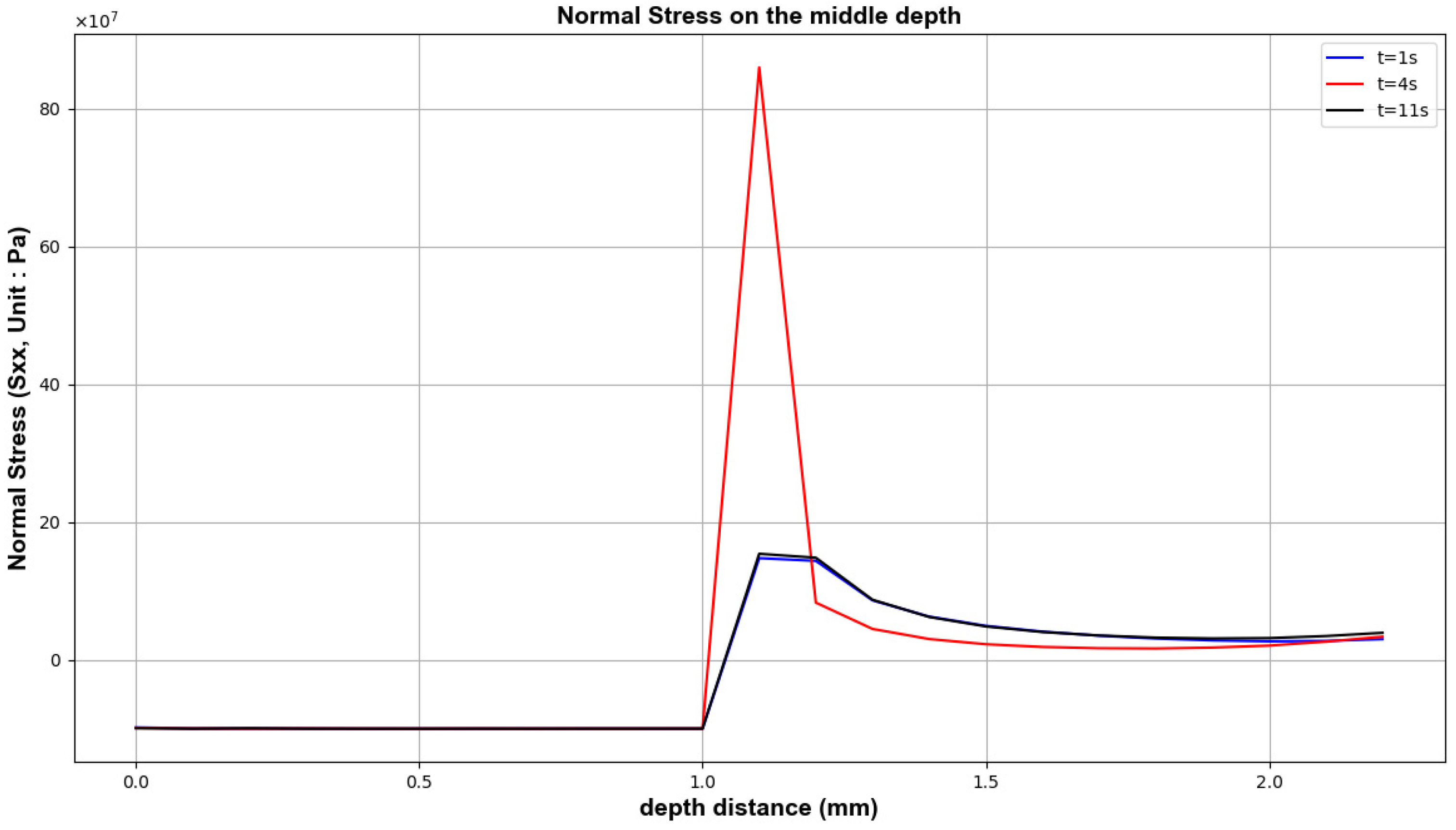
- Stress analysis at both the interface and surface reveals that the CPI 10 design effectively reduces tensile and shear stresses at the interface as the volume fraction mixture increases. Although the CPI 10 design does not lower the surface tensile stress, the two-phase functionally graded coatings can reduce it to below 1.15 MPa, even rendering it compressive. This is an acceptable level for ceramic materials. Furthermore, evaluations of the normal stress distribution along the TBC surface and coating central line indicate that a higher volume ratio of yttria-stabilized zirconia (YSZ) relative to the NiCoCrAlY bond coat results in lower thermal stress during shock testing. This suggests that further optimization of the stress distribution is possible by applying the CPI 10 design to the surface and adopting a three-rule linear distribution (N = 3) in the functionally graded coatings.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yanyan, Z.; Wei, Z.; Licheng, G. Investigations of the Fracture Mechanics of Nonhomogeneous Materials Under Thermal Shock Loading. Doctoral Dissertation, Harbin Institute of Technology, Harbin, China, 2014. [Google Scholar]
- Erdogan, F.; Wu, B.H. Crack problems in FGM layers under thermal stress. J. Therm. Stress. 1995, 19, 237–265. [Google Scholar] [CrossRef]
- Cho, J.R.; Ha, D.Y. Averaging and finite element discretization approaches in the numerical analysis of functionally graded materials. Mater. Sci. Eng. A 2001, 302, 187–196. [Google Scholar] [CrossRef]
- Wang, T.; Xueling, F.; Yongle, S. The stress and cracks in thermal barrier coating system: A review. Chin. J. Solid Mech. 2016, 37, 477–517. [Google Scholar]
- Xu, Z.; Huang, G.; He, L.; Mu, R.; Wang, K.; Dai, J. Effect of grit blasting on the thermal cycling behavior of diffusion aluminide/YSZ TBCs. J. Alloys Compd. 2014, 586, 1–9. [Google Scholar] [CrossRef]
- Jian, C.Y.; Hashida, T.; Takahashi, H.; Saito, M. Thermal shock and fatigue resistance evaluation of functionally graded coating for gas turbine blades by laser heating method. Compos. Eng. 1995, 5, 879–889. [Google Scholar]
- Xiong, H.P.; Kawasaki, A.; Kang, Y.S.; Watanabe, R. Experimental study on heat insulation performance of functionally graded metal/ceramic coatings and their fracture behavior at high surface temperatures. Surf. Coat. Technol. 2005, 194, 203–214. [Google Scholar] [CrossRef]
- Taylor, T.A.; Appleby, D.L.; Weatherill, A.E. Plasma-sprayed yttria-stabilized zirconia coatings: Structure-property relationships. Surf. Coat. Technol. 1990, 43, 470–480. [Google Scholar] [CrossRef]
- Zhou, B.; Kokini, K. Effect of preexisting surface cracks on the interfacial thermal fracture of thermal barrier coatings: An experimental study. Surf. Coat. Technol. 2004, 187, 17–25. [Google Scholar] [CrossRef]
- Bhattacharya, S.; Singh, I.V.; Mishra, B.K. Fatigue-life estimation of functionally graded materials using XFEM. Eng. Comput. 2013, 29, 427–448. [Google Scholar] [CrossRef]
- Thamburaja, P.; Sarah, K.; Srinivasa, A.; Reddy, J.N. Fracture of viscoelastic materials: FEM implementation of a non-local & rate form-based finite-deformation constitutive theory. Comput. Methods Appl. Mech. Eng. 2019, 354, 871–903. [Google Scholar] [CrossRef]
- Paulino, H.; Kim, J.H. Mixed-mode J-integral formulation and implementation using graded elements for fracture analysis of nonhomogeneous orthotropic materials. Mech. Mater. 2003, 35, 107–128. [Google Scholar]
- Kim, J.H.; Paulino, G.H. Finite element evaluation of mixed mode stress intensity factors in functionally graded materials. Int. J. Numer. Methods Eng. 2001, 53, 1903–1935. [Google Scholar] [CrossRef]
- Ozturk, M.; Erdogan, F. Mode I crack problem in an inhomogeneous orthotropic medium. Int. J. Eng. Sci. 1997, 35, 869–883. [Google Scholar] [CrossRef]
- Erdogan, F.; Wu, B.H. The surface crack problem for a plate with functionally graded properties. J. Appl. Mech. 1997, 64, 449–456. [Google Scholar] [CrossRef]
- Shih, C.F.; Loreniz, H.G.; German, M.D. Crack extension modeling with singular quadratic isoparametric elements. Int. J. Fract. 1976, 12, 647–651. [Google Scholar] [CrossRef]
- Leslie, B.S.; Hershokovitz, I.; Wawrzynek, P.A. Methods for calculating stress intensity factors in anisotropic materials: Part I-z=0 is a symmetric plane. Eng. Fract. Mech. 2004, 72, 2328–2358. [Google Scholar]
- Zhou, B.; Kokini, K. Effect of surface pre-crack morphology on the fracture of thermal barrier coatings under thermal shock. Acta Mater. 2004, 52, 4189–4197. [Google Scholar] [CrossRef]
- Mehboob, G.; Xu, T.; Li, G.-R.; Hussain, S.; Mehboob, G.; Tahir, A. Strain-Induced Cracking Behavior of Coating/Substrate Systems and Strain Tolerant Design for Thick Coatings. Coatings 2020, 10, 1066. [Google Scholar] [CrossRef]
- Mehboob, G.; Xu, T.; Li, G.-R.; Yang, G.-J.; Tahir, A.; Ragab, M.; Hussain, S. Tailoring Periodic Vertical Cracks in Thermal Barrier Coatings Enabling High Strain Tolerance. Coatings 2021, 11, 720. [Google Scholar] [CrossRef]
- Lee, S.S.; Yoon, D.J.; Lee, J.H. Finite element analysis of residual stress in NiCrAlY/yttria-stabilized Zirconia Coatings by Nanoscale Multi-layered Deposition. Key Eng. Mater. 2004, 270–273, 58–63. [Google Scholar]
- Kim, J.H.; Paulino, G.H. Mixed-mode fracture of orthotropic functionally graded materials using finite elements and the modified crack closure method. Eng. Fract. Mech. 2002, 69, 1557–1586. [Google Scholar] [CrossRef]
- Rangaraj, S.; Kokini, K. Interface thermal fracture in functionally graded zirconia-mullite-bond coat alloy thermal barrier coatings. Acta Mater. 2003, 51, 251–267. [Google Scholar] [CrossRef]
- Zhou, B.; Kokini, K. The Effect of the Pre-Existing Surface Crack Morphologies on the Thermal Fracture of Ceramic Coatings. Doctoral Dissertation, Purdue University, West Lafayette, IN, USA, 2003. [Google Scholar]
- Gilber, A.; Kokini, A. Thermal fracture of zirconia-mullite composite thermal barrier coatings under thermal shock: A numerical study. Surf. Coat. Technol. 2008, 202, 2152–2161. [Google Scholar] [CrossRef]
- Khor, K.A.; Gu, Y.W. Effects of residual stress on the performance of plasma sprayed functionally graded ZrO2/NiCoCrAlY coatings. Mater. Sci. Eng. 2000, 277, 64–76. [Google Scholar] [CrossRef]
- Dombrovsky, L.A.; Rousseau, B.; Echegut, P.; Randrianalisoa, J.H.; Baillis, D. High Temperature Infrared Properties of YSZ Electrolyte Ceramics for SOFCs: Experimental Determination and Theoretical Modeling. Am. Ceram. Soc. 2011, 94, 4310–4316. [Google Scholar] [CrossRef]
- Patricio, M.; Mattheij, R.M.M. Crack Propagation Analysis; (CASA-Report; Vol. 0723); Eindhoven University of Technology: Eindhoven, The Netherlands, 2007. [Google Scholar]
- Eischien, J.R. Fracture of nonhomogeneous materials. Int. J. Fract. 1987, 34, 3–22. [Google Scholar] [CrossRef]
- Sollero, P.; Aliabodi, M.H. Fracture mechanics analysis of anisotropic plates by the boundary element method. Int. J. Fract. 1993, 64, 269–284. [Google Scholar] [CrossRef]
- Liebowitz, H.; Sih, G. Mathematical Theory of Brittle Fracture; Academic Press: New York, NY, USA, 1968. [Google Scholar]
- Batra, R.C.; Jin, Z.H. Some basic fracture mechanics concepts in functionally graded materials. J. Mech. Phys. Solids 1996, 44, 1221–1235. [Google Scholar]
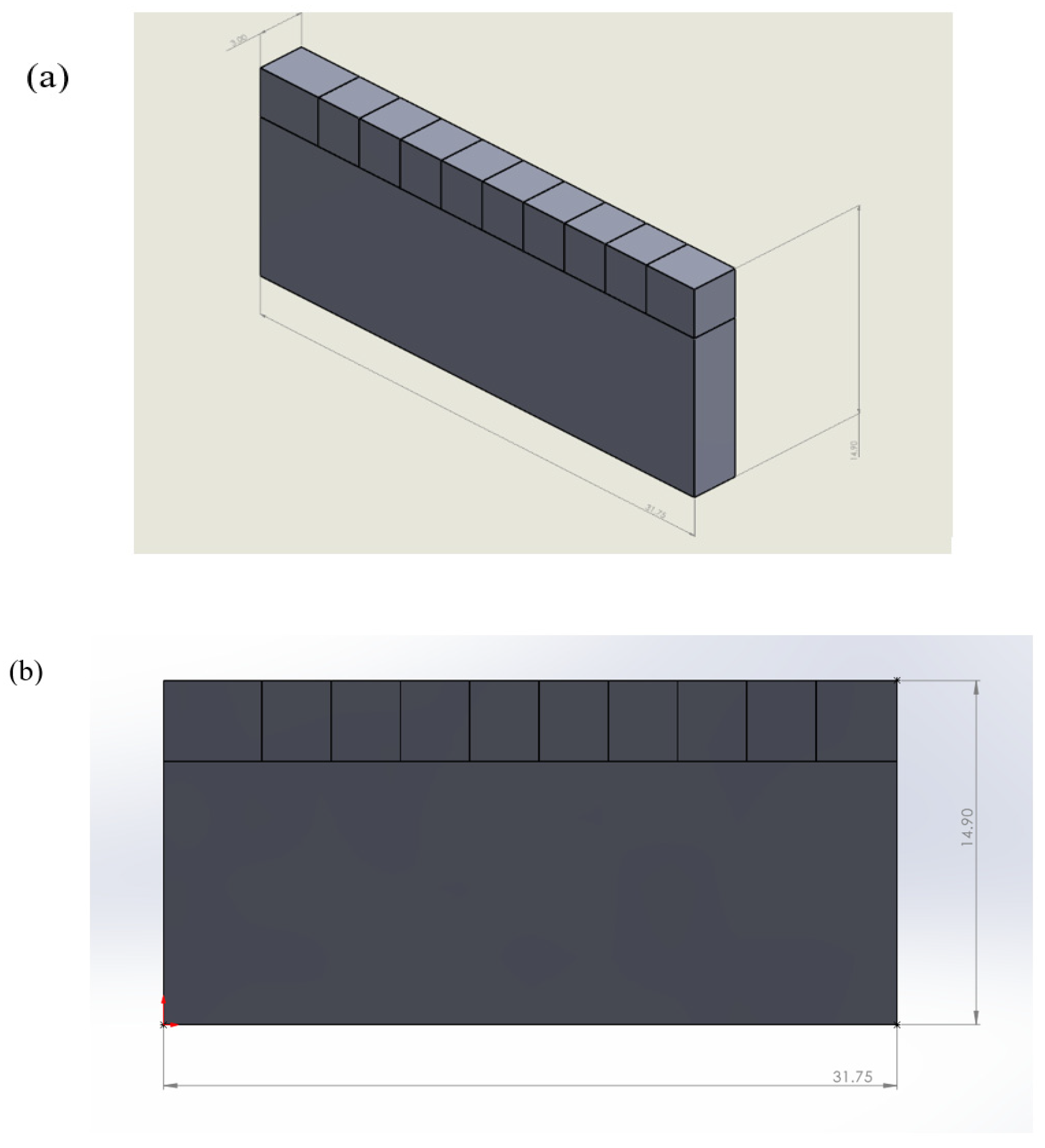
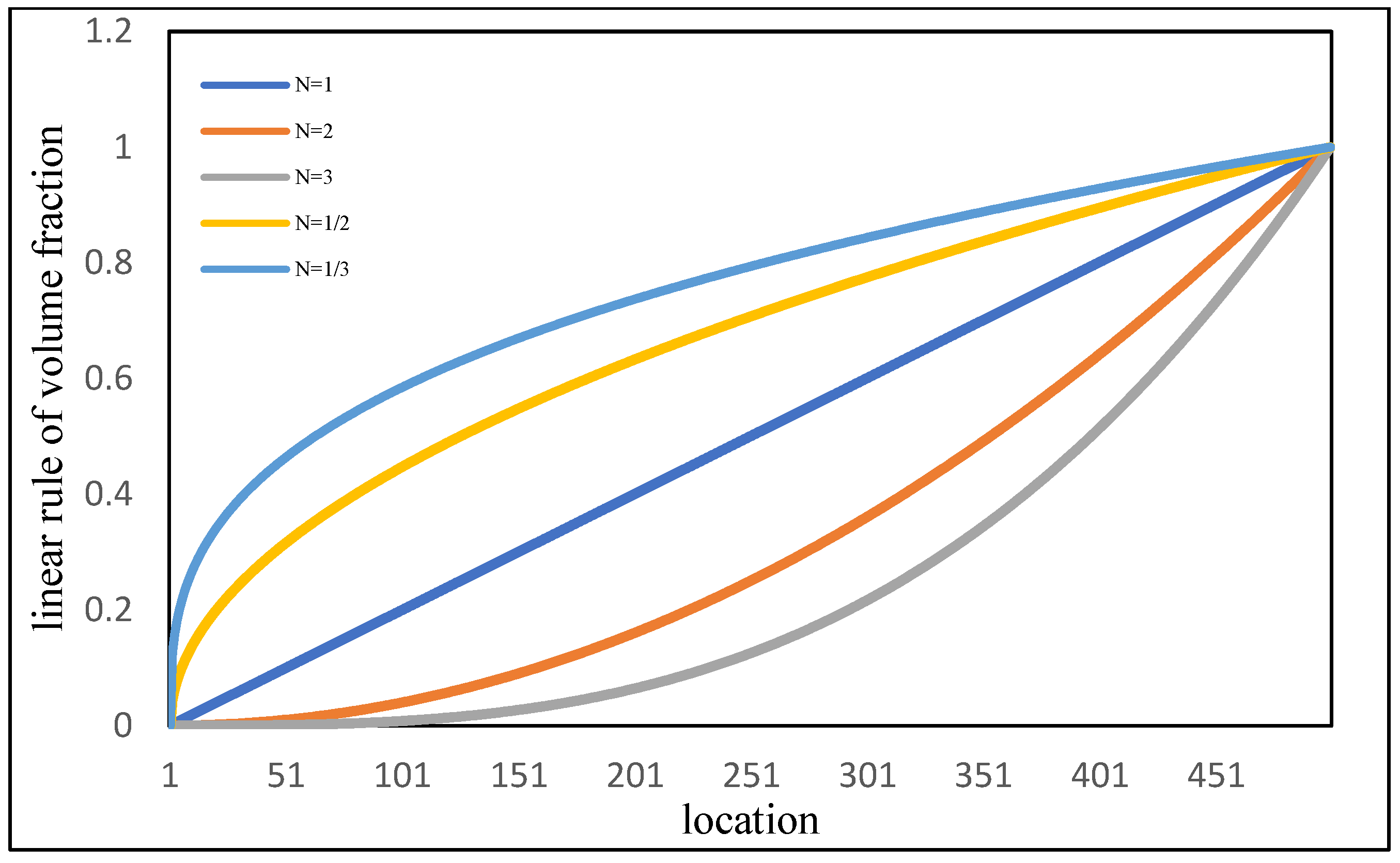
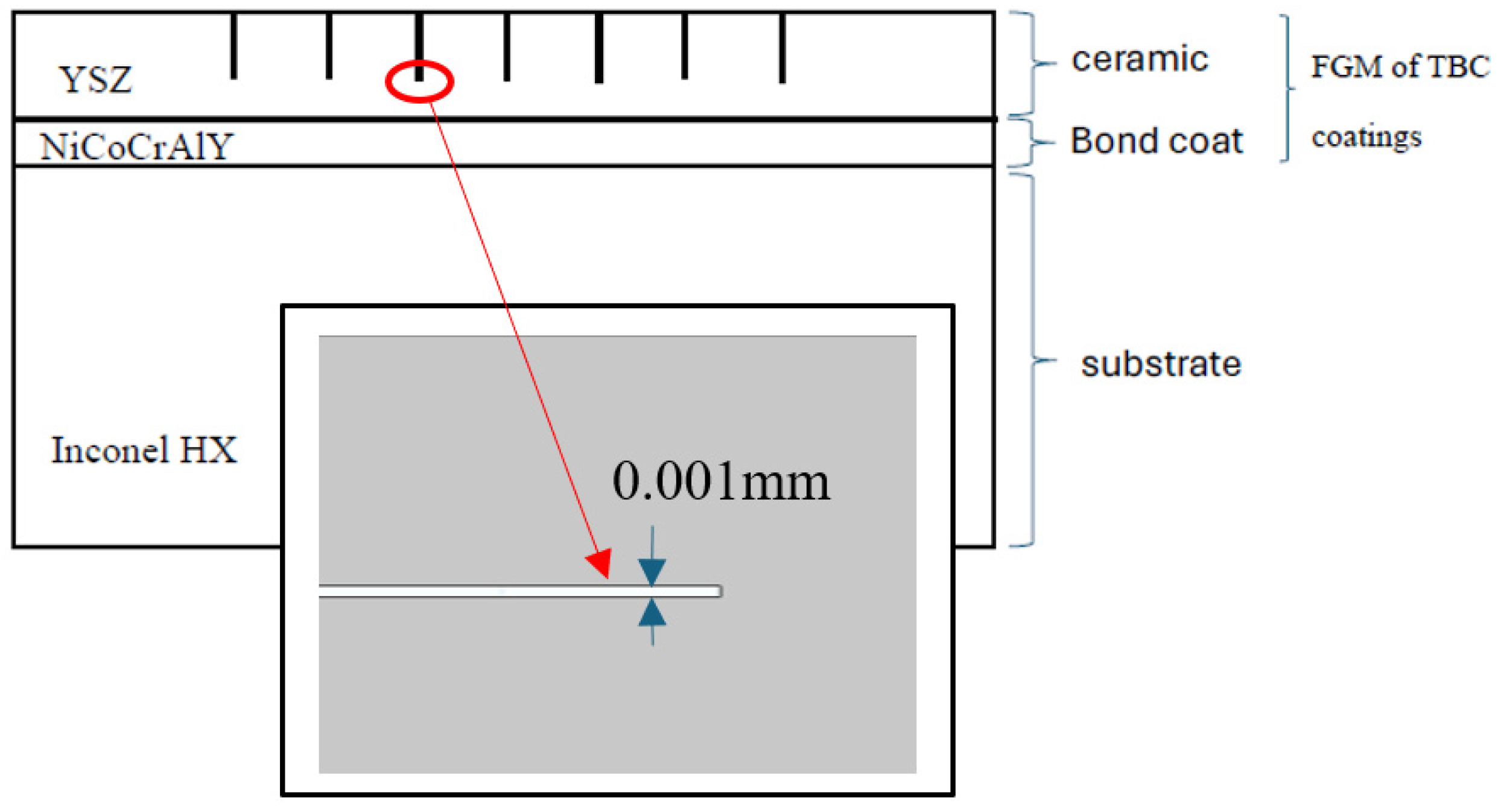
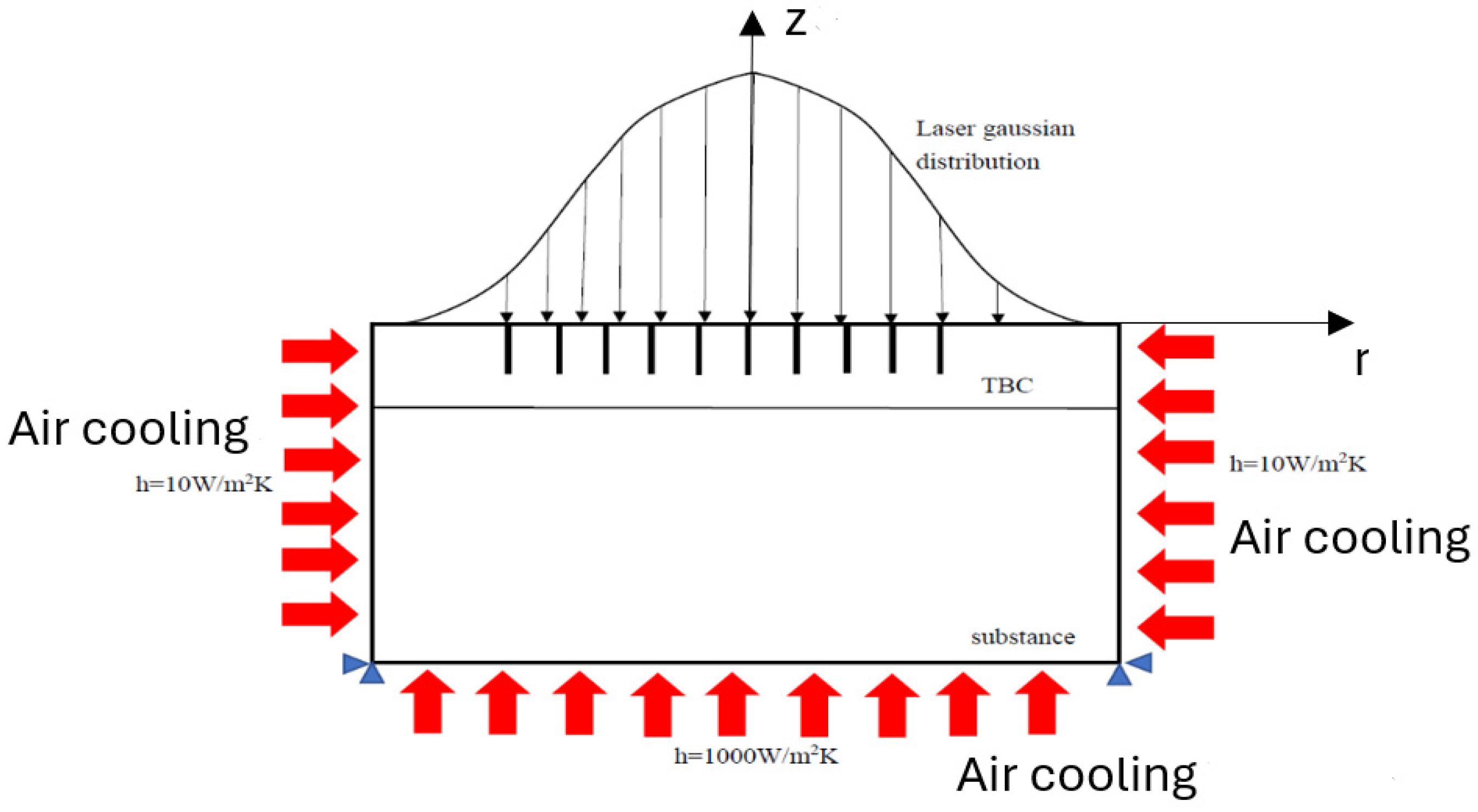












| Temperature (°K) | Young’s Modulus (GPa) | Density (kg/m3) | Poisson’s Ratio (m/m) | Thermal Conductivity (W/m°K) | Specific Heat | Thermal Expansion Coefficient (10−6/°K) | |
|---|---|---|---|---|---|---|---|
| INCO HX | 293 | 207 | 8880 | 0.312 | 90.5 | 461 (J/kg·K) | 12.7 |
| 673 | 182 | 8880 | 0.312 | 65.3 | 460 (J/kg·K) | 16.4 | |
| 1073 | 150 | 8880 | 0.312 | 73.9 | 460 (J/kg·K) | - | |
| NiCoCrAlY (BC, bond coating) | 300 | 64.5 | 6291 | 0.3 | 3.82 | 460 (J/kg·K) | 10.3 |
| 1000 | 53.0 | 6291 | 0.3 | 7.93 | 617 (J/kg·K) | 10.5 | |
| 1500 | 43.0 | 6291 | 0.3 | 9.86 | 617 (J/kg·K) | 11.4 | |
| YSZ | 300 | 13.6 | 5600 | 0.25 | 1.01 | 500 (J/kg·K) | 7.5 |
| 1000 | 10.4 | 5600 | 0.25 | 0.83 | 637 (J/kg·K) | 9.0 | |
| 1500 | 8.0 | 5600 | 0.25 | 0.83 | 656 (J/kg·K) | 9.7 |
| a/w | KI Calculated Using DCT Method | KI Calculated Using J-Integral |
|---|---|---|
| 0.25 | 1.129 | 1.109 |
| 0.50 | 1.181 | 1.162 |
| 0.75 | 1.465 | 1.437 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lo, K.-C.; Kuo, J.-K.; Huang, P.-H.; Hsiao, C.-Y. Using Preexisting Surface Cracks to Prevent Thermal Fatigue Failure and Crack Delamination in FGM Thermal Barrier Coatings. Coatings 2025, 15, 362. https://doi.org/10.3390/coatings15030362
Lo K-C, Kuo J-K, Huang P-H, Hsiao C-Y. Using Preexisting Surface Cracks to Prevent Thermal Fatigue Failure and Crack Delamination in FGM Thermal Barrier Coatings. Coatings. 2025; 15(3):362. https://doi.org/10.3390/coatings15030362
Chicago/Turabian StyleLo, Kai-Chien, Jenn-Kun Kuo, Pei-Hsing Huang, and Chang-Yu Hsiao. 2025. "Using Preexisting Surface Cracks to Prevent Thermal Fatigue Failure and Crack Delamination in FGM Thermal Barrier Coatings" Coatings 15, no. 3: 362. https://doi.org/10.3390/coatings15030362
APA StyleLo, K.-C., Kuo, J.-K., Huang, P.-H., & Hsiao, C.-Y. (2025). Using Preexisting Surface Cracks to Prevent Thermal Fatigue Failure and Crack Delamination in FGM Thermal Barrier Coatings. Coatings, 15(3), 362. https://doi.org/10.3390/coatings15030362








