Residual Stress Model in Laser Direct Deposition Based on Energy Equation
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Residual Stresses
3.2. Mathematical Thermal Strain/Stress Model Under CW and PW Laser Modes
Methodology
3.3. Model Validation
- (1)
- A new process (PW laser process) has been provided to reduce and eliminate residual stress in additive manufacturing deposits. This process can achieve the effect of reducing accumulated thermal strain and residual stress by forming compressive strain and partially offsetting tensile strain during the laser additive manufacturing process under the same heat input as traditional continuous laser additive manufacturing;
- (2)
- By establishing mathematical models of CW and PW thermal stress evolution, the mechanism of residual stress elimination in PW laser mode was fundamentally explained, and the correctness of the model was verified through experiments. The relevant laser process parameters, deposition part size parameters, and material parameters can be directly input into the mathematical model to obtain the thermal stress evolution process of the corresponding materials and processes of the deposited parts in the laser additive manufacturing process. This lays the foundation for predicting the thermal stress of deposited parts in multi-size/multi-material laser additive manufacturing and eliminating residual stress. In the future, the PW process and the impact of pulsed laser technology on residual stress in additive manufacturing deposited parts will be further optimized and explored, laying the foundation for manufacturing low-stress additive manufacturing workpieces.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xie, D.; Lv, F.; Yang, Y.; Shen, L.; Tian, Z.; Shuai, C.; Chen, B.; Zhao, J. A Review on Distortion and Residual Stress in Additive Manufacturing. Chin. J. Mech. Eng. Addit. Manuf. Front. 2022, 1, 100039. [Google Scholar] [CrossRef]
- Zhao, H.; Liu, Z.; Yu, C.; Liu, C.; Zhan, Y. Finite element analysis for residual stress of TC4/Inconel718 functionally gradient materials produced by laser additive manufacturing. Opt. Laser Technol. 2022, 152, 108146. [Google Scholar] [CrossRef]
- Chen, S.-G.; Gao, H.-J.; Zhang, Y.-D.; Wu, Q.; Gao, Z.-H.; Zhou, X. Review on residual stresses in metal additive manufacturing: Formation mechanisms, parameter dependencies, prediction and control approaches. J. Mater. Res. Technol. 2022, 17, 2950–2974. [Google Scholar] [CrossRef]
- Bartlett, J.L.; Li, X. An overview of residual stresses in metal powder bed fusion. Addit. Manuf. 2019, 27, 131–149. [Google Scholar] [CrossRef]
- Fang, Z.-C.; Wu, Z.-L.; Huang, C.-G.; Wu, C.-W. Review on residual stress in selective laser melting additive manufacturing of alloy parts. Opt. Laser Technol. 2020, 129, 106283. [Google Scholar] [CrossRef]
- Bin Lai, Y.; Liu, W.J.; Bin Zhao, J.; Zhao, Y.H.; Wang, F.Y.; Han, W.C. Experimental Study on Residual Stress in Titanium Alloy Laser Additive Manufacturing. Appl. Mech. Mater. 2013, 431, 20–26. [Google Scholar]
- Zaeh, M.F.; Branner, G. Investigations on residual stresses and deformations in selective laser melting. Prod. Eng. 2009, 4, 35–45. [Google Scholar] [CrossRef]
- Vasinonta, A.; Beuth, J.; Griffith, M. Process Maps for Controlling Residual Stress and Melt Pool Size in Laser-Based SFF Processes; Department of Mechanical Engineering Carnegie Mellon University: Pittsburgh, PA, USA, 2000. [Google Scholar]
- Shiomi, M.; Osakada, K.; Nakamura, K.; Yamashita, T.; Abe, F. Residual Stress within Metallic Model Made by Selective Laser Melting Process. CIRP Ann. 2004, 53, 195–198. [Google Scholar] [CrossRef]
- Wu, A.S.; Brown, D.W.; Kumar, M.; Gallegos, G.F.; King, W.E. An Experimental Investigation into Additive Manufacturing-Induced Residual Stresses in 316L Stainless Steel. Metall. Mater. Trans. A 2014, 45, 6260–6270. [Google Scholar] [CrossRef]
- Aggarangsi, P.; Beuth, J.L. Localized Preheating Approaches for Reducing Residual Stress. In Proceedings of the 2006 International Solid Freeform Fabrication Symposium, Austin, TX, USA, 14–16 August 2006; pp. 710–720. [Google Scholar]
- Martina, F.; Roy, M.J.; Szost, B.A.; Terzi, S.; Colegrove, P.A.; Williams, S.W.; Withers, P.J.; Meyer, J.; Hofmann, M. Residual stress of as-deposited and rolled wire+arc additive manufacturing Ti–6Al–4V components. Mater. Sci. Technol. 2016, 32, 1439–1448. [Google Scholar] [CrossRef]
- Zhao, J.; Quan, G.-Z.; Zhang, Y.-Q.; Ma, Y.-Y.; Jiang, L.-H.; Dai, W.-W.; Jiang, Q. Influence of deposition path strategy on residual stress and deformation in weaving wire-arc additive manufacturing of disc parts. J. Mater. Res. Technol. 2024, 30, 2241–2256. [Google Scholar] [CrossRef]
- Chen, X.; Xie, X.; Wu, H.; Ji, X.; Shen, H.; Xue, M.; Wu, H.; Chao, Q.; Fan, G.; Liu, Q. In-situ control of residual stress and its distribution in a titanium alloy additively manufactured by laser powder bed fusion. Mater. Charact. 2023, 201, 112953. [Google Scholar] [CrossRef]
- Mathews, R.; Karandikar, J.; Tyler, C.; Smith, S. Residual stress accumulation in large-scale Ti-6Al-4V wire-arc additive manufacturing. Procedia CIRP 2024, 121, 180–185. [Google Scholar] [CrossRef]
- Ghanavati, R.; Naffakh-Moosavy, H.; Moradi, M.; Gadalińska, E.; Saboori, A. Residual stresses and distortion in additively-manufactured SS316L-IN718 multi-material by laser-directed energy deposition: A validated numerical-statistical approach. J. Manuf. Process. 2023, 108, 292–309. [Google Scholar] [CrossRef]
- Klein, G.H.; Karunakaran, R.; Sealy, M.P. Thermal redistribution of compressive residual stress introduced by interlayer laser shock peening in hybrid additive manufacturing. Procedia CIRP 2024, 121, 67–72. [Google Scholar] [CrossRef]
- Zou, X.; Chang, T.; Yan, Z.; Zhao, Z.S.; Pan, Y.; Liu, W.; Song, L. Control of thermal strain and residual stress in pulsed-wave direct laser deposition. Opt. Laser Technol. 2023, 163, 109386. [Google Scholar] [CrossRef]
- Yan, Z.; Song, L.; Liu, W.; Zou, X.; Zhou, Z. Numerical analysis of thermal stress evolution of pulsed-wave laser direct energy deposition. Int. J. Adv. Manuf. Technol. 2021, 115, 1399–1410. [Google Scholar] [CrossRef]
- Prime, M.B.; Sebring, R.J.; Edwards, J.M.; Hughes, D.J.; Webster, P.J. Laser Surface-contouring and Spline Data-smoothing for Residual Stress Measurement. Exp. Mech. 2004, 44, 176–184. [Google Scholar] [CrossRef]
- Biegler, M.; Graf, B.; Rethmeier, M. In-situ distortions in LMD additive manufacturing walls can be measured with digital image correlation and predicted using numerical simulations. Addit. Manuf. 2018, 20, 101–110. [Google Scholar] [CrossRef]
- Xie, R.; Chen, G.; Zhao, Y.; Zhang, S.; Yan, W.; Lin, X.; Shi, Q. In-situ observation and numerical simulation on the transient strain and distortion prediction during additive manufacturing. J. Manuf. Process. 2019, 38, 494–501. [Google Scholar] [CrossRef]
- Leggatt, R.H. Residual stresses in welded structures. Int. J. Press. Vessel. Pip. 2008, 85, 144–151. [Google Scholar] [CrossRef]
- Yakout, M.; Elbestawi, M.; Veldhuis, S.C. Density and mechanical properties in selective laser melting of Invar 36 and stainless steel 316L. J. Mater. Process. Tech. 2019, 266, 397–420. [Google Scholar] [CrossRef]
- DHann, D.B.; Iammi, J.; Folkes, J. A simple methodology for predicting laser-weld properties from material and laser parameters. J. Phys. D Appl. Phys. 2011, 44, 445401. [Google Scholar]
- Shamsundar, N. Approximate Calculation of Multidimensional Solidification by Using Conduction Shape Factors. J. Heat Transf. 1982, 104, 8–12. [Google Scholar] [CrossRef]
- Bejan, A.; Kraus, A.D. Heat Transfer Handbook; Wiley: Hoboken, NJ, USA, 2003. [Google Scholar]

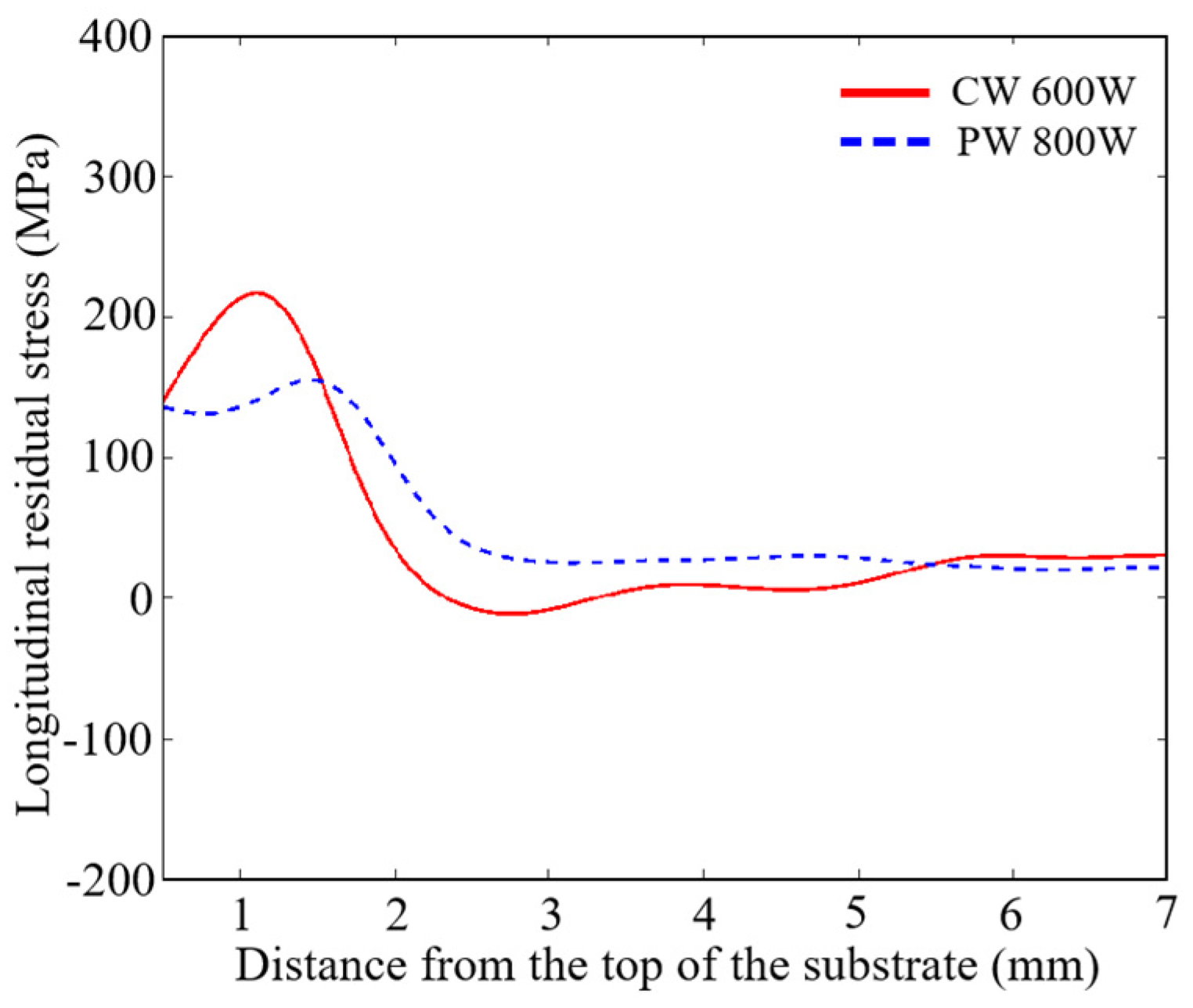


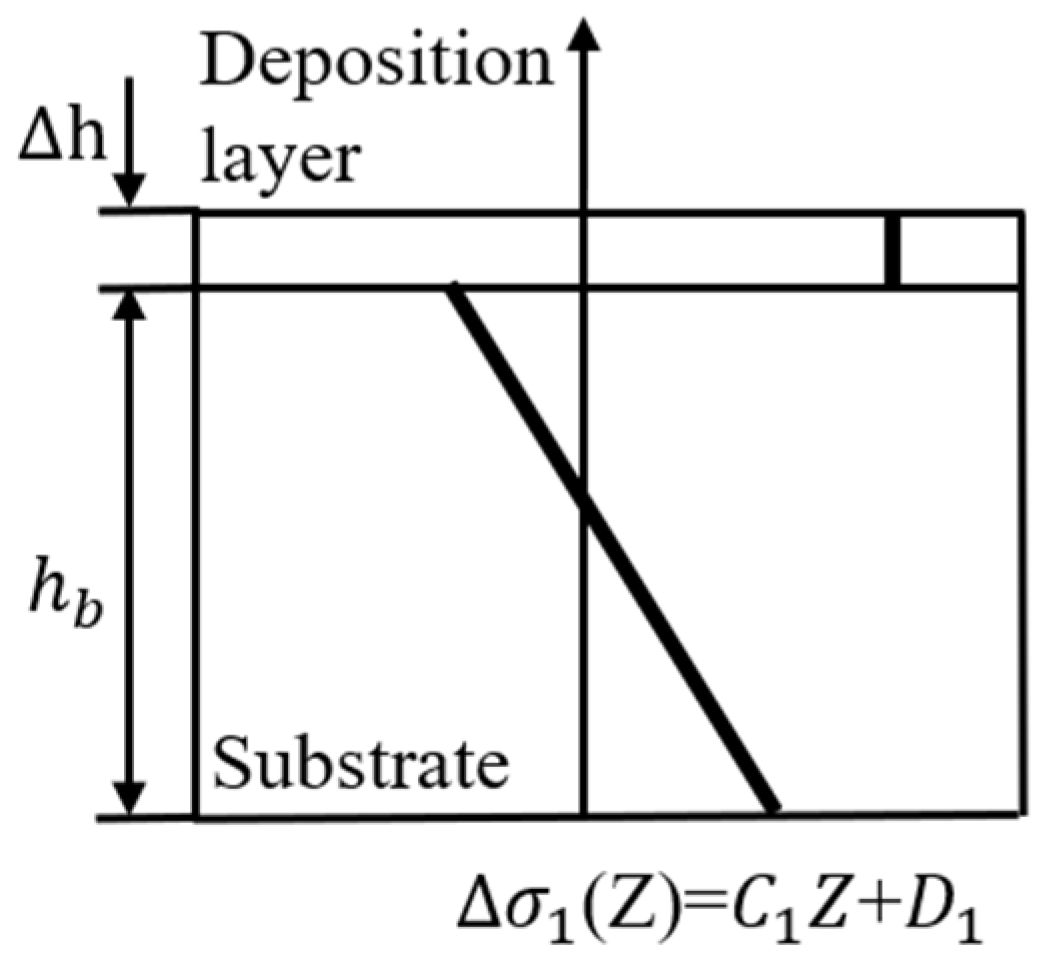
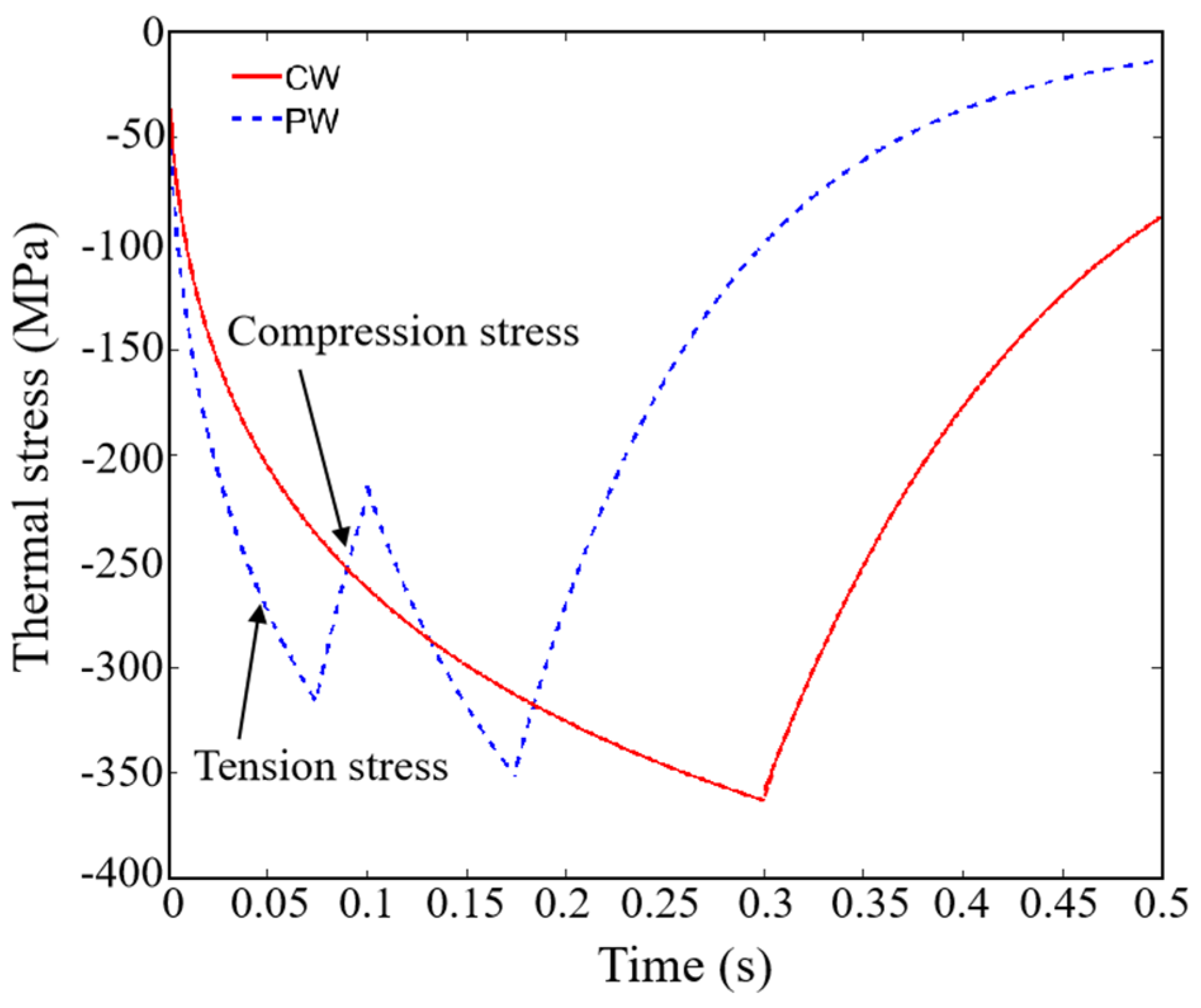
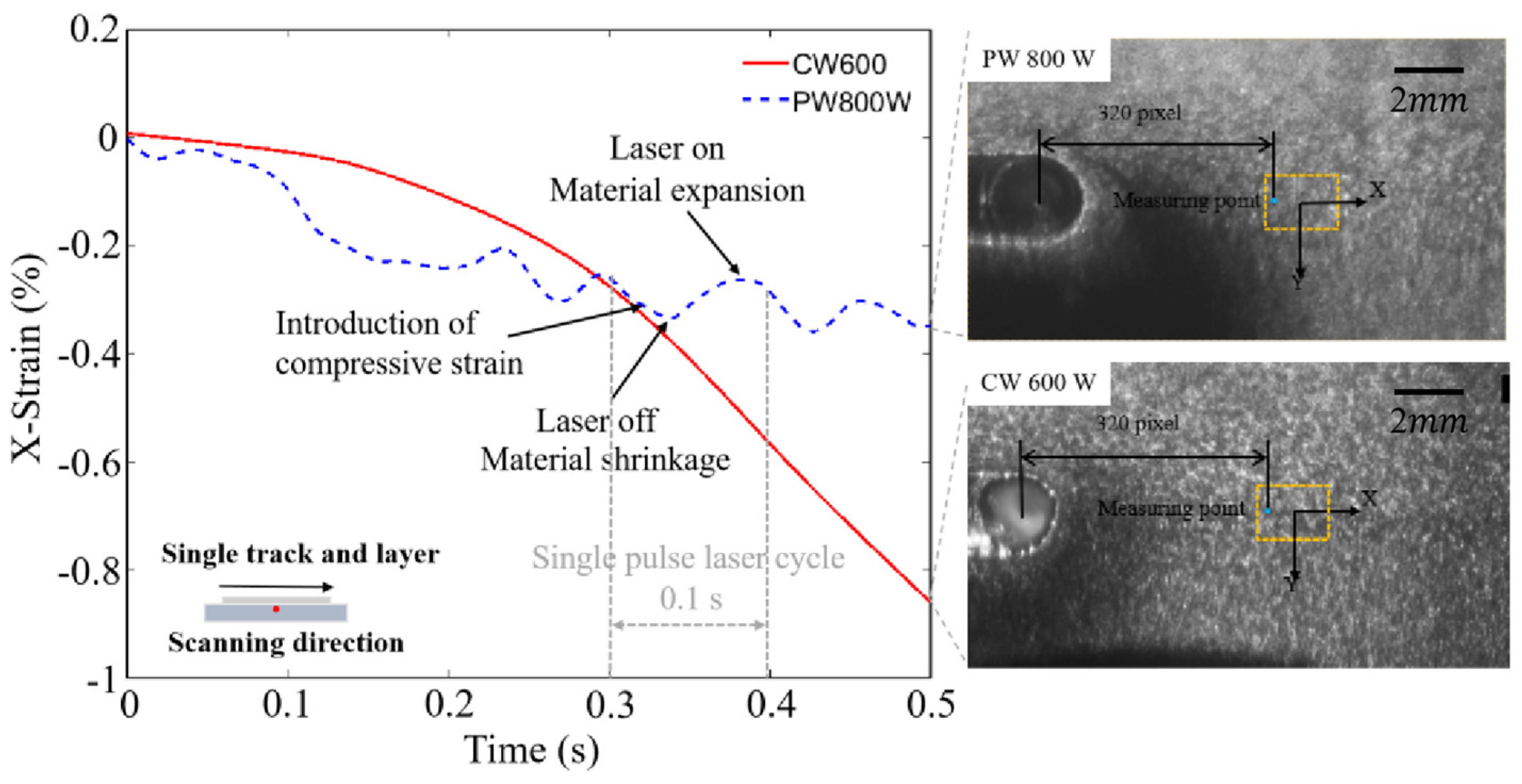
| Sample Number | Laser Mode | Laser Power (W) | Scanning Speed (mm/s) | Duty Cycle | Frequency (HZ) |
|---|---|---|---|---|---|
| S1 | CW | 600 | 8 | / | / |
| S2 | PW | 800 | 8 | 75% | 10 |
| Laser Mode | CW | PW | |
|---|---|---|---|
| Process parameters | Laser power: W | 600 | 800 |
| Laser absorptivity | 0.4 | ||
| scanning speed: m/s | 0.008 | ||
| Deposition height: m | 0.0002 | ||
| Substrate height m | 0.01 | ||
| Spot radius: m | 0.0006 | ||
| Duty cycle: | - | 75% | |
| Frequency: Hz | - | 10 | |
| Material parameters | Thermal diffusivity: m2/s | 5.38 × 10−6 | |
| Specific heat capacity: J/(kg.)) | 0.46 × 103 | ||
| Material density: kg/m3 | 7950 | ||
| Coefficient of thermal expansion: /K | 1.2 × 10−5 | ||
| Modulus of elasticity: pa | 2 × 1011 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, M.; Zou, X.; Gong, M.; Chang, T.; Cao, Q.; Ju, H. Residual Stress Model in Laser Direct Deposition Based on Energy Equation. Coatings 2025, 15, 217. https://doi.org/10.3390/coatings15020217
Cheng M, Zou X, Gong M, Chang T, Cao Q, Ju H. Residual Stress Model in Laser Direct Deposition Based on Energy Equation. Coatings. 2025; 15(2):217. https://doi.org/10.3390/coatings15020217
Chicago/Turabian StyleCheng, Manping, Xi Zou, Muhong Gong, Tengfei Chang, Qi Cao, and Houlai Ju. 2025. "Residual Stress Model in Laser Direct Deposition Based on Energy Equation" Coatings 15, no. 2: 217. https://doi.org/10.3390/coatings15020217
APA StyleCheng, M., Zou, X., Gong, M., Chang, T., Cao, Q., & Ju, H. (2025). Residual Stress Model in Laser Direct Deposition Based on Energy Equation. Coatings, 15(2), 217. https://doi.org/10.3390/coatings15020217





