Analysis of Motile Gyrotactic Micro-Organisms for the Bioconvection Peristaltic Flow of Carreau–Yasuda Bionanomaterials
Abstract
1. Introduction
2. Problem Formulation
3. Numerical Method
4. Results and Discussion
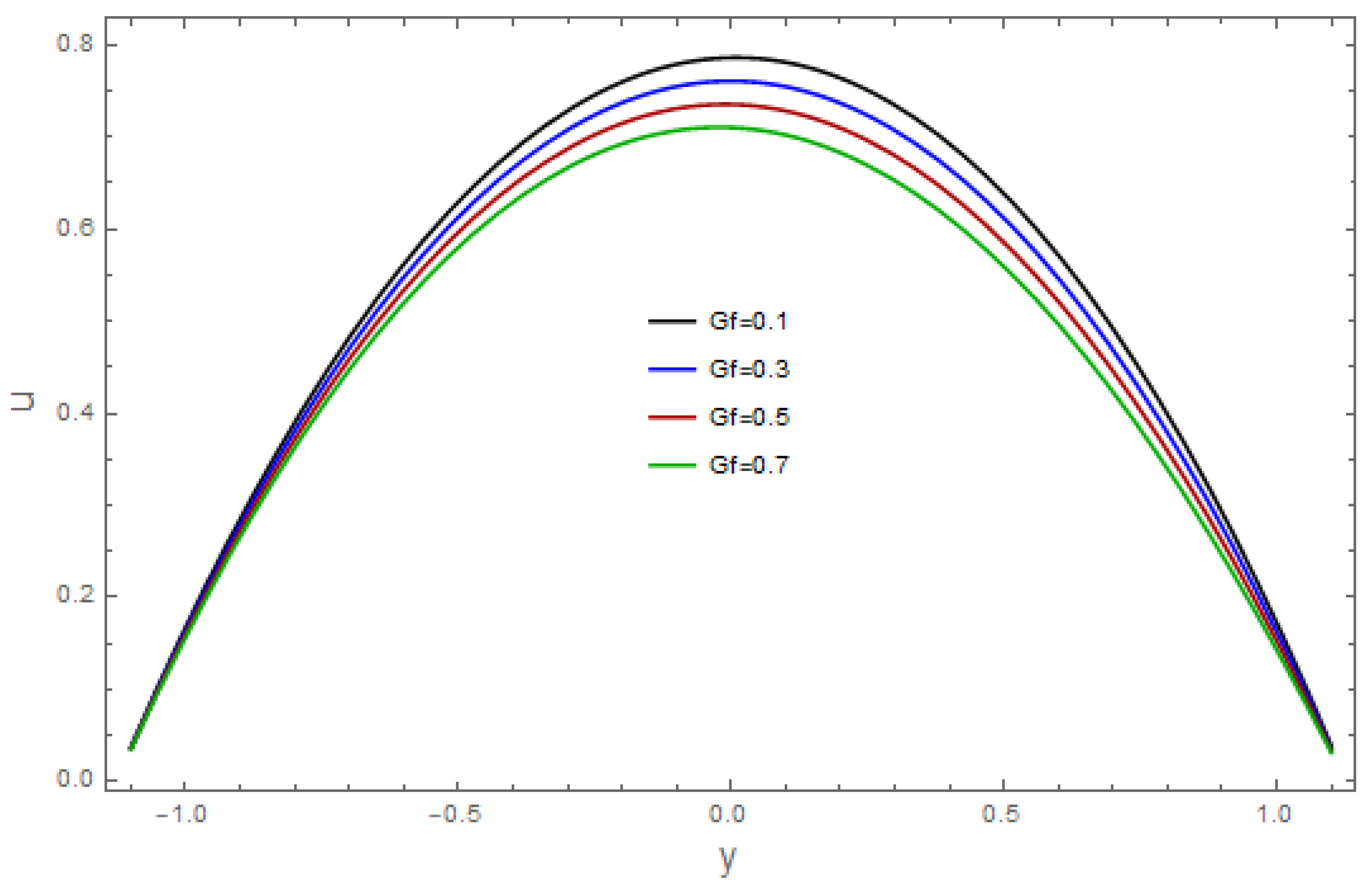
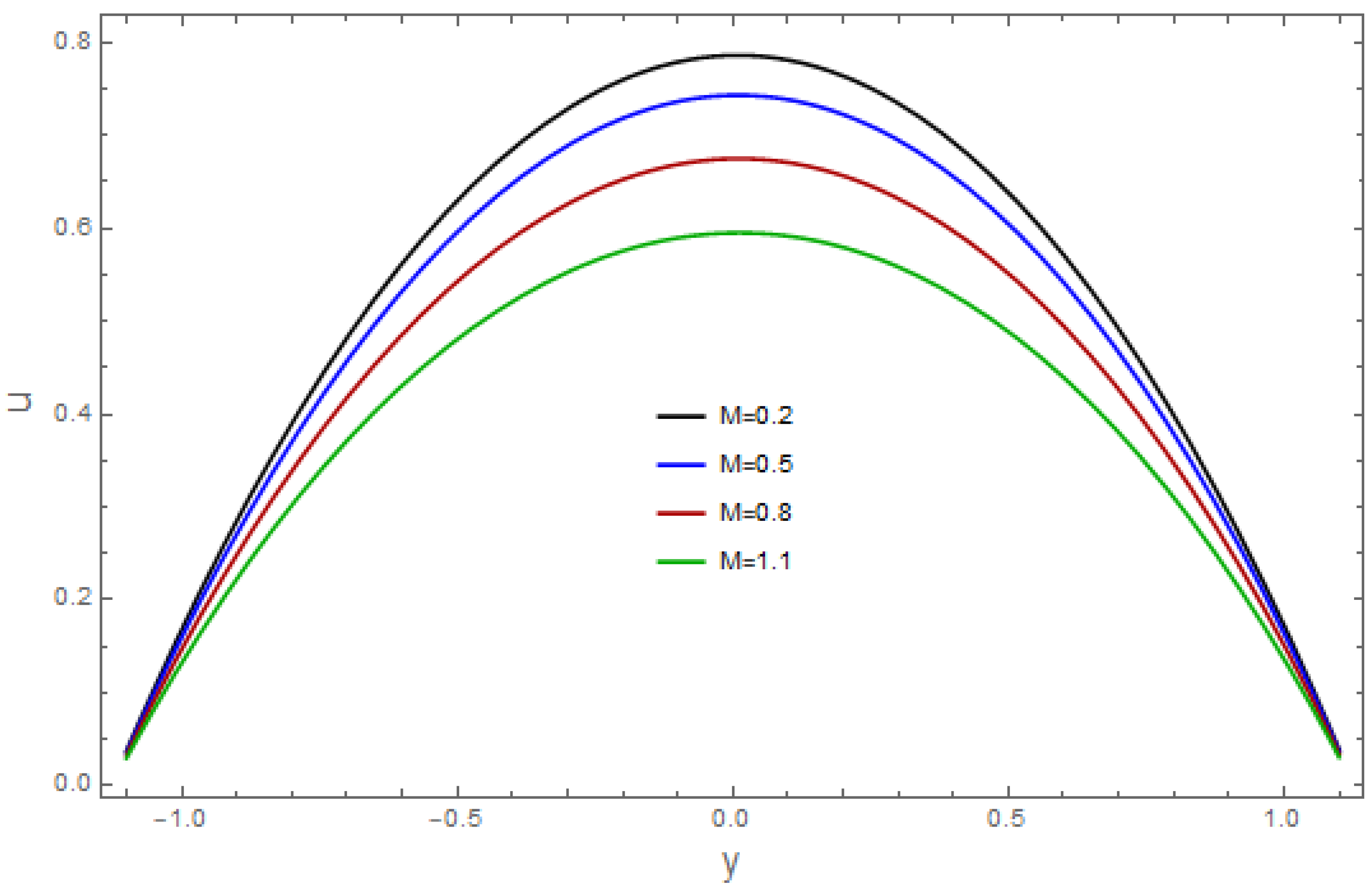
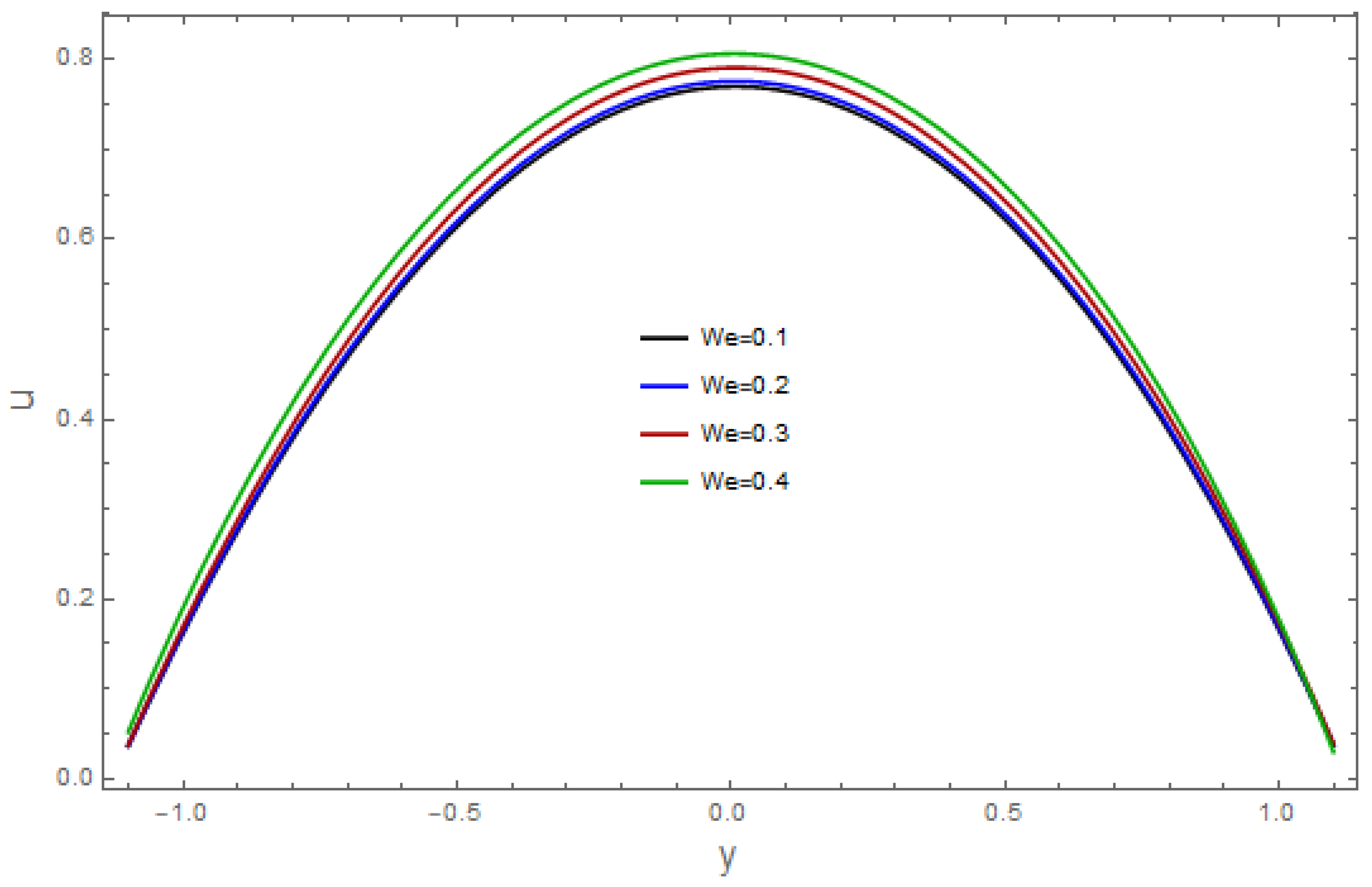
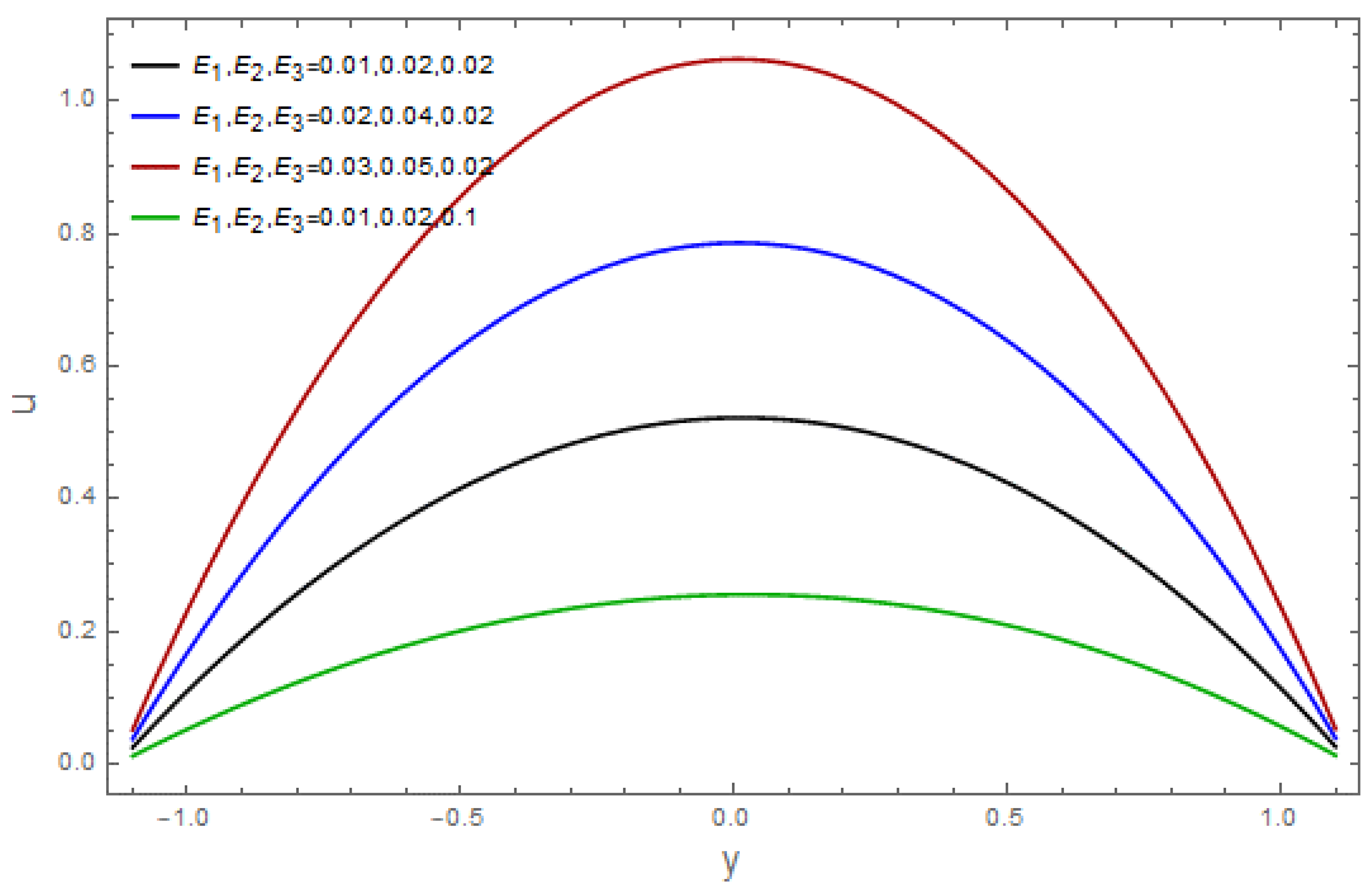
4.1. Velocity
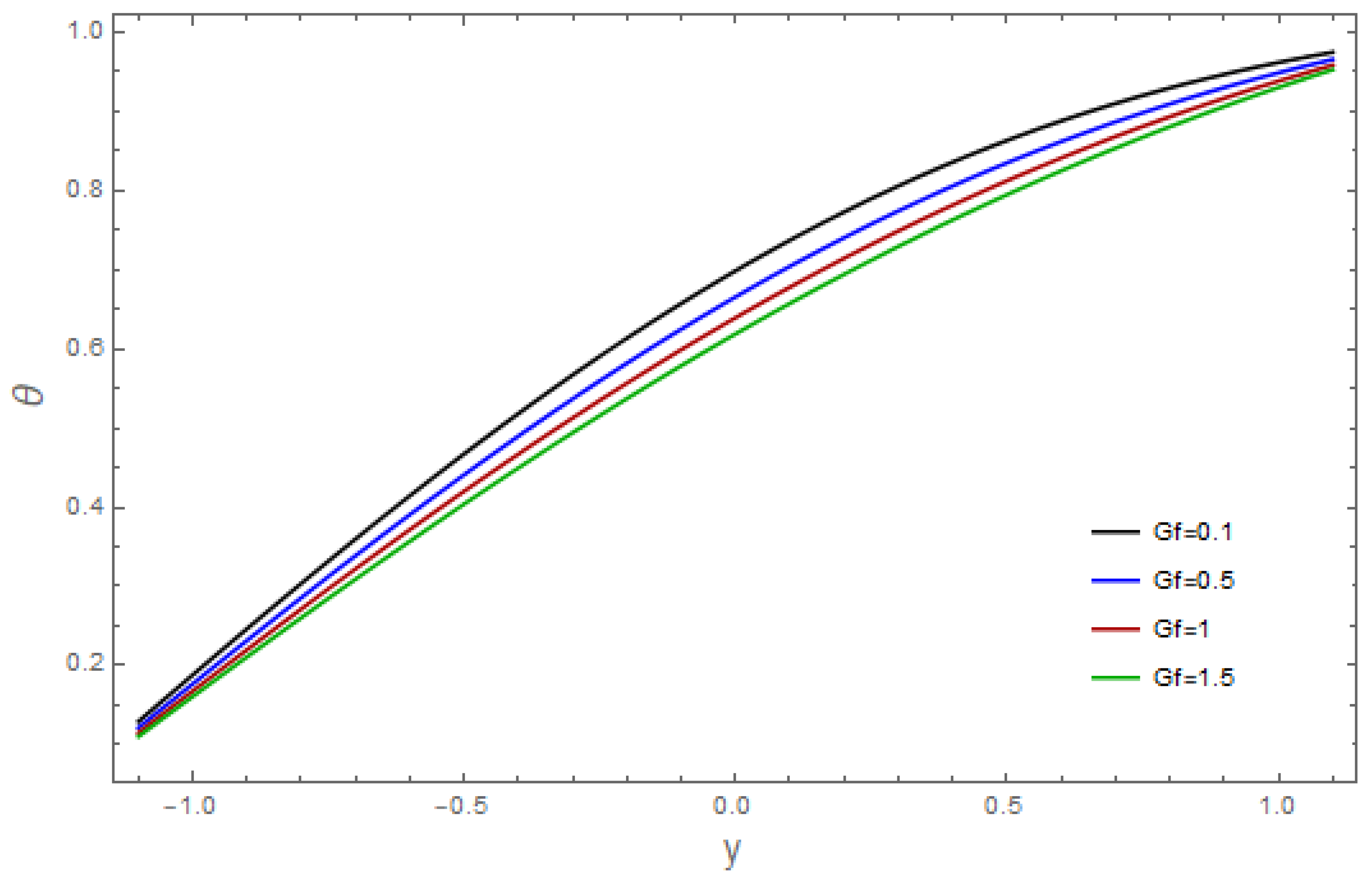
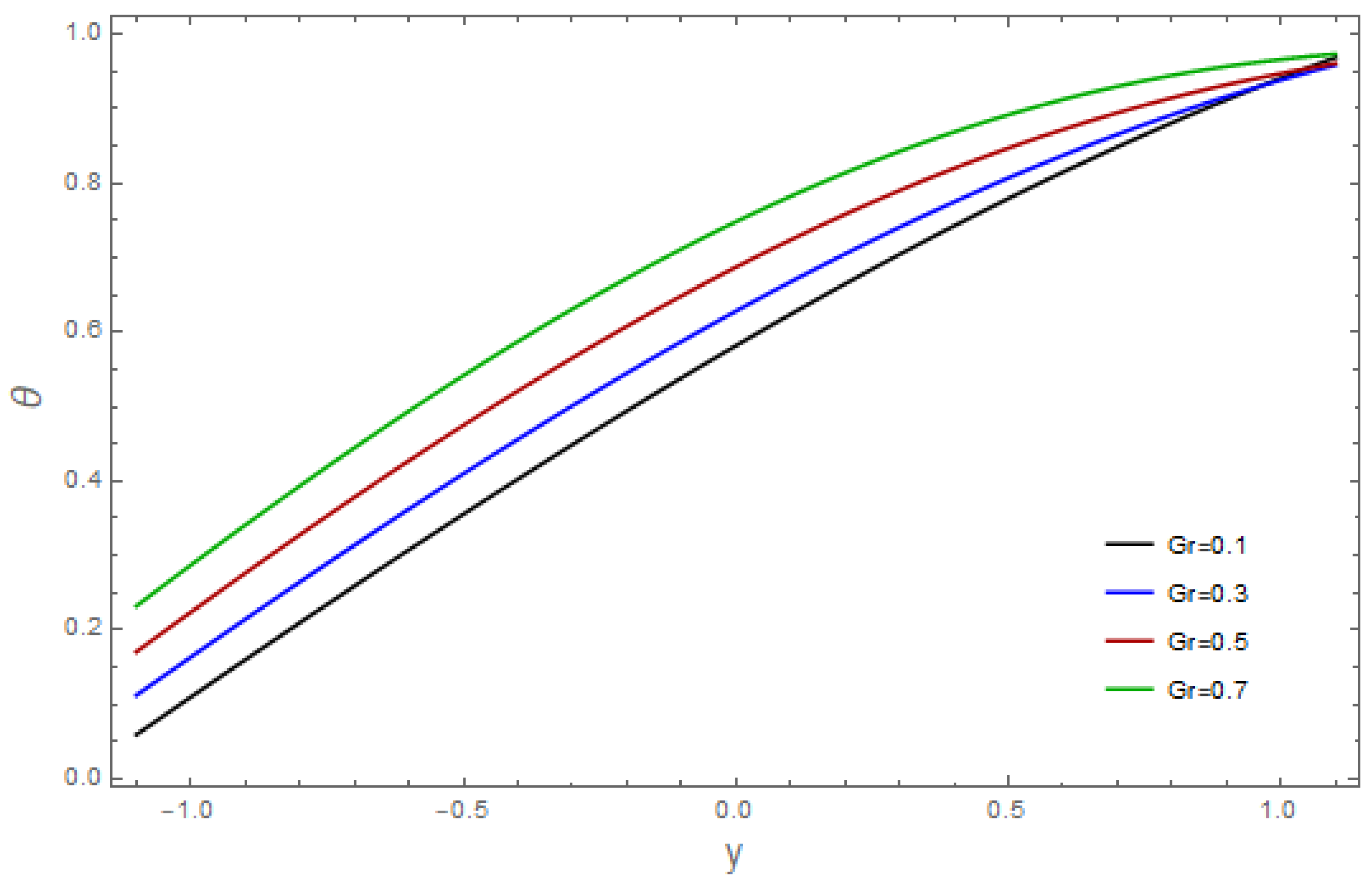
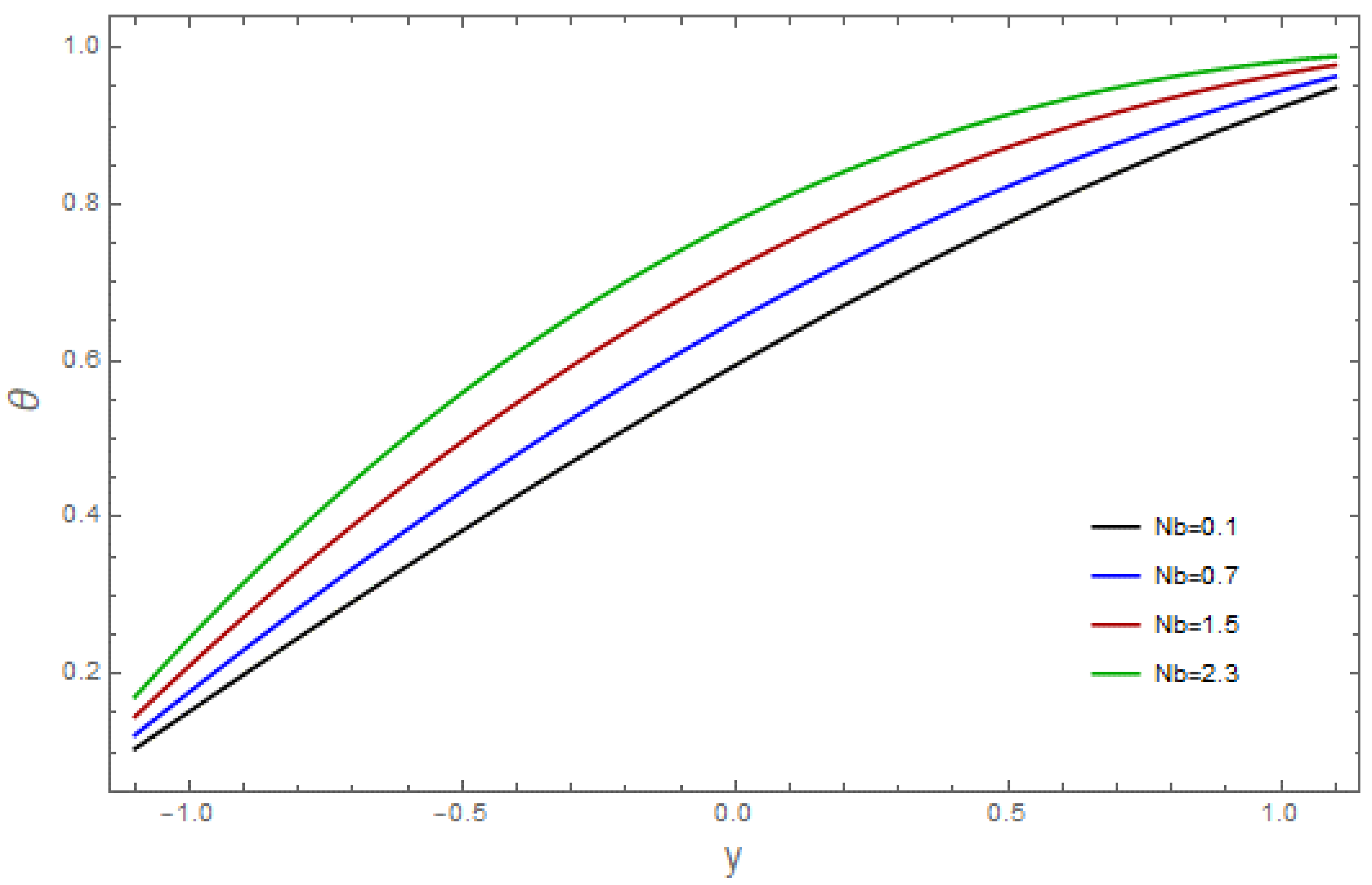
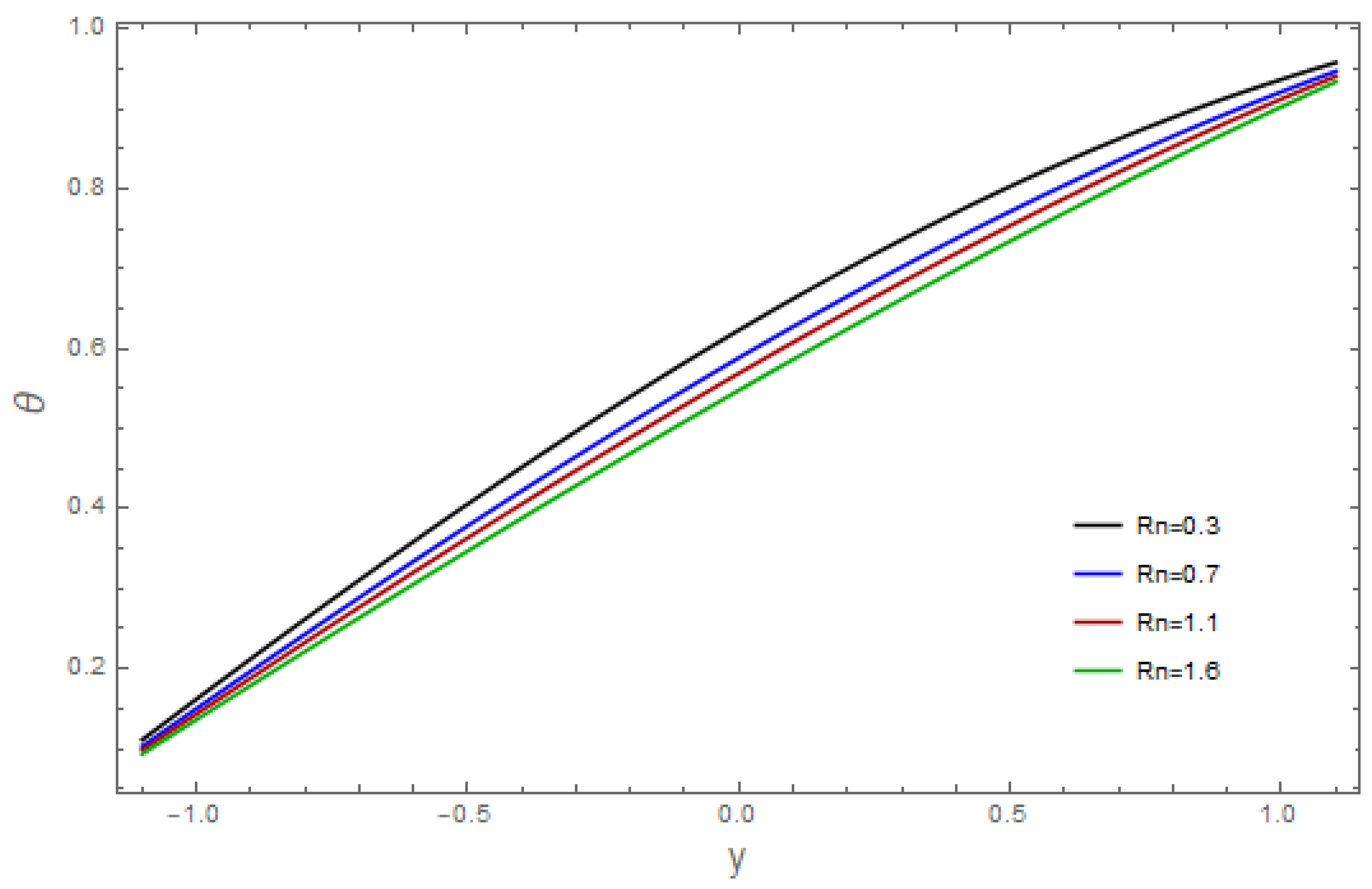
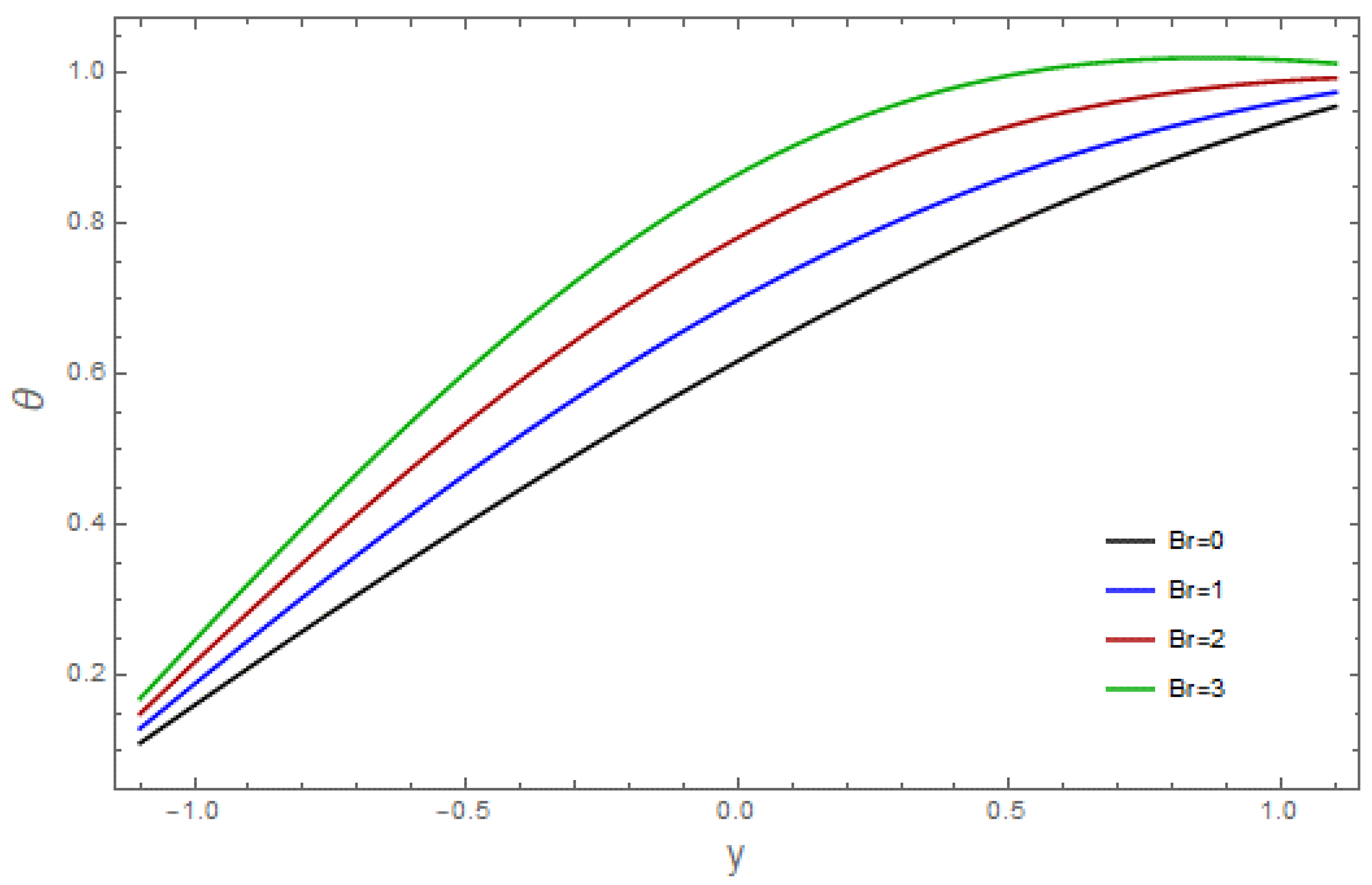
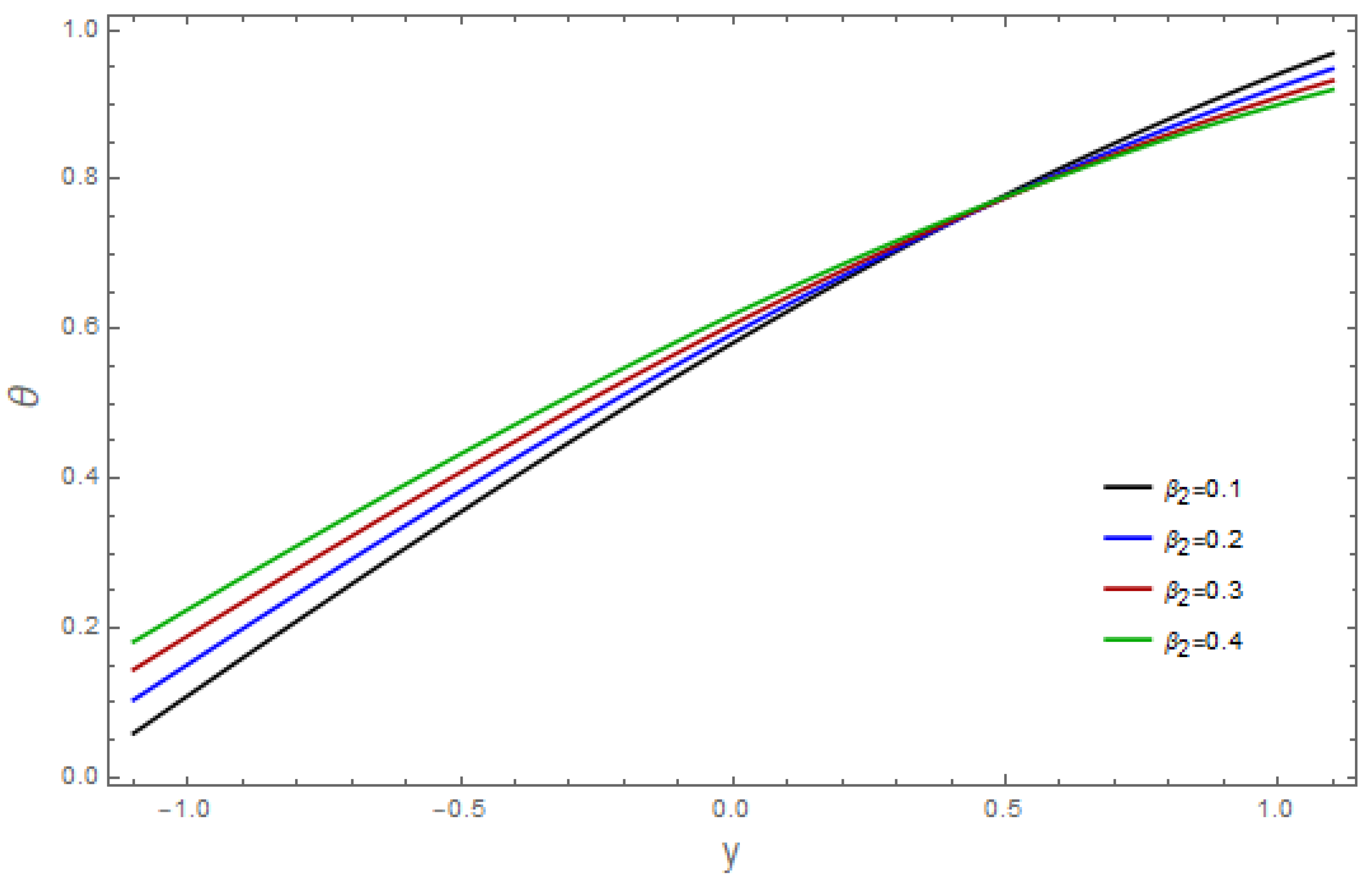
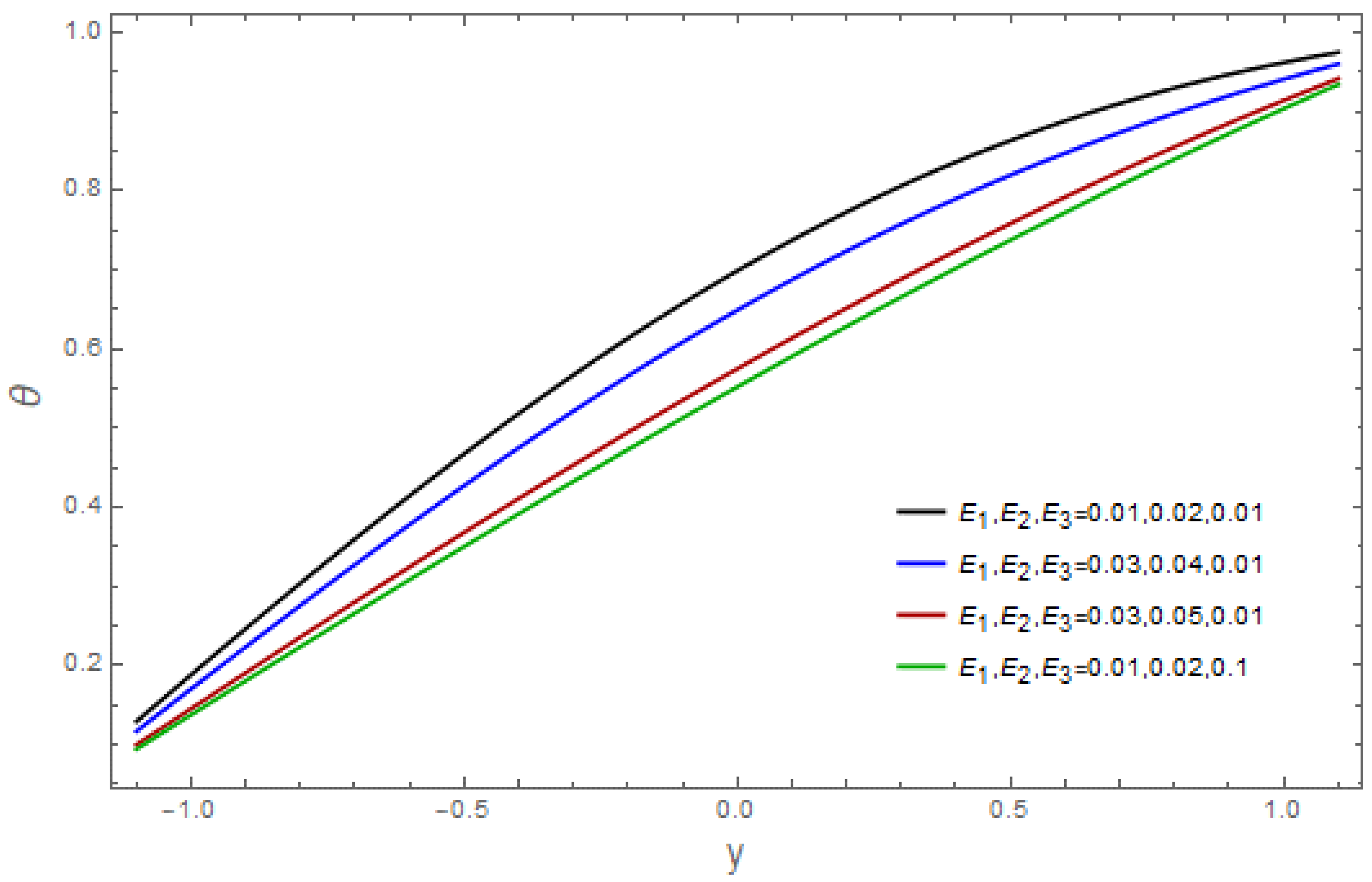
4.2. Temperature
4.3. Profiles of Motile Micro–Organisms
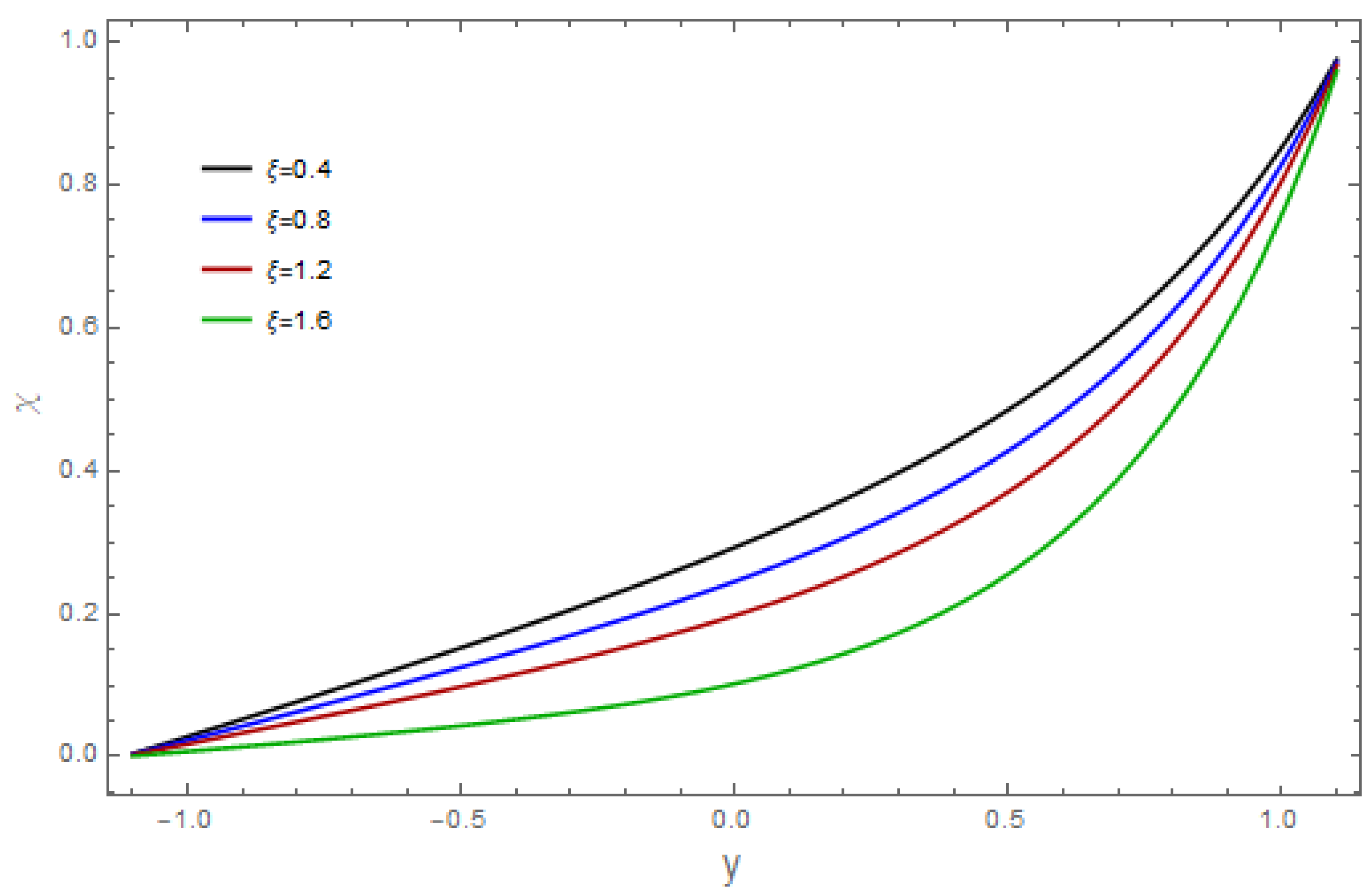
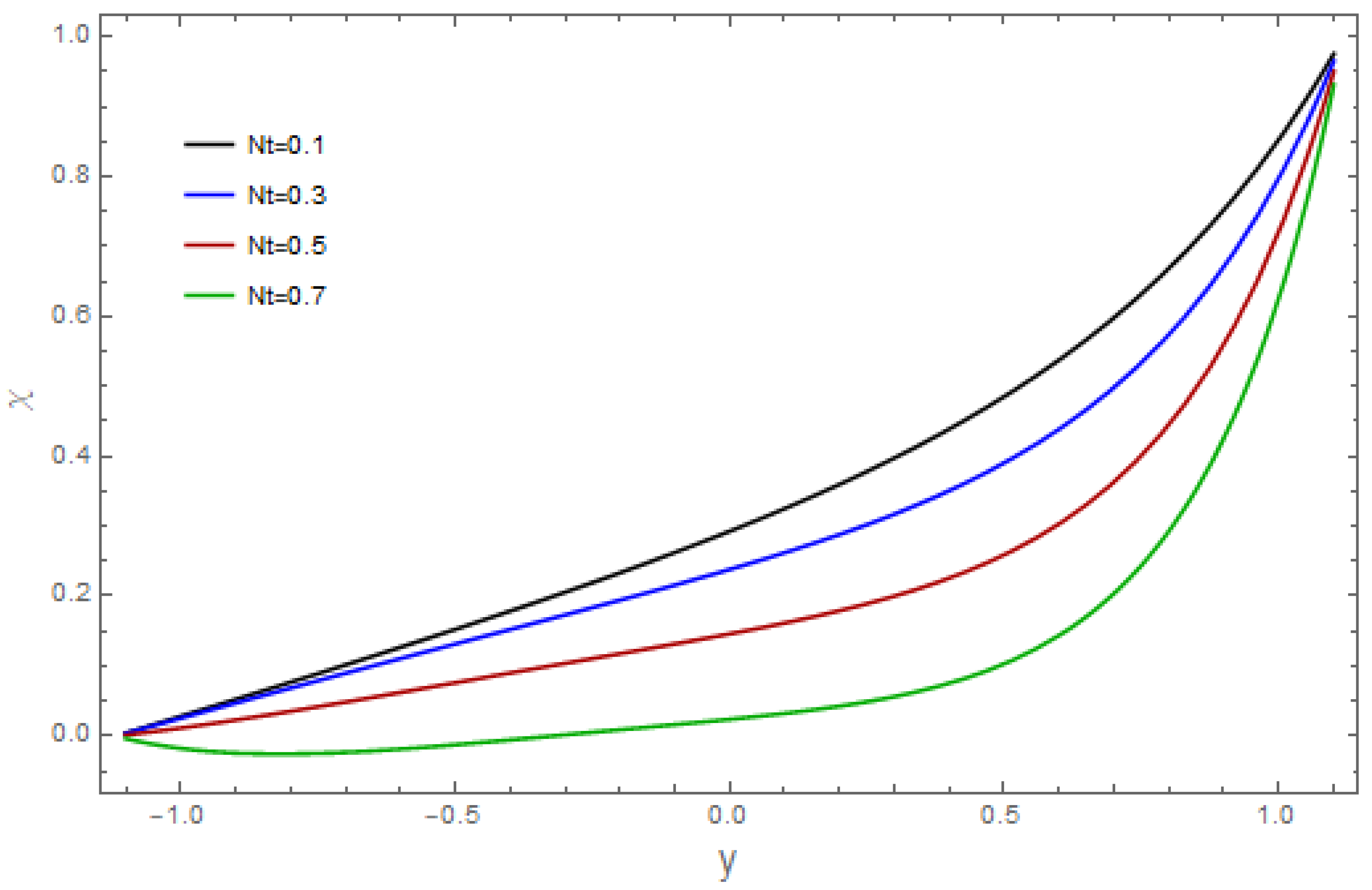
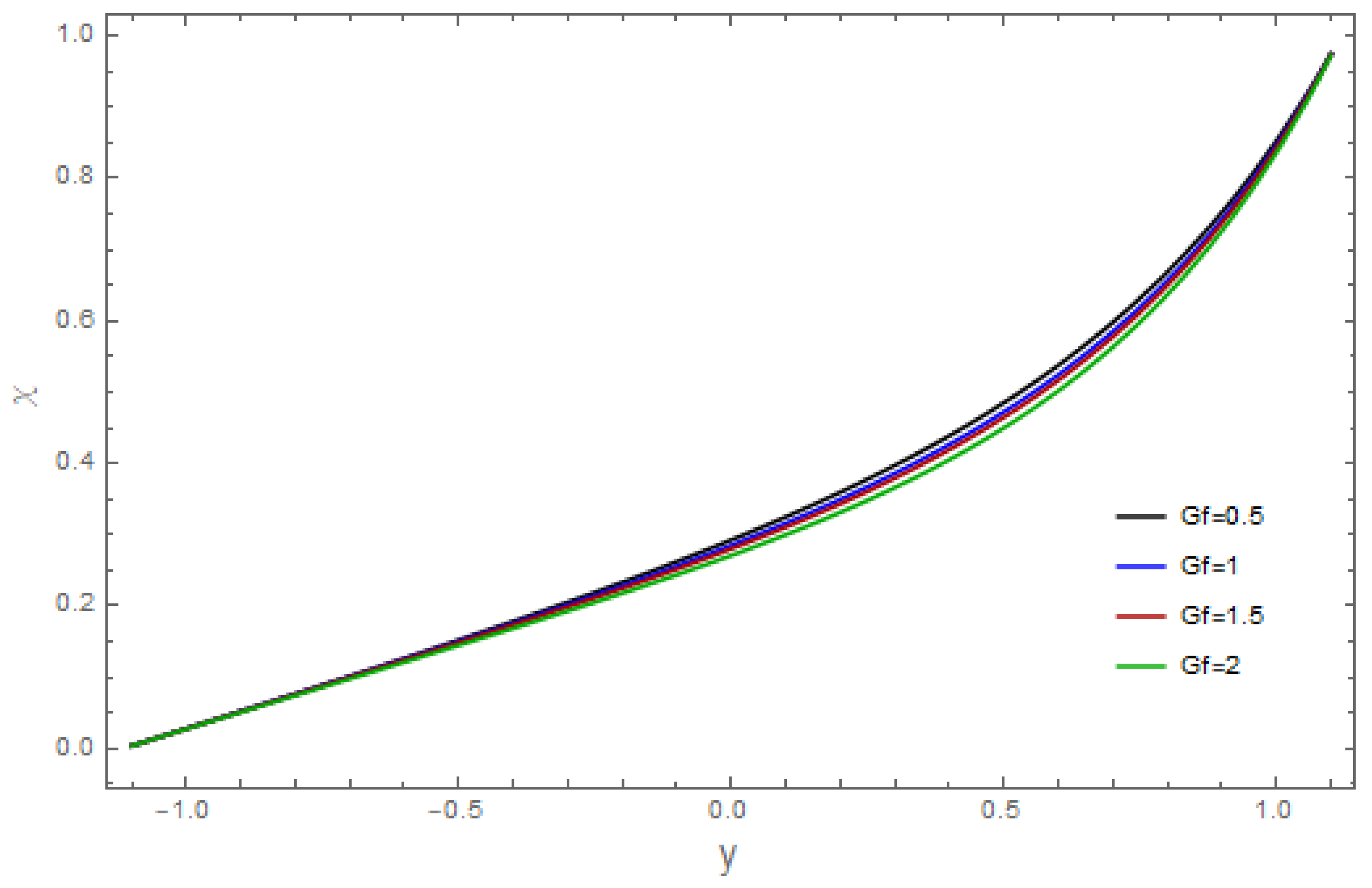
4.4. Concentration
4.5. Heat Transfer Rate
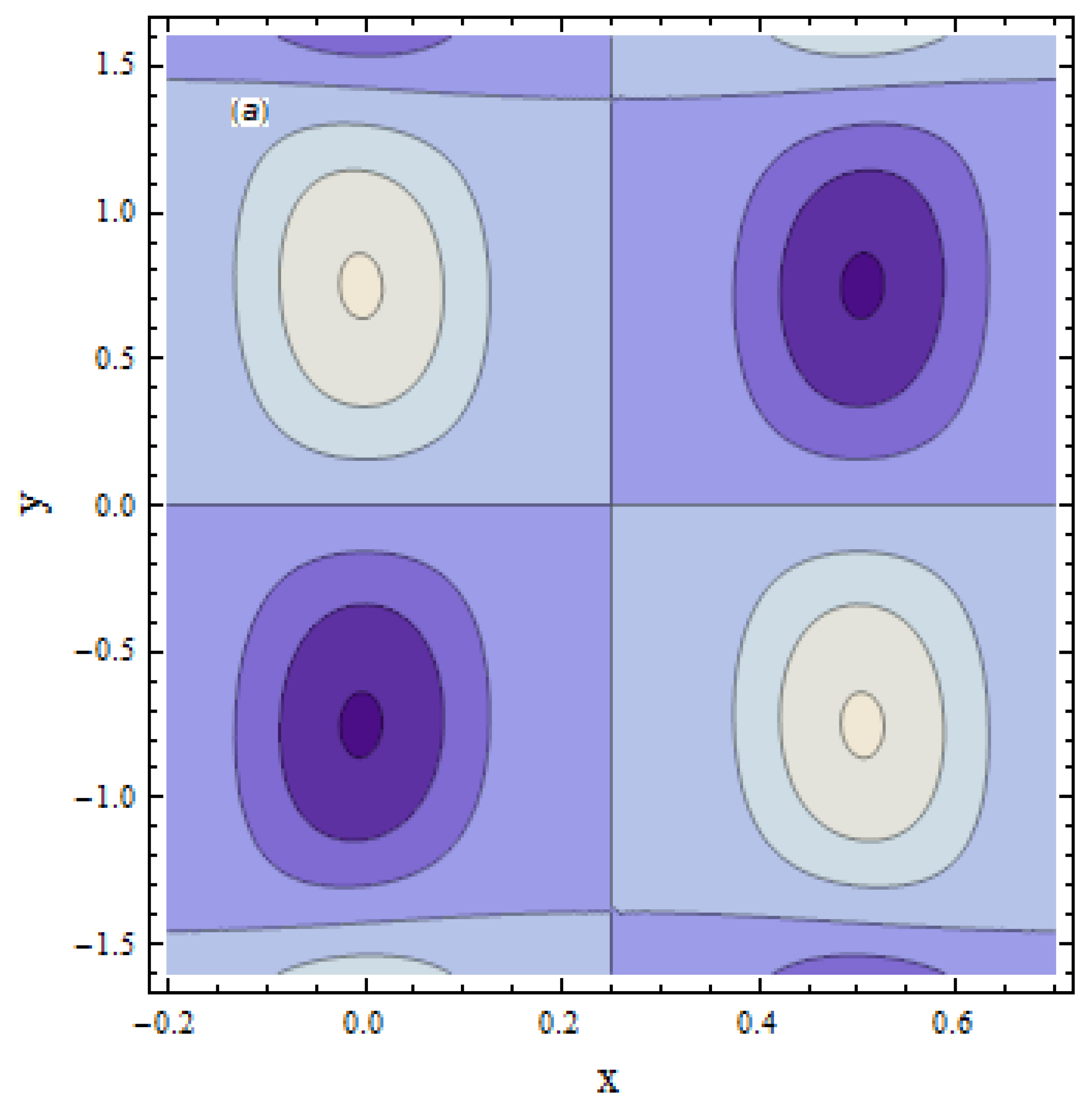
4.6. Trapping
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| (x,y) | Cartesian co-ordinates |
| a | wave amplitude |
| c | wave speed |
| p | pressure |
| d | coefficient of viscous damping |
| n | power law index |
| C | concentration |
| (u,v) | velocity components |
| t | time |
| d1 | half channel width |
| g | gravitational acceleration |
| k | thermal conductivity |
| T | temperature |
| Wc | maximum cell swimming speed |
| Greek symbols | |
| α | thermal diffusivity |
| ρ | density, kg/m3 |
| σ | electric conductions |
| Γ | material constant |
| σ | electric conductions |
| λ | wave length |
| δ | wave number |
| (β1,β2,β3) | slip parameters |
| ψ | stream function |
References
- Latham, T. Fluid motion in a peristaltic pump. Master’s Thesis, Massachusetts Institute of Technology, Cambridge, UK, 1966. [Google Scholar]
- Shapiro, A.H.; Jaffrin, M.Y.; Weinberg, S.L. Peristaltic pumping with long wavelength at low Reynolds number. J. Fluid Mech. 1969, 37, 799–825. [Google Scholar] [CrossRef]
- Mekheimer, K.S. Effect of the induced magnetic field on peristaltic flow of a couple stress fluid. Phys. Lett. A 2008, 372, 4271–4278. [Google Scholar] [CrossRef]
- Ali, N.; Sajid, M.; Javed, T.; Abbas, Z. Heat transfer analysis of peristaltic flow in a curved channel. Int. J. Heat Mass Transf. 2010, 53, 3319–3325. [Google Scholar] [CrossRef]
- Mustafa, M.; Hina, S.; Hayat, T.; Alsaedi, A. Influence of wall properties on the peristaltic flow of a nanofluid: Analytic and numerical solutions. Int. J. Heat Mass Transf. 2012, 55, 4871–4877. [Google Scholar] [CrossRef]
- Hayat, T.; Iqbal, M.; Yasmin, H.; Alsaadi, F.E.; Gao, H. Simultaneous effects of Hall and convective conditions on peristaltic flow of couple-stress fluid in an inclined asymmetric channel. Pramana 2015, 85, 125–148. [Google Scholar] [CrossRef]
- Sinnott, M.D.; Cleary, P.W.; Harrison, S.M. Peristaltic transport of a particulate suspension in the small intestine. Appl. Math. Model. 2017, 44, 143–159. [Google Scholar] [CrossRef]
- Yasmin, H.; Iqbal, N.; Tanveer, A. Engineering applications of peristaltic fluid flow with hall current, thermal deposition and convective conditions. Mathematics 2020, 8, 1710. [Google Scholar] [CrossRef]
- Nisar, Z.; Hayat, T.; Alsaedi, A.; Ahmad, B. Significance of activation energy in radiative peristaltic transport of Eyring-Powell nanofluid. Int. Commun. Heat Mass Transf. 2020, 116, 104655. [Google Scholar] [CrossRef]
- Yasmin, H.; Iqbal, N.; Hussain, A. Convective heat/mass transfer analysis on Johnson-Segalman fluid in a symmetric curved channel with peristalsis: Engineering applications. Symmetry 2020, 12, 1475. [Google Scholar] [CrossRef]
- Hina, S.; Kayani, S.M.; Mustafa, M. Aiding or opposing electro-osmotic flow of Carreau–Yasuda nanofluid induced by peristaltic waves using Buongiorno model. Waves Random Complex Media 2022, 1–17. [Google Scholar] [CrossRef]
- Abbasi, F.M.; Shehzad, S.A. Magnetized peristaltic transportation of boron-nitride and ethylene-glycol nanofluid through a curved channel. Chem. Phys. Lett. 2022, 803, 139860. [Google Scholar]
- Hayat, T.; Nisar, Z.; Ahmad, B.; Yasmin, H. Simultaneous effects of slip and wall properties on MHD peristaltic motion of nanofluid with Joule heating. J. Magn. Magn. Mater. 2015, 395, 48–58. [Google Scholar] [CrossRef]
- Hayat, T.; Nisar, Z.; Yasmin, H.; Alsaedi, A. Peristaltic transport of nanofluid in a compliant wall channel with convective conditions and thermal radiation. J. Mol. Liq. 2016, 220, 448–453. [Google Scholar] [CrossRef]
- Rafiq, M.; Sajid, M.; Alhazmi, S.E.; Khan, M.I.; El-Zahar, E.R. MHD electroosmotic peristaltic flow of Jeffrey nanofluid with slip conditions and chemical reaction. Alex. Eng. J. 2022, 61, 9977–9992. [Google Scholar] [CrossRef]
- Choi, S.U.S. Enhancing Thermal Conductivity of Fluids with Nanoparticles; Siginer, D., Wang, H., Eds.; Developments and Applications of Non-Newtonian Flows; ASME FE Argonne National Laboratory: Argonne, IL, USA, 1995; Volume 231, pp. 99–105. [Google Scholar]
- Buongiorno, J.D. Convective transport in nanofluids. ASME J. Heat Transf. 2006, 128, 240–250. [Google Scholar] [CrossRef]
- Tsai, C.Y.; Chien, H.T.; Ding, P.P.; Chan, B.; Luh, T.Y.; Chen, P.H. Effect of structural character of gold nanoparticles in nanofluid on heat pipe thermal performance. Mater. Lett. 2004, 58, 1461–1465. [Google Scholar] [CrossRef]
- Li, Y.; Tung, S.; Schneider, E.; Xi, S. A review on development of nanofluid preparation and characterization. Powd. Techn. 2009, 196, 89–101. [Google Scholar] [CrossRef]
- Yan, Q.; Lu, J.; Ni, X.W. Measurement of the Velocities of Nanoparticles in Flowing Nanofluids using the Zero-Crossing Laser Speckle Method. Chin. Phys. Lett. 2012, 29, 044207. [Google Scholar] [CrossRef]
- Abbasi, F.M.; Hayat, T.; Ahmad, B. Peristaltic transport of copper–water nanofluid saturating porous medium. Physica E 2015, 67, 47–53. [Google Scholar] [CrossRef]
- Akram, S.; Afzal, Q.; Aly, E.H. Half-breed effects of thermal and concentration convection of peristaltic pseudoplastic nanofluid in a tapered channel with induced magnetic field. Case Stud. Therm. Eng. 2020, 22, 100775. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Abdelsalam, S.I. Bio-inspired peristaltic propulsion of hybrid nanofluid flow with Tantalum (Ta) and Gold (Au) nanoparticles under magnetic effects. Waves Random Complex Media 2021, 1–26. [Google Scholar] [CrossRef]
- Alsaedi, A.; Nisar, Z.; Hayat, T.; Ahmad, B. Analysis of mixed convection and hall current for MHD peristaltic transport of nanofluid with compliant wall. Int. Commun. Heat Mass Transf. 2021, 121, 105121. [Google Scholar] [CrossRef]
- Abbasi, F.M.; Zahid, U.M.; Akbar, Y.; Saba, B.; Hamida, M.B. Thermodynamic analysis of electroosmosis regulated peristaltic motion of Fe 3 O 4-Cu/H 2 O hybrid nanofluid. Int. J. Modern Phys. B 2022, 36, 2250060. [Google Scholar] [CrossRef]
- Nisar, Z.; Hayat, T.; Alsaedi, A.; Ahmad, B. Mathematical modeling for peristalsis of couple stress nanofluid. Math. Meth. Appl. Sci. 2022, 1–19. [Google Scholar] [CrossRef]
- Hussain, A.; Wang, J.; Akbar, Y.; Shah, R. Enhanced thermal effectiveness for electroosmosis modulated peristaltic flow of modified hybrid nanofluid with chemical reactions. Sci. Rep. 2022, 12, 13756. [Google Scholar] [CrossRef]
- Yasmin, H.; Giwa, S.O.; Noor, S.; Sharifpur, M. Experimental exploration of hybrid nanofluids as energy-efficient fluids in solar and thermal energy storage applications. Nanomaterials 2023, 13, 278. [Google Scholar] [CrossRef] [PubMed]
- Singh, K.R.B.; Nayak, V.; Singh, R.P. Introduction to bionanomaterials: An overview. In Bionanomaterials Fundamentals and Biomedical Applications; IOP Publishing, Ltd.: Bristol, UK, 2021; pp. 1–21. [Google Scholar]
- Jeevanandam, J.; Ling, J.K.U.; Barhoum, A.; Chan, Y.S.; Danquah, M.K. Bionanomaterials: Definitions, Sources, Types, Properties, Toxicity, And Regulations, Fundamentals of Bionanomaterials. Micro Nano Technol. 2022, 15, 1–29. [Google Scholar]
- Hayat, T.; Abbasi, F.M.; Alsaedi, A.; Alsaadi, F. Hall and Ohmic heating effects on the peristaltic transport of a Carreau-Yasuda fluid in an asymmetric channel. Z. Naturforschung A 2014, 69, 43–51. [Google Scholar] [CrossRef]
- Kayani, S.M.; Hina, S.; Mustafa, M. A new model and analysis for peristalsis of Carreau-Yasuda (CY) nanofluid subject to wall properties. Arab. J. Sci. Eng. 2020, 45, 5179–5190. [Google Scholar] [CrossRef]
- Khan, M.I.; Alzahrani, F.; Hobiny, A.; Ali, Z. Estimation of entropy generation in Carreau-Yasuda fluid flow using chemical reaction with activation energy. J. Mater. Res. Techn. 2020, 9, 9951–9964. [Google Scholar] [CrossRef]
- Nisar, Z.; Hayat, T.; Alsaedi, A.; Momani, S. Peristaltic flow of chemically reactive Carreau-Yasuda nanofluid with modified Darcy’s expression. Mater. Today Commun. 2022, 33, 104532. [Google Scholar] [CrossRef]
- Iqbal, J.; Abbasi, F.M. Analysis of entropy generation for Magnetohydrodynamics peristaltic motion of Carreau-Yasuda nanofluid through a curved channel with variable thermal conductivity and Joule heating. Waves Random Complex Media 2022, 1–20. [Google Scholar] [CrossRef]
- Pedley, T.J.; Hill, N.A.; Kessler, J.O. The growth of bioconvection patterns in a uniform suspension of gyrotactic micro-organisms. J. Fluid Mech. 1988, 195, 223–237. [Google Scholar] [CrossRef] [PubMed]
- Waqas, H.; Khan, S.U.; Hassan, M.; Bhatti, M.M.; Imran, M. Analysis on the bioconvection flow of modified second-grade nanofluid containing gyrotactic microorganisms and nanoparticles. J. Mol. Liq. 2019, 291, 111231. [Google Scholar] [CrossRef]
- Rao, M.; Gangadhar, K.; Chamkha, A.J.; Surekha, P. Bioconvection in a convectional nanofluid flow containing gyrotactic microorganisms over an isothermal vertical cone embedded in a porous surface with chemical reactive species. Arab. J. Sci. Eng. 2021, 46, 2493–2503. [Google Scholar] [CrossRef]
- Hayat, T.; Nisar, Z.; Alsaedi, A. Bioconvection and Hall current analysis for peristalsis of nanofluid. Int. Commun. Heat Mass Transf. 2021, 129, 105693. [Google Scholar] [CrossRef]
- Hussein, S.A.; Ahmed, S.E.; Arafa, A.A. Electrokinetic peristaltic bioconvective Jeffrey nanofluid flow with activation energy for binary chemical reaction, radiation and variable fluid properties. Z. Angew. Math. Mech. 2022, 103, e202200284. [Google Scholar] [CrossRef]
- Akbar, Y.; Huang, S. Enhanced thermal effectiveness for electrokinetically driven peristaltic flow of motile gyrotactic microorganisms in a thermally radiative Powell Eyring nanofluid flow with mass transfer. Chem. Phys. Lett. 2022, 808, 140120. [Google Scholar] [CrossRef]
- Avramenko, A.; Kovetska, Y.Y.; Shevchuk, I.V. Lorenz approach for analysis of bioconvection instability of gyrotactic motile microorganisms. Chaos Solit. Fractals 2023, 166, 112957. [Google Scholar] [CrossRef]
- Gad-el-Hak, M. Compliant coatings: The simpler alternative. Exp. Therm. Fluid Sci. 1998, 16, 141–156. [Google Scholar] [CrossRef]
- Gad-El-Hak, M. Drag Reduction Using Compliant Walls. In Flow Past Highly Compliant Boundaries and in Collapsible Tubes. Fluid Mechanics and Its Applications; Carpenter, P.W., Pedley, T.J., Eds.; Springer: Dordrecht, The Netherlands, 2003; p. 72. [Google Scholar]
- Kramer, M.O. Boundary-layer stabilization by distributed damping. J. Aeronaut. Sci. 1957, 24, 459–460. [Google Scholar]











| ξ | β3 | Gf | Gc | Pe | Nb | Nt | ϕ(0) |
|---|---|---|---|---|---|---|---|
| 0.5 | 0.1 | 0.3 | 0.2 | 2 | 0.1 | 0.1 | 0.18773 |
| 1 | |||||||
| 0.5 | 0.2 | 0.16171 | |||||
| 0.3 | |||||||
| 0.1 | 0.5 | 0.18979 | |||||
| 0.9 | 0.19382 | ||||||
| 0.3 | 0.5 | 0.18171 | |||||
| 0.9 | 0.17599 | ||||||
| 0.2 | 3 | 0.18593 | |||||
| 4 | 0.18468 | ||||||
| 2 | 0.4 | 0.27067 | |||||
| 0.7 | 0.28265 | ||||||
| 0.1 | 0.2 | 0.06122 | |||||
| 0.3 | 0.03627 |
| Gf | We | Nb | Gr | Pe | Rn | β | −θ′(η) |
|---|---|---|---|---|---|---|---|
| 0.5 | 0.3 | 4 | 0.5 | 1 | 1.5 | 0.1 | 0.0367749 |
| 0.7 | 0.0279817 | ||||||
| 0.5 | 0.4 | 0.0342317 | |||||
| 0.5 | 0.0127678 | ||||||
| 0.3 | 5 | 0.048036 | |||||
| 6 | 0.0553885 | ||||||
| 4 | 0.7 | 0.0651841 | |||||
| 0.9 | 0.0974947 | ||||||
| 0.5 | 1.5 | 0.0435089 | |||||
| 2 | 0.0492939 | ||||||
| 1 | 1 | 0.0836686 | |||||
| 1.8 | 0.0112577 | ||||||
| 1.5 | 0.2 | 0.0367803 | |||||
| 0.5 | 0.0365506 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nisar, Z.; Yasmin, H. Analysis of Motile Gyrotactic Micro-Organisms for the Bioconvection Peristaltic Flow of Carreau–Yasuda Bionanomaterials. Coatings 2023, 13, 314. https://doi.org/10.3390/coatings13020314
Nisar Z, Yasmin H. Analysis of Motile Gyrotactic Micro-Organisms for the Bioconvection Peristaltic Flow of Carreau–Yasuda Bionanomaterials. Coatings. 2023; 13(2):314. https://doi.org/10.3390/coatings13020314
Chicago/Turabian StyleNisar, Zahid, and Humaira Yasmin. 2023. "Analysis of Motile Gyrotactic Micro-Organisms for the Bioconvection Peristaltic Flow of Carreau–Yasuda Bionanomaterials" Coatings 13, no. 2: 314. https://doi.org/10.3390/coatings13020314
APA StyleNisar, Z., & Yasmin, H. (2023). Analysis of Motile Gyrotactic Micro-Organisms for the Bioconvection Peristaltic Flow of Carreau–Yasuda Bionanomaterials. Coatings, 13(2), 314. https://doi.org/10.3390/coatings13020314







