An Estimation of Local Residual Stresses in Amorphous and Crystallized Trivalent Chromium Coatings
Abstract
1. Introduction
2. Materials and Methods
2.1. Coating Deposition
2.2. Coating Characterization
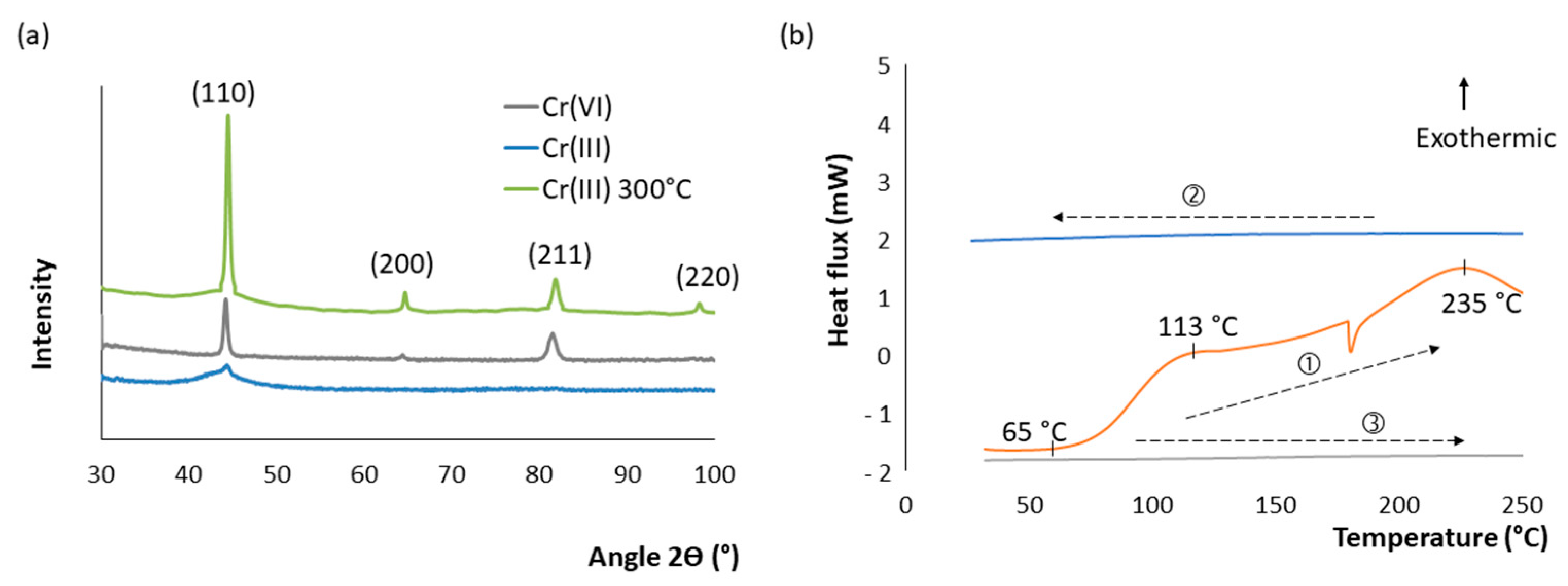
2.2.1. XRD Method
- θ: from 71.5° to 85.5°, by 0.02° steps (plane 211),
- ψ: from −60° to 55°, by 5° steps,
- φ: from 0° to 90°, by 45° steps, (0° corresponds to the longitudinal direction of the plate and 90° to the transverse direction),
- acquisition time: 10 s by steps.
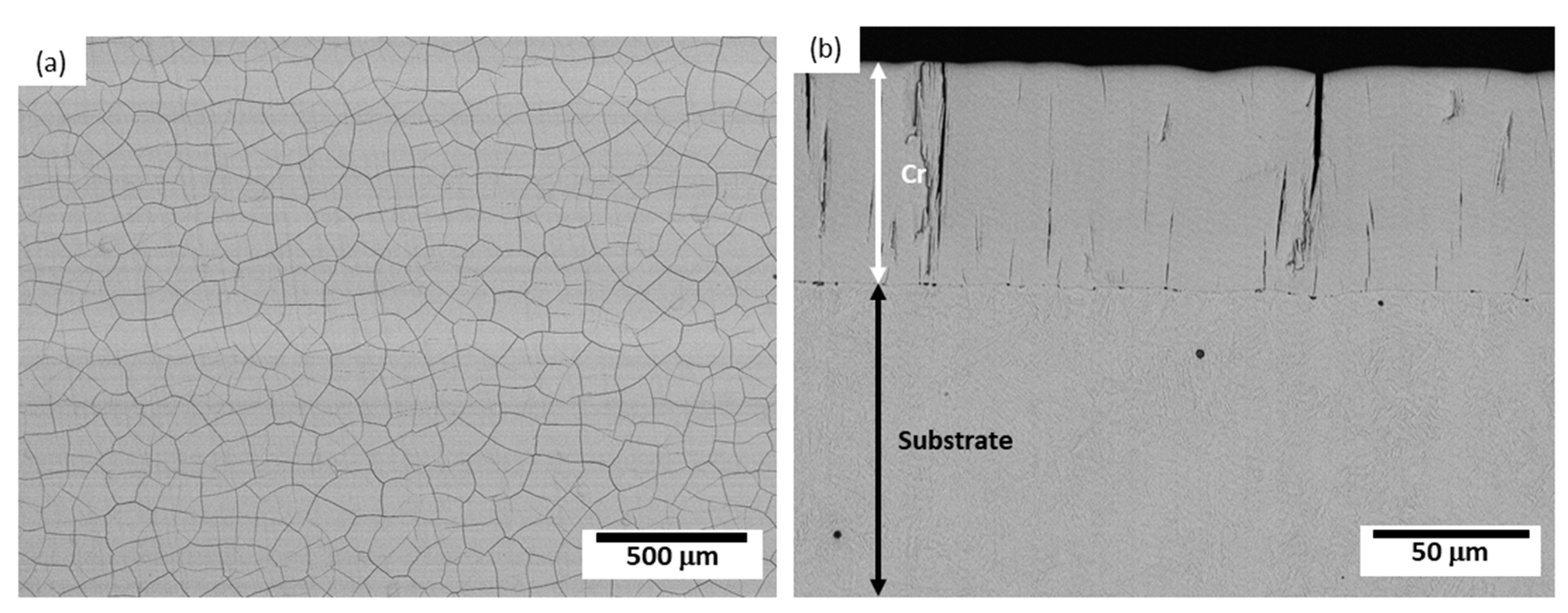
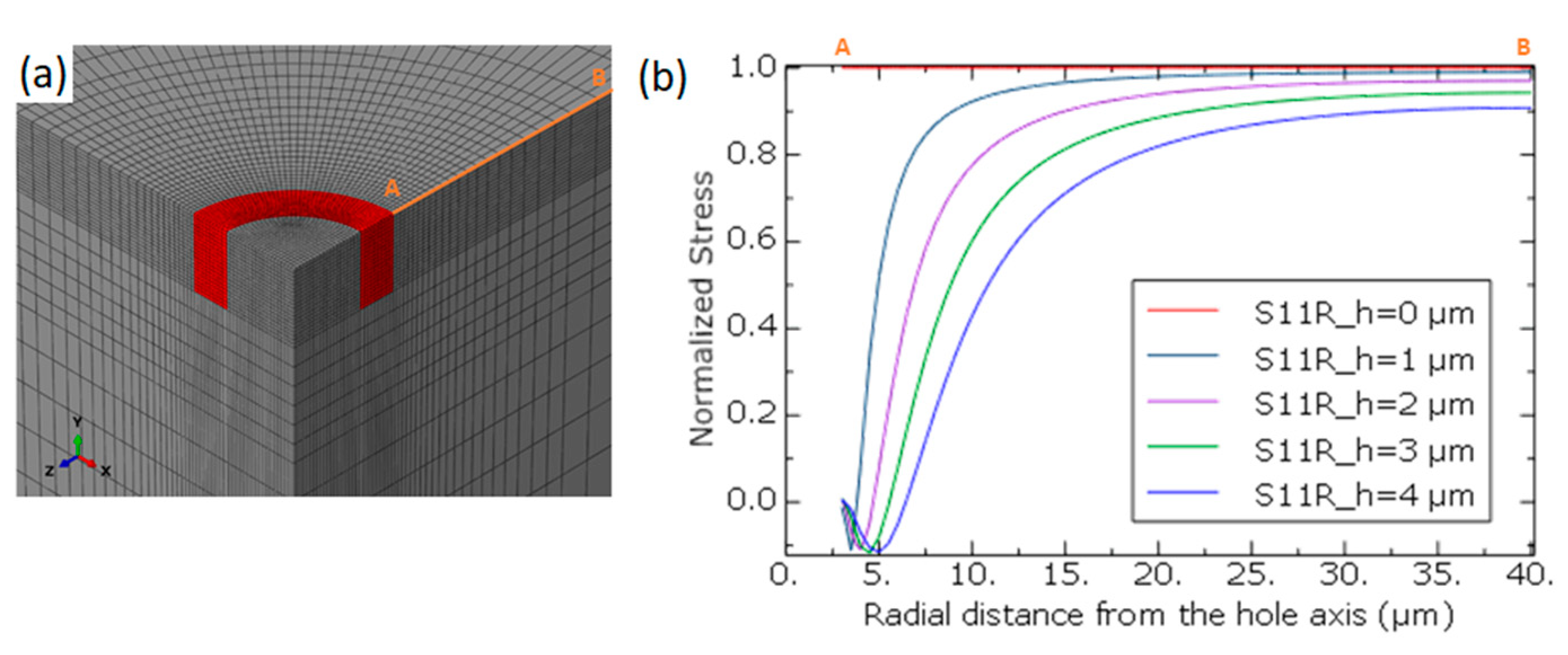
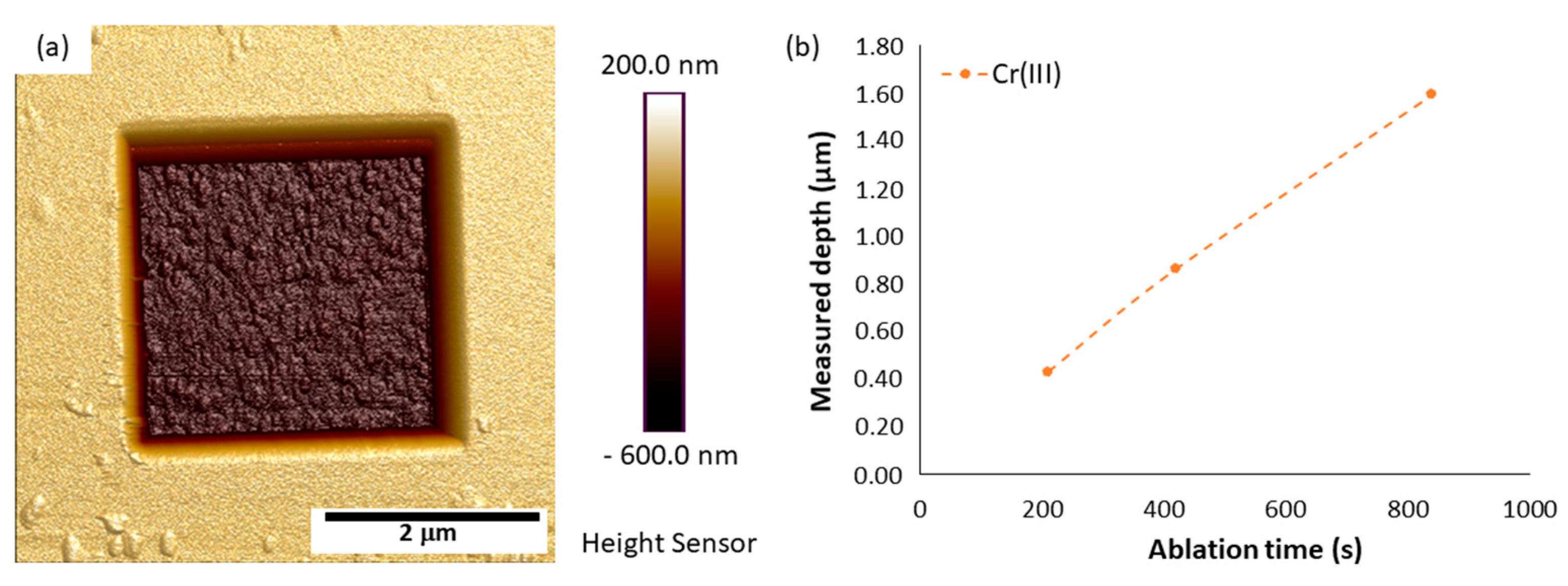
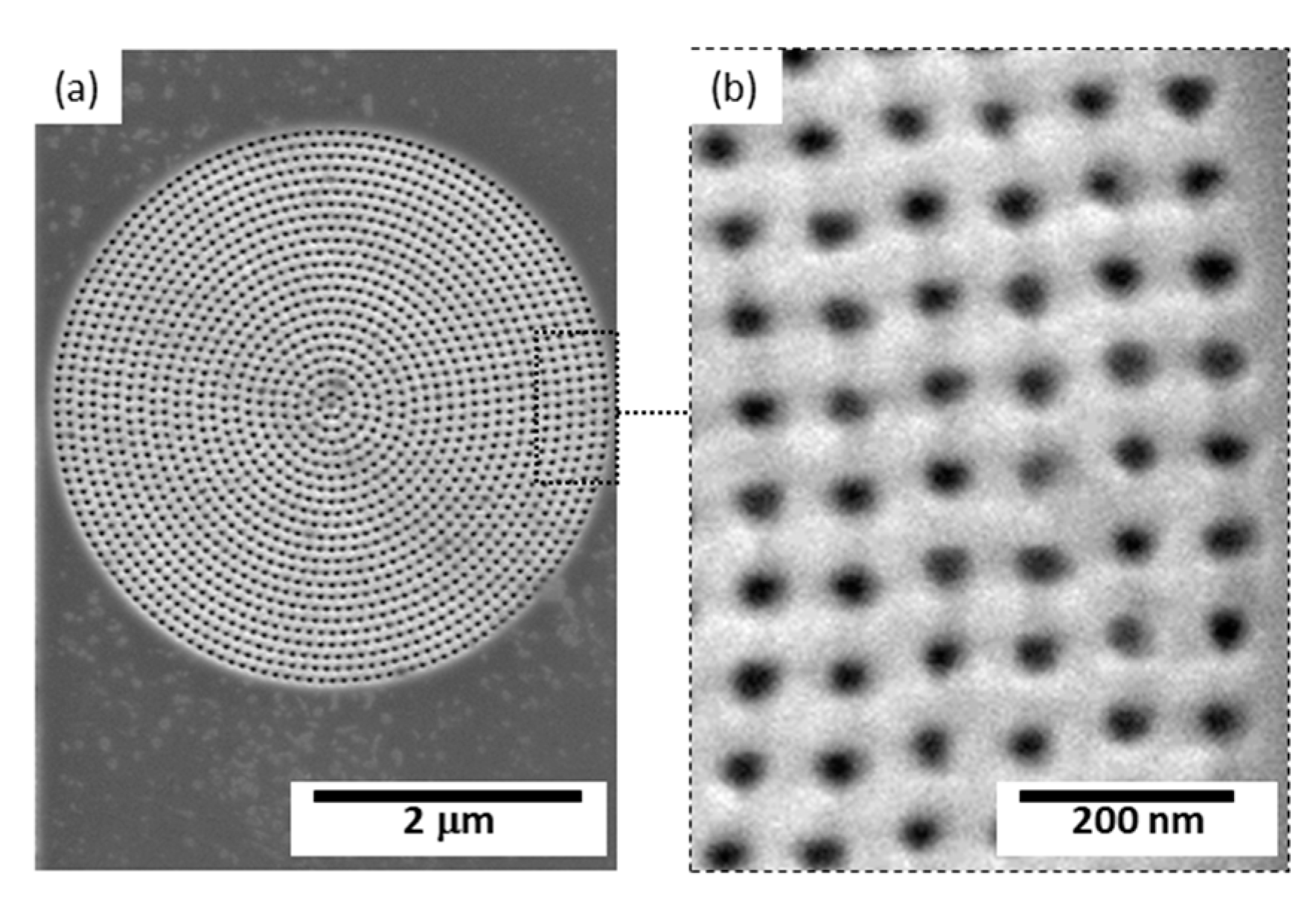
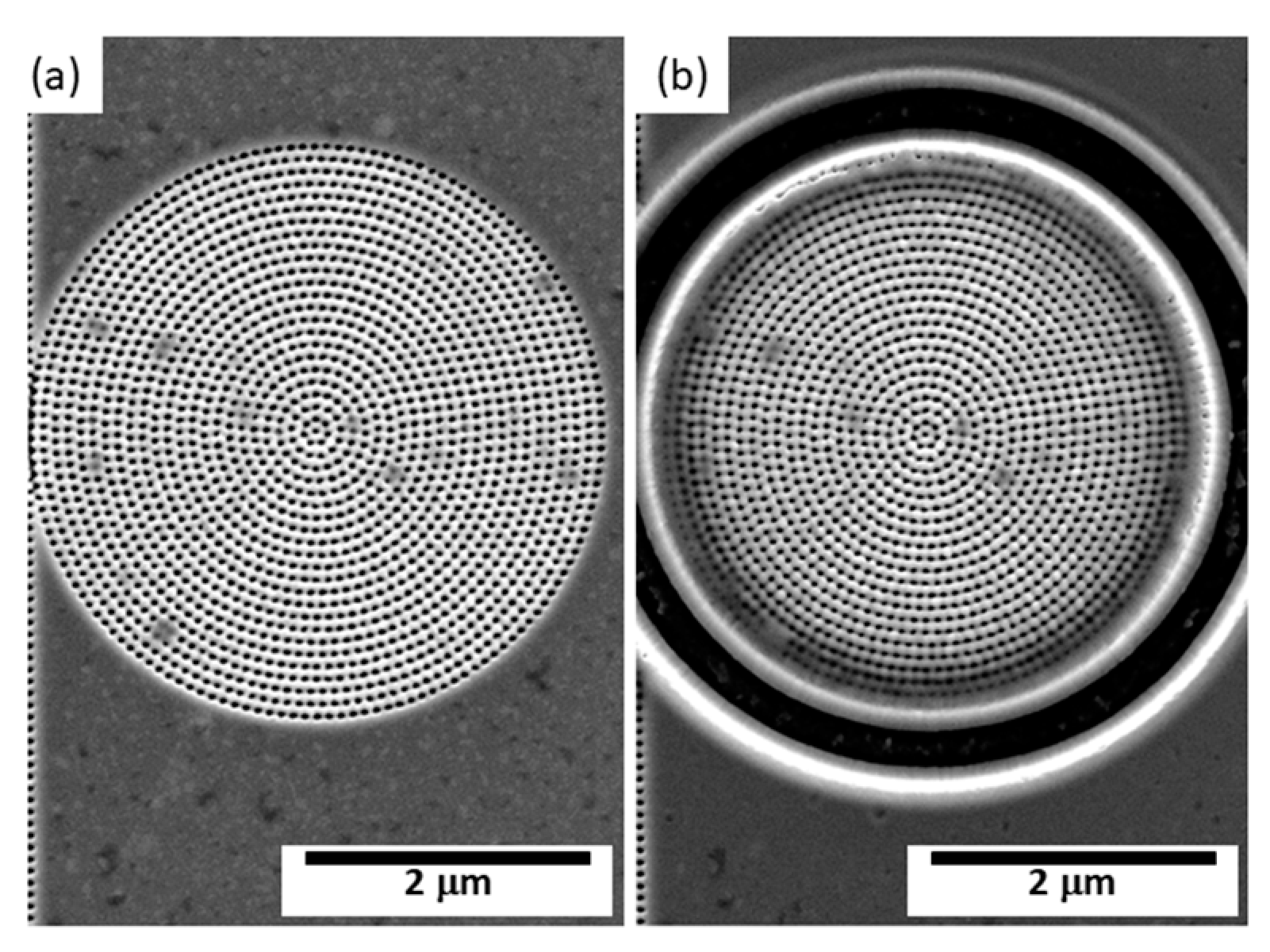
2.2.2. FIB-DIC Method
3. Results
3.1. Determination of Residual Stresses in Heat-Treated Coatings
3.1.1. XRD Method
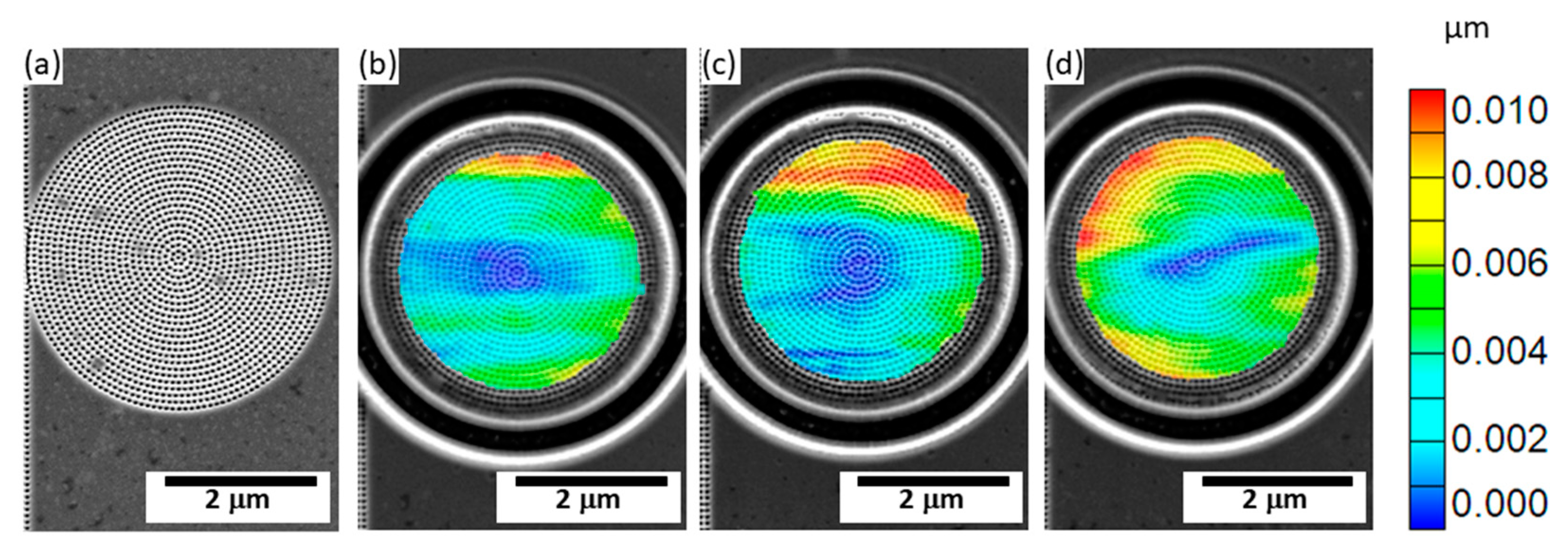
3.1.2. FIB-DIC Method
3.2. Determination of Residual Stresses in Untreated Trivalent Chromium Coatings
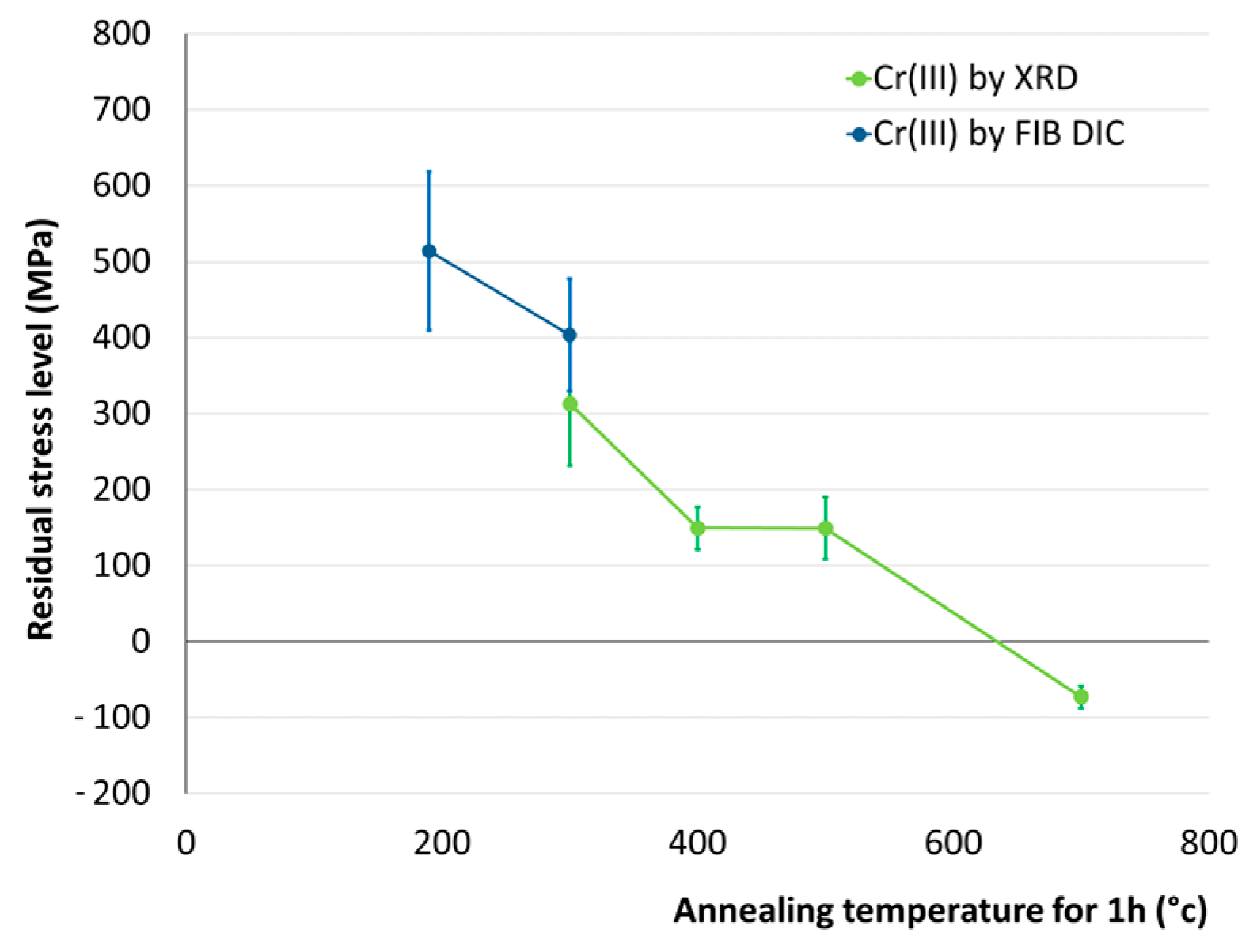
3.3. Determination of Residual Stresses in Annealed Coatings
4. Discussion
5. Conclusions
- Residual stress determination by FIB-DIC was successfully implemented. Comparing the results obtained from XRD measurements on the crystallized deposit show similar values, which allowed us to validate the method.
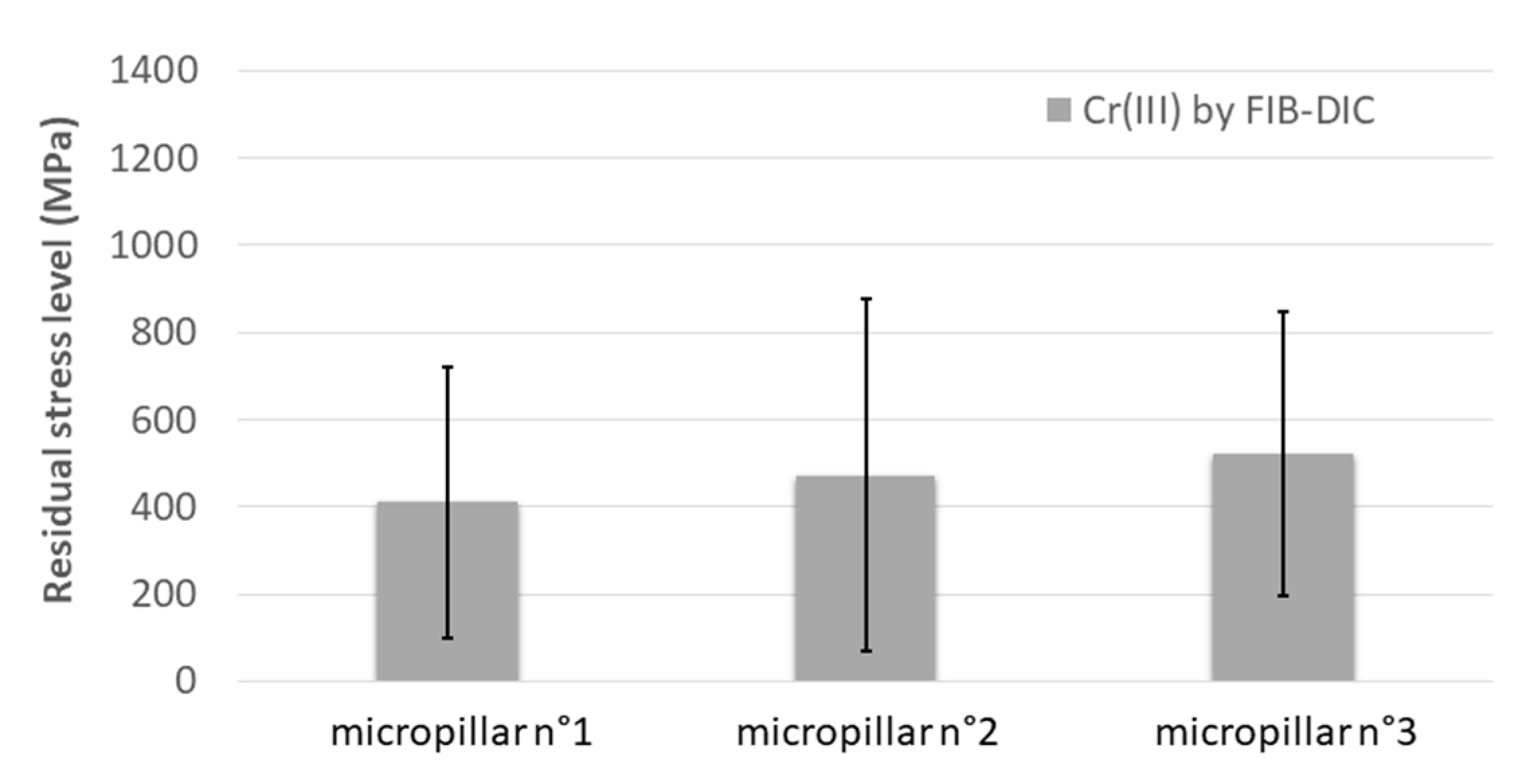
- The estimated stress level is 479 ± 359 MPa for amorphous untreated samples. With the crystallization of the deposit, the residual stresses decrease to about 377 ± 275 MPa.
- A relaxation of the residual stresses is observed as a function of the post-heat treatment temperature. At a heat treatment temperature of 700 °C for one hour, the stresses initially in tension are converted to compression.
- FIB-DIC observes a significant variation in stresses within the milled pillar. We attribute this phenomenon to a subsurface microcracking in these deposits.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vaiopoulou, E.; Gikas, P. Regulations for Chromium Emissions to the Aquatic Environment in Europe and Elsewhere. Chemosphere 2020, 254, 126876. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Ma, C.; Walsh, F.C. Alternative Tribological Coatings to Electrodeposited Hard Chromium: A Critical Review. Trans. IMF 2020, 98, 173–185. [Google Scholar] [CrossRef]
- Protsenko, V.S.; Danilov, F.I. Chromium Electroplating from Trivalent Chromium Baths as an Environmentally Friendly Alternative to Hazardous Hexavalent Chromium Baths: Comparative Study on Advantages and Disadvantages. Clean Technol. Environ. Policy 2014, 16, 1201–1206. [Google Scholar] [CrossRef]
- Liang, A.; Li, Y.; Liang, H.; Ni, L.; Zhang, J. A Favorable Chromium Coating Electrodeposited from Cr(III) Electrolyte Reveals Anti-Wear Performance Similar to Conventional Hard Chromium. Mater. Lett. 2017, 189, 221–224. [Google Scholar] [CrossRef]
- Mahdavi, S.; Allahkaram, S.; Heidarzadeh, A. Characteristics and Properties of Cr Coatings Electrodeposited from Cr(III) Baths. Mater. Res. Express 2019, 6, 026403. [Google Scholar] [CrossRef]
- Danilov, F.I.; Protsenko, V.S.; Gordiienko, V.O.; Kwon, S.C.; Lee, J.Y.; Kim, M. Nanocrystalline Hard Chromium Electrodeposition from Trivalent Chromium Bath Containing Carbamide and Formic Acid: Structure, Composition, Electrochemical Corrosion Behavior, Hardness and Wear Characteristics of Deposits. Appl. Surf. Sci. 2011, 257, 8048–8053. [Google Scholar] [CrossRef]
- Benaben, P. An Overview of Hard Chromium Plating Using Trivalent Chromium Solutions. Plat. Surf. Finish. 2011, 98, 7–14. [Google Scholar]
- Dennis, J.K.; Such, T.E. Nickel and Chromium Plating; Woodhead Publishing Series in Metals and Surface Engineering; Elsevier Science: Amsterdam, The Netherlands, 1993; ISBN 978-1-84569-863-8. [Google Scholar]
- Willis, D.J.; Hammond, C. Structure of Chromium Deposits from Plating Solutions Containing Trivalent and Hexavalent Chromium. Mater. Sci. Technol. 1986, 2, 630–636. [Google Scholar] [CrossRef]
- Ghaziof, S.; Golozar, M.A.; Raeissi, K. Characterization of As-Deposited and Annealed Cr–C Alloy Coatings Produced from a Trivalent Chromium Bath. J. Alloy. Compd. 2010, 496, 164–168. [Google Scholar] [CrossRef]
- Tsai, R.; Wu, S. Phase Stability of Chromium Plating from Chromic Acid Electrolyte Containing Formic Acid. J. Electrochem. Soc. 1990, 137, 3057–3060. [Google Scholar] [CrossRef]
- El-Sharif, M.; Chisholm, C.U. Characteristics of Electrodeposited Chromium. Trans. IMF 1997, 75, 208–212. [Google Scholar] [CrossRef]
- Li, J.; Li, Y.; Tian, X.; Zou, L.; Zhao, X.; Wang, S.; Wang, S. The Hardness and Corrosion Properties of Trivalent Chromium Hard Chromium. MSA 2017, 8, 1014–1026. [Google Scholar] [CrossRef]
- Durut, F. Recherche des Mécanismes Microstructuraux Qui rEgissent Les Propriétés Macroscopiques de Dépôts de Chrome: Influence des Paramètres D’élaboration. Ph.D. Thesis, Grenoble INPG, Grenoble, France, 1999. [Google Scholar]
- Pina, J.; Dias, A.; François, M.; Lebrun, J.L. Residual Stresses and Crystallographic Texture in Hard-Chromium Electroplated Coatings. Surf. Coat. Technol. 1997, 96, 148–162. [Google Scholar] [CrossRef]
- Durut, F.; Benaben, P. Détermination des contraintes résiduelles de dépôts électrolytiques de chrome réalisés à partir d’une solution de chrome hexavalent. MatÉRiaux Tech. 2000, 88, 51–59. [Google Scholar] [CrossRef]
- Keil, S. Experimental Determination of Residual Stresses with the Ring-Core Method and an on-Line Measuring System. Exp. Tech. 1992, 16, 17–24. [Google Scholar] [CrossRef]
- Abadias, G.; Chason, E.; Keckes, J.; Sebastiani, M.; Thompson, G.B.; Barthel, E.; Doll, G.L.; Murray, C.E.; Stoessel, C.H.; Martinu, L. Review Article: Stress in Thin Films and Coatings: Current Status, Challenges, and Prospects. J. Vac. Sci. Technol. A Vac. Surf. Film. 2018, 36, 020801. [Google Scholar] [CrossRef]
- Korsunsky, A.M.; Salvati, E.; Lunt, A.G.J.; Sui, T.; Mughal, M.Z.; Daniel, R.; Keckes, J.; Bemporad, E.; Sebastiani, M. Nanoscale Residual Stress Depth Profiling by Focused Ion Beam Milling and Eigenstrain Analysis. Mater. Des. 2018, 145, 55–64. [Google Scholar] [CrossRef]
- Sebastiani, M.; Eberl, C.; Bemporad, E.; Pharr, G.M. Depth-Resolved Residual Stress Analysis of Thin Coatings by a New FIB–DIC Method. Mater. Sci. Eng. A 2011, 528, 7901–7908. [Google Scholar] [CrossRef]
- Dang, N.M.; Ku, W.Y.; Wang, Z.Y.; Lin, C.H.; Chen, T.F.; Lin, M.T. Incremental FIB-DIC Ring-Core Methods for the Residual Stress Measurement of Bilayer Thin Films. Exp. Mech. 2022, 62, 1489–1499. [Google Scholar] [CrossRef]
- Sebastiani, M.; Rossi, E.; Zeeshan Mughal, M.; Benedetto, A.; Jacquet, P.; Salvati, E.; Korsunsky, A.M. Nano-Scale Residual Stress Profiling in Thin Multilayer Films with Non-Equibiaxial Stress State. Nanomaterials 2020, 10, 853. [Google Scholar] [CrossRef]
- Salvati, E.; Romano-Brandt, L.; Mughal, M.Z.; Sebastiani, M.; Korsunsky, A.M. Generalised residual stress depth profiling at the nanoscale using focused ion beam milling. J. Mech. Phys. Solids 2019, 125, 488–501. [Google Scholar] [CrossRef]
- Winiarski, B.; Gholinia, A.; Tian, J.; Yokoyama, Y.; Liaw, P.K.; Withers, P.J. Submicron-Scale Depth Profiling of Residual Stress in Amorphous Materials by Incremental Focused Ion Beam Slotting. Acta Mater. 2012, 60, 2337–2349. [Google Scholar] [CrossRef]
- Krottenthaler, M.; Schmid, C.; Schaufler, J.; Durst, K.; Göken, M. A Simple Method for Residual Stress Measurements in Thin Films by Means of Focused Ion Beam Milling and Digital Image Correlation. Surf. Coat. Technol. 2013, 215, 247–252. [Google Scholar] [CrossRef]
- Kang, K.J.; Yao, N.; He, M.Y.; Evans, A.G. A Method for in Situ Measurement of the Residual Stress in Thin Films by Using the Focused Ion Beam. Thin Solid Film. 2003, 443, 71–77. [Google Scholar] [CrossRef]
- Bemporad, E.; Brisotto, M.; Depero, L.E.; Gelfi, M.; Korsunsky, A.M.; Lunt, A.J.G.; Sebastiani, M. A Critical Comparison between XRD and FIB Residual Stress Measurement Techniques in Thin Films. Thin Solid Film. 2014, 572, 224–231. [Google Scholar] [CrossRef]
- Chen, T.Y.-F.; Chou, Y.-C.; Wang, Z.-Y.; Lin, W.-Y.; Lin, M.-T. Using Digital Image Correlation on SEM Images of Strain Field after Ion Beam Milling for the Residual Stress Measurement of Thin Films. Materials 2020, 13, 1291. [Google Scholar] [CrossRef]
- Romano-Brandt, L.; Salvati, E.; Le Bourhis, E.; Moxham, T.; Dolbnya, I.P.; Korsunsky, A.M. Nano-Scale Residual Stress Depth Profiling in Cu/W Nano-Multilayers as a Function of Magnetron Sputtering Pressure. Surf. Coat. Technol. 2020, 381, 125142. [Google Scholar] [CrossRef]
- Guillon, R.; Dalverny, O.; Fori, B.; Gazeau, C.; Alexis, J. Mechanical Behaviour of Hard Chromium Deposited from a Trivalent Chromium Bath. Coatings 2022, 12, 354. [Google Scholar] [CrossRef]
- Brandes, E.A.; Smithells, C.J. Metals Reference Book. Reed Elsevier plc Group: Woburn, MA, USA, 1976. [Google Scholar]
- Guillon, R. Amélioration de L’adhérence de Revêtements Electrolytiques de Chrome: Étude Expérimentale et Numérique. Ph.D. Thesis, Université de Toulouse, Toulouse, France, 20 June 2022. [Google Scholar]
- Huang, C.A.; Liu, Y.W.; Yu, C.; Yang, C.-C. Role of Carbon in the Chromium Deposit Electroplated from a Trivalent Chromium-Based Bath. Surf. Coat. Technol. 2011, 205, 3461–3466. [Google Scholar] [CrossRef]
- Del Pianta, D. Détermination Des Paramètres Physicochimiques Régissant L’électrodéposition D’une Couche de Chrome Métallique à Partir D’Une Solution de Chrome Trivalent. Ph.D. Thesis, University of Pau (UPPA), Pau, France, 2017. [Google Scholar]


| Fe | C | Si | Mn | P | S | Cr | Mo | Ni |
|---|---|---|---|---|---|---|---|---|
| Bal. | 0.088 | 0.01 | 0.343 | 0.012 | 0.006 | 0.054 | 0.005 | 0.009 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guillon, R.; Stéphan, C.; Balcaen, Y.; Josse, C.; Fori, B.; Dalverny, O.; Alexis, J. An Estimation of Local Residual Stresses in Amorphous and Crystallized Trivalent Chromium Coatings. Coatings 2023, 13, 124. https://doi.org/10.3390/coatings13010124
Guillon R, Stéphan C, Balcaen Y, Josse C, Fori B, Dalverny O, Alexis J. An Estimation of Local Residual Stresses in Amorphous and Crystallized Trivalent Chromium Coatings. Coatings. 2023; 13(1):124. https://doi.org/10.3390/coatings13010124
Chicago/Turabian StyleGuillon, Robin, Cédric Stéphan, Yannick Balcaen, Claudie Josse, Benoit Fori, Olivier Dalverny, and Joel Alexis. 2023. "An Estimation of Local Residual Stresses in Amorphous and Crystallized Trivalent Chromium Coatings" Coatings 13, no. 1: 124. https://doi.org/10.3390/coatings13010124
APA StyleGuillon, R., Stéphan, C., Balcaen, Y., Josse, C., Fori, B., Dalverny, O., & Alexis, J. (2023). An Estimation of Local Residual Stresses in Amorphous and Crystallized Trivalent Chromium Coatings. Coatings, 13(1), 124. https://doi.org/10.3390/coatings13010124






