Computation of Vascular Parameters: Implementing Methodology and Performance Analysis
Abstract
:1. Introduction
2. Materials and Methods
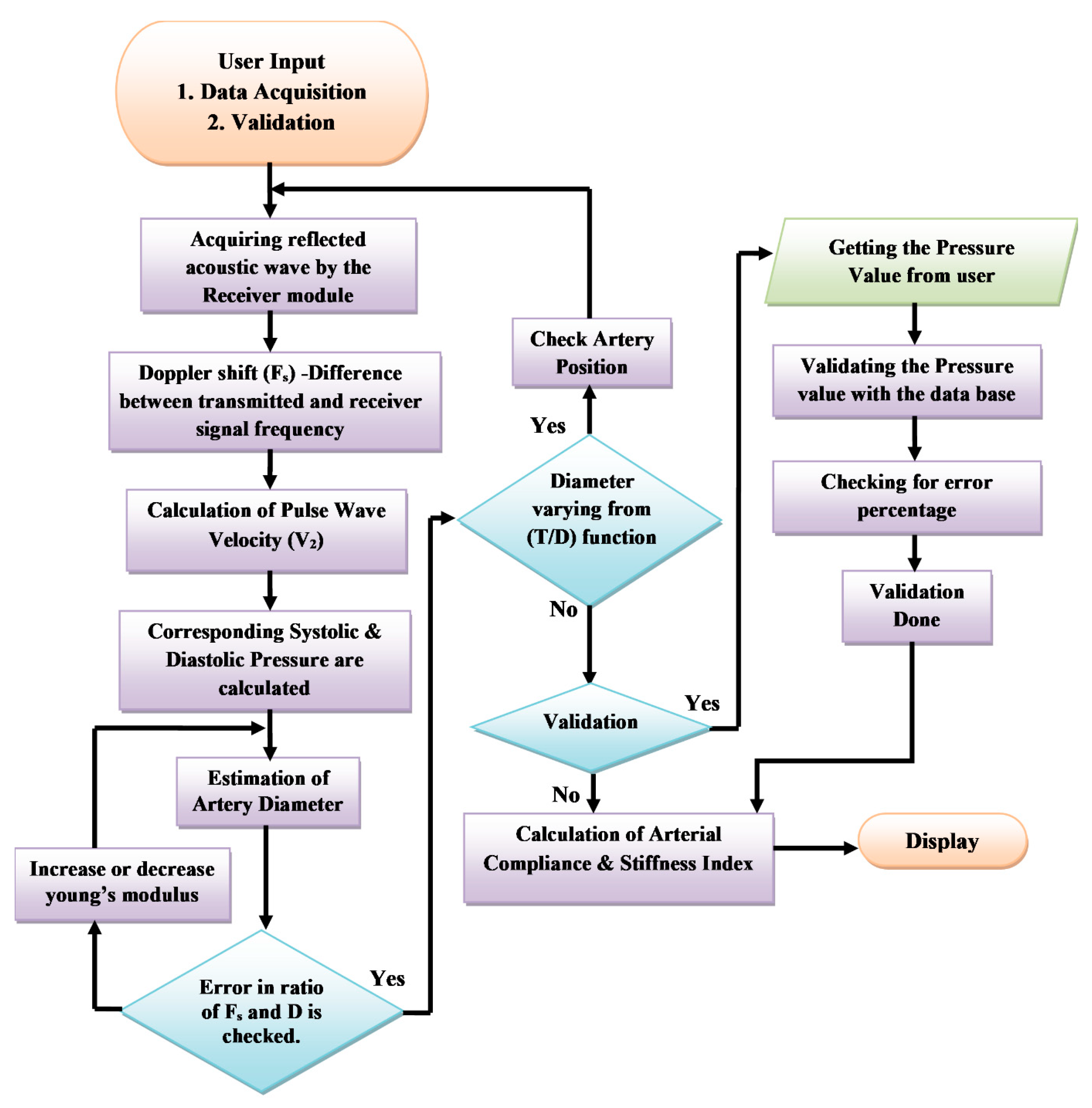
2.1. CaNVAS: System Architecture
- (i)
- A sensor positioning module equipped with infrared illumination to mark the artery’s position.
- (ii)
- An embedded ultrasound sensor module housing a piezoelectric crystal oscillator with an appropriate frequency.
- (iii)
- A transmitter unit, receiver unit, and pre-processing module encompassing an acquisition unit, operational amplifier, and high-pass filter.
- (iv)
- A microprocessor and signal processing module responsible for extracting vascular parameters.
- (v)
- A display module featuring a manual setting mode and an output display unit.
2.1.1. Sensor Positioning Module
2.1.2. Pre-Processing Module
2.1.3. Computation of Vascular Parameters
3. Results
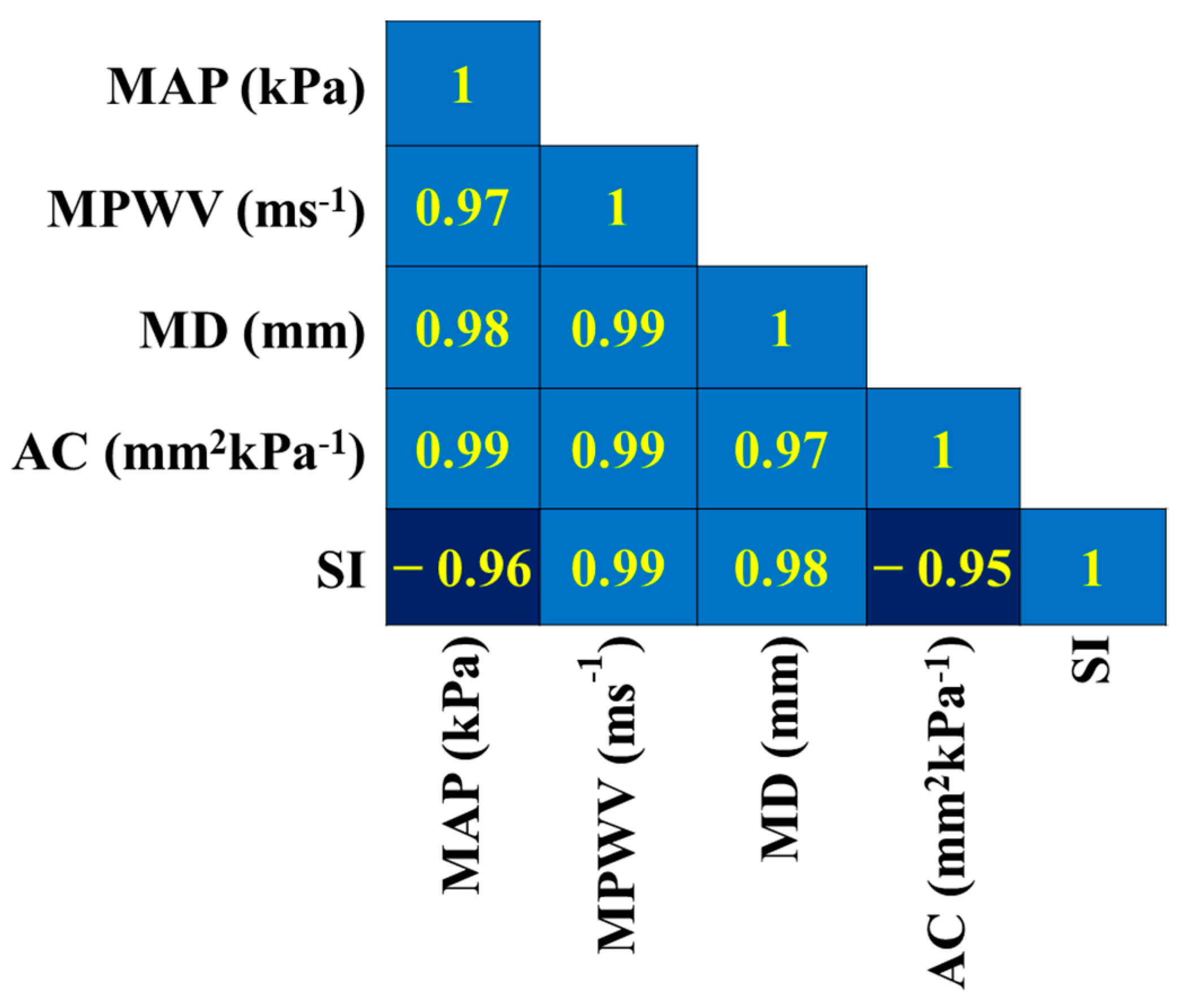
3.1. Linear Regression Analysis
3.2. Repeatability Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dart, A.M.; Lacombe, F.; Yeoh, J.K.; Cameron, J.D.; Jennings, G.L.; Laufer, E.; Esmore, D.S. Aortic distensibility in patients with isolated hypercholesterolaemia, coronary artery disease, or cardiac transplant. Lancet 1991, 338, 270–273. [Google Scholar] [CrossRef] [PubMed]
- Hirai, T.; Sasayama, S.; Kawasaki, T.; Yagi, S. Stiffness of systemic arteries in patients with myocardial infarction. A noninvasive method to predict severity of coronary atherosclerosis. Circulation 1989, 80, 78–86, Erratum in: Circulation 1989, 80, 1946. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zoungas, S.; Asmar, R.P. Arterial stiffness and cardiovascular outcome. Clin. Exp. Pharmacol. Physiol. 2007, 34, 647–651. [Google Scholar] [CrossRef] [PubMed]
- Blacher, J.; Pannier, B.; Guerin, A.P.; Marchais, S.J.; Safar, M.E.; London, G.M. Carotid arterial stiffness as a predictor of cardiovascular and all-cause mortality in end-stage renal disease. Hypertension 1998, 32, 570–574. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gatzka, C.D.; Cameron, J.D.; Kingwell, B.A.; Dart, A.M. Relation between coronary artery disease, aortic stiffness, and left ventricular structure in a population sample. Hypertension 1998, 32, 575–578. [Google Scholar] [CrossRef] [Green Version]
- Lehmann, E.D.; Hopkins, K.D.; Jones, R.L.; Rudd, A.G.; Gosling, R.G. Aortic distensibility in patients with cerebrovascular disease. Clin. Sci. 1995, 89, 247–253. [Google Scholar] [CrossRef]
- Pannier, B.M.; Avolio, A.P.; Hoeks, A.; Mancia, G.; Takazawa, K. Methods and devices for measuring arterial compliance in humans. Am. J. Hypertens. 2002, 15, 743–753. [Google Scholar] [CrossRef]
- Komine, H.; Asai, Y.; Yokoi, T.; Yoshizawa, M. Non-invasive assessment of arterial stiffness using oscillometric blood pressure measurement. Biomed. Eng. Online 2012, 11, 6. [Google Scholar] [CrossRef] [Green Version]
- Whitaker, T.; Wilson, S.C.; Spang, K.; Wawro, T.J.; Lane, J.A. Motion Management in a Fast Blood Pressure Measurement Device. U.S. Patent 7,429,245, 30 September 2008. [Google Scholar]
- Chen, Y.; Li, L.; Hershler, C.; Dill, R.P. Continuous Non-Invasive Blood Pressure Monitoring Method and Apparatus. U.S. Patent 6,599,251, 29 July 2003. [Google Scholar]
- Frankenreiter, M.; Rometsch, R.; Seher, J.P. HP Inc, Blood Pressure Monitor. U.S. Patent 4,953,557, 4 September 1990. [Google Scholar]
- Forstner, K.; Yen, G.F.A.C.Y. Non Invasive Blood Pressure Monitor and a Method for the Non-Invasive Measurement of the Blood Pressure. U.S. Patent 6,447,457, 10 September 2002. [Google Scholar]
- Vatner, S.F.; Zhang, J.; Vyzas, C.; Mishra, K.; Graham, R.M.; Vatner, D.E. Vascular Stiffness in Aging and Disease. Front. Physiol. 2021, 12, 762437. [Google Scholar] [CrossRef]
- Hoctor, R.T.; Thomenius, K.E.; Dentinger, A.M.; Mccarter, J.W. Method and Apparatus for Ultrasonic Continuous, Non-Invasive Blood Pressure Monitoring. U.S. Patent 7,125,383, 24 October 2006. [Google Scholar]
- Kuri, Y. System and Method for Determining Arterial Compliance and Stiffness. U.S. Patent 9,408,541, 9 August 2016. [Google Scholar]
- Xu, M. Local Measurement of the Pulse Wave Velocity Using Doppler Ultrasound. Master’s Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 24 May 2002. [Google Scholar]
- Polańczyk, A.; Woźniak, T.; Strzelecki, M.; Szubert, W.; Stefańczyk, L. Evaluating an algorithm for 3D reconstruction of blood vessels for further simulations of hemodynamic in human artery branches. In Proceedings of the 2016 Signal Processing: Algorithms, Architectures, Arrangements, and Applications (SPA), Poznan, Poland, 21–23 September 2016; pp. 103–107. [Google Scholar] [CrossRef]
- Polańczyk, A.; Strzelecki, M.; Woźniak, T.; Szubert, W.; Stefańczyk, L. 3D Blood Vessels Reconstruction Based on Segmented CT Data for Further Simulations of Hemodynamic in Human Artery Branches. Found. Comput. Decis. Sci. 2017, 42, 359–371. [Google Scholar] [CrossRef] [Green Version]
- Polanczyk, A.; Podgorski, M.; Polanczyk, M.; Veshkina, N.; Zbicinski, I.; Stefanczyk, L.; Neumayer, C. A novel method for describing biomechanical properties of the aortic wall based on the three-dimensional fluid-structure interaction model. Interact. Cardiovasc. Thorac. Surg. 2019, 28, 306–315. [Google Scholar] [CrossRef] [Green Version]
- Riley, W.A., Jr.; Barnes, R.W.; Schey, H.M. An approach to the noninvasive periodic assessment of arterial elasticity in the young. Prev. Med. 1984, 13, 169–184. [Google Scholar] [CrossRef]
- Ahlgren, A.R.; Hansen, F.; Sonesson, B.; Länne, T. Stiffness and diameter of the common carotid artery and abdominal aorta in women. Ultrasound. Med. Biol. 1997, 23, 983–988. [Google Scholar] [CrossRef]
- Gamble, G.; Zorn, J.; Sanders, G.; MacMahon, S.; Sharpe, N. Estimation of arterial stiffness, compliance, and distensibility from M-mode ultrasound measurements of the common carotid artery. Stroke 1994, 25, 11–16. [Google Scholar] [CrossRef] [Green Version]
- Kawasaki, T.; Sasayama, S.; Yagi, S.; Asakawa, T.; Hirai, T. Non-invasive assessment of the age related changes in stiffness of major branches of the human arteries. Cardiovasc. Res. 1987, 21, 678–687. [Google Scholar] [CrossRef]
- Magda, S.L.; Ciobanu, A.O.; Florescu, M.; Vinereanu, D. Comparative reproducibility of the noninvasive ultrasound methods for the assessment of vascular function. Heart Vessels 2013, 28, 143–150. [Google Scholar] [CrossRef]
- Nichols, W.W.; O’Rourke, M.F.; Kenney, W.L. McDonald’s Blood Flow in Arteries: Theoretical, Experimental and Clinical Principles, 7th ed.; CRC Press: Boca Raton, FL, USA, 2022. [Google Scholar]
- Nagai, Y.; Fleg, J.L.; Kemper, M.K.; Rywik, T.M.; Earley, C.J.; Metter, E.J. Carotid arterial stiffness as a surrogate for aortic stiffness: Relationship between carotid artery pressure-strain elastic modulus and aortic pulse wave velocity. Ultrasound. Med. Biol. 1999, 25, 181–188. [Google Scholar] [CrossRef]
- Peterson, L.H.; Jensen, R.E.; Parnell, J. Mechanical Properties of Arteries in Vivo. Circ. Res. 1960, 8, 622–639. [Google Scholar] [CrossRef]
- Xing, X.; Ma, Z.; Zhang, M.; Zhou, Y.; Dong, W.; Song, M. An Unobtrusive and Calibration-free Blood Pressure Estimation Method using Photoplethysmography and Biometrics. Sci. Rep. 2019, 9, 8611. [Google Scholar] [CrossRef] [Green Version]
- Zheng, D.; Yao, Y.; Morrison, I.; Greenwald, S. Photoplethysmographic assessment of arterial stiffness and endothelial function. In Photoplethysmography; Academic Press: London, UK, 2022; pp. 235–276. [Google Scholar]
- Danpinid, A.; Luo, J.; Vappou, J.; Terdtoon, P.; Konofagou, E.E. In vivo characterization of the aortic wall stress-strain relationship. Ultrasonics 2010, 50, 654–665. [Google Scholar] [CrossRef] [Green Version]
- Arnett, D.K.; Evans, G.W.; Riley, W.A. Arterial stiffness: A new cardiovascular risk factor? Am. J. Epidemiol. 1994, 140, 669–682. [Google Scholar] [CrossRef] [PubMed]
- Arnett, D.K.; Chambless, L.E.; Kim, H.; Evans, G.W.; Riley, W. Variability in ultrasonic measurements of arterial stiffness in the Atherosclerosis Risk in Communities study. Ultrasound. Med. Biol. 1999, 25, 175–180. [Google Scholar] [CrossRef] [PubMed]
- Bussy, C.; Boutouyrie, P.; Lacolley, P.; Challande, P.; Laurent, S. Intrinsic stiffness of the carotid arterial wall material in essential hypertensives. Hypertension 2000, 35, 1049–1054. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Claridge, M.W.; Bate, G.R.; Hoskins, P.R.; Adam, D.J.; Bradbury, A.W.; Wilmink, A.B. Measurement of arterial stiffness in subjects with vascular disease: Are vessel wall changes more sensitive than increase in intima-media thickness? Atherosclerosis 2009, 205, 477–480. [Google Scholar] [CrossRef] [PubMed]
- Nogata, F.; Yokota, Y.; Kawamura, Y.; Walsh, W.R. System for estimating sclerosis of in vivo arteries based on ultrasound B-mode image analysis. In Proceedings of the 10th International Conference on Control, Automation, Robotics and Vision, AIP Conference Proceedings, Hanoi, Vietnam, 17–20 December 2008; pp. 1779–1782. [Google Scholar]
- Selzer, R.H.; Mack, W.J.; Lee, P.L.; Kwong-Fu, H.; Hodis, H.N. Improved common carotid elasticity and intima-media thickness measurements from computer analysis of sequential ultrasound frames. Atherosclerosis 2001, 154, 185–193. [Google Scholar] [CrossRef]
- Mackenzie, I.S.; Wilkinson, I.B.; Cockcroft, J.R. Assessment of arterial stiffness in clinical practice. Qjm 2002, 95, 67–74. [Google Scholar] [CrossRef] [Green Version]
- Luo, J.; Konofagou, E. A fast normalized cross-correlation calculation method for motion estimation. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2010, 57, 1347–1357. [Google Scholar] [CrossRef] [Green Version]
- Drzewiecki, G.M.; Butterfield, R.D.; Ciaccio, E.J. Rutgers State University of New Jersey, Pressure Waveform Monitor. U.S. Patent 5,363,855, 14 November 1994. [Google Scholar]
- Joseph, J.; Radhakrishnan, R.; Kusmakar, S.; Thrivikraman, A.S.; Sivaprakasam, M. Technical Validation of ARTSENS-An Image Free Device for Evaluation of Vascular Stiffness. IEEE J. Transl. Eng. Health Med. 2015, 3, 1900213. [Google Scholar] [CrossRef]

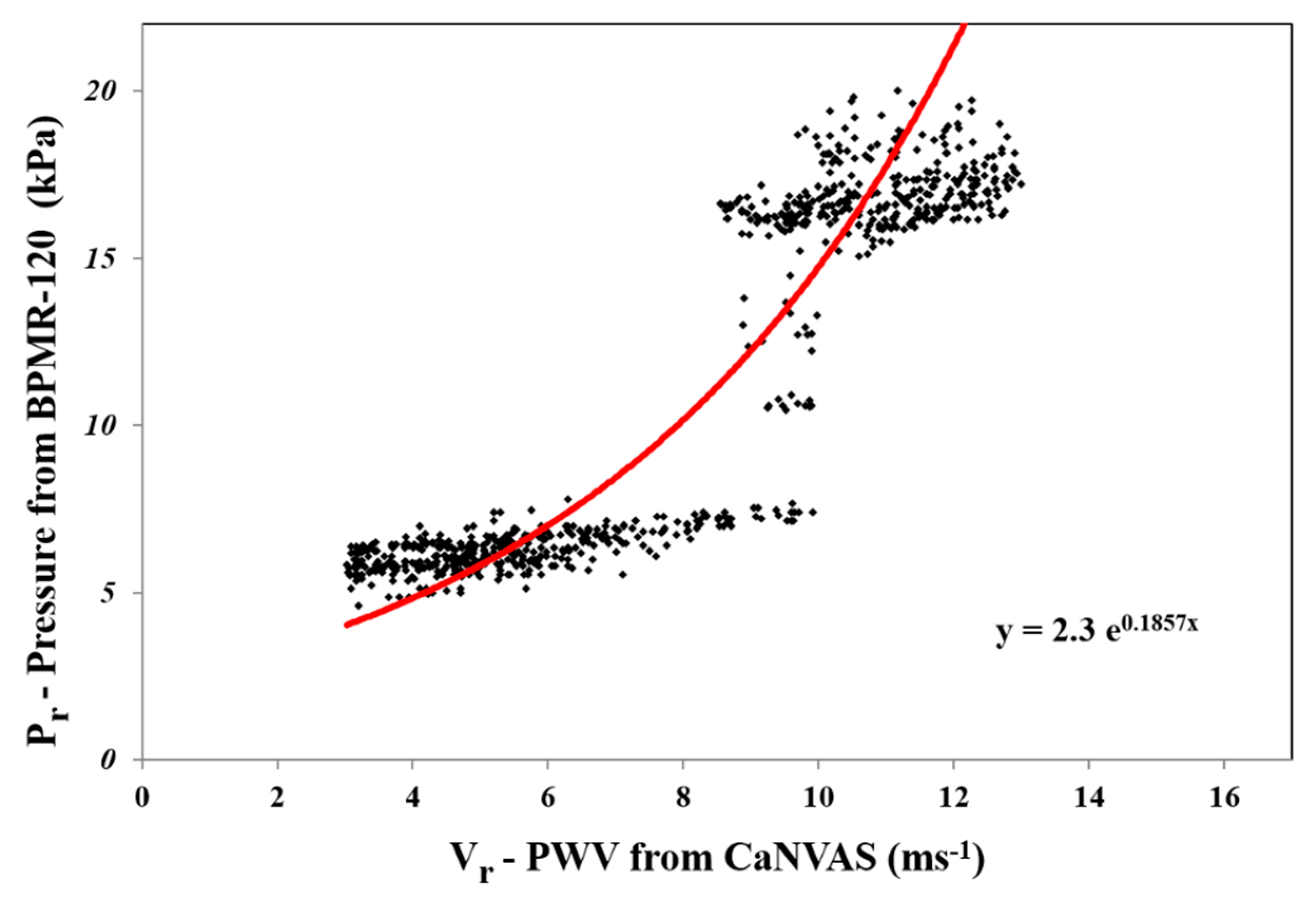
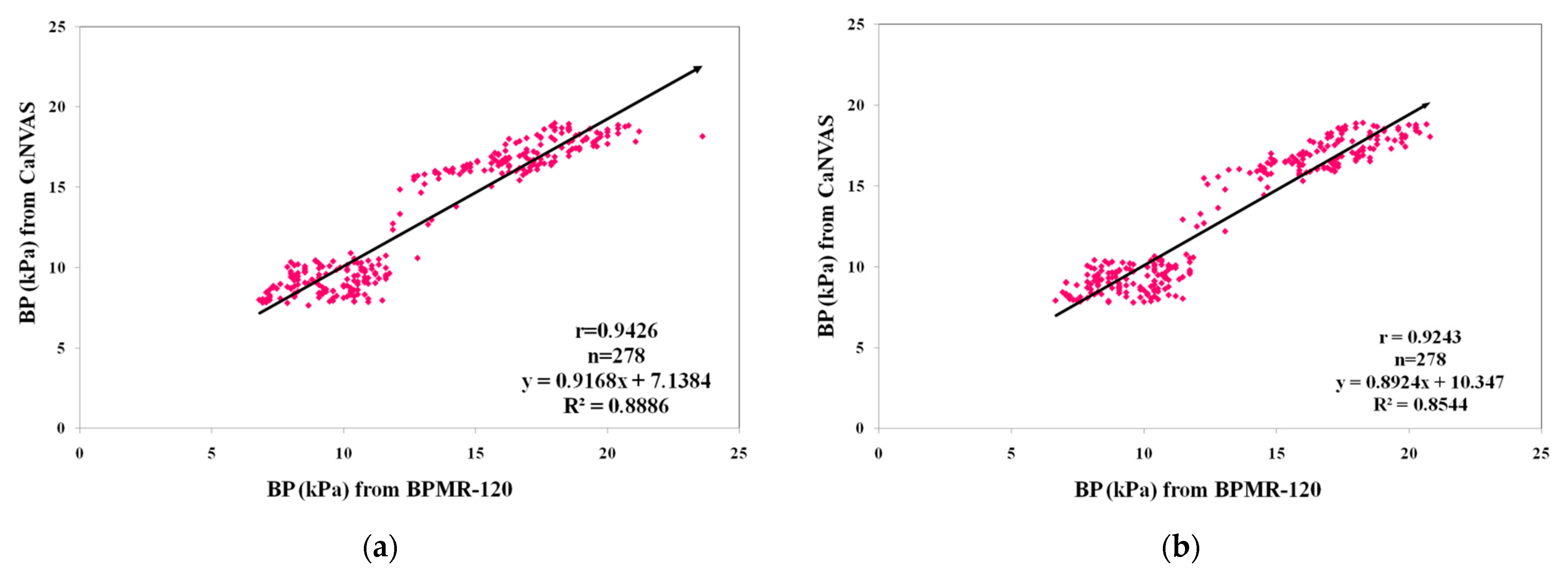
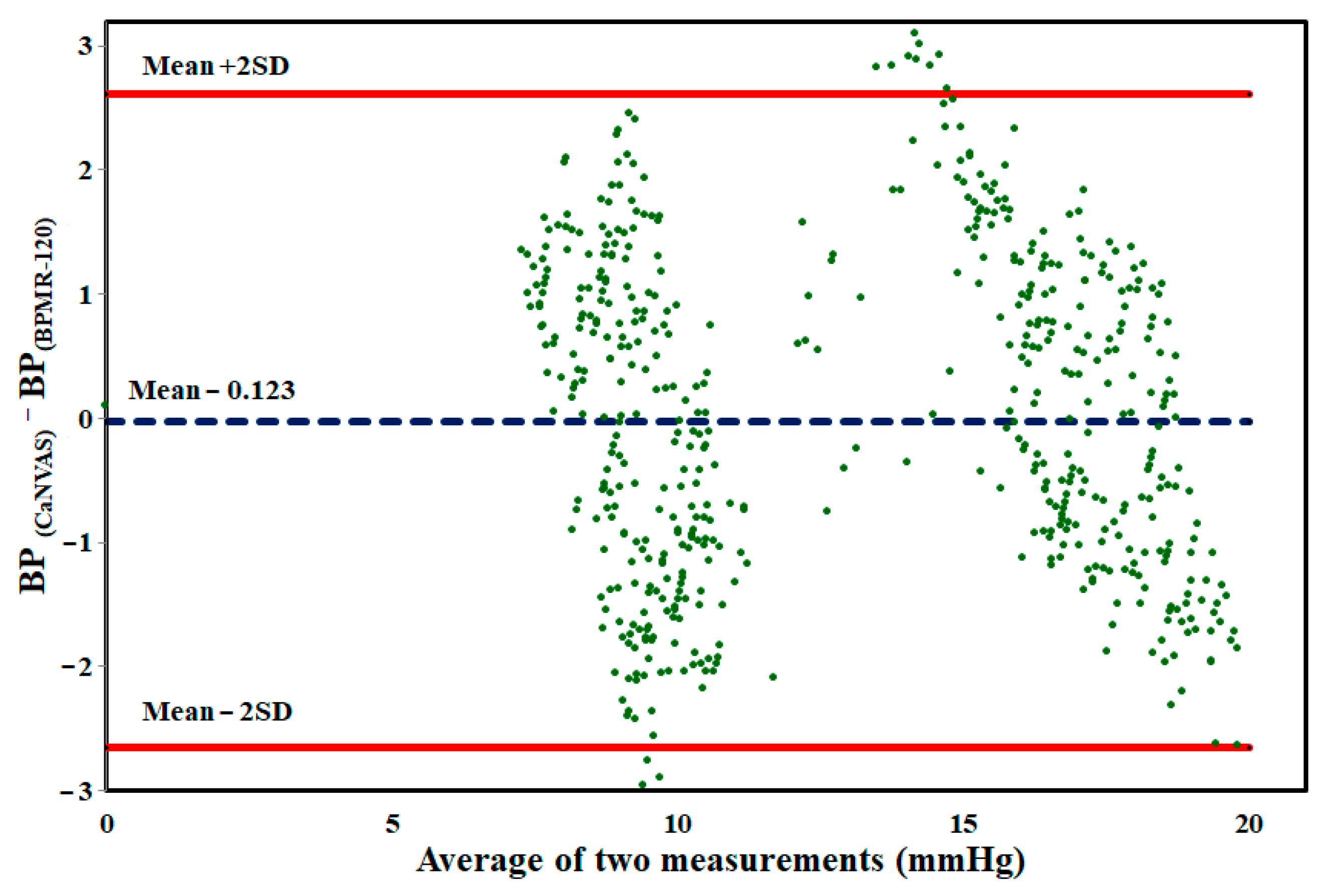

| Parameters Measured | Coefficient of Repeatability in % |
|---|---|
| Blood Pressure (kPa) | 10.2 |
| Pulse Wave Velocity (ms−1) | 11.3 |
| Arterial Compliance (mm2kPa−1) | 20.9 |
| Stiffness Index | 7.6 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sikkandar, M.Y.; Padmanabhan, S.; Mohan, B.; AlMohimeed, I.; Alassaf, A.; Alshewaier, S.A.; Almukil, A.A.; Begum, S. Computation of Vascular Parameters: Implementing Methodology and Performance Analysis. Biosensors 2023, 13, 757. https://doi.org/10.3390/bios13080757
Sikkandar MY, Padmanabhan S, Mohan B, AlMohimeed I, Alassaf A, Alshewaier SA, Almukil AA, Begum S. Computation of Vascular Parameters: Implementing Methodology and Performance Analysis. Biosensors. 2023; 13(8):757. https://doi.org/10.3390/bios13080757
Chicago/Turabian StyleSikkandar, Mohamed Yacin, Sridharan Padmanabhan, Bobby Mohan, Ibrahim AlMohimeed, Ahmad Alassaf, Shady A. Alshewaier, Ali Abdullah Almukil, and Sabarunisha Begum. 2023. "Computation of Vascular Parameters: Implementing Methodology and Performance Analysis" Biosensors 13, no. 8: 757. https://doi.org/10.3390/bios13080757
APA StyleSikkandar, M. Y., Padmanabhan, S., Mohan, B., AlMohimeed, I., Alassaf, A., Alshewaier, S. A., Almukil, A. A., & Begum, S. (2023). Computation of Vascular Parameters: Implementing Methodology and Performance Analysis. Biosensors, 13(8), 757. https://doi.org/10.3390/bios13080757





