Zero-Field and Field-Induced Interactions between Multicore Magnetic Nanoparticles
Abstract
1. Introduction
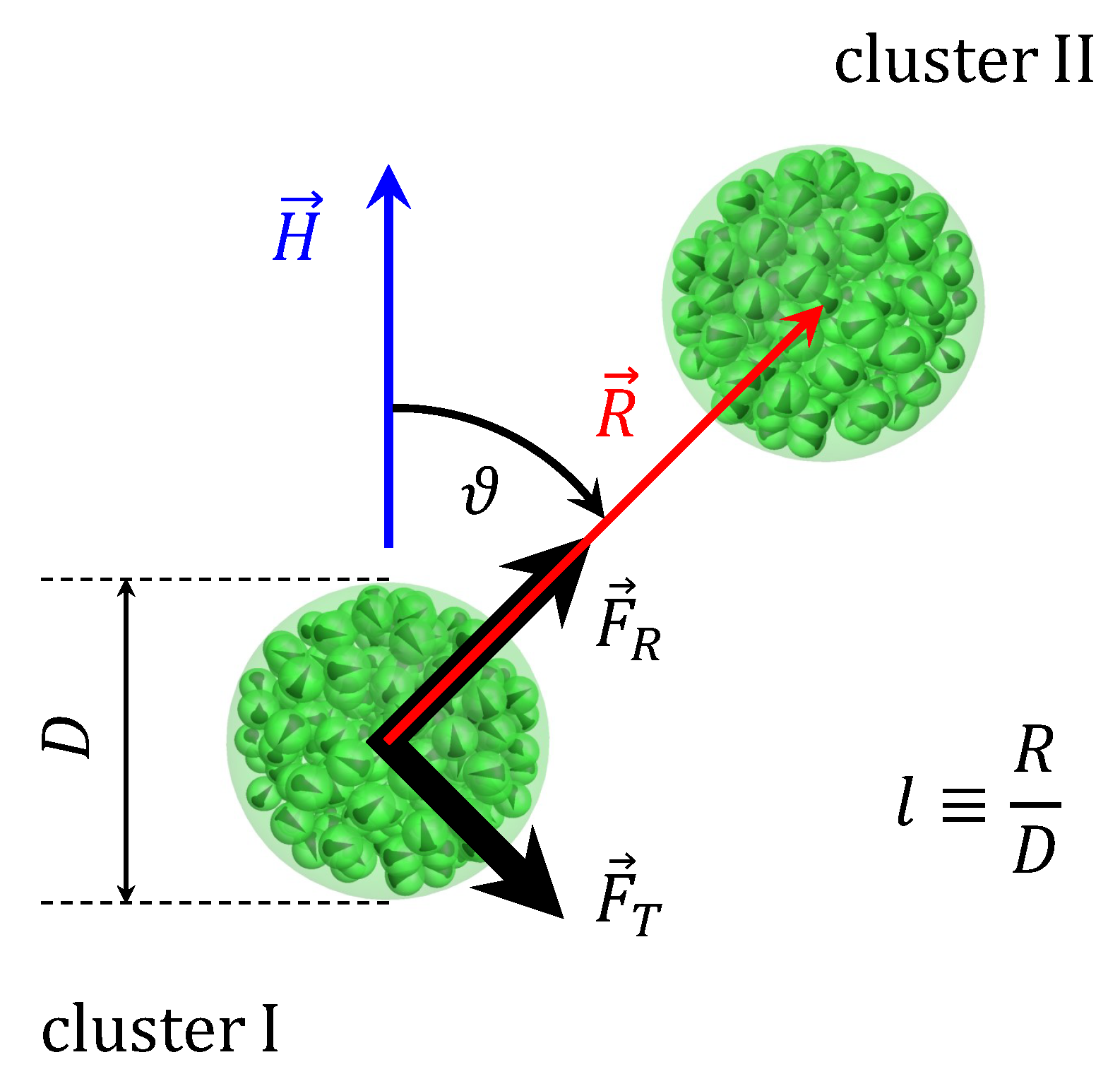
2. Model and Methods
3. Results and Discussion
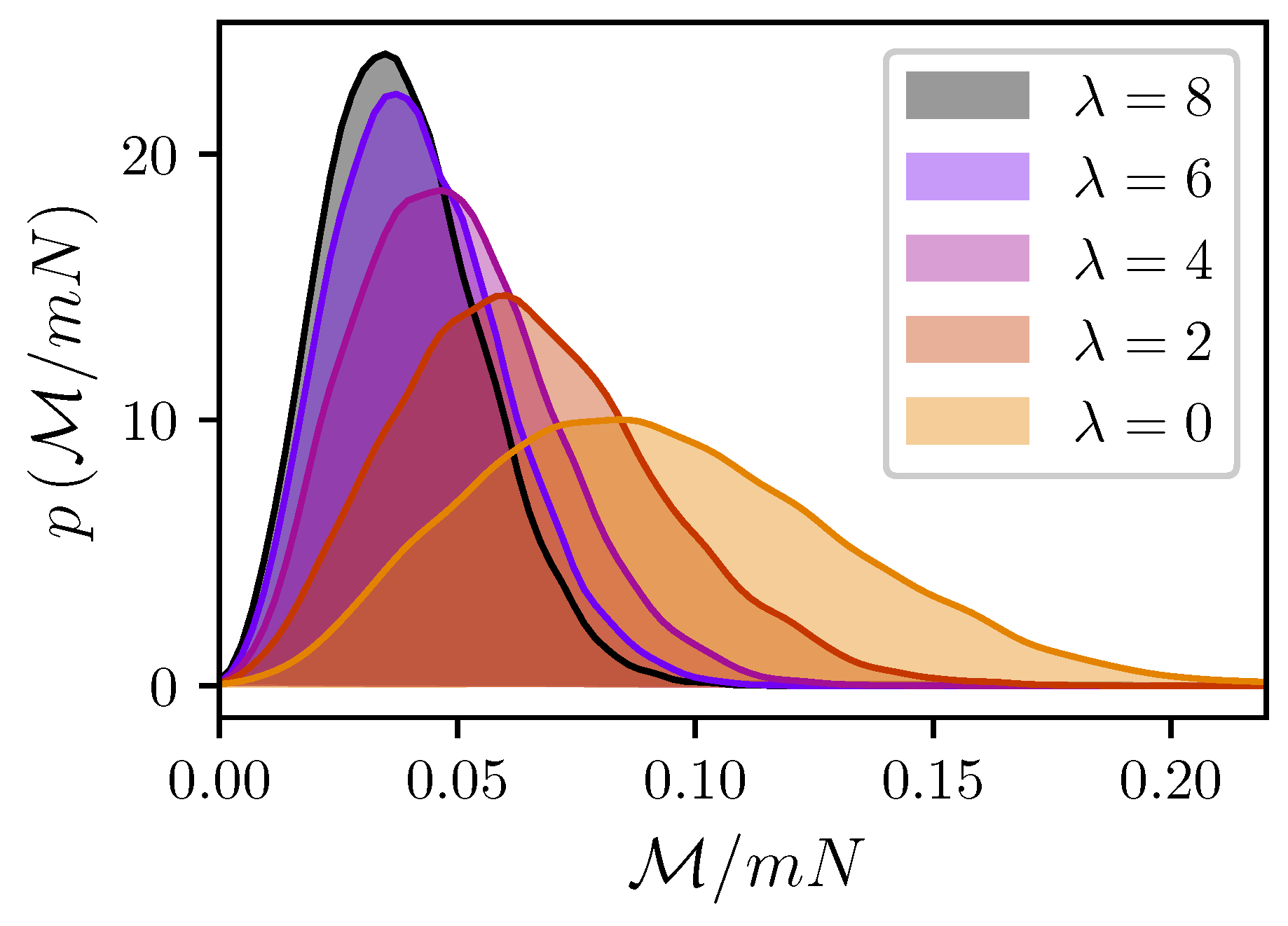
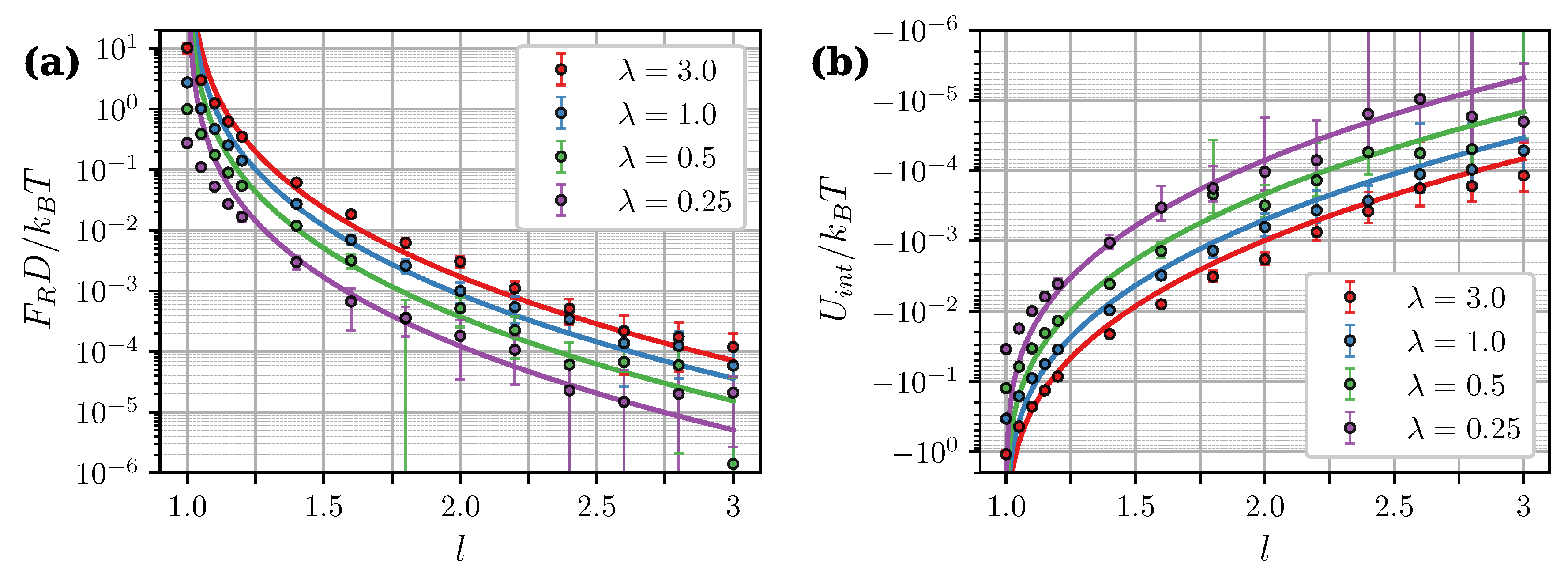
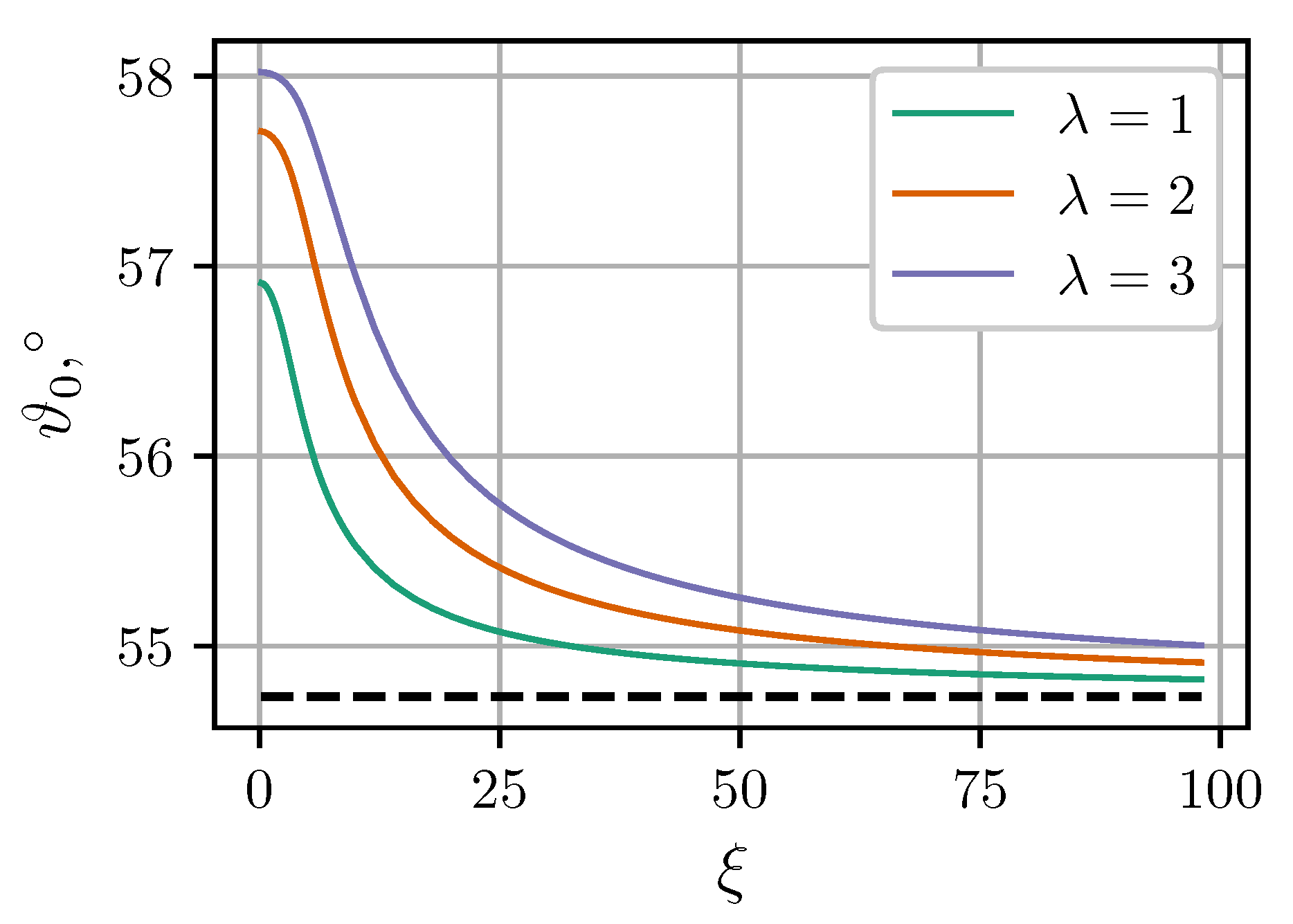
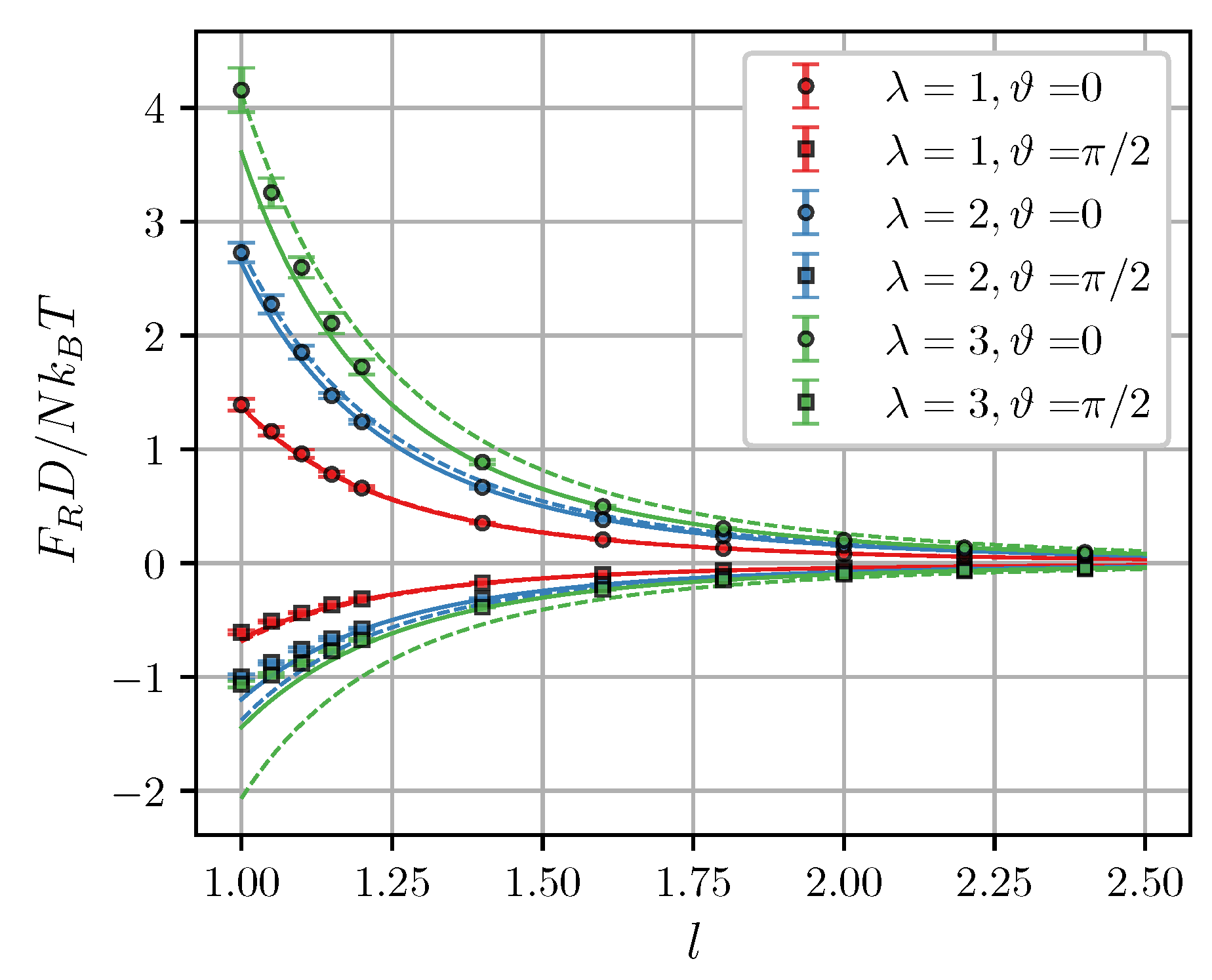
3.1. Zero-Field Interaction
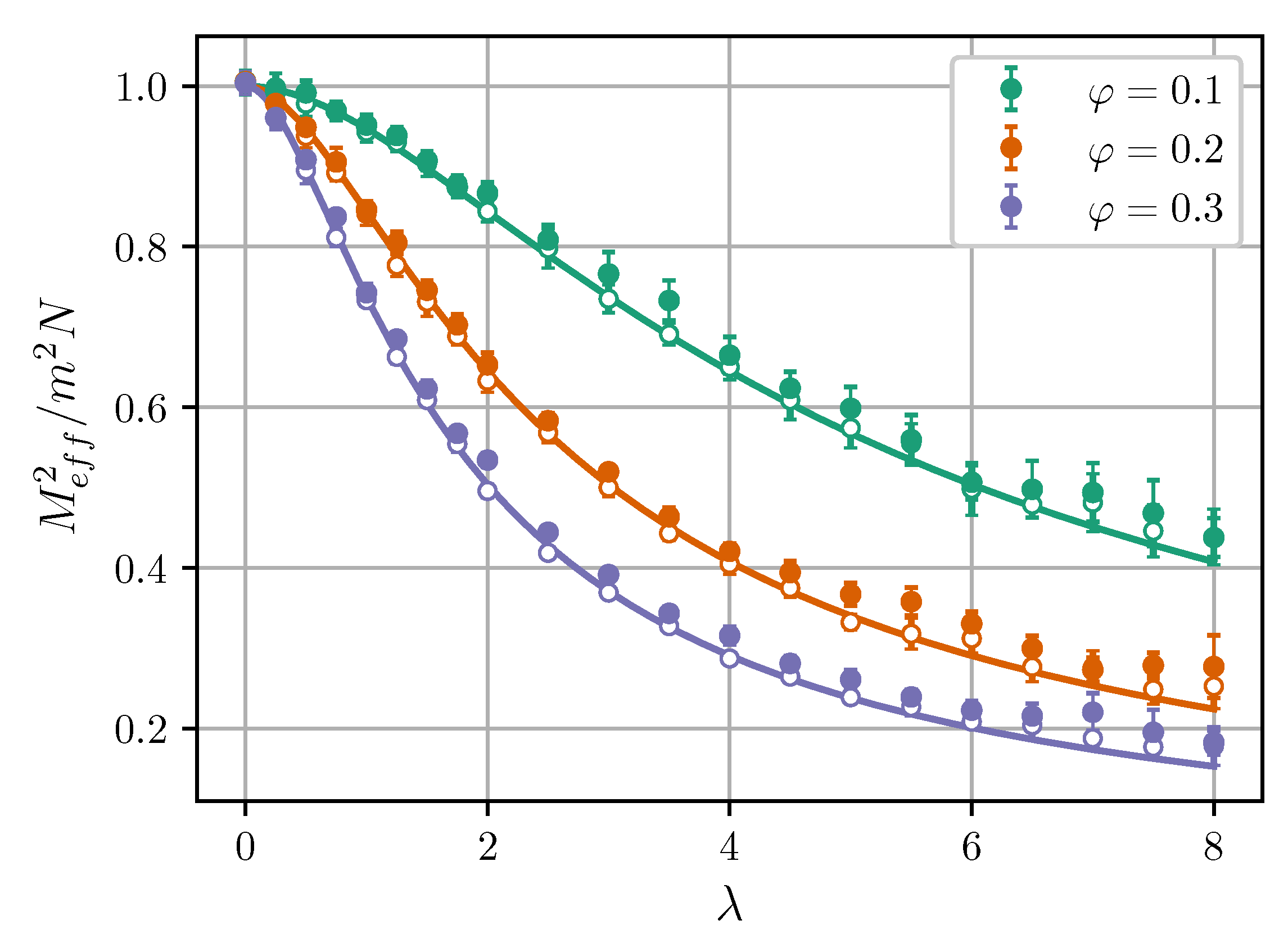
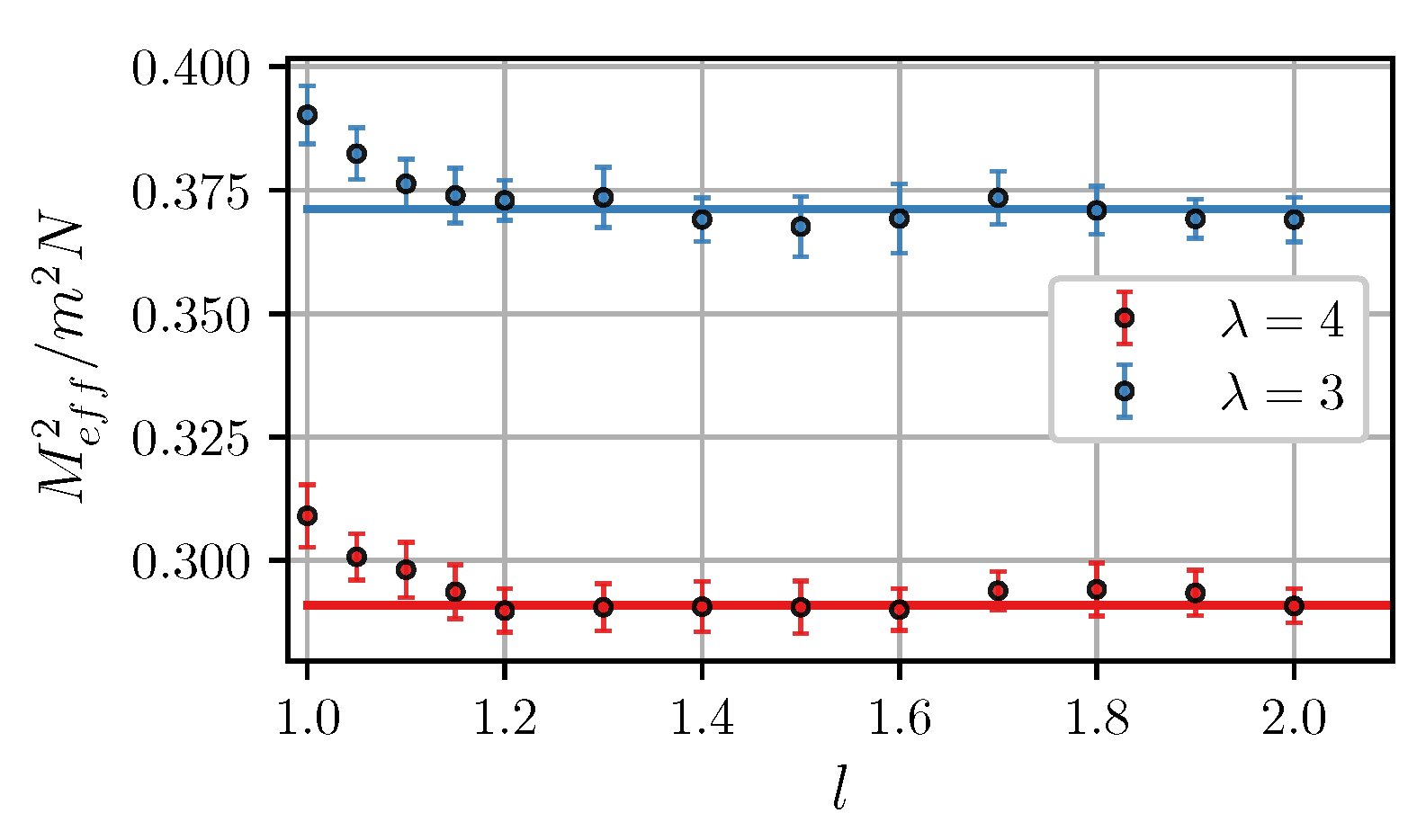
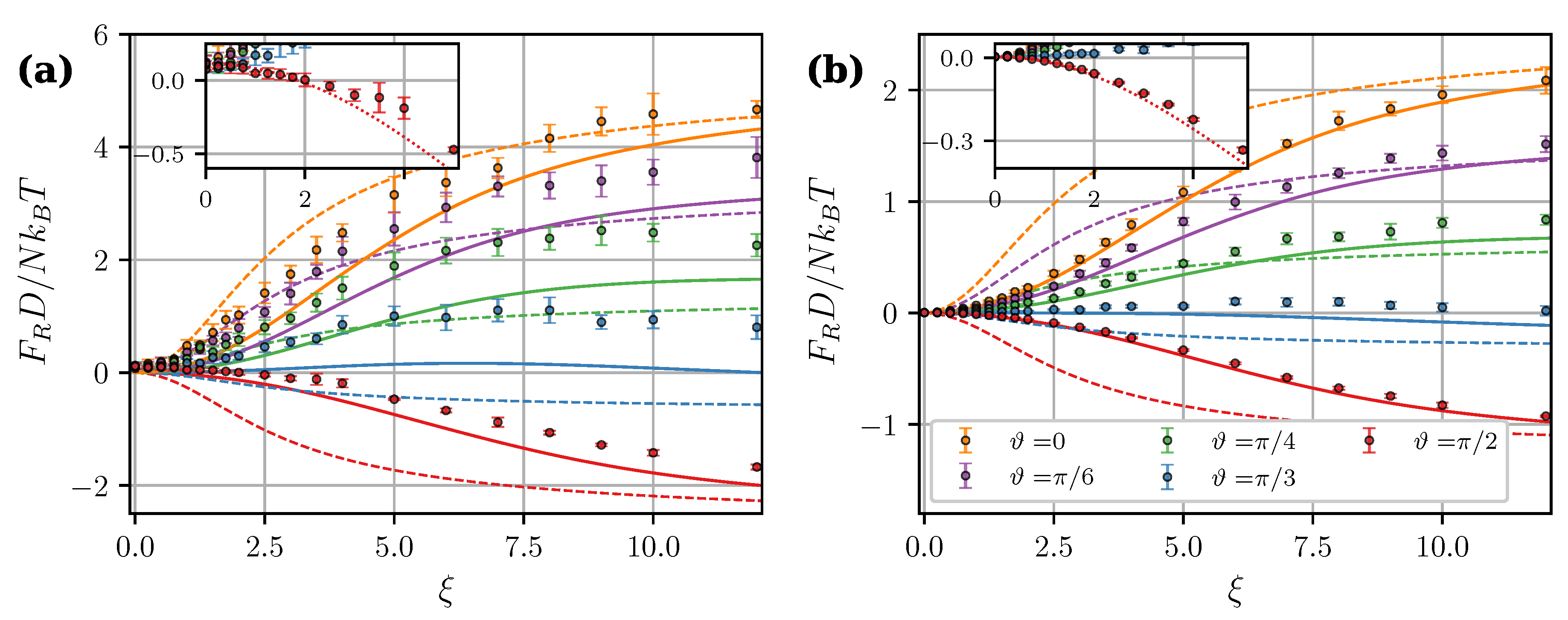
3.2. Field-Induced Interaction
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| MCMNP | Multicore Magnetic Nanoparticle |
| SCMNP | Single-core Magnetic Nanoparticle |
| MMFT | Modified Mean Field Theory |
| LDM | Langevin Dipoles Model |
| MDM | Mutual Dipoles Model |
References
- Gutiérrez, L.; Costo, R.; Grüttner, C.; Westphal, F.; Gehrke, N.; Heinke, D.; Fornara, A.; Pankhurst, Q.A.; Johansson, C.; Veintemillas-Verdaguer, S.; et al. Synthesis methods to prepare single- and multi-core iron oxide nanoparticles for biomedical applications. Dalton Trans. 2015, 44, 2943–2952. [Google Scholar] [CrossRef]
- Wells, J.; Kazakova, O.; Posth, O.; Steinhoff, U.; Petronis, S.; Bogart, L.K.; Southern, P.; Pankhurst, Q.; Johansson, C. Standardisation of magnetic nanoparticles in liquid suspension. J. Phys. D 2017, 50, 383003. [Google Scholar] [CrossRef]
- Dutz, S. Are magnetic multicore nanoparticles promising candidates for biomedical applications? IEEE Trans. Magn. 2016, 52, 1–3. [Google Scholar] [CrossRef]
- Ditsch, A.; Yin, J.; Laibinis, P.E.; Wang, D.I.C.; Hatton, T.A. Ion-Exchange Purification of Proteins Using Magnetic Nanoclusters. Biotechnol. Progress 2006, 22, 1153–1162. [Google Scholar] [CrossRef]
- Ko, J.; Lim, H.B. Multicore Magnetic Nanoparticles (MMNPs) Doped with Cs and FITC for the Determination of Biomarker in Serum using ICP-MS. Anal. Chem. 2014, 86, 4140–4144. [Google Scholar] [CrossRef]
- Jeong, A.; Lim, H. Magnetophoretic separation ICP-MS immunoassay using Cs-doped multicore magnetic nanoparticles for the determination of salmonella typhimurium. Talanta 2018, 178, 916–921. [Google Scholar] [CrossRef] [PubMed]
- Bakandritsos, A.; Papagiannopoulos, A.; Anagnostou, E.N.; Avgoustakis, K.; Zboril, R.; Pispas, S.; Tucek, J.; Ryukhtin, V.; Bouropoulos, N.; Kolokithas-Ntoukas, A.; et al. Merging High Doxorubicin Loading with Pronounced Magnetic Response and Bio-repellent Properties in Hybrid Drug Nanocarriers. Small 2012, 8, 2381–2393. [Google Scholar] [CrossRef] [PubMed]
- Lyer, S.; Tietze, R.; Unterweger, H.; Zaloga, J.; Singh, R.; Matuszak, J.; Poettler, M.; Friedrich, R.P.; Duerr, S.; Cicha, I.; et al. Nanomedical innovation: The SEON-concept for an improved cancer therapy with magnetic nanoparticles. Nanomedicine 2015, 10, 3287–3304. [Google Scholar] [CrossRef] [PubMed]
- Lim, E.K.; Kim, H.O.; Jang, E.; Park, J.; Lee, K.; Suh, J.S.; Huh, Y.M.; Haam, S. Hyaluronan-modified magnetic nanoclusters for detection of CD44-overexpressing breast cancer by MR imaging. Biomaterials 2011, 32, 7941–7950. [Google Scholar] [CrossRef]
- Pothayee, N.; Balasubramaniam, S.; Pothayee, N.; Jain, N.; Hu, N.; Lin, Y.; Davis, R.M.; Sriranganathan, N.; Koretsky, A.P.; Riffle, J.S. Magnetic nanoclusters with hydrophilic spacing for dual drug delivery and sensitive magnetic resonance imaging. J. Mater. Chem. B 2013, 1, 1142–1149. [Google Scholar] [CrossRef]
- Kim, M.H.; Kim, B.; Lim, E.K.; Choi, Y.; Choi, J.; Kim, E.; Jang, E.; Park, H.S.; Suh, J.S.; Huh, Y.M.; et al. Magnetic Nanoclusters Engineered by Polymer-Controlled Self-Assembly for the Accurate Diagnosis of Atherosclerotic Plaques via Magnetic Resonance Imaging. Macromol. Biosci. 2014, 14, 943–952. [Google Scholar] [CrossRef]
- Eberbeck, D.; Dennis, C.L.; Huls, N.F.; Krycka, K.L.; Gruttner, C.; Westphal, F. Multicore magnetic nanoparticles for magnetic particle imaging. IEEE Trans. Magn. 2013, 49, 269–274. [Google Scholar] [CrossRef]
- Zhou, X.Y.; Jeffris, K.E.; Elaine, Y.Y.; Zheng, B.; Goodwill, P.W.; Nahid, P.; Conolly, S.M. First in vivo magnetic particle imaging of lung perfusion in rats. Phys. Med. Biol. 2017, 62, 3510. [Google Scholar] [CrossRef]
- Ziemian, S.; Löwa, N.; Kosch, O.; Bajj, D.; Wiekhorst, F.; Schütz, G. Optimization of Iron Oxide Tracer Synthesis for Magnetic Particle Imaging. Nanomaterials 2018, 8, 180. [Google Scholar] [CrossRef]
- Qu, Y.; Li, J.; Ren, J.; Leng, J.; Lin, C.; Shi, D. Enhanced Magnetic Fluid Hyperthermia by Micellar Magnetic Nanoclusters Composed of Mn x Zn1–x Fe2O4 Nanoparticles for Induced Tumor Cell Apoptosis. ACS Appl. Mater. Interfaces 2014, 6, 16867–16879. [Google Scholar] [CrossRef]
- Hemery, G.; Genevois, C.; Couillaud, F.; Lacomme, S.; Gontier, E.; Ibarboure, E.; Lecommandoux, S.; Garanger, E.; Sandre, O. Monocore vs. multicore magnetic iron oxide nanoparticles: Uptake by glioblastoma cells and efficiency for magnetic hyperthermia. Mol. Syst. Des. Eng. 2017, 2, 629–639. [Google Scholar] [CrossRef]
- Sathya, A.; Kalyani, S.; Ranoo, S.; Philip, J. One-step microwave-assisted synthesis of water-dispersible Fe3O4 magnetic nanoclusters for hyperthermia applications. J. Magn. Magn. Mater. 2017, 439, 107–113. [Google Scholar] [CrossRef]
- Bender, P.; Fock, J.; Hansen, M.; Bogart, L.; Southern, P.; Ludwig, F.; Wiekhorst, F.; Szczerba, W.; Zeng, L.; Heinke, D.; et al. Influence of clustering on the magnetic properties and hyperthermia performance of iron oxide nanoparticles. Nanotechnology 2018, 29, 425705. [Google Scholar] [CrossRef]
- Schaller, V.; Wahnström, G.; Sanz-Velasco, A.; Enoksson, P.; Johansson, C. Monte Carlo simulation of magnetic multi-core nanoparticles. J. Magn. Magn. Mater. 2009, 321, 1400–1403. [Google Scholar] [CrossRef]
- Schaller, V.; Wahnström, G.; Sanz-Velasco, A.; Gustafsson, S.; Olsson, E.; Enoksson, P.; Johansson, C. Effective magnetic moment of magnetic multicore nanoparticles. Phys. Rev. B 2009, 80, 092406. [Google Scholar] [CrossRef]
- Kuznetsov, A.A. Equilibrium magnetization of a quasispherical cluster of single-domain particles. Phys. Rev. B 2018, 98, 144418. [Google Scholar] [CrossRef]
- Weddemann, A.; Auge, A.; Kappe, D.; Wittbracht, F.; Hütten, A. Dynamic simulations of the dipolar driven demagnetization process of magnetic multi-core nanoparticles. J. Magn. Magn. Mater. 2010, 322, 643–646. [Google Scholar] [CrossRef]
- Usov, N.A.; Serebryakova, O.N.; Tarasov, V.P. Interaction effects in assembly of magnetic nanoparticles. Nanoscale Res. Lett. 2017, 12, 489. [Google Scholar] [CrossRef]
- Ilg, P. Equilibrium magnetization and magnetization relaxation of multicore magnetic nanoparticles. Phys. Rev. B 2017, 95, 214427. [Google Scholar] [CrossRef]
- Kuznetsov, A.A. Force acting on a cluster of magnetic nanoparticles in a gradient field: A Langevin dynamics study. J. Magn. Magn. Mater. 2019, 475, 415–420. [Google Scholar] [CrossRef]
- Bean, C.P.; Livingston, J.D. Superparamagnetism. J. Appl. Phys. 1959, 30, S120–S129. [Google Scholar] [CrossRef]
- Ivanov, A.O.; Kuznetsova, O.B. Magnetic properties of dense ferrofluids: An influence of interparticle correlations. Phys. Rev. E 2001, 64, 041405. [Google Scholar] [CrossRef]
- Ivanov, A.O.; Zverev, V.S.; Kantorovich, S.S. Revealing the signature of dipolar interactions in dynamic spectra of polydisperse magnetic nanoparticles. Soft Matter 2016, 12, 3507–3513. [Google Scholar] [CrossRef]
- Wang, Z.; Holm, C.; Müller, H.W. Molecular dynamics study on the equilibrium magnetization properties and structure of ferrofluids. Phys. Rev. E 2002, 66, 021405. [Google Scholar] [CrossRef]
- Holm, C.; Weis, J.J. The structure of ferrofluids: A status report. Curr. Opin. Colloid Interface Sci. 2005, 10, 133–140. [Google Scholar] [CrossRef]
- Rovigatti, L.; Russo, J.; Sciortino, F. Structural properties of the dipolar hard-sphere fluid at low temperatures and densities. Soft Matter 2012, 8, 6310–6319. [Google Scholar] [CrossRef]
- Kantorovich, S.S.; Ivanov, A.O.; Rovigatti, L.; Tavares, J.M.; Sciortino, F. Temperature-induced structural transitions in self-assembling magnetic nanocolloids. Phys. Chem. Chem. Phys. 2015, 17, 16601–16608. [Google Scholar] [CrossRef] [PubMed]
- Ryzhkov, A.V.; Melenev, P.V.; Balasoiu, M.; Raikher, Y.L. Structure organization and magnetic properties of microscale ferrogels: The effect of particle magnetic anisotropy. J. Chem. Phys. 2016, 145, 074905. [Google Scholar] [CrossRef]
- Bossis, G.; Volkova, O.; Lacis, S.; Meunier, A. Magnetorheology: Fluids, structures and rheology. In Ferrofluids; Springer: Berlin, Germany, 2002; pp. 202–230. [Google Scholar] [CrossRef]
- Keaveny, E.E.; Maxey, M.R. Modeling the magnetic interactions between paramagnetic beads in magnetorheological fluids. J. Comput. Phys. 2008, 227, 9554–9571. [Google Scholar] [CrossRef]
- Biller, A.; Stolbov, O.; Raikher, Y.L. Dipolar models of ferromagnet particles interaction in magnetorheological composites. J. Optoelectron. Adv. Mater. 2015, 17, 1106–1113. [Google Scholar]
- Martin, J.E.; Anderson, R.A.; Tigges, C.P. Simulation of the athermal coarsening of composites structured by a uniaxial field. J. Chem. Phys. 1998, 108, 3765–3787. [Google Scholar] [CrossRef]
- Furst, E.M.; Gast, A.P. Dynamics and lateral interactions of dipolar chains. Phys. Rev. E 2000, 62, 6916. [Google Scholar] [CrossRef]
- Segovia-Gutierrez, J.P.; de Vicente, J.; Hidalgo-Alvarez, R.; Puertas, A.M. Brownian dynamics simulations in magnetorheology and comparison with experiments. Soft Matter 2013, 9, 6970–6977. [Google Scholar] [CrossRef]
- Ivanov, A.S.; Pshenichnikov, A.F. Magnetophoresis and diffusion of colloidal particles in a thin layer of magnetic fluids. J. Magn. Magn. Mater. 2010, 322, 2575–2580. [Google Scholar] [CrossRef]
- Borin, D.; Zubarev, A.; Chirikov, D.; Müller, R.; Odenbach, S. Ferrofluid with clustered iron nanoparticles: Slow relaxation of rheological properties under joint action of shear flow and magnetic field. J. Magn. Magn. Mater. 2011, 323, 1273–1277. [Google Scholar] [CrossRef]
- Magnet, C.; Kuzhir, P.; Bossis, G.; Meunier, A.; Suloeva, L.; Zubarev, A. Haloing in bimodal magnetic colloids: The role of field-induced phase separation. Phys. Rev. E 2012, 86, 011404. [Google Scholar] [CrossRef] [PubMed]
- Faraudo, J.; Andreu, J.S.; Calero, C.; Camacho, J. Predicting the Self-Assembly of Superparamagnetic Colloids under Magnetic Fields. Adv. Funct. Mater. 2016, 26, 3837–3858. [Google Scholar] [CrossRef]
- Lartigue, L.; Hugounenq, P.; Alloyeau, D.; Clarke, S.P.; Lévy, M.; Bacri, J.C.; Bazzi, R.; Brougham, D.F.; Wilhelm, C.; Gazeau, F. Cooperative organization in iron oxide multi-core nanoparticles potentiates their efficiency as heating mediators and MRI contrast agents. ACS Nano 2012, 6, 10935–10949. [Google Scholar] [CrossRef] [PubMed]
- Bender, P.; Fock, J.; Frandsen, C.; Hansen, M.F.; Balceris, C.; Ludwig, F.; Posth, O.; Wetterskog, E.; Bogart, L.K.; Southern, P.; et al. Relating magnetic properties and high hyperthermia performance of iron oxide nanoflowers. J. Phys. Chem. C 2018, 122, 3068–3077. [Google Scholar] [CrossRef]
- García-Palacios, J.L.; Lázaro, F.J. Langevin-dynamics study of the dynamical properties of small magnetic particles. Phys. Rev. B 1998, 58, 14937. [Google Scholar] [CrossRef]
- Ahrentorp, F.; Astalan, A.; Blomgren, J.; Jonasson, C.; Wetterskog, E.; Svedlindh, P.; Lak, A.; Ludwig, F.; van IJzendoorn, L.J.; Westphal, F.; et al. Effective particle magnetic moment of multi-core particles. J. Magn. Magn. Mater. 2015, 380, 221–226. [Google Scholar] [CrossRef]
- De Gennes, P.G.; Pincus, P.A. Pair correlations in a ferromagnetic colloid. Phys. Kondens. Mater. 1970, 11, 189–198. [Google Scholar] [CrossRef]
- Hamaker, H. The London—van der Waals attraction between spherical particles. Physica 1937, 4, 1058–1072. [Google Scholar] [CrossRef]
- Stenhammar, J.; Linse, P.; Wennerstroöm, H.; Karlström, G. An exact calculation of the van der Waals interaction between two spheres of classical dipolar fluid. J. Phys. Chem. B 2010, 114, 13372–13380. [Google Scholar] [CrossRef]
- Israelachvili, J.N. Intermolecular and Surface Forces; Academic Press: Cambridge, MA, USA, 2011. [Google Scholar]


© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuznetsov, A.A. Zero-Field and Field-Induced Interactions between Multicore Magnetic Nanoparticles. Nanomaterials 2019, 9, 718. https://doi.org/10.3390/nano9050718
Kuznetsov AA. Zero-Field and Field-Induced Interactions between Multicore Magnetic Nanoparticles. Nanomaterials. 2019; 9(5):718. https://doi.org/10.3390/nano9050718
Chicago/Turabian StyleKuznetsov, Andrey A. 2019. "Zero-Field and Field-Induced Interactions between Multicore Magnetic Nanoparticles" Nanomaterials 9, no. 5: 718. https://doi.org/10.3390/nano9050718
APA StyleKuznetsov, A. A. (2019). Zero-Field and Field-Induced Interactions between Multicore Magnetic Nanoparticles. Nanomaterials, 9(5), 718. https://doi.org/10.3390/nano9050718




