Abrasion Effect on Heating Performance of Carbon Nanotube/Epoxy Composites
Abstract
1. Introduction
2. Materials and Methods
2.1. Fabrication of CNT/Epoxy Composites
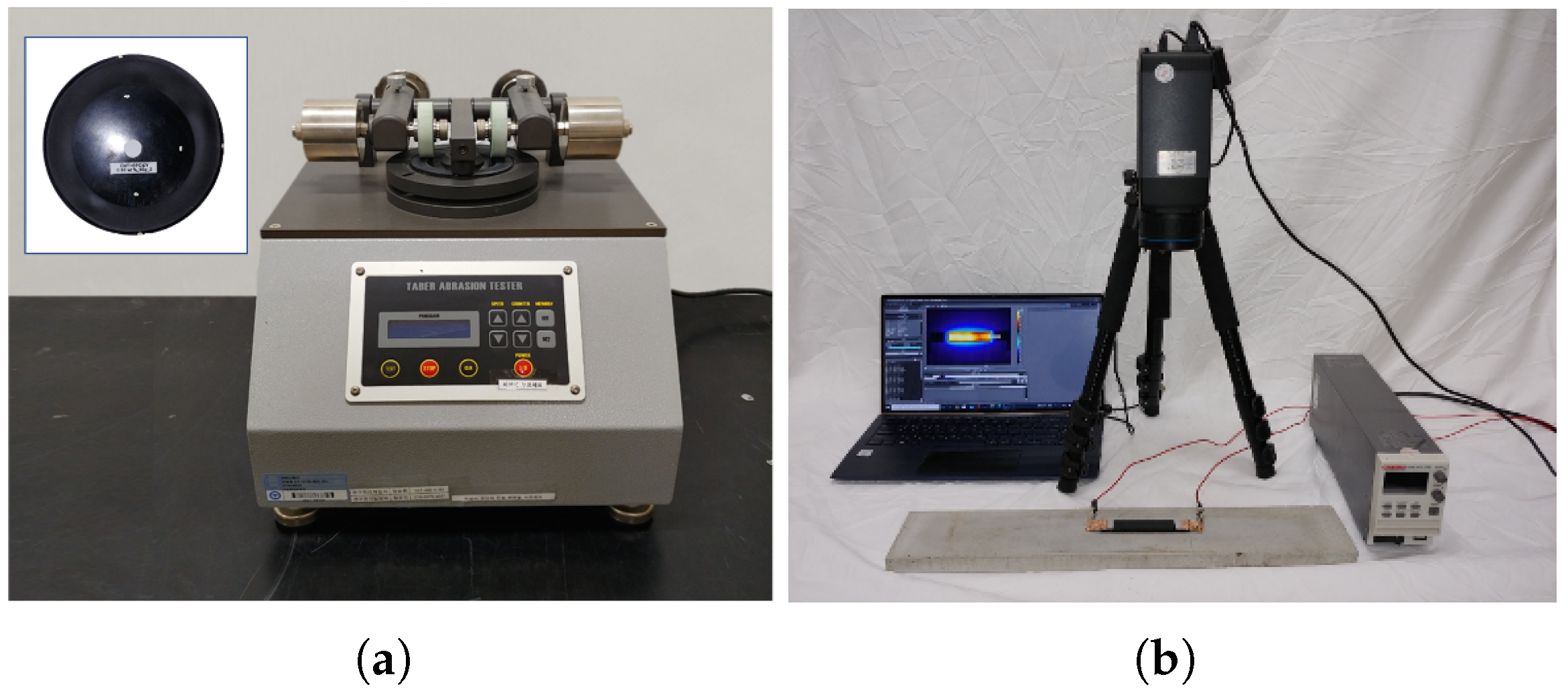
2.2. Characterizations
3. Results and Discussion
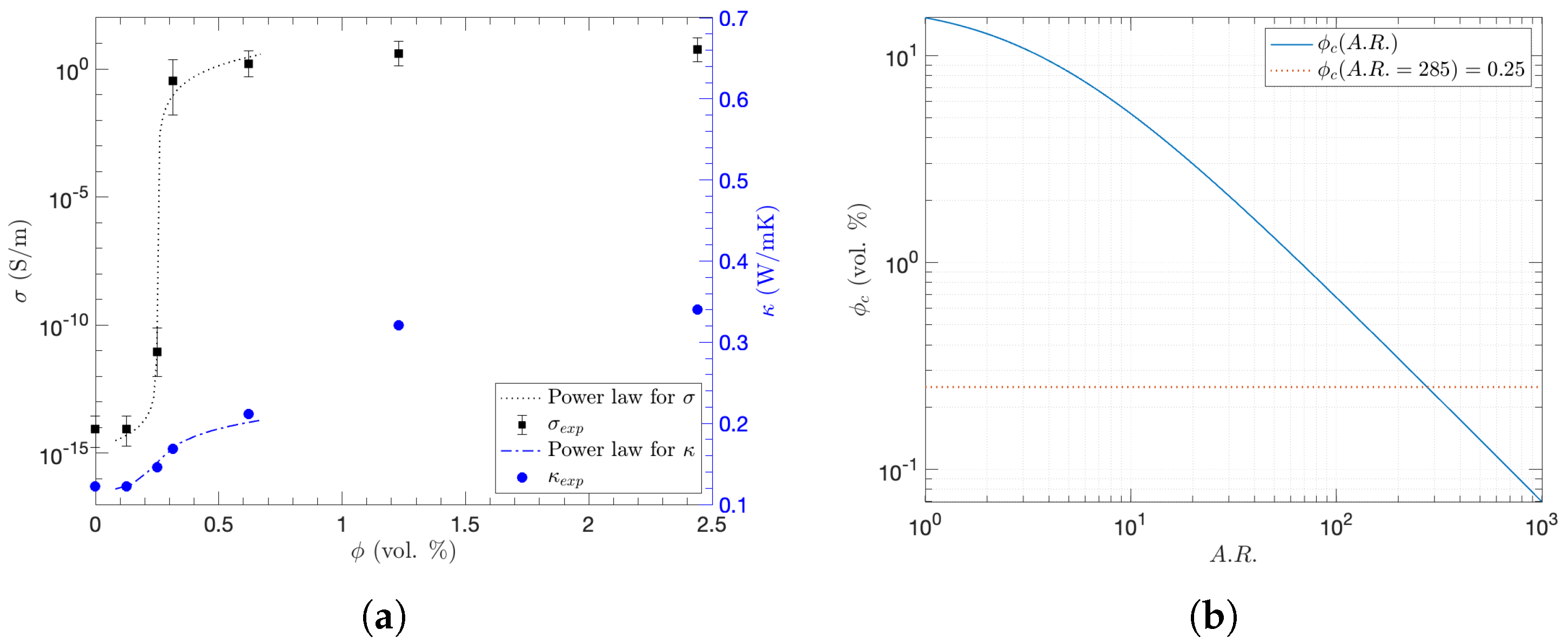
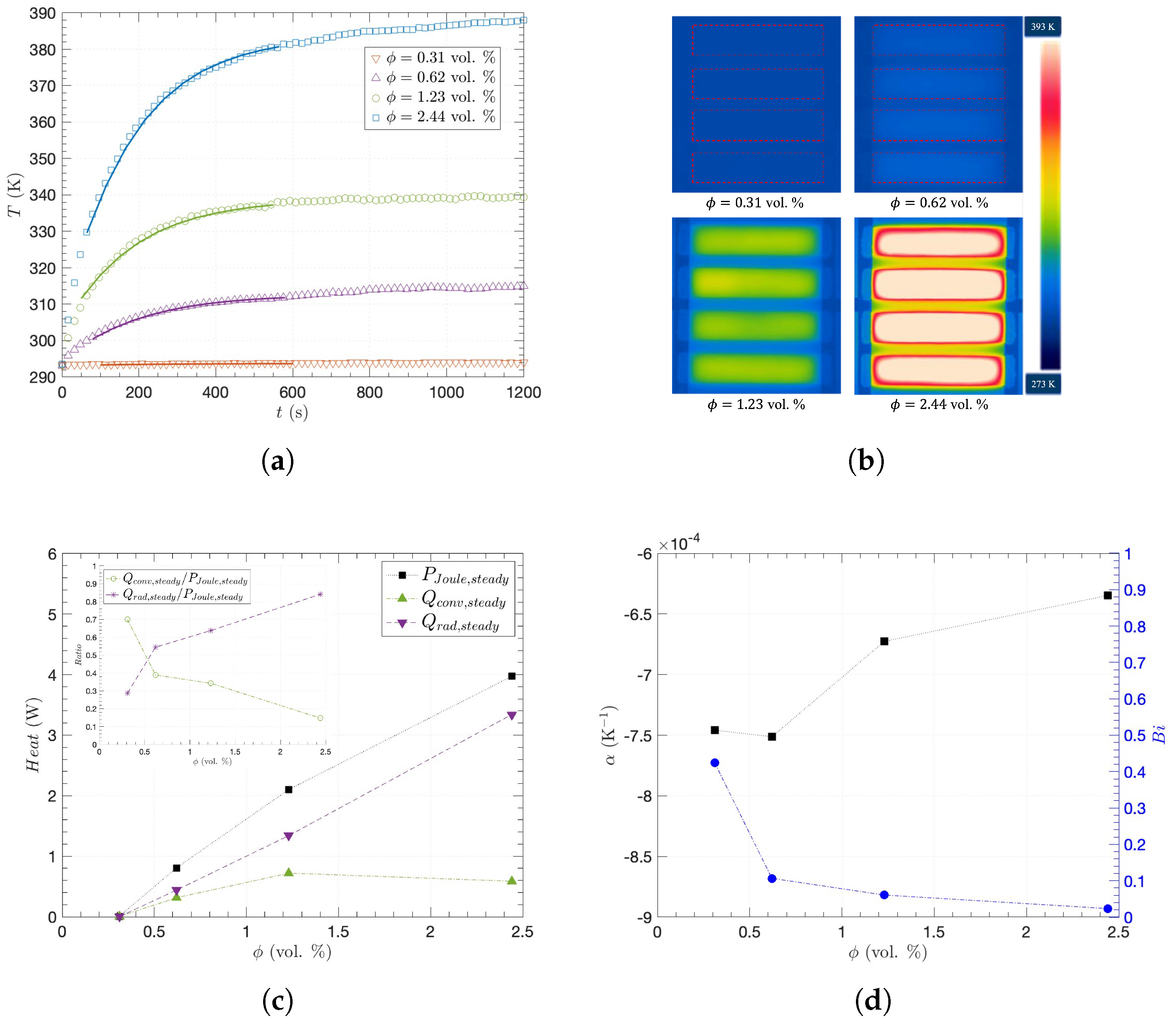
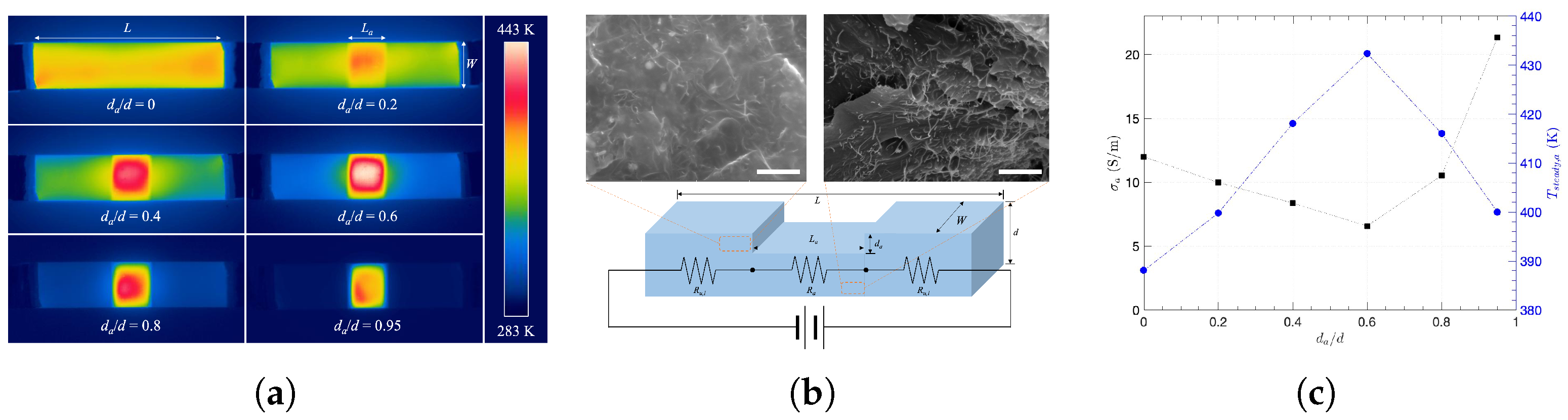
3.1. Electrical Percolation and Heating Performance
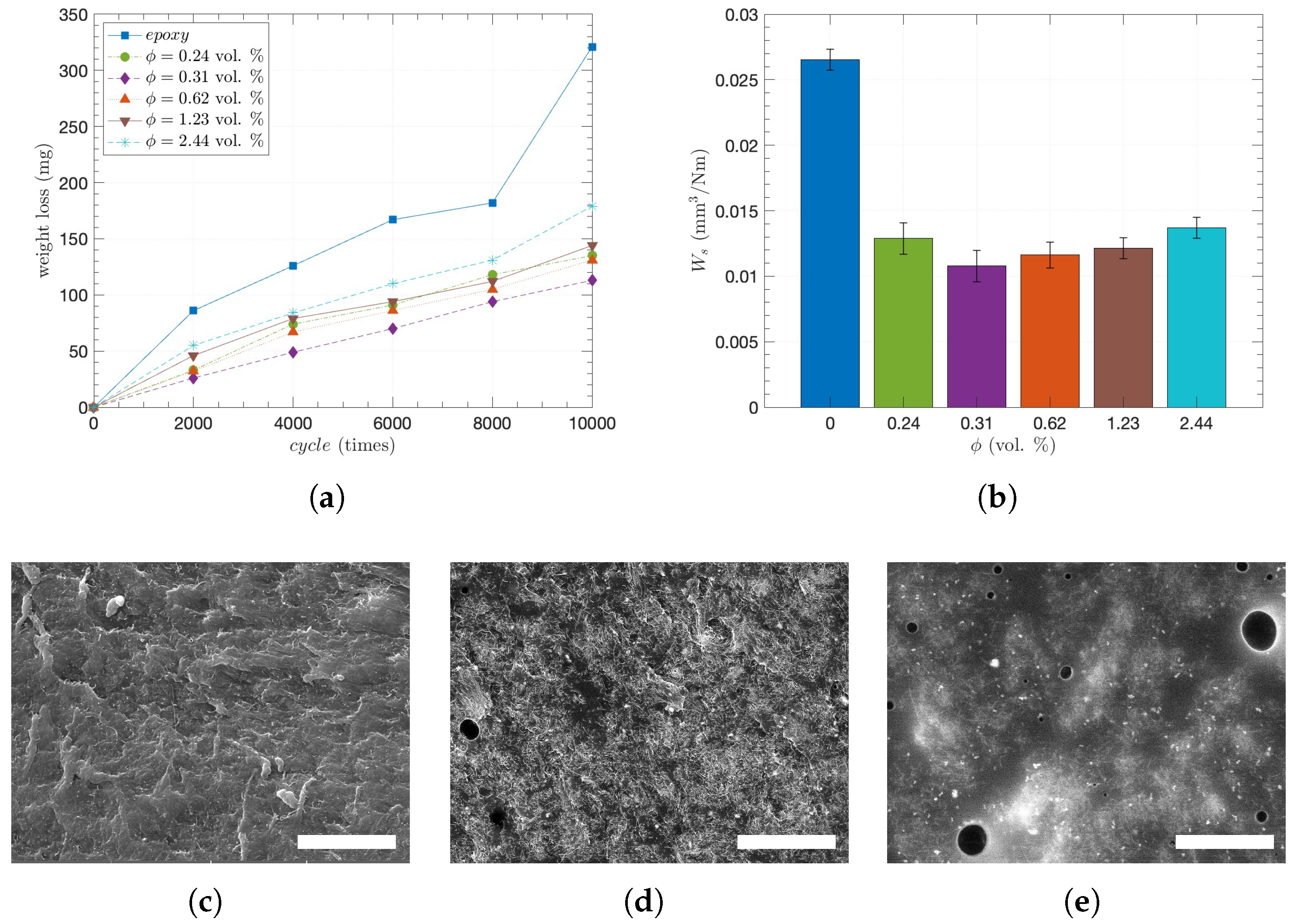
3.2. Abrasion Characterizations of CNT/Epoxy Composites
3.3. Abrasion Effect on Heating Performance of the CNT/Epoxy Composites
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Baughman, R.; Zakhidov, A.; Heer, W.D. Carbon nanotubes-the route toward applications. Science 2002, 297, 787–792. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Ma, P.; Chow, W.; To, C.; Tang, B.; Kim, J. Correlations between percolation threshold, dispersion state, and aspect ratio of carbon nanotubes. Adv. Funct. Mater. 2007, 17, 3207–3215. [Google Scholar] [CrossRef]
- Tjong, S. Structural and mechanical properties of polymer nanocomposites. Mater. Sci. Eng. R 2006, 53, 73–197. [Google Scholar] [CrossRef]
- Li, Y.; Wang, Q.; Wang, S. A review on enhancement of mechanical and tribological properties of polymer composites reinforced by carbon nanotubes and graphene sheet: Molecular dynamic simulations. Compos. Part B 2019, 160, 348–361. [Google Scholar] [CrossRef]
- Balguri, P.; Samuel, D.; Thumu, U. A review on mechanical properties of epoxy nanocomposites. Mater. Today Proc. 2021, 44, 346–355. [Google Scholar] [CrossRef]
- Ahir, S.; Terentjev, E. Photomechanical actuation in polymer-nanotube composites. Nat. Mater. 2005, 4, 491–495. [Google Scholar] [CrossRef]
- Azeez, A.; Rhee, K.; Park, S.; Hui, D. Epoxy clay nanocomposites—Processing, properties and application: A review. Compos. Part B 2013, 45, 308–320. [Google Scholar] [CrossRef]
- Vertuccio, L.; Foglia, F.; Pantani, R.; Romero-Sanchez, M.; Calderon, B.; Guadagno, L. Carbon nanotubes and expanded graphite based bulk nanocomposites for de-icing applications. Compos. Part B 2021, 207, 108583. [Google Scholar] [CrossRef]
- Lee, S.J.; Jung, Y.J.; Cho, C.; Jang, S.H. Effect of Atmospheric Temperature on Epoxy Coating Reinforced with Carbon Nanotubes for De-Icing on Road Systems. Nanomaterials 2023, 13, 2248. [Google Scholar] [CrossRef]
- Jala, J.; Nowacki, B.; Mistewicz, K.; Gradon, P. Graphite-epoxy composite systems for Joule heating based de-icing. Cold Reg. Sci. Technol. 2023, 216, 104024. [Google Scholar] [CrossRef]
- Raji, A.; Varadhachary, T.; Nan, K.; Wang, T.; Lin, J.; Ji, Y.; Genorio, B.; Zhu, Y.; Kittrell, C.; Tour, J. Composites of graphene nanoribbon stacks and epoxy for Joule heating and deicing of surface. ACS Appl. Mater. Interfaces 2016, 8, 3551–3556. [Google Scholar] [CrossRef] [PubMed]
- Yao, X.; Hawkins, S.; Falzon, B. An advanced anti-icing/de-icing system utilizing highly aligned carbon nanotube webs. Carbon 2018, 136, 130–138. [Google Scholar] [CrossRef]
- Fenta, E.W.; Mebratie, B.A. Advancements in carbon nanotube-polymer composites: Enhancing properties and applications through advanced manufacturing techniques. Heliyon 2024, 10, e36490. [Google Scholar] [CrossRef] [PubMed]
- Mucha, M.; Krzyzak, A.; Kosicka, E.; Coy, E.; Kościński, M.; Sterzyński, T.; Sałaciński, M. Effect of MWCNTs on Wear Behavior of Epoxy Resin for Aircraft Applications. Materials 2020, 13, 2696. [Google Scholar] [CrossRef]
- Grm, V.; Zave, D.; Drazic, G. A carbon-nanotubes-based heating fabric composite for automotive applications. Mater. Technol. 2020, 54, 761–768. [Google Scholar] [CrossRef]
- Park, Y.; Gwon, N.H.; Seong, W.K.; Kim, W. Heater-Integrated Flexible Piezoresistive Pressure Sensor Array for Smart-Car Seats. IEEE Sens. J. 2024, 24, 1255–1263. [Google Scholar] [CrossRef]
- Creech, S.; Guidi, J.; Elburn, D. Artemis: An overview of NASA’s activities to return humans to the Moon. In Proceedings of the 2022 IEEE Aerospace Conference, Big Sky, MT, USA, 5–12 March 2022; pp. 1–7. [Google Scholar]
- Gordon, G.D. Space Exploration: Mass Ratios for Different Missions. In Proceedings of the AIAA SPACE 2007 Conference & Exposition, Long Beach, CA, USA, 18–20 September 2007. [Google Scholar] [CrossRef]
- Kang, M.; Liu, Y.; Lin, W.; Liang, C.; Cheng, J. The thermal behavior and flame retardant performance of phase change material microcapsules with halloysite nanotube. J. Energy Storage 2023, 60, 106632. [Google Scholar] [CrossRef]
- Cheraghi, E.; Chen, S.; Yeow, J.T. Boron Nitride-Based Nanomaterials for Radiation Shielding: A Review. IEEE Nanotechnol. Mag. 2021, 15, 8–17. [Google Scholar] [CrossRef]
- Cha, J.H.; Jang, W.H.; Sarath Kumar, S.K.; Noh, J.E.; Choi, J.S.; Kim, C.G. Functionalized multi-walled carbon nanotubes/hydrogen-rich benzoxazine nanocomposites for cosmic radiation shielding with enhanced mechanical properties and space environment resistance. Compos. Sci. Technol. 2022, 228, 109634. [Google Scholar] [CrossRef]
- Liu, C.; Yin, H. Tailorable thermoelasticity of cubic lattice-based cellular and granular materials by prestress. Mater. Des. 2023, 233, 112223. [Google Scholar] [CrossRef]
- Ryan, E.; Seibers, Z.; Reynolds, J.; Shofner, M. Electrically conducting polymers and composites for applications in space exploration. J. Appl. Polym. Sci. 2024, 141, e55225. [Google Scholar] [CrossRef]
- Jang, S.H.; Kim, D.; Park, Y.L. Accelerated curing and enhanced material properties of conductive polymer nanocomposites by Joule heating. Materials 2018, 11, 1775. [Google Scholar] [CrossRef] [PubMed]
- Bond, D.; Goddard, B.; Singleterry, R.C., Jr.; y León, S.B. Evaluating the effectiveness of common aerospace materials at lowering the whole body effective dose equivalent in deep space. Acta Astronaut. 2019, 165, 68–95. [Google Scholar] [CrossRef]
- Mazumder, M.; Srirama, P.; Sharma, R.; Biris, A.; Hidetaka, I.; Trigwell, S.; Horenstein, M. Lunar and Martian dust dynamics. IEEE Ind. Appl. Mag. 2010, 16, 14–21. [Google Scholar] [CrossRef]
- Weiss, P.; Mohamed, M.; Gobert, T.; Chouard, Y.; Singh, N.; Chalal, T.; Schmied, S.; Schweins, M.; Stegmaier, T.; Gresser, G.; et al. Advanced materials for future lunar extravehicular activity space suit. Adv. Mater. Technol. 2020, 5, 2000028. [Google Scholar] [CrossRef]
- Jackson, T.; Farrell, W.; Zimmerman, M. Rover wheel charging on the lunar surface. Adv. Space Res. 2015, 55, 1710–1720. [Google Scholar] [CrossRef]
- Budzyń, D.; Zare-Behtash, H.; Cowley, A.; Cammarano, A. Implicit lunar dust mitigation technology: Compliant mechanisms. Acta Astronaut. 2023, 203, 146–156. [Google Scholar] [CrossRef]
- Gaier, J.; Meador, M.; Rogers, K.; Sheehy, B. Abrasion of Candidate Spacesuit Fabrics by Simulated Lunar Dust; Technical Report 2009-01-2473, SAE Technical Paper; NASA: Washington, DC, USA, 2009.
- Ryan, E.; Seibers, Z.; Reynolds, J.; Shofner, M. Surface-localized chemically modified reduced graphene oxide nanocomposites as flexible conductive surfaces for space applications. ACS Appl. Polym. Mater. 2023, 5, 5092–5102. [Google Scholar] [CrossRef]
- Chen, J.; Zhu, J.; Li, Q.; Wu, H.; Guo, S.; Qiu, J. Constructing 3D interconnected CNTs network in PA6 composites with well-dispersed UHMWPE for excellent tribological and heat dissipation properties. Compos. Part B 2022, 246, 110252. [Google Scholar] [CrossRef]
- Arif, M.; Alhashmi, H.; Varadarajan, K.; Koo, J.; Hart, A.; Kumar, S. Multifunctional performance of carbon nanotubes and graphene nanoplatelets reinforced PEEK composites enabled via FFF additive manufacturing. Compos. Part B 2020, 184, 107625. [Google Scholar] [CrossRef]
- Li, C.; Fei, J.; Zhang, T.; Zhao, S.; Qi, L. Relationship between surface characteristics and properties of fiber-reinforced resin-based composites. Compos. Part B Eng. 2023, 249, 110422. [Google Scholar] [CrossRef]
- Man, Z.; Wang, H.; He, Q.; Kim, D.E.; Chang, L. Friction and wear behaviour of additively manufactured continuous carbon fibre reinforced PA6 composites. Compos. Part B 2021, 226, 109332. [Google Scholar] [CrossRef]
- Jang, S.; Yin, H. Effect of aligned ferromagnetic particles on strain sensitivity of multi-walled carbon nanotube/polydimethylsiloxane sensors. Appl. Phys. Lett. 2015, 106, 141903. [Google Scholar] [CrossRef]
- Rebeque, P.V.; Silva, M.J.; Cena, C.R.; Nagashima, H.N.; Malmonge, J.A.; Kanda, D.H.F. Analysis of the electrical conduction in percolative nanocomposites based on castor-oil polyurethane with carbon black and activated carbon nanopowder. Polym. Compos. 2019, 40, 7–15. [Google Scholar] [CrossRef]
- Tregenza, O.; Saha, M.; Hutasoit, N.; Hulston, C.; Palanisamy, S. An experimental evaluation of the thermal interface resistance between cold spray copper/laser-textured alumina bi-layered composites. Int. J. Heat Mass Transf. 2022, 188, 122606. [Google Scholar] [CrossRef]
- Bossa, N.; Sipe, J.; Berger, W.; Scott, K.; Kennedy, A.; Thomas, T.; Hendren, C.; Wiesner, M. Quantifying mechanical abrasion of MWCNT nanocomposites used in 3D printing: Influence of CNT content on abrasion products and rate of microplastic production. Environ. Sci. Technol. 2021, 55, 10332. [Google Scholar] [CrossRef]
- Schlagenhauf, L.; Chu, B.; Buha, J.; Nuesch, F.; Wang, J. Release of carbon nanotubes from an epoxy-based nanocomposite during an abrasion process. Environ. Sci. Technol. 2012, 46, 7366. [Google Scholar] [CrossRef]
- Kohlmeyer, R.R.; Lor, M.; Deng, J.; Liu, H.; Chen, J. Preparation of stable carbon nanotube aerogels with high electrical conductivity and porosity. Carbon 2011, 49, 2352–2361. [Google Scholar] [CrossRef]
- Molla-Abbasi, P.; Ghaffarian, S.R.; Danesh, E. Porous carbon nanotube/PMMA conductive composites as a sensitive layer in vapor sensors. Smart Mater. Struct. 2011, 20, 105012. [Google Scholar] [CrossRef]
- Shklovskii, B.; Efros, A. Electronic Properties of Doped Semiconductors; Springer: New York, NY, USA, 1984. [Google Scholar]
- Kirkpatrick, S. Percolation and Conduction. Rev. Mod. Phys. 1973, 45, 574. [Google Scholar] [CrossRef]
- Stauffer, D.; Aharony, A. Introduction to Percolation Theory; CRC Press: Boca Raton, FL, USA, 1994. [Google Scholar]
- Kim, B.W.; Park, S.H.; Bandaru, P. Anomalous decrease of the specific heat capacity at the electrical and thermal conductivity percolation threshold in nanocomposites. Appl. Phys. Lett. 2014, 105, 253108. [Google Scholar] [CrossRef]
- Vionnet-Menot, S.; Grimaldi, C.; Maeder, T.; Strässler, S.; Ryser, P. Tunneling-percolation origin of nonuniversality: Theory and experiments. Phys. Rev. B 2005, 71, 064201. [Google Scholar] [CrossRef]
- Straley, J. Critical exponents for the conductivity of random resistor lattices. Phys. Rev. B 1977, 15, 5733. [Google Scholar] [CrossRef]
- Kim, B.W.; Park, S.H.; Kapadia, R.; Bandaru, P. Evidence of percolation related power law behavior in the thermal conductivity of nanotube/polymer composites. Appl. Phys. Lett. 2013, 102, 243105. [Google Scholar] [CrossRef]
- Yang, Y.; Grulke, E. Thermal and rheological properties of carbon nanotube-in-oil dispersion. J. Appl. Phys. 2006, 99, 114307. [Google Scholar] [CrossRef]
- Ruan, B.; Jacobi, A. Ultrasonication effects on thermal and rheological properties of carbon nanotubes suspension. Nanoscale Res. Lett. 2012, 7, 127. [Google Scholar] [CrossRef]
- Feng, C.; Jiang, L. Micromechanics modeling of the electrical conductivity of carbon nanotube (CNT)—Polymer nanocomposites. Compos. Part A Appl. Sci. Manuf. 2013, 47, 143–149. [Google Scholar] [CrossRef]
- Saberi, M.; Moradi, A.; Ansari, R.; Hassanzadeh-Aghdam, M.K.; Jamali, J. Developing an efficient analytical model for predicting the electrical conductivity of polymeric nanocomposites containing hybrid carbon nanotube/carbon black nanofillers. Compos. Part A Appl. Sci. Manuf. 2024, 185, 108374. [Google Scholar] [CrossRef]
- Li, C.; Thostenson, E.T.; Chou, T.W. Dominant role of tunneling resistance in the electrical conductivity of carbon nanotube—Based composites. Appl. Phys. Lett. 2007, 91, 223114. [Google Scholar] [CrossRef]
- Takeda, T.; Shindo, Y.; Kuronuma, Y.; Narita, F. Modeling and characterization of the electrical conductivity of carbon nanotube-based polymer composites. Polymer 2011, 52, 3852–3856. [Google Scholar] [CrossRef]
- Deng, F.; Zheng, Q.S. An analytical model of effective electrical conductivity of carbon nanotube composites. Appl. Phys. Lett. 2008, 92, 071902. [Google Scholar] [CrossRef]
- Chien, A.T.; Cho, S.; Joshi, Y.; Kumar, S. Electrical conductivity and Joule heating of polyacrylonitrile/carbon nanotube composite fibers. Polymer 2014, 55, 6896. [Google Scholar] [CrossRef]
- Krause, B.; Pötschke, P.; Ilin, E.; Predtechenskiy, M. Melt mixed SWCNT-polypropylene composites with very low electrical percolation. Polymer 2016, 98, 45–50. [Google Scholar] [CrossRef]
- Cheng, Y.; Zhang, H.; Wang, R.; Wang, X.; Zhai, H.; Wang, T.; Jin, Q.; Sun, J. Highly stretchable and conductive copper nanowire based fibers with hierarchical structure for wearable heaters. ACS Appl. Mater. Interfaces 2016, 8, 32925. [Google Scholar] [CrossRef]
- Vollmer, M. Newton’s law of cooling revisited. Eur. J. Phys. 2009, 30, 1063. [Google Scholar] [CrossRef]
- Yi, W.; Lu, L.; Zhang, D.L.; Pan, Z.; Xie, S. Linear specific heat of carbon nanotubes. Phys. Rev. B 1999, 59, R9015. [Google Scholar] [CrossRef]
- Lu, L.; Yi, W.; Zhang, D. 3ω method for specific heat and thermal conductivity measurements. Rev. Sci. Instrum. 2001, 72, 2996. [Google Scholar] [CrossRef]
- Masarapu, C.; Henry, H.; Wei, B. Specific heat of aligned multiwalled carbon nanotubes. Nanotechnology 2005, 16, 1490. [Google Scholar] [CrossRef]
- Kim, H.; Park, K.; Lee, D. A study on the epoxy resin concrete for the ultra-precision machine tool bed. J. Mater. Process. Technol. 1995, 48, 649. [Google Scholar] [CrossRef]
- Baller, J.; Becker, N.; Ziehmer, M.; Thomassey, M.; Zielinski, B.; Müller, U.; Sanctuary, R. Interaction between silica nanoparticles and an epoxy resin before and during network formation. Polymer 2009, 50, 3211. [Google Scholar] [CrossRef]
- Hubert, H. Aspect Flow and Compaction of Laminated Composite Shapes During Cure. Ph.D. Thesis, The University of British Columbia, Kelowna, BC, Canada, 1996. [Google Scholar]
- Li, C.; Medvedev, G.; Lee, E.W.; Kim, J.; Caruthers, J.; Strachan, A. Molecular dynamics simulations and experimental studies of the thermomechanical response of an epoxy thermoset polymer. Polymer 2012, 53, 4222. [Google Scholar] [CrossRef]
- Gong, S.; Zhu, Z.; Li, Z. Electron tunnelling and hopping effects on the temperature coefficient of resistance of carbon nanotube/polymer nanocomposites. Phys. Chem. Chem. Phys. 2017, 19, 5113. [Google Scholar] [CrossRef] [PubMed]
- Dai, H.; Thostenson, E.; Schumacher, T. Comparative study of the thermoresistive behavior of carbon nanotube-based nanocomposites and multiscale hybrid composites. Compos. Part B 2021, 222, 1090068. [Google Scholar] [CrossRef]
- Conti, R.; Gallitto, A.A.; Fiordilino, E. Measurement of the convective heat-transfer coefficient. Phys. Teach. 2014, 52, 109. [Google Scholar] [CrossRef]
- Bisht, A.; Dasgupta, K.; Debrupa, L. Effect of graphene and CNT reinforcement on mechanical and thermomechanical. J. Appl. Polym. Sci. 2018, 135, 46101. [Google Scholar] [CrossRef]
- Jang, S.; Li, L. Self-sensing carbon nanotube composites exposed to glass transition temperature. Materials 2020, 13, 259. [Google Scholar] [CrossRef]
- Jacobs, O.; Xu, W.; Schädel, B.; Wu, W. Wear behaviour of carbon nanotube reinforced epoxy resin composites. Tribol. Lett. 2006, 23, 65. [Google Scholar] [CrossRef]
- Tang, P.; Zhang, R.; Shi, R.; Bin, Y. Synergetic effects of carbon nanotubes and carbon fibers on electrical and self-heating properties of high-density polyethylene composites. J. Mater. Sci. 2015, 50, 1565–1574. [Google Scholar] [CrossRef]
- Sánchez-Romate, X.; García, A.; Sánchez, M.; Ureña, A. Electrical Transport Mechanisms in Graphene Nanoplatelet Doped Polydimethylsiloxane and Application to Ultrasensitive Temperature Sensors. ACS Appl. Mater. Interfaces 2023, 15, 22377–22394. [Google Scholar] [CrossRef]
- Luo, S.; Liu, T. SWCNT/Graphite Nanoplatelet Hybrid Thin Films for Self-Temperature-Compensated, Highly Sensitive, and Extensible Piezoresistive Sensors. Adv. Mater. 2013, 25, 5650–5657. [Google Scholar] [CrossRef]
- Parkhomenko, I.; Vlasukova, L.; Parfimovich, I.; Komarov, F.; Novikov, L.; Chernik, V.; Zhigulin, D. Atomic oxygen exposure effect on carbon nanotubes/epoxy composites for space systems. Acta Astronaut. 2023, 204, 124–131. [Google Scholar] [CrossRef]


| Space | Low Temp (K) | Atmosphere | Environment | Application | Required Properties |
|---|---|---|---|---|---|
| Earth Orbit | 153 | At. O, N | Micrometeroid, Electrostatic charge | G4C orbital EVA suit for Gemini, Orbital EVA suit for shuttle/ISS EMU | Micrometeroid and thermal protective |
| Moon | 25 | Ar, Ne, , He | Lunar dust, Electrostatic charge | A7L EVA suit for Apollo | Micrometeroid, abrasion, and thermal protective, Nonflammable |
| Mars | 133 | , , Ar, , O | Martian dust, Electrostatic charge | - | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, B.-W.; Lee, S.-J.; Jang, S.-H.; Yin, H. Abrasion Effect on Heating Performance of Carbon Nanotube/Epoxy Composites. Nanomaterials 2025, 15, 337. https://doi.org/10.3390/nano15050337
Kim B-W, Lee S-J, Jang S-H, Yin H. Abrasion Effect on Heating Performance of Carbon Nanotube/Epoxy Composites. Nanomaterials. 2025; 15(5):337. https://doi.org/10.3390/nano15050337
Chicago/Turabian StyleKim, Byung-Wook, Seung-Jun Lee, Sung-Hwan Jang, and Huiming Yin. 2025. "Abrasion Effect on Heating Performance of Carbon Nanotube/Epoxy Composites" Nanomaterials 15, no. 5: 337. https://doi.org/10.3390/nano15050337
APA StyleKim, B.-W., Lee, S.-J., Jang, S.-H., & Yin, H. (2025). Abrasion Effect on Heating Performance of Carbon Nanotube/Epoxy Composites. Nanomaterials, 15(5), 337. https://doi.org/10.3390/nano15050337








