Numerical Study on the Buckling Behavior of FG Porous Spherical Caps Reinforced by Graphene Platelets
Abstract
1. Introduction
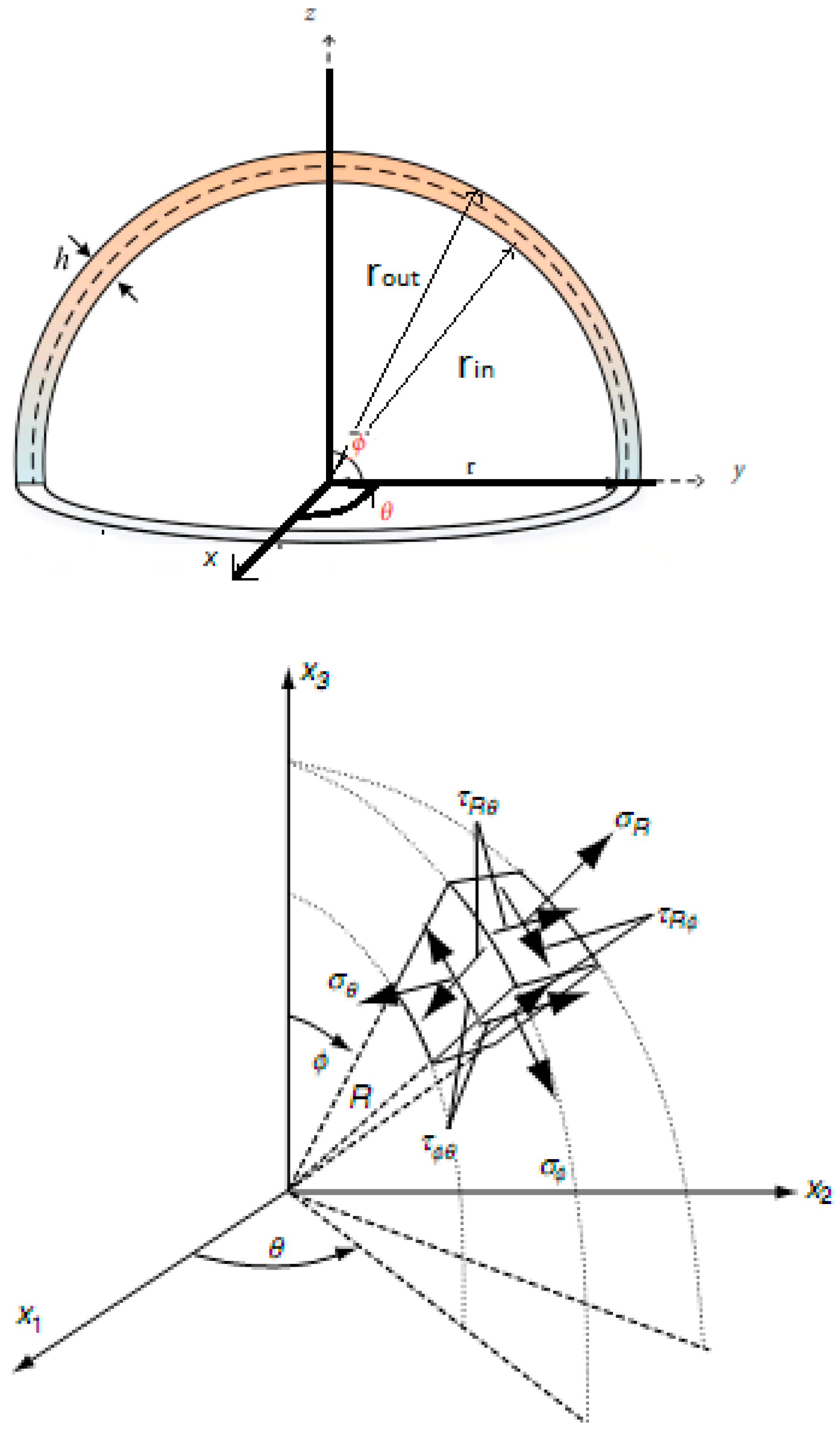
2. Theoretical Problem
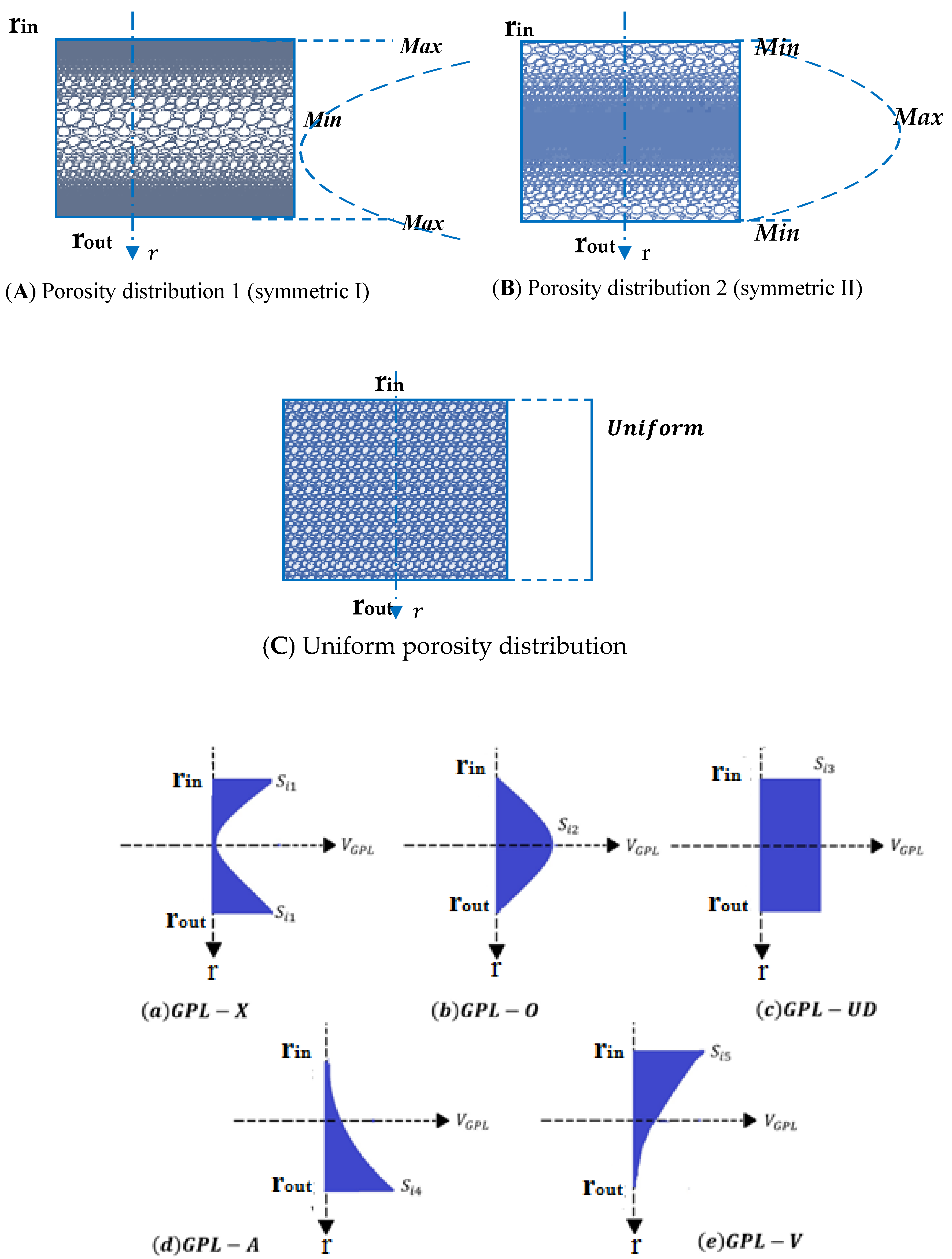
2.1. Description of Geometry and Mechanical Properties
- -
- Porosity distribution 1
- -
- Porosity distribution 2
- -
- Uniform porosity distribution
2.2. Governing Equations of the Problem
3. Finite Element Modeling
- -
- For a spherical cap with θ = 180°, ϕ = 180°, u, v, w (r, θ and ϕ = 0, 180°), = 1 at r = b.
- -
- For a spherical cap with θ = 180°, ϕ = 90°, u, v, w (r, θ and ϕ = 0, 90°), = 1 at r = b.
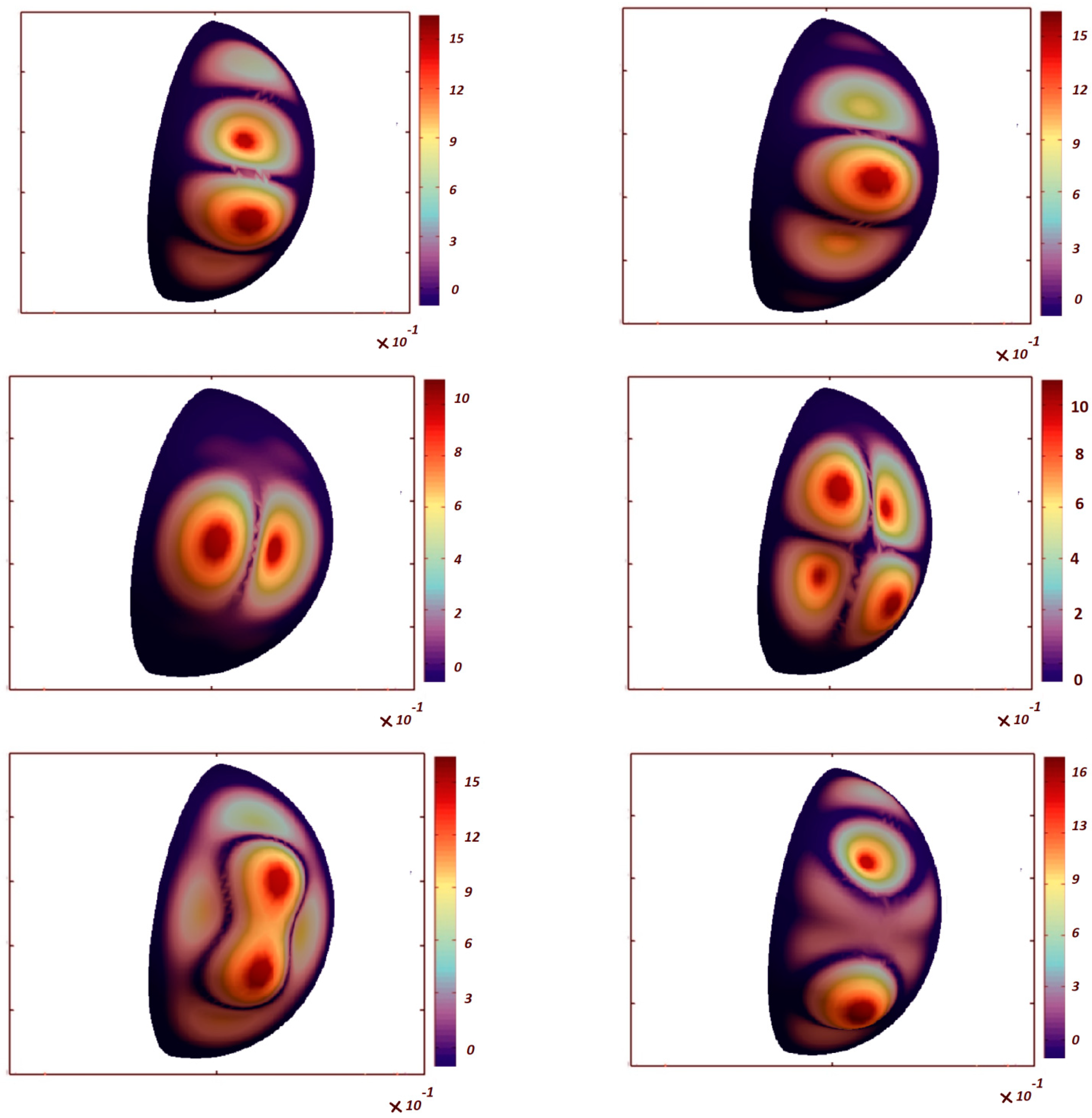
4. Numerical Results and Discussion
4.1. Validation
4.2. Parametric Analysis of the Buckling Load
5. Concluding Remarks
- (a)
- The maximum and minimum buckling loads seem to be reached for GPL-X and GPL-O distributions, respectively.
- (b)
- The maximum and minimum buckling loads belong to the PD1 and PD2 cases, respectively.
- (c)
- The difference between the maximum and minimum critical buckling loads for different porosity distributions is approximately equal to 90%, and the buckling loads of the selected structure increase considerably (approximately of 100%) with an increase in the weight fraction of GPLs.
- (d)
- The effect of the porosity coefficient on the critical buckling load for porous spherical cap shells made of FG-GPLs is lower than the weight fraction of the nanofillers, being approximately equal to 12.5%.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Molina, J.-M.; Rodríguez-Guerrero, A.; Louis, E.; Rodríguez-Reinoso, F.; Narciso, J. Porosity Effect on Thermal Properties of Al-12 wt % Si/Graphite Composites. Materials 2017, 10, 177. [Google Scholar] [CrossRef]
- Kiarasi, F.; Babaei, M.; Asemi, K.; Dimitri, R.; Tornabene, F. Free Vibration Analysis of Thick Annular Functionally Graded Plate Integrated with Piezo-Magneto-Electro-Elastic Layers in a Hygrothermal Environment. Appl. Sci. 2022, 12, 10682. [Google Scholar] [CrossRef]
- Narciso, J.; Molina, J.; Rodríguez, A.; Rodríguez-Reinoso, F.; Louis, E. Effects of infiltration pressure on mechanical properties of Al–12Si/graphite composites for piston engines. Compos. Part B Eng. 2016, 91, 441–447. [Google Scholar] [CrossRef]
- Prieto, R.; Molina, J.; Narciso, J.; Louis, E. Thermal conductivity of graphite flakes–SiC particles/metal composites. Compos. Part A Appl. Sci. Manuf. 2011, 42, 1970–1977. [Google Scholar] [CrossRef]
- Zhang, L.; Lai, S.; Wang, C.; Yang, J. DSC regularized Dirac-delta method for dynamic analysis of FG graphene platelet-reinforced porous beams on elastic foundation under a moving load. Compos. Struct. 2020, 255, 112865. [Google Scholar] [CrossRef]
- Priyanka, R.; Twinkle, C.M.; Pitchaimani, J. Stability and dynamic behavior of porous FGM beam: Influence of graded porosity, graphene platelets, and axially varying loads. Eng. Comput. 2021, 38, 4347–4366. [Google Scholar] [CrossRef]
- Binh, C.T.; Quoc, T.H.; Huan, D.T.; Hien, H.T. Vibration characteristics of rotating functionally graded porous beams reinforced by graphene platelets. J. Sci. Technol. Civ. Eng. (STCE) 2021, 15, 29–41. [Google Scholar] [CrossRef]
- Xu, H.; Wang, Y.Q.; Zhang, Y. Free vibration of functionally graded graphene platelet-reinforced porous beams with spinning movement via differential transformation method. Arch. Appl. Mech. 2021, 91, 4817–4834. [Google Scholar] [CrossRef]
- Ganapathi, M.; Anirudh, B.; Anant, C.; Polit, O. Dynamic characteristics of functionally graded graphene reinforced porous nanocomposite curved beams based on trigonometric shear deformation theory with thickness stretch effect. Mech. Adv. Mater. Struct. 2019, 28, 741–752. [Google Scholar] [CrossRef]
- Yas, M.H.; Rahimi, S. Thermal vibration of functionally graded porous nanocomposite beams reinforced by graphene platelets. Appl. Math. Mech. 2020, 41, 1209–1226. [Google Scholar] [CrossRef]
- Safarpour, M.; Rahimi, A.; Alibeigloo, A.; Bisheh, H.; Forooghi, A. Parametric study of three-dimensional bending and frequency of FG-GPLRC porous circular and annular plates on different boundary conditions. Mech. Based Des. Struct. Mach. 2019, 49, 707–737. [Google Scholar] [CrossRef]
- Nguyen, N.V.; Nguyen-Xuan, H.; Lee, D.; Lee, J. A novel computational approach to functionally graded porous plates with graphene platelets reinforcement. Thin-Walled Struct. 2020, 150, 106684. [Google Scholar] [CrossRef]
- Gao, K.; Gao, W.; Chen, D.; Yang, J. Nonlinear free vibration of functionally graded graphene platelets reinforced porous nanocomposite plates resting on elastic foundation. Compos. Struct. 2018, 204, 831–846. [Google Scholar] [CrossRef]
- Saidi, A.R.; Bahaadini, R.; Majidi-Mozafari, K. On vibration and stability analysis of porous plates reinforced by graphene platelets under aerodynamical loading. Compos. Part B Eng. 2019, 164, 778–799. [Google Scholar] [CrossRef]
- Asemi, K.; Babaei, M.; Kiarasi, F. Static, natural frequency and dynamic analyses of functionally graded porous annular sector plates reinforced by graphene platelets. Mech. Based Des. Struct. Mach. 2020, 50, 3853–3881. [Google Scholar] [CrossRef]
- Phan, D.H. Isogeometric Analysis of Functionally-Graded Graphene Platelets Reinforced Porous Nanocomposite Plates Using a Refined Plate Theory. Int. J. Struct. Stab. Dyn. 2020, 2020, 2050076. [Google Scholar] [CrossRef]
- Gao, W.; Qin, Z.; Chu, F. Wave propagation in functionally graded porous plates reinforced with graphene platelets. Aerosp. Sci. Technol. 2020, 102, 105860. [Google Scholar] [CrossRef]
- Zhou, C.; Zhang, Z.; Zhang, J.; Fang, Y.; Tahouneh, V. Vibration analysis of FG porous rectangular plates reinforced by graphene platelets. Steel Compos. Struct. 2020, 34, 215–226. [Google Scholar]
- Teng, M.W.; Wang, Y.Q. Nonlinear forced vibration of simply supported functionally graded porous nanocomposite thin plates reinforced with graphene platelets. Thin-Walled Struct. 2021, 164, 107799. [Google Scholar] [CrossRef]
- Kiarasi, F.; Babaei, M.; Sarvi, P.; Asemi, K.; Hosseini, M.; Omidi Bidgoli, M. A review on functionally graded porous structures reinforced by graphene platelets. J. Comput. Appl. Mech. 2021, 52, 731–750. [Google Scholar]
- Ton-That, H.L.; Nguyen-Van, H.; Chau-Dinh, T. A novel quadrilateral element for analysis of functionally graded porous plates/shells reinforced by graphene platelets. Arch. Appl. Mech. 2021, 91, 2435–2466. [Google Scholar] [CrossRef]
- Ansari, R.; Hassani, R.; Gholami, R.; Rouhi, H. Free vibration analysis of postbuckled arbitrary-shaped FG-GPL-reinforced porous nanocomposite plates. Thin-Walled Struct. 2021, 163, 107701. [Google Scholar] [CrossRef]
- Safarpour, M.; Rahimi, A.R.; Alibeigloo, A. Static and free vibration analysis of graphene platelets reinforced composite truncated conical shell, cylindrical shell, and annular plate using theory of elasticity and DQM. Mech. Based Des. Struct. Mach. 2019, 48, 496–524. [Google Scholar] [CrossRef]
- Bahaadini, R.; Saidi, A.R.; Arabjamaloei, Z.; Ghanbari-Nejad-Parizi, A. Vibration Analysis of Functionally Graded Graphene Reinforced Porous Nanocomposite Shells. Int. J. Appl. Mech. 2019, 11, 1950068. [Google Scholar] [CrossRef]
- Kiarasi, F.; Babaei, M.; Mollaei, S.; Mohammadi, M.; Asemi, K. Free vibration analysis of FG porous joined truncated conical-cylindrical shell reinforced by graphene platelets. Adv. Nanores. 2021, 11, 361–380. [Google Scholar]
- Babaei, M.; Kiarasi, F.; Marashi, S.M.H.; Ebadati, M.; Masoumi, F.; Asemi, K. Stress wave propagation and natural frequency analysis of functionally graded graphene platelet-reinforced porous joined conical–cylindrical–conical shell. Waves Random Complex Media 2021, 1–33. [Google Scholar] [CrossRef]
- Ye, C.; Wang, Y.Q. Nonlinear forced vibration of functionally graded graphene platelet-reinforced metal foam cylindrical shells: Internal resonances. Nonlinear Dyn. 2021, 104, 2051–2069. [Google Scholar] [CrossRef]
- Wang, Y.Q.; Ye, C.; Zu, J.W. Nonlinear vibration of metal foam cylindrical shells reinforced with graphene platelets. Aerosp. Sci. Technol. 2018, 85, 359–370. [Google Scholar] [CrossRef]
- Moradi-Dastjerdi, R.; Behdinan, K. Stress waves in thick porous graphene-reinforced cylinders under thermal gradient environments. Aerosp. Sci. Technol. 2021, 110, 106476. [Google Scholar] [CrossRef]
- Salehi, M.; Gholami, R.; Ansari, R. Analytical solution approach for nonlinear vibration of shear deformable imperfect FG-GPLR porous nanocomposite cylindrical shells. Mech. Based Des. Struct. Mach. 2021, 1–23. [Google Scholar] [CrossRef]
- Nejadi, M.; Mohammadimehr, M.; Mehrabi, M. Free vibration and stability analysis of sandwich pipe by considering porosity and graphene platelet effects on conveying fluid flow. Alex. Eng. J. 2020, 60, 1945–1954. [Google Scholar] [CrossRef]
- Zhou, X.; Wang, Y.; Zhang, W. Vibration and flutter characteristics of GPL-reinforced functionally graded porous cylindrical panels subjected to supersonic flow. Acta Astronaut. 2021, 183, 89–100. [Google Scholar] [CrossRef]
- Ebrahimi, F.; Seyfi, A.; Dabbagh, A.; Tornabene, F. Wave dispersion characteristics of porous graphene platelet-reinforced composite shells. Struct. Eng. Mech. 2019, 71, 99–107. [Google Scholar]
- Pourjabari, A.; Hajilak, Z.E.; Mohammadi, A.; Habibi, M.; Safarpour, H. Effect of Porosity on free and forced vibration characteristics of the GPL reinforcement composite nanostructures. Comput. Math. Appl. 2019, 77, 2608–2626. [Google Scholar] [CrossRef]
- Zhou, Z.; Ni, Y.; Tong, Z.; Zhu, S.; Sun, J.; Xu, X. Accurate nonlinear buckling analysis of functionally graded porous graphene platelet reinforced composite cylindrical shells. Int. J. Mech. Sci. 2018, 151, 537–550. [Google Scholar] [CrossRef]
- Shahgholian-Ghahfarokhi, D.; Rahimi, G.; Khodadadi, A.; Salehipour, H.; Afrand, M. Buckling analyses of FG porous nanocomposite cylindrical shells with graphene platelet reinforcement subjected to uniform external lateral pressure. Mech. Based Des. Struct. Mach. 2020, 49, 1059–1079. [Google Scholar] [CrossRef]
- Shahgholian-Ghahfarokhi, D.; Safarpour, M.; Rahimi, A. Torsional buckling analyses of functionally graded porous nanocomposite cylindrical shells reinforced with graphene platelets (GPLs). Mech. Based Des. Struct. Mach. 2019, 49, 81–102. [Google Scholar] [CrossRef]
- Yang, J.; Chen, D.; Kitipornchai, S. Buckling and free vibration analyses of functionally graded graphene reinforced porous nanocomposite plates based on Chebyshev-Ritz method. Compos. Struct. 2018, 193, 281–294. [Google Scholar] [CrossRef]
- Dong, Y.; He, L.; Wang, L.; Li, Y.; Yang, J. Buckling of spinning functionally graded graphene reinforced porous nanocomposite cylindrical shells: An analytical study. Aerosp. Sci. Technol. 2018, 82–83, 466–478. [Google Scholar] [CrossRef]
- Ansari, R.; Hassani, R.; Gholami, R.; Rouhi, H. Nonlinear bending analysis of arbitrary-shaped porous nanocomposite plates using a novel numerical approach. Int. J. Nonlinear Mech. 2020, 126, 103556. [Google Scholar] [CrossRef]
- Kitipornchai, S.; Chen, D.; Yang, J. Free vibration and elastic buckling of functionally graded porous beams reinforced by graphene platelets. Mater. Des. 2017, 116, 656–665. [Google Scholar] [CrossRef]
- Twinkle, C.M.; Pitchaimani, J. Free vibration and stability of graphene platelet reinforced porous nano-composite cylindrical panel: Influence of grading, porosity and non-uniform edge loads. Eng. Struct. 2021, 230, 111670. [Google Scholar]
- Nguyen, Q.H.; Nguyen, L.B.; Nguyen, H.B.; Nguyen-Xuan, H. A three-variable high order shear deformation theory for isogeometric free vibration, buckling and instability analysis of FG porous plates reinforced by graphene platelets. Compos. Struct. 2020, 245, 112321. [Google Scholar] [CrossRef]
- Anamagh, M.R.; Bediz, B. Free vibration and buckling behavior of functionally graded porous plates reinforced by graphene platelets using spectral Chebyshev approach. Compos. Struct. 2020, 253, 112765. [Google Scholar] [CrossRef]
- Barbaros, I.; Yang, Y.; Safaei, B.; Yang, Z.; Qin, Z.; Asmael, M. State-of-the-art review of fabrication, application, and mechanical properties of functionally graded porous nanocomposite materials. Nanotechnol. Rev. 2022, 11, 321–371. [Google Scholar] [CrossRef]
- Budiansky, B.; Roth, R.S. Axisymmetric Dynamic Buckling of Clamped Shallow Spherical Shells; NASA TND-1510; NASA: Washington, DC, USA, 1962; pp. 597–609.
- Simitses, G.J. Axisymmetric dynamic snap-through buckling of shallow spherical caps. AIAA J. 1967, 5, 1019–1021. [Google Scholar] [CrossRef]
- Kao, R.; Perrone, N. Dynamic buckling of axisymmetic spherical caps with initial imperfection. Comput. Struct. 1978, 9, 463–473. [Google Scholar] [CrossRef]
- Kao, R. Nonlinear dynamic buckling of spherical caps with initial imperfections. Comput. Struct. 1980, 12, 49–63. [Google Scholar] [CrossRef]
- Chao, C.; Lin, I. Static and dynamic snap-through of orthotropic spherical caps. Compos. Struct. 1990, 14, 281–301. [Google Scholar] [CrossRef]
- Alwar, R.; Reddy, B.S. Dynamic buckling of isotropic and orthotropic shallow spherical cap with circular hole. Int. J. Mech. Sci. 1979, 21, 681–688. [Google Scholar] [CrossRef]
- Haboussi, M.; Sankar, A.; Ganapathi, M. Nonlinear axisymmetric dynamic buckling of functionally graded graphene reinforced porous nanocomposite spherical caps. Mech. Adv. Mater. Struct. 2019, 28, 127–140. [Google Scholar] [CrossRef]
- Penna, R.; Feo, L.; Lovisi, G.; Fabbrocino, F. Application of the Higher-Order Hamilton Approach to the Nonlinear Free Vibrations Analysis of Porous FG Nano-Beams in a Hygrothermal Environment Based on a Local/Nonlocal Stress Gradient Model of Elasticity. Nanomaterials 2022, 12, 2098. [Google Scholar] [CrossRef] [PubMed]
- Penna, R.; Feo, L.; Lovisi, G.; Fabbrocino, F. Hygro-Thermal Vibrations of Porous FG Nano-Beams Based on Local/Nonlocal Stress Gradient Theory of Elasticity. Nanomaterials 2021, 11, 910. [Google Scholar] [CrossRef]
- Liu, Y.F.; Wang, Y.Q. Thermo-Electro-Mechanical Vibrations of Porous Functionally Graded Piezoelectric Nanoshells. Nanomaterials 2019, 9, 301. [Google Scholar] [CrossRef] [PubMed]
- Karami, B.; Shahsavari, D.; Janghorban, M.; Dimitri, R.; Tornabene, F. Wave Propagation of Porous Nanoshells. Nanomaterials 2018, 9, 22. [Google Scholar] [CrossRef]
- Kiarasi, F.; Babaei, M.; Asemi, K.; Dimitri, R.; Tornabene, F. Three-Dimensional Buckling Analysis of Functionally Graded Saturated Porous Rectangular Plates under Combined Loading Conditions. Appl. Sci. 2021, 11, 10434. [Google Scholar] [CrossRef]
- Babaei, M.; Asemi, K.; Kiarasi, F. Dynamic analysis of functionally graded rotating thick truncated cone made of saturated porous materials. Thin-Walled Struct. 2021, 164, 107852. [Google Scholar] [CrossRef]
- Arshid, E.; Khorshidvand, A.R. Free vibration analysis of saturated porous FG circular plates integrated with piezoelectric actuators via differential quadrature method. Thin-Walled Struct. 2018, 125, 220–233. [Google Scholar] [CrossRef]
- Babaei, M.; Kiarasi, F.; Tehrani, M.S.; Hamzei, A.; Mohtarami, E.; Asemi, K. Three-dimensional free vibration analysis of functionally graded graphene reinforced composite laminated cylindrical panel. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2022, 236, 1501–1514. [Google Scholar] [CrossRef]
- Liu, D. Free Vibration of Functionally Graded Graphene Platelets Reinforced Magnetic Nanocomposite Beams Resting on Elastic Foundation. Nanomaterials 2020, 10, 2193. [Google Scholar] [CrossRef]
- Wang, Y.; Xie, K.; Fu, T.; Shi, C. Bending and Elastic Vibration of a Novel Functionally Graded Polymer Nanocomposite Beam Reinforced by Graphene Nanoplatelets. Nanomaterials 2019, 9, 1690. [Google Scholar] [CrossRef] [PubMed]
- Babaei, M.; Kiarasi, F.; Asemi, K.; Dimitri, R.; Tornabene, F. Transient Thermal Stresses in FG Porous Rotating Truncated Cones Reinforced by Graphene Platelets. Appl. Sci. 2022, 12, 3932. [Google Scholar] [CrossRef]


| 0.1 | 0.1738 | 0.9361 |
| 0.2 | 0.3442 | 0.8716 |
| 0.3 | 0.5103 | 0.8064 |
| 0.4 | 0.6708 | 0.7404 |
| 0.5 | 0.8231 | 0.6733 |
| 0.6 | 0.9612 | 0.6047 |
| Polar Angle | |||||||
|---|---|---|---|---|---|---|---|
| (Ansys Workbench) | 2.890 | 2.901 | 2.990 | 3.001 | 3.012 | 3.078 | |
| 180° | (Present) | 2.908 | 2.924 | 3.008 | 3.021 | 3.033 | 3.132 |
| (Ansys Workbench) | 1.861 | 1.867 | 2.110 | 2.101 | 2.159 | 2.299 | |
| 90° | (Present) | 1.873 | 1.875 | 2.180 | 2.211 | 2.222 | 2.320 |
| GPL Pattern | λ1 | λ2 | λ3 | λ4 | λ5 | λ6 | |
|---|---|---|---|---|---|---|---|
| 90° | 3.174 | 3.174 | 3.670 | 3.775 | 3.778 | 3.939 | |
| GPL-X | 180° | 4.450 | 4.462 | 4.572 | 4.600 | 4.617 | 4.798 |
| 90° | 1.898 | 1.901 | 2.227 | 2.254 | 2.264 | 2.354 | |
| GPL-A | 180° | 2.914 | 2.925 | 2.997 | 3.009 | 3.018 | 3.109 |
| 90° | 1.885 | 1.888 | 2.212 | 2.238 | 2.248 | 2.336 | |
| GPL-V | 180° | 2.906 | 2.917 | 2.988 | 3.001 | 3.009 | 3.098 |
| 90° | 1.653 | 1.657 | 1.949 | 1.950 | 1.958 | 2.018 | |
| GPL-O | 180° | 2.731 | 2.747 | 2.801 | 2.821 | 2.824 | 2.894 |
| 90° | 1.903 | 1.906 | 2.233 | 2.259 | 2.270 | 2.359 | |
| GPL-UD | 180° | 2.926 | 2.937 | 3.009 | 3.022 | 3.030 | 3.121 |
| Porosity Distribution | λ1 | λ2 | λ3 | λ4 | λ5 | λ6 | |
|---|---|---|---|---|---|---|---|
| 90° | 2.842 | 2.842 | 3.268 | 3.346 | 3.359 | 3.473 | |
| PD1 | 180° | 3.964 | 3.974 | 4.076 | 4.092 | 4.111 | 4.259 |
| 90° | 1.581 | 1.584 | 1.862 | 1.863 | 1.870 | 1.926 | |
| PD2 | 180° | 2.613 | 2.632 | 2.684 | 2.703 | 2.707 | 2.774 |
| 90° | 2.249 | 2.249 | 2.607 | 2.677 | 2.683 | 2.799 | |
| PD3 | 180° | 3.191 | 3.199 | 3.280 | 3.297 | 3.310 | 3.434 |
| Weight Fraction of Nano-Fillers (%wt) | λ1 | λ2 | λ3 | λ4 | λ5 | λ6 | |
|---|---|---|---|---|---|---|---|
| 90° | 1.458 | 1.459 | 1.696 | 1.737 | 1.744 | 1.819 | |
| 0% | 180° | 2.102 | 2.107 | 2.162 | 2.171 | 2.180 | 2.258 |
| 90° | 2.191 | 2.191 | 2.528 | 2.601 | 2.602 | 2.710 | |
| 0.5% | 180° | 3.048 | 3.056 | 3.130 | 3.151 | 3.163 | 3.290 |
| 90° | 2.744 | 2.746 | 3.187 | 3.261 | 3.268 | 3.403 | |
| 1% | 180° | 3.927 | 3.940 | 4.032 | 4.062 | 4.076 | 4.246 |
| λ1 | λ2 | λ3 | λ4 | λ5 | λ6 | ||
|---|---|---|---|---|---|---|---|
| 90° | 3.262 | 3.262 | 3.780 | 3.881 | 3.889 | 4.055 | |
| 0.2 | 180° | 4.626 | 4.635 | 4.752 | 4.777 | 4.794 | 4.975 |
| 90° | 2.842 | 2.842 | 3.268 | 3.346 | 3.359 | 3.473 | |
| 0.4 | 180° | 3.964 | 3.974 | 4.076 | 4.092 | 4.111 | 4.259 |
| 90° | 2.744 | 2.746 | 3.187 | 3.261 | 3.268 | 3.403 | |
| 0.5 | 180° | 3.927 | 3.940 | 4.032 | 4.062 | 4.076 | 4.246 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Z.; Wang, Y.; Zhang, S.; Dimitri, R.; Tornabene, F.; Asemi, K. Numerical Study on the Buckling Behavior of FG Porous Spherical Caps Reinforced by Graphene Platelets. Nanomaterials 2023, 13, 1205. https://doi.org/10.3390/nano13071205
Zhou Z, Wang Y, Zhang S, Dimitri R, Tornabene F, Asemi K. Numerical Study on the Buckling Behavior of FG Porous Spherical Caps Reinforced by Graphene Platelets. Nanomaterials. 2023; 13(7):1205. https://doi.org/10.3390/nano13071205
Chicago/Turabian StyleZhou, Zhimin, Yun Wang, Suying Zhang, Rossana Dimitri, Francesco Tornabene, and Kamran Asemi. 2023. "Numerical Study on the Buckling Behavior of FG Porous Spherical Caps Reinforced by Graphene Platelets" Nanomaterials 13, no. 7: 1205. https://doi.org/10.3390/nano13071205
APA StyleZhou, Z., Wang, Y., Zhang, S., Dimitri, R., Tornabene, F., & Asemi, K. (2023). Numerical Study on the Buckling Behavior of FG Porous Spherical Caps Reinforced by Graphene Platelets. Nanomaterials, 13(7), 1205. https://doi.org/10.3390/nano13071205








