Uniaxial Strain Dependence on Angle-Resolved Optical Second Harmonic Generation from a Few Layers of Indium Selenide
Abstract
1. Introduction
2. Methods
2.1. Growth of InSe Single Crystals and Their Characterizations
2.2. Sample Preparation and Strain Engineering
2.3. SHG Measurements
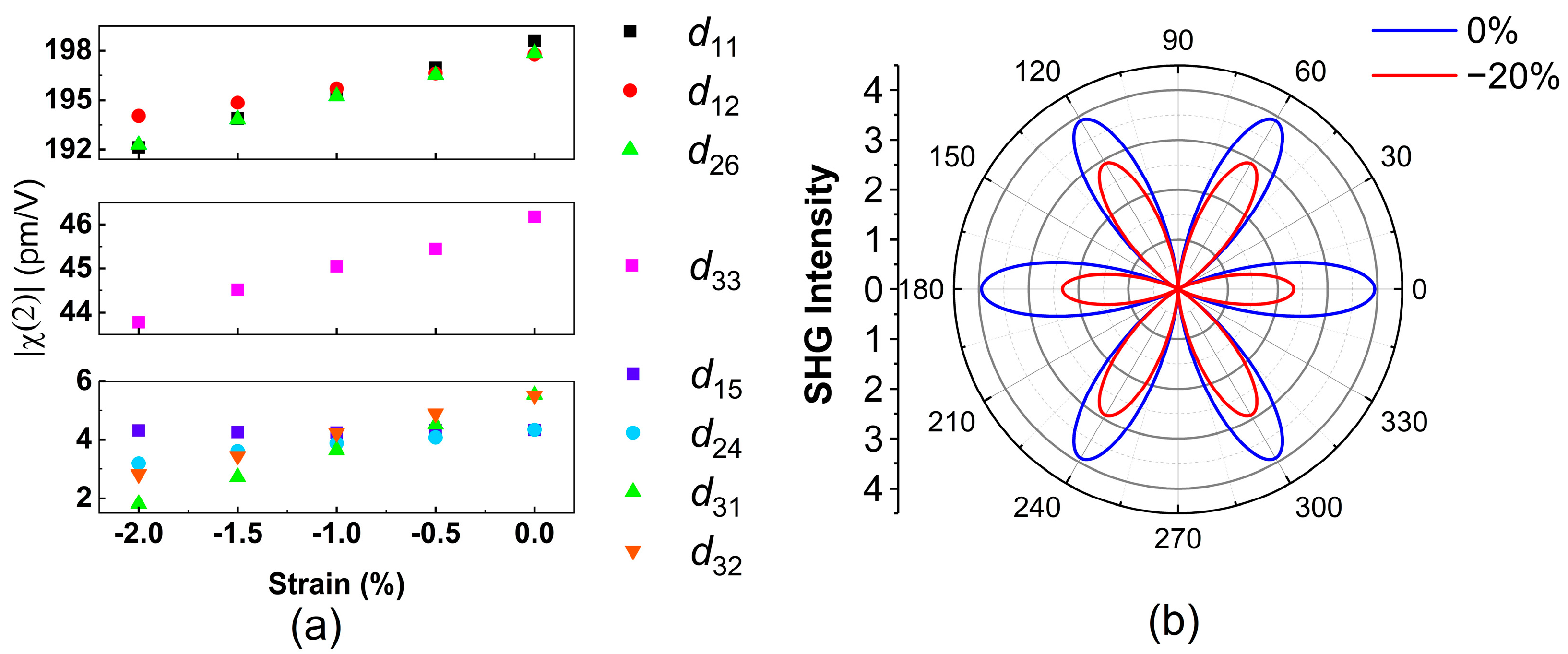
2.4. Theoretical Calculations
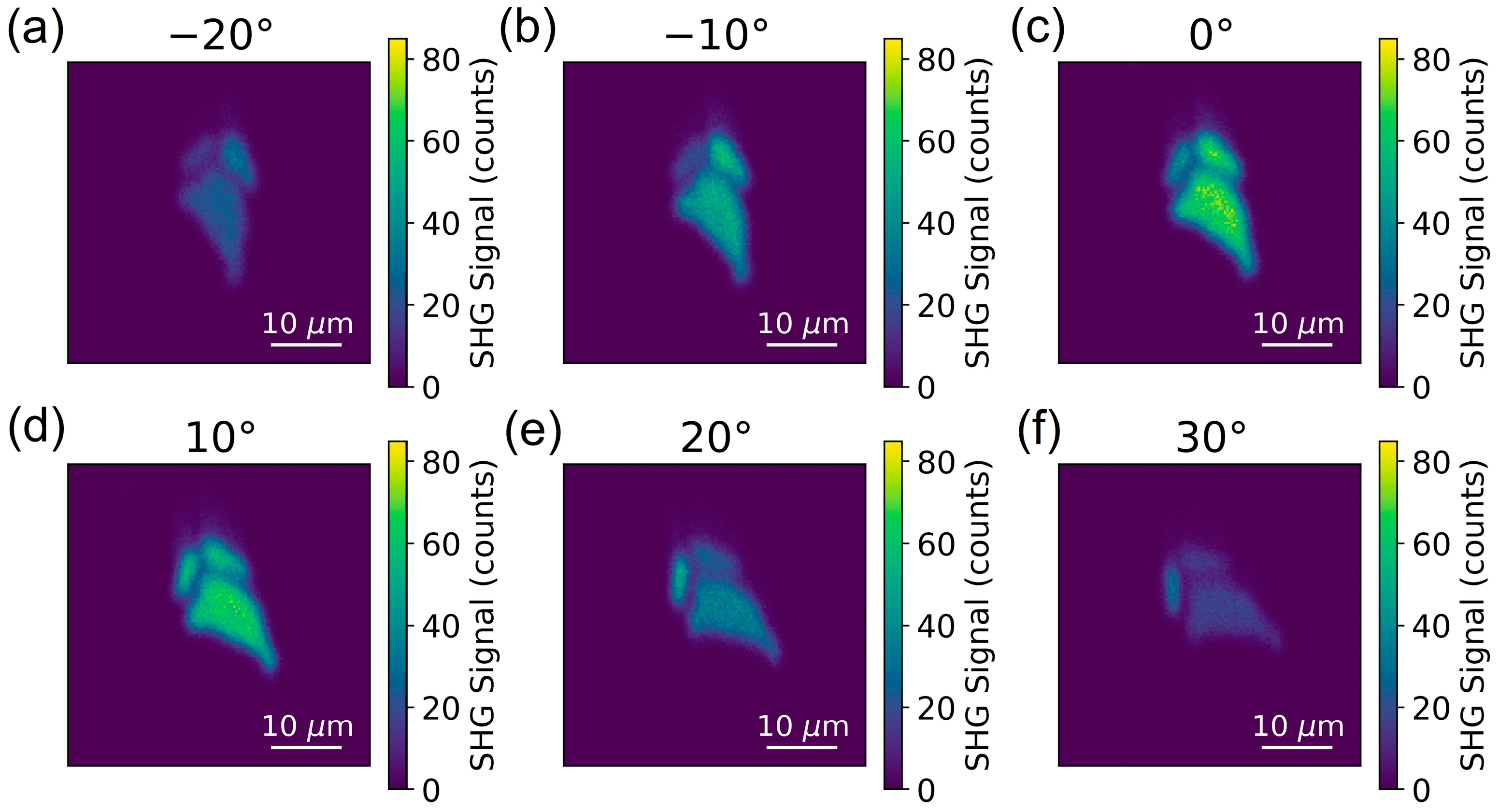
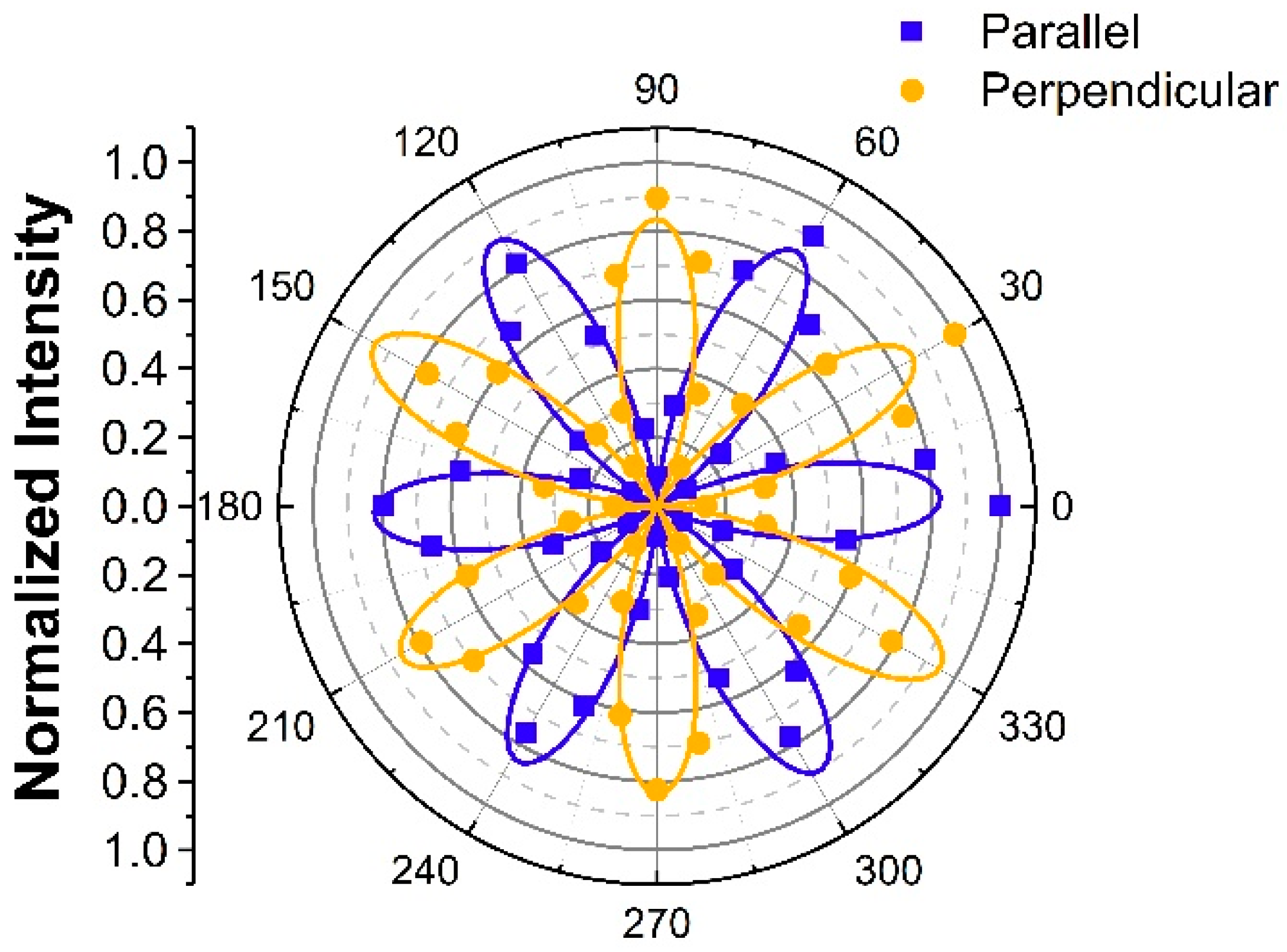
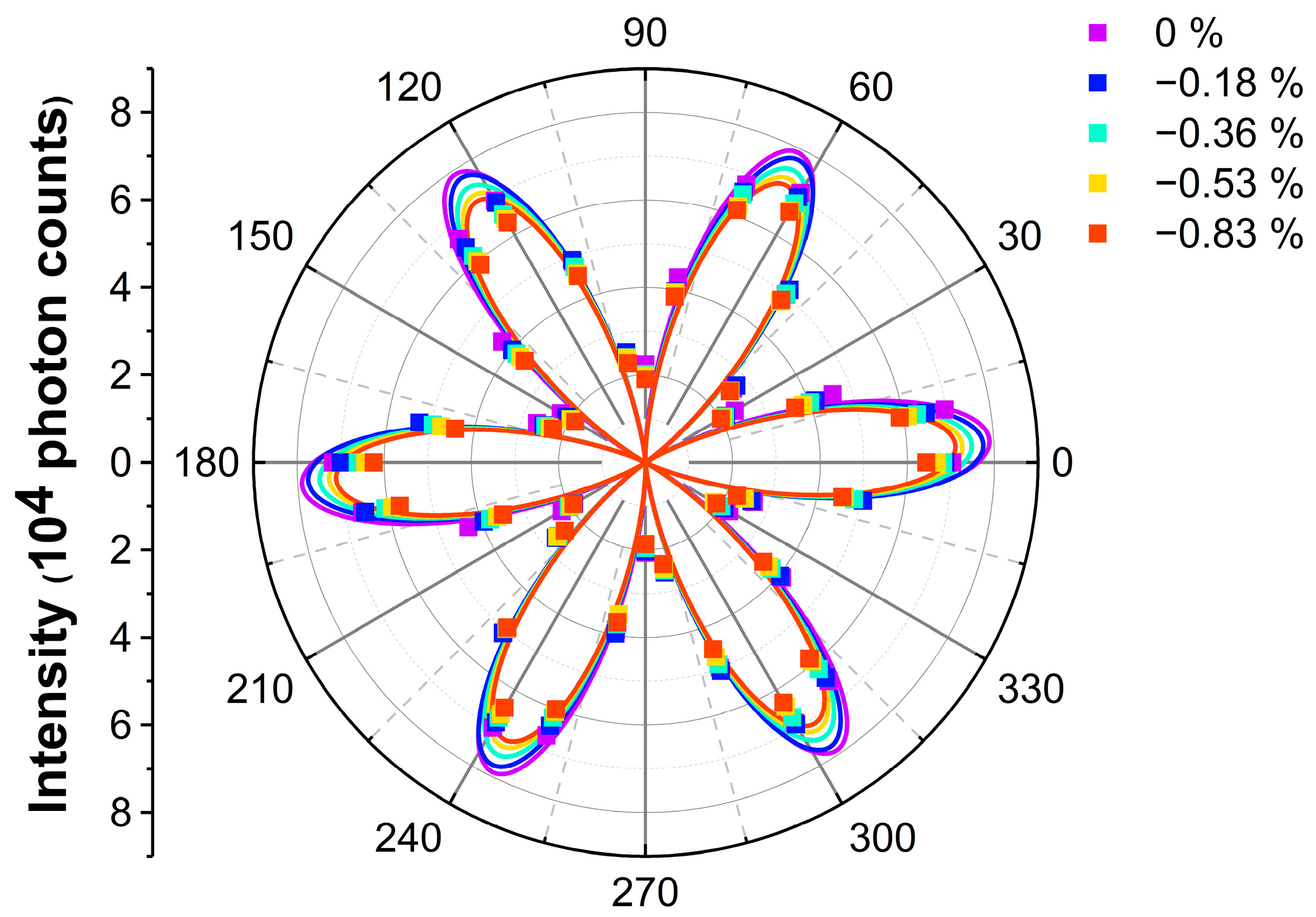
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.-E.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Rubio, A.; Le Lay, G. Emergent elemental two-dimensional materials beyond graphene. J. Phys. D Appl. Phys. 2017, 50, 053004. [Google Scholar] [CrossRef]
- Tan, T.; Jiang, X.; Wang, C.; Yao, B.; Zhang, H. 2D material optoelectronics for information functional device applications: Status and challenges. Adv. Sci. 2020, 7, 2000058. [Google Scholar] [CrossRef] [PubMed]
- Akinwande, D.; Petrone, N.; Hone, J. Two-dimensional flexible nanoelectronics. Nat. Commun. 2014, 5, 5678. [Google Scholar] [CrossRef]
- Gao, L. Flexible device applications of 2D semiconductors. Small 2017, 13, 1603994. [Google Scholar] [CrossRef]
- Dai, M.; Gao, C.; Nie, Q.; Wang, Q.J.; Lin, Y.F.; Chu, J.; Li, W. Properties, Synthesis, and Device Applications of 2D Layered InSe. Adv. Mater. Technol. 2022, 7, 2200321. [Google Scholar] [CrossRef]
- Sucharitakul, S.; Goble, N.J.; Kumar, U.R.; Sankar, R.; Bogorad, Z.A.; Chou, F.-C.; Chen, Y.-T.; Gao, X.P. Intrinsic electron mobility exceeding 103 cm2/(V s) in multilayer InSe FETs. Nano Lett. 2015, 15, 3815–3819. [Google Scholar] [CrossRef]
- Bandurin, D.A.; Tyurnina, A.V.; Yu, G.L.; Mishchenko, A.; Zólyomi, V.; Morozov, S.V.; Kumar, R.K.; Gorbachev, R.V.; Kudrynskyi, Z.R.; Pezzini, S. High electron mobility, quantum Hall effect and anomalous optical response in atomically thin InSe. Nat. Nanotechnol. 2017, 12, 223–227. [Google Scholar] [CrossRef]
- Wei, T.-R.; Jin, M.; Wang, Y.; Chen, H.; Gao, Z.; Zhao, K.; Qiu, P.; Shan, Z.; Jiang, J.; Li, R. Exceptional plasticity in the bulk single-crystalline van der Waals semiconductor InSe. Science 2020, 369, 542–545. [Google Scholar] [CrossRef]
- Zhao, Q.; Frisenda, R.; Wang, T.; Castellanos-Gomez, A. InSe: A two-dimensional semiconductor with superior flexibility. Nanoscale 2019, 11, 9845–9850. [Google Scholar] [CrossRef]
- Tamalampudi, S.R.; Lu, Y.-Y.; U., R.K.; Sankar, R.; Liao, C.-D.; Cheng, C.-H.; Chou, F.C.; Chen, Y.-T. High performance and bendable few-layered InSe photodetectors with broad spectral response. Nano Lett. 2014, 14, 2800–2806. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Wang, T.; Wu, M.; Cao, T.; Chen, Y.; Sankar, R.; Ulaganathan, R.K.; Chou, F.; Wetzel, C.; Xu, C.-Y. Ultrasensitive tunability of the direct bandgap of 2D InSe flakes via strain engineering. 2D Mater. 2018, 5, 021002. [Google Scholar] [CrossRef]
- Song, C.; Fan, F.; Xuan, N.; Huang, S.; Zhang, G.; Wang, C.; Sun, Z.; Wu, H.; Yan, H. Largely tunable band structures of few-layer InSe by uniaxial strain. ACS Appl. Mater. Interfaces 2018, 10, 3994–4000. [Google Scholar] [CrossRef] [PubMed]
- Cassidy, D.T.; Lam, S.; Lakshmi, B.; Bruce, D.M. Strain mapping by measurement of the degree of polarization of photoluminescence. Appl. Opt. 2004, 43, 1811–1818. [Google Scholar] [CrossRef]
- Tripathy, S.; Chua, S.; Chen, P.; Miao, Z. Micro-Raman investigation of strain in GaN and Alx Ga1− x N/GaN heterostructures grown on Si (111). J. Appl. Phys. 2002, 92, 3503–3510. [Google Scholar] [CrossRef]
- Mennel, L.; Furchi, M.M.; Wachter, S.; Paur, M.; Polyushkin, D.K.; Mueller, T. Optical imaging of strain in two-dimensional crystals. Nat. Commun. 2018, 9, 516. [Google Scholar] [CrossRef]
- Sugita, A.; Mochiduki, K.; Katahira, Y.; Ng, S.H.; Juodkazis, S.O.E. Augmentation of surface plasmon-enhanced second harmonic generation from Au nanoprisms on SiO2/Si: Interference contribution. Opt. Express 2022, 30, 22161–22177. [Google Scholar] [CrossRef]
- Sautter, D., Jr.; Xu, L.; Miroshnichenko, A.E.; Lysevych, M.; Volkovskaya, I.; Smirnova, D.A.; Camacho-Morales, R.; Zangeneh Kamali, K.; Karouta, F.; Vora, K. Tailoring second-harmonic emission from (111)-GaAs nanoantennas. Nano Lett. 2019, 19, 3905–3911. [Google Scholar] [CrossRef]
- Gili, V.F.; Carletti, L.; Locatelli, A.; Rocco, D.; Finazzi, M.; Ghirardini, L.; Favero, I.; Gomez, C.; Lemaître, A.; Celebrano, M. Monolithic AlGaAs second-harmonic nanoantennas. Opt. Express 2016, 24, 15965–15971. [Google Scholar] [CrossRef]
- Boyd, R.W. Nonlinear Optics; Academic Press: London, UK, 2020. [Google Scholar]
- Kumar, N.; Najmaei, S.; Cui, Q.; Ceballos, F.; Ajayan, P.M.; Lou, J.; Zhao, H. Second harmonic microscopy of monolayer MoS2. Phys. Rev. B 2013, 87, 161403. [Google Scholar] [CrossRef]
- Janisch, C.; Wang, Y.; Ma, D.; Mehta, N.; Elías, A.L.; Perea-López, N.; Terrones, M.; Crespi, V.; Liu, Z. Extraordinary second harmonic generation in tungsten disulfide monolayers. Sci. Rep. 2014, 4, 5530. [Google Scholar] [CrossRef] [PubMed]
- Ribeiro-Soares, J.; Janisch, C.; Liu, Z.; Elías, A.; Dresselhaus, M.; Terrones, M.; Cançado, L.; Jorio, A. Second harmonic generation in WSe2. 2D Mater. 2015, 2, 045015. [Google Scholar] [CrossRef]
- Mennel, L.; Paur, M.; Mueller, T. Second harmonic generation in strained transition metal dichalcogenide monolayers: MoS2, MoSe2, WS2, and WSe2. APL Photonics 2019, 4, 034404. [Google Scholar] [CrossRef]
- Liang, J.; Zhang, J.; Li, Z.; Hong, H.; Wang, J.; Zhang, Z.; Zhou, X.; Qiao, R.; Xu, J.; Gao, P. Monitoring local strain vector in atomic-layered MoSe2 by second-harmonic generation. Nano Lett. 2017, 17, 7539–7543. [Google Scholar] [CrossRef]
- Grimaldi, I.; Gerace, T.; Pipita, M.; Perrotta, I.; Ciuchi, F.; Berger, H.; Papagno, M.; Castriota, M.; Pacilé, D. Structural investigation of InSe layered semiconductors. Solid State Commun. 2020, 311, 113855. [Google Scholar] [CrossRef]
- Hao, Q.; Yi, H.; Su, H.; Wei, B.; Wang, Z.; Lao, Z.; Chai, Y.; Wang, Z.; Jin, C.; Dai, J. Phase identification and strong second harmonic generation in pure ε-InSe and its alloys. Nano Lett. 2019, 19, 2634–2640. [Google Scholar] [CrossRef]
- Koskinen, K.; Slablab, A.; Divya, S.; Czaplicki, R.; Chervinskii, S.; Kailasnath, M.; Radhakrishnan, P.; Kauranen, M. Bulk second-harmonic generation from thermally evaporated indium selenide thin films. Opt. Lett. 2017, 42, 1076–1079. [Google Scholar] [CrossRef]
- Zhou, J.; Shi, J.; Zeng, Q.; Chen, Y.; Niu, L.; Liu, F.; Yu, T.; Suenaga, K.; Liu, X.; Lin, J. InSe monolayer: Synthesis, structure and ultra-high second-harmonic generation. 2D Mater. 2018, 5, 025019. [Google Scholar] [CrossRef]
- Leisgang, N.; Roch, J.G.; Froehlicher, G.; Hamer, M.; Terry, D.; Gorbachev, R.; Warburton, R.J. Optical second harmonic generation in encapsulated single-layer InSe. AIP Adv. 2018, 8, 105120. [Google Scholar] [CrossRef]
- Sun, Z.; Zhang, Y.; Qian, J.; Qiao, R.; Li, X.; Wang, Z.; Zheng, C.; Liu, K.; Cao, T.; Liu, W.T. Compelling Evidence for the ε-Phase InSe Crystal by Oblique Incident Second Harmonic Generation. Adv. Opt. Mater. 2022, 10, 2201183. [Google Scholar] [CrossRef]
- Island, J.O.; Kuc, A.; Diependaal, E.H.; Bratschitsch, R.; Van Der Zant, H.S.; Heine, T.; Castellanos-Gomez, A. Precise and reversible band gap tuning in single-layer MoSe2 by uniaxial strain. Nanoscale 2016, 8, 2589–2593. [Google Scholar] [CrossRef] [PubMed]
- The Code, OpenMX, Pseudoatomic Basis Functions, and Pseudopotentials Are Available on a Website. Available online: https://www.openmx-square.org/ (accessed on 3 December 2019).
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef] [PubMed]
- Morrison, I.; Bylander, D.; Kleinman, L. Nonlocal Hermitian norm-conserving Vanderbilt pseudopotential. Phys. Rev. B 1993, 47, 6728. [Google Scholar] [CrossRef] [PubMed]
- Ozaki, T. Variationally optimized atomic orbitals for large-scale electronic structures. Phys. Rev. B 2003, 67, 155108. [Google Scholar] [CrossRef]
- Lee, C.-C.; Lee, Y.-T.; Fukuda, M.; Ozaki, T. Tight-binding calculations of optical matrix elements for conductivity using nonorthogonal atomic orbitals: Anomalous Hall conductivity in bcc Fe. Phys. Rev. B 2018, 98, 115115. [Google Scholar] [CrossRef]
- Ghahramani, E.; Moss, D.; Sipe, J. Full-band-structure calculation of second-harmonic generation in odd-period strained (Si)n/(Ge)n superlattices. Phys. Rev. B 1991, 43, 8990. [Google Scholar] [CrossRef] [PubMed]
- Duan, C.-G.; Li, J.; Gu, Z.-Q.; Wang, D.-S. First-principles calculation of the second-harmonic-generation coefficients of borate crystals. Phys. Rev. B 1999, 60, 9435. [Google Scholar] [CrossRef]
- Onida, G.; Reining, L.; Rubio, A. Electronic excitations: Density-functional versus many-body Green’s-function approaches. Rev. Mod. Phys. 2002, 74, 601. [Google Scholar] [CrossRef]
- Deckoff-Jones, S.; Zhang, J.; Petoukhoff, C.E.; Man, M.K.; Lei, S.; Vajtai, R.; Ajayan, P.M.; Talbayev, D.; Madéo, J.; Dani, K.M. Observing the interplay between surface and bulk optical nonlinearities in thin van der Waals crystals. Sci. Rep. 2016, 6, 22620. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Z.-Y.; Cheng, H.-Y.; Kung, S.-H.; Yao, H.-C.; Inbaraj, C.R.P.; Sankar, R.; Ou, M.-N.; Chen, Y.-F.; Lee, C.-C.; Lin, K.-H. Uniaxial Strain Dependence on Angle-Resolved Optical Second Harmonic Generation from a Few Layers of Indium Selenide. Nanomaterials 2023, 13, 750. https://doi.org/10.3390/nano13040750
Li Z-Y, Cheng H-Y, Kung S-H, Yao H-C, Inbaraj CRP, Sankar R, Ou M-N, Chen Y-F, Lee C-C, Lin K-H. Uniaxial Strain Dependence on Angle-Resolved Optical Second Harmonic Generation from a Few Layers of Indium Selenide. Nanomaterials. 2023; 13(4):750. https://doi.org/10.3390/nano13040750
Chicago/Turabian StyleLi, Zi-Yi, Hao-Yu Cheng, Sheng-Hsun Kung, Hsuan-Chun Yao, Christy Roshini Paul Inbaraj, Raman Sankar, Min-Nan Ou, Yang-Fang Chen, Chi-Cheng Lee, and Kung-Hsuan Lin. 2023. "Uniaxial Strain Dependence on Angle-Resolved Optical Second Harmonic Generation from a Few Layers of Indium Selenide" Nanomaterials 13, no. 4: 750. https://doi.org/10.3390/nano13040750
APA StyleLi, Z.-Y., Cheng, H.-Y., Kung, S.-H., Yao, H.-C., Inbaraj, C. R. P., Sankar, R., Ou, M.-N., Chen, Y.-F., Lee, C.-C., & Lin, K.-H. (2023). Uniaxial Strain Dependence on Angle-Resolved Optical Second Harmonic Generation from a Few Layers of Indium Selenide. Nanomaterials, 13(4), 750. https://doi.org/10.3390/nano13040750






