Waves Propagating in Nano-Layered Phononic Crystals with Flexoelectricity, Microstructure, and Micro-Inertia Effects
Abstract
:1. Introduction
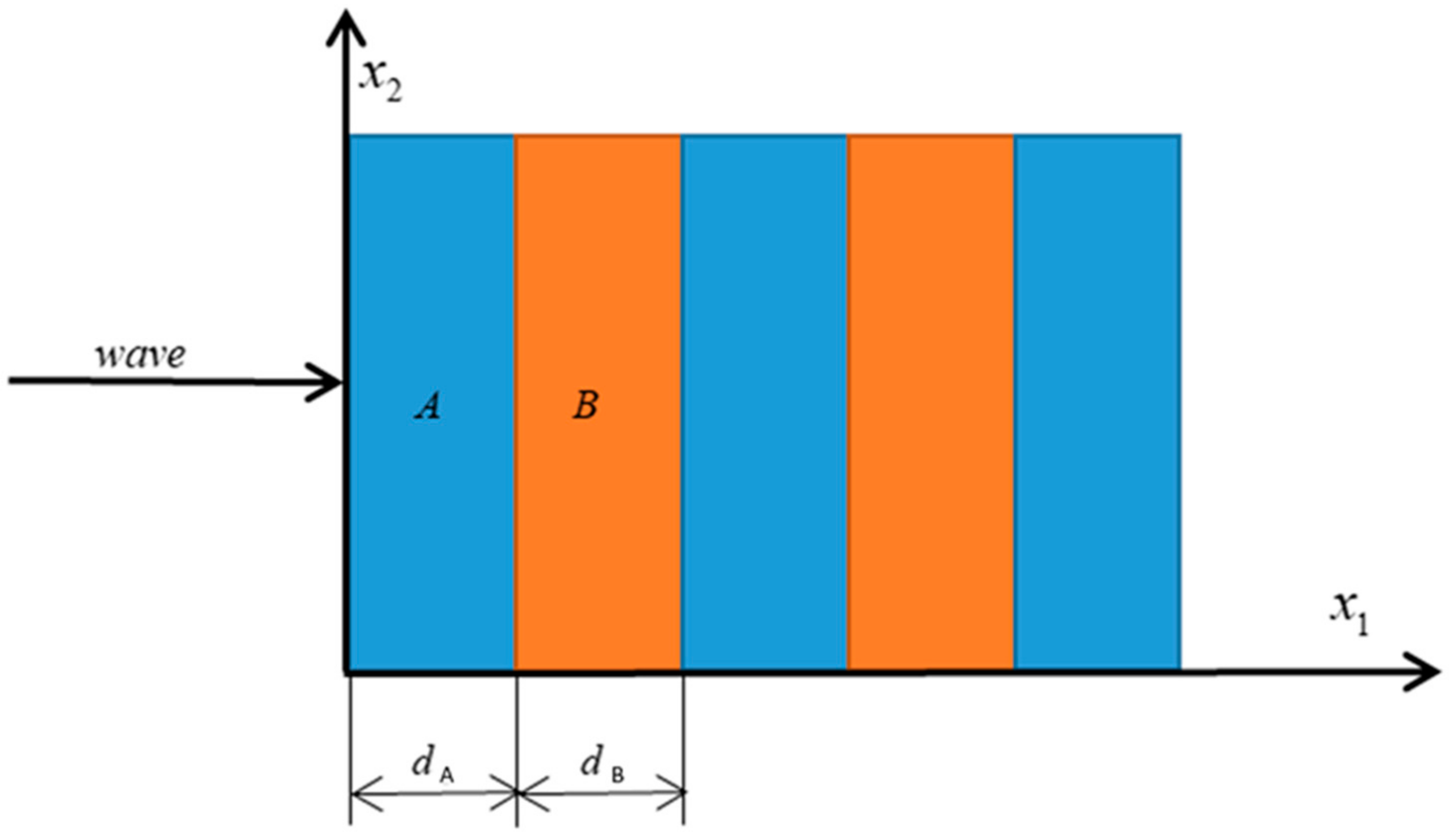
2. Materials and Methods
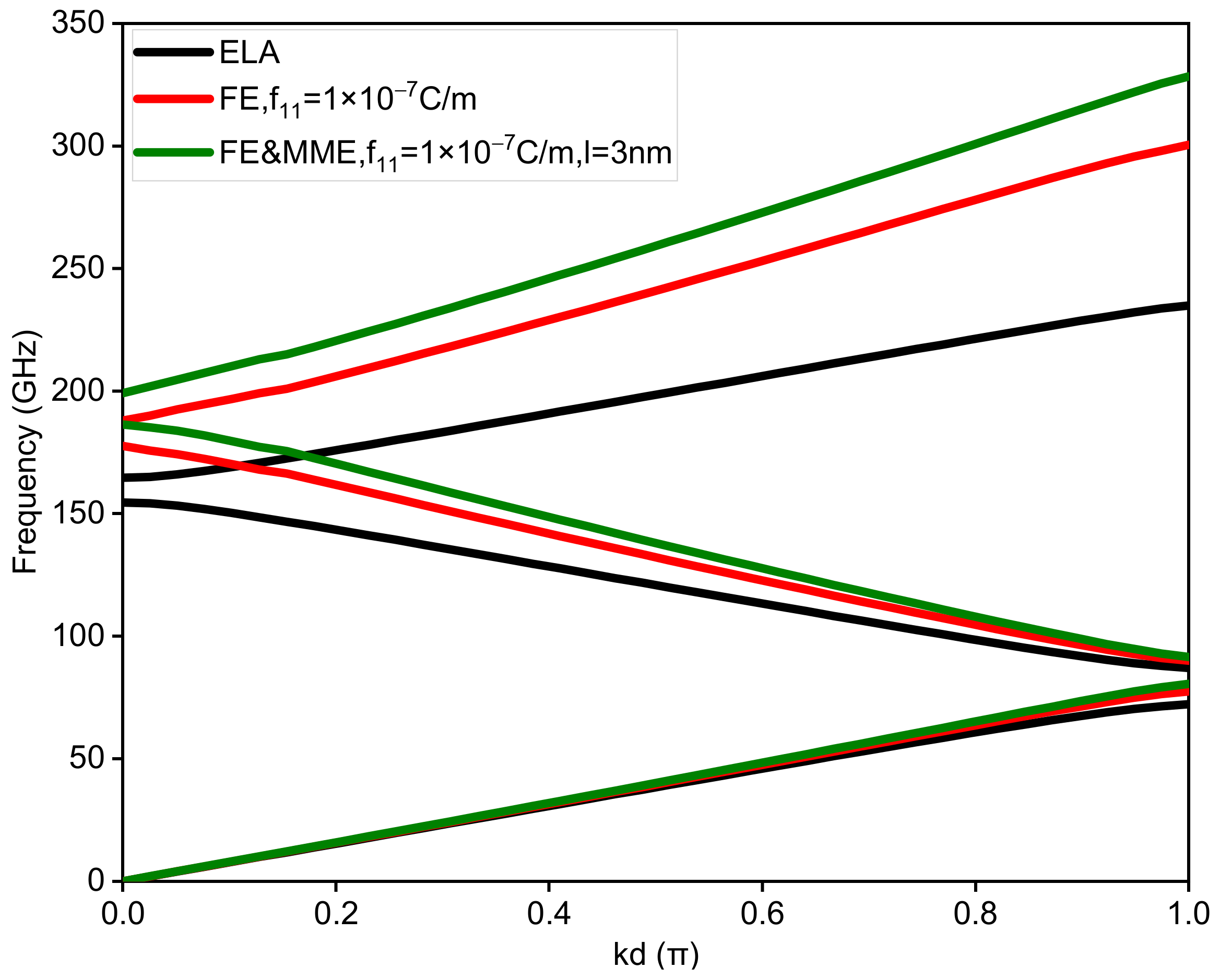
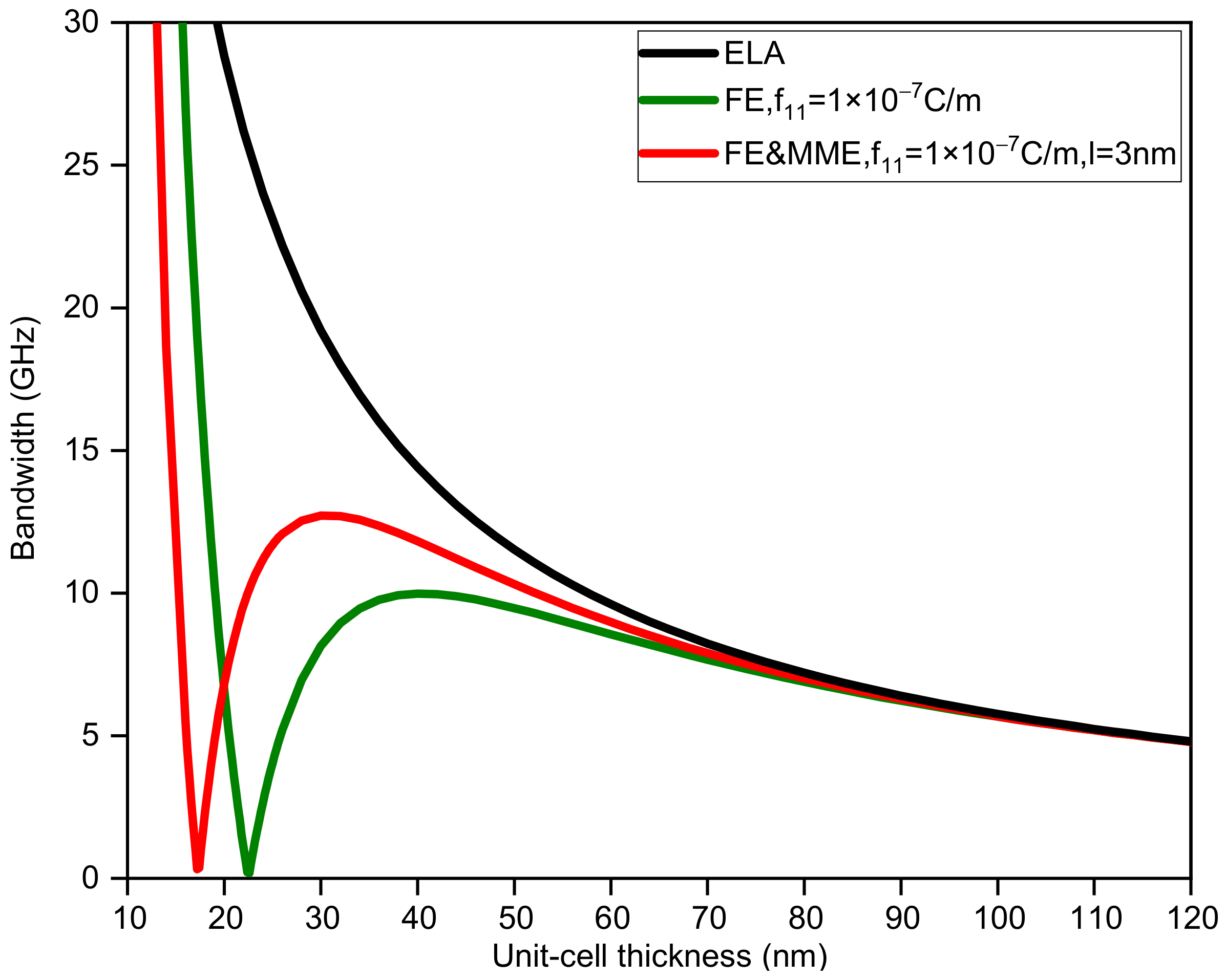
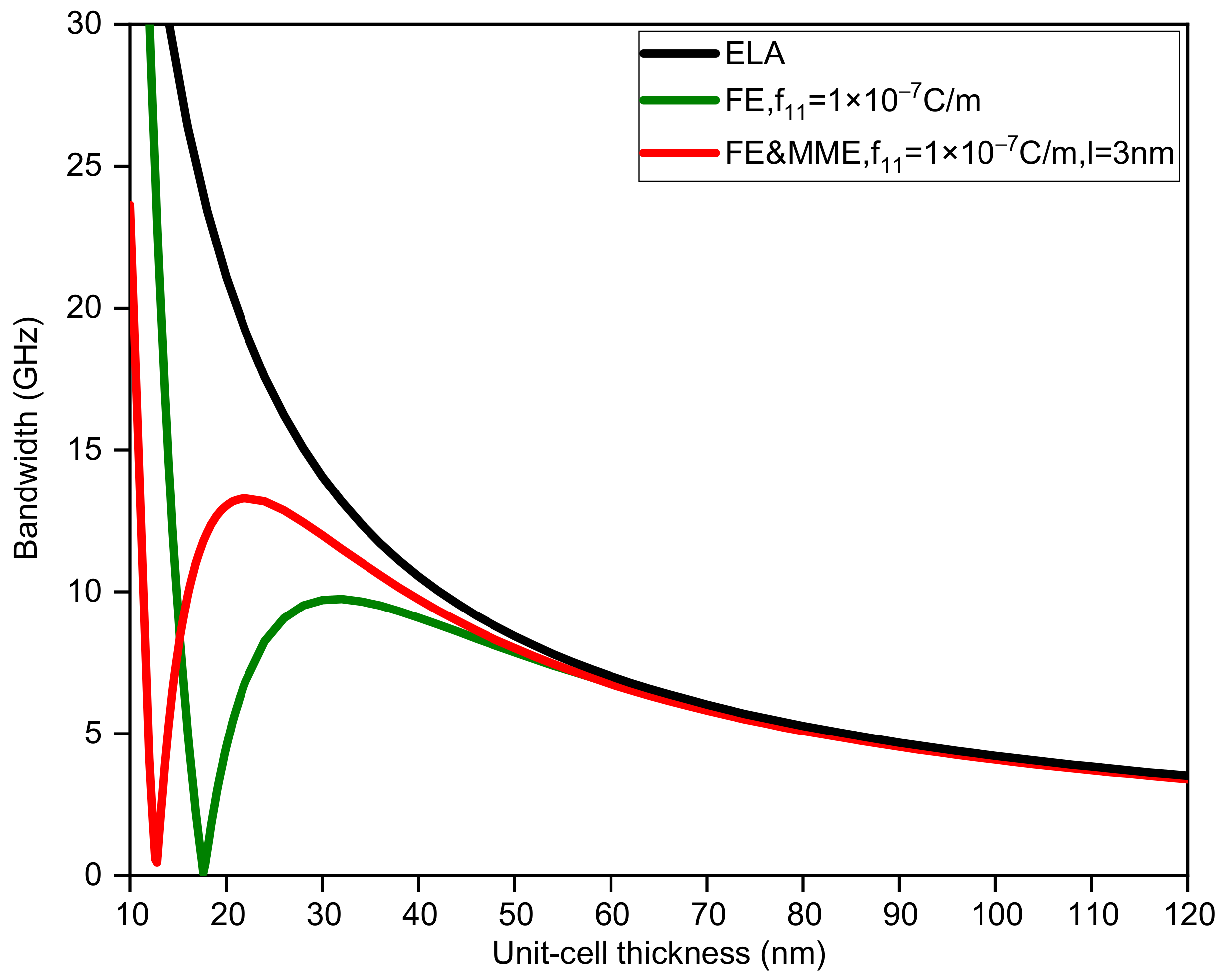
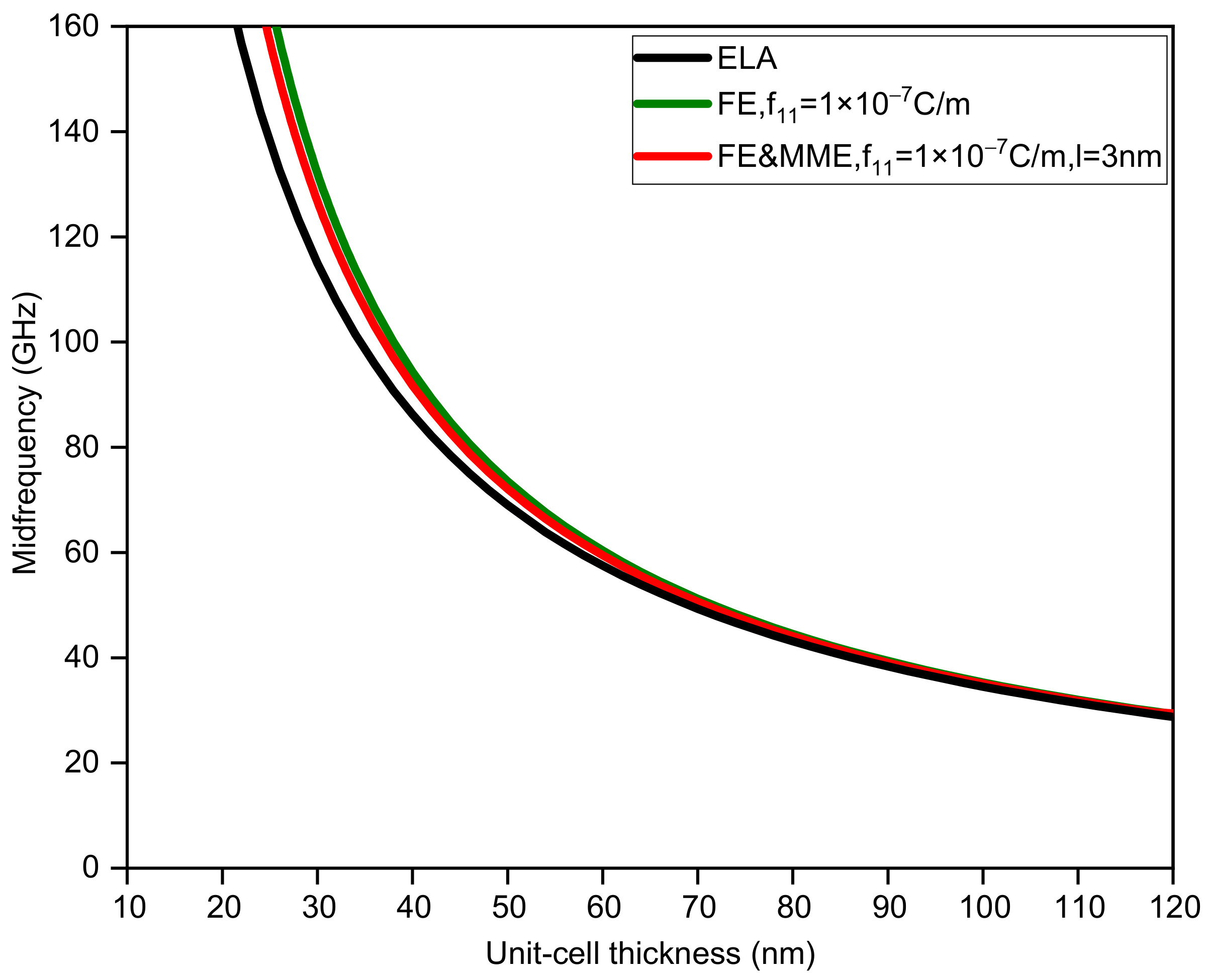
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kushwaha, M.S.; Halevi, P.; Dobrzynski, L.; Djafari-Rouhani, B. Acoustic band structure of periodic elastic composites. Phys. Rev. Lett. 1993, 71, 2022–2025. [Google Scholar] [CrossRef] [PubMed]
- Sigalas, M.M.; Economou, E.N. Elastic and acoustic wave band structure. J. Sound Vib. 1992, 158, 377–382. [Google Scholar] [CrossRef]
- Hussein, M.I.; Hulbert, G.M.; Scott, R.A. Dispersive elastodynamics of 1D banded materials and structures: Design. J. Sound Vib. 2007, 307, 865–893. [Google Scholar] [CrossRef] [Green Version]
- Assouar, M.B.; Senesi, M.; Oudich, M.; Ruzzene, M.; Hou, Z.L. Broadband plate-type acoustic metamaterial for low-frequency sound attenuation. Appl. Phys. Lett. 2012, 101, 173505. [Google Scholar] [CrossRef]
- Pennec, Y.; Djafari-Rouhani, B.; Vasseur, J.O.; Larabi, H.; Khelif, A.; Choujaa, A.; Benchabane, S.; Laude, V. Acoustic channel drop tunneling in a phononic crystal. Appl. Phys. Lett. 2005, 87, 261912. [Google Scholar] [CrossRef]
- Liu, Z.Y.; Zhang, X.X.; Mao, Y.W.; Zhu, Y.Y.; Yang, Z.Y.; Chan, C.T.; Sheng, P. Locally resonant sonic materials. Science 2000, 289, 1734–1736. [Google Scholar] [CrossRef]
- Vasseur, J.O.; Deymier, P.A.; Chenni, B.; Djafari-Rouhani, B.; Dobrzynski, L.; Prevost, D. Experimental and theoretical evidence for the existence of absolute acoustic band gaps in two-dimensional solid phononic crystals. Phys. Rev. Lett. 2001, 86, 3012. [Google Scholar] [CrossRef] [Green Version]
- Huang, Z.G. Silicon-based filters, resonators and acoustic channels with phononic crystal structures. J. Phys. D Appl. Phys. 2011, 44, 245406. [Google Scholar] [CrossRef]
- Khelif, A.; Djafari-Rouhani, B.; Vasseur, J.O.; Deymier, P.A.; Lambin, P.; Dobrzynski, L. Transmittivity through straight and stublike waveguides in a two-dimensional phononic crystal. Phys. Rev. B. 2002, 65, 174308. [Google Scholar] [CrossRef] [Green Version]
- Shakeri, A.; Darbari, S.; Moravvej-Farshi, M.K. Designing a tunable acoustic resonator based on defect modes, stimulated by selectively biased PZT rods in a 2D phononic crystal. Ultrasonics 2019, 92, 8–12. [Google Scholar] [CrossRef]
- Chandra, H.; Deymier, P.A.; Vasseur, J.O. Elastic wave propagation along waveguides in three-dimensional phononic crystals. Phys. Rev. B 2004, 70, 054302. [Google Scholar] [CrossRef] [Green Version]
- Wu, X.D.; Zhang, M.H.; Zuo, S.G.; Huang, H.D.; Wu, H. An investigation on interior noise reduction using 2D locally resonant phononic crystal with point defect on car ceiling. J. Vib. Control 2018, 25, 386–396. [Google Scholar] [CrossRef]
- Graczykowski, B.; Sledzinska, M.; Alzina, F.; Gomis-Bresco, J.; Reparaz, J.S.; Wagner, M.R.; Torres, C.S. Phonon dispersion in hypersonic two-dimensional phononic crystal membranes. Phys. Rev. B 2015, 91, 075414. [Google Scholar] [CrossRef] [Green Version]
- Ezzahri, Y.; Grauby, S.; Rampnoux, J.-M.; Michel, H.; Pernot, G.; Claeys, W.; Dilhaire, S.; Rossignol, C.; Zeng, G.; Shakouri, A. Coherent phonons in Si/Si Ge superlattices. Phys. Rev. B 2007, 75, 195309. [Google Scholar] [CrossRef]
- Lin, S.P.; Zhu, L.F.; Qiu, Y.; Jiang, Z.Y.; Wang, Y.F.; Zhu, J.; Wu, H.P. A self-powered multi-functional sensor based on triboelectric nanogenerator for monitoring states of rotating motion. Nano Energy 2021, 83, 105857. [Google Scholar] [CrossRef]
- Qiu, Y.; Tian, Y.; Sun, S.S.; Hu, J.H.; Wang, Y.Y.; Zhang, Z.; Liu, A.P.; Cheng, H.Y.; Gao, W.Z.; Zhang, W.N.; et al. Bioinspired, multifunctional dual -mode pressure sensors as electronic skin for decoding complex loading processes and human motions. Nano Energy 2020, 78, 105337. [Google Scholar] [CrossRef]
- Qiu, Y.; Wang, C.J.; Lu, X.Y.; Wu, H.P.; Ma, X.L.; Hu, J.H.; Qi, H.C.; Tian, Y.; Zhang, Z.; Bao, G.J. A Biomimetic drosera capensis with adaptive decision-predation behavior based on multifunctional sensing and fast actuating capability. Adv. Funct. Mater. 2022, 32, 2270077. [Google Scholar] [CrossRef]
- Scott, J.F. Lattice perturbations in CaWO4 and CaMoO4. J. Chem. Phys. 1968, 48, 874–876. [Google Scholar] [CrossRef]
- Tagantsev, A.K. Theory of flexoelectric effect in crystals. Zhurnal Eksperimental’noi I Teoreticheskoi Fiziki 1985, 88, 2108–2122. [Google Scholar]
- Kogan, S.M. Piezoelectric effect during inhomogeneous deformation and acoustic scattering of carriers in crystals. Sov. Phys. Solid State 1964, 5, 2069–2070. [Google Scholar]
- Tagantsev, A. K Piezoelectricity and flexoelectricity in crystalline dielectrics. Phys. Rev. B 1986, 34, 5883. [Google Scholar] [CrossRef]
- Hu, S.L.; Shen, S.P. Electric field gradient theory with surface effect for nano-dielectrics. Comput. Mater. Contin. 2009, 13, 63–87. [Google Scholar]
- Hu, S.L.; Shen, S.P. Variational principles and governing equations in nano-dielectrics with the flexoelectric effect. Sci. China Phys. Mech. 2010, 53, 1497–1504. [Google Scholar] [CrossRef]
- Shen, S.P.; Hu, S.L. A theory of flexoelectricity with surface effect for elastic dielectrics. J. Mech. Phys. Solids 2010, 58, 665–677. [Google Scholar] [CrossRef]
- Majdoub, M.S.; Sharma, P.; Cagin, T. Enhanced size-dependent piezoelectricity and elasticity in nanostructures due to the flexoelectric effect. Phys. Rev. B 2008, 77, 125424. [Google Scholar] [CrossRef] [Green Version]
- Yan, Z.; Jiang, L.Y. Size-dependent bending and vibration behaviour of piezoelectric nanobeams due to flexoelectricity. J. Phys. D Appl. Phys. 2013, 46, 355502. [Google Scholar] [CrossRef]
- Yan, Z.; Jiang, L.Y. Flexoelectric effect on the electroelastic responses of bending piezoelectric nanobeams. J. Appl. Phys. 2013, 113, 194102. [Google Scholar] [CrossRef]
- Yan, Z. Exact solutions for the electromechanical responses of a dielectric nano-ring. J. Intell. Mater. Syst. Struct. 2017, 28, 1140–1149. [Google Scholar] [CrossRef]
- Hu, T.T.; Yang, W.J.; Liang, X.; Shen, S.P. Wave propagation in flexoelectric microstructured solids. J. Elast. 2017, 130, 197–210. [Google Scholar] [CrossRef]
- Hu, T.T.; Wang, X.; Yan, Y.; Chen, L.J.; Xu, W. Influence of impact velocity on flexoelectric effect. Results Phys. 2019, 15, 102812. [Google Scholar] [CrossRef]
- Yang, W.J.; Deng, Q.; Liang, X.; Shen, S.P. Lamb wave propagation with flexoelectricity and strain gradient elasticity considered. Smart. Mater. Struct. 2018, 27, 085003. [Google Scholar] [CrossRef]
- Zheng, H.; Zhou, C.; Yan, D.J.; Wang, Y.S.; Zhang, C.Z. A meshless collocation method for band structure simulation of nanoscale phononic crystals based on nonlocal elasticity theory. J. Comput. Phys. 2020, 408, 109268. [Google Scholar] [CrossRef]
- Liu, C.C.; Hu, S.L.; Shen, S.P. Effect of flexoelectricity on band structures of one-dimensional phononic crystals. J. Appl. Mech. 2014, 81, 051007. [Google Scholar] [CrossRef]
- Yang, W.J.; Hu, T.T.; Liang, X.; Shen, S.P. On band structures of layered phononic crystals with flexoelectricity. Appl. Mech. Mater. 2017, 88, 629–644. [Google Scholar] [CrossRef]
- Qi, L. Rayleigh wave propagation in semi-infinite flexoelectric dielectrics. Phys. Scr. 2019, 94, 065803. [Google Scholar] [CrossRef]
- Yang, W.J.; Liang, X.; Deng, Q.; Shen, S.P. Rayleigh wave propagation in a homogeneous centrosymmetric flexoelectric half-space. Ultrasonics 2020, 103, 106105. [Google Scholar] [CrossRef]
- Georgiadis, H.G.; Vardoulakis, I.; Velgaki, E.G. Dispersive Rayleigh-wave propagation in microstructured solids characterized by dipolar gradient elasticity. J. Elast. 2004, 74, 17–45. [Google Scholar] [CrossRef]
- Shodja, H.M.; Goodarzi, A.; Delfani, M.R.; Haftbaradaran, H. Scattering of an anti-plane shear wave by an embedded cylindrical micro-/nano-fiber within couple stress theory with micro inertia. Int. J. Solids Struct. 2015, 58, 73–90. [Google Scholar] [CrossRef]
- Maranganti, R.; Sharma, P. Atomistic determination of flexoelectric properties of crystalline dielectrics. Phys. Rev. B 2009, 80, 054109. [Google Scholar] [CrossRef] [Green Version]
- Xu, L.; Shen, S.P. Size-dependent piezoelectricity and elasticity due to the electric field-strain gradient coupling and strain gradient elasticity. Int. J. Appl. Mech. 2013, 5, 1350015. [Google Scholar] [CrossRef]





| ELA | FE | FE&MME | ||
|---|---|---|---|---|
| 1st band gap | Range (GHz) | 72–87 | 79–89 | 80–92 |
| Midfrequency (GHz) | 79.5 | 84 | 86 | |
| 2nd band gap | Range (GHz) | 154–164 | 177–187 | 186–199 |
| Midfrequency (GHz) | 159 | 182 | 192 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, J.; Hu, P.; Chen, Y.; Chen, S.; Zhang, C.; Wang, Y.; Liu, D. Waves Propagating in Nano-Layered Phononic Crystals with Flexoelectricity, Microstructure, and Micro-Inertia Effects. Nanomaterials 2022, 12, 1080. https://doi.org/10.3390/nano12071080
Zhu J, Hu P, Chen Y, Chen S, Zhang C, Wang Y, Liu D. Waves Propagating in Nano-Layered Phononic Crystals with Flexoelectricity, Microstructure, and Micro-Inertia Effects. Nanomaterials. 2022; 12(7):1080. https://doi.org/10.3390/nano12071080
Chicago/Turabian StyleZhu, Jun, Puying Hu, Yudan Chen, Shaowei Chen, Chuanzeng Zhang, Yanzheng Wang, and Dongying Liu. 2022. "Waves Propagating in Nano-Layered Phononic Crystals with Flexoelectricity, Microstructure, and Micro-Inertia Effects" Nanomaterials 12, no. 7: 1080. https://doi.org/10.3390/nano12071080
APA StyleZhu, J., Hu, P., Chen, Y., Chen, S., Zhang, C., Wang, Y., & Liu, D. (2022). Waves Propagating in Nano-Layered Phononic Crystals with Flexoelectricity, Microstructure, and Micro-Inertia Effects. Nanomaterials, 12(7), 1080. https://doi.org/10.3390/nano12071080








