Time-Dependent Flow of Water-Based CoFe2O4-Mn-ZnFe2O4 Nanoparticles over a Shrinking Sheet with Mass Transfer Effect in Porous Media
Abstract
1. Introduction
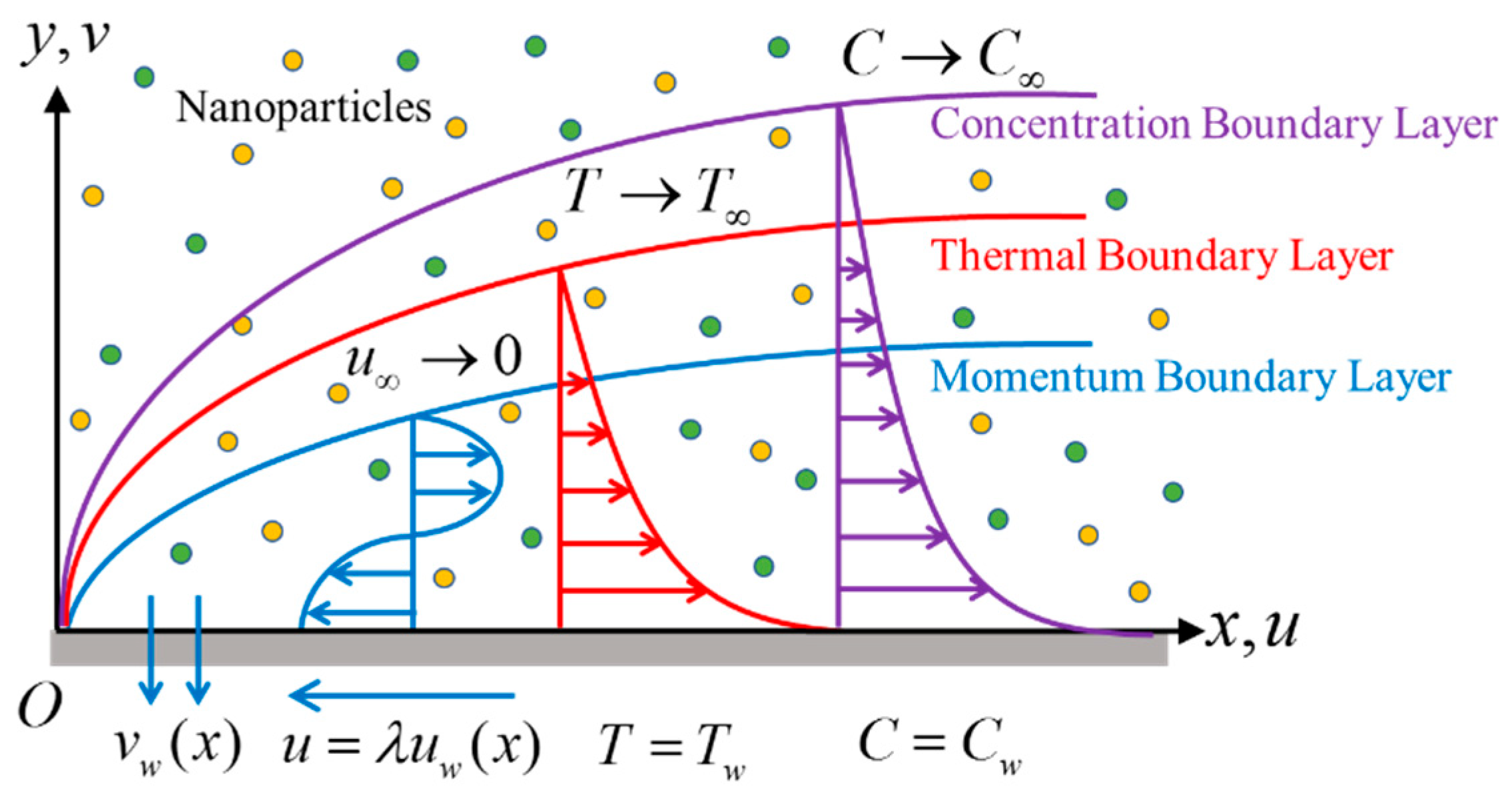
2. Description and Framework of the Mathematical Model
3. Time Stability Analysis
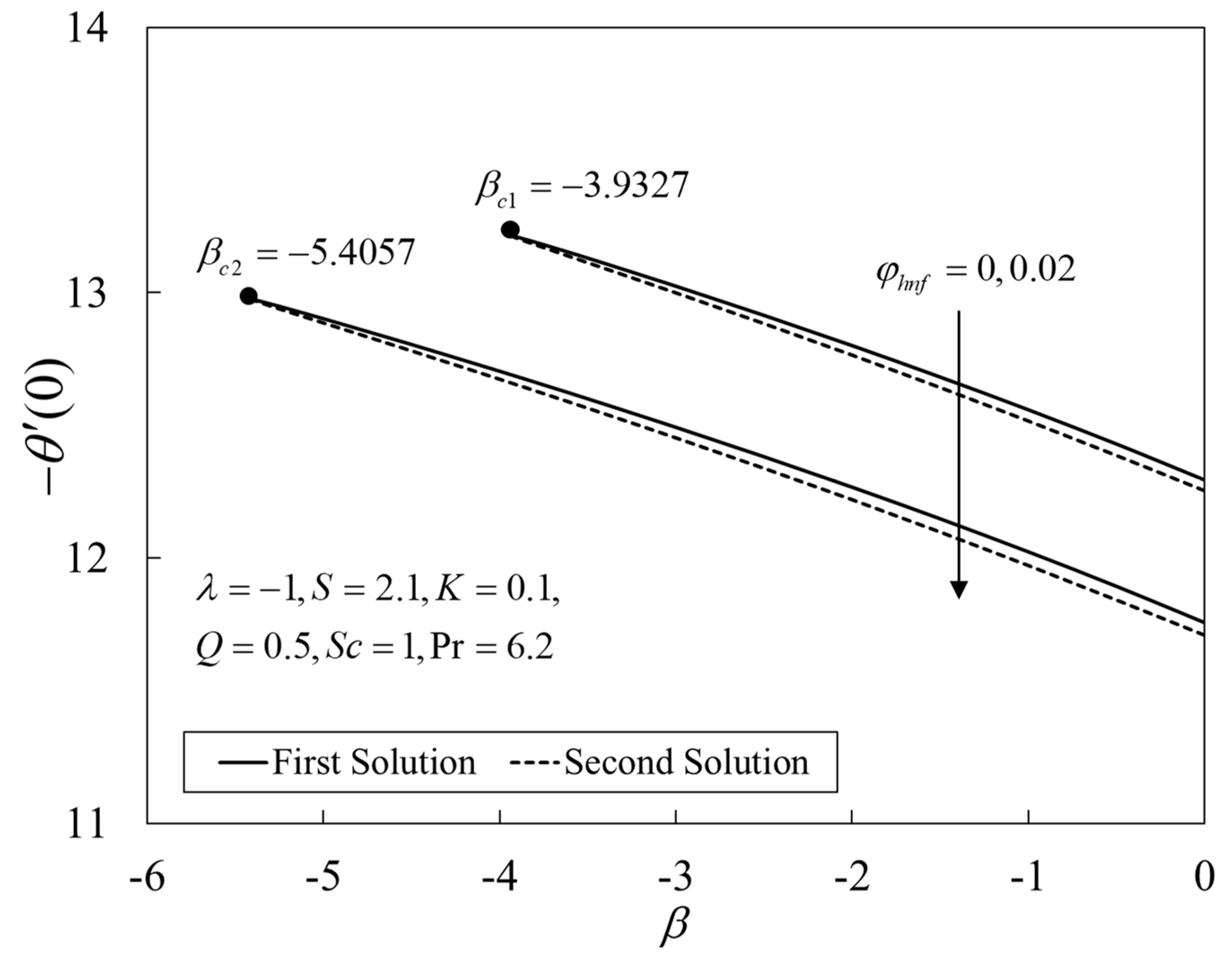
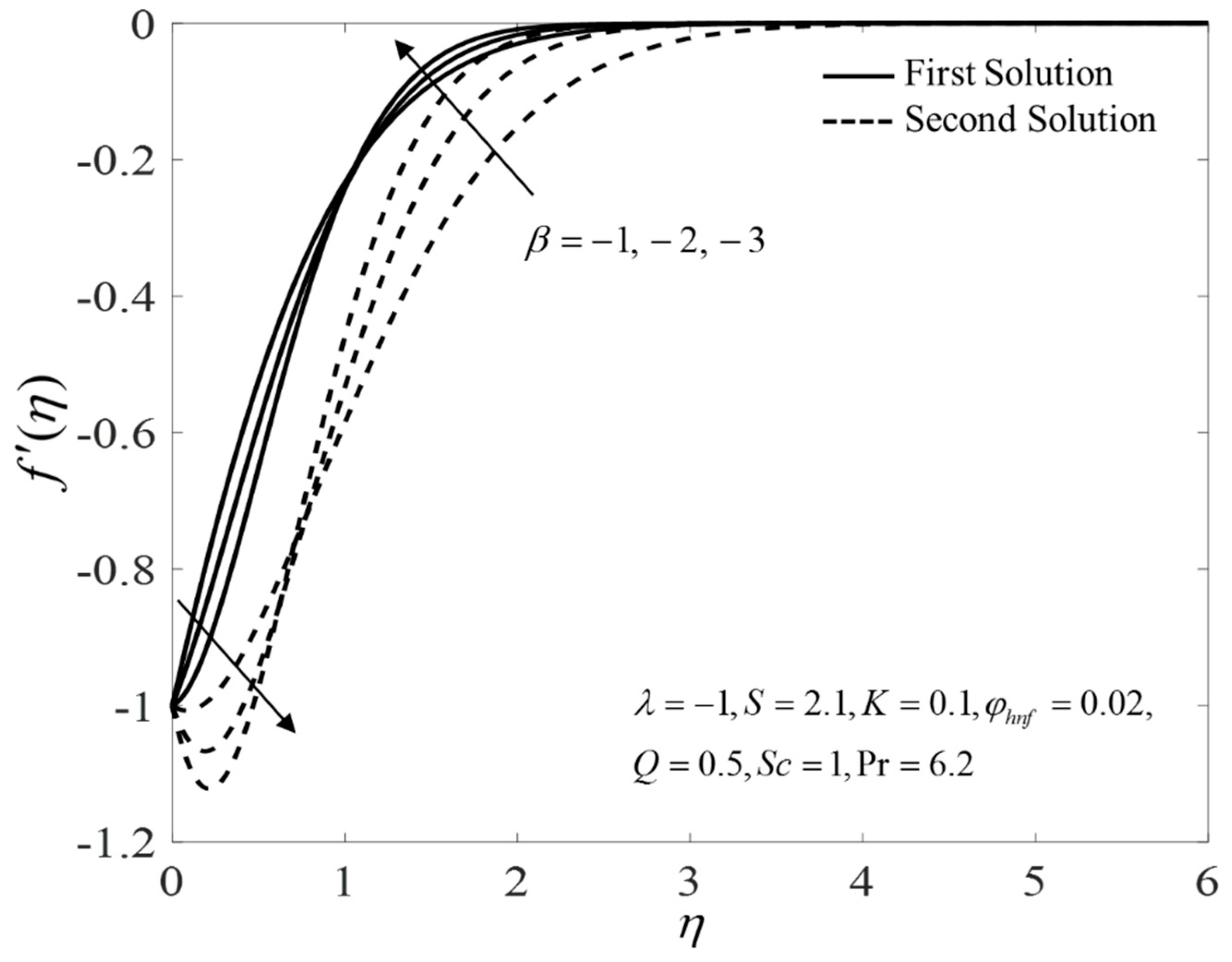
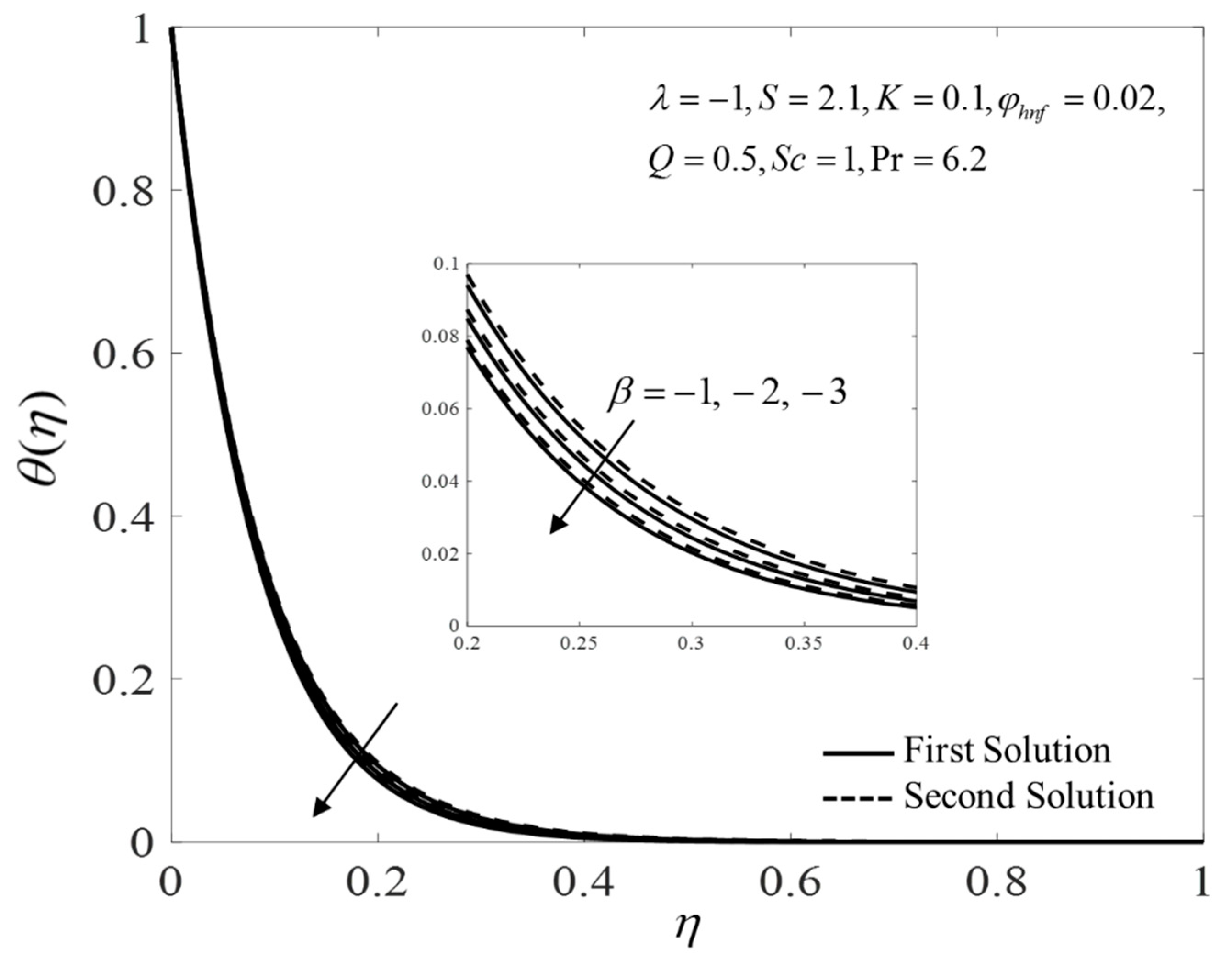
4. Analysis of Results and Discussion
5. Conclusions
- The mass transfer rate and the friction factor accelerate for the first branch solution, but decelerate for the second branch solution for high values of nanoparticle volume fraction , while the heat transfer rate abruptly diminished in both branch solutions.
- The heat and mass transfer rates, as well as the friction factor, enrich for the first solution for larger values of , while the opposite is seen for the second solution.
- The magnitude of the critical values augments with larger values of and , which ultimately causes the separations in the boundary layer to diminish.
- The first branch solution shows an inertial decay of disturbance, which is thus physically reliable as time passes, whereas the second branch solution shows the opposite response.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| constant | |
| C | concentration |
| ambient concentration | |
| surface concentration | |
| skin friction coefficient | |
| specific heat at constant pressure (J kg−1 K−1) | |
| mass diffusivity coefficient | |
| dimensionless stream function | |
| arbitrary functions | |
| permeability of the porous media | |
| porous medium parameter | |
| thermal conductivity of the fluid (W m−1 K−1) | |
| local Nusselt number | |
| Prandtl number | |
| heat sink or source coefficient | |
| heat sink or source parameter | |
| local Reynolds number | |
| mass flux velocity parameter | |
| Schmidt number | |
| local Sherwood number | |
| time (s) | |
| fluid temperature (K) | |
| ambient temperature (K) | |
| surface temperature (K) | |
| velocity component in the x- and y- directions (m s−1) | |
| velocity of the surface (m s−1) | |
| velocity of the mass flux (m s−1) | |
| Cartesian coordinates (m) | |
| Greek symbols | |
| constant | |
| unsteady parameter | |
| dimensionless time variable | |
| eigenvalue | |
| similarity variable | |
| dimensionless temperature | |
| stretching/shrinking parameter | |
| dynamic viscosity (kg m−1 s−1) | |
| kinematic viscosity of the fluid (m2 s−1) | |
| density of the fluid (kg m−3) | |
| nanoparticle volume fractions for CoFe2O4 (cobalt ferrite) | |
| nanoparticle volume fractions for MnZnFe2O4 (manganese-zinc ferrite) | |
| hybrid nanoparticle volume fractions | |
| dimensionless concentration | |
| stream function | |
| Subscripts | |
| base fluid | |
| hybrid nanofluid | |
| solid component for CoFe2O4, (cobalt ferrite) | |
| solid component for MnZnFe2O4 (manganese-zinc ferrite) | |
| Superscript | |
| differentiation with respect to | |
References
- Choi, S.U.; Eastman, J.A. Enhancing thermal conductivity of fluids with nanoparticles. In Proceedings of the 1995 International Mechanical Engineering Congress and Exhibition, San Francisco, CA, USA, 12–17 November 1995. [Google Scholar]
- Saidur, R.; Leong, K.Y.; Mohammed, H.A. A review on applications and challenges of nanofluids. Renew. Sustain. Energy Rev. 2019, 15, 1646–1668. [Google Scholar] [CrossRef]
- Motsumi, T.G.; Makinde, O.D. Effects of thermal radiation and viscous dissipation on boundary layer flow of nanofluids over a permeable moving flat plate. Phys. Scr. 2012, 86, 045003. [Google Scholar] [CrossRef]
- Sheikholeslami, M. Effect of uniform suction on nanofluid flow and heat transfer over a cylinder. J. Braz. Soc. Mech. Sci. Eng. 2015, 37, 1623–1633. [Google Scholar] [CrossRef]
- Naramgari, S.; Sulochana, C. MHD flow over a permeable stretching/shrinking sheet of a nanofluid with suction/injection. Alex. Eng. J. 2016, 55, 819–827. [Google Scholar] [CrossRef]
- Daniel, Y.S.; Aziz, Z.A.; Ismail, Z.; Salah, F. Effects of thermal radiation, viscous and Joule heating on electrical MHD nanofluid with double stratification. Chin. J. Phys. 2017, 55, 630–651. [Google Scholar] [CrossRef]
- Maleki, H.; Safaei, M.R.; Alrashed, A.A.; Kasaeian, A. Flow and heat transfer in non-Newtonian nanofluids over porous surfaces. J. Therm. Anal. Calorim. 2019, 135, 1656–1666. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Dufour and Soret effects on Al2O3-water nanofluid flow over a moving thin needle: Tiwari and Das model. Int. J. Num. Meth. Heat Fluid Flow 2020, 31, 766–782. [Google Scholar] [CrossRef]
- Shuaib, M.; Ali, A.; Khan, M.A.; Ali, A. Numerical investigation of an unsteady nanofluid flow with magnetic and suction effects to the moving upper plate. Adv. Mech. Eng. 2020, 12, 1687814020903588. [Google Scholar] [CrossRef]
- Ramzan, M.; Khan, N.S.; Kumam, P.; Khan, R. A numerical study of chemical reaction in a nanofluid flow due to rotating disk in the presence of magnetic field. Sci. Rep. 2021, 11, 19399. [Google Scholar] [CrossRef]
- Ghadikolaei, S.S.; Yassari, M.; Sadeghi, H.; Hosseinzadeh, K.; Ganji, D.D. Investigation on thermophysical properties of TiO2–Cu/H2O hybrid nanofluid transport dependent on shape factor in MHD stagnation point flow. Powder Technol. 2017, 322, 428–438. [Google Scholar] [CrossRef]
- Sundar, L.S.; Sharma, K.V.; Singh, M.K.; Sousa, A.C.M. Hybrid nanofluids preparation, thermal properties, heat transfer and friction factor—A review. Renew. Sustain. Energy Rev. 2017, 68, 185–198. [Google Scholar] [CrossRef]
- Devi, S.S.U.; Devi, S.P.A. Heat transfer enhancement of Cu-Al2O3/water hybrid nanofluid flow over a stretching sheet. J. Nigerian Mathem. Soc. 2017, 36, 419–433. [Google Scholar]
- Yousefi, R.M.; Dinarvand, S.; Yazdi, M.E.; Pop, I. Stagnation-point flow of an aqueous titania-copper hybrid nanofluid toward a wavy cylinder. Int. J. Numer. Method Heat Fluid Flow 2018, 28, 1716–1735. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Unsteady flow and heat transfer past a stretching/shrinking sheet in a hybrid nanofluid. Int. J. Heat Mass Transf. 2019, 136, 288–297. [Google Scholar] [CrossRef]
- Sreedevi, P.; Reddy, P.S.; Chamkha, A. Heat and mass transfer analysis of unsteady hybrid nanofluid flow over a stretching sheet with thermal radiation. SN Appl. Sci. 2020, 2, 1222. [Google Scholar] [CrossRef]
- Khan, U.; Ishak, A.; Zaib, A. Hybrid nanofluid flow containing single-wall and multi-wall CNTs induced by a slender stretchable sheet. Chin. J. Phys. 2021, 74, 350–364. [Google Scholar] [CrossRef]
- Wakif, A.; Chamkha, A.; Thumma, T.; Animasaun, I.L.; Sehaqui, R. Thermal radiation and surface roughness effects on the thermo-magneto-hydrodynamic stability of alumina–copper oxide hybrid nanofluids utilizing the generalized Buongiorno’s nanofluid model. J. Therm. Anal. Calorim. 2021, 143, 1201–1220. [Google Scholar] [CrossRef]
- Jyothi, A.M.; Naveen Kumar, R.; Punith Gowda, R.J.; Veeranna, Y.; Prasannakumara, B.C. Impact of activation energy and gyrotactic microorganisms on flow of Casson hybrid nanofluid over a rotating moving disk. Heat Transf. 2021, 50, 5380–5399. [Google Scholar] [CrossRef]
- Elnaqeeb, T.; Animasaun, I.L.; Shah, N.A. Ternary-hybrid nanofluids: Significance of suction and dual-stretching on three-dimensional flow of water conveying nanoparticles with various shapes and densities. Z. Naturforsch. A 2021, 76, 231–243. [Google Scholar] [CrossRef]
- Mabood, F.; Yusuf, T.A.; Khan, W.A. Cu–Al2O3–H2O hybrid nanofluid flow with melting heat transfer, irreversibility analysis and nonlinear thermal radiation. J. Therm. Anal. Calorim. 2021, 143, 973–984. [Google Scholar] [CrossRef]
- Merkin, J.H.; Pop, I.; Lok, Y.Y.; Grosan, T. Similarity Solutions for the Boundary Layer Flow and Heat Transfer of Viscous Fluids, Nanofluids, Porous Media, and Micropolar Fluids; Elsevier: Oxford, UK, 2021. [Google Scholar]
- Abel, M.S.; Khan, S.K.; Prasad, K.V. Study of visco-elastic fluid flow and heat transfer over a stretching sheet with variable viscosity. Int. J. Non-Linear Mech. 2002, 37, 81–88. [Google Scholar] [CrossRef]
- Ahmad, S.; Pop, I. Mixed convection boundary layer flow from a vertical flat plate embedded in a porous medium filled with nanofluids. Int. Commun. Heat Mass Transf. 2010, 37, 987–991. [Google Scholar] [CrossRef]
- Pal, D.; Mondal, H. Influence of chemical reaction and thermal radiation on mixed convection heat and mass transfer over a stretching sheet in Darcian porous medium with Soret and Dufour effects. Energy Convers. Manag. 2012, 62, 102–108. [Google Scholar] [CrossRef]
- Rosali, H.; Ishak, A.; Pop, I. Micropolar fluid flow towards a stretching/shrinking sheet in a porous medium with suction. Int. Commun. Heat Mass Transf. 2012, 39, 826–829. [Google Scholar] [CrossRef]
- Vyas, P.; Srivastava, N. Radiative boundary layer in porous medium due to exponentially shrinking permeable sheet. Int. Sch. Res. Not. 2012, 2012, 214362. [Google Scholar] [CrossRef]
- Pantokratoras, A. Forced convection in a Darcy–Brinkman porous medium with a convective thermal boundary condition. J. Porous Media 2015, 18, 873–878. [Google Scholar] [CrossRef]
- Kumar, R.; Sood, S. Effect of quadratic density variation on mixed convection stagnation point heat transfer and MHD fluid flow in porous medium towards a permeable shrinking sheet. J. Porous Media 2016, 19, 1083–1097. [Google Scholar] [CrossRef]
- Hussanan, A.; Salleh, M.Z.; Khan, I.; Tahar, R.M. Heat transfer in magnetohydrodynamic flow of a casson fluid with porous medium and Newtonian heating. J. Nanofluids 2017, 6, 784–793. [Google Scholar] [CrossRef]
- Hassan, M.; Marin, M.; Alsharif, A.; Ellahi, R. Convective heat transfer flow of nanofluid in a porous medium over wavy surface. Phys. Lett. A 2018, 382, 2749–2753. [Google Scholar] [CrossRef]
- Zaib, A.; Haq, R.U.; Sheikholeslami, M.; Chamkha, A.J.; Rashidi, M.M. Impact of non-Darcy medium on mixed convective flow towards a plate containing micropolar water-based TiO2 nanomaterial with entropy generation. J. Porous Media 2020, 23, 11–26. [Google Scholar] [CrossRef]
- Khan, U.; Zaib, A.; Bakar, S.A.; Roy, N.C.; Ishak, A. Buoyancy effect on the stagnation point flow of a hybrid nanofluid toward a vertical plate in a saturated porous medium. Case Stud. Therm. Eng. 2021, 27, 101342. [Google Scholar] [CrossRef]
- Tiwari, R.K.; Das, M.K. Heat transfer augmentation in a two-sided lid-driven differentially heated square cavity utilizing nanofluids. Int. J. Heat Mass Transf. 2007, 50, 2002–2018. [Google Scholar] [CrossRef]
- Kameswaran, P.K.; Makukula, Z.G.; Sibanda, P.; Motsa, S.S.; Murthy, P.V.S.N. A new algorithm for internal heat generation in nanofluid flow due to a stretching sheet in a porous medium. Int. J. Numer. Methods Heat Fluid Flow 2014, 24, 1020–1043. [Google Scholar] [CrossRef]
- Haq, R.U.; Noor, N.F.M.; Khan, Z.H. Numerical simulation of water based magnetite nanoparticles between two parallel disks. Adv. Powder Technol. 2016, 27, 1568–1575. [Google Scholar] [CrossRef]
- Ahmed, N.; Tassaddiq, A.; Alabdan, R.; Adnan; Khan, U.; Noor, S.; Mohyud-Din, S.T.; Khan, I. Applications of nanofluids for the thermal enhancement in radiative and dissipative flow over a wedge. Appl. Sci. 2019, 9, 1976. [Google Scholar] [CrossRef]
- Takabi, B.; Salehi, S. Augmentation of the heat transfer performance of a sinusoidal corrugated enclosure by employing hybrid nanofluid. Adv. Mech. Eng. 2014, 6, 147059. [Google Scholar] [CrossRef]
- Hussain, S.; Ahmed, S.E.; Akbar, T. Entropy generation analysis in MHD mixed convection of hybrid nanofluid in an open cavity with a horizontal channel containing an adiabatic obstacle. Int. J. Heat Mass Transf. 2017, 114, 1054–1066. [Google Scholar] [CrossRef]
- Waini, I.; Ishak, A.; Pop, I. Radiative and magnetohydrodynamic micropolar hybrid nanofluid flow over a shrinking sheet with Joule heating and viscous dissipation effects. Neural Comput. Appl. 2022, 34, 3783–3794. [Google Scholar] [CrossRef]
- Xu, Y.; Wang, X.; Hao, Q. A mini review on thermally conductive polymers and polymer-based composites. Compos. Commun. 2021, 24, 100617. [Google Scholar] [CrossRef]
- Fang, T.; Zhang, J.; Zhong, Y. Note on unsteady viscous flow on the outside of an expanding or contracting cylinder. Commun. Nonlinear Sci. Numer. Simulat. 2012, 12, 3124–3128. [Google Scholar] [CrossRef]
- Merkin, J.H. On dual solutions occurring in mixed convection in a porous medium. J. Eng. Math. 1986, 20, 171–179. [Google Scholar] [CrossRef]
- Weidman, P.D.; Kubitschek, D.G.; Davis, A.M.J. The effect of transpiration on self-similar boundary layer flow over moving surfaces. Int. J. Eng. Sci. 2006, 44, 730–737. [Google Scholar] [CrossRef]
- Harris, S.D.; Ingham, D.B.; Pop, I. Mixed convection boundary-layer flow near the stagnation point on a vertical surface in a porous medium: Brinkman model with slip. Transp. Porous Media 2009, 77, 267–285. [Google Scholar] [CrossRef]
- Shampine, L.F.; Gladwell, I.; Thompson, S. Solving ODEs with MATLAB; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Kameswaran, P.K.; Shaw, S.; Sibanda, P.; Murthy, P.V.S.N. Homogeneous-heterogeneous reactions in a nanofluid flow due to a porous stretching sheet. Int. J. Heat Mass Transf. 2013, 57, 465–472. [Google Scholar] [CrossRef]



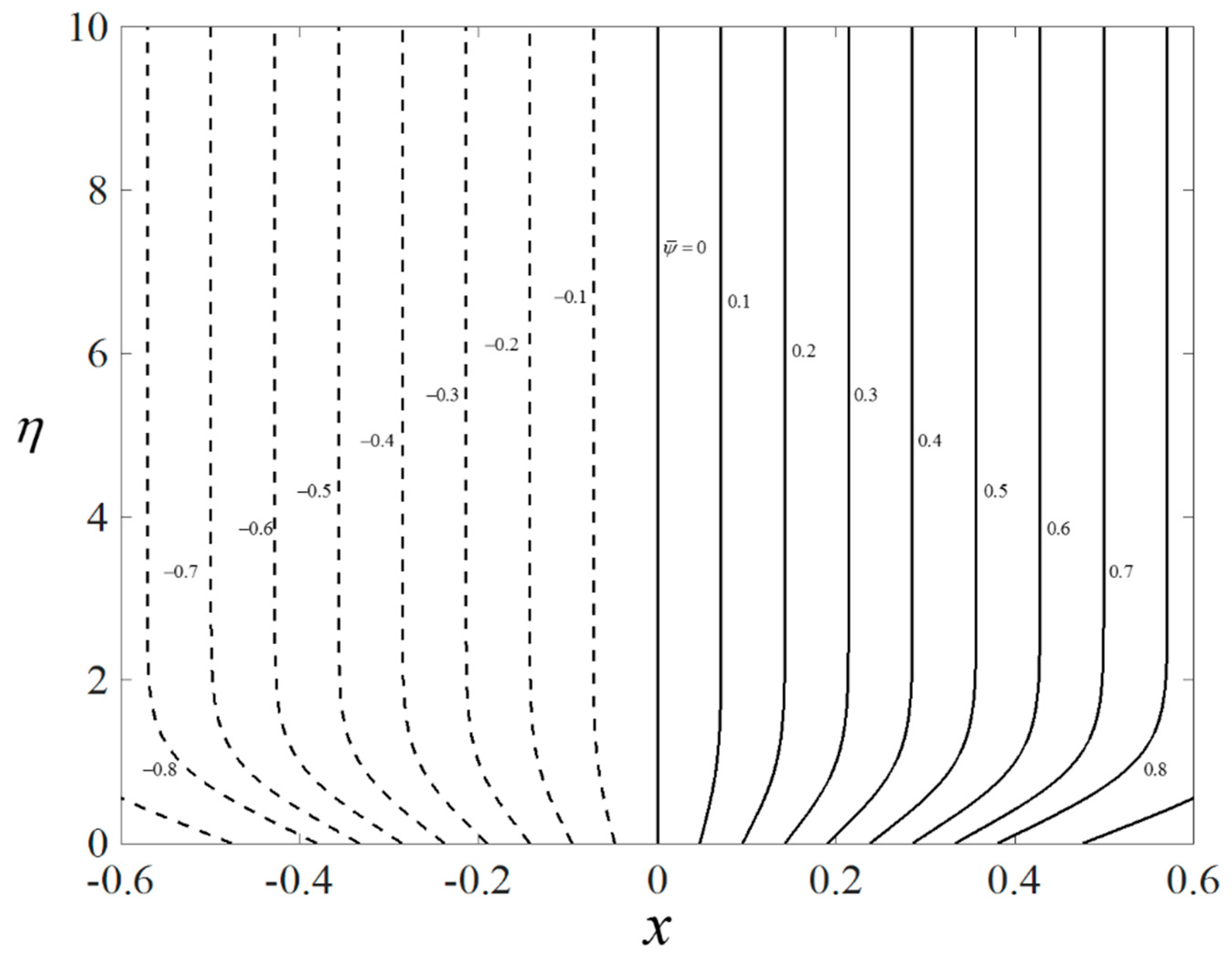

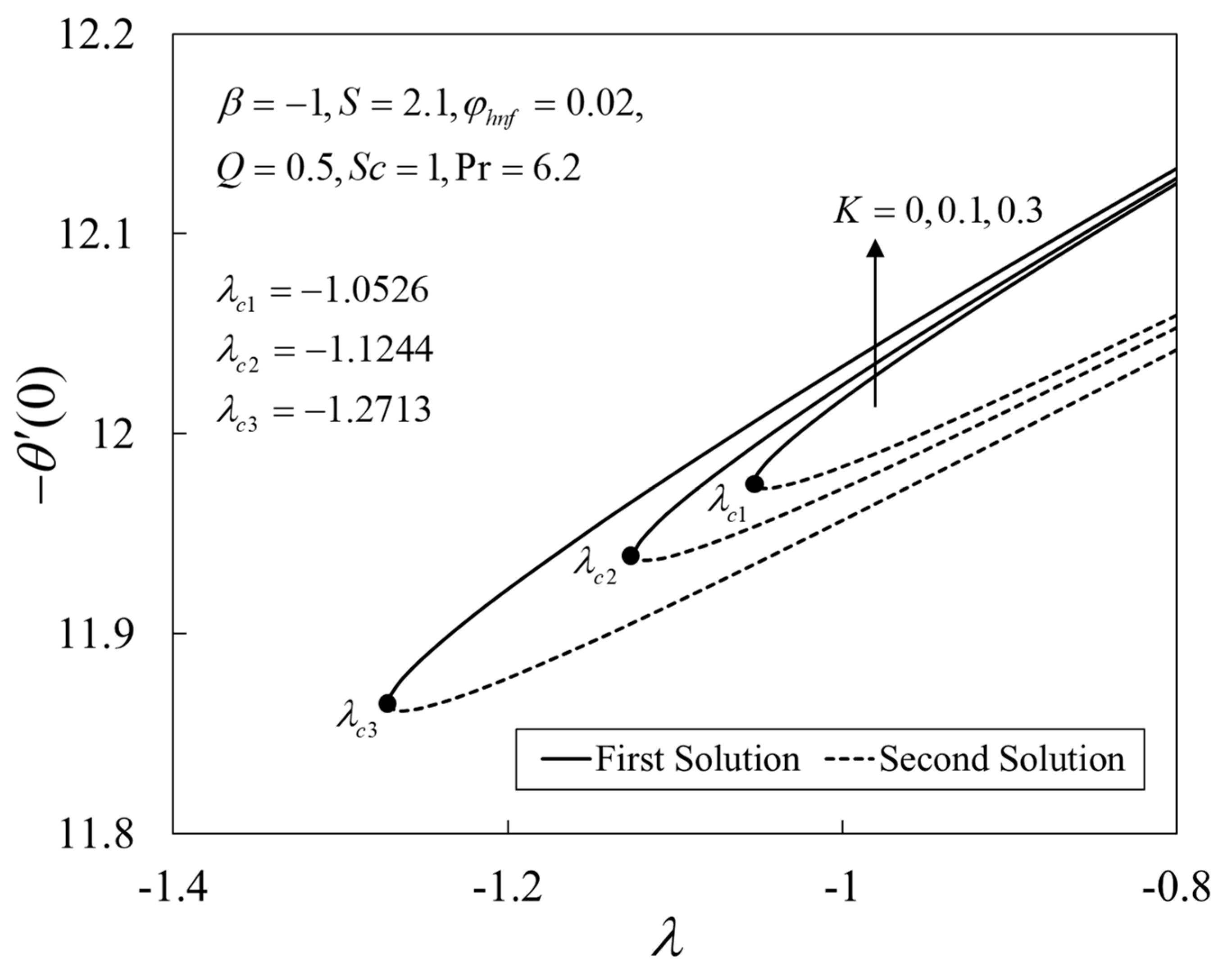
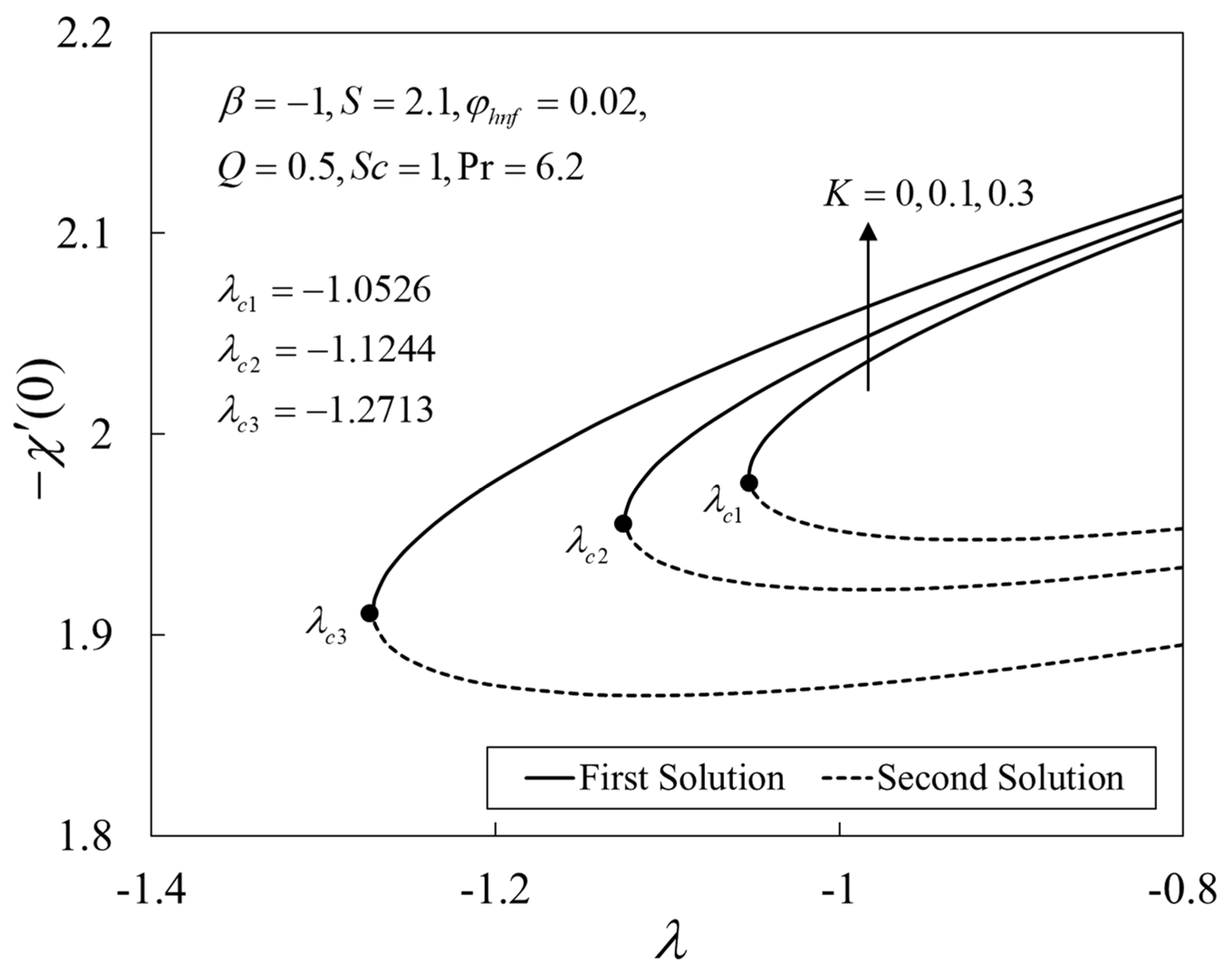
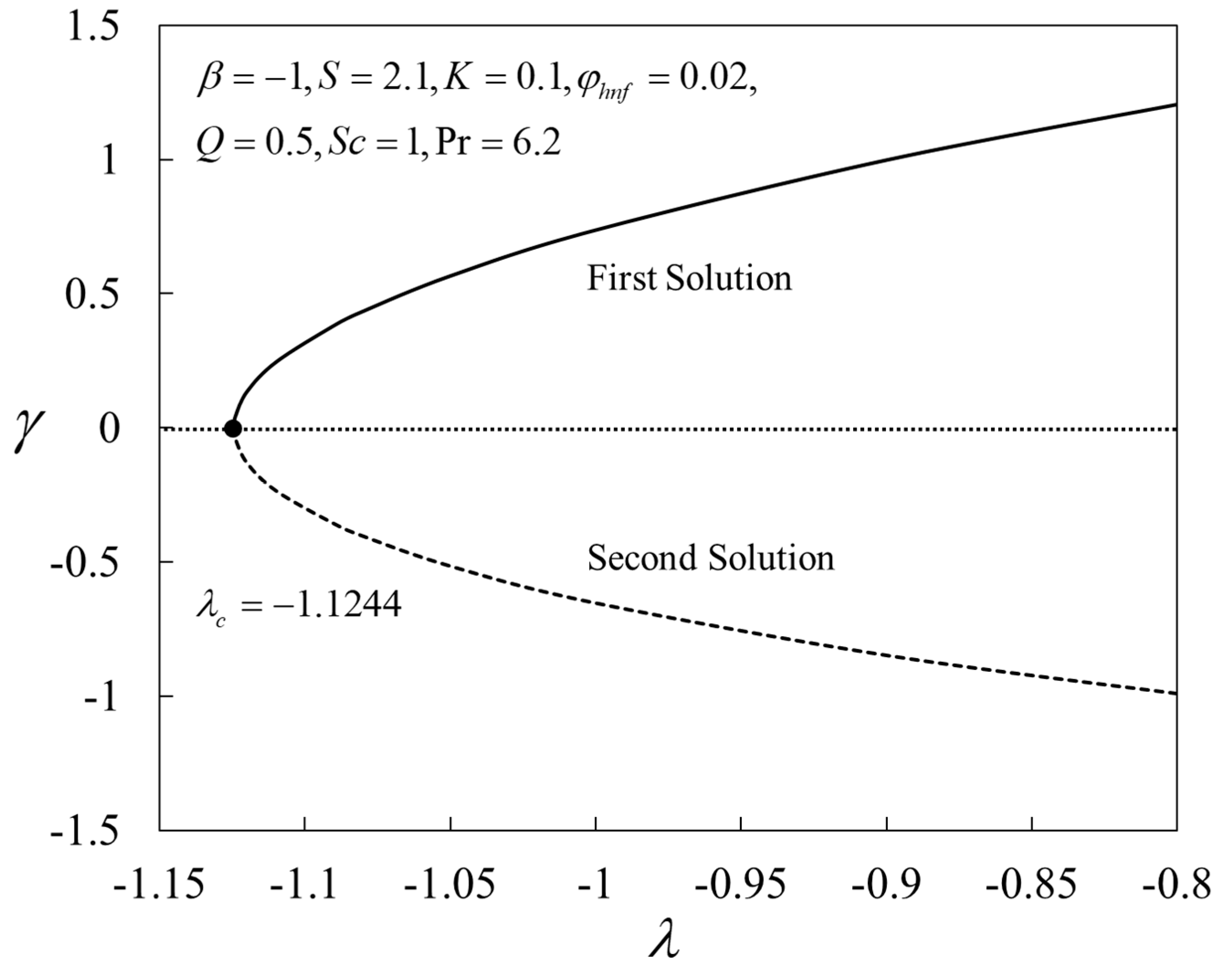
| Properties | ρ(kg/m3) | Cp(J/kg K) | k(W/mk) | Pr |
|---|---|---|---|---|
| Water | 997.1 | 4179 | 0.613 | 6.2 |
| CoFe2O4 | 4907 | 700 | 3.7 | |
| 4900 | 800 | 5 |
| Present Results | Kameswaran et al. [47] | |
|---|---|---|
| 0.5 | 1.22474487 | 1.22474487 |
| 1.0 | 1.41421356 | 1.41421356 |
| 1.5 | 1.58113883 | 1.58113883 |
| 2.0 | 1.73205081 | 1.73205081 |
| 5.0 | 2.44948974 | 2.44948974 |
| 0.00 | 0.1 | 2.1 | −1.0 | 0.5 | 1.0326 | 12.5602 | 2.0355 |
| 0.01 | 1.0759 | 12.2887 | 2.0389 | ||||
| 0.02 | 1.1129 | 12.0244 | 2.0418 | ||||
| 0.02 | 0.0 | 0.9167 | 12.0170 | 2.0277 | |||
| 0.2 | 1.2512 | 12.0295 | 2.0511 | ||||
| 0.3 | 1.3628 | 12.0335 | 2.0582 | ||||
| 0.1 | 2.15 | 1.2340 | 12.3364 | 2.0990 | |||
| 2.2 | 1.3409 | 12.6469 | 2.1546 | ||||
| 2.3 | 1.5297 | 13.2649 | 2.2630 | ||||
| 2.1 | −2.0 | 0.6321 | 12.2673 | 2.2218 | |||
| −3.0 | 0.1120 | 12.4926 | 2.3703 | ||||
| −4.0 | −0.4643 | 12.7034 | 2.4991 | ||||
| −1.0 | 0.0 | 1.1129 | 12.2791 | 2.0418 | |||
| 1.5 | 1.1129 | 11.4768 | 2.0418 | ||||
| 2.0 | 1.1129 | 11.1796 | 2.0418 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Waini, I.; Khan, U.; Zaib, A.; Ishak, A.; Pop, I.; Akkurt, N. Time-Dependent Flow of Water-Based CoFe2O4-Mn-ZnFe2O4 Nanoparticles over a Shrinking Sheet with Mass Transfer Effect in Porous Media. Nanomaterials 2022, 12, 4102. https://doi.org/10.3390/nano12224102
Waini I, Khan U, Zaib A, Ishak A, Pop I, Akkurt N. Time-Dependent Flow of Water-Based CoFe2O4-Mn-ZnFe2O4 Nanoparticles over a Shrinking Sheet with Mass Transfer Effect in Porous Media. Nanomaterials. 2022; 12(22):4102. https://doi.org/10.3390/nano12224102
Chicago/Turabian StyleWaini, Iskandar, Umair Khan, Aurang Zaib, Anuar Ishak, Ioan Pop, and Nevzat Akkurt. 2022. "Time-Dependent Flow of Water-Based CoFe2O4-Mn-ZnFe2O4 Nanoparticles over a Shrinking Sheet with Mass Transfer Effect in Porous Media" Nanomaterials 12, no. 22: 4102. https://doi.org/10.3390/nano12224102
APA StyleWaini, I., Khan, U., Zaib, A., Ishak, A., Pop, I., & Akkurt, N. (2022). Time-Dependent Flow of Water-Based CoFe2O4-Mn-ZnFe2O4 Nanoparticles over a Shrinking Sheet with Mass Transfer Effect in Porous Media. Nanomaterials, 12(22), 4102. https://doi.org/10.3390/nano12224102








