Electronic Properties of Hexagonal Graphene Quantum Rings from TAO-DFT
Abstract
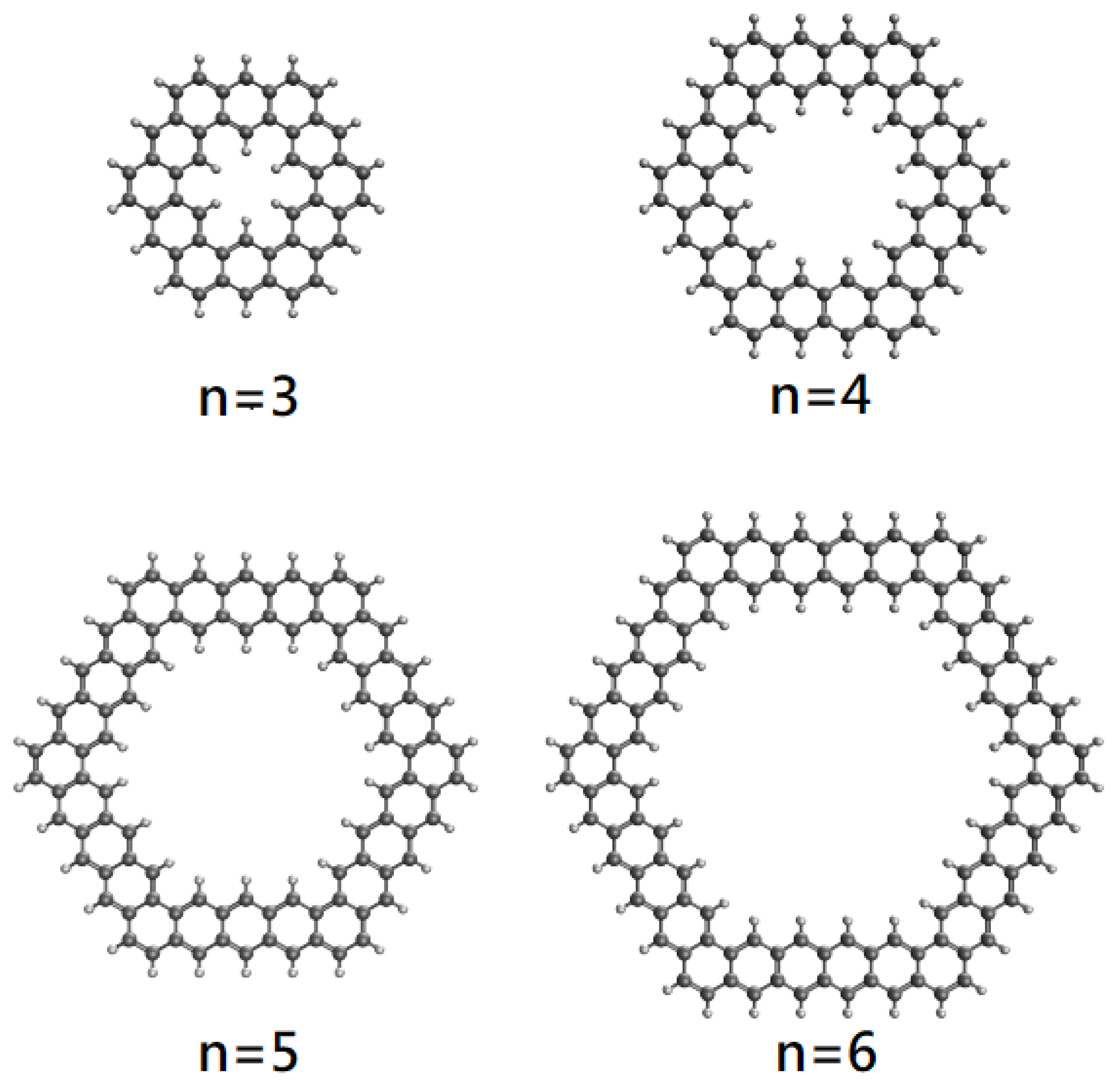
1. Introduction
2. Computational Details
3. Results and Discussion
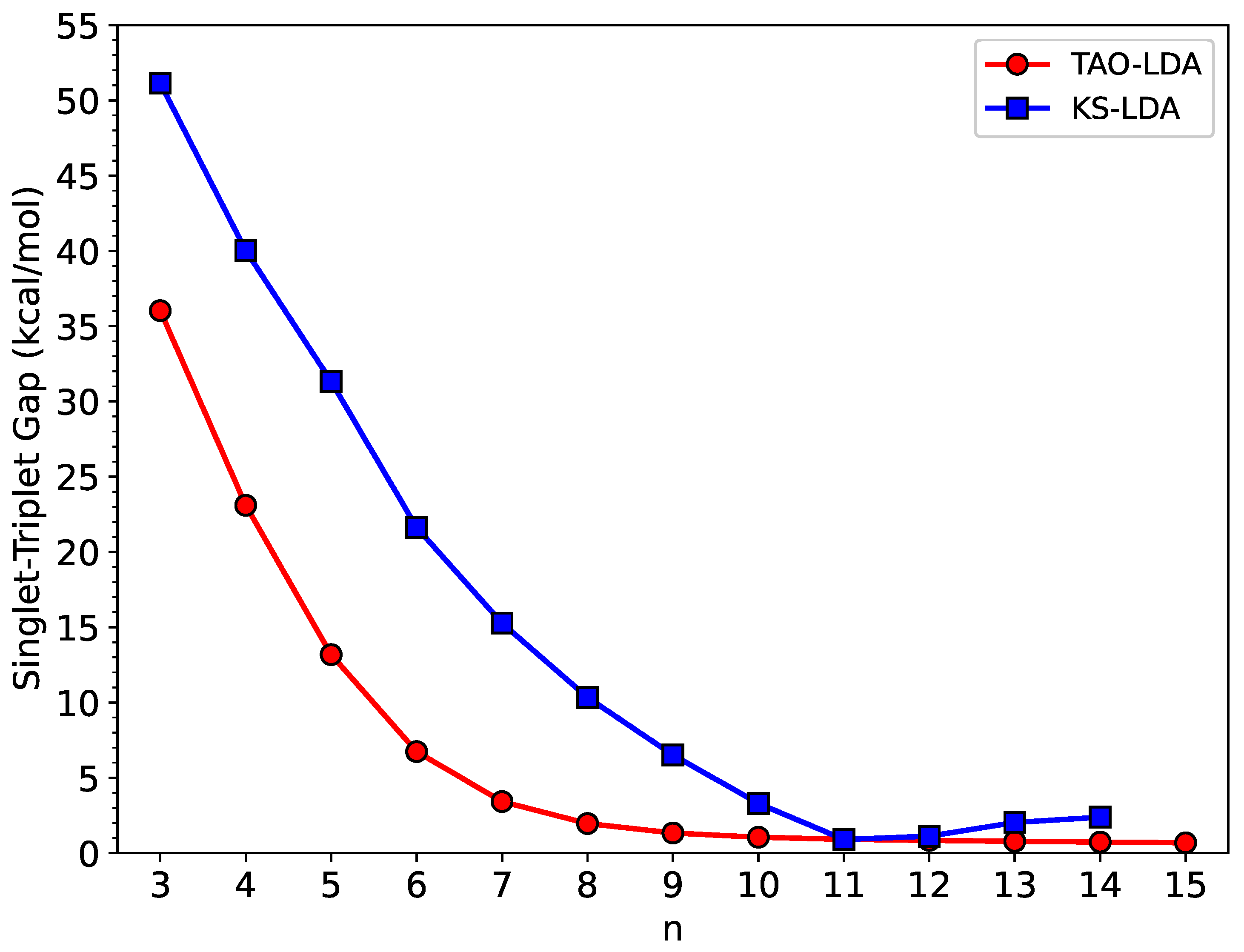
3.1. Singlet–Triplet Gap
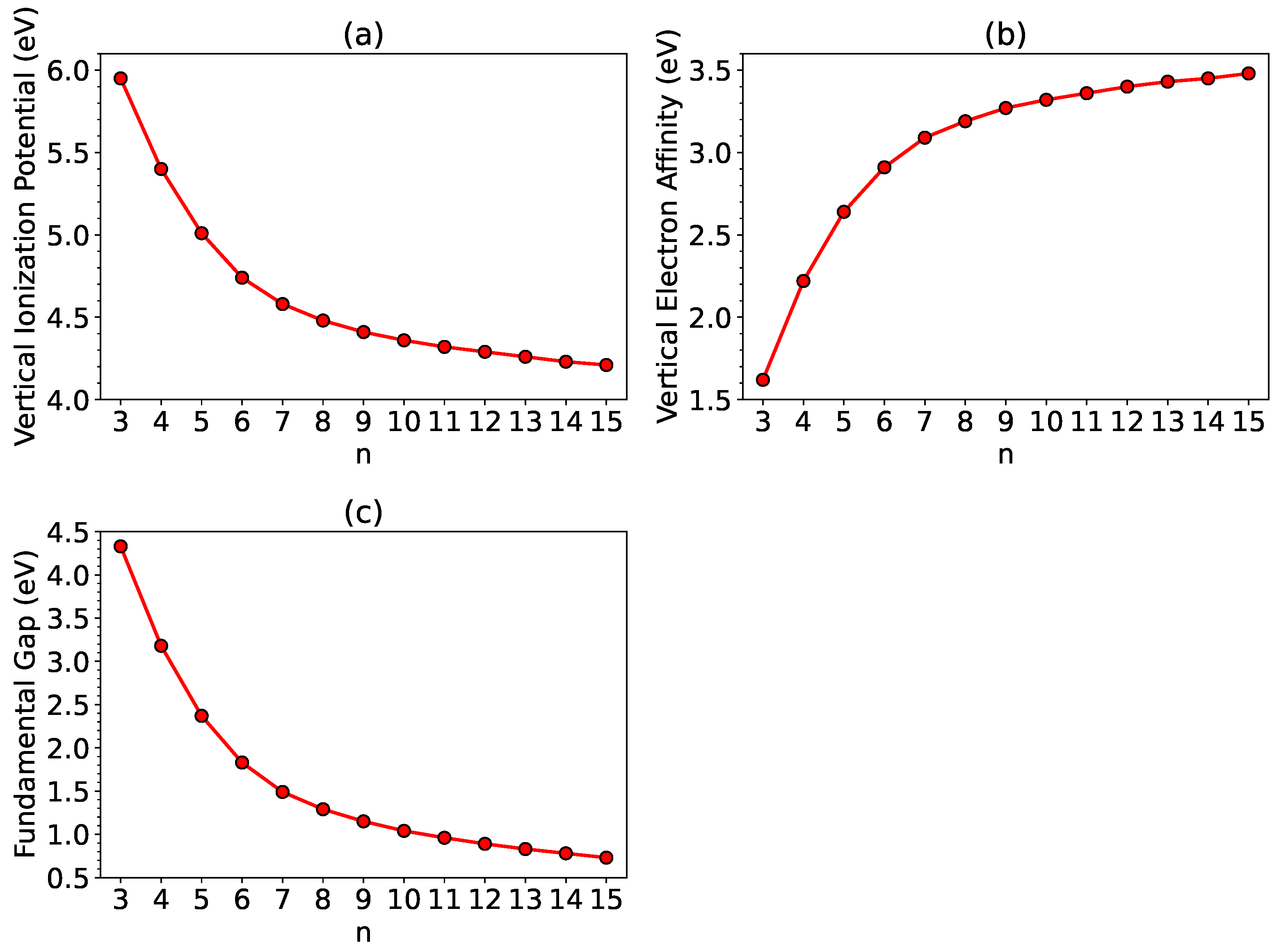
3.2. Fundamental Gap and Vertical Electron Affinity/Ionization Potential
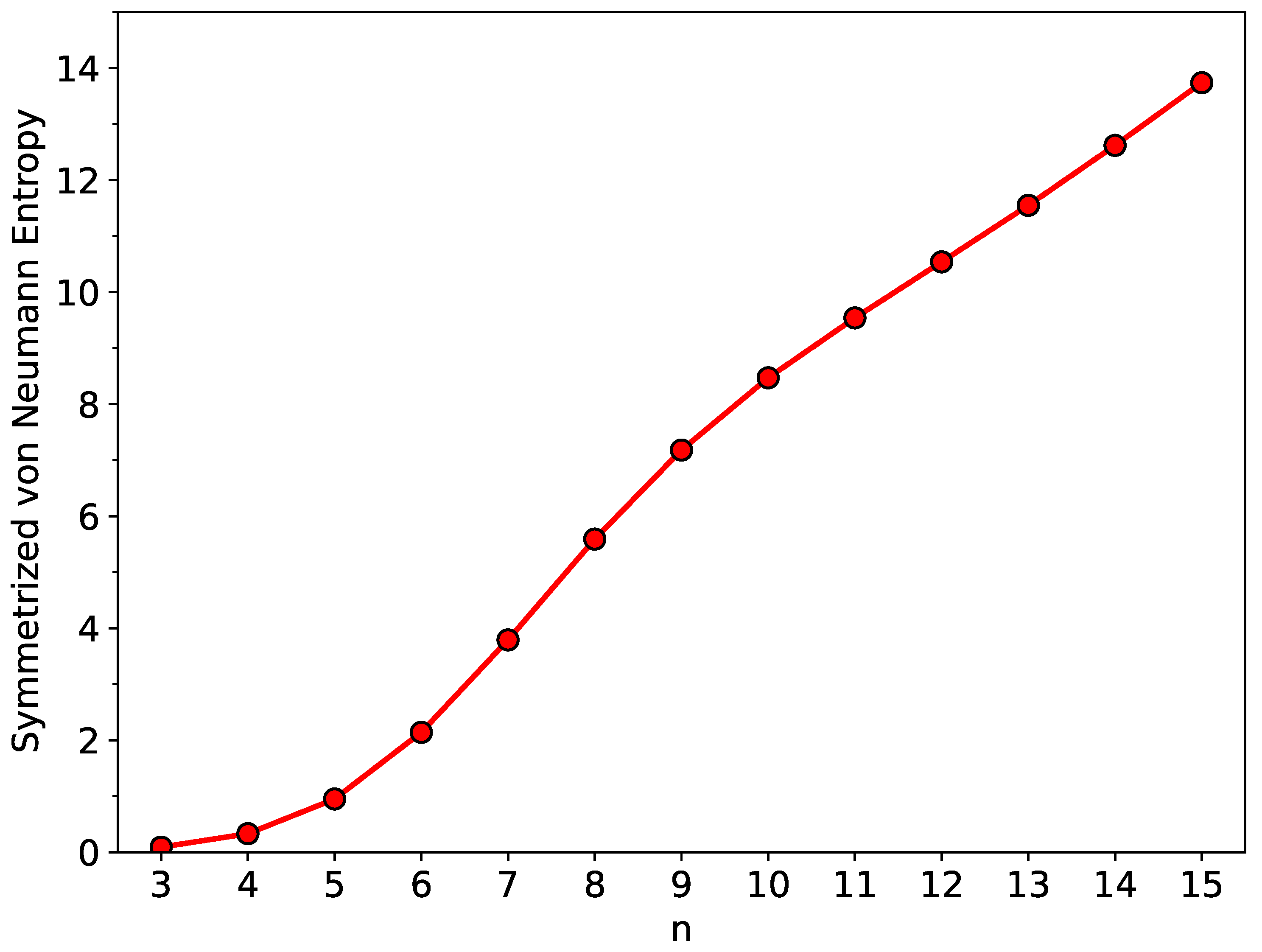
3.3. Symmetrized von Neumann Entropy
3.4. Active TAO-Orbital Occupation Numbers
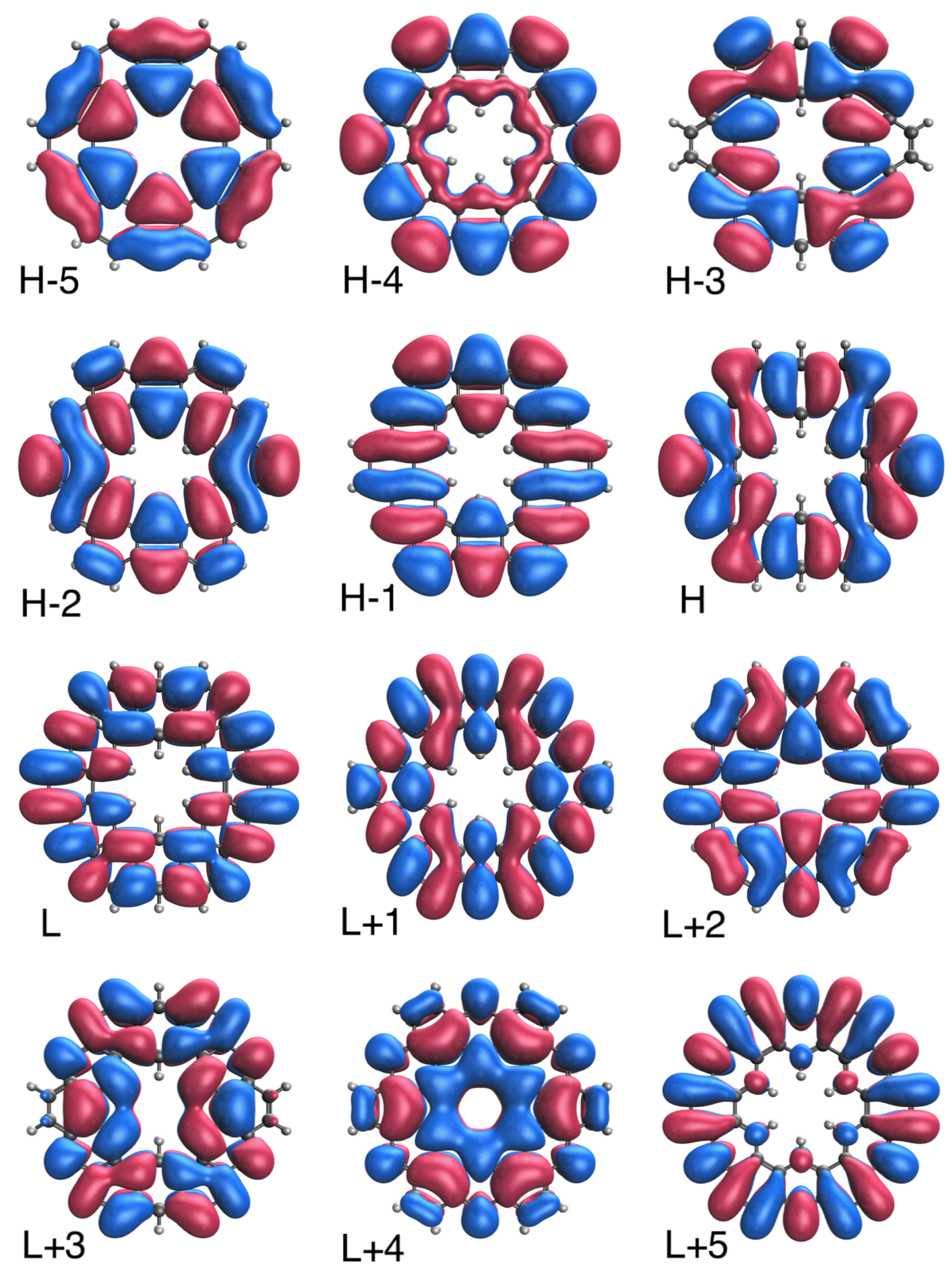
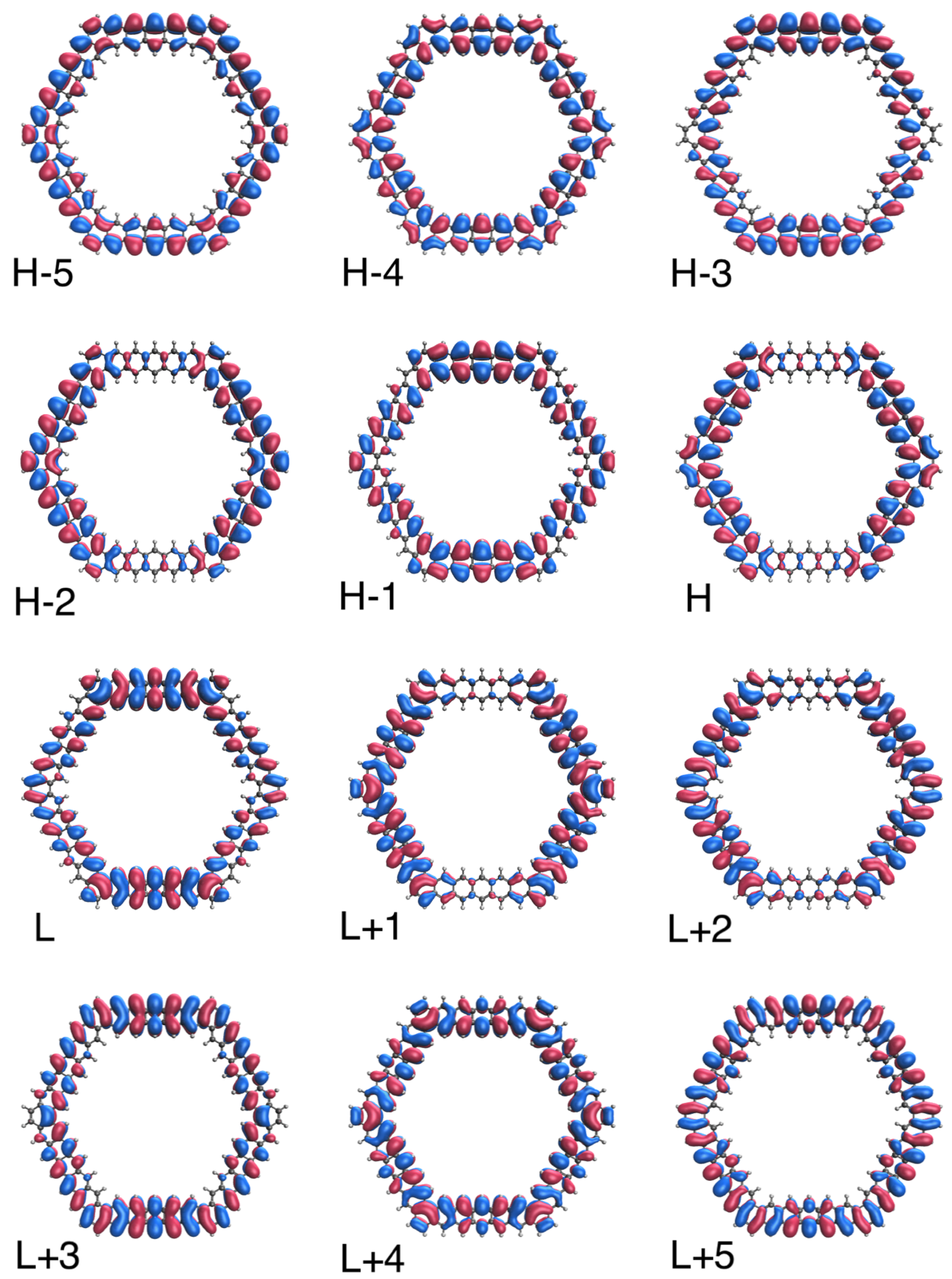
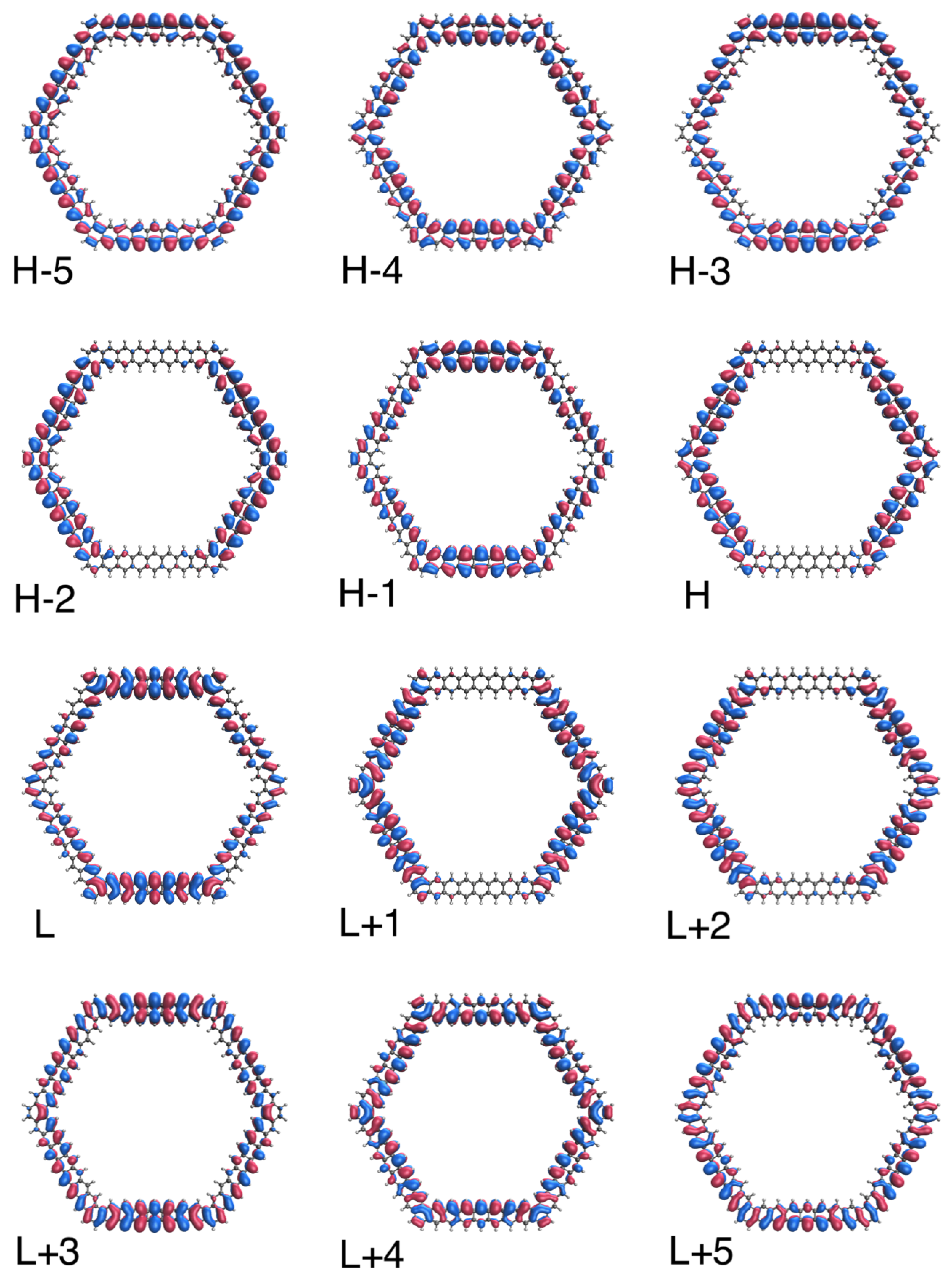
3.5. Real-Space TAO-Orbital Representation
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.E.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Katsnelson, M.I.; Grigorieva, I.; Dubonos, S.; Firsov, A. Two-dimensional gas of massless Dirac fermions in graphene. Nature 2005, 438, 197–200. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Tan, Y.W.; Stormer, H.L.; Kim, P. Experimental observation of the quantum Hall effect and Berry’s phase in graphene. Nature 2005, 438, 201–204. [Google Scholar] [CrossRef] [PubMed]
- Neto, A.C.; Guinea, F.; Peres, N.M.; Novoselov, K.S.; Geim, A.K. The electronic properties of graphene. Rev. Mod. Phys. 2009, 81, 109. [Google Scholar] [CrossRef]
- Geim, A.K. Graphene: Status and prospects. Science 2009, 324, 1530–1534. [Google Scholar] [CrossRef]
- Geim, A.K.; Novoselov, K.S. The rise of graphene. In Nanoscience and Technology: A Collection of Reviews from Nature Journals; World Scientific: Singapore, 2010; pp. 11–19. [Google Scholar]
- Stankovich, S.; Dikin, D.A.; Dommett, G.H.B.; Kohlhaas, K.M.; Zimney, E.J.; Stach, E.A.; Piner, R.D.; Nguyen, S.T.; Ruoff, R.S. Graphene-based composite materials. Nature 2006, 442, 282–286. [Google Scholar] [CrossRef]
- Nigar, S.; Zhou, Z.; Wang, H.; Imtiaz, M. Modulating the electronic and magnetic properties of graphene. RSC Adv. 2017, 7, 51546–51580. [Google Scholar] [CrossRef]
- Papageorgiou, D.G.; Kinloch, I.A.; Young, R.J. Mechanical properties of graphene and graphene-based nanocomposites. Prog. Mater. Sci. 2017, 90, 75–127. [Google Scholar] [CrossRef]
- Das, A.; Pisana, S.; Chakraborty, B.; Piscanec, S.; Saha, S.K.; Waghmare, U.V.; Novoselov, K.S.; Krishnamurthy, H.R.; Geim, A.K.; Ferrari, A.C.; et al. Monitoring dopants by Raman scattering in an electrochemically top-gated graphene transistor. Nat. Nanotechnol. 2008, 3, 210–215. [Google Scholar] [CrossRef]
- Britnell, L.; Gorbachev, R.; Jalil, R.; Belle, B.; Schedin, F.; Mishchenko, A.; Georgiou, T.; Katsnelson, M.; Eaves, L.; Morozov, S.; et al. Field-effect tunneling transistor based on vertical graphene heterostructures. Science 2012, 335, 947–950. [Google Scholar] [CrossRef]
- Stoller, M.D.; Park, S.; Zhu, Y.; An, J.; Ruoff, R.S. Graphene-based ultracapacitors. Nano Lett. 2008, 8, 3498–3502. [Google Scholar] [CrossRef] [PubMed]
- Pumera, M. Graphene-based nanomaterials for energy storage. Energy Environ. Sci. 2011, 4, 668–674. [Google Scholar] [CrossRef]
- Raccichini, R.; Varzi, A.; Passerini, S.; Scrosati, B. The role of graphene for electrochemical energy storage. Nat. Mater. 2015, 14, 271–279. [Google Scholar] [CrossRef]
- Steane, A. Quantum computing. Rep. Prog. Phys. 1998, 61, 117. [Google Scholar] [CrossRef]
- Calafell, I.A.; Cox, J.; Radonjić, M.; Saavedra, J.; de Abajo, F.G.; Rozema, L.; Walther, P. Quantum computing with graphene plasmons. NPJ Quantum Inf. 2019, 5, 1–7. [Google Scholar]
- Patel, S.K.S.; Choi, S.H.; Kang, Y.C.; Lee, J.K. Eco-Friendly Composite of Fe3O4-Reduced Graphene Oxide Particles for Efficient Enzyme Immobilization. ACS Appl. Mater. Interfaces 2017, 9, 2213–2222. [Google Scholar] [CrossRef] [PubMed]
- Otari, S.V.; Kumar, M.; Anwar, M.Z.; Thorat, N.D.; Patel, S.K.S.; Lee, D.; Lee, J.H.; Lee, J.K.; Kang, Y.C.; Zhang, L. Rapid synthesis and decoration of reduced graphene oxide with gold nanoparticles by thermostable peptides for memory device and photothermal applications. Sci. Rep. 2017, 7, 10980. [Google Scholar] [CrossRef]
- Williams, J.; DiCarlo, L.; Marcus, C. Quantum Hall effect in a gate-controlled pn junction of graphene. Science 2007, 317, 638–641. [Google Scholar] [CrossRef]
- Abanin, D.; Levitov, L. Quantized transport in graphene pn junctions in a magnetic field. Science 2007, 317, 641–643. [Google Scholar] [CrossRef]
- Özyilmaz, B.; Jarillo-Herrero, P.; Efetov, D.; Abanin, D.A.; Levitov, L.S.; Kim, P. Electronic transport and quantum Hall effect in bipolar graphene p-n-p junctions. Phys. Rev. Lett. 2007, 99, 166804. [Google Scholar] [CrossRef]
- Chen, H.; Müller, M.B.; Gilmore, K.J.; Wallace, G.G.; Li, D. Mechanically strong, electrically conductive, and biocompatible graphene paper. Adv. Mater. 2008, 20, 3557–3561. [Google Scholar] [CrossRef]
- Mak, K.F.; Sfeir, M.Y.; Wu, Y.; Lui, C.H.; Misewich, J.A.; Heinz, T.F. Measurement of the optical conductivity of graphene. Phys. Rev. Lett. 2008, 101, 196405. [Google Scholar] [CrossRef] [PubMed]
- Balandin, A.A.; Ghosh, S.; Bao, W.; Calizo, I.; Teweldebrhan, D.; Miao, F.; Lau, C.N. Superior thermal conductivity of single-layer graphene. Nano Lett. 2008, 8, 902–907. [Google Scholar] [CrossRef] [PubMed]
- Nakada, K.; Fujita, M.; Dresselhaus, G.; Dresselhaus, M.S. Edge state in graphene ribbons: Nanometer size effect and edge shape dependence. Phys. Rev. B 1996, 54, 17954. [Google Scholar] [CrossRef]
- Wakabayashi, K.; Fujita, M.; Ajiki, H.; Sigrist, M. Electronic and magnetic properties of nanographite ribbons. Phys. Rev. B 1999, 59, 8271. [Google Scholar] [CrossRef]
- Ezawa, M. Peculiar width dependence of the electronic properties of carbon nanoribbons. Phys. Rev. B 2006, 73, 045432. [Google Scholar] [CrossRef]
- Brey, L.; Fertig, H. Electronic states of graphene nanoribbons studied with the Dirac equation. Phys. Rev. B 2006, 73, 235411. [Google Scholar] [CrossRef]
- Sasaki, K.i.; Murakami, S.; Saito, R. Gauge field for edge state in graphene. J. Phys. Soc. Jpn. 2006, 75, 074713. [Google Scholar] [CrossRef]
- Son, Y.W.; Cohen, M.L.; Louie, S.G. Energy gaps in graphene nanoribbons. Phys. Rev. Lett. 2006, 97, 216803. [Google Scholar] [CrossRef]
- Fernández-Rossier, J.; Palacios, J.J. Magnetism in graphene nanoislands. Phys. Rev. Lett. 2007, 99, 177204. [Google Scholar] [CrossRef]
- Ponomarenko, L.A.; Schedin, F.; Katsnelson, M.I.; Yang, R.; Hill, E.W.; Novoselov, K.S.; Geim, A.K. Chaotic Dirac billiard in graphene quantum dots. Science 2008, 320, 356–358. [Google Scholar] [CrossRef] [PubMed]
- Hod, O.; Barone, V.; Scuseria, G.E. Half-metallic graphene nanodots: A comprehensive first-principles theoretical study. Phys. Rev. B 2008, 77, 035411. [Google Scholar] [CrossRef]
- Russo, S.; Oostinga, J.B.; Wehenkel, D.; Heersche, H.B.; Sobhani, S.S.; Vandersypen, L.M.; Morpurgo, A.F. Observation of Aharonov-Bohm conductance oscillations in a graphene ring. Phys. Rev. B 2008, 77, 085413. [Google Scholar] [CrossRef]
- Hewageegana, P.; Apalkov, V. Electron localization in graphene quantum dots. Phys. Rev. B 2008, 77, 245426. [Google Scholar] [CrossRef]
- Bahamon, D.; Pereira, A.; Schulz, P. Inner and outer edge states in graphene rings: A numerical investigation. Phys. Rev. B 2009, 79, 125414. [Google Scholar] [CrossRef]
- Downing, C.; Stone, D.; Portnoi, M. Zero-energy states in graphene quantum dots and rings. Phys. Rev. B 2011, 84, 155437. [Google Scholar] [CrossRef]
- Bacon, M.; Bradley, S.J.; Nann, T. Graphene quantum dots. Part. Part. Syst. Charact. 2014, 31, 415–428. [Google Scholar] [CrossRef]
- Recher, P.; Trauzettel, B.; Rycerz, A.; Blanter, Y.M.; Beenakker, C.; Morpurgo, A. Aharonov-Bohm effect and broken valley degeneracy in graphene rings. Phys. Rev. B 2007, 76, 235404. [Google Scholar] [CrossRef]
- Potasz, P.; Güçlü, A.; Voznyy, O.; Folk, J.; Hawrylak, P. Electronic and magnetic properties of triangular graphene quantum rings. Phys. Rev. B 2011, 83, 174441. [Google Scholar] [CrossRef]
- El Khatib, M.; Evangelisti, S.; Leininger, T.; Bendazzoli, G.L. A theoretical study of closed polyacene structures. Phys. Chem. Chem. Phys. 2012, 14, 15666–15676. [Google Scholar] [CrossRef]
- Schelter, J.; Recher, P.; Trauzettel, B. The Aharonov–Bohm effect in graphene rings. Solid State Commun. 2012, 152, 1411–1419. [Google Scholar] [CrossRef]
- Da Costa, D.; Chaves, A.; Zarenia, M.; Pereira, J., Jr.; Farias, G.; Peeters, F. Geometry and edge effects on the energy levels of graphene quantum rings: A comparison between tight-binding and simplified Dirac models. Phys. Rev. B 2014, 89, 075418. [Google Scholar] [CrossRef]
- Yan, C.H.; Wei, L.F. Size effects in Aharonov–Bohm graphene rings. J. Phys. Condens. Matter 2010, 22, 295503. [Google Scholar] [CrossRef]
- Schelter, J.; Bohr, D.; Trauzettel, B. Interplay of the Aharonov-Bohm effect and Klein tunneling in graphene. Phys. Rev. B 2010, 81, 195441. [Google Scholar] [CrossRef]
- Munárriz, J.; Domínguez-Adame, F.; Malyshev, A. Toward graphene-based quantum interference devices. Nanotechnology 2011, 22, 365201. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.; Zhang, Z.; Zhang, J.; Deng, X.; Fan, Z.; Tang, G. Electronic and magnetic properties of zigzag-edged hexagonal graphene ring nanojunctions. Carbon 2015, 94, 996–1002. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous electron gas. Phys. Rev. 1964, 136, B864. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-consistent equations including exchange and correlation effects. Phys. Rev. 1965, 140, A1133. [Google Scholar] [CrossRef]
- Hachmann, J.; Dorando, J.J.; Avilés, M.; Chan, G.K.L. The radical character of the acenes: A density matrix renormalization group study. J. Chem. Phys. 2007, 127, 134309. [Google Scholar] [CrossRef]
- Gidofalvi, G.; Mazziotti, D.A. Active-space two-electron reduced-density-matrix method: Complete active-space calculations without diagonalization of the N-electron Hamiltonian. J. Chem. Phys. 2008, 129, 134108. [Google Scholar] [CrossRef]
- Mizukami, W.; Kurashige, Y.; Yanai, T. More π electrons make a difference: Emergence of many radicals on graphene nanoribbons studied by ab initio DMRG theory. J. Chem. Theory Comput. 2013, 9, 401–407. [Google Scholar] [CrossRef] [PubMed]
- Chai, J.D. Density functional theory with fractional orbital occupations. J. Chem. Phys. 2012, 136, 154104. [Google Scholar] [CrossRef] [PubMed]
- Mermin, N. Thermal properties of the inhomogeneous electron gas. Phys. Rev. 1965, 137, A1441. [Google Scholar] [CrossRef]
- Chai, J.D. Thermally-assisted-occupation density functional theory with generalized-gradient approximations. J. Chem. Phys. 2014, 140, 18A521. [Google Scholar] [CrossRef] [PubMed]
- Chai, J.D. Role of exact exchange in thermally-assisted-occupation density functional theory: A proposal of new hybrid schemes. J. Chem. Phys. 2017, 146, 044102. [Google Scholar] [CrossRef]
- Xuan, F.; Chai, J.D.; Su, H. Local density approximation for the short-range exchange free energy functional. ACS Omega 2019, 4, 7675–7683. [Google Scholar] [CrossRef]
- Chen, B.J.; Chai, J.D. TAO-DFT fictitious temperature made simple. RSC Adv. 2022, 12, 12193–12210. [Google Scholar] [CrossRef]
- Lin, C.Y.; Hui, K.; Chung, J.H.; Chai, J.D. Self-consistent determination of the fictitious temperature in thermally-assisted-occupation density functional theory. RSC Adv. 2017, 7, 50496–50507. [Google Scholar] [CrossRef]
- Li, S.; Chai, J.D. TAO-DFT-based ab initio molecular dynamics. Front. Chem. 2020, 8, 589432. [Google Scholar] [CrossRef]
- Yeh, S.H.; Manjanath, A.; Cheng, Y.C.; Chai, J.D.; Hsu, C.P. Excitation energies from thermally assisted-occupation density functional theory: Theory and computational implementation. J. Chem. Phys. 2020, 153, 084120. [Google Scholar] [CrossRef]
- Wu, C.S.; Chai, J.D. Electronic Properties of Zigzag Graphene Nanoribbons Studied by TAO-DFT. J. Chem. Theory Comput. 2015, 11, 2003–2011. [Google Scholar] [CrossRef] [PubMed]
- Yeh, C.N.; Chai, J.D. Role of Kekulé and Non-Kekulé Structures in the Radical Character of Alternant Polycyclic Aromatic Hydrocarbons: A TAO-DFT Study. Sci. Rep. 2016, 6, 30562. [Google Scholar] [CrossRef] [PubMed]
- Seenithurai, S.; Chai, J.D. Effect of Li adsorption on the electronic and hydrogen storage properties of acenes: A dispersion-corrected TAO-DFT study. Sci. Rep. 2016, 6, 33081. [Google Scholar] [CrossRef] [PubMed]
- Wu, C.S.; Lee, P.Y.; Chai, J.D. Electronic properties of cyclacenes from TAO-DFT. Sci. Rep. 2016, 6, 37249. [Google Scholar] [CrossRef]
- Seenithurai, S.; Chai, J.D. Effect of Li Termination on the Electronic and Hydrogen Storage Properties of Linear Carbon Chains: A TAO-DFT Study. Sci. Rep. 2017, 7, 4966. [Google Scholar] [CrossRef]
- Seenithurai, S.; Chai, J.D. Electronic and Hydrogen Storage Properties of Li-Terminated Linear Boron Chains Studied by TAO-DFT. Sci. Rep. 2018, 8, 13538. [Google Scholar] [CrossRef]
- Yeh, C.N.; Wu, C.; Su, H.; Chai, J.D. Electronic properties of the coronene series from thermally-assisted-occupation density functional theory. RSC Adv. 2018, 8, 34350–34358. [Google Scholar] [CrossRef]
- Chung, J.H.; Chai, J.D. Electronic properties of Möbius cyclacenes studied by thermally-assisted-occupation density functional theory. Sci. Rep. 2019, 9, 2907. [Google Scholar] [CrossRef]
- Seenithurai, S.; Chai, J.D. Electronic properties of linear and cyclic boron nanoribbons from thermally-assisted-occupation density functional theory. Sci. Rep. 2019, 9, 12139. [Google Scholar] [CrossRef]
- Deng, Q.; Chai, J.D. Electronic properties of triangle-shaped graphene nanoflakes from TAO-DFT. ACS Omega 2019, 4, 14202–14210. [Google Scholar] [CrossRef]
- Huang, H.J.; Seenithurai, S.; Chai, J.D. TAO-DFT study on the electronic properties of diamond-shaped graphene nanoflakes. Nanomaterials 2020, 10, 1236. [Google Scholar] [CrossRef]
- Seenithurai, S.; Chai, J.D. TAO-DFT investigation of electronic properties of linear and cyclic carbon chains. Sci. Rep. 2020, 10, 13133. [Google Scholar] [CrossRef] [PubMed]
- Seenithurai, S.; Chai, J.D. Electronic properties of carbon nanobelts predicted by thermally-assisted-occupation DFT. Nanomaterials 2021, 11, 2224. [Google Scholar] [CrossRef] [PubMed]
- Hanson-Heine, M.W. Metal sandwich and ion complexes in cyclacene nanobelts. Mol. Phys. 2022, e2118187. [Google Scholar] [CrossRef]
- Hanson-Heine, M.W. Static correlation in vibrational frequencies studied using thermally-assisted-occupation density functional theory. Chem. Phys. Lett. 2020, 739, 137012. [Google Scholar] [CrossRef]
- Hanson-Heine, M.W. Static Electron Correlation in Anharmonic Molecular Vibrations: A Hybrid TAO-DFT Study. J. Phys. Chem. A 2022, 126, 7273–7282. [Google Scholar] [CrossRef] [PubMed]
- Shao, Y.; Gan, Z.; Epifanovsky, E.; Gilbert, A.T.; Wormit, M.; Kussmann, J.; Lange, A.W.; Behn, A.; Deng, J.; Feng, X.; et al. Advances in molecular quantum chemistry contained in the Q-Chem 4 program package. Mol. Phys. 2015, 113, 184–215. [Google Scholar] [CrossRef]
- Yeh, C.N.; Lee, P.Y.; Chai, J.D. Electronic and Optical Properties of the Narrowest Armchair Graphene Nanoribbons Studied by Density Functional Methods. Aust. J. Chem. 2016, 69, 960. [Google Scholar] [CrossRef]
- Perdew, J.P.; Yang, W.; Burke, K.; Yang, Z.; Gross, E.K.; Scheffler, M.; Scuseria, G.E.; Henderson, T.M.; Zhang, I.Y.; Ruzsinszky, A.; et al. Understanding band gaps of solids in generalized Kohn–Sham theory. Proc. Natl. Acad. Sci. USA 2017, 114, 2801–2806. [Google Scholar] [CrossRef]
- Takatsuka, K.; Fueno, T.; Yamaguchi, K. Distribution of odd electrons in ground-state molecules. Theor. Chim. Acta 1978, 48, 175–183. [Google Scholar] [CrossRef]
- Head-Gordon, M. Characterizing unpaired electrons from the one-particle density matrix. Chem. Phys. Lett. 2003, 372, 508–511. [Google Scholar] [CrossRef]
- Grimme, S.; Hansen, A. A Practicable Real-Space Measure and Visualization of Static Electron-Correlation Effects. Angew. Chem. Int. Ed. 2015, 54, 12308–12313. [Google Scholar] [CrossRef] [PubMed]


| n | Singlet | Triplet |
|---|---|---|
| 3 | 0.0000 | 2.0047 |
| 4 | 0.0000 | 2.0040 |
| 5 | 0.0000 | 2.0028 |
| 6 | 0.0000 | 2.0054 |
| 7 | 0.0000 | 2.0068 |
| 8 | 0.0000 | 2.0101 |
| 9 | 0.0001 | 2.0271 |
| 10 | 0.0004 | 2.8843 |
| 11 | 4.1432 | 5.1379 |
| 12 | 5.1804 | 6.2166 |
| 13 | 4.8429 | 5.9705 |
| 14 | 3.8338 | 5.0410 |
| n | KS-LDA | TAO-LDA |
|---|---|---|
| 3 | 0.000 | 0.000 |
| 4 | 0.000 | 0.000 |
| 5 | 0.000 | 0.000 |
| 6 | 0.000 | 0.000 |
| 7 | 0.000 | 0.000 |
| 8 | 0.000 | 0.000 |
| 9 | 0.000 | 0.000 |
| 10 | 0.000 | 0.000 |
| 11 | 1.609 | 0.000 |
| 12 | 7.633 | 0.000 |
| 13 | 5.261 | 0.000 |
| 14 | 2.489 | 0.000 |
| 15 | 0.000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, C.-C.; Chai, J.-D. Electronic Properties of Hexagonal Graphene Quantum Rings from TAO-DFT. Nanomaterials 2022, 12, 3943. https://doi.org/10.3390/nano12223943
Chen C-C, Chai J-D. Electronic Properties of Hexagonal Graphene Quantum Rings from TAO-DFT. Nanomaterials. 2022; 12(22):3943. https://doi.org/10.3390/nano12223943
Chicago/Turabian StyleChen, Chi-Chun, and Jeng-Da Chai. 2022. "Electronic Properties of Hexagonal Graphene Quantum Rings from TAO-DFT" Nanomaterials 12, no. 22: 3943. https://doi.org/10.3390/nano12223943
APA StyleChen, C.-C., & Chai, J.-D. (2022). Electronic Properties of Hexagonal Graphene Quantum Rings from TAO-DFT. Nanomaterials, 12(22), 3943. https://doi.org/10.3390/nano12223943







