Heat Transfer of Magnetohydrodynamic Stratified Dusty Fluid Flow through an Inclined Irregular Porous Channel
Abstract
1. Introduction
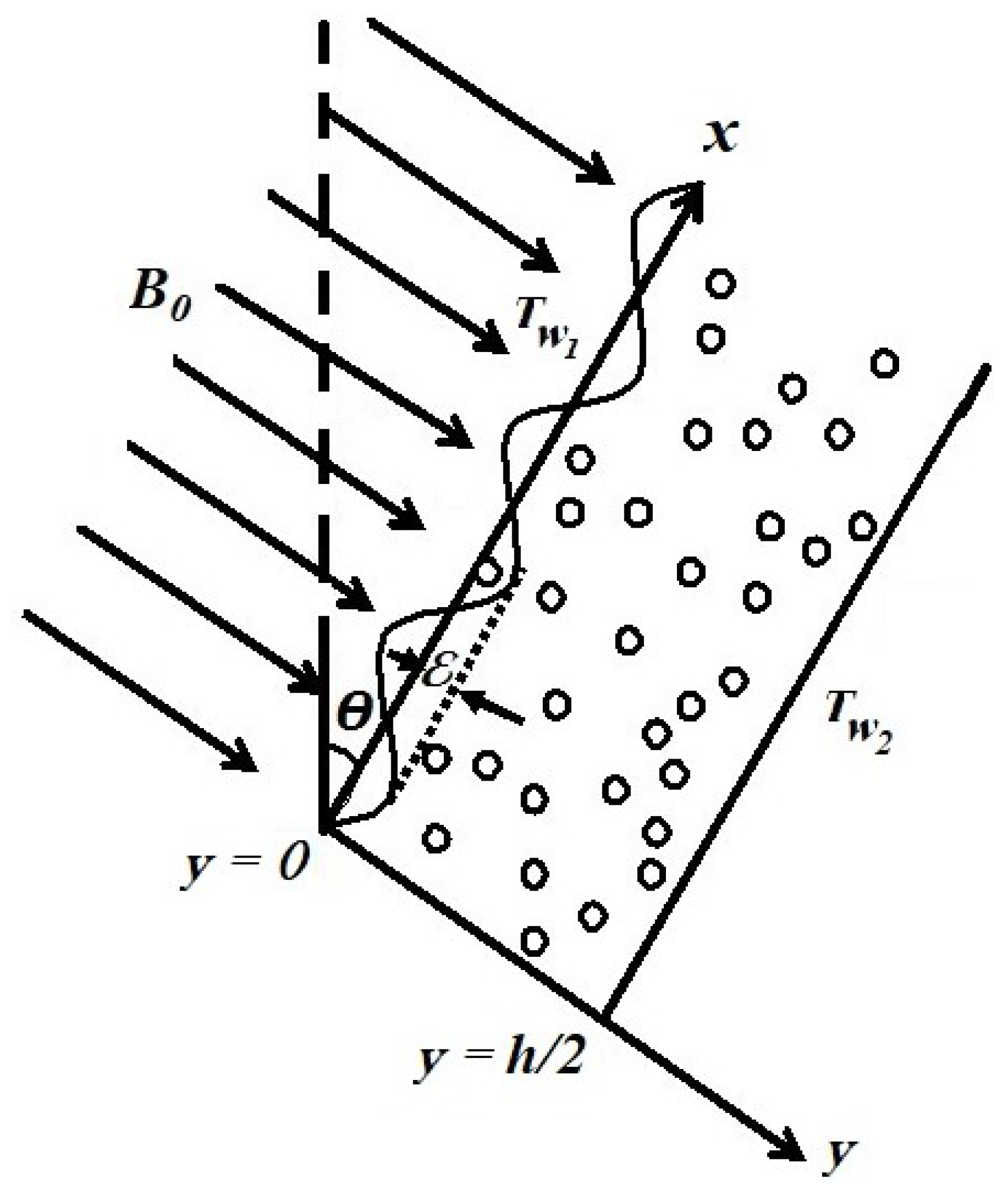
2. Mathematical Model
- The dust particles are distributed uniformly throughout the fluid flow.
- The number density of dust particles (N) is fixed.
- The dust particles are solid sphere shaped, identical and symmetrical in size.
- The nature of dust particles is elastic and electrically non-conducting.
- Except fluid density and viscosity all other physical properties are constant.
- i.
- when , , , for
- ii.
- when , , , for
- i.
- when , , , for
- ii.
- when , , , for
- i.
- when for
- ii.
- when
- i.
- when for
- ii.
- when , ,
3. Numerical Solution
- i.
- when , ,
- ii.
- when
- i.
- when ,
- ii.
- when
3.1. Skin-Friction ()
3.2. Nusselt Number (Nu)
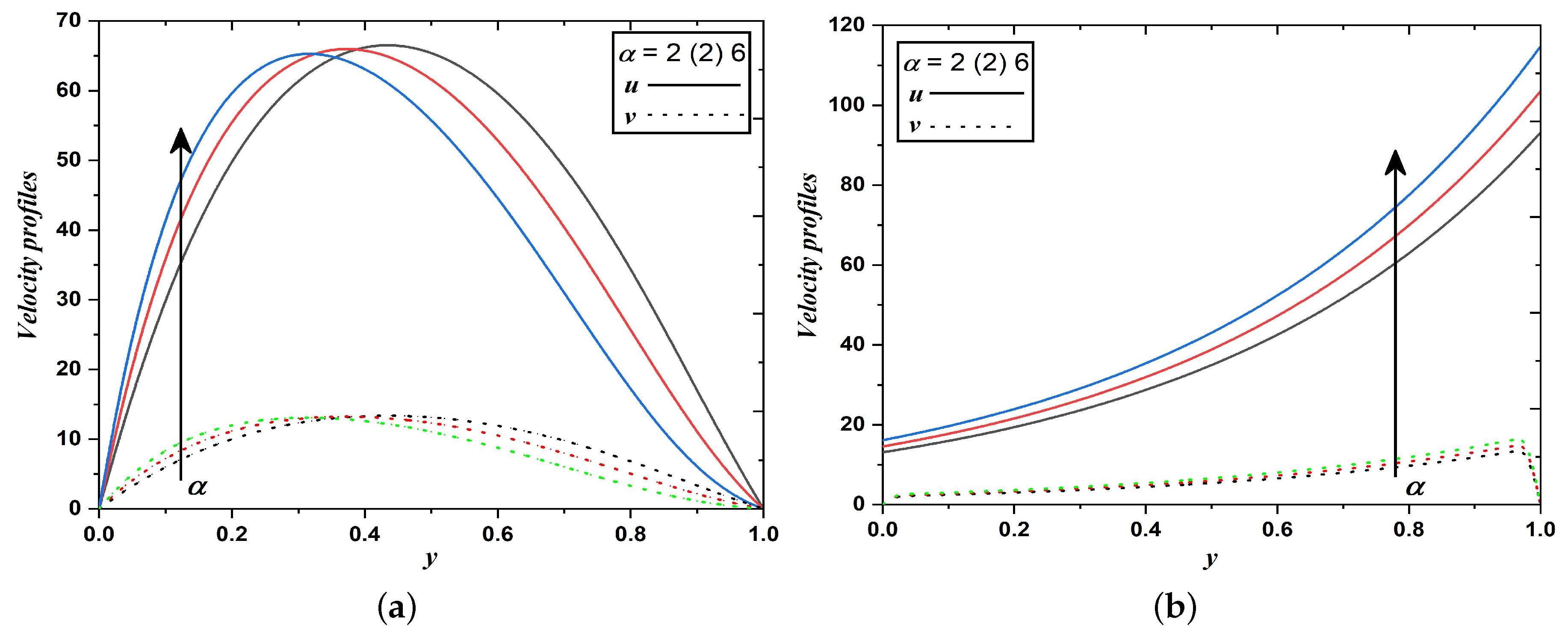
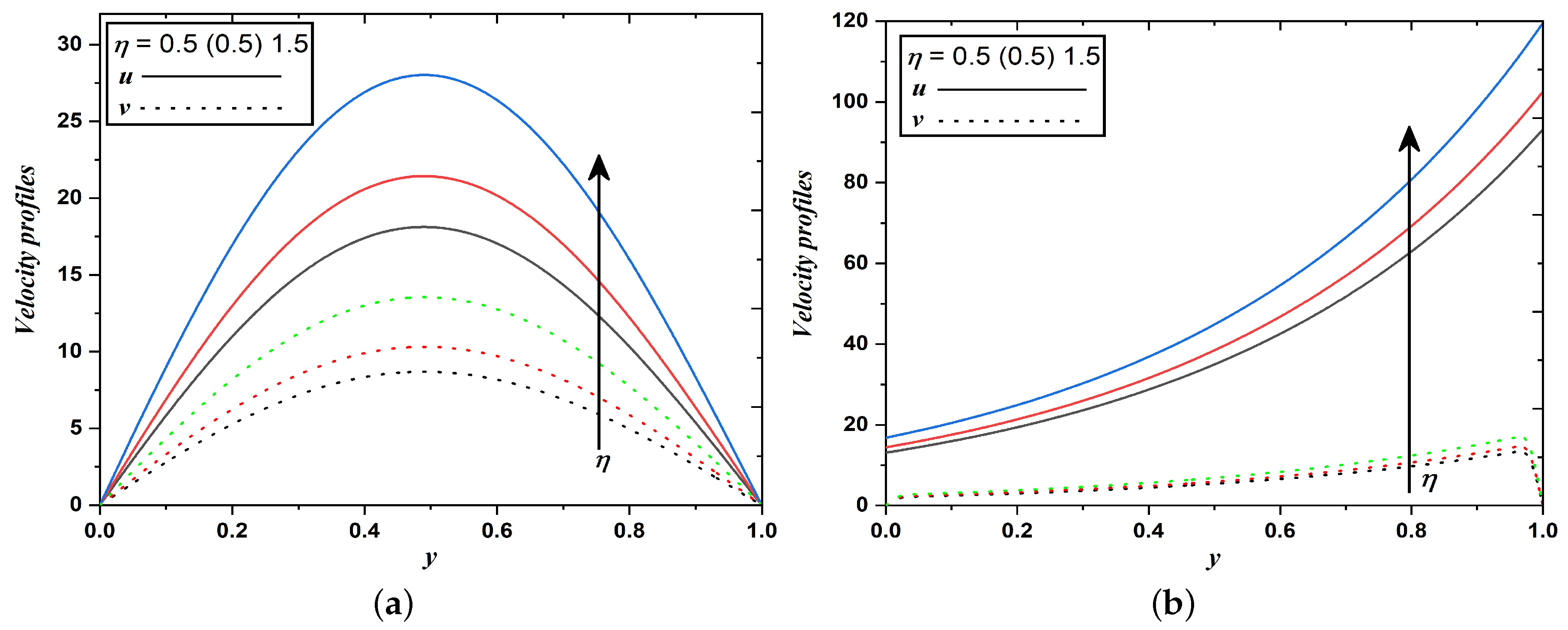
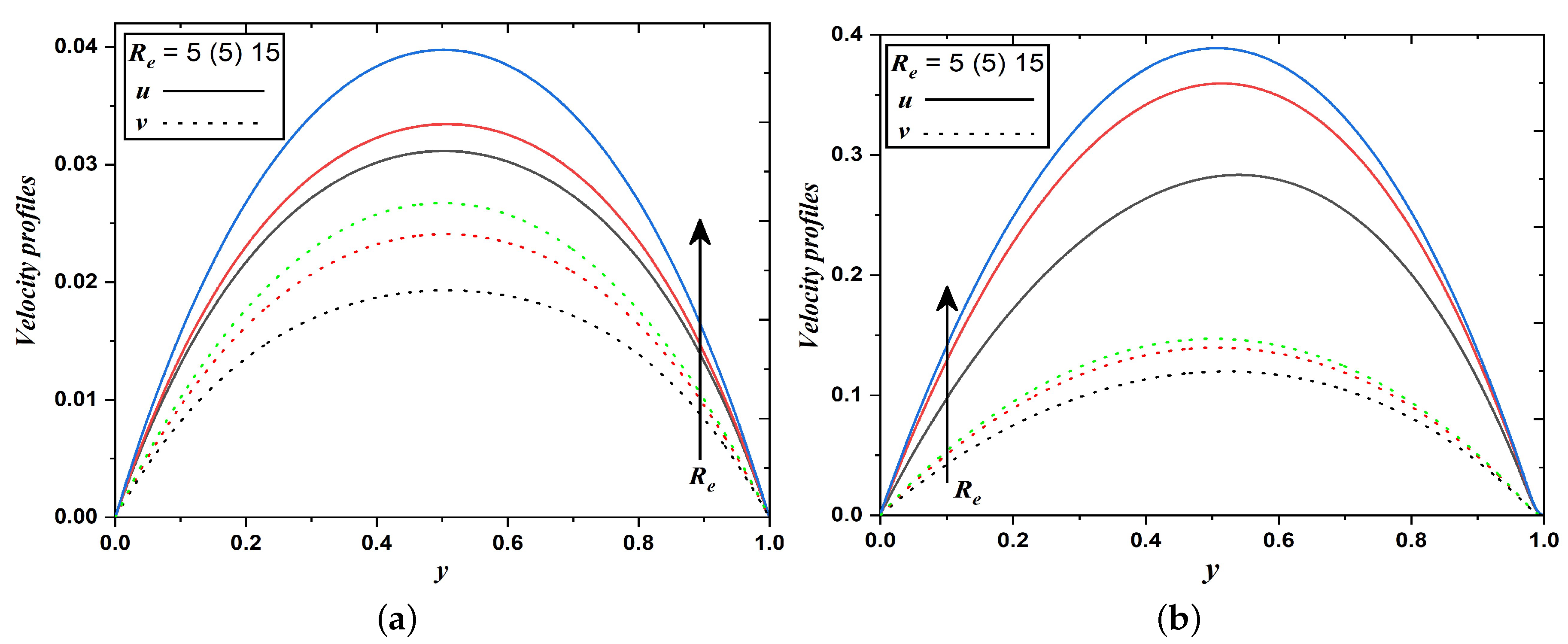
4. Results and Description
5. Conclusions
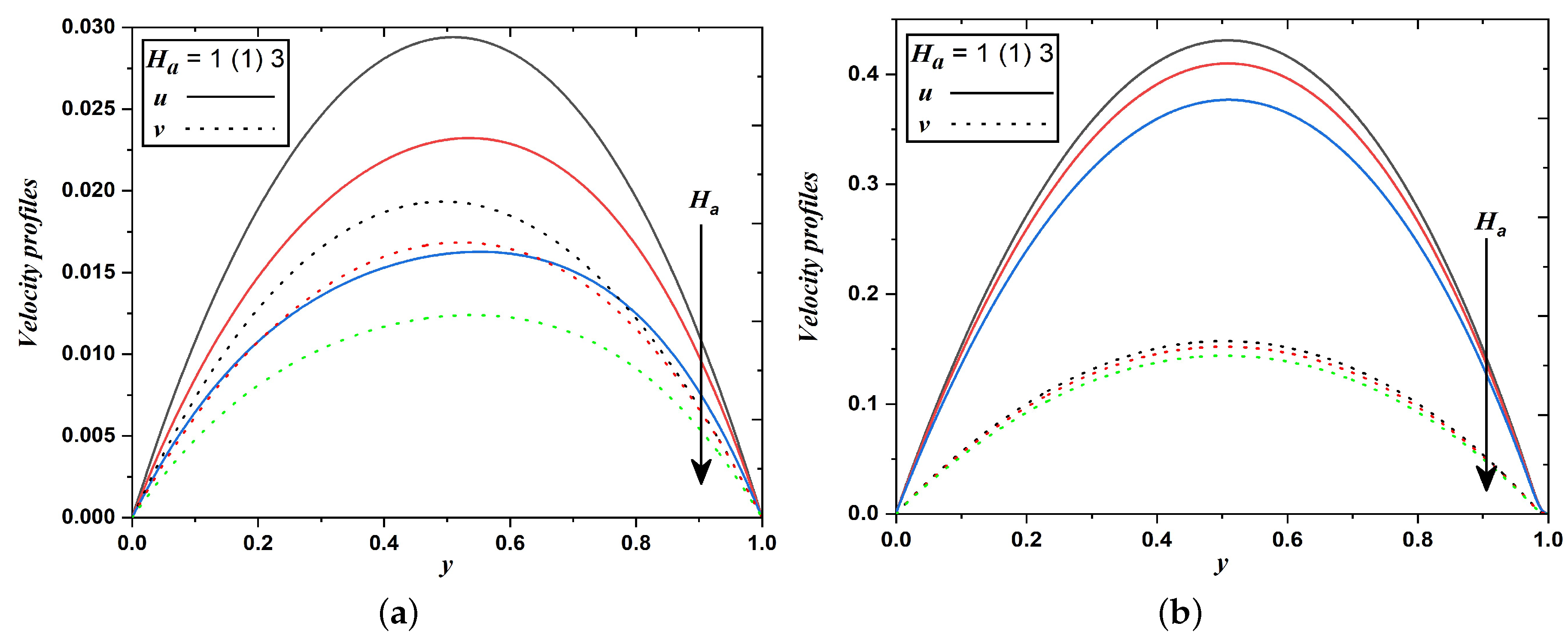
- The dusty fluid flow approaches high velocity when the stratified decay parameter, Reynolds number, and permeability of porous medium increase, whereas the effect is contrary for Hartmann number.
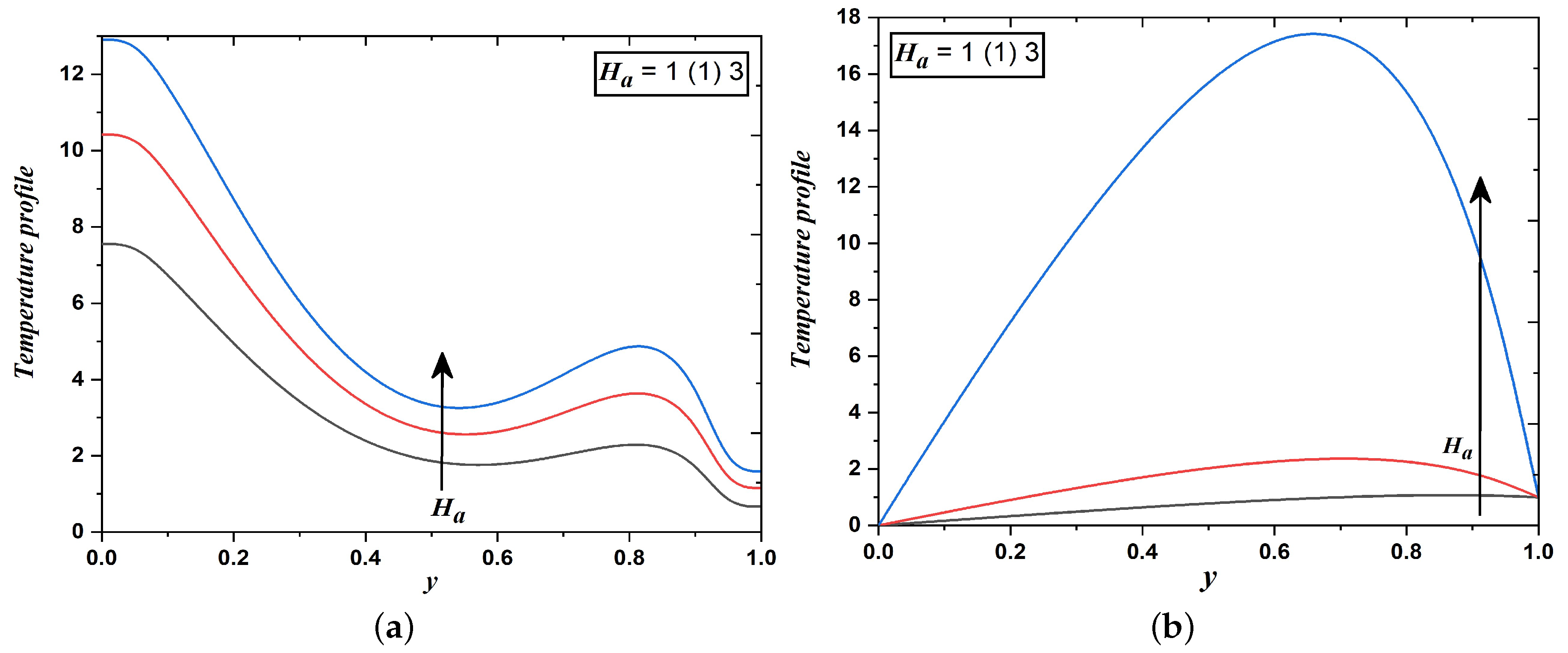
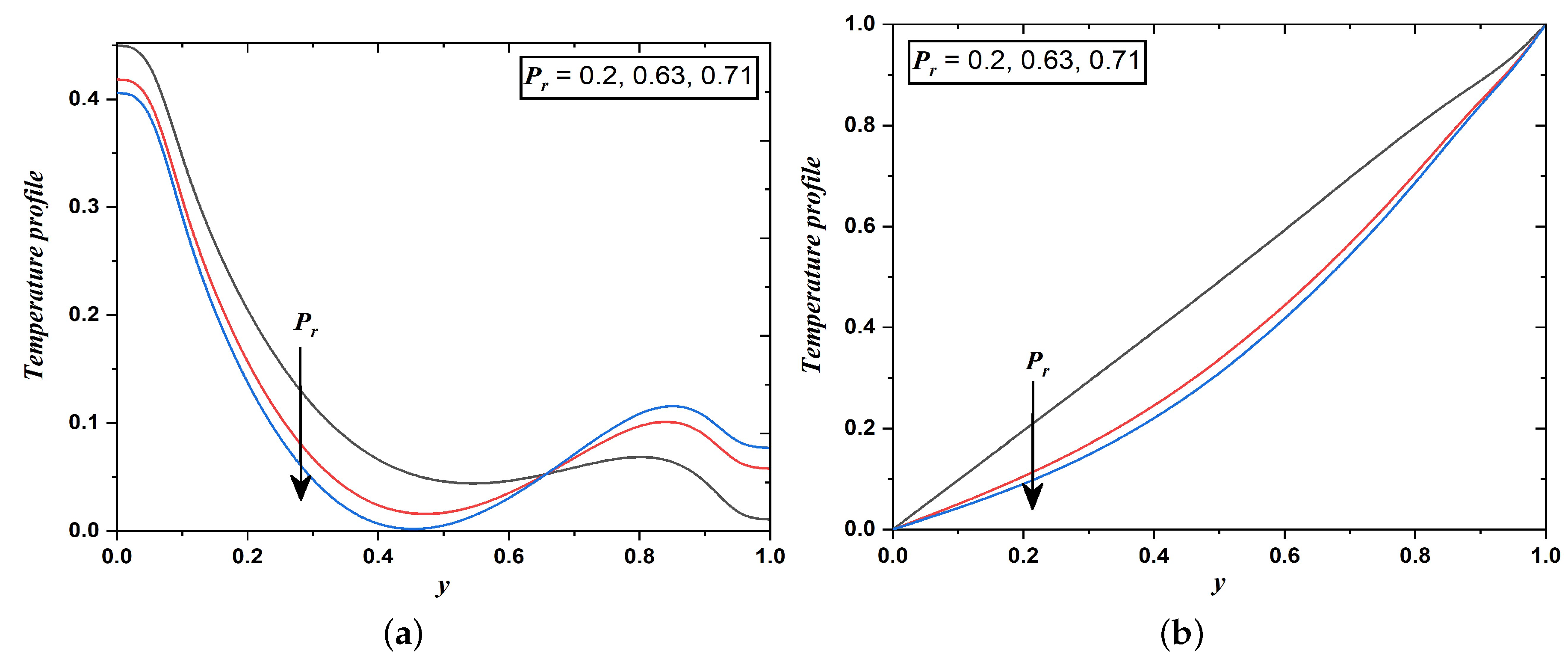
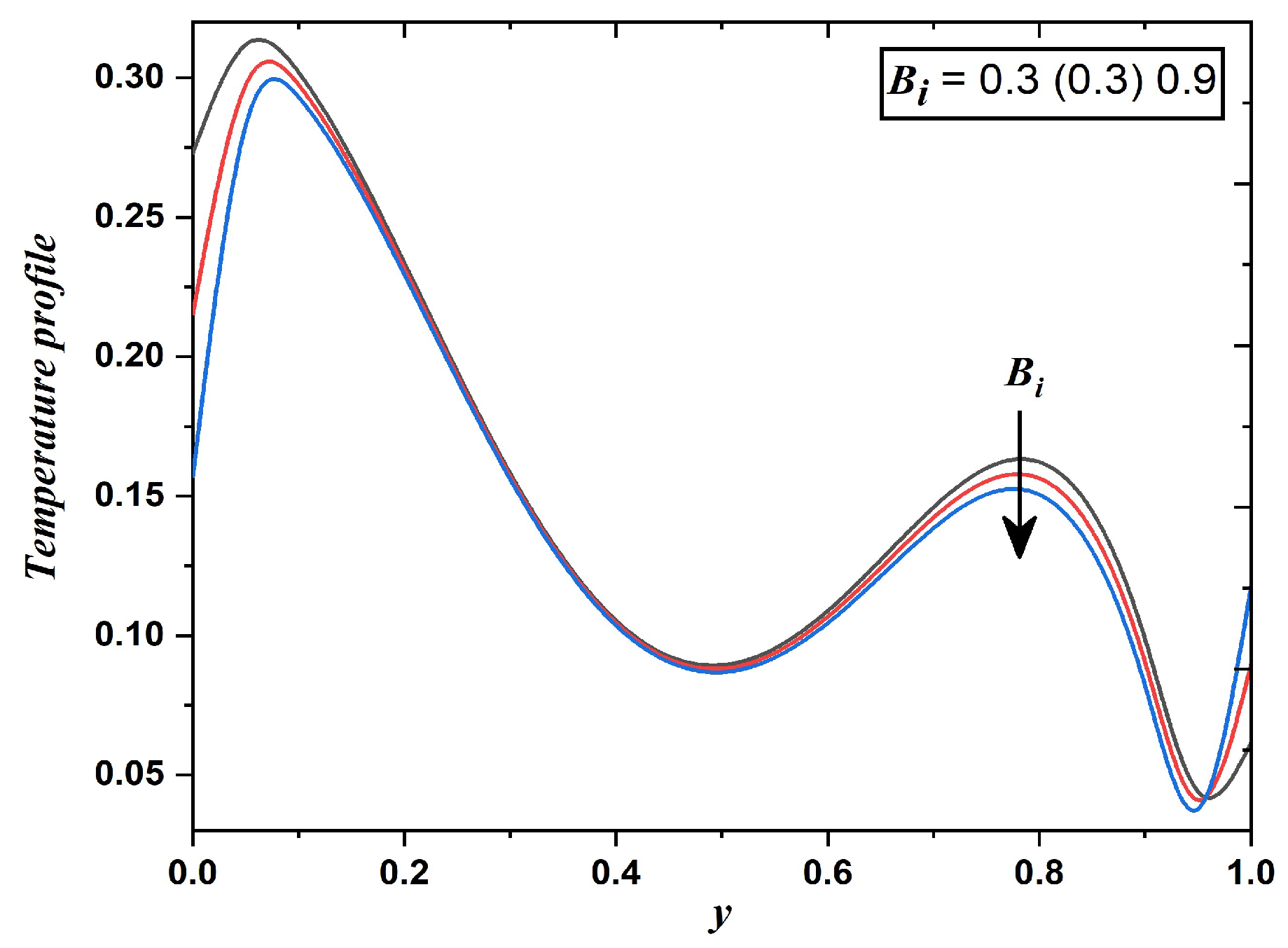
- Noticeable enhancement in temperature profile is observed for raising values of Eckert number, Hartmann number and reducing the value of Prandtl number and Biot number.
- Velocity fields of dusty fluid phase in case 1 is comparatively higher than in case 2 irrespective of variation in flow pertinent parameters.
- Temperature distribution is found to be more in the case of convective boundary than in Navier slip boundary, i.e., case 2.
- Increment in stratified decay parameter, Reynold number, and permeability of porosity yield reduction in friction factor, and the opposite effect is experienced with increment in Hartmann number.
- The heat transfer rate is magnified by boosting the values of , number, and and by reducing the magnitude of .
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| u | fluid velocity |
| v | dust velocity |
| fluid density | |
| t | time |
| co-ordinate axes | |
| T | fluid temperature |
| wall temperature | |
| viscoelasticity | |
| specific heat at fixed pressure | |
| permeability of the porous medium | |
| m | mass per unit volume of the dust particles |
| kinematic viscosity | |
| wave number | |
| viscoelastic parameter | |
| relaxation time parameter | |
| Prandtl number | |
| Biot number | |
| heat transfer coefficient | |
| p | fluid pressure |
| h | width of the channel |
| coefficient of thermal conductivity of the fluid | |
| A | positive real constant |
| k | stokes resistance coefficient |
| local temperature | |
| g | acceleration due to gravity |
| stratification decay parameter | |
| amplitude parameter | |
| viscosity of the fluid | |
| porous parameter | |
| Reynolds number | |
| Hartmann number | |
| l | mass concentration of dust particle |
| Eckert number |
References
- Saffman, P.G. On the stability of laminar flow of a dusty gas. J. Fluid Mech. 1962, 13, 120–129. [Google Scholar] [CrossRef]
- Gireesha, B.J.; Madhura, K.R.; Bagewadi, C.S. Flow of an unsteady dusty fluid through porous media in a uniform pipe with sector of a circle as cross-section. Int. J. Pure Appl. Math. 2012, 76, 29–47. [Google Scholar]
- Madhura, K.R.; Swetha, D.S.; Iyengar, S.S. The impact of Beltrami effect on dusty fluid flow through hexagonal channel in presence of porous medium. Appl. Math. Comput. 2017, 313, 342–354. [Google Scholar] [CrossRef]
- Madhura, K.R.; Swetha, D.S. Influence of volume fraction of dust particles on dusty fluid flow through porous rectangular channel. Int. J. Math. Trends Technol. 2017, 50, 261–275. [Google Scholar]
- Hamdan, M.H.; Barron, R.M. A dusty gas flow model in porous media. J. Comput. Appl. Math. 1990, 30, 21–37. [Google Scholar] [CrossRef]
- Wang, J.; Khan, M.I.; Khan, W.A.; Abbas, S.Z.; Khan, M.I. Transportation of heat generation/absorption and radiative heat flux in homogeneous—Heterogeneous catalytic reactions of non-Newtonian fluid (Oldroyd-B model). Comput. Methods Programs Biomed. 2020, 189, 105310. [Google Scholar] [CrossRef] [PubMed]
- Turkyilmazoglu, M. Slip flow and heat transfer over a specific wedge: An exactly solvable Falkner—Skan equation. J. Eng. Math. 2015, 92, 73–81. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Heat transfer enhancement feature of the non-Fourier Cattaneo—Christov heat flux model. J. Heat Transfer 2021, 143, 094501. [Google Scholar] [CrossRef]
- Hayat, T.; Tamoor, M.; Khan, M.I.; Alsaedi, A. Numerical simulation for nonlinear radiative flow by convective cylinder. Results Phys. 2016, 6, 1031–1035. [Google Scholar] [CrossRef]
- Manjunatha, P.T.; Gireesha, B.J.; Prasannakumara, B.C. Effect of radiation on flow and heat transfer of MHD dusty fluid over a stretching cylinder embedded in a porous medium in presence of heat source. Int. J. Appl. Comput. Math. 2017, 3, 293–310. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Zeeshan, A.; Ijaz, N.; Beg, A.O.; Kadir, A. Mathematical modelling of nonlinear thermal radiation effects of EMHD peristalic pumping of viscoelastic dusty fluid through a porous medium duct. Eng. Sci. Technol. Int. J. 2017, 20, 1129–1139. [Google Scholar]
- Rafiq, M.; Siddiqa, S.; Begum, N.; Al-Mdallal, Q.; Hossain, M.A.; Gorla, R.S.R. Non-linear radiation effect on dusty fluid flow near a rotating blunt-nosed body. Proc. Inst. Mech. Eng. Part E J. Process Mech. Eng. 2021, 235, 1775–1783. [Google Scholar] [CrossRef]
- Ibrahim, W. The effect of induced magnetic field and convective boundary condition on MHD stagnation point flow and heat transfer of upper-convected Maxwell fluid in the presence of nanoparticle past a stretching sheet. Propul. Power Res. 2016, 5, 164–175. [Google Scholar] [CrossRef]
- Nazeer, M.; Hussain, F.; Khan, M.I.; Rehman, A.; El-Zahar, E.R.; Chu, Y.M.; Malik, M.Y. Theoretical study of MHD electro-osmotically flow of third-grade fluid in micro channel. Appl. Math. Comput. 2022, 420, 126868. [Google Scholar] [CrossRef]
- Waini, I.; Ishal, A.; Pop, I. Magnetohydrodynamic flow past a shrinking vertical sheet in a dusty hybrid nanofluid with thermal radiation. Appl. Math. Mech. 2022, 43, 127–140. [Google Scholar] [CrossRef]
- Rehman, S.U.; Fatima, N.; Ali, B.; Imran, M.; Ali, L.; Shah, N.A.; Chung, J.D. Combined impact of Cattaneo-Christov double diffusion and radiative heat flux on bio-convective flow of Maxwell liquid configured by a stretched nano-material surface. Appl. Math. Comput. 2022, 10, 2877. [Google Scholar]
- Lou, Q.; Ali, B.; Rehman, S.U.; Habib, D.; Abdal, S.; Shah, N.A.; Chung, J.D. Micropolar dusty fluid: Coriolis force effects on dynamics of MHD rotating fluid when Lorentz force is significant. Mathematics 2022, 10, 2630. [Google Scholar] [CrossRef]
- Wei, Y.; Rehman, S.U.; Fatima, N.; Ali, B.; Ali, L.; Chung, J.D.; Shah, N.A. Significance of dust particles, nanoparticles radius, Coriolis and Lorentz forces: The case of Maxwell dusty fluid. Nanomaterials 2022, 12, 1512. [Google Scholar] [CrossRef]
- Wang, J.; Mustafa, Z.; Siddique, I.; Ajmal, M.; Jaradat, M.M.M.; Rehman, S.U.; Ali, B.; Ali, H.M. Computational analysis for bioconvection of microorganisms in Prandtl nanofluid Darcy—Forchheimer flow across an inclined sheet. Nanomaterials 2022, 12, 1791. [Google Scholar] [CrossRef]
- Saeed, M.; Ahmad, B.; Hassan, Q.M. Variable thermal effects of viscosity and radiation of ferrofluid submerged in porous medium. Ain Shams Eng. J. 2021, 13, 101653. [Google Scholar] [CrossRef]
- Nayak, M.K.; Dash, G.C.; Singh, L.P. Heat and mass transfer effects on MHD viscoelastic fluid over a stretching sheet through porous medium in presence of chemical reaction. Propul. Power Res. 2016, 5, 70–80. [Google Scholar] [CrossRef]
- Metri, P.G.; Metri, P.G.; Abel, S.; Silvestrov, S. Heat transfer in MHD mixed convection viscoelastic fluid flow over a stretching sheet embedded in a porous medium with viscous dissipation and non-uniform heat source/sink. Proc. Eng. 2016, 157, 309–316. [Google Scholar] [CrossRef]
- Madhura, K.R.; Gireesha, B.J.; Bagewadi, C.S. Exact solutions of unsteady dusty fluid flow through porous media in an open rectangular channel. Adv. Theor. Appl. Mech. 2009, 2, 1–17. [Google Scholar]
- Madhura, K.R.; Gireesha, B.J.; Bagewadi, C.S. Flow of an unsteady dusty fluid flow through porous media in a channel of triangular cross-section. Int. Rev. Phys. 2010, 4, 315–325. [Google Scholar]
- Chu, Y.M.; Shankaralingappa, B.M.; Gireesha, B.J.; Alzahrani, F.; Khan, M.I.; Khan, S.U. The Casson dusty nanofluid: Significance of Darcy—Forchheimer law, magnetic field, and non-Fourier heat flux model subject to stretch surface. Mathematics 2022, 10, 2877. [Google Scholar]
- Rathod, V.P.; Sridhar, N.G. Peristaltic flow of a couple stress fluids in an inclined channel. Int. J. Allied Pract. Res. Rev. 2015, 2, 27–38. [Google Scholar]
- Geentanjali, A.; Roy, A.S.; Kalyane, S.; Sonth, R.M. Unsteady flow of a dusty visco-elastic fluid through a inclined channel. Adv. Pure Math. 2011, 1, 187–192. [Google Scholar]
- Sivaraj, R.; Rushikumar, B. Unsteady MHD dusty viscoelastic fluid Couette flow in an irregular channel with varying mass diffusion. Int. J. Heat Mass Transfer 2012, 55, 3076–3089. [Google Scholar] [CrossRef]
- Sivaraj, R.; Rushikumar, B. Chemically reacting dusty viscoelastic fluid flow in an irregular channel with convective boundary. Ain Shams Eng. J. 2013, 4, 93–101. [Google Scholar] [CrossRef]
- Turkyilmazoglu, M. Suspension of dust particles over a stretchable rotating disk and two-phase heat transfer. Int. J. Multiphase Flow 2020, 127, 103260. [Google Scholar] [CrossRef]
- Ophori, D.U. The significance of viscosity in density-dependent flow of groundwater. J. Hydrol. 1998, 204, 261–270. [Google Scholar] [CrossRef]
- Lai, F.C.; Kulacki, F.A. The effect of variable viscosity on convective heat transfer along a vertical surface in a saturted porous medium. Int. J. Heat Mass Transfer 1990, 33, 1028–1031. [Google Scholar] [CrossRef]
- Chakraborty, S.; Medhi, N. MHD flow and heat transfer of a dusty visco-elastic liquid down an inclined channel in porous medium under variable viscosity and pressure. Indian J. Theor. Phys. 1995, 43, 293–302. [Google Scholar]
- Kalpana, G.; Madhura, K.R. Computational study on heat transfer of MHD dusty fluid flow under variable viscosity and variable pressure down an inclined irregular porous channel. Int. J. Emerg. Tech. Adv. Eng. 2017, 7, 358–369. [Google Scholar]

| Parameters | Friction Factor | Nusselt Number | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Case 1 | Case 2 | Case 1 | Case 2 | |||||||
| 2 | 1.655429831 | 1.522853027 | - | - | ||||||
| 4 | 5 | 0.5 | 2 | 0.63 | 2 | 0.3 | 0.267180727 | 0.051919641 | - | - |
| 6 | 0.047586102 | 0.042784836 | - | - | ||||||
| 5 | 0.05630821 | 0.051046954 | - | - | ||||||
| 2 | 10 | 0.5 | 2 | 0.63 | 2 | 0.3 | 0.041519842 | 0.011958089 | - | - |
| 15 | 0.012577104 | 0.006690786 | - | - | ||||||
| 0.5 | 0.072013672 | 0.184309882 | - | - | ||||||
| 2 | 5 | 1 | 2 | 0.63 | 2 | 0.3 | 0.35154075 | 0.093963489 | - | - |
| 1.5 | 0.50068426 | 0.070470312 | - | - | ||||||
| 1 | 0.00001230 | 0.014340811 | 2.668474288 | 0.16881461 | ||||||
| 2 | 5 | 1 | 2 | 0.63 | 2 | 0.3 | 0.000126263 | 0.017545688 | 3.097579452 | 0.471514472 |
| 1.5 | 0.018380056 | 0.043992287 | 3.389508739 | 3.781870901 | ||||||
| 0.2 | - | - | 0.747356087 | 0.097687653 | ||||||
| 2 | 5 | 1 | 2 | 0.63 | 2 | 0.3 | - | - | 0.728959459 | 0.049202599 |
| 0.71 | - | - | 0.855920223 | 0.041396851 | ||||||
| 1 | - | - | 0.079504801 | 0.410427683 | ||||||
| 2 | 5 | 1 | 2 | 0.63 | 2 | 0.3 | - | - | 0.160255859 | 0.721319137 |
| 3 | - | - | 0.241006918 | 1.032210592 | ||||||
| 0.3 | - | - | 0.378036935 | - | ||||||
| 2 | 5 | 1 | 2 | 0.63 | 2 | 0.6 | - | - | 0.732270204 | - |
| 0.9 | - | - | 1.093369217 | - | ||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kalpana, G.; Saleem, S. Heat Transfer of Magnetohydrodynamic Stratified Dusty Fluid Flow through an Inclined Irregular Porous Channel. Nanomaterials 2022, 12, 3309. https://doi.org/10.3390/nano12193309
Kalpana G, Saleem S. Heat Transfer of Magnetohydrodynamic Stratified Dusty Fluid Flow through an Inclined Irregular Porous Channel. Nanomaterials. 2022; 12(19):3309. https://doi.org/10.3390/nano12193309
Chicago/Turabian StyleKalpana, Gajendran, and Salman Saleem. 2022. "Heat Transfer of Magnetohydrodynamic Stratified Dusty Fluid Flow through an Inclined Irregular Porous Channel" Nanomaterials 12, no. 19: 3309. https://doi.org/10.3390/nano12193309
APA StyleKalpana, G., & Saleem, S. (2022). Heat Transfer of Magnetohydrodynamic Stratified Dusty Fluid Flow through an Inclined Irregular Porous Channel. Nanomaterials, 12(19), 3309. https://doi.org/10.3390/nano12193309







